Motion in a Plane Class 11 Important Extra Questions Physics Chapter 4

Here we are providing Class 11 Physics Important Extra Questions and Answers Chapter 4 Motion in a Plane. Important Questions for Class 11 Physics with Answers are the best resource for students which helps in Class 11 board exams.

Class 11 Physics Chapter 4 Important Extra Questions Motion in a Plane

Motion in a Plane Important Extra Questions Very Short Answer Type

Question 1.
Under what condition |a + b| = |a| + |b| holds good?
Answer:
When a and b act in the same direction i. e. when 0 = 0 between • them, then |a + b|=|a| + |b|.

Question 2.
Under what condition |a – b| = |a| – |b| holds good?
Answer:
The condition |a – b|=|a| – |b| holds goods when a and b act in the opposite direction.

Question 3.
The sum and difference of the two vectors are equal in magnitude
i. e. |a + b|=|a – b|. What conclusion do you draw from this?
Answer:
The two vectors are equal in magnitude and are perpendicular to each other.

Question 4.
What is the angle between \(\overrightarrow{\mathbf{A}} \times \overrightarrow{\mathbf{B}}\) and \(\overrightarrow{\mathbf{B}} \times \overrightarrow{\mathbf{A}}\)?
Answer:
The given vectors act along two parallel lines in opposite directions i.e. they are anti-parallel, so the angle between them is 180°.

Question 5.
What is the minimum number of coplanar vectors of different magnitudes which can give zero resultant?
Answer:
3, If three vectors can be represented completely by the three sides of a triangle taken in the same order, then their resultant is zero.

Question 6.
When a – b = a + b condition holds good than what can you say about b?
Answer:
For a – b = a + b condition to hold good, b must be a null vector.

Question 7.
What is the magnitude of the component of the 9î – 9ĵ + 19k̂ vector along the x-axis?
Answer:
9.

Question 8.
Can displacement vector be added to force vector?
Answer:
No.

Question 9.
What is the effect on the dimensions of a vector if it is multiplied by a non-dimensional scalar?
Answer:
There is no effect on the dimensions of a vector if it is multiplied by a non-dimensional scalar.

Question 10.
(a) What is the angle between î + ĵ and î vectors?
Answer:
45°

(h) What is the angle between î – ĵ and the x-axis?
Answer:
45°

(c) What is the angle between î + ĵ and î – ĵ?
Answer:
90°

Question 11.
What is the dot product of 2î + 4ĵ + 5k̂ and 3î + 2ĵ + k̂?
Answer:
19.

Question 12.
What must be the value of ‘a’ in 2î + 2ĵ – ak̂ so that it is perpendicular to 5î + 7ĵ – 3k̂?
Answer:
8.

Question 13.
Is finite rotation a vector quantity? Why?
Answer:
No. This is because the addition of two finite rotations does not obey commutative law.

Question 14.
Is infinitesimally small rotation a vector quantity? Why?
Answer:
Yes. This is because the addition of two infinitesimally small rotations obeys commutative law.

Question 15.
(a) Can the resultant of two vectors of different magnitudes be zero?
Answer:
No

(b) Can the magnitude of the rectangular component of a vector be greater than the magnitude of the vector itself?
Answer:
No.

Question 16.
A quantity has both magnitude and direction. Is it necessarily a vector? Why? Give an example.
Answer:
No. The given quantity will be a vector only if it obeys the laws of vector addition. Electric current.

Question 17.
In a vector equation, all the quantities are of similar nature but their directions are different. Does it mean that the vector equation is necessarily incorrect? Electric current.
Answer:
No. In a vector equation, the vectors need not be in the same direction.

Question 18.
Why vectors cannot be added algebraically?
Answer:
The vectors cannot be added algebraically because they possess both magnitude and direction.

Question 19.
Fifty vectors each of magnitude 10 units are completely represented by the sides of a polygon in the same order. What will be the resultant?
Answer:
Their resultant will be zero. This is because the vector sum of all the vectors represented by the sides of a closed polygon taken in the same order- is zero.

Question 20.
What will be the angle between \(\vec{A}\) and \(\vec{B}\) if \(\overrightarrow{\mathbf{A}}+\overrightarrow{\mathbf{B}}=\overrightarrow{\mathbf{B}}+\overrightarrow{\mathbf{A}}\)?
Answer:
The angle between A and B may be of any value if A + B = B + A.

Question 21.
How will you prove that the given vectors are neither parallel nor perpendicular?
Answer:
The given vectors will neither be parallel nor perpendicular if neither their cross product nor their dot product is zero.

Question 22.
How will you prove that the two vectors are parallel?
Answer:
If their cross product is zero, then the two vectors are said to be parallel.

Question 23.
How will you prove that two vectors are perpendicular?
Answer:
Two vectors will be perpendicular to each other if their dot product is zero.

Question 24.
(a) What is the minimum possible resultant of two forces 2N and 1N?
Answer:
1N

(b) What is the maximum possible resultant of two forces 2N and 1N?
Answer:
3N.

Question 25.
Is P² a scalar or a vector? Justify your answer.
Answer:
P2 is scalar because it is the dot product of P and P.

Question 26.
Is it necessary to mention the direction of a vector having zero magnitudes? Why?
Answer:
No. A vector having zero magnitudes is called a null vector. In this case, there is no sense of direction as the null vector is represented by a point.

Question 27.
Can a vector vary with time? Give example.
Answer:
Yes. For example, when an object moves, its position vector continuously changes with time.

Question 28.
Define a projectile.
Answer:
It is defined as an object thrown with some initial velocity moves under the effect of gravity alone.

Question 29.
Define trajectory.
Answer:
It is defined as the path followed by the projectile during its flight.

Question 30.
Define maximum height attained by a projectile.
Answer:
It is defined as the maximum vertical distance traveled by the projectile during its journey.

Question 31.
Define the horizontal range of the projectile.
Answer:
It is defined as the maximum horizontal distance between the point of projection and the point in the horizontal plane where the projectile hits it back after the journey.

Question 32.
At what angle a ball must be thrown to get maximum horizontal range?
Answer:
It must be thrown at an angle of 45° to the horizontal.

Question 33.
At what point in its trajectory does a projectile have its minimum velocity?
Answer:
At the highest point of the trajectory, a projectile has its minimum velocity.

Question 34.
How much is the velocity of the projectile at its highest point along the vertical direction?
Answer:
It is zero.

Question 35.
When a projectile is fired at an angle with the horizontal, then which of the components of its velocity remains constant throughout the trajectory?
Answer:
The horizontal component of its velocity remains constant throughout the trajectory.

Question 36.
At what point of the trajectory of a ball thrown upward is the acceleration perpendicular to the velocity?
Answer:
The acceleration is perpendicular to the velocity at the highest point of the trajectory.

Question 37.
A bob of mass 0.1 kg hung from the ceiling of a room by a string 2m long is set into oscillation. The speed of the bob at its mean position is 1 ms-1. What is the trajectory of the bob if its string is cut when the bob is: (a) at one of its extreme position (b) at its mean position.
Answer:
(a) straight line,
(b) parabolic path.

Question 38.
A cannon on a level plane is aimed at an angle P above the horizontal and a shell is fired with a muzzle speed v towards a vertical cliff a distance x away. At what height y from the bottom, the shell would hit the cliff?
Answer:
y = x tan β – \(\frac{\mathrm{gx}^{2}}{2 \mathrm{v}^{2} \cos ^{2} \beta}\)

Question 39.
A body is projected so that it has maximum range R. What is .the maximum height reached during the flight?
Answer:
Maximum height is \(\frac{1}{4}\) of maximum range i.e. h = \(\frac{R}{4}\).

Question 40.
A bullet x is fired from a gun when the angle of elevation of the gun is 30°. Another bullet y is fired from the gun at an angle of elevation 60°. Tell which of the two bullets would have a greater horizontal range?
Answer:
Both will have the same range.

Question 41.
A particle moves in a plane with uniform acceleration ¡n a direction different from its initial velocity. What is the nature of the path followed by it?
Answer:
It is a parabolic path in nature.

Question 42.
A projectile of mass m is fired with initial velocity u at angle 6 with the horizontal. What is the change in momentum as it rises to the highest point of the trajectory?
Answer:
Change in momentum = Force × time = mg × \(\frac{\mathrm{u} \sin \theta}{\mathrm{g}}\) = mu sinθ

Question 43.
Name the five physical quantities which change during the motion of an oblique projectile.
Answer:
Five physical quantities are velocity, the vertical component of velocity, momentum, kinetic energy, potential energy.

Question 44.
Name the two quantities which would be reduced if air resistance is taken into account in the study of the motion of the oblique projectile which quantity would be increased?
Answer:
The quantities which are reduced:

1. maximum height
2. horizontal range.

The angle at which the projectile hits the ground would be increased.

Question 45.
At which point of the trajectory of the projectile, the speed is maximum?
Answer:
The speed is maximum at two points which are:

1. The point from where the projectile is thrown.
2. The point where the projectile returns back to the plane of projection.

Question 46.
What is the condition for the following formula to be valid?
Average velocity = \(\)\frac{\text { Initial velocity + final velocity }}{2}
Answer:
It is valid only if acceleration is constant.

Question 47.
A body travels with uniform acceleration ai for time t, and with uniform acceleration a2 for time t2. What is the average acceleration?
Answer:
aaverage = \(\frac{a_{1} t_{1}+a_{2} t_{2}}{t_{1}+t_{2}}\).

Question 48.
Even when rain is falling vertically downwards the front screen of a moving car gets wet. On the other hand, the back screen remains dry. Why?
Answer:
The rain strikes the car in the direction of the relative velocity of the rain with respect to the car.

Question 49.
Can a body have a constant velocity and still have a varying speed?
Answer:
No. If the velocity of a body is constant then its speed cannot vary.

Question 50.
(a) What are the units of angular speed?
Answer:
Units of angular speed are radian/second (rad s-1]).

(b) What is the relation between time period (T) and frequency (v)?
Answer:
T= \(\frac{1}{v}\)

Question 51.
(a) Give an example of a body moving with uniform speed but having a variable velocity and an acceleration that remains constant in magnitude but changes in direction.
Answer:
A body having uniform circular motion.

(b) What is the direction of a centripetal acceleration with reference to the position vector of a particle moving in a circular path?
Answer:
It is always along the position vector but directed towards the center of the circle.

Motion in a Plane Important Extra Questions Short Answer Type

Question 1.
Name two quantities that are the largest when the maximum height attained by the projectile is largest.
Answer:
Time of flight and the vertical component of velocity are the two quantities that are the largest when the maximum height attained by the projectile is the largest.

Question 2.
A stone dropped from the window of a stationary railway carriage takes 2 seconds to reach the ground. At what time the stone will reach the ground when the carriage is moving with
(a) the constant velocity of 80kmh^-1
(b) constant acceleration of 2ms^-2?
Answer:
The time taken by the freely falling stone to reach the ground is given by
t = \(\sqrt{\frac{2 h}{g}}\)

In both cases, the stone will fall through the same height as it is falling when the railway carriage is stationary. Hence the stone will reach the ground after 2 seconds.

Question 3.
Can a particle accelerate when its speed is constant? Explain.
Answer:
Yes. A particle can be accelerated if its velocity changes. A particle having uniform circular motion has constant speed but its direction of motion changes continuously. Due to this, there is a change in velocity and hence the particle is moving with variable velocity. Thus particle is accelerating.

Question 4.
(a) Is circular motion possible at a constant speed or at constant velocity? Explain.
Answer:
Circular motion is possible at a constant speed because, in a circular motion, the magnitude of the velocity i.e. speed remains constant while the direction of motion changes continuously.

(b) Define frequency and time period.
Answer:
Frequency is defined as the number of rotations completed by a body in one second and the time period is defined as the time taken by an object to complete one rotation.

Question 5.
When the component of a vector A along the direction of vector B is zero, what can you conclude about the two vectors?
Answer:
The two vectors A and B are perpendicular to each other.
Explanation: Let θ = angle between the two vectors A and B component of vector A along the direction of B is obtained by resolving A i.e. A cos θ.

Now according to the statement
A cos θ = 0
or
cos θ = 0 = cos 90°
θ = 90°
i.e. A ⊥ B

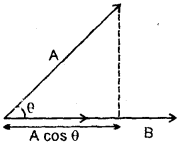
Hence proved.

Question 6.
Comment on the statement whether it is true or false “Displacement vector is fundamentally a position vector.’’ Why?
Answer:
The given statement is true. The displacement vector gives the position of a point just like the position vector. The only difference between the displacement and the position vector is that the displacement vector gives the position of a point with reference to a point other than the origin, while the position vector gives the position of a point with reference to the origin. Since the choice of origin is quite arbitrary, so the given statement.

Question 7.
Does the nature of a vector changes when it is multiplied by a scalar?
Answer:
The nature of a vector may or may not be changed when it is multiplied.

For example, when a vector is multiplied by a pure number like 1, 2, 3,…. etc., then the nature of the vector does not change. On the other hand, when a vector is multiplied by a scalar physical quantity, then the nature of the vector changes.

For example, when acceleration (vector) is multiplied by a mass (scalar) of a body, then it gives force (a vector quantity) whose nature is different than acceleration.

Question 8.
Can the walk of a man be an example of the resolution of vectors? Explain.
Answer:
Yes, when a man walks, he pushes the ground with his foot. In return, an equal and opposite reaction acts on his foot. The reaction is resolved into two components: horizontal and vertical components. The horizontal component of the reaction helps the man to move forward while the vertical component balances the weight of the man.

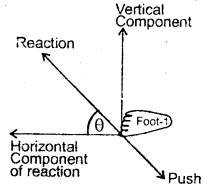
Question 9.
Explain under what conditions, the resultant of two vectors will be equal to either of them.
Answer:
The resultant of two vectors will be equal to either of them if:

1. The two vectors are of the same magnitude.
2. The two vectors are inclined to each other at 120°.

Explanation: Let x be the magnitude of each of the two vectors say P and Q. If 9 = angle between P and Q, then the magnitude of their resultant vector (R) is given by the relation

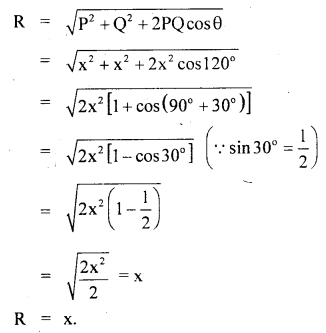
Question 10.
Why the magnitude of the rectangular components of a vector can’t be greater than the magnitude of the vector itself?
Answer:
The magnitude of rectangular components of a vector itself cannot be greater than the magnitude of the vector itself because the rectangular components of a vector A are A_x = A cos θ and A_y = A sin 9. As sin θ and cos θ both are ≤ 1, so both A_x and A_y cannot be greater than A.

Question 11.
Can a flight of a bird be an example of the composition of vectors? Explain.
Answer:
Yes, the flight of a bird can be an example of the composition of vector i.e. addition of vectors as is shown in the figure here. As it flies, it strikes the air with its wings W, W along with WO. So, according to Newton’s third law of motion, airstrikes the wings in opposite directions with the same force in reaction represented by OA and OB. Thus according to the parallelogram law of vectors, the resultant of OA and OB is OC. This resultant upward force OC is responsible for the flight of the bird.

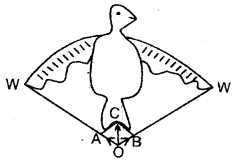
Question 12.
Can commutative law be applied to vector subtraction?
Answer:
Let P and Q be the two vectors.
∴ As P – Q ≠ Q – P, so commutative law is not applied to vector subtraction.

Question 13.
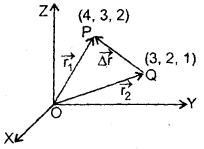
Write down the vector whose head is at (4, 3, 2) and whose tail is at (3, 2, 1).
Answer:
Let r, and r2 be the position vectors of the points P (4, 3, 2) and Q (3, 2, 1) respectively
∴ r₁ = 4î + 3ĵ + 2k̂
and r₂ = 3î + 2ĵ + 1k̂

Let QP = Δr be the required vector
Now applying triangle law of vector addition of ΔOQP, we get
r₂ + Δr = r₁
or
Δr = r₁ – r₂
= (4î + 3ĵ + 2k̂) – (3î + 2ĵ + 1k̂)
= (î + ĵ + k̂ ).

Question 14.
If A.B = A.C, is it safe to conclude that B = C?
Answer:
Let θ₁, θ₂ be the angler between A and B, B and C respectively


So we conclude that it is not safe to conclude that B = C until θ₁ = θ₂.

Question 15.
Define Tensor. Give example.
Answer:
It is defined as a physical quantity that has no direction but has different values in different directions. It is neither a vector nor a scalar, e.g. moment of Inertia has no direction but its values are different in different directions. Hence moment of inertia is neither a scalar nor a vector but a tensor. Other examples of the tensor are refractive index, stress, strain, density.

Question 16.
How does a sling work?
Answer:
It works on the principle of parallelogram law of vectors. A rubber sling is attached to the two ends of a sling. The stone to be thrown is held at point O. When the rubber string is pulled, the tensions are produced along with OA and OB. When the string is released, the stone moves under the effect of resultant force OC.

Question 17.
Why does a tennis ball bounce higher on hills than in plains?
Answer:
We know that the maximum height of a projectile is given by the relation

where θ = angle of projection
and u = initial velocity of projection.

From (i), we see that the maximum height is inversely proportional to the acceleration due to gravity (g). So smaller the g, greater shall be the maximum height. Since the value of g is lesser on the hills than on the plains, so a tennis ball will bounce more on hills than on plains.

Question 18.
Two particles are moving with equal and opposite velocities in such a way that they are always at a constant distance apart. Calculate the time after which the particles return to their initial positions.
Answer:
Clearly, the two particles are at the two ends of the diameter of a circular path. It is further clear that each particle will return to its initial position after describing one circle.

If t = required time, then
t = \(\frac{2 \pi r}{v}=\frac{\pi d}{v}\)
where v = speed of particles.
r = radius of the circular track,
d = constant distance between particles.
= diameter of the circular path = 2r.

Question 19.
A ball P is projected directly towards a second ball Q. The horizontal distance x of the second ball is lesser than the horizontal range R of the first ball. The second ball is released from the rest at the instant the first is projected, will the two balls collide.
Answer:
Yes, the initial elevation of 2nd ball = x tan θ
During time t, the second ball covers a distance = \(\frac{1}{2}\) gt2

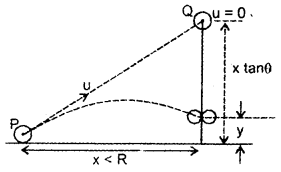
If y = elevation at the instant of collision, then

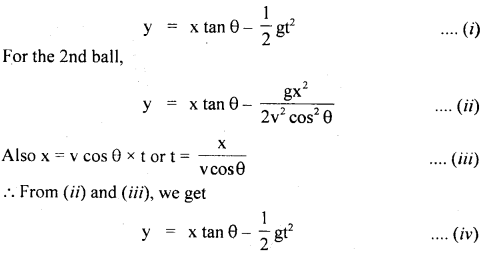
Thus from (i) and (iv), it is clear that both the balls attain the same elevation at time t. So that two must collide, whatever may be the initial velocity of the first ball.

Question 20.
Which one of the following is greater?
(a) The angular velocity of the hour hand of a watch.
(b) The angular velocity of the Earth around its own axis. Why?
Answer:
The angular velocity of the hour hand of a watch.is greater than the angular velocity of the earth around its own axis.

Explanation: We know that the angular velocity (w) of an object has
Time period (T) is given by
ω = \(\frac{2 \pi}{\mathrm{T}}\) ….(i)
(a) T for hour hand of a watch is 12h

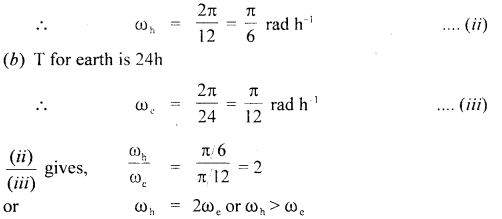
Thus clearly angular velocity of the hour hand is more than the angular velocity of the earth around its own axis because of the T_earth > T_{hour hands}.

Question 21.
A bomber in the horizontal flight drops a bomb when it is just above the target. Explain whether the bomb hits the target or misses it?
Answer:
The bomb will miss the target. This is due to the fact that at the time of dropping, the bomb possesses a horizontal speed equal to the speed of the bomber. So bomb will drop and hit the ground some distance away (= horizontal speed of bomber x time) from the place of dropping.

Question 22.
(a) What is the direction of the area of the vector?
Answer:
It acts perpendicular to the plane containing the area i.e. it acts normal to the surface area.

(b) What are the characteristics of the motion of an Earth satellite revolving around it in a fixed orbit?
Answer:
The satellite motion in a fixed orbit is characterized by the following factors:

1. The Earth’s gravitational pull at the height is equal to the centripetal force at that height.
2. The angular velocity of the satellite about the Earth’s center remains constant.
3. The linear speed of the satellite is also constant.

Question 23.
A ball is thrown horizontally and at the same time, another ball is dropped from the top of a tower with the same velocity.
(i) Will both the balls hit the ground with the same velocity?
Answer:
When the balls hit the ground, their vertical velocities are equal. However, the horizontal velocities will be different. Hence the resultant velocities of the two balls are different, so the balls would hit the ground with different velocities.

(ii) Will both the balls reach the ground at the same time?
Answer:
Both the balls would reach the ground at the same time because their initial vertical velocities, acceleration, and distances covered are all equal.

Question 24.
Three balls thrown at different angles reach the same maximum height (fig. given), then answer the following:

(a) Are the vertical components of the initial velocity the same for all the balls? If not, which one has the least vertical velocity component?
Answer:
Since maximum height is directly proportional to the vertical component of initial velocity hence the vertical components of velocity in the three cases are the same as the maximum height attained is the same.

(b) Will they all have the same time of flight?
Answer:
Yes, all will have same time of flight T = \(\frac{2 \mathrm{u} \sin \theta}{\mathrm{g}}\) as sin θ is same for all.

(c) Which one has the greatest horizontal velocity component?
Answer:
The projectile having maximum range has the greatest horizontal component of the initial velocity.

Question 25.
When a car is driven too fast around a curve it skids outward. How would a passenger sitting inside explain the car’s motion? How would an observer standing by the roadside explain the event?
Answer:
The passenger inside the car is in the reference frame of the car so he experiences centrifugal force \(\frac{\mathrm{mv}^{2}}{\mathrm{r}}\) acting on the car. So to remain in a circular path force should be larger as it is proportional to v₁₂.

An observer on the roadside finds that the centripetal force \(\frac{\mathrm{mv}^{2}}{\mathrm{r}}\) acting on the car becomes inadequate with an increase in v. Since again v₂ r and r is insufficient so the car skids.

Question 26.
Show that the horizontal range of projectile for two angles of projection α and β is same when α + β = 90°.
Answer:
The horizontal ranges for two angles of projections are

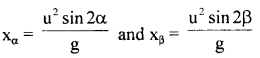
assuming that projection velocity is the same in both cases

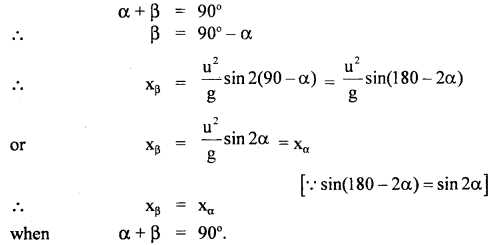
Question 27.
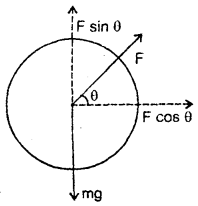
Why is it easier to pull a lawn roller than to push it? Explain.
Answer:
(a) Consider the case of the pull of roller as shown in Fig. (a):
Here the force of pull (F) has two components:
(i) F cos θ which moves the roller horizontally.
(ii) F sin θ which acts vertically upward and opposite to the weight (mg) of the roller.
∴ Net weight of the roller = mg – F sin θ ….(i)

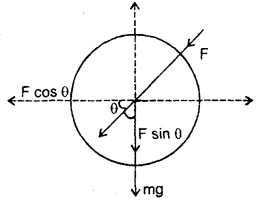
(b) Case of the push of roller: In this case, the components of F are:
(i) F cos θ which helps to move the roller horizontally.
(ii) F sin θ which acts vertically downward in the direction of the weight (mg) of the roller.
∴ Net weight of the roller = mg + F sin θ ….(ii)

Now from equations (i) and (ii), it is clear that the net weight (i.e. apparent weight) of the roller becomes greater in the case of pushing than in the case of pulling. But due to friction, the force required to move a body is directly proportional to the effective weight of the body. So a smaller force is required to move the roller in case of pulling than in case of pushing. Hence it is easier to pull than to push a lawn roller.

Question 28.
(a) Is the maximum height attained by a projectile largest when the maximum range is maximum?
Answer:
No. the angle for the maximum horizontal range is 45° but for the maximum height the largest range is 41°:

(b) A body in uniform horizontal circular motion possesses variable velocity. Does it mean that the kinetic energy of the body is also variable?
Answer:
No, since the magnitude of velocity in uniform motion is constant, hence the kinetic energy will also not change.

(c) Why vector cannot be added algebraically?
Answer:
The algebraic sum does not take into account the direction of a physical quantity. It adds only magnitudes, hence it is not suitable for vector sum.

Question 29.
(a) How can a vector be tripled?
Answer:
By multiplying the vector by number 3 (a scalar) or by adding two more similar vectors i.e. vectors of the same magnitude and direction to the given vector.

(b) State Triangle law of vector addition.
Answer:
It states that if two vectors are represented completely (i.e. both in magnitude and direction) by the two sides of a triangle taken in the same order then their resultant is represented completely by the third side of the triangle taken in the opposite order.

Question 30.
State polygon law of vector addition. Show it graphically.
Answer:
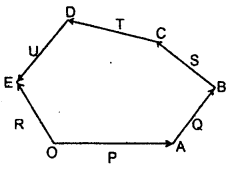
It states that if a number of vectors are represented completely by the sides of a polygon taken in the same order, then their resultant is represented completely by its closing side taken in the opposite order. If P, Q, S, T, and U be the vectors represented completely by the sides OA, AB, BC, CD, and DE of the polygon taken in the same order, then their resultant (R) is represented by OE taken in opposite order s.t.
R = P + Q + S + T + U

Question 31.
Define null vector. What are its properties? What is its physical significance?
Answer:
It is defined as a vector having zero magnitudes and acting in an arbitrary direction. It is denoted by O.
Properties of null vector:

1. The addition or subtraction of zero vector from a given vector is again the same vector.
   i.e. A + O = A
   A – O = A
2. The multiplication of zero vector by a non-zero real number is again the zero vector
   i.e. n.O = O
3. If n₁ A = n2B where n₁ and n₂ are non-zero real numbers, then the relation will hold good
   if A = B = O
   i.e. both A and B are null vectors.

The physical significance of null vector: It is useful in describing the physical situation involving vector quantities.
e.g. A – A = O
0 × A = O.

Motion in a Plane Important Extra Questions Long Answer Type

Question 1.
Discuss the problem of a swimmer who wants to cross the river in the shortest time.
Answer:
Let v_s and v_r be the velocities of swimmer and river respectively.
Let v = resultant velocity of v_s and v_r

1. Let the swimmer begins to swim at an angle θ with the line OA where OA is ⊥ to the flow of the river.
If t = time taken to cross the river, then
t = \(\frac{l}{v_{s} \cos \theta}\) …(i)

where l = breadth of the river
For t to be minimum, cos 0 should be maximum.
i.e. cos θ = 1

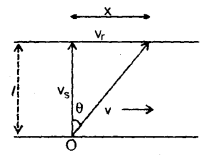
This is possible if θ = 0
Thus, we conclude that the swimmer should swim in a direction perpendicular to the direction of the flow of the river.

2. v = \(\sqrt{v_{s}^{2}+v_{r}^{2}}\)
where v\(\vec{v}\) is the resultant velocity of v_s and v_r.

3. tan θ = \(\frac{v_{\mathrm{r}}}{\mathrm{v}_{\mathrm{s}}}=\frac{\mathrm{x}}{l}\)
or
x = l\(\frac{\mathbf{v}_{\mathrm{r}}}{\mathbf{V}_{\mathrm{s}}}\)

4. t = \(\frac{l}{\mathrm{~V}_{\mathrm{s}}}\)

Question 2.
State and prove parallelogram law of vector addition. Discuss some special cases.
Answer:
It states that if two vectors can be represented completely (i.e. both in magnitude and direction) by the two adjacent sides of a parallelogram drawn from a point then their resultant is represented completely by its diagonal drawn from the same point.

Proof: Let P and Q be the two vectors represented completely by the adjacent sides OA and OB of the parallelogram OACB s.t.
\(\overrightarrow{\mathrm{OA}}\) = P, \(\overrightarrow{\mathrm{OB}}\) = Q
or
| \(\overrightarrow{\mathrm{OA}}\) | = |P|, | \(\overrightarrow{\mathrm{OB}}\) | = |Q|

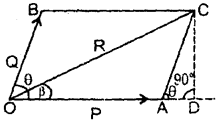
θ = angle between them = ∠AOB

If R be their resultant, then it will be represented completely by the diagonal OC through point O s.t. OC = R
The magnitude of R: Draw CD ⊥ to OA produced,


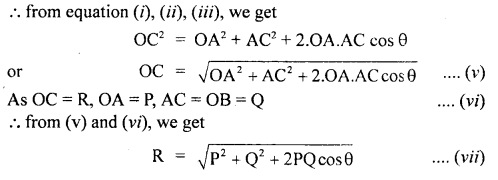
eqn. (vii) gives the magnitude of R.

The direction of R: Let β be the angle made by R with P
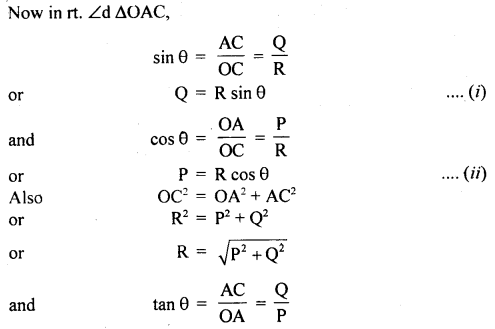
∴ in rt. ∠d ΔODC,

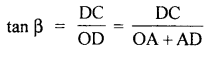
Special cases: (a) When two vectors are acting in the same direction:
Then θ = 0°

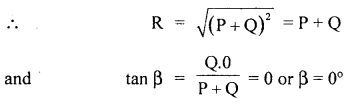
Thus, the magnitude of the resultant vector is equal to the sum of the magnitudes of the two vectors acting in the same direction, and their resultant acts in the direction of P and Q.

(b) When two vectors act in the opposite directions:
Then θ = 180°

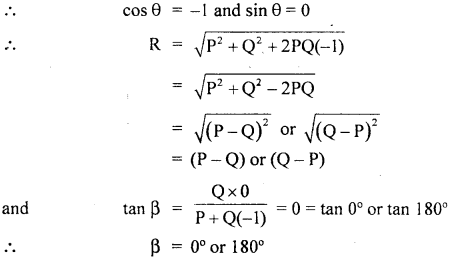
Thus, the magnitude of the resultant of two vectors acting in the opposite direction is equal to the difference of the magnitude of two vectors and it acts in the direction of the bigger vector.

(c) If θ = 90° i.e. if P ⊥ Q,
then cos 90° = 0
and
sin 90° = 1

R = \(\sqrt{P^{2}+Q^{2}}\)
and
tan β = \(\frac{O}{P}\).

Question 3.
Derive the relation between linear velocity and angular velocity. Also, deduce its direction.
Answer:
Let R be the radius of the circular path of center O on which an object is moving with uniform angular velocity co. Let v = its linear velocity. Let the object move from point P at time t to point Q at time t + Δt. If r and r + Δr be its position vectors at point P and Q respectively, then


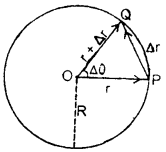
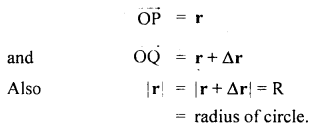
∴ Linear displacement of the particle from P to Q in small time interval Δt = Δr.
Let Δθ = its angular displacement
∴ ω = \(\frac{Δθ}{Δt}\)
or
Δθ = ωΔt ….(1)

Also we know that Δθ = \(\frac{\widehat{\mathrm{PQ}}}{\mathrm{R}}\) …(2)

∴ from (1) and (2), we get

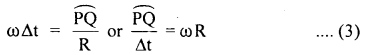
Now when Δt → 0, then from eqn. (1) Δθ → 0
so arc PQ = \(\widehat{\mathrm{PQ}}\) = chord PQ

Thus eqn. (3) reduces to

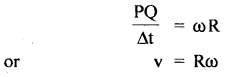
where v = \(\frac{PQ}{Δt}\) is the linear velocity of the object.

Direction of velocity vector: In isosceles ΔOPQ,

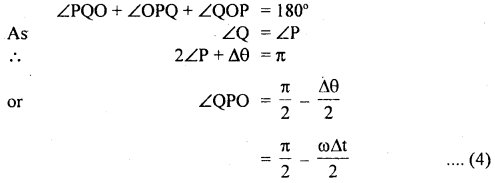
when Δt → 0, ∠QPO → \(\frac{π}{2}\)
i.e. \(\overrightarrow{\mathrm{OP}}\) tends to become ⊥ to \(\overrightarrow{\mathrm{OP}}\)
or
\(\overrightarrow{\mathrm{OP}}\) tends to lie along the tangent at P. Hence velocity vector at P is directed along the tangent to the circle in the direction of motion.

Question 4.
What do you understand by the rectangular resolution of a vector? Resolve it into its two rectangular components.
Answer:
It is defined as the process of splitting a given vector into two or three-component vectors at right angles to each other. The component vectors are called rectangular components of the given vector. Let R be the given vector acting in the X – Y plane at an angle θ with the x-axis. Let \(\overrightarrow{\mathrm{OC}}\) = R. From point C, draw perpendiculars CA and CB on X and Y axes respectively. If P and Q be the rectangular components of R along the X and Y axis respectively, then

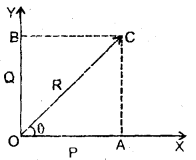
Also according to the triangle law of vector addition,
R = P + Q
= Pî + Qĵ
or
R= (R cosθ)î+ (R sinθ)ĵ
Thus if A_x and A_y be the two rectangular components of A along the X and Y axes respectively.
Then A = A_x + A_y
A = A_xî + A_yĵ

Note: Similarly in three dimensions,
A = A_xî +A_yĵ + A_zk̂

Position vector in two and three dimensions can be expressed as:
r = xî + yĵ
and
r = xî + yĵ + zk̂ .

Question 5.
Define centripetal acceleration and derive its expression. Also, deduce its direction.
Answer:
Centripetal acceleration: It is defined as the acceleration which always acts towards the center along the radius of the circular path.
“Centripetal” comes from a Greek term that means “centre¬seeking”.

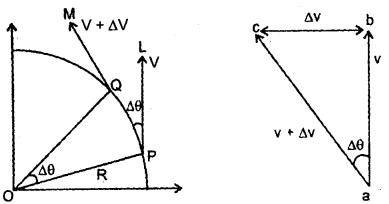
The expression for centripetal acceleration: Let a body be moving wi^h constant angular velocity 0) and constant speed v in a circular path of radius R and center O.

Let the body be at points P and Q at time t and t + Δt respectively.
Also, let Δθ = ∠POQ be the angular displacement in a small-time inverted Δt, thus

If v and v + Δv be the velocity vectors of the body at points P and Q acting along PL and QM respectively. As the body is moving with uniform speed, so lengths PL and PM are equal i.e. PL = PM. The change in velocity Δv from P to Q is due to the change in the direction of the velocity vector.

Hence the magnitude of the acceleration of the body is given by

From point ‘a’ draw \(\overrightarrow{\mathrm{ab}}\) parallel and equal to \(\overrightarrow{\mathrm{PL}}\) and \(\overrightarrow{\mathrm{ac}}\) parallel and equal to \(\overrightarrow{\mathrm{QM}}\), thus

Clearly, the angle between ab and ac is Δθ. As Δt is quite small so
b and c lie on very close to each other, thus be can be taken to be an arc \(\widehat{\mathrm{bc}}\) of a circle of radius ab = |v|.

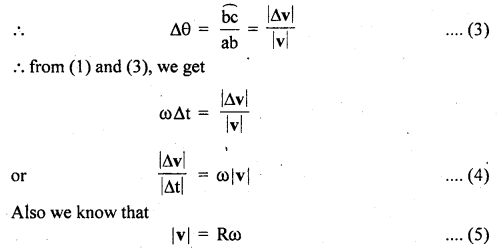
Also when Δt → 0, then L.H.S. of Eqn. (4) represents acceleration. Thus Eqn. (4) can be written as


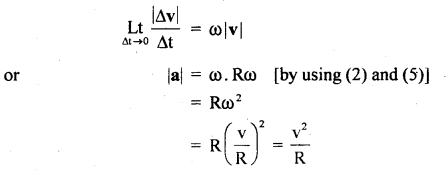
eqn. (6) gives the magnitude of the acceleration produced in the body.

The direction of acceleration: Acceleration always acts in the direction of Δv which acts from b to c. When Δt is decreased, Δθ also decreases. If Δt → 0, then Δθ → 0, so Δv tends to be perpendicular to ab. As ab ∥ PL, so Δv tends to act perpendicular to PL i.e. along PO i.e. along the radius towards the center of the circular path.

Since v and R are always constant, so the magnitude of centripetal acceleration is also constant. But the direction changes pointing always towards the center. So centripetal acceleration is not a constant vector.

Question 6.
A body is projected with some initial velocity making an angle θ with the horizontal. Show that its path is a parabola. Find the maximum height attained, time for maximum height, horizontal range, maximum horizontal range, and the time of flight.
Answer:
Let the body be projected with velocity u inclined at angle θ with the horizontal. The horizontal and vertical components of velocity and acceleration are
U_x, a_x, and U_y, a_y where
u_x = u cos θ, U_y = u sin θ, a_x = 0, a_y = -g
where g is the acceleration due to gravity.

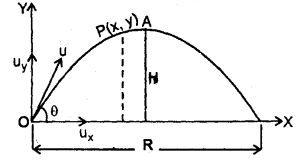
The coordinates of O are (0, 0). Considering horizontal motion, The position of the body after time t has coordinates (x, y) where
x (t) = X_o + u_x t + 1/2 a_x × t²

Substituting for various factors
x(t) = 0 + u cos θ . t + 0
x(t) = u cos θ t

Equation of trajectory:
Substituting for t from eq. (1) in eq. (2), we get

This is an equation of a parabola. Thus, the path of a projectile is a parabola.

Maximum height attained: At the maximum height, the vertical componènt of velocity becomes O (zero). Now using the equation of motion

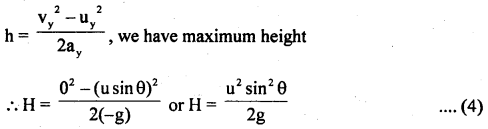
Time for maximum height: Using equation of motion, v = u + at or v_y = Uy + a_y t, we have

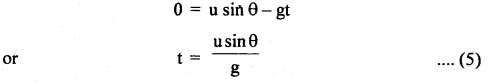
Horizontal range: Let the horizontal range be R. Since there is no acceleration in the horizontal direction, so
x = x(0) + u_xt + \(\frac{1}{2}\)a_x t²
Here x(0) = 0, u_x = u cos θ, a_x = 0, and t is the total time of flight which is twice the time for maximum height because the body takes the same time in rising to and falling from the highest point.

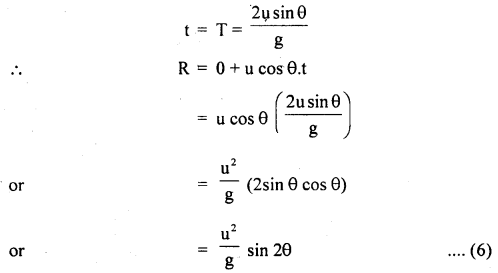
Maximum horizontal range: For R to be maximum, sin 2θ in Eqn. (6) must be maximum i.e. sin 2θ = 1,

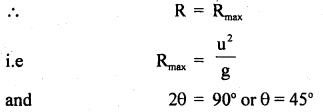
Thus, for R to be maximum, 0 must be 45°. It is independent of the mass of the body.

Time of flight of the projectile: The projectile after completing its flight returns back to the same horizontal level from which it was projected. Therefore, the vertical displacement in the whole flight is zero. Considering vertical motion.


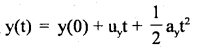
Therefore, either T = 0 or u sin θ – \(\frac{1}{2}\) g T = 0
T = 0 corresponds to the initial (starting) position,

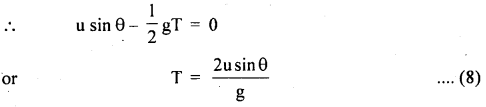
Equation (8) gives the total time of flight This is twice the time for maximum height.

Question 7.
A projectile is projected at an angle a with the vertical with initial velocity u. Find the maximum height attained, time of flight, and the horizontal range.

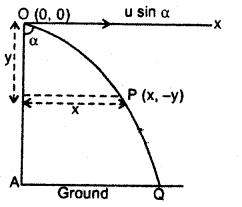
Answer:
Let the particle be projected from O with velocity u. The magnitude of horizontal and vertical components of the velocity are u sinα and u cosα respectively. Coordinates of O are (0, 0).

Maximum height: Consider the vertical motion. The initial speed Uy = u cosα and final speed v_y = 0, acceleration ay = – g, then using the
equation of motion s = \(\frac{v^{2}-u^{2}}{2 a}\) we have

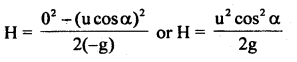
Time of flight: It is the total time taken by the projectile in going from O to A. The vertical displacement = 0. Considering vertical motion and using the equation of motion

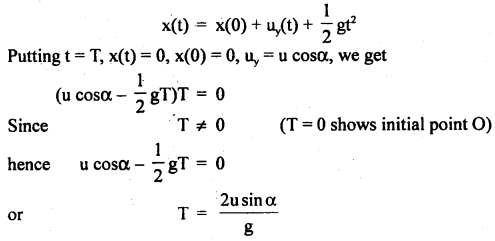
Horizontal range: Considering horizontal motion u_x = u sinα,
a_x = 0,
∴ using equation of motion

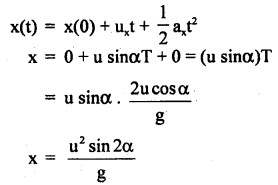
Numerical Problems:

Question 1.
What is the angle between the following pair of vectors?
A = î + ĵ + k̂,B = – 2î – 2ĵ – 2k̂
Answer:
We know that
A.B = AB cos θ

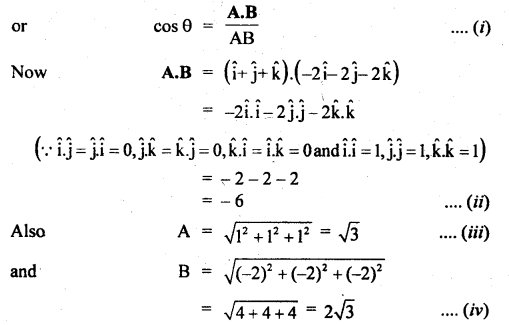
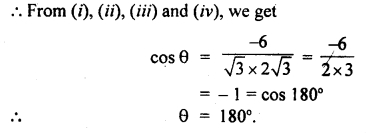
Question 2.
Prove that 2î – 3ĵ – k̂ and – 6î + 9ĵ + 3k̂ are parallel.
Answer:
Let A = 2î – 3ĵ – k̂
and B = – 6î + 9ĵ + 3k̂

These two vectors will be parallel if their cross-product is zero.
i.e. if A × B = 0


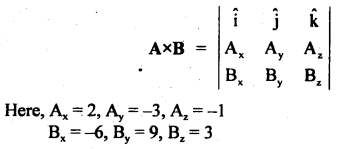
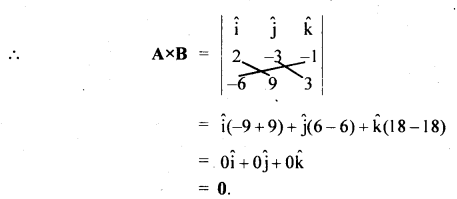
Hence proved.

Question 3.
Calculate the area of the triangle determined by two vectors
A = 3î + 4ĵ and B = – 3î + 7ĵ
Answer:
We know that area of a triangle is given by

Question 4.
A man wants to cross the river to an exactly opposite point on the other bank. If he can row his boat with twice the velocity of the current, then at what angle to the current, he must keep the boat pointed.
Answer:

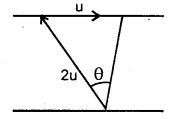
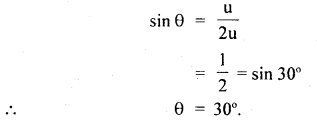
Question 5.
A body is acted upon by the following, velocities:
(i) 7 ms^-1 due to E,
(ii) 10 ms^-1 due S,
(iii) 5\(\sqrt{2}\) ms^-1 due N.E.
Find the magnitude and direction of the resultant velocity.

Answer:
Let OA, OB and OC represent the velocities given in the statement i.e.
OA = 7 ms^-1
OB = 10 ms^-1
and OC = 5\(\sqrt{2}\) ms^-1
To find their resultant velocity, resolve OC into two rectangular components along east and north.

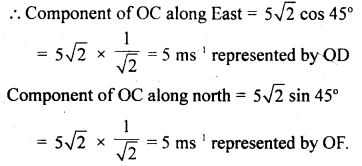
Hence resultant velocity along east = 7 + 5 = 12 ms^-1 and resultant velocity along south = OB – OF = 10 – 5 = 5 ms^-1.

If R be the resultant velocity, then the magnitude of R is obtained by applying the parallelogram law of vector addition as

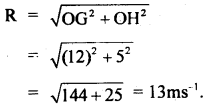
When OG = 12ms^-1 and OH 5ms^-1.

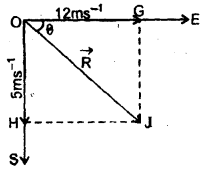
The direction of R: Let θ be the angle made by R with the east.
∴ in rt. ∠d ΔOGI,

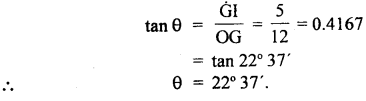
Question 6.
If \(\overrightarrow{\mathbf{A}}+\overrightarrow{\mathbf{B}}=\overrightarrow{\mathbf{C}}\), prove that C = (A² + B² + 2AB cosθ)^1/2 where θ ¡s the angle between \(\overrightarrow{\mathbf{A}}\) and \(\overrightarrow{\mathbf{B}}\).
Answer:

Question 7.
Determine the value of k so that the vectors \(\overrightarrow{\mathbf{A}}\) = 6î + kĵ – 4k̂ and \(\overrightarrow{\mathbf{B}}\) = 3î + 4ĵ + 5k̂ are perpendicular.
Answer:

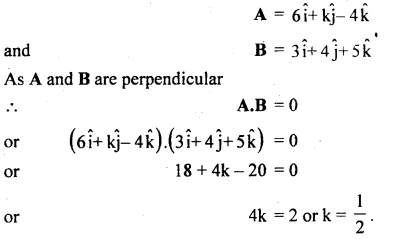
Question 8.
Determine a unit vector which is perpendicular to both \(\overrightarrow{\mathbf{A}}\) = 2î + ĵ + k̂ and \(\overrightarrow{\mathbf{B}}\) = î – ĵ + 2k̂
Answer:
Let n̂ be the unit vector perpendicular to both A and B.
Also, Let C be the vector that acts along n̂
∴ By definition of the cross product,

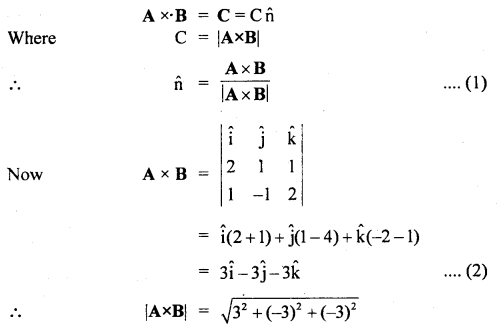
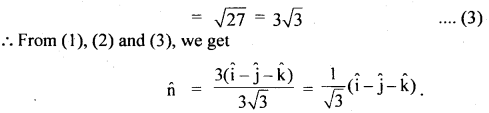
Question 9.
A projectile is fired horizontally with a velocity of 98 ms^-1 from the top of a hill 490 m high. Find:
(i) the velocity with which it strikes the ground.
(ii) the time is taken to reach the ground.
(iii) the distance of the target from the hill.
Answer:

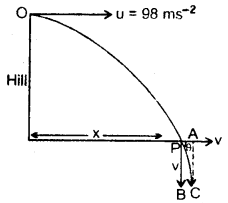
(i) h = 490 m, a = g = 9.8 ms²
U_y = initial velocity along the y-axis at the top of the tower = 0

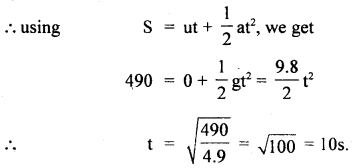
(ii) Let v be the velocity along the y-axis with which the projectile hits the ground.

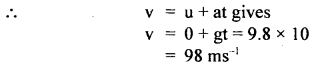
If V be the resultant velocity of hitting the ground

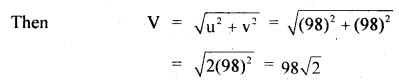
Let θ be the angle made by V with the horizontal

(iii) Let x, be the distance of the target from the hill.
∴ x = horizontal distance covered with u in a time t.
ut = 98 × 10 = 980 m.

Question 10.
A boy stands at 78.4 m from a building and throws a ball which just enters a window 39.2 m above the ground. Calculate the velocity of the projection of the ball.
Answer:
Let the boy standing at A throw a ball with initial velocity u.
θ = angle of the projection made with the horizontal.

As the boy is at 78.4 m from the building and the ball just enters above the ground.

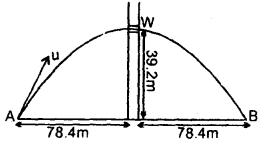
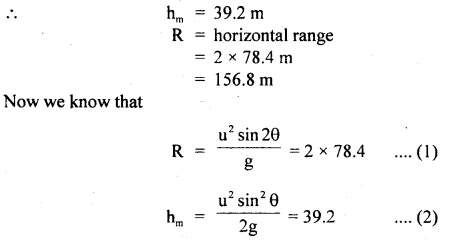
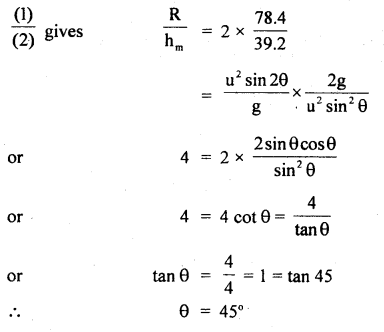
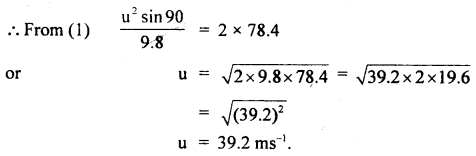
Question 11.
The equation of trajectory of an oblique projectile is
y = \(\sqrt{3}\)x – \(\frac{1}{2}\)gx²
What is the initial velocity in ms’ and the angle of projection of the projectile in degree?
Answer:
Comparing the given equation with the standard equation of the trajectory of an oblique projectile.

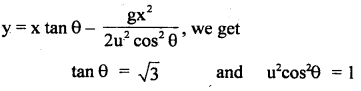
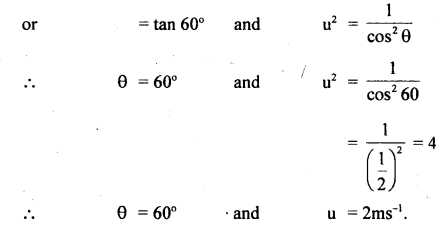
Question 12.
Two particles located at a point begin to move with velocities 4 ms^-1 and 1 ms^-1 horizontally in opposite directions. Determine the time when their velocity vectors become perpendicular. Assuming that the motion takes place in a uniform gravitational field of strength g.
Answer:
Let v₁ and v₂ be the velocities of first and 2nd particles respectively after a time t.
∴ v₁ = 4î – gt ĵ
v₂ = – î – gt ĵ
For v₁ and v₂ to be ⊥ to each other, then their dot product must be zero.

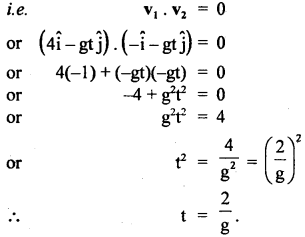
Question 13.
In the previous question, determine the distance between particles at t = \(\frac{2}{g}\).
Answer:
The separation between the particles is to take place only along the horizontal.
∴ the separation between the particles
= relative speed of the particles along horizontal × time

Question 14.
The maximum range of a projectile is range. What is the angle of projection for the actual range?
Answer:
We know that the horizontal range of a projectile is given by
R = \(\frac{\mathrm{u}^{2} \sin 2 \theta}{\mathrm{g}}\) …(i)

Where u = initial velocity of projection
θ = angle of projection with horizontal

Also, we know that the maximum horizontal range (Rmax) is given by

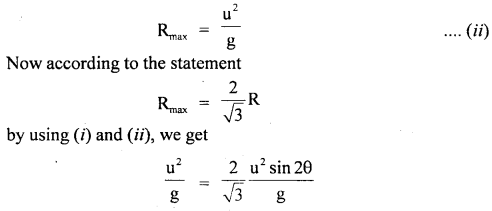
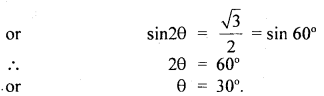
Question 15.
A body is projected with a velocity of 40 ms^-1. After two seconds, it crosses a verticle pole of 20.4 m. Find the angle of projection and the horizontal range.
Answer:
Here, u = 40 ms^-1
height of verticle pole, h = 20.4 m
t = 2 seconds

Let us take vertical motion

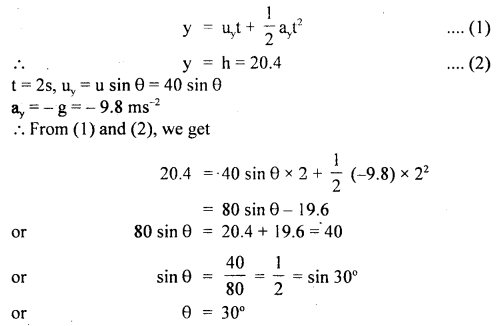
∴ The horizontal range is given by the relation,

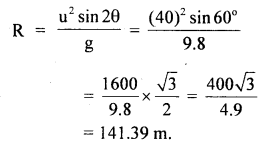
Question 16.
The greatest and the least resultant of two forces acting at a point are 29 N and 5 N respectively. If each force is increased by 3 N, find the resultant of two new forces when acting at a point at an angle of 90° with each other.
Answer:
Let A and B be the two forces.
∴ Greatest Resultant = A + B = 29 N ….(1)
least Resultant = A – B = 5 N ….(2)

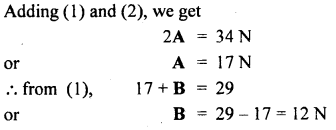
Let A and B be the new forces such that
A’ = A + 3 = 17 + 3 = 20N and
B’ = B + 3 = 12 + 3 = 15 N

Here, θ = angle between A’ and B’ = 90°
Let R be the resultant of A’ and B’.
∴ according to parallelogram law of vector addition

The direction of R:
Let β be the angle made by R with A’

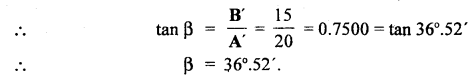
Question 17.
An aircraft is trying to fly due north at a speed of 100 ms^-1 but is subjected to a crosswind blowing from west to east at 50 ms^-1. What is the actual velocity of the aircraft relative to the surface of the earth?
Answer:
Let V_a and V_w be the velocities of aircraft and wind respectively.
∴ V_a = 100 ms^-1 along N direction
V_w = 50 ms^-1 along E direction

If V be the resultant velocity of the aircraft, then these may be represented as in the figure given below. So the magnitude of V is given by,


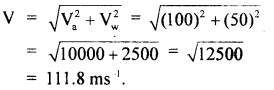
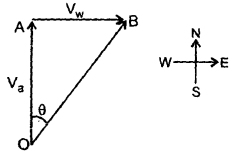
Let ∠AOB = θ be the angle which the resultant makes with the north direction.

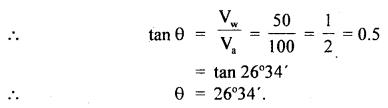
Question 18.
Calculate the total linear acceleration of a particle moving in a circle of radius 0.4 m at the instant when its angular velocity is 2 rad s^-1 and angular acceleration is 5 rad s^-2.
Answer:
Since the particle possesses angular acceleration, so its total linear acceleration (a) is the vector sum of the tangential acceleration (a,) and the centripetal acceleration (ac). a₁ and a_c, are at right angles to each other.
a = \(\sqrt{a_{t}^{2}+a_{c}^{2}}\) …. (1)

Now r = radius of the circular path = 0.4 m
ω = angular velocity of the particle = 2 rad s^-1
α = angular acceleration = 5 rad s^-2


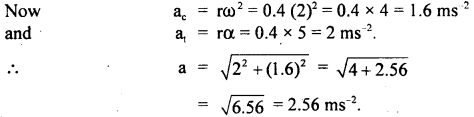
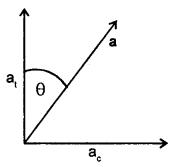
Let θ = angle made by a with a,

Question 19.
Calculate the greatest number of rotations per minute which can be given to a body of mass 500 gms tied to a string of length 1.5 m, if the string can withstand a maximum tension of 40 N.
Answer:
Here, m = mass of body = 500 gms = 0.5 kg
r = radius of circle = 1.5 m
F_c = Centripetal force = 40 N
Now we know that

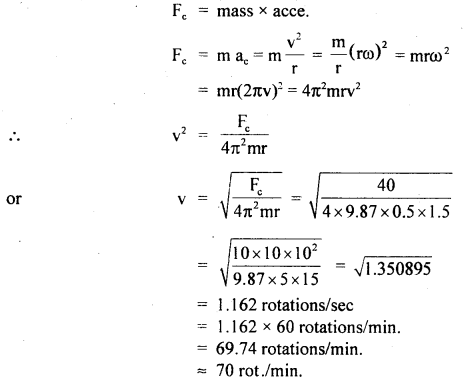
Hence the body can be given 70 rotations per minute without breaking the string.

Question 20.
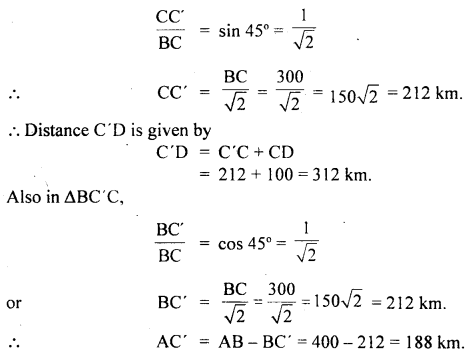
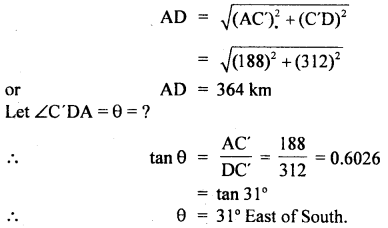
An airplane flies 400 km west from city A to city B then 300 km north-east to city C and finally 100 km north to city D. How far is it from city A to city D? In what direction must the airplane go to return directly to the city A from city D?
Answer:
Given, AB = 400 km
BC = 300 km
CD = 100 km
AD =?

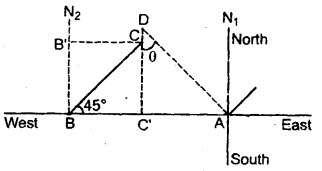
Let N₁, N₂ represent north directions.
∠ABC = 45°
Draw CC’ ⊥ AB, And CB’ ⊥ BN₂
Now in ΔBC’ C

From AAC’D, AD is given by

Question 21.
Which of the following quantities are independent of the choice of the orientation of the coordinates axes:
a + b, 3a_x + 2b_y, [a + b – c], angle between b and c, a?
Answer:
a + b, |a + b – c|, angle between b and c, a are the quantities that are independent of the choice of the orientation of the coordinate axes.

But the value of 3a_x + 2b_y depends on the orientation of the axes.

Question 22.
A machine gun is mounted on the top of a tower 100 m high. At what angle should the gun be inclined to cover a maximum range of firing on the ground below? The muzzle speed of the bullet is 150 ms^-1. (take g = 10 ms^-2)
Answer:
Here, u = 150 ms^-1
h = 100 m
g = 10 ms^-2

Let R = horizontal range of the projectile.
If t = time during which the bullet covers, horizontal range R = time during which the bullet falls through a height h (= 100 m).
Let θ = angle of projection with horizontal, then
u_x = u cos θ = 150 cos θ = horizontal component of speed of the projection,
u_y = 150 sin θ = vertical component of the speed of projection.

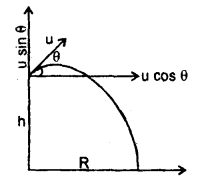
Considering the upward motion positive, then using the equation


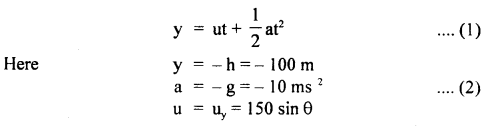
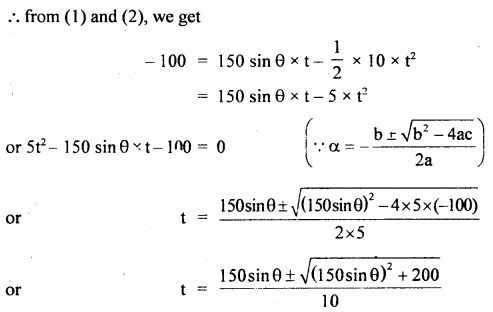
∴ The horizontal range covered by the (using positive sign only) projectile is given by
x = u_x × t

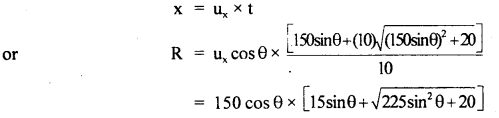
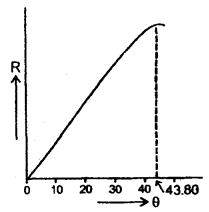
To maximize this function graphically, a graph between R and θ by taking arbitrary values of θ is plotted. We find from the graph that R is maximum for θ = 43.8°.

Question 23.
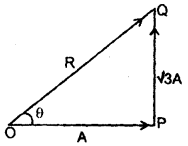
Two vectors of magnitude A and \(\sqrt{3}\) A are perpendicular to each other. What is the angle which their resultant makes with A?
Answer:
Let θ = angle made by R (resultant of A and \(\sqrt{3}\) A) with A
∴ In ΔOPQ

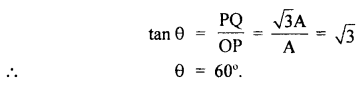
Question 24.
If |a + b| = |a – b|, then find the angle between a and b using properties of the dot product of vectors.
Answer:
Here, |a + b| = |a – b| …. (i) given
Squaring on both sides of eqn. (i), we get

Question 25.
The express cross product of two vectors in cartesian coordinates.
Answer:
Let A and B be the two vectors s.t.


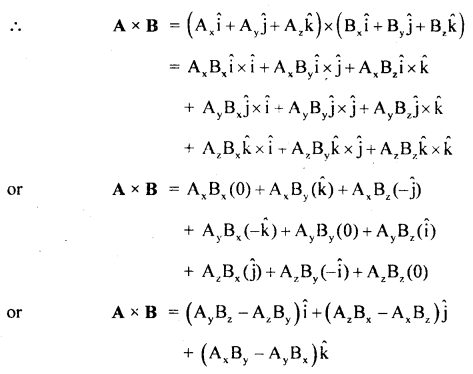
It can be written in a determinant form as

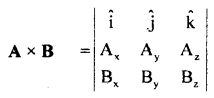
Value-Based Type:

Question 1.
Two friends Raman and Madhav arc playing on the bank of a river. They are throwing stones in the river. Raman is a student of the science stream whereas Madhav from the commerce stream. Every time Raman throws the stone far away than Madhav. Madhav was surprised and could not understand the reason. Finally, Raman explained the fact that it is relating to physics.
(i) What values are shown here by Roman?
Answer:
Intelligence, social, cooperative, and willing to share his knowledge,

(ii) Which concept is being used here?
Answer:
Horizontal range of a projectile

(iii) What is the minimum angle to get the maximum horizontal range?
Answer:
We know that: Maximum range: R = \(\frac{u^{2} \sin 2 \theta}{\mathrm{g}}\)

For maximum range
Sin 2θ = maximum value
i.e sin 2θ =1
⇒ sin 2θ = sin 90°
⇒ 2θ = 90° or θ =45°
Hence, if the body is projected at an angle of 45°, it will cause maximum horizontal range.

Question 2.
Himanshu and Shika are the two students of class XI. Himanshu fired a bullet at an angle of 30° with the horizontal which hits the ground 3 km away. Shika told that I could hit a bird that is 4 km away by adjusting its angle of projection. Himanshu immediately betted, He told if you can I would give you Its. 1000 otherwise you have to pay the same to me. Himanshu was sure that you can never hit that bird. However, killing a bird is not good for the environment. Assume the muzzle speed to be fixed, and neglect air resistance.
(i) Is betting a good practice?
Answer:
No

(ii) How Himanshu is sure that the bullet could not hit the target?
Answer:
Max horizonatal range = \(\frac{\mathrm{u}^{2}}{\mathrm{~g}}\) [∵ muzzle velocityin fixed]
ie R_max = \(\frac{\mathrm{u}^{2}}{\mathrm{~g}}\) = 2\(\sqrt{3}\) = 3.464 km g
So, the bullet can not hit the target which is 4 km away.

(iii) Which values are depicted in the above question?
Answer:
Values depicted are:
(a) Concern for environment and living creature
(b) Avoid betting
(c) Intelligent

Question 3.
Rain is falling vertically with a speed of 35 m s^-1. A girl of class XI rides a bicycle with a speed of 12 m s^-1 in the east to west direction. She could not understand that in which direction she should half her umbrella. When she came back to her home she asked her mother who was a physics teacher in a reputed school. She explained as under:
(i) Which value is displayed by the girl?
Answer:
Willing to know the scientific reason, curiosity

(ii) What justification was given by her mother?
Answer:
V_f = The velocity of rain
V_b = The velocity of bicycle
Both these velocities are with respect to the ground.
The velocity of rain relative to the velocity of the bicycle is given by
V_rb = V_r – V_b

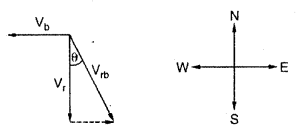
This relative velocity vector as shown above makes an angle θ with the vertical.
It is given by:

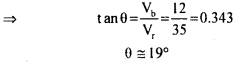
∴ The girl should hold her umbrella at an angle of about 19° with the vertical towards the west.

Question 4.
Sita a student of class XI was suffering from malaria. The area is full of mosquitoes. She was not having a mosquito net. Her friend Geeta has an extranet. She gave it to Sita. Also, she took Gita to Doctor, got her medicines. After a week Sita became normal.
(a) Comment upon the qualities of Sita.
Answer:
Sita has a caring attitude, and concern for others.

(b) The mosquito net over a 7 m × 4 m bed is 3 m high. The net has a hole at one corner of the bed through which a mosquito enters the net. It files and Sita at the diagonally opposite upper corner of the net
(i) Find the magnitude of the displacement of the mosquito
Answer:
We know that
A = \(\sqrt{\left(A_{x}\right)^{2}+\left(A_{y}\right)^{2}+\left(A_{x}\right)^{2}}=\sqrt{7^{2}+4^{2}+3^{2}}\)
= \(\sqrt{74}\) m

(ii) Taking the hole as the origin, the length of the bed as the X-axis, its width as the Y-axis and vertically up as the Z-axis, write the components of the displacement vector.
Answer:
The components of the vector are 7 m, 4 m, and 3 m