ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 18 Trigonometric Identities Ex 18
These Solutions are part of ML Aggarwal Class 10 Solutions for ICSE Maths. Here we have given ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 18 Trigonometric Identities Ex 18
More Exercises
- ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 18 Trigonometric Identities Ex 18
- ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 18 Trigonometric Identities MCQS
- ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 18 Trigonometric Identities Chapter Test
Question 1.
If A is an acute angle and sin A = \(\\ \frac { 3 }{ 5 } \) find all other trigonometric ratios of angle A (using trigonometric identities).
Solution:
sin A = \(\\ \frac { 3 }{ 5 } \)
In ∆ABC, ∠B = 90°
AC = 5 and BC = 3
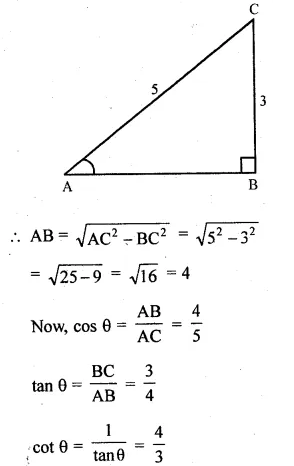
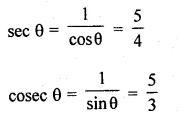
Question 2.
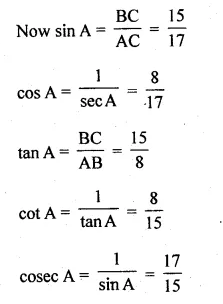
If A is an acute angle and sec A = \(\\ \frac { 17 }{ 8 } \), find all other trigonometric ratios of angle A (using trigonometric identities).
Solution:
sec A = \(\\ \frac { 17 }{ 8 } \) (A is an acute angle)
In right ∆ABC
sec A = \(\\ \frac { AC }{ AB } \) = \(\\ \frac { 17 }{ 8 } \)
AC = 17, AB = 8

Question 3.
Express the ratios cos A, tan A and sec A in terms of sin A.
Solution:
cos A = \(\sqrt { { 1-sin }^{ 2 }A } \)
tan A = \(\frac { SinA }{ CosA } =\frac { sinA }{ \sqrt { { 1-sin }^{ 2 }A } } \)
sec A = \(\frac { 1 }{ cosA } =\frac { 1 }{ \sqrt { { 1-sin }^{ 2 }A } } \)
Question 4.
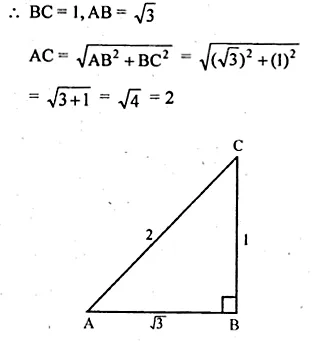
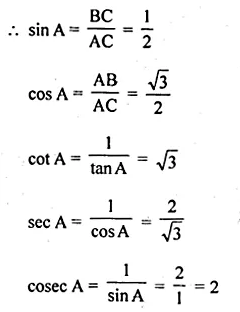
If tan A = \(\frac { 1 }{ \sqrt { 3 } } \), find all other trigonometric ratios of angle A.
Solution:
tan A = \(\frac { 1 }{ \sqrt { 3 } } \)
In right ∆ABC,
tan A = \(\\ \frac { BC }{ AB } \) = \(\frac { 1 }{ \sqrt { 3 } } \)
Question 5.
If 12 cosec θ = 13, find the value of \(\frac { 2sin\theta -3cos\theta }{ 4sin\theta -9cos\theta } \)
Solution:
12 cosec θ = 13
⇒ cosec θ = \(\\ \frac { 13 }{ 12 } \)
In right ∆ABC,
∠A = θ
cosec θ = \(\\ \frac { AC }{ BC } \) = \(\\ \frac { 13 }{ 12 } \)


Without using trigonometric tables, evaluate the following (6 to 10) :
Question 6.
(i) cos² 26° + cos 64° sin 26° + \(\frac { tan{ 36 }^{ O } }{ { cot54 }^{ O } } \)
(ii) \(\frac { sec{ 17 }^{ O } }{ { cosec73 }^{ O } } +\frac { tan68^{ O } }{ cot22^{ O } } \) + cos² 44° + cos² 46°
Solution:
Given that
(i) cos² 26° + cos 64° sin 26° + \(\frac { tan{ 36 }^{ O } }{ { cot54 }^{ O } } \)
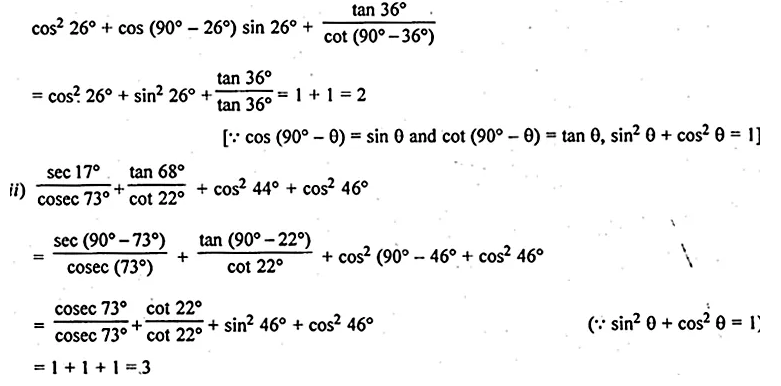
Question 7.
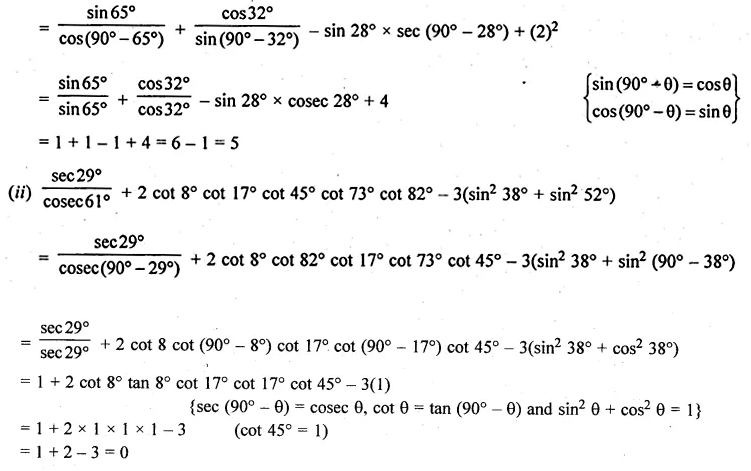
(i) \(\frac { sin65^{ O } }{ { cos25 }^{ O } } +\frac { cos32^{ O } }{ sin58^{ O } } \) – sin 28° sec 62° + cosec² 30° (2015)
(ii) \(\frac { sin29^{ O } }{ { cosec61 }^{ O } } \) + 2 cot 8° cot 17° cot 45° cot 73° cot 82° – 3(sin² 38° + sin² 52°).
Solution:
given that
(i) \(\frac { sin65^{ O } }{ { cos25 }^{ O } } +\frac { cos32^{ O } }{ sin58^{ O } } \) – sin 28° sec 62° + cosec² 30°
Question 8.
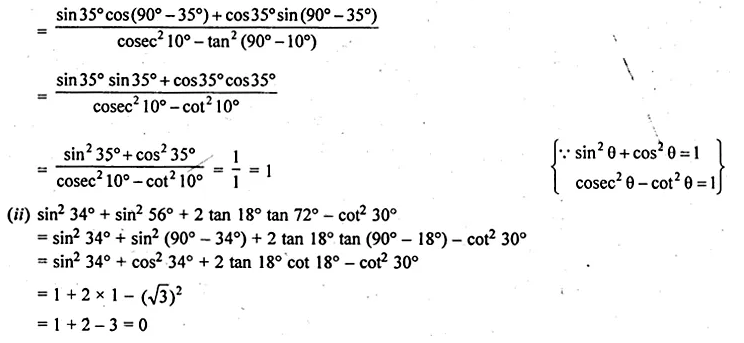
(i) \(\frac { { sin }35^{ O }{ cos55 }^{ O }+{ cos35 }^{ O }{ sin }55^{ O } }{ { cosec }^{ 2 }{ 10 }^{ O }-{ tan }^{ 2 }{ 80 }^{ O } } \)
(ii) \({ sin }^{ 2 }{ 34 }^{ O }+{ sin }^{ 2 }{ 56 }^{ O }+2tan{ 18 }^{ O }{ tan72 }^{ O }-{ cot }^{ 2 }{ 30 }^{ O }\)
Solution:
Given that
(i) \(\frac { { sin }35^{ O }{ cos55 }^{ O }+{ cos35 }^{ O }{ sin }55^{ O } }{ { cosec }^{ 2 }{ 10 }^{ O }-{ tan }^{ 2 }{ 80 }^{ O } } \)
Question 9.
(i) \({ \left( \frac { { tan25 }^{ O } }{ { cosec }65^{ O } } \right) }^{ 2 }+{ \left( \frac { { cot25 }^{ O } }{ { sec65 }^{ O } } \right) }^{ 2 }+{ 2tan18 }^{ O }{ tan }45^{ O }{ tan72 }^{ O } \)
(ii) \(\left( { cos }^{ 2 }25+{ cos }^{ 2 }65 \right) +cosec\theta sec\left( { 90 }^{ O }-\theta \right) -cot\theta tan\left( { 90 }^{ O }-\theta \right) \)
Solution:
(i) \({ \left( \frac { { tan25 }^{ O } }{ { cosec }65^{ O } } \right) }^{ 2 }+{ \left( \frac { { cot25 }^{ O } }{ { sec65 }^{ O } } \right) }^{ 2 }+{ 2tan18 }^{ O }{ tan }45^{ O }{ tan72 }^{ O } \)
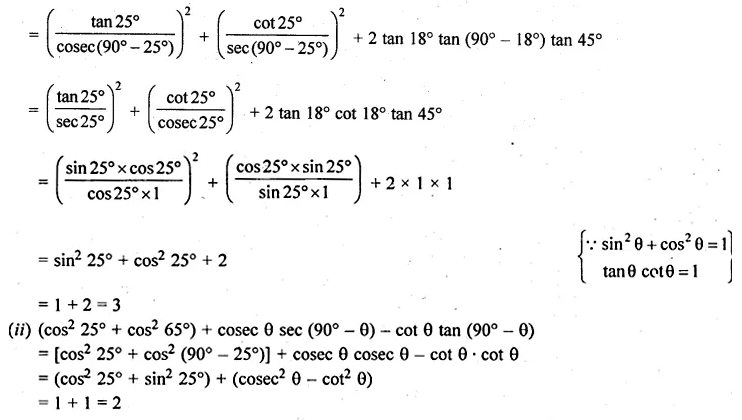
Question 10.
(i) 2(sec² 35° – cot² 55°) – \(\frac { { cos28 }^{ O }cosec{ 62 }^{ O } }{ { tan18 }^{ O }tan{ 36 }^{ O }{ tan30 }^{ O }{ tan54 }^{ O }{ tan72 }^{ O } } \)
(ii) \(\frac { { cosec }^{ 2 }(90-\theta )-{ tan }^{ 2 }\theta }{ 2({ cos }^{ 2 }{ 48 }^{ O }+{ cos }^{ 2 }{ 42 }^{ O }) } -\frac { { 2tan }^{ 2 }{ 30 }^{ O }{ sec }^{ 2 }{ 52 }^{ O }{ sin }^{ 2 }{ 38 }^{ O } }{ { cosec }^{ 2 }{ 70 }^{ O }-{ tan }^{ 2 }{ 20 }^{ O } } \)
Solution:
(i) 2(sec² 35° – cot² 55°) – \(\frac { { cos28 }^{ O }cosec{ 62 }^{ O } }{ { tan18 }^{ O }tan{ 36 }^{ O }{ tan30 }^{ O }{ tan54 }^{ O }{ tan72 }^{ O } } \)
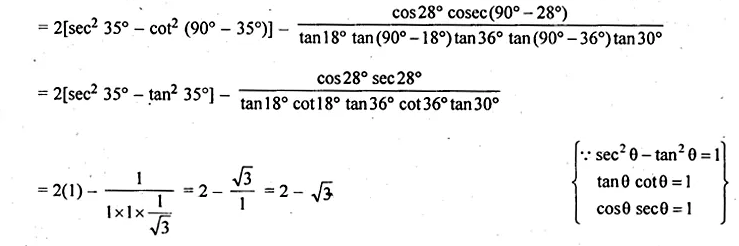
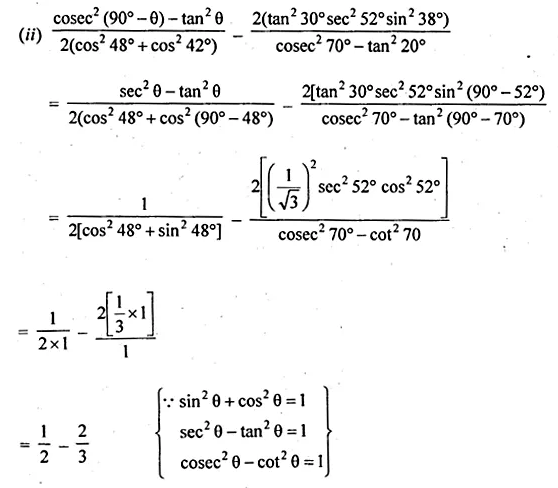
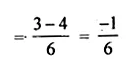
Question 11.
Prove that following:
(i) cos θ sin (90° – θ) + sin θ cos (90° – θ) = 1
(ii) \(\frac { tan\theta }{ tan({ 90 }^{ O }-\theta ) } +\frac { sin({ 90 }^{ O }-\theta ) }{ cos\theta } ={ sec }^{ 2 }\theta \)
(iii) \(\frac { cos({ 90 }^{ O }-\theta )cos\theta }{ tan\theta } +{ cos }^{ 2 }({ 90 }^{ O }-\theta )=1\)
(iv) sin (90° – θ) cos (90° – θ) = \(\frac { tan\theta }{ { 1+tan }^{ 2 }\theta } \)
Solution:
(i) cos θ sin (90° – θ) + sin θ cos (90° – θ) = 1
L.H.S. = cos θ sin (90° – θ) + sin θ cos (90° – θ)
= cos θ . cos θ + sin θ . sin θ
= cos2 θ + sin2 θ = 1 = R.H.S.

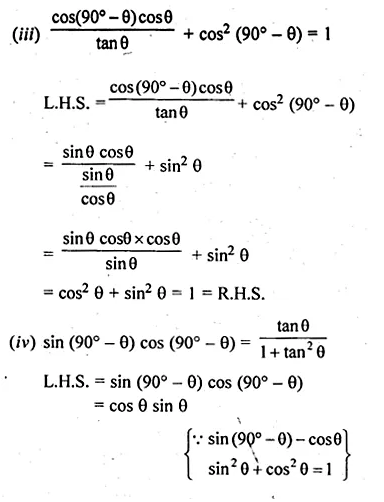
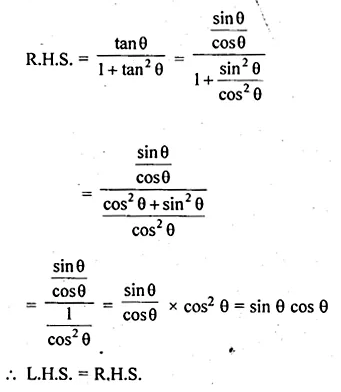
Prove that following (12 to 30) identities, where the angles involved are acute angles for which the trigonometric ratios as defined:
Question 12.
(i) (sec A + tan A) (1 – sin A) = cos A
(ii) (1 + tan2 A) (1 – sin A) (1 + sin A) = 1.
Solution:
(i) (sec A + tan A) (1 – sin A) = cos A
L.H.S. = (sec A + tan A) (1 – sin A)
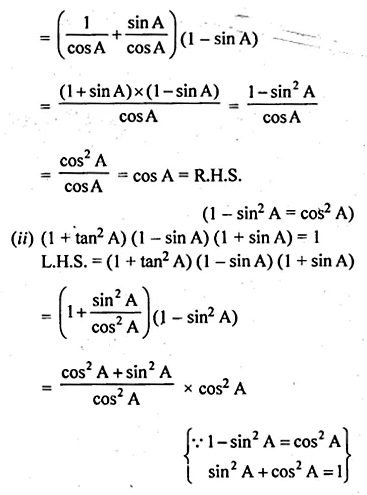
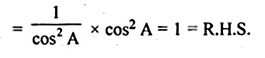
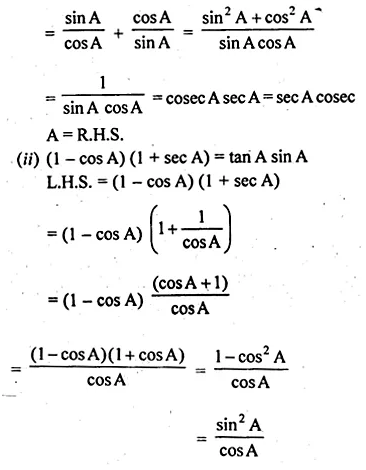
Question 13.
(i) tan A + cot A = sec A cosec A
(ii) (1 – cos A)(1 + sec A) = tan A sin A.
Solution:
(i) tan A + cot A = sec A cosec A
L.H.S. = tan A + cot A

Question 14.
(i) \(\frac { 1 }{ 1+cosA } +\frac { 1 }{ 1-cosA } =2{ cosec }^{ 2 }A\)
(ii) \(\frac { 1 }{ secA+tanA } +\frac { 1 }{ secA-tanA } =2{ sec }A\)
Solution:
(i) \(\frac { 1 }{ 1+cosA } +\frac { 1 }{ 1-cosA } =2{ cosec }^{ 2 }A\)
L.H.S = \(\frac { 1 }{ 1+cosA } +\frac { 1 }{ 1-cosA }\)
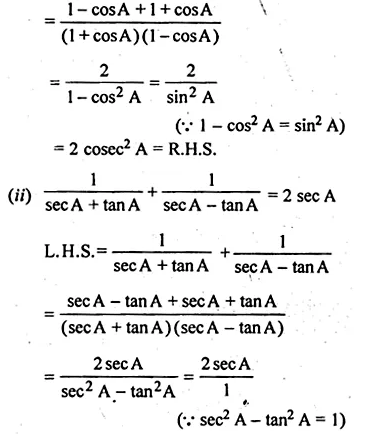
![]()
Question 15.
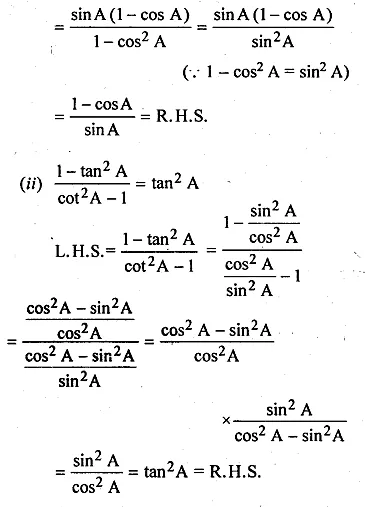
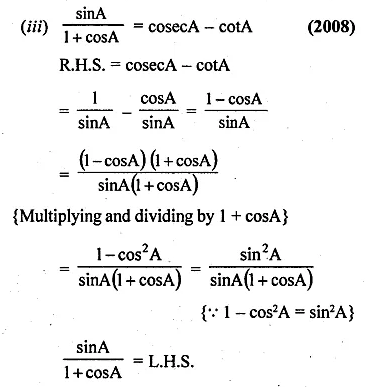
(i) \(\frac { sinA }{ 1+cosA } =\frac { 1-cosA }{ sinA } \)
(ii) \(\frac { 1-{ tan }^{ 2 }A }{ { cot }^{ 2 }A-1 } ={ tan }^{ 2 }A\)
(iii) \(\frac { sinA }{ 1+cosA } =cosecA-cotA\)
Solution:
(i) \(\frac { sinA }{ 1+cosA } =\frac { 1-cosA }{ sinA } \)
L.H.S = \(\frac { sinA }{ 1+cosA } \)
(multiplying and dividing by (1 – cosA))
Question 16.
(i) \(\frac { secA-1 }{ secA+1 } =\frac { 1-cosA }{ 1+cosA } \)
(ii) \(\frac { { tan }^{ 2 }\theta }{ { (sec\theta -1) }^{ 2 } } =\frac { 1+cos\theta }{ 1-cos\theta } \)
(iii) \({ (1+tanA) }^{ 2 }+{ (1-tanA) }^{ 2 }=2{ sec }^{ 2 }A\)
(iv) \({ sec }^{ 2 }A+{ cosec }^{ 2 }A={ sec }^{ 2 }A{ .cosec }^{ 2 }A\)
Solution:
(i) \(\frac { secA-1 }{ secA+1 } =\frac { 1-cosA }{ 1+cosA } \)
L.H.S = \(\frac { secA-1 }{ secA+1 } \)
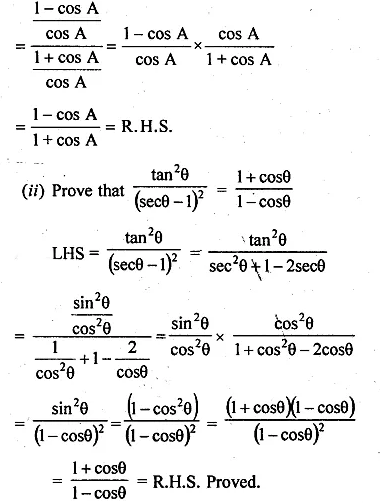
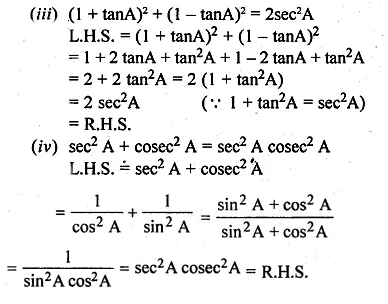
Question 17.
(i) \(\frac { 1+sinA }{ cosA } +\frac { cosA }{ 1+sinA } =2secA \)
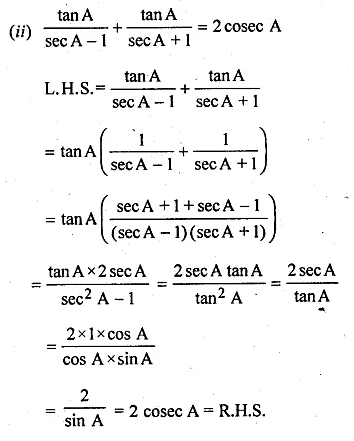
(ii) \(\frac { tanA }{ secA-1 } +\frac { tanA }{ secA+1 } =2cosecA\)
Solution:
(i) \(\frac { 1+sinA }{ cosA } +\frac { cosA }{ 1+sinA } =2secA \)
L.H.S = \(\frac { 1+sinA }{ cosA } +\frac { cosA }{ 1+sinA } \)
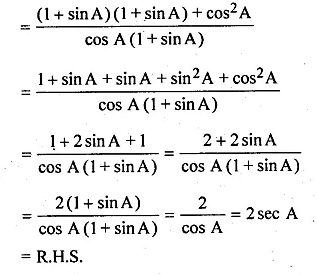
Question 18.
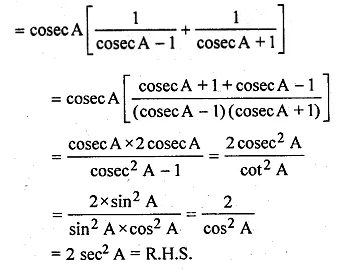
(i) \(\frac { cosecA }{ cosecA-1 } +\frac { cosecA }{ cosecA+1 } =2{ sec }^{ 2 }A\)
(ii) \(cotA-tanA=\frac { { 2cos }^{ 2 }A-1 }{ sinA-cosA } \)
(iii) \(\frac { cotA-1 }{ 2-{ sec }^{ 2 }A } =\frac { cotA }{ 1+tanA } \)
Solution:
(i) \(\frac { cosecA }{ cosecA-1 } +\frac { cosecA }{ cosecA+1 } =2{ sec }^{ 2 }A\)
L.H.S = \(\frac { cosecA }{ cosecA-1 } +\frac { cosecA }{ cosecA+1 } \)
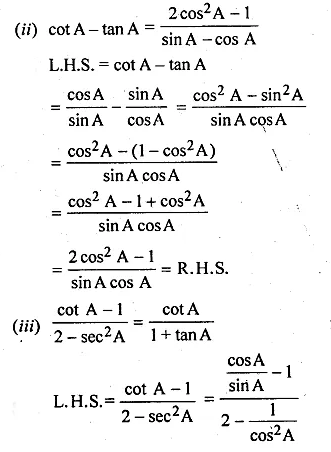
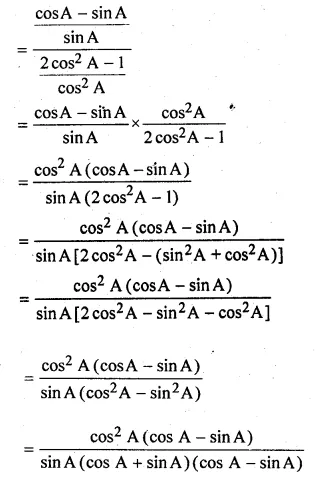
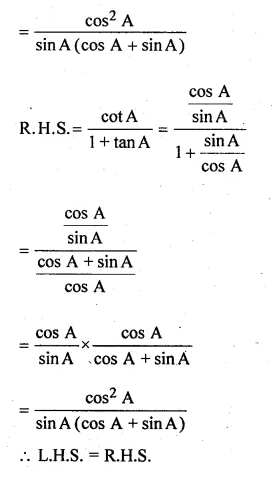
Question 19.
(i) \({ tan }^{ 2 }\theta -{ sin }^{ 2 }\theta ={ tan }^{ 2 }\theta { sin }^{ 2 }\theta \)
(ii) \(\frac { cos\theta }{ 1-tan\theta } -\frac { { sin }^{ 2 }\theta }{ cos\theta -sin\theta } =cos\theta +sin\theta \)
Solution:
(i) \({ tan }^{ 2 }\theta -{ sin }^{ 2 }\theta ={ tan }^{ 2 }\theta { sin }^{ 2 }\theta \)
L.H.S = \({ tan }^{ 2 }\theta -{ sin }^{ 2 }\theta \)
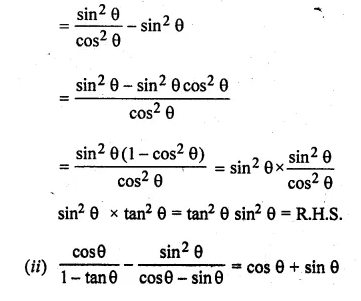

Question 20.
(i) cosec4 θ – cosec2 θ = cot4 θ + cot2 θ
(ii) 2 sec2 θ – sec4 θ – 2 cosec2 θ + cosec4 θ = cot4 θ – tan4 θ.
Solution:
(i) cosec4 θ – cosec2 θ = cot4 θ + cot2 θ
L.H.S = cosec4 θ – cosec2 θ
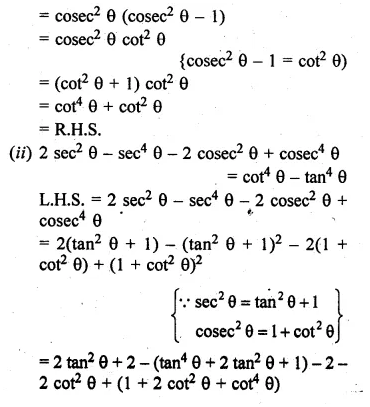
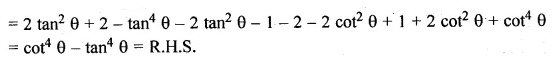
Question 21.
(i) \(\frac { 1+cos\theta -{ sin }^{ 2 }\theta }{ sin\theta (1+cos\theta ) } =cot\theta \)
(ii) \(\frac { { tan }^{ 3 }\theta -1 }{ tan\theta -1 } ={ sec }^{ 2 }\theta +tan\theta \)
Solution:
(i) \(\frac { 1+cos\theta -{ sin }^{ 2 }\theta }{ sin\theta (1+cos\theta ) } =cot\theta \)
L.H.S = \(\frac { 1+cos\theta -{ sin }^{ 2 }\theta }{ sin\theta (1+cos\theta ) }\)

Question 22.
(i) \(\frac { 1+cosecA }{ cosecA } =\frac { { cos }^{ 2 }A }{ 1-sinA } \)
(ii) \(\sqrt { \frac { 1-cosA }{ 1+cosA } } =\frac { sinA }{ 1+cosA } \)
Solution:
(i) \(\frac { 1+cosecA }{ cosecA } =\frac { { cos }^{ 2 }A }{ 1-sinA } \)
L.H.S = \(\frac { 1+cosecA }{ cosecA }\)

Question 23.
(i) \(\sqrt { \frac { 1+sinA }{ 1-sinA } } =tanA+secA\)
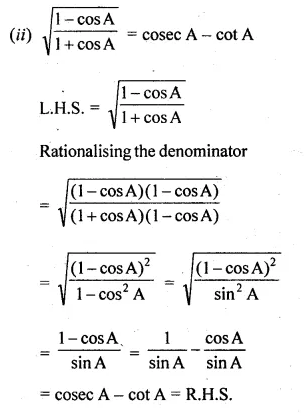
(ii) \(\sqrt { \frac { 1-cosA }{ 1+cosA } } =cosecA-cotA\)
Solution:
(i) \(\sqrt { \frac { 1+sinA }{ 1-sinA } } =tanA+secA\)
L.H.S = \(\sqrt { \frac { 1+sinA }{ 1-sinA } } \)
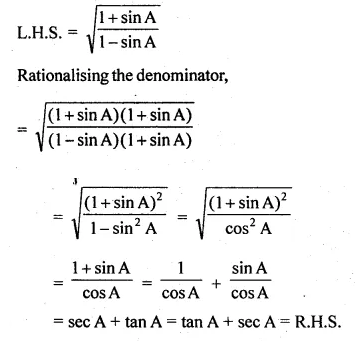
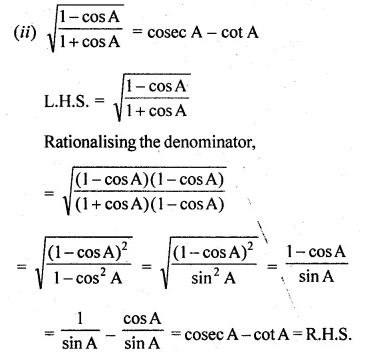
Question 24.
(i) \(\sqrt { \frac { secA-1 }{ secA+1 } } +\sqrt { \frac { secA+1 }{ secA-1 } } =2cosecA\)
(ii) \(\frac { cotAcotA }{ 1-sinA } =1+cosecA \)
Solution:
(i) \(\sqrt { \frac { secA-1 }{ secA+1 } } +\sqrt { \frac { secA+1 }{ secA-1 } } =2cosecA\)
L.H.S = \(\sqrt { \frac { secA-1 }{ secA+1 } } +\sqrt { \frac { secA+1 }{ secA-1 } } \)
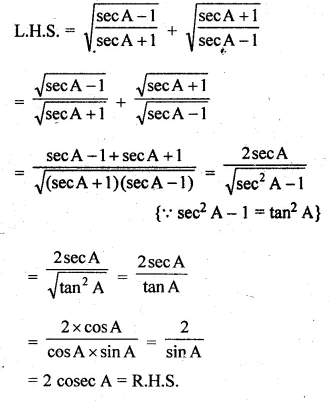
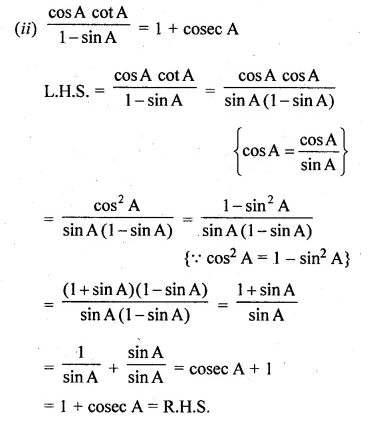
Question 25.
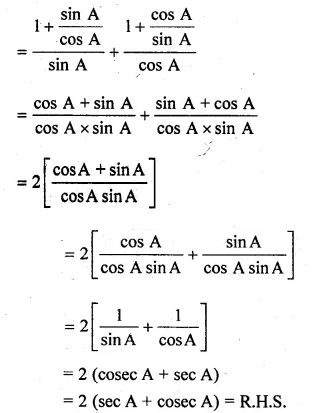
(i) \(\frac { 1+tanA }{ sinA } +\frac { 1+cotA }{ cosA } =2(secA+cosecA)\)
(ii) \({ sec }^{ 4 }A-{ tan }^{ 4 }A=1+2{ tan }^{ 2 }A \)
Solution:
(i) \(\frac { 1+tanA }{ sinA } +\frac { 1+cotA }{ cosA } =2(secA+cosecA)\)
L.H.S = \(\frac { 1+tanA }{ sinA } +\frac { 1+cotA }{ cosA } \)
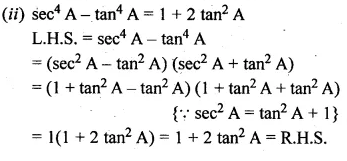
Question 26.
(i) cosec6 A – cot6 A = 3 cot2 A cosec2 A + 1
(ii) sec6 A – tan6 A = 1 + 3 tan2 A + 3 tan4 A
Solution:
(i) cosec6 A – cot6 A = 3 cot2 A cosec2 A + 1
L.H.S = cosec6 A – cot6 A
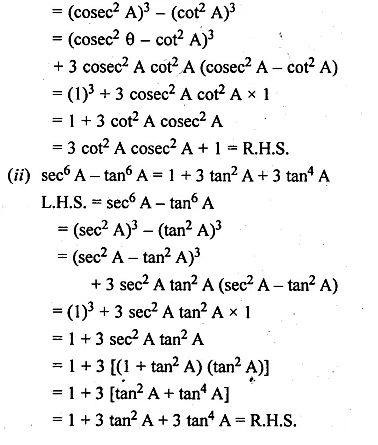
Question 27.
(i) \(\frac { cot\theta -cosec\theta -1 }{ cot\theta -cosec\theta +1 } =\frac { 1+cos\theta }{ sin\theta } \)
(ii) \(\frac { sin\theta }{ cot\theta +cosec\theta } =2+\frac { sin\theta }{ cot\theta -cosec\theta } \)
Solution:
(i) \(\frac { cot\theta -cosec\theta -1 }{ cot\theta -cosec\theta +1 } =\frac { 1+cos\theta }{ sin\theta } \)
L.H.S = \(\frac { cot\theta -cosec\theta -1 }{ cot\theta -cosec\theta +1 }\)

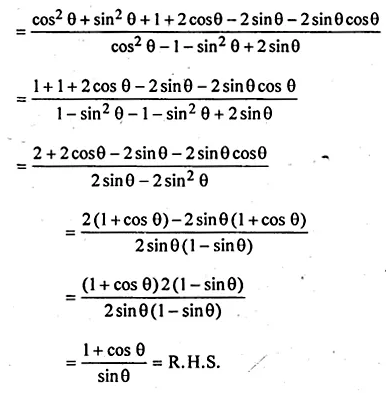
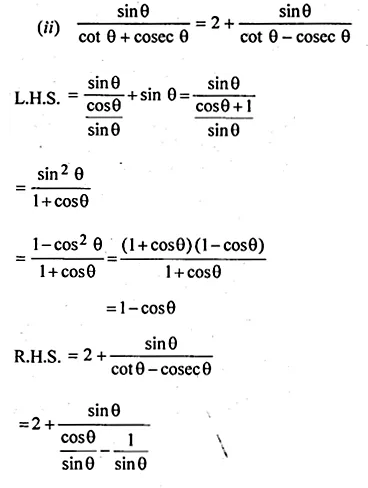
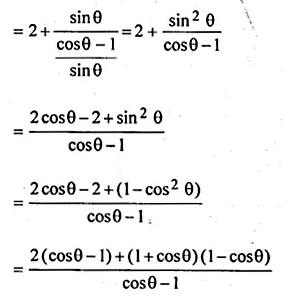
Question 28.
(i) (sinθ + cosθ)(secθ + cosecθ) = 2 + secθ cosecθ
(ii) (cosecA – sinA)(secA – cosA) sec2A = tanA
(iii) (cosecθ – sinθ)(secθ – cosθ)(tan θ + cotθ) = 1
Solution:
(i) (sinθ + cosθ)(secθ + cosecθ) = 2 + secθ cosecθ
L.H.S = (sinθ + cosθ)(secθ + cosecθ)
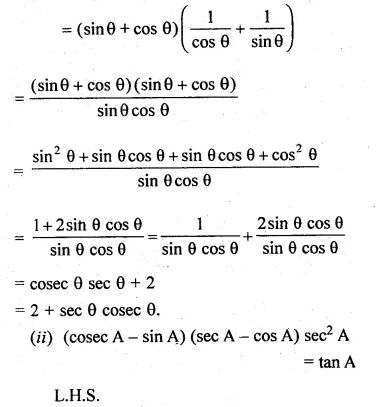

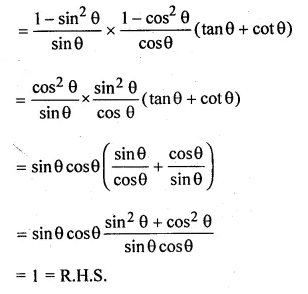
Question 29.
(i) \(\frac { { sin }^{ 3 }A+{ cos }^{ 3 }A }{ sinA+cosA } +\frac { { sin }^{ 3 }A-{ cos }^{ 3 }A }{ sinA-cosA } =2\)
(ii) \(\frac { { tan }^{ 2 }A }{ { 1+tan }^{ 2 }A } +\frac { cot^{ 2 }A }{ 1+{ cot }^{ 2 }A } =1\)
Solution:
(i) \(\frac { { sin }^{ 3 }A+{ cos }^{ 3 }A }{ sinA+cosA } +\frac { { sin }^{ 3 }A-{ cos }^{ 3 }A }{ sinA-cosA } =2\)
L.H.S = \(\frac { { sin }^{ 3 }A+{ cos }^{ 3 }A }{ sinA+cosA } +\frac { { sin }^{ 3 }A-{ cos }^{ 3 }A }{ sinA-cosA } \)
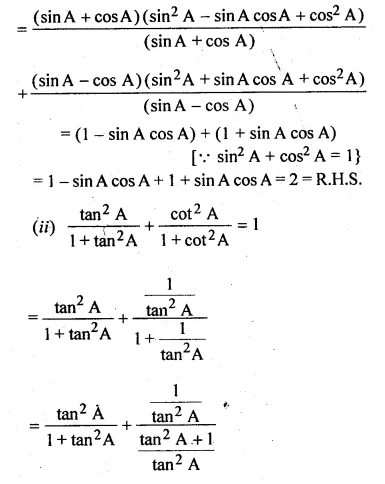

Question 30.
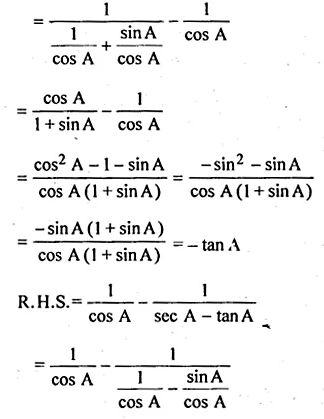
(i) \(\frac { 1 }{ secA+tanA } -\frac { 1 }{ cosA } =\frac { 1 }{ cosA } -\frac { 1 }{ secA-tanA } \)
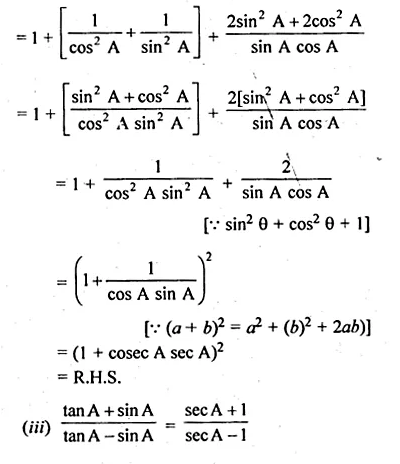
(ii) \({ (sinA+secA) }^{ 2 }+{ (cosA+cosecA) }^{ 2 }={ (1+secA\quad cosecA) }^{ 2 }\)
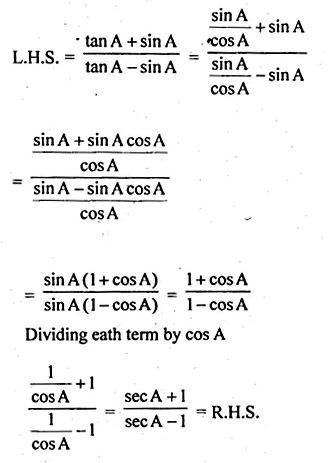
(iii) \(\frac { tanA+sinA }{ tanA-sinA } =\frac { secA+1 }{ secA-1 } \)
Solution:
(i) \(\frac { 1 }{ secA+tanA } -\frac { 1 }{ cosA } =\frac { 1 }{ cosA } -\frac { 1 }{ secA-tanA } \)
L.H.S = \(\frac { 1 }{ secA+tanA } -\frac { 1 }{ cosA } \)

Question 31.
If sin θ + cos θ = √2 sin (90° – θ), show that cot θ = √2 + 1
Solution:
sin θ + cos θ = √2 sin (90° – θ)
sin θ + cos θ = √2 cos θ
dividing by sin θ
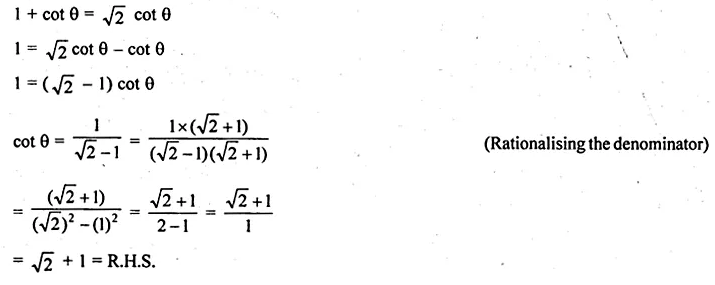
Question 32.
If 7 sin2 θ + 3 cos2 θ = 4, 0° ≤ θ ≤ 90°, then find the value of θ.
Solution:
7 sin2 θ + 3 cos2 θ = 4, 0° ≤ θ ≤ 90°
3 sin2 θ + 3 cos2 θ + 4 sin2 θ = 4
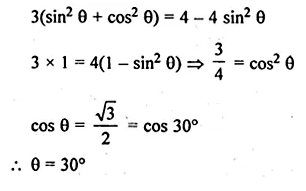
Question 33.
If sec θ + tan θ = m and sec θ – tan θ = n, prove that mn = 1.
Solution:
sec θ + tan θ = m and sec θ – tan θ = n
mn = (sec θ + tan θ) (sec θ – tan θ) = sec2 θ – tan2 θ = 1
(∴ sec2 θ – tan2 θ = 1)
Hence proved.
Question 34.
If x – a sec θ + b tan θ and y = a tan θ + b sec θ, prove that x2 – y2 = a2 – b2.
Solution:
x – a sec θ + b tan θ and y = a tan θ + b sec θ
To prove that x2 – y2 = a2 – b2.
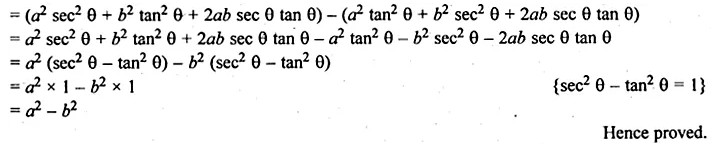
Question 35.
If x = h + a cos θ and y = k + a sin θ, prove that (x – h)2 + (y – k)2 = a2.
Solution:
x = h + a cos θ and y = k + a sin θ
To prove that (x – h)2 + (y – k)2 = a2.

We hope the ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 18 Trigonometric Identities Ex 18 help you. If you have any query regarding ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 18 Trigonometric Identities Ex 18, drop a comment below and we will get back to you at the earliest.