Free PDF Download of CBSE Maths Multiple Choice Questions for Class 12 with Answers Chapter 12 Linear Programming. Maths MCQs for Class 12 Chapter Wise with Answers PDF Download was Prepared Based on Latest Exam Pattern. Students can solve NCERT Class 12 Maths Linear Programming MCQs Pdf with Answers to know their preparation level.
Linear Programming Class 12 Maths MCQs Pdf
1. Of all the points of the feasible region, for maximum or minimum of objective function, the point lies
(a) inside the feasible region
(b) at the boundary line of the feasible region
(c) vertex point of the boundary of the feasible region
(d) none of these
Answer
Answer: c
2. A dealer wishes to purchase a number of fans and sewing machines. He has only ₹ 5,760 to invest and has space for at most 20 items. A fan costs him ₹ 360 and a sewing machine ₹ 240. He expects to sell a fan at a profit of ₹ 22 and a sewing machine at a profit of ₹ 18. Assinning that he can sell all the items that he buys, how should he invests his money to maximise the profit? The LPP for above question is
(a) x → fans, y → sewing machines
To maximise Z = 22x + 18y
subject to constraints
x ≥ 0, y ≥ 0, x + y ≤ 20
360x + 240y ≥ 5760
(b) x → fans, y → sewing machines
To maximise Z= 18x + 22y subject to constraints
x ≥ 0, y ≥ 0, x + y ≤ 20 360x + 240y ≥ 5760
(c) x → fans, y → sewing machines
To maximise Z = 22x + 18y
x ≥ 0, y ≥ 0, x + y ≥ 0
360x + 240y ≥ 5760
(d) x → fans, y → sewing machines
To maximise Z = 22x + 18j
x ≥ 0, y ≥ 0, x + y ≤ 20 360x + 240y ≤ 5760
Answer
Answer: d
3. Feasible region is the set of points which satisfy
(a) the objective functions
(b) some of the given constraints
(c) all of the given constraints
(d) none of these
Answer
Answer: c
4. An aeroplane can carry a maximum of 200 passangers. A profit of ₹ 400 is made on each first class ticket and a profit of ₹ 300 is made on each economy class ticket. An airline reserves at least 20 seats for first class. However, at least 4 times as many passengers prefer to travel by economy class than by first class. Find how many tickets of each type must be sold to maximise the profit? The LPP for the given situation is
(a) x → first class, y → economy class
To maximise Z = 400x + 300y
subject to constraints
x ≥ 0, y ≥ 0, x+y ≤ 200
x ≥ 20, y ≥ 80
(b) x → first class, y → economy class
To maximise Z 400x + 300y
subject to constraints
x ≥ 0, y ≥ 0, x+y ≥ 200
x ≥ 20, y ≥ 80
(c) x → first class, y → economy class
To maximise Z = 400x + 300y
subject to constraints
x ≥ 0, y ≥ 0, x ≥ 20
x + y ≤ 200
x ≥ 4y
(d) x → first class, y → economy class
To maximise Z = 400x + 300y
subject to constraints
x ≥ 20, y ≥ 0
x + y ≤ 200
y ≥ 4x
Answer
Answer: d
5. A manufacturer makes two types of furniture, chairs and tables. Both the products are processed on three machines A1 A2 and A3. Machine A1 requires 3 hours for a chair and 3 hours for a table, machine A2 requires 5 hours for a chair and 2 hours for a table and machine A3 requires 2 hours for a chair and 6 hours for a table. Maximum time available on machine A1, A2 and A3 is 36 hours, 50 hours and 60 hours respectively. Profits are ₹ 20 per chair and ₹ 30 per table. Formulate the above as a linear programming problem to maximise the profit. [HOTS]
Answer/Explanation
Answer:
Explaination:
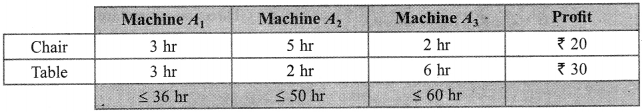
Let x chairs and y tables are manufactured.
Then LPP is Maximise P = 20x + 30y
subject to the constraints, x ≥ 0, y ≥ 0, 3x + 3y ≤ 36, 5x + 2y ≤ 50, 2x + 6y ≤ 60.
6. One kind of cake requires 200 g of flour and 25 g of fat and another kind of cake requires 100 g of flour and 50 g of fat, 5 kg of flour and 1 kg of fat is available, formulate the problem to find the maximum number of cakes which can be made, assuming that there is no shortage of the other ingredients used in making the cakes.
Answer/Explanation
Answer:
Explaination:
Let x cakes of kind I and y cakes of kind II are made.
Then LPP is
Maximise Z = x + y
subject to the constraints x ≥ 0, y ≥ 0
200x + 100y ≤ 5000
25x + 50y ≤ 1000
7. A small firm manufactures necklaces and bracelets. The total number of necklaces and bracelets that it can handle per day is at mo£ 24. It takes one hour to make a bracelet and half an hour to make a necklace. The maximum number of hours available per day is 16. If the profit on a necklace is ₹ 100 and that on a bracelet is ₹ 300. Formulate an LPP for finding how many of each should be produced daily to maximise the profit? It is being given that at lea£ one of each muft be produced. [Delhi 2017]
Answer/Explanation
Answer:
Explaination:
Let x necklaces and y bracelets be produced.
Then LPP is
To maximise profit
Z =100x + 300y
subject to constraints
x ≥ 1, y ≥ 1
x + y ≤ 24; \(\frac{1}{2}\)x + y ≤ 16
8. Two tailors, A and B, earn ₹ 300 and ₹ 400 per day respectively. A can stitch 6 shirts and 4 pairs of trousers while B can stitch 10 shirts and 4 pairs of trousers per day. To find how many days should each of them work and if it is desired to produce at least 60 shirts and 32 pairs of trousers at a minimum labour cost, formulate this as an LPP. [AI 2017]
Answer/Explanation
Answer:
Explaination:
Let A works for x days and B for y days
∴ LPP is to minimise Z = 300x + 400y
subject to the constraints 6x + 10y ≥ 60
4x + 4y ≥ 32
x ≥ 0, y ≥ 0
We hope the given Maths MCQs for Class 12 with Answers Chapter 12 Linear Programming will help you. If you have any query regarding CBSE Class 12 Maths Linear Programming MCQs Pdf, drop a comment below and we will get back to you at the earliest.