On this page, you will find Pair of Linear Equations in Two Variables Class 10 Notes Maths Chapter 3 Pdf free download. CBSE NCERT Class 10 Maths Notes Chapter 3 Pair of Linear Equations in Two Variables will seemingly help them to revise the important concepts in less time.
CBSE Class 10 Maths Chapter 3 Notes Pair of Linear Equations in Two Variables
Pair of Linear Equations in Two Variables Class 10 Notes Understanding the Lesson
Two linear equations in the same two variables are called a pair of linear equations in two variables. The most general form of a pair of linear equation is
a1x + b1y + c1 = 0
a2x+ b2y + c2 = 0
where a1,a2, b1,b2, c1 c2 are real numbers. For the pair of linear equations, the following situations can arise:
(i) \(\frac{a_{1}}{a_{2}} \neq \frac{b_{1}}{b_{2}}\) In this case, the pair of linear equations is consistent.
(ii) \(\frac{a_{1}}{a_{2}}=\frac{b_{1}}{b_{2}} \neq \frac{c_{1}}{c_{2}}\) The pair of linear equations in inconsistent.
(iii) \(\frac{a_{1}}{a_{2}}=\frac{b_{1}}{b_{2}}=\frac{c_{1}}{c_{2}}\) The pair of linear equations is consistent.
2. A pair of linear equations in two variables, can be represented, and solved by the
- Graphical method
- Algebraic method
3. Graphical Method: The graph of a pair of linear equations in two variables is represented by two lines, following three possibilities can occur.
- Two lines intersect at one point, then that point gives the unique solution of the two equations and the pair of equations is consistent.
- Two lines will not intersect, i.e. they are parallel, the pair of linear equations is inconsistent and the pair of equations will have no solution.
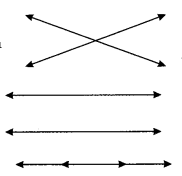
- The graph will be a pair of coincident lines. Each point on the lines will be a solution, so the pair of equations will have infinitely many solution and is consistent.
4. Algebraic Method: A pair of linear equations can be solved by any of the following three methods:
- Substitution method
- Elimination method
- Cross-multiplication method
5. Graphical Method of Solution of a pair of Linear Equations:
If the lines represented by the pair of linear equations in two variables are given by
a1x + b1y + c1 = 0
a2x+ b2y + c2 = 0
Following are the cases:
(i) If \(\frac{a_{1}}{a_{2}} \neq \frac{b_{1}}{b_{2}}\) then the lines are intersecting lines and intersect at one point. In this case, the pair of linear equations in consistent.
(ii) If \(\frac{a_{1}}{a_{2}}=\frac{b_{1}}{b_{2}}=\frac{c_{1}}{c_{2}}\), then the lines are coincident. In this case, the pair of linear equation is consistent (dependent)
(iii) If \(\frac{a_{1}}{a_{2}}=\frac{b_{1}}{b_{2}} \neq \frac{c_{1}}{c_{2}}\) then the lines are parallel to each other. In this case, the pair of linear equations inconsistent.