Here we are providing Areas Related to Circles Class 10 Extra Questions Maths Chapter 12 with Answers Solutions, Extra Questions for Class 10 Maths was designed by subject expert teachers. https://ncertmcq.com/extra-questions-for-class-10-maths/
Extra Questions for Class 10 Maths Areas Related to Circles with Answers Solutions
Extra Questions for Class 10 Maths Chapter 12 Areas Related to Circles with Solutions Answers
Areas Related to Circles Class 10 Extra Questions Very Short Answer Type
Area Related To Circle Class 10 Extra Questions With Solutions Question 1.
Find the area of a square inscribed in a circle of diameter p cm.

Solution:
Diagonal of the square = p cm
∴ p2 = side2 + side2
⇒ p2 = 2side2
or side2 = \(\frac{p^{2}}{2}\) cm2 = area of the square
Areas Related To Circles Class 10 Extra Questions Question 2.
Find the area of the circle inscribed in a square of side a cm.

Solution:
Diameter of the circle = a
![]()
Areas Related To Circles Extra Questions Question 3.
Find the area of a sector of a circle whose radius is and length of the arc is l.
Solution:
Area ola sector ola circle with radius r
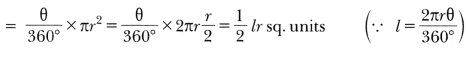
Area Related To Circle Class 10 Extra Questions Question 4.
Find the ratio of the areas of a circle and an equilateral triangle whose diameter and a side are respectively equal.
Solution:

Areas Related To Circles Class 10 Extra Questions With Answers Question 5.
A square inscribed in a circle of diameter d and another square is circumscribing the circle. Show that the area of the outer square is twice the area of the inner square.

Solution:
Side of outer square = d {Fig. 12.5]
∴ Its area = d
Diagonal of inner square = d
∴ Side = \(\frac{d}{\sqrt{2}}\)
⇒ Area = \(\frac{d^{2}}{2}\)
Area of outer square = 2 × Area of inner square.
Class 10 Maths Chapter 12 Extra Questions Question 6.
If circumference and the area of a circle are numerically equal, find the diameter of the circle.
Solution:
Given, 2πr = πr2
⇒ 2r = r2
⇒ r(r – 2) = 0 or r = 2
i.e. d = 4 units
Area Related To Circles Extra Questions Question 7.
The radius of a wheel is 0.25 m. Find the number of revolutions it will make to travel a distance of 11 km.
Solution:
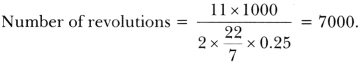
Area Related To Circle Class 10 Extra Questions With Solutions Pdf Question 8.
If the perimeter of a semi-circular protractor is 36 cm, find its diameter.
Solution:
Perimeter of a semicircular protractor = Perimeter of a semicircle
= (2r + πr) cm
⇒ 2r + πr = 36
⇒ r\(\left(2+\frac{22}{7}\right)\) = 36
⇒ r = 7cm
Diameter 2r = 2 × 7 = 14 cm.
Area Related To Circle Class 10 Extra Questions Pdf Question 9.
If the diameter of a semicircular protractor is 14 cm, then find its perimeter.
Solution:
Perimeter of a semicircle = πr + 2r
= \(\frac{22}{7}\) × 7 + 2 × 7 = 22 + 14 = 36cm
Areas Related to Circles Class 10 Extra Questions Short Answer Type 1
Area Related To Circle Class 10 Important Questions With Solutions Question 1.
If a square is inscribed in a circle, what is the ratio of the areas of the circle and the square?

Solution:
Let radius of the circle be r units.
Then, diagonal of the square = 2r

Area Related To Circle Difficult Questions Question 2.
What is the area of the largest triangle that is inscribed in a semi circle of radius r unit?
Solution:

Area of largest ∆ABC = \(\frac{1}{2}\) × AB × CD
\(\frac{1}{2}\) × 2r × r = r2 sq. units
Extra Questions Of Area Related To Circles Class 10 Question 3.
What is the angle subtended at the centre of a circle of radius 10 cm by an arc of length 5π cm?
Solution:
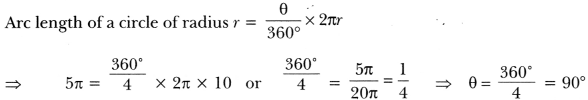
Areas Related To Circles Class 10 Important Questions Question 4.
What is the area of the largest circle that can be drawn inside a 4 rectangle of length a cm and breadth b cm (a > b)?
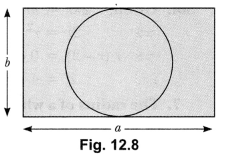
Solution:
Diameter of the largest circle that can be inscribed in the given b
rectangle = b cm
∴ Radius = \(\frac{b}{2}\) cm
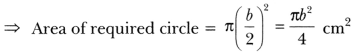
Ch 12 Maths Class 10 Extra Questions Question 5.
Difference between the circumference and radius of a circle is 37 cm. Find the area of circle.
Solution:
Given 2π r – r = 37
or r (2π – 1) = 37

Extra Questions For Class 10 Maths Areas Related To Circles Question 6.
The radii of two circles are 8 cm and 6 cm respectively. Find the radius of the circle having area equal to the sum of the areas of the two circles.
Solution:
Let r be the radius of required circle. Then, we have
πr2 = π(8)2 + π(6)2
⇒ πr2 = 64π + 36π
⇒ πr2 = 100π
∴ r2 = \(\frac{100 \pi}{\pi}\) = 100
⇒ r = 10cm
Hence, radius of required circle is 10 cm.
Class 10 Areas Related To Circles Extra Questions Question 7.
The radii of two circles are 19 cm and 9 cm respectively. Find the radius of the circle which has circumference equal to the sum of the circumferences of the two circles.
Solution:
Let R be the radius of required circle. Then, we have
2πR = 2π(19) + 2π (9)
⇒ 2πR = 2π (19 + 9)
⇒ R = \(\frac{2 \pi \times 28}{2 \pi}\) = 28
⇒ R = 28 cm
Hence, the radius of required circle is 28 cm.
Area Related To Circle Extra Questions Question 8.
Find the area of a circle whose circumference is 22 cm.
Solution:
Let r be the radius of the circle. Then,
Circumference = 22 cm

Class 10 Maths Ch 12 Extra Questions Question 9.
The area of a circular playground is 22176 m2. Find the cost of fencing this ground at the rate of ₹50 per m.
Solution:
Area of circular playground = 22176 m2
πr2 = 22176
⇒ \(\frac{22}{7}\) r2 = 2176
⇒ \(\frac{22176 \times 7}{22}\)
⇒ r = 84 m
∴ Circumference of the playground =2πr = 2 × \(\frac{22}{7}\) × 84 = 44 × 12 = 528 m .
∴ Cost of fencing this ground = ₹ 528 × 50 = ₹ 26400.
Class 10 Area Related To Circles Extra Questions Question 10.
Find the area of a sector of a circle with radius 6 cm if angle of the sector is 60°.
Solution:
We know that

Important Questions Of Area Related To Circle Class 10 Question 11.
Find the area of a quadrant of a circle whose circumference is 22 cm.
Solution:
Let r be the radius of circle, then circumference = 22 cm
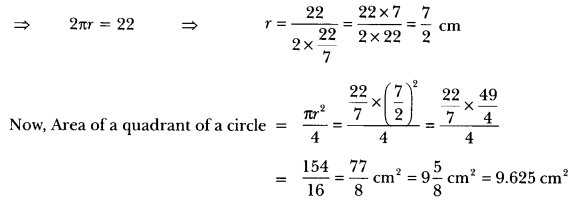
Chapter 12 Maths Class 10 Extra Questions Question 12.
The length of the minute hand of a clock is 14 cm. Find the area swept by the minute hand in 5 minutes.
Solution:
Since the minute hand rotates through 6° in one minute, therefore, area swept by the minute hand in one minute is the area of a sector of angle 6° in a circle of radius 14 cm.
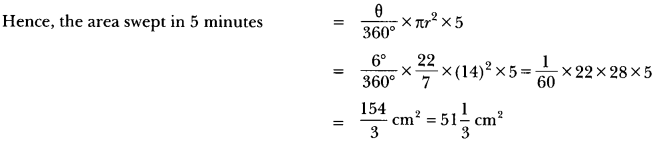
Extra Questions On Areas Related To Circles Question 13.
To warn ships for underwater rocks, a lighthouse spreads a red coloured light over a sector of angle 80° to a distance of 16.5 km. Find the area of the sea over which the ships are warned. (Use π = 3.14)
Solution:
We have, r = 16.5 km and 0 = 80°
∴ Area of the sea over which the ships are warned =
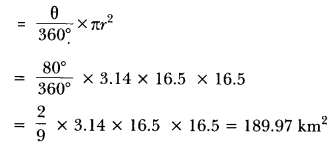
Areas Related to Circles Class 10 Extra Questions Short Answer Type 2
Area Related To Circles Class 10 Extra Questions Question 1.
If the perimeter of a semicircular protractor is 66 cm, find the diameter of the protractor
(Take π = \(\frac{22}{7}\)).
Solution:
Let the radius of the protractor be r сm. Then,
Perimeter = 66 cm
= πr + 2r = 66 [∴ Perimeter of a semicircle = πr + 2r]

Extra Questions Areas Related To Circles Class 10 Question 2.
The circumference of a circle exceeds the diameter by 16.8 cm. Find the radius of the circle.
Solution:
Let the radius of the circle be r сm. Then,
Diameter = 2r cm and Circumference = 2πr cm
According to question,
Circumference = Diameter + 16.8
⇒ 2πr = 2r + 16.8
⇒ 2 × \(\frac{22}{7}\) × r = 2r + 16.8
⇒ 44r = 14r + 16.8 × 7
⇒ 44r – 14r = 117.6 or 30r = 117.6
⇒ r = \(\frac{117.6}{30}\) = 3.92
Hence, radius = 3.92 cm.
Question 3.
A race track is in the form of a ring whose inner circumference is 352 m, and the outer circumference is 396 m. Find the width of the track.
Solution:
Let the outer and inner radii of the ring be R m and r m respectively. Then,
2πR = 396 and 2πr = 352
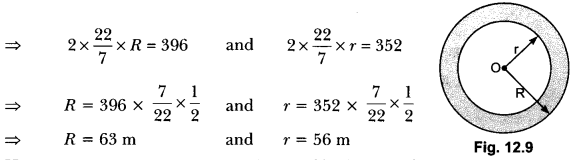
Hence, width of the track = (R – r) m = (63 – 56) m = 7 m
Question 4.
The inner circumference of a circular track [Fig. 12.10] is 220 m. The track is 7 m wide everywhere. Calculate the cost of putting up a fence along the outer circle at the rate of ₹2 per metre.
Solution:

Let the inner and outer radii of the circular track berm and R m respectively. Then,
Inner circumference = 2πr = 220 m
![]()
Since the track is 7 m wide everywhere. Therefore,
R = Outer radius = r + 7 = (35 + 7)m = 42 m
∴ Outer circumference = 2πR = 2 × \(\frac{22}{7}\) × 42m = 264m
Rate of fencing = ₹ 2 per metre
∴ Total cost of fencing = (Circumference × Rate) = ₹(264 × 2) = ₹ 528
Question 5.
The wheels of a car are of diameter 80 cm each. How many complete revolutions does each wheel make in 10 minutes when the car is travelling at a speed of 66 km per hour?
Solution:
The diameter of a wheel = 80 cm.
radius of the wheel = 40 cm.
Now, distance travelled in one complete revolution of wheel = 2π × 40 = 80π
Since, speed of the car is 66 km/h
So, distance travelled in 10 minutes = \(\frac{66 \times 100000 \times 10}{60}\)
= 11 × 100000 cm = 1100000 cm.
So, Number of complete revolutions in 10 minutes
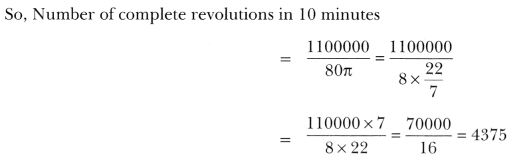
Question 6.
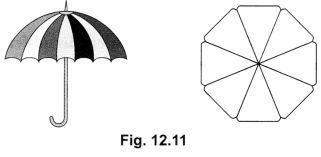
An umbrella has 8 ribs which are equally spaced (Fig. 12.11). Assuming umbrella to be a flat circle of radius 45 cm, find the area between the two consecutive ribs of the umbrella.
Solution:
We have, r = 45 cm
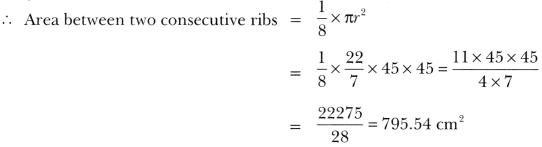
Question 7.
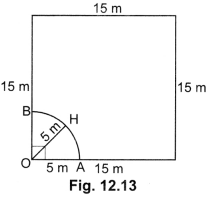
A horse is tied to a peg at one corner of a square shaped grass field of side 15 m by means of a 5 m long rope (Fig. 12.12). Find (i) the area of that part of the field in which the horse can graze;
(ii) the increase in the grazing area if the rope were 10 m long instead of 5 m. (Use π = 3.14)

Solution:
Let the horse be tied at point O and the length of the rope is OH (Fig. 12.13).
Thus, (i) the area of the part of the field in which the horse can graze
= Area of the quadrant of a circle (OAHB)
![]()
(ii) Now r = 10 m and
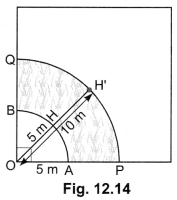
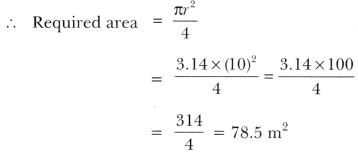
Increase in the grazing area
= (78.5 – 19.625) m2
= 58.875 m2
Question 8.
A car has two wipers which do not overlap. Each wiper has a blade of length 25 cm sweeping through an angle of 115o. Find the total area cleaned at each sweep of the blades.
Solution:
We have, r = 25 cm and θ = 115°.
∴ Total area cleaned at each sweep of the blades
= 2 × (Area of the sector having radius 25 cm and angle θ = 115°).
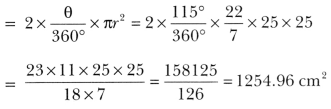
Question 9.
In Fig. 12.15, sectors of two concentric circles of radii 7 cm and 3.5 cm are shown. Find the area
of the shaded region.
Solution:
Let A1 and A2 be the areas of sectors OAB and OCD respectively. Then, A, = Area of a sector of angle 30° in a circle of radius 7 cm.
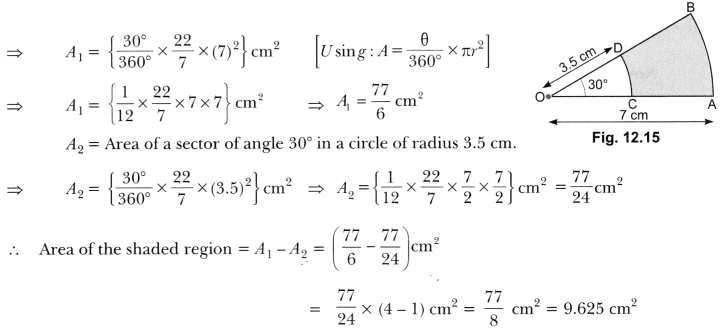
Question 10.
The minute hand of a clock is 10 cm long. Find the area of the face of the clock described by the minute hand between 9 AM and 9.35 AM.
Solution:
We have,
Angle described by the minute hand in one minute = 6°
∴ Angle described by the minute hand in 35 minutes = (6 × 35)° = 210°
Area swept by the minute hand in 35 minutes = Area of a sector of a circle of radius 10 cm

Question 11.
Find the area of the sector of a circle with radius 4 cm and of angle 30°. Also, find the area of the corresponding major sector. (Use π = 3.14)
Solution:
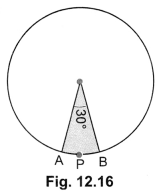
Area of the sector OAPB = \(\frac{\theta}{360^{\circ}} \times \pi r^{2}\)
\(\frac{30^{\circ}}{360^{\circ}}\) × 3.14 × 4 × 4 cm2
= \(\frac{12.56}{3}\)cm2
= 4.19 cm2 (approx.)
Area of the corresponding major sector = πr2 – Area of sector OAPB
= (3.14 × 4 × 4 – 4.19)cm2 = (50.24 – 4.19) cm2
= 46.05 cm2 = 46.1 cm2 (approx.)
Question 12.
A chord of a circle of radius 15 cm subtends an angle of 60° at the centre. Find the areas of the corresponding minor and major segments of the circle. (Use π = 3.14 and √3 = 1.73)
Solution:
We have, r = 15 cm and θ = 60°
Given segment is APB
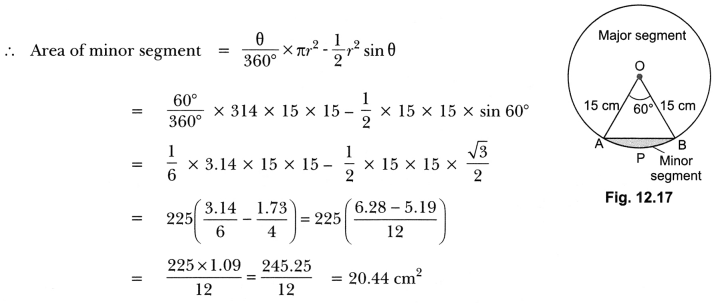
Now, area of major segment = Area of circle – Area of minor segment
= π(15)2 – 20.44 = 3.14 × 225 – 20.44
= 706.5 – 20.44 = 686.06 cm
Question 13.
A chord of a circle of radius 12 cm subtends an angle of 120° at the centre. Find the area of the corresponding segment of the circle. (Use π = 3.14 and √3 = 1.73)
Solution:
We have, r = 12 cm and θ = 120°
Given segment is APB
Now, area of the corresponding segment of circle
= Area of the minor segment
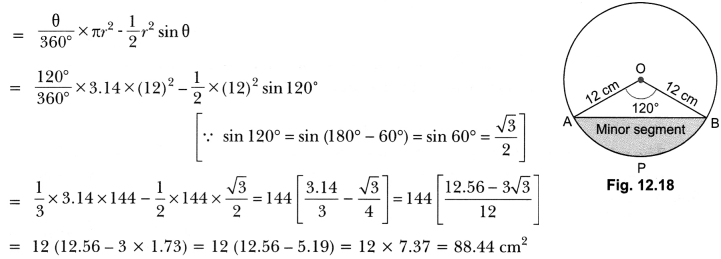
Question 14.
A round table cover has six equal designs as shown in Fig. 12.19. If the radius of the cover is 28 cm?, find the cost of making the designs at the rate of ₹ = 0.35 per cm2. (Use √3 = 1.7)
Solution:
Area of one design = Area of the sector OAPB – Area of ΔAOB
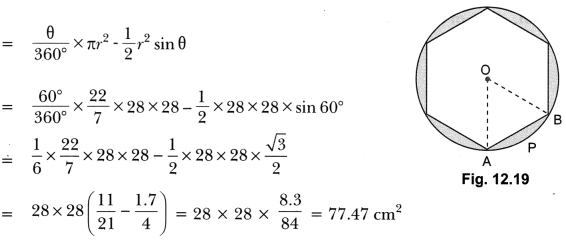
Area of 6 such designs = 77.47 × 6 = 464.8 cm2
Hence, cost of making such designs = ₹ 162.69
Question 15.
Find the area of the shaded region in Fig. 12.20, if PQ = 24 cm, PR = 7 cm and O is the centre of the circle.
Solution:
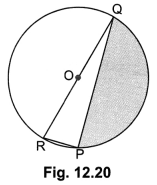
Here, ROQ is the diameter of given circle, therefore ∠RPQ = 90°
Now, in right angled ΔPRQ, we have
RQ2 = RP2 + PQ2 (by Pythagoras Theorem)
RQ2 = (7)2 + (24)2 = 49 + 576 = 625
RQ = \(\sqrt{625}\) = 25 cm
Therefore, radius r = \(\frac{25}{2}\) cm
Now, area of shaded region
= Area of the semi-circle – Area of ARPQ
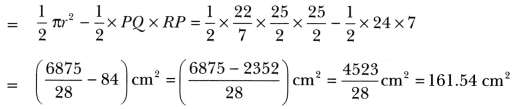
Question 16.
Find the area of the shaded region in Fig. 12.21, if radii of the two concentric circles with centre 0 are 7 cm and 14 cm respectively and ∠AOC = 40°.
Solution:
Area of shaded region
= Area of sector AOC – Area of sector OBD
Question 17.
Find the area of the shaded region in Fig. 12.22, if ABCD is a square of side 14 cm and APD and BPC are semicircles.
Solution:
We have, radius of semicircles = 7 cm
∴ Area of shaded region
= Area of square ABCD – Area of semi-circles (APD +BPC)

Question 18.
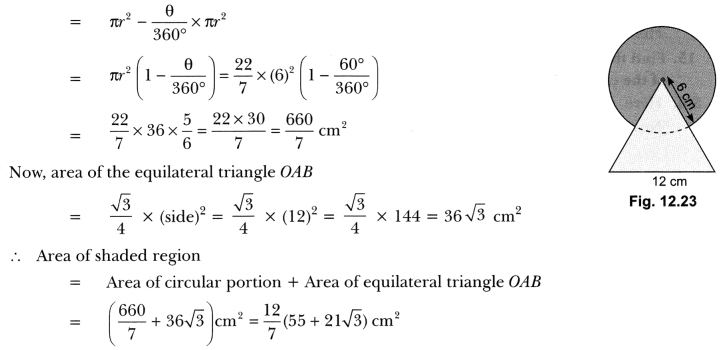
Find the area of the shaded region in Fig. 12.23, where a circular arc of radius 6 cm has been drawn with vertex 0 of an equilateral triangle OAB of side 12 cm as centre.
Solution:
We have, radius of circular region
= 6 cm and each side of ΔOAB = 12 cm.
∴ Area of the circular portion
= Area of circle – Area of the sector
Quesiton 19.
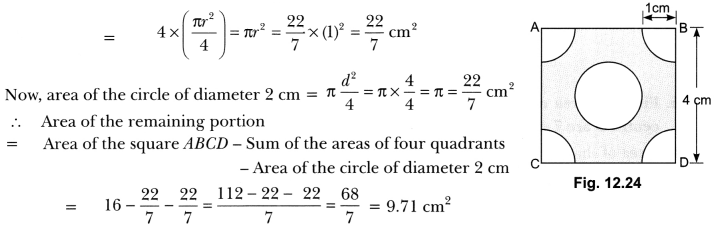
From each corner of a square of side 4 cm, a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in Fig. 12.24. Find the area of the remaining portion of the square.
Solution:
We have, the side of the square ABCD = 4 cm
Area of the square ABCD = (4)2 = 16 cm2
Since, each quadrant of a circle has radius 1 cm.
∴ The sum of the areas of four quadrants
Question 20.
In Fig. 12.25, ABCD is a square of side 14 cm. With centres A, B, C and D, four circles are drawn such that each circle touches externally two of the remaining three circles. Find the area of the shaded region.
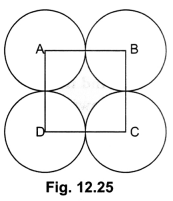
Solution:
We have, each side of square ABCD = 14 cm
∴ Area of square ABCD = (142)cm2 = 196 cm2
Now, radius of each quadrant of circle,
r = \(\frac{14}{2}\) = 7 cm
∴ The sum of the area of the four quadrants at the four corners of the square
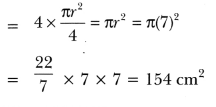
Now, area of shaded portion
= Area of square ABCD – The sum of the areas of four quadrants at the four corners of the square
= (196 – 154) cm2 = 42 cm2
Question 21.
On a square handkerchief, nine circular designs, each of radius 7 cm are made (see Fig. 12.26). Find the area of the remaining portion of the handkerchief.

Solution:
Total area of circular design = 9 × Area of one circular design
= 9 × π × (7)2
= 9 × \(\frac{22}{7}\) × 7 × 7 = 1386 cm2
Now, each side of square ABCD = 3 × diameter of circular design
= 3 × 14 = 42 cm
∴ Area of square ABCD = (42)2 = 1764 cm2
∴ Area of the remaining portion of handkerchief
= Area of square ABCD – Total area of circular design
= (1764 – 1386) cm = 378 cm2
Question 22.
In Fig. 12.27, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the (i) quadrant OACB, (ii) shaded region.
OR
In the given figure, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the shaded region.
Solution:

Question 23.
In Fig. 12.28, a square OABC is inscribed in a quadrant OPBQ. If OA = 20 cm, find the area of the shaded region. (Use π = 3.14).
Solution:
We have,
Radius of quadrant
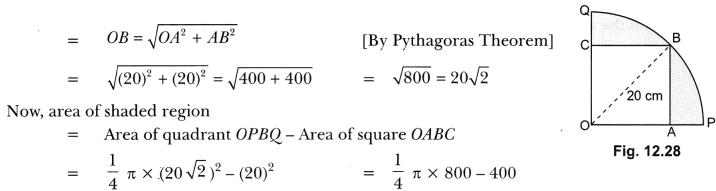
= 200 × 3.14 – 400 = 628 – 400 = 228 cm2
Question 24.
Calculate the area of the designed region in Fig. 12.29, which is common between the two quadrants of circles of radius, 8 cm each.
Solution:
Here, radius of each quadrant ABPD and BQDC = 8 cm
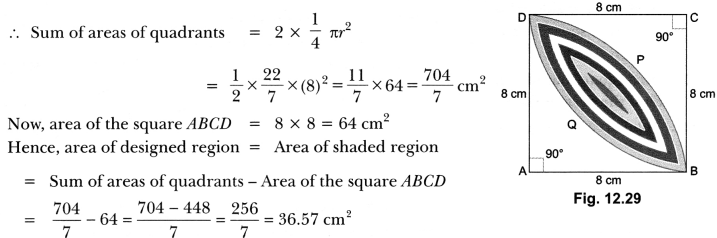
Question 25.
In the given Fig. 12.30, find the area of the shaded region.
Solution:
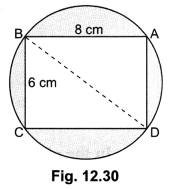
Clearly, diameter of the circle
= Diagonal BD of rectangle ABCD
Now, BD
![]()
Let r be the radius of the circle. Then, r = \(\left(\frac{10}{2}\right)\)cm = 5 cm
Hence, area of the shaded region = Area of the circle – Area of rectangle ABCD
= πr² – l × b = (3.14 × 5 × 5) – (8 × 6)
= (78.50 – 48) cm2 = 30.50 cm2
Question 26.
A square park has each side of 100 m. At each corner of the park, there is a flower bed in the form of a quadrant of radius 14 m as shown in Fig. 12.31. Find the area of the remaining part of the park.
Solution:
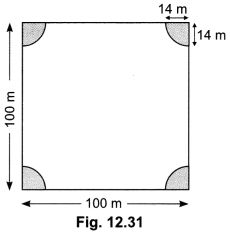
We have,
Area of 4 quadrants of a circle of radius 14 m
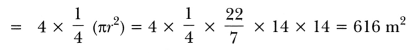
Area of square park having side 100 m long
= (100 × 100) m2 = 10,000 m2.
Hence, area of the remaining part of the park
= Area of square – Area of 4 quadrants at each corner
= (10,000 – 616) m2 = 9384 m2
Question 27.
Find the area of the shaded region in Fig. 12.32, where ABCD is a square of side 14 cm each.
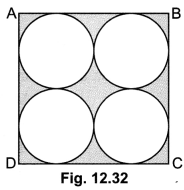
Solution:
Area of square ABCD = 14 × 14 cm2 = 196 cm2
Diameter of each circle = \(\frac{14}{2}\) cm = 7 cm
So, radius of each circle = \(\frac{7}{2}\) cm
![]()
∴ Area of shaded region = Area of square – Area of 4 circles
= (196 – 154) cm2 = 42 cm2
Question 28.
In Fig. 12.33, ABCD is a trapezium of area 24.5 sq. cm. In it, AD || BC, ∠DAB = 90°, AD = 10 cm and BC = 4 cm. If ABE is a quadrant of a circle, find the area of the shaded region. [Take π = \(\frac{22}{7}\)
Solution:
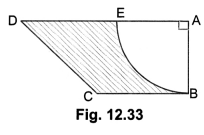
Area of trapezium = 24.5 cm2
\(\frac{1}{2}\)[AD + BC] AB = 24.5
\(\frac{1}{2}\)[10 + 4] × AB = 24.5
AB = 3.5 cm ⇒ r = 3.5 cm
Area of quadrant = \(\frac{1}{4}\)πr2
.025 × \(\frac{22}{7}\) × 3.5 × 3.5 = 9.625 cm2
The area of shaded region = 24.5 – 9.625 = 14.875 cm2
Question 29.
In Fig. 12.34, O is the centre of a circle such that diameter AB = 13 cm and AC = 12 cm. BC is joined. Find the area of the shaded region. (Take π = 3.14)
Solution:
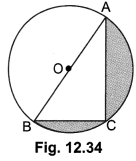
In ΔABC, ∠ACB = 90° (Angle in the semicircle)
∴BC2 + AC2 = AB2
∴ BC2 = AB2 – AC2
= 169 – 144 = 25
∴ BC = 5 cm
Area of the shaded region = Area of semicircle – Area of right ΔABC

Question 30.
In Fig. 12.35, are shown two arcs PAQ and PBQ. Arc PAQ is a part of circle with centre 0 and radius OP while arc PBQ is a semi-circle drawn on PQ as diameter with centre M.
If OP = PQ = 10 cm show that area of shaded region is \(25\left(\sqrt{3}-\frac{\pi}{6}\right)\)cm2.
Solution:
Since OP = PQ = QO
⇒ ΔPOQ is an equilateral triangle
∴ ∠POQ = 60°
Area of segment PAQM
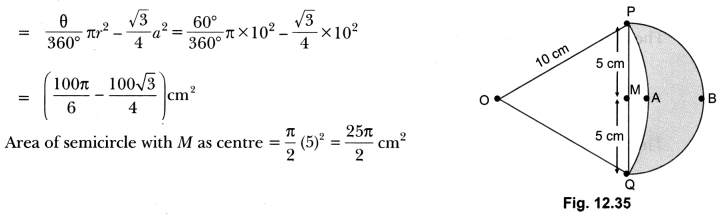

Areas Related to Circles Class 10 Extra Questions Long Answer Type
Question 1.
PQRS is a diameter of a circle of radius 6 cm. The lengths PQ, QR and RS are equal. Semicircles are drawn on PQ and QS as diameters is shown in Fig. 12.36. Find the perimeter and area of the shaded region.
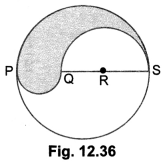
Solution:
We have,
PS = Diameter of a circle of radius 6 cm = 12 cm
∴ PQ = QR = RS = \(\frac{12}{3}\) = 4 cm
Fig. 12.36 QS = QR + RS = (4 + 4) cm = 8 cm
Hence, required perimeter
= Arc of semicircle of radius 6 cm + Arc of semi circle of radius 4 cm + Arc of semi-circle of radius 2 cm
= (π × 6 + π × 4 + π × 2) cm = 12π cm = 12 × = \(\frac{22}{7}\) = \(\frac{264}{7}\) = 37.71 cm.
Required area = Area of semicircle with PS as diameter + Area of semicircle with PQ as diameter – Area of semi-circle with QS as diameter
Question 2.
Figure 12.37 depicts an archery target marked with its five scoring areas from the centre outwards as Gold, Red, Blue, Black and White. The diameter of the region representing Gold score is 21 cm and each of the other bands is 10.5 cm wide. Find the area of each of the five scoring regions.
Solution:
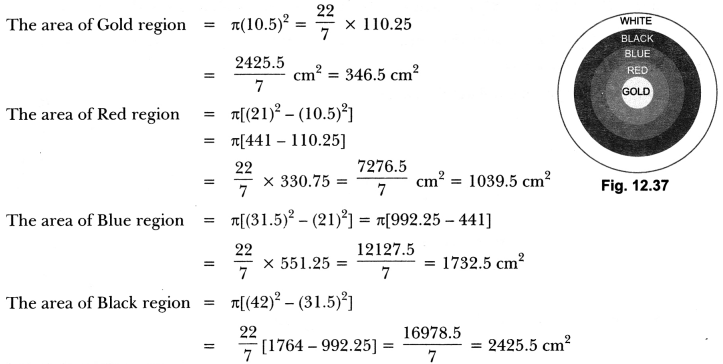
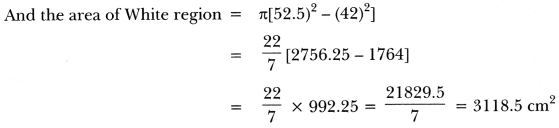
Question 3.
The short and long hands of a clock are 4 cm and 6 cm long respectively. Find the sum of distances travelled by their tips in 2 days.
Solution:
In 2 days, the short hand will complete 4 rounds.
Distance moved by its tip = 4 (Circumference of a circle of radius 4 cm)

Question 4.
Fig. 12.38, depicts a racing track whose left and right ends are semicircular. The distance between the two inner parallel line segments is 60 m and they are each 106 m long. If the track is 10 m wide, find:
(i) the distance around the track along its inner edge.
(ii) the area of the track.
Solution:
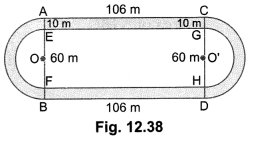
Here, we have
OE = O’G = 30 m
AE = CG = 10 m
OA = O’C = (30 + 10) m = 40 m
AC = EG = FH = BD = 106 m
(i) The distance around the track along its inner edge
= EG + FH + 2 × (circumference of the semicircle of radius OE = 30cm)
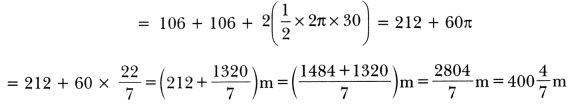
(ii) Area of the track = Area of the shaded region
= Area of rectangle AEGC + Area of rectangle BFHD + 2 (Area of the semicircle of radius 40 m – Area of the semicircle with radius 30 m)
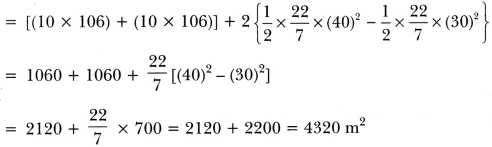
Question 5.
The area of an equilateral triangle ABC is 17320.5 cm. With each vertex of the triangle as centre, a circle is drawn with radius equal to half the length of the side of the triangle (see Fig. 12.39). Find the area of the shaded region. (Use π = 3.14 and √3 = 1.73205)
Solution:
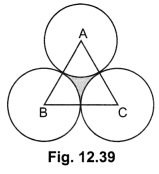
Let each side of the equilateral triangle be x cm. Then,
Area of equilateral triangle ABC = 17320.5 cm (Given)

Question 6.
In a circular table cover of radius 32 cm, a design is formed leaving an equilateral triangle ABC in the middle as shown in Fig. 12.40. Find the area of the design.
Solution:
Here, ∆ABC is an equilateral triangle. Let O be the circumcentre of circumcircle.
Radius, r = 32 cm.
Now, area of circle = πr2
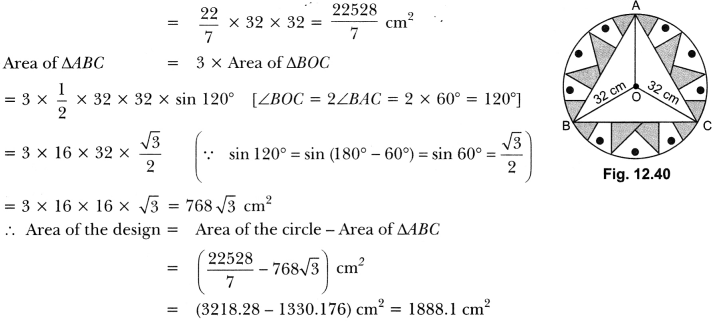
Question 7.
In Fig. 12.41, AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region.
Solution:
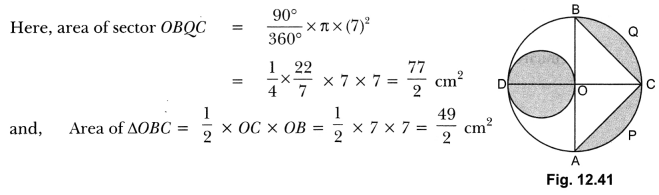
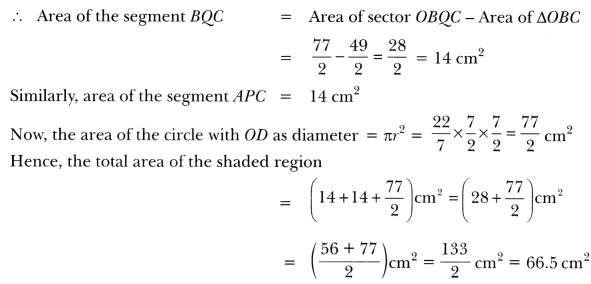
Question 8.
In Fig. 12.42 ABC is a quadrant of a circle of radius 14 cm and a semicircle is drawn with BC as diameter. Find the area of the shaded region.
Solution:
In ∆ABC, we have
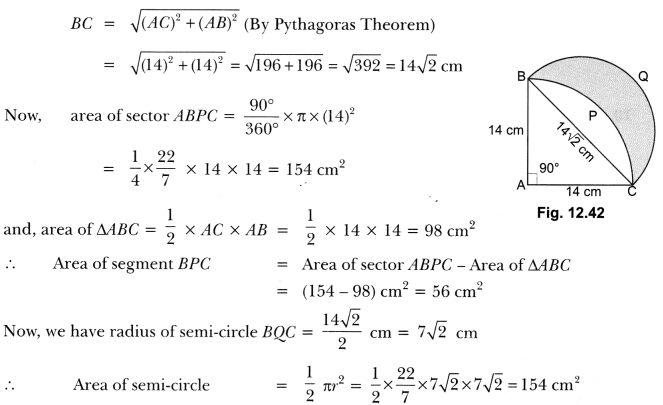
Hence, area of the shaded region = Area of the semi-circle BQC – Area of the segment BPC
= (154 – 56)cm2 = 98cm2
Question 9.
In Fig 12.43, a circle is inscribed in an equilateral triangle ABC of side 12 cm. Find the radius of inscribed circle and the area of the shaded region. [Use π = 3.14 and √3 = 1.73]
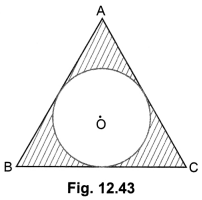
Solution:
Construction:
Join OA, OB and OC
Draw OZ ⊥ BC, OX ⊥ AB and OY ⊥ AC.
Let the radius of the circle be r сm.
Area of ∆ABC = Area of ∆AOB + Area of ∆BOC + Area of ∆AOC

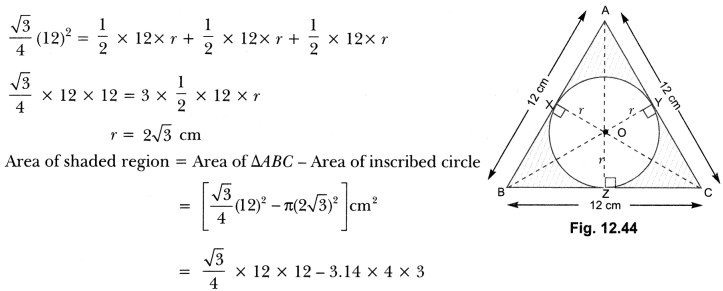
= 1.73 × 3 × 12 – 3.14 × 4 × 3
= 62.28 – 37.68 = 24.6 cm2
Question 10.
In Fig. 12.45, PSR, RTQ and PAQ are three semicircles of diameters 10 cm, 3 cm and 7 cm respectively. Find the perimeter of the shaded region. [Use π = 3.14]
Solution:
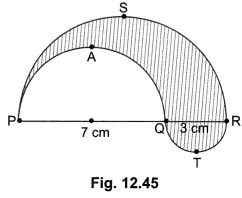
Radius of semicircle PSR = \(\frac{1}{2}\) × 10 cm = 5 cm
Radius of semicircle RTQ = \(\frac{1}{2}\) × 3 cm = 1.5 cm
Radius of semicircle PAQ = \(\frac{1}{2}\) × 7 em = 3.5 cm
Perimeter of shaded region = Circumference of semicircle PSR + Circumference of semicircle RTQ + Circumference of semicircle PAQ.

= π[5 + 1.5 + 3.5]= 3.14 × 10 = 31.4cm
Question 11.
An elastic belt is placed around the rim of a pulley of radius 5 cm. (Fig. 12.46). From one point C on the belt, the elastic belt is pulled directly away from the centre O of the pulley until it is at P, 10 cm from the point O. Find the length of the belt that is still in contact with the pulley. Also find the shaded area. (Use π = 3.14 and √3 = 1.73)
Solution:
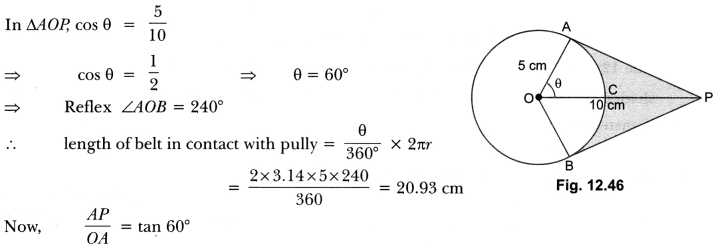
PA = 5√3 cm = BP [Tangents from an external point are equal]
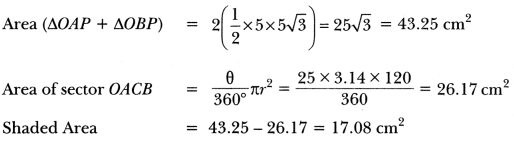
Question 12.
In Fig. 12.47, a sector OAP of a circle with centre O, containing ∠θ. AB is perpendicular to the radius OA and meets OP produced at B. Prove that the perimeter of shaded region is
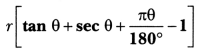
Solution:
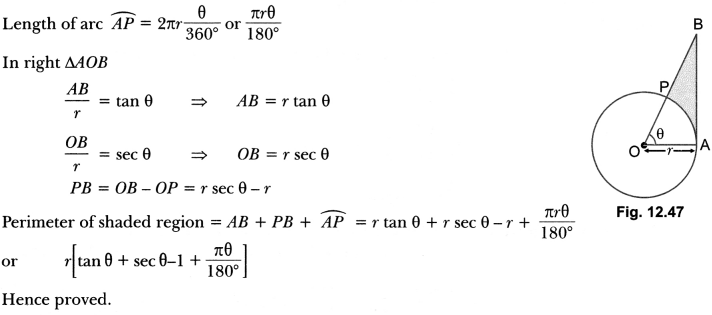
Question 13.
Find the area of the shaded region in Fig. 12.48, where APD, AQB, BRC , CSD are semi-circles of diameter 14 cm, 3.5 cm, 7 cm and 3.5 cm respectively. (Use π = \(\frac{22}{7}\) )
Solution:
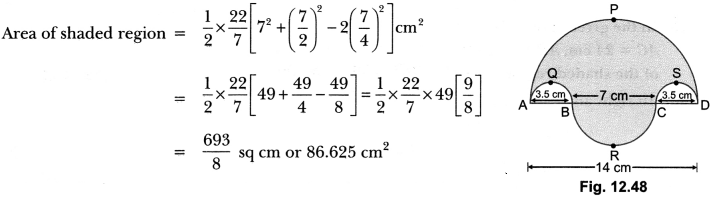
Question 14.
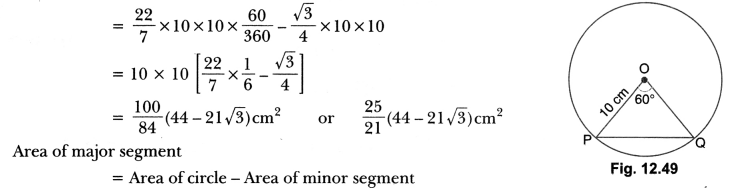
A chord PQ of a circle of radius 10 cm subtends an angle of 60° at the 14 cmcentre of circle. Find the area of major and minor segments of the Fig. 12.48 circle.
Solution:
Area of minor segment
= Area of minor sector having angle 60° at centre – area of equilateral ∆OPQ
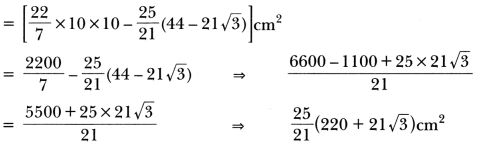
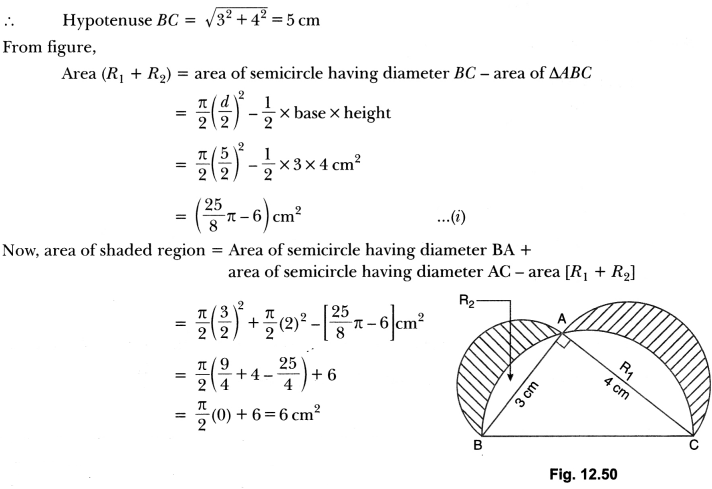
Question 15.
In the given figure, ∆ABC is a right-angled triangle in which ∠A is 90°. Semicircles are drawn on AB, AC and BC as diameters. Find the area of the shaded region.
Solution:
∵ ABC is right angled triangle
Question 16.
In the given figure, O is the centre of the circle with AC = 24 cm, AB = 7 cm and ∠BOD = 90°. Find the area of the shaded region.
Solution:
In right angle triangle ABC
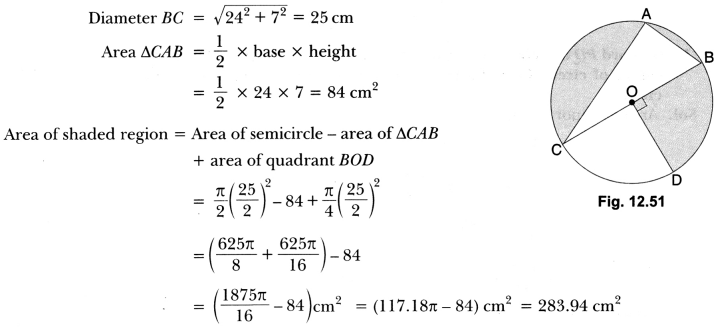
Areas Related to Circles Class 10 Extra Questions HOTS
Question 1.
Two circles touch internally. The sum of their areas is 116 ncmo and distance between their centres is 6 cm. Find the radii of the circles.
Solution:
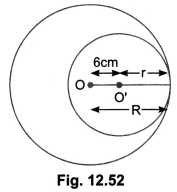
Let R and r be the radii of the circles [Fig. 12.52].
Then, according to question,
⇒ πR2 +πr2 = 116π
⇒ R2 + r2 = 116 …..(i)
Distance between the centres = 6 cm
⇒ OO’ = 6 cm
⇒ R – s = 6 …(ii)
Now, (R + r)2 + (R – 1)2 = 2(R2 + m2)
Using the equation (i) and (ii), we get
(R + r)2 + 36 = 2 × 116
= (R + r)2 = (2 x 116 – 36) = 196
= R + r = 14 …..(iii)
Solving (ii) and (iii), we get R = 10 and r = 4
Hence, radii of the given circles are 10 cm and 4 cm respectively.
Question 2.
A bicycle wheel makes 5000 revolutions in moving 11 km. Find the diameter of the wheel.
Solution:
Let the radius of the wheel be r сm.
∴ Diameter = 2r cm = (2 × 35) cm = 70 cm
Hence, the diameter of the wheel is 70 cm.
Question 3.
Find the area of the shaded design of Fig. 12.53, where ABCD is a square of side 10 cm and semicircles are drawn with each side of the square as diameter (use π = 3.14).
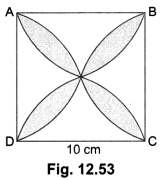
Solution:

Let us mark the four unshaded regions as I, II, III and IV (Fig. 12.53).
Area of I + Area of III
= Area of ABCD – Areas of two semicircles of radius 5 cm each
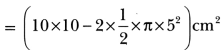
= (100 – 3.14 × 25) cm2 = (100 – 78.5) cm2 = 21.5 cm2
Similarly, Area of II + Area of IV = 21.5 cm2
So, Area of the shaded design
= Area of ABCD – Area of (I + II + III + IV)
= (100 – 2 × 21.5) cm2
= (100 – 43) cm2 = 57 cm2
Question 4.
A copper wire, when bent in the form of a square, encloses an area of 484 cm2. If the same wire is bent in the form of a circle, find the area enclosed by it.
Solution:
We have, Area of the square = a2 = 484 cm2
∴ Side of the square = √1484 cm = 22 cm
So, Perimeter of the square = 4 (side) = (4 × 22) cm = 88 cm
Let r be the radius of the circle. Then, according to question,
Circumference of the circle = Perimeter of the square
Question 5.
Two circles touch externally. The sum of their areas is 130 r sq. cm and the distance between their centres is 14 cm. Find the radii of the circles.
Solution:
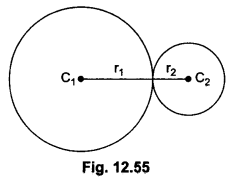
If two circles touch externally, then the distance between their centres is equal to the sum of their radii.
Let the radii of the two circles be r, cm and r2 cm respectively [Fig. 12.55).
Let C, and C, be the centres of the given circles. Then,
C1C2 = r1 +r2
= 14 = r1 +r2
[∵ C1C2 = 14 cm given]
= r1 +r2 = 14
It is given that the sum of the areas of two circles is equal to 130 n cm2

Solving (i) and (iv), we get r1 = 11 cm and r2 = 3 cm.
Hence, the radii of the two circles are 11 cm and 3 cm.
Question 6.
In Fig. 12.56, from a rectangular region ABCD with A
AB = 20 cm, a right triangle AED with AE = 9 cm and DE = 12 cm, is cut off. On the other end, taking BC as diameter, a semicircle is added on outside the region. Find the area of the shaded region. [Use π = 3.14]
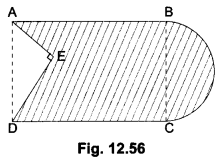
Solution:
Area of shaded region
= Area of rectangle – Area of triangle + Area of semicircle. In right ∆ADE.
AD2 = AE2 + DE2
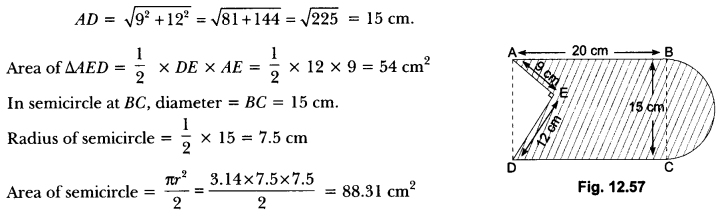
Area of rectangle = AB × BC = 20 × 15 = 300 cm2
Area of shaded region = 300 + 88.31 – 54 = 334.31 cm2
Question 7.
In the given Fig. 12.58, the side of square is 28 cm and radius of each circle is half of the length of the side of the square where O and O’ are centres of the circles. Find the area of shaded region.

Solution:
Area of shaded region
= Area of square + Area of 2 major sectors having angle 270° at centre
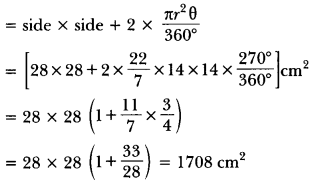
Question 8.
In the given Fig. 12.59, ABCD is a rectangle of dimensions 21 cm × 14 cm. A semicircle is drawn with BC as diameter. Find the area and the perimeter of the shaded region in the figure.
Solution:

Area of shaded region
Area of shaded region
= Area of rectangle – Area of semicircle
=(l × b) – \(\frac{1}{2}\) × π × r2
= (21 × 14) – \(\frac{1}{2}\) × π × 7 × 7
= 294 – \(\frac{1}{2}\) × \(\frac{22}{7}\) × 7 × 7
= 294 – 77 = 217 cm2
Now, perimeter of shaded region = 2l + b + circumference of semicircle i.e.; πr
= 2 × 21 + 14 + \(\frac{22}{7}\) × 7
= 56 + 22 = 78 cm
