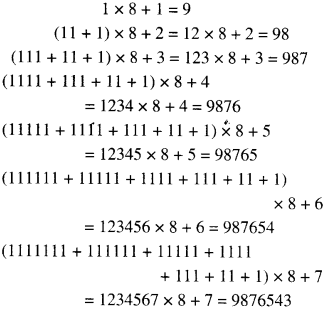Category: CBSE Class 6

NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.5 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.5.

• Basic Geometrical Ideas Class 6 Ex 4.1
• Basic Geometrical Ideas Class 6 Ex 4.2
• Basic Geometrical Ideas Class 6 Ex 4.3
• Basic Geometrical Ideas Class 6 Ex 4.4
• Basic Geometrical Ideas Class 6 Ex 4.6

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 4
Chapter Name	Basic Geometrical Ideas
Exercise	Ex 4.5
Number of Questions Solved	3
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.5

Question 1.
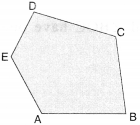
Draw a rough sketch of a quadrilateral PQRS. Draw its diagonals. Name them. Is the meeting point of the diagonal in the interior or exterior of the quadrilateral?
Solution :

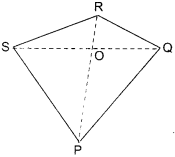
The meeting point O of the diagonals PR and QS of the quadrilateral PQRS is in the interior of the quadrilateral PQRS.

Question 2.
Draw a rough sketch of a quadrilateral KLMN. State :
(a) two pairs of opposite sides.
(b) two pairs of opposite angles.
(c) two pairs of adjacent sides.
(d) two pairs of adjacent angles.
Solution :
(a) \(\bar { KL }\), \(\bar { NM }\) and \(\bar { KN }\), \(\bar { ML }\)
(b) ∠K, ∠M and ∠N, ∠L
(c) \(\bar { KL }\) , \(\bar { KN }\) and \(\bar { NM }\), \(\bar { ML }\) or \(\bar { KL }\) , \(\bar { LM }\) and \(\bar { NM }\), \(\bar { ML }\)

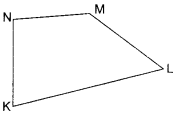
(d) ∠K, ∠L, and ∠M. ∠N or ∠K. ∠L and ∠L, ∠M etc.

Question 3.
Investigate:
Use strips and fasteners to make a triangle and a quadrilateral. Try to push inward at any one vertex of the triangle. Do the same to the quadrilateral. Is the triangle distorted? Is the quadrilateral distorted? Is the triangle rigid? Why is it that structures like electric towers make use of triangular shapes and not quadrilaterals?
Solution :
On pushing inward at any one vertex of the triangle, the triangle is not distorted, However, the quadrilateral is distorted. Hence, a triangle is a rigid figure. This is why structures like electric towers make use of triangular shapes and not quadrilaterals.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.5 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.5, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.4 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.4.

• Basic Geometrical Ideas Class 6 Ex 4.1
• Basic Geometrical Ideas Class 6 Ex 4.2
• Basic Geometrical Ideas Class 6 Ex 4.3
• Basic Geometrical Ideas Class 6 Ex 4.5
• Basic Geometrical Ideas Class 6 Ex 4.6

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 4
Chapter Name	Basic Geometrical Ideas
Exercise	Ex 4.4
Number of Questions Solved	2
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.4

Question 1.
Draw a rough sketch of a triangle ABC. Mark a point P in its interior and a point Q in its exterior. Is the point A in its exterior or in its exterior?
Solution :

The point A is neither in the exterior nor in the interior of triangle ABC. It is on the triangle ABC.

Question 2.
(a) Identify three triangles in the figure.
(b) Write the names of seven angles,
(c) Write the names of the six line segments,
(d) Which two triangles have ∠B as common?
Solution :
(a) Three triangles
Triangle ABC,
Triangle ABD,
Triangle ADC

(b) Seven Angles
∠ABC. ∠ACB, ∠BAC, ∠BAD, ∠CAD, ∠ADB and ∠ADC
(c) Six line segments
\(\bar { AB }\), \(\bar { AC }\), \(\bar { BC }\), \(\bar { AD }\), \(\bar { BD }\), \(\bar { DC }\)
(d) ΔABC. ΔABD.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.4 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.4, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.3 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.3.

• Basic Geometrical Ideas Class 6 Ex 4.1
• Basic Geometrical Ideas Class 6 Ex 4.2
• Basic Geometrical Ideas Class 6 Ex 4.4
• Basic Geometrical Ideas Class 6 Ex 4.5
• Basic Geometrical Ideas Class 6 Ex 4.6

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 4
Chapter Name	Basic Geometrical Ideas
Exercise	Ex 4.3
Number of Questions Solved	3
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.3

Question 1.
Name the angles in the given figure.

Solution :
∠A or ∠DAB; ∠B or ∠ABC; ∠C or ∠BCD; ∠Dor ∠CDA.

Question 2.
In the given diagram, name the point (s)
(a) in the interior of ∠DOE
(b) in the exterior of∠EOF
(c) on ∠EOF.

Solution :
(a) A
(b) C, A, D
(c) E, B,0, F.

Question 3.
Draw rough diagrams of two angles such that they have
(a) One point in common
(b) Two points in common
(c) Three points in common
(d) Four points in common
(e) One ray in common.
Solution :
(a)

∠AOB and ∠BOC have one point O in common.
(b) ∠AOB and ∠OBC have two points O and B in common.

(c) Not possible
(d) Not possible
(e)

∠AOB and ∠BOC have one ray \(\overrightarrow { OB }\) in common

 

We hope the NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.3 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.3, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.2 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.2.

• Basic Geometrical Ideas Class 6 Ex 4.1
• Basic Geometrical Ideas Class 6 Ex 4.3
• Basic Geometrical Ideas Class 6 Ex 4.4
• Basic Geometrical Ideas Class 6 Ex 4.5
• Basic Geometrical Ideas Class 6 Ex 4.6

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 4
Chapter Name	Basic Geometrical Ideas
Exercise	Ex 4.2
Number of Questions Solved	5
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.2

Question 1.
Classify the following curves as
(i) open
(ii) closed.

Solution :
(i) a, c
(ii) b,d,e.

Question 2.
Draw rough diagrams to illustrate the following :
(a) Open curve
(b) Closed curve.
Solution :
(a)

(b)

Question 3.
Draw any polygon and shade its interior.
Solution :

Question 4.
Consider the given figure and answer the questions:
(a) Is it a curve?

(b) Is it closed?
Solution :
(a) Yes! It is a curve
(b) Yes! It is closed.

Question 5.
Illustrate, if possible, each one of the following with a rough diagram :
(a) A closed curve that is not a polygon
(b) An open curve made up entirely of line segments.
(c) A polygon with two sides.
Solution :
(a)

(b)

(c) Not possible.

We hope the NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.2 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.2, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.7 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.7.

• Playing With Numbers Class 6 Ex 3.1
• Playing With Numbers Class 6 Ex 3.2
• Playing With Numbers Class 6 Ex 3.3
• Playing With Numbers Class 6 Ex 3.4
• Playing With Numbers Class 6 Ex 3.5
• Playing With Numbers Class 6 Ex 3.6

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 3
Chapter Name	Playing With Numbers
Exercise	Ex 3.7
Number of Questions Solved	11
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.7

Question 1.
Renu purchases two bags of fertilizer of weights 75 kg and 69 kg. Find the maximum value of weight which can measure the weight of the fertilizer e×act number of times.
Solution :
Factors of 75 are 1, 3, 5, 15, 25 and 75.
Factors of 69 are 1, 3, 23 and 69.
∴ Common factors of 75 and 69 are 1 and 3.
Highest of these common factors is 3.
∴ H.C.F. of 75 and 69 is 3.
Hence, the maximum value of weight which can measure the weight of the fertilizer e×act number of times is 3 kg.

Question 2.
Three boys step off together from the same spot. Their steps measure 63 cm, 70 cm, and 77 cm, respectively. What is the minimum distance each should cover so that all can cover the distance in complete steps?
Solution :

∴ L.C.M. of 63, 70 and 77
= 2 × 3 × 3 × 5 × 7 × 11 = 6930.
Hence, the minimum distance each should cover so that all can cover the distance in complete steps is 6930 cm.

Question 3.
The length, breadth, and height of a room are 825 cm, 675 cm, and 450 cm, respectively. Find the longest tape which can measure the three dimensions of the room exactly.
Solution :
Factors of 825 are 1, 3, 5, 11, 15, 25, 33, 55,75,165, 275 and 825.
Factors of 675 are 1, 3, 5,9,15, 25, 27,45,75, 135, 225 and 675.
Factors of 450 are 1,2,3,5,6,9,10,15,18,25, 30,45, 50, 75, 90, 150, 225 and 450.
∴ Common factors of 825, 675 and 450 are 1,3,5,15, 25 and 75.
Highest of these common factors is 75.
Hence, the length of the longest tape which can measure the three dimensions of the room exactly is 75 cm.

Question 4.
Determine the smallest 3-digit number which is exactly divisible by 6, 8 and 12.
Solution :

∴ L.C.M. of 6, 8 and 12 = 2 × 2 × 2 × 3 = 24. Multiples of 24 are 24,48,72,96,120,144,
Hence, the smallest 3-digit number which is exactly divisible by 6, 8 and 12 is 120.

Question 5.
Determine the largest 3-digit number exactly divisible by 8, 10 and 12.
Solution :

∴ L.C.M. of 8,10 and 12 = 2×2×2×3×5
= 120.
Multiples of 120 are :
120 × 1 = 120,120 × 2 = 240,120 × 3 = 360,120 × 4 = 480,120 × 5 = 600,120 × 6 = 720,120 × 7 = 840,
120 × 8 = 960,120 × 9 = 1080,
Hence, the largest 3-digit number exactly divisible by 8, 10 and 12 is 960.

Question 6.
The traffic lights at three different road crossings change after every 48 seconds, 72 seconds and 108 seconds respectively. If they change simultaneously at 7 am at what time will they change simultaneously again?
Solution :

∴ L.C.M. of 48,72 and 108 = 2 × 2 × 2 × 2 × 3 × 3 × 3 = 432.
432 seconds = 7 min 12 seconds.
Hence, they will change simultaneously again after 7 min 12 seconds from 7 a.m.

Question 7.
Three tankers contain 403 liters, 434 liters and 465 liters of diesel respectively. Find the ma×imum capacity of a container that can measure the diesel of the three containers e×act a number of times.
Solution :
Factors of 403 are 1, 13, 31 and 403. Factors of 434 are 1, 2, 7, 14, 31, 62, 217 and 434.
Factors of 465 are 1, 3, 5, 15, 31, 93, 155 and 465.
Common factors of 403,434 and 465 are 1 and 31.
Highest of these common factors is 31.
∴ H.C.F. of 403. 434 and 465 is 31.
Hence, the maximum capacity of the container that can measure the diesel of the three containers an e×act number of times is 31 litres.

Question 8.
Find the least number which when divided by 6, 15 and 18 leave remainder 5 in each case.
Solution :

∴ L.C.M. of 6, 15 and 18 = 2 × 3 × 3 × 5 = 90.
Hence, the required number is 90 + 5 i.e., 95.

Question 9.
Find the smallest four digit number which is divisible by 18, 24 and 32.
Solution :

∴ L.C.M. = 2 × 2 × 2 × 2 × 2 × 3 × 3 = 288.
Multiples of 288 are :
288 × 1 = 288, 288 × 2 = 576, 288 × 3 = 864, 288×4= 1152,
Hence, the smallest four digit number which is divisible by 18, 24 and 32 is 1152.

Question 10.
Find the L.C.M. of the following numbers:
(a) 9 and 4
(b) 12 and 5
(c) 6 and 5
(d) 15 and 4.
Observe a common property in the obtained L.C.M.s. Is L.C.M. the product of two numbers in each case?
Solution :
(a) 9 and 4

∴ L.C.M. of 9 and 4 = 2 × 2 × 3 × 3 = 36 (= 9 × 4).

(b) 12 and 5

∴ L.C.M. of 12 and 5 = 2×2×3×5 = 60 (= 12 × 5).

(c) 6 and 5

∴ L.C.M. of 6 and 5 = 2×3×5 = 30 (= 6 × 5).

(d) 15 and 4

∴ L.C.M. of 15 and 4 = 2 × 2 × 3 × 5 = 60 (=15×4).
We observe a common property in the obtained L.C.M.’s that L.C.M. is the product of two numbers in each case.

Question 11.
Find the L.C.M. of the following numbers in which one number is the factor of the other.
(a) 5, 20
(b) 6, 18
(c) 12, 48
(d) 9, 45.
What do you observe in the results obtained?
Solution :
(a) 5, 20
Prime factorisations of 5 and 20 are as follows: 5 = 5
20 = 2 × 2 × 5 ∴ L.C.M. of 5 and 20
=2×2×5 = 20.

(b) 6, 18
Prime factorisations of 6 and 18 are as follows:
6 = 2×3 18 = 2×3×3
∴ L.C.M. of 6 and 18 = 2×3×3 = 18.

(c) 12, 48
Prime factorisations of 12 and 48 are as follows:
12 = 2 × 2 × 3
48 = 2×2×2×2×3
∴ L.C.M. of 12 and 48 = 2 × 2 × 2 × 2 × 3 = 48.

(d) 9, 45
Prime factorisations of 9 and 45 are as follows:
9 = 3×3
45 = 3 × 3 × 5
∴ L.C.M. of 9 and 45 = 3 × 3 × 5 = 45.
In the results obtained, we observe that L.C.M. of the two numbers in which one number is the factor of the other is the greater number.

We hope the NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.7 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.7, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.6 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.6.

• Playing With Numbers Class 6 Ex 3.1
• Playing With Numbers Class 6 Ex 3.2
• Playing With Numbers Class 6 Ex 3.3
• Playing With Numbers Class 6 Ex 3.4
• Playing With Numbers Class 6 Ex 3.5
• Playing With Numbers Class 6 Ex 3.7

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 3
Chapter Name	Playing With Numbers
Exercise	Ex 3.6
Number of Questions Solved	3
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.6

Question 1.
Find the H.C.F. of the following numbers,
(a) 18, 48
(b) 30, 42
(c) 18, 60
(d) 27, 63
(e) 36, 84
(f) 34, 102
(g) 70, 105, 175
(h) 91, 112, 49
(i) 18, 54, 81
(j) 12 45 75
Solution :
(a) 18,48
Factors of 18 are 1, 2, 3, 6, 9 and 18.
Factors of 48 are 1, 2, 3,4, 6, 8, 12, 16, 24 and
∴ Common factors of 18 and 48 are 1, 2, 3
Highest of these common factors is 6. ∴ H.C.F. of 18 and 48 is 6.

(b) 30,42
Factors of 30 are 1, 2, 3, 5, 6, 10, 15 and 30.
Factors of 42 are 1, 2, 3. 6. 7, 14, 21 and 42. ,
∴ Common factors of 30 and 42 are 1,2, 3 and 6.
Highest of these common factors is 6.
∴ H.C.F. of 30 and 42 is 6.

(c) 18,60
Factors of 18 are 1, 2, 3, 6, 9 and 18. Factors of 60 are 1,2, 3,4, 5,6,10 12,15, 20, 30 and 60.
∴ Common factors of 18 and 60 are 1, 2, 3 and 6.
Highest of these common factors is 6.
∴ H.C.F. of 18 and 60 is 6.

(d) 27,63
Factors of 27 are 1, 3, 9 and 27.
Factors of 63 are 1, 3, 7, 9, 21 and 63.
Common factors of 27 and 63 are 1, 3 and 9.
Highest of these common factors is 9.
∴ H.C.F. of 27 and 63 is 9.

(e) 36,84
Factors of 36 are 1, 2, 3,4, 6, 9,12, 18 and 36.
Factors of 84 are 1,2, 3,4,6,7, 12, 14,21, 28, 42 and 84.
Common factors of 36 and 84 are 1,2, 3,4, 6 and 12.
Highest of these common factors is 12.
∴ H.C.F. of 36 and 84 is 12. if) 34,102 • ‘
Factors of 34 are 1,2, 17 and 34.
Factors of 102 are 1, 2, 3,6,17, 34. 51 and 102.
∴ Common factors of 34 and 102 are 1, 2, 17 and 34.
Highest of these common factors is 34.
∴ H.C.F. of 34 and 102 is 34.

(g) 70,105,175
Factors of 70 are 1. 2, 5. 7, 10. 14, 35 and 70.
Factors of 105 are 1, 3, 5. 7. 15. 21. 35 and 105.
Factors of 175 are 1. 5, 7. 25. 35 and 175. .’. Common factors of 70, 105 and 175 are 1, 5 and 35.
Highest of these common factors is 35.
∴ H.C.F. of 70. 105 and 175 are 35.

(h) 91,112,49
Factors of 91 are 1,7, 13 and 91.
Factors of 112 are 1,2. 4. 7, 8, 14. 16. 28, 56 and 112.
Factors of 49 are 1.7 and 49.
Common factors of 91,112 and 49 are 1 and 7.
Highest of these common factors is 7.
∴ H.C.F. of 91, 112 and 49 is 7.

(i) 18,54,81
Factors of 18 are 1. 2, 3. 6, 9 and 18. Factors of 54 are 1, 2. 3, 6. 9, 18. 27 and 54.
Factors of 81 are 1. 3, 9, 27 and 81.
∴ Common factors of 18,54 and 81 are 1, 3 and 9.
Highest of these common factors is 9.
∴ H.C.F. of 18, 54 and 81 is 9.
(j) 12, 45, 75
Factors of 12 are 1, 2, 3, 4, 6 and 12. Factors of 45 are 1, 3, 5, 9, 15 and 45.
: Factors of 75 are 1, 3, 5, 15, 25 and 75.
∴ Common factors of 12,45 and 75 are 1 and 3.
Highest of these common factors is 3.
H.C.F. of 12. 45 and 75 is 3.

Question 2.
What is the H.C.F. of two consecutive :
(a) numbers?
(b) even numbers?
(c) odd numbers?
Solution :
(a) The H.C.F. of two consecutive numbers is 1.
(b) The H.C.F. of two consecutive even numbers is 2.
(c) The H.C.F. of two consecutive odd numbers is 1.

Question 3.
H. C.F. of co-prime numbers 4 and 15 was found as follows factorization: 4 = 2 × 2 and 15 = 3 × 5 since there is no common prime factor, so H.C.F. of 4 and 15 is 0. Is the answer correct? If not, what is the correct H.C.F?
Solution :
No, the answer is not correct. The correct answer is as follows :
H.C.F. of 4 and 15 is 1.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.6 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.6, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.5 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.5.

• Playing With Numbers Class 6 Ex 3.1
• Playing With Numbers Class 6 Ex 3.2
• Playing With Numbers Class 6 Ex 3.3
• Playing With Numbers Class 6 Ex 3.4
• Playing With Numbers Class 6 Ex 3.6
• Playing With Numbers Class 6 Ex 3.7

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 3
Chapter Name	Playing With Numbers
Exercise	Ex 3.6
Number of Questions Solved	12
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.5

Question 1.
Which of the following statements are true?
(a) If a number is divisible by 3, it must be divisible by 9.
(b) If a number is divisible by 9, it must be divisible by 3.
(c) A number is divisible by 18 if it is divisible by both 3 and 6.
(d) If a number is divisible by 9 and 10 both, then it must be divisible by 90.
(e) If two numbers are co-primes, at least one of them must be prime.
(f) All numbers which are divisible by 4 must be divisible by 8.
(g) All numbers which are divisible by 8 must also be divisible by 4.
(h) If a number exactly divides two numbers separately, it must exactly divide their sum.
(i) If a number exactly divides the sum of two numbers, it must exactly divide the two numbers separately.
Solution :
(a) This statement is false.
(b) This statement is true.
(c) This statement is false.
(d) This statement is true.
(e) This statement is false.
(j) This statement is false.
(g) This statement is true.
(h) This statement is true.
(i) This statement is false.

Question 2.
Here are two different factor trees for 60. Write the missing numbers.
(a)

(b)

Solution :
(a)

(b)

Question 3.
Which factors are not included in the prime factorization of a composite number?
Solution :
1 and the number itself are not included in the prime factorization of a composite number.

Question 4.
Write the greatest 4-digit number and e×press it in terms of its prime factors.
Solution :
The greatest 4 digit number is 9999.

∴ 9999 = 3×3× 11 × 101.

Question 5.
Write the smallest 5-digit number and e×press it into the form of its prime factors.
Solution :
The smallest 5-digit number is 10000.

∴ 10000 = 2×2×2×2×5×5×5×5.

Question 6.
Find all the prime factors of 1729 and arrange them in ascending order. Now state the relation, if any; between two consecutive prime factors.
Solution :

All the prime factors of 1729 are 7, 13 and 19. When arranged in ascending order, these are 7, 13, 19. We observe that 13 – 7 = 6 19 – 13 = 6
Relation: The difference between two consecutive prime factors is 6.

Question 7.
The product of three consecutive numbers is always divisible by 6. E×plain this statement with the help of some examples.
Solution :
Example 1: Take three consecutive numbers 21, 22 and 23.
21 is divisible by 3.
22 is divisible by 2.
∴ 21 × 22 is divisible by 3 × 2 ( = 6)
∴ 21 × 22 × 23 is divisible by 6.

E×ample 2: Take three consecutive numbers 47, 48 and 49.
48 is divisible by 2 and 3 both.
∴ 48 is divisible by 2 × 3 (= 6)
47 × 48 × 49 is divisible by 6.

Question 8.
The sum of two consecutive odd numbers is divisible by 4. Verify this statement with the help of some examples.
Solution :
Example 1: Take two consecutive odd numbers 5 and 7.
Sum of these numbers = 5 + 7=12 12 is divisible by 4.
Example 2: 13 and 15
Sum of 13 and 15 =13+15 = 28
28 is divisible by 4.

Question 9.
In which of the following expressions, prime ffactorizationhas been done: j
(a) 24 = 2 × 3 × 4
(b) 56 = 7 × 2 × 2 × 2
(c) 70 = 2 × 5 × 7
(d) 54 = 2 × 3 × 9
Solution :
(a) Prime factorisation has not been done.
(b) Prime factorisation has been done.
(c) Prime factorisation has been done.
(d) Prime factorisation has not been done.

Question 10.
Determine, if 25110 is divisible by 45. [Hint : 5 and 9 are co-prime numbers. Test the divisibility of the number by 5 and 9]
Solution :
Divisibility of 25110 by 5
Number in the unit’s place of 25110 = 0
∴ 25110 is divisible by 5.
Divisibility of 25110 by 9
Sum of the digits of the number 25110 = 2+ 5 + 1 + 1 + 0 = 9
9 is divisible by 9.
∴ 25110 is divisible by 9
As 25110 is divisible by 5 and 9 both and 5 and 9 are co-prime numbers, so 25110 is divisible by 5 × 9 = 45.

Question 11.
18 is divisible by both 2 and 3. It is also divisible by 2 × 3 = 6. Similarly, a number is divisible by both 4 and 6. Can we say that the number must also be divisible by 4 × 6 = 24 ? If not, give an example to justify your answer.
Solution :
No we cannot say that the number will be divisible by 4 × 6 = 24, if it is divisible by both 4 and 6 because 4 and 6 are not co-prime numbers (they have two common factors 1 and 2).
Example : 36 is divisible by both 4 and 6.
But, 36 is not divisible by 24.

Question 12.
am the smallest number, having four different prime factors. Can you find me ?
Solution :
The smallest four different prime numbers are 2. 3. 5 and 7.
∴ The smallest number, having four different prime factors is 2 × 3 × 5 × 7 = 210.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.5 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.5, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.4 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.4.

• Playing With Numbers Class 6 Ex 3.1
• Playing With Numbers Class 6 Ex 3.2
• Playing With Numbers Class 6 Ex 3.3
• Playing With Numbers Class 6 Ex 3.5
• Playing With Numbers Class 6 Ex 3.6
• Playing With Numbers Class 6 Ex 3.7

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 3
Chapter Name	Playing With Numbers
Exercise	Ex 3.4
Number of Questions Solved	7
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.4

Question 1.
Find the common factors of:
(a) 20 and 28
(b) 15 and 25
(c) 35 and 50
(d) 56 and 120.
Solution :
(a) 20 and 28
Factors of 20 are 1, 2,4, 5, 10 and 20.
Factors of 28 are 1, 2,4, 7, 14 and 28.
Hence, the common factors of 20 and 28 are 1, 2 and 4.

(b) 15 and 25
Factors of 15 are 1, 3, 5 and 15.
Factors of 25 are 1, 5 and 25.
Hence, the common factors of 15 and 25 are 1 and 5.

(c) 35 and 50
Factors of 35 are 1, 5, 7 and 35.
Factors of 50 are 1, 5, 10, 25 and 50.
Hence, the common factors of 35 and 50 are 1 and 5.

(d) 56 and 120
Factors of 56 are 1, 2,4, 7, 8, 14, 28 and 56. Factors of 120 are 1, 2, 3,4, 5, 6, 8,10, 12,15, 20, 24, 30,40, 60 and 120. ,
Hence, the common factors of 56 and 120 are 1, 2,4 and 8.

Question 2.
Find the common factors of:
(a) 4, 8 and 12
(b) 5, 15 and 25.
Solution :
(a) 4,8 and 12 Factors of 4 are 1,2 and 4.
Factors of 8 are 1, 2,4 and 8.
Factors of 12 are 1, 2, 3,4,6 and 12.
Hence, the common factors of 4,8 and 12 are 1, 2 and 4.

(b) 5,15 and 25
Factors of 5 are 1 and 5.
Factors of 15 are 1, 3 and 5.
Factors of 25 are 1,5 and 25.
Hence, the common factors of 5,15 and 25 are 1 and 5.

Question 3.
Find the first three common multiples of:
(a) 6 and 8
(b) 12 and 18.
Solution :
(a) 6 and 8
Multiples of 6 are 6, 12, 18, 24, 30, 36, 42, 48, 54, 60, 66, 72,78, 84,90,96,
Multiples of 8 are 8, 16, 24, 32, 40, 48, 56, 64, 72, 80, 88, 96
Common multiples of 6 and 8 are 24, 48, 72, 96,
∴ First three common multiples of 6 and 8 are 24, 48 and 72.

(b) 12 and 18
Multiples of 12 are 12, 24, 36, 48, 60, 72, 84, 96, 108, 120, 132, 144, *
Multiples of 18 are 18,36,54,72,90,108,126, 144,
∴ Common multiples of 12 and 18 are 36, 72,108, 144,
∴ First three common multiples of 12 and 18 are 36, 72 and 108.

Question 4.
Write all the numbers less than 100 which are common multiples of 3 and 4.
Solution :
Multiples of 3 are : 3, 6, 9, 12, 15, 18, 21, 24, 27, 30, 33, 36, 39, 42, 45, 48, 51, 54, 57, 60, 63, 66, 69, 72, 75, 78, 81, 84, 87, 90, 93, 96, 99, 102, 105, 108,
Multiples of 4 are : 4, 8, 12, 16, 20, 24, 28, 32, 36, 40, 44, 48, 52, 56, 60, 64, 68, 72, 76, 80, 84, 88, 92, 96, 100, 104, 108,
Common multiples of 3 and 4 are : 12, 24, 36, 48, 60, 72, 84, 96, 108,
∴ All the numbers less than 100 which are common multiples of 3 and 4 are 12, 24, 36, 48, 60, 72, 84 and 96.

Question 5.
Which of the following numbers are co-prime :
(a) 18 and 35
(b) 15 and 37
(c) 30 and 415
(d) 17 and 68
(e) 216 and 215
(f) 81 and 16.
Solution :
(a) 18 and 35
Factors of 18 are 1, 2, 3, 6, 9 and 18.
Factors of 35 are 1, 5, 7 and 35.
∴ A common factor of 18 and 35 is 1.
∴ 18 and 3 5 have only 1 as the common factor
∴ 18 and 35 are co-prime numbers.

(b) 15 and 37
Factors of 15 are 1, 3, 5 and 15.
Factors of 37 are 1 and 37.
∴ A common factor of 15 and 37 is 1. v 15 and 37 have only 1 as the common factor ∴ 15 and 37 are co-prime numbers.

(c) 30 and 415
Factors of 30 are 1, 2, 3, 5, 6, 10, 15 and 30.
Factors of 415 are 1, 5, 83 and 415.
∴ Common factors of 30 and 415 are 1 and 5. v
∴ 30 and 415 have two common factors
∴ 30 and 45 are not co-prime numbers.

(d) 17 and 68 Factors of 17 are 1 and 17.
Factors of 68 are 1, 2, 4, 17, 34 and 68.
∴ Common factors of 17 and 68 are 1 and 17.
∴ 17 and 68 have two common factors
∴ 17 and 68 are not co-prime numbers.

(e) 216 and 215
Factors of 216 are 1,2, 3, 4, 6, 8, 9, 12, 18, 24, 27, 36, 54, 72, 108 and 216.
Factors of 215 are 1, 5 and 43
∴ A common factor of 216 and 215 is 1.
∴ 216 and 215 have only 1 as the common factor
∴ 216 and 215 are co-prime numbers.

(f) 81 and 16
Factors of 81 are 1, 3, 9, 27 and 81.
Factors of 16 are 1, 2, 4, 8 and 16.
∴ A common factor of 81 and 16 is 1.
∴ 81 and 16 have only 1 as the common factor
∴ 81 and 16 are co-prime numbers.

Question 6.
A number is divisible by both 5 and 12. By which other numbers will that number be always divisible?
Solution :
The number will be always divisible by 5 × 12 = 60.

Question 7.
A number is divisible by 12. By what other numbers will that number be divisible?
Solution :
Factors of 12 are 1, 2, 3, 4, 6 and 12. So, that number will be divisible by other numbers 1, 2, 3, 4 and 6.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.4 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.4, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.3 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.3.

• Playing With Numbers Class 6 Ex 3.1
• Playing With Numbers Class 6 Ex 3.2
• Playing With Numbers Class 6 Ex 3.4
• Playing With Numbers Class 6 Ex 3.5
• Playing With Numbers Class 6 Ex 3.6
• Playing With Numbers Class 6 Ex 3.7

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 3
Chapter Name	Playing With Numbers
Exercise	Ex 3.3
Number of Questions Solved	6
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.3

Question 1.
Using divisibility tests, determine which the following numbers are divisible by 2; by 3; by 4; by 5; by 6; by 8; by 9; by 10; by 11; (say yes or no):

Solution :

Question 2.
Using divisibility tests, determine which of the following numbers are divisible by 4: by 8:
(a) 572
(b) 726352
(C) 5500
(d) 6000
(e) 12159
(f) 14560
(g) 21084
(h) 31795072
(i) 1700
(j) 2150.
Solution :
(a) 572
(i) Divisibility by 4
The number formed by last two digits = 72

∵ Remainder is 0
∴ 72 is divisible by 4
∴ 572 is divisible by 4.

(ii) Divisibility by 8
The number formed by last three digits = 572

∵ Remainder = 4 ≠ 0
∴ 572 is not divisible by 8.

(b) 726352
(i) Divisibility by 4
The number formed by last two digits = 52

∵ Remainder is 0
∴ 52 is divisible by 4
∴ 726352 is divisible by 4.

(ii) Divisibility by 8
The number formed by last three digits = 352

∵ Remainder is 0
∴ 352 is divisible by 8
∴ 726352 is divisible by 8.

(c) 5500
(i) Divisibility by 4
The number formed by last two digits = 00, which is divisible by 4 5500 is divisible by 4.
∴ 500 is Divisibility by 4

(ii)
The number formed by last three digits = 500

∵ Remainder 4 0
∴ 500 is not divisible by 8
∴ 5500 is not divisible by 8.

(d) 6000
(i) Divisibility by 4
The number formed by last two digits = 00, which is divisible by 4
6000 is divisible by 4.

(ii) Divisibility by 8
The number formed by last three digits = 000, which is divisible by 8 6000 is divisible by 8.
6000 is divisible by 8

(e) 12159
(i) Divisibility by 4
The number formed by last two digits = 59

∵ Remainder = 3 ≠ 0
∴ 59 is not divisible by 4.
∴ 12159 is not divisible by 4.

(ii) Divisibility by 8
The number formed by last three digits =159

∵ Remainder 7 ≠ 0
∴ 159 is not divisible by 8
∴ 12159 is not divisible by 8.

(f) 14560
(i) Divisibility by 4
The number formed by last two digits = 60

∵ Remainder is 0
∴ 60 is divisible by 4
∴ 14560 is divisible by 4.

(ii) Divisibility by 8
The number formed by last three digits = 560

∵ Remainder is 0
∴ 560 is divisible by 8
∴ 14560 is divisible by 8.

(g) 21084
(i) Divisibility by 4
The number formed by last two digits = 84

∵ Remainder is 0
∴ 484 is divisible by 4
∴ 21084 is divisible by 4.

(ii) Divisibility by 8
The number formed by last three digits = 84

∵ The remainder is not 0
∴ 84 is not divisible by 8
∴ 21084 is not divisible by 8.

(h) 31795072
(i) Divisibility by 4
The number formed by last two digits = 72

∵ Remainder is 0
∴ 72 is divisible by 4
∴ 31795072 is divisible by 4.

(ii) Divisibility by 8
The number formed by last three digits = 72

∵ Remainder is 0
∴ 72 is divisible by 8
∴ 31795072 is divisible by 8.

(i) 1700
(i) Divisibility by 4
The number formed by last two digits = 00, which is divisible by 4
∴ 1700 is divisible by

(ii) Divisibility by 8
The number formed by last three digits = 700

∵ Remainder 4 ≠ 0
∴ 700 is not divisible by 8
∴ 1700 is not divisible by 8.

(j) 2150
(i) Divisibility by 4
The number formed by last two digits = 50

∵ Remainder = 2 ≠ 0
∴ 50 is not divisible by 4
∴ 2150 is not divisible by 4.

(ii) Divisibility by 8
The number formed by last three digits =150

∵ Remainder 6 ≠ 0
∴ 150 is not divisible by 8
∴ 2150 is not divisible by 8.

Question 3.
Using divisibility tests, determine which of following numbers are divisible by 6 :
(a)297144
(b) 1258
(c) 4335
(d) 61233
(e) 901352
(f) 438750
(g) 1790184
(h) 12583
(i) 639210
(j) 17852.
Solution :
We know that number is divisible by 6 if it is divisible by 2 and 3 both.
(a) 297144
(i) Divisibility by 2
Unit’s digit = 4
297144 is divisible by 2.

(ii) Divisibility by 3
Sum of the digits = 2 + 9 + 7 + 1+ 4 + 4 = 27, which is divisible by 3 297144 is divisible by 3 Since, 297144 is divisible by 2 and 3 both, so it is divisible by 6.

(b) 1258
(i) Divisibility by 2 Unit’s digit = 8 1258 is divisible by 2.
(ii) Divisibility by 3 Sum of the digits =1+2 + 5 + 8=16 which is not divisible by 3 1258 is not divisible by 3. Since 1258 is divisible by 2 but not by 3. so 1258 is not divisible by 6.

(c) 4335
(i) Divisibility by 2
Unit’s digit = 5, which is not any of the digits 0, 2, 4, 6 or 8 4335 is not divisible by 2 4335 is not divisible by 6.

(d) 61233
(i) Divisibility by 2
Unit’s digit = 3, which is not any of the digits 0, 2, 4, 6 or 8

61233 is not divisible by 2
61233 is not divisible by 6.

(e) 901352
(i) Divisibility by 2 v Unit’s digit = 2
901352 is divisible by 2.
(ii) Divisibility by 3
Sum of the digits = 9 + 0+1+3 + 5 + 2 = 20, which is not divisible by 3
901352 is not divisible by 3 Since, 901352 is divisible by 2 but not by 3, so it is not divisible by 6.

(f) 438750
(i) Divisibility by 2 v Unit’s digit = 0
438750 is divisible by 2.

(ii) Divisibility by 3
Sum of the digits = 4 + 3 + 8 + 7 + 5+ 0 = 27, which is divisible by 3
438750 is divisible by 3 Since, 438750 is divisible by 2 and 3 both, so it is divisible by 6.

(g) 1790184
(i) Divisibility by 2 v Unit’s digit = 4
1790184 is divisible by 2.
(ii) Divisibility by 3
Sum of the digits =1+7 + 9 + 0+1 + 8 + 4=30, which is divisible by 3
1790184 is divisible by 3 Since, 1790184 is divisible by 2 and 3 both, so it is divisible by 6.

(h) 12583,
(i) Divisibility by 2
Unit’s digit = 3, which is not any of the digits 0, 2, 4, 6 or 8
12583 is not divisible by 2
12583 is not divisible by 6.

(i) 639210
(i) Divisibility by 2
Unit’s digit = 0
639210 is divisible by 2.
(ii) Divisibility by 3
Sum of the digits = 6 + 3 + 9 + 2+1+0 = 21, which is divisible by 3
639210 is divisible by 3 Since, 639210 is divisible by 2 and 3 both, so it is divisible by 6.

(j) 17852
(i) Divisibility by 2
Unit’s digit = 2
∴ 17852 is divisible by 2.
(ii) Divisibility by 3 Sum of the digits =1+7 + 8 + 5 + 2 = 23, which is not divisible by 3
17852 is not divisible by 3
Since 17852 is divisible by 2 but not by 3, so it is not divisible by 6.

Question 4.
Using divisibility tests, determine which of the following numbers are divisible by 11:
(a) 5445
(b) 10824
(c) 7138965
(d) 70169308
(e) 10000001
(f) 901153.
Solution :
(a) 5445
Sum of the digits (at odd places) from the right =5+4=9
Sum of the digits (at even places) from the right =4+5=9
Difference of these sums = 9-9 = 0 v 0 is divisible by 11
5445 is divisible by 11.

(b) 10824
Sum of the digits (at odd places) from the right = 4 + 8+1 = 13
Sum of the digits (at even places) from the right = 2 + 0 = 2
Difference of these sums = 13 – 2 = 11
11 is divisible by 11
10824 is divisible by 11.

(c) 7138965
Sum of the digits (at odd places) from the right =5+9+3+7=24
Sum of the digits (at even places) from the right = 6 + 8 + 1 = 15
Difference of these sums = 24 – 15 = 9
Y 9 is not divisible by 11
∴ 7138965 is not divisible by 11.

(d) 70169308
Sum of the digits (at odd places) from the right = 8 + 3+6 + 0=17
Sum of the digits (at even places) from the right = 0 + 9 + 1+7=17 Difference of these sums = 17-17=0
Y 0 is divisible by 11
∴ 70169308 is divisible by 11.

(e) 10000001
Sum of the digits (at odd places) from the right =l+0+0+0=l
Sum of the digits (at even places) from the right =0+0+0+1=1 Difference of these sums =1-1=0 0 is divisible by 11
∴ 10000001 is divisible by 11.

(f) 901153
Sum of the digits (at odd places) from the right = 3+ 1 +0 = 4
Sum of the digits (at even places) from the right = 5+1+9=15
Difference of these sums =15-4=11
Y 11 is divisible by 11
∴ 901153 is divisible by 11.

Question 5.
Write the smallest digit and the largest digit in the blank space of each of the following numbers so that the number is divisible by 3:
(a) __ 6724
(b) 4765 __ 2.
Solution :
(a) __ 6724
(i) Smallest digit
Sum of the given digits = 6 + 7 + 2 + 4=19
19 is not divisible by 3
∴ Smallest digit (non-zero) is 2.

(ii) Largest digit The largest digit is 8.

(b) 4765 _ 2
(i) Smallest digit
Sum of the given digits = 4 + 7 + 6 + 5 + 2 = 24 Y 24 is divisible by 3
∴ Smallest digit is 0.

(ii) Largest digit The largest digit is 9.

Question 6.
Write digit in the blank space of each of the following numbers so that the number is divisible by 11:
(a) 92 __ 389
(b) 8 __ 9484.
Solution :
(a) 92 __ 389
Sum of the given digits (at odd places) from the right = 9 + 3 + 2= 14
Sum of the given digits (at even places) from the right = 8 + required digit + 9 = required digit + 17
Difference of these sums = required digit + 3 For the above difference to be divisible by 11, required digit = 8.
Hence, the required number is 92 8 389.

(b) 8 __ 9484
Sum of the given digits (at odd places) from the right = 4 + 4 + required digit = 8 + required digit
Sum of the given digits (at even places) from the right = 8 + 9 + 8 = 25
Difference of the sums = 17 – required digit For the above difference to be divisible by 11, required digit = 6.
Hence, the required number is 8 6 9484.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.3 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.3, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.2 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.2.

• Playing With Numbers Class 6 Ex 3.1
• Playing With Numbers Class 6 Ex 3.3
• Playing With Numbers Class 6 Ex 3.4
• Playing With Numbers Class 6 Ex 3.5
• Playing With Numbers Class 6 Ex 3.6
• Playing With Numbers Class 6 Ex 3.7

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 3
Chapter Name	Playing With Numbers
Exercise	Ex 3.2
Number of Questions Solved	12
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.2

Question 1.
What is the sum of any two :
(a) Odd numbers
(b) Even numbers.
Solution :
(a) The sum of any two odd numbers is an even number.
(b) The sum of any two even numbers is an even number.

Question 2.
State whether the following statements are True or False :

1. The sum of three odd numbers is even.
2. The sum of two odd numbers and one even number is even.
3. The product of three odd numbers is odd.
4. If an even number is divided by 2, the quotient is always odd.
5. All prime numbers are odd.
6. Prime numbers do not have any factors.
7. Sum of two prime numbers is always even.
8. 2 is the only even prime number.
9. All even numbers are composite numbers.
10. The product of two even numbers is always even.

Solution :

1. This statement is false.
2. This statement is true.
3. This statement is true.
4. This statement is false.
5. This statement is false.
6. This statement is false.
7. This statement is false.
8. This statement is true.
9. This statement is false.
10. This statement is true.

Question 3.
The numbers 13 and 31 are prime numbers. Both these numbers have the same digits 1 and 3. Find such pairs of prime numbers up to 100.
Solution :
All other such pairs of prime numbers up to 100 are as follows :
17 and 71; 37 and 73; 79 and 97.

Question 4.
Write down separately the prime and composite numbers less than 20.
Solution :

Question 5.
What is the greatest prime number between 1 and 10?
Solution :
The greatest prime number between 1 and 10 is 7.

Question 6.
Express the following as the sum of two odd primes :
(a) 44
(b) 36
(c) 24
(d) 18.
Solution :
(a) 44 = 3+41
(b) 36 = 5 + 31
(c) 24 = 5+19
(d) 18 = 5+13.

Question 7.
Give three pairs of prime numbers whose difference is 2.
[Remark: Two prime numbers whose difference is 2 are called twin primes],
Solution :
The three pairs of prime numbers, whose difference is 2, are as follows :
3, 5 ; 5, 7 ; 11, 13.

Question 8.
Which of the following numbers are prime?
(a) 23
(b) 51
(c) 37
(d) 26.
Solution :
(a) 23,
(c) 37 are prime numbers.

Question 9.
Write seven consecutive composite numbers less than 100 so that there is no prime number between them.
Solution :
90, 91, 92, 93, 94, 95, 96.

Question 10.
Express each of the following numbers as the sum of three odd primes :
(a) 21
(b) 31
(c) 53
(d) 61.
Solution :
(a) 21 = 3 + 5 + 13
(b) 31 = 3 + 5 + 23
(c) 53 = 13+ 17 + 23
(d) 61 =7;+ 13+41.

Question 11.
Write five pairs of prime numbers below 20 whose sum is divisible by 5. (Hint: 3 + 7 – 10).
Solution :
2, 3 ; 2, 13 ; 3, 17 ; 7, 13 ; 11, 19.

Question 12.
Fill in the blanks in the following :

1. A number which has only two factors is called a
2. A number which has more than two factors is called a
3. 1 is neither nor
4. The smallest prime number is
5. The smallest composite number is
6. The smallest even number is

Solution :

1. A number which has only two factors is called a prime number.
2. A number which has more than two factors is called a composite number.
3. 1 is neither prime number nor composite number.
4. The smallest prime number is 2.
5. The smallest composite number is 4.
6. The smallest even number is 2.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.2 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 3 Playing With Numbers Ex 3.2, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.3 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.3.

• Whole Numbers Class 6 Ex 2.1
• Whole Numbers Class 6 Ex 2.2

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 2
Chapter Name	Whole Numbers
Exercise	Ex 2.3
Number of Questions Solved	5
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.3

Question 1.
Which of the following will not represent
(a) 1 + 0
(b) 0 × 0
(c) \(\frac { 0 }{ 2 }\)
(d) \(\frac { 10\quad 10 }{ 2 }\)
Solution :
(a) 1 + 0 = 1 ≠ 0
(b) 0 × 0 = 0
(c) \(\frac { 0 }{ 2 }\)
(d) \(\frac { 10-10 }{ 2 } =\frac { 0 }{ 2 } =0\)
Hence, (a) 1 + 0 will not represent zero.

Question 2.
If the product of two whole numbers is zero, can we say that one or both of them will be zero? Justify through examples.
Solution :
Yes! For example :
2 × 0 = 0
0 × 3 = 0
0 × 0 = 0.

Question 3.
If the product of two whole numbers is 1. can we say that one or both of them will be 1? Justify through examples.
Solution :
Both of them must be ‘ 1’ as 1 × 1 = 1.

Question 4.
Find using distributive property :
(a) 728 × 101
(b) 5437 × 1001
(c) 824 × 25
(d) 4275 × 125
(e) 504 × 35
Solution :

Question 5.
Study the pattern :

Write the next two steps. Can you say how the pattern works ?
(Hint : 12345 =11111 + 1 1 11 + 111 + 11 + 1). Sol. Next two steps are as follows :
Solution :
Next two steps are as follows :
123456 x 8 + 6 = 987654
1234567 x 8 + 7 = 9876543.
Working of the pattern

We hope the NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.3 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.3, drop a comment below and we will get back to you at the earliest.