Free PDF Download of CBSE Maths Multiple Choice Questions for Class 12 with Answers Chapter 13 Probability. Maths MCQs for Class 12 Chapter Wise with Answers PDF Download was Prepared Based on Latest Exam Pattern. Students can solve NCERT Class 12 Maths Probability MCQs Pdf with Answers to know their preparation level.
Probability Class 12 Maths MCQs Pdf
1. Let A and B be two given events such that P(A) = 0.6, P(B) = 0.2 and P(A/B) = 0.5. Then P(A’/B’) is

Answer/Explanation
Answer: c
Explaination:
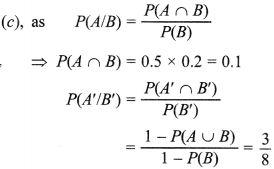
2. Let A and B be two given independent events such that P(A) =p and P(B) = q and P(exactly one of A, B) = \(\frac{2}{3}\), then value of 3p + 3q – 6pq is
(a) 2
(b) -2
(c) 4
(d) -4
Answer/Explanation
Answer: a
Explaination:

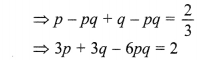
3. If P(A ∩ B) = 70% and P(B) = 85%, then P(A/B) is equal to

Answer/Explanation
Answer: a
Explaination:
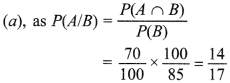
4. Two dice are thrown once. If it is known that the sum of the numbers on the dice was less than 6 the probability of getting a sum 3 is

Answer/Explanation
Answer: c
Explaination: (c), as favourable cases for sum less than 6 are 10 and favourable for a total of 3 is 2.
5. Three balls are drawn from a bag containing 2 red and 5 black balls, if the random variable X represents the number of red balls drawn, then X can take values
(a) 0, 1, 2
(b) 0, 1, 2, 3
(c) 0
(d) 1, 2
Answer/Explanation
Answer: a
Explaination: (a), as there are 2 red balls, so maximum ’ red balls can be 2.
6. The probability distribution of the discrete variable X is given as:
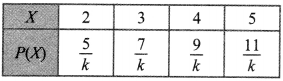
The value of k is
(a) 8
(b) 16
(c) 32
(d) 48
Answer/Explanation
Answer:
Explaination:

7. Let A and B be two given mutually exclusive events. Then P(A/B) is _________ .
Answer/Explanation
Answer:
Explaination: 0, as P(A ∩ B) = 0 and P(A/B) = \(\frac{P(A \cap B)}{P(B)}\)
8. If A and B are two independent events such that P(A) = \(\frac{1}{7}\) and P(B) = \(\frac{1}{6}\) then P(A’ ∩ B’) is _________ .
Answer/Explanation
Answer:
Explaination:

9. Three persons A, B and C, fire a target in turn. Their probabilities of hitting the target are 0.2,0.3 and 0.5 respectively, the probability that target hit is _________ .
Answer/Explanation
Answer:
Explaination:
0.72, as /^target hit)
= P(at least one hits the target)
= 1 – P( none hits) =1 \(-P(\bar{A} \bar{B} \bar{C})\)
10. If A and B are independent events, then P(A and B) = P(A) + P(B). State true or false.
Answer/Explanation
Answer:
Explaination: False, as P(A and B) = P(A) P(B).
11. A speaks truth in 70% cases and B speaks truth in 85% cases. The probability that they speak the same fact is ______ .
Answer/Explanation
Answer:
Explaination:

12. Given P(A) = 0.4, P(B) = 0.7 and P(B/A) = 0.6. Find P(A ∪ B).
Answer/Explanation
Answer:
Explaination: .
P(B/A) = \(\frac{P(A \cap B)}{P(A)}\)
⇒ 0.6 × 0.4 = P (A ∩ B)
⇒ P(A ∩ B) = 0.24
Now, P(A ∪ B) = P(A) + P(B) – P(A ∩ B)
= 0.4 + 0.7 – 0.24
= 0.86
13. Given P(A) = \(\frac{1}{2}\) p(B) = \(\frac{1}{3}\) and P(A ∩ B) = \(\frac{1}{6}\). Are the events A and B independent?
Answer/Explanation
Answer:
Explaination:
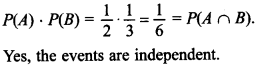
14. Prove that if E and F are independent events, then the events E and F are also independent. [Delhi 2017]
Answer/Explanation
Answer:
Explaination:
As E and F are independent events
∴ P(E ∩ F) = P(E)P(F) …(i)
Consider,
P(E)P(F) = P(E)[1 – P(F)]
= P(E) – P(E)P(F)
= P(E) – P(E ∩ F)
P(E)P(F’) = P(E ∩ F’)
⇒ E and F’ are independent events.
15. A die, whose faces are marked 1, 2, 3 in red and 4,5,6 in green, is tossed. Let A be the event “number obtained is even” and B be the event “number obtained is red”. Find if A and B are independent events. [AI 2017]
Answer/Explanation
Answer:
Explaination:
A : number is even, i.e. 2, 4, 6; P(A) = \(\frac{3}{6}\)
= \(\frac{1}{2}\)
B : number is red, i.e. 1, 2, 3; P(B) = \(\frac{3}{6}\)
= \(\frac{1}{2}\)
A ∩ B : number is even and red, i.e. 2;
P(A ∩ B) = \(\frac{1}{6}\)
P(A) × P(B) = \(\frac{1}{2} \times \frac{1}{2}=\frac{1}{4}\)
As P(A ∩ B) ≠ P(A) . P(B)
Hence, not independent.
16. A black and a red die are rolled together. Find the conditional probability of obtaining the sum 8, given that the red die resulted in a number less than 4. [CBSE 2018]
Answer/Explanation
Answer:
Explaination:
A : sum 8, i.e. (2, 6), (3, 5), (4, 4), (5, 3), (6, 2)
B : red die number less than 4.
B : (1, 1), (2, 1), (3, 1), (4, 1), (5, 1), (6, 1), (1, 2), (2, 2), (3, 2), (4, 2), (5, 2), (6, 2), (1, 3), (2, 3), (3, 3), (4, 3), (5, 3), (6, 3).
A ∩ B : (6, 2), (5, 3)
∴ Conditional probability of obtaining sum 8, given that red die resulted in number less than 4.
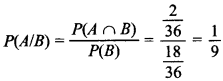
17. 12 cards numbered 1 to 12 are placed in a box, mixed up thoroughly and then a card is drawn at random from the box. If it is known that the number on the drawn card is more than 3, find the probability that it is an even number.
Answer/Explanation
Answer:
Explaination:
Total cards are 12
A : number drawn is more than 3, i.e. 4, 5, 6, …, 12.
B : getting an even number, i.e. 2, 4, 6, 8, 10, 12.
A ∩ B : 4, 6, 8, 10, 12
![]()
18. One card is drawn from a well shuffled pack of 52 cards. If E is the event “the card drawn is a king or a queen” and F is the event “the card drawn is an ace or a queen,” then find the probability of the conditional event E/F. [HOTS]
Answer/Explanation
Answer:
Explaination:

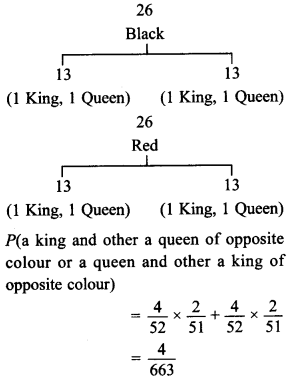
19. Two cards are drawn from a well shuffled pack of 52 cards one after the other without replacement. Find the probability that one of them is a queen and the other is a king of opposite colour.
Answer/Explanation
Answer:
Explaination:
20. A coin is tossed thrice and all eight outcomes are assumed equally likely. Let the event E be “the first throw results in head” and event F be “the last throw results in tail”. Find whether events E and F are independent.
Answer/Explanation
Answer:
Explaination:
Sample space
S = {HHH, HHT, HTH, THH, HTT, THT, TTH, TTT}
E: first throw results in head = {HHH,HHT,HTH,HTT}
F: Last throw result in tail = {HHT, HTT, THT, TTT}

21. Two dice are thrown. Find the probability that the numbers appeared have a sum 8 if it is known that the second die always exhibits 4. [HOTS]
Answer/Explanation
Answer:
Explaination:
A: Sum of the numbers is 8, i.e. {(2,6), (3,5), (4,4), (5,3), (6,2)}
B: the second die always exhibits 4, i.e. {(1,4), (2,4), (3,4), (4,4), (5,4), (6,4)} A ∩ B= {(4, 4)}
Required probability,
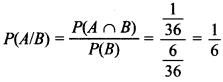
22. If P(A)=0.8,P(B)=0.5 andP(B/A)=0.4, find (i) P(A ∩ B) (ii) P(A/B) (iii) P(A ∪ B). [NCERT]
Answer/Explanation
Answer:
Explaination:
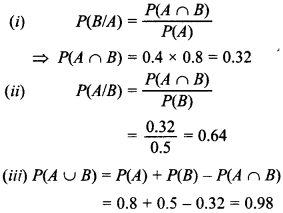
23. The value of k, for which the following distribution is a probability distribution

Answer/Explanation
Answer:
Explaination:

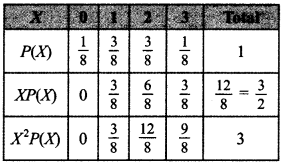
24. Find mean (µ) and variance (a2) for the following probability distribution:
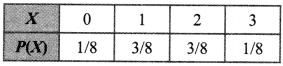
Answer/Explanation
Answer:
Explaination:
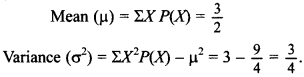
25. A random variable X has a probability distribution P(X) of the following form where k is some number:Determine (i) k (ii) P(X ≤ 2) (iii) P(X ≤ 2) (iv) P(X ≥ 2). [NCERT; Delhi 2019]
Answer/Explanation
Answer:
Explaination:
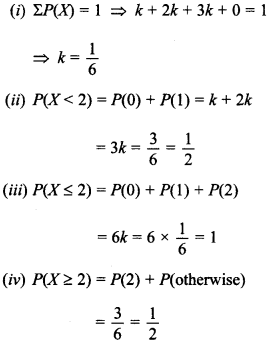
We hope the given Maths MCQs for Class 12 with Answers Chapter 13 Probability will help you. If you have any query regarding CBSE Class 12 Maths Probability MCQs Pdf, drop a comment below and we will get back to you at the earliest.