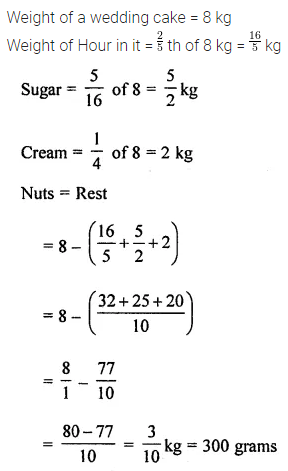ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 1 Rational Numbers Check Your Progress
Question 1.
Evaluate the following:
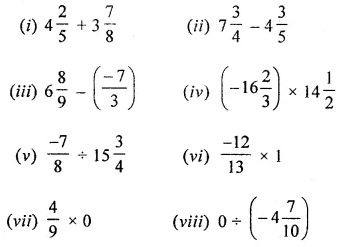
Solution:
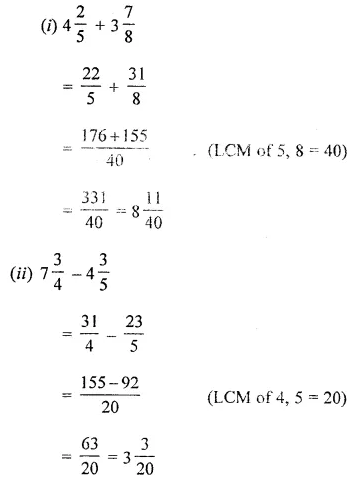

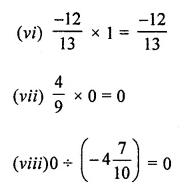
Question 2.
What number should be added to \(\frac { -4 }{ 11 }\) to get \(\frac { -3 }{ 8 }\)?
Solution:
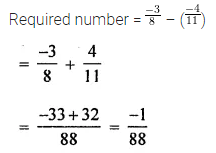
Question 3.
What rational number should be subtracted from the sum of \(\frac { 3 }{ 14 }\) and \(\frac { -4 }{ 7 }\) to get \(\frac { 13 }{ 21 }\)?
Solution:
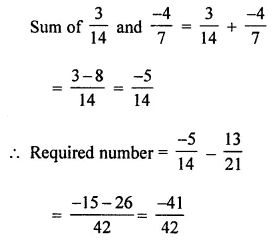
Question 4.
If the product of two rational numbers is \(\frac { 25 }{ 42 }\) and one of them -2\(\frac { 6 }{ 7 }\), find the other.
Solution:

Question 5.
Divide the sum of \(\frac { 4 }{ 13 }\) and \(\frac { -3 }{ 2 }\) by their product.
Solution:
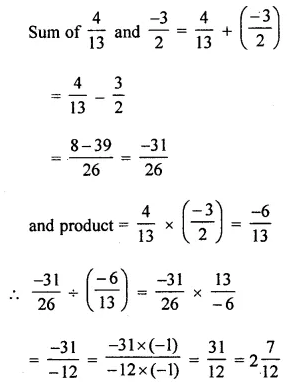
Question 6.
Using the appropriate properties of operations of rational numbers, evaluate the following:
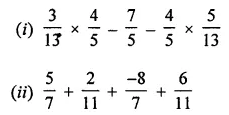
Solution:

Question 7.
Find the additive inverse of the following:
(i) -13\(\frac { 7 }{ 8 }\)
(ii) 4\(\frac { 3 }{ 6 }\)
Solution:

Question 8.
Find the multiplicative inverse of the following:
(i) \(\frac { -23 }{ 46 }\)
(ii) 0
Solution:

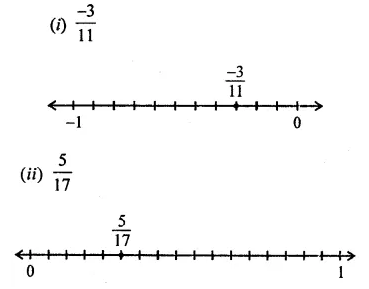
Question 9.
Represent the following rational numbers on the number line:
(i) \(\frac { -3 }{ 11 }\)
(ii) \(\frac { 5 }{ 17 }\)
Solution:
Question 10.
Insert five rational numbers between \(\frac { -3 }{ 7 }\) and \(\frac { 2 }{ 5 }\)
Solution:

Question 11.
If p = \(\frac { -4 }{ 9 }\) , q = \(\frac { 2 }{ 3 }\) and r = \(\frac { -8 }{ 11 }\), then verily the foflowing:
(i) p + (q + r) = (p + q) + r
(ii) p × q = q × p
(iii) p × (q + r) = p × q + p × r
(iv) (p + q) ÷ r = p ÷ r + q ÷ r.
Solution:
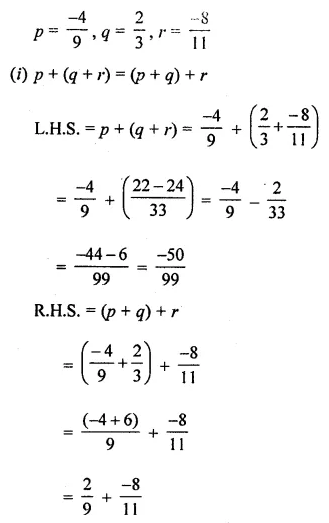
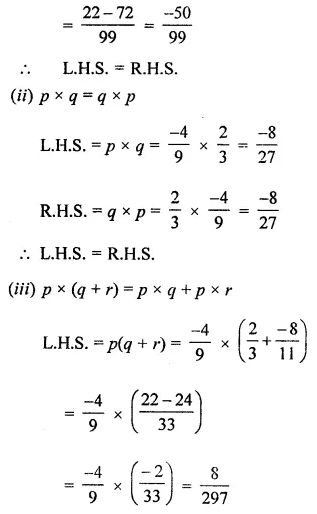
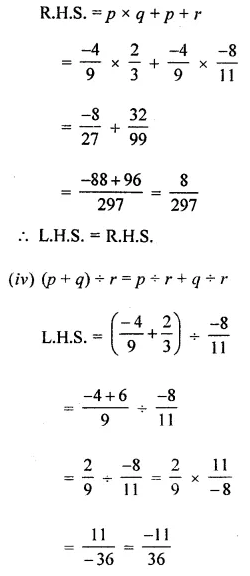
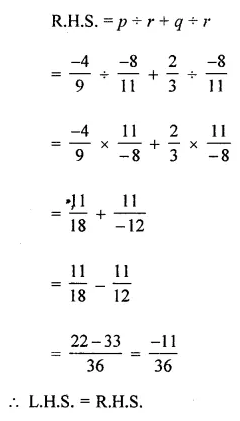
Question 12.
A wedding cake weighed 8 kg. If \(\frac { 2 }{ 5 }\) th of its weight was flour, \(\frac { 5 }{ 16 }\) th was sugar, \(\frac { 1 }{ 4 }\) thwas cream and the rest were nuts, find the weight of nuts.
Solution: