ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2

Question 1.
Write five numbers which you can decide by looking at their one’s digit that they are not square numbers.
Solution:
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 1

Question 2.
What will be the unit digit of the squares of the following numbers?
(i) 951
(ii) 502
(iii) 329
(iv) 643
(v) 5124
(vi) 7625
(vii) 68327
(viii) 95628
(ix) 99880
(x) 12796
Solution:
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 2

Question 3.
The following numbers are obviously not perfect. Give reason.
(i) 567
(ii) 2453
(iii) 5298
(iv) 46292
(v) 74000
Solution:
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 3

Question 4.
The square of which of the following numbers would be an odd number or an even number? Why?
(i) 573
(ii) 4096
(iii) 8267
(iv) 37916
Solution:
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 4

Question 5.
How many natural numbers lie between the square of the following numbers?
(i) 12 and 13
(ii) 90 and 91
Solution:
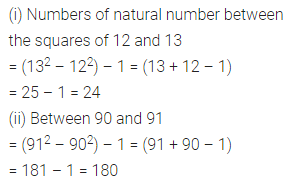
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 5

Question 6.
Without adding, find the sum.
(i) 1 + 3 + 5 + 7 + 9 + 11 + 13 + 15
(ii) 1 + 3 + 5 + 7 + 9 + 11 + 13 + 15 + 17 + 19 + 21 + 23 + 25 + 27 + 29
Solution:
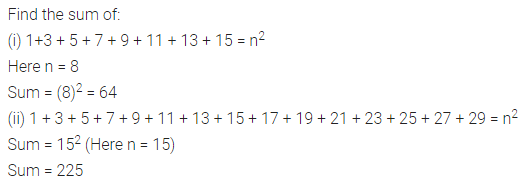
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 6

Question 7.
(i) Express 64 as the sum of 8 odd numbers.
(ii) 121 as the sum of 11 odd numbers.
Solution:
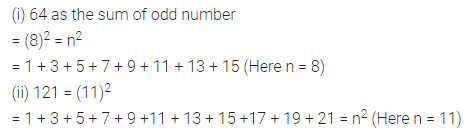
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 7

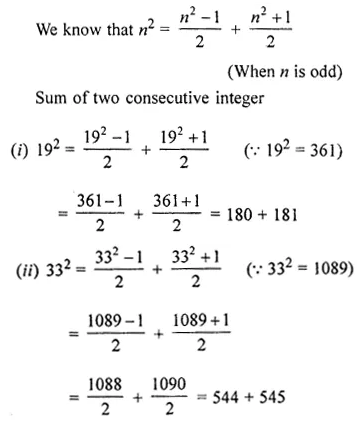
Question 8.
Express the following as the sum of two consecutive integers:
(i) 19²
(ii) 33²
(iii) 47²
Solution:
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 8

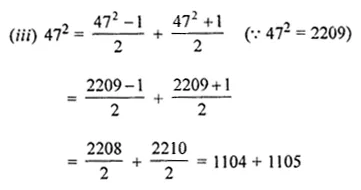
Question 9.
Find the squares of the following numbers without actual multiplication:
(i) 31
(ii) 42
(iii) 86
(iv) 94
Solution:
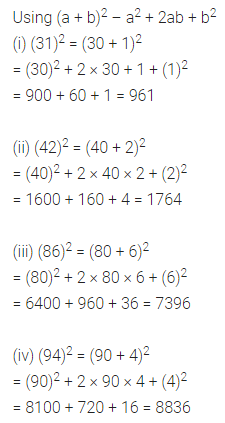
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 10

Question 10.
Find the squares of the following numbers containing 5 in unit’s place:
(i) 45
(ii) 305
(iii) 525
Solution:
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 11

Question 11.
Write a Pythagorean triplet whose one number is
(i) 8
(ii) 15
(iii) 63
(iv) 80
Solution:
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 12

Question 12.
Observe the following pattern and find the missing digits:
21² = 441
201² = 40401
2001² = 4004001
20001² = 4 – – – 4 – – – 1
200001² = ————–
Solution:
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 14

Question 13.
Observe the following pattern and find the missing digits:
9² = 81
99² = 9801
999² = 998001
9999² = 99980001
99999² = 9——–8———01
999999² = 9——–0———1
Solution:
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 15

Question 14.
Observe the following pattern and find the missing digits:
7² = 49
67² = 4489
667² = 444889
6667² = 44448889
66667² = 4 ———–8 ————– 9
666667² = 4———–8————8 –
Solution:
ML Aggarwal Class 8 Solutions for ICSE Maths Chapter 3 Squares and Square Roots Ex 3.2 16