Polynomials Class 10 Notes Maths Chapter 2

On this page, you will find Polynomials Class 10 Notes Maths Chapter 2 Pdf free download. CBSE NCERT Class 10 Maths Notes Chapter 2 Polynomials will seemingly help them to revise the important concepts in less time.

CBSE Class 10 Maths Chapter 2 Notes Polynomials

Polynomials Class 10 Notes Understanding the Lesson

1. The value of the polynomial p(x) at x = a is p(a).

2. Zeroes of the polynomial p(x) can be find by equating p(x) to zero and solving the equation for

3. If for p(x) = ax² + bx + c = 0, a ≠ 0; α and β are the zeroes, then

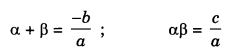
4. If for p(x) = ax³ + bx² + cx + d = 0; a ≠ 0; α, β,γ are the zeroes, then
α + β + γ = \(\frac{-b}{a}\)
αβ+βγ + αγ = \(\frac{c}{a}\)
αβγ =\(\frac{-d}{a}\)

5. If α and β are the zeroes; then quadratic polynomial will be given by K[x² – Sx + P]
where
S = α +β
P = αβ
K (≠0) is real.

6. The cubic polynomial with zeroes α, β and γ is given by
K[x³ – S₁x² + S₁x² S₃]
where
S₁ = α + β + γ
S₂ = αβ + βγ + αγ
S₃= αβγ
K(≠ 0) is real.

Degree of a Polynomial:

1. The degree of a polynomial p(x) in x is the highest power of x in p(x)

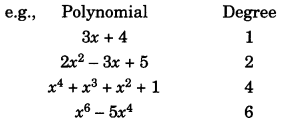
Note: Expressions like \(\frac{1}{\sqrt{x}}, \frac{1}{x^{2}+1}, \sqrt{x+2}\)

2. (i) Polynomial with degree 1, i.e., polynomial of the form ax + b; a ≠ 0 is called linear polynomial.
(ii) Polynomial with degree 2, i.e., polynomial of the form ax2 + bx + c; a ≠ 0 is called quadratic polynomial.
(iii) Polynomial with degree 3, i.e. polynomial of the form ax³ + bx² + cx + d ; a ≠ 0 is called cubic polynomial.
(iv) Polynomial with degree 4, i.e. polynomial of the form ax⁴ + bx³ + cx² + dx + e; a ≠ 0 is called biquadratic polynomial.

Geometrical Meaning of the Zeroes of a Polynomial:

1. For any polynomial y = f(x), the number of points on which the graph of y = f(x) intersects at x-axis is called the number of the zeroes of the polynomial and the x-coordinates of these points are called the zeroes of the polynomial y = f(x).

2. Polynomial with degree ‘n’ has maximum ‘n’ number of zeroes. A constant polynomial has no zeroes.

3. Geometrical representation of a linear polynomial is always a straight line.

4. Geometrical representation of a quadratic polynomial is the graph of the shape either open upwards like ‘∪’ or open downwards like ‘∩’ according to a > 0 or a < 0. These curves are called Parabola.

Relationship Between Zeroes and Coefficient of a Quadratic Polynomial:

1. If α and β are the zeroes of the quadratic polynomial  p(x) = ax² + bx + c a≠0 then

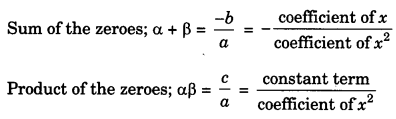
Relationship Between Zeroes and Coefficient of a Cubic Polynomial

1. If α, β and γ are the zeroes of the cubic polynomial

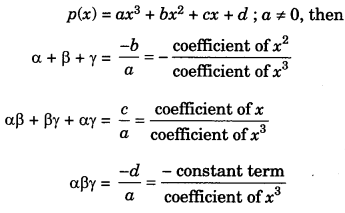
2. A quadratic polynomial p(x) with zeroes α and β is given by
p(x) = K[x² – (α + β)x + αβ]
where K(≠0) is real.

3. A cubic polynomial p(x) with α, β and γ as zeroes is given by
p(x) = K[x³ – (α + β + γ)x² + (αβ +βγ + αγ)x – αβγ
where K(≠0) is real.

Division Algorithm for Polynomials:
If p(x) and g(x) are any two polynomials where g(x) ≠ 0. Then on dividing p(x) by g(x), we find other two polynomials q(x) and r(x) such that
p(x) = g(x) x q(x) + r(x);
where deg. of r(x) < deg. of gix)
or Dividend = Divisor x Quotient + Remainder

Note:

• If r(x) = 0, then g(x) will be a factor of p(x) otherwise not.
• If any real number ‘a’ is a zero of the polynomial p(x), then (x – a) will be a factor of p(x).