RD Sharma Class 10 Solutions Chapter 2 Polynomials Ex 2.1

RD Sharma Class 10 Solutions Chapter 2 Polynomials Ex 2.1

These Solutions are part of RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 2 Polynomials Ex 2.1

Other Exercises

• RD Sharma Class 10 Solutions Chapter 2 Polynomials Ex 2.1
• RD Sharma Class 10 Solutions Chapter 2 Polynomials Ex 2.2
• RD Sharma Class 10 Solutions Chapter 2 Polynomials Ex 2.3
• RD Sharma Class 10 Solutions Chapter 2 Polynomials VSAQS
• RD Sharma Class 10 Solutions Chapter 2 Polynomials MCQS

Question 1.
Find the zeros of each of the following quadratic polynomials and verify the relationship between the zeros and their co-efficients :

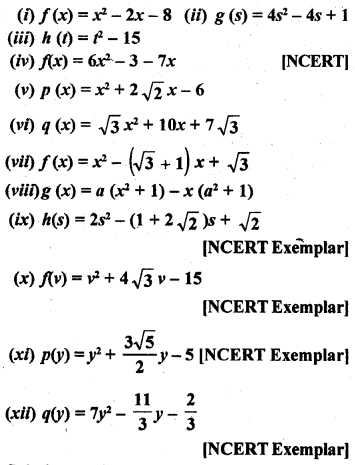
Solution:
(i) f(x) = x² – 2x – 8

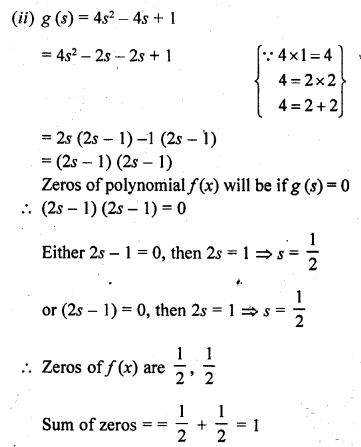
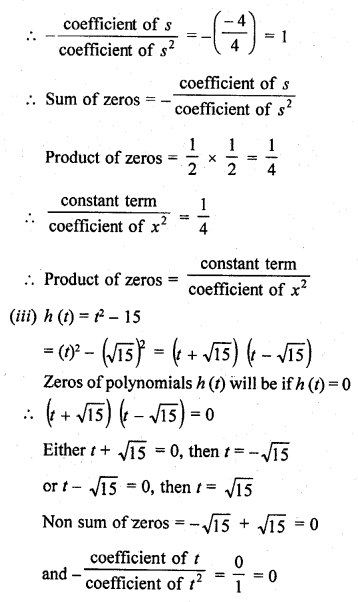
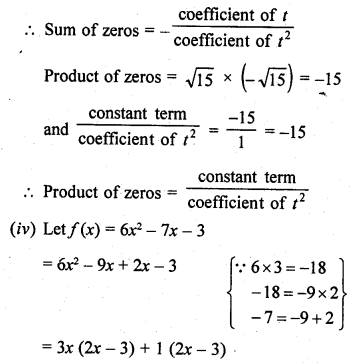
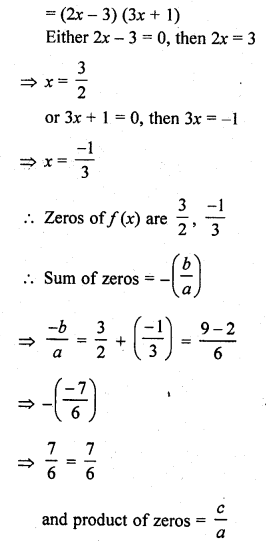
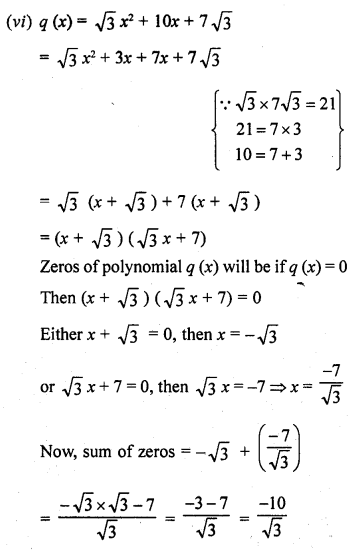
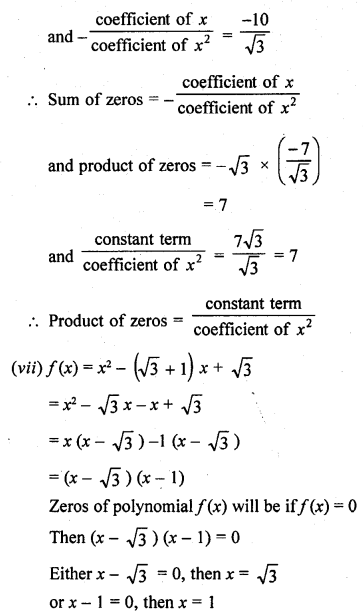
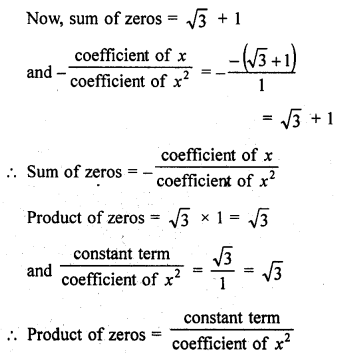
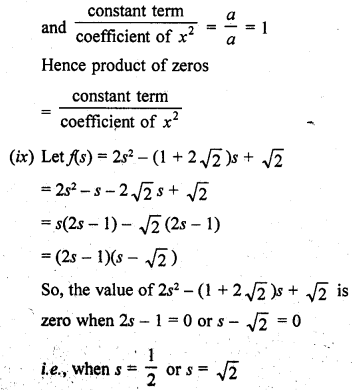
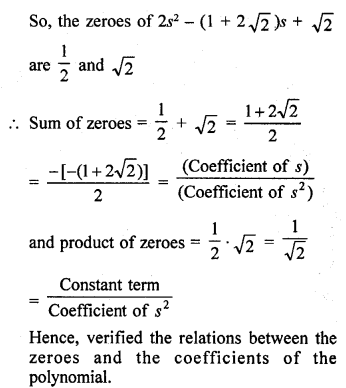
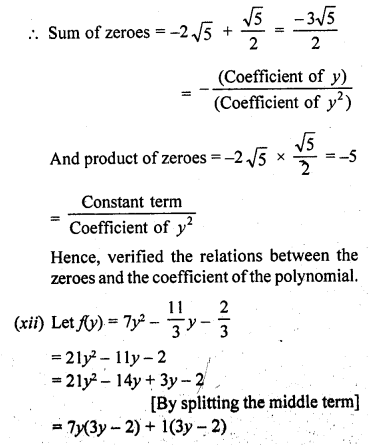
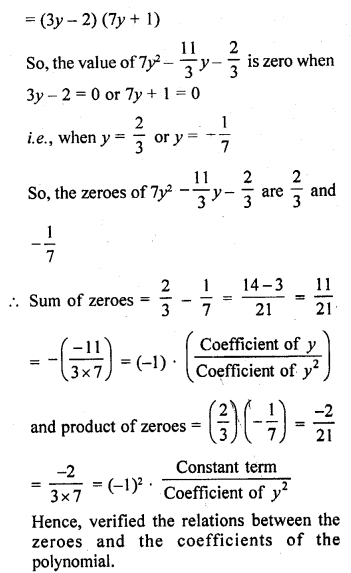
Question 2.
For each of the following, find a quadratic polynomial whose sum and product respectively of the zeroes are as given. Also, find the zeroes of these polynomials by factorization.

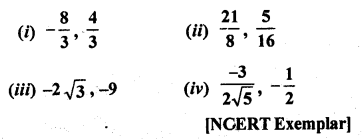
Solution:
(i) Given that, sum of zeroes (S) = – \(\frac { 8 }{ 3 }\)
and product of zeroes (P) = \(\frac { 4 }{ 3 }\)
Required quadratic expression,

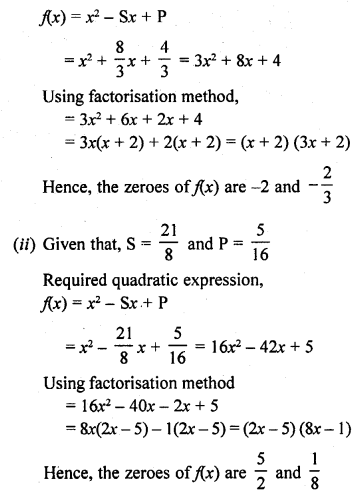
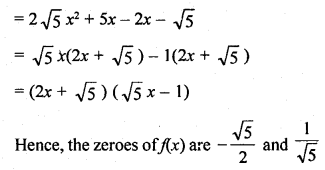
Question 3.
If α and β are the zeros of the quadratic polynomial f(x) = x² – 5x + 4, find the value of \(\frac { 1 }{ \alpha } +\frac { 1 }{ \beta } -2\alpha \beta\).
Solution:

Question 4.
If α and β are the zeros of the quadratic polynomial p(y) = 5y² – 7y + 1, find the value of \(\frac { 1 }{ \alpha } +\frac { 1 }{ \beta }\)
Solution:

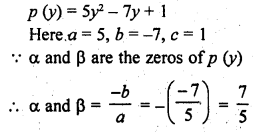
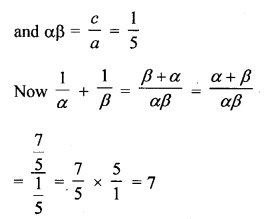
Question 5.
If α and β are the zeros of the quadratic polynomial f(x) = x² – x – 4, find the value of \(\frac { 1 }{ \alpha } +\frac { 1 }{ \beta } -\alpha \beta\)
Solution:

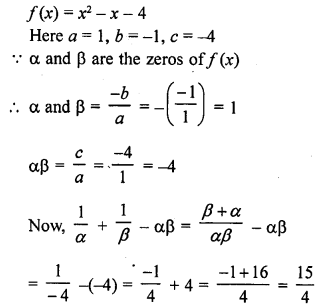
Question 6.
If α and β are the zeros of the quadratic polynomial f(x) = x² + x – 2, find the value of \(\frac { 1 }{ \alpha } -\frac { 1 }{ \beta }\)
Solution:

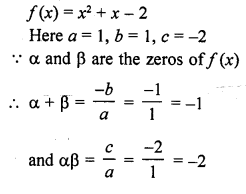
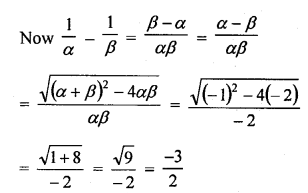
Question 7.
If one zero of the quadratic polynomial f(x) = 4x² – 8kx – 9 is negative of the other, find the value of k.
Solution:

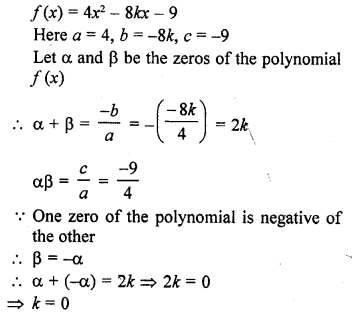
Question 8.
If the sum of the zeros of the quadratic polynomial f(t) = kt² + 2t + 3k is equal to their product, find the value of k.
Solution:

Question 9.
If α and β are the zeros of the quadratic polynomial p(x) = 4x² – 5x – 1, find the value of α²β + αβ².
Solution:

Question 10.
If α and β are the zeros of the quadratic polynomial f(t) = t² – 4t + 3, find the value of α⁴β³ + α³β⁴.
Solution:

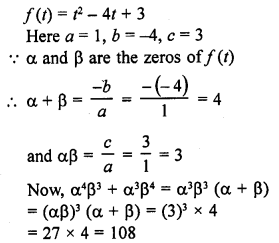
Question 11.
If α and β are the zeros of the quadratic polynomial f (x) = 6x⁴ + x – 2, find the value of \(\frac { \alpha }{ \beta } +\frac { \beta }{ \alpha }\)
Solution:

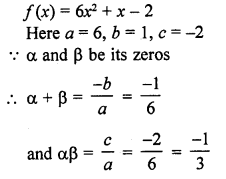
Question 12.
If α and β are the zeros of the quadratic polynomial p(s) = 3s² – 6s + 4, find the value of \(\frac { \alpha }{ \beta } +\frac { \beta }{ \alpha } +2\left( \frac { 1 }{ \alpha } +\frac { 1 }{ \beta } \right) +3\alpha \beta\)
Solution:

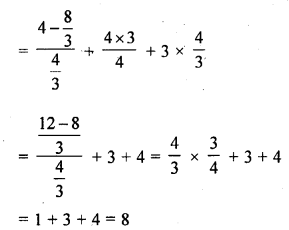
Question 13.
If the squared difference of the zeros of the quadratic polynomial f(x) = x² + px + 45 is equal to 144, find the value of p
Solution:

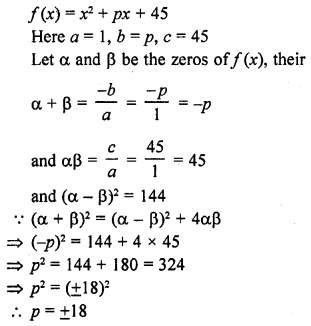
Question 14.
If α and β are the zeros of the quadratic polynomial f(x) = x² – px + q, prove that:

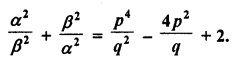
Solution:

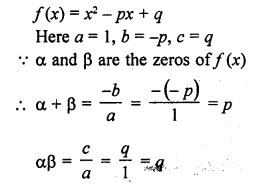
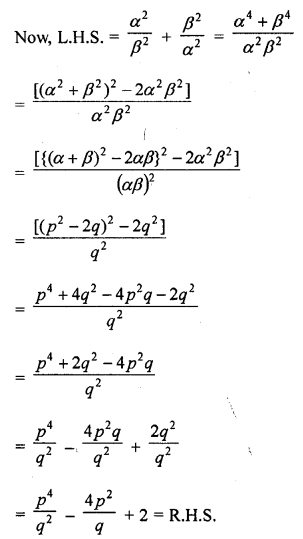
Question 15.
If α and β are the zeros of the quadratic polynomial f(x) = x² – p(x + 1) – c, show that (α + 1) (β + 1) = 1 – c.
Solution:

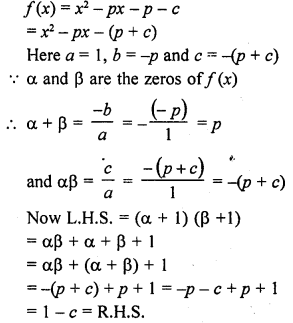
Question 16.
If α and β are the zeros of the quadratic polynomial such that α + β = 24 and α – β = 8, find a quadratic polynomial having α and β as its zeros.
Solution:

Question 17.
If α and β are the zeros of the quadratic polynomial f(x) = x² – 1, find a quadratic polynomial whose zeros are \(\frac { 2\alpha }{ \beta }\) and \(\frac { 2\beta }{ \alpha }\)
Solution:

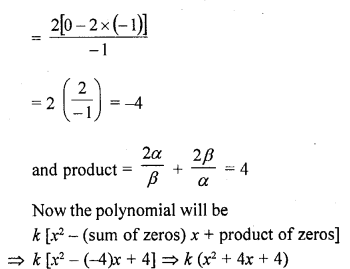
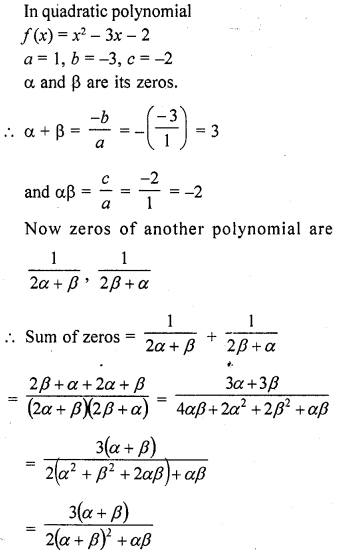
Question 18.
If α and β are the zeros of the quadratic polynomial f(x) = x² – 3x – 2, find a quadratic polynomial whose zeros are \(\frac { 1 }{ 2\alpha +\beta }\) and \(\frac { 1 }{ 2\beta +\alpha }\)
Solution:

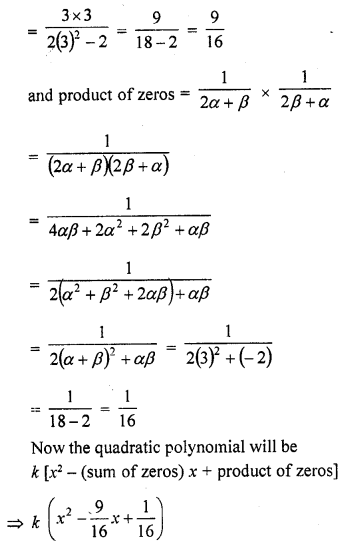
Question 19.
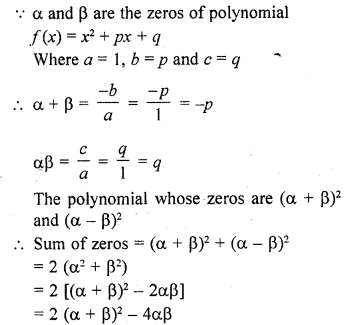
If α and β are the zeroes of the polynomial f(x) = x² + px + q, form a polynomial whose zeros are (α + β)² and (α – β)².
Solution:

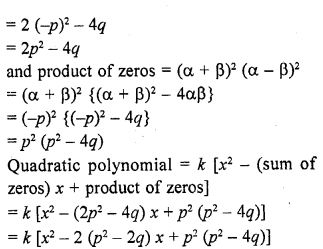
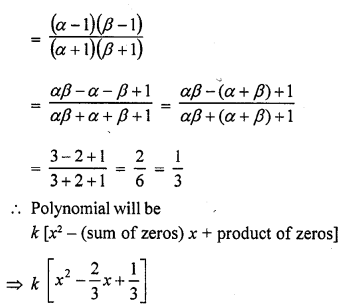
Question 20.
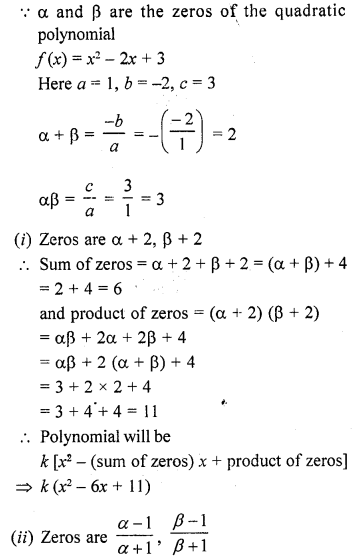
If α and β are the zeros of the quadratic polynomial f(x) = x² – 2x + 3, find a polynomial whose roots are :
(i) α + 2, β + 2
(ii) \(\frac { \alpha -1 }{ \alpha +1 } ,\frac { \beta -1 }{ \beta +1 }\)
Solution:

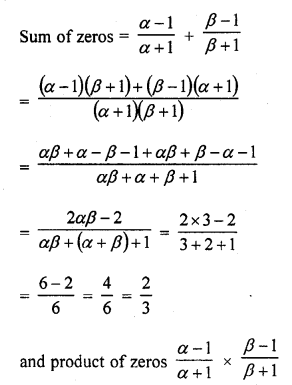
Question 21.
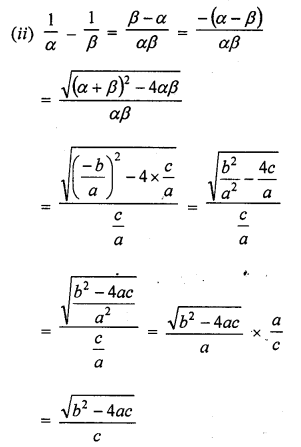
If α and β are the zeros of the quadratic polynomial f(x) = ax² + bx + c, then evaluate :


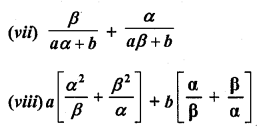
Solution:

 

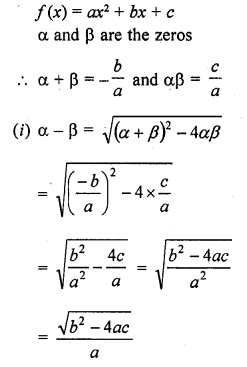
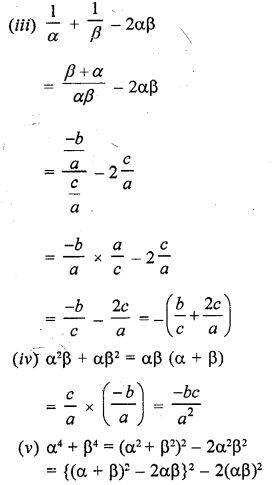
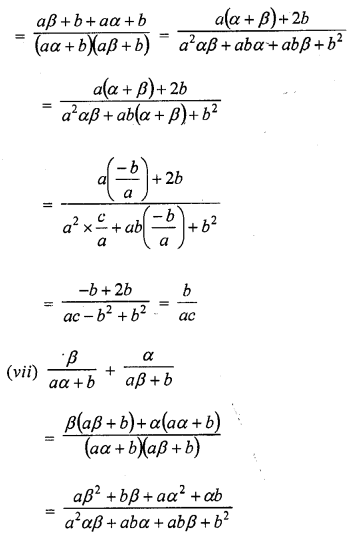
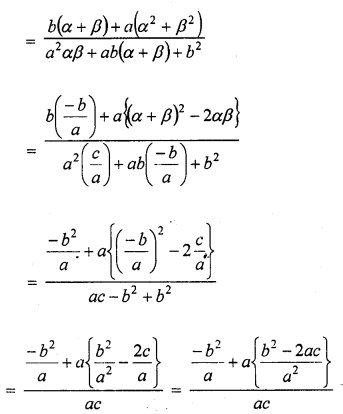
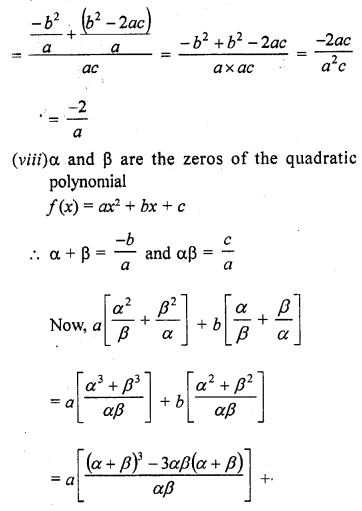
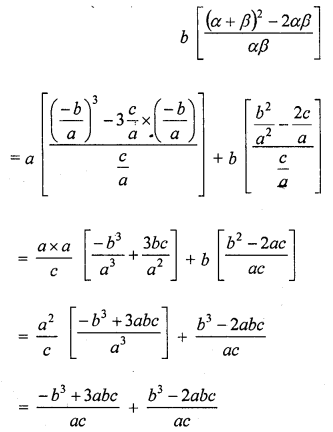
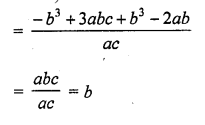
Hope given RD Sharma Class 10 Solutions Chapter 2 Polynomials Ex 2.1 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.