RS Aggarwal Class 9 Solutions Chapter 10 Area Ex 10A
These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Class 9 Solutions Chapter 10 Area Ex 10A.
Question 1.
Solution:
Given : In the figure, ABCD is a quadrilateral and
AB = CD = 5cm

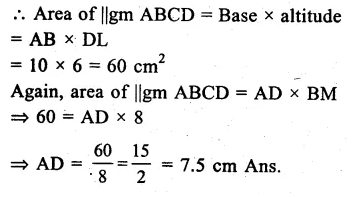
Question 2.
Solution:
In ||gm ABCD,
AB = 10cm, altitude DL = 6cm
and BM is altitude on AD, and BM = 8 cm.
Question 3.
Solution:
Diagonals of rhombus are 16cm and 24 cm.
Area = \(\frac { 1 }{ 2 } \) x product of diagonals
= \(\frac { 1 }{ 2 } \) x 1st diagonal x 2nd diagonal
= \(\frac { 1 }{ 2 } \) x 16 x 24
= 192 cm² Ans.
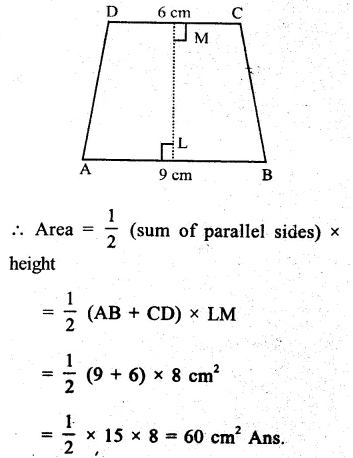
Question 4.
Solution:
Parallel sides of a trapezium are 9cm and 6cm and distance between them is 8cm
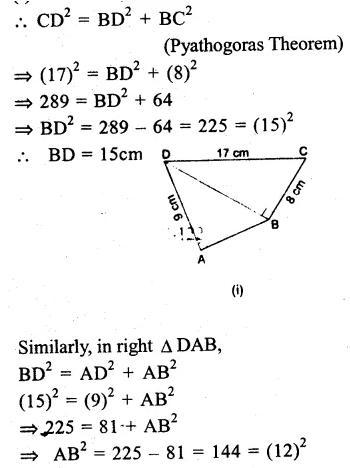
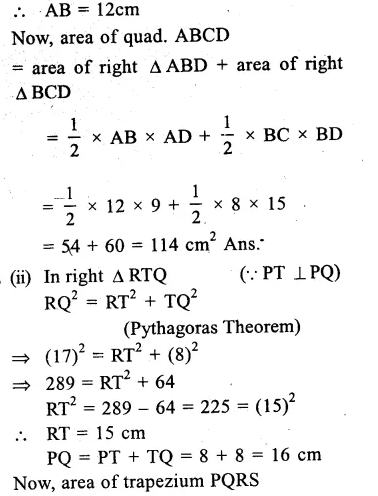
Question 5.
Solution:
from the figure
(i) In ∆ BCD, ∠ DBC = 90°

Question 6.
Solution:
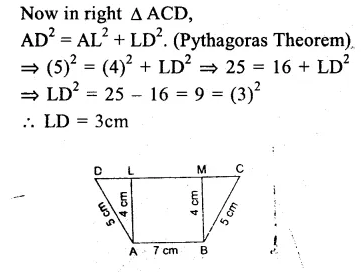
In the fig, ABCD is a trapezium. AB || DC
AB = 7cm, AD = BC = 5cm.
Distance between AB and DC = 4 cm.
i.e. ⊥AL = ⊥BM = 4cm.

Question 7.
Solution:
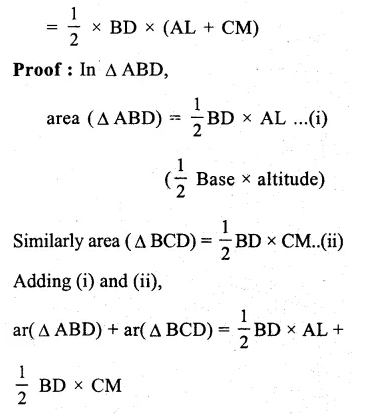
Given : In quad. ABCD. AL⊥BD and CM⊥BD.
To prove : ar(quad. ABCD)
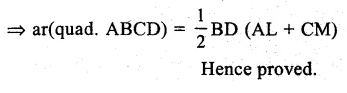
Question 8.
Solution:
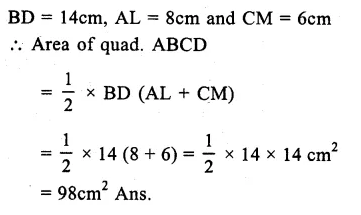
In quad. ABCD, BD is its diagonal and AL⊥BD, CM⊥BD
Question 9.
Solution:
Given : ABCD is a trapezium in which AB || DC and its diagonals AC and BD intersect each other at O.
To prove : ar(∆ AOD) = ar(∆ BOC)

Question 10.
Solution:
Given : In the figure,
DE || BC.
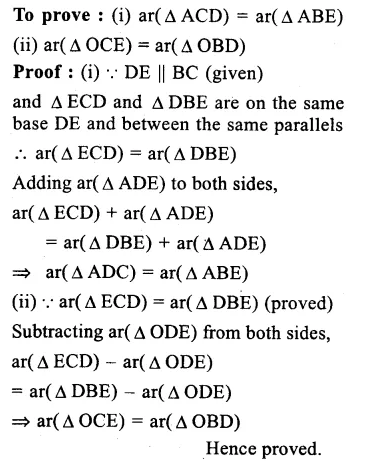
Question 11.
Solution:
Given : In ∆ ABC, D and E are the points on AB and AC such that
ar( ∆ BCE) = ar( ∆ BCD)
To prove : DE || BC.
Proof : (∆ BCE) = ar(∆ BCD)
But these are on the same base BC.
Their altitudes are equal.
Hence DE || BC
Hence proved.
Question 12.
Solution:
Given : In ||gm ABCD, O is any. point inside the ||gm. OA, OB, OC and OD are joined.
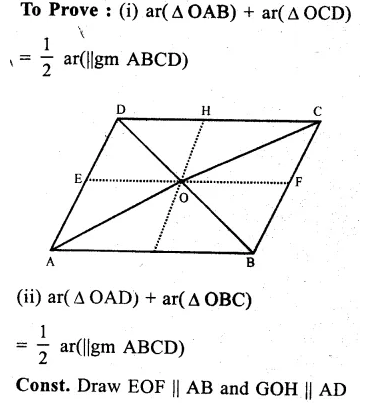
Question 13.
Solution:
Given : In quad. ABCD.
A line through D, parallel to AC, meets ‘BC produced in P. AP in joined which intersects CD at E.
To prove : ar( ∆ ABP) = ar(quad. ABCD).
Const. Join AC
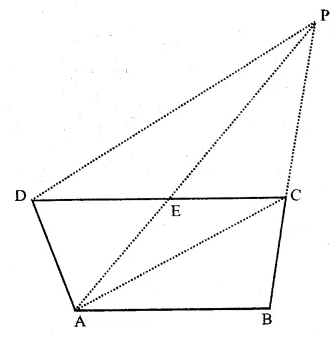
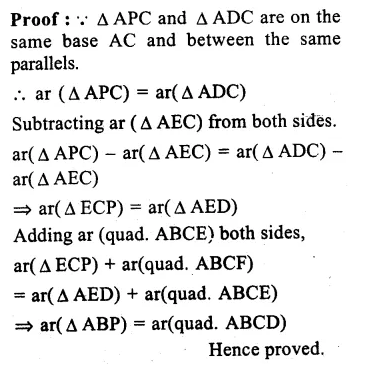
Question 14.
Solution:
Given : ∆ ABC and ∆ DBC are on the same base BC with points A and D on , opposite sides of BC and
ar( ∆ ABC) = ar( ∆ DBC).
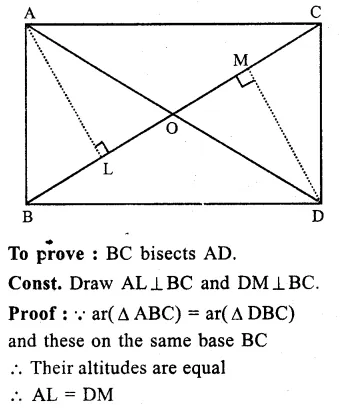
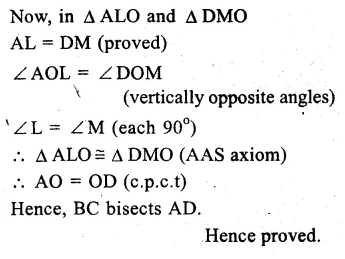
Question 15.
Solution:
Given : In ∆ ABC, AD is the median and P is a point on AD
BP and CP are joined
To prove : (i) ar(∆BDP) = ar(∆CDP)
(ii) ar( ∆ ABP) = ar( ∆ ACP)

Question 16.
Solution:
Given : In quad. ABCD, diagonals AC and BD intersect each other at O and BO = OD
To prove : ar(∆ ABC) = ar(∆ ADC)
Proof : In ∆ ABD,
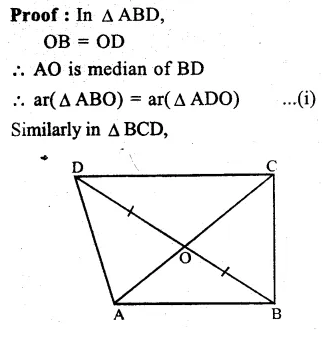
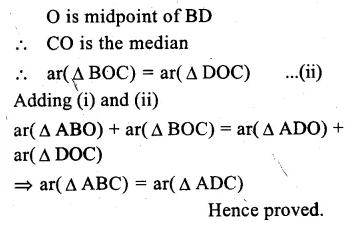
Question 17.
Solution:
In ∆ ABC,D is mid point of BC
and E is midpoint of AD and BE is joined.
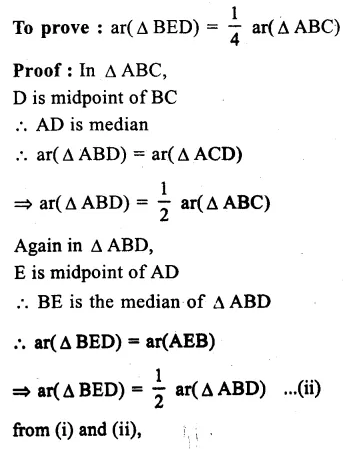
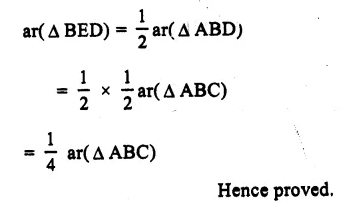
Question 18.
Solution:
Given : In ∆ ABC. D is a point on AB and AD is joined. E is mid point of AD EB and EC are joined.
To prove : ar( ∆ BEC) = \(\frac { 1 }{ 2 } \) ar( ∆ ABC)
Proof : In ∆ ABD,
E is midpoint of AD
BE is its median
ar(∆ EBD) = ar(∆ ABE)

Question 19.
Solution:
Given : In ∆ ABC, D is midpoint of BC and E is die midpoint of BO is the midpoint of AE.
To prove that ar( ∆ BOE) = \(\frac { 1 }{ 8 } \) ar(∆ ABC).
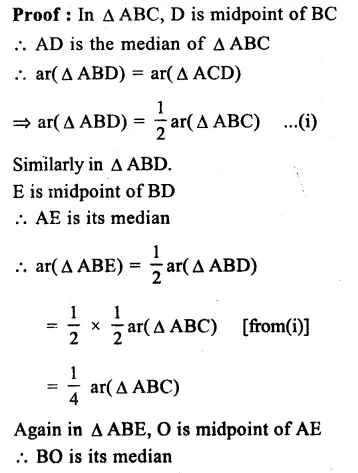
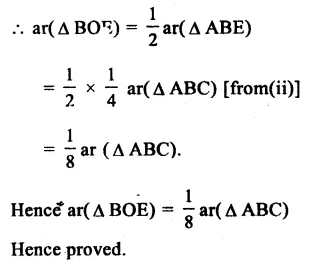
Question 20.
Solution:
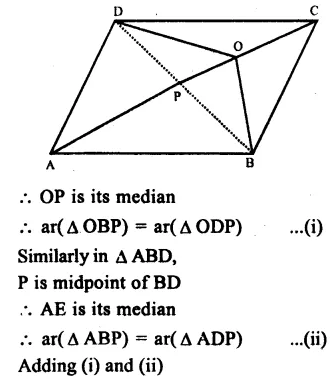
Given : In ||gm ABCD, O is any point on diagonal AC.
To prove : ar( ∆ AOB) = ar( ∆ AOD)
Const. Join BD which intersects AC at P
Proof : In ∆ OBD,
P is midpoint of BD
(Diagonals of ||gm bisect each other)
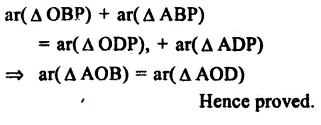
Question 21.
Solution:
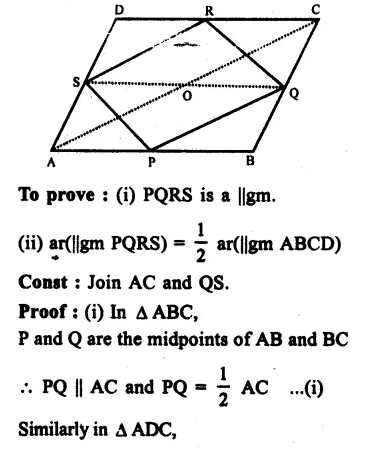
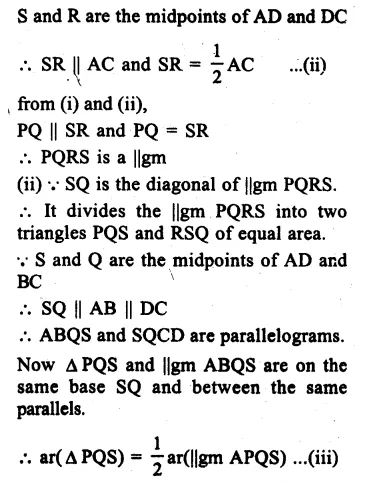
Given : ABCD is a ||gm.
P, Q, R and S are the midpoints of sides AB, BC, CD, DA respectively.
PQ, QR, RS and SP are joined.
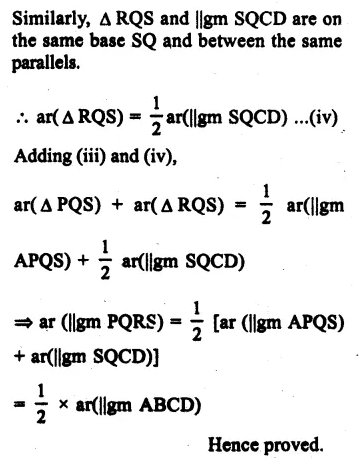
Question 22.
Solution:
Given : In pentagon ABCDE,
EG || DA meets BA produced and
CF || DB, meets AB produced.
To prove : ar(pentagon ABCDE) = ar(∆ DGF)
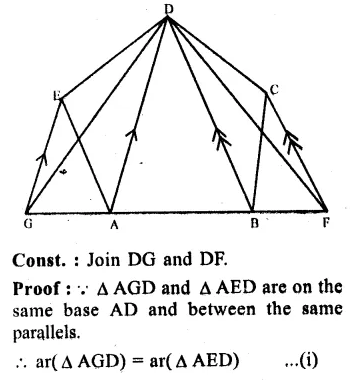
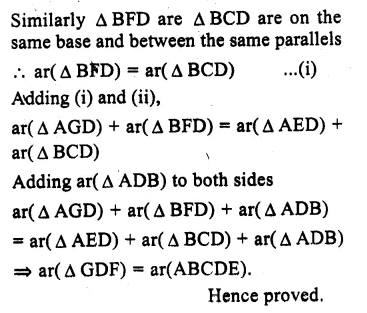
Question 23.
Solution:
Given ; A ∆ ABC in which AD is the median.
To prove ; ar( ∆ ABD) = ar( ∆ ACD)
Const : Draw AE⊥BC.
Proof : Area of ∆ ABD
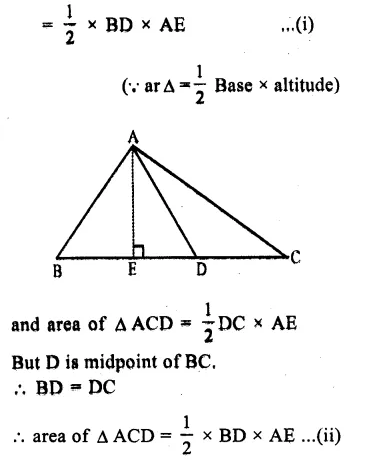

Question 24.
Solution:
Given : A ||gm ABCD in which AC is its diagonal which divides ||gm ABCD in two ∆ ABC and ∆ ADC.

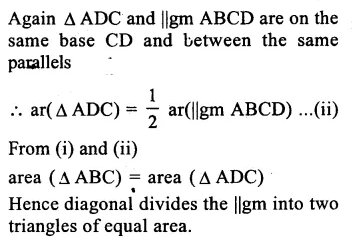
Question 25.
Solution:
Given : In ∆ ABC,
D is a point on BC such that
BD = \(\frac { 1 }{ 2 } \) DC
AD is joined.

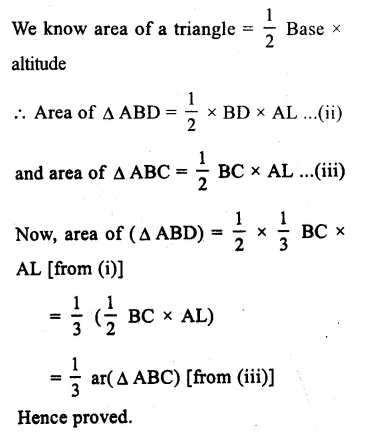
Question 26.
Solution:
Given : In ∆ ABC, D is a point on BC such that
BD : DC = m : n
AD is joined.
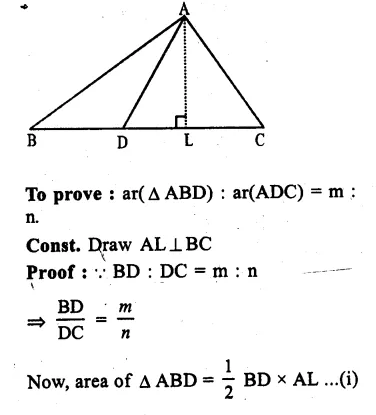
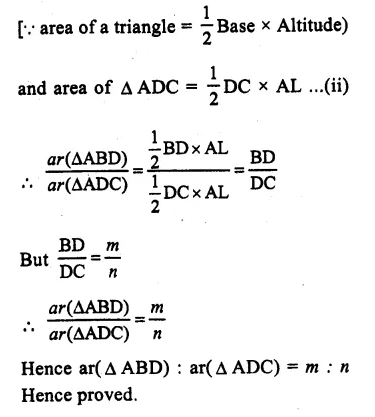
Hope given RS Aggarwal Class 9 Solutions Chapter 10 Area Ex 10A are helpful to complete your math homework.
If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.