ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 7 Ratio and Proportion Ex 7.3

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 7 Ratio and Proportion Ex 7.3

These Solutions are part of ML Aggarwal Class 10 Solutions for ICSE Maths. Here we have given ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 7 Ratio and Proportion Ex 7.3

More Exercises

• ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 7 Ratio and Proportion Ex 7.1
• ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 7 Ratio and Proportion Ex 7.2
• ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 7 Ratio and Proportion Ex 7.3
• ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 7 Ratio and Proportion MCQS
• ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 7 Ratio and Proportion Chapter Test

Question 1.
If a : b : : c : d, prove that
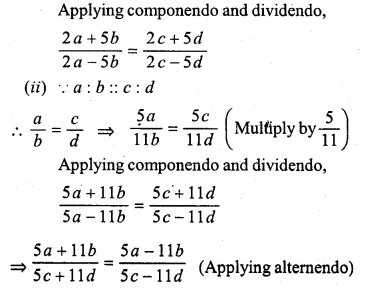
(i) \(\frac { 2a+5b }{ 2a-5b } =\frac { 2c+5d }{ 2c-5d } \)
(ii) \(\frac { 5a+11b }{ 5c+11d } =\frac { 5a-11b }{ 5c-11d } \)
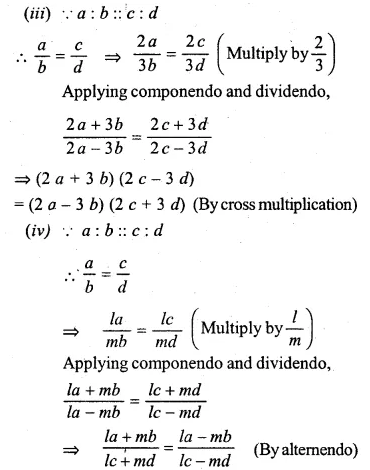
(iii) (2a + 3b)(2c – 3d) = (2a – 3b)(2c + 3d)
(iv) (la + mb) : (lc + mb) :: (la – mb) : (lc – mb)
Solution:
(i) a : b : : c : d
then \(\frac { a }{ b } =\frac { c }{ d } \)
⇒ \(\frac { 2a }{ 5b } =\frac { 2c }{ 5d } \) (multiply by \(\\ \frac { 2 }{ 5 } \) )

Question 2.
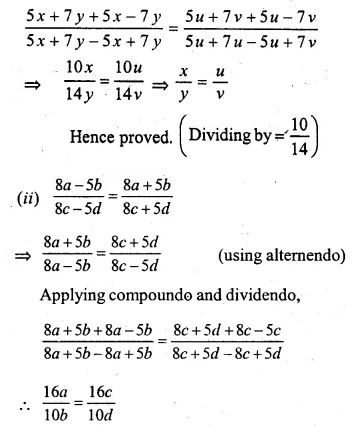
(i) If \(\frac { 5x+7y }{ 5u+7v } =\frac { 5x-7y }{ 5u-7v } \) , Show that \(\frac { x }{ y } =\frac { u }{ v } \)
(ii) \(\frac { 8a-5b }{ 8c-5d } =\frac { 8a+5b }{ 8c+5d } \) , prove that \(\frac { a }{ b } =\frac { c }{ d } \)
Solution:
(i) \(\frac { 5x+7y }{ 5u+7v } =\frac { 5x-7y }{ 5u-7v } \)
Applying alternendo \(\frac { 5x+7y }{ 5u+7v } =\frac { 5x-7y }{ 5u-7v } \)
Applying componendo and dividendo

Question 3.
If (4a + 5b) (4c – 5d) = (4a – 5d) (4c + 5d), prove that a, b, c, d are in proporton.
Solution:
(4a + 5b) (4c – 5d) = (4a – 5d) (4c + 5d)
⇒ \(\frac { 4a+5b }{ 4a-5b } =\frac { 4c+5d }{ 4c-5d } \)
Applying componendo and dividendo

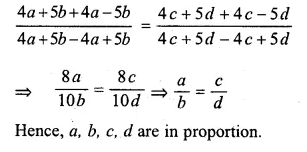
Question 4.
If (pa + qb) : (pc + qd) :: (pa – qb) : (pc – qd) prove that a : b : : c : d
Solution:
(pa + qb) : (pc + qd) :: (pa – qb) : (pc – qd)
⇒ \(\frac { pa+qb }{ pc+qd } =\frac { pq-qb }{ pc-qd } \)
⇒ \(\frac { pa+qb }{ pc-qd } =\frac { pq+qb }{ pc-qd } \)
Applying componendo and dividendo
⇒ \(\frac { pa+qb+pa-qb }{ pa+qb-pa+qb } =\frac { pc+qd+pc-qd }{ pc-qd-pc+qd } \)

Question 5.
If (ma + nb): b :: (mc + nd) : d, prove that a, b, c, d are in proportion.
Solution:
(ma + nb): b :: (mc + nd) : d
⇒ \(\frac { ma+nb }{ b } =\frac { mc+nd }{ d } \)
⇒ mad + nbd = mbc + nbd
⇒ mad = mbc
⇒ ad = bc
⇒ \(\frac { a }{ b } =\frac { c }{ d } \)
Hence a : b :: c : d.

Question 6.
If (11a² + 13b²) (11c² – 13d²) = (11a² – 13b²)(11c² + 13d²), prove that a : b :: c : d.
Solution:
(11a² + 13b²) (11c² – 13d²) = (11a² – 13b²)(11c² + 13d²)
⇒ \(\frac { 11a+{ 13b }^{ 2 } }{ { 11a }^{ 2 }-{ 13b }^{ 2 } } =\frac { { 11c }^{ 2 }+{ 13d }^{ 2 } }{ { 11c }^{ 2 }-{ 13d }^{ 2 } } \)
Applying componendo and dividendo

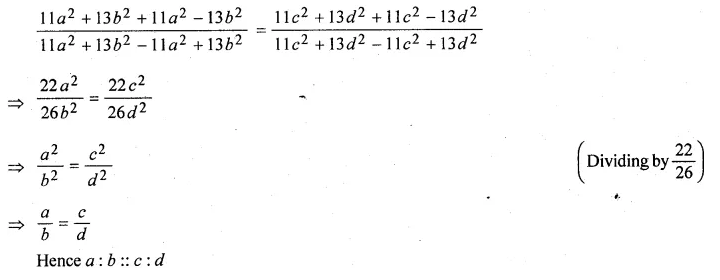
Question 7.
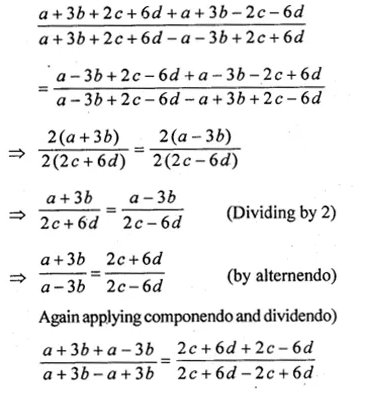
If (a + 3b + 2c + 6d) (a – 3b – 2c + 6d) = (a + 3b – 2c – 6d) (a – 3b + 2c – 6d), prove that a : b :: c : d.
Solution:
\(\frac { a + 3b + 2c + 6d }{ a – 3b + 2c – 6d } =\frac { a + 3b – 2c – 6d }{ a – 3b – 2c + 6d } \)
⇒ \(\frac { a + 3b + 2c + 6d }{ a + 3b – 2c – 6d } =\frac { a – 3b + 2c – 6d }{ a – 3b – 2c + 6d } \) (by altenendo)
Applying componendo and dividendo

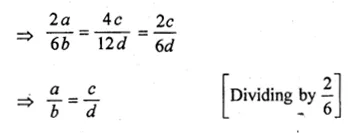
Question 8.
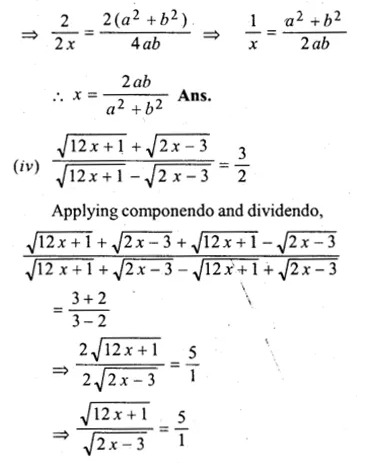
If \(x=\frac { 2ab }{ a+b } \) find the value of \(\frac { x+a }{ x-a } +\frac { x+b }{ x-b } \)
Solution:
\(x=\frac { 2ab }{ a+b } \)
⇒ \(\frac { x }{ a } =\frac { 2b }{ a+b } \)
Applying componendo and dividendo,

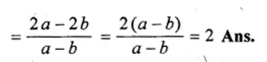
Question 9.
If \(x=\frac { 8ab }{ a+b } \) find the value of \(\frac { x+4a }{ x-4a } +\frac { x+4b }{ x-4b } \)
Solution:
\(x=\frac { 8ab }{ a+b } \)
⇒ \(\frac { x }{ 4a } =\frac { 2b }{ a+b } \)
Applying componendo and dividendo,

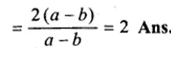
Question 10.
If \(x=\frac { 4\sqrt { 6 } }{ \sqrt { 2 } +\sqrt { 3 } } \) find the value of \(\frac { x+2\sqrt { 2 } }{ x-2\sqrt { 2 } } +\frac { x+2\sqrt { 3 } }{ x-2\sqrt { 3 } } \)
Solution:
\(x=\frac { 4\sqrt { 6 } }{ \sqrt { 2 } +\sqrt { 3 } } \)
⇒ \(\frac { 4\sqrt { 2 } \times \sqrt { 3 } }{ \sqrt { 2 } +\sqrt { 3 } } \)

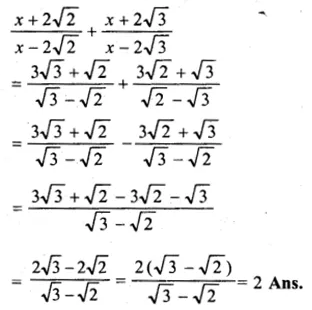
Question 11.
Solve \(x:\frac { \sqrt { 36x+1 } +6\sqrt { x } }{ \sqrt { 36x+1 } -6\sqrt { x } } =9 \)
Solution:
\(\frac { \sqrt { 36x+1 } +6\sqrt { x } }{ \sqrt { 36x+1 } -6\sqrt { x } } =\frac { 9 }{ 1 } \)
Applying componendo and dividendo,

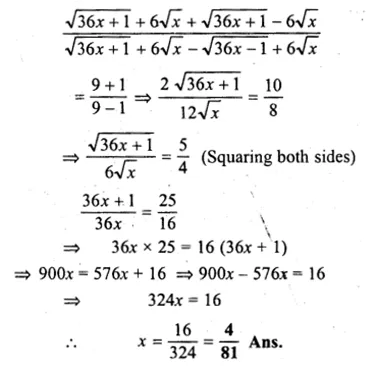
Question 12.
Find x from the following equations :
(i) \(\frac { \sqrt { 2-x } +\sqrt { 2+x } }{ \sqrt { 2-x } -\sqrt { 2+x } } =3 \)
(ii) \(\frac { \sqrt { x+4 } +\sqrt { x-10 } }{ \sqrt { x+4 } -\sqrt { x-10 } } =\frac { 5 }{ 2 } \)
(iii) \(\frac { \sqrt { 1+x } +\sqrt { 1-x } }{ \sqrt { 1+x } -\sqrt { 1-x } } =\frac { a }{ b } \)
(iv) \(\frac { \sqrt { 12x+1 } +\sqrt { 2x-3 } }{ \sqrt { 12x+1 } -\sqrt { 2x-3 } } =\frac { 3 }{ 2 } \)
(v) \(\frac { 3x+\sqrt { { 9x }^{ 2 }-5 } }{ 3x-\sqrt { { 9x }^{ 2 }-5 } } =5 \)
(vi) \(\frac { \sqrt { a+x } +\sqrt { a-x } }{ \sqrt { a+x } -\sqrt { a-x } } =\frac { c }{ d } \)
Solution:
(i) \(\frac { \sqrt { 2-x } +\sqrt { 2+x } }{ \sqrt { 2-x } -\sqrt { 2+x } } =3 \)
Applying componendo and dividendo,

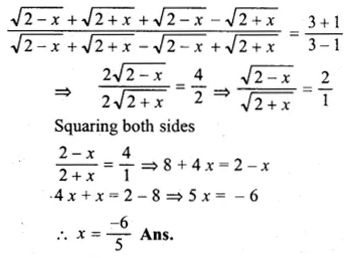
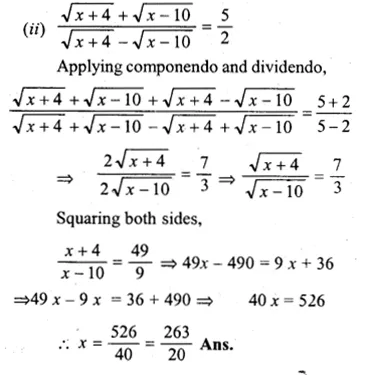
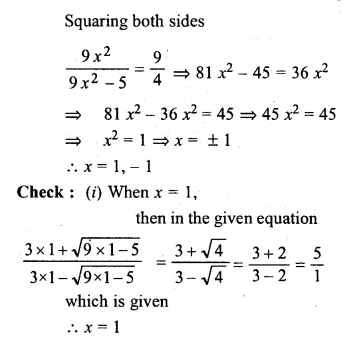
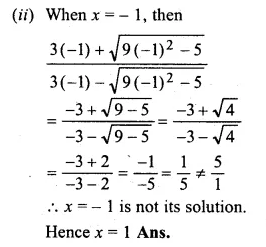
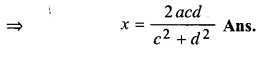
Question 13.
Solve \(\frac { 1+x+{ x }^{ 2 } }{ 1-x+{ x }^{ 2 } } =\frac { 62\left( 1+x \right) }{ 63\left( 1-x \right) } \)
Solution:
\(\frac { 1+x+{ x }^{ 2 } }{ 1-x+{ x }^{ 2 } } =\frac { 62\left( 1+x \right) }{ 63\left( 1-x \right) } \)
⇒ \(\frac { \left( 1-x \right) \left( 1+x+{ x }^{ 2 } \right) }{ \left( 1+x \right) \left( 1-x+{ x }^{ 2 } \right) } =\frac { 62 }{ 63 } \)
⇒ \(\frac { \left( 1+x \right) \left( 1-x+{ x }^{ 2 } \right) }{ \left( 1-x \right) \left( 1+x+{ x }^{ 2 } \right) } =\frac { 63 }{ 62 } \)

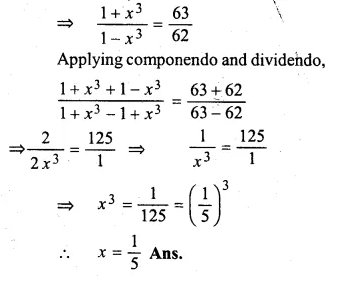
Question 14.
Solve for \(x:16{ \left( \frac { a-x }{ a+x } \right) }^{ 3 }=\frac { a+x }{ a-x } \)
Solution:
\(x:16{ \left( \frac { a-x }{ a+x } \right) }^{ 3 }=\frac { a+x }{ a-x } \)
⇒ \(\left( \frac { a+x }{ a-x } \right) \times { \left( \frac { a+x }{ a-x } \right) }^{ 3 }=16 \)

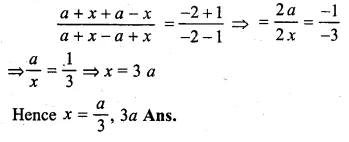
Question 15.
If \(x=\frac { \sqrt { a+x } +\sqrt { a-1 } }{ \sqrt { a+1 } -\sqrt { a-1 } } \) , using properties of proportion , show that x² – 2ax + 1 = 0
Solution:
We have \(x=\frac { \sqrt { a+x } +\sqrt { a-1 } }{ \sqrt { a+1 } -\sqrt { a-1 } } \)
⇒ \(\frac { x+1 }{ x-1 } =\frac { 2\sqrt { a+1 } }{ 2\sqrt { a-1 } } \)

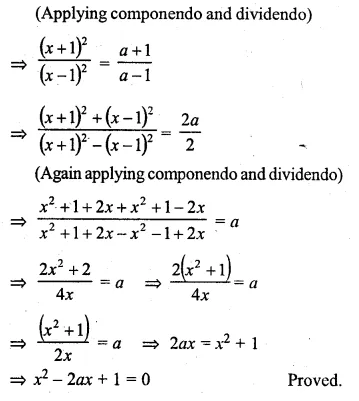
Question 16.
Given \(x=\frac { \sqrt { { a }^{ 2 }+{ b }^{ 2 } } +\sqrt { { a }^{ 2 }-{ b }^{ 2 } } }{ \sqrt { { a }^{ 2 }+{ b }^{ 2 } } -\sqrt { { a }^{ 2 }-{ b }^{ 2 } } } \) Use componendo and dividendo to prove that \({ b }^{ 2 }=\frac { { 2a }^{ 2 }x }{ { x }^{ 2 }+1 } \)
Solution:
If \(\frac { x }{ 1 } =\frac { \sqrt { { a }^{ 2 }+{ b }^{ 2 } } +\sqrt { { a }^{ 2 }-{ b }^{ 2 } } }{ \sqrt { { a }^{ 2 }+{ b }^{ 2 } } -\sqrt { { a }^{ 2 }-{ b }^{ 2 } } } \)
Applying componendo and dividendo both sides

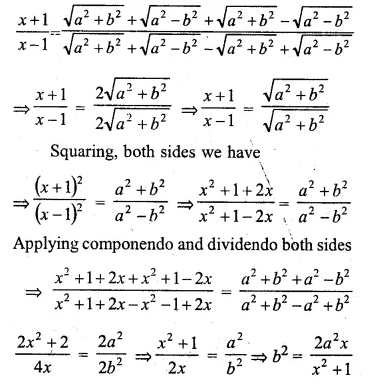
Question 17.
Given that \(\frac { { a }^{ 3 }+3{ ab }^{ 2 } }{ { b }^{ 3 }+{ 3a }^{ 2 }b } =\frac { 63 }{ 62 } \). Using componendo and dividendo find a: b. (2009)
Solution:
Given that \(\frac { { a }^{ 3 }+3{ ab }^{ 2 } }{ { b }^{ 3 }+{ 3a }^{ 2 }b } =\frac { 63 }{ 62 } \)
By componendo and dividendo

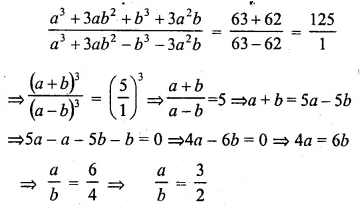
a : b = 3 : 2

Question 18.
Give \(\frac { { x }^{ 3 }+12x }{ { 6x }^{ 2 }+8 } =\frac { { y }^{ 3 }+27y }{ { 9y }^{ 2 }+27 } \) Using componendo and dividendo find x : y.
Solution:
Give \(\frac { { x }^{ 3 }+12x }{ { 6x }^{ 2 }+8 } =\frac { { y }^{ 3 }+27y }{ { 9y }^{ 2 }+27 } \)
Using componendo-dividendo, we have

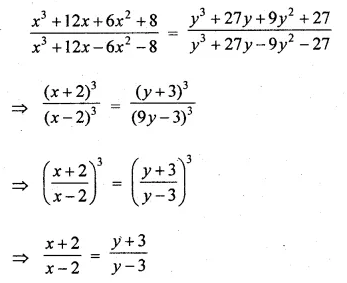
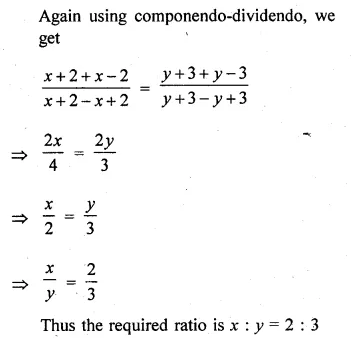
Question 19.
Using the properties of proportion, solve the following equation for x; given
\(\frac { x^{ 3 }+3x }{ { 3x }^{ 2 }+1 } =\frac { 341 }{ 91 } \)
Solution:
\(\frac { x^{ 3 }+3x }{ { 3x }^{ 2 }+1 } =\frac { 341 }{ 91 } \)
Applying componendo and dividendo

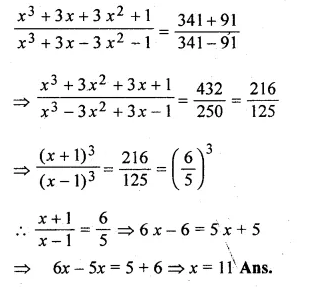
Question 20.
If \(\frac { x+y }{ ax+by } =\frac { y+z }{ ay+bz } =\frac { z+x }{ az+bx } \) , prove that each of these ratio is equal to \(\\ \frac { 2 }{ a+b } \) unless x + y + z = 0
Solution:
\(\frac { x+y }{ ax+by } =\frac { y+z }{ ay+bz } =\frac { z+x }{ az+bx } \)
= \(\frac { x+y+y+z+z+x }{ ax+by+ay+bz+az+bx } \)

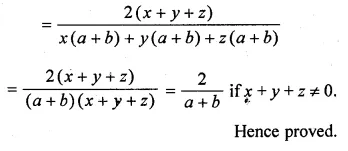
Hope given ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 7 Ratio and Proportion Ex 7.3 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.