Category: CBSE Class 6

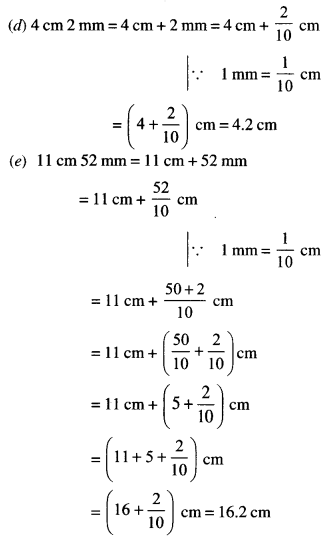
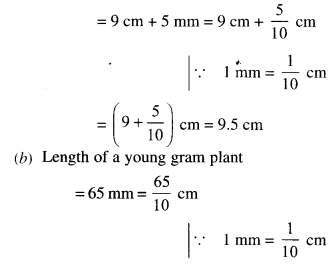
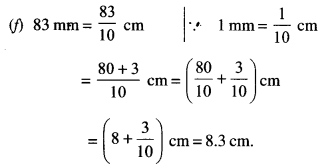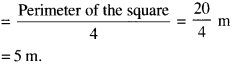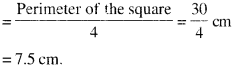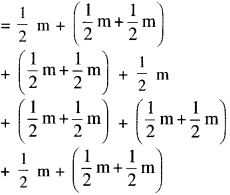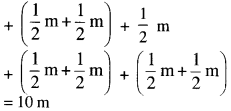NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.2 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.2.

• Whole Numbers Class 6 Ex 2.1
• Whole Numbers Class 6 Ex 2.3

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 2
Chapter Name	Whole Numbers
Exercise	Ex 2.2
Number of Questions Solved	7
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.2

Question 1.
Find the sum by suitable rearrangement :
(a) 837 + 208 + 363
(b) 1962 + 453 + 1538 + 647.
Solution :
(a) 837 + 208 + 363
= 837 + 363 + 208 = (837 + 363) + 208 = 1200 + 208 = 1408
(b) 1962+ 453+ 1538 + 647
= 1962 + 1538 + 453 + 647 = (1962 + 1538) + (453 + 647)
= 3500+ 1100 = 4600.

Question 2.
Find the product by a suitable rearrangement :
(a) 2 × 1768 × 50
(b) 4 × 166 × 25
(c) 8 × 291 × 125
(d) 625 × 279 × 16
(e) 285 × 5 × 60
(f) 125 × 40 × 8 × 25.
Solution :
(a) 2 × 1768 × 50
= 2 × 50 × 1768 = (2 × 50) × 1768 = 100 × 1768= 1,76,800
(b) 4 × 166 × 25 = 4 × 25 × 166 = (4 × 25) × 166 = 100 × 166 = 16,600
(c) 8 × 291 × 125 = 8 × 125 × 291
= (8 × 125) × 291 = 1000 × 291 =2,91,000
(d) 625 × 279 × 16 = 625 × 16 × 279
= (625 × 16) × 279 = 10000 ×279 = 27,90,000
(e) 285 × 5 × 60 = 285 × (5 × 60) = 285 × 300
= 85,500
(f) 125 × 40 × 8 × 25 = (125 × 40) × (8 × 25) = 5000 × 200 = 10,00,000.

Question 3.
Find the value of the following :
(a) 297 × 17+297 × 3
(b) 54279 × 92 + 8 × 54279
(c) 81265 × 169 – 81265 × 69
(d) 3845 × 5 × 782 + 769 × 25 × 218.
Solution :
(a) 297 × 17 + 297 × 3 = 297 × (17+ 3)
= 297 × 20 = 5940
(b) 54279 × 92 + 8 × 54279
= 54279 × 92 + 54279 × 8 = 54279 × (92 + 8)
= 54279 × 100 = 54,27,900
(c) 81265 × 169-81265 × 69
= 81265 × (169-69)
= 81265 × 100 = 81,26,500
(d) 3845 × 5 × 782 + 769 × 25 × 218
= 3845 × 5 × 782 + 769 × 5 × 5 × 218 = 3845 × 5 × 782 + (769 × 5) × 5 × 218 = 3845 × 5 × 782 + 3845 × 5 × 218 = 3845 × 5 × (782 + 218)
= 3845 × 5 × 1000 = 19225 × 1000 = 1,92,25,000.

Question 4.
Find the product, using suitable properties :
(a) 738 × 103
(b) 854 × 102
(c) 258 × 1008
(d) 1005 × 168.
Solution :
(a) 738 × 103
= 738 × (100+ 3)
= 738 × 100 + 738 × 3 = 73,800 + 2,214 = 76,014
(b) 854 × 102
= 854 × (100 + 2)
= 854 × 100 + 854 × 2 = 85,400+ 1,708 = 87,108
(c) 258 × 1008
= 258 × (1000+ 8)
= 258 × 1000 + 258 × 8 = 2,58,000 + 2,064 = 2,60,064
(d) 1005 × 168
= 168 × 1005
= 168 × (1000+ 5)
= 168 × 1000+ 168 × 5
= 1,68,000 + 840 = 1,68,840.

Question 5.
A taxi driver filled his car petrol tank with 40 liters of petrol on Monday. The next day he filled the tank with 50 liters of petrol. If the petrol costs ₹ 44 per liter, how much did he spend all on petrol?
Solution :
Petrol filled on Monday = 40 litres
Petrol filled the next day = 50 litres
∴ Total petrol filled on the two days = 40 litres + 50 litres = 90 litres
∴ Cost of petrol per litre = ₹ 44
∴ Cost of 90 litres of 7 petrol = ₹ 44 × 90 = ? 3960.

Question 6.
A vendor’supplies 32 liters of milk to a hotel in the morning and 68 liters of milk in the evening. If the milk costs ₹ 15 per liter, how much money is due to the vendor per day?
Solution :
Milk supplied in the morning = 32 liters
Milk supplied in the evening = 68 liters
∴ Milk supplied per day = 32 litres + 68 litres = 100 litres
Cost of milk per liter = ₹ 15
Money due to the vendor per day = Cost of
100 litres of milk = ₹ 15 × 100 = ₹ 1500.

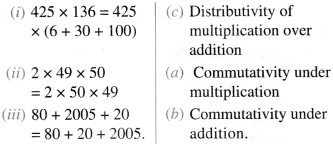
Question 7.
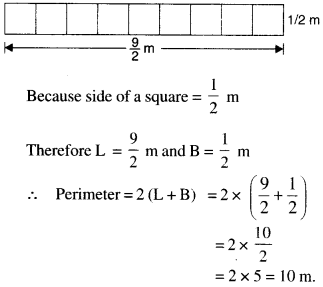
Match the following :

Solution :

We hope the NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.2 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.2, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.3 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.3.

• Knowing Our Numbers Class 6 Ex 1.1
• Knowing Our Numbers Class 6 Ex 1.2

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 1
Chapter Name	Knowing Our Numbers
Exercise	Ex 1.3
Number of Questions Solved	3
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.3

Question 1.
Estimate each of the following using general rule:
(a) 730 + 998
(b) 796 – 314
(c) 12,904 + 2,888
(d) 28,292 – 21,496
Make ten more such examples of addition, subtraction and estimation of their outcome.
Solution :
(a) 730 + 998 = 700 + 1000 = 1700
(b) 796 – 314 = 800 – 300 = 500
(c) 12,904 + 2,888 = 13,000 + 3,000 = 16,000
(d) 28,292 – 21,496 = 28,000 – 21,000 = 7,000
Ten more such examples of addition, subtraction and estimation of their outcome are as follows:
Ex. 1.720 + 990
Ex. 2.640 + 880
Ex. 3. 749 + 740
Ex. 4.890 – 420
Ex. 5.680 – 370
Ex. 6.585 – 220
Ex. 7.10803 + 3777
Ex. 8.15663 + 2125
Ex. 9. 30990 – 21660
Ex. 10. 40870-19530
Solution :
1. 720 + 990 = 700 + 1000 = 1700
2. 640 + 880 = 600 + 900 = 1500
3. 749 + 740 = 700 + 700 = 1400
4. 890 – 420 = 900 – 400 = 500
5. 680 – 370 = 700 – 400 = 300
6. 585 – 220 = 600 – 200 = 400
7. 10803 + 3777 = 11000 + 4000 = 15000
8. 15663 + 2125 = 16000 + 2000 = 18000
9. 30990 – 21660 = 31000 – 22000 = 9000
10. 40870 – 19530 = 41000 – 20000 = 21000

Question 2.
Give a rough estimate (by rounding off to nearest hundreds) and also a closer estimate (by rounding off to nearest tens):
(a) 439 + 334 4,317
(b) 1,08,734 – 47,599
(c) 8,325 – 491
(d) 4,89,348-48,365 Make four more such examples.
Solution :
(a)

• Rough estimate (Rounding off to nearest hundreds)
  439 + 334 + 4317
  = 400 + 300 + 4,300 = 5000
• Closer estimate (Rounding off to nearest tens) 439 + 334 + 4317
  = 440 + 330 + 4,320 = 5,090.

(b)

• Rough estimate (Rounding off to nearest hundreds) 1,08,734 – 47,599
  = 1,08,700 – 47,600 = 61,100
• Closer estimate (Rounding off to nearest tens) 1,08,734 – 47,599
  = 1,08,730 – 47,600 = 61,130.

(c)

• Rough estimate (Rounding off to nearest hundreds) 8325 – 491
  = 8300 – 500 = 7800
• Closer estimate (Rounding off to nearest tens)
  8325 – 491
  = 8330 – 490 = 7840.

(d)

• Rough estimate (Rounding off to nearest hundreds)
  4,89,348 – 48,365
  = 4,89,300 – 48,400 = 4,40,900
• Closer estimate (Rounding off to nearest tens)
  4,89,348 – 48,365
  = 4,89,350 – 48,370 = 4,40,980

Four more such examples are as follows:
1. 538 + 432 + 5326
2. 2,09, 849 – 57,698
3. 9426 – 395
4. 5,98,459 – 36,463 Sol.
solution :
1. 538 + 432 + 5326
= 500 + 400 + 5300
(Rough estimate Rounding off to nearest hundreds)
= 6200
538 + 432 + 5326
= 540 + 430 + 5330
(Closer estimate Rounding off to nearest tens) = 6300

2. 2,09,849 – 57.698
= 2.09.800 – 57,700
(Rough estimate Rounding off to nearest hun-dreds)
=152100 2,09, 849 – 57,698
= 2,09, 850 – 57,700
(Closer estimate Rounding off to nearest tens) =152150

3. 9426-395
= 9400 – 400 ..
(Rough estimate Rounding off to nearest hundreds)
= 9000 9426 – 395
= 9430 – 400
(Closer estimate Rounding off to nearest tens) = 9030

4. 5,98,459 – 36,463
= 5,98,500 – 36,500
(Rough estimate Rounding off to nearest hundreds)
= 5,62,000 5,98,459 – 36,463
= 5,98,460 – 36,460
(Closer estimate Rounding off to nearest tens) = 5,62,000

Question 3.
Estimate the following products using the general rule:
(a) 578 ×161
(b) 5281 × 3491
(c) 1291 × 592
(d) 9250 × 29
Make four more such examples.
Solution :
(a) 578 × 161
Estimated product = 600 × 200 = 1,20,000
(b) 5281 × 3491
Estimated product = 5000 × 3500 = 1,75,00,000
(c) 1291 × 592
Estimated product = 1300 × 600 = 7,80,000
(d) 9250 × 29
Estimated product = 10000 × 30 = 3,00,000

Four more such examples are:
1. 678 × 261
2. 4271 × 4391
3. 2391 × 629
4. 8250 × 39

We hope the NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.3 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.3, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.2 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.2.

• Knowing Our Numbers Class 6 Ex 1.1
• Knowing Our Numbers Class 6 Ex 1.3

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 1
Chapter Name	Knowing Our Numbers
Exercise	Ex 1.2
Number of Questions Solved	12
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.2

Question 1.
A book exhibition was held for four days in a school. The number of tickets sold at the counter on the first, second, third and final day was respectively 1094, 1812, 2050 and 2751. Find the total number of tickets sold on all four days.
Solution :
Number of tickets sold on the first day = 1094
Number of tickets sold on the second day =1812
Number of tickets sold on the third day = 2050
Number of tickets sold on the final day = 2751
Total number of tickets sold on all the four days = 1094 + 1812 + 2050 + 2751 = 7707.

Question 2.
Shekhar is a famous cricket player. He has so far scored 6980 runs in test matches. He wishes to complete 10000 runs. How many more runs does he need?
Solution :
Runs scored so far = 6980
Runs wished to be scored = 10000
Runs needed more = 10000 – 6980 = 3020.

Question 3.
In an election, the successful candidate registered 5,77,500 votes and his nearest rival secured 3,48,700 votes. By what margin did the successful candidate win the election?
Solution :
Votes registered by the successful candidate = 5,77,500
Votes secured by the nearest rival = 3,48.700
Margin by which the successful candidate won the election = 5,77,500 – 3,48,700 = 2,28,800.

Question 4.
Kirti bookstore sold books worth? 2,85,891 in the first week of June and books worth ?. 4,00,768 in the second week of the month. How much was the sale for the two weeks together? In which week was the sale greater and by how much?
Solution :
Sale of books in the first week = ₹ 2,85,891
Sale of books in the second week = ₹ 4,00,768
∴ Sale for the two weeks together = ₹ 2,85,891 + ₹ 4,00,768 = ₹ 6,86,659.
The sale was greater in the second week by ₹ 4,00,768 – ₹ 2,85,891 i.e., by ₹ 1,14,877.

Question 5.
Find the difference between the greatest and least numbers that can be written using the digits 6, 2, 7, 4, 3 each only once.
Sol.
Greatest number that can be written using the digits 6, 2, 7, 4, 3 each only once = 76,432
Least number that can be written using the digits 6, 2, 7, 4, 3 each only once = 23,467
∴ Difference between the greatest and least numbers that can be written using the digits 6,2,7,4, 3 each only once = 76,432 – 23,467 = 52,965.

Question 6.
A machine, on an average, manufactures 2,825 screws a day. How many screws did it produce in the month of January 2006?
Solution :
The number of screws manufactured by the machine a day on an average = 2,825.
Number of days in the month of January 2006 = 31
The number of screws produced by the machine in the month of January 2006 = 2,825 × 31 =87,575.

Question 7.
A merchant had ₹ 78,592 with her. She placed an order for purchasing 40 radio sets at ? 1200 each. How much money will remain with her after the purchase?
Sol.
Money which the merchant had = ₹ 78,592
Cost of 40 radio sets at ? 1200 each = ₹ 1200 × 40 = ₹ 48,000
Money that will remain with the merchant after the purchase = ₹ 78,592 – ₹ 48,000 = ₹ 30,592.

Question 8.
A student multiplied 7236 by 65 instead of multiplying by 56. By how much was his answer greater than the. correct answer?
[Hint: Do you need to do both the multiplications?]
Solution :
The wrong answer was greater than the correct answer by
= 7236 × 65 – 7236 × 56
= 7236 × (65 – 56)
= 7236 × 9 = 65.124

Question 9.
To stitch a shirt, 2 m 15 cm cloth is needed. Out of 40 m cloth, how many shirts can be stitched and how much cloth will remain ?
[Hint: convert data in cm.]
Solution :
2 m 15 cm = 2 m + 15 cm = 2 × 100 cm + 15 cm = 200 cm + 15 cm = 215 cm
40 m = 40 × 100 cm = 4000 cm

Hence, 18 shirts can be stitched and 130 cm,
i. e., 1 m 30 cm cloth will remain.

Question 10.
Medicine is packed in boxes, each weighing 4 kg 500 g. Mow many such boxes can be loaded in a van which cannot carry beyond 800 kg?
Solution :
4 kg 500 g = 4 kg + 500 g
= 4 × 1000 g + 500 g
= 4000 g + 500 g
= 4500 g 800 kg
= 800 × 1000 g = 800000 g

Hence, 177 such boxes can be loaded.

Question 11.
The distance between the school and the house of a student’s house is 1 km 875 m. Every day she walks both ways. Find the total distance covered by her in six days.
Solution :
1 km 875 m = 1 km + 875 m
= 1 × 1000 m + 875 m
= 1000 m + 875 m = 1875 m
Distance covered by her in a day in walking both ways between school and home = 1875 × 2 m = 3750 m
∴ Total distance covered by her in six days in walking both ways between school and home = 3750 m × 6 = 22500 m
= 22000 m + 500 m = \(\frac { 22000 }{ 1000 }\) km + 500 m
= 22 km + 500 m = 22 km 500 m.

Question 12.
A vessel has 4 liters and 500 ml of curd. In how many glasses, each of 25 ml capacity, can it be filled?
Solution :
41500 ml = 41 + 500 ml
= 4 × 1000 ml + 500 ml
= 4000 ml + 500 ml = 4500 ml

Hence, it can be filled in 180 glasses.

We hope the NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.2 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.2, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 14 Practical Geometry Ex 14.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 14 Practical Geometry Ex 14.1.

• Practical Geometry Class 6 Ex 14.2
• Practical Geometry Class 6 Ex 14.3
• Practical Geometry Class 6 Ex 14.4
• Practical Geometry Class 6 Ex 14.5
• Practical Geometry Class 6 Ex 14.6

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 14
Chapter Name	Practical Geometry
Exercise	Ex 14.1
Number of Questions Solved	5
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 14 Practical Geometry Ex 14.1

Question 1.
Draw a circle of radius 3.2 cm.
Solution :

Steps of Construction

• Open the compasses for the required radius 3.2 cm, by putting the pointer on 0 and opening the pencil upto 3.2 cm.
• Draw a point with a sharp pencil and marks it as O in the centre.
• Place the pointer of the compasses where the centre has been marked.
• Turn the compasses slowly to draw the circle.

Question 2.
With the same centre O, draw two circles of radii 4 cm and 2.5 cm.
Solution :
Steps of Construction
1. For circle of radius 4 cm

• Open the compasses for the required radius 4 cm, by putting the pointer on 0 and opening the pencil upto 4 cm.
• Place the pointer of the compasses at O.
• Turn the compasses slowly to draw the circle.

2. For circle of radius 2.5 cm

• Open the compasses for the required radius 2.5 cm. by putting the pointer on 0 and opening the pencil upto 2.5 cm.
• Place the pointer of the compasses at O.
• Turn the compasses slowly to draw the circle.

Question 3.
Draw a circle and any two of its diameters. If you join the ends of these diameters, what is the figure obtained? What figure is obtained if the diameters are perpendicular to each other? How do you check your answer?
Solution :
(i) On joining the ends of any two diameters of the circle, the figure obtained is a rectangle.

(ii) On joining the ends of any two diameters of the circle, perpendicular to each other, the figure obtained is a square.
To check the answer, we measured the sides and angles of the figure obtained.

Question 4.
Draw any circle and mark points A, B and C such that:
(a) A is on the circle.
(b) B is in the interior of the circle.
(c) C is in the exterior of the circle.
Solution :

Question 5.
Let A, B be the centres of two circles of equal radii; draw them so that each one of them passes We need a ruler here. through the centre of the other. Let them intersect at C and D. Examine whether \(\overline { AB }\) and \(\overline { CD }\) are at right angles.
Solution :

Yes! \(\overline { AB }\) and \(\overline { CD }\) are at right angles.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 14 Practical Geometry Ex 14.1 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 14 Practical Geometry Ex 14.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 7 Fractions Ex 7.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 7 Fractions Ex 7.1.

• Fractions Class 6 Ex 7.2
• Fractions Class 6 Ex 7.3
• Fractions Class 6 Ex 7.4
• Fractions Class 6 Ex 7.5
• Fractions Class 6 Ex 7.6

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 7
Chapter Name	Fractions
Exercise	Ex 7.1
Number of Questions Solved	11
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 7 Fractions Ex 7.1

Question 1.
Write the fraction representing the shaded portion.



Solution :

Question 2.
Color the part according to the given fraction.

Solution :

Question 3.
Identify the error, if any

Solution :
Neither of the shaded portions represents the corresponding given fractions. 4.

Question 4.
What fraction of a day is 8 hours?
Solution :
1 day = 24 hours
∴ Required fraction = \(\frac { 8 }{ 24 }\)

Question 5.
What fraction of an hour is 40 minutes?
Solution :
1 hour = 60 minutes
∴ Required fraction = \(\frac { 40 }{ 60 }\)

Question 6.
Arya, Abhimanyu, and Vivek shared lunch. Arya brings two sandwiches, one made of vegetable and one of jam. The other two boys forgot to bring their lunch. Arya agreed to share his sandwiches so that each person will have an equal share of each sandwich.
(a) How can Arya divide his sandwiches so that each person has an equal share?
(b) What part of a sandwich will each boy receive?
Solution :
(a) Arya will divide each sandwich into three equal parts, and give one part of each sandwich to each one of them.
(b) Each boy will receive \(\frac { 1 }{ 3 }\) part of a sandwich.

Question 7.
Kanchan dyes dresses. She had to dye 30 dresses. She has so far finished 20 dresses. What fraction of dresses has she finished?
Solution :
She has finished \(\frac { 2 }{ 3 }\) fraction of the dresses.

Question 8.
Write the natural numbers from 2 to 12. What fraction of them are prime numbers?
Solution :
The natural numbers from 2 to 12 are 2, 3, 4,5,6, 7, 8, 9, 10, 11 and 12
Total number of natural numbers = 11
Out of these, the prime numbers are 2, 3, 5, 7, 11

Total number of these prime numbers = 5 5
∴ Required fraction = \(\frac { 5 }{ 11 }\).

Question 9.
Write the natural numbers from 102 to 113. What fraction of them are prime numbers?
Solution :
The natural numbers from 102 to 113 are
102, 103, 104, 105, 106, 107, 108, 109, 110, 111, 112 and 113
Total number of natural numbers =12
Out of these, the prime numbers are 103, 107, 109, 113.
Total number of these prime numbers = 4 . 4
∴ Required fraction = \(\frac { 4 }{ 12 }\).

Question 10.
What fraction of these circles have X’s in them?

Solution :
Total number of circles = 8
Number of circles which have X’s in them = 4
∴ Required fraction = \(\frac { 4 }{ 8 }\).

Question 11.
Kristin received a CD player for her birthday. She bought 3 CDs and received 5 others as gifts. What fraction of her total CDs did she buy and what fraction did she receive as gifts?
Solution :
Number of CDs bought = 3
Number of CDs received as gifts = 5
∴ Total number of CDs = 3 + 5 = 8
Fraction of her total CDs that she bought \(\frac { 3 }{ 8 }\) and, fraction of her total CDs that she received as gifts \(\frac { 5 }{ 8 }\).

 

We hope the NCERT Solutions for Class 6 Maths Chapter 7 Fractions Ex 7.1 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 7 Fractions Ex 7.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 8 Decimals Ex 8.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 8 Decimals Ex 8.1.

• Decimals Class 6 Ex 8.2
• Decimals Class 6 Ex 8.3
• Decimals Class 6 Ex 8.4
• Decimals Class 6 Ex 8.5
• Decimals Class 6 Ex 8.6

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 8
Chapter Name	Decimals
Exercise	Ex 8.1
Number of Questions Solved	10
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 8 Decimals Ex 8.1

Question 1.
Write the following as numbers in the given table:
(a)

(b)

Hundreds (100)	Tens (10)	Ones (1)	Tenths (\(\frac { 1 }{ 10 } \))

Solution.

	Hundreds (100)	Tens (10)	Ones (1)	Tenths (\(\frac { 1 }{ 10 } \))
(a)	0	3	1	2
(b)	1	1	0	4

Question 2.
Write the following decimals in the place value table :
(a) 19.4
(b) 0.3
(c) 10.6
(d) 205.9
Solution.

	Hundreds	Tens	Ones	Tenths
(a)	0	1	9	4
(b)	0	0	0	3
(c)	0	1	0	6
(d)	2	0	5	9

Question 3.
Write each of the following as decimals:
(a) Seven-tenths
(b) Two tens and nine-tenths
(c) Fourteen point six
(d) One hundred and two ones
(e) Six hundred point eight
Solution.
(a) 7 tenths : \(\frac { 7 }{ 10 } \) =0.7.
(b) Two tens and nine-tenths = 20 + \(\frac { 9 }{ 10 } \)  =20.9
(c) Fourteen point six = 14.6
(d) One hundred and two-ones =100 + 2=102
(e) Six hundred point eight = 600.8

Question 4.
Write each of the following as decimals:
(a) \(\frac { 5 }{ 10 } \)
(b) 3 +\(\frac { 7 }{ 10 } \)
(c) 200+60+5+\(\frac { 1 }{ 10 } \)
(d) 70+\(\frac { 8 }{ 10 } \)
(e) 4 \(\frac { 2 }{ 10 } \)
(g) \(\frac { 3 }{ 2 } \)
(h) \(\frac { 2 }{ 5 } \)
(i) \(\frac { 12 }{ 5 } \)
(j) 3 \(\frac { 3 }{ 5 } \)
(k) 4\(\frac { 1 }{ 2 } \)
Solution.

Question 5.
Write the following decimals as fractions. Reduce the fractions to lowest form :
(a) 0.6
(b) 205
(c) 1.0
(d) 3.8
(e) 13.7
(f) 21.2
(g) 6.4
Solution.
(a)
 \(0.6=\frac { 6 }{ 10 } =\frac { 6\div 2 }{ 10\div 5 } \)
∵  H.C.F.(6,10) = 2
= \(\frac { 3 }{ 5 } \)

(b)
\(2.5=\frac { 25 }{ 10 } =\frac { 25\div 5 }{ 10\div 5 } \)
∵  H.C.F.(25,10) = 5
= \(\frac { 5 }{ 2 } \)

(c)
\(1.5=\frac { 10 }{ 10 } =\frac { 10\div 10 }{ 10\div 10 } \)
∵  H.C.F.(10,10) = 10
= \(\frac { 1 }{ 1 } \) =1

(d)
\(3.8 =\frac { 38 }{ 10 } =\frac { 38\div 2 }{ 10\div 2 } \)
∵  H.C.F.(38,10) = 2
= \(\frac { 19 }{ 5 } \)

(e)
\(13.7=\frac { 137 }{ 10 } \)

(f)
\( 21.2=\frac { 212 }{ 10 } =\frac { 212\div 2 }{ 10\div 5 } \)
∵  H.C.F.(212,10) = 5
= \(\frac { 106 }{ 5 } \)

(g)
\(6.4=\frac { 64 }{ 10 } =\frac { 64\div 2 }{ 10\div 5 } \)
∵  H.C.F.(64,10) = 2
= \(\frac { 32 }{ 5 } \)

Question 6.
Express the following as cm using decimals:
(a) 2 mm
(b) 30 mm
(c) 116 mm
(d) 4cm 2mm
(e) 11cm 52mm
(f) 83 mm
Solution.
(a)
\(2mm =\frac { 2 }{ 10 } \)
∵  \( 1mm =\frac { 1 }{ 10 } \)
= 0.2cm

(b)
\(30mm =\frac { 30 }{ 10 } \)
∵  \( 1mm =\frac { 1 }{ 10 } \)
= 3cm = 3.0cm

Question 7.
Between which two whole numbers on the number line are the given numbers lie? Which of these whole numbers is nearer the number?
(a) 0.8
(b) 5.1
(c) 2.6
(d) 6.4
(e) 9.0
(f) 4.9

Solution.
(a) 0.8. The number 0.8 is between the two whole numbers 0 and 1. The whole number 1 is nearer the number 0.8.

(b) 5.1. The number 5.1 is between the two whole numbers 5 and 6. The whole number 5 is nearer the number 5.1.

(c) 2.6. The number 2,6 is between the two whole numbers 2 and 3. The whole number 3 is nearer the number 2.6.

(d) 6.4 The number 6.4 is between the two whole numbers 6 and 7. The whole number 6 is nearer the number 6.4.

(e) 0. 9.0 itself is a whole number.

(f) 4.9. The number 4.9 is between the two whole numbers 4 and 5. The whole number 5 is nearer the number 4.9.

Question 8.
Show the following numbers on the number line:
(a) 2
(b) 1.9
(c) 1
(d) 2.5.
Solution.
(a) 0.2. We know that 0.2 is more than zero but less than one. There are 2-tenths in it. Divide the unit length between 0 and 1 into 10 equal parts and take 2 parts as shown below:

(b) 1.9. We know that 1.9 is more than one but less than two. There are 9-tenths in’it. Divide the unit length between 1 and 2 into 10 equal parts and take 9 parts as shown below:

(c) 1.1. We know that 1.1 is more than one but less than two. There is one-tenth in it. Divide the unit length between 1 and 2 into 10 equal parts and take 1 part as shown below:

(d) 2.5. We know that 2.5 is more than two but less than three. There are 5-tenths in it. Divide the unit length between 2 and 3 into 10 equal parts and take 5 parts as shown below :

Question 9.
Write the decimal number represented by the points A, B, C, D on the given number line :

Solution.
(i) A. The decimal number represented by the point A is 0.8 as the unit length between 0 and 1 has been divided into 10 equal parts and 8 parts have been taken.

(ii) B. The decimal number represented by the point B is 1.3 as the unit length between 1 and 2 has been divided into 10 equal parts and 3 parts have been taken.

(iii) C. The decimal number represented by the point C is 2.2 as the unit length between 2 and 3 has been divided into 10 equal parts and 2 parts have been taken.

(iv) D. The decimal number represented by the point D is 2.9 as the unit length between 2 and 3 has been divided into 10 equal parts and 9 parts have been taken.

Question 10.
(a) The length of Ramesh’s notebook is 9 cm 5 mm. What will be its length in cm?
(b) The length of a young gram plant is 65 mm. Express its length in cm.
Solution.
(a) Length of Ramesh’s notebook = 9 cm 5mm

 

We hope the NCERT Solutions for Class 6 Maths Chapter 8 Decimals Ex 8.1 help you. If you have any query regarding. NCERT Solutions for Class 6 Maths Chapter 8 Decimals Ex 8.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 13 Symmetry Ex 13.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 13 Symmetry Ex 13.1.

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 13
Chapter Name	Symmetry
Exercise	Ex 13.1
Number of Questions Solved	6
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 13 Symmetry Ex 13.1

Question 1.
List any four symmetrical objects from your home or school.
Solution :
The blackboard, the table top, a pair of scissors, the computer disc.

Question 2.
For the given figure, which one is the mirror line, l₁ or l₂?

Solution :
I₂ is the mirror line.

Question 3.
Identify the shapes given below. Check whether they are symmetric or not. Draw the line of symmetry as well.

Solution :
(a) Symmetric

(b) Symmetric

(c) Not symmetric
(d) Symmetric

(e) Symmetric

(f) Symmetric

Question 4.
Copy the following on a squared paper. A square paper is what you would have used in your arithmetic notebook in earlier classes. Then complete them such that the dotted line is the line of symmetry.



Solution :

Question 5.
In the figure, l is the line of symmetry. Complete the diagram to make it symmetric.

Solution :

Question 6.
In the figure, l is the line of symmetry. Draw the image of the triangle and complete the diagram so that it becomes symmetric.

Solution :

 

We hope the NCERT Solutions for Class 6 Maths Chapter 13 Symmetry Ex 13.1 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 13 Symmetry Ex 13.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes Ex 5.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes Ex 5.1.

• Understanding Elementary Shapes Class 6 Ex 5.2
• Understanding Elementary Shapes Class 6 Ex 5.3
• Understanding Elementary Shapes Class 6 Ex 5.4
• Understanding Elementary Shapes Class 6 Ex 5.5
• Understanding Elementary Shapes Class 6 Ex 5.6
• Understanding Elementary Shapes Class 6 Ex 5.7
• Understanding Elementary Shapes Class 6 Ex 5.8
• Understanding Elementary Shapes Class 6 Ex 5.9

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 5
Chapter Name	Understanding Elementary Shapes
Exercise	Ex 5.1
Number of Questions Solved	7
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes Ex 5.1

Question 1.
What is the disadvantage in comparing line segments by mere observation?
Solution :
Sometimes the difference in lengths betweenthe two line segments is not obvious. So, we are not always sure about our usual judgment.

Question 2.
Why is it better to use a divider than with a ruler, while measuring the length of a line segment?
Solution :
There may be errors due to the thickness of the ruler and angular viewing by using a ruler. These errors are eradicated by using a divider. So, it is better to use a divider, than a ruler, while measuring the length of a line segment.

Question 3.
Draw any line segment, say \(\overline { AB }\). Take any point C lying in between A and B. Measure the lengths of AB, BC and AC. Is AB = AC + CB? [Note : If A, B, C are any three points on a line such that AC + CB = AB, then we can be sure that C lies between A and B.]
Solution :

Length of AB = 7 cm
Length of BC = 3 cm
Length of AC = 4 cm
AC + CB = 4 cm + 3 cm = 7 cm
But AB = 7 cm
So, AB = AC A- CB.

Question 4.
If A, B, C are three points on a line such that AB = 5 cm, BC – 3 cm and AC – 8 cm, which one of them lies between the other two?

Solution :
AB + BC = AC, so, the point B lies between the point A and point C.

Question 5.
Verify, whether D is the mid-point of AG.
Solution :
AD = AB + BC + CD = 3 units
DG = OE + EF + FG = 3 units
∴ Yes ! D is the mid-point of AG.

Question 6.
If B is the mid-point of \(\overline { AC }\) and C is the mid-point of \(\overline { BD }\), where A, B, C, D lie on a straight line, say why AB = CD ?
Solution :

∴ B is the mid-point of \(\overline { AC }\)
∴ AB = BC …(1)
∴ C is the mid-point of \(\overline { BD }\)
∴ BC = CD … (2)
In view of (1) and (2), we get AB = CD.

Question 7.
Draw five triangles and measure their sides. Check in each case, if the sum of the lengths of any two sides is always less than the third side.
Solution :
(i) AB = 3.7 cm
BC = 3 cm
AC = 3.8 cm

Clearly, AB + BC > AC
BC + AC > AB
AC + AB > BC

(ii) AB = 3 cm
BC = 3 cm
CA = 3 cm

Clearly, AB + BC > AC
BC + AO AB
AC + AB > B C

(iii) AB = 4 cm
BC = 3 cm
AC = 5 cm

Clearly, AB + BC > AC
BC+ AC > AB
AC + AB > BC.

(iv) AB = 2 cm
BC = 2 cm
AC = 2.8 cm

Clearly, AB + BC > AC
BC + AC > AB
AC +AB> BC

(v) AB = 3 cm
BC = 4 cm
CA = 3 cm

Clearly, AB + BC > AC
BC + AC> AB
AC + AB> BC
In each case, we observe that the sum of the lengths of any two sides is always greater than the third side.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes Ex 5.1 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes Ex 5.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 10 Mensuration Ex 10.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 10 Mensuration Ex 10.1.

• Mensuration Class 6 Ex 10.2
• Mensuration Class 6 Ex 10.3

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 10
Chapter Name	Mensuration
Exercise	Ex 10.1
Number of Questions Solved	17
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 10 Mensuration Ex 10.1

Question 1.
Find the perimeter of each of the following figures:



Solution :
(a) Perimeter
= 5 cm + I cm + 2 cm + 4 cm
= 12 cm
(b) Perimeter
= 40 cm + 35 cm + 23 cm + 35 cm
= 133 cm
(c) Perimeter
= 15 cm + 15 cm + 15 cm+ 15 cm
= 60 cm
(d) Perimeter
= 4 cm + 4 cm + 4 cm + 4 cm + 4 cm
= 20 cm
(e) Perimeter
= 1 cm + 4 cm + 0.5 cm + 2.5 cm + 2.5 cm + 0.5 cm + 4 cm
= 15 cm
(f) Perimeter = 1 cm + 3 cm + 2 cm + 3 cm + 4 cm + 1 cm + 3 cm + 2 cm + 3 cm + 4 cm + 1 cm + 3 cm + 2 cm + 3 cm + 4 cm + 1 cm + 3 cm + 2 cm + 3 cm + 4 cm
= 52 cm

Question 2.
The lid of a rectangular box of sides 40 cm by 10 cm is sealed all around with tape. What is the length of the tape required?
Solution :
Length of the tape required
= Perimeter of the rectangular box = 2 × (Length + Breadth)
= 2 × (40 cm + 10 cm)
= 2 × (50 cm)
= 100 cm = l m.

Question 3.
A table-top measures 2 m 25 cm by l m 50 cm. What is the perimeter of the table-top?
Solution :
Perimeter of the table-top
= 2 × (Length + Breadth)
= 2 × (2 m 25 cm + 1 m 50 cm)
= 2 × (2.25 m+1.50 m)
= 2 × (3.75 m)
= 7.5 m

Question 4.
What is the length of the wooden strip required to frame a photograph of length and breadth 32 cm and 21 cm respectively?
Solution :
Length of the wooden strip required = 2 × (Length + Breadth)
= 2 × (32 cm + 21 cm)
= 2 × (53 cm)
= 106 cm = 1.06 m.

Question 5.
A rectangular piece of land measures 0.7 km by 0.5 km. Each side is to be fenced with 4 rows of wires. What is the length of the wire needed?
Solution :
Perimeter of the rectangle
= 2 × (Length + Breadth )
= 2 × (0.7 km + 0.5 km)
= 2 × (1.2km)
= 2.4 km
Length of the wire needed
= 4 × Perimeter of the rectangle = 4 × (2.4 km)
= 9.6 km.

Question 6.
Find the perimeter of each of the following shapes:
(a) A triangle of sides 3 cm, 4 cm, and 5 cm
(b) An equilateral triangle of side 9 cm
(c) An isosceles triangle with equal sides 8 cm each a mi third side 6 cm.
Solution :
(a) Perimeter of the triangle = 3 cm + 4 cm + 5 cm = 12 cm
(b) Perimeter of the equilateral triangle = 3 × Length of a side = 3 × (9 cm) = 27 cm
(c) Perimeter of the isosceles triangle = 8 cm + 8 cm + 6 cm = 22 cm.

Question 7.
Find the perimeter of a triangle with sides measuring 10 cm, 14 cm, and 15 cm.
Solution :
Perimeter of the triangle
= Sum of the lengths of its three sides
= 10 cm + 14 cm + 15 cm
= 39 cm.

Question 8.
Find the perimeter of a regular hexagon with each side measuring 8 m.
Solution :
Perimeter of the regular hexagon
= 6 × Length of a side
= 6 × (8m)
= 48 m.

Question 9.
Find the side of the square whose perimeter is 20 m.
Solution :
Perimeter of the square
= 4 × Length of a side
⇒ Length of one side

Question 10.
The perimeter of a regular pentagon is 100 cm. How long is its every side?
Solution :
Perimeter of the regular pentagon = 5 × Length of a side
⇒ Length of one (each) side

Question 11.
A piece of string is 30 cm long. What will be the length of each side if the string is used to form:
(a) a square?
(b) an equilateral triangle?
(c) a regular hexagon?
Solution :
(a) Perimeter of the square = 4 × Length of a side
⇒ Length of a side

(b) Perimeter of the equilateral triangle
= 3 × Length of a side
⇒ Length of a side


(c) Perimeter of the regular hexagon = 6 × Length of a side.
⇒ Length of a side

Question 12.
Two sides of a triangle are 12 cm and 14 cm. The perimeter of the triangle is 36 cm. What is the third side?
Solution :
Perimeter of a triangle = Sum of the lengths of its three sides
⇒ 36 cm = 12 cm + 14 cm + Length of the third side
⇒ 36 cm = 26 cm + Length of the third side
⇒ Length of the third side = 36 cm – 26 cm = 10 cm.

Question 13.
Find the cost of fencing a square park of side 250 m at the rate of ₹ 20 per meter.
Solution :
Perimeter of the square park = 4 × Length of a side = 4 × (250m)
= 1000 m
∴ Cost of fencing the square park at the rate of?
20 per metre = ₹ 1000 × 20
= ₹ 20,000

Question 14.
Find the cost of fencing a rectangular park of length 175 m and breadth 125 m at the rate of? 12 per meter.
Solution :
Perimeter of the rectangular park = 2 × (Length + Breadth)
= 2 × (175m + 125 m)
= 2 × (300 m)
= 600 m
Cost of fencing the rectangular park at the rate of ?
12 per metre = ₹ 600 × 12
= ₹ 7200.

Question 15.
Sweety runs around a square park of side 75 m. Bulbul runs around a rectangular park with length 60 m and breadth 45 m. Who covers less distance?
Solution :
Perimeter of the square park = 4 × Length of a side = 4 × (75 m)
= 300 m
Perimeter of the rectangular park
= 2 × (Length + Breadth)
= 2 × (60 m + 45 m)
= 2 × (105 m)
= 210 m.
Since, the perimeter of the rectangular park is less than the perimeter of the square park, therefore. Bulbul covers less distance.

Question 16.
What is the perimeter of each of the following figures? What do you infer from the answers?


Solution :
(a) Perimeter
= Sum of the lengths of all the sides
= 25 cm + 25 cm + 25 cm + 25 cm
= 100 cm.

(b) Perimeter
= Sum of the lengths of all the sides
= 40 cm + 10 cm + 40 cm + 10 cm
= 100 cm.

(c) Perimeter
= Sum of the lengths of all the sides
= 30 cm + 20 cm + 30 cm + 20 cm
= 100 cm.

(d) Perimeter
= Sum of the lengths of all the sides
= 30 cm + 40 cm + 30 cm
= 100 cm.
The inference from the answers. All the figures have the same perimeter.

Question 17.
Avneet buys 9 square paving slabs, each with a side of \(\frac { 1 }{ 2 }\) m. He lays them in the form of a square.

(a) What is the perimeter of his arrangement (Fig. i)?
(b) Shari does not like his arrangement. She gets him to lay them out like a cross. What is the perimeter of her arrangement (Fig. ii)?
(c) Which has a greater perimeter?
(d) Avneet wonders if there is a way of getting an even greater perimeter. Can you find a way of doing this? (The paving slabs must meet along complete edges i.e. they cannot be broken.)
Solution :
(a) Perimeter of his arrangement = 4 × Length of one side

(b) Perimeter of her arrangement = Sum of the lengths of all the sides


(c) Cross has greater perimeter.
(d) Yes ! there is a way of getting an even greater perimeter. It is shown below:

 

We hope the NCERT Solutions for Class 6 Maths Chapter 10 Mensuration Ex 10.1 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 10 Mensuration Ex 10.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 9 Data Handling Ex 9.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 9 Data Handling Ex 9.1.

• Data Handling Class 6 Ex 9.2
• Data Handling Class 6 Ex 9.3
• Data Handling Class 6 Ex 9.4

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 9
Chapter Name	Data Handling
Exercise	Ex 9.1
Number of Questions Solved	7
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 9 Data Handling Ex 9.1

Question 1.
In a Mathematics test, the following marks were obtained by 40 students. Arrange these marks in a table using, tally marks.

8	I	3	7	6	5	5	4	4	2
4	9	5	3	7	I	6	5	2	7
7	3	8	4	2	8	9	5	8	6
7	4	5	6	9	6	4	4	6	6

(a) Find how many students obtained marks equal to or more than 7.
(b) How many students obtained marks below 4?
Solution.

(a) 5 + 4 + 3=12 students obtained marks equal to or more than 7.
(b) 3 + 3 + 2 = 8 students obtained marks below 4.

Question 2.
Following is the choice of sweets of 30 students of Class VI
Ladoo, Barfi, Ladoo, Jalebi, Ladoo, Rasgulla, Jalebi, Ladoo, Barfi, Rasgulla, Ladoo, Jalebi Jalebi, Rasgulla, Ladoo, Rasgulla, Jalebi, Ladoo, Rasgulla, Ladoo, Ladoo, Barfi, Rasgulla, Rasgulla, Jalebi, Rasgulla, Ladoo, Rasgulla, Jalebi, Ladoo.
(a) Arrange the names of sweets in a table using tally marks.
(b) Which sweet is preferred by most of the students?
Solution.
(a)

(b) Ladoo is preferred by most of the students.

1	3	5	6	6	3	5	4	1	6
2	5	3	4	6	1	5	5	6	1
1	2	2	3	5	2	4	5	5	6
5	1	6	2	3	5	2	4	1	5

Question 3.
Make a table and enter the data using tally- marks. Find the number that appeared.
(a) The minimum number of times.
(b) The maximum number of times.
(c) Find those numbers that appear an equal number of limes.
Solution.

(a) The number that appeared the minimum number of times is 4.
(b) The number that appeared the maximum number of times is 5.
(c) The numbers that appeared an equal number of times are 1 and 6.

Question 4.
Following pictograph shows the number of tractors in five villages:

Observe the pictograph and answers the following questions:
(i) Which village has the minimum number of tractors?
(ii) Which village has the maximum number of tractors?
(iii) How many more tractors village C has as compared to village B?
(iv) What is the total number of tractors in all the five villages?
Solution.
(i) Village D has the minimum number of tractors.
(ii) Village C has the maximum number of tractors.
(iii) Village C has 8-5 = 3 more tractors as compared to village B.
(iv) Total number of tractors in all the five villages = 6 +5+ 8 + 3 + 6 = 28.

Question 5.
The number of girl students in each class of a co-educational middle school is depicted by the pictograph:

Observe this pictograph and answer the following questions:
(a) Which class has the minimum number of girl students?
(b) Is the number of girls in class VI less than the number of girls in class V?
(c) How many girls are there in VII class?
Solution.
(a) Class VIII has the minimum no. of girl students.
(b) No! the number of girls in class VI is not less than the number of girls in class V.
(c) Number of girls in class VII – 3 x 4 = 12.

Question 6.
The sale of electric bulbs on different days of a week is shown below:

What can we conclude from the said pictograph?
Observe the pictograph and answer the following questions:
(a) How many bulbs were sold on Friday?
(b) On which day the maximum number of bulbs were sold?
(c) If one bulb was sold at the rate off 10, what was the total sale on Sunday?
(d) Can you find out the total sale of the week?
(e) If one big carton can hold 9 bulbs, how many cartons were needed in the given week?
Solution.
(a) Number of bulbs sold on Friday = 7×2 = 14.
(b) The maximum number of bulbs were sold on Sunday.
(c) Number of bulbs sold on Sunday
= 9 x 2=18.
∴ Total sale on Sunday
= Rs.18 x 10 = Rs. 180.
(d) Total number of bulbs sold in the week
= (6 + 8 + 4 + 5 + 7 + 4 + 9) x 2
= 43 x 2 = 86.

Hence, 10 cartons were needed in the given week.

Question 7.
In a village six fruit merchants sold the following number of fruit baskets in a particular season:

Observe this pictograph and answer the following questions:
(a) Which merchant sold the maximum number of baskets?
(b) How many fruit baskets were sold by Anwar?
(c) The merchants who have sold 600 or number of baskets are planning to buy a godown for the next season. Can you name them?
Solution.
(a) Martin sold the maximum number of baskets.
(b) 7 x 100 = 700 fruit baskets were sold by Anwar.
(c) Yes! Anwar. Martin and Ranjit Singh are planning to buy a godown for the next season.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 9 Data Handling Ex 9.1 help you. If you have any query regarding. NCERT Solutions for Class 6 Maths Chapter 9 Data Handling Ex 9.1, drop a comment below and we will get back to you at the earliest.