NCERT Solutions for Class 7 Maths Chapter 5 Lines and Angles Ex 5.1 are part of NCERT Solutions for Class 7 Maths. Here we have given NCERT Solutions for Class 7 Maths Chapter 5 Lines and Angles Ex 5.1.
| Board | CBSE |
| Textbook | NCERT |
| Class | Class 7 |
| Subject | Maths |
| Chapter | Chapter 5 |
| Chapter Name | Lines and Angles |
| Exercise | Ex 5.1, Ex 5.2. |
| Number of Questions Solved | 14 |
| Category | NCERT Solutions |
NCERT Solutions for Class 7 Maths Chapter 5 Lines and Angles Ex 5.1
Question 1.
Find the complement of each of the following angles:
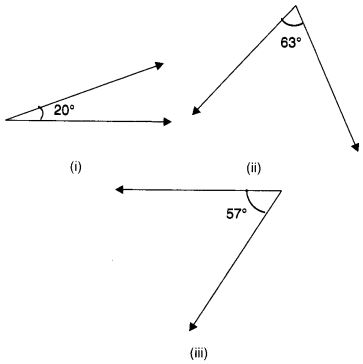
Solution:
Since, the sum of the measures of an angle and its complement is 90°, therefore,
- The complement of an angle of measure 20° is the angle of (90° – 20°), f.e., 70°.
- The complement of an angle of measure 63° is the angle of (90° – 63°), i.e., 27°.
- The complement of an angle of measure 57° is the angle of (90° – 57°), i.e., 33°.
Question 2.
Find the supplement of each of the following angles:
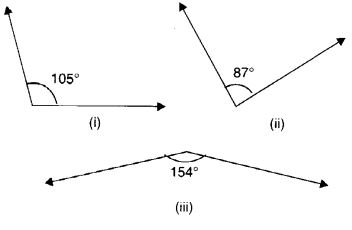
Solution:
- Supplement of the angle 105° = 180° – 105° = 75°
- Supplement of the angle 87° = 180° – 87° = 93°
- Supplement of the angle 154° = 180° – 154° = 26°
Question 3.
Identify which of the following pairs of angles are complementary and which are supplementary.
- 65°, 115°
- 63°, 27°
- 112°, 68°
- 130°, 50°
- 45°,45°
- 80°, 10°.
Solution:
- Since, 65°+ 115° = 180°
So, this pair of angles are supplementary. - Since, 63°+ 27° = 90°
So, this pair of angles are complementary. - Since, 112° + 68° = 180°
So, this pair of angles are supplementary. - Since, 130°+50° = 180°
So, this pair of angles are supplementary. - Since, 45°+ 45° = 90°
So, this pair of angles are complementary. - Since, 80°+ 10° = 90°
So, this pair of angles are complementary.
Question 4.
Find the angle which is equal to its complement.
Solution:
Let the measure of the angle be x°. Then, the measure of its complement is given to be x°.
Since, the sum of the measures of an angle and its complement is 90°, therefore,
x° + x° = 90°
⇒ 2x° = 90°
⇒ x° = 45°
Thus, the required angle is 45°.
Question 5.
Find the angle which is equal to its supplement.
Solution:
Let the measure of the angle be x°. Then,
a measure of its supplement = x°
Since, the sum of the measures of an angle and its supplement is 180°, therefore,
x° + x° = 180°
⇒ 2x° =180°
⇒ x° = 90°
Hence, the required angle is 90°.
Question 6.
In the given figure, ∠ 1 and ∠ 2 are supplementary angles.

If ∠1 is decreased, what changes should take place in ∠ 2 so that both the angles still remain supplementary?
Solution:
∠ 2 will increase as much as ∠ 1 decreases.
Question 7.
Can two angles be supplementary if both of them are:
- acute?
- obtuse?
- right?
Solution:
- No! two acute angles cannot be a supplement.
- No! Two obtuse angles cannot be supplementary.
- Yes! Two right angles are always supplementary.
Question 8.
An angle is greater than 45°. Is its complementary angle greater than 45° or equal to 45° or less than 45°.
Solution:
Since the sum of the measure of ah angle and its complement is 90°.
∴ The complement of an angle of measures 45° + x°,
where x > 0 is the angle of [90° – (45° + x°)] = 90° – 45° – x°= 45° – x°.
Clearly, 45° + x° > 45° – x°
Hence, the complement of an angle > 45° is less than 45°.
Question 9.
In the adjoining figure:
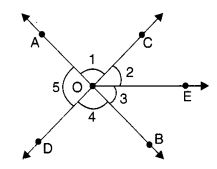
- Is ∠1 adjacent to ∠2 ?
- Is ∠ AOC adjacent to ∠ AOE?
- Do ∠ COE and ∠ EOD form a linear pair?
- Are ∠ BOD and ∠ DOA supplementary?
- Is ∠ 1 vertically opposite to ∠ 4?
- What is the vertically opposite angle of ∠ 5?
Solution:
- Yes ! ∠ 1 is adjacent to ∠ 2.
- No ! ∠ AOC is not adjacent to ∠ AOE.
- Yes! ∠ COE and ∠ EOD form a linear pair.
- Yes ! ∠ BOD and ∠ DOA are supplementary.
- Yes ! ∠ 1 is vertically opposite to ∠ 4.
- The vertically opposite angle of ∠ 5 is ∠ 2 + ∠ 3, i.e., ∠ COB.
Question 10.
Indicate which pairs of angles are:
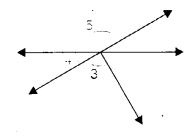
- Vertically opposite angles.
- Linear pairs.
Solution:
- The pair of vertically opposite angles are ∠1, ∠4; ∠5, ∠2 + ∠3.
- The pair of linear angles are ∠1, ∠5; ∠4, ∠5.
Question 11.
In the following figure, is ∠ 1 adjacent to ∠ 2? Give reasons.
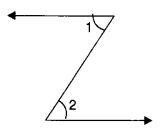
Solution:
∠1 is not adjacent to ∠2 because they have no common vertex.
Question 12.
Find the values of the angles x, y, and z in each of the following:
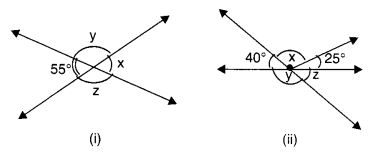
Solution:
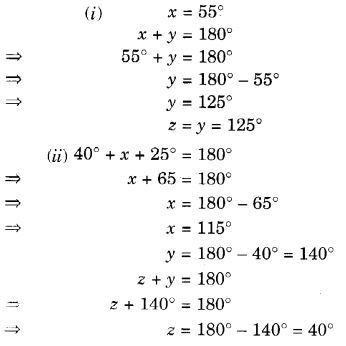
Question 13.
Fill in the blanks:
- If two angles are complementary, then the sum of their measures is
- If two angles are supplementary, then the sum of their measures is
- Two angles forming a linear pair are
- If two adjacent angles are supplementary, they form a
- If two lines intersect at a point, then the vertically opposite angles are always
- If two lines intersect at a point, and if one pair of vertically opposite angles are acute angles, then the other pair of vertically opposite angles are
Solution:
- 90°
- 180°
- supplementary
- linear pair
- equal
- obtuse angles
Question 14.
In the adjoining figure, name the following pairs of angles.
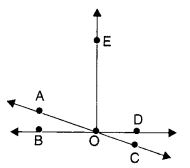
- Obtuse vertically opposite angles
- Adjacent complementary angles
- Equal supplementary angles
- Unequal supplementary angles
- Adjacent angles that do not form a linear pair.
Solution:
- Obtuse vertically opposite angles are ∠AOD and ∠BOC.
- Adjacent complementary angles are ∠BOA and ∠AOE.
- Equal supplementary angles are ∠BOE and ∠EOD.
- Unequal supplementary angles are ∠BOA and ∠AOD, ∠BOC and ∠COD, ∠EOA, and ∠EOC.
- Adjacent angles that do not form a linear pair are ∠AOB and ∠AOE, ∠AOE and ∠EOD; ∠EOD and ∠COD.
We hope the NCERT Solutions for Class 7 Maths Chapter 5 Lines and Angles Ex 5.1 help you. If you have any query regarding NCERT Solutions for Class 7 Maths Chapter 5 Lines and Angles Ex 5.1, drop a comment below and we will get back to you at the earliest.