Online Education for RD Sharma Class 10 Solutions Chapter 5 Arithmetic Progressions Ex 5.6

Online Education for RD Sharma Class 10 Solutions Chapter 5 Arithmetic Progressions Ex 5.6

These Solutions are part of Online Education RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 5 Arithmetic Progressions Ex 5.6

Other Exercises

• RD Sharma Class 10 Solutions Chapter 5 Arithmetic Progressions Ex 5.1
• RD Sharma Class 10 Solutions Chapter 5 Arithmetic Progressions Ex 5.2
• RD Sharma Class 10 Solutions Chapter 5 Arithmetic Progressions Ex 5.3
• RD Sharma Class 10 Solutions Chapter 5 Arithmetic Progressions Ex 5.4
• RD Sharma Class 10 Solutions Chapter 5 Arithmetic Progressions Ex 5.5
• RD Sharma Class 10 Solutions Chapter 5 Arithmetic Progressions Ex 5.6
• RD Sharma Class 10 Solutions Chapter 5 Arithmetic Progressions VSAQS
• RD Sharma Class 10 Solutions Chapter 5 Arithmetic Progressions MCQS

Question 1.
Find the sum of the following arithmetic progressions :

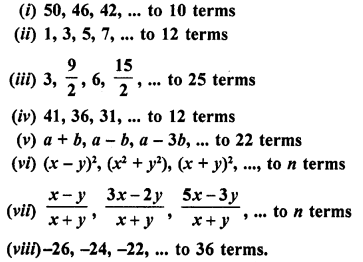
Solution:

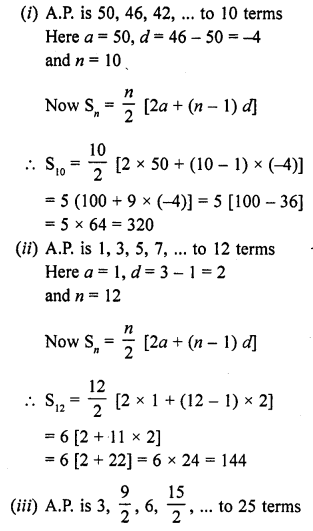
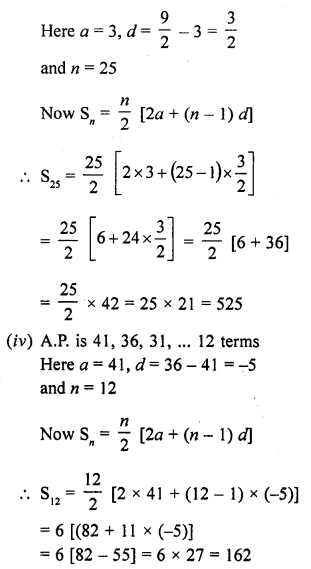
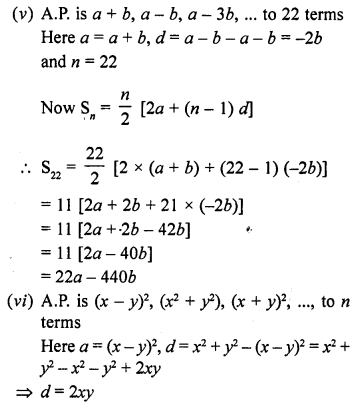
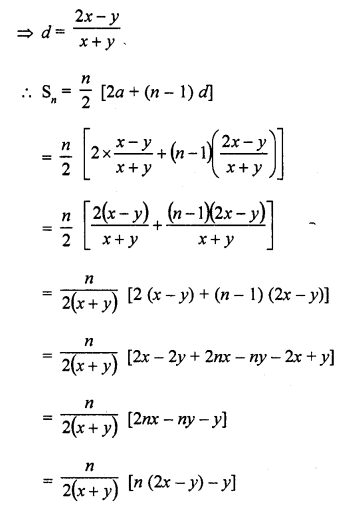
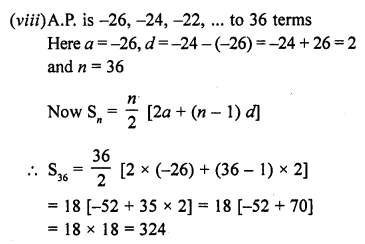
Question 2.
Find the sum to n term of the A.P. 5, 2, – 1, 4, -7, …,
Solution:

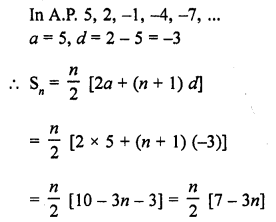
Question 3.
Find the sum of n terms of an A.P. whose nth terms is given by an = 5 – 6n.
Solution:

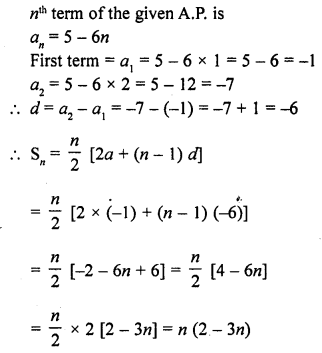
Question 4.
Find the sum of last ten terms of the A.P.: 8, 10, 12, 14,…, 126. [NCERT Exemplar]
Solution:

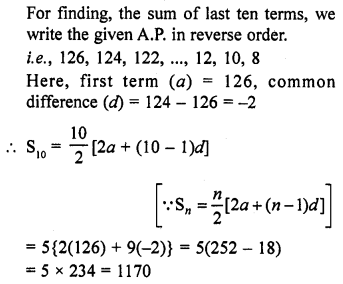
Question 5.
Find the sum of the first 15 terms of each of the following sequences having nth term as
(i) a_n = 3 + 4n
(ii) b_n = 5 + 2n
(iii) x_n = 6 – n
(iv) y_n = 9 – 5n
Solution:

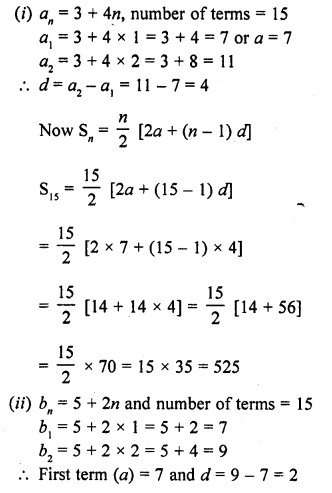
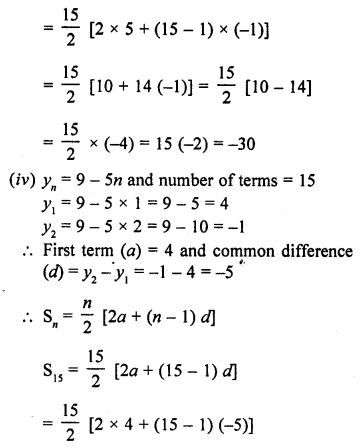
Question 6.
Find the sum of first 20 terms of the sequence whose nth term is a_n = An + B.
Solution:

Question 7.
Find the sum of the first 25 terms of an A.P. whose nth term is given by a_n = 2 – 3n. [CBSE 2004]
Solution:

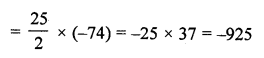
Question 8.
Find the sum of the first 25 terms of an A.P. whose nth term is given by a_n = 7 – 3n. [CBSE 2004]
Solution:

Question 9.
If the sum of a certain number of terms starting from first term of an A.P. is 25, 22, 19, …, is 116. Find the last term.
Solution:

Question 10.
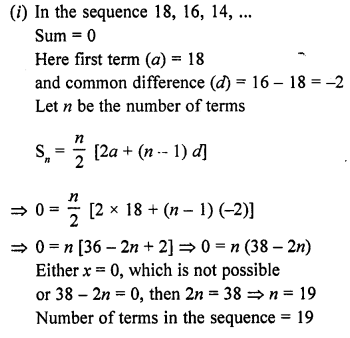
(i) How many terms of the sequence 18, 16, 14, … should be taken so that their sum is zero ?
(ii) How many terms are there in the A.P. whose first and fifth terms are -14 and 2 respectively and the sum of the terms is 40?
(iii) How many terms of the A.P. 9, 17, 25,… must be taken so that their sum is 636 ? [NCERT]
(iv) How many terms of the A.P. 63, 60, 57, ……… must be taken so that their sum is 693 ? [CBSE 2005]
(v) How many terms of the A.P. 27, 24, 21, …, should be taken so that their sum is zero? [CBSE 2016]
Solution:

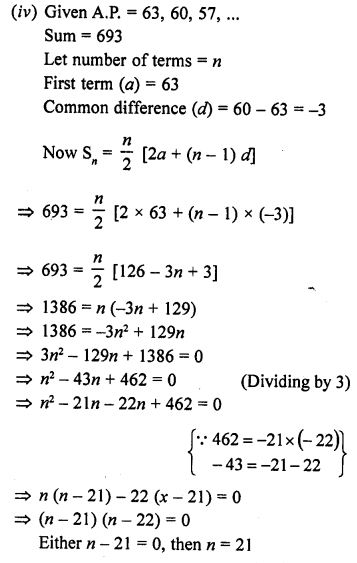
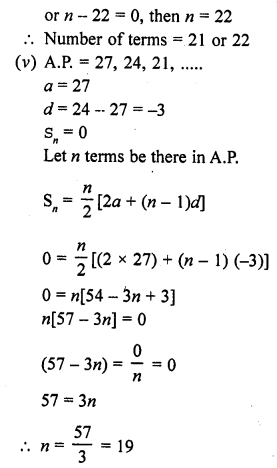
Question 11.
Find the sum of the first
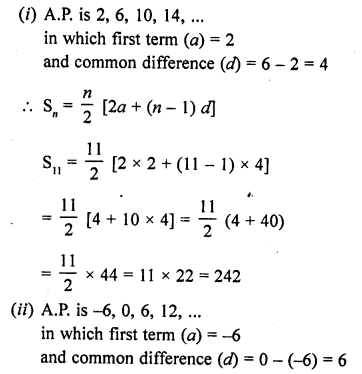
(i) 11 terms of the A.P. : 2, 6, 10, 14,…
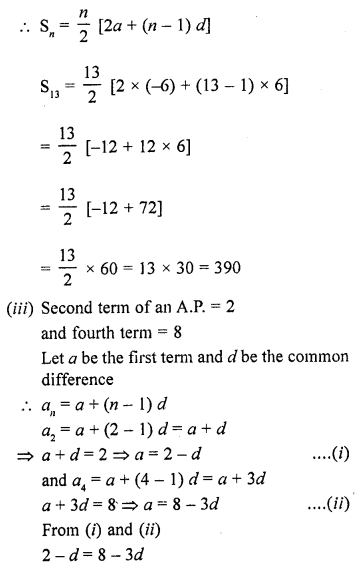
(ii) 13 terms of the A.P. : -6, 0, 6, 12,…
(iii) 51 terms of the A.P.: whose second term is 2 and fourth term is 8.
Solution:

Question 12.
Find the sum of
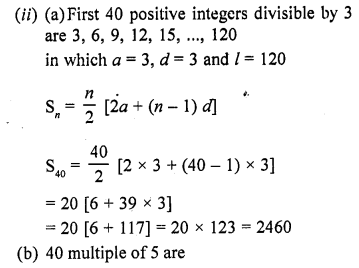
(i) the first 15 multiples of 8
(ii) the first 40 positive integers divisible by
(a) 3, (b) 5, (c) 6
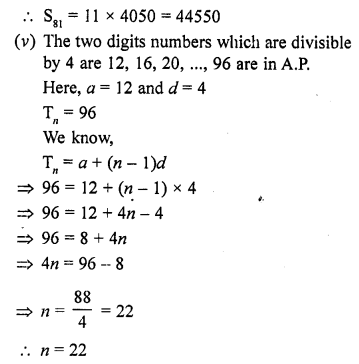
(iii) all 3-digit natural numbers which are divisible by 13. [CBSE 2006C]
(iv) all 3-digit natural numbers, which are multiples of 11. [CBSE 2012]
(v) all 2-digit natural numbers divisible by 4. [CBSE 2017]
Solution:

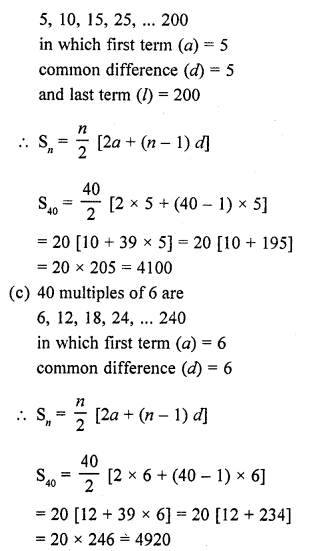
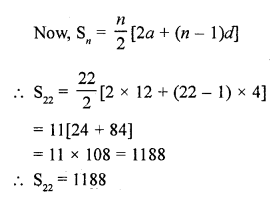
Question 13.
Find the sum :
(i) 2 + 4 + 6 + ……….. + 200
(ii) 3 + 11 + 19 + ………. + 803
(iii) (-5) + (-8) + (-11) + ……. + (-230)
(iv) 1 + 3 + 5 + 7 + …….. + 199
(v) 7 + 10\(\frac { 1 }{ 2 }\) + 14 + ……… + 84
(vi) 34 + 32 + 30 + ………. + 10
(vii) 25 + 28 + 31 + ……….. + 100 [CBSE 2006C]
(viii) 18 + 15\(\frac { 1 }{ 2 }\) + 13 + ……… + (-49\(\frac { 1 }{ 2 }\))
Solution:

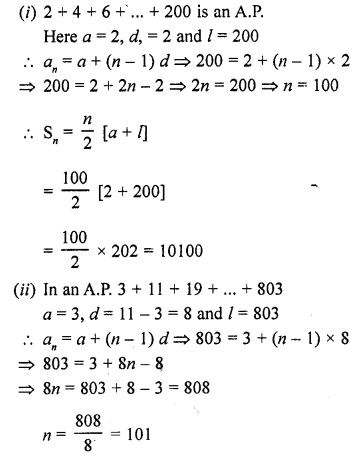
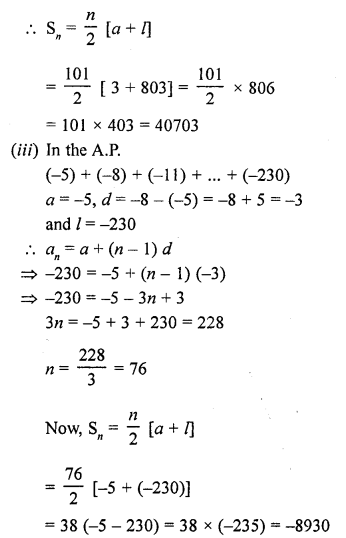
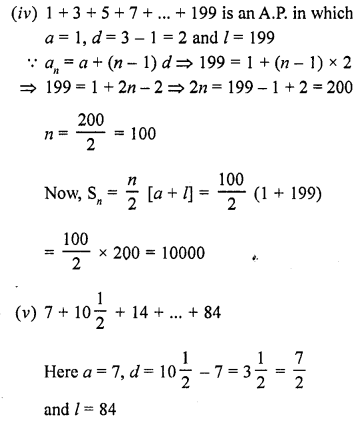
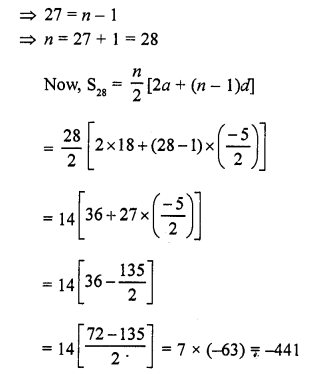
Question 14.
The first and the last terms of an A.P. are 17 and 350 respectively. If the common difference is 9, how many terms are there and what is their sum ?
Solution:
First term of an A.P. (a) = 17
Last term (l) = 350
Common difference (d) = 9
Let n be the number of terms Then an = a + (n – 1) d
=> 350 = 17 + (n – 1) x 9
=> 350 = 17 + 9n – 9
=> 9n = 350 – 17 + 9 = 342
n = 38
Number of terms = 38
Now Sn = \(\frac { n }{ 2 }\) [a + l]
= \(\frac { 38 }{ 2 }\) [17 + 350] = 19 (367) = 6973

Question 15.
The third term of an A.P. is 7 and the seventh term exceeds three times the third term by 2. Find the first term, the common difference and the sum of first 20 terms.
Solution:

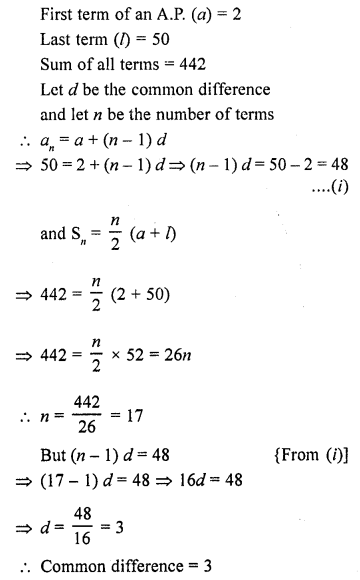
Question 16.
The first term of an A.P. is 2 and the last term is 50. The sum of all these terms is 442. Find the common difference.
Solution:

Question 17.
If 12th term of an A.P. is -13 and the sum of the first four terms is 24, what is the sum of first 10 terms ?
Solution:
12th term of an A.P. = -13
Sum of first 4 terms = 24
Let a be the first term and d be the common difference

Question 18.
Find the sum of n terms of the series

Solution:

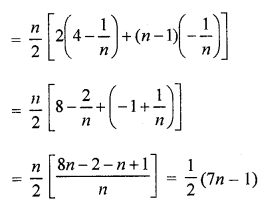
Question 19.
In an A.P., if the first term is 22, the common difference is -4 and the sum to n terms is 64, find n.
Solution:

Question 20.
In an A.P., if the 5th and 12th terms are 30 and 65 respectively, what is the sum of first 20 terms ?
Solution:
In an A.P.
5th term = 30

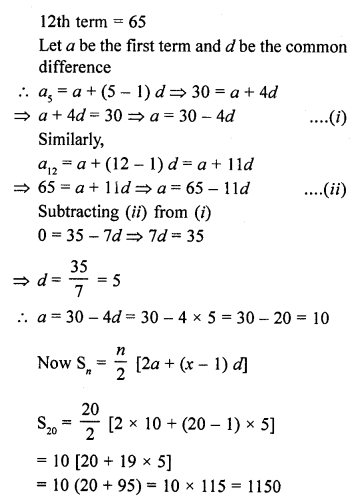
Question 21.
Find the sum of first 51 terms of an A.P. whose second and third terms are 14 and 18 respectively.
Solution:
In an A.P.
No. of terms = 51
Second term a₂ = 14
and third term a₃ = 18
Let a be the first term and d be the common
difference, then

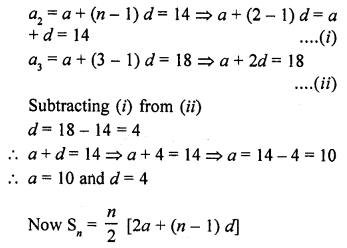
Question 22.
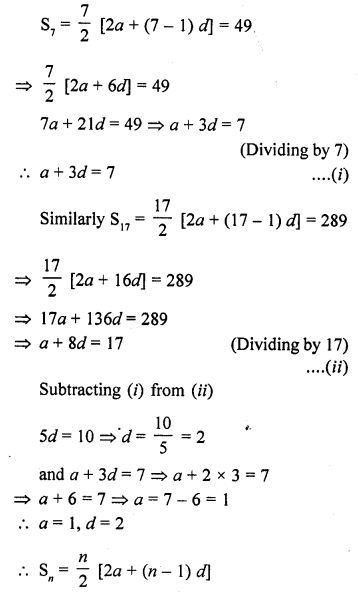
If the sum of 7 terms of an A.P. is 49 and that of 17 terms is 289, find the sum of n terms.
Solution:
Let a be the first term and d be the common difference of an A.P.
Sum of 7 terms = 49
and sum of 17 terms = 289

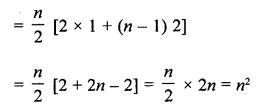
Question 23.
The first term of an A.P. is 5, the last term is 45 and the sum is 400. Find the number of terms and the common difference.
Solution:

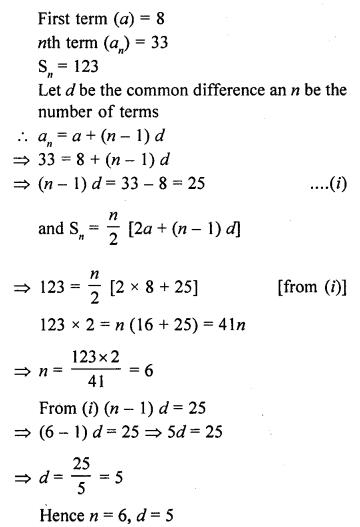
Question 24.
In an A.P. the first term is 8, nth term is 33 and the sum to first n terms is 123. Find n and d, the common differences. [CBSE 2008]
Solution:
In an A.P.

Question 25.
In an A.P., the first term is 22, nth term is -11 and the sum to first n terms is 66. Find n and d, the common difference. [CBSE 2008]
Solution:

Question 26.
The first and the last terms of an AP are 7 and 49 respectively. If sum of all its terms is 420, find its common difference. [CBSE 2014]
Solution:

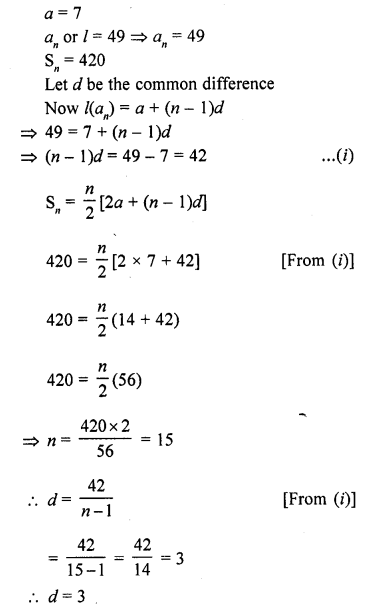
Question 27.
The first and the last terms of an A.P. are 5 and 45 respectively. If the sum of all its terms is 400, find its common difference. [CBSE 2014]
Solution:

Question 28.
The sum of first q terms of an A.P. is 162. The ratio of its 6th term to its 13th term is 1 : 2. Find the first and 15th term of the A.P. [CBSE 2015]
Solution:

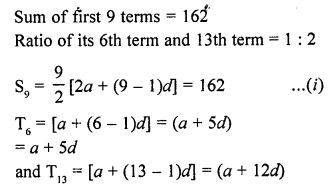
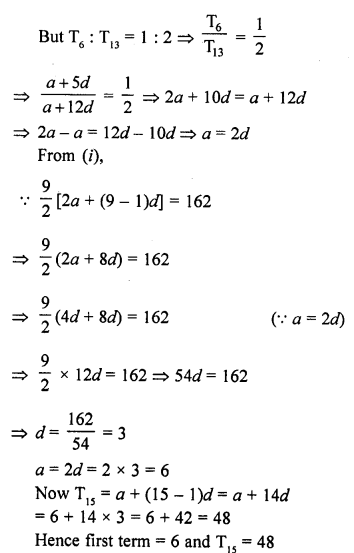
Question 29.
If the 10th term of an A.P. is 21 and the sum of its first ten terms is 120, find its nth term. [CBSE 2014]
Solution:

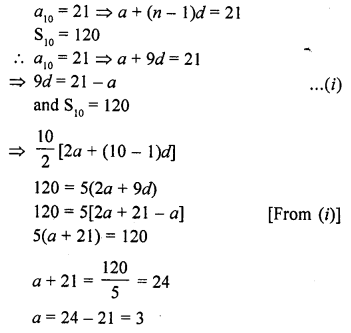
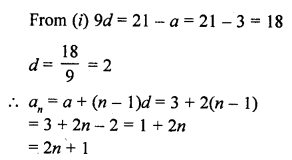
Question 30.
The sum of the first 7 terms of an A.P. is 63 and the sum of its next 7 terms is 161. Find the 28th term of this A.P. [CBSE 2014]
Solution:

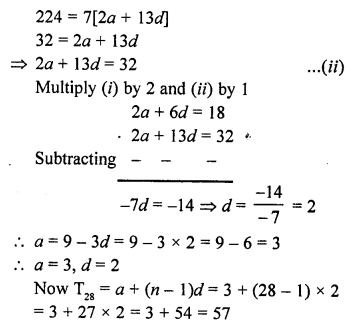
Question 31.
The sum of first seven terms of an A.P. is 182. If its 4th and the 17th terms are in the ratio 1 : 5, find the A.P. [CBSE 2014]
Solution:

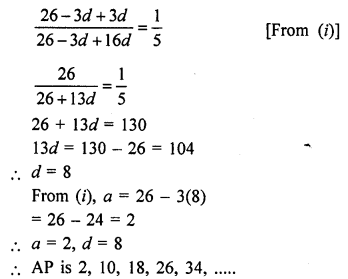
Question 32.
The nth term of an A.P. is given by (-4n + 15). Find the sum of first 20 terms of this A.P. [CBSE 2013]
Solution:

Question 33.
In an A.P., the sum of first ten terms is -150 and the sum of its next ten terms is -550. Find the A.P. [CBSE 2010]
Solution:

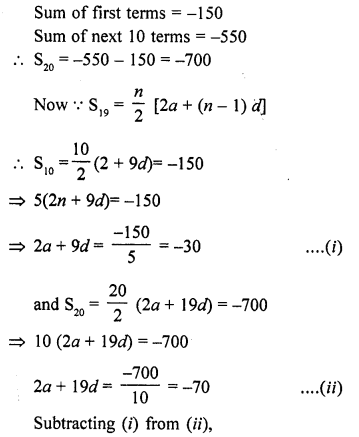
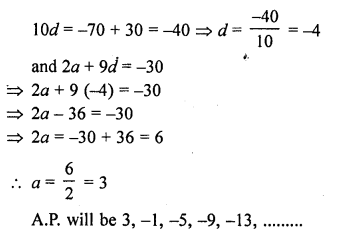
Question 34.
Sum of the first 14 terms of an A.P. is 1505 and its first term is 10. Find its 25th term. [CBSE 2012]
Solution:

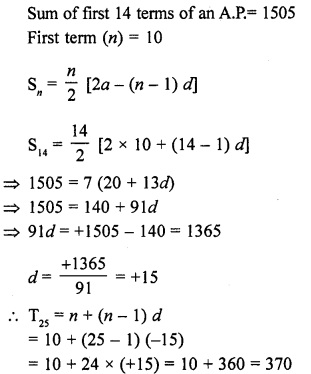
Question 35.
In an A.P., the first term is 2, the last term is 29 and the sum of the terms is 155. Find the common difference of the A.P. [CBSE 2010]
Solution:

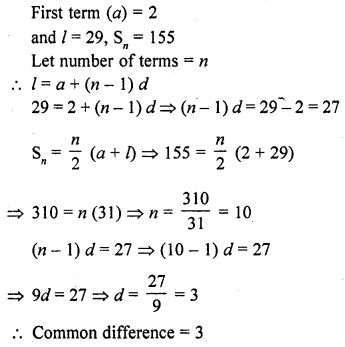
Question 36.
The first and the last term of an A.P. are 17 and 350 respectively. If the common difference is 9, how many terms are there and what is their sum ? [NCERT]
Solution:

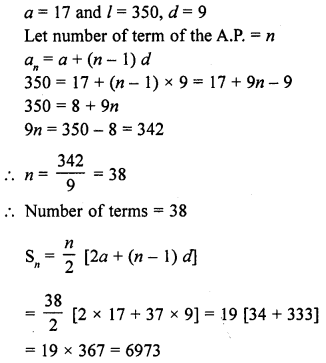
Question 37.
Find the number of terms of the A.P. -12, -9, -6,…, 21. If 1 is added to each term of this A.P., then find the sum ofi all terms of the A.P. thus obtained. [CBSE 2013]
Solution:

= 6 x 9 = 54
If we add 1 to each term, then the new sum of so formed A.P.
= 54 + 1 x 12 = 54 + 12 = 66

Question 38.
The sum of the first n terms of an A.P. is 3n² + 6n. Find the nth term of this A.P. [CBSE 2014]
Solution:

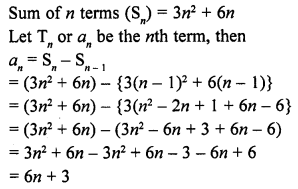
Question 39.
The sum of first n terms of an A.P. is 5n – n². Find the nth term of this A.P. [CBSE 2014]
Solution:

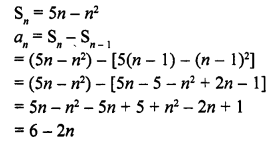
Question 40.
The sum of the first n terms of an A.P. is 4n² + 2n. Find the nth term of this A.P. [CBSE 2014]
Solution:

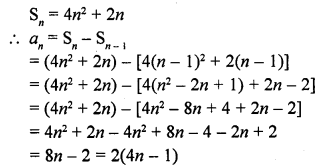
Question 41.
The sum of first n terms of an A.P. is 3n² + 4n. Find the 25th term of this A.P. [CBSE 2013]
Solution:
Let a be the first term and d be common difference

Question 42.
The sum of first n terms of an A.P. is 5n² + 3n. If its mth term is 168, find the value of m. Also, find the 20th term of this A.P. [CBSE 2013]
Solution:

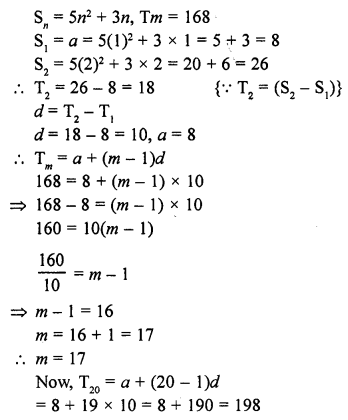
Question 43.
The sum of first q terms of an A.P. is 63q – 3q². If its pth term is -60, find the value of p, Also, find the 11th term of this A.P. [CBSE 2013]
Solution:

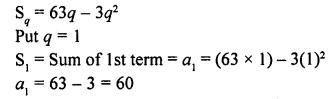
Question 44.
The sum of first m terms of an A.P. is 4m² – m. If its nth term is 107, find the value of n. Also, find the 21st term of this A.P. [CBSE 2013]
Solution:

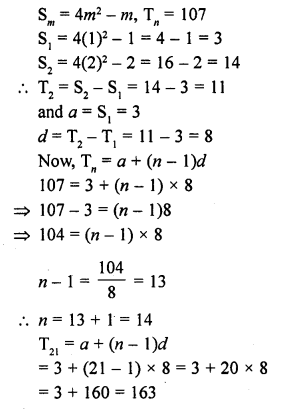
Question 45.
If the sum of the first n terms of an A.P. is 4n – n², what is the first term ? What is the sum of first two terms ? What is the second term ? Similarly, find the third, the tenth and the nth terms. [NCERT]
Solution:

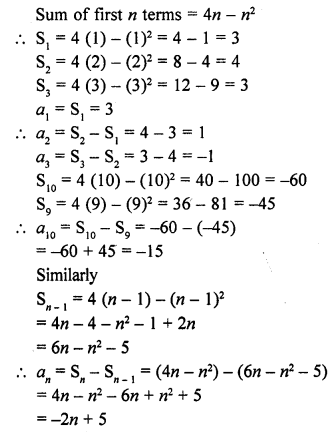
Question 46.
If the sum of first n terms of an A.P. is \(\frac { 1 }{ 2 }\) (3n² + 7n), then find its nth term. Hence write its 20th term. [CBSE 2015]
Solution:

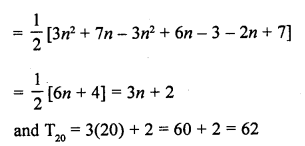
Question 47.
In an A.P., the sum of first n terms is \(\frac { { 3n }^{ 2 } }{ 2 } +\frac { 13 }{ 2 } n\). Find its 25th term. [CBSE 2006C]
Solution:


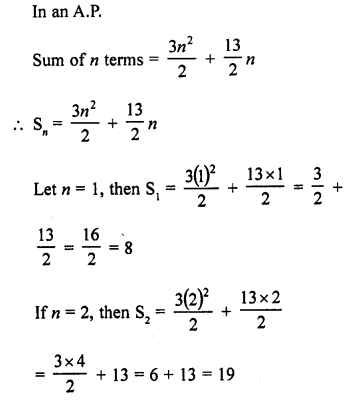
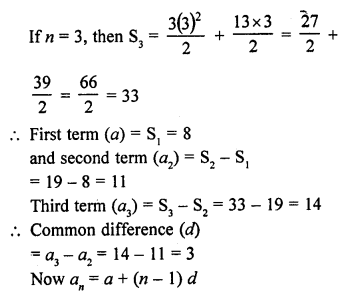
a25 = 8 + (25 – 1) x 3 = 8 + 24 x 3 = 8 + 72 = 80
Hence 25th term = 80

Question 48.
Find the sum of all natural numbers between 1 and 100, which are divisible by 3.
Solution:

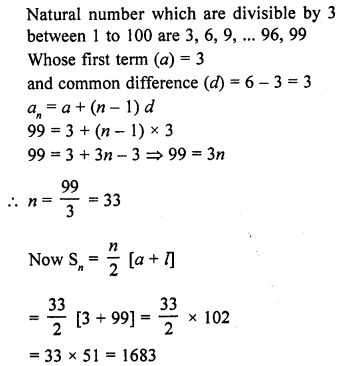
Question 49.
Find the sum of first n odd natural numbers.
Solution:

Question 50.
Find the sum of all odd numbers between
(i) 0 and 50
(ii) 100 and 200
Solution:
(i) Odd numbers between 0 and 50 are = 1, 3, 5, 7, …, 49 in which

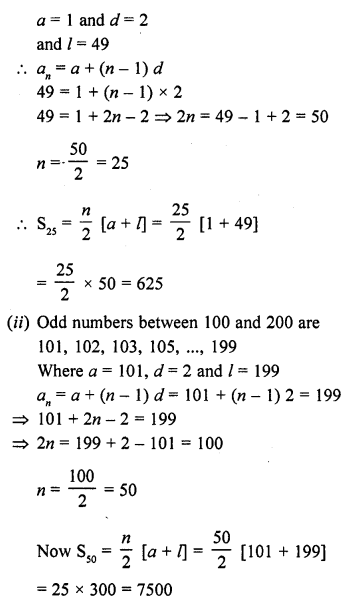
Question 51.
Show that the sum of all odd integers between 1 and 1000 which are divisible by 3 is 83667.
Solution:

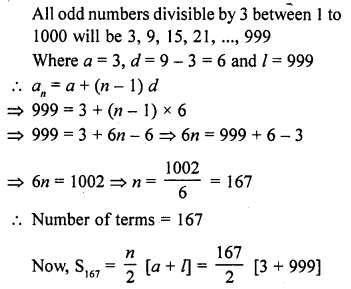
Hence proved.

Question 52.
Find the sum of all integers between 84 and 719, which are multiples of 5.
Solution:

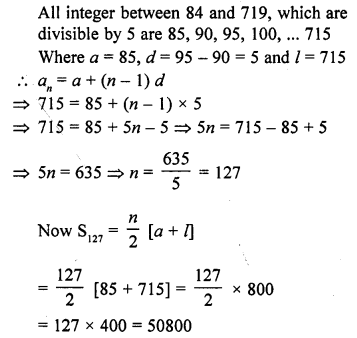
Question 53.
Find the sum of all integers between 50 and 500, which are divisible by 7.
Solution:

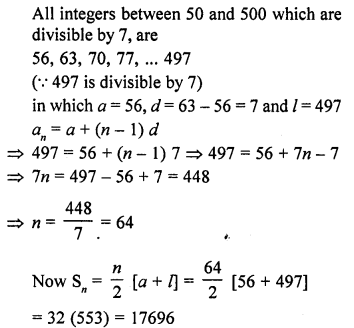
Question 54.
Find the sum of all even integers between 101 and 999.
Solution:
All integers which are even, between 101 and 999 are = 102, 104, 106, 108, … 998

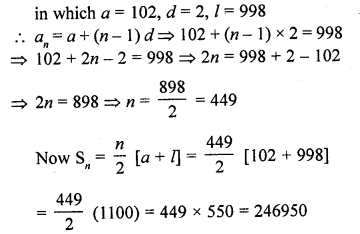
Question 55.
(i) Find the sum of all integers between 100 and 550, which are divisible by 9.
(ii) all integers between 100 and 550 which are not divisible by 9.
(iii) all integers between 1 and 500 which are multiplies of 2 as well as of 5.
(iv) all integers from 1 to 500 which are multiplies 2 as well as of 5.
(v) all integers from 1 to 500 which are multiplies of 2 or 5.
Solution:





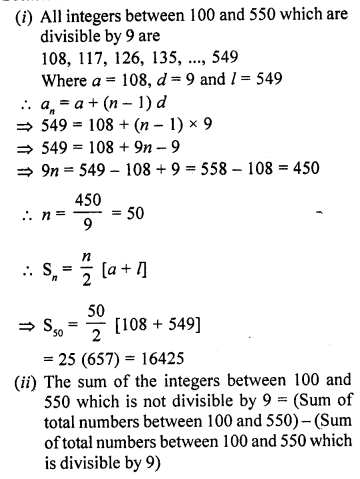
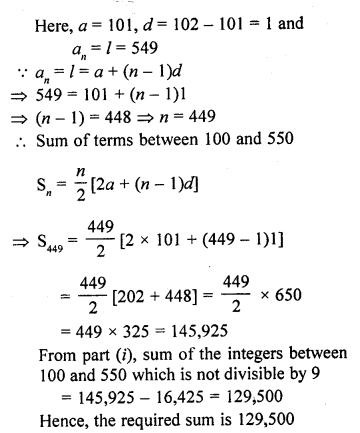
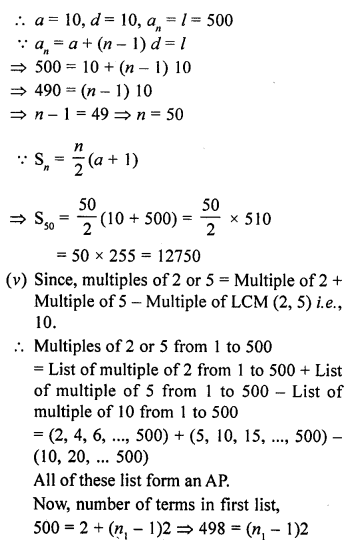
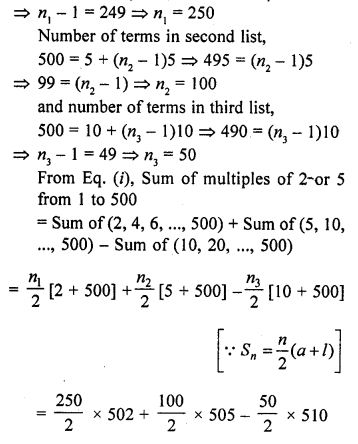
= 250 x 251 + 505 x 50 – 25 x 510
= 62750 + 25250 – 12750
= 88000 – 12750
= 75250

Question 56.
Let there be an A.P. with first term ‘a’, common difference d. If an denotes its nth term and S the sum of first n terms, find.
(i) n and S , if a = 5, d = 3 and a_n = 50.
(ii) n and a, if an = 4, d = 2 and S_n = -14.
(iii) d, if a = 3, n = 8 and S_n = 192.
(iv) a, if an = 28, S_n = 144 and n = 9.
(v) n and d, if a = 8, a_n = 62 and S_n = 210.
(vi) n and a_n, if a = 2, d = 8 and S_n = 90.
(vii) k, if S_n = 3n² + 5n and a_k = 164.
Solution:
In an A.P. a is the first term, d, the common difference a is the nth term and S_n is the sum of first n terms,

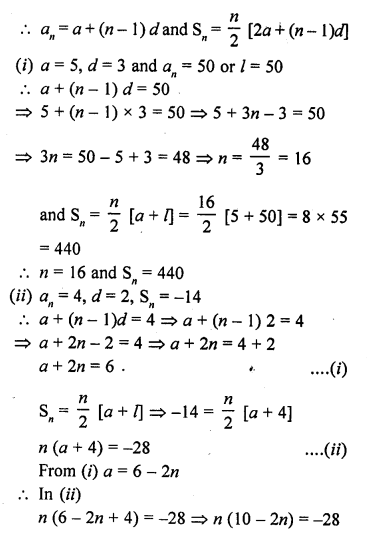
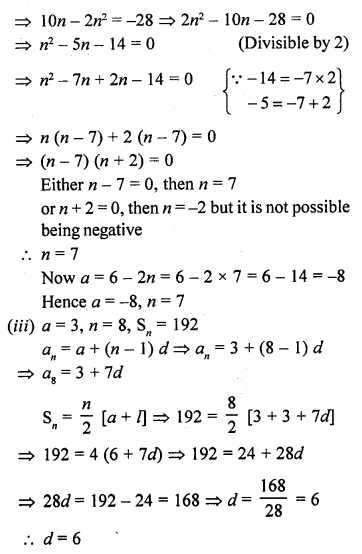
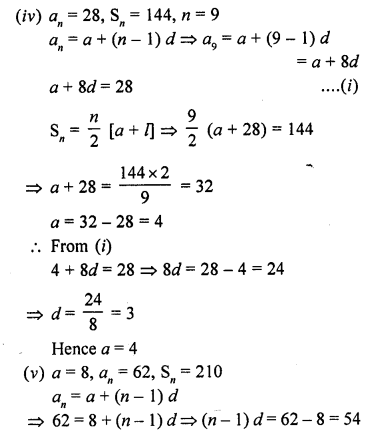
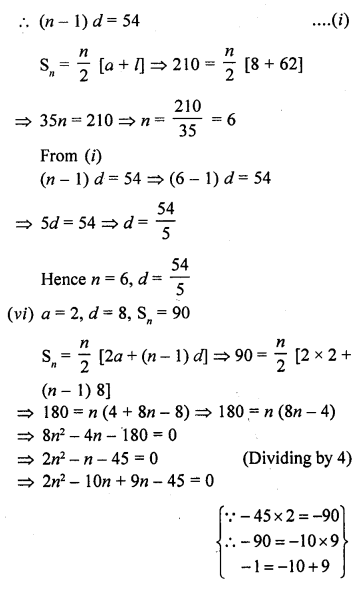
Question 57.
If S_n denotes the sum of first n terms of an A.P., prove that S₁₂ = 3(S₈ – S₄). [NCERT Exemplar, CBSE 2015]
Solution:

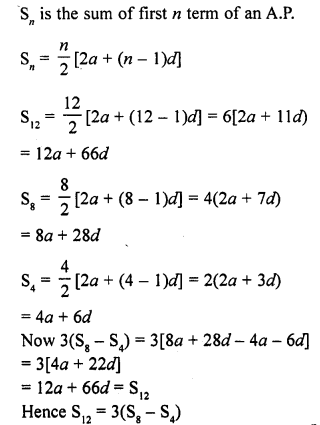
Question 58.
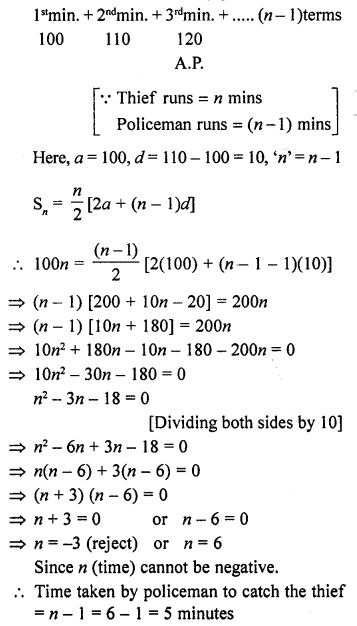
A thief, after committing a theft runs at a uniform speed of 50 m/minute. After 2 minutes, a policeman runs to catch him. He goes 60 m in first minute and increases his speed by 5m/minute every succeeding minute. After how many minutes, the policeman will catch the thief? [CBSE 2016]
Solution:
Let total time be 22 minutes.
Total distance covered by thief in 22 minutes = Speed x Time
= 100 x n = 100n metres
Total distance covered by policeman

Question 59.
The sums of first n terms of three A.P.S are S₁, S₂ and S₃. The first term of each is 5 and their common differences are 2, 4 and 6 respectively. Prove that S₁ + S₃ = 2S₂. [CBSE 2016]
Solution:

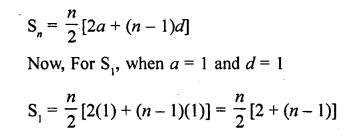
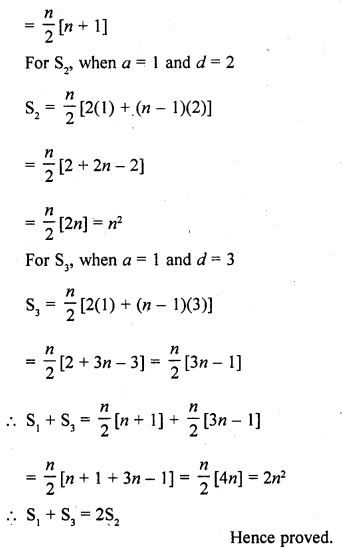
Question 60.
Resham wanted to save at least 76500 for sending her daughter to school next year (after 12 months). She saved ₹450 in the first month and raised her savings by ₹20 every next month. How much will she be able to save in next 12 months? Will she be able to send her daughter to the school next year?
Solution:
Given : Resham saved ₹450 in the first month and raised her saving by ₹20 every month and saved in next 12 months.
First term (a) = 450
Common difference (d) = 20
and No. of terms (n) = 12
We know sum of n terms is in A.P.
S_n = \(\frac { n }{ 2 }\) [2a + (n – 1) d]
S_n = \(\frac { 12 }{ 2 }\) [2 x 450 + (12 – 1) x 20]
=> S_n = 6[900 + 240]
=> S_n = 6720
Here we can see that Resham saved ₹ 6720 which is more than ₹ 6500.
So, yes Resham shall be able to send her daughter to school.

Question 61.
In a school, students decided to plant trees in and around the school to reduce air pollution. It was decided that the number of trees, that each section of each class will plant, will be double of the class in which they are studying. If there are 1 to 12 classes in the school and each class has two sections, find how many trees were planted by the students. [CBSE 2014]
Solution:

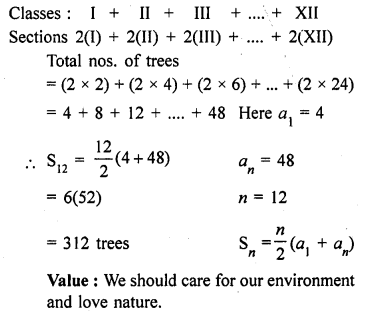
Question 62.
Ramkali would need ₹ 1800 for admission fee and books etc., for her daughter to start going to school from next year. She saved ₹ 50 in the first month of this year and increased her monthly saving by ₹ 20. After a year, how much money will she save? Will she be able to fulfill her dream of sending her daughter to school? [CBSE 2014]
Solution:
Admission fee and books etc. = ₹ 1800
First month’s savings = ₹ 50
Increase in monthly savings = ₹ 720
Period = 1 year = 12 months
Here a = 50, d = 20 and n = 12
S₁₂ = \(\frac { n }{ 2 }\) [2a + (n – 1) d]
= \(\frac { 12 }{ 2 }\) [2 x 50 + (12 – 1) x 20]
= 6[100 + 11 x 20]
= 6[100 + 220]
= 6 x 320 = ₹ 1920
Savings = ₹ 1920
Yes, she will be able to send her daughter.

Question 63.
A man saved ₹ 16500 in ten years. In each year after the first he saved ₹ 100 more than he did in the preceding year. How much did he save in the first year ?
Solution:
Savings in 10 years = ₹ 16500
S₁₀ = ₹ 16500 and d = 7100
S_n = \(\frac { n }{ 2 }\) [2a + (n – 1)d]
16500= \(\frac { 10 }{ 2 }\) [2 x a + (10 – 1) x 100]
16500 = 5 (2a + 900)
16500 = 10a + 4500
=> 10a = 16500 – 4500 = 12000
a = 1200
Saving for the first year = ₹ 1200

Question 64.
A man saved ₹ 32 during the first year, ₹ 36 in the second year and in this way he increases his savings by ₹ 4 every year. Find in what time his saving will be ₹ 200.
Solution:
Savings for the first year = ₹ 32
For the second year = ₹ 36

Question 65.
A man arranges to pay off a debt of ₹ 3600 by 40 annual installments which form an arithmetic series. When 30 of the installments are paid, he dies leaving one – third of the debt unpaid, find the value of the first installment.
Solution:

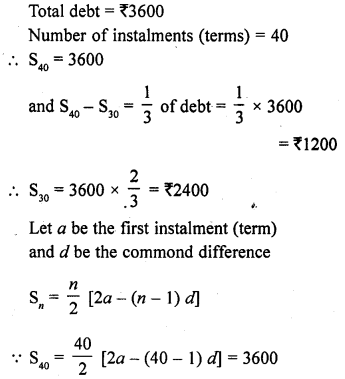
Question 66.
There are 25 trees at equal distances of 5 metres in a line with a well, the distance of the well from the nearest tree being 10 metres. A gardener waters all the trees separately starting from the well and he returns to the well after watering each tree to get water for the next. Find the total distance the gardener will cover in order to water all the trees.
Solution:
Number of trees = 25

Distance between one to other tree = 5 m
Distance between first near and the well = 10 m
Now in order to water the first tree, the gardener has to cover 10m + 10m = 20m
and to water the second tree, the distance to covered is 15 + 15 = 30 m
To water the third tree, the distance to cover is = 20 + 20 = 40 m
The series will be 20, 30, 40, ……….
where a = 20, d = 30 – 20 = 10 and n = 25

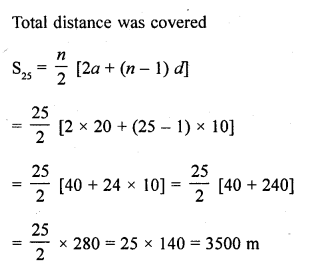
Question 67.
A man is employed to count ₹ 10710. He counts at the rate of ₹ 180 per minute for half an hour. After this he counts at the rate of ₹ 3 less every minute than the preceding minute. Find the time taken by him to count the entire amount.
Solution:

=> (n – 59) (n – 60) = 0
Either n – 59 = 0, then n – 59 or n – 60 = 0, then n = 60
Total time = 59 + 30 = 89 minutes or = 60 + 30 = 90 minutes

Question 68.
A piece of equipment cost a certain factory ₹ 600,000. If it depreciates in value, 15% the first, 13.5% the next year, 12% the third year, and so on. What will be its value at the end of 10 years, all percentages applying to the original cost ?
Solution:
Cost of a piece of equipment = ₹ 600,000
Rate of depreciation for the first year = 15%
for the second year = 13.5%
for the third year = 12.0% and so on
The depreciation is in A.P.
whose first term (a) = 15
and common difference (d) = 13.5 – 15.0 = -1.5
Period (n) = 10

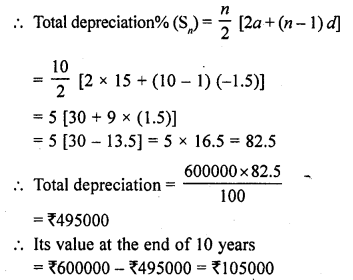
Question 69.
A sum of ₹ 700 is to be used to give seven cash prizes to students of a school for their overall academic performance. If each prize is ₹ 20 less than its preceding prize, find the value of each prize.
Solution:
Total sum = ₹ 700

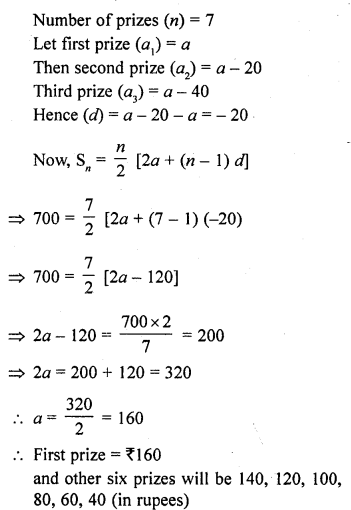
Question 70.
If S_n denotes the sum of the first n terms of an A.P., prove that S₃₀ = 3 (S₂₀ – S₁₀). [CBSE 2014]
Solution:

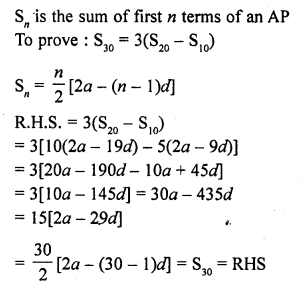
Question 71.
Solve the question: (-4) + (-1) + 2 + 5 + … + x = 437. [NCERT Exemplar]
Solution:
Given equation is,

Question 72.
Which term of the A.P. -2, -7, -12, … will be -77 ? Find the sum of this A.P. up to the term -77.
Solution:

Question 73.
The sum of first n terms of an A.P. whose first term is 8 and the common difference is 20 is equal to the sum of first 2n terms of another A.P. whose first term is -30 and common difference is 8. Find n. [NCERT Exemplar]
Solution:
Given that, first term of the first A.P. (a) = 8
and common difference of the first A.P. (d) = 20
Let the number of terms in first A.P. be n
Sum of first n terms of an A.P., S_n

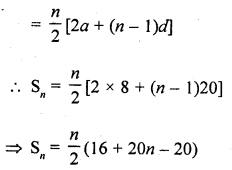
Question 74.
The students of a school decided to beautify the school on the annual day by fixing colourful on the straight passage of the school. They have 27 flags to be fixed at intervals of every 2 metre. The flags are stored at the position of the middle most flag Ruchi was given the responsibility of placing the flags. Ruchi kept her books where the flags were stored. She could carry only one flag at a time. How much distance did she cover in completing this job and returning back to collect her books? What is the maximum distance she travelled carrying a flag? [NCERT Exemplar]
Solution:
Given that, the students of a school decided to beautify the school on the annual day by fixing colourful flags on the straight passage of the school.
Given that, the number of flags = 27
and distance between each flag = 2 m.
Also, the flags are stored at the position of the middle most flag i. e., 14th flag and Ruchi was given the responsibility of placing the flags.
Ruchi kept her books, where the flags were stored i.e., 14th flag and she could carry only one flag at a time.
Let she placed 13 flags into her left position from middle most flag i.e., 14th flag.
For placing second flag and return his initial position distance travelled = 2 + 2 = 4 m.
Similarly, for placing third flag and return his initial position, distance travelled = 4 + 4 = 8 m.
For placing fourth flag and return his initial position, distance travelled = 6 + 6 = 12 m.
For placing fourteenth flag and return his initial position, distance travelled = 26 + 26 = 52 m.
Proceed same manner into her right position from middle most flag i.e., 14th flag.
Total distance travelled in that case = 52 m.
Also, when Ruchi placed the last flag she return her middle position and collect her books.
This distance also included in placed the last flag.
So, these distances from a series.
4 + 8 + 12 + 16 + … + 52 [for left]
and 4 + 8 + 12 + 16 + … + 52 [for right] .
Total distance covered by Ruchi for placing these flags
= 2 x (4 + 8 + 12 + … +52)


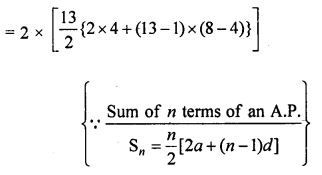
Hence, the required is 728 m in which she did cover in completing this job and returning back to collect her books.
Now, the maximum distance she travelled carrying a flag = Distance travelled by Ruchi during placing the 14th flag in her left position or 27th flag in her right position
= (2 + 2 + 2 + … + 13 times)
= 2 x 13 = 26 m
Hence, the required maximum distance she travelled carrying a flag is 26 m.

Hope given RD Sharma Class 10 Solutions Chapter 5 Arithmetic Progressions Ex 5.6 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.