Tag: NCERT Solutions for Class 6 Maths

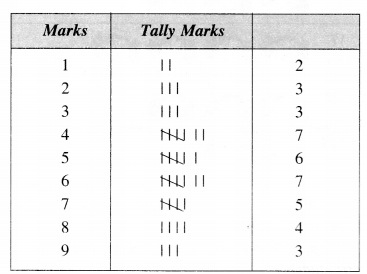
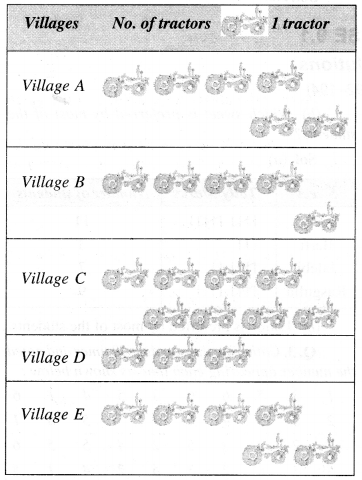
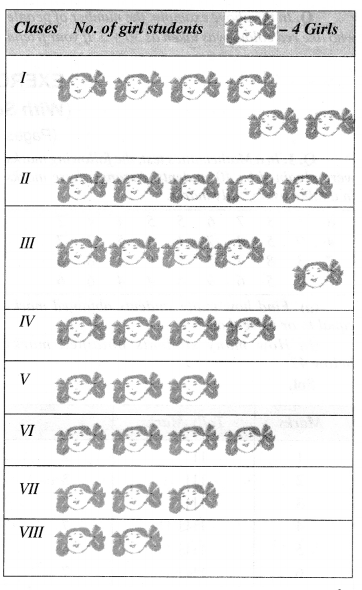
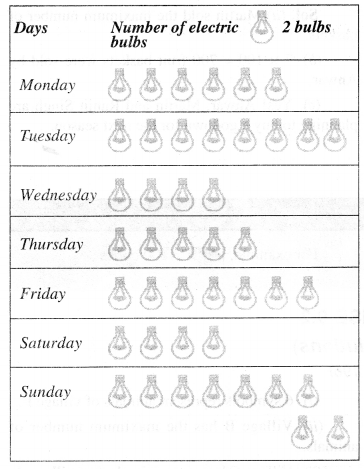
NCERT Solutions for Class 6 Maths Chapter 13 Symmetry Ex 13.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 13 Symmetry Ex 13.1.

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 13
Chapter Name	Symmetry
Exercise	Ex 13.1
Number of Questions Solved	6
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 13 Symmetry Ex 13.1

Question 1.
List any four symmetrical objects from your home or school.
Solution :
The blackboard, the table top, a pair of scissors, the computer disc.

Question 2.
For the given figure, which one is the mirror line, l₁ or l₂?

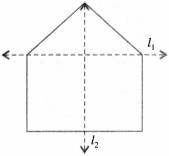
Solution :
I₂ is the mirror line.

Question 3.
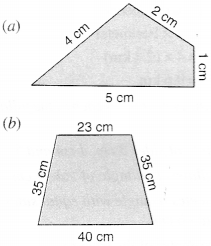
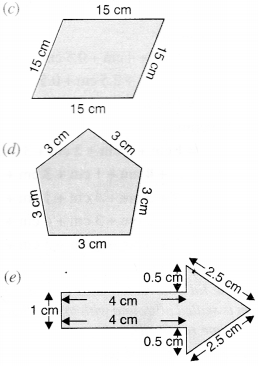
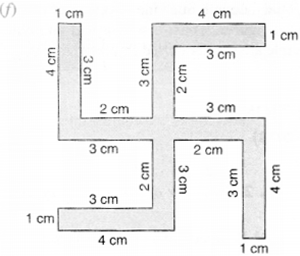
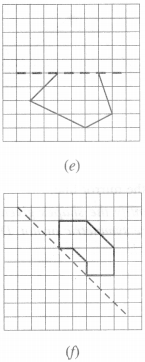
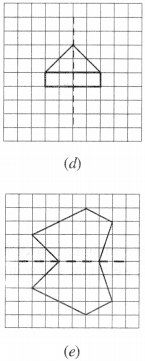
Identify the shapes given below. Check whether they are symmetric or not. Draw the line of symmetry as well.

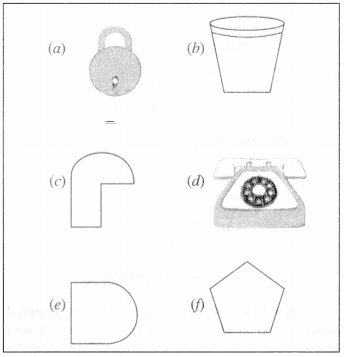
Solution :
(a) Symmetric

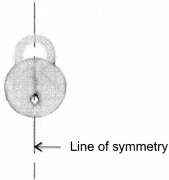
(b) Symmetric

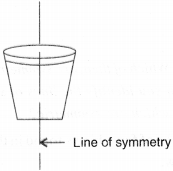
(c) Not symmetric
(d) Symmetric

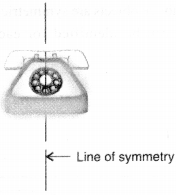
(e) Symmetric

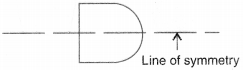
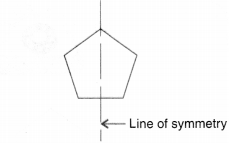
(f) Symmetric

Question 4.
Copy the following on a squared paper. A square paper is what you would have used in your arithmetic notebook in earlier classes. Then complete them such that the dotted line is the line of symmetry.



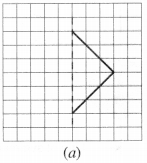
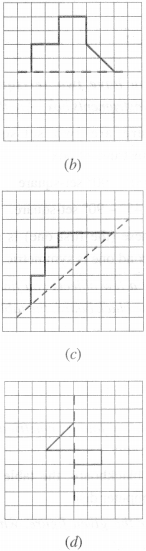
Solution :

Question 5.
In the figure, l is the line of symmetry. Complete the diagram to make it symmetric.

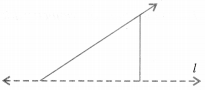
Solution :

Question 6.
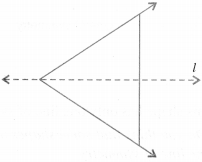
In the figure, l is the line of symmetry. Draw the image of the triangle and complete the diagram so that it becomes symmetric.

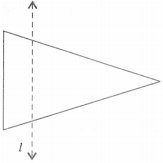
Solution :

 

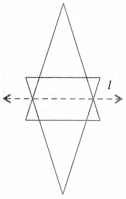
We hope the NCERT Solutions for Class 6 Maths Chapter 13 Symmetry Ex 13.1 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 13 Symmetry Ex 13.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes Ex 5.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes Ex 5.1.

• Understanding Elementary Shapes Class 6 Ex 5.2
• Understanding Elementary Shapes Class 6 Ex 5.3
• Understanding Elementary Shapes Class 6 Ex 5.4
• Understanding Elementary Shapes Class 6 Ex 5.5
• Understanding Elementary Shapes Class 6 Ex 5.6
• Understanding Elementary Shapes Class 6 Ex 5.7
• Understanding Elementary Shapes Class 6 Ex 5.8
• Understanding Elementary Shapes Class 6 Ex 5.9

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 5
Chapter Name	Understanding Elementary Shapes
Exercise	Ex 5.1
Number of Questions Solved	7
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes Ex 5.1

Question 1.
What is the disadvantage in comparing line segments by mere observation?
Solution :
Sometimes the difference in lengths betweenthe two line segments is not obvious. So, we are not always sure about our usual judgment.

Question 2.
Why is it better to use a divider than with a ruler, while measuring the length of a line segment?
Solution :
There may be errors due to the thickness of the ruler and angular viewing by using a ruler. These errors are eradicated by using a divider. So, it is better to use a divider, than a ruler, while measuring the length of a line segment.

Question 3.
Draw any line segment, say \(\overline { AB }\). Take any point C lying in between A and B. Measure the lengths of AB, BC and AC. Is AB = AC + CB? [Note : If A, B, C are any three points on a line such that AC + CB = AB, then we can be sure that C lies between A and B.]
Solution :

Length of AB = 7 cm
Length of BC = 3 cm
Length of AC = 4 cm
AC + CB = 4 cm + 3 cm = 7 cm
But AB = 7 cm
So, AB = AC A- CB.

Question 4.
If A, B, C are three points on a line such that AB = 5 cm, BC – 3 cm and AC – 8 cm, which one of them lies between the other two?

Solution :
AB + BC = AC, so, the point B lies between the point A and point C.

Question 5.
Verify, whether D is the mid-point of AG.
Solution :
AD = AB + BC + CD = 3 units
DG = OE + EF + FG = 3 units
∴ Yes ! D is the mid-point of AG.

Question 6.
If B is the mid-point of \(\overline { AC }\) and C is the mid-point of \(\overline { BD }\), where A, B, C, D lie on a straight line, say why AB = CD ?
Solution :

∴ B is the mid-point of \(\overline { AC }\)
∴ AB = BC …(1)
∴ C is the mid-point of \(\overline { BD }\)
∴ BC = CD … (2)
In view of (1) and (2), we get AB = CD.

Question 7.
Draw five triangles and measure their sides. Check in each case, if the sum of the lengths of any two sides is always less than the third side.
Solution :
(i) AB = 3.7 cm
BC = 3 cm
AC = 3.8 cm

Clearly, AB + BC > AC
BC + AC > AB
AC + AB > BC

(ii) AB = 3 cm
BC = 3 cm
CA = 3 cm

Clearly, AB + BC > AC
BC + AO AB
AC + AB > B C

(iii) AB = 4 cm
BC = 3 cm
AC = 5 cm

Clearly, AB + BC > AC
BC+ AC > AB
AC + AB > BC.

(iv) AB = 2 cm
BC = 2 cm
AC = 2.8 cm

Clearly, AB + BC > AC
BC + AC > AB
AC +AB> BC

(v) AB = 3 cm
BC = 4 cm
CA = 3 cm

Clearly, AB + BC > AC
BC + AC> AB
AC + AB> BC
In each case, we observe that the sum of the lengths of any two sides is always greater than the third side.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes Ex 5.1 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 5 Understanding Elementary Shapes Ex 5.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 10 Mensuration Ex 10.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 10 Mensuration Ex 10.1.

• Mensuration Class 6 Ex 10.2
• Mensuration Class 6 Ex 10.3

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 10
Chapter Name	Mensuration
Exercise	Ex 10.1
Number of Questions Solved	17
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 10 Mensuration Ex 10.1

Question 1.
Find the perimeter of each of the following figures:



Solution :
(a) Perimeter
= 5 cm + I cm + 2 cm + 4 cm
= 12 cm
(b) Perimeter
= 40 cm + 35 cm + 23 cm + 35 cm
= 133 cm
(c) Perimeter
= 15 cm + 15 cm + 15 cm+ 15 cm
= 60 cm
(d) Perimeter
= 4 cm + 4 cm + 4 cm + 4 cm + 4 cm
= 20 cm
(e) Perimeter
= 1 cm + 4 cm + 0.5 cm + 2.5 cm + 2.5 cm + 0.5 cm + 4 cm
= 15 cm
(f) Perimeter = 1 cm + 3 cm + 2 cm + 3 cm + 4 cm + 1 cm + 3 cm + 2 cm + 3 cm + 4 cm + 1 cm + 3 cm + 2 cm + 3 cm + 4 cm + 1 cm + 3 cm + 2 cm + 3 cm + 4 cm
= 52 cm

Question 2.
The lid of a rectangular box of sides 40 cm by 10 cm is sealed all around with tape. What is the length of the tape required?
Solution :
Length of the tape required
= Perimeter of the rectangular box = 2 × (Length + Breadth)
= 2 × (40 cm + 10 cm)
= 2 × (50 cm)
= 100 cm = l m.

Question 3.
A table-top measures 2 m 25 cm by l m 50 cm. What is the perimeter of the table-top?
Solution :
Perimeter of the table-top
= 2 × (Length + Breadth)
= 2 × (2 m 25 cm + 1 m 50 cm)
= 2 × (2.25 m+1.50 m)
= 2 × (3.75 m)
= 7.5 m

Question 4.
What is the length of the wooden strip required to frame a photograph of length and breadth 32 cm and 21 cm respectively?
Solution :
Length of the wooden strip required = 2 × (Length + Breadth)
= 2 × (32 cm + 21 cm)
= 2 × (53 cm)
= 106 cm = 1.06 m.

Question 5.
A rectangular piece of land measures 0.7 km by 0.5 km. Each side is to be fenced with 4 rows of wires. What is the length of the wire needed?
Solution :
Perimeter of the rectangle
= 2 × (Length + Breadth )
= 2 × (0.7 km + 0.5 km)
= 2 × (1.2km)
= 2.4 km
Length of the wire needed
= 4 × Perimeter of the rectangle = 4 × (2.4 km)
= 9.6 km.

Question 6.
Find the perimeter of each of the following shapes:
(a) A triangle of sides 3 cm, 4 cm, and 5 cm
(b) An equilateral triangle of side 9 cm
(c) An isosceles triangle with equal sides 8 cm each a mi third side 6 cm.
Solution :
(a) Perimeter of the triangle = 3 cm + 4 cm + 5 cm = 12 cm
(b) Perimeter of the equilateral triangle = 3 × Length of a side = 3 × (9 cm) = 27 cm
(c) Perimeter of the isosceles triangle = 8 cm + 8 cm + 6 cm = 22 cm.

Question 7.
Find the perimeter of a triangle with sides measuring 10 cm, 14 cm, and 15 cm.
Solution :
Perimeter of the triangle
= Sum of the lengths of its three sides
= 10 cm + 14 cm + 15 cm
= 39 cm.

Question 8.
Find the perimeter of a regular hexagon with each side measuring 8 m.
Solution :
Perimeter of the regular hexagon
= 6 × Length of a side
= 6 × (8m)
= 48 m.

Question 9.
Find the side of the square whose perimeter is 20 m.
Solution :
Perimeter of the square
= 4 × Length of a side
⇒ Length of one side

Question 10.
The perimeter of a regular pentagon is 100 cm. How long is its every side?
Solution :
Perimeter of the regular pentagon = 5 × Length of a side
⇒ Length of one (each) side

Question 11.
A piece of string is 30 cm long. What will be the length of each side if the string is used to form:
(a) a square?
(b) an equilateral triangle?
(c) a regular hexagon?
Solution :
(a) Perimeter of the square = 4 × Length of a side
⇒ Length of a side

(b) Perimeter of the equilateral triangle
= 3 × Length of a side
⇒ Length of a side


(c) Perimeter of the regular hexagon = 6 × Length of a side.
⇒ Length of a side

Question 12.
Two sides of a triangle are 12 cm and 14 cm. The perimeter of the triangle is 36 cm. What is the third side?
Solution :
Perimeter of a triangle = Sum of the lengths of its three sides
⇒ 36 cm = 12 cm + 14 cm + Length of the third side
⇒ 36 cm = 26 cm + Length of the third side
⇒ Length of the third side = 36 cm – 26 cm = 10 cm.

Question 13.
Find the cost of fencing a square park of side 250 m at the rate of ₹ 20 per meter.
Solution :
Perimeter of the square park = 4 × Length of a side = 4 × (250m)
= 1000 m
∴ Cost of fencing the square park at the rate of?
20 per metre = ₹ 1000 × 20
= ₹ 20,000

Question 14.
Find the cost of fencing a rectangular park of length 175 m and breadth 125 m at the rate of? 12 per meter.
Solution :
Perimeter of the rectangular park = 2 × (Length + Breadth)
= 2 × (175m + 125 m)
= 2 × (300 m)
= 600 m
Cost of fencing the rectangular park at the rate of ?
12 per metre = ₹ 600 × 12
= ₹ 7200.

Question 15.
Sweety runs around a square park of side 75 m. Bulbul runs around a rectangular park with length 60 m and breadth 45 m. Who covers less distance?
Solution :
Perimeter of the square park = 4 × Length of a side = 4 × (75 m)
= 300 m
Perimeter of the rectangular park
= 2 × (Length + Breadth)
= 2 × (60 m + 45 m)
= 2 × (105 m)
= 210 m.
Since, the perimeter of the rectangular park is less than the perimeter of the square park, therefore. Bulbul covers less distance.

Question 16.
What is the perimeter of each of the following figures? What do you infer from the answers?


Solution :
(a) Perimeter
= Sum of the lengths of all the sides
= 25 cm + 25 cm + 25 cm + 25 cm
= 100 cm.

(b) Perimeter
= Sum of the lengths of all the sides
= 40 cm + 10 cm + 40 cm + 10 cm
= 100 cm.

(c) Perimeter
= Sum of the lengths of all the sides
= 30 cm + 20 cm + 30 cm + 20 cm
= 100 cm.

(d) Perimeter
= Sum of the lengths of all the sides
= 30 cm + 40 cm + 30 cm
= 100 cm.
The inference from the answers. All the figures have the same perimeter.

Question 17.
Avneet buys 9 square paving slabs, each with a side of \(\frac { 1 }{ 2 }\) m. He lays them in the form of a square.

(a) What is the perimeter of his arrangement (Fig. i)?
(b) Shari does not like his arrangement. She gets him to lay them out like a cross. What is the perimeter of her arrangement (Fig. ii)?
(c) Which has a greater perimeter?
(d) Avneet wonders if there is a way of getting an even greater perimeter. Can you find a way of doing this? (The paving slabs must meet along complete edges i.e. they cannot be broken.)
Solution :
(a) Perimeter of his arrangement = 4 × Length of one side

(b) Perimeter of her arrangement = Sum of the lengths of all the sides


(c) Cross has greater perimeter.
(d) Yes ! there is a way of getting an even greater perimeter. It is shown below:

 

We hope the NCERT Solutions for Class 6 Maths Chapter 10 Mensuration Ex 10.1 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 10 Mensuration Ex 10.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 9 Data Handling Ex 9.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 9 Data Handling Ex 9.1.

• Data Handling Class 6 Ex 9.2
• Data Handling Class 6 Ex 9.3
• Data Handling Class 6 Ex 9.4

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 9
Chapter Name	Data Handling
Exercise	Ex 9.1
Number of Questions Solved	7
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 9 Data Handling Ex 9.1

Question 1.
In a Mathematics test, the following marks were obtained by 40 students. Arrange these marks in a table using, tally marks.

8	I	3	7	6	5	5	4	4	2
4	9	5	3	7	I	6	5	2	7
7	3	8	4	2	8	9	5	8	6
7	4	5	6	9	6	4	4	6	6

(a) Find how many students obtained marks equal to or more than 7.
(b) How many students obtained marks below 4?
Solution.

(a) 5 + 4 + 3=12 students obtained marks equal to or more than 7.
(b) 3 + 3 + 2 = 8 students obtained marks below 4.

Question 2.
Following is the choice of sweets of 30 students of Class VI
Ladoo, Barfi, Ladoo, Jalebi, Ladoo, Rasgulla, Jalebi, Ladoo, Barfi, Rasgulla, Ladoo, Jalebi Jalebi, Rasgulla, Ladoo, Rasgulla, Jalebi, Ladoo, Rasgulla, Ladoo, Ladoo, Barfi, Rasgulla, Rasgulla, Jalebi, Rasgulla, Ladoo, Rasgulla, Jalebi, Ladoo.
(a) Arrange the names of sweets in a table using tally marks.
(b) Which sweet is preferred by most of the students?
Solution.
(a)

(b) Ladoo is preferred by most of the students.

1	3	5	6	6	3	5	4	1	6
2	5	3	4	6	1	5	5	6	1
1	2	2	3	5	2	4	5	5	6
5	1	6	2	3	5	2	4	1	5

Question 3.
Make a table and enter the data using tally- marks. Find the number that appeared.
(a) The minimum number of times.
(b) The maximum number of times.
(c) Find those numbers that appear an equal number of limes.
Solution.

(a) The number that appeared the minimum number of times is 4.
(b) The number that appeared the maximum number of times is 5.
(c) The numbers that appeared an equal number of times are 1 and 6.

Question 4.
Following pictograph shows the number of tractors in five villages:

Observe the pictograph and answers the following questions:
(i) Which village has the minimum number of tractors?
(ii) Which village has the maximum number of tractors?
(iii) How many more tractors village C has as compared to village B?
(iv) What is the total number of tractors in all the five villages?
Solution.
(i) Village D has the minimum number of tractors.
(ii) Village C has the maximum number of tractors.
(iii) Village C has 8-5 = 3 more tractors as compared to village B.
(iv) Total number of tractors in all the five villages = 6 +5+ 8 + 3 + 6 = 28.

Question 5.
The number of girl students in each class of a co-educational middle school is depicted by the pictograph:

Observe this pictograph and answer the following questions:
(a) Which class has the minimum number of girl students?
(b) Is the number of girls in class VI less than the number of girls in class V?
(c) How many girls are there in VII class?
Solution.
(a) Class VIII has the minimum no. of girl students.
(b) No! the number of girls in class VI is not less than the number of girls in class V.
(c) Number of girls in class VII – 3 x 4 = 12.

Question 6.
The sale of electric bulbs on different days of a week is shown below:

What can we conclude from the said pictograph?
Observe the pictograph and answer the following questions:
(a) How many bulbs were sold on Friday?
(b) On which day the maximum number of bulbs were sold?
(c) If one bulb was sold at the rate off 10, what was the total sale on Sunday?
(d) Can you find out the total sale of the week?
(e) If one big carton can hold 9 bulbs, how many cartons were needed in the given week?
Solution.
(a) Number of bulbs sold on Friday = 7×2 = 14.
(b) The maximum number of bulbs were sold on Sunday.
(c) Number of bulbs sold on Sunday
= 9 x 2=18.
∴ Total sale on Sunday
= Rs.18 x 10 = Rs. 180.
(d) Total number of bulbs sold in the week
= (6 + 8 + 4 + 5 + 7 + 4 + 9) x 2
= 43 x 2 = 86.

Hence, 10 cartons were needed in the given week.

Question 7.
In a village six fruit merchants sold the following number of fruit baskets in a particular season:

Observe this pictograph and answer the following questions:
(a) Which merchant sold the maximum number of baskets?
(b) How many fruit baskets were sold by Anwar?
(c) The merchants who have sold 600 or number of baskets are planning to buy a godown for the next season. Can you name them?
Solution.
(a) Martin sold the maximum number of baskets.
(b) 7 x 100 = 700 fruit baskets were sold by Anwar.
(c) Yes! Anwar. Martin and Ranjit Singh are planning to buy a godown for the next season.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 9 Data Handling Ex 9.1 help you. If you have any query regarding. NCERT Solutions for Class 6 Maths Chapter 9 Data Handling Ex 9.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 6 Integers Ex 6.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 6 Integers Ex 6.1.

• Integers Class 6 Ex 6.2
• Integers Class 6 Ex 6.3

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 6
Chapter Name	Integers
Exercise	Ex 6.1
Number of Questions Solved	10
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 6 Integers Ex 6.1

Question 1.
Write opposite of the following:
(a) Increase in weight
(b) 30 km north
(c) 326 BC
(d) Loss of Rs. 700
(e) 100 m above sea level.
Solution.
(a) Decrease in weight
(b) 30 km south
(c) 326 AD
(d) Gain of Rs. 700
(e) 100 m below sea level

Question 2.
Represent the following numbers as integers with appropriate signs.
(a) An airplane is flying at a height two thousand meters above the ground.
(b) A submarine is moving at depth, eight hundred meters below the sea level.
(c) A deposit of rupees two hundred.
(d) Withdrawal of rupees seven hundred.
Solution.
(a) + 2000
(b) – 800
(c) + 200
(d) – 700.

Question 3.
Represent the following numbers on a number line:
(a) + 5
(b) -10
(c) + 8
(d) -7
Solution.

Question 4.
Adjacent figure is a vertical number line, representing integers. Observe it and locate the following points :
(a) If point D is + 8, then which point is – 8?
(b) Is point G a negative integer or a positive integer?
(c) Write integers for points B and E.
(d) Which point marked on this number line has the least value?
(e) Arrange all the points in p-decreasing order of value.
Solution.

(a) F
(b) negative integer
(c) B → + 4, E → 10
(d) E
(e) D, C, B, A, O, H, G, F, E.

Question 5.
Following is the list of temperatures of five places in India, on a particular day of the year.
Solution.

Place	Temperature
Siachin	10°C below 0°C	………….
Shimla	2°C below 0°C	…………
Ahmedabad	30°C above 0°C	………….
Delhi	20°C above 0°C	………..
Srinagar	5°C below 0°C	………….

(a) Write the temperature of these places in the form of integers in the blank column.
(b) Following is the number line representing the temperature in degree Celsius.
Plot the name of the city against its temperature.
(c) Which is the coolest place?
(d) Write the names of the places whose temperature are above 10°C.
Solution.
(a)

Place	Temperature
Siachin	10°C below 0°C	– 10°C
Shimla	2°C below 0°C	– 2°C
Ahmedabad	30°C above 0°C	+ 30°C
Delhi	20°C above 0°C	+ 20°C
Srinagar	5°C below 0°C	– 5°C

(b)

(c) Siachin is the coolest place.
(d) Ahmedabad and Delhi.

Question 6.
In each of the following pairs, which number is to the right of the other on the number line?
(a) 2, 9
(b) -3,-8
(c) 0,-1
(d) – 11, 10
(e) -6,6
(f) 1,- 100
Solution.
(a) 2, 9
The number 9 is to the right of the number 2.
(b) -3,-8
The number – 3 is to the right of the number -8.
(c) 0,-1
The number 0 is to the right of the number – 1.
(d) – 11,10
The number 10 is to the right of the number -11.
(e) -6, 6
The number 6 is to the right of the number – 6.
(f) 1, -100
The number 1 is to the right of the number – 100.

Question 7.
Write all the integers between the given pairs (write them in the increasing order)
(a) 0 and – 7
(b) -4 and 4
(c) – 8 and -15
(d) – 30 and – 23.
Solution.
(a) 0 and – 7
The integers between 0 and – 7 in increasing order are – 6, – 5, – 4, – 3, – 2 and – 1.
(b) – 4 and 4
The integers between – 4 and 4 in increasing order are – 3, – 2, – 1, 0, 1, 2 and 3.
(c) – 8 and – 15
The integers between – 8 and – 15 in increasing order are – 14, – 13, – 12, – 11, – 10 and – 9.
(d) – 30 and – 23
The integers between – 30 and – 23 in increasing order are – 29, – 28, – 27, – 26, – 25 and – 24.

Question 8.
(a) Write four negative integers greater than – 20.
(b) Write four negative integers less than -10.
Solution.
(a) Four negative integers greater than 20 are – 19, – 18, – 17 and – 16.
(b) Four negative integers less than – 10 and 11,- 12,- 13 and -14

Question 9.
For the following statements write True (T) or False (F). If the statement is false, correct the state­ment.
(a) -8 is to the right of- 50 on a number line.
(b) – 100 is to the right of – 50 on a number line.
(c) A smallest negative integer is – 1.
(d) – 26 is larger than -25
Solution.
(a) True (T)
(b) False (F); – 100 is to the left of – 50 on a number line.
(c) False (F); Greatest negative integer is – 1.
(d) False (F); – 26 is smaller than – 25.

Question 10.
Draw a number line and answer the following:
(a) Which number will we reach if we move 4 numbers to the right of-2?
(b) Which number will we reach if we move 5 numbers to the left of 1?
(c) If we are at -8 on the number line, in which direction should we move to reach -13?
(d) If we are at-6 on the number line, in which direction should we move to reach -l?
Solution.
(a) We will reach number 2.
(b) We will reach the number – 4.
(c) We should move in the left direction.
(d) We should move in the right direction

 

We hope the NCERT Solutions for Class 6 Maths Chapter 6 Integers Ex 6.1 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 6 Integers Ex 6.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.1.

• Basic Geometrical Ideas Class 6 Ex 4.2
• Basic Geometrical Ideas Class 6 Ex 4.3
• Basic Geometrical Ideas Class 6 Ex 4.4
• Basic Geometrical Ideas Class 6 Ex 4.5
• Basic Geometrical Ideas Class 6 Ex 4.6

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 4
Chapter Name	Basic Geometrical Ideas
Exercise	Ex 4.1
Number of Questions Solved	6
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.1

Question 1.
Use the figure to name:
(a) Five points
(b) A line
(c) Four rays
(d) Five line segments.
Solution :
(a) O, B, C. D, E

Question 2.
Name the line given in all possible (twelve) ways, choosing only two letters at a time from the four given.
Solution :

Question 3.
Use the figure to name:
(a) The line containing point E.
(b) The line passing through A.
(c) The line on which O lies
(d) Two pairs of intersecting lines.

Solution :
(a) \(\overleftrightarrow { AE }\), etc.
(b) \(\overleftrightarrow { AE }\), etc.
(c) \(\overleftrightarrow { CO } or\overleftrightarrow { OC }\)
(d) \(\overleftrightarrow { CO, } \overleftrightarrow { AE } ;\overleftrightarrow { AE } ,\overleftrightarrow { EF }.\)

Question 4.
How many lines can pass through
(a) one given point?
(b) two given points?
Solution :
(a) Countless lines can pass through one given point.
(b) One and only one line can pass through two given points.

Question 5.
Draw a rough figure and label suitably in each of the following cases :
(a) Point P lies on \(\bar { AB } \)
(b) \(\overleftrightarrow { XY }\) and \(\overleftrightarrow { PQ }\) intersect at M.
(c) Line contains E and F but not D.
(d) \(\bar { Op } \) and \(\bar { OQ } \) meet at O.
Solution :
(a)

(b)

(c)

(d)

Question 6.
Consider the following figure of line \(\bar { MN } \) Say whether following statements are true or false in context of the given figure.
(a) Q, M, O, N, P are points on the line \(\bar { MN } \)
(b) M, O, N are points on a line segment \(\bar { MN } \).
(c) M and N are end points of line segment \(\bar { MN } \).

(d) O and N are end points of line segment \(\bar { OP } \).
(e) M is one of the end points of line segment \(\bar { QO } \).
(f) M is point on ray \(\overrightarrow { OP }\).
(g) Ray \(\overrightarrow { OP }\) is different from ray \(\overrightarrow { QP }\).
(h) Ray \(\overrightarrow { OP }\) is same as ray \(\overrightarrow { OM }\).
(i) Ray \(\overrightarrow { OM }\) is not opposite to ray \(\overrightarrow { OP }\).
(j) O is not an initial point of \(\overrightarrow { OP }\).
(k) N is the initial point of \(\overrightarrow { NP }\) and \(\overrightarrow { NM }\).
Solution :
(a) True
(b) True
(c) True
(d) False
(e) False
(f) False
(g) True
(h) False
(i) False
(j) False
(k) True.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.1 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 4 Basic Geometrical Ideas Ex 4.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.1.

• Whole Numbers Class 6 Ex 2.2
• Whole Numbers Class 6 Ex 2.3

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 2
Chapter Name	Whole Numbers
Exercise	Ex 2.1
Number of Questions Solved	8
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.1

Question 1.
Write the next three natural numbers after 10999.
Solution :
The next three natural numbers after 10999 are 11000, 11001 and 11002.

Question 2.
Write the three whole numbers occurring just before 10001.
Solution :
The three whole numbers occurring just before 10001 are 10000, 9999 and 9998.

Question 3.
Which is the smallest whole number?
Solution :
0 is the smallest whole number.

Question 4.
How many whole numbers are there between 32 and 53?
Solution :
There are 20 whole numbers between 32 and 53.
These are 33, 34, 35, 36, 37, 38, 39, 40, 41, 42,43, 44, 45, 46, 47,48, 49, 50, 51 and 52.

Question 5.
Write successor of:
(a) 2440701
(b) 100199
(c) 1099999
(d) 2345670
Solution :
(a) The successor of 2440701 is 24,40,702.
(b) The successor of 100199 is 1,00,200.
(c) The successor of 1099999 is 11,00,000.
(d) The successor of 2345670 is 23,45,671.

Question 6.
Write the predecessor of:
(a) 94
(b) 10000
(c) 208090
(d) 7654321.
Solution :
(a) The predecessor of 94 is 93.
(b) The predecessor of 10000 is 9,999.
(c) The predecessor of 208090 is 2,08,089.
(d) The predecessor of 7654321 is 76,54,320.

Question 7.
In each of the following pairs of numbers, the state which the whole number is on the left of the other number on the number line. Also write them with the appropriate sign (>, <) between them.
(a) 530, 503
(b) 370, 307
(c) 98765, 56789
(d) 9830415, 10023001.
Solution :
(a) The whole number 503 is on the left of the whole number 530 on the number line. So, 503 < 530.
(b) The whole number 307 is on the left of the whole number 370 on the number line. So, 307 < 370.
(c) The whole number 56789 is on the left of the whole number 98765 on the number line. So, 56789 < 98765. ;
(d) The whole number 9830415 is on the left of the whole number 10023001 on the number line. So, 98,30,415 < 100,23,001.

Question 8.
Which of the following statements are true (T) and which are false (F)?
(a) Zero is the smallest natural number.
(b) 400 is the predecessor of 399.
(c) Zero is the smallest whole number.
(d) 600 is the successor of 599.
(e) All natural numbers are whole numbers.
(f) All whole numbers are natural numbers,
(g) The predecessor of a two digit number is never a single digit number.
(h) 1 is the smallest whole number.
(i) The natural number 1 has no predecessor,
(j) The whole number 1 has no predecessor.
(k) The whole number 13 lies between 11 and 12.
(l) The whole number 0 has no predecessor.
(m) The successor of a two digit number is always a two digit number.
Solution :
(a) This statement is false (F).
(b) This statement is false (F).
(c) This statement is true (T).
(d) This statement is true (T).
(e) This statement is true (T).
(f) This statement is false (F).
(g) This statement is false (F).
(h) This statement is false (F).
(i) This statement is true (T).
(j) This statement is false (F).
(k) This statement is false (F).
(l) This statement is true (T).
(m) This statement is false (F).

 

We hope the NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.1 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 2 Whole Numbers Ex 2.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.1.

• Knowing Our Numbers Class 6 Ex 1.2
• Knowing Our Numbers Class 6 Ex 1.3

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 1
Chapter Name	Knowing Our Numbers
Exercise	Ex 1.1
Number of Questions Solved	4
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.1

Question 1.
Fill in the blanks:
(a) 1 lakh = …………………. ten thousand.
(b) 1 million = …………………. hundred thousand.
(c) 1 crore = …………………. ten lakh.
(d) 1 crore = …………………. million.
(e) 1 million = …………………. lakh.
Solution :
(a) 1 lakh = ten ten thousand.
(b) 1 million = ten hundred thousand.
(c) 1 crore = ten ten lakh
(d) 1 crore = ten million
(e) 1 million = ten lakh

Question 2.
Place commas correctly and write the numerals :
(a) Seventy-three lakh seventy’ five thousand three hundred seven.
(b) Nine crore five lakh forty-one.
(c) Seven crores fifty-two lakh twenty-one thousand three hundred two.
(d) Fifty-eight million four hundred twenty-three thousand two hundred two.
(e) Twenty-three lakh thirty thousand ten.
Solution :
(a) 73,75,307
(b) 9.05.00.041
(c) 7,52.21,302
(d) 58.423.202
(e) 23.30,010.

Question 3.
Insert commas suitably and write the names according to Indian System of Numeration :
(a) 87595762
(b) 8546283
(c)99900046
(d) 98432701.
Solution :
(a) 8, 75, 95, 762. Eight crores seventy-five lakh ninety-five thousand seven hundred and sixty-two.
(b) 85, 46, 283. Eighty-five lakh forty-six thousand two hundred and eighty-three.
(c) i 9, 99, 00, 046. Nine crore ninety-nine lakh and forty-six.
(d) 9, 84, 32, 701. Nine crore eighty-four lakh thirty-two thousand seven hundred and one.

Question 4.
Insert commas suitably and write the names according to International System of Numeration :
(a) 78921092
(b) 7452283
(c) 99985102
(d) 48049831.
Solution :
(a) 78,921,092. Seventy-eight million nine hundred twenty-one thousand and ninety-two.
(b) 7.452,283. Seven million four hundred fifty-two thousand two hundred and eighty-three.
(c) 99. 985. 102. Ninety-nine million nine hundred eighty-five thousand one hundred and two.
(d) 48. 049. 831. Forty-eight million forty-nine thousand eight hundred and thirty-one.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.1 help you. If you have any query regarding NCERT Solutions for Class 6 Maths Chapter 1 Knowing Our Numbers Ex 1.1, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 6 Maths Chapter 11 Algebra Ex 11.1 are part of NCERT Solutions for Class 6 Maths. Here we have given NCERT Solutions for Class 6 Maths Chapter 11 Algebra Ex 11.1.

• Algebra Class 6 Ex 11.2
• Algebra Class 6 Ex 11.3
• Algebra Class 6 Ex 11.4
• Algebra Class 6 Ex 11.5

Board	CBSE
Textbook	NCERT
Class	Class 6
Subject	Maths
Chapter	Chapter 11
Chapter Name	Algebra
Exercise	Ex 11.1
Number of Questions Solved	1
Category	NCERT Solutions

NCERT Solutions for Class 6 Maths Chapter 11 Algebra Ex 11.1

Question 1.
Find the rule which gives the number of matchsticks required to make the following matchstick patterns. Use a variable to write the rule.
(a) A pattern of letter T as
(b) A pattern of letter Z as
(c) A pattern of letter U as
(d) A pattern of letter V as
(e) A pattern of letter E as
(f) A pattern of letter S as
(g) A pattern of letter A as
Solution.
(a) Number of matchsticks required = 2n
(b) Number of matchsticks required = 3n
(c) Number of matchsticks required = 3n
(d) Number of matchsticks required = 2n
(e) Number of matchsticks required = 5n
(f) Number of matchsticks required = 5n
(g) Number of matchsticks required = 6

Question 2.
We already know the rule for the pattern of letters L, C and F. Some of the letters from Q. 1 (given above) give us the same rule as that given by L. Which are these? Why does this happen?
Solution.
These letters are T and V. This happens since the number>of matchsticks require,d in each of them is 2.

Question 3.
Cadets are marching in a parade. There are 5 cadets in a row. What is the rule, which gives the number of cadets, given the number of rows? (Use n for the number of rows.)
Solution.
The number of cadets = 5n.

Question 4.
If there are 50 mangoes in a box, how will you write the total number of mangoes in terms of the number of boxes? (Use b for the number of boxes.)
Solution.
Total number of mangoes = 50b.

Question 5.
The teacher distributes 5 pencils per student. Can you tell how many pencils are needed, given the number of students? (Use for the number of students.)
Solution.
Number of pencils needed = 5s

Question 6.
A bird flies 1 kilometer in one minute. Can you express the distance covered by the bird in tertns of its flying time in minutes? (Use t for flying time in minutes.)
Solution.
Yes! / kilometers
The bird flies in one minute = 1 kilometer
The bird flies in / minutes = 1 x t kilometers
= kilometers

Question 7.
Radha is drawing a dot Rangoli (a beautified pattern of lines joining dots with chalk powder. She has 9 dots in a row. How many dots will her Rangoli have for r rows? How many dots are there if there are 8 rows? If there are 10 rows?

Solution.
∵ Number of dots in 1 row = 9
∴ Number of dots in r rows = 9 x r=9r
Number of dots in 8 rows = 9 x 8 = 72
Number of dots in 10 row = 9 x 10 = 90

Question 8.
Leela is Radha’s younger sister. Leela is 4 years younger than Radha. Can you write Leela’s age in terms of Radha’s age? Take Radha’s age to be x years.
Solution.
Yes! we can write Leela’s age in terms of Radha’s age.
Age of Radha = x years
∵ Leela is 4 years younger than Radha.
∴ Age of Leela = (x – 4) years

Question 9.
Mother has made laddus. She gives some laddus to guests and family members; still, 5 laddus remain. If the number of laddus mother gave away is l, how many laddus did she make?
Solution.
Number of laddus given away to guests and family members = l
Number of laddus remained = 5
∴ Number of laddus she made = 1 + 5

Question 10.
Oranges are to be transferred from larger boxes into smaller boxes. When a large box is emptied, the oranges from it fill two smaller boxes and still, 10 oranges remain, outside. If the number of oranges in a small box is taken to be x, what is the number of oranges in the larger box?
Solution.
Let the number of oranges in a smaller box box
∴ Number of oranges in two smaller boxes = 2x
Number of oranges remained outside = 10
∴ Number of oranges in the larger box = 2x+ 10

Question 11.
(a) Look at the following matchstick pattern of squares (figure). The squares are not separate. Two neighboring squares have a common matchstick. Observe the pattern and find the rule that gives the number of matchsticks in terms of the number of squares.
(Hint: If you remove the vertical stick at the end, you will get a pattern of Cs.)

(b) Figure gives a matchstick pattern of triangles. Av in Exercise 11(a) above, find the general rule that gives the number of matchsticks in terms of the number of triangles.


Solution.
(a)

 Rule: Number of matchsticks required = 3x + I
where x is the number of squares.

(b)

Rule: Number of matchsticks required = 2x + 1,
where x is the number of triangles.

 

We hope the NCERT Solutions for Class 6 Maths Chapter 11 Algebra Ex 11.1 help you. If you have any query regarding. NCERT Solutions for Class 6 Maths Chapter 11 Algebra Ex 11.1, drop a comment below and we will get back to you at the earliest.