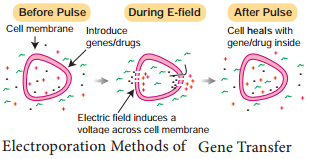
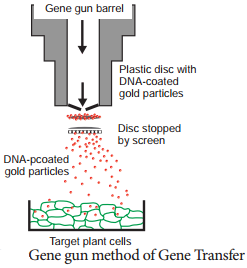
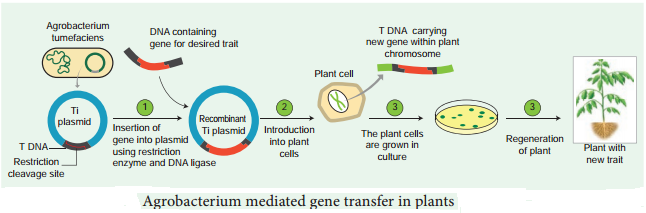
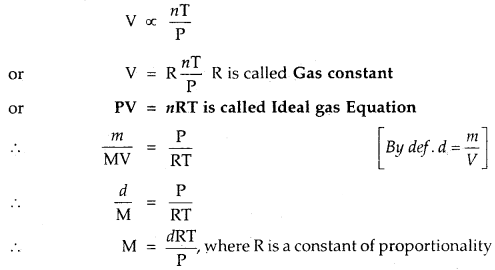
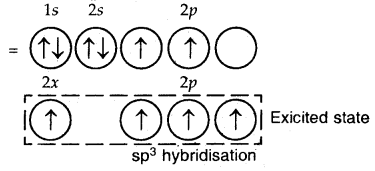
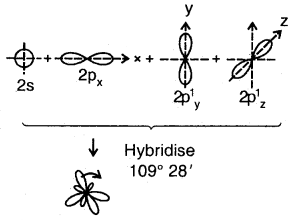
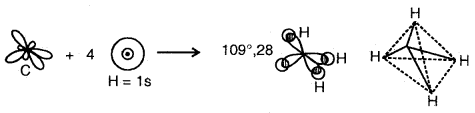
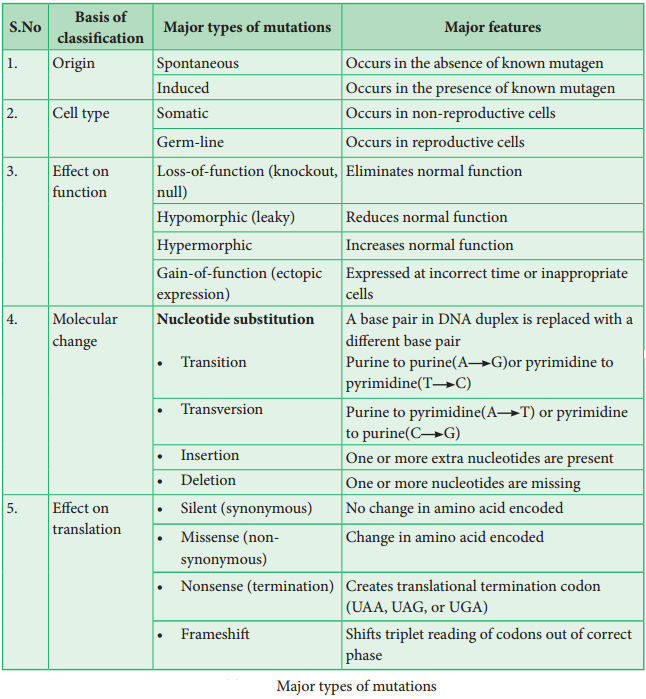
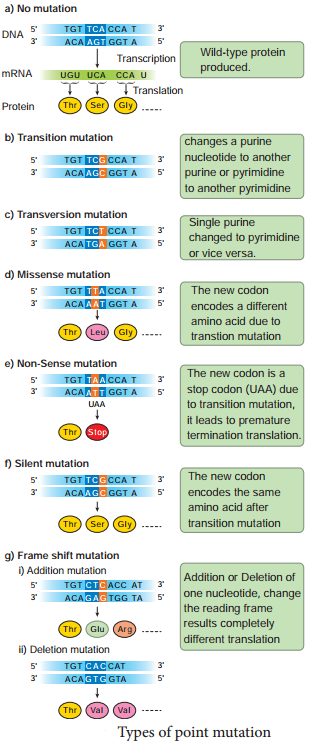
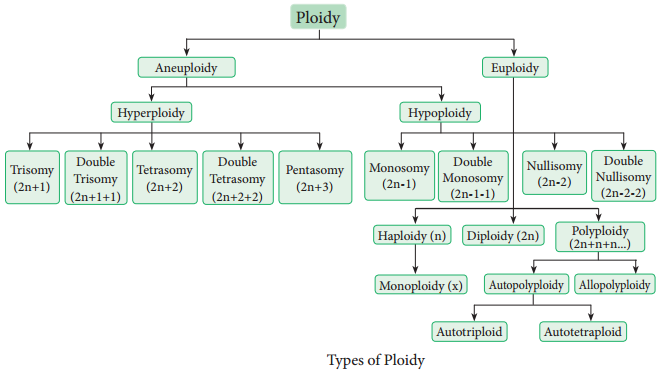
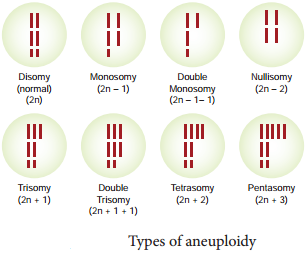
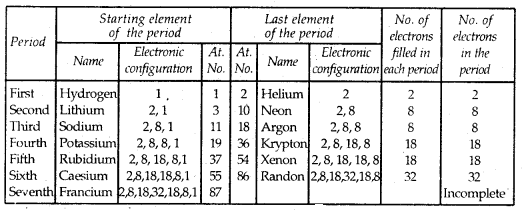
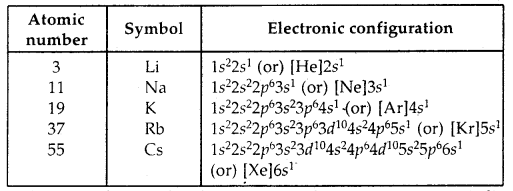
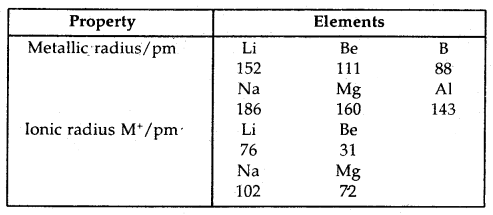
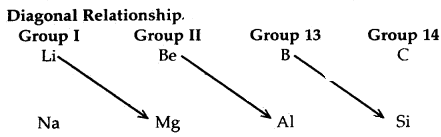
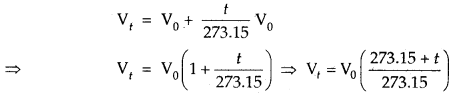Learninsta presents the core concepts of Biology with high-quality research papers and topical review articles.
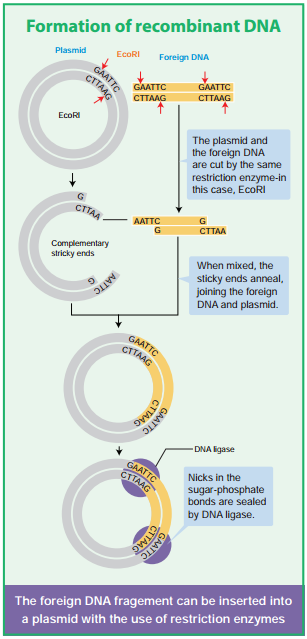
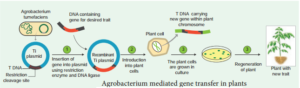
Screening For Recombiants
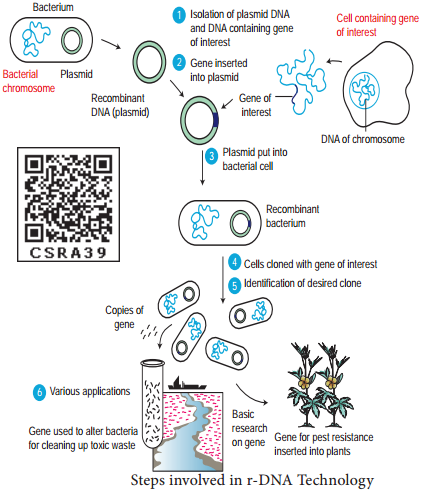
After the introduction of r-DNA into a suitable host cell, it is essential to identify those cells which have received the r-DNA molecule. This process is called screening. The vector or foreign DNA present in recombinant cells expresses the characters, while the non-recombinants do not express the characters or traits. For this some of the methods are used and one such method is Blue-White Colony Selection method.
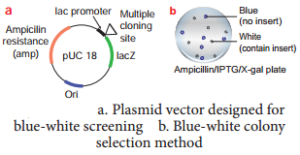
Insertional Inactivation – BlueWhite Colony Selection Method
It is a powerful method used for screening of recombinant plasmid. In this method, a reporter gene lacZ is inserted in the vector. The lacZ encodes the enzyme β-galactosidase and contains several recognition sites for restriction enzyme.
β-galactosidase breaks a synthetic substrate called X-gal (5-bromo-4-chloro-indolyl-β-D-galacto-pyranoside) into an insoluble blue coloured product. If a foreign gene is inserted into lacZ, this gene will be inactivated. Therefore, no-blue colour will develop (white) because β-galactosidase is not synthesized due to inactivation of lacZ.
Therefore, the host cell containing r-DNA form white coloured colonies on the medium contain X-gal, whereas the other cells containing non-recombinant DNA will develop the blue coloured colonies.
On the basis of colony colour, the recombinants can be selected.
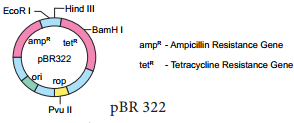
Antibiotic resistant markers
An antibiotic resistance marker is a gene that produces a protein that provides cells with resistance to an antibiotic. Bacteria with transformed DNA can be identifid by growing on a medium containing an antibiotic. Recombinants will grow on these media as they contain genes encoding resistance to antibiotics such as ampicillin, chloro amphenicol, tetracycline or kanamycin, etc., while others may not be able to grow in these media, hence it is considered useful selectable marker.
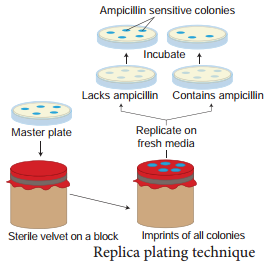
Replica plating technique
A technique in which the pattern of colonies growing on a culture plate is copied. A sterile filter plate is pressed against the culture plate and then lifted. Then the filter is pressed against a second sterile culture plate. This results in the new plate being infected with cell in the same relative positions as the colonies in the original plate. Usually, the medium used in the second plate will differ from that used in the first. It may
include an antibiotic or exclude a growth factor. In this way, transformed cells can be selected.
Molecular Techniques – Isolation of Genetic Material and Gel Electrophoresis
Electrophoresis is a separating technique used to separate diffrent biomolecules with positive and negative charges.
Principle
By applying electricity (DC) the molecules migrate according to the type of charges they have. The electrical charges on different molecules are variable.

Agarose GEL Electrophoresis
It is used mainly for the purifiation of specific DNA fragments. Agarose is convenient for separating DNA fragments ranging in size from a few hundred to about 20000 base pairs. Polyacrylamide is preferred for the purifiation of smaller DNA fragments. The gel is complex network of polymeric molecules.
DNA molecule is negatively charged molecule – under an electric field DNA molecule migrates through the gel. The electrophoresis is frequently performed with marker DNA fragments of known size which allow accurate size determination of an unknown DNA molecule by interpolation. The advantages of agarose gel electrophoresis are that the DNA bands can be readily detected at high sensitivity.
The bands of DNA in the gel are stained with the dye Ethidium Bromide and DNA can be detected as visible florescence illuminated in UV light will give orange florescence, which can be photographed.
Nucleic Acid Hybridization Blotting Techniques
Blotting techniques are widely used analytical tools for the specifi identification of desired DNA or RNA fragments from larger number of molecules. Blotting refers to the process of immobilization of sample nucleic acids or solid support (nitrocellulose or nylon membranes.) The blotted nucleic acids are then used as target in the hybridization experiments for their specific detection.
Types of Blotting Techniques
Southern Blotting:
The transfer of DNA from agarose gels to nitrocellulose membrane.
Northern Blotting:
The transfer of RNA to nitrocellulose membrane.
Western Blotting:
Electrophoretic transfer of Proteins to nitrocellulose membrane.
Southern Blotting Techniques – DNA:
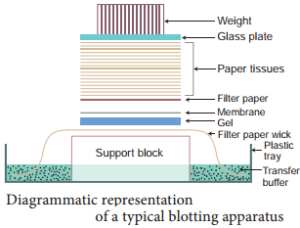
The transfer of denatured DNA from Agarose gel to Nitrocellulose Blotting or Filter Paper technique was introduced by Southern in 1975 and this technique is called Southern Blotting Technique.
Steps
The transfer of DNA from agarose gel to nitrocellulose filter paper is achieved by Capillary Action. A buffer Sodium Saline Citrate (SSC) is used, in which DNA is highly soluble, it can be drawn up through the gel into the Nitrocellulose membrane.
By this process ss-DNA becomes ‘Trapped’ in the membrane matrix. This DNA is hybridized with a nucleic acid and can be detected by autoradiography.
Autoradiography – A technique that captures the image formed in a photographic emulsion due to emission of light or radioactivity from a labelled component placed together with unexposed film.
Northern Blot
It was found that RNA is not binding to cellulose nitrate. Therefore, Alwin et al. (1979) devised a procedure in which RNA bands are transferred from the agarose gel into nitrocellulose filter paper. This transfer of RNA from gel to special filter paper is called Northern Blot hybridization. The filter paper used for Northern blot is Amino Benzyloxymethyl Paper which can be prepared from Whatman 540 paper.
Western Blot
Refers to the electrophoretic transfer of proteins to blotting papers. Nitrocellulose filter paper can be used for western blot technique. A particular protein is then identified by probing the blot with a radio-labelled antibody which binds on the specific protein to which the antibody was prepared.
Bioassay for Target Gene Effect
Target gene is target DNA, foreign DNA, passenger DNA, exogenous DNA, gene of interest or insert DNA that is to be either cloned or specifially mutated. Gene targeting experiments have been targeting the nuclei and this leads to ‘gene knock-out’. For this purpose, two types of targeting vectors are used. They are insertion vectors and replacement or transplacement vectors.
Insertion vectors are entirely inserted into targeted locus as the vectors are linearized within the homology region. Initially, these vectors are circular but during insertion, become linear. It leads to duplication of sequences adjacent to selectable markers.

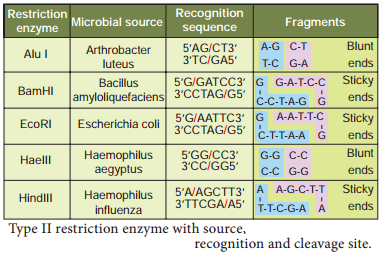
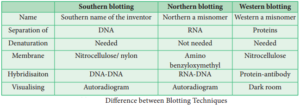
Differences between Blotting Techniques
The replacement vector has the homology region and it is co-linear with target. This vector is linearized prior to transfection outside the homology region and then consequently a crossing over occurs to replace the endogenous DNA with the incoming DNA.
Genome Sequencing and Plant Genome Projects
The whole complement of genes that determine all characteristics of an organism is called genome. Which may be nuclear genome, mitochondrial genome or plastid genome. Genome of many plants contain both functional and non-expressive DNA proteins.
Genome project refers to a project in which the whole genome of plant is analysed using sequence analysis and sequence homology with other plants. Such genome projects have so far been undertaken in Chlamydomonas(algae), Arabidopsis thaliana, rice and maize plants. Genome content of an organism is expressed in terms of number of base pairs or in terms of the content of DNA which is expressed as c-value.
Evolutionary pattern assessed using DNA
In recent years the evolutionary relationship between different plant taxa is assessed using DNA content as well as the similarities and differences in the DNA sequence (sequence homology). Based on such analysis the taxa and their relationship are indicated in cladogram. Which will show the genetic distance between two taxa. It also shows antiquity or modernity of any taxon with respect to one another (See also Unit-2, Chapter-5 of XI Std.)
Genome editing and CRISPR – Cas9
Genome editing or gene editing is a group of technologies that has the ability to change an organism’s DNA. These technologies allow genetic material to be added, removed, or altered at particular locations in the genome. Several approaches to genome editing have been developed. A recent one is known as CRISPR-Cas9, which is short form of Clustered Regularly Interspaced Short Palindromic Repeats and CRISPR-associated protein 9.
The CRISPR-Cas9 system has generated a lot of excitement in the scientific community because it is faster, cheaper, more accurate, and more efficient than other existing genome editing methods. Rice, was among the first plants to be used to demonstrate the feasibility of CRISPR mediated targeted mutagenesis and gene replacement.
The gene editing tool CRISPR can be used to make hybrid rice plants that can clone their seed. Imtiyaz Khand and Venkatesan Sundaresan and colleagues reported in a new study which clearly shows one can re-engineer rice to switch it from a sexual to an asexual mode.
RNA Interference (RNAi)
All characters of organism are the result of expression of different genes which are regions of nuclear DNA. This expression involves transcription and translation. Transcription refers to the copying of genetic information from one strand of the DNA (called sense strand) by RNA. This RNA, as soon as it formed cannot be straight away sent to the cytoplasm to undertake the process of translation.
It has to be edited and made suitable for translation which brings about protein synthesis. One of the main
items removed from the RNA strand are the introns. All these changes before translation normally take place whereby certain regions of DNA are silence. However, there is an (RNAi) pathway. RNA interference is a biological process in which RNA molecules inhibit gene expression or translation. This is done by neutralising targetd mRNA molecules.
A simplified model for the RNAi pathway is based on two steps, each involving ribonuclease enzyme. In the first step, the trigger RNA (either dsRNA or miRNA primary transcript) is processed into a short interfering RNA (siRNA) by the RNase II enzymes called Dicer and Drosha. In the second step, siRNAs are loaded into the effector complex RNA-induced silencing complex (RISC). The siRNA is unwound during RISC assembly and the single-stranded RNA hybridizes with mRNA target. This RNAi is seen in plant feeding nematodes.