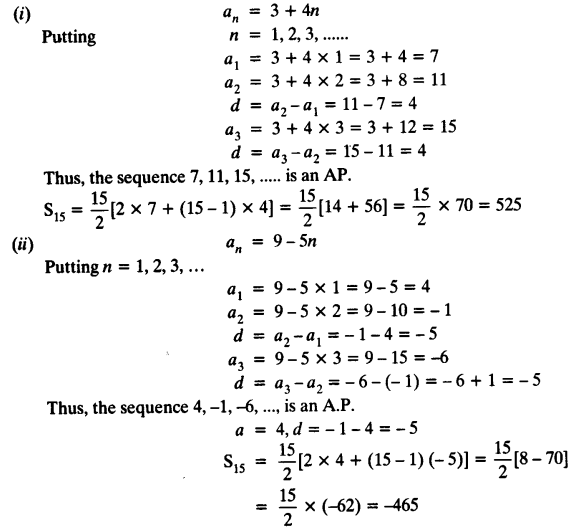
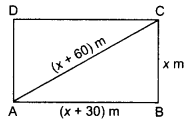
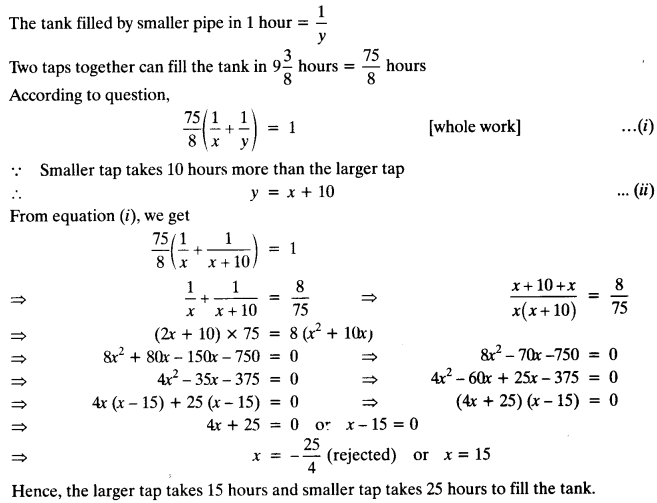
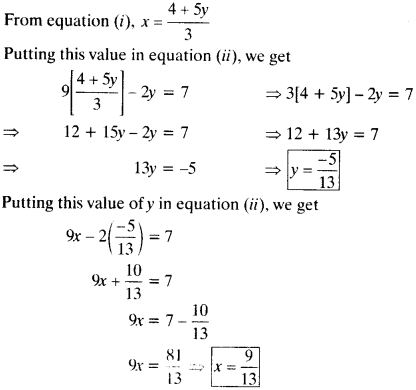
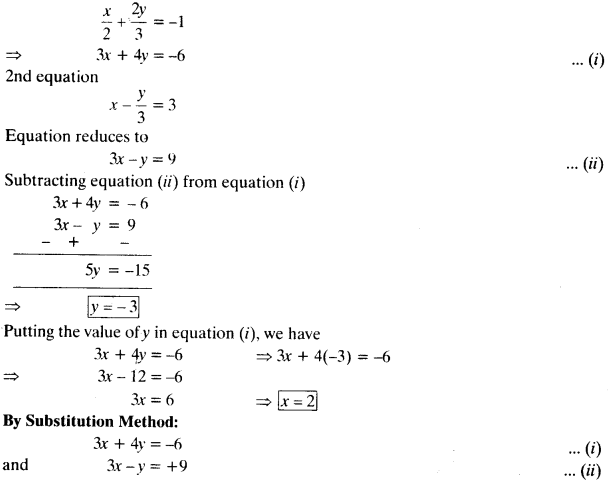
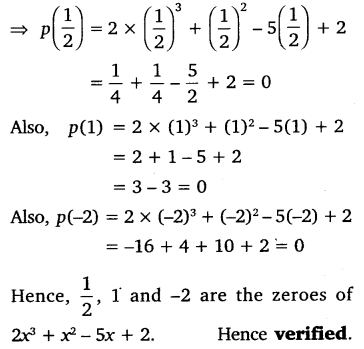
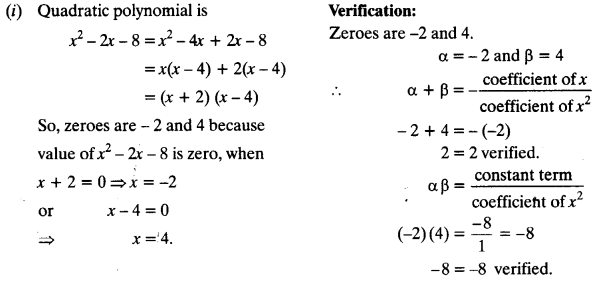
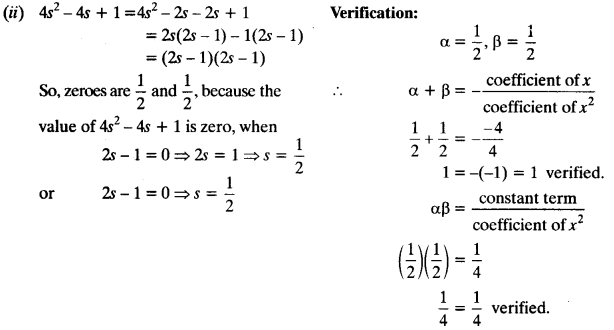
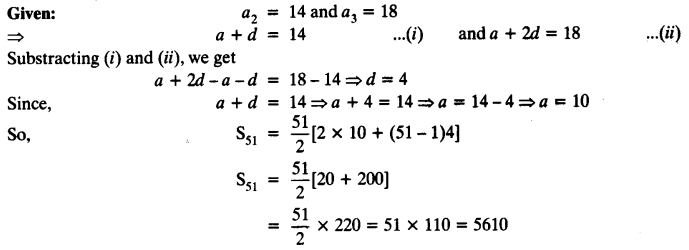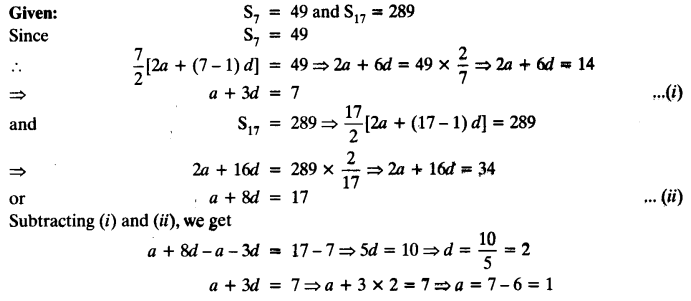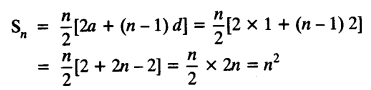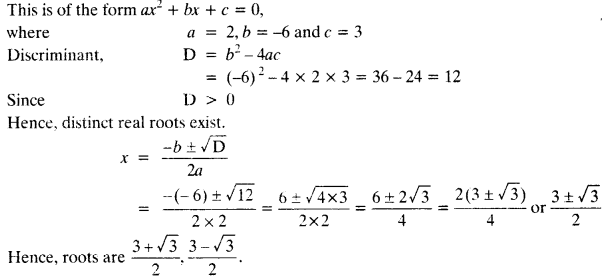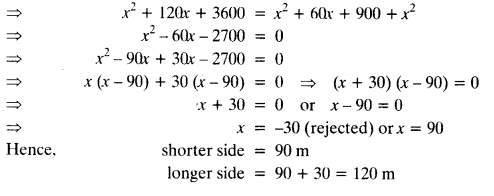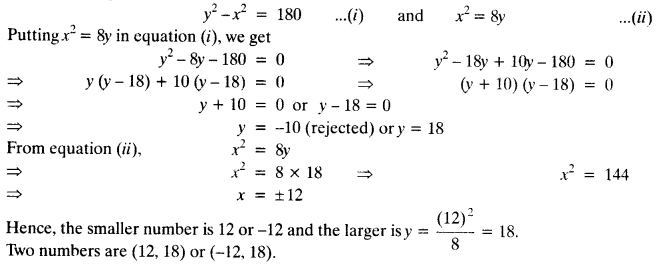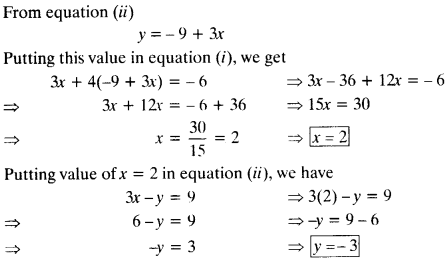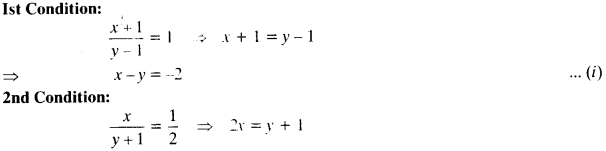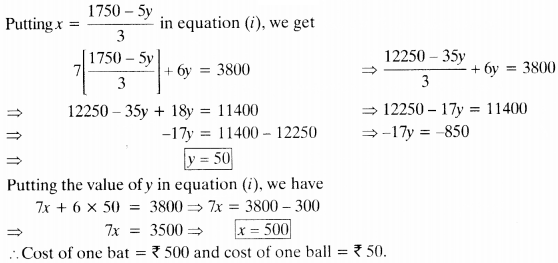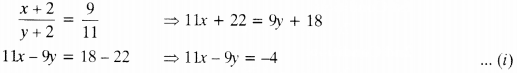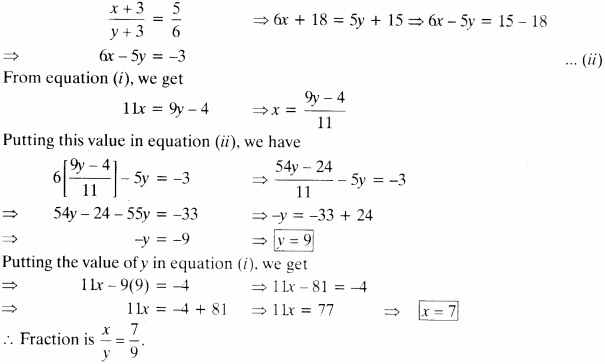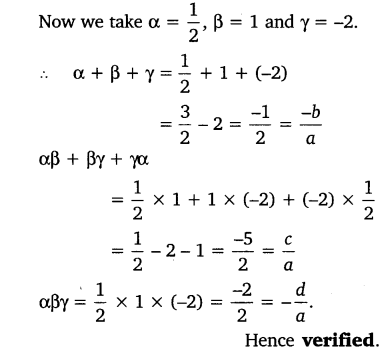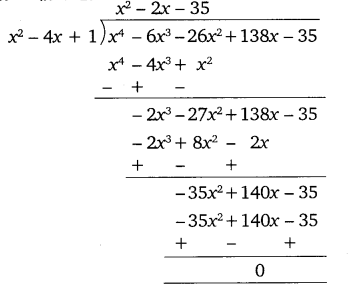Dattu – Page 19 – NCERT MCQ

NCERT Solutions for Class 9 Maths Chapter 1 Number Systems Ex 1.2

March 14, 2023

NCERT Solutions for Class 9 Maths Chapter 1 Number Systems Ex 1.2 are part of NCERT Solutions for Class 9 Maths. Here we have given NCERT Solutions for Class 9 Maths Chapter 1 Number Systems Ex 1.2.

Board	CBSE
Textbook	NCERT
Class	Class 9
Subject	Maths
Chapter	Chapter 1
Chapter Name	Number Systems
Exercise	Ex 1.2
Number of Questions Solved	4
Category	NCERT Solutions

NCERT Solutions for Class 9 Maths Chapter 1 Number Systems Ex 1.2

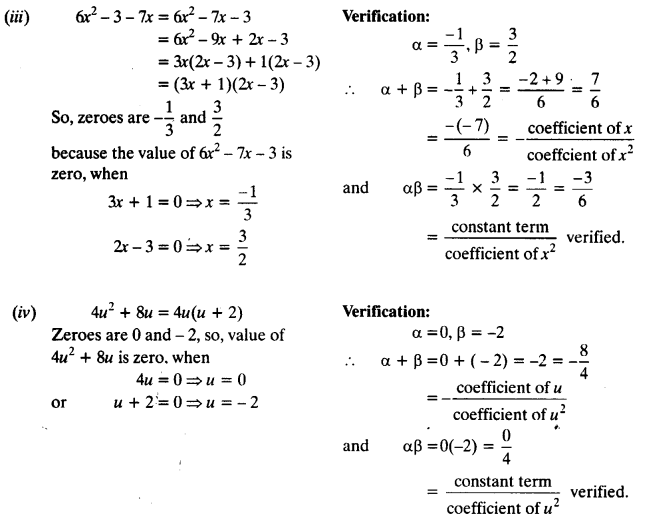
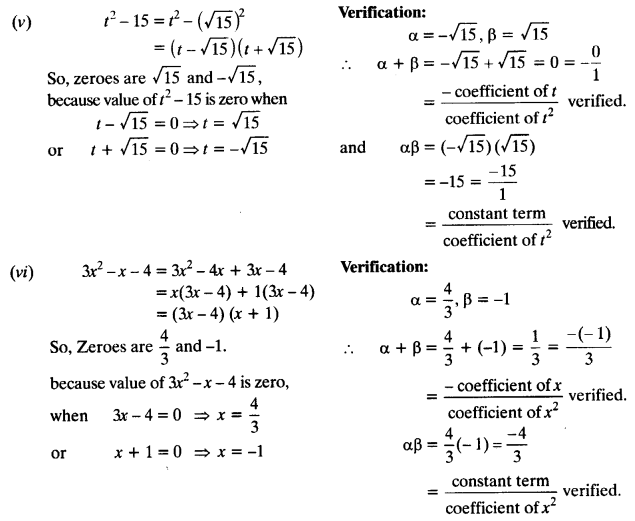
Question 1.
State whether the following statements are true or false. Justify your answers.
(i) Every irrational number is a real number.
(ii) Every point on the number line is of the form √m , where m is a natural number.
(iii) Every real number is an irrational number.
Solution:
(i) True (∵ Real numbers = Rational numbers + Irrational numbers.)
(ii) False (∵ no negative number can be the square root of any natural number.)
(iii) False (∵ rational numbers are also present in the set of real numbers.)

Question 2.
Are the square roots of all positive integers irrational? If not, give an example of the square root of a number that is a rational number.
Solution:
No, the square roots of all positive integers are not irrational.
e.g., √l6 = 4
Here, ‘4’ is a rational number.

Question 3.
Show how √5 can be represented on the number line.
Solution:
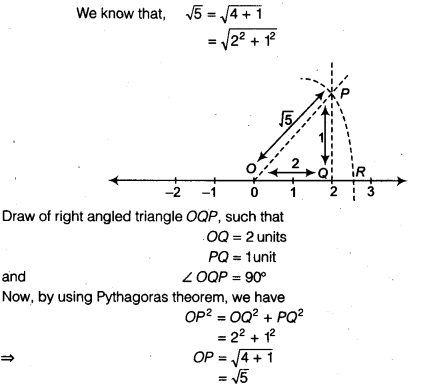
NCERT Solutions for Class 9 Maths Chapter 1 Number Systems Ex 1.2 img 1
Now, take O as centre OP = √5 as radius, draw an arc, which intersects the line at point R. .
Hence, the point R represents √5.

Question 4.
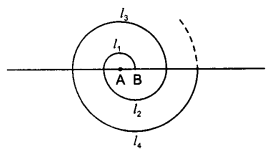
Classroom activity (constructing the ‘square root spiral’).
Solution:
Take a large sheet of paper and construct the ‘square root spiral’ in the following fashion. Start with a point O and draw a line segment OP₁, of unit lengths Draw a line segment P₁, P₂ perpendicular to OP₁ of unit length (see figure).
NCERT Solutions for Class 9 Maths Chapter 1 Number Systems Ex 1.2 img 2
Now, draw a line segment P₂P₃ perpendicular to OP₂. Then draw a line segment P₃P₄ perpendicular to OP₃. Continuing in this manner, you can get the line segment P_n-1 P_n by drawing a line segment of unit length perpendicular to
OP_n-1. In this manner, you will have created the points P₂, P₃,…… P_n,….. and
joined them to create a beautiful spiral depicting √2,√3,√4,……

We hope the NCERT Solutions for Class 9 Maths Chapter 1 Number Systems Ex 1.2, help you. If you have any query regarding NCERT Solutions for Class 9 Maths Chapter 1 Number Systems Ex 1.2, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2

March 13, 2023

NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2 are part of NCERT Solutions for Class 10 Maths. Here we have given NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 6
Chapter Name	Triangles
Exercise	Ex 6.2
Number of Questions Solved	10
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2

Question 1.
In the given figure (i) and (ii), DE || BC. Find EC in (i) and AD in (ii).
NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2 1
Solution:

Question 2.
E and F are points on the sides PQ and PR respectively of a ∆PQR. For each of the following cases, state whether EF || QR:
(i) PE = 3.9 cm, EQ = 3 cm, PF = 3.6 cm and FR = 2.4 cm
(ii) PE = 4 cm, QE = 4.5 cm, PF = 8 cm and RF = 9 cm
(iii) PQ = 1.28 cm, PR = 2.56 cm, PE = 0.18 cm and PF = 0.36 cm
Solution:
NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2 3

Question 3.
In the given figure, if LM || CB and LN || CD.
NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2 4

Solution:

Question 4.
In the given figure, DE || AC and DF || AE.
NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2 8

Solution:

Question 5.
In the given figure, DE || OQ and DF || OR. Show that EF || QR.
NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2 11
Solution:

Question 6.
In the given figure, A, B and C are points on OP, OQ and OR respectively such that AB || PQ and AC || PR. Show that BC || QR.
NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2 14
Solution:

Question 7.
Using B.P.T., prove that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side.
Solution:
Given: A ∆ABC in which D is the mid-point of AB and DE || BC
NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2 17

Question 8.
Using converse of B.P.T., prove that the line joining the mid-points of any two sides of a triangle is parallel to the third side.
Solution:
Given: A ΔABC in which D and E are mid-points of sides AB and AC respectively.
To Prove: DE || BC
NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2 18

Question 9.
ABCD is a trapezium in which AB || DC and its diagonals intersect each other at the
Solution:
NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2 20

Question 10.
The diagonals of a quadrilateral ABCD intersect each other at the point O such that \(\frac { AO }{ BO }\) = \(\frac { CO }{ DO }\) Show that ABCD is a trapezium.
Solution:
NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2 22

We hope the NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2 help you. If you have any query regarding NCERT Solutions for Class 10 Maths Chapter 6 Triangles Ex 6.2, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3

March 13, 2023

NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 are part of NCERT Solutions for Class 10 Maths. Here we have given NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 5
Chapter Name	Arithmetic Progressions
Exercise	Ex 5.3
Number of Questions Solved	20
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3

Question 1.
Find the sum of the following APs:
(i) 2, 7, 12,…… to 10 terms.
(ii) -37, -33, -29, …… to 12 terms.
(iii) 0.6, 1.7, 2.8, ……, to 100 terms.
(iv) \(\frac { 1 }{ 15 }\), \(\frac { 1 }{ 12 }\), \(\frac { 1 }{ 10 }\), …….., to 11 terms.
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 1

Question 2.
Find the sums given below:
(i) 7 + 10\(\frac { 1 }{ 2 }\) + 14 + … + 84
(ii) 34 + 32 + 30 + … + 10
(iii) -5 + (-8) + (-11) + ….. + (-230)
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 3

Question 3.
In an AP:
(i) given a = 5, d = 3, a_n = 50, find n and S_n.
(ii) given a = 7, a₁₃ = 35, find d and S₁₃.
(iii) given a₁₂ = 37, d = 3, find a and S₁₂.
(iv) given a₃ = -15, S₁₀ = 125, find d and a₁₀.
(v) given d = 5, S₉ = 75, find a and a₉.
(vi) given a = 2, d = 8, S_n = 90, find n and a_n.
(vii) given a = 8, a_n = 62, S_n = 210, find n and d.
(viii) given a_n = 4, d = 2, S_n = -14, find n and a.
(ix) given a = 3, n = 8, S = 192, find d.
(x) given l = 28, S = 144, and there are total 9 terms. Find a.
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 4

Question 4.
How many terms of AP: 9, 17, 25, … must be taken to give a sum of 636?
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 8

Question 5.
The first term of an AP is 5, the last term is 45 and the sum is 400. Find the number of terms and the common difference.
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 9

Question 6.
The first and the last terms of an AP are 17 and 350 respectively. If the common difference is 9, how many terms are there and what is their sum?
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 10

Question 7.
Find the sum of first 22 terms of an AP in which d = 7 and 22nd term is 149.
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 11

Question 8.
Find the sum of first 51 terms of an AP whose second and third terms are 14 and 18 respectively.
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 12

Question 9.
If the sum of first 7 terms of an AP is 49 and that of 17 terms is 289, find the sum of first n terms.
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 13

Question 10.
Show that a₁, a₂, ……. a_n,…… form an AP where an is defined as below:
(i) a_n = 3 + 4n
(ii) a_n = 9 – 5n
Also find the sum of the first 15 terms in each case.
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 15

Question 11.
If the sum of the first n terms of an AP is 4n – n², what is the first term (that is S₁)? What is the sum of first two terms? What is the second term? Similarly, find the 3rd, the 10th and the nth terms.
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 16

Question 12.
Find the sum of the first 40 positive integers divisible by 6.
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 17

Question 13.
Find the sum of the first 15 multiples of 8.
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 18

Question 14.
Find the sum of the odd numbers between 0 and 50.
Solution:
Let odd numbers between 0 and 50 be 1, 3, 5, 7,…….., 49.
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 19

Question 15.
A contract on construction job specifies a penalty for delay of completion beyond a certain date as follows:
₹ 200 for the first day, ₹ 250 for the second day, ₹ 300 for the third day, etc. the penalty for each succeeding day being ₹ 50 more than for the preceding day. How much money the contractor has to pay as penalty, if he has delayed the work by 30 days?
Solution:
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 20

Question 16.
A sum of ₹ 700 is to be used to give seven cash prizes to students of a school for their overall academic performance. If each prize is ₹ 20 less than its preceding prize, find the value of each of the prizes.
Solution:
Let 1st prize be of ₹ a
2nd prize be ₹ (a – 20) and
3rd prize be ₹ (a – 20 – 20) = ₹ (a – 40)
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 21

Question 17.
In a school, students thought of planting trees in and around the school to reduce air pollution. It was decided that the number of trees, that each section of each class will plant, will be the same as the class, in which they are studying, eg. a section of Class I will plant 1 tree, a section of Class II will plant 2 trees and so on till Class XII. There are three sections of each class. How many trees will be planted by the students?
Solution:
Let the trees be planted 1, 2, 3, 4, 5 , …… 12
Here, a = 1, d = 1, n = 12
Total number of trees planted by each section
S₁₂ = \(\frac { 12 }{ 2 }\) [2a + (n – 1) d] = 6 [2 x 1 + (12 – 1) x 1]
= 6 [2 + 11] = 6 x 13 = 78
Total number of trees planted by 3 sections = 78 x 3 = 234

Question 18.
A spiral is made up of successive semicircles, with centres alternately at A and B, starting with centre at A, of radii 0.5 cm, 1.0 cm, 1.5 cm, 2.0 cm,… as shown in figure. What is the total length of such a spiral made up of thirteen consecutive semicircles?
(Take π = \(\frac { 22 }{ 7 }\))
[Hint: Length of successive semicircles is l₁, l₂, l₃, l₄, … with centres at A, B, respectively.]
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 22
Solution:

Question 19.
200 logs are stacked in the following manner 20 logs in the bottom row, 19 in the next row, 18 in the row next to it and so on (see Figure). In how many rows are the 200 logs placed and how many logs are in the top row?
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 24
Solution:

Question 20.
In a potato race, a bucket is placed at the starting point, which is 5 m from the first potato, and the other potatoes are placed 3 m apart in a straight line. There are ten potatoes in the line (see Fig.) A competitor starts from the bucket, picks up the nearest potato, runs back with it, drops it in the bucket, runs back to pick up the next potato, runs to the bucket to drop it in, and she continues in the same way until all the potatoes are in the bucket. What is the total distance the competitor has to run?
[Hint: To pick up the first potato and the second potato, the total distance (in metres) run by a competitor is 2 x 5 + 2 x (5 + 3)]
NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3 26

Solution:
Distance between the first potato and the bucket = 5 m
Distance between next 2 potatoes = 3 m each
So, series is 5 m, 8 m, 11 m,
Here, a = 5 m, d = (8 – 5) m = 3 m
Total distance travelled for 10 potatoes = 2 [5 + 8 + 11 + …….. + 10 terms]
= 2[\(\frac { 10 }{ 2 }\){2 x 5 + (10 – 1) 3}]
= 2[5{10 + 27}] = 2(37 x 5) = 37 x 10 = 370 m.

We hope the NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3, help you. If you have any query regarding NCERT Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.3, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.4

March 13, 2023

NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.4 are part of NCERT Solutions for Class 10 Maths. Here we have given NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.4.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 4
Chapter Name	Quadratic Equations
Exercise	Ex 4.4
Number of Questions Solved	5
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.4

Question 1.
Find the nature of the roots of the following quadratic equations. If the real roots exist, find them:
(i) 2x² -3x + 5 = 0
(ii) 3x² – 4√3x + 4 = 0
(iii) 2x²-6x + 3 = 0
Solution:
(i) 2x² – 3x + 5 = 0
This is of the form ax² + bx + c = 0,
where a = 2, b = -3 and c = 5
Discriminant, D = b²-4ac
= (-3) ²-4 x 2 x 5 = 9 – 40 = -31
Since, D < 0
Hence, no real roots exist.

(ii) 3x² – 4√3x + 4 = 0
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.4 1
(iii) 2x²-6x + 3 = 0

Question 2.
Find the values of k for each of the following quadratic equations, so that they have two equal roots.
(1) 2x² + kx + 3 = 0
(2) kx (x – 2) + 6 = 0
Solution:
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.4 3

Question 3.
Is it possible to design a rectangular mango grove whose length is twice its breadth, and the area is 800 m²? If so, find its length and breadth.
Solution:
Let breadth of the rectangular mango grove be x m
Then, the length of rectangular mango grove be 2xm
According to question,
x x 2x = 800
⇒ 2x² = 800
⇒ x² = 400
⇒ x = ±20 [-20 is rejected]
Hence, breadth = 20 m and length = 2 x 20 = 40 m
So, it is possible to design a rectangular mango grove whose length is twice its breadth.

Question 4.
Is the following situation possible? If so, determine their present ages.
The sum of the ages of two friends is 20 years. Four years ago, the product of their ages in years was 48.
Solution:
Let the present age of one friend be x years
Then, the present age of other friend be (20 -x) years
4 years ago, one friend’s age was (x – 4) years
4 years ago, other friend’s age was (20 -x – 4) = (16 -x) years
According to question,
(x-4) (16-x) = 48
⇒ 16x – x² – 64 + 4x = 48
⇒ x² – 20x + 112 = 0
This is of the form ax² + bx + c = 0, where, a = 1, b = -20 and c = 112
Discriminant, D = b² – 4ac
= (-20)² – 4 x 1 x 112 = 400 – 448 = – 48 < 0
Since, no real roots exist.
So, the given situation is not possible.

Question 5.
Is it possible to design a rectangular park of perimeter 80 m and area 400 m²? If so, find its length and breadth.
Solution:
Let the length of rectangular park be x m and breadth be y m.
Given: area = 400 m²
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.4 4

We hope the NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.4 help you. If you have any query regarding NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.4, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3

March 13, 2023

NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 are part of NCERT Solutions for Class 10 Maths. Here we have given NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 4
Chapter Name	Quadratic Equations
Exercise	Ex 4.3
Number of Questions Solved	11
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3

Question 1.
Find the roots of the following quadratic equations, if they exist, by the method of completing the square:
(i) 2x² – 7x + 3 = 0
(ii) 2x² + x – 4 = 0
(iii) 4x² + 4√3x + 3 = 0
(iv) 2x² + x + 4 = 0
Solution:
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 1

Question 2.
Find the roots of the quadratic equations by applying the quadratic formula.
(i) 2x² – 7x + 3 = 0
(ii) 2x² – x + 4 = 0
(iii) 4x² – 4√3x + 3 = 0
(iv) 2x² – x + 4 = 0
Solution:
(i) 2x² – 7x + 3 = 0
This is of the form ax² + bx + c = 0,
where a = 2, b = -7 and c = 3
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 5

(ii) 2x² – x + 4 = 0
This is of the form ax² + bx + c = 0,
where a = 2, b = 1 and c = – 4
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 7

(iii) 4x² – 4√3x + 3 = 0
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 8

(iv) 2x² – x + 4 = 0

Question 3.
Find the roots of the following equations:

NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 11
Solution:

Question 4.
The sum of the reciprocals of Rehman’s ages, (in years) 3 years ago and 5 years from now is \(\frac { 1 }{ 3 }\) Find his present age.
Solution:
Let the present age of Rehman be x years
3 years ago Rehman’s age was = (x – 3) years
5 years from now Rehman’s age will be = (x + 5) years
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 13

Question 5.
In a class test, the sum of Shefali’s marks in Mathematics and English is 30. Had she got 2 marks more in Mathematics and 3 marks less in English, the product of their marks would have been 210. Find her marks in the two subjects.
Solution:
Let the marks secured by Shefali in Mathematics = x Then,
According to question,
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 14

Question 6.
The diagonal of a rectangular field is 60 metres more than the shorter side. If the longer side is 30 metres more than the shorter side, find the sides of the field.
Solution:
Let the shorter side of rectangle = x m
Then, longer side = (x + 30) m and diagonal = (x + 60) m
In ΔABC, (x + 60)² = (x + 30)² + (x)² [Pythagoras Theorem]
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 15

Question 7.
The difference of squares of two numbers is 180. The square of the smaller number is 8 times the larger number. Find the two numbers.
Solution:
Let the smaller number = x
and the larger number = y
According to question,
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 17

Question 8.
A train travels 360 km at a uniform speed. If the speed had been 5 km/h more, it would have taken 1 hour less for the same journey. Find the speed of the train.
Solution:
Total distance travelled = 360 km
Let uniform speed be x km/h
Then, increased speed = (x + 5) km/h
According to question,
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 18

Question 9.
Two water taps together can fill a tank in 9\(\frac { 3 }{ 8 }\) hours. The tap of larger diameter takes 10 hours less than the smaller one to fill the tank separately. Find the time in which each tap can separately fill the tank.
Solution:
Let larger pipe fills the tank in x hours and the smaller pipe fills the tank in y hours.
The tank filled by the larger pipe in 1 hour = \(\frac { 1 }{ x }\)
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 19

Question 10.
An express train takes 1 hour less than a passenger train to travel 132 km between Mysore and Bengaluru (without taking into consideration the time they stop at intermediate stations). If the average speed of the express train is 11 km/h more than that of the passenger train, find the average speed of the two trains.
Solution:
Let the speed of passenger train = x km/h
Then, the speed of express train be (x + 11) km/h
According to question,
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 20

Question 11.
Sum of the areas of two squares is 468 m². If the difference of their perimeters is 24 m, find the sides of the two squares.
Solution:
Let the sides of two squares be x m and y m
According to question,
NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 21

We hope the NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3 help you. If you have any query regarding NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations Ex 4.3, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7

March 13, 2023

NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7 are part of NCERT Solutions for Class 10 Maths. Here we have given NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 3
Chapter Name	Pair of Linear Equations in Two Variables
Exercise	Ex 3.7
Number of Questions Solved	8
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7

Question 1.
The age of two friends Ani and Biju differ by 3 years. Ani’s father Dharam is twice as old as Ani and Biju is twice as old as his sister Cathy. The ages of Cathy and Dharam differ by 30 years. Find the ages of Ani and Biju.
Solution:
Let the ages of Ani and Biju be x years and y years respectively.
If Ani is older than Biju
x – y =3
If Biju is older than Ani
y – x = 3
-x + y =3 [Given]
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7 1
Subtracting equation (i) from equation (ii), we get:
3x – 57
⇒ x = 19
Putting x = 19 in equation (i), we get
19-y = 3
⇒ y = 16
Again subtracting equation (iv) from equation (iii), we get
3x = 63
⇒ x = 21
Putting x = 21 in equation (iii) we get
21 -y= -3
⇒ y = 24
Hence, Ani’s age is either 19 years or 21 years and Biju’s age is either 16 years or 24 years.

Question 2.
One says, “Give me a hundred, friend! I shall then become twice as rich as you”. The other replies, “If you give me ten, I shall be six times as rich as you”. Tell me what is the amount of their (respective) capital?
Solution:
Let the two friends have ₹ x and ₹ y.
According to the first condition:
One friend has an amount = ₹(x + 100)
Other has an amount = ₹ (y – 100
∴ (x + 100) =2 (y – 100)
⇒ x + 100 = 2y – 200
⇒ x – 2y = -300       …(i)
According to the second condition:
One friend has an amount = ₹(x – 10)
Other friend has an amount =₹ (y + 10)
∴ 6(x – 10) = y + 10
⇒ 6x – 60 = y + 10
⇒ 6x-y = 70                                        …(ii)
Multiplying (ii) equation by 2 and subtracting the result from equation (i), we get:
x – 12x = – 300 – 140
⇒ -11x = -440
⇒ x = 40
Substituting x = 40 in equation (ii), we get
6 x 40 – y = 70
⇒ -y = 70- 24
⇒ y   = 170
Thus, the two friends have ₹ 40 and ₹ 170.

Question 3.
A train covered a certain distance at a uniform speed. If the train would have been 10 km/h faster, it would have taken 2 hours less than the scheduled time. And, if the train were slower by 10 km/h, it would have taken 3 hours more than the scheduled time. Find the distance covered by the train.
Solution:
Let the original speed of the train be x km/h
and the time taken to complete the journey be y hours. ‘
Then the distance covered = xy km

Case I: When speed = (x + 10) km/h and time taken = (y – 2) h
Distance = (x + 10) (y – 2) km
⇒ xy = (x + 10) (y – 2)
⇒ 10y – 2x = 20
⇒ 5y – x = 10
⇒ -x + 5y = 10 …(i)

Case II: When speed = (x – 10) km/h and time taken = (y + 3) h
Distance = (x – 10) (y + 3) km
⇒ xy = (x – 10) (y + 3)
⇒ 3x- 10y = 30 …(ii)
Multiplying equation (i) by 3 and adding the result to equation (ii), we get
15y – 10y = 30 f 30
⇒ 5y = 60
⇒ y   = 12
Putting y = 12 in equation (ii), we get
3x- 10 x 12= 30
⇒ 3x   = 150
⇒ x   = 50
∴ x = 50 and y = 12
Thus, original speed of train is 50 km/h and time taken by it is 12 h.
Distance covered by train = Speed x Time
= 50 x 12 = 600 km.

Question 4.
The students of a class are made to stand in rows. If 3 students are extra in a row, there would be 1 row less. If 3 students are less in a row, there would be 2 rows more. Find the number of students in the class.
Solution:
Let the number of rows be x and the number of students in each row be y.
Then the total number of students = xy
Case I: When there are 3 more students in each row
Then the number of students in a row = (y + 3)
and the number of rows = (x – 1)
Total number of students = (x – 1) (y + 3)
∴ (x – 1) (y + 3) = xy
⇒ 3x -y =3 …(i)
Case II: When 3 students are removed from each row
Then the number of students in each row = (y-3)
and the number of rows = (x + 2)
Total number of students = (x + 2) (y – 3)
∴ (x + 2) (y – 3) = xy
⇒ -3x + 2y = 6 …(ii)
Adding the equations (i) and (ii), we get
-y + 2y = 3 + 6
⇒ y = 9
Putting y = 9 in the equation (ii), we get
-3x + 18 = 6
⇒ x = 4
∴ x = 4 and y = 9
Hence, the total number of students in the class is 9 x 4 = 36.

Question 5.
In a ∆ABC, ∠C = 3 ∠B = 2(∠A + ∠B). Find the three angles.
Solution:
Let ∠A = x° and ∠B = y°.
Then ∠C = 3∠B = (3y)°.
Now ∠A + ∠B + ∠C = 180°
⇒ x + y + 3y = 180°
⇒ x + 4y = 180° …(i)
Also, ∠C = 2(∠A + ∠B)
⇒ 3y – 2(x + y)
⇒ 2x – y = 0° …(ii)
Multiplying (ii) by 4 and adding the result to equation (i), we get:
9x = 180°
⇒ x = 20°
Putting x = 20 in equation (i), we get:
20 + 4y = 180°
⇒ 4y = 160°
⇒ y = \(\frac { 160 }{ 40 }\) = 40°
∴ ∠A = 20°, ∠B = 40° and ∠C = 3 x 40° = 120°.

Question 6.
Draw the graphs of the equations 5x – y = 5 and 3x – y = 3. Determine the coordinates of the vertices of the triangle formed by these lines and the y-axis.
Solution:
5x – y = 5 …(i)
3x-y = 3 …(ii)
For graphical representation:
From equation (i), we get: y = 5x – 5
When x = 0, then y -5
When x = 2, then y = 10 – 5 = 5
When x = 1, then y = 5 – 5 = 10
Thus, we have the following table of solutions:
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7 2
From equation (ii), we get:
⇒ y = 3x – 3
When x = 0, then y = -3
When x = 2, then y = 6 – 3 = 3
When x = 1, then y = 3 – 3 = 0
Thus, we have the following table of solutions:

Plotting the points of each table of solutions, we obtain the graphs of two lines intersecting each other at a point C(1, 0).
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7 4
The vertices of ΔABC formed by these lines and the y-axis are A(0, -5), B(0, -3) and C(1, 0).

Question 7.
Solve the following pairs of linear equations:
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7 5
Solution:
(i) The given equations are
px + qy = p – q …(1)
qx – py = p + q …(2)
Multiplying equation (1) byp and equation (2) by q and then adding the results, we get:
x(p² + q²) = p(p – q) + q(p + q)
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7 6

(ii) The given equations are
ax + by = c …(1)
bx – ay = 1 + c …(2)
Multiplying equation (1) by b and equation (2) by a, we get:
abx + b²y = cb …(3)
abx + a²y = a(1+ c) …(4)
Subtracting (3) from (4), we get:
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7 8

(iii) The given equations may be written as: bx – ay = 0 …(1)
ax + by = a² + b² …(2)
Multiplying equation (1) by b and equation (2) by a, we get:
b²x + aby = 0 ….(3)
a²x + aby = a(a² + b²) …..(4)
Adding equation (3) and equation (4), we get:
(a² + b²)x = a (a² + b²) a(a² + b²)
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7 10

(iv) The given equations may be written as:
(a – b)x + (a + b)y = a² – 2ab – b² …(1)
(a + b)x + (a + b)y = a² + b² …(2)
Subtracting equation (2) from equation (1), we get:
(a – b)x – (a + b)x
= (a² – 2ab – b²) – (a² + b²)
⇒ x(a – b- a-b) = a² – 2ab – b² – a² – b²⇒ -2bx = -2ab – 2b²⇒ 2bx = 2b² + 2ab
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7 11

(v) The given equations may be written as:
76x – 189y = -37 …(1)
-189x + 76y = -302 …(2)
Multiplying equation (1) by 76 and equation (2) by 189, we get:
5776x – 14364y = -2812 …(3)
-35721x + 14364y = -57078 …(4)
Adding equations (3) and (4), we get:
5776x – 35721x = -2812 – 57078
⇒ – 29945x = -59890
⇒ x = 2
Putting x = 2 in equation (1), we get:
76 x 2 – 189y = -37
⇒ 152 – 189y = -37
⇒ -189y = -189
⇒ y = 1
Thus, x = 2 and y = 1 is the required solution.

Question 8.
ABCD is a cyclic quadrilateral (see figure). Find the angles of the cyclic quadrilateral.
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7 13
Solution:

We hope the NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7 help you. If you have any query regarding NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.7, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.5

March 13, 2023

NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.5 are part of NCERT Solutions for Class 10 Maths. Here we have given NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.5.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 3
Chapter Name	Pair of Linear Equations in Two Variables
Exercise	Ex 3.5
Number of Questions Solved	4
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.5

Question 1.
Which of the following pairs of linear equations has unique solution, no solution, or infinitely many solutions. In case there is a unique solution, find it by using cross multiplication method.
(i) x – 3y – 3 = 0
3x – 9y – 2 = 0
(ii) 2x + y = 5
3x + 2y = 8
(iii) 3x – Sy = 20
6x – 10y = 40
(iv) x – 3y – 7 = 0
3x – 3y – 15 = 0
Solution:
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.5 1
(iii) Equations are 3x – 5y = 20 and 6x – 10y = 40
Here,

(iv) Equations are x – 3y = 7 and 3x – 3y = 15

Question 2.
(i) for which values of a and b does the following pai of linea equation have an infinite number of solutions₹
2x + 3y =7
(a – b)x + (a + b)y = 3a + b – 2
(ii) For which value of K will the following pair of linear equation have no solution₹
3x + y = 1
(2k – 1)x + (k – 1)y = 2k + 1
Solution:
(i) Equations are
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.5 5
⇒ a – 2b = 3 Solving (iii) and (iv) for a and b
By cross multiplication method.

Question 3.
Solve the following pair of linear equations by the substitution and cross-multiplication methods:
8x + 5y = 9
3x + 2y = 4
Solution:
Equations are
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.5 7
By coss multiplication Method :
Equations are

Question 4.
Form the pair of linear equations in the following problems and find their solutions (if they exist) by any algebraic method:
(i) A part of monthly hostel charges is fixed and the remaining depends on the number of days one has taken food in the mess. When a student A takes food for 20 days she has to pay ₹ 1000 as hostel charges whereas a student B, who takes food for 26 days, pays ₹ 1180 as hostel charges. Find the fixed charges and the cost of food per day.
(ii) A fraction becomes 1/3 when 1 is subtracted from the numerator and it becomes 1/4 when 8 is added to its denominator. Find the fraction.
(iii) Yash scored 40 marks in a test, getting 3 marks for each right answer and losing 1 mark for each wrong answer. Had 4 marks been awarded for each correct answer and 2 marks been deducted for each incorrect answer, then Yash would have scored 50 marks. How many questions were there in the test₹
(iv) Places A and B are 100 km apart on a highway. One car starts from A and another from B at the same time. If the cars travel in the same direction at different speeds, they meet in 5 hours. If they travel towards each other, they meet in 1 hour. What are the speeds of the two cars ₹
(v) The area of a rectangle gets reduced by 9 square units, if its length is reduced by 5 units and breadth is increased by 3 units. If we increase the length by 3 units and the breadth by 2 units, the area increases by 67 square units. Find the dimensions of the rectangle.
Solution:
(i) Let fixed monthly hostel charges = and charges per day = ₹ y
A.T.Q.
As per condition of student A
x + 20y = 1000
As per condition of student B
x + 26y = 1180

By cross multiplication method
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.5 9
∴ Fixed monthly hostel charges = ₹ 400 and charges per day = ₹ 30

By cross multiplication method

Solving (i) and (ii) for x and y
By cross multiplication method

By cross multiplication method
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.5 13

We hope the NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.5 help you. If you have any query regarding NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.5, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.4

March 13, 2023

NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.4 are part of NCERT Solutions for Class 10 Maths. Here we have given NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.4.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 3
Chapter Name	Pair of Linear Equations in Two Variables
Exercise	Ex 3.4
Number of Questions Solved	2
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.4

Question 1.
Solve the following pair of linear equations by the elimination method and the substitution
(i) x + y = 5 and 2x – 3y = 4
(ii) 3x + 4y = 10 and 2x – 2y = 2
(iii) 3x – 5y – 4 = 0 and 9x = 2y + 7
(iv) x/2 + 2y/3 = -1 and x – y/3 = 3
Solution:
(i) By Elimination Method:
Fquations are x + y = 5
and 2x – 3y = 4
Multiply equation (i) by 2 and subtract equation (ii) from it, we have
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.4 1

(ii) By Elimination method:
Equations are 3x + 4y = 10
and 2x – 2y = 2
Multiplying equation (ii) by 2 and adding to equation (i), we

(iii) By Elimination Method:

(iv) By Elimination Method:
1st equation :
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.4 5

Question 2.
Form the pair of linear equations in the following problems, and find their solutions (if they exist) by the elimination method:
(i) If we add 1 to the numerator and subtract 1 from the denominator, a fraction reduces to 1. It becomes – if we only add 1 to the denominator. What is the fraction₹
(ii) Five years ago, Nuri was thrice as old as Sonu. Ten years later, Nuri will be twice as old as Sonu. How old are Nuri and Sonu₹
(iii) The sum of the digits of a two-digit number is 9. Also, nine times this number is twice the number obtained by reversing the order of the digits. Find the number.
(iv) Meena went to a bank to withdraw ₹ 2000. She asked the cashier to give her ₹ 50 and ₹ 100 notes only. Meena got 25 notes in all. Find how many notes of ₹ 50 and ₹ 100 she received.
(v) A lending library has a fixed charge for the first three days and an additional charge for each day thereafter. Saritha paid ₹ 27 for a book kept for seven days, while Susy paid ₹ 21 for the book she kept for five days. Find the fixed charge and the charge for each extra day.
Solution:
(i) Let numerator be x and denominator be y.
Fraction = x/y
A.T.Q.

(ii) Let present age of Nuri be x years and Sonu’s present age bey years.
A.T.Q.
1st Condition :

2nd Condition :
Subtractomg equation (ii) from equetion (i), we get

Hence, present age of Nuri is 50 years and sonu’s present age is 20 years.

(iii) Let digit at unit place = x and digit at ten’s place = y.
Two digit number is lOy + x
A.T.Q.
1st Condition :
x + y = 9
2nd Condition :
9(10y + x) = 2(10k + y) ⇒ 90y + 9x = 20x + 2y
⇒ 88y – 11x = 0 ⇒ -11y + 88y = 0
⇒ -x + 8y = 0
Adding equestion (i) and (ii), we get
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.4 12

(iv) Let the number of notes of ₹ 50 = x and the number of notes of ₹ 100 = y
A.T.Q
1st Condition :
50x + 100y = 2000
⇒ x + 2y = 40
2nd Condition :

(v) Let, fixed charge for first 3 days be ₹ x and additional charge per day after 3 days be y.
A.T.Q.
1st Condition : as per Saritha
x + 4y = 27
2nd Condition : as per Susy
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.4 14
Putting y = 3 in equation (i),
x + 4(3) = 27 ⇒ x + 12 = 27 ⇒ x = 15
Hence, fixed charge is ₹ 15 and charge for each extra day is ₹ 3.

We hope the NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.4 help you. If you have any query regarding NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.4, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.3

March 13, 2023

NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.2 are part of NCERT Solutions for Class 10 Maths. Here we have given NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.2.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 3
Chapter Name	Pair of Linear Equations in Two Variables
Exercise	Ex 3.3
Number of Questions Solved	3
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.3

Question 1.
Solve the following pair of linear equations by the substitution method,
(i) x + y = 14, x – y = 4
(ii) s – t = 3, s/3 + t/2 = 6
(iii) 3x – y = 3, 9x – 3y = 9
(iv) 0.2x + 0.3y = 1.3, 0.4x + 0.5y = 2.3
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.3 1
Solution:
From equation (i),
x + y – 14 ⇒ y = 14x
Putting the value ofy in equation (ii), we get
x – (14 – x) = 4 ⇒ x – 14 + x = 4 ⇒ 2x = 4 + 14
2x = 18 ⇒ x = 9
Now, puttingx = 9 in equation (i), we have
9 + y = 14 ⇒ y = 14 – 9 ⇒ y = 5
so, x = 9, y = 5
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.3 2
∴ y can have infinite real values
∴ x can have infinite real values because x = \(\frac { y+3 }{ 3 }\)

Question 2.
Solve 2x + 3y = 11 and 2x – 4y = – 24 and hence find the value of ‘m’ for which y = mx + 3.
Solution:
Equations are 2x + 3y = 11
and 2x – 4y = -24
From equation (i)
2x = 11 – 3y
Putting this value in equation (ii), we get
11 – 3y – 4y = -24 ⇒ 11 – 7y = -24 ⇒ – 7y = – 35
y = \(\frac { 35 }{ 7 }\) ⇒ y = 5
Putting y = 5 in equation (i). we have
2x + 3 x 5 = 11 ⇒ 2x + 15 = 11 ⇒ 2x = 11 – 15 ⇒ 2x = -4 ⇒ x = -2
Now. putting the value of x andy in equation
y = mx + 3 ⇒ 5 = -2m + 3 ⇒ 2 = -2m ⇒ m = -1

Question 3.
Form the pair of linear equations for the following problems and find their solution by substitution method.
(i) The difference between two numbers is 26 and one number is three times the other. Find them.
(ii) The larger of two supplementary angles exceeds the smaller by 18 degrees. Find them.
(iii) The coach of a cricket team buys 7 bats and 6 balls for ₹ 3800. Later, she buys 3 bats and 5 balls for ₹ 1750. Find the cost of each bat and each ball,
(iv) The taxi charges in a city consist of a fixed charge together with the charge for the distance covered. For a distance of 10 km, the charge paid is ₹ 105 and for a journey of 15 km, the charge paid is ₹ 155. What are the fixed charges and the charge per km₹ How much does a person have to pay for travelling a distance of 25 km₹
(v) A fraction becomes 9/2, if 2 is added to both the numerator and the denominator. If, 3 is added to both the numerator and denominator it becomes 5/6, Find the fraction.
(vi) Five years hence, the age of Jacob will be three times that of his son. Five years ago, Jacob’s age was seven times that of his son. What are their present ages?
Solution:
(i) Let 1st number be x and 2nd number be y.
Let x >y
1st condition :
x – y = 26
2nd condition :
x = 3y
Putting x = 3y in equation (i)
3y – y = 26 ⇒ 2y = 26 ⇒ y = 13
From (ii)
x = 3 x 13 = 39
∴ One number is 13 and the other number is 39.

(ii) Let one angle be x and its supplementary angle = y
Let x > y
1st Condition :
x + y = 180°
2nd Condition :
x – y = 18° ⇒ X = 18° + y
From equation (ii), putting the value ofx in equation (i),
18° + y + y = 180° ⇒ 18° + 2y = 180°
2y = 162° ⇒ y = 81°
From (ii) x = 18° + 81° = 99° ⇒ x = 99°
∴ One angle is 81° and another angle is 99°.

(iii) Let cost of 1 bat = ₹x and cost of 1 ball = ₹y
1st Condition:
7x + 6y = 3800
2nd Condition:
3x + 5y = 1750
From equation (ii), we get
NCERT Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables Ex 3.3 6
putting x = 1750-5y/3 in equation (i), we get
Cost of one bat = ₹ 500 and cost of one ball = ₹ 50.

(iv) Let fixed charges be ₹.v and charge for per km be ₹y.
A.T.Q.
1st Condition :
x + lOy = 105
2nd Condition :
x + 15y = 155
From equation (i), we get
x= 105 – 10y
Putting this value in equation (ii), we have
105 – 10y + 15y = 155 ⇒ 105 + 5y = 155
⇒ 5y = 155 – 105 ⇒ 5y = 50 ⇒ y = 10
Now, puttingy = 10 in equation (i), we have
x + 10(10) = 105 ⇒ x + 100 = 105 ⇒ x = 5
Fixed charges is ₹ 5 and charges per km is ₹ 10.

3rd Condition :
For distance of 25 km
x + 25y = 5 + 25(10) = 5 + 250 = 255
Amount paid for travelling 25 km is ₹ 255.

(v) Let numerator be x and denominator be y.
∴ Fraction is x/y
A.T.Q.
1st condition :

2nd condition :

(vi) Let present age of Jacob be x years and that of his son bey years.
A.T.Q.
1st Condition :
x + 5 = 3(y + 5) ⇒ x + 5 = 3y + 15 ⇒ x – 3y = 15 – 5 ⇒ x – 3y = 10
2nd Condition:
x – 5 = 7(y – 5) ⇒ x – 5 = 7y – 35 ⇒ x = 7y – 35 + 5
⇒ x = 7y – 30
Putting the value of ‘x’ in equation (i), we get
7y – 30 – 3y = 10
4y – 30 = 10
4y = 40 y = 10 ⇒ y = 10
putting the value of y in equation(ii), we get
x = 7(10) – 30 = 70 – 30 ⇒ x = 40
Hence, the present age of Jacob is 40 years and that of his son is 10 years.

We hope the NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.3 help you. If you have any query regarding NCERT Solutions for Class 10 Mathematics Chapter 3 Pair of Linear Equations in Two Variables Ex 3.3, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.4

March 13, 2023

NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.4 are part of NCERT Solutions for Class 10 Maths. Here we have given NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.4.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 2
Chapter Name	Polynomials
Exercise	Ex 2.4
Number of Questions Solved	5
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.4

Question 1.
Verify that the numbers given alongside of the cubic polynomials below are their zeroes. Also, verify the relationship between the zeroes and the coefficients in each case:
(i) 2x³ + x² – 5x + 2; \(\frac { 1 }{ 4 }\), 1, -2
(ii) x³ – 4x² + 5x – 2; 2, 1, 1
Solution:
(i) Comparing the given polynomial with ax³ + bx² + cx + d, we get:
a = 2, b – 1, c = -5 and d = 2.
∴ p(x) = 2x³ + x² – 5x + 2
NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.4 1

(ii) Compearing the given polynomial with ax³ + bx² + cx + d, we get:
a = 1, b = -4, c = 5 and d = – 2.
∴ p (x) = x³ – 4x² + 5x – 2
⇒ p(2) = (2)³ – 4(2)² + 5 x 2 – 2
= 8 – 16+ 10 – 2 = 0
p(1) = (1)³ – 4(1)² + 5 x 1-2
= 1 – 4 + 1 – 2
= 6-6 = 0
Hence, 2, 1 and 1 are the zeroes of x³ – 4x²+ 5x – 2.
Hence verified.
Now we take α = 2, β = 1 and γ = 1.
α + β + γ = 2 + 1 + 1 = \(\frac { 4 }{ 1 }\) = \(\frac { -b }{ a }\)
αβ + βγ + γα = 2 + 1 + 2 = \(\frac { 5 }{ 1 }\) = \(\frac { c }{ a }\)
αβγ = 2 x 1 x 1 = \(\frac { 2 }{ 1 }\) = \(\frac { -d }{ a }\).
Hence verified.

Question 2.
Find a cubic polynomial with the sum, sum of the product of its zeroes taken two at a time, and the product of its zeroes as 2, -7, -14 respectively.
Solution:
Let α , β and γ be the zeroes of the required polynomial.
Then α + β + γ = 2, αβ + βγ + γα = -7 and αβγ = -14.
∴ Cubic polynomial
= x³ – (α + β + γ)x² + (αβ + βγ + γα)x – αβγ
= x³ – 2x² – 1x + 14
Hence, the required cubic polynomial is x³ – 2x² – 7x + 14.

Question 3.
If the zeroes of the polynomial x³ – 3x² + x + 1 are a-b, a, a + b, find a and b.
Solution:
Let α , β and γ be the zeroes of polynomial x³ – 3x² + x + 1.
Then α = a-b, β = a and γ = a + b.
∴ Sum of zeroes = α + β + γ
⇒   3 = (a – b) + a + (a + b)
⇒ (a – b) + a + (a + b) = 3
⇒ a-b + a + a + b = 3
⇒ 3a = 3
⇒ a = \(\frac { 3 }{ 3 }\) = 1 …(i)
Product of zeroes = αβγ
⇒ -1 = (a – b) a (a + b)
⇒ (a – b) a (a + b) = -1
⇒   (a² – b²)a = -1
⇒ a³ – ab² = -1 … (ii)
Putting the value of a from equation (i) in equation (ii), we get:
(1)³-(1)b² = -1
⇒ 1 – b² = -1
⇒ – b² = -1 – 1
⇒  b² = 2
⇒ b = ±√2
Hence, a = 1 and b = ±√2.

Question 4.
If two zeroes of the polynomial x⁴ – 6x³ – 26x²+ 138x – 35 are 2 ± √3, finnd other zeroes.
Solution:
Since two zeroes are 2 + √3 and 2 – √3,
∴ [x-(2 + √3)] [x- (2 – √3)]
= (x-2- √3)(x-2 + √3)
= (x-2)²– (√3)²x² – 4x + 1 is a factor of the given polynomial.
Now, we divide the given polynomial by x² – 4x + 1.
NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.4 3
So, x⁴ – 6x³ – 26x² + 138x – 35
= (x² – 4x + 1) (x² – 2x – 35)
= (x² – 4x + 1) (x² – 7x + 5x – 35)
= (x²-4x + 1) [x(x- 7) + 5 (x-7)]
= (x² – 4x + 1) (x – 7) (x + 5)
Hence, the other zeroes of the given polynomial are 7 and -5.

Question 5.
If the polynomial x⁴ – 6x³ + 16x² – 25x + 10 is divided by another polynomial x² – 2x + k, the remainder comes out to be x + a, find k and a.
Solution:
We have
p(x) = x⁴ – 6x³ + 16x² – 25x + 10
Remainder = x + a   … (i)
Now, we divide the given polynomial 6x³ + 16x² – 25x + 10 by x² – 2x + k.
NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.4 4
Using equation (i), we get:
(-9 + 2k)x + 10-8 k + k² = x + a
On comparing the like coefficients, we have:
-9 + 2k = 1
⇒ 2k = 10
⇒ k = \(\frac { 10 }{ 2 }\) = 5 ….(ii)
and 10 -8k + k²– a ….(iii)
Substituting the value of k = 5, we get:
10 – 8(5) + (5)² = a
⇒   10 – 40 + 25 = a
⇒ 35 – 40 = a
⇒ a =   -5
Hence, k = 5 and a = -5.

We hope the NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.4 help you. If you have any query regarding NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.4, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.2

March 13, 2023

NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.2 are part of NCERT Solutions for Class 10 Maths. Here we have given NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.2.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 2
Chapter Name	Polynomials
Exercise	Ex 2.2
Number of Questions Solved	2
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.2

Question 1.
Find the zeroes of the following quadratic polynomials and verify the relationship between the zeroes and the coefficients.
(i) x² – 2x – 8
(ii) 4s² – 4s + 1
(iii) 6x² – 3 – 7x
(iv) 4u² + 8u
(v) t² -15
(vi) 3x² – x – 4
Solution:
NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.2 1

Question 2.
Find a quadratic polynomial each with the given numbers as the sum and product of its zeroes respectively.
NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.2 5
Solution:
(i) Zeroes of polynomial are not given, sum of zeroes = \(\frac { 1 }{ 4 }\) and product of zeroes = -1
If ax² + bx + c is a quadratic polynomial, then
α + β = sum of zeroes = \(\frac { -b }{ a }\) = \(\frac { 1 }{ 4 }\) and αβ = product of zeroes = \(\frac { c }{ a }\) = -1
Quadratic polynomial is ax² + bx + c
Let a = k, ∴ b = \(\frac { -k }{ 4 }\) and c = -k
Putting these values, we get

For different values of k, we can have quadratic polynomials all having sum of zeroes as \(\frac { 1 }{ 4 }\) and product of zeroes as -1.

(ii) Sum of zeroes = α + β = √2 = \(\frac { -b }{ a }\); product of zeroes = αβ = \(\frac { 1 }{ 3 }\) = \(\frac { c }{ a }\)
Quadratic polynomial is ax² + bx + c
Let a = k,b = -√2k and c = \(\frac { k }{ 3 }\)
Putting these values we get
NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.2 7
For all different real values of k, we can have different quadratic polynomials of the form 3×2 – 3√2x +1 having sum of zeroes = √2 and product of zeroes = \(\frac { 1 }{ 3 }\)

(iii) Sum of zeroes = α + β = 0 = \(\frac { -b }{ a }\); product of zeroes = αβ = √5 = \(\frac { c }{ a }\)
Let quadratic polynomial is ax² + bx + c
Let a = k,b = 0, c = √5 k
Putting these values, we get
k[x² – 0x + √5 ] = k(x² + √5).
For different real values of k, we can have different quadratic polynomials of the form
x² + √5, having sum of zeroes = 0 and product of zeroes = √5

(iv) Sum of zeroes = α + β = 1= \(\frac { -b }{ a }\); product of zeroes = αβ = 1 = \(\frac { c }{ a }\)
Let quadratic polynomial is ax² + bx + c.
Let a=k, c = k, b = -k
Putting these values, we get k[x² -x +1]
Quadratic polynomial is of the form x² -x + 1 for different values of k.

(v) Sum of zeroes = α + β = \(\frac { -1 }{ 4 }\)= \(\frac { -b }{ a }\); product of zeroes = αβ = \(\frac { 1 }{ 4 }\) = \(\frac { c }{ a }\)
Let quadratic polynomial is ax² + bx + c
Let a=k, b= \(\frac { k }{ 4 }\), c= \(\frac { k }{ 4 }\)
Putting these values, we get k

Quadratic polynomial is of the form 4x² +x + 1 for different values of k.

(vi) Sum of zeroes = α + β = 4 = \(\frac { -b }{ a }\); product of zeroes = αβ = 1 = \(\frac { c }{ a }\)
Let quadratic polynomial is ax² + bx + c
Let a = k,b = -4k and c = k
Putting these values, we get
k[x² – 4x + 1]
Quadratic polynomial is of the form x² – 4x + 1 for different values of k.

We hope the NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.2 help you. If you have any query regarding NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.2, drop a comment below and we will get back to you at the earliest.