Category: CBSE Class 11

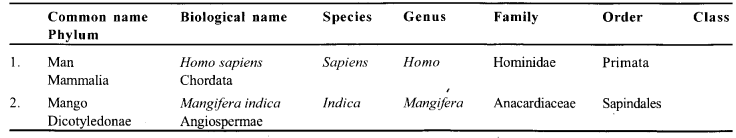
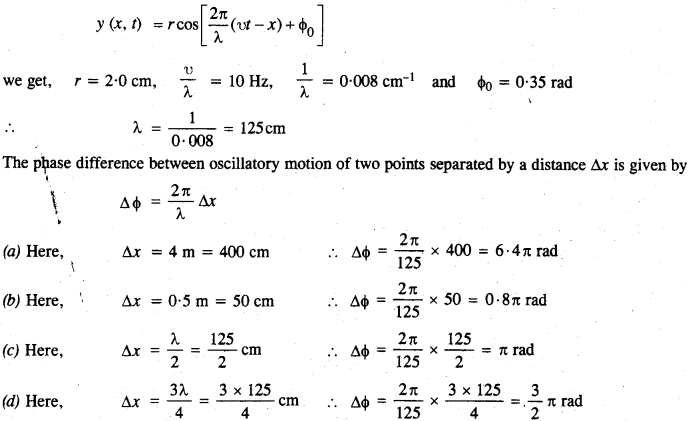
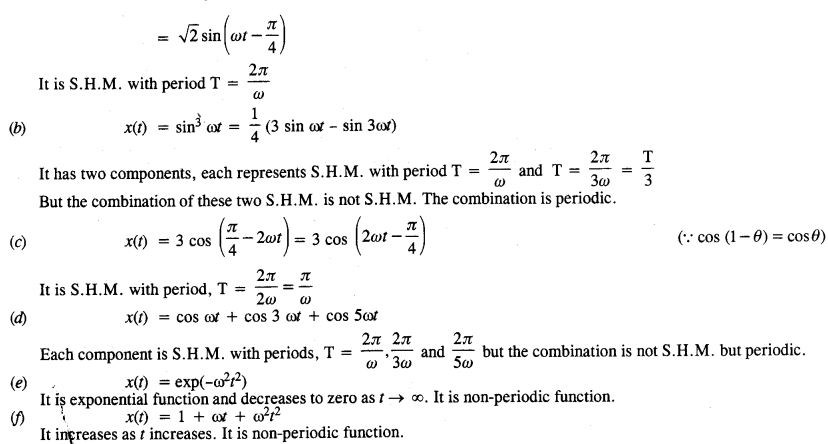
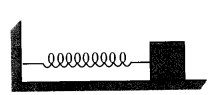
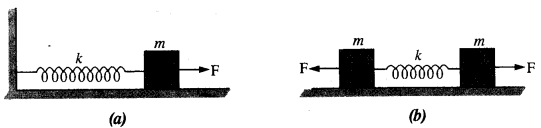
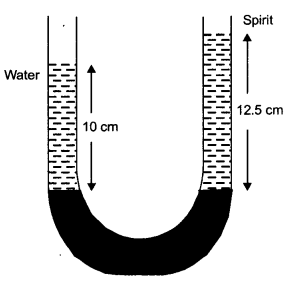
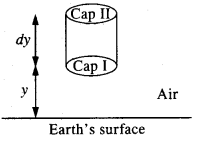
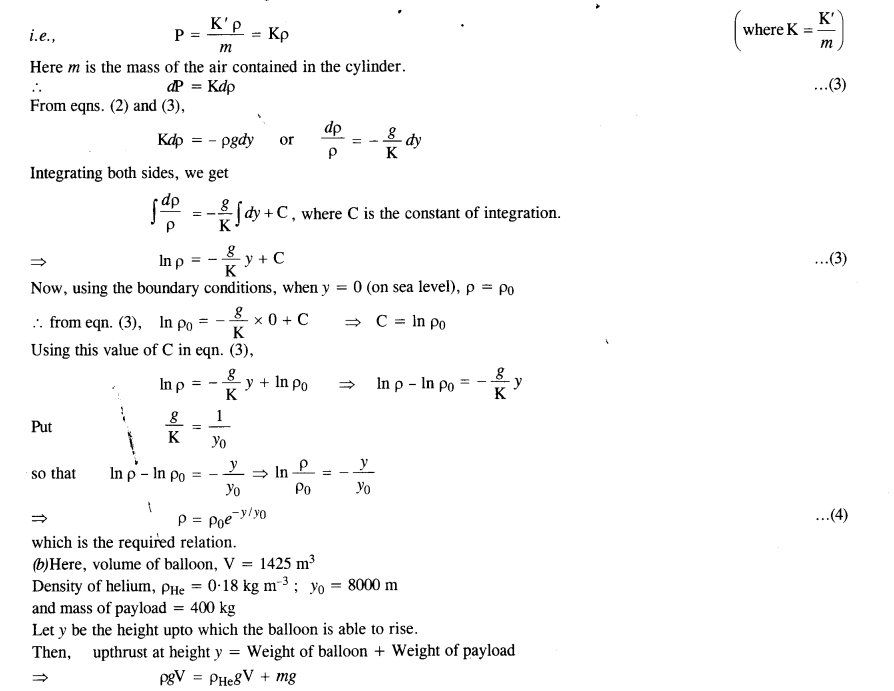
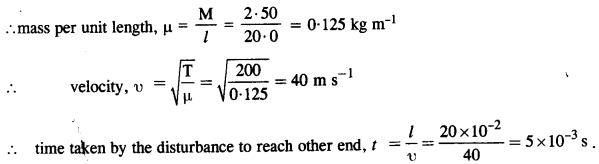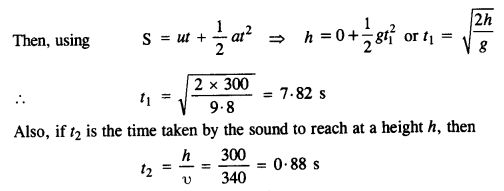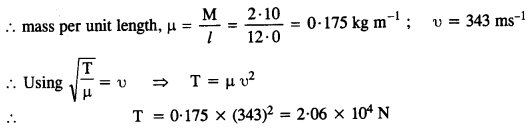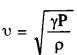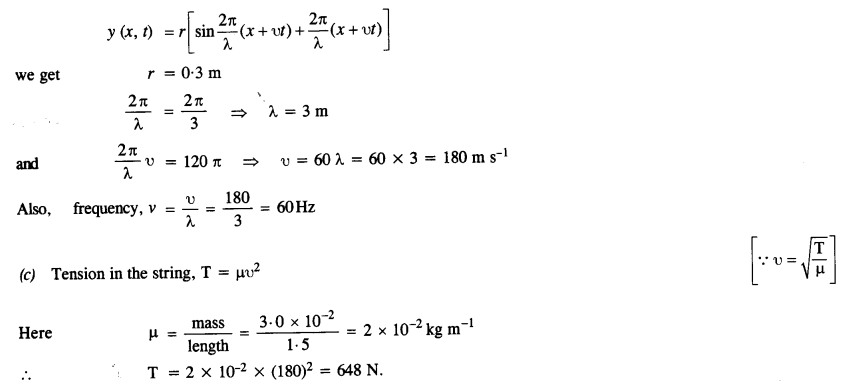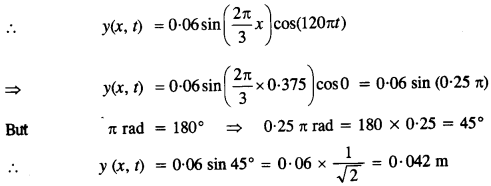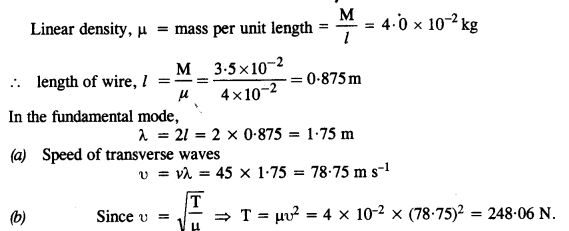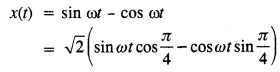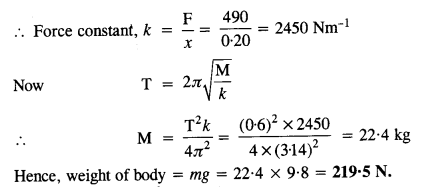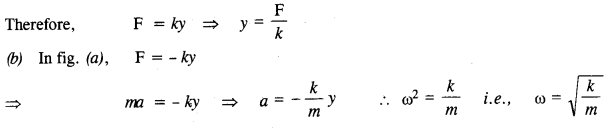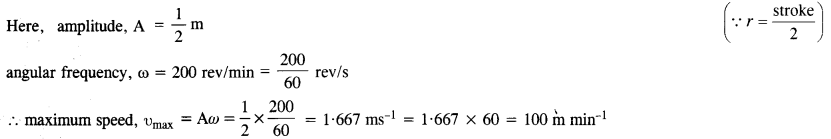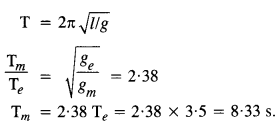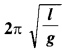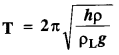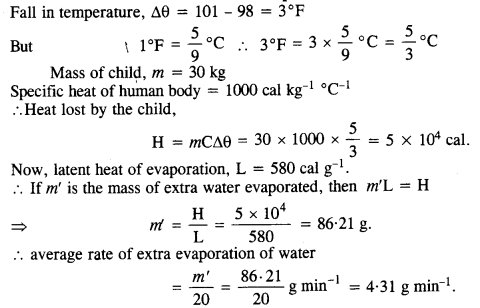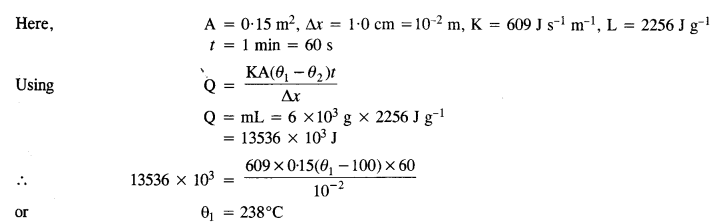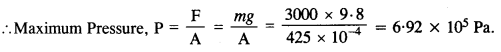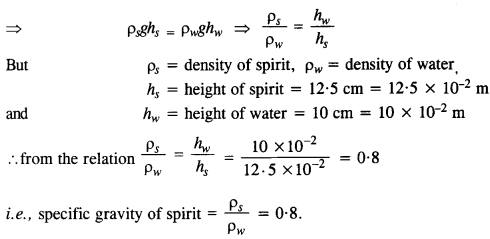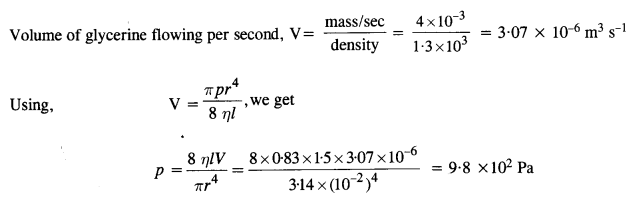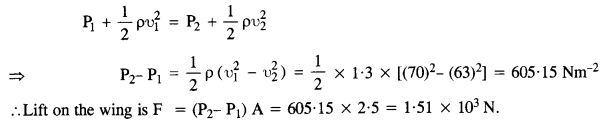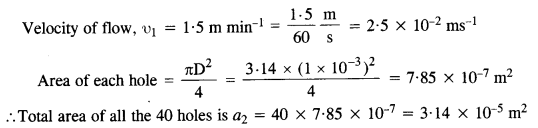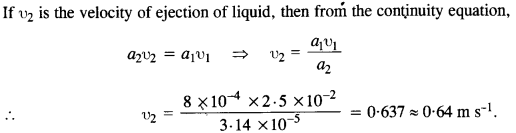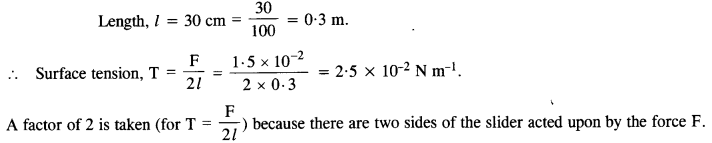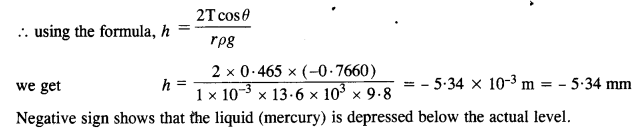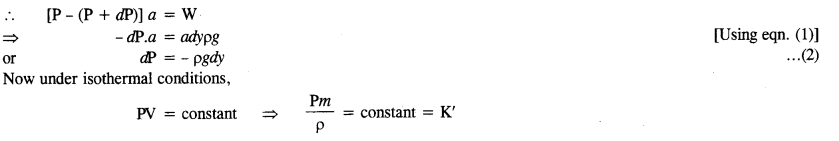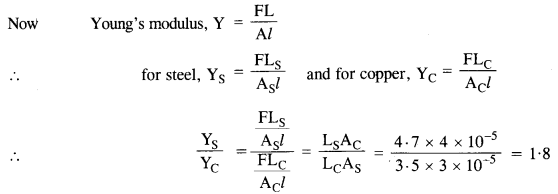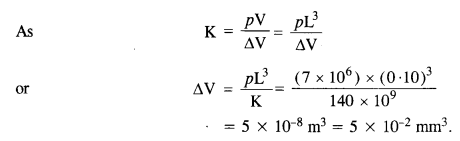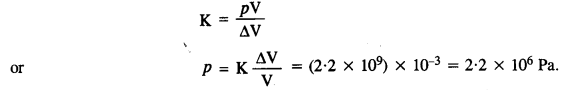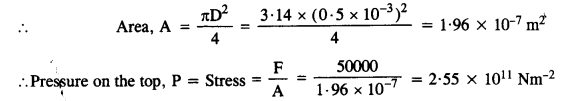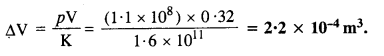Here we are providing NCERT Solutions for Class 11 English Hornbill Chapter 3 Discovering Tut: The Saga Continues. Students can get Class 11 English Discovering Tut: The Saga Continues NCERT Solutions, Questions and Answers designed by subject expert teachers.

Discovering Tut: The Saga Continues NCERT Solutions for Class 11 English Hornbill Chapter 3

Discovering Tut: The Saga Continues NCERT Text Book Questions and Answers

Discovering Tut: The Saga Continues Understanding the text

Question 1.
Give reasons for the following.

(i) King Tut’s body has been subjected to repeated scrutiny.
Answer:
1. In 1922, Howard Carter, A British archaeologist, discovered Tut’s tomb and in the process, cut the body to remove it from the coffin.

2. In 1968, a professor of anatomy x-rayed the mummy. He discovered that Tut’s breastbone and front ribs were missing.

3. In January 2005, the mummy was taken out for a CT scan.
King Tut was just a teenager when he died. He was the last heir of a powerful family that had ruled Egypt for centuries. He was laid to rest, laden with large quantities of gold, and eventually forgotten.

It was when his tomb was discovered that the modem world wondered why he had died at such an early age. The possibility of him being murdered could not be ruled out.

(ii) How are Carter’s investigation was resented.
Answer:
Howard Carter was the British archaeologist who in 1922 discovered Tut’s tomb. His investigation was resented because Carter’s men removed the mummy’s head and cut off nearly every major joint to separate Tut from his adornments. They, then, reassembled the remains on a layer of sand in a wooden box and put him back.

(iii) Carter had to chisel away the solidified resins to raise the king’s remains.
Answer:
The solidified material had to be cut away from below the limbs and chest before it was possible to raise King Tut out. This had to be done because if Carter had not cut the mummy free, thieves would have evaded the guards and tom the mummy apart to remove the gold that was buried with Tut.

(iv) Tut’s body was buried along with gilded treasures.
Answer:
Tut’s body was buried along with gilded treasures that remain the richest royal collection ever found. The beautiful works of art in gold were buried with everyday things he would want in the afterlife: board games, a bronze razor, linen undergarments, cases of food and wine. The Egyptian royals believed that they could take their riches with them after death.

(v) The boy king changed his name from Tutankhaten to Tutankhamun.
Answer:
King Tutankhaten changed his name to Tutankhamun, which meant “living image of Amun”, to show that he meant to restore the old ways. This was because Amenhotep IV, his predecessor, promoted the worship of the Aten, the sun disk, changed his name to Akhenaten, or “servant of the Aten”. He shocked the country by attacking Amun, a major god, smashing his images and closing his temples.

Question 2.
(i) List the deeds that led Ray Johnson to describe Akhenaten as “wacky”.
Answer:
According to Ray Johnson, Akhenaten was crazy because he started one of the strangest periods in the history of ancient Egypt. He promoted the worship of the Aten, the sun disk, changed his name to Akhenaten, or “servant of the Aten”, and moved the religious capital from the old city of Thebes to the new city of Akhetaten, known now as Amama. He further shocked the country by attacking Amun, a major god, smashing his images and closing his temples.

(ii) What were the results of the CT scan?
Answer:
A CT machine scanned the mummy from head to toe and created 1,700 digital X-ray images in cross section. Tut’s head was scanned in 0.62 millimetre slices to register its complicated structures to probe the secrets of his death. The neck vertebrae, other images of a hand, several views of the rib cage, and a transection of the skull showed that there was nothing amiss in his death.

(iii) List the advances in technology that have improved forensic analysis.
Answer:
Today diagnostic imaging can be done with computed tomography, or CT, by which hundreds of X-rays in cross section are put together like slices of bread to create a three-dimensional virtual body.

(iv) Explain the statement, “King Tut is one of the first mummies to be scanned—in death, as in life… ”
Answer:
King Tut is one of the first mummies to be studied under a CT scan. In real life, he was the Pharaoh—the leader of his country. Hence both in life and death he moved majestically ahead of his countrymen.

Discovering Tut: The Saga Continues Talking about the text

Discuss the following in groups of two pairs, each pair in a group taking opposite points of view.

Question 1.
Scientific intervention is necessary to unearth buried mysteries.
Answer:
Necessary

• Better tools for locating sites/information/life and death
• Better equipment to study unearthed facts for example, murder/natural death
• Evidence is evaluated scientifically
• Helps in ending doubts/fallacies through improved equipment
• Ends idle speculation through empirical proof

Unnecessary

• Engineers and scientists from Japan have not been able to find out how pyramids were built (quarrying/ transporting/placing stone) despite recreating/studying available data
• Scientific intervention destroys evidence at times
• Scientific knowledge is biased starts with a hypothesis. Investigation that begins with a predetermined outcome and searches for evidence to prove a foregone conclusion, disregards other perspectives. Research showed, for example, that the fact that Tut died of head injury was false

Question 2.
Advanced technology gives us conclusive evidence of past events.
Answer:
Gives evidence

• Recreates any event
• Better equipment and tools help in better understanding of events and situations
• Recreation and simulation of events helps in study
• Processes like carbon-dating help fix events in a timeline
• Technology like CT scans help in the study of unearthed material
• Remove fallacious beliefs

Does not give conclusive evidence

• Recreated events may not be authentic in context as information gaps may exist
• Science deals with hardcore facts; there maybe external factors in history that are as yet unknown
• Science judges and draws conclusions based on facts as they exist today; at times they may not be accurate in historical perspective
• Sometimes theories propagated may not be actual facts, for example, the theory that Tut was murdered
• Not an accurate determinant for human behaviour and other sociological facts

Question 3.
Traditions, rituals and funerary practices must be respected.
Answer:
Should be respected

• They are based on beliefs people held dear and they should be respected
• Disrespect to Amun the god most popular in ancient Egypt probably led to the downfall of Amonhotep IV
• People do not like these beliefs questioned
• These beliefs often prove to be scientifically beneficial
• We do not have the right to disrespect views of others

Question 4.
Knowledge about the past is useful to complete our knowledge of the world we live in.
Answer:
Useful

• Helps one to draw conclusions from the past events
• Makes one’s life richer by giving meaning to the books one reads, the cities one visits or the music one hears
• Broadens one’s outlook by presenting to one an admixture of races, a mingling of cultures and a spectacular drama of the making of the modem world out of diverse forces
• Enables one to grasp one’s relationship with one’s past
• Preserves the traditional and cultural values of a nation, and serves as a beacon of light, guiding society in confronting various crises
• A bridge connecting the past with the present and pointing the road to the future

Not useful

• History is layered, tribes came and went, kings, priests, religions and ideologies came and went, what spot of geography remained eternal, unnecessarily causes a divide
• Politicians appeal (selectively) to history, to rouse the rabble, inflaming people to violence, and naturally, the other
• Side will retaliate, justifying their equally murderous actions with their version of history

Discovering Tut: The Saga Continues Thinking about language

Question 1.
Read the following piece of information from The Encyclopedia of Language by David Crystal.
Answer:
Egyptian is now extinct: its history dates from before the third millennium B.C., preserved in many hieroglyphic inscriptions and papyrus manuscripts. Around the second century A.D., it developed into a language known as Coptic. Coptic may still have been used as late as the early nineteenth century and is still used as a religious language by Monophysite Christians in Egypt.

Question 2.
What do you think are the reasons for the extinction of languages?
Answer:

• With increasing disuse, not because the peoples themselves, with their cultural traditions, have dwindled away, but because the language has been overwhelmed by a more dominant one.
• A language needs a nation (in the broad sense of people conscious of a group identity) that sees it as ‘its own’failing this the language dies out
• A language’s social status determines its life or death. If its speakers turn away more and more from using a language that is perceived as conferring real benefits in everyday life, it dies out
• The introduction of a non-indigenous language that takes over all social functions
• The disappearance of a population that speaks that language
• Parents do not pass on a language to their children
• Population dislocation or relocation due to events like war, famine, earthquakes
• The emergence of a new world language
• The emergence of supra linguistic functions like banking information in another language

Question 3.
Do you think it is important to preserve languages?
Answer:
Language diversity is essential to the human heritage. Each and every language embodies the unique cultural wisdom of a group of people. The loss of any language is thus a loss for all humanity. It is essential to preserve languages in order to preserve fundamental human rights, and for the protection of minority groups. Language is an important marker of identity. Even when speaking the same language, social groups differentiate themselves by their dialect or the way they talk.

So, language offers a way of stating a resistance to cultural homogenisation. A native language goes beyond differentiation. It represents a whole cultural history. The need to define one’s roots, especially in the face of what can look like foreign hegemony, is powerful. ‘Linguistic diversity’ is a benchmark of cultural diversity. The death of a language is symptomatic of cultural death: a way of life disappears with the death of a language. Language is a cultural resource, and must be transmitted to children.

Question 4.
In what ways do you think we could help prevent the extinction of languages and dialects?
Answer:
Although approximately 6,000 languages still exist, many are under threat. There is an imperative need for language documentation, new methods, new policy initiatives and safeguarding strategies to enhance the vitality of these languages. The cooperative efforts of language communities, language professionals, NGOs and governments will be indispensable in countering this threat.

There is a pressing need to build support for language communities in their efforts to establish meaningful new roles for their endangered languages. One important issue in preserving a language is how widely it is used in written form. Prerequisites for the written use of a language are orthography development, literature production, and the teaching of mother-tongue literacy.

Discovering Tut: The Saga Continues Working with words

Question 1.
Given below are some interesting combinations of words. Explain why they have been used together.
Answer:

• ghostly dust devils – a dust devil is a whirlwind into which dust and debris gets caught up, making it visible and making it look like a ghost
• desert sky – blank/lifeless sky
• stunning artefacts – breathtakingly beautiful objects made by humans
• funerary treasures – jewels or precious objects relating to or suitable for a burial or funeral
• scientific detachment – methodical aloofness
• dark-bellied clouds – dark, bulging clouds
• casket grey – ash-coloured like a coffin
• eternal brilliance – endless lustre/radiance
• ritual resins – resins used in a system of rites
• virtual body – figure of the body generated by the computer
• The above descriptions are very vivid and make understanding/visualisation better.

Question 2.
Here are some commonly used medical terms. Find out their meanings.
(a) CT scan – A CT (computerised tomography) scanner is a medical imaging method employing tomography where digital geometry processing is used to generate a three-dimensional image of the internals of an object from a large series of two-dimensional X-ray images taken around a single axis of rotation.

(b) MRI – Magnetic Resonance Imaging (MRI) is a diagnostic medical imaging technique utilising the principles of nuclear magnetic resonance. MRI is viewed by many as the most versatile, powerful and sensitive diagnostic imaging modality available. Its medical importance can be summarised briefly as having the ability to non- invasively generate thin sections, functional images of any part of the body at any angle and direction in a relatively short period of time.

(c) tomography – production of body image; the technique of using ultrasound, gamma rays, or X-rays to produce a focussed image of the structures across a specific depth within the body, while blurring details at other depths

(d) autopsy – examination to find cause of death: the medical examination of a dead body in order to establish the cause and circumstances of death

(e) dialysis – medical filtering process: the process of filtering the accumulated waste products of metabolism from the blood of a patient whose kidneys are not functioning properly, using a kidney machine

(f) ECG – an electrocardiogram is a graphic produced by an electrocardiograph, which records the changes in the electrical current in the heart during heartbeats in the form of a continuous strip graph. The results of the ECG are used to tell whether the heart is performing normally or suffering from abnormalities.

(g) post mortem – examination made after death to determine the cause of death

(h) angiography – Angiography is the X-ray (radiographic) study of the blood vessels. Angiography is used to detect abnormalities, including narrowing or blockages in the blood vessels throughout the circulatory system and in some organs.

The procedure is commonly used to diagnose heart disease; to evaluate kidney function and detect kidney cysts or tumours; to map renal anatomy in transplant donors; to detect an abnormal bulge of an artery that can rupture leading to haemorrhage, tumour, blood clot, or abnormal tangles of arteries and veins in the brain; and to diagnose problems with the retina of the eye.

i) biopsy – removal of living tissue: the removal of a sample of tissue from a living person for laboratory examination

Discovering Tut: The Saga Continues Things to do

Question 1.
The constellation Orion is associated with the legend of Osiris, the god of the afterlife. Find out the astronomical
descriptions and legends associated with the following.
Answer:
(i) Ursa Major (Saptarishi mandala) – This is also known as the Great Bear, because of its shape, recognised early on by Romans and Native Americans. In Hindu mythology each of the stars represents one of the Saptarshis or seven sages.

(ii) Polaris (Dhruva tara) – Also known as the North star or pole star, the brightest star in the Ursa Minor constellation. In ancient Hindu literature Polaris was given the name Dhruva or immovable, fixed in one place.

(iii) Pegasus (Winged horse) – A bright constellation in the northern sky. Pegasus was depicted as a white winged stallion, one of the children of the Greek god Poseidon.

(iv) Sirius (Dog star) – From the ancient greek term for glowing, one of the brightest stars in the Earth’s night sky.
This is presented as Orion’s dog, who hunts for the Greek god Zeus.

(v) Gemini (Mithuna) – One of the zodiac constellations. The Latin word Gemini translates to twins, representing the pair Castor and Pollux, sons of the Spartan Queen Leda. Similarly, Mithuna in Indian astrology stands for couple or union.

Question 2.
Some of the leaves and flowers mentioned in the passage for adorning the dead are willow, olive, celery, lotus, cornflower. Which of these are common in our country?
Answer:
The Indian willow, lotus, and cornflower are found in several places across the country.

Question 3.
Name some leaves and flowers that are used as adornments in our country.
Answer:
Leaves used for adornment in India include mango leaves, banana leaves, tulsi leaves, banyan leaves, peepal tree leaves and so on. Flowers used for adornment include marigolds, roses, lotus flowers, jasmine flowers, hibiscus flowers and so on.

Here we are providing NCERT Solutions for Class 11 English Hornbill Chapter 4 Landscape of the Soul. Students can get Class 11 English Landscape of the Soul NCERT Solutions, Questions and Answers designed by subject expert teachers.

Landscape of the Soul NCERT Solutions for Class 11 English Hornbill Chapter 4

Landscape of the Soul NCERT Text Book Questions and Answers

Landscape of the Soul Understanding the text

Question 1.
(i) Contrast the Chinese view of art with the European view with examples.
Answer:
In the Chinese view, art is a representation of the mind or the spirit, whereas in the European view, it is of the figure or the body. While Chinese paintings reveal the inner world, the European paintings lay emphasis on a true representation of the physical appearance of the subject.

The examples of paintings by Wu Daozi and Quinten Metsys are representative of this difference. The painting by Metsys is life-like. It is an exact representation of a fly. The painting of Daozi, on the other hand, is not only beautiful but alive too. It has a way within that only the painter is aware of.

(ii) Explain the concept of shanshul
Answer:
Shanshui expresses the Chinese view of art as a conceptual space. It literally means mountain-water, which, when used together, represent the word ‘landscape’. Mountain and water represent two complementary poles of an image. They are essentially Yang and Yin, two seemingly opposing forces complementing each other for a unified whole, the landscape. The mountain is Yang – stable, warm and dry in the sun, reaching vertically towards the sky, while the water is Yin – fluid, moist and cool, horizontal and resting on the earth.

Question 2.
(i) What do you understand by the terms ‘outsider art’ and ‘art brut’ or ‘raw art’?
Answer:
Outsider art is a term that describes artistic creations by someone who has no formal training to be an artist. However, they show unmistakable artistic talent and insight, and their work poses a stimulating contrast to the mainstream art. Art brut or raw art, a term used by the painter Jean Dubuffet, refers to art forms which are outside the conventions of the mainstream art world. They are in their raw state in respect of their cultural and artistic influences.

(ii) Who was the “untutored genius who created a paradise ” and what is the nature of his contribution to art?
Answer:
The untutored genius was Nek Chand Saini. He created a rock garden in Chandigarh. The garden is entirely sculpted with stones and recycled materials.

Landscape of the Soul Talking about the text 

Discuss the following statements in groups of four.

Question 1.
“The Emperor may rule over the territory he has conquered, but only the artist knows the way within.”
Answer:
The Tang Emperor Xuanzong may have commissioned Wu Daozi to create a great work of art. The Emperor wields power over his territory and wealth to control the lives of his people. However, the artist who creates a work of art understands his work in a manner that nobody can. In Wu Daozi’s case, we see that the Emperor cannot enter the work of art as Wu does, because despite all his power, he can never be a part of the space shared by Wu and his work of art. That relationship is too sacred and personal a space for anybody to enter.

Question 2.
“The landscape is an inner one, a spiritual and conceptual space.”
Answer:
This landscape is described by the article as Shanshui, which translates to mountain-water. Two different sides to an artwork, just like Yin and Yang. The mountain which reaches towards heaven and the water which treads across the earth. These two sides therefore also portray the spiritual which transcends into the heavenly, and the conceptual which wades through the earth. In this landscape the artist has been able to capture a scene not just as he would view it, but more than that, subjectively the multiple ways in which his mind would think about a landscape. He wants the viewer to be able to enter and understand his mind.

Landscape of the Soul Thinking about language

Question 1.
Find out the correlates of Yin and Yang in other cultures.
Answer:
In Christianity, this can be seen in the struggle between being heaven for those who repent of sin and hell for those who do not. In Vedanta philosophy in the Gita this can be seen in the division between Akshara, the invisible soul and the Kshara, the visible body. Similarly many other cultures have similar binaries between black and white, good and evil and so on.

Question 2.
What is the language spoken in Flanders?
Answer:
Although Flanders is in Belgium, the language primarily spoken in the region is Dutch.

Landscape of the Soul Working with words

I. The following common words are used in more than one sense.
panel , studio , brush , essence , material

Examine the following sets of sentences to find out what the words, ‘panel’ and ‘essence’ mean in different contexts.

Question 1.
(i) The masks from Bawa village in Mali look like long panels of decorated wood.
Answer:
A flat rectangular piece of wood

(ii) Judge H. Hobart Grooms told the jury panel he had heard the reports.
Answer:
A small group of people selected to pass a judgement

(iii) The panel is laying the groundwork for an international treaty.
Answer:
A small group of people selected to represent a larger group

(iv) The glass panels of the window were broken.
Answer:
A flat rectangular piece of glass, which is part of a window

(v) Through the many round tables, workshops and panel discussions, a consensus was reached.
Answer:
A small group of people that participates in a discussion amongst themselves

(vi) The sink in the hinged panel above the bunk drains into the head.
Answer:
A rectangular case used to keep something

Question 2.
(i) Their repetitive structure must have taught the people around the great composer the essence of music.
Answer:
What goes into composing music

(ii) Part of the answer is in the proposition; but the essence is in the meaning.
Answer:
The actual answer

(iii) The implications of these schools of thought are of practical essence for the teacher.
Answer:
Practically the most important aspect

(iv) They had added vanilla essence to the pudding.
Answer:
A strong liquid used to add flavour

II. Now find five sentences each for the rest of the words to show the different senses in which each of them is used.

Studio

• I live in a studio apartment.
• They hired a studio to record their music album.
• That is one of the most famous studios because the biggest actors work for them.
• Can we get a studio audience for the news channel’s evening show?
• He owns a ballet studio on the other side of the city.

Material

• This factory produces a lot of waste material.
• Thankfully the storm did not cause any material damage in the town.
• The material foundations of the building have been laid.
• Do you have the material needed to make the dress?
• He is very fond of material things, and therefore can never be trusted.

Brush

• He needs to brush up on his knowledge of the subject.
• I used to brush my shoes but then I could no longer find the time.
• She needs a new brush to paint with.
• He would brush past me every day on the metro because he was in such a hurry.

Noticing form

• A classical Chinese landscape is not meant to reproduce an actual view, as would a Western figurative painting.
• Whereas the European painter wants you to borrow his eyes and look at a particular landscape exactly as he saw it, from a specific angle, the Chinese painter does not choose a single viewpoint.
  The above two examples are ways in which contrast may be expressed. Combine the following sets of ideas to show the contrast between them.

Question 1.
(i) European art tries to achieve a perfect, illusionistic likeness
(ii) Asian art tries to capture the essence of inner life and spirit.
Answer:
European art tries to perfect the image of the world it captures, trying to be as close to real life as possible, whereas Asian art tries to go beyond the physical world to capture the subjective picture, as one’s inner life and spirit would see it.

Question 2.
(i) The Emperor commissions a painting and appreciates its outer appearance,
(ii) The artist reveals to him the true meaning of his work.
Answer:
While the Emperor pays for the artwork and appreciates the physical depiction that is portrayed, only the artist who carefully crafts the painting understands the true meaning of his work.

Question 3.
(i) The Emperor may rule over the territory he has conquered
(ii) The artist knows the way within.
Answer:
While the Emperor wields power to rule over his kingdom, only the artist understands the method he uses in his artwork, and how the work reflects the mysterious nature of the universe.

Landscape of the Soul Things to do

Question 1.
Find out about as many Indian schools of painting as you can. Write a short note on the distinctive features of each school.
Answer:
Mughal Painting: This began during the reign of Humayun, the 2nd Mughal emperor. This style of painting was influenced heavily by Persian art. Mughal painters used bright colours, focussed on the fine details of costumes and gold, and also captured scenes of wildlife and nature. Most portraits of famous courtiers and royalty had the subjects posing with particular gestures of hands and face. Considered the golden period for miniature painting in India.

Rajasthani Painting: This style of painting began under the Rajput emperors after the 17th century. This began after the relationship between the Mughal and the Rajasthani empires improved, leading to influence of Mughal painting on local artists. Most of the painters chose their subjects as scenes taken from Hindu religious poetry. Traditional painting on walls was replaced by miniature paintings.

Pahari Painting: Most of these painters practiced their art in places like Kashmir, Punjab, Uttar Pradesh and neighbouring territories. Here also a lot of bright colours were used, with themes that varied from focussing on nature to depicting the gracefulness of female subjects .

Bengal School of Art: A more recent school which began during the British Raj in the early 20 century. It was a part of the Indian nationalist movement, which sought to distinguish itself from art forms promoted in British art schools across India. Therefore the themes focussed on by painters were also those that may help promote Indian nationalism. Some other schools of art in Indian include Tribal painting, Kerala mural painting and so on.

Question 2.
Find out about experiments in recycling that help in environmental conservation.
Answer:
Some recent efforts to cut down on environmental pollution include the setting up of recycling centers to collect material that can be used again like tin cans, plastic bottles and containers, electronic equipment and waste paper. The setting up of trash bins to separate degradable and non-degradable waste has also helped reduce the amount of waste in garbage dumps. Further inventions like biodegradable bags and bottles, cars running on electricity or water instead of petrol, robots to sift through trash cans to utilise waste, and so on have helped the process of environmental conservation.

Here we are providing NCERT Solutions for Class 11 English Snapshots Chapter 3 Ranga’s Marriage. Students can get Class 11 English Ranga’s Marriage NCERT Solutions, Questions and Answers designed by subject expert teachers.

Ranga’s Marriage NCERT Solutions for Class 11 English Snapshots Chapter 3

Ranga’s Marriage NCERT Text Book Questions and Answers

Question 1.
Comment on the influence of English – the language and the way of life – on Indian life as reflected in the story. What is the narrator’s attitude to English?
Answer:
The narrator has very poignantly brought out the influence of English language on the way of life in the story. As the title reflects, it is not Vivaha but “marriage” because Ranga, having had the opportunity to go for higher education to Bangalore is to an extent influenced by the West and he could talk in English. Ranga’s homecoming was a great event. People rushed announcing his arrival and went to look at him.

Unlike the people in the village, Ranga said he would not get married immediately but would wait and find the right girl to get married to. He quoted the example of an officer who got married six months back when he was about thirty and his wife, twenty-five. He liked the idea of marrying a mature girl who would understand him, unlike a childish bride. Quoting the classic tale of Shakuntala, he said that Dushyantha would not have fallen in love with Shakuntala if she were young. He said that a man should marry a girl he admires and it would be impossible to admire an immature girl.

Question 2.
Astrologers’ perceptions are based more on hearsay and conjecture than what they learn from the study of the stars. Comment with reference to the story.
Answer:
Astrologers’ perceptions are based more on tittle-tattle and assumption than what they learn from the study of the stars. This is brought out effectively through the character of a Shastri in the story. The narrator told the Shastri about his ploy to bring Ranga and Ratna together before he took Ranga to him. As planned, the Shastri pretended to make certain calculations and said that his problem had something to do with a girl.

He added that the name of the girl was something found in the ocean such as Kamala (the lotus), Pachchi, (the moss) or Ratna (the precious stone). The narrator said that the girl in Rama Rao’s house was Ratna. The Shastri was very positive about the proposal working out. Later that . evening, the narrator joked with the Shastri about his predictions based on the information he gave but Shastri did not like it. He said “…Don’t forget, I developed on the hints you had given me.”

Question 3.
Indian society has moved a long way from the way the marriage is arranged in the story. Discuss.
Answer:
The Indian society has certainly moved a long way from the way the marriage is arranged in the story. In the story, firstly, Ratna is a child of eleven. The marriage of a girl of this age is now a criminal offense. Ranga falls in love with Ratna, who is no more than a child when he hears her sing. Unlike the story, marriages are arranged but based on compatibility and maturity of the couple. The predictions of an astrologer, like the Shastri in the story, are no longer the gospel truth. Mutual consent of the couple is given more importance than that of the matchmakers, like the narrator.

Question 4.
What kind of a person do you think the narrator is?
Answer:
The narrator is an affable man who is intelligent and a keen observer. He notices Ranga’s expressions of delight and disappointment and deals with the situation accordingly. He is proud of his roots and talking of his village he says, “I am not the only one who speaks glowingly of Hosahalli.” He does not like the idea of people aping the West blindly. He talks disparagingly of them, “they are like a flock of sheep.

One sheep walks into a pit, the rest blindly follow it.” The influence of English, on the native language, too meets with criticism—“What has happened is disgraceful, believe me.” Ranga’s western concepts of marriage, too, do not appeal to him. He feels “distressed (as) the boy who (he) thought would make a good husband, had decided to remain a bachelor.” But he anyway decides to play matchmaker and arrange Ranga’s marriage.

His curiosity to know what the people were up to when they went to Ranga’s house makes him follow them. He writes, “Attracted by the crowd, I too went and stood in the courtyard.” A traditionalist by nature he is happy to note that,Ranga “bent low to touch my feet.” However, he knows how to use situations to his advantage. He decides that Ratna is just the right girl for Ranga. He plots a situation, wherein Ranga hears her sing and falls in love with her. Then, he takes him to the Shastri who has been tutored by him. He is a traditionalist but a well-meaning person. He takes onto himself the responsibility of getting Ranga married and sees it through.

NCERT Solutions for Class 11 Physics Chapter 15 Waves

These Solutions are part of NCERT Solutions for Class 11 Physics. Here we have given NCERT Solutions for Class 11 Physics Chapter 15 Waves

Question 1.
A string of mass 2.50 kg is under a tension of 200 N. The length of the stretched string is 20.0 cm. If the transverse jerk is struck at one end of the string, how long does the disturbance take to reach the other end ?
Answer:
Tension in the string, T = 200 N ; Mass of string, M = 2.50 kg ;
Length, l= 20.0 cm = 20 x 10^-2 m

Question 2.
A stone dropped from the top of a tower of height 300 m high splashes into the water of a pond near the base of the tower. When is the splash heard at the top? Given that the speed of sound in air is 340 m s^-1. (g = 9.8 m s^-2)
Answer:
Here, h=300 m, g = 9.8 m s^-2 and velocity of sound,υ= 340 m s^-1
Let t₁ be the time taken by the stone to reach the surface of the pond.

.’. Total time after which sound of splash is heard = t₁+ t₂= 7.82 + 0.88 = 8.7 s.

Question 3.
A steel wire has a length of 12.0 m and a mass of 2.10 kg. What should be the tension in the wire so that the speed of a transverse wave on the wire equals the speed of sound in dry air at 20°C = 343 m s^-1.
Answer:
Mass, M= 2.10 kg, Length, l = 12.0 m.

Question 4.
Use the formula

to explain why the speed of sound in air
(a) is independent of pressure.
(b) increases with humidity.
(c) increases with temperature
Answer:


Question 5.
You have learnt that a travelling wave in one dimension is represented by a function y = f(x,t) where x and t must appear in the combination x – υt or x + υt, i.e. y = F(x ± υt.) Is the converse true ? Examine if the following functions for y can possibly represent a travelling wave:
(a) (x – υt)²
(b) log [(x +υt )/x₀]
(c)exp [-(x + υt)/x₀]
(d) 1/(x + υt)
Answer:
The converse of the above statement is not true. For a function to represent a travelling wave, the function must be finite and well defined at any point and at any time. Only the function (C) satisfies this condition and is a wave function.

Question 6.
A bat emits the ultrasonic sound of frequency 1000 kHz in air. If the sound meets a water surface, what is the wavelength of (a) the reflected sound, (b) the transmitted sound?
Speed of sound in air is 340 m s^-1 and in water 1486 m s-1.
Answer:
Here v = 1000 kHz = 1000 x 10³ Hz = 10⁶ Hz
Velocity of sound in air, υ_a = 340 ms^-1; the velocity of sound in water, υ_w = 1486 ms^-1
(a) For reflected sound, medium remains the same

Question 7.
A hospital uses an ultrasonic scanner to locate tumours in a tissue. What is the wavelength of sound in the tissue in which the speed of sound is 1.7 km s^-1 ? The operating frequency of the scanner is 4.2 MHz.
Answer:
Here,υ = 1.7kms^_1 = 1.7 x 1000 ms^_1 = 1700 ms^_1
Frequency, v= 4.2 MHz = 4.2 X 10⁶ Hz

Question 8.
A transverse harmonic wave on a string is described by
y (x, t) = 3.0 sin (361 + 0.018 x + π/4) where x and y are in cm and t in s. The positive direction of x is from left to right.
(a) Is this a travelling wave or a stationary wave?
(b) If it is travelling, what are the speed and direction of its propagation?
(c) What are its amplitude and frequency ?|
(d) What is the initial phase at the origin?
(e) What is the least distance between two successive crests in the wave?
Answer:
(a) It is traveling which is propagating from right to left. Comparing the given equation with y (x, t) = r sin (ωt+kr+φ)

Question 9.
For the wave described in Exercise 8, plot the displacement (y) versus (f) graphs for x = 0, 2, and 4 cm. What is the shape of these graphs? In which aspects do the oscillatory motion in travelling waves differ from one point to another: amplitude, frequency, or phase?
Answer:
The transverse harmonic wave is

The graph obtained is sinusoidal. Similar graphs are obtained for x = 2 cm and x = 4 cm. The oscillatory motion in the travelling wave only differs in respect of phase. The amplitude and frequency of oscillatory motion remain the same in all the cases.

Question 10.
For the traveling harmonic wave
y (x, t) = 2.0 cos 2π(10t – 0.0080 x + 0.35)
where x and y are in cm and t in s. Calculate the phase difference between the oscillatory motion of two points separated by a distance of
(a)4 m
(b) 0.5 m
(c) λ/2
(d) 3λ/4.
Answer:
Comparing the equation given in the question, with the standard equation of travelling wave:

Question 11.
The transverse displacement of a string (clamped at its both ends) is given by

where x and y are in m and t in s. The length of the string is 1.5 m and its mass is 3.0 x 10^-2 kg.
Answer the following:
(a) Does the function represent a travelling wave or a stationary wave?
(b) Interpret the wave as a superposition of two waves traveling in opposite directions. What are the wavelength, frequency, and speed of each wave?
(c) Determine the tension in the string.
Answer:
(a) The function does not represent a travelling wave. It represents a stationary wave.
(b) Using the relation

Question 12.
(1) For the wave on a string described in Exercise 11, do all the points on the string oscillate with the same (a) frequency, (b) phase, (c) amplitude? Explain your answers, (2) What is the amplitude of a point 0.375 m away from one end?
(a) All the points on the wave have
(b) same frequently everywhere except at the nodes.
(c) same phase everywhere in a loop except at the nodes.
(d) different amplitude.
(2) Here, x = 0.375 m, 1 = 0

Question 13.
Given below are some functions of x and y to represent the displacement (transverse or longitudinal) of an elastic wave. State which of these represent
(1) a travelling wave,
(2) a stationary wave or
(3) none at all:
(a) y = 2 cos (3x) sin (10t)
(b)
(c) y = 3 sin (5x – 0.5t) + 4 cos (5x – 0.5t)
(d) y = cos x sin t- cos 2x sin 2t
Answer:
(a) It represents a stationary wave.
(b) It does not represent either a travelling wave or a stationary wave.
(c) It is a representation of the travelling wave.
(d) It is a superposition of two stationary waves.

Question 14.
A wire stretched between two rigid supports vibrates in its fundamental mode with a frequency of 45 Hz. The mass of the wire is 3.5 x 10^-2 kg and its linear density is
4.0 x 10^-2 kg m^_1. What is
(a) the speed of a transverse wave on the string, and
(b) the tension in the string?
Answer:
Here, v = 45 Hz ; mass of wire, M = 3.5 x 10^-2 kg

Question 15.
A meter-long tube open at one end, with a movable piston at the other end, shows resonance with a fixed frequency source (a tuning fork of frequency 340 Hz) when this tube length is 25.5 cm or 79.3 cm. Estimate the speed of sound in the air at the temperature of the experiment. The edge effects may be neglected.
Answer:
Let v be the fundamental frequency of the closed-end organ pipe for the length
l_x = 25.5 cm

Question 16.
A steel rod 100 cm long is clamped at its middle. The fundamental frequency of longitudinal vibrations of the rod are given to be 2.53 kHz. What is the speed of sound in steel?
Answer:
l = 100 cm = 1 m  f = 2.53 kHz
Since the rod is champed at the middle, a node is formed and since the rod is oscillating at a fundamental frequency of 2.53 kHz antinodes are formed at both ends as shown in the figure.
∴ l = λ/4 × 2 = λ/2 ⇒ λ = 2l
λ = 2 × 1 = 2 cm
We know that
v = fλ = 2.53 × 10³ × 2
= 5.06 × 10 ³ ms^-1

Question 17.
A pipe 20 cm long is closed at one end. Which harmonic mode of the pipe is resonantly excited by a 430 Hz source? Will the same source be in resonance with the pipe if both ends are open? (speed of sound in air is 340 ms^-1).
Answer:

This is the first harmonic. The second harmonic is 3v i.e., 1275 Hz, the third harmonic is 5v i.e., 2125 Hz etc. Therefore, only the first harmonic of frequency 425 is rasonantly excited by a 430 Hz source.If both the ends of the pipe are open, the fundamental frequency is given by

The second, third, fourth… etc. harmonics have frequencies 2v’, 3v’, 4v’……. i.e., 1700 Hz, 2550 Hz, 3400 Hz etc.No harmonic can be excited by the 430 Hz source in this case.

Question 18.
Two sitar strings A and B playing the note ‘Ga’ are slightly out of tune and produce beats of frequency 6 Hz. The tension in string A is slightly reduced and the beat frequency is found to reduce to 3 Hz. If the original frequency of A is 324 Hz, what is the frequency of B?
Answer:
Let v₁ and v₂ be the frequencies of strings A and B respectively
Then, v₁= 324 Hz, v₂ = ?
Number of beats, b = 6
v₂ = v₁± b = 324 ± 6
i.e.,   v₂ = 330 Hz or 318Hz.
Since the frequency is directly proportional to square root of tension, on decreasing the tension in the string A, its frequency v₁; will be reduced i.e., number of beats will increase if v₂ = 330 Hz. This is not so because a number of beats becomes 3. Therefore, it is concluded that the frequency v₂ = 318 Hz because on reducing the tension in string A, its frequency may be reduced to 32l Hz, thereby giving 3 beats with v₂ = 318 Hz.

Question 19.
Explain why (or how) :
(a) in a sound wave, a displacement node is a pressure antinode and vice versa.
(b) bats can ascertain distances, directions, nature and sizes of the obstacles without any “eyes”.
(c) a violin note and sitar note may have the same frequency, yet we can distinguish between the two notes.
(d) solids can support both longitudinal and transverse waves, but only longitudinal waves can propagate in gases, and
(e) The shape of a pulse gets distorted during propagation in a dispersive medium.
Answer:
(a) In a sound wave, a decrease in displacement i..e. displacement node causes an increase in the pressure there i.e, a pressure antinode is formed. Also, an increase in displacement is due to the decrease in pressure.

(b) Bats emit ultrasonic waves of high frequency from their mouths. These waves after being reflected back from the obstacles on their path are observed by the bats. These waves give them an idea of distance, direction, nature and size of the obstacles.

(c) The quality of a violin note is different from the quality of sitar. Therefore, they emit different harmonics which can be observed by human ear to differentiate between the two notes.

(d) This is due to the fact that gases have only the bulk modulus of elasticity whereas solids have both, the shear modulus as well as the bulk modulus of elasticity.

(e) A pulse of sound consists of a combination of waves of different wavelength. In a dispersive medium, these waves travel with different velocities giving rise to the distortion in the wave.

Question 20.
A train, standing at the outer signal of a railway station blows a whistle of frequency 400 Hz in still air. (1) What is the frequency of the whistle for a platform observer when the train (a) approaches the platform with a speed of 10 ms^-1, (b) recedes from the platform with a speed of 10 ms^-1 ? (2) What is the speed of sound in each case ? The speed of sound in still air can be taken as 340 ms^-1.
Answer:
Here, frequency of whistle, v = 400 Hz,
Speed of sound, v = 340 ms^-1; Speed of train, v_s = 10 m s^-1,
(1) (a) When the train approaches the platform i.e., the observer at rest,

Question 21.
A train, standing in a station-yard, blows a whistle of frequency 400 Hz in still air. The wind starts blowing in the direction from the yard to the station at a speed of 10 ms^-1. What are the frequency, wavelength, and speed of sound for an observer standing on the station’s platform? Is the situation exactly identical to the case when the air is still and the observer runs towards the yard at a speed of 10 ms^-1? The speed of sound in still air can be taken as 340 ms^-1.
Answer:
Frequency of source of the sound, v = 400 Hz.
Speed of sound, υ= 340 ms^-1; Speed of wind, υ_m = 10 ms^-1.
Effective speed of sound = Speed of sound + Speed of air
= υ + υ_m = 340 + 10 = 350 ms^-1.
The source, as well as the listener, are both at rest. Therefore, there is no relative motion.

Since the source of sound is at rest, the wavelength A does not change
.’. wavelength = 0.875 m.
Also, speed of sound = 340 + 0 = 340 ms^-1
The situations are altogether different, because both the observer and the source are in motion with respect to the medium in this case.

Question 22.
A traveling harmonic wave on a string is described by
y (x,t) = 7.5 sin (0.0050 x + 12t + π/4)
(a) What are the displacement and velocity of oscillation of a point at x = 1 cm, and t = 1 s? Is this velocity equal to the velocity of wave propagation?
(b) Locate the points of the string which have the same transverse displacements and velocity as the x = 1 cm point at t = 2s, 5s, and 11s.
Answer:
The traveling harmonic wave is

(b) All the point, located at distance nλ, (where n is an integer) from the point x = 1 have the same velocity and displacement.

Question 23.
A narrow sound pulse (for example, a short pip by a whistle) is sent across a medium, (a) Does the pulse have a definite (1) frequency, (2) wavelength, (3) speed of propagation ? (b) If the pulse rate is 1 after every 20 s, (that is the whistle is blown for a split of second after every 20 s), is the frequency of the note produced by the whistle equal to 1/20 or 0.05 Hz? ¹
Answer:
1.  The pulse does not have a definite frequency and definite wavelength but has a definite speed of propagation.
2.  The frequency of the note is not 1/20 Hz or 0.05 Hz. It is only the frequency of repetition of the whistle.

Question 24.
One end of a long string of linear mass density 8.0 x 10^-3 kg m^-1 is connected to an electrically driven tuning fork of frequency 256 Hz. The other end passes over a pulley and is tied to a pan containing a mass of 90 kg. The pulley end absorbs all the incoming energy so that reflected waves at this end have negligible amplitude. At t = 0, the left end (fork end) of the string x = 0 has zero transverse displacement (y = 0) and is moving along positive y- direction. The amplitude of the wave is 5.0 cm. Write down the transverse displacement y as function of x and t that describes the wave on the string.
Answer:
Here, mass per unit length, m = linear mass density = 8 x 10^-3 kg m³.
Tension in the string, T = 90 kg = 90 x 9.8 = 882 N
Frequency,  v = 256 Hz ; Amplitude, r = 5.0 cm = 5 x 10^-2 m. Hz
The wave produced in the string has velocity υ given by


Question 25.
A SONAR system fixed in a submarine operates at a frequency of 40.0 kHz. An enemy submarine moves towards the SONAR with a speed of 360 km h^-1. What is the frequency of sound reflected by the submarine? Take the speed of sound in water to be 1450 ms^-1.
Answer:
Here, frequency of SONAR(source )=40.0 kHz = 40 x10³

Question 26.
Earthquakes generate sound waves inside the earth. Unlike a gas, the earth can experience both transverse (S) and longitudinal (P) sound waves. Typically the speed of the S wave is about 4.0 kms^-1, and that of P wave is 8.0 kms^-1. A seismograph records P and S waves from an earthquake. The first P wave arrives 4 min before the first S wave. Assuming the waves travel in a straight line, how far away does the earthquake occur?
Answer:
Let υ₁ and υ₂ be the velocities of two waves respectively and t₁ and t₂ is the time taken by them to travel to the position of a seismograph.

Question 27.
A bat is fitting about in a cave, navigating via ultrasonic bleeps. Assume that the sound emission frequency of the bat is 40 kHz. During one fast swoop directly toward a flat wall surface, the bat is moving at 0.03 times the speed of sound in air. What frequency does the bat hear reflected off the wall ?
Answer:
Here, frequency of sound emitted by bat, v = 40 kHz
Velocity of bat υ_s= 0.03 u, where 0 is the velocity of sound.
The bat is moving towards the flat wall. This is the case of source in motion and the observer at rest. Therefore, the frequency of sound reflected at the wall is

The frequency v’ is reflected by the wall and is again received by the bat moving towards the wall. This is the case of an observer moving towards the source with velocity
υ₀= 0.03υ.
.’. frequency observed by bat,

We hope the NCERT Solutions for Class 11 Physics Chapter 15 Waves, help you. If you have any query regarding NCERT Solutions for Class 11 Physics Chapter 15 Waves, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 11 Physics Chapter 14 Oscillations

These Solutions are part of NCERT Solutions for Class 11 Physics. Here we have given NCERT Solutions for Class 11 Physics Chapter 14 Oscillations

Question 1.
Which of the following examples represent periodic motion?
(a) A swimmer completing one (return) trip from one bank of a river to the other and back.
(b) A freely suspended bar magnet displayed from its N-S direction and released.
(c) A hydrogen molecule rotating about its center of mass.
(d) An arrow released from a bow.
Answer:
(b) and (c).
(Swimmer does not repeat trip after regular interval of time. Similarly, arrow released from a bow is not periodic).

Question 2.
Which of the following examples represent (nearly) simple harmonic motion and which represent periodic but not simple harmonic motion?
(a) the rotation of earth about its axis.
(b) motion of an oscillating mercury column in a U-tube.
(c) motion of a ball bearing inside a smooth curved bowl, when released from a point slightly above the lower most point.
(d) general vibrations of a polyatomic molecule about its equilibrium position.
Answer:
(b) and (c) represent SHM in particular, (a) represents the period motion, (d) represents a superposition of a number of simple harmonic motions and is a periodic motion not necessarily S.H.M.

Question 3.
Figure depicts’four x-t plots for linear motion of a particle. Which of the plots represents periodic motion ? What is the period of motion (in case of periodic motion) ?

Answer:
Fig (b) and (d) represent periodic motions and the time period of each of these is 2 second, (a) and (c) are non periodic motions.

Question 4.
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion ? Give period for each case of periodic motion : (ω is any positive constant).
(a) sin ωt – cos ωt
(b) sin² ωt
(c) 3 cos (π/4 — 2ωt)
(d) cos ωt + cos 3ωt + cos 5ωt
(e) exp (- ω²t² )
(f) 1 +ωt +ω²t²
Answer:

Question 5.
A particle is in linear simple harmonic motion between two points, A and B, 10 cm apart. Take the direction from A to B as the positive direction and give the signs of velocity, acceleration and force on the particle when it is (a) at the end A, (b) at the end B, (c) at the mid-point of AB going towards A, (d) at 2 cm away from B going towards A, (e) at 3 cm away from A going towards B, and (f) at 4 cm away from A going towards A.
Answer:
A and B form the extreme positions and the center of line AB (let it be O) forms the mean position.

(a) At the end A, e., extreme position, velocity is zero, acceleration and force are directed towards O and are positive.

(b) At the end B, e., second extreme position, velocity is zero whereas the acceleration and force are directed towards the point O and are negative.

(c) At the mid point 0, while going towards A, velocity is negative and maximum. The acceleration and force are both zero.

(d) At a distance 2 cm away from B going towards A, velocity is negative. Since acceleration and force are also directed towards O, both of them are negative.

(e) At a distance 3 cm away from A going towards B, velocity is positive. Since the acceleration and force are directed towards 0 from A, both of these are positive.

(f) At a distance of 4 cm away from A and going towards A, velocity is directed along BA, therefore, it is positive.Since acceleration and force are directed towards OB, both of them are positive.

Question 6.
Which of the following relationships between the acceleration a and the displacement x of a particle involve simple harmonic motion ?
(a) a = 0.7x           (b) a = – 200 x²        (c) a = -10x                   (d) a = 100³
Answer:
Only the relation (c) represents the simple harmonic motion because for S.H.M., a a – x.

Question 7.
The motion of a particle executing simple harmonic motion is described by the displacement function, x(t) = A cos (cos ωt + Φ)
If the initial (t = 0) position of the particle is 1 cm and its initial velocity is co cm/s, what are its amplitude and initial phase angle ? The angular frequency of the particle is n s^_1. If instead of the cosine function, we choose the sine function to describe the SHM : x = B sin (at + a), what are the amplitude and initial phase of the particle with the above initial conditions ?
Answer:

Question 8.
A spring balance has a scale that reads from 0 to 50 kg. The length of the scale is 20 cm. A body suspended from this balance,,when displaced and released, oscillates with a period of 0.6 s. What is the weight of the body ?
Answer:
Length of scale = 20 cm = 0.20 m
Total scale reading = 50 kg
∴ F = mg = 50 x 9.8 = 490 N
x= 20 cm = 0.20 m

Question 9.
A spring with a spring constant 1200 N m^-1 is mounted on a horizontal table as shown in Fig. A mass of 3 kg is attached to the free end of the spring. The mass is then pulled sideways to a distance of 2.0 cm and released. Determine
(1) the frequency of oscillations,
(2) maximum acceleration of the mass, and
(3) the maximum speed of the mass.

Answer:
Here k = 1200 N m^_1, m = 3 kg, A = 2.0 cm = 2 x 10^-2 m

Question 10.
In Exercise 9, let us take the position of mass when the spring is unstreched as x= 0, and the direction from left to right as the positive direction of x-axis. Give x as a function of time t for the oscillating mass if at the moment we start the stopwatch (t = 0), the mass is
(a) at the mean position,
(b) at the maximum stretched position, and
(c) at the maximum compressed position.
In what way do these functions for SHM differ from each other, in frequency, in amplitude or the initial phase ?
Answer:
Here, the maximum displacement = Amplitude A = 2 cm
(a) If the time t = 0 at x = 0, the displacement can be expressed as the sine function of t.
x(t) = A sin ωt

Question 11.
Following figures correspond to two circular motions. The radius of the circle, the period of revolution, the initial position, and the sense of revolution (i.e clockwise or anti-clockwise) are indicated on each figure.

Obtain the corresponding simple harmonic motions of the x-projection of the radius vector of the revolving particle P in each case.
Answer:

Question 12.
Plot the corresponding reference circle for each of the following simple harmonic motions. Indicate the initial (t = 0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case (or is in cm and t is in s) :
(a) x = – 2 sin (3t + π/3)
(b) x = cos (π/6 – t)
(c) x = 3 sin (2nt + tc/4)
(d) x = 2 cos πt
Answer:

Question 13.
Figure (a) shows a spring of force constant k clamped rigidly at one end and a mass m attached to its free end. A Figure (a) shows a spring of force constant k clamped rigidly at one end and a mass m attached to its free end. A force F applied at the free end stretches the spring. Figure (b) shows the same spring with both ends free and attached to a mass m at either end. Each end of the spring in Fig. (b) is stretched by the same force F.

(a) What is the maximum extension of the spring in the two cases ?
(b) If the mass in Fig. (a) and the two masses in Fig. (b) are released free, what is the period of oscillation in each case ?
Answer:
(a) Let y be the maximum extension produced in the spring in fig. (a).
Then   F = Ay (in magnitude)   ∴y =  \(\frac { F }{ K } \)
In fig. (b), the force on one mass acts as the force of reaction due to the force on the other mass. Therefore, each mass behaves as if it is fixed with respect to the other.

Question 14.
The piston in the cylinder head of a locomotive has a stroke (twice the amplitude) of 1.0 m. If the piston moves with simple harmonic motion with an angular frequency of 200 rev/min,
what its maximum speed ?
Answer:

Question 15.
The acceleration due to gravity on the surface of moon is 1.7 ms^-2. What is the time period of a simple pendulum on the surface of moon if its time period on the surface of earth is 3.5 s ? (g on the surface of earth is 9.8 ms^-2)
Answer:

Question 16.
Answer the following questions :
(a) Time period of a particle in SHM depends on the force constant k and mass m of the particle :

A simple pendulum executes SHM approximately. Why then is the time period of a pendulum independent of the mass of the pendulum ?

(b) The motion of a simple pendulum is approximately simple harmonic for small angle oscillations. For larger angles of oscillation ,a more involved analysis shows that T is grater than

Think of a qualitative argument to appreciate this results.

(c) A man with a wristwatch on his hand falls from the top of a tower. Does the watch give correct time during the free fall ?

(d) What is the frequency of oscillation of a simple pendulum mounted in a cabin that is freely falling under gravity
Answer:
In case of a spring, k does not depend upon m. However, in case of a simple pendulum, k is directly proportional to

(c) The wrist watch uses an electronic system t>r spring system to give correct time, which does not change with acceleration due to gravity. Therefore, watch gives the correct time.

(d) During free fall of the cabin, the acceleration due to gravity is zero. Therefore, the frequency of oscillations is also zero e., the pendulum will not vibrate at all.

Question 17.
A simple pendulum of length l and having a bob of mass M is suspended in a car. The car is moving on a circular track of radius R with a uniform speed v. If the pendulum makes small oscillations in a radial direction about its equilibrium position, what will be its time period ?
Answer:
In this case, the bob of the pendulum is under the action of two accelerations.
(1) Acceleration due to gravity ‘g’ acting vertically downwards.

Question 18.
A cylindrical piece of cork of base area A and height h floats in a liquid of density ρ. The cork is depressed slightly and then released. Show that the cork oscillates up and down simple harmonically with a period

where ρ is the density of cork. (Ignore damping due to viscosity of the liquid).
Answer:
Let l be the height of the cork which is inside the liquid. Then, at equilibrium,
weight of the cork = upthrust of the portion of cork inside the liquid .

Question 19.
One end of a U-tube containing mercury is connected to a suction pump and the other end to atmosphere. A small pressure difference is maintained between the two columns. Show that, when the suction pump is removed, the column of mercury in the U-tube executes simple harmonic motion.
Answer:
The suction pump creates the pressure difference, thus mercury rises in one limb of the U-tube. When it is removed, a net force acts on the liquid column due to the difference in levels of mercury in the two limbs and hence the liquid column executes S.H.M. which can be expressed as:
Consider the mercury contained in a vertical U-tube upto the level P and Q in its two limbs.
Let P = density of the mercury.
L = Total length of the mercury column in both the limbs.
A = internal cross-sectional area of U-tube. m = mass of mercury in U-tube = LAP.
Assume, the mercury be depressed in left limb to F by a small distance y, then it rises by the same amount in the right limb to position Q’.
.’. Difference in levels in the two limbs = P’ Q’ = 2y.
:. Volume of mercury contained in the column of length 2y = A X 2y
.•. m – A x 2y x ρ.
If W = weight of liquid contained in the column of length 2y.
Then W = mg = A x 2y x ρ x g
This weight produces the restoring force (F) which tends to bring back the mercury to its equilibrium position.

Question 20.
An air chamber of volume V has a neck area of cross-section a into which a ball of mass m can move up and down without any friction (Fig.). Show that when the ball is pressed down a little and released, it executes SHM. Obtain an expression for the time period of oscillations assuming pressure-volume variations of air to be isothermal [see Fig.]

Answer:
When the ball is lying at rest in the long neck, the pressure of air below the ball inside the chamber is equal to the atmospheric pressure outside. If the ball is slightly depressed below through a distance y, then change in volume of air inside the chamber,

Question 21.
You are riding in an automobile of mass 3000 kg. Assuming that you are examining the oscillation characteristics of its suspension system. The suspension sags 15 cm when the entire automobile is placed on it. Also, the amplitude of oscillation decreases by 50% during one complete oscillation. Estimate the values of (a) the spring constant k and (b) the damping constant b for the spring and shock absorber system of one wheel, assuming that each wheel supports 750 kg.
Answer:
(a) Here, mass M = 3000 kg, displacement x = 15 cm = 015 m, g = 10 m/s². There are four spring systems. If k is the spring constant of each spring, then total spring constant of all the four springs in parallel is

Question 22.
Show that for a particle in linear SHM the average kinetic energy over a period of oscillation equals the average potential energy over the same period.
Answer:
Let the SHM be represented as
y = r sin ωt, where r – amplitude…..(1)


Question 23.
A circular disc of mass 10 kg is suspended by a wire attached to its center. The wire is twisted by rotating the disc and released. The period of torsional oscillations is found to be 1.5 s. The radius of the disc is 15 cm. Determine the torsional spring constant of the wire. (Torsional spring constant a is defined by the relation J = – αθ, where J is the restoring couple and 0 the angle of twist).
Answer:
Here, mass of disc, m = 10 kg,
radius r =15 cm = 0.15 m Tortional spring constant a = ?
Time period of tortional oscillations, T = 1.5 s

Question 24.
A body describes a S.H.M. with an amplitude of 5 cm and period of 0.2 s. Find the acceleration and velocity of the body, when displacement is (a) 5 cm, (b) 3 cm, (c) 0 cm.
Answer:
A = 5 cm, T = 0.2 s.

Question 25.
A mass attached to a spring is free to oscillate with angular velocity to in a horizontal plane without friction or damping. It is pulled to a distance x₀ and pushed towards the center with velocity υ₀ at time
t = 0. Determine the amplitude of the resulting oscillations in terms of the parameters oo, x₀ and υ₀ .
Answer:
According to the law of conservation of energy
Total energy at x₀ = Total energy at extreme position.

We hope the NCERT Solutions for Class 11 Physics Chapter 14 Oscillations, help you. If you have any query regarding . NCERT Solutions for Class 11 Physics Chapter 14 Oscillations, drop a comment below and we will get back to you at the earliest.

NCERT Solutions for Class 11 Physics Chapter 12 Thermodynamics

These Solutions are part of NCERT Solutions for Class 11 Physics. Here we have given NCERT Solutions for Class 11 Physics Chapter 12 Thermodynamics

Question 1.
A geyser heats water flowing at the rate of 3.0 liters per minute from 27 °C to 77 °C. If the geyser operates on a gas burner, what is the rate of consumption of the fuel if its heat of combustion is 4-0 x 10⁴ J/g ?
Answer:

Question 2.
What amount of heat must be supplied to 2.0 x 10^-2 kg of nitrogen (at room temperature) to raise its temperature by 45°C at constant pressure ? (Molecular) mass of N₂ = 28 ; R = 8.3 J mol^-1 K^-1).
Answer:
Nitrogen is a diatomic gas. Therefore its molar specific heat at constant pressure is

Question 3.
Explain why
(a) Two bodies at different temperatures T₁ and T₂ if brought in thermal contact do not necessarily settle to the mean temperature (T₁ + T₂)/2.
(b) The coolant in a chemical or a nuclear plant (i.e., the liquid used to prevent the different parts of a plant from getting too hot) should have high specific heat.
(c) Air pressure in a car type increases during driving.
(d) The climate of a harbor town is more temperate than that of a town in a desert at the same latitude.
Answer:
(a) Two bodies would settle at the mean temperature (T₁+ T₂)/2 only if they have equal thermal capacities.
(b) A coolant of high specific heat can withdraw more heat out of the chemical or nuclear plant than a coolant of ordinary specific heat.
(c) During driving, the tyres of the car are heated up due to friction between tyres and road. Since heating of tyres causes an increase in temperature, the pressure in the tyres increases (P a T at constant volume).
(d) The relative humidity in a harbour town is more than the relative humidity in a desert town. Therefore, the climatic conditions of a harbour town do not reach extremes of hot or cold making it a temperate zone.

Question 4.
A cylinder with a movable piston contains 3 moles of hydrogen at standard temperature and pressure. The walls of the cylinder are made of a heat insulator, and the piston is insulated by having a pile of sand on it. By what factor does the pressure of the gas increase if the gas is compressed to half its original volume?
Answer:
Hydrogen is a diatomic gas. Therefore, for hydrogen, ratio of two specific heats is

Question 5.
In changing the state of a gas adiabatically from an equilibrium state A to another equilibrium state B, an amount of work equal to 22.3 J is done on the system. If the gas is taken form state A to B via a process in which the net heat absorbed by the system is 9.35 cal, how much is the net work done by the system in the latter case ? [Take 1 cal = 4.19 J]
Answer:
When the state of the gas changes adiabatically from A to B, amount of work done is used to increase the internal energy of the gas.
Increase in internal energy, ΔU = 22.3 J.
In the second case, when state A changes to state B, heat absorbed by the system
ΔQ = 9.35 cal = 9.35 x 4.19J = 39 18 J
Now, using the first law of thermodynamics,
ΔQ =ΔU + ΔW
=> ΔW =ΔQ-ΔU = 39 18-22.3 = 16.88 J

Question 6.
Two cylinders A and B of equal capacity are connected to each other via a stopcock. A contains gas at standard temperature and pressure. B is completely evacuated. The entire system is thermally insulated. The stopcock is suddenly opened. Answer the following :
(a) What is the final pressure of the gas in A and B?
(b) What is the change in the internal energy of the gas?
(c) What is the change in the temperature of the gas?
Do the intermediate states of the system (before settling to the final equilibrium state) lie of its P-V-T surface ?
Answer:
(a) Since the final temperature and initial temperature remain the same (isothermal process).

(b) Since the temperature of the system remains unchanged, the change in internal energy is zero.

(c) The system being thermally insulated, there is no change in temperature (because of free expansion)

(d) The expansion is a free expansion. Therefore, the intermediate states are non-equilibrium states and the gas equation is not satisfied in these states. As a result, the gas can not return to an equilibrium state which lie on the P-V-T surface.

Question 7.
A steam engine delivers 5.4 x 10⁸ J of work per minute and services 3.6 x 10⁹ J of heat per minute from its boiler. What is the efficiency of the engine? How much heat is wasted per minute?
Answer:
Output i.e., useful work done per minute = 5.4 x 10⁸J
Input i.e., heat absorbed per minute = 3.6 X 10⁹ J

Question 8.
An electric heater supplies heat to a system at a rate of 100 W. If the system performs work at a rate of 75 joules per second, at what rate is the internal energy increasing?
Answer:
Rate of heat supplied to the system is 100 W = 100 Js^-1
Rate of work done by the system is 75Js^-1.
From the first law of thermodynamics, we have
∆ Q = ∆ U + ∆ W ……..(1)
If the above parameters are observed for a time‘∆ t’,
\(\frac{\Delta \mathrm{Q}}{\Delta \mathrm{t}}=\frac{\Delta \mathrm{U}}{\Delta \mathrm{t}}+\frac{\Delta \mathrm{W}}{\Delta \mathrm{t}}\) …….(2)
where \(\frac{\Delta Q}{\Delta t}\) is the rate of heat supplied to the system for the given time, \(\frac{\Delta U}{\Delta t}\) is the rate of increase in internal energy of the system for the given time and \(\frac{\Delta W}{\Delta t}\) is the
rate of work done by the system in the given time.
∴ From equation (2), we have
100 = \(\frac{\Delta U}{\Delta t}\) + 75
∴ \(\frac{\Delta U}{\Delta t}\) = 25 Js^-1
Therefore the internal energy increases at rate of 25 Joules per second.

Question 9.
A thermodynamic system is taken from an original state to an intermediate state by the linear process shown in Fig.

Its volume is then reduced to the original value from E to F by an isobaric process. Calculate the work done by the gas from D to E to F.
Answer:
As is clear from above Fig.,
Change in pressure, dP = EF = 5.0 – 2.0 = 3.0 N m^-2
Change in volume, dW = DF = 600 – 300 = 300 m³
Work done by the gas from D to E to F = area of ADEF

Question 10.
A refrigerator is to remove heat from the eatables kept inside at 10°C. Calculate the coefficient of performance, if room temperature is 36°C.
Answer:
Here, T₁ = 36°C = 36 + 273 = 309 K
T₂ = 10°C = 10 + 273 = 283 K

We hope the NCERT Solutions for Class 11 Physics Chapter 12 Thermodynamics, help you. If you have any query regarding NCERT Solutions for Class 11 Physics Chapter 12 Thermodynamics, drop a comment below and we will get back to you at the earliest

NCERT Solutions for Class 11 Physics Chapter 11 Thermal Properties of Matter

These Solutions are part of NCERT Solutions for Class 11 Physics. Here we have given NCERT Solutions for Class 11 Physics Chapter 11 Thermal Properties of Matter

Question 1.
The triple points of neon and carbon dioxide are 24.57 K and 216.55 K respectively. Express these temperatures on the Celsius and Fahrenheit scales.
Answer:
The relation between Kelvin scale and Celcius scale is

Question 2.
Two absolute scales A and B have triple points of water defined to be 200 A and 350 B. What is the relation between T_A and T_B?
Answer:
The triple point of water is T = 273.16 K

Question 3.
The electrical resistance in ohms of a certain thermometer varies with temperature according to the approximate law:
R = R₀ [1 + 5 x 10^-3 (T – T₀)]
The resistance is 101.6 Ω at the triple-point of water, and 165.5 Ω at the normal melting point of lead (600.5 K). What is the temperature when the resistance is 123.4 Ω ?
Answer:
At the triple point of water, T = 273.16 K and R = 101.6 Ω(given)
R = R₀ [1 + 5 X 10^-3  (T – T0)]
=>  101.6 = Ro [1 + 5 X 10^-3 (273-16-T₀)]  …(1)
At temperature T = 600.5 K, R = 165.5 Q
165.5 = Ro [1 + 5 X 10^-3 (600-5 – T0)]……………(2)


Answer the following:
(a) The triple-point of water is a standard fixed point in modern thermometry. Why? What is wrong with taking the melting point of ice and the boiling point of water as standard fixed points (as was originally done in the Celsius scale)?
(b) There were two fixed points in the original Celsius scale as mentioned above which were assigned the number 0 °C and 100 °C respectively. On the absolute scale, one of the fixed points is the triple-point of water, which on the kelvin absolute scale is assigned the number 273.16 K. What is the other fixed point on this (Kelvin) scale?
(c) The absolute temperature (Kelvin scale) T is related to the temperature t_c on the Celsius scale by
t_c = T – 273.15
(d) Why do we have 273.15 in this relation, and not 273.16?
What is the temperature of the triple-point of water on an absolute scale whose unit interval size is equal to that of the Fahrenheit scale?
Answer:
(a) Triple point of water has a unique value e. 273.16 K. The melting point and boiling points of ice, and water respectively do not have unique values and change with the change in pressure.
(b) The other fixed point is absolute zero of temperature.
(c) On the Celcius scale 0 °C corresponds to the melting point of ice at normal pressure and the value of absolute temperature is 273.15 K. The temperature 273.16 K corresponds to the triple point of water.
(d) The Fahrenheit scale and Absolute scale are related as

Question 5.
Two ideal gas thermometers A and B use oxygen and hydrogen respectively. The following observations are made :

Temperature	Pressure thermometer A	Pressure thermometer B
Triple-point of water Normal melting point of sulphur	1.250 x 105 Pa 1.797 x 105 Pa	0.200 x 105 Pa 0.287 x 105 Pa

(a) What is the absolute temperature of the normal melting point of sulphur as read by thermometers A and B?
(b) What do you think is the reason for the slightly different answers from A and B? (The thermometers are not faulty). What further procedure is needed in the experiment to reduce the discrepancy between the two readings?
Answer:

(b) The discrepancy between the two readings is due to the fact that the gases are not perfectly ideal gases. To reduce the discrepancy, the readings should be taken at low pressures so that the gases could show prefect behaviour.

Question 6.
A steel tape 1 m long is correctly calibrated for a temperature of 27.0 °C. The length of a steel rod measured by this tape is found to be 63.0 cm on a hot day when the temperature is 45.0 °C. What is the actual length of the steel rod on that day? What is the length of the same steel rod on a day when the temperature is 27.0°C? Coefficient of linear expansion of steel = 1.20 x 10^-5 °C^_1.
Answer:
The length of the steel tape at 27 °C is 100 cm
∴  L = 100 m and T = 27°

Length of the same steel rod shall again be 63 cm when measured by the same steel tape at 27°.

Question 7.
A large steel wheel is to be fitted onto a shaft of the same material. At 27°C, the outer diameter of the shaft is 8.70 cm and the diameter of the central holes in the wheel is 8.69 cm. The shaft is cooled using ‘dry ice’. At what temperature of the shaft does the wheel slip on the shaft? Assume the coefficient of linear expansion of the steel to be constant over the required temperature range.
Answer:

Question 8.
A hole is drilled in copper steel. The diameter of the hole is 4.24 cm at 27.0 °C. What is the change in the diameter of the hole when the steel is heated to 227 °C? Coefficient of linear expansion of copper = 1.70 x 10^-5 °C¹
Answer:
Given α = 1.70 × 10^-5K^-1
β = 2α = 3.40 × 10^-5K^-1
d₁ = 4.24 cm
Initial area A₁ at 27°C = 300K = T₁
is \(\pi\left(\frac{\mathrm{d}_{1}}{2}\right)^{2}=\pi\left(\frac{4.24}{2}\right)^{2}\)
⇒ A₁ = 4.4944 π cm²
Area A₂ at 227°C = 500K = T₂ is
∆₁ [1 + β(T₂ – T₁)]
i.e. A₂ = 4.4944 π [1 + 3.40 × 10^-5 (500 – 300)]
A₂ = 4.5250 π cm²
⇒ \(\pi\left(\frac{\mathrm{d}_{2}}{2}\right)^{2}\) = 4.5250 π cm²
⇒ d₂ = \(\sqrt{18.100}\) =4.2544 cm
∴ Change in diameter = d₂ – d₁
= 4.2544 – 4.25 = 0.0144 cm

Question 9.
A brass wire 1.8 m long at 27°C is held taut with little tension between two rigid supports. If the wire is cooled to a temperature of – 39 °C, what is the tension developed in the wire, if its diameter is 2.0 mm? Co-efficient of linear expansion of brass = 2.0 x 10^-5 °C’ ; Young’s modulus of brass = 0.91 x 10¹¹ Pa.
Answer:

Question 10.
A brass rod of length 50 cm and diameter 3.0 mm is joined to a steel rod of the same length and diameter. What is the change in length of the combined rod at 250 °C, if the original lengths are at 40*0 °C? Is there a ‘thermal stress’ developed at the junction? The ends of the rod are free to expand (Co-efficient of linear expansion of brass = 2.0 x 10^-5 °C¹, steel = 1.2 x 10⁵ °C¹.
Answer:

Question 11.
The coefficient of volume expansion of glycerin is 49 x 10^-5 °C^-1. What is the fractional change in its density for a 30 °C rise in temperature?
Answer:

Question 12.
A 10 kW drilling machine is used to drill a bore in a small aluminium block of mass 8.0 kg. How much is the rise in temperature of the block in 2.5 minutes, assuming 50% of power is used up in beating the machine itself or lost to the surroundings? Specific heat of aluminium = 0.91Jg^-1°C^-1.
Answer:

Question 13.
A copper block of mass 2.5 kg is heated in a furnace to a temperature of 500°C and then placed on a large ice block. What is the maximum amount of ice that cari melt?
(Specific heat of copper = 0.39J g^-1 °C^-1, and heat of fusion of water = 335 J g^-1).
Answer:
Let m be the mass of ice melted when hot copper block is placed over an ice block.
Heat lost of copper block = Heat gained by ice

Question 14.
In an experiment on the specific heat of a metal, a 0.20 kg block of metal at 150°C is dropped in a copper calorimeter (of water equivalent 0.025 kg) containing 150 cm³ of water at 27°C. The final temperature is 40°C. Compute the specific heat of the metal.
Answer:

Question 15.
Given below are observations on molar-specific heats at room temperature of some common gases.

Gas	Molar specific heat (Cv) (cal mol-1 K-1)
Hydrogen	4.87
Nitrogen	4.97
Oxygen	5.02
Nitric oxide	4.99
Carbon monoxide	5.01
Chlorine	6.17

The measured molar specific heats of these gases are markedly different from those for monoatomic gases. [Typically, the molar specific heat of a monoatomic gas is 2.92 cal/mol K, as you must have worked out in 16.] Explain this difference. What can you infer from the somewhat larger (than the rest) value for chlorine?
Answer:
All the gases mentioned above are diatomic, and hence have other degrees of freedom in addition to the translational degrees of freedom. To raise the temperature of the gas, heat has to be supplied to increase the average energy of all the modes.

Consequently, the molar specific heats for diatomic gases is higher than that for monoatomic gases. The higher value of the molar specific heat of chlorine indicates that at room temperature, vibrational modes are also present In addition to the rotational modes of freedom.

Question 16.
Answer the following questions based on the P – T phase diagram of carbon dioxide (Figure shown below) :

(a) At what temperature and pressure can the solid, liquid and vapour phase of CO2 co-exist in equilibrium ?
(b) What is the effect of decrease of pressure on the fusion and boiling point of CO₂ ?
(c) What are the critical temperature and pressure for CO₂ ? What is their significance ?
(d) Is CO₂ solid, liquid or gas at

(a) – 70 °C under 1 atm,
(b) – 60 °C under 10 atm
(c) 15 °C under 56 atm ?

Answer:
(a) Liquid and vapour phases coexist with solid phase at the triple point temperature – 56.6 °C and a pressure of 5.11 atm.
(b) The boiling point as well as freezing point decrease with the decrease in pressure.
(c) The critical temperature is 3T1 °C and critical pressure is’73.0 atm.
(d) (a) It is vapour (b) It is solid and (c) It is liquid.

Question 17.
Answer the following questions based on the P – T phase diagram of CO₂ (Fig. of question 17).
(a) CO₂ at 1 atm pressure and temperature – 60 °C is compressed isothermally. Does it go through a liquid phase?
(b) What happens when CO₂ at 4 atm pressure is cooled from room temperature at constant pressure?
(c) Describe qualitatively the changes in a given mass of solid CO₂ at 10 atm pressure and temperature – 65 °C as it is heated up at room temperature at constant pressure.
(d) CO₂ is heated to a temperature of 70° C and compressed isothermally. What changes in its properties do you expect to observe?
Answer:

1. When subjected to isothermal compression, Co₂ will condense to solid directly without passing through the liquid phase.
2. When cooled at constant pressure, Co₂ will condense directly to solid phase without passing through the liquid phase,
3. As the solid Co₂ is heated up, it first turns to liquid phase and then solid phase. The fusion and boiling points can be obtained from finding out the points of intersection between the horizontal 10 atm line on the P-T diagram with the fusion and vaporization curves.
4. Co₂ will not exhibit any clear transition to the liquid phase but will deviate more and more from ideal behaviour ps the pressure increases.

Question 18.
A child running a temperature of 101 °F is given an antipyrin (i. e. a medicine that lowers fever) which causes an increase in the rate of evaporation of sweat from his body. If the fever is brought down to 98 °F in 20 min, what is the average Irate of extra evaporation caused by the drug? Assume the evaporation mechanism to be the only way by which heat is lost. The mass of the child is 30 kg. The specific heat of the human body is approximately the same as that of water, and the latent heat of evaporation of water at that temperature is about 580 cal g^_1.
Answer:

Question 19.
A cubical icebox of thermocouples has each side = 30 cm and a thickness of 5 cm. 4 kg of ice is put in the box. If the outside temp is 45° C and coeff. of thermal conductivity = 0.01 Js^-1 mf¹ °C^-1, calculate the mass of ice left after 6 hours. Take latent heat of fusion of ice = 335 x 10³ J/kg.
Answer:


Question 20.
A brass boiler has a base area of 0.15 m² and a thickness of 1.0 cm. It boils water at the rate of 6.0 kg/min when placed on a gas stove. Estimate the temperature of the part of the flame in contact with the boiler. Thermal conductivity of brass = 609 Js^-1 m^-1 °C^-1. Heat of vaporisation of water = 2256 Jg^-1.
Answer:

Question 21.
Explain why :
(a) a body with large reflectivity is a poor emitter
(b) a brass tumbler feels much colder than a wooden tray on a chilly day
(c) an optical pyrometer (for measuring high temperatures) calibrated for ideal black body radiation gives too low a value for the temperature of a read hot iron piece in the open, but gives a correct value for the temperature when the same piece is in the furnace
(d) the earth without its atmosphere would be inhospitably cold
(e) Heat systems based on the circulation of steam are more efficient in warming a building than those based on circu|ation of hot water.
Answer:
(a) According to Kirchhoff s law of black body radiations, good emitters are good absorbers and bad emitters are bad absorbers. A body with large reflectivity is a poor absorber of heat and consequently, it is also a poor emitter.

(b) Brass is a good conductor of heat, while wood is a bad conductor. When we touch the brass tumbler on a chilly day, heat starts flowing from our body to the tumbler and we feel it cold. However, when the wooden tray is touched, heat does mot flow from our hands to the tray and we do not feel cold.

(c) An optical pyrometer is based on the principle that the brightness of a glowing surface of a body depends upon its temperature. Therefore, if the temperature of the body is less than 600°C, the image formed by the optical pyrometer is not brilliant and we do not get a reliable result. It is for this reason that the pyrometer gives a too low value for the temperature of red hot iron in the open.

(d) The atmosphere of earth acts as an insulator to stop the infrared radiations which fall on the surface of earth during day time, to escape during the night. If there were no atmosphere, the whole of heat radiated by the earth during night would escape to the universe leaving it under the intense cold.

(e) Steam at a temperature of 100°C has more heat (because its latent heat is 540 cal g^-1) compared to the same amount of water at the same temperature. Therefore, heating systems based on the circulation of steam are more efficient in warming a building than those based on the circulation of hot water.

Question 22.
A body cools from 80°C to 50°C in 5 minutes. Calculate the time it takes to cool from 60°C to 30°C. The temperature of the surroundings is 20°C.
Answer:
If θ₀ is the temperature of surrounding, time taken t to cool a body from temperature θ₁, to θ₂ is given by

We hope the NCERT Solutions for Class 11 Physics Chapter 11 Thermal Properties of Matter, help you. If you have any query regarding NCERT Solutions for Class 11 Physics Chapter 11 Thermal Properties of Matter, drop a comment below and we will get back to you at the earliest

NCERT Solutions for Class 11 Physics Chapter 10 Mechanical Properties of Fluids

These Solutions are part of NCERT Solutions for Class 11 Physics. Here we have given NCERT Solutions for Class 11 Physics Chapter 10 Mechanical Properties of Fluids

Question 1.
Explain why
1. The blood pressure In humans is greater at the feet than at the brain.
Answer:
The height of the blood column in the human body is more at the feet than at the brain. As the pressure increases with the height of the column, blood exerts  more pressure at the feet than at the brain (P = ρgh)

2. Atmospheric pressure at a height of about 6 km decreases to nearly half of its value at the sea level, though the height of the atmosphere is more than 100 km.
Answer:
The density of air is maximum near the surface of the earth (at sea level) and it decreases rapidly with height. The density of air at a height of 6 km reduces to almost half it’s value at sea level. Hence the atmospheric pressure at a height of 6 km decreases to nearly half of its value at sea level even though the height of the atmosphere is more than 100km.

3. Hydrostatic pressure is a scalar quantity even though pressure is force divided by area.
Answer:
The pressure created while applying a force on a liquid is transmitted equally in all direction inside the liquid. That is why there is no fixed direction for the pressure transmitted. Hence we can say that hydrostatic pressure is a scalar quantity.

Question 2.
Explain why
(a) The angle of contact of mercury with glass is obtuse, while that of water with glass is acute.
(b) Water on a clean glass surface tends to spread out while mercury on the same surface tends to form drops. (Put differently, water wets the glass while mercury does not.)
(c) Surface tension of a liquid is independent of the area of the surface.
(d) Detergents should have small angles of contact.
(e) A drop of liquid under no external forces is always spherical in shape.
Answer:
(a) The cohesive force between mercury molecules is greater than the adhesive force between mercury and glass molecules. Therefore, the meniscus of mercury in a glass tube is convex and hence the angle of contact is obtuse. In the case of water, the cohesive force between water molecules is less than the adhesive force between water and glass molecules. Therefore, the meniscus of water in the glass tube is concave and hence the angle of contact is acute.
(b) The cohesive force between water molecules is less than the adhesive force between glass and water molecules. On the other hand, the cohesive force between mercury molecules is greater than the adhesive force between glass and mercury molecules.
(c) Surface tension is defined as the force per unit length of an imaginary line drawn tangentially on the surface of the liquid at rest. Therefore, surface tension is also independent of the area of the liquid surface.
(d) So that the detergent has low surface tension and greater ability to wet a surface.
(e) In the absence of external force, liquid drop takes a spherical shape due to surface tension.

Question 3.
Fill in the blanks using the words from the list appended with each statement:
(a) Surface tension of liquids generally……………. with temperature (increases/decreases)
(b) Viscosity of gases……………… with temperature, whereas viscosity of liquids………………. with temperature (increases/decreases)
(c) For solids with the elastic modulus of rigidity, the shearing force is proportional to………………….. while for fluids it is proportional to…………. (shear strain/rate of shear strain)
(d) For a fluid in steady flow, the increase in flow speed at a constriction follows from…………….. while the decrease of pressure there follows from………….. (conservation of mass/Bernoulli’s principle)
(e) For the model of a plane in a wind tunnel, turbulence occurs at a…………………… speed than the critical speed for turbulence for an actual plane (greater/smaller)
Answer:
(a) decreases
(b) increases ; decreases
(c) shear strain ; rate of shear strain
(d) conservation of mass ; Bernoulli’s principle

Question 4.
Explain why
(a) To keep a piece of paper horizontal, you should blow over, not under, it.
(b) When we try to close a water tap with our fingers, fast jets of water gush through the openings between our fingers.
(c) The size of a needle of a syringe controls flow rate better than the thumb pressure exerted by a doctor while administering an injection.
(d) A fluid flowing out of a small hole in a vessel results in a backward thurst on the vessel.
(e) a spinning cricket ball in air does not follow a parabolic trajectory.
Answer:
(a) When we blow over the piece of paper, the velocity of air increases. As a result, the pressure over it decreases in accordance with the Bernoulli’s theorem whereas the pressure below it is higher (atmospheric pressure) which keeps the paper horizontal.

(b) This is due to the continuity equation of flow of the liquids. When we close the water tap, the area of cross-section decreases and according to the equation of continuity (aυ= constant), the velocity of flow of the liquid increases.

(c) For a> constant height, the Bernoulli’s theorem is expressed as
p + \(\frac { 1}{ 2 } \) pυ² = constant
In this equation, the pressure P occur with a single power whereas the velocity occurs with a square power. Therefore, the velocity has more effect compared to the pressure. It is for this reason that needle of the syringe controls flow rate better than the thumb pressure exerted by the doctor.

(d) When a fluid flows out of a small hole, it carries momentum with it. According to the law of conservation of momentum, the vessel gets a recoil momentum. Thus, there is a change in momentum of the vessel which means that a backward thurst acts on it.

(e) When ball is given spin at the top, the velocity of air above the ball is higher than below the ball. According to Bernoulli’s theorem, die pressure above the ball is less than the pressure below the ball. Thus, there is net upward force on the spinning ball and hence the ball follows a curved path. This effect is known as the Magnus effect.

Question 5.
A 50 kg girl wearing high heel shoes balances on a single heel. The heel is circular with a diameter 1.0 cm. What is the pressure exerted by the heel on the horizontal floor?
Answer:
Mass of girl, m= 50 kg.
Force on the heel, F = mg = 50 x 9.8 = 490 N
Diameter, D = 1.0 cm = 1 x 10^-2 m

Question 6.
Toricelli’s barometer used mercury. Pascal duplicated it using French wine of density 984 kg m^-3. Determine the height of the wine column for normal atmospheric pressure.
Answer:
At normal pressure of atmosphere,


Question 7.
A vertical off-shore structure is built to withstand maximum stress of 10⁹ Pa. Is the structure suitable for putting up on top of an oil well in the ocean? Take the depth of the ocean to be roughly 3 km, and ignore ocean currents.
Answer:
Maximum stress that can be taken by the off-share structure, P_max = 10⁹Pa.
density of water = 10³ kg /m³ and g = 9.8 m/s² and h = 3km (depth of the sea)
∴ Pressure exerted by seawater, P = ρgh
= 10³ × 9.8 × 3 × 10³
P = 2.94 × 10⁷ Pa
Note that, P < P_max
Since the pressure exerted by the seawater is less than the maximum pressure the structure can withstand, the structure is suitable for putting on the top of the oil well.

Question 8.
A hydraulic automobile lift is designed to lift cars with a maximum mass of 3000 kg. The area of cross-section of the piston carrying the load is 425 cm². What maximum pressure would the smaller piston have to bear?
Answer:
Maximum mass, m= 3000 kg ; Area, A = 425 cm² = 425 x 10^-4 m².

Question 9.
A U-tube contains water and methylated spirit separated by mercury. The mercury columns in the two arms in level with 10.0 cm of water in one arm and 12.5 cm of spirit in the other. What is the specific gravity of spirit?
Answer:

Since the mercury column in the two arms is in level, therefore,
Pressure due to spirit = Pressure due to water

Question 10.
In the previous problem, if 15.0 cm of water and spirit each are further poured into the respective arms of the tube, what is the difference in the levels of mercury in the two arms? (Specific gravity of mercury = 13.6)
Answer:
If p_m be the density of mercury, then

Question 11.
Can Bernoulli’s equation be used to describe the flow of water through a rapid motion in a river? Explain.
Answer:
No. The flow of water through a rapid in a river is turbulent and hence Bernoulli’s principle cannot be applied.

Question 12.
Does it matter if one uses gauge instead of absolute pressures in applying Bernoulli’s equation? Explain.
Answer:
If the pressures at the two places where Bernoulli’s theorem is applied are not much different, it does not matter if one uses gauge pressure instead of absolute pressure.

Question 13.
Glycerine flows steadily through a horizontal tube of length 1.5 m and radius 1.0 cm. If the amount of glycerine collected per second at one end is 4.0 x 10^-3 kg s^-1, what is the pressure difference between the two ends of the tube? Density of glycerine = 1.3 X 10³ kg m^-3 and viscosity of
glycerine = 0.83 N s m^-2 to
Answer:
Here, l= 1.5 m, r =1.0 cm = 10^-2 q = 0.83 N s m^-2

Question 14.
In a test experiment on a model airplane in a wind tunnel, the flow speeds on the upper and lower surfaces of the wing are 70 m s^-1 and 63 m s^-1 respectively. What is the lift on the wing if its area is 2.5 m² ? Take the density of air to be 1.3 kg m^-3.
Answer:
Here υ₁ 70 ms^-1, υ₂ = 63 ms ‘, p = 1.3 kg m ³ and A = 2.5 m².
Now, using Bernoulli’s theorem (for constant height),

Question 15.
Figures (a) and (b) refer to the steady flow of a (non-viscous) liquid. Which of the two figures is incorrect? Why?

Answer:
Figure (a) is incorrect. It is because of the fact that at the kink, the velocity of flow of liquid is large and hence using the Bernoulli’s theorem the pressure is less. As a result, the water should not rise higher in the tube where there is a kink (i. e. where the area of cross-section is small).

Question 16.
The cylindrical tube of a spray pump has a cross-section of 8.0 cm² one end of which has 40 fine holes each of diameter 1.0 mm. If the liquid flow inside the tube is 1.5 m min^-1, what is the speed of ejection of the liquid through the holes ?
Answer:
Here, area of cylindrical tube, a_x = 8.0 cm² = 8 x 10^-4 m²

Question 17.
A U-shaped wire is dipped in a soap solution and removed. The thin soap film formed between the wire and the light slider supports a weight of 1.5 x 10^-2 N (which includes the small weight of the silder). The length of the silder is 30 cm. What is the surface tension of the film ?
Answer:
Force on the silder, F= 1.5 x 10^-2 N

Question 18.
(a) shows a thin liquid film supporting a small weight = 4.5 x 10^-2 N. What is the weight supported by a film of the same liquid at the same temperature in Fig. (b) and (c) ? Explain your answer physically.

Answer:
As shown in fig(a)

Question 19.
What is the pressure inside the drop of mercury of radius 3.00 mm at room temperature ? Surface tension of mercury at that temperature (20 °C) is 4.65 x 10^-1 N m^_1. The atmospheric pressure is 1.01 x 10⁵ Pa. Also give the excess pressure inside the drop.
Answer:
Here, surface tension, T = 4.65 x 10^-1 Nm^-1
Radius of drop, r =3.00 mm = 3 x 10^-3 m.
Outside pressure, p₀ = 1.01 x 10⁵ Pa.
Using the relation,

Question 20.
What is the excess pressure inside a bubble of soap solution of radius 5-00 mm, given that the surface tension of soap solution at the temperature (20 °C) is 2.50 x 10^-2 N m^-1 ? If an air bubble of the same dimension were formed at depth of 40.0 cm inside a container containing the soap solution (of relative density 1.20), what would be the pressure inside the bubble? (1 atmospheric pressure is 1.01 x 10^s Pa).
Answer:
Here, surface tension, T = 2.50 x 10^-2 N m^-1
Radius, r= 5.00 mm = 5 x 10^-3 m.

Question 21.
A tank with a square base of area 1.0 m² is divided by a vertical partition in the middle. The bottom of the partition has a small-hinged door of area 20 cm². The tank is filled with water in one compartment, and an acid (or relative density 1.7) in the other, both to a height of 4.0 m. compute the force necessary to keep the door close.
Answer:

Question 22.
A manometer reads the pressure of a gas in an enclosure as shown in Fig. (a). When a pump removes some of the gas, the manometer reads as in Fig. (b). The liquid used in the manometers is mercury and the atmospheric pressure is 76 cm of mercury.
(a) Give the absolute and gauge pressure of the gas in the enclosure for cases (a) and (b), in units of cm of mercury.
(b) How would the levels change in case (b) if 13.6 cm of water (immiscible with mercury) are poured into the right limb of the manometer? (Ignore the small change in the volume of the gas).

Answer:
The atmospheric pressure, P = 76 cm of mercury
(a) From figure (a),
Pressure head, h = 20 cm of mercury
∴ Absolute pressure = P + h = 76 + 20 = 96 cm of mercury
Also, Gauge pressure = h =20 cm of mercury
From figure (b),
Pressure head,h = -18 cm of mercury
∴ Absolute pressure = P + h = 76 + (- 18) = 58 cm of mercury
Also, (Gauge pressure = h = – 18 cm of mercury
(b) When 13.6 cm of water is poured into the right limb of the manometer of figure (b), then, using the relation : pressure, p = pgh = p’g’h’,

Therefore, pressure at the point B,
P_B = P + h’ = 76 + 1 = 77 cm of mercury
If h is the difference in the mercury levels in the two limbs, then taking P_A = P_B
=>  58 + h” = 11 => h” = 77 – 58 = 19 cm of mercury.

Question 23.
Two vessels have the same area but different shapes. The first vessel takes twice the volume of water that the second vessel requires to fill upto a particular common height. Is the force exerted by the water on the base of the vessel the same in the two cases? If so, why do the vessels filled with water to that same height give different readings on a weighing scale?
Answer:

Question 24.
During blood transfusion, the needle is inserted in a vein where the gauge pressure is 2000 Pa. At what height must the blood container be placed so that blood may just enter the vein ? [Use the density of whole blood as 1.06 x 10³ kg m^-3 ]
Answer:

Question 25.
In deriving Bernoulli’s equation, we equated the work done on the fluid in the tube to its change in the potential and kinetic energy,
(a) How does the pressure change as the fluid moves along the tube if dissipative forces are present?
(b) Do the dissipative forces become more important as the fluid velocity increases? Discuss qualitatively.
Answer:
(a) If the dissipative forces are present, some of the pressure energy of the liquid is used in doing some work against these forces. Hence, the fluid pressure decreases.
(b) With the increase in velocity, the rate of loss of energy also increases. As a result, the dissipative forces become more significant.

Question 26.
(a) What is the largest average velocity of blood flow in an artery of radius 2 x 10^-3 m if the flow must remain steady? (b) What is the corresponding flow rate?
(Take viscosity of blood to be 2.084 x 10^-3 Pa s). Take the density of blood as 1.06 kg m^-3.
Answer:
Here, r = 2 x 10^-3 m, η = 2.084 x 10^-3 Pa s
Taking the maximum value of Reynold number for streamlined flow as N_R = 2000, the maximum velocity is

Question 27.
A plane is in level flight at constant speed and each of its two wings has an area of 25 m². If the speed of the air is 180 km/h over the lower wing and 234 km/h over the upper wing surface, determine the plane’s mass. (Take air density to be 1 kg m^-3).
Answer:

Question 28.
In Millikan’s oil drop experiment, what is the terminal speed of an uncharged drop of radius 2.0 x 10^-5 m and density 1.2 x 10³ kg m^-3. Take the viscosity of air at the temperature of the experiment to be
1.8 x 10^-5 How much is the viscous force on the drop at that speed ? Neglect buoyancy of the drop due to air.
Answer:

Question 29.
Mercury has an angle of contact equal to 140° with soda lime glass. A narrow tube of radius 1.00 mm made of this glass is dipped in a trough containing mercury. By what amount does the mercury dip down in the tube relative to the liquid surface outside ? Surface tension of mercury at the temperature of the experiment is 0.465 N m^_1. Density of mercury = 13.6 x 10³ kg m³.
Answer:
Here, angle of contact, θ = 140°                 .’. cos θ= cos 140° = – 0.7660
Also,  r = 1.00 mm = 1 x 10^-3 m, surface tension, T = 0.465 N nr¹
density ρ= 13.6 x 10³ kg m^-3

Question 30.
Two narrow bores of diameters 3.0 mm and 6.0 mm are joined together to form a U-tube open at both ends. If the U-tube contains water, what is the difference in its levels in the two limbs of the tube ? Surface tension of water at the temperature of the experiment is 7.3 x 10^-2 N m^-1. Take the angle of contact to be zero and density of water to be 1.0 x 10³ kg m^-3 (g = 9.8 ms’²)
Answer:
Let r_x be the radius of one bore and r₂ be the radius of second bore of the U-tube. Then, if h_x and h₂ are the heights of water on two sides,

Question 31.
(a) It is known that density p of air decreases with height y (in metres) as

where ρo = 1.25 kg m^-3 is the density at sea level, and y₀ is a constant. This density variation is called the law of atmospheres. Obtain this law assuming that the temperature of the atmosphere remains constant (isothermal conditions). Also, assume that the value of g remains constant.
(b) A large He balloon of volume 1425 m³ is used to lift a payload of 400 kg. Assume that the balloon maintains a constant radius as it rises. How high does it rise?
[Take yo = 8000 m and p_He = 0.18 kg m^-3]
Answer:
(a) Consider an imaginary cylinder of air having an area ‘a’ of a cross-section of each end face and placed vertically above the surface of the earth. Let the cap I of the cylinder be at a height of y and the cap II be at the height y + dy. Let P and P + dP be the pressures at caps I and II respectively. Then, the net force acting on the cap due to gravity is the weight of the cylinder i. e.,
W = mass of cylinder xg = ady.pg    …(1)
Since the cylinder is considered to be placed in the air, therefore, the force due to the difference in pressure is balanced by the weight of the cylinder under the equilibrium conditions.

We hope the NCERT Solutions for Class 11 Physics Chapter 10 Mechanical Properties of Fluids, help you. If you have any query regarding NCERT Solutions for Class 11 Physics Chapter 10 Mechanical Properties of Fluids, drop a comment below and we will get back to you at the earliest

 

NCERT Solutions for Class 11 Physics Chapter 9 Mechanical Properties of Solids

These Solutions are part of NCERT Solutions for Class 11 Physics. Here we have given NCERT Solutions for Class 11 Physics Chapter 9 Mechanical Properties of Solids

Question 1.
A steel wire of length 4.7 m and cross-section 3.0 x 10^-5 m² stretches by the same amount as a copper wire of length 3.5 m and cross-section 4.0 x 10^-5 m² under a given load. What is the ratio of Young’s modulus of steel to that of copper?
Answer:
For steel, length of wire, L_s = 4.7 m
Area of cross-section, A_s = 3 x 10^-5 m²
For copper, length of wire, L_c = 3.5 m
Area of cross-section, A_c = 4.0 x 10^-5 m²
Also, extension
l_s = l_c = l

Question 2.
Fig. shows the stress-strain curve for a given material. What are
(a) Young’s modulus and
(b) approximate yield strength for this material?

Answer:
(a) From graph, for stress = 150 x 10⁶ Nm^-2
the corresponding strain = 0.002

Question 3.
The stress-strain graphs for materials A and B are shown in Figure.

The graphs are drawn to the same scale.
(a) Which of the material has greater Young’s modulus?
(b) Which material is more ductile?
(c) Which is more brittle?
(d) Which of the two is stronger material?
Answer:
(a) material A has a greater Young’s modulus because the slope of the graph of material A is more.
(b) Material A is more ductile than material B because it has a large plastic deformation between the elastic limit and the breaking point.
(c) Material B is more brittle than material A because it has a small plastic deformation between the elastic limit and the breaking point.
(d) Material A is stronger than B because more stress is required to break it.

Question 4.
Read each of the statements below carefully and state, with reasons, if it is true or false.
(a) The modulus of elasticity of rubber is greater than that of steel.
(b) The stretching of a coil is determined by its shear modulus.
Answer:
(a) False. Young’s modulus for steel is more than rubber.
(b) True

Question 5.
Calculate the elongation of the steel and brass wire in the Fig. Unloaded length of steel wire is 1.5 m and of brass wire = 1.0.m, the diameter of each wire = 0.25 cm. Young’s modulus of steel is 2.0 x 10¹¹ Pa and that of brass is 0.91 x 10¹¹ Pa.

Answer:

Question 6.
The edges of an aluminium cube are 10 cm long. One face of the cube is firmly fixed to a vertical wall. A mass of 100 kg is then attached to the opposite face of the cube. The shear modulus of aluminium is 25 GPa. What is the vertical deflection of this face? (1 Pa = 1 Nm^-2)
Answer:

Question 7.
Four identical hollow cylindrical columns of steel support a big structure of mass 50,000 kg. The inner and outer radii of each column are 30 and 60 cm respectively. Assuming the load distribution to be uniform, calculate the compressional strain of each column. The Young’s modulus of steel is 2.0 x 10¹¹ Pa (1 Pa = 1 N m^-2).
Answer:
Here, outer radius of each column, r₀ = 60 cm = 60 x 10“² m
Inner radius, r,= 30 cm = 30 x 10^-2 m

Question 8.
A piece of copper having a rectangular cross-section of 15.2 mm x 19.1 mm is pulled in tension with 44,500 N force-producing only elastic deformation. Calculate the resulting strain. The shear modulus of elasticity of copper is 42 x 10⁹ Nm-².
Answer:
Here, A = 15.2 x 19.1 x 10-⁶m² . F = 44,500 N ; G = 42 X 10⁹ Nm-².

Question 9.
A steel cable with a radius of 1.5 cm supports a chairlift at a ski area. If the maximum stress is not to exceed 10⁸ Nm^-2, what is the maximum load the cable cab support?
Answer:
r = radius of cable = 1.5 cm.
A = π(0.015)² m²
max stress = 10⁸ Nm^-2
Let F be the maximum force.
stress = \(\frac{F}{A}\)
∴ F = max stress × area.
= 10⁸ × π × (0.015)²
= 7.065 × 10⁴N
= 70,650 N
∴ The maximum load is 70,650 N

Question 10.
A rigid bar of mass 15 kg is supported symmetrically by three wires each 2 m long. These at each end are of copper and middle one is of iron. Determine the ratio of their diameters if each is to have the same tension. Young’s modulus of elasticity for copper and steel are 120 x 10⁹ Nm^-2 and 190 x 10⁹ Nm^-2 respectively.
Answer:
As each wire has same tension F, so each wire has same extension due to mass of rigid bar. As each wire is of same length, hence each wire has same strain. If D is the diameter of wire, then


Question 11.
A 14.5 kg mass, fastened to the end of a steel wire of unstretched length 1 m, is Whirled in a vertical circle with an angular velocity of 2 rad/s at the bottom of the circle. The cross-sectional area of the wire is 0.065 cm². Calculate the elongation of the wire when the mass is at the lowest point of its path.
Y_steel = 2 x 10¹¹ Nm^-2.
Answer:
Here, m = 14.5 kg ;l = r = lm;v = 2rps;A = 0065 x 10^-4 m²
Total pulling force on mass, when it is at the lowest position of the vertical circle is
F = mg + mr ω²
= 14 .5 x 9.8 + 14.5 x 1 x 4
= 200.1 N

Question 12.
Compute the bulk modulus of water from the following data : Initial volume = 100.0 litre, Pressure Increase =100.0 atm (1 atm = 1.013 x 10⁵Pa), Final volume = 100.5 liter. Compare the bulk modulus of water with that of air (at constant temperature). Explain in simple terms why the ratio is so large.
(1 Pa = 1 Nm^-2 ).
Answer:
Given, V = 100 litre; Vf = 100.5 litre
:. Change in volume, ΔV = V_f – V_i= 100.5 – 100 = 0.5 liter

Question 13.
What is the density of water at a depth where pressure is 80.0 atm, given that its density at the surface is 1.03 x 10³ kg m^-3? (Compressibility of water is 45.8 x 10^-11 Pa^-1 ; 1 Pa = 1 N m^-2)
Answer:
The increase in density is given by dρ = CPρ
where C is compressibility, P is pressure and p is the density at the surface.
Here, C = 45.8 x 10^-11 Pa^-1, P = 80 x 1 x 10⁵ Nm^-2, p = 1.03 x 10³kg m³
∴   dρ= 45.8 x 10^-11 x 80 x 10⁵ x 1.03 x 10³ = 3.774 kg m³
∴Total density at the given depth = ρ+ dp = 1.03 x 10³ + 3.774
= 1.03 x 1000 + 3.774 = 1033.774 kg m^-3 ≈1 .034 X 10³ kg m^-3

Question 14.
Compute the fractional change in volume of a glass slab, when subjected to a hydraulic pressure of 10 atmospheres. Bulk modulus of elasticity of glass = 37 x 10⁹ Nm^-2 and 1 atm = 1.103 x 10^s Pa
Answer:
Here ρ= 10 atm = 10 x 1.013 x 10⁵ Pa ; K = 37 x 10⁹ Nm^-2

Question 15.
Determine the volume contraction of a solid copper cube, 10 cm on an edge when subjected to a hydraulic pressure of 7 x 10⁶ Pa. Bulk modulus of copper = 140 G Pal
Answer:
Here,  L = 10 cm = 0.10 m ; p = 7 x 10⁶ Pa ;
K = 140 G Pa = 140 x 10⁹ Pa

Question 16.
How much should be pressure on a liter of water be changed to compress it by 0.10% ? Bulk modulus of elasticity of water = 2.2 x 10⁹ Nm^-2.
Answer:
V = 1 liter = 10^-3 m³ ; ΔV/V = 0.10/100 = 10^-3

Question 17.
Anvils made of single crystals of diamond, with the shape as shown in Fig. are used to investigate behavior of materials under very high pressures. Flat faces at the narrow end of the anvil have a diameter of 0.5 mm, and the wide ends are subjected to a compressional force of 50,000 N. What is the pressure at the tip of the anvil?

Answer:
Here, compressional force, F = 50000 N
Diameter, D = 0.5 mm = 0.5 x 10^-3 m

Question 18.
A rod of length 1.05 m having negligible mass is supported at its ends by two wires of steel (wire A) and aluminium (wire B) of equal lengths as shown in Fig. The cross-sectional area of wires A and B are 1 mm² and 2 mm² respectively. At what point along the rod should a mass m be suspended in order to produce equal stresses and equal strains in both steel and aluminium wires? Given,

Answer:

Question 19.
A mild steel wire of length 1 m and cross-sectional area 0.5 x 10^-2 cm² is stretched, well within its elastic limit, horizontally between two pillars. A mass of 100 g is suspended from the midpoint of the wire. Calculate the depression at the midpoint, g = 10 ms^-2 ; Y = 2 x 10¹¹ Nm^-2.
Answer:


Question 20.
Two strips of metal are riveted together at their ends by four rivets, each of diameter 6 mm. What is the maximum tension that can be exerted by the riveted strip if the shearing stress on the rivet is not to
Answer:
Here, r = 6/2 = 3 mm = 3 x 10^-3 m; Max. stress = 2.9 x 10⁷ Pa ;
Max. load on a rivet = Max. stress x area of cross-section
= 2.9 x 10⁷ x (22/7) x (3 x 10^-3)²

Question 21.
The Marina Trench is located in the Pacific Ocean and at one place it is nearly eleven km beneath the surface of the water. The water pressure at the bottom of the trench is about 1.1 x 10⁸ Pa. A steel ball of initial volume 0.32 m³ is dropped into the ocean and falls to the bottom of the Trench. What is the change in the volume of the ball when it reaches the bottom?
Answer:
p = 1.1 x 10⁸ Pa ; V = 0.32 m³ ; K = 1.6 x 10¹¹ Pa

We hope the NCERT Solutions for Class 11 Physics Chapter 9 Mechanical Properties of Solids, help you. If you have any query regarding NCERT Solutions for Class 11 Physics Chapter 9 Mechanical Properties of Solids, drop a comment below and we will get back to you at the earliest.