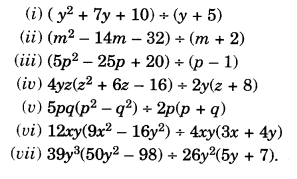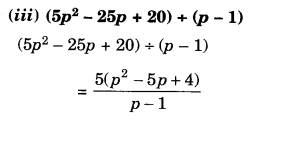Category: CBSE Class 8

RD Sharma Class 8 Solutions Chapter 17 Understanding Shapes III (Special Types of Quadrilaterals) Ex 17.1

These Solutions are part of RD Sharma Class 8 Solutions. Here we have given RD Sharma Class 8 Solutions Chapter 17 Understanding Shapes III Ex 17.1

Other Exercises

• RD Sharma Class 8 Solutions Chapter 17 Understanding Shapes III Ex 17.1
• RD Sharma Class 8 Solutions Chapter 17 Understanding Shapes III Ex 17.2
• RD Sharma Class 8 Solutions Chapter 17 Understanding Shapes III Ex 17.3

Question 1.
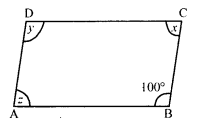
Given below is a parallelogram ABCD. Complete each statement along with the definition or property used:
(i) AD = ………
(ii) ∠DCB = ……….
(iii) OC’ = …….
(iv) ∠DAB + ∠CDA = …….

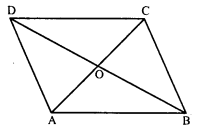
Solution:
(i) AD = BC
(ii) ∠DCB = ∠ADC

(iii) OC = OA
(iv) ∠DAB + ∠CDA = 180°

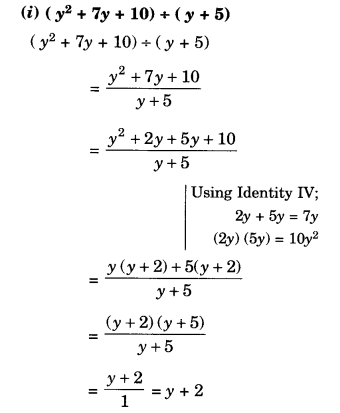
Question 2.
The following figures are parallelograms. Find the degree values of the unknowns x,y, z.


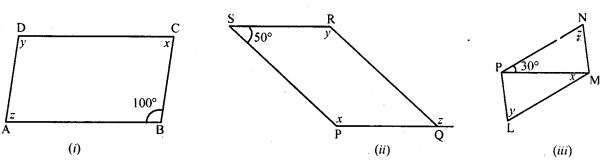
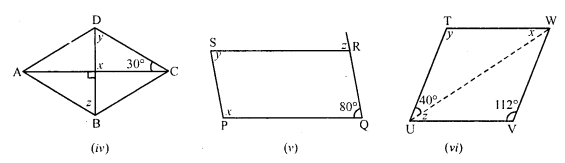
Solution:
In a parallelogram, opposite angles are equal and sum of adjacent angle is 180°.
(i) In parallelogram ABCD,
∠B = 100°
∠A = ∠C = 180° (Co-interior angles)

⇒ x + 100° = 180°
⇒ x = 180° – 100°
⇒ x = 80°
But ∠A = ∠C and ∠B = ∠D (Opposite angles)
A = x
⇒ z = x
⇒ z = 80°
and ∠D = ∠B
⇒ y = 100°
x = 80°, y = 100° and z = 80°
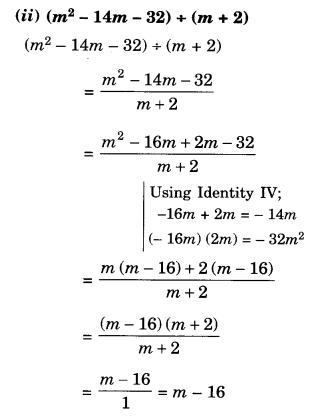
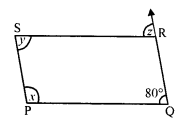
(ii) In parallelogram PQRS, side PQ is produced to T.

∠S = 50°
∠PQR = ∠S (Opposite angles)
w = 50°
But ∠P + ∠PQR = 180° (Sum of adjacent angles)
⇒ x + 50° = 180°
⇒ x = 180° – 50° = 130°
⇒ But ∠P = ∠R (Opposite angles)
x = y
⇒ y = 130°
But w + z = 180° (A linear pair)
⇒ 50° + z = 180°
⇒ z = 180° – 50° = 130°
⇒ x = 130°, y = 130°, ∠ = 130
(iii) In parallelogram LMNP, PM is its diagonal ∠NPM = 30°, ∠PMN = 90°

PN || LM (Opposite sides of a parallelogram) and PM is its transversal
∠NPM = ∠PML
⇒ 30° = x
x = 30°
In ∆PMN,
∠P = 30°, ∠M = 90°
But ∠P + ∠M + ∠N = 180° (Sum of angles of a triangle)
⇒ 30° + 90° + z = 180°
⇒ 120° + z = 180°
⇒ z = 180° – 120° = 60°
But ∠L = ∠N (Opposite angles of a parallelogram)
y = z
⇒ y = 60°
Hence x = 30°, y = 60° and ∠ = 60°
(iv) In rhombus ABCD, diagonals AC and BD bisect each other at right angles.

x = 90°
In ∆OCD,
∠O + ∠C + ∠D = 180°
⇒ 90° + 30° + y = 180°
⇒ 120° + y = 180°
⇒ y = 180° – 120° = 60°
y = 60°
CD || AB, BD is the transversal
y = z (Alternate angles)
z = 60°
Hence x = 90°, y = 60° and z = 60°
(v) In parallelogram PQRS, side QR is produced to T

∠Q = 80°
∠P + ∠Q = 180° (Sum of adjacent angles)
⇒ x + 80° = 180°
⇒ x = 180° – 80° = 100°
∠Q = ∠S (Opposite angles of a parallelogram)
⇒ 80° = y
⇒ y = 80°
PQ || SR and QRT is transversal
∠TRS = ∠RQP (Corresponding angles)
⇒ ∠ = 80°
Hence x = 100°, y = 80° and z = 80°
(vi) In parallelogram TUVW, UW is its diagonal

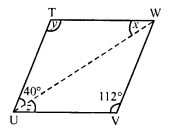
∠TUW = 40° and ∠V = 112°
∠T = ∠V (Opposite angles)
y = 112°
In ∆TUW,
∠T + ∠V + ∠W = 180° (Sum of angles of a parallelogram)
⇒ y + 40° + x = 180°
⇒ 112° + 40° + x = 180°
⇒ 152° + x = 180°
⇒ x = 180° – 152° = 28°
UV || TW and UW is its transversal
∠WUV = ∠TWU (Alternate angles)
⇒ z = x
⇒ z = 28°
Hence x = 28°, y = 112°, z = 28°

Question 3.
Can the following figures be parallelograms. Justify your answer.

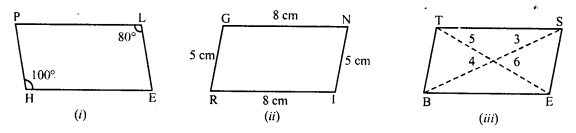
Solution:
(i) In quadrilateral PLEH
∠H = 100°, ∠L = 80°
But there are opposite angles
∠H ≠ ∠L
PLEH is not a parallelogram.
(ii) In quadrilateral GNIR,
RI = 8 cm, GN = 8 cm, RG = 5 cm and IN = 5 cm
PI = GH and RG = IN
But there are opposite sides of the quadrilateral.
GNIR is a parallelogram.
(iii) In quadrilateral BEST,
BS and ET are its diagonals
But these diagonal do not bisect each other.
BEST is not a parallelogram

Question 4.
In the adjacent figure HOPE is a parallelogram. Find the angle measures x, y and z. State the geometrical truths you use to find them.

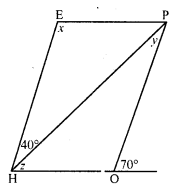
Solution:
In the figure, HOPE is a parallelogram in which HG is produced and HP is the diagonal

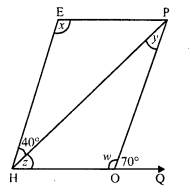
∠EHP = 40° and ∠POQ = 70°
But ∠POQ + ∠POH = 180° (Linear pair)
⇒ 70° + w = 180°
⇒ w = 180° – 70° = 110°
But ∠E = ∠POE (Opposite angles of a parallelogram)
x = 110°
HE || OP and HP is its transversal.
∠EHP = ∠HPO (Alternate angles)
⇒40° = y
⇒ y = 40°
In ∆PHO,
Ext. ∠POQ = ∠PHO + ∠HPO
⇒ 70° = z + y
⇒ 70° = z + 40°
⇒ z = 70° – 40° = 30°
Hence x = 110°, y = 40°, z = 30°

Question 5.
In the following figures GUNS and RUNS are parallelograms. Find x and y.

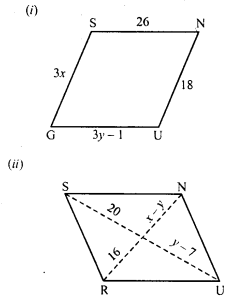
Solution:
(i) In parallelogram GUNS,
Opposite sides are parallel and equal
3x = 18
⇒ x = 6
and 3y – 1 = 26
⇒ 3y = 26 + 1 = 27
⇒ y = 9
x = 6, y = 9
(ii) Diagonals of a parallelogram bisect each other.
y – 7 = 20
⇒ y = 20 + 7 = 27
and x – 27 = 16
⇒ x – 27 = 16
⇒ x = 16 + 27 = 43
x = 43, y = 27

Question 6.
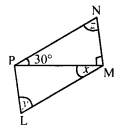
In the following figure RISK and CLUE are parallelograms. Find the measure of x.

Solution:
In the figure, RISK and CLUE are parallelograms
∠K = 120° and ∠L = 70°
In parallelogram RISK

RK || IS
∠RKS = ∠ISU (Corresponding angles)
⇒ ∠ISU = 120°
In parallelogram CLUE,
∠E = ∠L (Opposite angles of a parallelogram)
∠E = 70° (∠L = 70°)
Now in ∆EOS,
Ext. ∠ISU = x + ∠E
⇒ 120° = x + 70°
⇒ x = 120° – 70° = 50°
x = 50°

Question 7.
Two opposite angles of a parallelogram are (3x – 2)° and (50 – x)°. Find the measure of each angle of the parallelogram.
Solution:
In parallelogram ABCD,

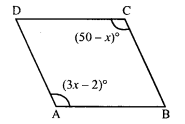
∠A = (3x – 2)° and ∠C = (50 – x)°
∠A = ∠C (Opposite angles of a parallelogram)
⇒ 3x – 2° = 50° – x
⇒ 3x + x = 50° + 2°
⇒ 4x = 52°
x = 13°

Question 8.
If an angle of a parallelogram is two- third of its adjacent angle, find the angles of the parallelogram.
Solution:
Let the parallelogram is ABCD and ∠A of a parallelogram ABCD be x

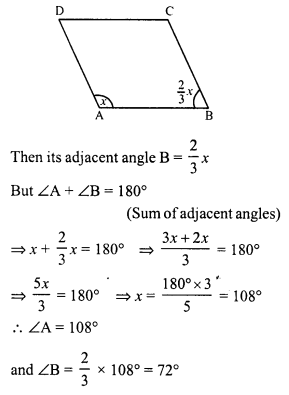
Hence other angles will be
∠C = ∠A = 108° (Opposite angles)
and ∠D = ∠B = 72° (Opposite angles)
Hence ∠A = 108°, ∠B = 72°, ∠C = 108° and ∠D = 72°

Question 9.
The measure of one angle of a parallelogram is 70°. What are the measures of the remaining angles ?
Solution:
Let in parallelogram ABCD,

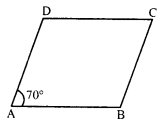
∠A = 70°
But ∠A + ∠B= 180° (Sum of adjacent angles)
⇒ 70° + ∠B = 180°
⇒ ∠B = 180° – 70°
⇒ ∠B = 110°
But ∠C = ∠A and ∠D = ∠B (Opposite angles)
∠C = 70° and ∠D = 110°
Hence ∠B = 110°, ∠C = 70° and ∠D = 110°

Question 10.
Two adjacent angles of a parallelogram are as 1 : 2. Find the measures of all the angles of the parallelogram.
Solution:
Let in parallelogram ABCD,

∠A : ∠B = 1 : 2
Let ∠A = x, then ∠B = 2x
But ∠A + ∠B = 180° (Sum of adjacent angles)
⇒ x + 2x = 180°
⇒ 3x = 180°
∠A = x = 60°
and ∠B = 2x = 2 x 60°= 120°
But ∠C = ∠A and ∠D = ∠B (Opposite angles)
∠C = 60° and ∠D = 120°
Hence ∠A = 60°, ∠B = 120°, ∠C = 60° and ∠D = 120°

Question 11.
In a parallelogram ABCD, ∠D = 135°, determine the measure of ∠A and ∠B.
Solution:
In parallelogram ABCD,

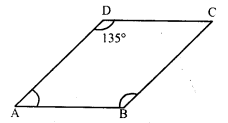
∠D = 135°
But ∠A + ∠D= 180° (Sum of adjacent angles)
∠A + 135° = 180°
∠A = 180° – 135° = 45°
But ∠B = ∠D (Opposite angles)
∠B = 135°
Hence ∠A = 45° and ∠B = 135°

Question 12.
ABCD is a parallelogram in which ∠A = 70°, compute ∠B, ∠C and ∠D.
Solution:
In parallelogram ABCD,

∠A = 70°
But ∠A + ∠B = 180° (Sum of adjacent angles)
⇒ 70° + ∠B = 180°
⇒ ∠B = 180° – 70° = 110°
But ∠C = ∠A and ∠D = ∠B (Opposite angles)
∠C = 70° and ∠D = 110°
Hence ∠B = 110°, ∠C = 70° and ∠D = 110°

Question 13.
The sum of two opposite angles of a parallelogram is 130°. Find all the angles of the parallelogram.
Solution:
Let in parallelogram

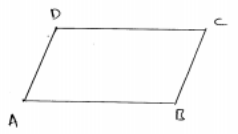
∠A + ∠C = 130°
But ∠A = ∠C (Opposite angles)
⇒ ∠C = \(\frac { 130 }{ 2 }\) = 65°
∠B + ∠D = 180° (Sum of adjacent angles)
⇒ 65° + ∠B = 180°
⇒ ∠B = 180° – 65° = 115°
⇒ ∠B = 115°
But ∠D = ∠B (Opposite angles)
∠D = 115°
Hence ∠A = 65°, ∠B = 115°, ∠C = 65° and ∠D = 115°

Question 14.
All the angles of a quadrilateral are equal to each other. Find the measure of each. Is the quadrilateral a parallelogram ? What special type of parallelogram is it ?
Solution:
All the angles of a quadrilateral are equal and sum of the four angles = 360°
Each angle will be = \(\frac { 360 }{ 4 }\) = 90°
Let in quadrilateral ABCD,
∠A = ∠B = ∠C = ∠D = 90°
It is a parallelogram as opposite angles are equal
i.e., ∠A = ∠C and ∠B = ∠D.
Each angle is of 90°
This parallelogram is a rectangle.

Question 15.
Two adjacent sides of a parallelogram are 4 cm and 3 cm respectively. Find its perimeter.
Solution:
Length of two adjacent sides = 4 cm and 3 cm
i.e., l = 4 cm and b = 3 cm
Perimeter = 2 (l + b) = 2 (4 + 3) cm = 2 x 7 = 14 cm

Question 16.
The perimeter of a parallelogram is 150 cm. One of its sides is greater ii.au the other by 25 cm. Find the length of the sides of the parallelogram.
Solution:
Perimeter of a parallelogram = 150 cm
Let l he the longer side and b be the shorter side
l = b + 25 cm.
⇒ 2 (l + b) = 150
⇒ l + b = 75
⇒ b + 25 + b = 75
⇒ 2b = 75 – 25 = 50
⇒ b = 25
l = b + 25 = 25 + 25 = 50
Sides are 50 cm, 25 cm

Question 17.
The shorter side of a parallelogram is 4.8 cm and the longer side is half as much again as the shorter side. Find the perimeter of the parallelogram.
Solution:
In a parallelogram shorter side (b) = 4.8 cm.
longer side (l) = 4.8 + \(\frac { 1 }{ 2 }\) x 4.8
= 4.8 + 2.4 = 7.2 cm
Perimeter = 2 (l + b) = 2 (7.2 + 4.8) cm = 2 x 12.0 = 24 cm

Question 18.
Two adjacent angles of a parallelogram are (3x – 4)° and (3x + 10)°. Find the angles of the parallelogram.
Solution:
Let in parallelogram ABCD,

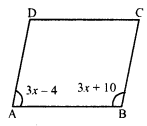
∠A = (3x + 4)° and ∠B = (3x + 10)°
But ∠A + ∠B = 180° (Sum of adj adjacent angles)
⇒ 3x – 4 + 3x + 10 = 180°
⇒ 6x + 6° = 180°
⇒ 6x = 180° – 6° = 174°
⇒ x = 29°
∠A = 3x – 4 = 3 x 29 – 4 = 87° – 4° = 83°
∠B = 3x + 10 = 3 x 29° + 10° = 87° + 10° = 97°
But ∠C = ∠A and ∠D = ∠B (Opposite angles)
∠C = 83° and ∠D = 97°
Hence ∠A = 83°, ∠B = 97°, ∠C = 83° and ∠D = 97°

Question 19.
In a parallelogram ABCD, the diagonals bisect each other at O. If ∠ABC = 30°, ∠BDC = 10° and ∠CAB = 70°.
Find : ∠DAB, ∠ADC, ∠BCD, ∠AOD, ∠DOC, ∠BOC, ∠AOB, ∠ACD, ∠CAB, ∠ADB, ∠ACB, ∠DBC and ∠DBA.
Solution:
In parallelogram ABCD, diagonal AC and ED bisect each other at O.

∠ABC = 30°, ∠CAB = 70° and ∠BDC = 10°
∠ADC = ∠ABC = 30° (Opposite angles)
and ∠ADB = ∠ADC – ∠BDC = 30° – 10° = 20°
AB || DC and AC is the transversal
∠ACD = ∠CAB = 70°(Altemate angles)
AB || DC and BD is transversal
∠CDB = ∠ABD = 10°(Altemate angles)
In ∆ABC
∠CAB + ∠ABC + ∠BCA = 180° (Sum of anlges of a triangle)
⇒ 70° + 30° + ∠BCA = 180°
⇒ 100° + ∠BCA = 180°
∠BCA = 180° – 100° = 80°
∠BCD = ∠BCA + ∠ACD = 80° + 70° = 150°
∠BCD = ∠DAB (Opposite angles)
⇒ ∠DAB = 150° and ∠CAD = 150° – 70° = 80°
In ∆OCD,
∠ODC + ∠OCD + ∠COD = 180° (Angles of a triangle)
⇒ ∠ACD + ∠ACD + ∠COD = 180°
⇒ 70° + 10° + ∠COD = 180°
⇒ 80° + ∠COD = 180°
⇒ ∠COD = 180° – 80° = 100°
∠COD = 100°
But ∠AOD + ∠COD = 180° (Linear pair)
⇒ ∠AOD + 100° = 180°
⇒ ∠AOD = 180° – 100° = 80°
⇒ ∠AOD = 80°
But ∠AOB = ∠COD
and ∠BOC = ∠AOD (Vertically opposite angles)
∠AOB = 100° and ∠BOC = 80°
Hence ∠DAB = 150°, ∠ADC = 30°, ∠BCD = 150°
∠AOD = 80° ∠DOC = 100°
∠BOC = 80° ∠AOB = 100°
∠ACD = 70°, ∠CAB = 70°, ∠AD = 20°, ∠ACB = 80°, ∠DBC = 20°, ∠DBA = 10

Question 20.
Find the angles marked with a question mark shown in the figure.

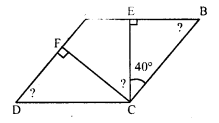
Solution:
In parallelogram ABCD,

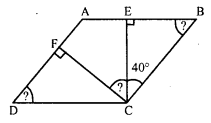
CE ⊥ AB and CF ⊥ AD
∠BCE = 40°
In ∆BCE,
∠BCE + ∠CEB + ∠EBC = 180° (Sum of angles of a triangle)
⇒ 40° + 90° + ∠EBC = 180°
⇒ 130° + ∠EBC = 180°
⇒ ∠EBC = 180° – 130° = 50°
or ∠B = 50°
But ∠D = ∠B (Opposite angles)
∠D = 50° or ∠ADC = 50°
Similarly in ∆DCF,
∠DCF + ∠CFD + ∠FDC = 180°
⇒ ∠DCF + 90° + 50° = 180°
⇒ ∠DCF + 140° = 180°
⇒ ∠DCF = 180° – 140° = 40°
But ∠C + ∠B= 180° (Sum of adjacent angles)
⇒ ∠BCE + ∠ECF + ∠DCF + ∠B = 180°
⇒ 40° + ∠ECF + 40° + 50° = 180°
⇒ ∠ECF + 130° = 180°
⇒ 40° + ∠ECF + 40° + 50° = 180°
⇒ ∠ECF + 130° = 180°
⇒ ∠ECF = 180° – 130° = 50°
∠ECF = 50°

Question 21.
The angle between the altitudes of a parallelogram through the same vertex of an obtuse angle of the parallelogram is 60°. Find the angles of the parallelogram.
Solution:
In parallelogram ABCD,

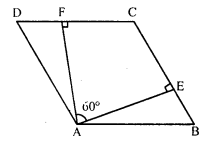
∠A is an obtused angle
AE ⊥ BC and AF ⊥ DC
∠EAF = 60°
In quadrilateral AECF,
∠EAF + ∠F + ∠C + ∠E = 360° (Sum of angles of a quadrilateral)
⇒ 60° + 90° + ∠C + 90° = 360°
⇒ 240° + ∠C = 360°
⇒ ∠C = 360° – 240° = 120°
∠C = 120°
But ∠A = ∠C (Opposite angles)
∠A = 120°
But ∠A + ∠B = 180° (Sum of adjacent angles)
⇒ 120° + ∠B = 180°
⇒ ∠B = 180° – 120° = 60°
∠B = 60°
But ∠D = ∠B (Opposite angles)
∠D = 60°
Hence ∠A = 120°, ∠B = 60°, ∠C = 120° and ∠D = 60°

Question 22.
In the figure, ABCD and AEFG are parallelograms. If ∠C = 55°, what is the measure of ∠F ?

Solution:
In the parallelogram ABCD,
∠A = ∠C …..(i) (Opposite angles of a parallelogram)
Similarly in parallelogram AEFG,
∠A = ∠F …(ii)
From (i) and (ii),
∠C = ∠F = 55° (∠C = 55°)
Hence ∠F = 55°

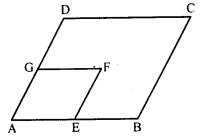
Question 23.
In the figure, BDEF and DCEF are each a parallelogram. It is true that BD = DC ? Why or why not ?

Solution:
In parallelogram BDEF,
BD = EF ……(i) (Opposite sides of a parallelogram)
Similarly, in parallelogram DCEF
DC = EF
From (i) and (ii),
BD = DC
Hence it is true that BD = DC.

Question 24.
In the figure, suppose it is known that DE = DF. Then is ∆ABC isosceles ? Why or why not ?
Solution:
In parallelogram BDEF,

∠B = ∠E ……(i) (Opposite angles of a parallelogram)
Similarly, in parallelogram DCEF,
∠C = ∠F ……(ii)
But DE = DF (Given)
In ∆DEF
∠E = ∠F
From (i) and (ii),
∠B = ∠C
AC = AB (Sides opposite to equal angles)
∆ABC is an isosceles triangle.

Question 25.
Diagonals of parallelogram ABCD intersect at O as shown in the figure. XY contains O, and X, Y are points on opposite sides of the parallelogram. Give reasons for each of the following:
(i) OB = OD
(ii) ∠OBY = ∠ODX
(iii) ∠BOY = ∠DOX
(iv) ∆BOY = ∆DOX
Now, state if XY is bisected at O.
Solution:
In parallelogram ABCD,

diagonals AC and BP intersect each other at O
O is the mid-point of AC and BD.
Through O, XY is draw such that X lies on AD and Y, on BC.
(i) OB = OD (O is mid-point of BD)
(ii) AD || BC and BD is transversal
∠OBY = ∠ODX (Alternate angles)
(iii) ∠BOY = ∠DOX (Vertically opposite angles)
(iv) Now in ∆BOY and ∆DOX,
OB = OD
∠OBY = ∠ODX
∠BOY = ∠DOX
∆BOY = ∆DOX (ASA axiom)
OY = OX (c.p.c.t.)
Hence XY is bisected at O.

Question 26.
In the figure ABCD is a parallelogram, CE bisects ∠C and AF bisects ∠A. In each of the following, if the statement is true, give a reason for the same.
(i) ∠A = ∠C
(ii) ∠FAB = \(\frac { 1 }{ 2 }\) ∠A
(iii) ∠DCE = \(\frac { 1 }{ 2 }\) ∠C
(iv) ∠CEB = ∠FAB
(v) CE || AF.
Solution:
In parallelogram ABCD,
CE is the bisector of ∠C and and AF is the bisector of ∠A.

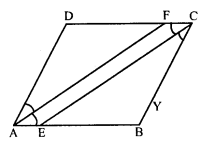
(i) ∠A = ∠C (Opposite angles of a parallelogram)
(ii) AF is the bisector of ∠A
∠FAB = \(\frac { 1 }{ 2 }\) ∠A
(iii) CE is the bisector of ∠C
∠DCE = \(\frac { 1 }{ 2 }\) ∠C
(iv) From (i), (ii) and (iii)
∠FAB = ∠DCE
(v) ∠FAB = ∠DCE
But these are opposite angles of quadrilateral AECF
AB or AE || DC or FC
AECF is a parallelogram
CE || AF
Hence proved

Question 27.
Diagonals of a parallelogram ABCD intersect at O. AL and CM are drawn perpendiculars to BD such that L and M lie on BD. Is AL = CM ? Why or why not ?
Solution:
In parallelogram ABCD, diagonals AC and BD intersect each other at O.

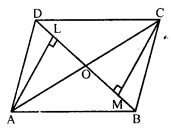
O is the mid-point of AC and BD.
AL ⊥ BD and CM ⊥ BD.
In ∆ALO and ∆CMO
∠L = ∠M (Each 90°)
∠AOL = ∠COM (Vertically opposite angles)
AO = CO (O is mid-point of AC)
∆ALO = ∆CMO (AAS axiom)
AL = CM (c.p.c.t.)
Hence proved

Question 28.
Points E and F lie on diagonal AC of a parallelogram ABCD such that AE = CF. What type of quadrilateral is BFDE ?
Solution:
In parallelogram ABCD,

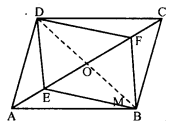
AC is its diagonal. E and F are points on AC such that AE = CF
Join EB, BF, FD and DE .
Join also diagonal BD which intersects AC at O
O is the mid-point of AC and BD
AO = OC
But AE = CF
⇒ AO – AE = CO – CF
⇒ EO = OF
But BO = OD (O is mid-point of BD)
Diagonals EF and BD of quadrilateral bisect each other at O.
BFDE is a parallelogram.

Question 29.
In a parallelogram ABCD, AB = 10 cm, AD = 6 cm. The bisector of ∠A meets DC in E. AE and BC produced meet at F. Find the length of CF.
Solution:
AB || DC

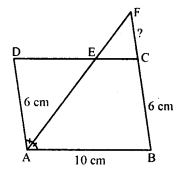
∠DEA = ∠EAB (Alternate angles)
= ∠DAE (EA is bisector of ∠A)
In ∆DAE,
∠DEA = ∠DAE
AD = DE = 6 cm
But DE = AB = 10 cm.
EC = DC – DE = 10 – 6 = 4 cm
AD || BC or BF and AF is transversal
∠DAE = ∠EFC (Alternate angle)
But ∠DAE = ∠DEA Prove
= ∠FEC (DEA = FEC vertically opposite angles)
In ∆ECF,
CE = CF = 4 cm (CE = 4 cm)

 

Hope given RD Sharma Class 8 Solutions Chapter 17 Understanding Shapes III Ex 17.1 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 8 Solutions Chapter 12 Percentage Ex 12.2

These Solutions are part of RD Sharma Class 8 Solutions. Here we have given RD Sharma Class 8 Solutions Chapter 12 Percentage Ex 12.2

Other Exercises

• RD Sharma Class 8 Solutions Chapter 12 Percentage Ex 12.1
• RD Sharma Class 8 Solutions Chapter 12 Percentage Ex 12.2

Question 1.
Find :
(i) 22% of 120
(ii) 25% of Rs. 1000
(iii) 25% of 10 kg
(iv) 16.5% of 5000 metres
(v) 135% of 80 cm
(vi) 2.5% of 10000 ml
Solution:

Question 2.
Find the number ‘a’, if
(i) 8.4% of a is 42
(ii) 0.5% of a is 3
(iii) \(\frac { 1 }{ 2 }\) % of a is 50
(iv) 100% of a is 100
Solution:

Question 3.
x is 5% of y, y is 24% of z. If x = 480, find the values of y and z.
Solution:
x = 5% of y, y = 24% of z.
x = 480

Question 4.
A coolie deposits Rs. 150 per month in his post office Saving Bank account. If this is 15% of his monthly income, find his monthly income.
Solution:
Let his monthly income = Rs. x
15% of x = Rs. 150

Question 5.
Asha got 86.875% marks in the annual examination. If she got 695 marks, find the number of marks of the Examination.
Solution:
Let total marks of the examination = x
86.875% of x = 695
=> 86.875 x \(\frac { 1 }{ 100 }\) x x = 695

Question 6.
Deepti went to school for 216 days in a full year. If her attendance is 90%, find the number of days on which the school was opened ?
Solution:
Let the school opened for = x days = 90% of x = 216

Question 7.
A garden has 2000 trees. 12% of these are mango trees, 18% lemon and the rest are orange trees. Find the number of orange trees.
Solution:
Number of total trees = 2000

Rest trees = 2000 – (240 + 360) = 2000 – 600 = 1400
Number of orange trees = 1400

Question 8.
Balanced diet should contain 12% of protein, 25% of fats and 63% of carbohydrates. If a child needs 2600 calories in this food daily, find in calories the amount of each of these in his daily food in take.
Solution:
Balance diet contains
Protein = 12%
Fats = 25%
Carbohydrates = 63%
Number of total calories = 2600
Number of calories of proteins = 12% of 2600 = \(\frac { 12 }{ 100 }\) x 2600 = 312
Number of calories of fats = 25% of 2600 = \(\frac { 25 }{ 100 }\) x 2600 = 650
Number of calories of carbohydrates = 63% of 2600 = \(\frac { 63 }{ 100 }\) x 2600 = 1638

Question 9.
A cricketer scored a total of 62 runs in 96 balls. He hits 3 sixes, 8 fours, 2 twos and 8 singles. What percentage of the total runs came in :
(i) Sixes
(ii) Fours
(iii) Twos
(iv) Singles
Solution:
Total score of a cricketer = 62 runs
(z) Number of sixes = 3
Run from 3 sixes = 3 x 6 = 18
Percentage = \(\frac { 18 }{ 62 }\) x 100 = 29.03%
(ii) Number of fours = 8
Total run from 8 fours = 4 x 8 = 32
Percentage = \(\frac { 32 }{ 62 }\) x 100 = 51.61%
(iii) Number of twos = 2
Total score from 2 twos = 2 x 2 = 4
Percentage = \(\frac { 4 }{ 62 }\) x 100 = \(\frac { 400 }{ 62 }\) = 6.45%
(iv) Number of single run = 8
Percentage = \(\frac { 8 }{ 62 }\) x 100 = \(\frac { 800 }{ 62 }\) = 12.9%

Question 10.
A cricketer hits 120 runs in 150 balls during a test match. 20% of the runs came in 6’s, 30% in 4’s, 25% in 2’s and the rest in 1’s. How many runs did he score in :
(i) 6’s
(ii) 4’s
(iii) 2’s
(iv) singles
What % of his shots were scoring ones ?
Solution:
Total runs scored by a cricketer =120
(i) Number of runs from sixes (6’s) = 20% of 120

Question 11.
Radha earns 22% of her investment. If she earns Rs. 187, then how much did she invest ?
Solution:
Total earning from investment = Rs. 187
Percent earning = 22%
Let his investment = x
Then 22% of x = Rs. 187

Question 12.
Rohit deposits 12% his income in a bank. He deposited Rs. 1440 in the bank during 1997. What was his total income for the year 1997 ?
Solution:
Deposit in the bank = Rs. 1440
Percentage = 12% of his total income
Let his total income = Rs. x

Question 13.
Gunpowder contains 75% nitre and 10% sulphur. Find the amount of the gunpowder which carries 9 kg nitre. What amount of gunpowder would contain 2.3 kg sulphur ?
Solution:
(i) In gunpowder,
Nitre = 75%
Sulphur = 10%
Let total amount of gunpowder = x kg
Nitre = 9 kg

Question 14.
An alloy of tin and copper consists of 15 parts of tin and 105 parts of copper. Find the percentage of copper in the alloy ?
Solution:
In an alloy,
Number of parts of tin = 15
and number of parts of copper = 105
Total parts = 15 + 105 = 120
Percentage of copper in the alloy = \(\frac { 105 }{ 120 }\) x 100 = 87.5%

Question 15.
An alloy contains 32% copper, 40% nickel and rest zinc. Find the mass of the zinc in 1 kg of the alloy.
Solution:
In an alloy,
Copper = 32%
Nickel = 40%
Rest is zinc = 100 – (32 + 40) = 100 – 72 = 28%
Mass of zinc in 1 kg = 28% of 1 kg = \(\frac { 28 }{ 100 }\) x 100 gm = 280 gm.

Question 16.
A motorist travelled 122 kilometres before his first stop. If he had 10% of his journey to complete at this point, how long was the total ride ?
Solution:
Distance travelled before first stop = 122 km
Let total journey = x km
10% of x = 122

Question 17.
A certain school has 300 students, 142 of whom are boys. It has 30 teachers, 12 of whom are men. What percent of the total number of students and teachers in the school is female ?
Solution:
Total numbers of teachers = 30
Number of male teachers = 12
Number of female teacher = 30 – 12 = 18
Percentage of female teachers = \(\frac { 18 x 100 }{ 30 }\) = 60%

Question 18.
Aman’s income is 20% less than that of Anil. How much percent is Anil’s income more than Aman’s income ?
Solution:
Let Anil’s income = Rs. 100
Then Aman’s income = Rs, 100 – 20 = Rs. 80
Now, difference of both’s incomes = 100 – 80 = Rs. 20
Anil income is Rs. 20 more than that of Aman’s
Percentage = \(\frac { 20 x 100 }{ 80 }\) = 25%

Question 19.
The value of a machine depreciates every year by 5%. If the present value of the machine be Rs. 100000, what will be its value after 2 years ?
Solution:
Present value of machine = Rs. 100000
Rate of depreciation per year = 5%
Period = 2 years
Value of machine after 2 years

Question 20.
The population of a town increases by 10% annually. If the present population is 60000, what will be its population after 2 years ?
Solution:
Present population of the town = 60000
Increase annually = 10%
Period = 2 years
Population after 2 years will be

Question 21.
The population of a town increases 10% annually. If the present population is 22000, find its population a year ago.
Solution:
Let the population of the town a year ago was = x
Increase in population = 10%

Question 22.
Ankit was given an increment of 10% on his salary. His new salary is Rs. 3575. What was his salary before increment ?
Solution:
Let the salary of Ankit before increment = x
Increment given = 10% of the salary
Salary after increment will be

Question 23.
In the new budget, the price of petrol rose by 10%. By how much percent must one reduce the consumption so that the expenditure does not increase ?
Solution:
Let price of petrol before budged = Rs. 100
Increase = 10%
Price after budget = Rs. 100 + 10 = Rs. 110
Let the consumption of petrol before budget = 100 l
Price pf 100 l = Rs. 110
Now of new price is Rs. 110, consumption = 100 l
are of new price will be 100, then

Question 24.
Mohan’s income is Rs. 15500 per month. He saves 11% of his income. If his income increases by 10% then he reduces his saving by 1%, how much does he save now ?
Solution:
Mohan’s income = Rs. 15500

We see that the savings is same
There is no change in savings.

Question 25.
Shikha’s income is 60% more than that of Shalu. What percent is Shalu’s income less than Shikha’s ?
Solution:
Let Shalu’s income = Rs. 100
Then Shikha’s income will be = Rs. 100 + 60 = Rs. 160
Now difference in their incomes = Rs. 160 – 100 = Rs. 60
Shalu’s income is less than Shikha’s income by Rs. 60
Percentage less = \(\frac { 60 x 100 }{ 160 }\) = \(\frac { 75 }{ 2 }\) % = 37.5%

Question 26.
Rs. 3500 is to be shared among three people so that the first person gets 50% of the second who in turn gets 50% of the third. How much will each of them get ?
Solution:
Let the third person gets = Rs. x
Then second person will get

Question 27.
After a 20% hike, the cost of Chinese Vase is Rs. 2000. What was the original price of the object ?
Solution:
Let the original price of the vase = Rs. x
Hike in price = 20%

Original price of the vase = Rs. 1666.66

Hope given RD Sharma Class 8 Solutions Chapter 12 Percentage Ex 12.2 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.