Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.
Enzyme Catalysis
Enzymes are complex protein molecules with three dimensional structures. They catalyse the chemical reaction in living organism. They are often present in colloidal state and extremely specific in catalytic action. Each enzyme produced in a particular living cell can catalyse a particular reaction in the cell.
Some common examples for enzyme catalysis
1. The peptide glycyl L-glutamyl L-tyrosin is hydrolysed by an enzyme called pepsin.
2. The enzyme diastase hydrolyses starch into maltose
2(C6H10O5)n + nH2O → nC12H22O11
3. The yeast contains the enzyme zymase which converts glucose into ethanol.
C6H12O6 → 2C2H5OH + 2CO2
4. The enzyme micoderma aceti oxidises alcohol into acetic acid.
C2H5OH + O2 → CH3COOH + H2O
5. The enzyme urease present in soya beans hydrolyses the urea.
NH2 – CO – NH2 + H2O → 2NH3 + CO2
Mechanism of Enzyme Catalysed Reaction
The following mechanism is proposed for the enzyme catalysis
E + S ⇄ ES → P + E
Where E is the enzyme, S the substrate (reactant), Es represents activated complex and P the products.
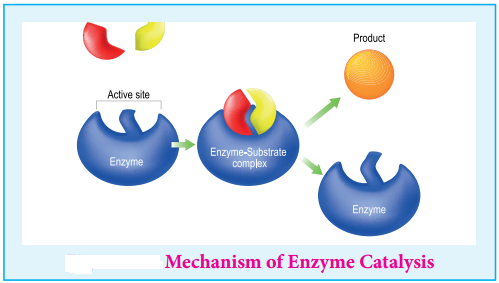
Enzyme Catalysed Reaction show Certain General Special Characteristics.
(i) Effective and efficient conversion is the special characteristic of enzyme catalysed reactions. An enzyme may transform a million molecules of reactant into product in a minute.
For eg. 2H2O2 → 2H2O + O2
For this reaction, the activation energy is 18k cal/mole without a catalyst. With colloidal platinum as a catalyst the activation energy is 11.7 kcal/mole.
But with the enzyme catalyst the activation energy of this reaction is less than 2kcal/mole.
(ii) Enzyme catalysis is highly specific in nature.
H2N-CO-NH2 + H2O → 2NH3 + CO2
The enzyme urease which catalyses the reaction of urea does not catalyse the following reaction of methyl urea
H2N-CO-NH-CH3 + H2O → No reaction
(iii) Enzyme catalysed reaction has maximum rate at optimum temperature. At first rate of reaction increases with the increase of temperature, but above a particular temperature the activity of enzyme is destroyed. The rate may even drop to zero. The temperature at which enzymic activity is high or maximum is called as optimum temperature.
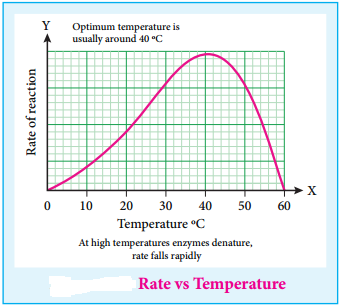
For Example:
- Enzymes involved in human body have an optimum temperature 37°C/98°F
- During high fever, as body temperature rises the enzymatic activity may collapse and lead to danger.
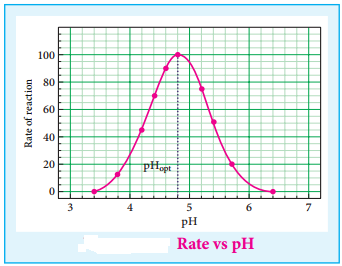
(iv) The rate of enzyme catalysed reactions varies with the pH of the system. The rate is maximum at a pH called optimum pH.
(v) Enzymes can be inhibited i.e. poisoned. Activity of an enzyme is decreased and destroyed by a poison. The physiological action of drugs is related to their inhibiting action.
Example: Sulpha drugs. Penicillin inhibits the action of bacteria and used for curing diseases like pneumonia, dysentery, cholera and other infectious diseases.
(vi) Catalytic activity of enzymes is increased by coenzymes or activators. A small non protein (vitamin) called a coenzyme promotes the catalytic activity of enzyme.
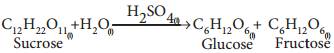



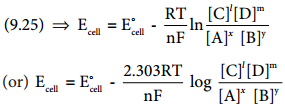 ………… (9.31)
………… (9.31)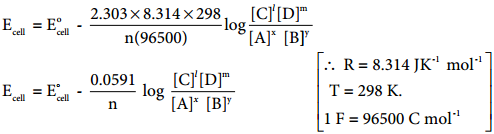 ……………… (9.32)
……………… (9.32)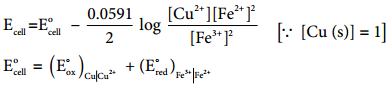
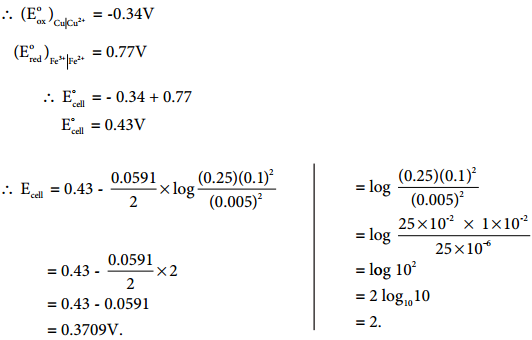

 …………… (9.35)
…………… (9.35)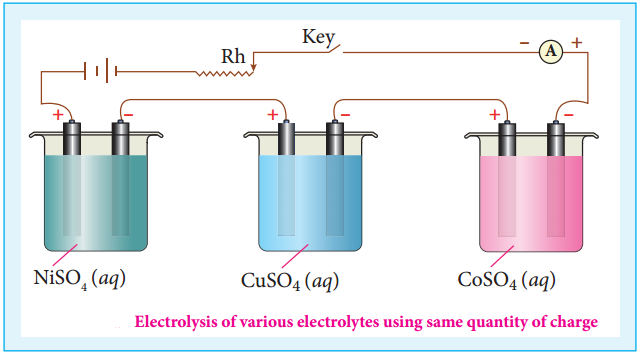
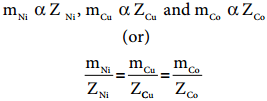 ………….. (9.36)
………….. (9.36)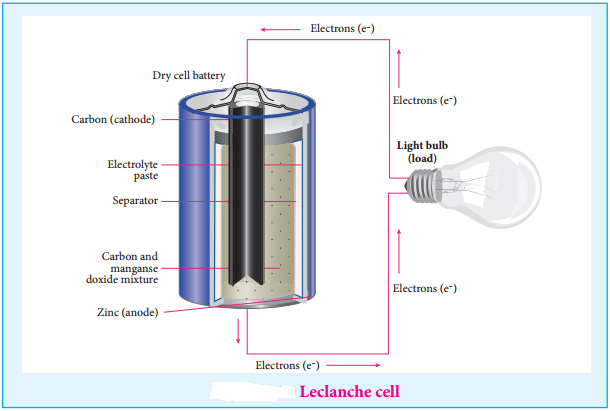
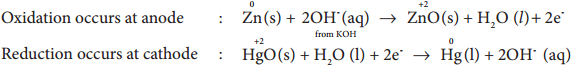

 ………… (9.14)
………… (9.14)
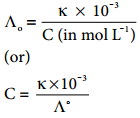
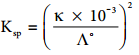 ………… (9.17)
………… (9.17)

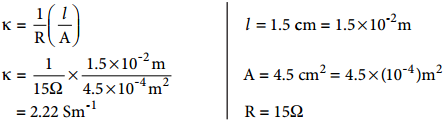
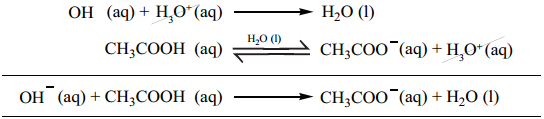
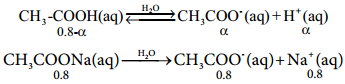
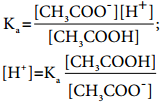
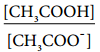
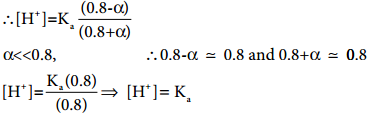
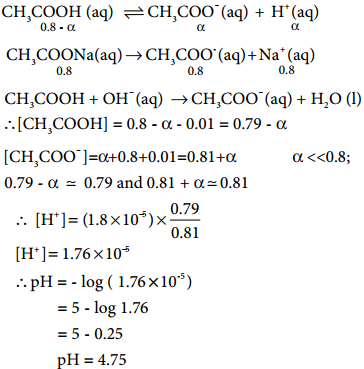
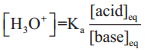 ………….. (8.20)
………….. (8.20)