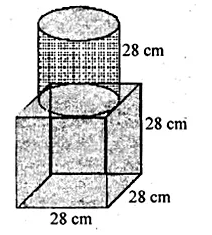
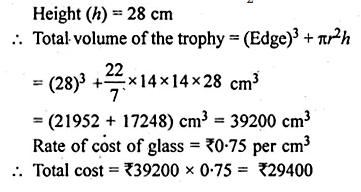
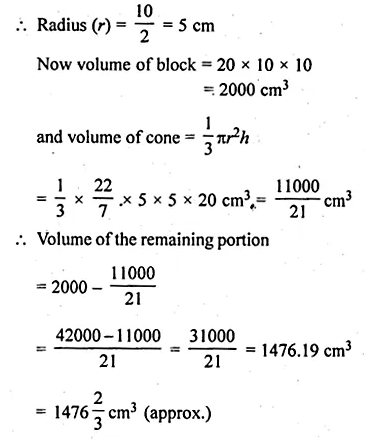
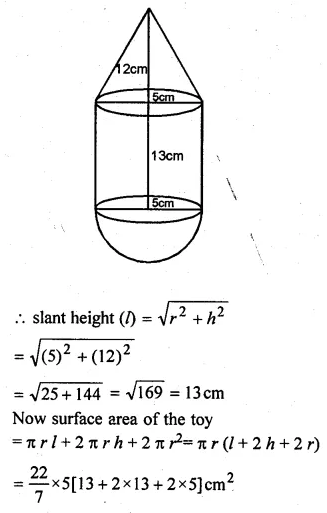
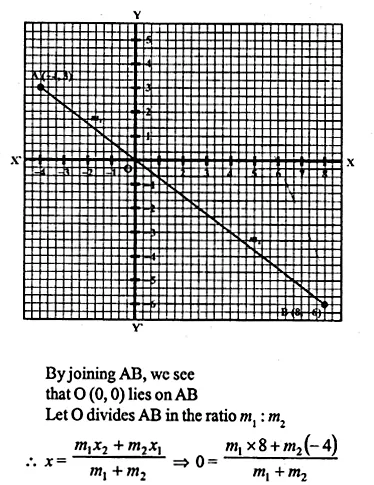
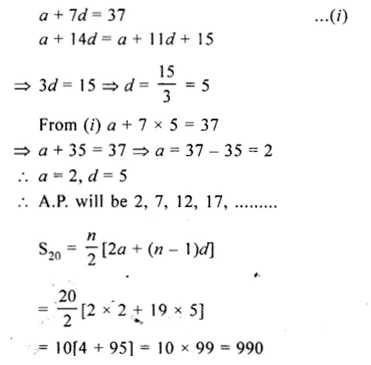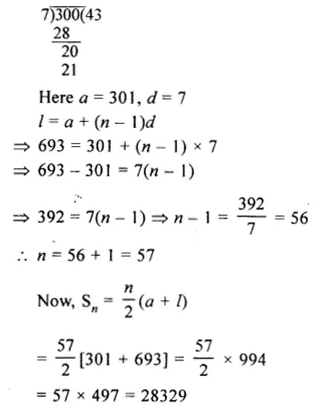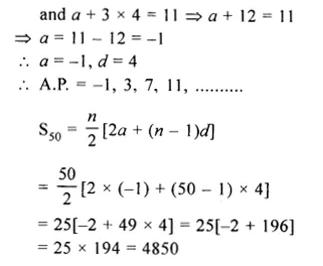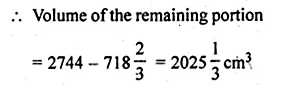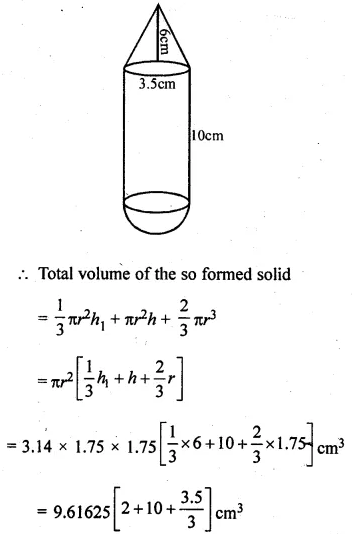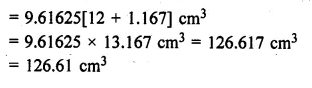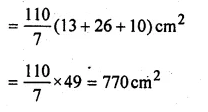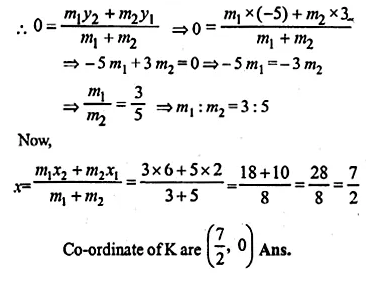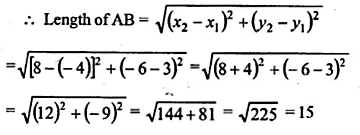ICSE Class 10 – Page 17

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations Ex 4A

February 2, 2023

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations (In one variable) Ex 4A

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations Ex 4A.

Other Exercises

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations Ex 4A
Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations Ex 4B

Question 1.
State, true or false :
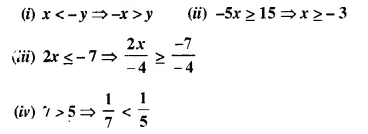
Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations Ex 4A 1.1
Solution:

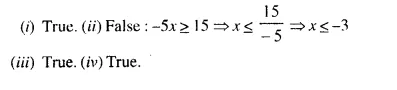
Question 2.
State whether the following statements are true or false:
(i) If a < b, then a – c < b – c (ii) If a > b, then a + c > b + c
(iii) If a < b, then ac > bc
(iv) If a > b, then \(\frac { a }{ b }\) < \(\frac { b }{ c }\) (v) If a – c > b – d; then a + d > b + c
(vi) If a < b, and c > 0, then a – c > b – c where a, b, c and d are real numbers and c ≠ 0.
Solution:
(i) True
(ii) True
(iii) False
(iv) False
(v) True
(vi) False

Question 3.
If x ∈ N, find the solution set of inequations,
(i) 5x + 3 ≤ 2x + 18
(ii) 3x – 2 < 19 – 4x
Solution:
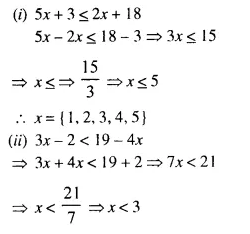
Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations Ex 4A 3.1
x = {1, 2}

Question 4.
If the replacement set is the set of whole numbers, Solve:
(i) x + 7 ≤ 11
(ii) 3x – 1 > 8
(iii) 8 – x > 5
(iv) 7 – 3x ≥ – \(\frac { 1 }{ 2 }\)
(v) x – \(\frac { 3 }{ 2 }\) < \(\frac { 3 }{ 2 }\) – x
(vi) 18 ≤ 3x – 2
Solution:
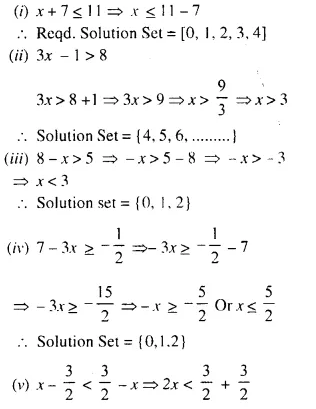
Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations Ex 4A 4.1

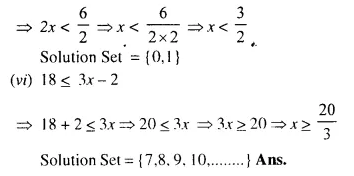
Question 5.
Solve the inequation :
3 – 2x ≥ x – 12 given that x ∈ N. [1987]
Solution:
3 – 2x ≥ x – 12
⇒ – 2x – x ≥ – 12 – 3
⇒ – 3x ≥ -15
⇒ – x ≥ – 5
⇒ x ≤ 5
Solution Set= {1, 2, 3, 4, 5} or {x ∈ N : x ≤ 5}

Question 6.
If 25 – 4x ≤ 16, find:
(i) the smallest value of x, when x is a real number
(ii) the smallest value of x, when x is an integer.
Solution:
25 – 4x ≤ 16
⇒ – 4x ≤ 16 – 25
⇒ – 4x ≤ – 9
⇒ 4x ≥ 9
x ≥ \(\frac { 9 }{ 4 }\)
(i) The smallest value of x, when x is a real number \(\frac { 9 }{ 4 }\) or 2.25
(ii) The smallest value of x, when x is an integer 3.

Question 7.
If the replacement set is the set of real numbers, solve:
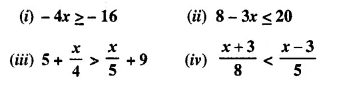
Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations Ex 4A 7.1
Solution:

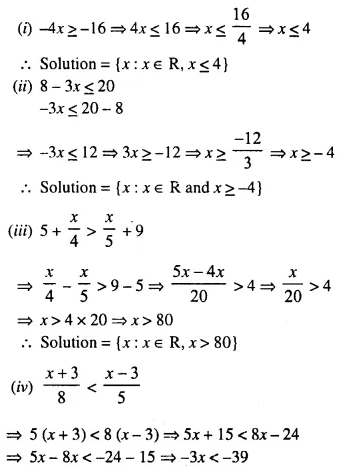
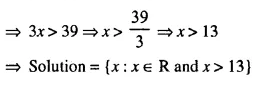
Question 8.
Find the smallest value of x for which 5 – 2x < 5\(\frac { 1 }{ 2 }\) – \(\frac { 5 }{ 3 }\) x, where x is an integer.
Solution:
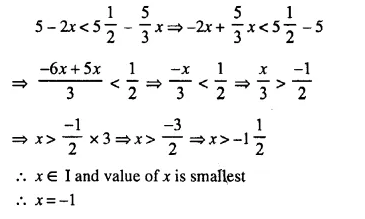
Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations Ex 4A 8.1

Question 9.
Find the largest value of x for which 2 (x – 1) ≤ 9 – x and x ∈ W.
Solution:
2 (x – 1) ≤ 9 – x
⇒ 2x – 2 ≤ 9 – x
⇒ 2x + x ≤ 9 + 2
⇒ 3x ≤ 11
⇒ x ≤ \(\frac { 11 }{ 3 }\)
⇒ x ≤ 3\(\frac { 2 }{ 3 }\)
x ∈ W and value of x is largest x = 3

Question 10.
Solve the inequation:
12 + 1\(\frac { 5 }{ 6 }\) x ≤ 5 + 3x and x ∈ R. (1999)
Solution:
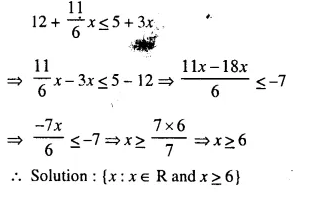
Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations Ex 4A 10.1

Question 11.
Given x ∈ (integers), find the solution set of: -5 ≤ 2x – 3 < x + 2.
Solution:
-5 ≤ 2x – 3 < x + 2
(i) -5 ≤ 2x – 3
⇒ -2x ≤ -3 + 5
⇒ -2x ≤ 2
⇒ x ≤ -1
⇒ -1 ≤ x
(ii) 2x – 3 < x + 2
⇒ 2x – x < 2 + 3
⇒ x < 5
From (i) and (ii),
-1 ≤ x < 5
x = {-1, 0, 1, 2, 3, 4}

Question 12.
Given x ∈ (whole numbers), find the solution set of: -1 ≤ 3 + 4x < 23.
Solution:
-1 ≤ 3 + 4x < 23
(i) -1 ≤ 3 + 4x
⇒ -1 – 3 ≤ 4x
⇒ -4 < 4x
⇒ -1 ≤ x
(ii) 3 + 4x < 23
⇒ 4x < 23 – 3
⇒ 4x < 20
⇒ x < 5
From (i) and (ii)
-1 ≤ x < 5 and x ∈ W
Solution set = {0, 1, 2, 3, 4}

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations Ex 4A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2

January 1, 2023

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2

These Solutions are part of ML Aggarwal Class 10 Solutions for ICSE Maths. Here we have given ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2

More Exercises

Question 1.
Draw an equilateral triangle of side 4 cm. Draw its circumcircle.
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2 Q1.1
Solution:
Steps of Construction :
(i) Draw a line segment BC = 4 cm
(ii) With centres B and C, draw two arcs of radius 4 cm
which intersect each other at A.
(iii) Join AB and AC. ∆ ABC is an equilateral triangle.
(iv) Draw the right bisector of BC and AC intersecting each other at O.
(v) Join OA, OB and OC.
(vi) With centre O, and radius equal to OB or OC or OA,
draw a circle which will pass through A, B and C.
This is the required circumcircle of ∆ ABC.

Question 2.
Using a ruler and a pair of compasses only, construct: (i) a triangle ABC given AB = 4cm, BC = 6 cm and ∠ABC = 90°.
(ii) a circle which passes through the points A, B and C and mark its centre as O. (2008)
Solution:
Steps of Construction:
(i) Draw a line segment AB = 4cm
(ii) At B, draw a ray BX making an angle of 90°
and cut off BC = 6 cm.
(iii) Join AC.
(iv) Draw the perpendicular bisectors of sides
AB and AC intersecting each other at O.
(v) With centre O, and radius equal to OB or OA or OC,
draw a circle which passes through A, B and C.
This is the required circle.
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2 Q2.1

Question 3.
Construct a triangle with sides 3 cm, 4 cm and 5 cm. Draw its circumcircle and measure its radius.
Solution:
Steps of Construction
(i) Draw a line segment BC = 4 cm.
(ii) With centre B and radius 3 cm and with centre C
and radius 5 cm draw two arcs which intersect each other at A.
(iii) Join AB and AC.
(iv) Draw the perpendicular bisector of sides BC and AC
which intersect each other at O.
(v) Join OB.
(vi) With centre O and radius OB, draw a circle
which will pass through A, B and C.
(vii) On measuring the radius OB = 2.5cm
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2 Q3.1

Question 4.
Using ruler and compasses only :
(i) Construe a triangle ABC with the following data: Base AB = 6 cm, AC = 5.2 cm and ∠CAB = 60°.
(ii) In the same diagram, draw a circle which passes through the points A, B and C. and mark its centre O.
Solution:
Steps of Construction :
(i) Draw a line segment AB = 6 cm.
(ii) At A, draw a ray making an angle of 60°.
(iii) With centre B and radius 5-2 cm.
draw an arc which intersects the ray at C.
(iv) Join BC
(v) Draw the perpendicular bisector of AB and BC
intersecting each other at O.
(vi) With O as a centre and OA as a radius
draw a circle which touches the ∆ABC at A, B and C.
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2 Q4.1

Question 5.
Using ruler and compasses only, draw an equilateral triangle of side 5 cm and draw its inscribed circle. Measure the radius of the circle.
Solution:
Steps of Construction :
(i). Draw a line segment BC = 5 cm
(ii) With centre B and C and radius 5 cm,
draw two arcs intersecting each other at A.
(iii) Join AB and AC.
(iv) Draw the angle bisectors of ∠B and ∠C intersecting each other at I.
(v) From I, draw a perpendicular ID on BC.
(vi) With centre I and radius ID, draw a circle
which touches the sides of the triangle internally.
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2 Q5.1
This is the required in circle.
Measure the radius ID which is 1.5 cm (approx)

Question 6.
(i) Conduct a triangle ABC with BC = 6.4 cm, CA = 5.8 cm and ∠ ABC = 60°. Draw its incircle. Measure and record the radius of the incircle.
(ii) Construct a ∆ABC with BC = 6.5 cm, AB = 5.5 cm, AC = 5 cm. Construct the incircle of the triangle. Measure and record the radius of the incircle. (2014)
Solution:
Steps of Construction :
(i) Draw a line segment BC = 6.4 cm
(ii) Construct ∠ DBC = 60° at B.
(iii) With C as centre and radius CA = 5.8 cm.
Draw an arc cutting BD at A.
(iv) Join AC. Then ABC is the required triangle.
(v) Draw the angle bisectors of ∠B and ∠C which intersect each other at O.
(vi) Draw OE ⊥ BC, intersecting BC in E.
(vii) With O as centre and OE as radius draw the required incircle.
Measure the radius OE which is = 1.5cm
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2 Q6.1
Steps of construction:
1. Draw a line BC = 6.5 cm.
2. With centre B and C draw arcs AB = 5.5 cm and AC = 5 cm
3. Join AB and AC, ABC is the required triangle.
4. Draw the angle bisetors of B and C. Let these bisectors meet at O.
5. Taking O as centre. Draw a incircle which touches all the sides of the ∆ABC.
6. From O draw a perpendicular to side BC which cut at N.
7. Measure ON which is required radius of the incircle. ON = 1.5 cm
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2 Q6.2

Question 7.
The bisectors of angles A and B of a scalene triangle ABC meet at O.
(i) What is the point O called?
(ii) OR and OQ are drawn a perpendicular to AB and CA respectively. What is the relation between OR and OQ ?
(iii) What is the relation between ∠ACO and ∠BCO?
Solution:
(i) The point O where the angle bisectors meet is called the incentre of the triangle.
(ii) The perpendiculars drawn from O to AB and CA are equal i.e. OR and OQ.
(iii) ∠ACO = ∠BCO
OC will bisect the ∠C
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2 Q7.1

Question 8.
Using ruler and compasses only, construct a triangle ABC in which BC = 4 cm, ∠ACB = 45° and the perpendicular from A on BC is 2.5 cm. Draw the circumcircle of triangle ABC and measure its radius.
Solution:
Steps of Construction
(i) Draw a line segment BC = 4 cm.
(ii) At B, draw a perpendicular and cut off BE = 2.5 cm.
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2 Q8.1
(iii) From E, draw a line EF parallel to BC.
(iv) From C, draw a ray making an angle of 45° which intersects EF at A.
(v) Join AB.
(vi) Draw the perpendicular bisectors of
sides BC and AC intersecting each other at O.
(vii) Join OB, OC and OA.
(viii) With centre 0 and radius OB or OC or OA draw a circle
which will pass through A, B and C.
This circle is the circumcircle of ∆ABC. On measuring its radius OB = 2 cm.

Question 9.
Construct a regular hexagon of side 4 cm. Construct a circle circumscribing the hexagon.
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2 Q9.1
Solution:
Steps of Construction
(i) Draw a line segment BC = 4 cm.
(ii) At A and B draw rays making on angle of 120° each and cut off AF = BC = 4cm.
(iii) At F and C, draw rays making angle of 120° each and cut off EF = CD = 4cm.
(iv) Join ED.
ABCDEF is the required hexagon.
(v) Draw perpendicular bisectors of sides AB and BC intersecting each other at O.
(vi) With centre O and radius equal OA or OB draw a circle
which passes through the vertices of the hexagon.
This is the required circumcircle of hexagon ABCDEF.

Question 10.
Draw a regular hexagon of side 4 cm and construct its incircie.
Solution:
Steps of constructions :
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2 Q10.1
(i) Draw a regular hexagon ABCDEF of side 4 cm.
(ii) Draw the angle bisectors of ∠A and ∠B
which intersect each other at O.
(iii) Draw OL ⊥ AB.
(iv) With centre O and radius OB, draw a circle
which touches the sides of the hexagon

Hope given ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.1

January 1, 2023

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.1

These Solutions are part of ML Aggarwal Class 10 Solutions for ICSE Maths. Here we have given ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.1 More Exercises

Question 1. Use a ruler and compass only in this question. (i) Draw a circle, centre O and radius 4 cm. (ii) Mark a point P such that OP = 7 cm. Construct the two tangents to the circle from P. Measure and record the length of one of the tangents. Solution: Steps of Construction:

Draw a circle with centre O and radius 4 cm.
Take a point P such that OP = 7 cm.
Bisect OB at M.
With centre M and diameter OP, draw another circle intersecting the given circle at A and B.
Join PA and PB. PA and PB is a pair of tangents to the circle.
On measuring PA, it is equal to 5.5 cm.

Question 2. Draw a line AB = 6 cm. Construct a circle with AB as diameter. Mark a point P at a distance of 5 cm from the mid-point of AB. Construct two tangents from P to the circle with AB as diameter. Measure the length of each tangent Solution: Steps of Construction:

Take a line segment AB = 6 cm.
Draw its perpendicular bisector bisecting it at O.
With centre O and radius OB, draw a circle.
Produce AB to P such that OP = 5 cm.
Draw its perpendicular bisector intersecting it at M.
With centre M and radius OM, draw a circle which intersects the given circle at T and S.
Join OT, OS, TP and SP. PT and PS are the required tangents to the given circle.
On measuring, each tangent is 4 cm long i.e. PT = PS = 4 cm.

Question 3. Construct a tangent to a circle of radius 4cm from a point on the concentric circle of radius 6 cm and measure its length. Also, verify the measurement by actual calculation. Solution: Steps of construction:

Take a point O.
With centre O and radii 4 cm and 6 cm, draw two concentric circles.
Join OA and take its mid-point M.
With centre M and radius MA, draw another circle which intersects the first circle at P and Q.
Join AP and AQ. AP and AQ are the required tangents to the first circle from point A.

Question 4. Draw a circle of radius 3 cm. Take two points P and Q on one of its extended diameter each at a distance of 7 cm from its centre. Draw tangents to the circle from these two points P and Q. Solution: Steps of construction:

Take a point O and with centre O, and radius 3 cm, draw a circle.
Produce its diameter both sides and cut off OP = OQ = 7 cm.
Take mid-points of OP and OQ as M and N respectively.
With centres M and N and OP and OQ as diameters, draw circles which intersect the given circle at A, B and C and D respectively.
Join PA, PB, QC and QD. PA, PB and QC and QD are the required tangents.

Question 5. Draw a line segment AB of length 8 cm. Taking A as centre, draw a circle of radius 4 cm and taking B as centre, draw another circle of radius 3 cm. Construct tangents to each circle from the centre of the other circle. Solution: Steps of construction:

Draw a line segment AB = 8 cm.
With centre A and radius 4 cm and with centre B and radius 3 cm, draw circles.
Draw a third circle AB as diameter which intersects the given two circles at C and D and P and Q respectively.
Join AC and AD, BP and BQ.
AC and AD, BP and BQ are the required tangents.

Hope given ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.1 are helpful to complete your math homework. If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10C

November 5, 2021

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10C

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10C. https://ncertmcq.com/selina-concise-mathematics-class-10-icse-solutions/

Other Exercises

Question 1.
Find the sum of the first 22 terms of the A.P. : 8, 3, – 2,…..
Solution:
A.P. = 8, 3, – 2,…..
Here, a = 8, d = 3 – 8 = – 5, n = 22

Question 2.
How many terms of the A.P. : 24, 21, 18, must be taken so that their sum is 78 ?
Solution:
Let n term of the given A.P. be taken
and A.P. = 24, 21, 18……
Let n be the number of terms.
Here, a = 24, d = 21 – 24 = – 3, S_n = 78

Question 3.
Find the sum of 28 terms of an A.P. whose nth term is 8n – 5.
Solution:
nth term (T_n) = 8n – 5
T₁ = 8 – 5 = 3
T₂ = 8 x 2 – 5 = 16 – 5 = 11
T₃ = 8 x 3 – 5 = 24 – 5 = 19
A.P. is 3, 11, 19,
Here, a = 3,d = 11 – 3 = 8 and n = 28

Question 4.
Find the sum of :
(i) all odd natural numbers less than 50.
(ii) first 12 natural numbers each of which is a multiple of 7.
Solution:
(i) Sum of all odd natural numbers less then 50
1 + 3 + 5 + 7 +…….+ 49
Here a = 1, d = 3 – 1 = 2
Let n be the number of term, then
49 = a + (n – 1)d
=> 49 = 1 + (n – 1) x 2
=> 49 – 1 = (n – 1) x 2

Question 5.
Find the sum of first 51 terms of an A.P. whose 2nd and 3rd terms are 14 and 18 respectively.
Solution:
Sum of first 51 terms of an A.P. in which T₂ = 14 and T₃ = 18
d = T₃ – T₂ = 18 – 14 = 4
and T₂ = a + d
=> 14 = a + 4
=> a = 14 – 4 = 10
A.P. = 10, 14, 18, 22,….

Question 6.
The sum of first 7 terms of an A.P. is 49 and that of first 17 terms of it is 289. Find the sum of first tt terms.
Solution:
S₇ = 49,
S₁₇ = 289

Question 7.
The first term of an A.P. is 5, the last term is 45 and the sum of its terms is 1000. Find the number of terms and the common difference of the A.P.
Solution:
First term of an AP (a) = 5
Last term = 45
and S_n = 1000

Question 8.
Find the sum of all natural numbers between 250 and 1000 which are divisible by 9.
Solution:
All natural numbers between 250 and 1000 which are divisible by 9 are
252, 261, 270……999

Question 9.
The first and the last terms of an A.P. are 34 and 700 respectively. If the common difference is 18, how many terms are there and what is their sum?
Solution:
In an A.P.
T₁ = a = 34, l = 700, d = 18
Let n be the number of terms, then
T_n = a + (n – 1 )d
=> 700 = 34 + (n – 1) x 18
=> 700 – 34 = 18(n – 1)
=> 180 – 0 = 666

Question 10.
In an A.P. the first term is 25, nth term is – 17 and the sum of n terms is 132. Find n and the common difference.
Solution:
In an A.P.
First term (a) = 25
nth term = – 17
and S_n = 132
Let d be the common difference

Question 11.
If the 8th term of an A.P. is 37 and the 15th term is 15 more than the 12th term, find the A.P. Also, find the sum of first 20 terms of this A.P.
Solution:
In an A.P.
8th term = 37
15th term = 12th term + 15
Let a be the first term and d be the common difference, then

Question 12.
Find the sum of all multiples of 7 lying between 300 and 700.
Solution:
Multiples of 7 lying between 300 and 700 are 301, 308, 315, 322,…., 693

Question 13.
The sum of n natural numbers is 5n² + 4n. Find its 8th term.
Solution:
Sum of n natural number = 5n² + 4n
S_n = 5n² + 4n
S₁(a) = 5 x (1)² + 4 x 1
= 5 + 4 = 9
S₂ = 5(2)² + 4 x 2 = 20 + 8 = 28
S₂ – S₁ = T₂ = 28 – 9 = 19
=> a + d = 19 => 9 + d = 19
d = 19 – 9 = 10
a = 9, d = 10
T₈ = a + (n – 1 )d = 9 + (8 – 1) x 10
= 9 + 7 x 10 = 9 + 70 = 79

Question 14.
The fourth term of an A.P. is 11 and the eighth term exceeds twice the fourth term by 5. Find the A.P. and the sum of first 50 terms.
Solution:
In an A.P.
T₄= 11
T₈ = 2T₄ + 5
Now, a + 3d = 11
a + 7d = 2 x 11 + 5 = 22 + 5 = 27
Subtracting, 4d = 16
=> d = \(\\ \frac { 16 }{ 4 } \) = 4

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 17 Mensuration Ex 17.4

November 2, 2021

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 17 Mensuration Ex 17.4

These Solutions are part of ML Aggarwal Class 10 Solutions for ICSE Maths. Here we have given ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 17 Mensuration Ex 17.4 https://ncertmcq.com/ml-aggarwal-icse-solutions-for-class-10-maths/

More Exercise

Take π = \(\\ \frac { 22 }{ 7 } \), unless stated otherwise.

Ex 17.4 Class 10 Ml Aggarwal Question 1.
The adjoining figure shows a cuboidal block of wood through which a circular cylinderical hole of the biggest size is drilled. Find the volume of the wood left in the block.

Solution:
Diameter of the biggest hole = 30 cm.
Radius (r) = \(\\ \frac { 30 }{ 2 } \) = 15 cm
and height (h) = 70 cm.

Ml Aggarwal Class 10 Ex 17.4. Solutions Question 2.
The given figure shows a solid trophy made of shining glass. If one cubic centimetre of glass costs Rs 0.75, find the cost of the glass for making the trophy.

Solution:
Edge of cubical part = 28 cm
and radius of cylindrical part (r) = \(\\ \frac { 28 }{ 2 } \) = 14cm

Ml Aggarwal Class 10 Solutions Mensuration Question 3.
From a cube of edge 14 cm, a cone of maximum size is carved out. Find the volume of the remaining material.
Solution:
Edge of a cube = 14 cm
Volume = (side)³ = (14)³ = 14 × 14 × 14 cm³ = 2744 cm³
Now diameter of the cone cut out from it = 14 cm

Ml Aggarwal Class 10 Mensuration Question 4.
A cone of maximum volume is curved out of a block of wood of size 20 cm x 10 cm x 10 cm. Find the volume of the remaining wood.
Solution:
Size of wooden block = 20 cm × 10 cm × 10 cm
Maximum diameter of the cone = 10 cm
and height (h) = 20 cm

Ml Aggarwal Class 10 Mensuration Solutions Question 5.
16 glass spheres each of radius 2 cm are packed in a cuboidal box of internal dimensions 16 cm x 8 cm x 8 cm and then the box is filled with water. Find the volume of the water filled in the box.
Solution:
Given
Radius of each glass sphere = 2 cm

Ml Aggarwal Class 10 Solutions Chapter Mensuration Question 6.
A pen stand made of wood is in the shape of a cuboid with four conical depressions to hold pens. The dimensions of the cuboid are 15 cm by 10 cm by 3.5 cm. The radius of each of the depression is 0.5 cm and the depth is 1.4 cm. Find the volume of the wood in the entire stand, correct to 2 decimal places.

Solution:
Dimensions of cuboid = 15 cm × 10 cm × 3.5 cm
and radius of each conical depression (r) = 0.5 cm
and depth (h) = 1.4 cm
Volume of cuboid = l × b × h

Ml Aggarwal Class 10 Solutions Gst Question 7.
A cuboidal block of side 7 cm is surmounted by a hemisphere. What is the greatest diameter that the hemisphere can have? Also, find the surface area of the solid.
Solution:
Side of cuboidal = 7 cm
Diameter of hemisphere = 7 cm
and radius (r) = \(\\ \frac { 7 }{ 2 } \) cm

Ml Aggarwal Class 10 Solutions Question 8.
A wooden article was made by scooping out a hemisphere from each end of a solid cylinder (as shown in the given figure). If the height of the cylinder is 10 cm and its base is of radius 3.5 cm, find the total surface area of the article.

Solution:
Height of the cylinder = 10 cm
and radius of the base = 3.5 cm
Total surface area
= Curved surface area of cylinder + 2 × Curved surface area of hemisphere

Www.Ilovemaths.Com For Class 10 Icse Solutions Question 9.
A toy is in the form of a cone of radius 3.5 cm mounted on a hemisphere of same radius. If the total height of the toy is 15.5 cm, find the total surface area of the toy.
Solution:
Total height of the toy = 15.5 cm
Radius of the base of the conical part (r) = 3.5 cm

Ml Aggarwal Class 10 Solutions Icse Gst Question 10.
A circus tent is in the shape of a cylinder surmounted by a cone. The diameter of the cylindrical portion is 24 m and its height is 11 m. If the vertex of the cone is 16 m above the ground, find the area of the canvas used to make the tent.
Solution:
Radius of base of cylindrical portion of tent (r) = \(\\ \frac { 24 }{ 2 } \) = 12 m

Ml Aggarwal Class 10 Solutions Icse Question 11.
An exhibition tent is in the form of a cylinder surmounted by a cone. The height of the tent above the ground is 85 m and the height of the cylindrical part is 50 m. If the diameter of the base is 168 m, find the quantity of canvas required to make the tent. Allow 20% extra for folds and stitching. Give your answer to the nearest m².
Solution:
Total height of the tent = 85 m
Height of cylindrical part (h₁) = 50 m

Question 12.
From a solid cylinder of height 30 cm and radius 7 cm, a conical cavity of height 24 cm and of base radius 7 cm is drilled out. Find the volume and the total surface of the remaining solid.
Solution:
Radius of solid cylinder (r₁) = 7 cm

Question 13.
The given figure shows a wooden toy rocket which is in the shape of a circular cone mounted on a circular cylinder. The total height of the rocket is 26 cm, while the height of the conical part is 6 cm. The base of the conical portion has a diameter of 5 cm, while the base diameter of the cylindrical portion is 3 cm. If the conical portion is to be painted green and the cylindrical portion red, find the area of the rocket painted with each of these colours. Also, find the volume of the wood in the rocket. Use π = 3.14 and give answers correct to 2 decimal places.

Solution:
In the given figure,
The total height of the toy rocket = 26 cm
Diameter of cylindrical portion = 3 cm

Question 14.
The given figure shows a hemisphere of radius 5 cm surmounted by a right circular cone of base radius 5 cm. Find the volume of the solid if the height of the cone is 7 cm. Give your answer correct to two places of decimal.
Solution:
Height of the conical part (h) = 7 cm
and radius of the base (r) = 5 cm

Question 15.
A buoy is made in the form of a hemisphere surmounted by a right cone whose circular base coincides with the plane surface of the hemisphere. The radius of the base of the cone is 3.5 metres and its volume is \(\\ \frac { 2 }{ 3 } \) of the hemisphere. Calculate the height of the cone and the surface area of the buoy correct to 2 places of decimal
Solution:
Radius of base of hemisphere = \(\\ \frac { 7 }{ 2 } \) m

Question 16.
A circular hall (big room) has a hemispherical roof. The greatest height is equal to the inner diameter, find the area of the floor, given that the capacity of the hall is 48510 m³.
Solution:
Let h be the greatest height
and r be the radius of the base
Then 2r = h + r ⇒ h = r

Question 17.
A building is in the form of a cylinder surmounted by a hemisphere valted dome and contains \(41 \frac { 19 }{ 21 } \) m3 of air. If the internal diameter of dome is equal to its total height above the floor, find the height of the building
Solution:
Volume of air in dome = \(41 \frac { 19 }{ 21 } \) m³
= \(\\ \frac { 880 }{ 21 } \) m³
Let radius of the dome = r m
Then height (h) = r m

Question 18.
A rocket is in the form of a right circular cylinder closed at the lower end and surmounted by a cone with the same radius as that of the cylinder. The diameter and the height of the cylinder are 6 cm and 12 cm respectively. If the slant height of the conical portion is 5 cm, find the total surface area and the volume of the rocket. (Use π = 3.14).
Solution:
Height of the cylindrical part (A) = 12 cm
Diameter = 6 cm
Radius (r) = \(\\ \frac { 6 }{ 2 } \) = 3 cm
Slant height of the conical part (l) = 5 cm

Question 19.
A solid is in the form of a right circular cylinder with a hemisphere at one end and a cone at the other end. Their common diameter is 3.5 cm and the height of the cylindrical and conical portions are 10 cm and 6 cm respectively. Find the volume of the solid. (Take π = 3.14)
Solution:
Diameter = 3.5 cm
Radius (r) = \(\\ \frac { 3.5 }{ 2 } \) = 1.75 cm
Height of cylindrical part (h₁) = 10 cm
and height of conical part (h₂) = 6 cm

Question 20.
A toy is in the shape of a right circular cylinder with a hemisphere on one end and a cone on the other. The height and radius of the cylindrical part are 13 cm and 5 cm respectively. The radii of the hemispherical and conical parts are the same as that of the cylindrical part. Calculate the surface area of the toy if the height of the conical part is 12 cm.
Solution:
Height of cylindrical part = 13 cm
Radius = 5 cm
Radius of cone (r) = 5 cm
Height of cone (h) = 12 cm

Question 21.
The given figure shows a model of a solid consisting of a cylinder surmounted by a hemisphere at one end. If the model is drawn to a scale of 1 : 200, find
(i) the total surface area of the solid in π m².
(ii) the volume of the solid in π litres.

Solution:
(i) In the given figure,
Height of cylindrical portion (h) = 8 cm
Radius (r) = 3 cm
Scale = 1 : 200

We hope the ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 17 Mensuration Ex 17.4 help you. If you have any query regarding ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 17 Mensuration Ex 17.4, drop a comment below and we will get back to you at the earliest.

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11

July 20, 2021

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11

These Solutions are part of ML Aggarwal Class 10 Solutions for ICSE Maths. Here we have given ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11

More Exercises

Midpoint Calculator is used to find the midpoint between 2 line segments using the midpoint formula. Use our calculator to find accurate midpoints step by step.

Question 1.
Find the co-ordinates of the mid-point of the line segments joining the following pairs of points:
(i) (2, – 3), ( – 6, 7)
(ii) (5, – 11), (4, 3)
(iii) (a + 3, 5b), (2a – 1, 3b + 4)
Solution:
(i) Co-ordinates of the mid-point of (2, -3), ( -6, 7)
\(\left( \frac { { x }_{ 1 }+{ x }_{ 2 } }{ 2 } ,\frac { { y }_{ 1 }+{ y }_{ 2 } }{ 2 } \right) or \)
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q1.1

Question 2.
The co-ordinates of two points A and B are ( – 3, 3) and (12, – 7) respectively. P is a point on the line segment AB such that AP : PB = 2 : 3. Find the co-ordinates of P.
Solution:
Points are A (-3, 3), B (12, -7)
Let P (x₁, y₁) be the point which divides AB in the ratio of m₁ : m₂ i.e. 2 : 3
then co-ordinates of P will be
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q2.1

Question 3.
P divides the distance between A ( – 2, 1) and B (1, 4) in the ratio of 2 : 1. Calculate the co-ordinates of the point P.
Solution:
Points are A (-2, 1) and B (1, 4) and
Let P (x, y) divides AB in the ratio of m₁ : m₂ i.e. 2 : 1
Co-ordinates of P will be
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q3.1

Question 4.
(i) Find the co-ordinates of the points of trisection of the line segment joining the point (3, – 3) and (6, 9).
(ii) The line segment joining the points (3, – 4) and (1, 2) is trisected at the points P and Q. If the coordinates of P and Q are (p, – 2) and \(\left( \frac { 5 }{ 3 } ,q \right) \) respectively, find the values of p and q.
Solution:
(i) Let P (x₁, y₁) and Q (x₂, y₂) be the points
which trisect the line segment joining the points
A (3, -3) and B (6, 9)
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q4.1

Question 5.
(i) The line segment joining the points A (3, 2) and B (5, 1) is divided at the point P in the ratio 1 : 2 and it lies on the line 3x – 18y + k = 0. Find the value of k.
(ii) A point P divides the line segment joining the points A (3, – 5) and B ( – 4, 8) such that \(\frac { AP }{ PB } =\frac { k }{ 1 } \) If P lies on the line x + y = 0, then find the value of k.
Solution:
(i) The point P (x, y) divides the line segment joining the points
A (3, 2) and B (5, 1) in the ratio 1 : 2
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q5.1

Question 6.
Find the coordinates of the point which is three-fourth of the way from A (3, 1) to B ( – 2, 5).
Solution:
Let P be the required point, then
\(\frac { AP }{ AB } =\frac { 3 }{ 4 } \)
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q6.1

Question 7.
Point P (3, – 5) is reflected to P’ in the x- axis. Also P on reflection in the y-axis is mapped as P”.
(i) Find the co-ordinates of P’ and P”.
(ii) Compute the distance P’ P”.
(iii) Find the middle point of the line segment P’ P”.
(iv) On which co-ordinate axis does the middle point of the line segment P P” lie ?
Solution:
(i) Co-ordinates of P’, the image of P (3, -5)
when reflected in x-axis will be (3, 5)
and co-ordinates of P”, the image of P (3, -5)
when reflected in y-axis will be (-3, -5)
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q7.1

Question 8.
Use graph paper for this question. Take 1 cm = 1 unit on both axes. Plot the points A(3, 0) and B(0, 4).
(i) Write down the co-ordinates of A1, the reflection of A in the y-axis.
(ii) Write down the co-ordinates of B1, the reflection of B in the x-axis.
(iii) Assign.the special name to the quadrilateral ABA1B1.
(iv) If C is the mid point is AB. Write down the co-ordinates of the point C1, the reflection of C in the origin.
(v) Assign the special name to quadrilateral ABC1B1.
Solution:
Two points A (3, 0) and B (0,4) have been plotted on the graph.
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q8.1
(i)∵ A1 is the reflection of A (3, 0) in the v-axis Its co-ordinates will be ( -3, 0)
(ii)∵ B1 is the reflection of B (0, 4) in the .x-axis co-ordinates of B, will be (0, -4)
(iii) The so formed figure ABA1B1 is a rhombus.
(iv) C is the mid point of AB co-ordinates of C” will be \(\frac { AP }{ AB } =\frac { 3 }{ 4 } \)
∵ C, is the reflection of C in the origin
co-ordinates of C, will be \(\left( \frac { -3 }{ 2 } ,-2 \right) \)
(v) The name of quadrilateral ABC1B1 is a trapezium because AB is parallel to B1C1.

Question 9.
The line segment joining A ( – 3, 1) and B (5, – 4) is a diameter of a circle whose centre is C. find the co-ordinates of the point C. (1990)
Solution:
∵ C is the centre of the circle and AB is the diameter
C is the midpoint of AB.
Let co-ordinates of C (x, y)
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q9.1

Question 10.
The mid-point of the line segment joining the points (3m, 6) and ( – 4, 3n) is (1, 2m – 1). Find the values of m and n.
Solution:
Let the mid-point of the line segment joining two points
A(3m, 6) and (-4, 3n) is P( 1, 2m – 1)
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q10.1

Question 11.
The co-ordinates of the mid-point of the line segment PQ are (1, – 2). The co-ordinates of P are ( – 3, 2). Find the co-ordinates of Q.(1992)
Solution:
Let the co-ordinates of Q be (x, y)
co-ordinates of P are (-3, 2) and mid-point of PQ are (1, -2) then
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q11.1

Question 12.
AB is a diameter of a circle with centre C ( – 2, 5). If point A is (3, – 7). Find:
(i) the length of radius AC.
(ii) the coordinates of B.
Solution:
AC = \(\sqrt { { \left( 3+2 \right) }^{ 2 }+{ \left( -7-5 \right) }^{ 2 } } \)
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q12.1

Question 13.
Find the reflection (image) of the point (5, – 3) in the point ( – 1, 3).
Solution:
Let the co-ordinates of the images of the point A (5, -3) be
A1 (x, y) in the point (-1, 3) then
the point (-1, 3) will be the midpoint of AA1.
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q13.1

Question 14.
The line segment joining A \(\left( -1,\frac { 5 }{ 3 } \right) \) the points B (a, 5) is divided in the ratio 1 : 3 at P, the point where the line segment AB intersects y-axis. Calculate
(i) the value of a
(ii) the co-ordinates of P. (1994)
Solution:
Let P (x, y) divides the line segment joining
the points \(\left( -1,\frac { 5 }{ 3 } \right) \), B(a, 5) in the ratio 1 : 3
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q14.1

Question 15.
The point P ( – 4, 1) divides the line segment joining the points A (2, – 2) and B in the ratio of 3 : 5. Find the point B.
Solution:
Let the co-ordinates of B be (x, y)
Co-ordinates of A (2, -2) and point P (-4, 1)
divides AB in the ratio of 3 : 5
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q15.1

Question 16.
(i) In what ratio does the point (5, 4) divide the line segment joining the points (2, 1) and (7 ,6) ?
(ii) In what ratio does the point ( – 4, b) divide the line segment joining the points P (2, – 2), Q ( – 14, 6) ? Hence find the value of b.
Solution:
(i) Let the ratio be m₁ : m₂ that the point (5, 4) divides
the line segment joining the points (2, 1), (7, 6).
\(5=\frac { { m }_{ 1 }\times 7+{ m }_{ 2 }\times 2 }{ { m }_{ 1 }+{ m }_{ 2 } } \)
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q16.1

Question 17.
The line segment joining A (2, 3) and B (6, – 5) is intercepted by the x-axis at the point K. Write the ordinate of the point k. Hence, find the ratio in which K divides AB. Also, find the coordinates of the point K.
Solution:
Let the co-ordinates of K be (x, 0) as it intersects x-axis.
Let point K divides the line segment joining the points
A (2, 3) and B (6, -5) in the ratio m₁ : m₂.
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q17.1

Question 18.
If A ( – 4, 3) and B (8, – 6), (i) find the length of AB.
(ii) in what ratio is the line joining AB, divided by the x-axis? (2008)
Solution:
Given A (-4, 3), B (8, -6)
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q18.1

Question 19.
(i) Calculate the ratio in which the line segment joining (3, 4) and( – 2, 1) is divided by the y-axis.
(ii) In what ratio does the line x – y – 2 = 0 divide the line segment joining the points (3, – 1) and (8, 9)? Also, find the coordinates of the point of division.
Solution:
(i) Let the point P divides the line segment joining the points
A (3, 4) and B (-2, 3) in the ratio of m₁ : m₂ and
let the co-ordinates of P be (0, y) as it intersects the y-axis
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q19.1

Question 20.
Given a line segment AB joining the points A ( – 4, 6) and B (8, – 3). Find:
(i) the ratio in which AB is divided by the y-axis.
(ii) find the coordinates of the point of intersection.
(iii)the length of AB.
Solution:
(i) Let the y-axis divide AB in the ratio m : 1. So,
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q20.1

Question 21.
(i) Write down the co-ordinates of the point P that divides the line joining A ( – 4, 1) and B (17,10) in the ratio 1 : 2.
(ii)Calculate the distance OP where O is the origin.
(iii)In what ratio does the y-axis divide the line AB ?
Solution:
(i) Let co-ordinate of P be (x, y) which divides the line segment joining the points
A ( -4, 1) and B(17, 10) in the ratio of 1 : 2.
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q21.1

Question 22.
Calculate the length of the median through the vertex A of the triangle ABC with vertices A (7, – 3), B (5, 3) and C (3, – 1)
Solution:
Let D (x, y) be the median of ΔABC through A to BC.
∴ D will be the midpoint of BC
∴ Co-ordinates of D will be,
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q22.1

Question 23.
Three consecutive vertices of a parallelogram ABCD are A (1, 2), B (1, 0) and C (4, 0). Find the fourth vertex D.
Solution:
Let O in the mid-point of AC the diagonal of ABCD
∴ Co-ordinates of O will be
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q23.1

Question 24.
If the points A ( – 2, – 1), B (1, 0), C (p, 3) and D (1, q) from a parallelogram ABCD, find the values of p and q.
Solution:
A (-2, -1), B (1, 0), C (p, 3) and D (1, q)
are the vertices of a parallelogram ABCD
∴ Diagonal AC and BD bisect each other at O
O is the midpoint of AC as well as BD
Let co-ordinates of O be (x, y)
When O is mid-point of AC, then
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q24.1

Question 25.
If two vertices of a parallelogram are (3, 2) ( – 1, 0) and its diagonals meet at (2, – 5), find the other two vertices of the parallelogram.
Solution:
Two vertices of a ||gm ABCD are A (3, 2), B (-1, 0)
and point of intersection of its diagonals is P (2, -5)
P is mid-point of AC and BD.
Let co-ordinates of C be (x, y), then
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q25.1

Question 26.
Prove that the points A ( – 5, 4), B ( – 1, – 2) and C (5, 2) are the vertices of an isosceles right angled triangle. Find the co-ordinates of D so that ABCD is a square.
Solution:
Points A (-5, 4), B (-1, -2) and C (5, 2) are given.
If these are vertices of an isosceles triangle ABC then
AB = BC.
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q26.1

Question 27.
Find the third vertex of a triangle if its two vertices are ( – 1, 4) and (5, 2) and mid point of one sides is (0, 3).
Solution:
Let A (-1, 4) and B (5, 2) be the two points and let D (0, 3)
be its the midpoint of AC and co-ordinates of C be (x, y).
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q27.1

Question 28.
Find the coordinates of the vertices of the triangle the middle points of whose sides are \(\left( 0,\frac { 1 }{ 2 } \right) ,\left( \frac { 1 }{ 2 } ,\frac { 1 }{ 2 } \right) and\left( \frac { 1 }{ 2 } ,0 \right) \)
Solution:
Let ABC be a ∆ in which \(D\left( 0,\frac { 1 }{ 2 } \right) ,E\left( \frac { 1 }{ 2 } ,\frac { 1 }{ 2 } \right) andF\left( \frac { 1 }{ 2 } ,0 \right) \),
the mid-points of sides AB, BC and CA respectively.
Let co-ordinates of A be (x₁, y₁), B (x₂, y₂), C (x₃, y₃)
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q28.1

Question 29.
Show by section formula that the points (3, – 2), (5, 2) and (8, 8) are collinear.
Solution:
Let the point (5, 2) divides the line joining the points (3, -2) and (8, 8)
in the ratio of m₁ : m₂
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q29.1

Question 30.
Find the value of p for which the points ( – 5, 1), (1, p) and (4, – 2) are collinear.
Solution:
Let points A (-5, 1), B (1, p) and C (4, -2)
are collinear and let point A (-5, 1) divides
BC in the ratio in m₁ : m₂
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q30.1

Question 31.
A (10, 5), B (6, – 3) and C (2, 1) are the vertices of triangle ABC. L is the mid point of AB, M is the mid-point of AC. Write down the co-ordinates of L and M. Show that LM = \(\\ \frac { 1 }{ 2 } \) BC.
Solution:
Co-ordinates of L will be
\(\left( \frac { 10+6 }{ 2 } ,\frac { 5-3 }{ 2 } \right) or\left( \frac { 16 }{ 2 } ,\frac { 2 }{ 2 } \right) or(8,1)\)
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q31.1

Question 32.
A (2, 5), B ( – 1, 2) and C (5, 8) are the vertices of a triangle ABC. P and.Q are points on AB and AC respectively such that AP : PB = AQ : QC = 1 : 2.
(i) Find the co-ordinates of P and Q.
(ii) Show that PQ = \(\\ \frac { 1 }{ 3 } \) BC.
Solution:
A (2, 5), B (-1, 2) and C (5, 8) are the vertices of a ∆ABC,
P and Q are points on AB
and AC respectively such that \(\frac { AP }{ PB } =\frac { AQ }{ QC } =\frac { 1 }{ 2 } \)
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q32.1

Question 33.
The mid-point of the line segment AB shown in the adjoining diagram is (4, – 3). Write down die co-ordinates of A and B.
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q33.1
Solution:
A lies on x-axis and B on the y-axis.
Let co-ordinates of A be (x, 0) and of B be (0, y)
P (4, -3) is the mid-point of AB

Question 34.
Find the co-ordinates of the centroid of a triangle whose vertices are A ( – 1, 3), B(1, – 1) and C (5, 1) (2006)
Solution:
Co-ordinates of the centroid of a triangle,
whose vertices are (x1, y1), (x2, y2) and
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q34.1

Question 35.
Two vertices of a triangle are (3, – 5) and ( – 7, 4). Find the third vertex given that the centroid is (2, – 1).
Solution:
Let the co-ordinates of third vertices be (x, y)
and other two vertices are (3, -5) and (-7, 4)
and centroid = (2, -1).
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q35.1

Question 36.
The vertices of a triangle are A ( – 5, 3), B (p – 1) and C (6, q). Find the values of p and q if the centroid of the triangle ABC is the point (1, – 1).
Solution:
The vertices of ∆ABC are A (-5, 3), B (p, -1), C (6, q)
and the centroid of ∆ABC is O (1, -1)
co-ordinates of the centroid of ∆ABC will be
ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 Q36.1

Hope given ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Category: ICSE Class 10

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 4 Linear Inequations (In one variable) Ex 4A

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.2

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 16 Constructions Ex 16.1

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10C

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 17 Mensuration Ex 17.4

ML Aggarwal Class 10 Solutions for ICSE Maths Chapter 11 Section Formula Ex 11