Linear Inequalities Class 11 MCQ Online Test With Answers Questions

Answer: (a) rational
Hint:
The sum of two rational numbers is a rational number.
Ex: Let two rational numbers are 1/2 and 1/3
Now, 1/2 + 1/3 = 5/6 which is a rational number.

Answer: (d) No solution
Hint:
Given, x² = -4
Since LHS ≥ 0 and RHS < 0
So, No solution is possible.

Answer: (b) x = -1
Hint:
Given, (x + 1)² + (x² + 3x + 2)² = 0
This is true when each term is equal to zero simultaneously,
So, (x + 1)² = 0 and (x² + 3x + 2)² = 0
⇒ x + 1 = 0 and x² + 3x + 2 = 0
⇒ x = -1, and x = -1, -2
Now, the common solution is x = -1
So, solution of the equation is x = -1

Answer: (a) (-8, ∞)
Hint:
Given,
(x + 3)/(x – 2) > 1/2
⇒ 2(x + 3) > x – 2
⇒ 2x + 6 > x – 2
⇒ 2x – x > -2 – 6
⇒ x > -8
⇒ x ∈ (-8, ∞)

Answer: (c) none of these
Hint:
Given inequalities x ≥ 6, y ≥ 2, 2x + y ≤ 10
Now take x = 6, y = 2 and 2x + y = 10
when x = 0, y = 10
when y = 0, x = 5
So, the points are A(6, 2), B(0, 10) and C(5, 0)

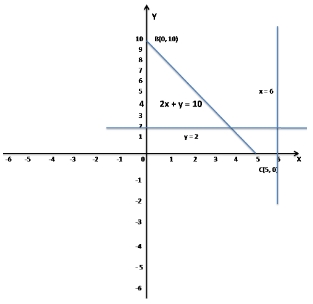
So, the region of the XOY-plane represented by the inequalities x ≥ 6, y ≥ 2, 2x + y ≤ 10 is not defined.

Answer: (d) 2 < x < 3 and x < 1
Hint:
Given, f(x) = (x – 1) × (x – 2) × (x – 3) has all factors with odd powers.
So, put them zero
i.e. x – 1 = 0, x – 2 = 0, x – 3 = 0
⇒ x = 1, 2, 3
Now, f(x) < 0 when 2 < x < 3 and x < 1

Answer: (b) (-1/2, 3/2)
Hint:
Given, -2 < 2x – 1 < 2
⇒ -2 + 1 < 2x < 2 + 1
⇒ -1 < 2x < 3
⇒ -1/2 < x < 3/2
⇒ x ∈(-1/2, 3/2)

Answer: (b) (-1, 3)
Hint:
Given, |x – 1| < 2
⇒ -2 < x – 1 < 2
⇒ -2 + 1 < x < 2 + 1
⇒ -1 < x < 3
⇒ x ∈ (-1, 3)

Answer: (d) x∈(−∞, −4)∪(6, ∞)
Hint:
Given |x−1| >5
Case 1:
(x – 1) > 5
⇒ x > 6
⇒ x ∈ (6,∞)
Case 2:
-(x – 1) > 5
⇒ -x + 1 > 5
⇒ -x > 4
⇒ x < -4
⇒ x ∈ (−∞, −4)
So the range of x is (−∞, −4)∪(6, ∞)

Answer: (b) (2, 4) ∪ (4, 6)
Hint:
Given, |2/(x – 4)| > 1
⇒ 2/|x – 4| > 1
⇒ 2 > |x – 4|
⇒ |x – 4| < 2
⇒ -2 < x – 4 < 2
⇒ -2 + 4 < x < 2 + 4
⇒ 2 < x < 6
⇒ x ∈ (2, 6) , where x ≠ 4
⇒ x ∈ (2, 4) ∪ (4, 6)

Answer: (d) (-∞, -2) ∪ [-1, 1] ∪ (2, ∞)
Hint:
Given, (|x| – 1)/(|x| – 2) ‎≥ 0
Let y = |x|
So, (y – 1)/(y – 2) ‎≥ 0
⇒ y ≤ 1 or y > 2
⇒ |x| ≤ 1 or |x| > 2
⇒ (-1 ≤ x ≤ 1) or (x < -2 or x > 2)
⇒ x ∈ [-1, 1] ∪ (-∞, -2) ∪ (2, ∞)
Hence the solution set is:
x ∈ (-∞, -2) ∪ [-1, 1] ∪ (2, ∞)

Answer: (d) -56/3 < x < 14/3
Hint:
Given inequality is :
-12 < (4 -3x)/(-5) < 2
⇒ -2 < (4-3x)/5 < 12
⇒ -2 × 5 < 4 – 3x < 12 × 5
⇒ -10 < 4 – 3x < 60
⇒ -10 – 4 < -3x < 60-4
⇒ -14 < -3x < 56
⇒ -56 < 3x < 14
⇒ -56/3 < x < 14/3

Answer: (d) No solution
Hint:
Given, x² = -4
Since LHS ≥ 0 and RHS < 0
So, No solution is possible.

Answer: (b) (-2, 8)
Hint:
Given, |x – 3| < 5
⇒ -5 < (x – 3) < 5
⇒ -5 + 3 < x < 5 + 3
⇒ -2 < x < 8
⇒ x ∈ (-2, 8)

Answer: (b) interior of a triangle including the points on the sides
Hint:
Given inequalities x ≥ 0, y ≥ 0, 3x + 4y ≤ 12
Now take x = 0, y = 0 and 3x + 4y = 12
when x = 0, y = 3
when y = 0, x = 4
So, the points are A(0, 0), B(0, 3) and C(4, 0)

So, the graph of the inequations x ≥ 0, y ≥ 0, 3x + 4y ≤ 12 is interior of a triangle including the points on the sides.

Answer: (d) (-5, 5)
Hint:
Given, |x| < 5
It means that x is the number which is at distance less than 5 from 0
Hence, -5 < x < 5
⇒ x ∈ (-5, 5)

Answer: (b) (-∞, 1] ∪ (2, 3)
Hint:
Given, f(x) = {(x – 1)×(2 – x)}/(x – 3) ≥ 0
or f(x) = -{(x – 1)×(2 – x)}/(x – 3)
which gives x – 3 ≠ 0
⇒ x ≠ 3

Using number line rule as shown in the figure,
which gives f(x) ≥ 0 when x ≤ 1 or 2 ≤ x < 3
i.e. x ∈ (-∞, 1] ∪ (2, 3)

Answer: (c) -2, 2
Hint:
Given, x² = 4
⇒ x² – 4 = 0
⇒ (x – 2)×(x + 2) = 0
⇒ x = -2, 2

Answer: (a) 27 < x < 2
Hint:
Given inequality is:
15 < 3(x-2)/5 < 0
⇒ 15 × 5 < 3(x-2) < 0 × 5
⇒ 75 < 3(x-2) < 0
⇒ 75/3 < x-2 < 0
⇒ 25 < x-2 < 0
⇒ 25 +2 < x <0+2
⇒ 27 < x < 2

Answer: (c) [-2, 0] ∪ [2, 4]
Hint:
Given, 1 ≤ |x – 1| ≤ 3
⇒ -3 ≤ (x – 1) ≤ -1 or 1 ≤ (x – 1) ≤ 3
i.e. the distance covered is between 1 unit to 3 units
⇒ -2 ≤ x ≤ 0 or 2 ≤ x ≤ 4
Hence, the solution set of the given inequality is
x ∈ [-2, 0] ∪ [2, 4]