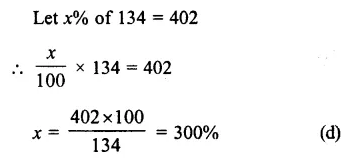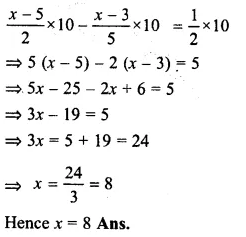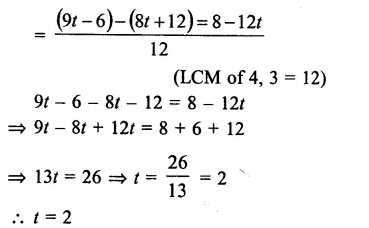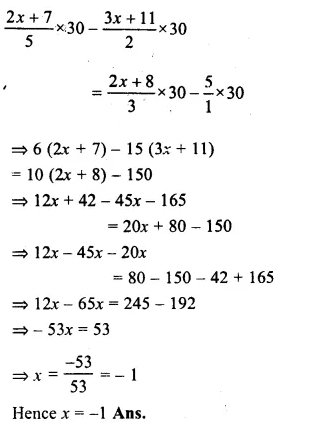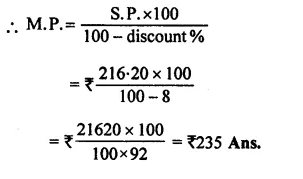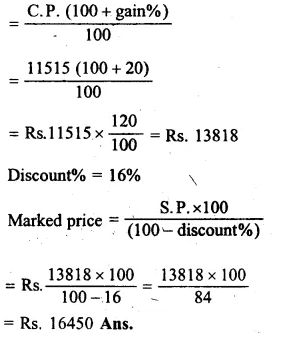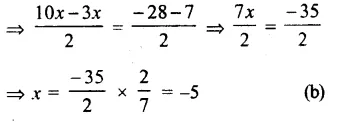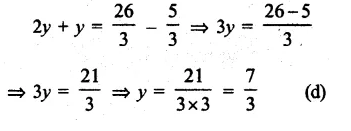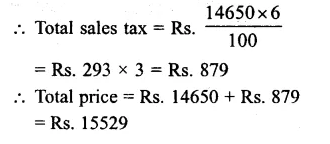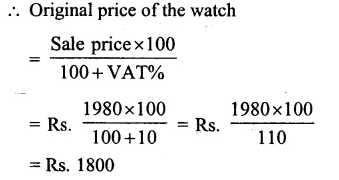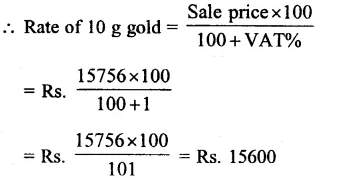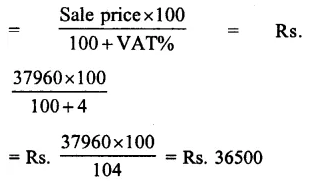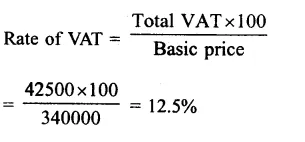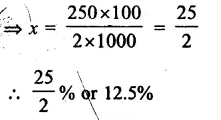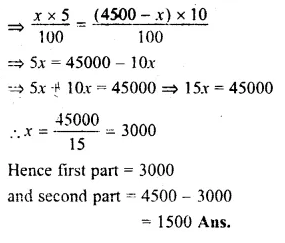Category: CBSE Class 8

RS Aggarwal Class 8 Solutions Chapter 10 Profit and Loss Ex 10D

These Solutions are part of RS Aggarwal Solutions Class 8. Here we have given RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10D.

Other Exercises

• RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10A
• RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10B
• RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10C
• RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10D

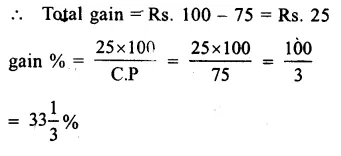
Question 1.
Solution:
Answer = (c)
C.P. of toy Rs. = 75
S.P. = Rs. 100

Question 2.
Solution:
Answer = (b)
C.P. of bat = Rs. 120
S.P. = Rs. 105
Loss = Rs. 120 – Rs. 105 = Rs. 15

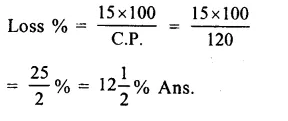
Question 3.
Solution:
Answer = (b)
S.P. of book = Rs. 100
gain = Rs. 20
C.P. = 100 – 20 = Rs. 80

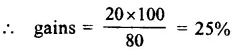
Question 4.
Solution:
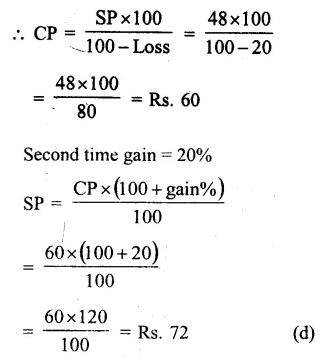
SP of an article = Rs. 48
Loss = 20%

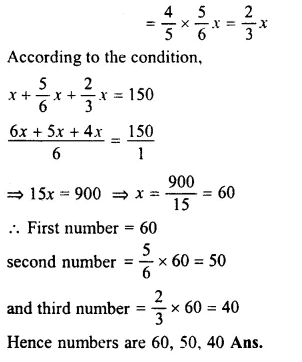
Question 5.
Solution:
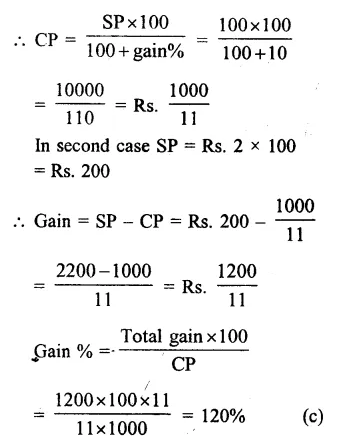
First time gain = 10%
Let SP – Rs. 100

Question 6.
Solution:
Let no. of bananas bought = 6
Now C.P. of bananas at the sale of 3

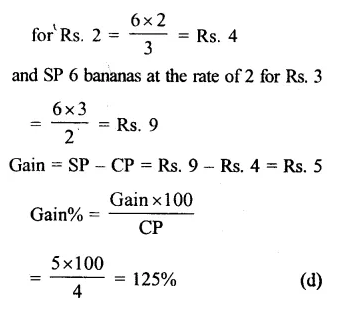
Question 7.
Solution:
SP of 10 pens = CP of 12 pens
= Rs. 100 (suppose)

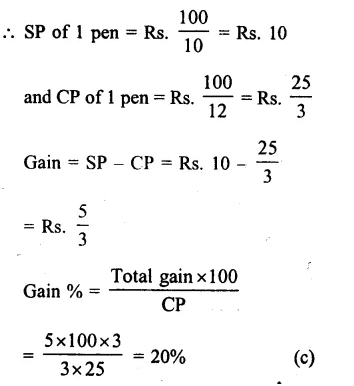
Question 8.
Solution:
Gain on 100 pencils = SP of 20 pencils
SP of 100 pencils gains = CP of 100 pencils
=> SP of 100 pencils – SP of 20 pencils = CP of 100 pencils
=> SP of 80 pencils – CP of 100 pencils = Rs 100 (suppose)

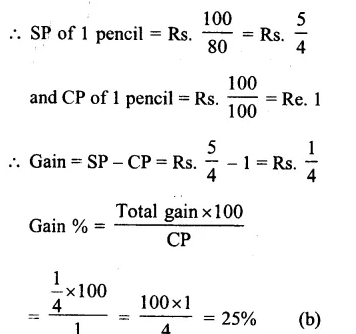
Question 9.
Solution:
SP of 5 toffees = Re. 1
SP of 2 toffees = Re. 1
Now CP of 1 toffee = Rs. \(\\ \frac { 1 }{ 5 } \)
and SP of 1 toffee = Rs. \(\\ \frac { 1 }{ 2 } \)

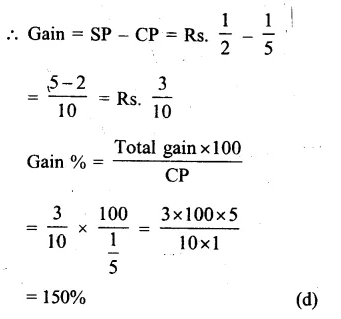
Question 10.
Solution:
CP of 5 oranges = Rs. 10
SP of 6 oranges = Rs. 15

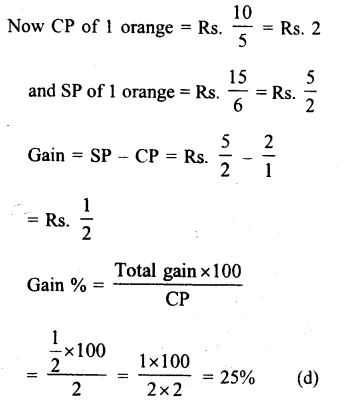
Question 11.
Solution:
SP of a radio = Rs. 950
Loss = 5%

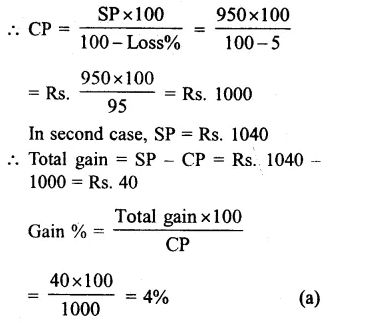
Question 12.
Solution:
Let CP of an article = Rs. 100
SP = \(\\ \frac { 6 }{ 5 } \) of CP = \(\\ \frac { 6 }{ 5 } \) x 100 = Rs. 120

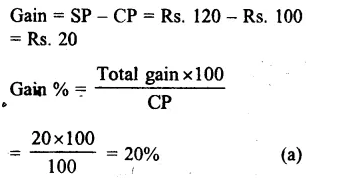
Question 13.
Solution:
SP of a chair = Rs. 720
Loss = 25%

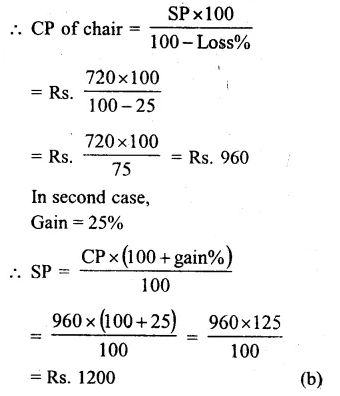
Question 14.
Solution:
Ratio in CP and SP = 20 : 21
Let CP = Rs. 20
and SP = Rs. 21
Gain = SP – CP = Rs. 21 – 20 = Re. 1

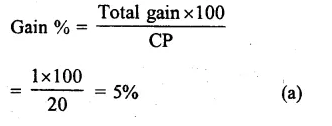
Question 15.
Solution:
SP of first chair = Rs. 500
Gain = 20%

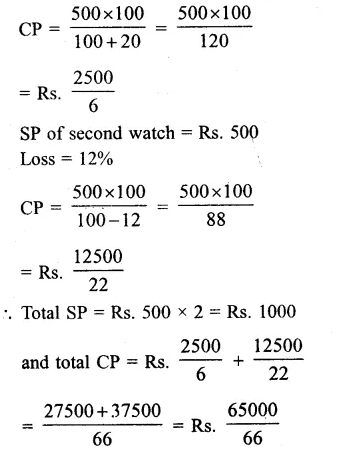
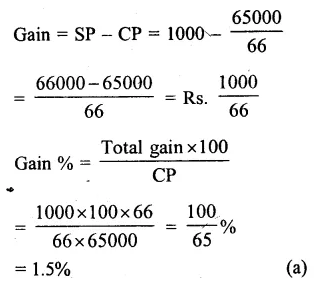
Question 16.
Solution:
Gain % SP of Rs. 625 = Loss on SP of Rs. 435
CP of an article = x

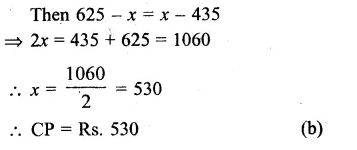
Question 17.
Solution:
CP of an article = Rs. 150
Overhead expenses = 10% of CP

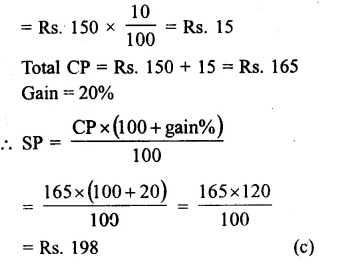
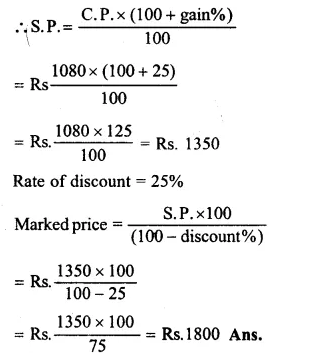
Question 18.
Solution:
In first case, gain = 5%
and in second case, loss = 5%
and difference = Rs. 5 more
But difference in % = 5 + 5 = 10%

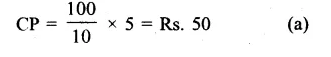
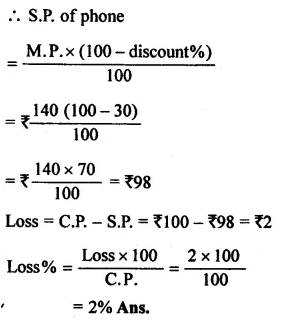
Question 19.
Solution:
Let CP of an article = Rs. 100
List price = Rs. 100 + 20% of Rs. 100
= Rs. 100 + 20 = Rs. 120
Discount = 10%

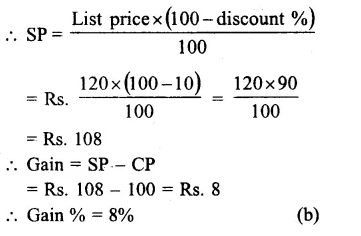
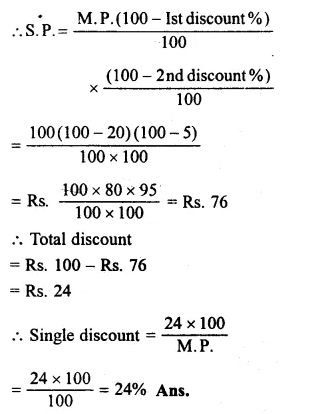
Question 20.
Solution:
Let CP of an article = Rs. 100
Then Marked price
= Rs. 100 + 10% of 100
= Rs. 100 + 10 = Rs. 110
Discount = 10%

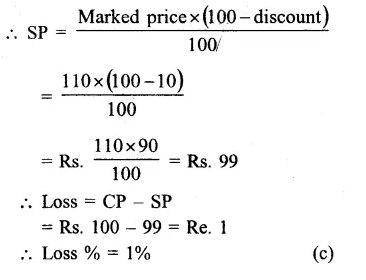
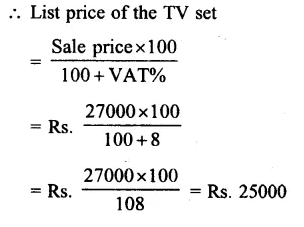
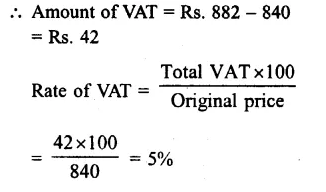
Question 21.
Solution:
Price of watch including VAT = Rs. 825
VAT% = 10%

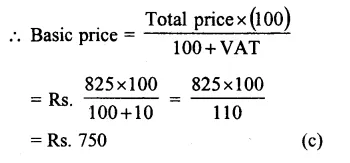
Hope given RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 8 Solutions Chapter 7 Factorisation Ex 7C

These Solutions are part of RS Aggarwal Solutions Class 8. Here we have given RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7C.

Other Exercises

• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7A
• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7B
• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7C
• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7D
• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7E

Question 1.
Solution:
x² + 8x + 16
= (x)² + 2 × x × 4 + (4)²
= (x + 4)²

Question 2.
Solution:
x² + 14x + 49
= (x)² + 2 × x × 7 + (7)²
= (x + 7)² Ans.

Question 3.
Solution:
1 + 2x + x²
= (1)² + 2 × 1 × x + (x)²
= (1 + x)² Ans.

Question 4.
Solution:
9 + 6z + z²
= (3)² + 2 x 3 x z + (z)²
= (3 + z)² Ans.

Question 5.
Solution:
x² + 6ax + 9a²
= (x)² + 2 × x × 3a + (3a)²
= (x + 3a)² Ans.

Question 6.
Solution:
4y² + 20y + 25
= (2y)² + 2 x 2y x 5 + (5)²
= (2y² + 5)²
{ ∵ a² + 2ab + b² = (a + b)²}

Question 7.
Solution:
36a² + 36a + 9
= 9 [4a² + 4a + 1]
= 9 [(2a)² + 2 x 2a x 1 + (1)²]
= 9 [2a + 1]²

Question 8.
Solution:
9m² + 24m + 16
= (3m)² + 2 x 3m x 4 + (4)²
= (3m + 4)²
{ ∵ a² + 2ab + b² = (a + b)²}

Question 9.
Solution:
z² + z + \(\\ \frac { 1 }{ 4 } \)
= (z)² + 2 x z x \(\\ \frac { 1 }{ 2 } \) + \({ \left( \frac { 1 }{ 2 } \right) }^{ 2 }\)
= \({ \left( z+\frac { 1 }{ 2 } \right) }^{ 2 }\)

Question 10.
Solution:
49a² + 84ab + 36b²
= (7a)² + 2 x 7a x 6b + (6b)²
{ ∵ a² + 2ab + b² = (a + b)²}
= (7a + 6b)²

Question 11.
Solution:
p² – 10p + 25
= (p)² – 2 x p x 5 + (5)²
= (p – 5)²
{ ∵ a² – 2ab + b² = (a – b)²}

Question 12.
Solution:
121a² – 88ab + 16b²
= (11a)² – 2 x 11a x 4b + 4(b)²
= (11a – 4b)²

Question 13.
Solution:
1 – 6x + 9x²
= (1)² – 2 x 1 x 3x + (3x)²
= (1 – 3x)²
{ ∵ a² – 2ab + b² = (a – b)²}

Question 14.
Solution:
9y² – 12y + 4
= (3y)² – 2 x 3y x 2 + (2)²
{ ∵ a² – 2ab + b² = (a – b)²}
= (3y – 2)²

Question 15.
Solution:
16x² – 24x + 9
= (4x)² – 2 x 4x x 3 + (3)²
= (4x – 3)² Ans.

Question 16.
Solution:
m² – 4mn + 4n²
= (m)² -2 x m x 2n + (2n)²
= (m – 2n)² Ans.

Question 17.
Solution:
a²b² – 6abc + 9c²
= (ab)² – 2 x ab x 3c + (3c)²
= (ab – 3c)² Ans.

Question 18.
Solution:
m⁴ + 2m²n² + n⁴
= (m²)² + 2m²n² + (n²)²
= (m² + n²)²
{ ∵ a² + 2ab + b² = (a + b)²}

Question 19.
Solution:
(l + m)² – 4lm
= l² + m² + 2lm – 4lm
= l² + m² – 2lm
= l² – 2lm + m²
= (l – m)²
{ ∵ a² – 2ab + b² = (a – b)}

 

Hope given RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 8 Solutions Chapter 9 Percentage Ex 9B

These Solutions are part of RS Aggarwal Solutions Class 8. Here we have given RS Aggarwal Solutions Class 8 Chapter 9 Percentage Ex 9B.

Other Exercises

• RS Aggarwal Solutions Class 8 Chapter 9 Percentage Ex 9A
• RS Aggarwal Solutions Class 8 Chapter 9 Percentage Ex 9B

Tick the correct answer in each of the following:

Question 1.
Solution:
\(\\ \frac { 3 }{ 5 } \)
= \(\\ \frac { 3X20 }{ 5X20 } \)
= \(\\ \frac { 60 }{ 100 } \)
= 60% (d)

Question 2.
Solution:
0.8%
= \(\\ \frac { 0.8 }{ 100 } \)
= \(\\ \frac { 8 }{ 10X100 } \)
= \(\\ \frac { 8 }{ 1000 } \)
= 0.008 (b)

Question 3.
Solution:
6 : 5
= \(\\ \frac { 6 }{ 5 } \)
= \(\\ \frac { 6X20 }{ 5X20 } \)
= \(\\ \frac { 120 }{ 100 } \)
= 120% (c)

Question 4.
Solution:
5% of a number is 9
Number = \(\\ \frac { 9X100 }{ 5 } \)
= 180 (d)

Question 5.
Solution:
Let x% of 90 = 120
=> \(\\ \frac { x }{ 100 } \) x 120 = 90
=> x% = \(\\ \frac { 120X100 }{ 90 } \)
= \(133\frac { 1 }{ 3 } %\) (c)

Question 6.
Solution:
Let x% 10 kg = 250 g
\(\\ \frac { x }{ 100 } \) x 10 kg
= \(\\ \frac { 250 }{ 1000 } \) kg
x = \(\\ \frac { 250X100 }{ 1000X10 } \)
= \(\\ \frac { 25 }{ 10 } \)%
= 2.5% (d)

Question 7.
Solution:
40% of x = 240
=> x = \(\\ \frac { 240 }{ 40 } \) x 100
= 600 (b)

Question 8.
Solution:
?% of 400 = 60
=> x% of 400 = 60
\(\\ \frac { x }{ 100 } \) x 400 = 60
x = \(\\ \frac { 60X100 }{ 400 } \)
= 15 (c)

Question 9.
Solution:
(180% of ?)÷2 = 504
\(\left( \frac { 180 }{ 100 } \times x \right) \div 2\) = 504
\(\frac { 180 }{ 100 } x\) = 504 x 2
\(x=\frac { 504\times 2\times 100 }{ 180 }\)
= 560 (d)

Question 10.
Solution:
20% of Rs. 800
= \(\\ \frac { 20 }{ 100 } \) x 800
= Rs 160 (a)

Question 11.
Solution:
Nitin gets = 98 marks
and it is 56% of total marks
Total-marks = \(\\ \frac { 98X100 }{ 56 } \)
= 175 (c)

Question 12.
Solution:
Let a number be = 1000
Then increase = 10%
Increased number = \(\\ \frac { 100X110 }{ 100 } \) = 110
Now decrease = 1%
Decreased number = \(\\ \frac { 110X90 }{ 100 } \) = 99
Difference = 100 – 99 = 1
% decrease = 1% (b)

Question 13.
Solution:
4 hours 30 min = \(4\frac { 1 }{ 2 } %\)
= \(\\ \frac { 9 }{ 2 } \) hours
% of a day = \(\\ \frac { 9 }{ 2 } \) x \(\\ \frac { 100 }{ 24 } \)%
= \(\\ \frac { 75 }{ 4 } \)%
= \(18\frac { 3 }{ 4 } %\) (a)

Question 14.
Solution:
Let total number of examinees = 100
Passed = 65
Failed = 100 – 65 = 35
Now 35% of total examinees = 420
Total examinees = \(\\ \frac { 420X100 }{ 35 } \)
= 1200 (c)

Question 15.
Solution:
Let number = x
Then x = \(\\ \frac { xX20 }{ 100 } \) = 40
=> 100x – 200x = 4000
80x = 4000
=> x = \(\\ \frac { 4000 }{ 80 } \) = 50
Number = 50 (a)

Question 16.
Solution:
Rate of decrease = \(27\frac { 1 }{ 2 } %\)
Let number = x

Question 17.
Solution:
Let x% of 20 = 0.05
\(\\ \frac { x }{ 100 } \) x 20 = 0.05
x = \(\\ \frac { 0.05X100 }{ 20 } \)
= 0.25% (c)

Question 18.
Solution:
\(\\ \frac { 1 }{ 3 } \) = 1206 = 402

Question 19.
Solution:
x% of y is y% = \(\\ \frac { xy }{ 100 } \)
\(\\ \frac { y }{ 100 } \) × x
= y% of x (a)

Question 20.
Solution:
Let x% of \(\\ \frac { 2 }{ 7 } \) = \(\\ \frac { 1 }{ 35 } \)
=>\(\\ \frac { x }{ 100 } \) x \(\\ \frac { 2 }{ 7 } \) = \(\\ \frac { 1 }{ 35 } \)
x = \(\\ \frac { 1X100X7 }{ 35X2 } \)
= 10% (b)

Hope given RS Aggarwal Solutions Class 8 Chapter 9 Percentage Ex 9B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 8 Solutions Chapter 8 Linear Equations Ex 8A

These Solutions are part of RS Aggarwal Solutions Class 8. Here we have given RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8A.

Other Exercises

• RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8A
• RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8B
• RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8C

Solve :

Question 1.
Solution:
8x + 3 = 27 + 2x
=> 8x – 2x
=> 27 – 3
=> 6x = 24
=> x = \(\\ \frac { 24 }{ 6 } \) = 4
x = 4

Question 2.
Solution:
5x + 7 = 2x – 8
=> 5x – 2x = – 8 – 7
=> 3x = – 15
=> x = \(\\ \frac { -15 }{ 3 } \) = – 5
x = – 5

Question 3.
Solution:
2z – 1 = 14 – z
=> 2z + z = 14 + 1
=> 3z = 15
=> z = \(\\ \frac { 15 }{ 3 } \) = 5
z = 5

You can also Download NCERT Solutions for Class 8 Maths to help you to revise complete Syllabus and score more marks in your examinations.

Question 4.
Solution:
9x + 5 = 4(x – 2) +8
=> 9x + 5 = 4x – 8 + 8
=> 9x – 4x = – 8 + 8 – 5
=> 5x = – 5
=> x = \(\\ \frac { -5 }{ 5 } \) = – 1
x = – 1

Question 5.
Solution:
\(\\ \frac { 7y }{ 5 } \) = y – 4
Multiplying both sides by 5,
\(\\ \frac { 7y }{ 5 } \) x 5 = 5(y – 4)
=> 5 (y-4)
=> 7y = 5y – 20
=> 7y – 5y = – 20
=> 2y = – 20
=> y = \(\\ \frac { -20 }{ 2 } \) = – 10
Hence y = – 10 Ans.

Question 6.
Solution:
3x + \(\\ \frac { 2 }{ 3 } \) = 2x + 1
=> 3x – 2x = 1 – \(\\ \frac { 2 }{ 3 } \)
=> x = \(\\ \frac { 3-2 }{ 3 } \) = \(\\ \frac { 1 }{ 3 } \)
Hence x = \(\\ \frac { 1 }{ 3 } \) Ans.

Question 7.
Solution:
15(y – 4) – 2(y – 9) + 5(y + 6) = 0
15y – 60 – 2y + 18 + 5y + 30 = 0
=> 15y – 2y + 5y = 60 – 18 – 30
=> 18y = 12
=> y = \(\\ \frac { 12 }{ 18 } \) = \(\\ \frac { 2 }{ 3 } \)
=> y = \(\\ \frac { 2 }{ 3 } \)

Question 8.
Solution:
3(5x – 7) – 2(9x – 11) = 4(8x – 13) – 17
=> 15x – 21 – 18x + 22 = 32x – 52 – 17
=> 15x – 18x – 32x = – 52 – 17 + 21 – 22
=> 15x – 50x = – 70
=> – 35x = – 70
=> x = \(\\ \frac { -70 }{ -35 } \) = 2
x = 2

Question 9.
Solution:
\(\\ \frac { x-5 }{ 2 } \) – \(\\ \frac { x-3 }{ 5 } \) = \(\\ \frac { 1 }{ 2 } \)
Multiplying each term by 10, the L.C.M. of 2 and 5

Question 10.
Solution:
\(\\ \frac { 3t-2 }{ 4 } \) – \(\\ \frac { 2t+3 }{ 3 } \) = \(\\ \frac { 2 }{ 3 } \) – t

Question 11.
Solution:
\(\frac { 2x+7 }{ 5 } +\frac { 3x+11 }{ 2 } =\frac { 2x+8 }{ 3 } -5\)
Multiplying by 30, the L.C.M. of 5, 2 and 3.

Question 12.
Solution:
\(\frac { 5x-4 }{ 6 } =4x+1-\frac { 3x+10 }{ 2 } \)
Multiplying by 6, the L.C.M. of 6 and 2

Question 13.
Solution:
\(5x-\frac { 1 }{ 3 } \left( x+1 \right) =6\left( x+\frac { 1 }{ 30 } \right) \)
=> 5x – \(\\ \frac { x+1 }{ 3 } \) = 6x + \(\\ \frac { 1 }{ 5 } \)
Multiplying by 15, the L.C.M. of 3 and 5

Question 14.
Solution:
\(4-\frac { 2\left( z-4 \right) }{ 3 } =\frac { 1 }{ 2 } \left( 2z+5 \right)\)

Question 15.
Solution:
\(\frac { 3\left( y-5 \right) }{ 4 } -4y=3-\frac { \left( y-3 \right) }{ 2 } \)
Multiplying by 4, the L.C.M. of 4 and 2

Question 16.
Solution:
\(\\ \frac { 8x-3 }{ 3x } \) = \(\\ \frac { 2 }{ 1 } \)
By cross multiplication,
8x – 3 = 6x
=> 8x – 6x = 3
=> 2x = 3
=> x = \(\\ \frac { 3 }{ 2 } \)
x = \(\\ \frac { 3 }{ 2 } \)

Question 17.
Solution:
\(\\ \frac { 9x }{ 7-6x } \) = \(\\ \frac { 15 }{ 1 } \)
By cross multiplication,
9x = 105 – 90x
=> 9x + 90x = 105
=> 99x = 105
=> x = \(\\ \frac { 105 }{ 99 } \) = \(\\ \frac { 35 }{ 33 } \)
x = \(\\ \frac { 35 }{ 33 } \)

Question 18.
Solution:
\(\\ \frac { 3x }{ 5x+2 } \) = \(\\ \frac { -4 }{ 1 } \)
By cross multiplication,
3x × 1 = – 4×(5x + 2)
=> 3x = – 20x – 8
=> 3x + 20x = – 8
=> 23x = – 8
=> x = \(\\ \frac { -8 }{ 23 } \)
Hence x = \(\\ \frac { -8 }{ 23 } \)

Question 19.
Solution:
\(\\ \frac { 6y-5 }{ 2y } \) = \(\\ \frac { 7 }{ 9 } \)
By cross multiplication,
9(6y – 5) = 7 × 2y
=> 54y – 45 = 14y
=> 54y – 14y = 45
=> 40y = 45
=> y = \(\\ \frac { 45 }{ 40 } \) = \(\\ \frac { 9 }{ 8 } \)
Hence y = \(\\ \frac { 9 }{ 8 } \) Ans.

Question 20.
Solution:
\(\\ \frac { 2-9z }{ 17-4z } \) = \(\\ \frac { 4 }{ 5 } \)
By cross multiplication,
5 (2 – 9z) = 4(17 – 4z)
=> 10 – 45z = 68 – 16z
=> – 45z + 16z = 68 – 10
=> – 29 = 58
=> z = \(\\ \frac { 58 }{ -29 } \) = – 2
Hence z = – 2 Ans.

Question 21.
Solution:
\(\\ \frac { 4x+7}{ 9-3x } \) = \(\\ \frac { 1 }{ 4 } \)
By cross multiplication,
4(4x + 7) = 1 (9 – 3x)
=> 16x + 28 = 9 – 3x
=> 16x + 3x = 9 -28
=> 19x = – 19
=> x = \(\\ \frac { -19 }{ 19 } \) = – 1
Hence x = – 1 Ans.

Question 22.
Solution:
\(\\ \frac { 7y+4}{ y+2 } \) = \(\\ \frac { -4 }{ 3 } \)
By cross multiplication,
3 (7y + 4) = – 4 (y + 2)
=> 21y + 12 = – 4y – 8
=> 21y + 4y = – 8 – 12
=> 25y = – 20
=> y = \(\\ \frac { -20 }{ 25 } \) = \(\\ \frac { -4 }{ 5 } \)
y = \(\\ \frac { -4 }{ 5 } \)

Question 23.
Solution:
\(\\ \frac { 15(2-y)-5(y+6) }{ 1-3y } \) = \(\\ \frac { 10 }{ 1 } \)
By cross multiplication,
15 (2 – y) – 5(y + 6) = 10 (1 – 3y)
=> 30 – 15y – 5y – 30 = 10 – 30y
=> – 15y – 5y + 30y = 10 – 30 + 30
=> 30y – 20y = 10
=> 10y = 10
y = \(\\ \frac { 10 }{ 10 } \) = 1
Hence y = 1 Ans.

Question 24.
Solution:
\(\\ \frac { 2x-(7-5x) }{ 9x-(3x+4x) } \) = \(\\ \frac { 7 }{ 6 } \)

Question 25.
Solution:
\(m-\frac { \left( m-1 \right) }{ 2 } =1-\frac { \left( m-2 \right) }{ 3 } \)

Question 26.
Solution:
\(\\ \frac { 3x+5 }{ 4x+2 } \) = \(\\ \frac { 3x+4 }{ 4x+7 } \)
By cross multiplication,
(3x + 5)(4x + 7) = (3x + 4)(4x + 2)
=> 12x² + 21x + 20x + 35

Question 27.
Solution:
\(\\ \frac { 9x-7 }{ 3x+5 } \) = \(\\ \frac { 3x-4 }{ x+6 } \)

Question 28.
Solution:
\(\\ \frac { 2-7x }{ 1-5x } \) = \(\\ \frac { 3+7x }{ 4+5x } \)

 

Hope given RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 8 Solutions Chapter 10 Profit and Loss Ex 10B

These Solutions are part of RS Aggarwal Solutions Class 8. Here we have given RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10B.

Other Exercises

• RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10A
• RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10B
• RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10C
• RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10D

Question 1.
Solution:
Marked price of cooler = Rs 4650
Rate of discount = 18%
Selling price

Question 2.
Solution:
Marked price = Rs 960
Selling price = Rs 816
Total Discount = M.P. – S.P.
= Rs 960 – Rs 816
= Rs 144

You can also Download NCERT Solutions for Class 8 Maths to help you to revise complete Syllabus and score more marks in your examinations.

Question 3.
Solution:
S.P. of shirt = Rs 1092
Discount = Rs 208
M.P. of shirt = S.P. + discount

Question 4.
Solution:
S.P. of toy = Rs 216.20
Discount = 8%

Question 5.
Solution:
S.P. of tea set = Rs 528
Rate of discount = 12%

Question 6.
Solution:
Let C.P. of goods = Rs 100
Marked price = Rs 100 + 35
= Rs 135
Rate of discount = 20%

Question 7.
Solution:
Let C.P. of phone = Rs 100
.’. Marked price = Rs 100 + 40
= Rs 140
Rate of discount = 30%

Question 8.
Solution:
C.P. of fan = Rs. 1080
Gain = 25%

Question 9.
Solution:
C.P of refrigerator = Rs. 11515
and gain % = 20%
S.P. of refrigerator

Question 10.
Solution:
C.P. of ring = Rs. 1190
Gain = 20%

Question 11.
Solution:
Let Marked price = Rs. 100
Discount = 10%

Question 12.
Solution:
Let C.P. = Rs. 100
Gain = 8%

Question 13.
Solution:
Marked price of TV = Rs. 18500
Series of two successive discounts = 20% and 5%

Question 14.
Solution:
Let M.P. = Rs. 100
First discount = 20%
and second discount = 5%

 

Hope given RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 8 Solutions Chapter 7 Factorisation Ex 7D

These Solutions are part of RS Aggarwal Solutions Class 8. Here we have given RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7D.

Other Exercises

• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7A
• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7B
• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7C
• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7D
• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7E

Question 1.
Solution:
x² + 5x + 6
= x² + 2x + 3x + 6
{6 = 2 x 3, 5 = 2 + 3}
= x (x + 2) + 3 (x + 2)
= (x + 2) (x + 3) Ans.

Question 2.
Solution:
y² + 10y + 24
= y² + 6y + 4y + 24
{24 = 6 x 4, 10 = 6 + 4}
= y (y + 6) + 4 (y + 6)
= (y + 6) (y + 4)

Question 3.
Solution:
z² + 12x + 27
{27 = 9 x 3, 12 = 9 + 3}
= z² + 9z + 3z + 27
= z (z + 9) + 3 (z + 9)
= (z + 9) (z + 3)

Question 4.
Solution:
p² + 6p + 8
= p² + 4p + 2p + 8
{ 8 = 4 x 2, 6 = 4 + 2}
= p (p + 4) + 2 (p + 4)
= (p + 4) (p + 2)

Question 5.
Solution:
x² + 15x + 56
= x² + 8x + 7x + 56
{56 = 8 x 7, 15 = 8 x 7}
= x(x + 8) + 7(x + 8)
= (x + 8) (x + 7) Ans.

Question 6.
Solution:
y² + 19y + 60
= y² + 15y + 4y + 60
{60 = 15 x 4, 19 = 15 + 4}
= y (y + 15) + 4 (y + 15)
= (y + 15) (y + 4)

Question 7.
Solution:
x² + 13x + 40
= x² + 5x + 8x + 40
{40 = 5 x 8, 13 = 5 + 8}
= x(x + 5) + 8(x + 5)
= (x + 5) (x + 8) Ans.

Question 8.
Solution:
q² – 10q + 21
= q² – 7q – 3q + 21
{21 = ( – 7)x( – 3), – 10 = – 7 – 3}
= q {q – 7) – 3 (q – 7)
= (q – 7)(q – 3)

Question 9.
Solution:
p² + 6p – 16
= p² + 8p – 2p – 16
{ – 16 = 8x( – 2),6 = 8 – 2}
= p (p + 8) – 2 (p + 8)
= (p + 8) (p – 2)

Question 10.
Solution:
x² – 10x + 24
= x² – 6x – 4x + 24
{24 = ( – 6) x ( – 4), – 10 = – 6 – 4 }
= x(x – 6) – 4(x – 6)
= (x – 6) (x – 4) Ans.

Question 11.
Solution:
x² – 23x + 42
= x² – 2x – 21x + 42
{42 = ( – 2) x ( – 21), – 23 = – 2 – 21}
= x(x – 2) – 21(x – 2)
= (x – 2) (x – 21) Ans.

Question 12.
Solution:
x² – 17x + 16
= x² – x – 16x + 16
{ 16 = ( – 1) x ( – 16), 17 = – 1 – 16}
= x (x – 1) – 16(x – 1)
= (x – 1) (x – 16) Ans.

Question 13.
Solution:
y² – 21y + 90
= y² – 15y – 6y + 90
{90 = ( – 15)x ( – 6), – 21 = – 15 – 6}
= y(y – 15) – 6 (y – 15)
= (y – 15) (y – 6)

Question 14.
Solution:
x² – 22x + 117
= x² – 13x – 9x + 117
{117 = ( – 13) x ( – 9), – 22 = – 13 – 9}
= x(x – 13) – 9(x – 13)
= (x – 9) (x – 13) Ans.

Question 15.
Solution:
x² – 9x + 20
= x² – 5x – 4x + 20
{ 20 = ( – 5) x ( – 4), – 9 = – 5 – 4}
= x(x – 5) – 4(x – 5)
= (x – 5) (x – 4) Ans.

Question 16.
Solution:
x² + x – 132
= x² + 12x – 11x – 132
{ – 132 = 12 x ( – 11), 1 = 12 – 11}
= x(x + 12) – 11(x + 12)
= (x + 12) (x – 11) Ans.

Question 17.
Solution:
x² + 5x – 104
= x² + 13x – 8x – 104
{ – 104 = 13 x ( – 8), 5 = 13 – 8}
= x(x + 13) – 8(x + 13)
= (x + 13) (x – 8) Ans.

Question 18.
Solution:
y² + 7y – 144
= y² + 16y – 9y – 144
{144 = – 16 x 9, 7 = 16 – 9}
= y(y +16) – 9(y + 16)
= (y + 16) (y – 9) Ans.

Question 19.
Solution:
z² + 19z – 150
= z² + 25z – 6z – 150
{ – 150 = 25 x ( – 6), 19 = 25 – 6}
= z(z + 25) – 6(z + 25)
= (z + 25) (z – 6) Ans.

Question 20.
Solution:
y² + y – 72
= y² + 9y – 8y – 72
{ – 72 = 9x( – 8), 1 = 9 – 8}
= y(y + 9) – 8(y + 9)
= (y + 9) (y – 8) Ans

Question 21.
Solution:
a² + 6a – 91
= a² + 13a – 7a – 91
{ – 91 = 13x( – 7), 6 = 13 – 7}
= a (a + 13) – 7 (a + 13)
= (a + 13) (a – 7)

Question 22.
Solution:
p² – 4p – 11
= p² – 11p + 7p – 77
{ – 77 = – 11 x 7, – 4 = – 11 + 7}
= p(p – 11) + 7 (p – 11)
= (p – 11)(p + 7)

Question 23.
Solution:
x² – 7x – 30
= x² – 10x + 3x – 30
{ – 30 = – 10 x 3, – 7 = – 10 + 3}
= x(x – 10) + 3(x – 10)
= (x – 10) (x + 3) Ans.

Question 24.
Solution:
x² – 14 x + 3 x – 42
{ – 11 = – 14 + 3], – 42 = – 14 x 3}
= x (x – 14) + 3 (x – 14)
= (x – 14) (x + 3) Ans.

Question 25.
Solution:
x² – 5x – 24
= x² – 8x + 3x – 24
{ – 24 = – 8 x 3, – 5 = – 8 + 3}
= x(x – 8) + 3(x – 8)
= (x – 8) (x + 3) Ans.

Question 26.
Solution:
y² – 6y – 135
= y² – 15y + 9y – 135
{ – 135 = – 15 x 9, – 6 = – 15 + 9}
= y (y – 15) + 9 (y – 15)
= (y – 15) (y + 9)

Question 27.
Solution:
z² – 12z – 45
= z² – 15z + 3z – 45
= z (z – 15) + 3 (z – 15)
{ – 45 = – 15 x 3, – 12 = – 15 + 3}
= (z – 15)(z + 3)

Question 28.
Solution:
x² – 4x – 12
= x² – 6x + 2x – 12
{ – 12 = – 6 x 2, – 4 = – 6 + 2}
= x (x – 6) + 2 (x – 6)
= (x – 6) (x + 2)

Question 29.
Solution:
3x² + 10x + 8
= 3x² + 6x + 4x + 8
{ 3 x 8 = 24, 24 = 6 x 4,10 = 6 + 4}
= 3x(x + 2) + 4(x + 2)
= (x + 2) (3x + 4) Ans.

Question 30.
Solution:
3y² + 14y + 8
= 3y² + 12y + 2y + 8
{3 x 8 = 24, 24 = 12 x 2, 14 = 12 + 2}
= 3y (y + 4) + 2 (y + 4)
= (y + 4) (3y + 2)

Question 31.
Solution:
3z² – 10z + 8
= 3z² – 6z – 4z + 8
{ 3 x 8 = 24, 24 = ( – 6)x( – 4), – 10 = – 6 – 4}
= 3z (z – 2) – 4 (z – 2)
= (z – 2) (3z – 4)

Question 32.
Solution:
2x² + x – 45
= 2x² + 10x – 9x – 45
{2 x ( – 45) = – 90,- 90 = (10)x( – 9), 1 = 10 – 9}
= 2x (x – 5) + 9 (x – 5)
= (x – 5) (2x + 9)

Question 33.
Solution:
6p² + 11p – 10
= 6x² + 15x – 4x – 10
{6x ( – 10) = 60, – 60 = 15 x ( – 4),11 = 15 – 4}
= 3x (2x + 5) – 2 (2x + 5)
= (3x – 2) (2x + 5) Ans.

Question 34.
Solution:
2x² – 20x + 3x – 30
{2 x ( – 30) = – 60, – 17 = – 20 + 3, – 60 = – 20 x 3}
= 2x (x – 10) + 3 (x – 10)
= (x – 10) (2x + 3) Ans.

Question 35.
Solution:
7y² -19y – 6
= 7y² – 21 y + 2y – 6
{7 x ( – 6) = – 42, – 42 = – 21 x 2, – 19 = – 21 + 2}
= 7y(y – 3) + 2 (y – 3)
= (y – 3) (7y + 2)

Question 36.
Solution:
28 – 31x – 5x²
= 28 – 35x + 4x – 5x²
{28 x ( – 5)= – 140, -140 = – 35 x 4, – 31 = – 35 + 4}
= 7 (4 – 5x) + x (4 – 5x)
= (4 – 5x) (7 + x)

Question 37.
Solution:
3 + 23z – 8z²
= 3 + 24z – z – 8z²
{3 x ( – 8) = – 24, – 24 = 24 x ( – 1), 23 = 24 – 1}
= 3 (1 + 8z) – z (1 + 8z)
= (1 + 8z) (3 – z)

Question 38.
Solution:
6x² – 5x – 6
= 6x² – 9x + 4x – 6
{ 6 x ( – 6) = – 36,- 36 = – 9 x 4, – 5 = – 9 + 4}
= 3x (2x – 3) + 2 (2x – 3)
= (2x – 3) (3x + 2)

Question 39.
Solution:
3m² + 24m + 36
= 3 {m² + 8m + 12}
= 3 {m² + 6m + 2m + 12)
{12 = 6 x 2, 8 = 6 + 2}
= 3 {m (m + 6) + 2 (m + 6)}
= 3 (m + 6) (m + 2)

Question 40.
Solution:
4n² – 8n + 3
= 4n² – 6n – 2n + 3
{4 x 3 = 12, 12 = ( – 6)x( – 2), – 8 = – 6 – 2}
= 2n (2n – 3) – 1 (2n- 3)
= (2n – 3) (2n – 1)

Question 41.
Solution:
6x² – 17x – 3
= 6x² – 18x + x – 3
{6 x ( – 3)= – 18, – 18 = – 18 x 1, – 17 = – 18 + 1}
= 6x (x – 3) + 1 (x – 3)
= (x – 3) (6x + 1)

Question 42.
Solution:
7x² – 19x – 6
7x² – 19x – 6
= 7x² – 21x + 2x – 6
{7 x ( – 6) = – 42, – 42 = – 21 x 2, – 19 = – 21 + 2}
= 7x (x – 3) + 2 (x – 3)
= (x – 3) (7x + 2)

Hope given RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 8 Solutions Chapter 8 Linear Equations Ex 8C

These Solutions are part of RS Aggarwal Solutions Class 8. Here we have given RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8C.

Other Exercises

• RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8A
• RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8B
• RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8C

Objective Questions :
Tick the correct answer in each of the following:

Question 1.
Solution:
2x – 3 = x + 2
=> 2x – x
= 2 + 3
= 5 (c)

Question 2.
Solution:
5x + \(\\ \frac { 7 }{ 2 } \) = \(\\ \frac { 3 }{ 2 } \) x – 14
=> 5x – \(\\ \frac { 3 }{ 2 } \) x = – 14 – \(\\ \frac { 7 }{ 2 } \)

Question 3.
Solution:
z = \(\\ \frac { 4 }{ 5 } \)(z + 10)
=> 5z = 4z + 40
=> 5z – 4z = 40
=> z = 40 (a)

Question 4.
Solution:
3m = 5m – \(\\ \frac { 8 }{ 5 } \)
=> 3m – 5m = \(\\ \frac { -8 }{ 5 } \)
=> – 2m = \(\\ \frac { -8 }{ 5 } \)
=> m = \(\\ \frac { -8 }{ -5×2 } \) = \(\\ \frac { 4 }{ 5 } \) (c)

Question 5.
Solution:
5t – 3 = 3t, – 5
=> 5t – 3t = – 5 + 3
=> 2t = – 2
=> t = \(\\ \frac { -2 }{ 2 } \) = – 1 (b)

Question 6.
Solution:
2y + \(\\ \frac { 5 }{ 3 } \) = \(\\ \frac { 26 }{ 3 } \) – y

Question 7.
Solution:
\(\\ \frac { 6x+1 }{ 3 } \) +1 = \(\\ \frac { x-3 }{ 6 } \)
\(\\ \frac { 12x+2+6=x-3 }{ 6 } \)
12x – x = – 3 – 2 – 6
11x – 11
=> x = \(\\ \frac { -11 }{ 11 } \) = – 1 (b)

Question 8.
Solution:
\(\\ \frac { n }{ 2 } \) – \(\\ \frac { 3n }{ 4 } \) + \(\\ \frac { 5n }{ 6 } \) = 21
\(\\ \frac { 6n-9n+10n= 252 }{ 12 } \)
LCM of 2, 4, 6 = 12
16n – 9n = 252
=> 7n = 252
=> n = \(\\ \frac { 252 }{ 7 } \) = 36 (c)

Question 9.
Solution:
\(\\ \frac { x+1 }{ 2x+3 } \) = \(\\ \frac { 3 }{ 8 } \)
=> 8 (x + 1) = 3 (2x + 3)
(By cross multiplication)
x + 8x + 9 = 8x – 6x = 9 – 8
=> 2x = 1
=> x = \(\\ \frac { 1 }{ 2 } \)
x = \(\\ \frac { 1 }{ 2 } \) (d)

Question 10.
Solution:
\(\\ \frac { 4x+8 }{ 5x+8 } \) = \(\\ \frac { 5 }{ 6 } \)
6(4x + 8) = 5(5x + 8)
(By cross multiplication)
24x + 48 = 25x + 40
=> 24x – 25x = 40 – 48
=> – x = – 8
=> x = 8 (c)

Question 11.
Solution:
\(\\ \frac { n }{ n+15 } \) = \(\\ \frac { 4 }{ 9 } \)
9n = 4n + 60
(By cross multiplication)
9n – 4n = 60
=> 5n = 60
=> n = \(\\ \frac { 60 }{ 5 } \) = 12
n = 12 (d)

Question 12.
Solution:
3(t – 3) = 5 (2t + 1)
3t – 9 = 10t + 5
=> 3t – 10t = 5 + 9
=> – 7t = 14
=> t = \(\\ \frac { 14 }{ -7 } \) = – 2
t = – 2 (a)

Question 13.
Solution:
Let number = x
Then \(\\ \frac { 4 }{ 5 } \)x = \(\\ \frac { 3 }{ 4 } \)x + 4
=> \(\\ \frac { 16x=15x+80 }{ 20 } \)
16x – 15x = 80
=> x = 80
:. Number = 80 (c)

Question 14.
Solution:
Ages of A : B = 5 : 7
Let A’s age = 5x
Then B’s age = 7x
After 4 years
A’s age = 5x + 4
and B’s age = 7x + 4
\(\\ \frac { 5x+4 }{ 7x+5 } \) = \(\\ \frac { 3 }{ 4 } \)
=> 3(7x + 4) = 4(5x + 4)
21x + 12 = 20x + 16
=>21x – 20x = 16 – 12
x = 4
B’s age = 7x
= 7 x 4
= 28 years (b)

Question 15.
Solution:
Perimeter of an isosceles triangle = 16 cm
and base = 6 cm
Let each equal side = x cm
x + x + 6 = 16
=> 2x = 16 – 6 = 10
=> x = \(\\ \frac { 10 }{ 2 } \) = 5
Each equal side = 5 cm (b)

Question 16.
Solution:
Let first number = x
Then second number = x + 1
and third number = x+ 2
x + x + 1 + x + 2 = 51
=> 3x + 3 = 51
=> 3x = 51 – 3 = 48
=> x = \(\\ \frac { 48 }{ 3 } \) = 16
Middle number = x + 1 = 16 + 1 = 17 (b)

Question 17.
Solution:
Let first number = x
Then second number = x + 15
x + x + 15 = 95
=> 2x = 95 – 15 = 80
=> x= \(\\ \frac { 80 }{ 2 } \) = 40
=> Smaller number = 40

Question 18.
Solution:
Ratio in boys and girls in a class = 7:5
Let no. of boys = 7x
Then no. of girls = 5x
7x – 5x = 8
=> 2x = 8
x = \(\\ \frac { 8 }{ 2 } \) = 4
Total strength = 7x + 5x = 12x
= 12 x 4
= 48 (c)

Hope given RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 8 Solutions Chapter 7 Factorisation Ex 7E

These Solutions are part of RS Aggarwal Solutions Class 8. Here we have given RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7E.

Other Exercises

• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7A
• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7B
• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7C
• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7D
• RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7E

OBJECTIVE QUESTIONS.
Tick the correct answer in each of the following :

Question 1.
Solution:
7a² – 63b² = 7 (a² – 9b²)
= 7 {(a)² – (3b)²}
= 7 (a – 3b) (a + 36) (d)

Question 2.
Solution:
2x – 32x³ = 2x (1 – 16x²)
= 2x {(1)² – (4x)²}
= 2x (1 – 4x) (1 + 4x) (d)

Question 3.
Solution:
x³ – 144x
= x (x² – 144)
= x {(x)² – (12)²}
= x (x – 12) (x + 12) (c)

Question 4.
Solution:
2 – 50x² = 2 (1 – 25x²)
= 2 {(1)² – (5x)²}
= 2 (1 – 5x) (1 + 5x) (d)

Question 5.
Solution:
a² + bc + ab + ac
= a2 + ab + ac + bc
= a (a + b) + c (a + b)
= (a + b) (a + c)   (a)

Question 6.
Solution:
pq² + q (p – 1) – 1
= pq² + pq – q – 1
= pq (q + 1) – 1 (q + 1)
= (q + 1) (pq – 1)
= (pq – 1) (q + 1) (d)

Question 7.
Solution:
ab – mn + an – bm
= ab + an – bm – mn
= a (b + n) – m (b + n)
= (b + n) (a – m) (b)

Question 8.
Solution:
ab – a – b + 1
= a (b – 1) – 1 (b – 1)
= b – 1) (a – 1) (a)

Question 9.
Solution:
x² – xz + xy – yz
= x (x – z) + y (x – z)
= (x – z) (x + y) (c)

Question 10.
Solution:
12m² – 21
= 3 (4m² – 9)
= 3 {(2m)² – (3)²}
= 3 (2m – 3) (2m + 3) (c)

Question 11.
Solution:
x³ – x
= x (x² – 1)
= x (x – 1) (x + 1) (d)

Question 12.
Solution:
1 – 2ab – (a² + b²)
= 1 – 2ab – a² – b²
= 1 – (a² + b² + 2ab)
= 1 – (a + b)²
= (1 + a + b) (1 – a – b) (c)

Question 13.
Solution:
x² + 6x + 8
= x² + 4x + 2x + 8
{8 = 4 x 2, 6 = 4 + 2}
= x (x + 4) + 2 (x + 4)
= (x + 4) (x + 2) (c)

Question 14.
Solution:
x² + 4x – 21
= x² + 7x – 3x – 21
{ – 21 = + 7 x ( – 3), 4 = 7 – 3}
= x (x + 7) – 3 (x + 7)
= (x + 7) (x – 3) (b)

Question 15.
Solution:
y² + 2y – 3
{ – 3 = 3 x ( – 1), 2 = 3 – 1}
= y² + 3y – y – 3
= y (y + 3) – 1 (y + 3)
= (y + 3) (y – 1) (a)

Question 16.
Solution:
40 + 3x – x²
= 40 + 8x – 5x -x²
{40 = 8 x ( – 5), 3 = 8 – 5}
= 8(5 + x) – x(5 + x)
= (5 + x)(8 – x) (c)

Question 17.
Solution:
2x² + 5x + 3
= 2x² + 2x + 3x + 3
{2 x 3 = 6, 6 = 2 x 3, 5 = 2 + 3}
= 2x(x + 1) + 3(x + 1)
= (x + 1)(2x + 3) (b)

Question 18.
Solution:
6a² – 13a + 6
= 6a² – 9a – 4a + 6
{6 x 6 = 36, 36 = ( – 9)x( – 4), – 13 = – 9 – 4}
= 3a (2a – 3) – 2 (2a – 3)
= (2a – 3) (3a – 2) (c)

Question 19.
Solution:
4z² – 8z + 3
= 4z² – 6z – 2z + 3
{4 x 3 = 12,12 = ( – 6)x( – 2), – 8 = – 6 – 2}
= 2z (2z – 3) – 1 (2z – 3)
= (2z – 3) (2z – 1) (a)

Question 20.
Solution:
3 + 23y – 8y²
= 3 + 24y – y – 8y²
{3 x ( – 8) = – 24, – 24 = 24 x ( – 1), 23 = 24 – 1}
= 3(1 + 8y) – y(1 + 8y)
= (1 + 8y) (3 – y) (b)

Hope given RS Aggarwal Solutions Class 8 Chapter 7 Factorisation Ex 7E are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 8 Solutions Chapter 10 Profit and Loss Ex 10C

These Solutions are part of RS Aggarwal Solutions Class 8. Here we have given RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10C.

Other Exercises

• RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10A
• RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10B
• RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10C
• RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10D

Question 1.
Solution:
List price of refrigerator = Rs. 14650
Sales tax = 6%

Question 2.
Solution:
(i) Lost of tie = Rs. 250
ST = 6%

Question 3.
Solution:
Price of watch including VAT = Rs. 1980
Rate of VAT = 10%

Question 4.
Solution:
Price of shirt including VAT = Rs. 133750
Rate of VAT = 7%
∴ Original price of the shirt

Question 5.
Solution:
Sale price of 10 g gold including VAT = Rs. 15756
Rate of VAT = 1%

Question 6.
Solution:
Sale price of computer including VAT = Rs. 37960
Rate of VAT = 4%
∴ Original price of computer

Question 7.
Solution:
Sale price of car parts including VAT = Rs. 20776
Rate of VAT = 12%
∴ Original price of car parts

Question 8.
Solution:
Sale price of TV set including VAT = Rs. 27000
Rate of VAT = 8%

Question 9.
Solution:
Sale price of shoes including VAT = Rs. 882
Original price = Rs 840

Question 10.
Solution:
Sale price of VCR including VAT = Rs. 19980
Original price = Rs. 18500
∴Amount of VAT

Question 11.
Solution:
Sale price of car including VAT = Rs. 382500
Basic price of the car = Rs. 340000
Amount of VAT = Rs. 382500 – 340000
= Rs. 42500

Hope given RS Aggarwal Solutions Class 8 Chapter 10 Profit and Loss Ex 10C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 8 Solutions Chapter 9 Percentage Ex 9A

These Solutions are part of RS Aggarwal Solutions Class 8. Here we have given RS Aggarwal Solutions Class 8 Chapter 9 Percentage Ex 9A.

Other Exercises

• RS Aggarwal Solutions Class 8 Chapter 9 Percentage Ex 9A
• RS Aggarwal Solutions Class 8 Chapter 9 Percentage Ex 9B

Question 1.
Solution:
(i) 48% = \(\\ \frac { 48 }{ 100 } \) = \(\\ \frac { 12 }{ 25 } \)
(ii) 220% = \(\\ \frac { 220 }{ 100 } \) = \(\\ \frac { 11 }{ 5 } \)
(iii) 2.5% = \(\\ \frac { 2.5 }{ 100 } \) = \(\\ \frac { 25 }{ 10X100 } \) = \(\\ \frac { 1 }{ 40 } \)

Question 2.
Solution:
(i) 6% = \(\\ \frac { 6 }{ 100 } \) = 0.06
(ii) 72% = \(\\ \frac { 72 }{ 100 } \) = 0.72
(iii) 125% = \(\\ \frac { 125 }{ 100 } \) = 1.25

Question 3.
Solution:

You can also Download NCERT Solutions for Class 8 Maths to help you to revise complete Syllabus and score more marks in your examinations.

Question 4.
Solution:
Ratio is 4:5 or \(\\ \frac { 4 }{ 5 } \)
\(\\ \frac { 4 }{ 5 } \)
= \(\\ \frac { 4X20 }{ 5X20 } \)
= \(\\ \frac { 80 }{ 100 } \)
= 80%

Question 5.
Solution:
125% = \(\\ \frac { 125 }{ 100 } \)
= \(\\ \frac { 5 }{ 4 } \) (dividing by 25)
Ratio = 5:4

Question 6.
Solution:

We see that 15% or \(\\ \frac { 3 }{ 20 } \) is the largest

Question 7.
Solution:
(i) Let x% of 150
= 96

Question 8.
Solution:
\(6\frac { 2 }{ 3 } %\) of Rs 3600
= Rs \(\\ \frac { 9 }{ 2 } \) x \(\\ \frac { 3600 }{ 100 } \)
= Rs 162

Question 9.
Solution:
16% of a number = 72
Number = \(\\ \frac { 72 }{ 16 }\)%
= \(\\ \frac { 72X100 }{ 16 } \)
= 450

Question 10.
Solution:
Let monthly income = Rs x
Savings = 18%

Question 11.
Solution:
Let total games = x
Then game which a team wins = 73
and it is 35% of total games
35% of x = 7
=> x = \(\\ \frac { 7X100 }{ 35 } \) = 20
Number of total games = 20

Question 12.
Solution:
Let salary = Rs x
Increment = 20%
Total salary = x + 20% of x

Question 13.
Solution:
No. of days, Sonal attended = 204 days
and her attendance = 85% of total days
85% of total days = 204
Total number of days = \(\\ \frac { 204X100 }{ 85 } \)
= 240 days

Question 14.
Solution:
Let B’s income = Rs. 100
Then A’s income = 20% less than B’s
= 100 – 20 = Rs. 80
Difference = 100 – 80 = 20
B’s more income that A’s = \(\\ \frac { 20 }{ 80 } \) x 100
= 25%

Question 15.
Solution:
Increase in price of petrol = 10%
Let first price = Rs. 100 p.l.
Increased price = 100 + 10 = Rs. 110
Now reduction in consumption = 110 – 100= 10
Percentage reduced consumption
= \(\\ \frac { 10X100 }{ 110 } \)
= \(\\ \frac { 100 }{ 11 } \)
= \(9\frac { 1 }{ 11 } %\)

Question 16.
Solution:
Present population of a town = 54000
Rate of increase = 8% annually
Population a year ago = \(\\ \frac { 54000X100 }{ 100+8 } \)
= \(\\ \frac { 54000X100 }{ 108 } \)
= 50000

Question 17.
Solution:
Depreciation in the value of machine = 20%
Present value = Rs. 160000
Then value of machine one year ago
= \(\\ \frac { 160000X100 }{ 100-20 } \)
= \(\\ \frac { 160000X100 }{ 80 } \)
= Rs 200000

Question 18.
Solution:
In an alloy,
Copper = 40%
Nickel = 32%
Zinc = 100 – (40% + 32%)
= 100 – 72 = 28%
Then mass of zinc in one kg of alloy
= 1 kg X 28%
= \(\\ \frac { 1000X28 }{ 100 } \) g
= 280 gm

Question 19.
Solution:
In balance diet,
Protein = 12%
Fats = 25%
Carbohydrates = 63%
Total number of dories = 2600

Question 20.
Solution:
In gunpowder,
Nitre = 75%
Sulphur = 10%
(i) Amount of gunpowder if nitre is 9 kg
= \(\\ \frac { 100 }{ 75 } \) x 9
= 12 kg
(ii) Amount of gunpowder if sulphur is 2.5kg 100
= \(\\ \frac { 100 }{ 10 } \) x 2.5
= 25 kg

Question 21.
Solution:
Let C get = Rs. x

Question 22.
Solution:
24-carat gold is 100% pure
22 parts out of 24 part in 22-carat gold

Question 23.
Solution:
Let present salary = Rs. 100
Increase = 25%
Increased salary = Rs. 100 + 25
= Rs. 125
To receive the original salary, amount to be decreased = Rs. 125 – 100 = Rs. 25
∴ % decrease = \(\\ \frac { 25X100 }{ 125 } \) = 20%

 

Hope given RS Aggarwal Solutions Class 8 Chapter 9 Percentage Ex 9A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 8 Solutions Chapter 8 Linear Equations Ex 8B

These Solutions are part of RS Aggarwal Solutions Class 8. Here we have given RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8B.

Other Exercises

• RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8A
• RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8B
• RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8C

Question 1.
Solution:
The numbers be 8x and 3x (∵ Ratio is 8 : 3)
Sum = 143
According to the condition
8x + 3x = 143
=> 11x = 143
=> x = \(\\ \frac { 143 }{ 11 } \) = 13
First number = 8x = 8 x 13 = 104
and second number = 3x = 3 x 13 = 39 Ans.

Question 2.
Solution:
Let the number = x
According to the condition,
x – \(\frac { 2 }{ 3 } x\) = 20
=> \(\\ \frac { 3x-2x }{ 3 } \) = 20
=> \(\\ \frac { x }{ 3 } \) = 20
=> x = 20 x 3 = 60
Hence original number = 60 Ans.

You can also Download NCERT Solutions for Class 8 Maths to help you to revise complete Syllabus and score more marks in your examinations.

Question 3.
Solution:
Let the number = x
According to the condition
\(\frac { 4 }{ 5 } x-\frac { 2 }{ 3 } x\) = 10
=> \(\\ \frac { 12x-10x }{ 15 } \) = 10
=> \(\\ \frac { 2x }{ 15 } \) = 10
=> 2x = 10 x 15
x = \(\\ \frac { 10 x 15 }{ 2 } \) = 75
Hence number = 75 Ans.

Question 4.
Solution:
Let first part = x
then second part = 24 – x (∵ Sum = 24)
According to the condition,
7x + 5 (24 – x) = 146
=> 7x + 120 – 5x = 146
=> 2x = 146 – 120 = 26
=> x = \(\\ \frac { 26 }{ 2 } \) = 13
First part = 13
and second part = 24 – 13 = 11 Ans.

Question 5.
Solution:
Let number = x
According to the condition
\(\frac { x }{ 5 } +5=\frac { x }{ 4 } -5\)
=> \(\\ \frac { x }{ 5 } \) – \(\\ \frac { x }{ 4 } \)= – 5 – 5
=> \(\\ \frac { 4x-5x }{ 20 } \) = – 10
=> \(\\ \frac { -x }{ 20 } \) = – 10 = \(\\ \frac { x }{ 20 } \) = 10
=> x = 10 × 20 = 200
Hence number = 200 Ans.

Question 6.
Solution:
Ratio between three numbers = 4:5:6
Let the largest number = 6x
smallest number = 4x
and third number = 5x
According to the condition,
6x + 4x = 5x + 55
=> 10x = 5x + 55
=> 10x – 5x = 55
=> 5x = 55
=> x = \(\\ \frac { 55 }{ 5 } \) = 11
Numbers will be 4x = 4 × 11 = 44,
5x = 5 × 31 = 55 and 6x = 6 × 11 = 66
Hence numbers are 44, 55, 66 Ans.

Question 7.
Solution:
Let the number = x
According to the condition,
4x + 10 = 5x – 5
=> 4x – 5x = – 5 – 10
=> – x = – 15
x = 15
Hence number = 15 Ans.

Question 8.
Solution:
Ratio between two numbers 3: 5
Let first number = 3x
and second number = 5x
Now according to the condition.
3x + 10 : 5x + 10 = 5 : 7

Question 9.
Solution:
Let first odd number = 2x + 1
second number 2x + 3
and third number = 2 + 5
According to the condition.
2x + 1 + 2x + 3 + 2x + 5 = 147
=> 6x + 9 = 147
=> 6x = 147 – 9 = 138
=> x = \(\\ \frac { 138 }{ 6 } \) = 23
Hence first odd number 2x + 1
= 23 x 2 + 1 = 46 + 1 = 47
Second number 47 + 2 = 49
and third number = 49 + 2 = 51 Ans.

Question 10.
Solution:
Let first even number = 2x
second number = 2x + 2
and third number = 2x + 4
According to the condition,
2x + 2x + 2 + 2x + 4 = 234
=> 6x + 6 = 234
=> 6x = 234 – 6
=> 6x = 228
=> x = \(\\ \frac { 228 }{ 6 } \) = 38
First even number = 2x = 38 x 2 = 76
second number = 76 + 2 = 78
and third number 78 + 2 = 80 Ans.

Question 11.
Solution:
The sum of two digits = 12
Let the ones digit of the number = x
then tens digit = 12 – x
and number = x + 10 (12 – x)
= x + 120 – 10x = 120 – 9x
Reversing the digits,
ones digit of new number = 12 – x
and tens digit = x
the number = 12 – x + 10x = 12 + 9x
According to the condition,
12 + 9x = 120 – 9x + 54
=> 9x + 9x
=> 120 + 54 – 12
=> 174 – 12
=> 18x = 162
=> x = \(\\ \frac { 162 }{ 18 } \) = 9
Original number = 120 – 9x
= 120 – 9 x 9
= 120 – 81
= 39
Hence number 39 Ans.
Check :Original number= 39
Sum of digits = 3 + 9 = 12
Now reversing its digit the new number
will be = 93
and 93 – 39 = 54 which is given.

Question 12.
Solution:
Let units digit of the number = x
then tens digit = 3x
and number = x + 10
3x = x + 30x = 31x
on reversing the digits.
units digit = 3x
and tens digit = x
then number 3x + 10x = 13x
According to the condition,
31x – 36 = 13x
=> 31x – 13x = 36
=> 18 x 36
=> x = \(\\ \frac { 36 }{ 18 } \) = 2
The original number = 31x = 31 x 2 = 62
Hence number = 62 Ans.
Check : Number = 62
tens digit = 2 x 3 = 6
On reversing the digit, the new number will be = 26
62 – 26 = 36 which is given.

Question 13.
Solution:
Let numerator of a rational number = x
Then its denominator = x + 7

Question 14.
Solution:
Let numerator of a fraction = x
The denominator = 2x – 2

Question 15.
Solution:
Let breadth of the rectangle = x cm
then length = x + 7
Area = l × b = (x + 7) × x
In second case,
Length of the new rectangle = x + 7 – 4
= x + 3 cm
and breadth = x + 3
Area = (x + 3)(x + 3)
According to the condition,
(x + 3)(x + 3) = x(x + 7)
x2 + 3x + 3x + 9 = x2 + 7x
=> x2 + 6x – 7x – x2 = – 9
=> x = – 9
=> x = 9
Length of the original rectangle
= > x + 7 = 9 + 7 = 16 cm
and breadth = x = 9 cm. Ans.

Question 16.
Solution:
Let length of rectangle = x m
then width = \(\frac { 2 }{ 3 } x\)m
Perimeter = 2 (l + b) m
=> \(2\left( x+\frac { 2 }{ 3 } x \right) =180\)

Question 17.
Solution:
Let the length of the base of the triangle = x cm
then altitude = \(\frac { 5 }{ 3 } x\)cm
Area = \(\\ \frac { 1 }{ 2 } \) base x altitude

Question 18.
Solution:
Let ∠A, ∠B and ∠C are the three angles of a triangle and
Let ∠A + ∠B = ∠C
But ∠A + ∠B + ∠C = 180°
∠C + ∠C = 180°
=> 2∠C = 180°
∠C = 90°
and ∠A + ∠B = 90°
Let ∠A = 4x and ∠B = 5x
4x + 5x = 90°
=> 9x = 90°
x = \(\frac { { 90 }^{ o } }{ 9 } \) = 10°
∠A = 4x = 4 x 10° = 40°
and ∠B = 5x = 5 x 10° = 50°
and ∠C = 90° Ans.

Question 19.
Solution:
Time taken downstream = 9 hour
and time taken upstream = 10 hour
Speed of stream = 1 km/h
Let the speed of a steamer in still water
= x km/h.
Distance downstream = 9(x + 1) km.
and upstream = 10 (x – 1) km.
According to the condition,
10(x – 1) = 9(x + 1)
l0x – 10 = 9x + 9
=> 10x – 9x = 9 + 10
=> x = 19
Hence speed of steamer in still water = 19 km/h
and distance = 9(x + 1)
= 9(19 + 1)
= 9 x 20km
= 180km. Ans.

Question 20.
Solution:
The distance between two stations = 300 km
Let the speed of first motorcyclists = x km/h
and speed of second motorcyclists = (x + 7)km/h
Distance covered by the first = 2x km
and distance covered by the second = 2 (x + 7) km
= 2x + 14 km
Distance uncovered by them = 300 – (2x + 2x + 14)kms.
According to the condition,
300 – (4x + 4) = 34
=> 300 – 4x – 14 = 34
=> 300 – 14 – 34 = 4x
=> 4x = 300 – 48
=> 4x = 252
=> x = \(\\ \frac { 252 }{ 4 } \) = 63
Speed of the first motorcyclists = 63km/h
and speed of second = 63 + 7 = 70 km/h
Check. Distance covered by both of them
= 2 x 63 + 2 x 70 = 126 + 140 = 266
Total distance = 300 km.
Distance between them = 300 – 266 = 34 km.
which is given.

Question 21.
Solution:
Sum of three numbers = 150
Let first number = x
then second number = \(\frac { 5 }{ 6 } x\)
and third number = \(\\ \frac { 4 }{ 5 } \) of second

Question 22.
Solution:
Sum of two pans = 4500
Let first part = x
then second part = 4500 – x
According to the condition,
5% of x = 10% of (4500 – x)

Question 23.
Solution:
Let age of Rakhi = x years
then her mother’s age = 4x
After 5 years,
Rakhi’s age = x + 5
and her mother’s age = 4x + 5
According to the conditions,
4x + 5 = 3 (x + 5)
=> 4x + 5 = 3x + 15
=> 4x – 3x = 15 – 5
=> x = 10
Rakhi ‘s present age = 10 years
and her mother’s age = 4 x 10
= 40 years Ans.

Question 24.
Solution:
Let age of Monu = x year
His father’s age = x + 29
and his grandfather’s age = x + 29 + 26
= x + 55
and sum of their ages = 135 years
Now,
x + x + 29 + x + 55 = 135
=> 3x + 84 = 135
=> 3x = 135 – 84 = 51
=> x = \(\\ \frac { 51 }{ 3 } \) = 17 years
Monu’s age = 17 years
His father age = 17 + 29 = 46 years
and his grandfather’s age = 46 + 26 = 72 years

Question 25.
Solution:
Let age of grandson = x year
Then his age = 10x
But 10x = x + 54
=> 10x – x = 54
=> 9x = 54
=> x = \(\\ \frac { 54 }{ 9 } \) = 6
Grand’s son age = 6 years
and his age = 6 x 10 = 60 years

Question 26.
Solution:
Let age of elder cousin = x years
and age of younger = (x – 10) years.
15 years ago,
age of older, cousin = x – 15 years
and age of younger = x – 10 – 15
= (x – 25) years.
According to the condition,
x – 15 = 2(x – 25)
=> x – 15 = 2x – 50
=> 2x – x = 50 – 15
=> x = 35
Age of elder cousin = 35 years
and age of younger = 35 – 10
= 25 years Ans.

Question 27.
Solution:
Let number of deer = x

Hope given RS Aggarwal Solutions Class 8 Chapter 8 Linear Equations Ex 8B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.