Category: Chemistry

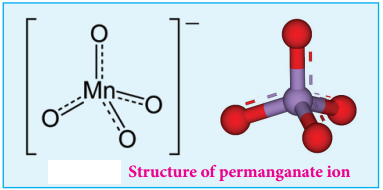
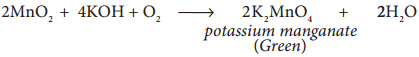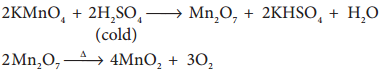Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.

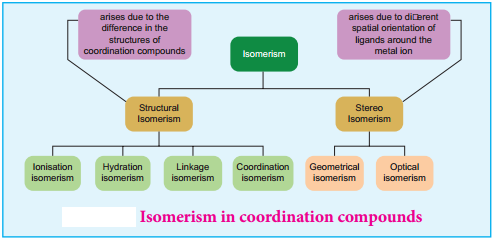
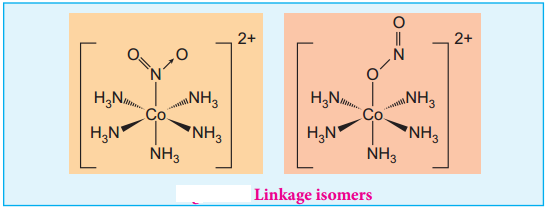
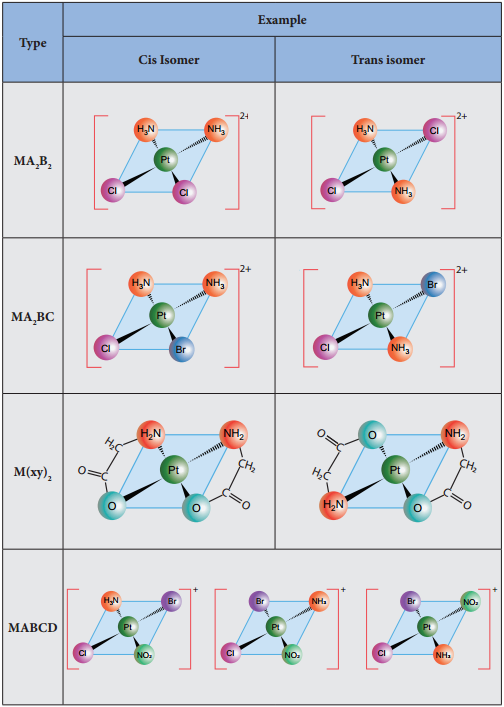
Theories of Coordination Compound

Alfred Werner considered the bonding in coordination compounds as the bonding between a lewis acid and a lewis base. His approach is useful in explaining some of the observed properties of coordination compounds. However, properties such as colour, magnetic property etc of complexes could not be explained on the basis of his approach.

Following werner theory, Linus pauling proposed the Valence Bond Thory (VBT) which assumes that the bond formed between the central metal atom and the ligand is purely covalent. Bethe and Van vleck treated the interaction between the metal ion and the ligands as electrostatic and extended the Crystal Field Theory (CFT) to explain the properties of coordination compounds. Further, Ligand field theory and Molecular orbital have been developed to explain the nature of bonding in the coordination compounds. In this porton we learn the elementry treatment of VBT and CFT to simple coordination compounds.

Valence Bond Theory

According to this theory, the bond formed between the central metal atom and the ligand is due to the overlap of filed ligand orbitals containing a lone pair of electron with the vacant hybrid orbitals of the central metal atom.

Main assumptions of VBT:

1. The ligand → metal bond in a coordination complex is covalent in nature. It is formed by sharing of electrons (provided by the ligands) between the central metal atom and the ligand.
2. Each ligand should have at least one filled orbital containing a lone pair of electrons.
3. In order to accommodate the electron pairs donated by the ligands, the central metal ion present in a complex provides required number (coordination number) of vacant orbitals.
4. These vacant orbitals of central metal atom undergo hybridisation, the process of mixing of atomic orbitals of comparable energy to form equal number of new orbitals called hybridised orbitals with same energy.
5. The vacant hybridised orbitals of the central metal ion, linearly overlap with filled orbitals of the ligands to form coordinate covalent sigma bonds between the metal and the ligand.
6. The hybridised orbitals are directional and their orientation in space gives a definite geometry to the complex ion.
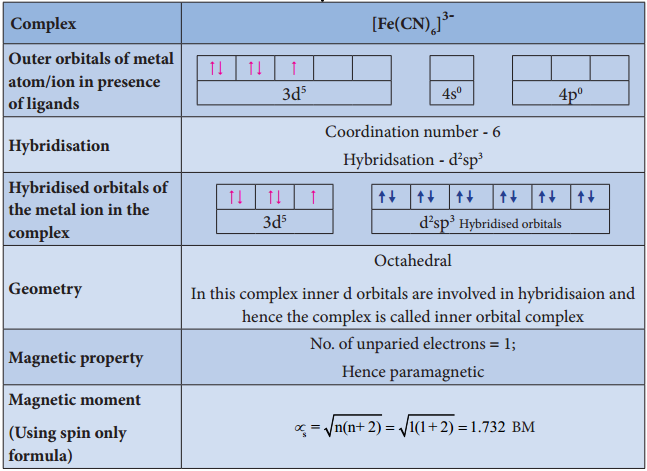
7. In the octahedral complexes, if the (n-1) d orbitals are involved in hybridisation, then they are called inner orbital complexes or low spin complexes or spin paired complexes.
8. If the nd orbitals are involved in hybridisation, then such complexes are called outer orbital or high spin or spin free complexes.
9. Here n represents the principal quantum number of the outermost shell.
10. The complexes containing a central metal atom with unpaired electron(s) are paramagnetic. If all the electrons are paired, then the complexes will be diamagnetic.
11. Ligands such as CO, CN^–, en, and NH₃ present in the complexes cause pairing of electrons present in the central metal atom. Such ligands are called strong field ligands.
12. Greater the overlapping between the ligand orbitals and the hybridised metal orbital, greater is the bond strength.

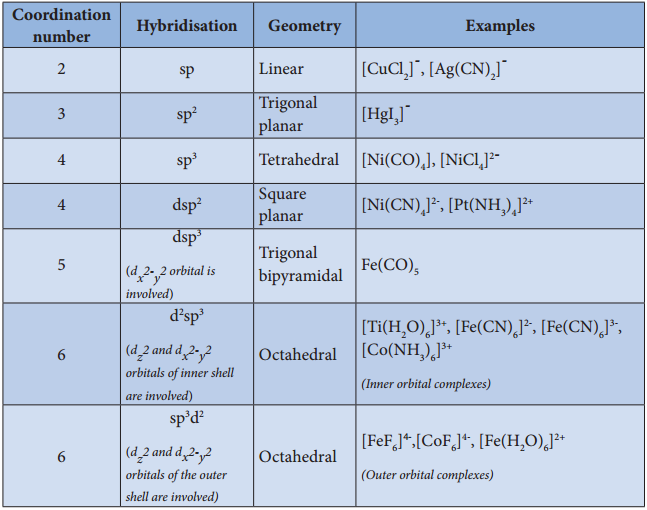
Let us illustrate the VBT by considering the following examples.

Illustration 1

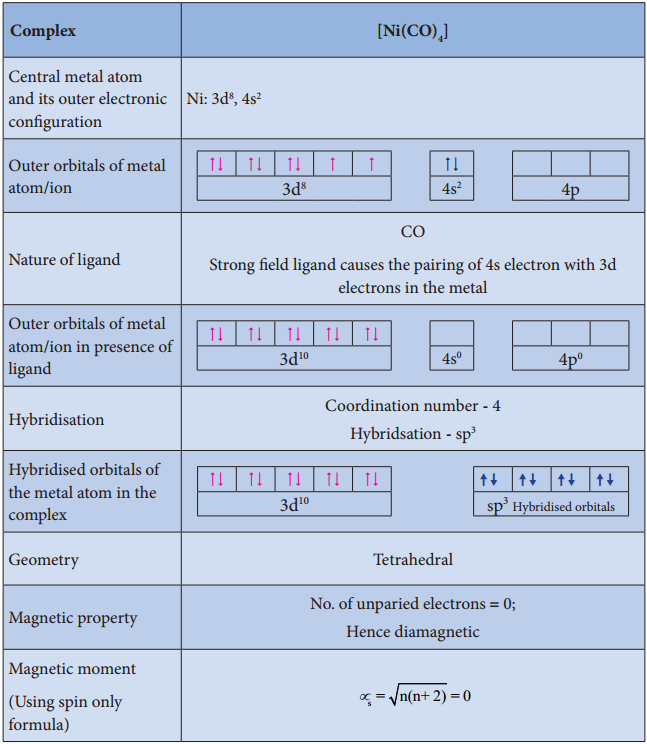
Illustration 2

Illustration 3

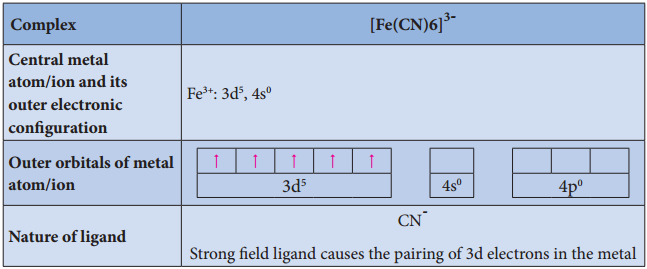
Illustration 4

Limitations of VBT

Eventhough VBT explains many of the observed properties of complexes, it still has following limitations

1. It does not explain the colour of the complex
2. It considers only the spin only magnetic moments and does not consider the other components of magnetic moments.
3. It does not provide a quantitative explanation as to why certain complexes are inner orbital complexes and the others are outer orbital complexes for the same metal.
4. For example, [Fe(CN)₆]^4- is diamagnetic (low spin) whereas [FeF₆]^4- is paramagnetic (high spin).

Crystal Field Theory

Valence bond theory helps us to visualise the bonding in complexes. However, it has limitations as mentioned above. Hence Crystal Field Theory to expalin some of the properties like colour, magnetic behaviour etc., This theory was originally used to explain the nature of bonding in ionic crystals. Later on, it is used to explain the properties of transition metals and their complexes. The salient features of this theory are as follows.

1. Crystal Field Theory (CFT) assumes that the bond between the ligand and the central metal atom is purely ionic. i.e. the bond is formed due to the electrostatic attraction between the electron rich ligand and the electron deficient metal.

2. In the coordination compounds, the central metal atom/ion and the ligands are considered as point charges (in case of charged metal ions or ligands) or electric dipoles (in case of metal atoms or neutral ligands).

3. According to crystal fild theory, the complex formation is considered as the following series of hypothetical steps.

Step 1:

In an isolated gaseous state, all the five d orbitals of the central metal ion are degenerate. Initially, the ligands form a spherical field of negative charge around the metal. In this field, the energies of all the five d orbitals will increase due to the repulsion between the electrons of the metal and the ligand.

Step 2:

The ligands are approaching the metal atom in actual bond directions. To illustrate this let us consider an octahedral field, in which the central metal ion is located at the origin and the six ligands are coming from the +x, -x, +y, -y, +z and -z directions as shown below.

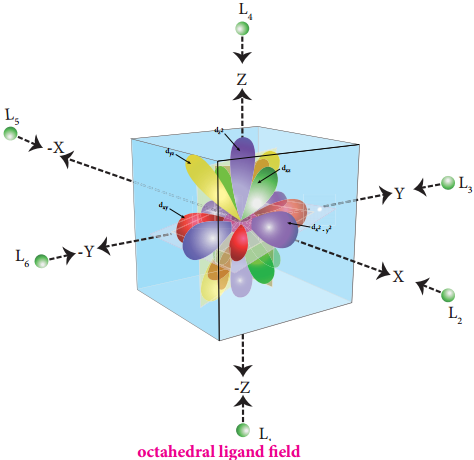
As shown in the figure, the orbitals lying along the axes dx²-y² and dz² orbitals will experience strong repulsion and raise in energy to a greater extent than the orbitals with lobes directed between the axes (d_xy, d_yz and d_zx). Thus the degenerate d orbitals now split into two sets and the process is called crystal field splitting.

Step 3:

Up to this point the complex formation would not be favoured. However, when the ligands approach further, there will be an attraction between the negatively charged electron and the positively charged metal ion, that results in a net decrease in energy. This decrease in energy is the driving force for the complex formation.

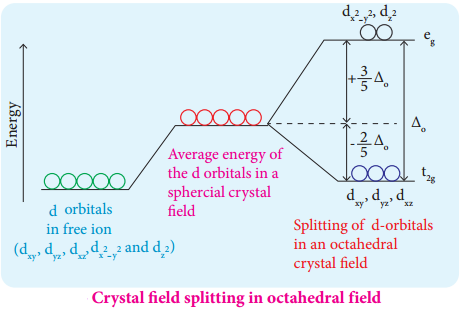
Crystal Field Splitting in Octahedral Complexes:

During crystal field splitting in octahedral field, in order to maintain the average energy of the orbitals (barycentre) constant, the energy of the orbitals (barycentre) constant, the energy of the orbitals \(\mathrm{d}_{\mathrm{x}}^{2}-\mathrm{y}^{2}\) and \(\mathrm{d}_{\mathrm{z}} 2\) (represented as e_g orbitals) will increase by 3/5 Δ_° while that of the other three orbitals d_xy, d_yz and d_zx (represented as t_2g orbitals) decrease by 2/5 Δ_°. Here, Δ_° represents the crystal field splitting energy in the octahedral field.

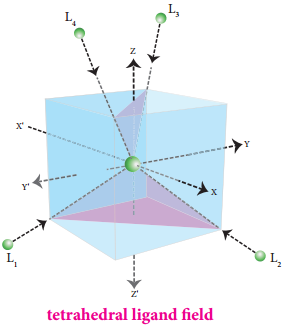
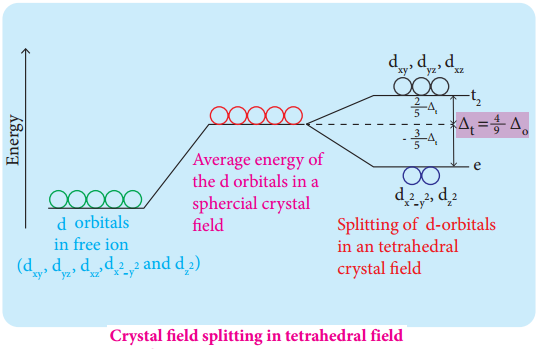
Crystal Field Splitting in Tetrahedral Complexes:

The approach of ligands in tetrahedral field can be visualised as follows. Consider a cube in which the central metal atom is placed at its centre (i.e. origin of the coordinate axis as shown in the figure). The four ligands approach the central metal atom along the direction of the leading diagonals drawn from alternate corners of the cube.

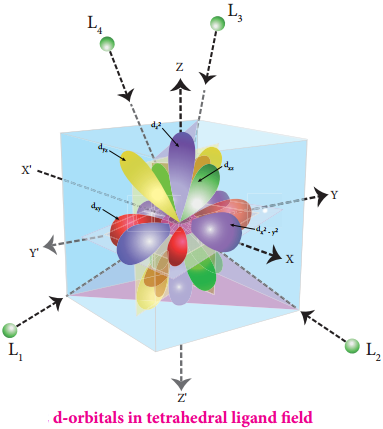
In this field, none of the d orbitals point directly towards the ligands, however the t₂ orbitals (d_xy, d_yz and d_zx) are pointing close to the direction in which ligands are approaching than the e orbitals (\(\mathrm{d}_{\mathrm{x}}^{2}-\mathrm{y}^{2}\) and \(\mathrm{d}_{\mathrm{z}} 2\)).

As a result, the energy of t₂ orbitals increases by 2/5Δ_t and that of e orbitals decreases by 3/5Δ_t as shown below. when compared to the octahedral field, this splitting is inverted and the spliting energy is less. The relation between the crystal field splitting energy in octahedral and tetrahedral ligand field is given by the expression; ∆_t = \(\frac{4}{9}\)∆_°

Crystal Filed Splitting Energy and Nature of Ligands:

The magnitude of crystal field splitting energy not only depends on the ligand field as discussed above but also depends on the nature of the ligand, the nature of the central metal atom/ion and the charge on it. Let us understand the effect of the nature of ligand on crystal field splitting by calculating the crystal field splitting energy of the octahedral complexes of titanium(III) with different ligands such as fluoride, bromide and water using their absorption spectral data.

The absorption wave numbers of complexes [TiBr₆]^3-, [TiF₆]^3- and [Ti(H₂O)₆]³⁺ are 12500, 19000 and 20000 cm^-1 respectively. The energy associated with the absorbed wave numbers of the light, corresponds to the crystal field splitting energy (Δ) and is given by the following expression,

Δ = hν = hc/λ = hc\(\bar {V} \)

where h is the Plank’ s constant; c is velocity of light, υ is the wave number of absorption maximum which is equal to 1/λ

From the above calculations, it is clear that the crystal filed splitting energy of the Ti³⁺ in complexes, the three ligands is in the order; Br^– < F^– < H₂O. Similarly, it has been found form the spectral data that the crystal field splitting power of various ligands for a given metal ion, are in the following order.

I^– < Br^– < SCN^– < Cl^– < S^2- < F^– < OH^– ~ urea < ox^2-
< H₂O < NCS^– < EDTA^4- < NH₃ < en < NO₂^– < en < NO₂^– < CN^– < CO

The above series is known as spectrochemical series. The ligands present on the right side of the series such as carbonyl causes relatively larger crystal field splitting and are called strong ligands or strong field ligands, while the ligands on the left side are called weak field ligands and causes relatively smaller crystal field splitting.

Distribution of D Electrons in Octahedral Complexes:

The filing of electrons in the d orbitals in the presence of ligand field also follows Hund’s rule. In the octahedral complexes with d² and d³ configurations, the electrons occupy different degenerate t_2g orbitals and remains unpaired. In case of d⁴ configuration, there are two possibilities. The fourth electron may either go to the higher energy e_g orbitals or it may pair with one of the t_2g electrons. In this scenario, the preferred confiuration will be the one with lowest energy.

If the octahedral crystal field splitting energy (Δ_°) is greater than the pairing energy (P), it is necessary to cause pairing of electrons in an orbital, then the fourth electron will pair up with an the electron in the t_2g orbital. Conversely, if the Δ_° is lesser than P, then the fourth electron will occupy one of the degenerate higher energy e_g orbitals.

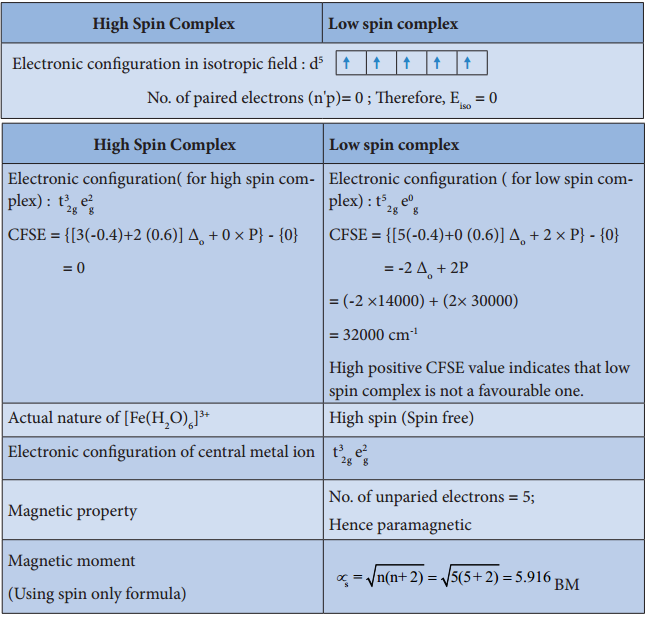
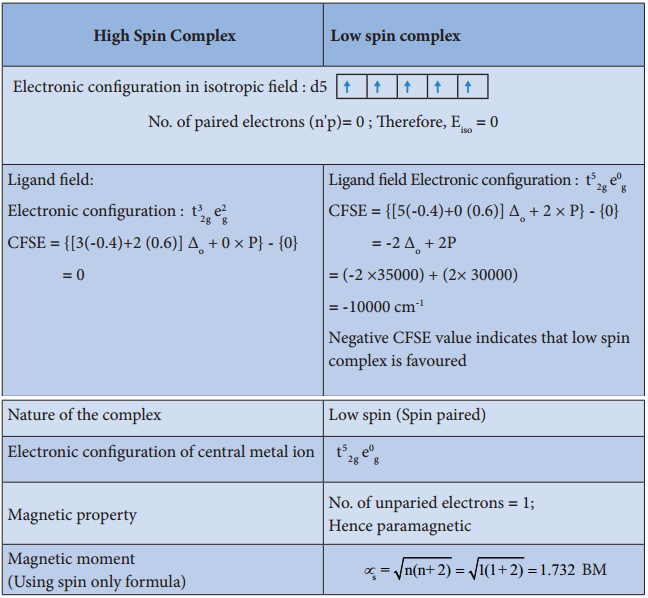
For example, let us consider two diffrent iron(III) complexes [Fe(H₂O₆)]³⁺ (weak field complex; wave number corresponds to Δ_° is 14000 cm^-1) and [Fe(CN)₆]^3- (Strong field complex; wave number corresponds to Δ_° is 35000 cm^-1. The wave number corresponds to the pairing energy of Fe³⁺ is 30000 cm^-1.

In both these complexes the Fe³⁺ has d⁵ configuration. In aqua complex, the Δ_° < P hence, the fourth & fifth electrons enter e_g orbitals and the configuration is t_2g³, e_g². In the cyanido complex Δ_° < P and hence the fourth & fit electrons pair up with the electrons in the t_2g orbitals and the electronic configuration is t_2g³, e_g².

The actual distribution of electrons can be ascertained by calculating the crystal field stabilisation energy (CFSE). The crystal field stabilisation energy is defined as the energy difference of electronic confiurations in the ligand field (E_LF) and the isotropic field/barycentre (E_iso).

CFSE (∆E_°) = {E_LF} – {E_iso}
= {[n_t2g(- 0.4) + n_eg(0.6)] Δ_° + n_p} – {n’_pP}

Here, n_t2g is the number of electrons in t_2g orbitals; neg is number of electrons in eg orbitals; n_p is number of electron pairs in the ligand field; & n’_P is the number of electron pairs in the isotropic field (barycentre).

Calculating the CFSE for the Iron Complexes

Complex: [Fe(H₂O)₆]³⁺

Complex: [Fe(CN)₆]^3-

Colour of the Complex and Crystal Field Splitting Energy:

Most of the transition metal complexes are coloured. A substance exhibits colour when it absorbs the light of a particular wavelength in the visible region and transmit the rest of the visible light. When this transmitted light enters our eye, our brain recognises its colour. The colour of the transmitted light is given by the complementary colour of the absorbed light.

For example, the hydrated copper (II) ion is blue in colour as it absorbs orange light, and transmit its complementary colour, blue. A list of absorbed wavelength and their complementary colour is given in the following table.

Wave length (λ) of absorbed light (Å)	Wave number (υ) of the absorbed light (cm-1)	Colour of absorbed light	Observed Colour
4000	25000	Violet	Yellow
4750	21053	Blue	Orange
5100	19608	Green	Red
5700	17544	Yellow	Violet
5900	16949	Orange	Blue
6500	15385	Red	Green

The observed colour of a coordination compound can be explained using crystal field theory. We learnt that the ligand field causes the splitting of d orbitals of the central metal atom into two sets (t_2g and e_g). When the white light falls on the complex ion, the central metal ion absorbs visible light corresponding to the crystal field splitting energy and transmits rest of the light which is responsible for the colour of the complex.

This absorption causes excitation of d-electrons of central metal ion from the lower energy t_2g level to the higher energy e_g level which is known as d-d transition.

Let us understand the d-d transitions by considering [Ti(H₂O)₆]³⁺ as an example. In this complex the central metal ion is Ti³⁺, which has d¹ configuration. This single electron occupies one of the t_2g orbitals in the octahedral aqua ligand field. When white light falls on this complex the d electron absorbs light and promotes itself to e_g level. The spectral data show the absorption maximum is at 20000 cm^-1 corresponding to the crystal field splitting energy (Δ_°) 239.7 kJ mol^-1.

The transmitted colour associated with this absorption is purple and hence ,the complex appears purple in colour. The octahedral titanium (III) complexes with other ligands such as bromide and flouride have different colours. This is due to the difference in the magnitude of crystal field splitting by these ligands (Refer page 156).

However, the complexes of central metal atom such as of Sc³⁺, Ti⁴⁺, Cu²⁺, Zn²⁺, etc are colourless. This is because the d-d transition is not possible in complexes with central metal having d^° or d¹⁰ configuration.

Metallic Carbonyls

Metal carbonyls are the transition metal complexes of carbon monoxide, containing MetalCarbon bond. In these complexes CO molecule acts as a neutral ligand. The first homoleptic carbonyl [Ni(CO)₄] nickel tetra carbonyl was reported by Mond in 1890. These metallic carbonyls are widely studied because of their industrial importance, catalytic properties and their ability to release carbon monoxide.

Classification:

Generally metal carbonyls are classifid in two different ways as described below.

(i) Classification Based on the Number of Metal Atoms Present.

Depending upon the number of metal atoms present in a given metallic carbonyl, they are classified as follows.

a. Mononuclear Carbonyls

These compounds contain only one metal atom, and have comparatively simple structures. For example, [Ni(CO)₄] – nickel tetracarbonyl is tetrahedral, [Fe(CO)₅] – Iron pentacarbonyl is trigonalbipyramidal, and [Cr(CO)₆] – Chromium hexacarbonyl is octahedral.

b. Poly Nuclear Carbonyls

Metallic carbonyls containing two or more metal atoms are called poly nuclear carbonyls. Poly nuclear metal carbonyls may be Homonuclear ([Co₂(CO)₈], [Mn₂(CO)₁₀], [Fe₃(CO)₁₂]) or hetero nuclear ([MnCo(CO)₉], [MnRe(CO)₁₀]) etc.

(ii) Classification Based on the Structure:

The structures of the binuclear metal carbonyls involve either metal-metal bonds or bridging CO groups, or both. The carbonyl ligands that are attached to only one metal atom are referred to as terminal carbonyl groups, whereas those attached to two metal atoms simultaneously are called bridging carbonyls. Depending upon the structures, metal carbonyls are classified as follows.

a. Non-Bridged Metal Carbonyls:

These metal carbonyls do not contain any bridging carbonyl ligands. They may be of two types.

(i) Non – bridged metal carbonyls which contain only terminal carbonyls.
Examples: [Ni(CO)₄], [Fe(CO)₅] and [Cr(CO)₆]

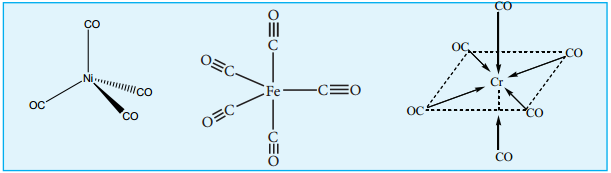
(ii) Non- bridged metal carbonyls which contain terminal carbonyls as well as Metal-Metal bonds. For examples, the structure of Mn₂(CO)₁₀ actually involve only a metal-metal bond, so the formula is more correctly represented as (CO)₅Mn – Mn(CO)₅.

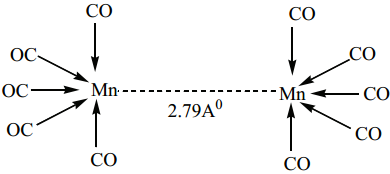
Other examples of this type are, Tc₂(CO)₁₀, and Re₂(CO)₁₀.

b. Bridged Carbonyls:

These metal carbonyls contain one or more bridging carbonyl ligands along with terminal carbonyl ligands and one or more Metal-Metal bonds. For example,

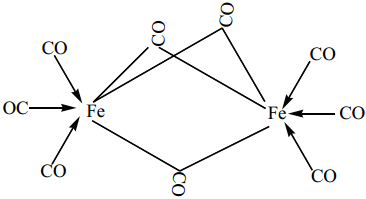
(i) The structure of Fe₂(CO)₉, di-iron nona carbonyl molecule consists of three bridging CO ligands, six terminal CO groups

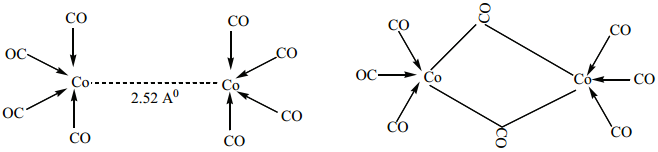
(ii) For dicobaltoctacarbonylCo₂(CO)₈ two isomers are possible. The one has a metal-metal bond between the cobalt atoms, and the other has two bridging CO ligands.

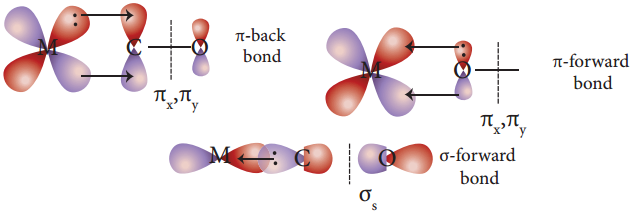
Bonding in Metal Carbonyls

In metal carbonyls, the bond between metal atom and the carbonyl ligand consists of two components. The first component is an electron pair donation from the carbon atom of carbonyl ligand into a vacant d-orbital of central metal atom.

This electron pair donation forms sigma bond. This sigma bond formation increases the electron density in metal d orbitals and makes the metal electron rich. In order to compensate for this increased electron density, a filled metal d-orbital interacts with the empty π* orbital on the carbonyl ligand and transfers the added electron density back to the ligand.

This second component is called π-back bonding. This in metal carbonyls, electron density moves from ligand to metal through sigma bonding and from metal to ligand through pi bonding, this synergic effect accounts for strong M ← O bond in metal carbonyls. This phenomenon is shown diagrammatically as follows.

Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.

Isomerism in Coordination Compounds

We have already learnt the concept of isomerism in the context of organic compounds, in the previous year  chemistry classes. Similarly, coordination compounds also exhibit isomerism. Isomerism is the phenomenon in which more than one coordination compounds having the same molecular formula have different physical and chemical properties due to different arrangement of ligands around the central metal atom. The following flow chart gives an overview of the common types of isomerism observed in coordination compounds,

Structural Isomers:

The coordination compounds with same formula, but have different connections among their constituent atoms are called structural isomers or constitutional isomers. Four common types of structural isomers are discussed below.

Linkage Isomers:

This type of isomers arises when an ambidentate ligand is bonded to the central metal atom/ion through either of its two different donor atoms. In the below mentioned examples, the nitrite ion is bound to the central metal ion Co³⁺ through a nitrogen atom in one complex, and through oxygen atom in other complex. [Co(NH₃)₅(NO₂)]²⁺

Coordination Isomers:

This type of isomers arises in the coordination compounds having both the cation and anion as complex ions. The interchange of one or more ligands between the cationic and the anionic coordination entities result in different isomers.

For example, in the coordination compound, [Co(NH₃)₆][Cr(CN)₆] the ligands ammonia and cyanide were bound respectively to cobalt and chromium while in its coordination isomer [Cr(NH₃)₆][Co(CN)₆] they are reversed.

Some more examples for coordination isomers

1. [Cr(NH₃)₅CN][Co(NH₃)(CN)₅] and [Co(NH₃)₅CN)] [Cr(NH₃)(CN)₅]
2. [Pt(NH₃)₄][Pd(Cl)₄] and [Pd(NH₃)₄][Pt(Cl)₄]

Ionisation Isomers:

This type of isomers arises when an ionisable counter ion (simple ion) itself can act as a ligand. The exchange of such counter ions with one or more ligands in the coordination entity will result in ionisation isomers. These isomers will give different ions in solution. For example, consider the coordination compound [Pt(en)₂Cl₂]Br₂.

In this compound, both Brand Cl^– have the ability to act as a ligand and the exchange of these two ions result in a different isomer [Pt(en)₂Br₂]Cl₂. In solution the first compound gives Br^– ions while the later gives Clions and hence these compounds are called ionisation isomers.

Some more example for the isomers,

1. [Cr(NH₃)₄ClBr]NO₂ and [Cr(NH₃)₄Cl NO₂]Br
2. [Co(NH₃)₄Br₂]Cl and [Co(NH₃)₄Cl Br] Br

Solvate Isomers:

The exchange of free solvent molecules such as water, ammonia, alcohol etc in the crystal lattice with a ligand in the coordination entity will give different isomers. These type of isomers are called solvate isomers. If the solvent molecule is water, then these isomers are called hydrate isomers. For example, the complex with chemical formula CrCl₃.6H₂O has three hydrate isomers as shown below.

Stereoisomers:

Similar to organic compounds, coordination compounds also exhibit stereoisomerism. The stereoisomers of a coordination compound have the same chemical formula and connectivity between the central metal atom and the ligands. But they differ in the spatial arrangement of ligands in three dimensional space. They can be further classified as geometrical isomers and optical isomers.

Geometrical Isomers:

Geometrical isomerism exists in heteroleptic complexes due to different possible three dimensional spatial arrangements of the ligands around the central metal atom. This type of isomerism exists in square planer and octahedral complexes. In square planar complexes of the form [MA₂B₂]ⁿ⁺ and [MA₂BC]ⁿ⁺ (where A, B and C are mono dentate ligands and M is the central metal ion/atom), Similar groups (A or B) present either on same side or on the opposite side of the central metal atom (M) give rise to two different geometrical isomers, and they are called, cis and trans isomers respectively.

The square planar complex of the type [M(xy)₂]ⁿ⁺ where xy is a bidentate ligand with two different coordinating atoms also shows cis-trans isomerism. Square planar complex of the form [MABCD]ⁿ⁺ also shows geometrical isomerism. In this case, by considering any one of the ligands (A, B, C or D) as a reference, the rest of the ligands can be arranged in three different ways leading to three geometrical isomers.

Figure 5.4 MA₂B₂MA₂BC M(xy)₂ MABCD – isomers

Octahedral Complexes:

Octahedral complexes of the type [MA₂B₄]ⁿ⁺, [M(xx)₂B₂]ⁿ⁺ shows cis-trans isomerism. Here A and B are monodentate ligands and xx is bidentate ligand with two same kind of donor atoms. In the octahedral complex, the position of ligands is indicated by the following numbering scheme.

In the above scheme, the positions (1, 2), (1, 3), (1, 4), (1, 5), (2, 3), (2, 5), (2, 6), (3, 4), (3, 6), (4, 5), (4, 6), and (5, 6) are identical and if two similar groups are present in any one of these positions, the isomer is referred as a cis isomer. Similarly, positions (1, 6), (2, 4), and (3, 5) are identical and if similar ligands are present in these positions it is referred as a trans-isomer.

Octahedral complex of the type [MA₃B₃]ⁿ⁺ also shows geometrical isomerism. If the three similar ligands (A) are present in the corners of one triangular face of the octahedron and the other three ligands (B) are present in the opposing triangular face, then the isomer is referred as a facial isomer (fac isomer) – Figure 5.6 (a).

If the three similar ligands are present around the meridian which is an imaginary semicircle from one apex of the octahedral to the opposite apex as shown in the figure 5.6(b), the isomer is called as a meridional isomer (mer isomer). This is called meridional because each set of ligands can be regarded as lying on a meridian of an octahedron.

As the number of different ligands increases, the number of possible isomers also increases. For the octahedral complex of the type [MABCDEF]ⁿ⁺, where A, B, C, D, E and F are monodentate ligands, fifteen different orientation are possible corresponding to 15 geometrical isomers. It is difficult to generate all the possible isomers.

Optical Isomerism

Coordination compounds which possess chairality exhibit optical isomerism similar to organic compounds. The pair of two optically active isomers which are mirror images of each other are called enantiomers. Their solutions rotate the plane of the plane polarised light either clockwise or anticlockwise and the corresponding isomers are called ‘d’ (dextro rotatory) and ‘l’ (levo rotatory) forms respectively. The octahedral complexes of type [M(xx)₃]ⁿ⁺, [M(xx)₂AB]ⁿ⁺ and [M(xx)₂B₂]ⁿ⁺ exhibit optical isomerism.

Examples:

The optical isomers of [Co(en)₃]³⁺ are shown in figure 5.7.

The coordination complex [CoCl₂(en)₂]⁺ has three isomers, two optically active cis forms and one optically inactive transform. These structures are shown below.

Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.

Nomenclature of Coordination Compounds

In the earlier days, the compounds were named after their discoverers. For example, K[PtCl₃(C₂H₄)] was called Zeise’s salt and [Pt(NH₃)₄][PtCl₄] is called Magnus’s green salt etc. There are numerous coordination compounds that have been synthesised and characterised.

The International Union of Pure and Applied Chemistry (IUPAC) has developed an elaborate system of nomenclature to name them systematically. The guidelines for naming coordination compounds based on IUPAC recommendations (2005) are as follows:

1. The cation is named first, followed by the anion regardless of whether the ion is simple or complex. For example

• In K₄[Fe(CN)₆], the cation K⁺ is named first followed by [Fe(CN)₆]^4-.
• In [Co(NH₃)₆]Cl₃, the complex cation [Co(NH₃)₆]³⁺ is named first followed by the anion Cl^–
• In [Pt(NH₃)₄][PtCl₄], the complex cation [Pt(NH₃)₄]²⁺ is named first followed by the complex anion [PtCl₄]^2-

2. The simple ions are named as in other ionic compounds. For example,

Simple Cation	Symbol	Simple Anion	Symbol
Sodium	Na+	Chloride	Cl–
Potassium	K+	Nitrate	NO3–
Copper	Cu2+	Sulphate	SO42-

3. To name a complex ion, the ligands are named first followed by the central metal atom/ion. When a complex ion contains more than one kind of ligands they are named in alphabetical order.

a. Naming the ligands:

(i) The name of anionic ligands ends with the letter ‘o’ and the cationic ligand ends with ‘ium’. The neutral ligands are usually called with their molecular names with fewer exceptions namely, H₂O (aqua), CO (carbonyl), NH₃ (ammine) and NO (nitrosyl).

(ii) A κ-term is used to denote an ambidendate ligand in which more than one coordination mode is possible. For example, the ligand thiocyanate can bind to the central atom/ion, through either the sulphur or the nitrogen atom. In this ligand, if sulphur forms a coordination bond with metal then the ligand is named thiocyanato-κS and if nitrogen is involved, then it is named thiocyanato-κN.

(iii) If the coordination entity contains more than one ligand of a particular type, the multiples of ligand (2, 3, 4 etc…) is indicated by adding appropriate Greek prefixes (di, tri, tetra, etc…) to the name of the ligand. If the name of a ligand itself contains a Greek prefix (eg. ethylenediamine), use an alternate prefies (bis, tris, tetrakis etc..) to specify the multiples of such ligands. These numerical prefixes are not taken into account for alphabetising the name of ligands.

b. Naming the Central Metal:

In cationic/neutral complexes, the element name is used as such for naming the central metal atom/ion, whereas, a suffix ‘ate’ is used along with the element name in anionic complexes. The oxidation state of the metal is written immediately after the metal name using roman numerals in parenthesis.

Naming of coordination compounds using IUPAC guidelines.

Example 1:

More examples with names are given in the list below for better understanding of IUPAC Nomenclature:

Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.

Definition of Important Terms Pertaining to Co-Ordination Compounds

Coordination Entity:

Coordination entity is an ion or a neutral molecule, composed of a central atom, usually a metal and the array of other atoms or groups of atoms (ligands) that are attached to it. In the formula, the coordination entity is enclosed in square brackets. For example, in potassium ferrocyanide, K₄[Fe(CN)₆], the coordination entity is [Fe(CN)₆]^4-. In nickel tetracarbonyl, the coordination entity is [Ni(CO)₄].

Central Atom/Ion:

The central atom/ion is the one that occupies the central position in a coordination entity and binds other atoms or groups of atoms (ligands) to itself, through a coordinate covalent bond. For example, in K₄[Fe(CN)₆], the central metal ion is Fe²⁺. In the coordination entity [Fe(CN)₆]^4-, the Fe²⁺ accepts an electron pair from each ligand, CN^– and thereby forming six coordinate covalent bonds with them. since, the central metal ion has an ability to accept electron pairs, it is referred to as a Lewis acid.

Ligands:

The ligands are the atoms or groups of atoms bound to the central atom/ion. The atom in a ligand that is bound directly to the central metal atom is known as a donor atom. For example, in K₄[Fe(CN)₆]^4- the ligand is CN^– ion, but the donor atom is carbon and in [Co(NH₃)₆]Cl₃ the ligand is NH₃ molecule and the donor atom is nitrogen.

Coordination Sphere:

The complex ion of the coordination compound containing the central metal atom/ion and the ligands attached to it, is collectively called coordination sphere and are usually enclosed in square brackets with the net charge. The other ionisable ions, are written outside the bracket are called counter ions. For example, the coordination compound K₄[Fe(CN)₆] contains the complex ion [Fe(CN)₆]^4- and is referred as the coordination sphere. The other associated ion K⁺ is called the counter ion.

Coordination Polyhedron:

The three dimensional spacial arrangement of ligand atoms/ions that are directly attached to the central atom is known as the coordination polyhedron (or polygon). For example, in K₄[Fe(CN)₆], the coordination polyhedra is octrahedral. The coordination polyhedra of [Ni(CO)₄] is tetrahedral.

Coordination Number:

The number of ligand donor atoms bonded to a central metal ion in a complex is called the coordination number of the metal. In other words, the coordination number is equal to the number of σ-bonds between ligands and the central atom.

For example,

• In K₄[Fe(CN)₆], the coordination number of Fe²⁺ is 6.
• In [Ni(en)₃]Cl₂, the coordination number of Ni²⁺ is also 6. Here the ligand ‘en’ represents ethane-1,2-diamine (NH₂-CH₂-CH₂-NH₂) and it contains two donor atoms (Nitrogen).
• Each ligand forms two  coordination bonds with nickel. So,totally there are six coordination bonds between them.

Oxidation State (Number):

The oxidation state of a central atom in a coordination entity is defined as the charge it would bear if all the ligands were removed along with the electron pairs that were shared with the central atom. In naming a complex, it is represented by a Roman numeral.

For example, in the coordination entity [Fe(CN)₆]^4-, the oxidation state of iron is represented as (II). The net charge on the complex ion is equal to the sum the oxidation state of the central metal and the charge the on the ligands attached to it. Using this relation the oxidation number can be calculated as follows Net charge = (oxidation state of the central metal) + [(No. of ligands) × (charge on the ligand)]

Example 1:

In [Fe(CN)₆]^4-, let the oxidation number of iron is x:
The net charge: – 4 = x + 6 (-1) ⇒ x = +2

Example 2:

In [Co(NH₃)₅Cl]²⁺, let the oxidation number of cobalt is x:
The net charge: +2 = x + 5 (0) + 1 (-1) ⇒ x = +3

Types of Complexes:

The coordination compounds can be classified into the following types based on

• The net charge of the complex ion
• Kinds of ligands present in the coordination entity.

Classification based on the net charge on the complex:

A coordination compound in which the complex ion

(i) Carries a net positive charge is called a cationic complex. Examples: [Ag(NH₃)₂]⁺, [Co(NH₃)₆]³⁺,
[Fe(H₂)O₆]²⁺, etc

(ii) Carries a net negative charge is called an anionic complex. Examples: [Ag(CN)₂]^–, [Co(CN)₆]^3-,
[Fe(CN)₆]^4-, etc

(iii) Bears no net charge, is called a neutral complex. Examples: [Ni(CO)₄], [Fe(CO)₅], [Co(NH₃)₃(Cl₃)].

Classification Based on Kind of Ligands:

A coordination compound in which

(i) The central metal ion/atom is coordinated to only one kind of ligands is called a homoleptic complex.
Examples: [Co(NH₃)₆]³⁺, [Fe(H₂O)₆]²⁺.

(ii) The central metal ion/atom is coordinated to more than one kind of ligands is called a heteroleptic complex. Example, [Co(NH₃)₅Cl]²⁺, [Pt(NH₃)₂Cl₂)].

Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.

Werner’s theory of Coordination Compounds

Swiss chemist Alfred Werner was the first one to propose a theory of coordination compounds to explain the observed behaviour of them. Let us consider the different coloured complexes of cobalt (III) chloride with ammonia which exhibit different properties as shown below.

In this case, the valences of the elements present in both the reacting molecules, cobalt (III) chloride and ammonia are completely satisfied. Yet these substances react to form the above mentioned complexes.

To explain this behaviour Werner postulated his theory as follows:

1. Most of the elements exhibit, two types of valence namely primary valence and secondary valence and each element tend to satisfy both the valences. In modern terminology, the primary valence is referred as the oxidation state of the metal atom and the secondary valence as the coordination number. For example, according to Werner, the primary and secondary valences of cobalt are 3 and 6 respectively.

2. The primary valence of a metal ion is positive in most of the cases and zero in certain cases. They are always satisfied by negative ions. For example in the complex CoCl₃.6NH₃, The primary valence of Co is +3 and is satisfied by 3Cl^– ions.

3. The secondary valence is satisfied by negative ions, neutral molecules, positive ions or the combination of these. For example, in CoCl₃.6NH₃ the secondary valence of cobalt is 6 and is satisfied by six neutral ammonia molecules, whereas in CoCl₃.5NH₃ the secondary valence of cobalt is satisfied by five neutral ammonia molecules and a Cl^– ion.

4. According to Werner, there are two spheres of attraction around a metal atom/ion in a complex. The inner sphere is known as coordination sphere and the groups present in this sphere are firmly attached to the metal. The outer sphere is called ionisation sphere. The groups present in this sphere are loosely bound to the central metal ion and hence can be separated into ions upon dissolving the complex in a suitable solvent.

1. The primary valences are non-directional while the secondary valences are directional. The geometry of the complex is determined by the spacial arrangement of the groups which satisfy the secondary valence. For example, if a metal ion has a secondary valence of six, it has an octahedral geometry. If the secondary valence is 4, it has either tetrahedral or square planar geometry.

The following table illustrates the Werner’s postulates.

Limitations of Werner’s Theory:

Even though, Werner’s theory was able to explain a number of properties of coordination compounds, it does not explain their colour and the magnetic properties.

Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.

Important Compound of Transition Elements

Oxides and Oxoanions of Metals

Generally, transition metal oxides are formed by the reaction of transition metals with molecular oxygen at high temperatures. Except the first member of 3d series, Scandium, all other transition elements form ionic metal oxides. The oxidation number of metal in metal oxides ranges from +2 to +7. As the oxidation number of a metal increases, ionic character decreases, for example, Mn₂O₇ is covalent.

Mostly higher oxides are acidic in nature, Mn₂O₇ dissolves in water to give permanganic acid (HMnO₄), similarly CrO₃ gives chromic acid (H₂CrO₄) and dichromic acid (H₂Cr₂O₇). Generally lower oxides may be amphoteric or basic, for example, Chromium (III) oxide – Cr₂O₃, is amphoteric and Chromium(II) oxide, CrO, is basic in nature.

Potassium Dichromate K₂Cr₂O₇

Preparation:

Potassium dichromate is prepared from chromate ore. The ore is concentrated by gravity separation. It is then mixed with excess sodium carbonate and lime and roasted in a reverbratory furnace.

The roasted mass is treated with water to separate soluble sodium chromate from insoluble iron oxide. The yellow solution of sodium chromate is treated with concentrated sulphuric acid which converts sodium chromate into sodium dichromate.

The above solution is concentrated to remove less soluble sodium sulphate. The resulting solution is filtered and further concentrated. It is cooled to get the crystals of Na₂SO₄.2H₂O.

The saturated solution of sodium dichromate in water is mixed with KCl and then concentrated to get crystals of NaCl. It is filtered while hot and the filtrate is cooled to obtain K₂Cr₂O₇ crystals.

Physical Properties:

Potassium dichromate is an orange red crystalline solid which melts at 671K and it is moderately soluble in cold water, but very much soluble in hot water. On heating it decomposes and forms Cr₂O₃ and molecular oxygen. As it emits toxic chromium fumes upon heating, it is mainly replaced by sodium dichromate.

Structure of Dichromate Ion:

Both chromate and dichromate ion are oxo anions of chromium and they are moderately strong oxidizing agents. In these ions chromium is in +6 oxidation state. In an aqueous solution, chromate and dichromate ions can be interconvertible, and in an alkaline solution chromate ion is predominant, whereas dichromate ion becomes predominant in acidic solutions. Structures of these ions are shown in the figure.

Chemical Properties:

1. Oxidation

Potassium dichromate is a powerful oxidising agent in acidic medium. Its oxidising action in the presence of H⁺ ions is shown below. You can note that the change in the oxidation state of chromium from Cr⁶⁺ to Cr³⁺. Its oxidising action is shown below.

Cr₂O₇^2- + 14H⁺ + 6e^– → 2Cr³⁺ + 7H₂O

The oxidising nature of potassium dichromate (dichromate ion) is illustrated in the following examples.

(i) It oxidises ferrous salts to ferric salts.

Cr₂O₇^2- + 6Fe²⁺ + 14H⁺ → 2Cr³⁺ + 6Fe³⁺ + 7H₂O

(ii) It oxidises iodide ions to iodine

Cr₂O₇^2- + 6I^– + 14H⁺ → 2Cr³⁺ + 3I₂ + 7H₂O

(iii) It oxidises sulphide ion to sulphur

Cr₂O₇^2- + 3S^2- + 14H⁺ → 2Cr³⁺ + 3S + 7H₂O

(iv) It oxidises sulphur dioxide to sulphate ion

Cr₂O₇^2- + 3SO₂ + 2H⁺ → 2Cr³⁺ + 3SO^2-₄ + H₂O

(v) It oxidises stannous salts to stannic salt

Cr₂O₇^2- + 3Sn²⁺ + 14H⁺ → 2Cr³⁺ + 3Sn⁴⁺ + 7H₂O

(vi) It oxidises alcohols to acids.

2K₂Cr₂O₇ + 8H₂SO₄ + 3CH₃CH₂OH →
2K₂SO₄ + 2Cr₂(SO₄)₃ + 3CH₃COOH + 11H₂O

Chromyl Chloride Test:

When potassium dichromate is heated with any chloride salt in the presence of Conc H₂SO₄, orange red vapours of chromyl chloride (CrO₂Cl₂) is evolved. This reaction is used to confirm the presence of chloride ion in inorganic qualitative analysis.

The chromyl chloride vapours are dissolved in sodium hydroxide solution and then acidified with acetic acid and treated with lead acetate. A yellow precipitate of lead chromate is obtained.

CrO₂Cl₂ + 4NaOH → Na₂CrO₄ + 2NaCl + 2H₂O

Uses of Potassium Dichromate:

Some important uses of potassium dichromate are listed below.

1. It is used as a strong oxidizing agent.
2. It is used in dyeing and printing.
3. It used in leather tanneries for chrome tanning.
4. It is used in quantitative analysis for the estimation of iron compounds and iodides.

Potassium Permanganate – KMnO₄

Preparation:
Potassium permanganate is prepared from pyrolusite (MnO₂) ore. The preparation involves the following steps.

(i) Conversion of MnO₂ to potassium manganate:

Powdered ore is fused with KOH in the presence of air or oxidising agents like KNO₃ or KClO₃. A green coloured potassium manganate is formed.

(ii) Oxidation of potassium manganate to potassium permanganate:

Potassium manganate thus obtained can be oxidised in two ways, either by chemical oxidation or electrolytic oxidation.

Chemical Oxidation:

In this method potassium manganate is treated with ozone (O₃) or chlorine to get potassium permanganate.

2MnO₄^2- + O₃ + H₂O → 2MnO₄^– + 2OH^– + O₂
2MnO₄^2- + Cl₂ → 2MnO₄^– + 2Cl^–

Electrolytic Oxidation

In this method aqueous solution of potassium manganate is electrolyzed in the presence of little alkali.

K₂MnO₄ ⇄ 2K⁺ + MnO₄^2-
H₂O ⇄ H⁺ + OH^–

Manganate ions are converted into permanganate ions at anode.

H₂ is liberated at the cathode.

2H⁺ + 2e^– → H₂↑

The purple coloured solution is concentrated by evaporation and forms crystals of potassium permanganate on cooling.

Physical Properties:

Potassium permanganate exists in the form of dark purple crystals which melts at 513 K. It is sparingly soluble in cold water but, fairly soluble in hot water.

Structure of Permanganate ion

Permanganate ion has tetrahedral geometry in which the central Mn⁷⁺ is sp³ hybridised.

Chemical Properties:

1. Action of Heat:

When heated, potassium permanganate decomposes to form potassium manganate and manganese dioxide.

2KMnO₄ → 2K₂MnO₄ + MnO₂ + O₂

2. Action of conc H₂SO₄

On treating with cold conc H₂SO₄, it decomposes to form manganese heptoxide, which subsequently decomposes explosively.

But with hot conc H₂SO₄, Potassium permanganate give MnSO_4. 

3. Oxidising Property:

Potassium permanganate is a strong oxidising agent, its oxidising action differs in different reaction medium.

(a) In neutral medium:

In neutral medium, it is reduced to MnO₂

MnO₄^– + 2H₂O + 3e^– → MnO₂ + 4OH^–

(i) It oxidises H₂S to sulphur

2MnO₄^– + 2H₂O + 3e^– → MnO₂ + 4OH^–

(ii) It oxidises thiosulphate into sulphate

8MnO₄^– + 3S₂O₃^2- + H₂O → 6SO₄^2- + 8MnO₂ + 2OH^–

(b) In alkaline medium:

In the presence of alkali metal hydroxides, the permanganate ion is converted into manganate.

MnO₄^– + e^– → MnO₄^2-

This manganate is further reduced to MnO2 by some reducing agents.

MnO₄^2- + H₂O → MnO₂ + 2OH^– + [O]

So the overall reaction can be written as follows.

MnO₄^– + 2H₂O + 3e^– → MnO₂ + 4OH^–

This reaction is similar as that for neutral medium.

Bayer’s Reagent:

Cold dilute alkaline KMnO₄ is known as Bayer’s reagent. It is used to oxidise alkenes into diols. For example, ethylene can be converted into ethylene glycol and this reaction is used as a test for unsaturation.

(c) In acid medium:

In the presence of dilute sulphuric acid, potassium permanganate acts as a very strong oxidising agent. Permanganate ion is converted into Mn²⁺ ion.

MnO₄^– + 8H⁺ + 5e^– → Mn²⁺ + 4H₂O

The oxidising nature of potassium permanganate (permanganate ion) in acid medium is illustrated in the following examples.

(i) It oxidises ferrous salts to ferric salts.

2MnO₄^– + 10Fe₂₊ + 16H⁺ → 2Mn²⁺ + 10Fe³⁺ + 8H₂O

(ii) It oxidises iodide ions to iodine

2MnO₄^– + 10 I^– + 16H⁺ → 2Mn²⁺ + 5I₂ + 8H₂O

(iii) It oxidises oxalic acid to CO₂

2MnO₄^– + 5(COO)^2-₂ + 16H⁺ → 2Mn²⁺ + 10CO₂ + 8H₂O

(iv) It oxidises sulphide ion to sulphur

2MnO^–₄ + 5 S^2- + 16H⁺ → 2Mn²⁺ + 5 S + 8H₂O

(v) It oxidises nitrites to nitrates

2MnO₄^– + 5 NO₂^– + 6H⁺ → 2Mn²⁺ + 5NO^–₃ + 3H₂O

(vi) It oxidises alcohols to aldehydes.

2KMnO₄ + 3H₂SO₄ + 5CH₃CH₂OH → K₂SO₄ + 2MnSO₄ + 5CH₃CHO + 8H₂O

(vii) It oxidises sulphite to sulphate

2MnO₄^– + 5SO₃^2- + 6H⁺ → 2Mn²⁺ + 5SO₄^2- + 3H₂O

Uses of Potassium Permanganate:

Some important uses of potassium permanganate are listed below.

1. It is used as a strong oxidizing agent.
2. It is used for the treatment of various skin infections and fungal infections of the foot.
3. It used in water treatment industries to remove iron and hydrogen sulphide from well water.
4. It is used as Bayer’s reagent for detecting unsaturation in an organic compound.
5. It is used in quantitative analysis for the estimation of ferrous salts, oxalates, hydrogen peroxide and iodides.

F-Block Elements – Inner Transition Elements

In the inner transition elements there are two series of elements.

1. Lanthanoids (previously called lanthanides)
2. Actinoids (previously called actinides)

Lanthanoid series consists of fourteen elements from Cerium (₅₈Ce) to Lutetium (₇₁Lu) following Lanthanum (₅₇La). These elements are characterised by the preferential filling of 4f orbitals, Similarly actinoids consists of 14 elements from Thrium (₉₀Th) to Lawrencium (₁₀₃Lr) following Actinium (₈₉Ac). These elements are characterised by the preferential filling of 5f orbital.

The position of Lanthanoids in the periodic table

The actual position of Lanthanoids in the periodic table is at group number 3 and period number 6. However, in the sixth period after lanthanum, the electrons are preferentially filled in inner 4f sub shell and these fourteen elements following lanthanum show similar chemical properties. Therefore these elements are grouped together and placed at the bottom of the periodic table. This position can be justified as follows.

1. Lanthanoids have general electronic configuration [Xe] 4f^1-14 5d^10-1 6s²
2. The common oxidation state of lanthanoides is +3
3. All these elements have similar physical and chemical properties.

Similarly the fourteen elements following actinium resemble in their physical and chemical properties. If we place these elements after Lanthanum in the periodic table below 4d series, the properties of the elements belongs to a group would be different and it would affect the proper structure of the periodic table. Hence a separate position is provided to the inner transition elements as shown in the figure.

Electronic Configuration of Lanthanoids:

We know that the electrons are filled in different orbitals in the order of their increasing energy in accordance with Aufbau principle. As per this rule after filling 5s, 5p and 6s and 4f level begin to fill from lanthanum, and hence the expected electronic configuration of Lanthanum(La) is [Xe] 4f¹ 5d° 6s² but the actual electronic configuration of Lanthanum is [Xe] 4f° 5d¹ 6s² and it belongs to d block.

Filling of 4f orbital starts from Cerium (Ce) and its electronic configuration is [Xe] 4f¹ 5d¹ 6s². As we move from Cerium to other elements the additional electrons are progressively filled in 4f orbitals as shown in the table.

Table: Electronic Configuration of Lanthanum and Lanthanoids

In Gadolinium (Gd) and Lutetium (Lu) the 4f orbitals, are half-filled and completely filled, and one electron enters 5d orbitals. Hence the general electronic configuration of 4f series of elements can be written as [Xe] 4f^1-14 5d^0-1 6s²

Oxidation State of Lanthanoids:

The common oxidation state of lanthanoids is +3. In addition to that some of the lanthanoids also show either +2 or +4 oxidation states. Gd³⁺ and Lu³⁺ ions have extra stability, it is due to the fact that they have exactly half filled and completely filled f-orbitals respectively their electronic configurations are

Gd³⁺: [Xe]4f⁷
Lu³⁺: [Xe]4f¹⁴

Similarly Cerium and terbium attain 4f° and 4f⁷ configurations respectively in the +4 oxidation states. Eu²⁺
and Yb²⁺ ions have exactly half filled and completely filled f orbitals respectively.

The stability of different oxidation states has an impact on the properties of these elements the following table shows the different oxidation states of lanthanoids.

Atomic and Ionic Radii:

As we move across 4f series, the atomic and ionic radii of lanthanoids show gradual decrease with increse in atomic number. This decrease in ionic size is called lanthanoid contraction.

Cause of Lanthanoid Contraction:

As we move from one element to another in 4f series (Ce to Lu) the nuclear charge increases by one unit and an additional electron is added into the same inner 4f sub shell. We know that 4f sub shell have a diffused shapes and therefore the shielding effect of 4f elelctrons relatively poor hence, with increase of nuclear charge, the valence shell is pulled slightly towards nucleus. As a result, the effective nuclear charge experienced by the 4f elelctorns increases and the size of Ln³⁺ ions decreases. Lanthanoid contraction of various lanthanoids is shown in the graph.

Consequences of Lanthanoid Contraction:

1. Basicity Differences

As we from Ce³⁺ to Lu³⁺, the basic character of Ln³⁺ ions decrease. Due to the decrease in the size of Ln³⁺ ions, the ionic character of Ln – OH bond decreases (covalent character increases) which results in the decrease in the basicity.

2. Similarities Among Lanthanoids:

In the complete f – series only 10 pm decrease in atomic radii and 20 pm decrease in ionic radii is observed because of this very small change in radii of lanthanoids, their chemical properties are quite similar.

The elements of the second and third transition series resemble each other more closely than the elements of the first and second transition series. For example

Series	Element	Atomic Radius
3d Series	Ti	132 pm
4d Series	Zr	145 pm
5d Series	Hf	144 pm

Actinoids:

The fourteen elements following actinium, i.e., from thorium (Th to lawrentium (Lr) are called actinoids. Unlike the lanthanoids, all the actinoids are radioactive and most of them have short half lives. Only thorium and uranium (U) occur in significant amount in nature and a trace amounts of Plutonium (Pu) is also found in Uranium ores. Neptunium (Np) and successive heavier elements are produced synthetically by the artificial transformation of naturally occuring elements by nuclear reactions. Similar to lanthanoids, they are placed at the bottom of the periodic table.

Electronic Configuration:

The electronic configuration of actinoids is not definite. The general valence shell electronic configuration of 5f elements is represented as [Rn]5f^1-146d^0-27s². The following table show the electronic configuration of actinoids.

Table: Electronic configuration of actinoids

Oxidation State of Actinoids:

Like lanthanoids, the most common state of actinoids is +3. In addition to that actinoids show variable oxidation states such as +2 , +3 , +4 ,+5,+6 and +7. The elements Americium(Am) and Thrium (Th show +2 oxidation state in some compounds, for example thorium iodide (ThI₂). The elements T, Pa, U, Np, Pu and Am show +5 oxidation states. Np and Pu exhibit +7 oxidation state.

Differences Between Lanthanoids and Actinoids:

Lanthanoids	Actinoids
1. Differentiating electron enters in 4f orbital	1. Differentiating electron enters in 5f orbital
2. Binding energy of 4f orbitals are higher	2. Binding energy of 5f orbitals are lower
3. They show less tendency to form complexes	3. They show greater tendency to form complexes
4. Most of the lanthanoids are colourless	4. Most of the actinoids are coloured. For example. U3+(red), U4+(green), UO22+(yellow)
5. They do not form oxo cations	5. They do form oxo cations such as UO22+, NPO22+ etc
6. Besides +3 oxidation states lanthanoids show +2 and +4 oxidation states in few cases	6. Besides +3 oxidation states actinoids show higher oxidation states such as +4, +5, +6 and +7

Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.

General Trend in Properties

Metallic Behaviour:

All the transition elements are metals. Similar to all metals the transition metals are good conductors of heat and electricity. Unlike the metals of Group-1 and group-2, all the transition metals except group 11 elements are hard. Of all the known elements, silver has the highest electrical conductivity at room temperature. Most of the transition elements are hexagonal close packed, cubic close packed or body centrered cubic which are the characteristics of true metals.

Figure 4.2 Lattice Structures of 3d, 4d, and 5d transistion metals

As we move from left to right along the transition metal series, melting point first increases as the number of unpaired d electrons available for metallic bonding increases, reach a maximum value and then decreases, as the d electrons pair up and become less available for bonding.

For example, in the first series the melting point increases from Scandium (m.pt 1814K) to a maximum of 2183 K for vanadium, which is close to 2180K for chromium. However, manganese in 3d series and Tc in 4d series have low melting point. The maximum melting point at about the middle of transition metal series indicates that d⁵ configuration is favourable for strong interatomic attraction. The following figure shows the trends in melting points of transition elements.

Variation of Atomic and Ionic Size:

It is generally expected a steady decrease in atomic radius along a period as the nuclear charge increases and the extra electrons are added to the same sub shell. But for the 3d transition elements, the expected decrease in atomic radius is observed from Sc to V, thereafter up to Cu the atomic radius nearly remains the same.

As we move from Sc to Zn in 3d series the extra electrons are added to the 3d orbitals, the added 3d electrons only partially shield the increased nuclear charge and hence the effective nuclear charge increases slightly. However, the extra electrons added to the 3d sub shell strongly repel the 4s electrons and these two forces are operated in opposite direction and as they tend to balance each other, it leads to constancy in atomic radii.

At the end of the series, d – orbitals of Zinc contain 10 electrons in which the repulsive interaction between the electrons is more than the effective nuclear charge and hence, the orbitals slightly expand and atomic radius slightly increases. Generally as we move down a group atomic radius increases, the same trend is expected in d block elements also. As the electrons are added to the 4d sub shell, the atomic radii of the 4d elements are higher than the corresponding elements of the 3d series.

However there is an unexpected observation in the atomic radius of 5d elements which have nearly same atomic radius as that of corresponding 4d elements. T is is due to lanthanoide contraction which is to be discussed later in this unit under inner transition elements.

Ionization Enthalpy:

Ionization energy of transition element is intermediate between those of s and p block elements. As we move from left to right in a transition metal series, the ionization enthalpy increases as expected. This is due to increase in nuclear charge corresponding to the filling of d electrons. The following figure show the trends in ionisation enthalpy of transition elements.

The increase in first ionisation enthalpy with increase in atomic number along a particular series is not regular. The added electron enters (n-1)d orbital and the inner electrons act as a shield and decrease the effect of nuclear charge on valence ns electrons. Therefore, it leads to variation in the ionization energy values.

The ionisation enthalpy values can be used to predict the thermodynamic stability of their compounds. Let us compare the ionisation energy required to form Ni²⁺ and Pt²⁺ ions.

For Nickel, IE₁ + IE₂
= (735 + 1753)
= 2490 kJmol^-1

For Platinum, IE₁ + IE₂
= (864 + 1791)
= 2655 kJmol^-1

Since, the energy required to form Ni²⁺ is less than that of Pt²⁺, Ni(II) compounds are thermodynamically more stable than Pt(II) compounds.

Oxidation State:

The first transition metal Scandium exhibits only +3 oxidation state, but all other transition elements exhibit variable oxidation states by loosing electrons from (n-1)d orbital and ns orbital as the energy difference between them is very small. Let us consider the 3d series; the following table summarizes the oxidation states of the 3d series elements.

At the beginning of the series, +3 oxidation state is stable but towards the end +2 oxidation state becomes stable. The number of oxidation states increases with the number of electrons available, and it decreases as the number of paired electrons increases.

Hence, the first and last elements show less number of oxidation states and the middle elements with more number of oxidation states. For example, the first element Sc has only one oxidation state +3; the middle element Mn has six different oxidation states from +2 to +7. The last element Cu shows +1 and +2 oxidation states only.

The relative stability of different oxidation states of 3d metals is correlated with the extra stability of half filled and fully filled electronic confiurations. Example: Mn²⁺(3d⁵) is more stable than Mn⁴⁺(3d³)

The oxidation states of 4d and 5d metals vary from +3 for Y and La to +8 for Ru and Os. The highest oxidation state of 4d and 5d elements are found in their compounds with the higher electronegative elements like O, F and Cl. for example: RuO₄, OsO₄ and WCl₆. Generally in going down a group, a stability of the higher oxidation state increases while that of lower oxidation state decreases.

It is evident from the Frost diagram (ΔG° vs oxidation number) as shown below. For titanium, vanadium and chromium, the most thermodynamically stable oxidation state is +3. For iron, the stabilities of +3 and +2 oxidation states are similar. Copper is unique in 3d series having a stable +1 oxidation state. It is prone to disproportionate to the +2 and 0 oxidation states.

Standard Electrode Potentials of Transition Metals

Redox reactions involve transfer of electrons from one reactant to another. Such reactions are always coupled, which means that when one substance is oxidised, another must be reduced. The substance which is oxidised is a reducing agent and the one which is reduced is an oxidizing agent. The oxidizing and reducing power of an element is measured in terms of the standard electrode potentials.

Standard electrode potential is the value of the standard emf of a cell in which molecular hydrogen under standard pressure (1 atm) and temperature (273 K) is oxidised to solvated protons at the electrode. If the standard electrode potential (E°), of a metal is large and negative, the metal is a powerful reducing agent, because it loses electrons easily. Standard electrode potentials (reduction potential) of few first transition metals are given in the following table.

In 3d series as we move from Ti to Zn, the standard reduction potential (\(E^{0}{ }_{M^{2+}} /_{M}\)) value is approaching towards less negative value and copper has a positive reduction potential. i.e., elemental copper is more stable than Cu²⁺.

There are two deviations., In the general trend, Fig shows that (\(E^{0}{ }_{M^{2+}} /_{M}\)) value for manganese and zinc are more negative than the regular trend. It is due to extra stability which arises due to the half filled d⁵ configuration in Mn²⁺ and completely filled d¹⁰ configuration in Zn²⁺.

Transition metals in their high oxidation states tend to be oxidizing. For example, Fe³⁺ is moderately a strong oxidant, and it oxidises copper to Cu²⁺ ions. The feasibility of the reaction is predicted from the following standard electrode potential values.

Fe³⁺(aq) + e^– ⇄ Fe²⁺ E° = 0.77V
Cu²⁺(aq) + 2e^– ⇄ Cu(s) E° = +0.34V

The standard electrode potential for the M³⁺/M²⁺ half-cell gives the relative stability between M³⁺ and M²⁺. The reduction potential values are tabulated as below.

The negative values for titanium, vanadium and chromium indicate that the higher oxidation state is preferred. If we want to reduce such a stable Cr³⁺ ion, strong reducing agent which has high negative value for reduction potential like metallic zinc (E° = – 0.76 V) is required.

The high reduction potential of Mn³⁺/Mn²⁺ indicates Mn²⁺ is more stable than Mn³⁺. For Fe³⁺/Fe²⁺ the reduction potential is 0.77V, and this low value indicates that both Fe³⁺ and Fe²⁺ can exist under normal conditions. The drop from Mn to Fe is due to the electronic structure of the ions concerned.

Mn³⁺ has a 3d⁴ configuration while that of Mn²⁺ is 3d⁵. The extra stability associated with a half filled d sub shell makes the reduction of Mn³⁺ very feasible (E° = +1.51V).

Magnetic Properties

Most of the compounds of transition elements are paramagnetic. Magnetic properties are related to the electronic configuration of atoms. We have already learnt in XI STD that the electron is spinning around its own axis, in addition to its orbital motion around the nucleus. Due to these motions, a tiny magnetic field is generated and it is measured in terms of magnetic moment. On the basis of magnetic properties, materials can be broadly classified as

• Paramagnetic Materials
• Diamagnetic materials, besides these there are ferromagnetic and antiferromagnetic materials.

Materials with no elementary magnetic dipoles are diamagnetic, in other words a species with all paired electrons exhibits diamagnetism. This kind of materials are repelled by the magnetic field because the presence of external magnetic field, a magnetic induction is introduced to the material which generates weak magnetic field that oppose the applied field.

Paramagnetic solids having unpaired electrons possess magnetic dipoles which are isolated from one another. In the absence of external magnetic field, the dipoles are arranged at random and hence the solid shows no net magnetism. But in the presence of magnetic field, the dipoles are aligned parallel to the direction of the applied field and therefore, they are attracted by an external magnetic field.

Ferromagnetic materials have domain structure and in each domain the magnetic dipoles are arranged. But the spin dipoles of the adjacent domains are randomly oriented. Some transition elements or ions with unpaired d electrons show ferromagnetism.

3d transition metal ions in paramagnetic solids often have a magnetic dipole moments corresponding to the electron spin contribution only. The orbital moment L is said to be quenched. So the magnetic moment of the ion is given by µ = g\(\sqrt{S(S+1)}\) µ_B

Where S is the total spin quantum number of the unpaired electrons and is µ_B Bohr Magneton. For an ion with n unpaired electrons S = \(\frac{n}{2}\) and for an electron g = 2.

Therefore the spin only magnetic moment is given by

The magnetic moment calculated using the above equation is compared with the experimental values in the following table. In most of the cases, the agreement is good.

Catalytic Properties

The chemical industries manufacture a number of products such as polymers, flavours, drugs etc., Most of the manufacturing processes have adverse effect on the environment so there is an interest for eco friendly alternatives. In this context, catalyst based manufacturing processes are advantageous, as they require low energy, minimize waste production and enhance the conversion of reactants to products.

Many industrial processes use transition metals or their compounds as catalysts. Transition metal has energetically available d orbitals that can accept electrons from reactant molecule or metal can form bond with reactant molecule using its d electrons. For example, in the catalytic hydrogenation of an alkene, the alkene bonds to an active site by using its π electrons with an empty d orbital of the catalyst.

The σ bond in the hydrogen molecule breaks, and each hydrogen atom forms a bond with a d electron on an atom in the catalyst. The two hydrogen atoms then bond with the partially broken π – bond in the alkene to form an alkane.

In certain catalytic processes the variable oxidation states of transition metals find applications. For example, in the manufacture of sulphuric acid from SO₃, vanadium pentoxide (V₂O₅) is used as a catalyst to oxidise SO₂. In this reaction V₂O₅ is reduced to vanadium (IV) Oxide (VO₂).

Some more examples are discussed below,

(i) Hydroformylation of Olefins

(ii) Preparation acetic acid from acetaldehyde.

(iii) Zeigler – Natta Catalyst

A mixture of TiCl₄ and trialkyl aluminium is used for polymerization.

Alloy Formation

An alloy is formed by blending a metal with one or more other elements. The elements may be metals or non metals or both. The bulk metal is named as solvent, and the other elements in smaller portions are called solute. According to Hume-Rothery rule to form a substitute alloy the difference between the atomic radii of solvent and solute is less than 15%.

Both the solvent and solute must have the same crystal structure and valence and their electro negativity difference must be close to zero. Transition metals satisfying these mentioned conditions form a number of alloys among themselves, since their atomic sizes are similar and one metal atom can be easily replaced by another metal atom from its crystal lattice to form an alloy. The alloys so formed are hard and often have high melting points. Examples: Ferrous alloys, gold – copper alloy, chrome alloys etc.

Formation of Interstitial Compounds

An interstitial compound or alloy is a compound that is formed when small atoms like hydrogen, boron, carbon or nitrogen are trapped in the interstitial holes in a metal lattice. They are usually non-stoichiometric compounds. Transition metals form a number of interstitial compounds such as TiC, ZrH_1.92, Mn₄N etc. The elements that occupy the metal lattice provide them new properties.

• They are hard and show electrical and thermal conductivity
• They have high melting points higher than those of pure metals
• Transition metal hydrides are used as powerful reducing agents
• Metallic carbides are chemically inert

Formation of Complexes

Transition elements have a tendency to form coordination compounds with a species that has an ability to donate an electron pair to form a coordinate covalent bond. Transition metal ions are small and highly charged and they have vacant low energy orbitals to accept an electron pair donated by other groups. Due to these properties, transition metals form large number of complexes. Examples: [Fe(CN)₆]^4-, [Co(NH₃)₆]³⁺, etc. The chemistry of coordination compound is discussed in unit 5.

Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.

Coordination Compounds and Double Salts

When two or more stable compounds in solution are mixed together and allowed to evaporate, in certain cases there is a possibility for the formation of double salts or coordination compounds. For example when an equimolar solution of ferrous sulphate and ammonium sulphate are mixed and allowed to crystallise, a double salt namely Mohr’s salt (Ferrous ammonium sulphate, FeSO₄.(NH₄)₂SO₄.6H₂O) is formed.

Let us recall the blood red colour formation in the inorganic qualitative analysis of ferric ion, the reaction between ferric chloride and potassium thiocyanate solution gives a blood red coloured coordination compound, potassium ferrithiocyanate K₃[Fe(SCN)₆].

If we perform a qualitative analysis to identify the constituent ions present in both the compounds, Mohr’s salt answers the presence of Fe²⁺, NH₄⁺ and SO₄^2- ions, whereas the potassium ferrithiocyanate will not answer Fe³⁺ and SCN^–ions. From this we can infer that the double salts lose their identity and dissociates into their constituent simple ions in solutions, whereas the complex ion in coordination compound, does not loose its identity and never dissociate to give simple ions.

Double salts and coordination compounds are complex compounds. The difference between double salt and coordination compound is that a double salt contains two salts with different crystal structures whereas a coordination compound contains a central metal ion surrounded by molecules or ions known as ligands.

A salt is essentially composed of an anion and a cation. But the main difference between a double salt and a complex salt is that a double salt is a combination of two salt compounds whereas a complex salt is a molecular structure that is composed of one or more complex ions.

A complex salt is a compound composed of a central metal atom having coordination bonds with ligands around it. Do not completely dissociate into its ions in water. It cannot be easily analyzed by determining the ions in the aqueous solution.

Both double salt as well sas complexes are formed by the combination of two or more stabel compounds in stoichiometic reatio. However they differ in the fact that double salt disssociate ito simple ions cmpletely with dissolved in water. However complex ions do not simple ions completely.

A double salt is a salt that contains more than one cation or more than one anion. Other examples include potassium sodium tartrate, ammonium iron (II) sulfate (Mohr’s salt), and bromlite. The fluorocarbonates contain fluoride and carbonate anions.

Double salts and coordination compounds are complex compounds. The difference between double salt and coordination compound is that a double salt contains two salts with different crystal structures whereas a coordination compound contains a central metal ion surrounded by molecules or ions known as ligands.

A double salt is formed from a three-component system, comprising two separate salts and water, and at a given temperature this may be represented by a triangular diagram. Phase diagram of a three-component system.

Double salts are addition compounds which lose their identity in aqueous solution whereas complexes which are also addition compounds do not lose their identity in aqueous solution.

In chemistry, a double bond is a covalent bond between two atoms involving four bonding electrons as opposed to two in a single bond. Double bonds occur most commonly between two carbon atoms, for example in alkenes. Other common double bonds are found in azo compounds (N=N), imines (C=N), and sulfoxides (S=O).

A complex salt is a salt that contains one or more complex ions – ions with metal centers and different molecules attached. Complex salts include potassium ferricyanide (used to create dyes and in blueprint paper) and potassium argentocyanide (used in silver plating).

The molecularity of the chemical reaction is equal to the sum of the stochiometric coefficients of the reactants in the chemical equation of the reaction. It is also defined as the number of reactant molecules taking part in a single step of the reaction.

Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.

Electronic Configuration – Detailed Explanation with Examples

We have already learnt in XI STD to write the electronic configuration of the elements using Aufbu principle, Hund’s rule etc. According to Aufbau principle, the electron first fills the 4s orbital before 3d orbital. Therefore filling of 3d orbital starts from Sc, its electronic configuration is [Ar]3d¹4s² and the electrons of successive elements are progressively filled in 3d orbital and the filling of 3d orbital is complete in Zinc, whose electronic configuration is [Ar]3d¹⁰4s².

However, there are two exceptions in the above mentioned progressive filling of 3d orbitals; if there is a chance of acquiring half filled or fully filled 3d sub shell, it is given priority as they are the stable configuration, for example Cr and Cu. The electronic configurations of Cr and Cu are [Ar] 3d⁵4s¹ respectively.

The extra stability of half filled and fully filled d orbitals, as already explained in XI STD, is due to symmetrical distribution of electrons and exchange energy. Note: The extra stability due to symmetrical distribution can also be visualized as follows. When the d orbitals are considered together, they will constitute a sphere.

So the half filled and fully filled configuration leads to complete symmetrical distribution of electron density. On the other hand, an unsymmetrical distribution of electron density as in the case of partially filled configuration will result in building up of a potential difference.

To decrease this and to achieve a tension free state with lower energy, a symmetrical distribution is preferred. With these two exceptions and minor variation in certain individual cases, the general electronic configuration of d – block elements can be written as [Noble gas] (n – 1)d^1-10 ns^1-2

To calculate an electron configuration, divide the periodic table into sections to represent the atomic orbitals, the regions where electrons are contained. Groups one and two are the s-block, three through 12 represent the d-block, 13 to 18 are the p-block and the two rows at the bottom are the f-block.

Electronic configuration, also called electronic structure, the arrangement of electrons in energy levels around an atomic nucleus. In terms of a more refined, quantum-mechanical model, the K-Q shells are subdivided into a set of orbitals (see orbital), each of which can be occupied by no more than a pair of electrons.

There are different orbital shapes (s, p, d, f) Each orbital can only hold 2 electrons max. There is a hierarchy, i.e. s orbitals will be filled before p orbitals which will be filled before d orbitals and so on. (s<p<d<f) (note, this is a general rule but there are exceptions).

The electron configuration is the standard notation used to describe the electronic structure of an atom. When assigning electrons to orbitals, we must follow a set of three rules: the Aufbau Principle, the Pauli Exclusion Principle, and Hund’s Rule.

If you are given with the atomic number of an element you can find it’s period number and group number. The period number is related to the number of electron occupied shells in the element and the period number is linked to its valence electrons.

There are two main exceptions to electron configuration: chromium and copper. In these cases, a completely full or half full d sub-level is more stable than a partially filled d sub-level, so an electron from the 4s orbital is excited and rises to a 3d orbital.

Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.

Position of D – Block Elements in the Periodic Table

We have already learnt the periodic classification of elements in XI std. the transition metals occupy from group – 3 to group – 12 of the modern periodic table.

Figure 4.1 – Position of d – block elements in the periodic table

D – Block elements composed of 3d series (4th period) Scandium to Zinc (10 elements), 4d series (5^th period) Yttrium to Cadmium (10 elements) and 5d series (6^th period) Lanthanum, Haffinium to mercury. As we know that the group-12 elements Zinc, Cadmium and Mercury do not have partially filled d-orbital either in their elemental state or in their normal oxidation states.

However they are treated as transition elements, because their properties are an extension of the properties of the respective transition elements. As per the IUPAC definition, the seventh period elements, starting from Ac, Rf to Cn also belong to transition metals. All of them are radioactive. Except Actinium; all the remaining elements are synthetically prepared and have very low half life periods.

The d-block elements are found in the middle of the period table. The d-block elements are called transition metals and have valence electrons in d orbital’s. The f-block elements,found in the two rows at the bottom of the periodic table, are called inner transition metals and have valence electrons in the f-orbital’s.

Transition elements are the elements that are found in Groups 3-12 (old groups IIA-IIB) on the periodic table (salmon-colored block in the middle of the table).

The Periodic Table provides a section for each of these groups of orbitals. The 10 electrons of the five d orbitals are filled by the elements found in the dropped central section of the table. This section is referred to as the d block elements, or the transition metals.

The d-block of the periodic table contains the elements of the groups 3-12 in which the d orbitals are progressively filled in each of the four long periods. The f-block consists of elements in which 4 f and 5 f orbitals are progressively filled. They are placed in a separate panel at the bottom of the periodic table.

The d-block elements are called transition elements because they exhibit transitional behaviour between s block and p-block elements. Their properties are transitional between highly reactive metallic elements of s block which are ionic in nature and the elements of p-block which are covalent in nature.

According to Aufbau principle , electrons first occupy the lowest energy orbital available to them and enter into higher energy orbitals only after the lower energy orbitals are filled. Therefore, 3d orbital is higher in energy than 4s. And hence electrons fill up in 4s before filling up in 3d.

The p sublevel has 3 orbitals, so can contain 6 electrons max. The d sublevel has 5 orbitals, so can contain 10 electrons max. And the 4 sublevel has 7 orbitals, so can contain 14 electrons max. In the picture below, the orbitals are represented by the boxes.

D-Block Elements:

Elements in which the last electron enters any one of the five d-orbitals of their respective penultimate shells are called d-block elements. The Importance of d-block Transition Metals. Transition metals, for the most part, are good conductors. They are also malleable, ductile, lustrous, and sliver-white in color. The d-block metals, and some of it’s key alloys, shaped the Bronze Age, Iron Age, and most importantly the steel age.

The p-block elements are found on the right side of the periodic table. They include the boron, carbon, nitrogen, oxygen and flourine families in addition to the noble gases. The noble gases have full p-orbital’s and are nonreactive.

In chemistry and atomic physics, the main group is the group of elements (sometimes called the representative elements) whose lightest members are represented by helium, lithium, beryllium, boron, carbon, nitrogen, oxygen, and fluorine as arranged in the periodic table of the elements.

Chlorine is in group 17 of periodic table, also called the halogens, and is not found as the element in nature – only as a compound. The most common of these being salt, or sodium chloride, and the potassium compounds sylvite (or potassium chloride) and carnallite (potassium magnesium chloride hexahydrate).

Find free online Chemistry Topics covering a broad range of concepts from research institutes around the world.

Group 18 (Inert gases) Elements

Occurrence:

All the noble gases occur in the atmosphere.

Physical Properties:

As we move along the noble gas elements, their atomic radius and boiling point increases from helium to radon. The first ionization energy decreases from helium to radon. Noble gases have the largest ionisation energy compared to any other elements in a given row as they have completely filled orbital in their outer most shell. They are extremely stable and have a small tendency to gain or lose electrons. The common physical properties of the group 18 elements are listed in the Table.

Physical Properties of group 18 Elements

Properties of Inert Gases:

Physical Properties:

Noble gases are monoatomic, odourless, colourless, tasteless, and non-inflammable. They are highly unreactive. They are non-metallic in nature.

Chemical Properties:

Only the xenon and krypton show some chemical reactivity. Xenon fluorides are prepared by direct reaction of xenon and fluorine under different conditions as shown below.

When XeF₆ is heated at 50°C in a sealed quartz vessel it forms XeOF₄.

When the reaction is continued the following reaction takes place.

2XeOF₄ + SiO₂ → 2XeO₂F₂ + SiF₄
2XeO₂F₂ + SiO₂ → 2XeO₃ + SiF₄

On hydrolysis with water vapour XeF₆ gives XeO₃

XeF₆ + 3H₂O → XeO₃ + 6HF

When XeF₆ reacts with 2.5 M NaOH, sodium per xenate is obtained.

2XeF₆ + 16NaOH → Na₄XeO₆ + Xe + O₂ + 12NaF + 8H₂O

Sodium per xenate is very much known for its strong oxidizing property. For example, it oxidises manganese (II) ion into permanganate ion even in the absence of the catalyst.

5XeO₆^4- + 2Mn²⁺ + 14H⁺ → 2MnO₄^– + 5XeO₃ + 7H₂O

Xenon reacts with PtF₆ and gave an orange yellow solid [XePtF₆] and this is insoluble in CCl₄.

Xenon difluoride forms addition compounds XeF₂.2SbF₅ and XeF₂.2TaF₅. Xenon
hexa fluorides forms compound with boron and alkali metals. Eg: XeF₆.BF₃, XeF₆MF, M-alkali metals.

There is some evidence for existence of xenon dichloride XeCl₂.

Krypton form krypton difluoride when an electric discharge is passed through Kr and flourine at 183°C or when gases are irradiated with SbF₅ it forms KrF₂.2SbF₃.

Structures of Compounds of Xenon:

Compound	Hybridaisation	Shape/Structure
XeF	sp3d	Linear
XeF4	sp3d2	Square planar
XeF6	sp3d3	Distorted octahedron
XeOF2	sp3d	T Shaped
XeOF4	sp3d2	Square pyramidal
XeO3	sp3	Pyramidal

Uses of Noble Gases:

The inertness of noble gases is an important feature of their practical uses.

Helium:

1. Helium and oxygen mixture is used by divers in place of air oxygen mixture. This prevents the painful dangerous condition called bends.
2. Helium is used to provide inert atmosphere in electric arc welding of metals.
3. Helium has lowest boiling point hence used in cryogenics (low temperature science)
4. It is much less denser than air and hence used for filing air balloons.

Neon:

Neon is used in advertisement as neon sign and the brilliant red glow is caused by passing electric current through neon gas under low pressure.

Argon:

Argon prevents the oxidation of hot filament and prolongs the life in filament bulbs

Krypton:

Krypton is used in florescent bulbs, flash bulbs etc. Lamps filed with krypton are used in airports as approaching lights as they can penetrate through dense fog.

Xenon:

Xenon is used in florescent bulbs, flash bulbs and lasers. Xenon emits an intense light in discharge tubes instantly. Due to this it is used in high speed electronic flash bulbs used by photographers.

Radon:

Radon is radioactive and used as a source of gamma rays. Radon gas is sealed as small capsules and implanted in the body to destroy malignant i.e. cancer growth.