Tag: Class 9 Maths RD Sharma Solutions

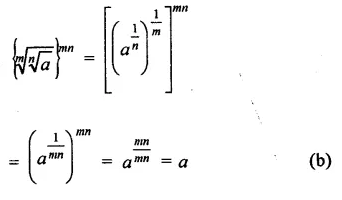
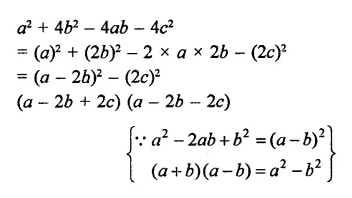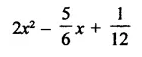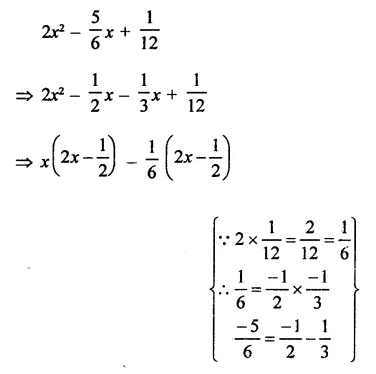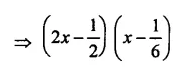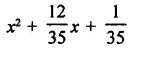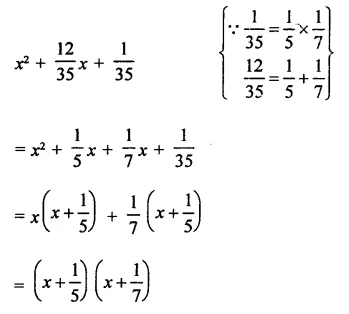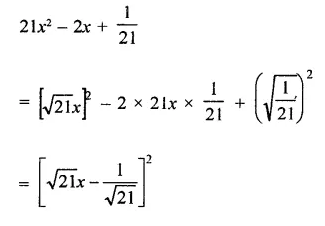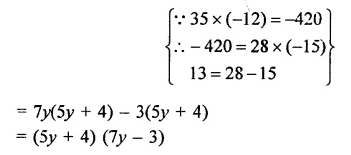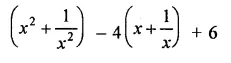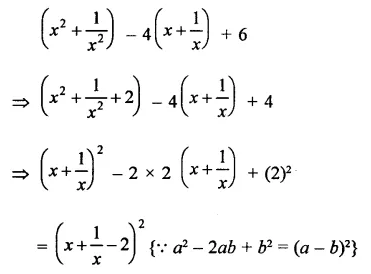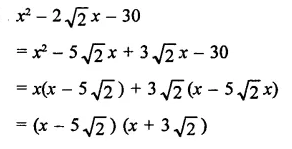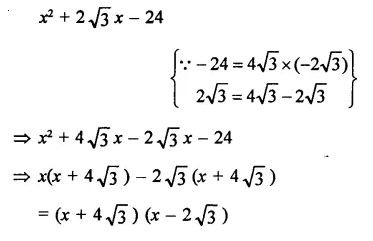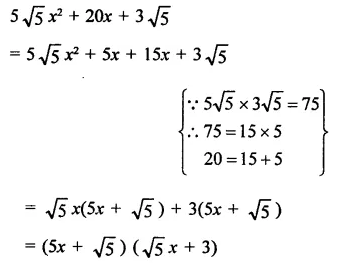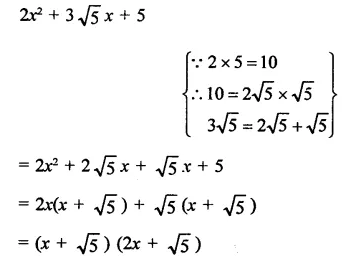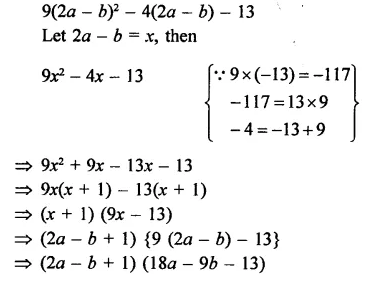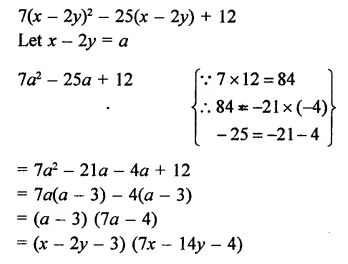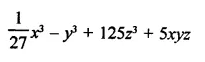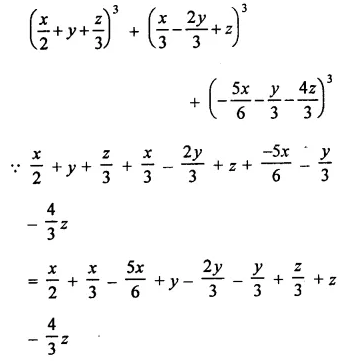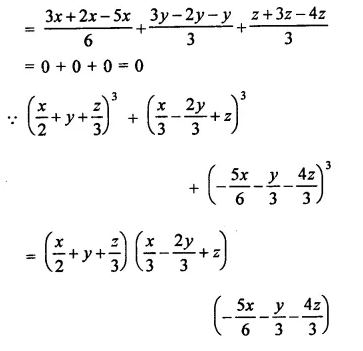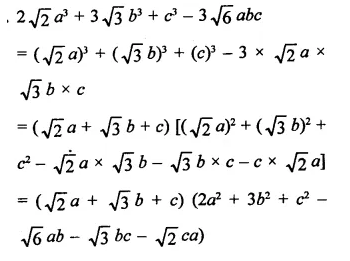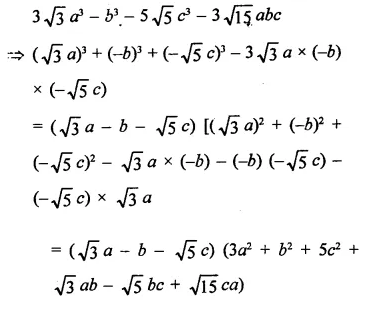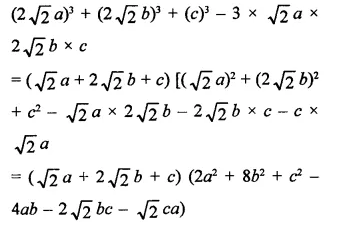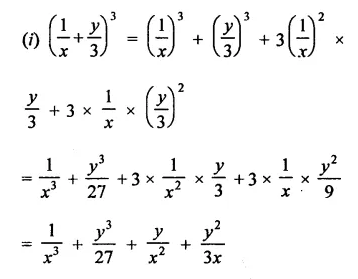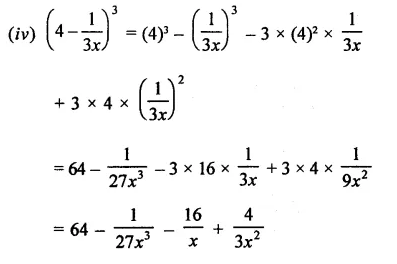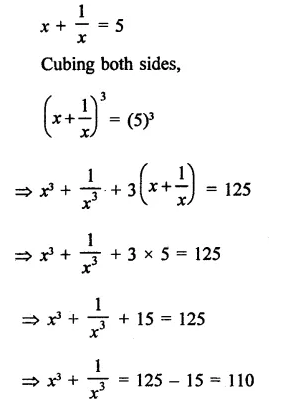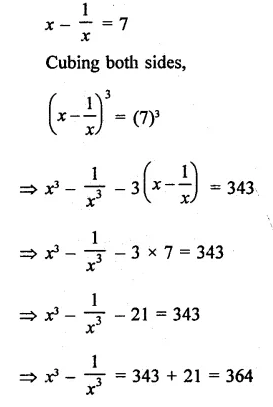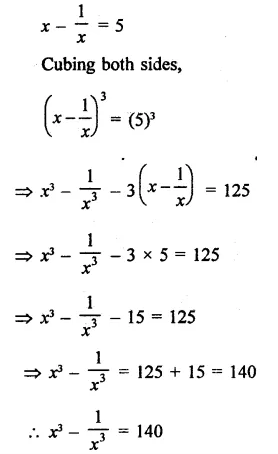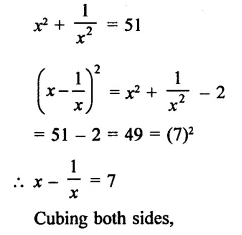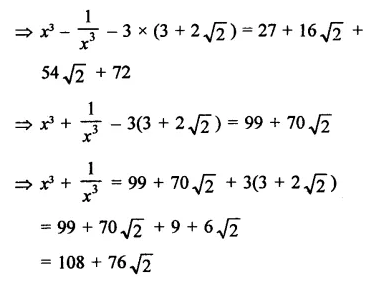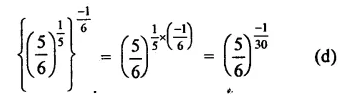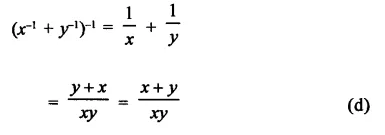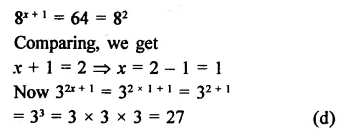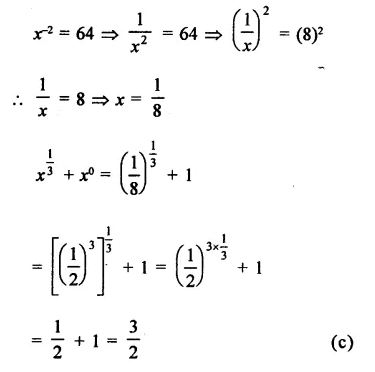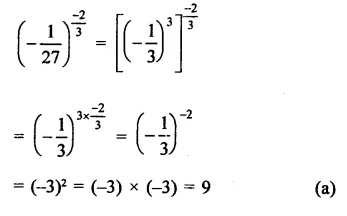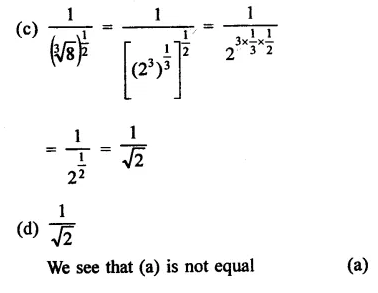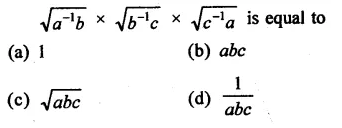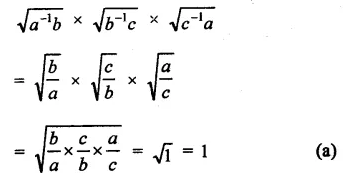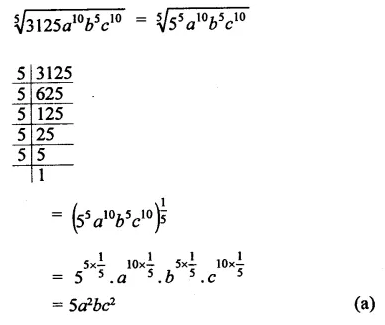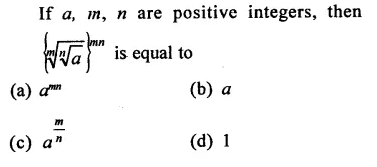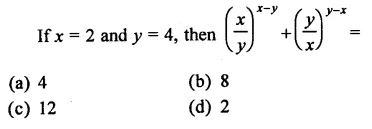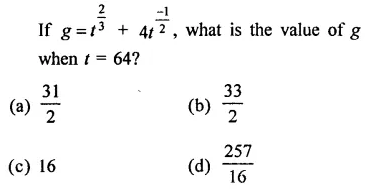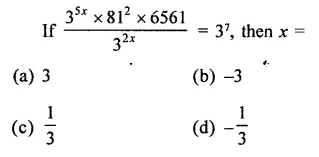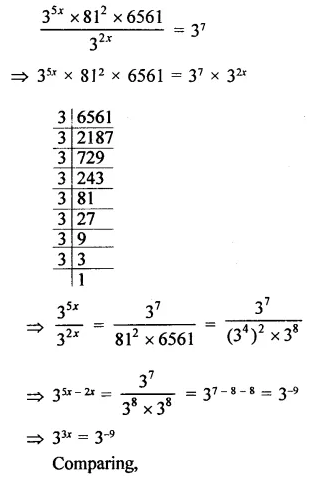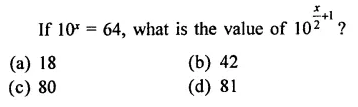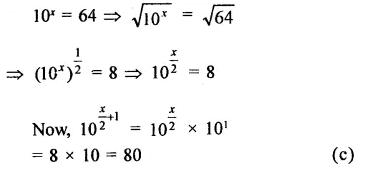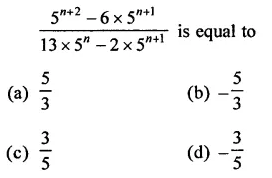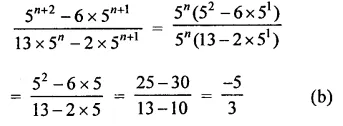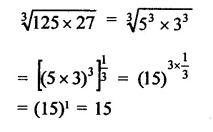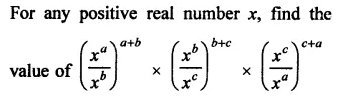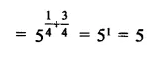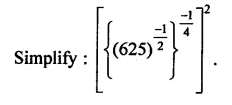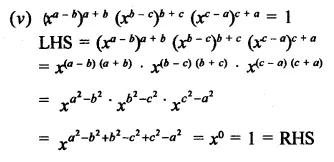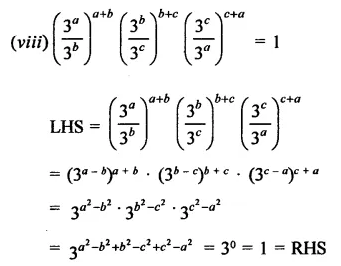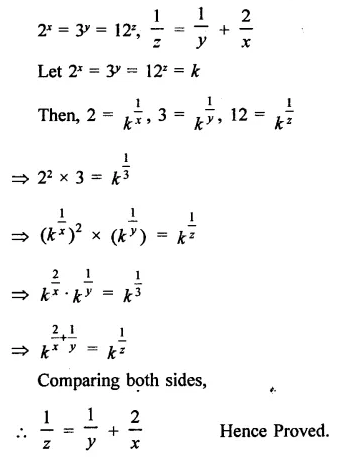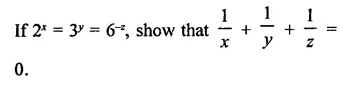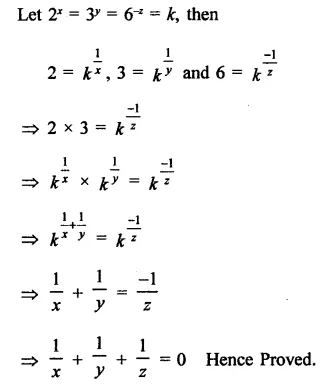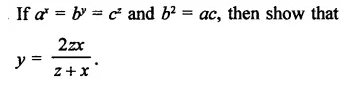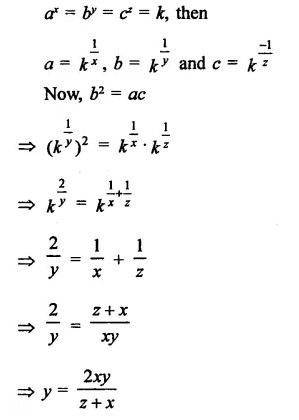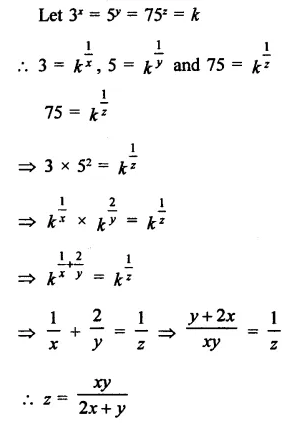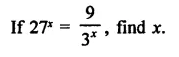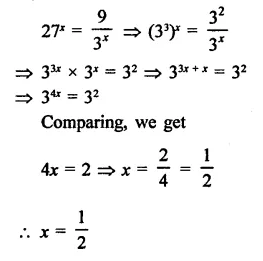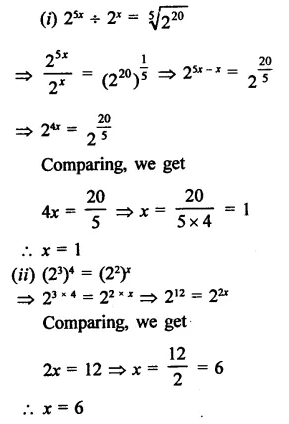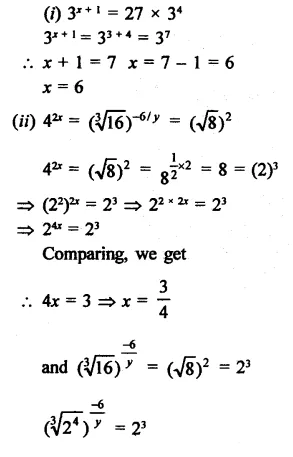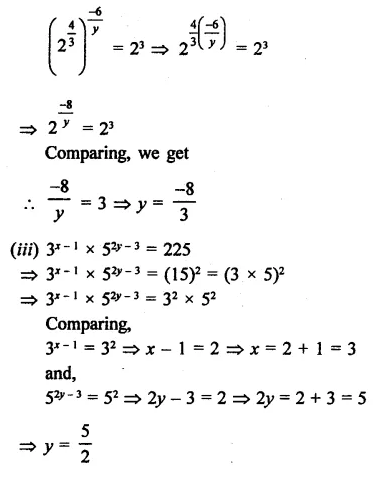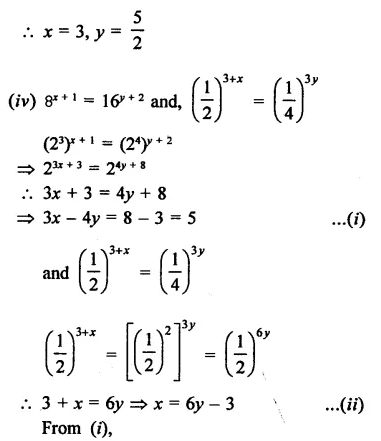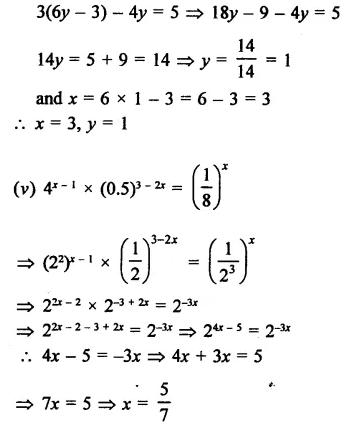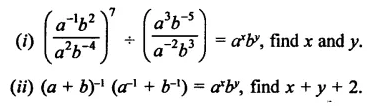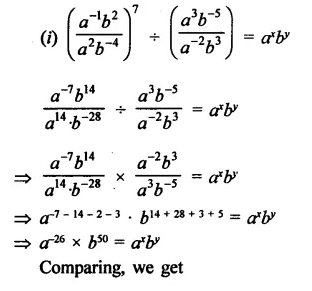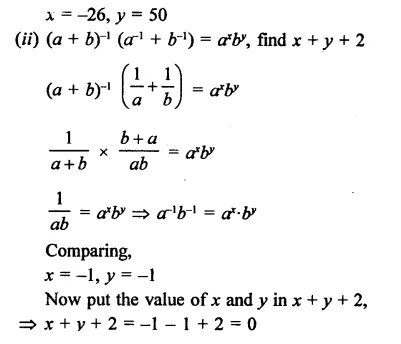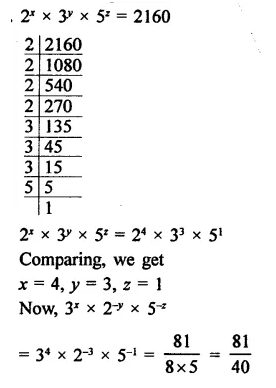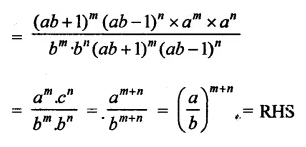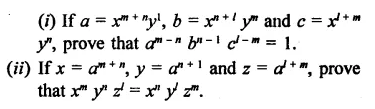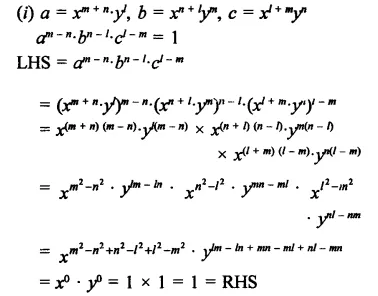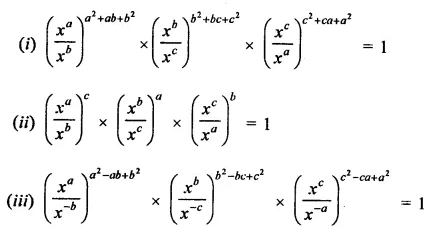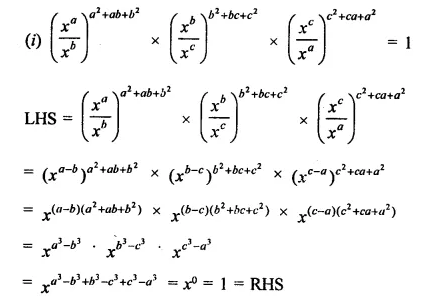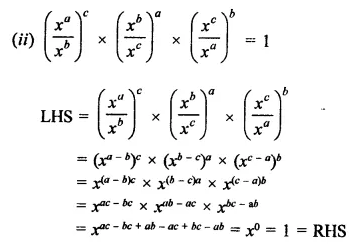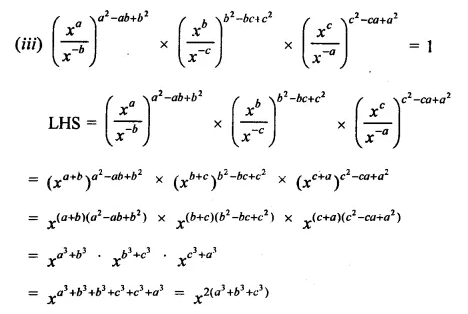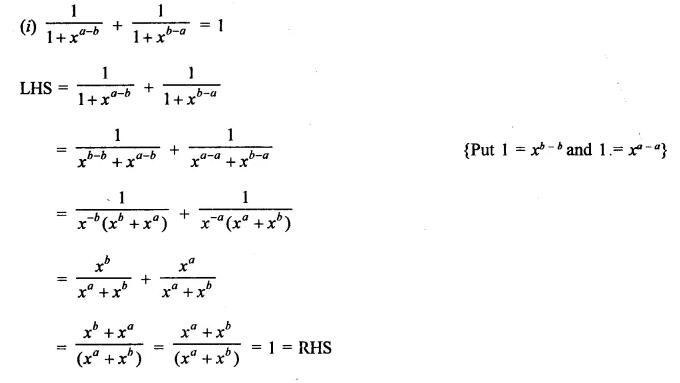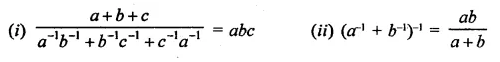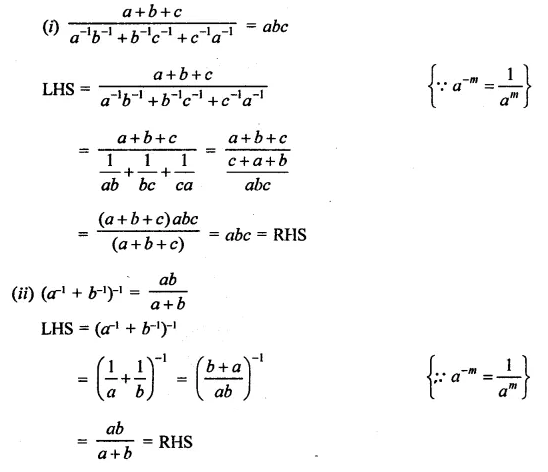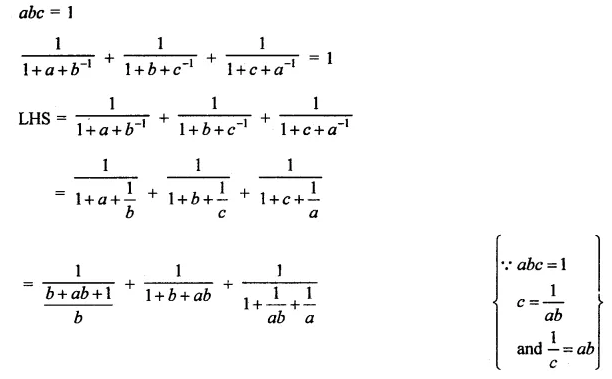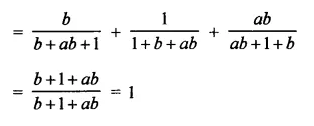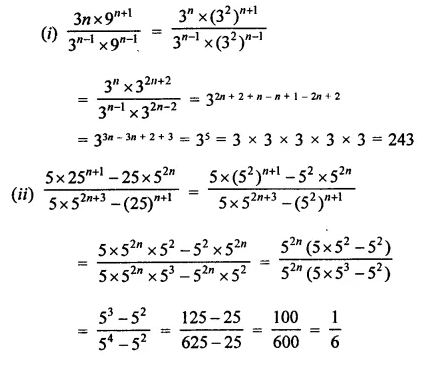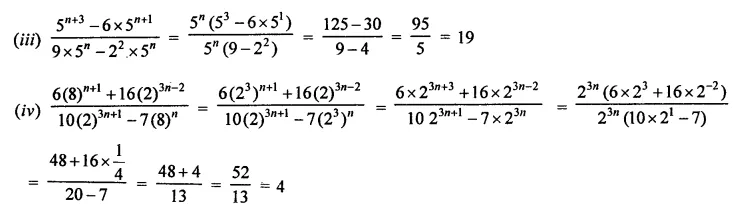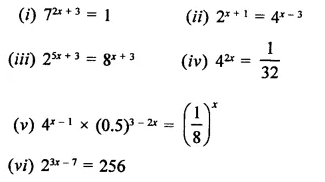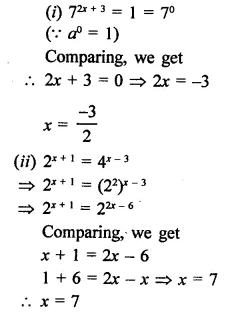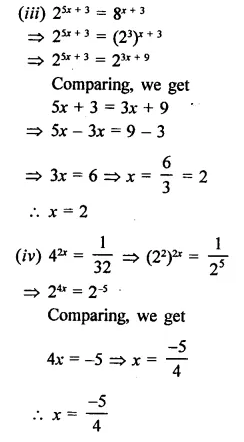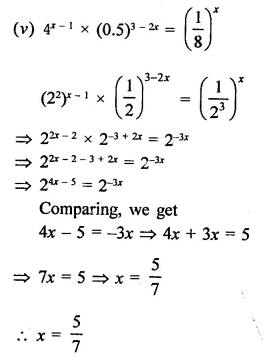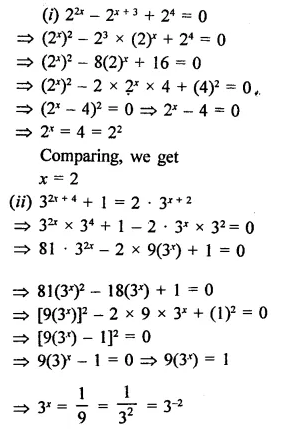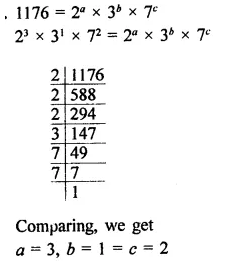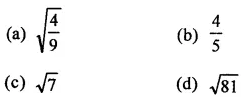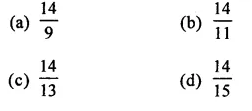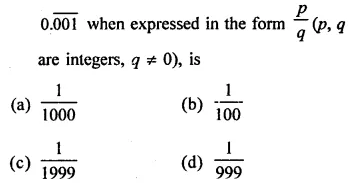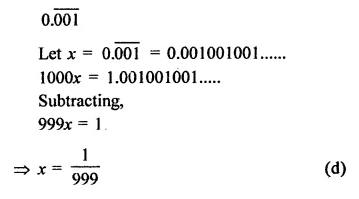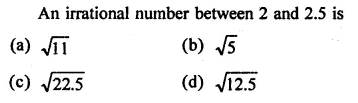RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions MCQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions MCQS

Other Exercises

• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.1
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.2
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.3
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.4
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions VSAQS
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions MCQS

Mark the correct alternative in each of the following:
Question 1.
The factors of x³ – x²y -xy² + y³ are
(a) (x + y) (x² -xy + y²)
(b) (x+y)(x² + xy + y²)
(c) (x + y)² (x – y)
(d) (x – y)² (x + y)
Solution:
x³ – x²y – xy² + y³
= x³ + y³ – x²y – xy²
= (x + y) (x² -xy + y²)- xy(x + y)
= (x + y) (x² – xy + y² – xy)
= (x + y) (x² – 2xy + y²)
= (x + y) (x – y)²         (d)

Question 2.
The factors of x³ – 1 +y³ + 3xy are
(a) (x – 1 + y)  (x² + 1 + y² + x + y – xy)
(b) (x + y + 1)  (x² + y² + 1- xy – x – y)
(c) (x – 1 + y)   (x² – 1 – y² + x + y + xy)
(d) 3(x + y – 1) (x² + y² – 1)
Solution:
x³ – 1 + y³ + 3xy
= (x)³ + (-1)³ + (y)³ – 3 x  x  x (-1) x y
= (x – 1 + y) (x² + 1 + y² + x + y – xy)
= (x- 1 + y) (x²+ 1 + y² + x + y – xy)      (a)

Question 3.
The factors of 8a³ + b³ – 6ab + 1 are
(a) (2a + b – 1) (4a² + b² + 1 – 3ab – 2a)
(b) (2a – b + 1) (4a² + b² – 4ab + 1 – 2a + b)
(c) (2a + b+1) (4a² + b² + 1 – 2ab – b – 2a)
(d) (2a – 1 + b)(4a² + 1 – 4a – b – 2ab)
Solution:
8a³ + b³ – 6ab + 1
= (2a)³ + (b)³ + (1)³ – 3 x 2a x b x 1
= (2a + b + 1) [(2a)² + b²+1-2a x b- b x 1 – 1 x 2a]
= (2a + b + 1) (4a² + b²+1-2ab-b- 2a)            (c)

Question 4.
(x + y)³ – (x – v)³ can be factorized as
(a) 2y (3x² + y²)                
(b) 2x (3x² + y²)
(c) 2y (3y² + x²)                
(d) 2x (x² + 3y²)
Solution:
(x + y)³ – (x – y)³
= (x + y -x + y) [(x + y)² + (x +y) (x -y) + (x – y)²]
= 2y(x² + y² + 2xy + x²-y² + x²+y² – 2xy)
= 2y(3x² + y²)          (a)

Question 5.
The expression (a – b)³ + (b – c)³ + (c – a)³ can be factorized as
(a) (a -b) (b- c) (c – a) 
(b) 3(a – b) (b – c) (c – a)
(c) -3(a – b) (b – c) (a – a)
(d) (a + b + c) (a² + b² + c² – ab – bc – ca)
Solution:
(a – b)³ + (b – c)³ + (c – a)³
Let a – b = x, b – a = y, c – a = z
∴ x³ + y³ + z³
x+y + z = a- b + b- c + c – a = 0
∴ x³ +y³ + z³ = 3xyz
(a – b)³ + (b – a)³ + (c – a)³
= 3 (a – b) (b – c) (c – a)        (b)

Question 6.

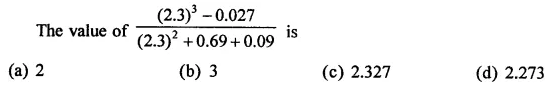
Solution:

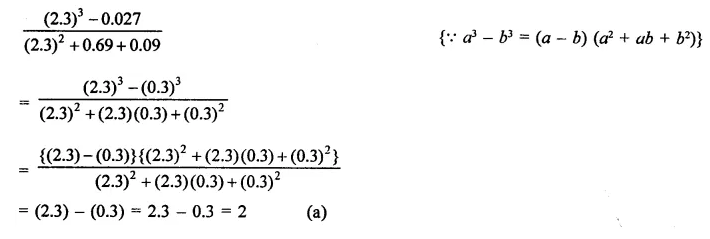
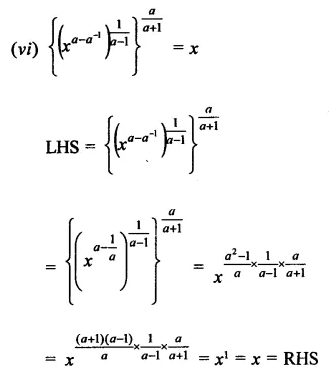
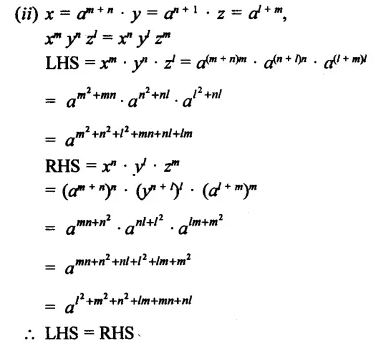
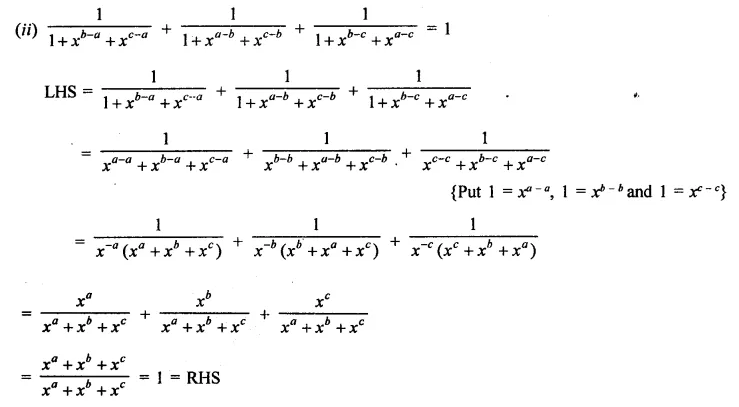
Question 7.

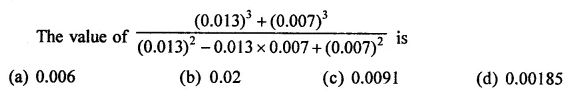
Solution:

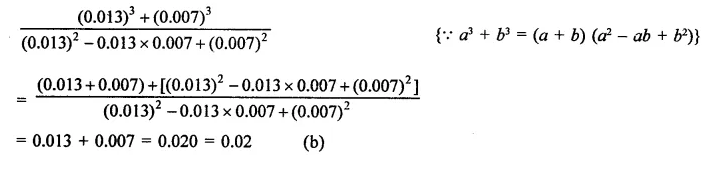
Question 8.
The factors of a² – 1 – 2x – x² are
(a) (a – x + 1) (a – x – 1)                                
(b) (a + x – 1) (a – x + 1)
(c) (a + x + 1) (a – x – 1)                               
(d) none of these
Solution:
a² – 1- 2x – x²
⇒ a² – (1 + 2x + x²)
= (a)² – (1 + x)²
= (a + 1 + x) (a – 1 – x)                         (c)

Question 9.
The factors of x⁴ + x² + 25 are
(a) (x² + 3x + 5) (x² – 3x + 5)                      
(b) (x² + 3x + 5) (x² + 3x – 5)
(c) (x² + x + 5) (x² – x + 5)                           
(d) none of these
Solution:
x⁴ + x² + 25 = x⁴ + 25 +x²
= (x²)² + (5)² + 2 x x² x 5- 9x²
= (x² + 5)² – (3x)²
= (x² + 5 + 3x) (x² + 5 – 3x)
= (x² + 3x + 5) (x² – 3x + 5)                 (a)

Question 10.
The factors of x² + 4y² + 4y – 4xy – 2x – 8 are
(a) (x – 2y – 4) (x – 2y + 2)                            
(b)  (x – y  +   2) (x – 4y – 4)
(c) (x + 2y – 4) (x + 2y + 2)                         
(d)    none of these
Solution:
x² + 4y² + 4y – 4xy – 2x – 8
⇒  x² + 4y + 4y – 4xy – 2x – 8
= (x)² + (2y)²– 2 x x x 2y + 4y-2x-8
= (x – 2y)² – (2x – 4y) – 8
= (x – 2y)² – 2 (x – 2y) – 8
Let x – 2y = a, then
a²– 2a – 8 = a²– 4a + 2a – 8
= a(a – 4) + 2(a – 4)
= (a-4) (a + 2)
= (x² -2y-4) (x² -2y + 2)                       (a)

Question 11.
The factors of x³ – 7x + 6 are
(a) x(x – 6) (x – 1)                                           
(b) (x² – 6) (x – 1)
(c) (x + 1) (x + 2) (x – 3)                               
(d) (x – 1) (x + 3) (x – 2)
Solution:
x³ -7x + 6= x³-1-7x + 7
= (x – 1) (x² + x + 1) – 7(x – 1)
= (x – 1) (x² + x + 1 – 7)
= (x – 1) (x² + x – 6)
= (x – 1) [x² + 3x – 2x – 6]
= (x – 1) [x(x + 3) – 2(x + 3)]
= (x – 1) (x+ 3) (x – 2)                           (d)

Question 12.
The expression x⁴ + 4 can be factorized as
(a) (x² + 2x + 2) (x² – 2x + 2)                       
(b) (x² + 2x + 2) (x² + 2x – 2)
(c) (x² – 2x – 2) (x² – 2x + 2)                         
(d) (x² + 2) (x² – 2)
Solution:
x⁴ + 4 = x⁴ + 4 + 4x² – 4x²                (Adding and subtracting 4x²)
= (x²)² + (2)² + 2 x x² x 2 – (2x)²
= (x² + 2)² – (2x)²
= (x² + 2 + 2x) (x² + 2 – 2x)                {∵ a² – b² = (a + b) (a – b)}
= (x² + 2x + 2) (x² – 2x + 2)                  (a)

Question 13.
If 3x = a + b + c, then the value of (x – a)³ + (x –    bf + (x – cf – 3(x – a) (x – b) (x – c) is
(a) a + b + c                                                
(b) (a – b) {b – c) (c – a)
(c) 0                                                                  
(d) none of these
Solution:
3x = a + b + c                                                                      .
⇒ 3x-a-b-c = 0
Now, (x – a)³+ (x – b)³ + (x – c)³ – 3(x – a) (x -b)  (x – c)
= {(x – a) + (x – b) + (x – c)} {(x – a)² + (x – b)² + (x – c)²  – (x – a) (x – b) (x – b) (x – c) – (x – c) (x – a)}
= (x – a + x – b + x – c) {(x – a)² + (x – b)²  + (x – c)² – (x – a) (x – b) – (x – b) (x – c) – (x – c) (x – a)}
= (3x – a – b -c) {(x – a)² + (x -b)²+ (x – c)² – (x – a) (x – b) – (x – b) (x – c) – (x – c) (x – a)}
But 3x-a-b-c = 0, then
= 0 x {(x – a)² + (x – b)² + (x – c)² – (x – a) (x – b) – (x – b) (x – c) – (x – c) (x – a)}
= 0                                                         (c)

Question 14.
If (x + y)³ – (x – y)³ – 6y(x² – y²) = ky², then k =
(a) 1                                   
(b) 2                                
(c) 4                                     
(d) 8
Solution:
(x + y)³ – (x – y)³ – 6y(x² – y²) = ky²
LHS = (x + y)³ – (x – y)³ – 3 x (x + y) (x – y) [x + y – x + y]
= (x+y-x + y)³       {∵ a³ – b³ – 3ab (a – b) = a³ – b³}
= (2y)³ = 8y³
Comparing with ky³, k = 8                     (d)

Question 15.
If x³ – 3x² + 3x – 7 = (x + 1) (ax² + bx + c), then a + b + c =
(a) 4                                   
(b) 12                             
(c) -10                                 
(d) 3
Solution:
x³ – 3x² + 3x + 7 = (x + 1) (ax² + bx + c)
= ax³ + bx² + cx + ax² + bx + c
x³ – 3x² + 3x – 7 = ax³ + (b + a)² + (c + b)x + c
Comparing the coefficient,
a = 1
b + a = -3 ⇒ b+1=-3 ⇒ b = -3-1=-4
c + b = 3 ⇒ c- 4 = 3 ⇒ c = 3 + 4 = 7
a + b + c = 1- 4 + 7 = 8- 4 = 4             (a)

Hope given RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions MCQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.1

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.1

Other Exercises

• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.1
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.2
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.3
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.4
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions VSAQS
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions MCQS

Factorize
Question 1.
x³ + x – 3x² – 3
Solution:
x³ + x – 3x² – 3
x³ – 3a² + x – 3
⇒  x²(x – 3) + 1(x – 3)
= (x – 3) (x² + 1)

Question 2.
a(a + b)³ – 3a²b(a + b)
Solution:
a(a + b)³ – 3a²b(a + b)
= a(a + b) {(a + b)² – 3ab}
= a(a + b) {a² + b² + 2ab – 3ab}
= a{a + b) {a² – ab + b²)

Question 3.
x(x³ – y³) + 3xy(x – y)
Solution:
x(x³ – y³) + 3xy(x – y)
= x(x – y) (x² + xy + y²) + 3xy(x – y)
= x(x – y) (x² + xy + y² + 3y)
= x(x – y) (x² + xy + y² + 3y)

Question 4.
a²x² + (ax² +1)x + a
Solution:
a²x² + (ax² + 1)x + a
= a²x² + a + (ax² + 1)x
= a(ax² + 1) + x(ax² + 1)
= (ax² + 1) (a + x)
= (x + a) (ax² + 1)

Question 5.
x² + y – xy – x
Solution:
x² + y – xy – x
= x²-x-xy + y = x(x- l)-y(*- 1)
= (x – 1) (x – y)

Question 6.
X³ – 2x²y + 3xy² – 6y³
Solution:
x³ – 2x²y + 3xy² – 6y³
= x²(x – 2y) + 3y²(x – 2y)
= (x – 2y) (x² + 3y²)

Question 7.
6ab – b² + 12ac – 2bc
Solution:
6ab – b² + 12ac – 2bc
= 6ab + 12ac – b² – 2bc
= 6a(b + 2c) – b(b + 2c)
= (b + 2c) (6a – b)

Question 8.
x(x – 2) (x – 4) + 4x – 8
Solution:
x(x – 2) (x – 4) + 4x – 8
= x(x – 2) (x – 4) + 4(x – 2)
= (x – 2) [x(x – 4) + 4]
= (x – 2) (x² – 4x + 4)
= (x – 2) [(x)² – 2 x x x 2 + (2)²]
= (x – 2) (x – 2)² = (x – 2)³

Question 9.
(a – b + c)² + (b – c + a)² + 2(a – b + c) (b – c + a)
Solution:
(a – b + c)² + ( b- c+a)² + 2(a – b + c) (b – c + a)      {∵ a² + b² + 2ab = (a + b)²}
= [a – b + c + b- c + a]²
= (2a)² = 4a²

Question 10.
a² + 2ab + b² – c²
Solution:
a² + 2ab + b² – c²
= (a² + 2ab + b²) – c²
= (a + b)² – (c)²         {∵  a² – b² = (a + b) (a – b)}
= (a + b + c) (a + b – c)

Question 11.
a² + 4b² – 4ab – 4c²
Solution:

Question 12.
x² – y² – 4xz + 4z²
Solution:
x² – y² – 4xz + 4z²
= x² – 4xz + 4z² – y²
= (x)² – 2 x x x 2z + (2z)² – (y)²
= (x – 2z)² – (y)²
= (x – 2z + y) (x – 2z – y)
= (x +y – 2z) (x – y – 2z)

Question 13.

Solution:

Question 14.

Solution:

Question 15.

Solution:

Question 16.
Give possible expression for the length and breadth of the rectangle having 35y² + 13y – 12 as its area.
Solution:
Area of a rectangle = 35y² + 13y – 12
= 35y² + 28y- 15y- 12

(i) If length = 5y + 4, then breadth = 7y – 3
(ii) and if length = 7y-3, then length = 5y+ 4

Question 17.
What are the possible expressions for the dimensions of the cuboid whose volume is 3x² – 12x.
Solution:
Volume 3x² – 12x
= 3x(x – 4)
∴ Factors are 3, x, and x – 4
Now, if length = 3, breadth = x and height = x – 4
if length =3, breadth = x – 4, height = x
if length = x, breadth = 3, height = x – 4
if length = x, breadth = x – 4, height = 3
if length = x – 4, breadth = 3, height = x
if length – x – 4, breadth = x, height = 3

Question 18.

Solution:

Question 19.
(x + 2) (x² + 25) – 10x² – 20x
Solution:
(x + 2) (x² + 25) – 10x² – 20x
= (x + 2) (x² + 25) – 10x(x + 2)
= (x + 2) [x² + 25 – 10x]
= (x + 2) [(x)² – 2 x x x 5 + (5)²]
= (x + 2) (x – 5)²

Question 20.
2a² + 2\(\sqrt { 6 } \) ab +3b²
Solution:
2a² + 2\(\sqrt { 6 } \)  ab +3 b²
= (\(\sqrt { 2 } \) a)²+ \(\sqrt { 2 } \) a x \(\sqrt { 3 } \) b+ (\(\sqrt { 3 } \) b)²
= (\(\sqrt { 2 } \)a + \(\sqrt { 3 } \) b)2

Question 21.
a² + b² + 2(ab + bc + ca)
Solution:
a² + b² + 2(ab + bc + ca)
= a² + b² + 2 ab + 2 bc + 2 ca
= (a + b)² + 2c(b + a)
= (a + b)² + 2c(a + b)
= (a + b) (a + b + 2c)

Question 22.
4(x – y)² – 12(x -y) (x + y) + 9(x + y)²
Solution:
4(x – y)² – 12(x – y) (x + y) + 9(x + y)²
= [2(x – y)² + 2 x 2(x – y) x 3(x + y) + [3 (x+y]²        {∵ a² + b² + 2 abc = (a + b)²}
= [2(x – y) + 3(x + y)]²
= (2x-2y + 3x + 3y)²
= (5x + y)²

Question 23.
a² – b² + 2bc – c²
Solution:
a² – b² + 2bc – c²
= a² – (b² – 2bc + c²)                                           {∵ a² + b² – 2abc = (a – b)²}
= a² – (b – c)²
= (a)² – (b – c)²          {∵ a² – b² = (a + b) (a – b)}
= (a + b – c) (a – b + c)

Question 24.
xy⁹ – yx⁹
Solution:
xy⁹ – yx⁹ = xy(y⁸ – x⁸)
= -xy(x⁸ – y⁸)
= -xy[(x⁴)² – (y⁴)²]
= -xy (x⁴ + y⁴) (x⁴ – y⁴)                                         {∵ a²-b² = (a + b) (a – b)}
= -xy (x⁴ + y⁴) {(x²)² – (y²)²}
= -xy(x⁴ + y⁴) (x² + y²) (x² – y²)
= -xy (x⁴ +y⁴) (x² + y²) (x + y) (x -y)
= -xy(x – y) (x + y) (x² + y²) (x⁴ + y⁴)

Question 25.
x⁴ + x²y² + y⁴
Solution:
x⁴ + x²y² + y⁴  = (x²)² + 2x²y² + y⁴ – x²y²           (Adding and subtracting x²y²)
= (x² + y²)² – (xy)²                                                        {∵ a² – b² = (a + b) (a – b)}
= (x² + y² + xy) (x² + y² – xy)
= (x² + xy + y²) (x² – xy + y²)

Question 26.
x² + 6\(\sqrt { 2 } \)x + 10
Solution:

Question 27.
x² + 2\(\sqrt { 2 } \)x- 30
Solution:

Question 28.
x² – \(\sqrt { 3 } \)x – 6
Solution:

Question 29.
x² + 5 \(\sqrt { 5 } \)x + 30
Solution:

Question 30.
x² + 2 \(\sqrt { 3 } \)x – 24
Solution:

Question 31.
5 \(\sqrt { 5 } \)x² + 20x + 3\(\sqrt { 5 } \)
Solution:

Question 32.
2x² + 3\(\sqrt { 5 } \) x + 5
Solution:

Question 33.
9(2a – b)² – 4(2a – b) – 13
Solution:

Question 34.
7(x-2y) – 25(x-2y) +12
Solution:

Question 35.
2(x+y) – 9(x+y) -5
Solution:
2(x+y) – 9(x+y) -5

Hope given RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.1 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.4

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.4

Other Exercises

• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.1
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.2
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.3
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.4
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions VSAQS
• RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions MCQS

Factorize each of the following expressions:
Question 1.
a³ + 8b³ + 64c³ – 24abc
Solution:
We know that
a³ + b³ + c³ – 3abc = (a + b + c) (a² + b² + c² – ab – bc – ca)
a³ + 8b³ + 64c³ – 24abc
= (a)³ + (2b)³ + (4c)³ – 3 x a x 2b x 4c
= (a + 2b + 4c) [(a)² + (2b)² + (4c)² -a x 2b – 2b x 4c – 4c x a]
= (a + 2b + 4c) (a² + 4b² + 16c² – 2ab – 8bc – 4ca)

Question 2.
x³ – 8y³ + 27z³ + 18xyz
Solution:
x³ – 8y³ + 27z³ + 18xyz
= (x)³ + (-2y)³ + (3z)³ – 3 x x x (-2y) (3 z)
= (x – y + 3z) (x² + 4y² + 9z² + 2xy + 6yz – 3zx)

Question 3.
27x³ – y³ – z³ – 9xyz          [NCERT]
Solution:
27x³-y³-z³-9xyz
= (3x)³ + (-y)³ + (-z)³ – 3 x 3x x (-y) (-z)
= (3x – y – z) [(3x)² + (-y)² + (-z)² – 3x x (-y) – (-y) (-z)-  (- z x 3x)]
= (3x-y – z) (9x² + y² + z² + 3xy – yz + 3zx)

Question 4.

Solution:

Question 5.
8x³ + 27y³ – 216z³ + 108xyz
Solution:
8x³ + 27y³ – 216z³ + 108xyz
= (2x)³ + (3y)³ + (6z)³ – 3 x (2x) (3y) (-6z)
= (2x + 3y – 6z) [(2x)² + (3y)² + (-6z)² – 2x x 3y – 3y x (-6z) – (-6z) x 2x]
= (2x + 3y – 6z) (4x² + 9² + 36z² – 6xy + 18yz + 12zx)

Question 6.
125 + 8x³ – 27y³ + 90xy
Solution:
125 + 8X³ – 27y³ + 90xy
= (5)³ + (2x)³ + (-3y)³ – [3 x 5 x 2x x (-3y)]
= (5 + 2x – 3y) [(5)² + (2x)² + (-3y)² – 5 x 2x – 2x (-3y) – (-3y) x 5]
= (5 + 2x – 3y) (25 + 4x² + 9y²– 10x + 6xy + 15y)

Question 7.
8x³ – 125y³ + 180xy + 216
Solution:
8x³ – 125y³ + 180xy + 216
= (2x)³ + (-5y)³ + (6)³ – 3 x 2x (-5y) x 6
= (2x – 5y + 6) [(2x)² + (-5y)² + (6)² – 2x x (-5y) – (-5y) x 6 – 6 x 2x]
= (2x -5y + 6) (4x² + 25y² + 36 + 10xy + 30y – 12x)

Question 8.
Multiply:
(i) x² +y² + z² – xy + xz + yz by x + y – z
(ii) x² + 4y² + z² + 2xy + xz – 2yz by x- 2y-z
(iii) x² + 4y² + 2xy – 3x + 6y + 9 by x – 2y + 3
(iv) 9x² + 25y² + 15xy + 12x – 20y + 16 by 3x  – 5y + 4
Solution:
(i)  (x² + y² + z² – xy + yz + zx) by (x + y – z)
= x³ +y³ – z³ + 3xyz
(ii) (x² + 4y² + z² + 2xy + xz – 2yz) by (x – 2y – z)
= (x -2y-z) [x² + (-2y)² + (-z)² -x x (- 2y) – (-2y) (z) – (-z) (x)]
= x³ + (-2y)³ + (-z)³ – 3x (-2y) (-z)
= x³ – 8y³ – z³ – 6xyz
(iii) x² + 4y² + 2xy – 3x + 6y + 9 by x – 2y + 3
= (x – 2y + 3) (x² + 4y² + 9 + 2xy + 6y – 3x)
= (x)³ + (-2y)³ + (3)³ – 3 x x x (-2y) x 3 = x³ – 8y³ + 27 + 18xy
(iv) 9x² + 25y³ + 15xy + 12x – 20y + 16 by 3x – 5y + 4
= (3x -5y + 4) [(3x)² + (-5y)² + (4)² – 3x x (-5y) (-5y x 4) – (4 x 3x)]
= (3x)³ + (-5y)³ + (4)³ – 3 x 3x (-5y) x 4
= 27x³ – 1257³ + 64 + 180xy

Question 9.
(3x – 2y)³ + (2y – 4z)³ + (4z – 3x)³
Solution:
(3x – 2y)³ + (2y – 4z)³ +   (4z – 3x)³
 ∵ 3x – 2y + 2y – 4z + 4z – 3x = 0
∴ (3x – 2y)³ + (2y – 4z)³ + (4z – 3x)³
= 3(3x – 2y) (2y – 4z) (4z – 3x)               {∵ x³ + y³ + z³ = 3xyz if x + y + z = 0}

Question 10.
(2x – 3y)³ + (4z – 2x)³  + (3y – 4z)³
Solution:
(2x – 3y)³ + (4z – 2x)³ + (3y – 4z)³
∵  2x – 3y + 4z – 2x + 3y – 4z = 0
∴ (2x – 3y)³ + (4z – 2x)³ + (3y – 4z)³
= (2x – 3y) (4z – 2x) (3y – 4z)                {∵ x³ + y³ + z³ = 3xyz if x + y + z = 0}

Question 11.

Solution:

Question 12.
(a – 3b)³ + (3b – c)³ + (c – a)³
Solution:
(a- 3b)³ + (3b – c)³ + (c – a)³
∵ a – 3b + 3b – c + c – a = 0
∴  (a – 3b)³ + (3b – c)³ + (c – a)³
= 3(a – 3b) (3b – c) (c – a)                       {∵ a³ + b³ + c³ = 3abc if a + b + c = 0}

Question 13.

Solution:

Question 14.

Solution:

Question 15.
2 \(\sqrt { 2 } \) a³+ 16\(\sqrt { 2 } \) b³ + c³ – 12abc
Solution:

Question 16.
Find the value of x³ + y³ – 12xy + 64, when x + y = -4
Solution:
x³ + y³ – 12xy + 64
x + y = -4
Cubing both sides,
x³ + y³ + 3 xy(x + y) = -64
Substitute the value of (x + y)
⇒ x² + y² + 3xy x (-4) = -64
⇒  x³ + y² – 12xy + 64 = 0

Hope given RD Sharma Class 9 Solutions Chapter 5 Factorisation of Algebraic Expressions Ex 5.4 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 4 Algebraic Identities Ex 4.3

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 4 Algebraic Identities Ex 4.3

Question 1.
Find the cube of each of the following binomial expressions:

Solution:

Question 2.
If a + b = 10 and ab = 21, find the value of a³ + b³.
Solution:
a + b = 10, ab = 21
Cubing both sides,
(a + b)³ = (10)³
⇒ a³ + 6³ + 3ab (a + b) = 1000
⇒  a³ + b³ + 3 x 21 x 10 = 1000
⇒  a³ + b³ + 630 = 1000
⇒  a³ + b³ = 1000 – 630 = 370
∴ a³ + b³ = 370

Question 3.
If a – b = 4 and ab = 21, find the value of a³-b³.
Solution:
a – b = 4, ab= 21
Cubing both sides,
⇒ (a – A)³ = (4)³
⇒ a³ – b³ – 3ab (a – b) = 64
⇒ a³-i³-3×21 x4 = 64
⇒  a³ – 6³ – 252 = 64
⇒  a³ – 6³ = 64 + 252 =316
∴ a³ – b³ = 316

Question 4.

Solution:

Question 5.

Solution:

Question 6.

Solution:

Question 7.

Solution:

Question 8.

Solution:

Question 9.
If 2x + 3y = 13 and xy = 6, find the value of 8x³ + 21y³.
Solution:
2x + 3y = 13, xy = 6
Cubing both sides,
(2x + 3y)³ = (13)³
⇒ (2x)³ + (3y)³ + 3 x 2x x 3X2x + 3y) = 2197
⇒ 8x³ + 27y³ + 18xy(2x + 3y) = 2197
⇒ 8x³ + 27y³ + 18 x 6 x 13 = 2197
⇒ 8X³ + 27y³ + 1404 = 2197
⇒  8x³ + 27y³ = 2197 – 1404 = 793
∴ 8x³ + 27y³ = 793

Question 10.
If 3x – 2y= 11 and xy = 12, find the value of 27x³ – 8y³.
Solution:
3x – 2y = 11 and xy = 12 Cubing both sides,
(3x – 2y)³ = (11)³
⇒  (3x)³ – (2y)³ – 3 x 3x x 2y(3x – 2y) =1331
⇒  27x³ – 8y³ – 18xy(3x -2y) =1331
⇒   27x³ – 8y³ – 18 x 12 x 11 = 1331
⇒  27x³ – 8y³ – 2376 = 1331
⇒  27X³ – 8y³ = 1331 + 2376 = 3707
∴ 2x³ – 8y³ = 3707

Question 11.
Evaluate each of the following:
(i)  (103)³
(ii) (98)³
(iii) (9.9)³
(iv) (10.4)³
(v) (598)³
(vi) (99)³
Solution:
We know that (a + bf = a³ + b³ + 3ab(a + b) and (a – b)³= a³ – b³ – 3 ab(a – b)
Therefore,
(i)  (103)³ = (100 + 3)³
= (100)³ + (3)³ + 3 x 100 x 3(100 + 3)    {∵ (a + b)³ = a³ + b³ + 3ab(a + b)}
= 1000000 + 27 + 900 x 103
= 1000000 + 27 + 92700
= 1092727
(ii) (98)³ = (100 – 2)³
= (100)³ – (2)³ – 3 x 100 x 2(100 – 2)
= 1000000 – 8 – 600 x 98
= 1000000 – 8 – 58800
= 1000000-58808
= 941192
(iii) (9.9)³ = (10 – 0.1)³
= (10)³ – (0.1)³ – 3 X 10 X 0.1(10 – 0.1)
= 1000 – 0.001 – 3 x 9.9
= 1000 – 0.001 – 29.7
= 1000 – 29.701
= 970.299
(iv) (10.4)³ = (10 + 0.4)³
= (10)³ + (0.4)³ + 3 x 10 x 0.4(10 + 0.4)
= 1000 + 0.064 + 12(10.4)
= 1000 + 0.064 + 124.8 = 1124.864
(v) (598)³ = (600 – 2)³
= (600)³ – (2)³ – 3 x 600 x 2 x (600 – 2)
= 216000000 – 8 – 3600 x 598
= 216000000 – 8 – 2152800
= 216000000 – 2152808
= 213847192
(vi) (99)³ = (100 – 1)³
= (100)³ – (1)³ – 3 x 100 x 1 x (100 – 1)
= 1000000 – 1 – 300 x 99
= 1000000 – 1 – 29700
= 1000000 – 29701
= 970299

Question 12.
Evaluate each of the following:
(i)  111³ – 89³
(ii) 46³ + 34³
(iii) 104³ + 96³
(iv) 93³ – 107³
Solution:
We know that a³ + b³ = (a + bf – 3ab(a + b) and a³ – b³ = (a – bf + 3 ab(a – b)
(i) 111³ – 89³
= (111 – 89)³ + 3 x ill x 89(111 – 89)
= (22)³ + 3 x 111 x 89 x 22
= 10648 + 652014 = 662662
(OR)
(a + b)³ – (a – b)³ = 2(b³ + 3a²b)
= 111³ – 89³ = (100 + 11)³ – (100 – 11)³
= 2(11³ + 3 x 100² x 11]
= 2(1331 + 330000]
= 331331 x 2 = 662662
(a + b)³ + (a- b)³ = 2(b³ + 3ab²)
(ii) 46³ + 34³ = (40 + 6)³ + (40 – 6)³
= 2[(40)³ + 3 x 40 x 6²]
= 2[64000 + 3 x 40 x 36]
= 2[64000 + 4320]
= 2 x 68320 = 136640
(iii) 104³ + 96³ = (100 + 4)³ + (100 – 96)³
= 2 [a³ + 3 ab²]
= 2[(100)³ + 3 x 100 x (4)²]
= 2[ 1000000 + 300 x 16]
= 2[ 1000000 + 4800]
= 1004800 x 2 = 2009600
(iv) 93³ – 107³ = -[(107)³ – (93)³]
= -[(100 + If – (100 – 7)³]
= -2[b³ + 3a²b)]
= -2[(7)³ + 3(100)² x 7]
= -2(343 + 3 x 10000 x 7]
= -2[343 + 210000]
= -2[210343] = -420686

Question 13.

Solution:

Question 14.
Find the value of 27X³ + 8y³ if
(i) 3x + 2y = 14 and xy = 8
(ii) 3x + 2y = 20 and xy = \(\frac { 14 }{ 9 }\)
Solution:

Question 15.
Find the value of 64x³ – 125z³, if 4x – 5z = 16 and xz = 12.
Solution:
4x – 5z = 16, xz = 12
Cubing both sides,
(4x – 5z)³ = (16)³
⇒ (4x)³ – (5y)³ – 3 x 4x x 5z(4x – 5z) = 4096
⇒ 64x³ – 125z³ – 3 x 4 x 5 x xz(4x – 5z) = 4096
⇒  64x³ – 125z³ – 60 x 12 x 16 = 4096
⇒ 64x³ – 125z³ – 11520 = 4096
⇒  64x³ – 125z³ = 4096 + 11520 = 15616

Question 16.

Solution:

Question 17.
Simplify each of the following:

Solution:

Question 18.

Solution:

Question 19.

Solution:

Hope given RD Sharma Class 9 Solutions Chapter 4 Algebraic Identities Ex 4.3 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 3 Rationalisation VSAQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 3 Rationalisation VSAQS

Question 1.
Write the value of (2 + \(\sqrt { 3 } \) ) (2 – \(\sqrt { 3 } \)).
Solution:
(2+ \(\sqrt { 3 } \) )(2- \(\sqrt { 3 } \) ) = (2)²-(\(\sqrt { 3 } \) )²
{∵ (a + b) (a – b) = a² – b²}
= 4-3=1

Question 2.
Write the reciprocal of 5 + \(\sqrt { 2 } \).
Solution:

Question 3.
Write the rationalisation factor of 7 – 3\(\sqrt { 5 } \) .
Solution:
Rationalising factor of 7 – 3\(\sqrt { 5 } \) is 7 + 3\(\sqrt { 5 } \)
{∵ (\(\sqrt { a } \) + \(\sqrt { b } \)  ) (\(\sqrt { a } \) – \(\sqrt { b } \)) = a-b}

Question 4.

Solution:

Question 5.
If x =\(\sqrt { 2 } \) – 1 then write the value of \(\frac { 1 }{ x }\).
Solution:

Question 6.
If a = \(\sqrt { 2 } \) + h then find the value of a –\(\frac { 1 }{ a }\)
Solution:

Question 7.
If x = 2 + \(\sqrt { 3 } \), find the value of x + \(\frac { 1 }{ x }\).
Solution:

Question 8.
Write the rationalisation factor of \(\sqrt { 5 } \) – 2.
Solution:
Rationalisation factor of \(\sqrt { 5 } \) – 2 is \(\sqrt { 5 } \) + 2 as
(\(\sqrt { a } \) + \(\sqrt { b } \))(\(\sqrt { a } \) – \(\sqrt { b } \)) = a – b

Question 9.
Simplify : \(\sqrt { 3+2\sqrt { 2 } }\).
Solution:

Question 10.
Simplify : \(\sqrt { 3-2\sqrt { 2 } }\).
Solution:

Question 11.
If x = 3 + 2 \(\sqrt { 2 } \), then find the value of  \(\sqrt { x } \) – \(\frac { 1 }{ \sqrt { x } }\).
Solution:

Hope given RD Sharma Class 9 Solutions Chapter 3 Rationalisation VSAQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1

Question 1.
Simplify each of the following:

Solution:

Question 2.
Simplify the following expressions:
(i)  (4 + \(\sqrt { 7 } \)) (3 + \(\sqrt { 2 } \))
(ii) (3 + \(\sqrt { 3 } \) )(5- \(\sqrt { 2 } \))
(iii) (\(\sqrt { 5 } \) -2)(\(\sqrt { 3 } \) – \(\sqrt { 5 } \))
Solution:

Question 3.
Simplify the following expressions:
(i)  (11 + \(\sqrt { 11 } \)) (11 – \(\sqrt { 11 } \))
(ii) (5 + \(\sqrt { 7 } \) ) (5 – \(\sqrt { 7 } \) )
(iii) (\(\sqrt { 8 } \) – \(\sqrt { 2 } \)) (\(\sqrt { 8 } \)+ \(\sqrt { 2 } \))
Solution:

Question 4.
Simplify the following expressions:
(i) (\(\sqrt { 3 } \)+\(\sqrt { 7 } \))²
(ii) (\(\sqrt { 5 } \) – \(\sqrt { 3 } \) )²
(iii) (2\(\sqrt { 5 } \) + 3 \(\sqrt { 2} \))²
Solution:
(i)


Hope given RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS

Mark the correct alternative in each of the following:
Question 1.
The value of {2 – 3 (2 – 3)³}³ is
(a) 5
(b) 125
(c) \(\frac { 1 }{ 5 }\)
(d) -125
Solution:
{2 – 3 (2 – 3)³}³ = {2 – 3 (-1)³}³
= {2 – 3 x (-1)}³
= (2 + 3)³ = (5)³
= 125    (b)

Question 2.
The value of x – y^x-y when x = 2 and y = -2 is
(a) 18
(b) -18
(c) 14
(d) -14
Solution:
x = 2, y = -2
x-y^x-y = 2 – (-2)^{2 – (-2)}
= 2 – (-2)^{2 + 2} = 2 – (-2)⁴
= 2 – (+16) = 2 – 16 = -14        (d)

Question 3.
The product of the square root of x with the cube root of x, is
(a) cube root of the square root of x
(b) sixth root of the fifth power of x
(c) fifth root of the sixth power of x
(d) sixth root of x
Solution:

Question 4.
The seventh root of x divided by the eighth root of x is

Solution:

Question 5.
The square root of 64 divided by the cube root of 64 is
(a) 64
(b) 2
(c) \(\frac { 1 }{ 2 }\)
(d) 64^{\(\frac { 2 }{ 3 }\)}
Solution:

Question 6.
Which of the following is (are) not equal to

Solution:

Question 7.
When simplified (x^–1 + y^–1)^–1 is equal to

Solution:

Question 8.
If 8^x+1 = 64, what is the value of 3 ^{2x +1}?
(a) 1
(b) 3
(c) 9
(d) 27
Solution:

Question 9.
If (2³)² = 4^x then   3^x =
(a) 3
(b) 6
(c) 9
(d) 27
Solution:

Question 10.
If x^-2= 64, then x^{\(\frac { 1 }{ 3 }\)} + x°=
(a) 2
(b) 3
(c) \(\frac { 3 }{ 2 }\)
(c) \(\frac { 2 }{ 3 }\)
Solution:

Question 11.
When simplified ( –\(\frac { 1 }{ 27 }\))^{\(\frac { -2 }{ 3 }\)}
(a) 9
(b) -9
(c) \(\frac { 1 }{ 9 }\)
(d) –\(\frac { 1 }{ 9 }\)
Solution:

Question 12.
Which one of the following is not equal to

Solution:

Question 13.
Which one of the following is not equal to

Solution:

Question 14.
If a, b, c are positive real numbers, then

Solution:

Question 15.

Solution:

Question 16.

Solution:

Question 17.

Solution:

Question 18.

Solution:

Question 19.

Solution:

Question 20.

Solution:

Question 21.
The value of {(23 + 2²)^2/3+ (150 -29)^1/2}²  is
(a) 196
(b) 289
(c) 324
(d) 400
Solution:
{(23 + 2²)^2/3 + (150 – 29)^1/2}²
= [(23×4)^{\(\frac { 2 }{ 3 }\)}  +(150 – 29)^{\(\frac { 1 }{ 2 }\)} ]²
= [(27)^{\(\frac { 2 }{ 3 }\)} + (121)^{\(\frac { 1 }{ 2 }\)} ]²
= [(3³)³ +(11²)^{\(\frac { 1 }{ 2 }\)}]² = (9 + 11)²
= (20)² = 400  (d)

Question 22.
(256)^0.16x (256)^0.09
(a) 4
(b) 16
(c) 64
(d) 256.25
Solution:

Question 23.
If 10^2y = 25, then 10^-y equals

Solution:

Question 24.
If 9^{X + 2} = 240 + 9^X. then x =
(a) 0.5
(b) 0.2
(c) 0.4
(d) 0.1
Solution:

Question 25.
If x is a positive real number and x² = 2, then x³ =
(a) \(\sqrt { 2 } \)
(b) 2\(\sqrt { 2 } \)
(c) 3\(\sqrt { 2 } \)
(d) 4
Solution:

Question 26.

Solution:

Question 27.

Solution:

Question 28.

Solution:

Question 29.

Solution:

Question 30.

Solution:

Question 31.

Solution:

Question 32.

Solution:

Question 33.
If (16)^{2x + 3} = (64)^{x + 3} , then 4^{2x – 2}  =
(a) 64
(b) 256
(c) 32
(d) 512
Solution:

Question 34.

Solution:

Question 35.

Solution:

Question 36.

Solution:

Question 37.

Solution:

Question 38.

Solution:

Question 39.

Solution:

Question 40.

Solution:

Hope given RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS

Question 1.
Write (625)^–1/4 in decimal form.
Solution:

Question 2.
State the product law of exponents:
Solution:
x^m x xⁿ = x^{m +n}

Question 3.
State the quotient law of exponents.
Solution:
x^m ÷ xⁿ = x^{m -n}

Question 4.
State the power law of exponents.
Solution:
(x^m)ⁿ =x^{m x n} = x^mn

Question 5.
If 2⁴ x 4² – 16^x, then find the value of x.
Solution:

Question 6.

Solution:

Question 7.
Write the value of \(\sqrt [ 3 ]{ 7 }\)  x \(\sqrt [ 3 ]{ 49 }\) .
Solution:

Question 8.

Solution:

Question 9.
Write the value of \(\sqrt [ 3 ]{ 125×27 }\)
Solution:

Question 10.

Solution:

Question 11.

Solution:

Question 12.

Solution:

Question 13.

Solution:

Question 14.
If (x – 1)³ = 8, what is the value of (x + 1)²?
Solution:

Hope given RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers Ex 2.2

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers Ex 2.2

Question 1.
Assuming that x, y, z are positive real numbers, simplify each of the following:

Solution:

Question 2.
Simplify:

Solution:

Question 3.
Prove that:

Solution:

Question 4.
Show that:


Solution:

Question 5.

Solution:

Question 6.

Solution:

Question 7.

Solution:

Question 8.

Solution:

Question 9.

Solution:

Question 10.
Find the values of x in each  of the following:

Solution:

Question 11.
If x = 2^1/3 + 2^2/3, show that x³ – 6x = 6.
Solution:

Question 12.
Determine (8x)^x, if 9^{x+ 2} = 240 + 9^x.
Solution:

Question 13.
If 3^x+1 = 9^x-2, find the value of 2^{1 +x}.
Solution:

Question 14.
If 3^4x = (81)^-1 and 10^1/y = 0.0001, find the value of 2^-x+4y
Solution:

Question 15.
If 5^3x = 125 and 10^y = 0.001 find x and y.
Solution:

Question 16.
Solve the following equations:

Solution:

Question 17.

Solution:

Question 18.
If a and b are different positive primes such that

Solution:

Question 19.
If 2^x x 3^y x 5^z = 2160, find x, y and z. Hence, compute the value of 3^x x 2^-y x 5^-z.
Solution:

Question 20.
If 1176 = 2^a x 3^b x 7^c, find the values of a, b and c. Hence, compute the value of 2^a x 3^b x 7^-c as a fraction.
Solution:

Question 21.
Simplify:

Solution:

Question 22.
Show that:

Solution:

Question 23.

Solution:

Hope given RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers Ex 2.2 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers Ex 2.1

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers Ex 2.1

Question 1.
Simplify the following:

Solution:

Question 2.
If a = 3 and b =-2, find the values of:
(i) a^a+ b^b
(ii) a^b + b^a
(iii) (a+b)^ab
Solution:

Question 3.
Prove that:

Solution:

Question 4.
Prove that

Solution:

Question 5.
Prove that

Solution:

Question 6.

Solution:

Question 7.
Simplify the following:

Solution:

Question 8.
Solve the following equations for x:

Solution:

Question 9.
Solve the following equations for x:

Solution:

Question 10.
If 49392 = a⁴b²V³, find the values.of a, b and c, where a, b and c are different positive primes.
Solution:

Question 11.
If 1176 = 2^a x 3^b x T^c, find a, 6 and c.
Solution:

Question 12.
Given 4725 = 3^a5^b7^c, find:
(i) the integral values of a, b and c
(ii) the value of 2^-a 3^b 7^c
Solution:

Question 13.
If a = xy^p-1, b = xy ^q-1 and c = xy^r-1, prove that a^q-r b^r-p c^p-q = 1
Solution:

Hope given RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers Ex 2.1 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 1 Number Systems MCQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 1 Number Systems MCQS

Other Exercises

• RD Sharma Class 9 Solutions Chapter 1 Number Systems Ex 1.1
• RD Sharma Class 9 Solutions Chapter 1 Number Systems Ex 1.2
• RD Sharma Class 9 Solutions Chapter 1 Number Systems Ex 1.3
• RD Sharma Class 9 Solutions Chapter 1 Number Systems Ex 1.4
• RD Sharma Class 9 Solutions Chapter 1 Number Systems Ex 1.5
• RD Sharma Class 9 Solutions Chapter 1 Number Systems Ex 1.6
• RD Sharma Class 9 Solutions Chapter 1 Number Systems MCQS

the correct alternative in each of the following:
Question 1.
Which one of the following is a correct statement?
(a) Decimal expansion of a rational number is terminating
(b) Decimal expansion of a rational number is non-terminating
(c) Decimal expansion of an irrational number is terminating
(d) Decimal expansion of an irrational number is non-terminating and non-repeating
Solution:
Decimal expansion of an irrational number is non-terminating and non-repeating . (d)

Question 2.
Which one of the following statements is true?
(a) The sum of two irrational numbers is always an irrational-number            
(b) The sum of two irrational numbers is always a rational number
(c) The sum of two irrational numbers may be a rational number or an irrational number
(d) The sum of two irrational numbers is always an integer
Solution:
The sum of two irrational numbers may be a rational number or an irrational number (c)

Question 3.
Which of the following is a correct statement?
(a) Sum of two irrational numbers is always irrational
(b) Sum of a rational and irrational number is always an irrational number
(c) Square of an irrational number is always a rational number
(d) Sum of two rational numbers can never be an integer
Solution:
Sum of a rational and irrational number is always an irrational number         (b)

Question 4.
Which of the following statements is true?
(a) Product of two irrational numbers is always irrational
(b) Product of a rational and an irrational number is always irrational
(c) Sum of two irrational numbers can never be irrational
(d) Sum of an integer and a rational number can never be an integer
Solution:
Product of a rational and an irrational number is always irrational    (b)

Question 5.
Which of the following is irrational?

Solution:

Question 6.
Which of the following is irrational?
(a) 14
(b)  0.14\(\overline { 16 }\)
(c)   0.\(\overline { 1416 }\)                  
(d)  0.1014001400014
Solution:
0.1014001400014…….. is irrational as it is non-terminating nor repeating decimal, (d)

Question 7.
Which of the following is rational?

Solution:

Question 8.
The number 0.318564318564318564… is:
(a) a natural number
(b) an integer
(c) a rational number
(d) an irrational number
Solution:
The number = 0.318564318564318564…………
= 0.\(\overline { 318564 }\)
∵ The decimal is non-terminating and recurring
∴ It is rational number.   (c)

Question 9.
If n is a natural number, then \(\sqrt { n } \)is
(a) always a natural number
(b) always a rational number
(c) always an irrational number
(d) sometimes a natural number and sometimes an irrational number
Solution:
If n is a natural number then \(\sqrt { n } \) may sometimes a natural number and sometime an irrational number e.g.
If n = 2 then \(\sqrt { n } \) =\(\sqrt { 2 } \) which is are irrational and if n = 4, then \(\sqrt { n } \)= \(\sqrt { 4 } \) =  2 which is a rational number.       (d)

Question 10.
Which of the following numbers can be represented as non-terminating, repeating decimals?

Solution:

Question 11.
Every point on a number line represents
(a) a unique real number
(b) a natural number
(c) a rational number
(d) an irrational number
Solution:
Every point on a number line represents a unique real number.         (a)

Question 12.
Which of the following is irrational?
(a) 0.15                     
(b) 0.01516
(c) 0.\(\overline { 1516 }\)                
(d) 0.5015001500015..
Solution:
As it is non-terminating non-repeating decimals while others are terminating or non-terminating repeating decimals. (d)

Question 13.
The number 1.\(\overline { 27 }\) in the form \(\frac { p }{ q }\)  , where p and q are integers and q ≠ 0, is

Solution:

Question 14.

Solution:

Question 15.

Solution:

Question 16.

Solution:

Question 17.

Solution:

Question 18.

Solution:

Question 19.

Solution:
An irrational number between 2 and 2.5 is \(\sqrt { 5 } \) as it has approximate value 2.236… (b)

Question 20.
The number of consecutive zeros in 2³ x 3⁴ x 5⁴ x 7, is
(a) 3                            
(b) 2
(c) 4
(d) 5
Solution:
In 2³ x 3⁴ x 5⁴ x 7, number of consecutive zero will be 3 as 2³ x 5⁴ = 2 x 2 x 2 x 5x 5 x 5 x 5 = 5000      (a)

Question 21.
The smallest rational number by which \(\frac { 1 }{ 3 }\) should be multiplied so that its decimal expansion terminates after one place of decimal, is

Solution:

Hope given RD Sharma Class 9 Solutions Chapter 1 Number Systems MCQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.