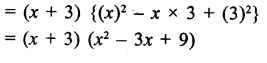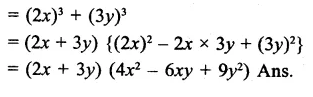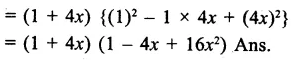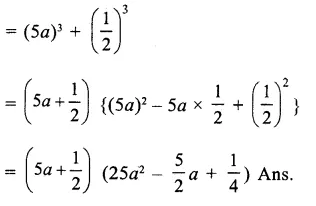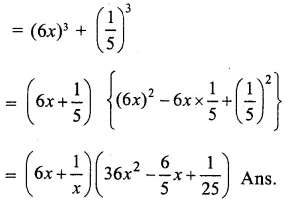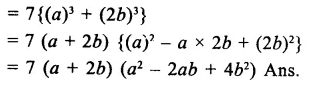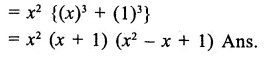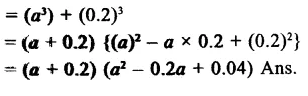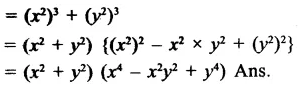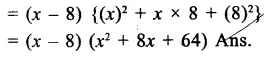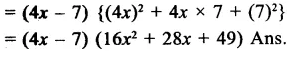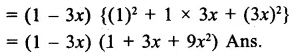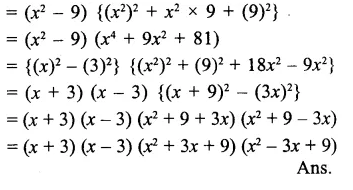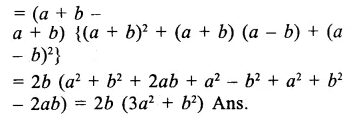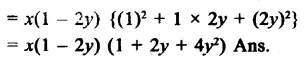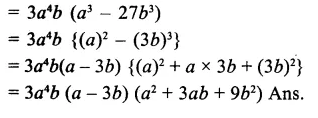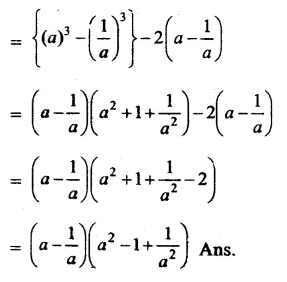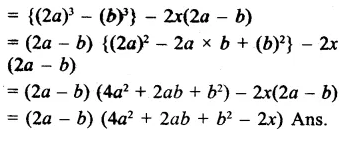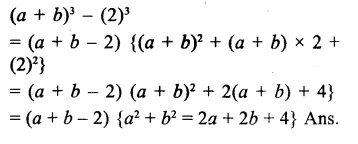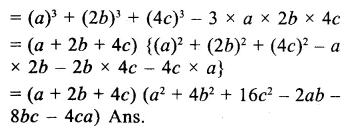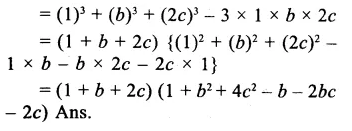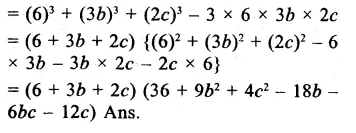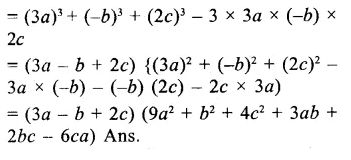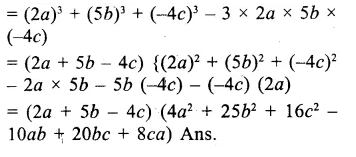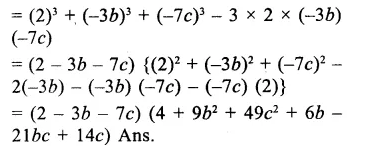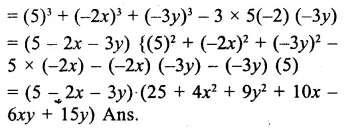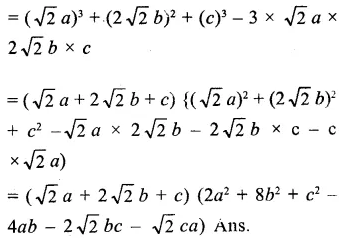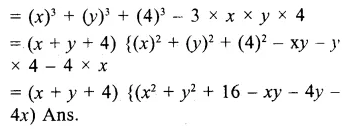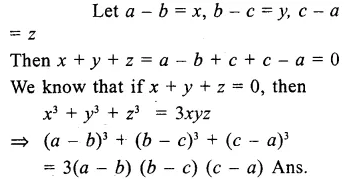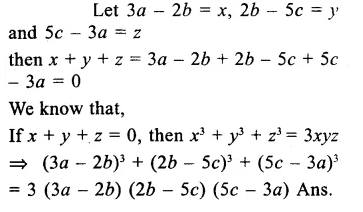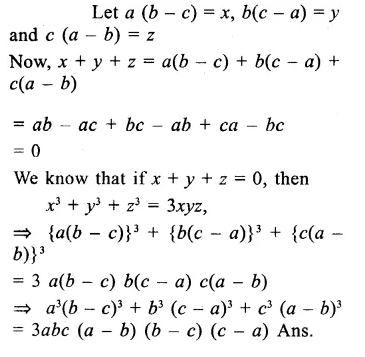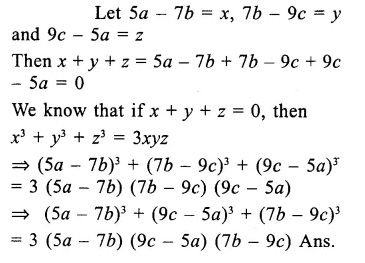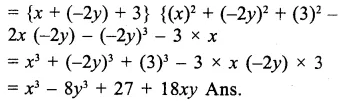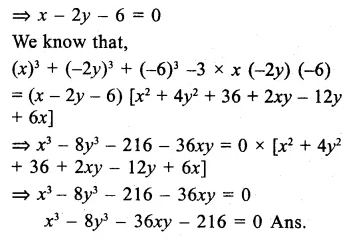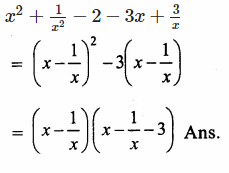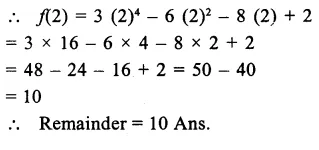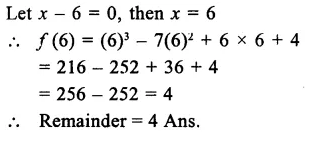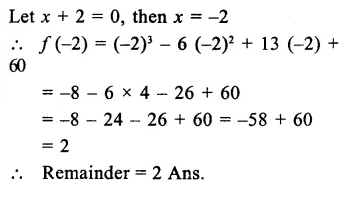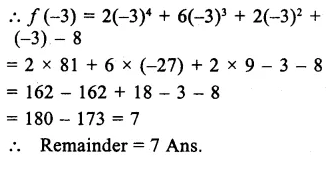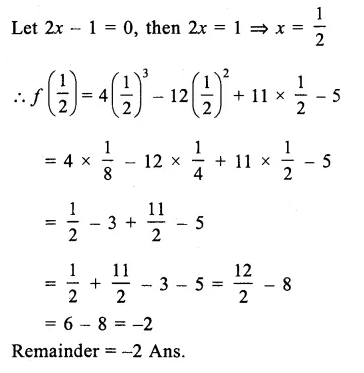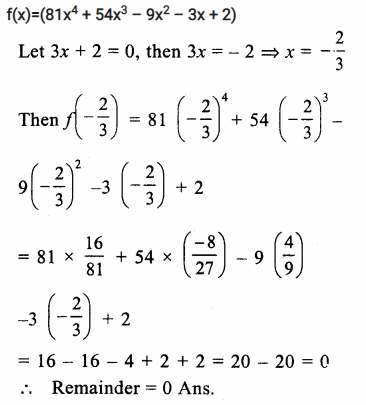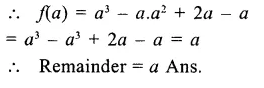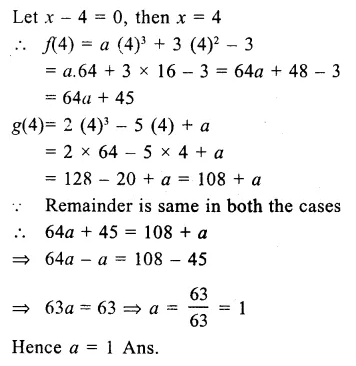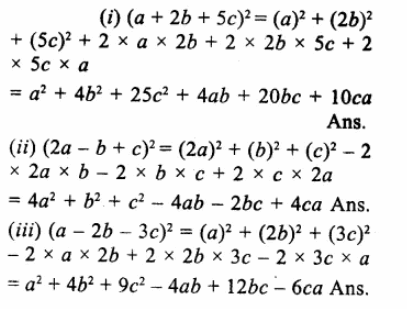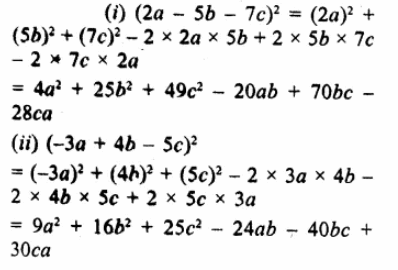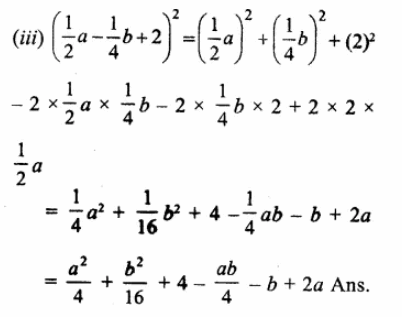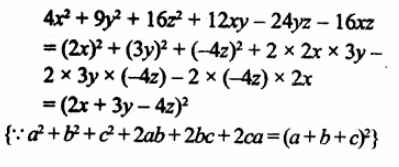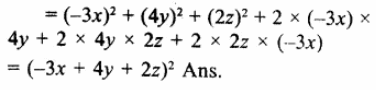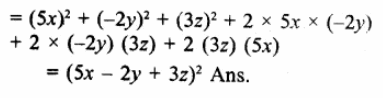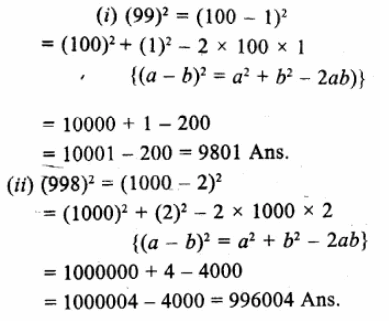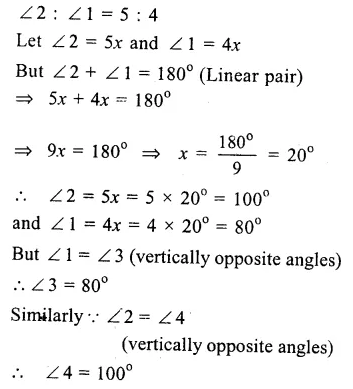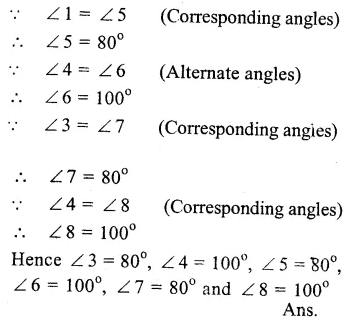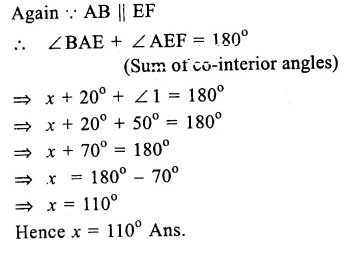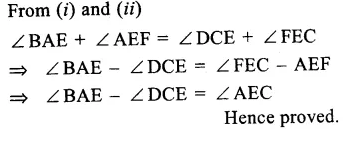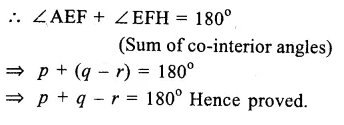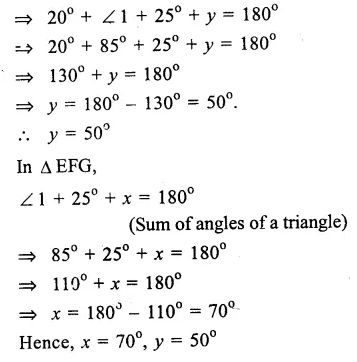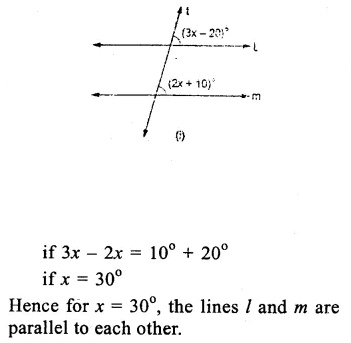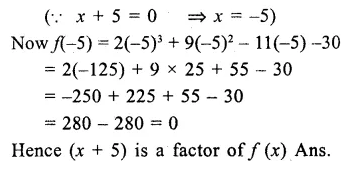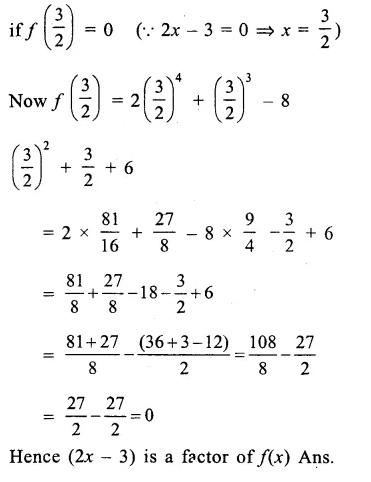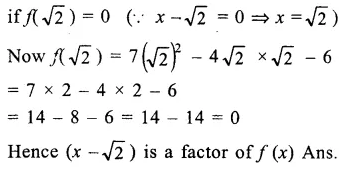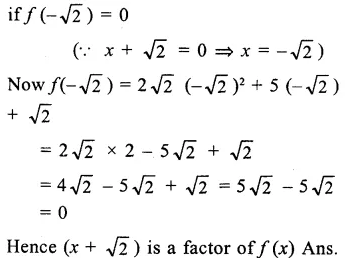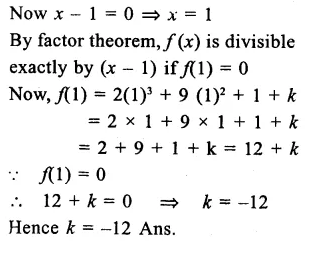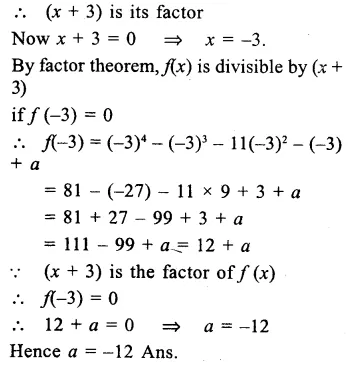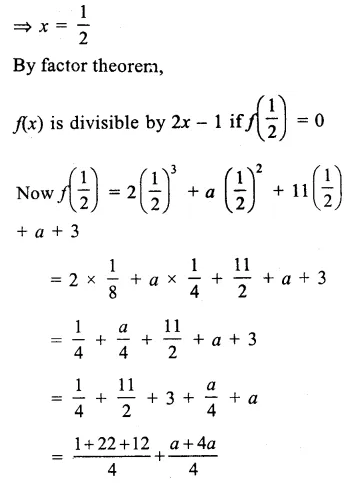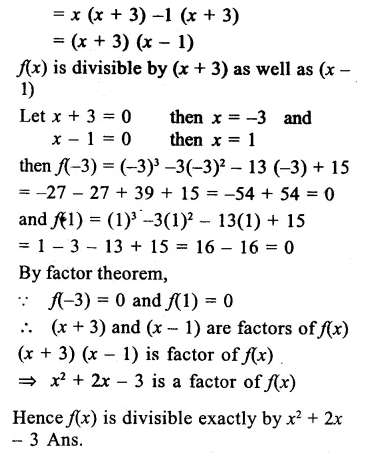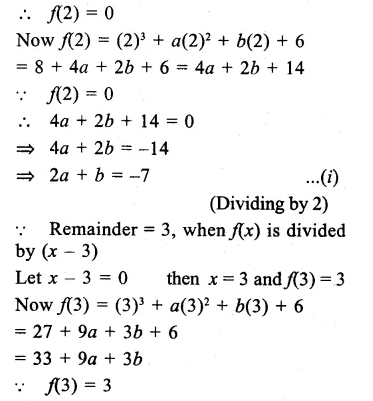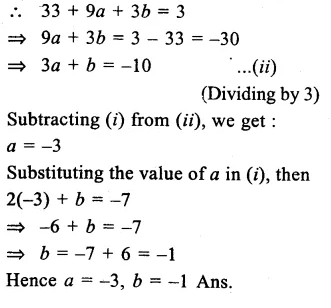RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2I
These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2I.
Other Exercises
- RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2A
- RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2B
- RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2C
- RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2D
- RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2E
- RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2F
- RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2G
- RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2H
- RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2I
- RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2J
- RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2K
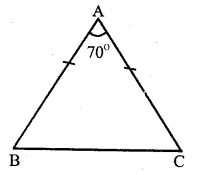
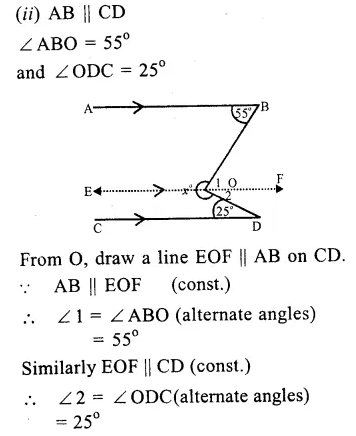
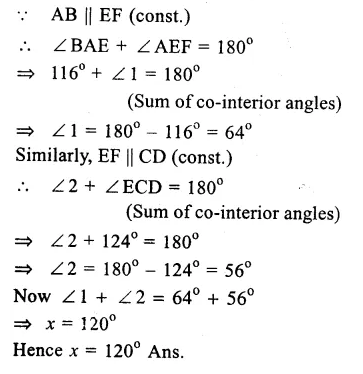
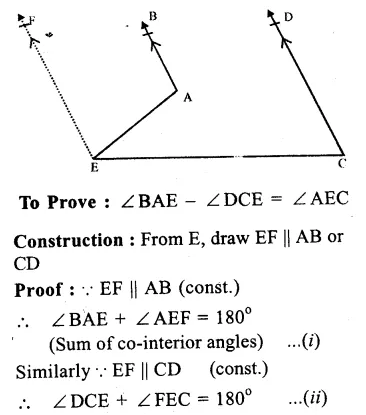
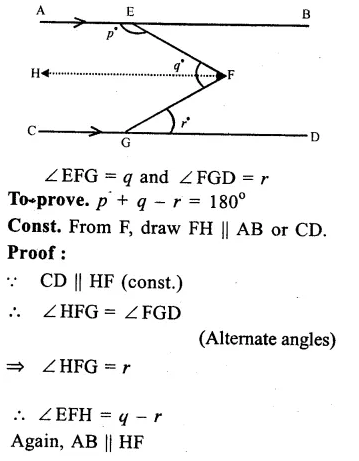
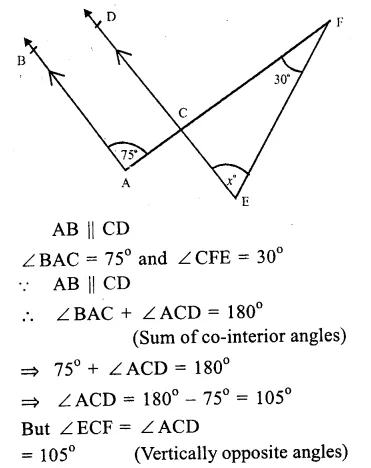
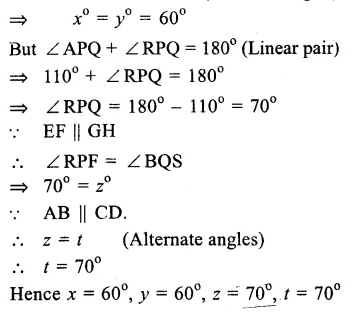
Question 1.
Solution:
(i)(3x+2)3
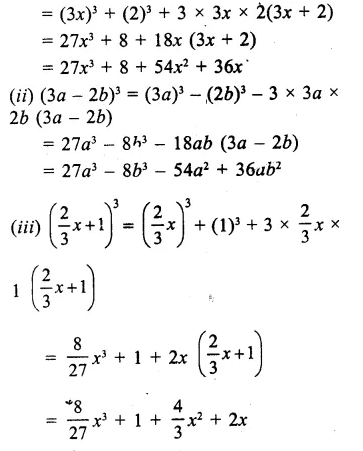
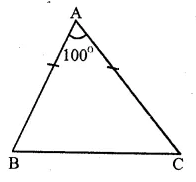
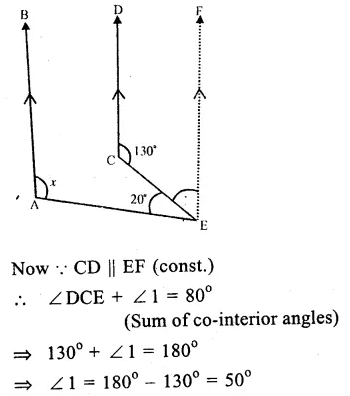
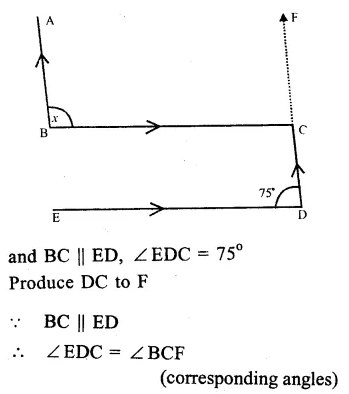
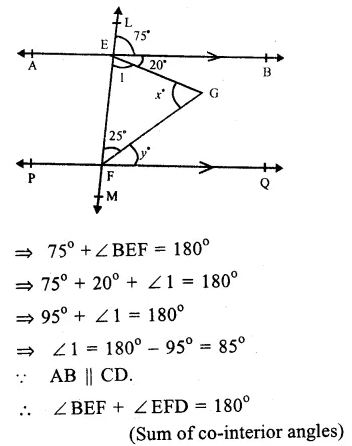
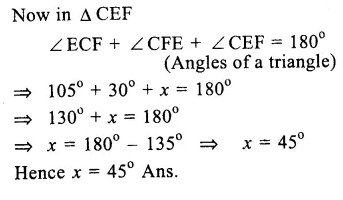
Question 2.
Solution:
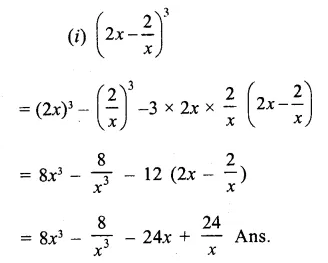
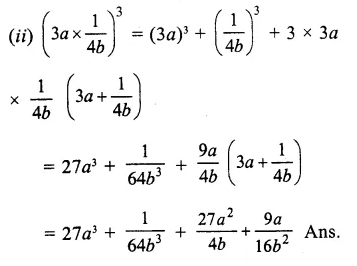
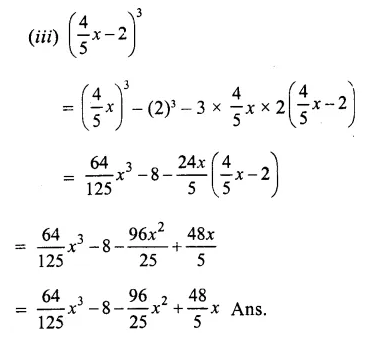
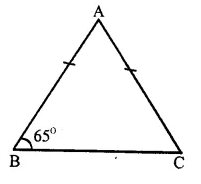
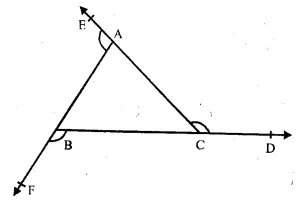
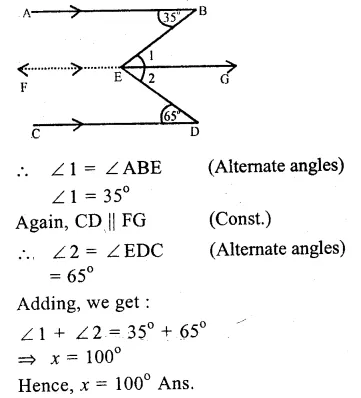
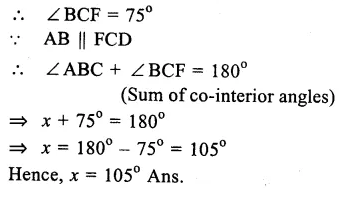
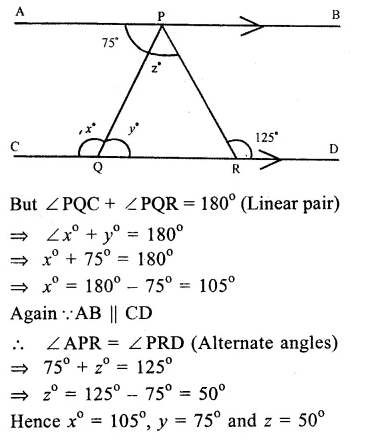
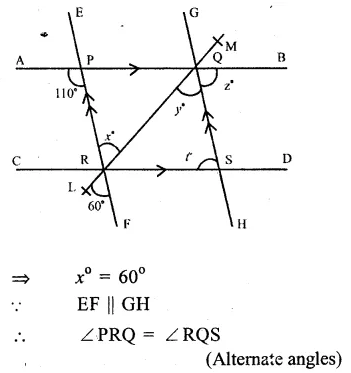
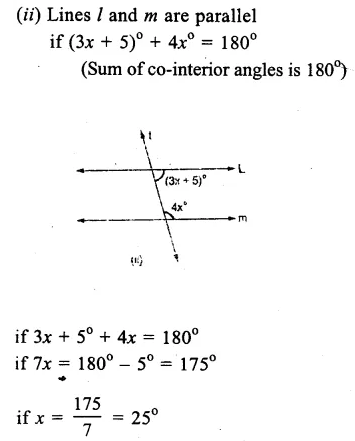
Question 3.
Solution:
(i)(95)3 = (100 – 5)3

Hope given RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2I are helpful to complete your math homework.
If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.