RS Aggarwal Class 6 Solutions Chapter 2 Factors and Multiples Ex 2D
These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2D.
Other Exercises
- RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2A
- RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2B
- RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2C
- RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2D
- RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2E
- RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2F
Find the H.C.F. of the numbers in each of the following, using the prime factorization method :
Question 1.
Solution:
We have

84 = 2 x 2 x 3 x 7
= 22 x 3 x 7
98 = 2 x 7 x 7 = 2 x 72
∴H.C.F. =2 x 7 = 14.
Question 2.
Solution:
We have

So, 170 = 2 x 5 x 17
238 = 2 x 7 x 17
∴ H.C.F. of 170 and 238 = 2 x 17 = 34
Question 3.
Solution:
We have

So, 504 = 2 x 2 x 2 x 3 x 3 x 7 = 23 x 32 x 7
980 = 2 x 2 x 5 x 7 x 7 = 22 x 5 x 72
∴ H.C.F. of 504 and 980 = 22 x 7
= 4 x 7 = 28.
Question 4.
Solution:
We have

So, 72 = 2 x 2 x 2 x 3 x 3 = 23 x 32
108 = 2 x 2 x 3 x 3 x 3 = 22 x 33
180 = 2 x 2 x 3 x 3 x 5 = 22 x 32 x 5
∴ H.C.F. of 72, 108,
180 = 22 x 32
= 4 x 9 = 36
Question 5.
Solution:
We have
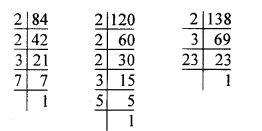
84 = 2 x 2 x 3 x 7 = 22 x 3 x 7
120 = 2 x 2 x 2 x 3 x 5 = 23 x 3 x 5
138 = 2 x 3 x 23
∴ H.C.F. of 84, 120 and 138 = 2 x 3 = 6
Question 6.
Solution:
We have
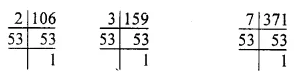
106 = 2 x 53
159 = 3 x 53
371 = 7 x 53
∴ H.C.F. of 106, 159, 371 = 53
Question 7.
Solution:
We have

272 = 2 x 2 x 2 x 2 x 17 = 24 x 17
425 = 5 x 5 x 17
= 52 x 17
∴ H.C.F. of 272 and 425 = 17.
Question 8.
Solution:
We have
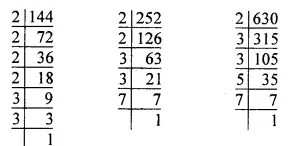
So, 144 = 2 x 2 x 2 x 2 x 3 x 3 = 24 x 32
252 = 2 x 2 x 3 x 3 x 7 = 22 x 32 x 7
630 = 2 x 3 x 3 x 5 x 7 = 2 x 32 x 5 x 7
∴ H.C.F. of 144, 252 and 630 = 2 x 32
= 2 x 9 = 18.
Question 9.
Solution:
We have
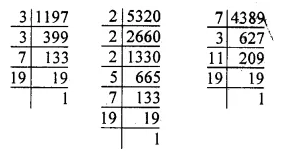
So, 1197 = 3 x 3 x 7 x 19 = 32 x 7 x 19
5320 = 2 x 2 x 2 x 5 x 7 x 19 = 23 x 5 x 7 x 19
4389 = 7 x 3 x 11 x 19
∴ H.C.F. of 1197, 5320,
4389 = 7 x 19 = 133.
Find the H.C.F. of the numbers in each of the following using division method:
Question 10.
Solution:
By division method, we have :
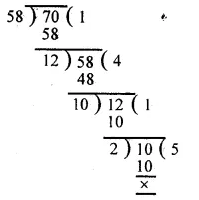
∴ H.C.F. of 58 and 70 = 2.
Question 11.
Solution:
By division method, we have
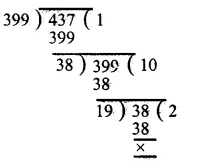
∴ H.C.F. of 399 and 437 = 19
Question 12.
Solution:
By division method, we have

∴ H.C.F. of 1045 and 1520 = 95.
Question 13.
Solution:
By division method, we have
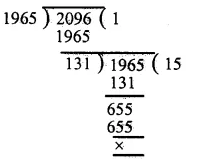
∴ H.C.F. of 1965 and 2096 = 131
Question 14.
Solution:
By division method, we have
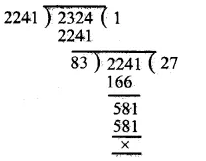
∴ H.C.F. of 2241 and 2324 = 83.
Question 15.
Solution:
First, we find the H.C.F. of 658 and 940

∴ H.C.F. of 658 and 940 is 94.
Now, we find the H.C.F. of 94 and 1128.
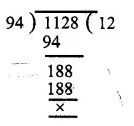
∴ H.C.F. of 94 and 1128 = 94
Hence, H.C.F. of 658, 940 and 1128 = 94.
Question 16.
Solution:
First, we find the H.C.F. of 754 and 1508

∴ H.C.F. of 754 and 1508 is 754
Now, we find the H.C.F. of 754 and 1972

∴ H.C.F. of 754 and 1972 = 58
Hence, the H.C.F. of 754,1508 and 1972 = 58.
Question 17.
Solution:
First, we find the H.C.F. of 391 and 425
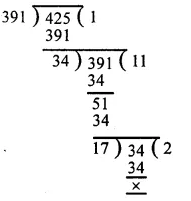
∴ H.C.F. of 391 and 425 is 17.
Now, we find the H.C.F. of 17 and 527
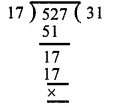
∴ H.C.F. of 17 and 527 is 17 Hence, H.C.F. of 391, 425 and 527 = 17.
Question 18.
Solution:
First, we find the H.C.F. of 1794 and 2346

H.C.F. of 1794 and 2346 is 138.
Now, we find the H.C.F. of 138 and 4761
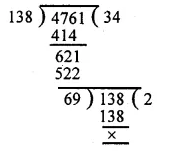
∴ H.C.F. of 138 and 4761 is 69.
Hence, the H.C.F. of 1794, 2346 and 4761 = 69.
Show that the following pairs are co-primes :
Question 19.
Solution:
First, we find the H.C.F. of 59, 97.

∴H.C.F. of 59 and 97 is 1.
Hence 59 and 97 are co-prime.
Question 20.
Solution:
First, we find the H.C.F. of 161 and 192.
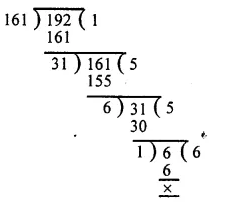
∴ H.C.F. of 161 and 192 is 1.
Hence 161 and 192 are co-prime.
Question 21.
Solution:
First, we find the H.C.F. of 343 and 432

∴ H.C.F. of 343 and 432 is 1.
Hence 343 and 432 are co-prime.
Question 22.
Solution:
First, we find the H.C.F. of 512 and 945.
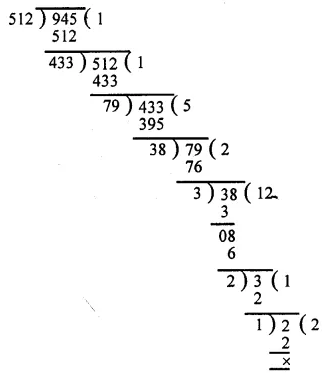
∴ H.C.F. of 512 and 945 is 1.
Hence 512 and 945 are co-prime.
Question 23.
Solution:
First, we find the H.C.F. of385 and 621
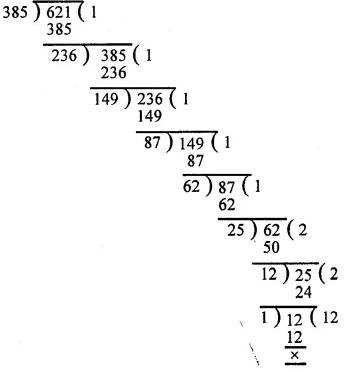
∴ H.C.F. of 385 and 621 is 1.
Hence the numbers 385 and 621 are co-prime
Question 24.
Solution:
First, we find the H.C.F. of 847 and 1014.

∴ H.C.F. of 847 and 1014 is 1.
Hence 847 and 1014 are co-prime.
Question 25.
Solution:
Clearly, we have to find the greatest number which divides (615 – 6) and (963 – 6) exactly.
So, the required number = H.C.F. of 615 – 6 = 609 and 963 – 6 = 957
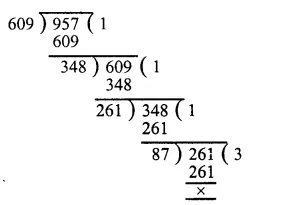
The required greatest number = 87.
Question 26.
Solution:
Clearly, we have to find the greatest number which divides 2011 – 9 = 2002 and 2623 – 5 = 2618.
So, the required greatest number = H.C.F. of 2002 and 2618
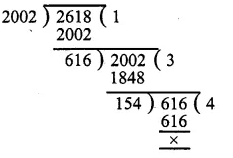
∴ Required greatest number = 154.
Question 27.
Solution:
Clearly, we have to find the greatest number which divides (445 4), (572 – 5) and (699 – 6). So, the required number = H.C.F. of 441, 567 and 693. First we find the H.C.F. of 441 and 567
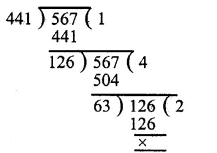
∴ H.C.F. of 441 and 567 is 63
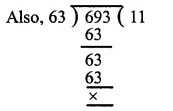
So H.C.F. of 63 and 693 is 63
Hence the required number = 63.
Question 28.
Solution:
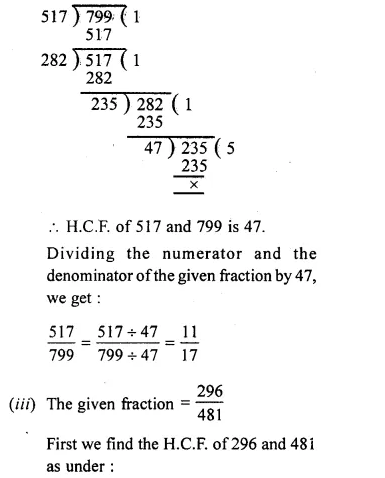
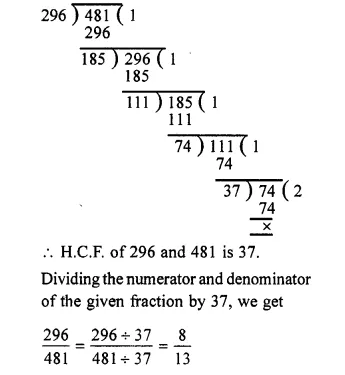
(i) The given fraction = \(\\ \frac { 161 }{ 207 } \)
First we find the H.C.F. of 161 and 207
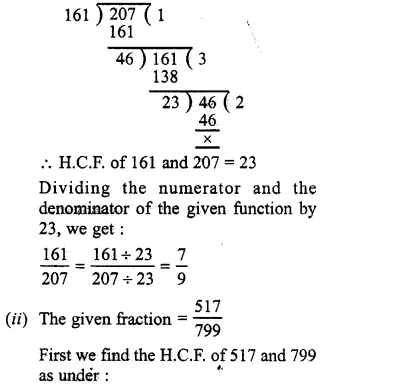
Question 29.
Solution:
Lengths of three pieces of timber = 42 metres, 49 metres, 63 metres Greatest possible length of each plank = H.C.F. of 42 metres, 49 metres and 63 metres
First we find the H.C.F. of 42 and 49
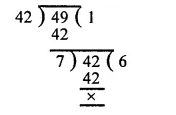
∴ H.C.F. of 42 and 49 = 7
Now we find the H.C.F. of 7 and 63
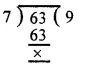
So, the H.C.F. of 7 and 63 is 7
∴ H.C.F. of 42 metres, 49 metres of 63 metres = 7 metres
Hence required possible length of each plank = 7 metres.
Question 30.
Solution:
Quantity of milk in three different containers = 403 L, 434 L and 465 L Clearly, the maximum capacity of the required container = H.C.F. of 403 L, 434 L, 465 L, we have
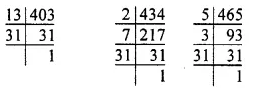
∴ 403 = 13 x 31
434 = 2 x 7 x 31
465 = 5 x 3 x 31
So the H.C.F. of 403 L, 434 L and 465 L = 31 L
Maximum capacity of the required container = 31 L.
Question 31.
Solution:
The given fruits = 527 apples, 646 pears and 748 oranges
Clearly, the greatest number of fruits in each heap = H.C.F. of 527, 646 and 748 we have

∴ 527 = 17 x 31
646 = 2 x 17 x 19
748 = 2 x 2 x 11 x 17
So, the H.C.F. of 527, 646 and 748 = 17
∴ Required number of fruits in each heap = 17
Total number of fruits = 527 + 646 + 748 = 1921
Number of heaps = \(\frac { Total\quad number\quad of\quad fruits }{ Number\quad of\quad fruits\quad in\quad one\quad heap } \)
= \( \frac { 1921 }{ 17 } \)
=113
Question 32.
Solution:
The given lengths are :
7 metres = 7 x 100 cm
= 700 cm 3 metres 85 cm
= (3 x 100 + 85) cm
= 385 cm
12 metres 95 cm = (12 x 100 + 95) cm
= 1295 cm
Clearly, the length of the required longest tape = H.C.F. of 700 cm, 385 cm and 1295 cm
We have
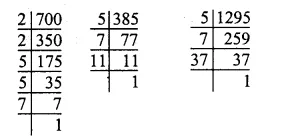
So, 700 = 2 x 2 x 5 x 5 x 7
= 22 x 52 x 7
385 = 5 x 7 x 11
1295 = 5 x 7 x 37
∴ H.C.F. of 700, 385 and 1295 = 5 x 7 = 35
∴ The required length of the longest tape
= 35 cm.
Question 33.
Solution:
Length of the courtyard = 18 m 72 cm = (18 x 100 + 72) cm = 1872 cm
Breadth of the courtyard = 13 m 20 cm = (13 x 100 + 20) cm = 1320 cm
Greatest side of each of the square tiles = H.C.F. of 1872 cm and 1320 cm
Now

1872 = 2 x 2 x 2 x 2 x 9 x 13
= 24 x 32 x 13
1320 = 2 x 2 x 2 x 3 x 5 x 11
= 23 x 3 x 5 x 11
So, the H.C.F. of 1872 and 1320
= 23 x 3 = 8 x 3 = 24
Greatest side of the square tile = 24 cm
Now Area of the courtyard = Length x Breadth = 1872 x 1320 cm2
Area of one square tile = Side x Side
= 24 x 24 cm2
∴ Least possible number of such tiles
= \(\frac { Area\quad of\quad the\quad courtyard }{ Area\quad of\quad the\quad tile } \)
= \( \frac { 1872\times 1320 }{ 24\times 24 } \)
= 78 x 55
= 4290
Question 34.
Solution:
Let the two prime numbers be 13 and 17, we find the H.C.F. of 13 and 17 as under
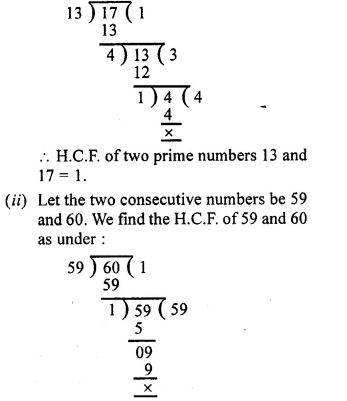
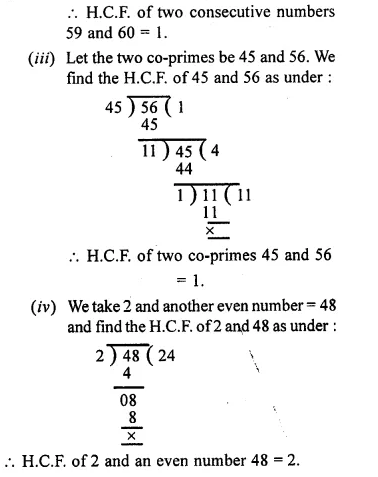
Hope given RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2D are helpful to complete your math homework.
If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.