RS Aggarwal Class 6 Solutions Chapter 5 Fractions Ex 5D
These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5D.
Other Exercises
- RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5A
- RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5B
- RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5C
- RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5D
- RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5E
- RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5F
- RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5G
Question 1.
Solution:
(i) Like fraction : Fractions having the same denominators are called like fractions. For examples:
\(\frac { 2 }{ 11 } ,\frac { 3 }{ 11 } ,\frac { 4 }{ 11 } ,\frac { 5 }{ 11 } ,\frac { 8 }{ 11 } \)
(ii) Unlike fraction : Fraction having the different denominators, are called unlike fractions. For examples:
\(\frac { 1 }{ 3 } ,\frac { 4 }{ 7 } ,\frac { 5 }{ 9 } ,\frac { 3 }{ 8 } ,\frac { 7 }{ 11 } \)
Question 2.
Solution:
We know that like fractions have same denominator
Now \(\frac { 3 }{ 5 } ,\frac { 7 }{ 10 } ,\frac { 8 }{ 15 } ,\frac { 11 }{ 30 } \)
LCM of 5, 10, 15 and 30 = 30
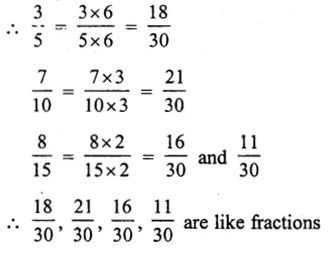
Question 3.
Solution:
We know that like fraction have same denominators
\(\frac { 1 }{ 4 } ,\frac { 5 }{ 8 } ,\frac { 7 }{ 12 } ,\frac { 13 }{ 24 } \)
LCM of 4, 8, 12, 24 = 24
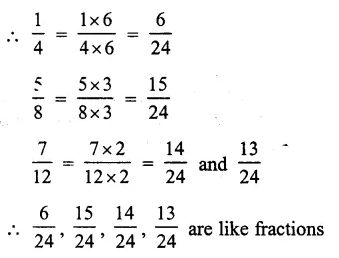
Question 4.
Solution:
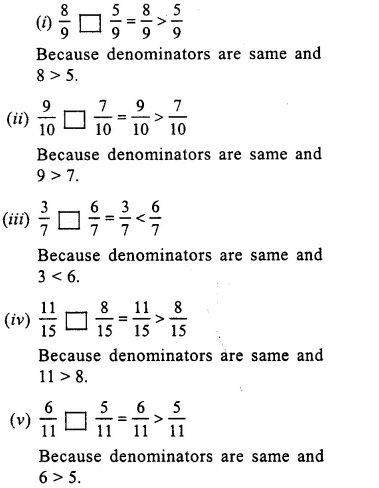
Question 5.
Solution:
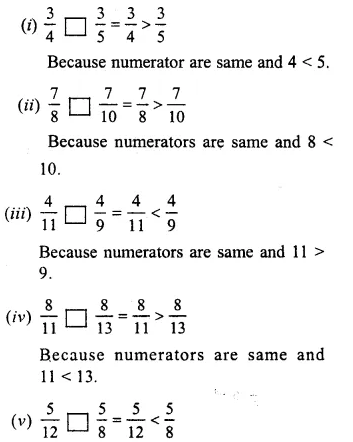
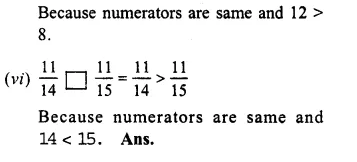
Compare the fractions given below :
Question 6.
Solution:
\(\frac { 4 }{ 5 } and\frac { 5 }{ 7 } \)
LCM of 5 and 7 = 35
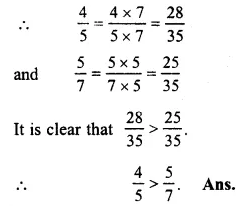
Question 7.
Solution:
\(\frac { 3 }{ 8 } and\frac { 5 }{ 6 } \)
LCM of 8 and 6 = 24
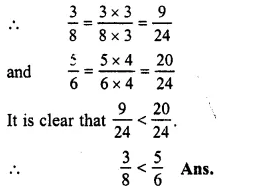
Question 8.
Solution:
\(\frac { 7 }{ 11 } and\frac { 6 }{ 7 } \)
LCM of 11 and 7 = 77
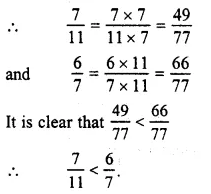
Question 9.
Solution:
\(\frac { 5 }{ 6 } and\frac { 9 }{ 11 } \)
LCM of 6 and 11 = 66

Question 10.
Solution:
\(\frac { 2 }{ 3 } and\frac { 4 }{ 9 } \)
LCM of 3 and 9 = 9
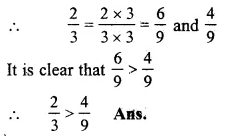
Question 11.
Solution:
\(\frac { 6 }{ 13 } and\frac { 3 }{ 4 } \)
LCM of 13 and 4 = 52

Question 12.
Solution:
\(\frac { 3 }{ 4 } and\frac { 5 }{ 6 } \)
LCM of 4 and 6 = 12
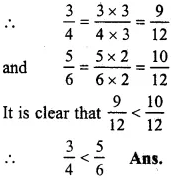
Question 13.
Solution:
\(\frac { 5 }{ 8 } and\frac { 7 }{ 12 } \)
LCM of 8 and 12 = 24
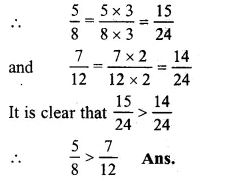
Question 14.
Solution:
\(\frac { 4 }{ 9 } and\frac { 5 }{ 6 } \)
LCM of 9 and 6 = 18
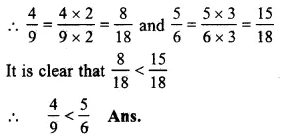
Question 15.
Solution:
\(\frac { 4 }{ 5 } and\frac { 7 }{ 10 } \)
LCM of 5 and 10 = 10
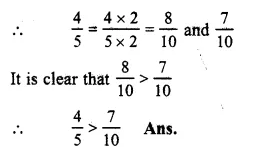
Question 16.
Solution:
\(\frac { 7 }{ 8 } and\frac { 9 }{ 10 } \)
LCM of 8 and 10 = 40
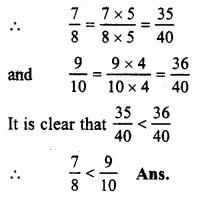
Question 17.
Solution:
\(\frac { 11 }{ 12 } and\frac { 13 }{ 15 } \)
LCM of 12 and 15 = 60
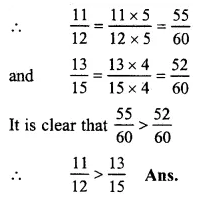
Question 18.
Solution:
\(\frac { 1 }{ 2 } ,\frac { 3 }{ 4 } ,\frac { 5 }{ 6 } and\frac { 7 }{ 8 } \)
LCM of 2, 4, 6 and 8 = 24

Question 19.
Solution:
\(\frac { 2 }{ 3 } ,\frac { 5 }{ 6 } ,\frac { 7 }{ 9 } and\frac { 11 }{ 18 } \)
LCM of 3, 6, 9 and 18 = 18

Question 20.
Solution:
\(\frac { 2 }{ 5 } ,\frac { 7 }{ 10 } ,\frac { 11 }{ 15 } and\frac { 17 }{ 30 } \)
LCM of 5, 10, 15 and 30 = 30

Question 21.
Solution:
\(\frac { 3 }{ 4 } ,\frac { 7 }{ 8 } ,\frac { 11 }{ 16 } and\frac { 23 }{ 32 } \)
LCM of 4, 8, 16 and 32 = 32

Arrange the following fractions in the descending order :
Question 22.
Solution:
\(\frac { 3 }{ 4 } ,\frac { 5 }{ 8 } ,\frac { 11 }{ 12 } and\frac { 17 }{ 24 } \)
LCM of 4, 8, 12 and 24 = 24

Question 23.
Solution:
\(\frac { 7 }{ 9 } ,\frac { 5 }{ 12 } ,\frac { 11 }{ 18 } and\frac { 17 }{ 36 } \)
LCM of 9, 12, 18 and 36 = 36

Question 24.
Solution:
\(\frac { 2 }{ 3 } ,\frac { 3 }{ 5 } ,\frac { 7 }{ 10 } and\frac { 8 }{ 15 } \)
LCM of 3, 5, 10 and 15 = 30

Question 25.
Solution:
\(\frac { 5 }{ 7 } ,\frac { 9 }{ 14 } ,\frac { 17 }{ 21 } and\frac { 31 }{ 42 } \)
LCM of 7, 14, 21 and 42 = 42

Question 26.
Solution:
∴ the numerators are equal
∴ The fraction having small denominator is greater than the fraction having large denominator
∴ In descending order, we can write
\(\frac { 1 }{ 12 } ,\frac { 1 }{ 23 } ,\frac { 1 }{ 7 } ,\frac { 1 }{ 9 } ,\frac { 1 }{ 17 } ,\frac { 1 }{ 50 } \)
Question 27.
Solution:
Here, the numerators of all fractions are equal
∴ The fraction having small denominator is greater than the fraction having large denominator
Now in descending order is
\(\frac { 3 }{ 4 } ,\frac { 3 }{ 5 } ,\frac { 3 }{ 7 } ,\frac { 3 }{ 11 } ,\frac { 3 }{ 13 } ,\frac { 3 }{ 17 } \)
Question 28.
Solution:
Lalita read 30 pages of a book containing 100 pages
She read \(\\ \frac { 30 }{ 100 } \) = \(\\ \frac { 3 }{ 10 } \) part of the book and Sarita read \(\\ \frac { 2 }{ 5 } \) of the book
Now in \(\\ \frac { 3 }{ 10 } \) and \(\\ \frac { 2 }{ 5 } \), LCM of 10, 5 = 10
\(\\ \frac { 3 }{ 10 } \) = \(\\ \frac { 3 }{ 10 } \)
\(\\ \frac { 2 }{ 5 } \) = \(\\ \frac { 2\times 2 }{ 5\times 2 } \) = \(\\ \frac { 4 }{ 10 } \)
From above, Sarita read more
as \(\\ \frac { 4 }{ 10 } \) or \(\frac { 2 }{ 5 } >\frac { 3 }{ 10 } \)
Question 29.
Solution:
Rafiq exercised for \(\\ \frac { 2 }{ 3 } \) hour and Rohit exercised for \(\\ \frac { 3 }{ 4 } \) hour
In \(\\ \frac { 2 }{ 3 } \) and \(\\ \frac { 3 }{ 4 } \), LCM of 3 and 4 = 12
\(\\ \frac { 2 }{ 3 } \) = \(\\ \frac { 2\times 4 }{ 3\times 4 } \) = \(\\ \frac { 8 }{ 12 } \)
\(\\ \frac { 3 }{ 4 } \) = \(\\ \frac { 3\times 3 }{ 4\times 3 } \) = \(\\ \frac { 9 }{ 12 } \)
\(\frac { 9 }{ 12 } >\frac { 8 }{ 12 } \)
=> \(\frac { 3 }{ 4 } >\frac { 2 }{ 3 } \)
∴ Rohit exercised more time
Question 30.
Solution:
In VI A, 20 student passed out of 25 or \(\\ \frac { 20 }{ 25 } \) or \(\\ \frac { 4 }{ 5 } \) students passed
But in VI B, 24 out of 30 passed 24 or \(\\ \frac { 24 }{ 30 } \) or \(\\ \frac { 4 }{ 5 } \) students passed
Now \(\\ \frac { 4 }{ 5 } \) = \(\\ \frac { 4 }{ 5 } \)
∴ Both sections gave same result
Hope given RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5D are helpful to complete your math homework.
If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.