Prasanna

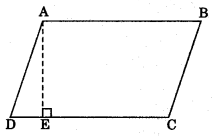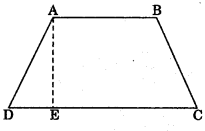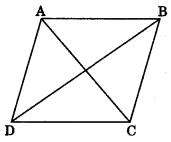
On this page, you will find Probability Class 9 Notes Maths Chapter 15 Pdf free download. CBSE NCERT Class 9 Maths Notes Chapter 15 Probability will seemingly help them to revise the important concepts in less time.

CBSE Class 9 Maths Chapter 15 Notes Probability

Probability Class 9 Notes Understanding the Lesson

1. Experiment: A procedure which produces some well-defined possible outcomes.

2. Random experiment: An experiment which when performed produces one of the several possible outcomes called a random experiment.

3. Trial: When we perform an experiment it is called a trial of the experiment.

4. Event: The set of outcomes of an experiment to which probability is assigned.

5. The empirical (or experimental) probability P(E) of an event E is given by
\(\mathrm{P}(\mathrm{E})=\frac{\text { Number of trials in which } \mathrm{E} \text { has happened }}{\text { Total number of trials }}\)
So, Probability of not happening an event \(\mathrm{P}(\overline{\mathrm{E}})\)= 1 – P(E)

6. The probability of an event lies between 0 and 1 (0 and 1 are included).

7. Impossible event: An event which never happens.

8. Certain event : An event which definitely happens.

On this page, you will find Statistics Class 9 Notes Maths Chapter 14 Pdf free download. CBSE NCERT Class 9 Maths Notes Chapter 14 Statistics will seemingly help them to revise the important concepts in less time.

CBSE Class 9 Maths Chapter 14 Notes Statistics

Statistics Class 9 Notes Understanding the Lesson

There are two types of data:

• Primary
• Secondary.

We can represent the data by:

• Ungrouped and
• Grouped frequency distribution.

Data can also be represented by:

• Bar graph
• Histogram
• Frequency polygons.

Class mark of grouped data
\(=\frac{\text { lower limit }+\text { upper limit }}{2}\)

Measure of central tendencies are mean, median and mode.

Mean:

where, Σf_ix_i = Sum of all observations
Σf_i = Total frequency.

Median: Arrange the observations in ascending or descending order,

(i) If numbers of observations (x) are odd, then median \(\left(\frac{n+1}{2}\right)^{t h}\) terms

(ii) If number of observations (x) are even, then median \(\frac{n^{t h}}{2} \text { and }\left(\frac{n}{2}+1\right)^{t h}\)

Mode: The observation whose frequency is highest.

Relationship between mean, median and mode:
Mode = 3 Median – 2 Mean.

Graphical Representation of Data

• Bar graphs: A bar graph is a pictorial representation of the numerical data by a number of bars (rectangles) of uniform width erected horizontally or vertically with equal space between them. Each rectangle or bar represents only one value of the numerical data and the height or length of bar indicates the corresponding value of the numerical data.
• Histogram: A histogram or frequency histogram is a representation of a frequency distribution in the form of rectangles such that there is no gap between any two successive rectangles.
• Frequency polygon: It is another method of representing frequency distribution graphically.

On this page, you will find Surface Areas and Volumes Class 9 Notes Maths Chapter 13 Pdf free download. CBSE NCERT Class 9 Maths Notes Chapter 13 Surface Areas and Volumes will seemingly help them to revise the important concepts in less time.

CBSE Class 9 Maths Chapter 13 Notes Surface Areas and Volumes

Surface Areas and Volumes Class 9 Notes Understanding the Lesson

Cuboid: With length T, breadth ‘b’ and height ‘h’
(a) Volume = lbh
(b) Total surface area = 2(lb + bh + hl)
(c) Lateral surface area = 2h(l + b) (c)

(d) Diagonal = \(\sqrt{l^{2}+b^{2}+h^{2}}\)
(e) Perimeter = 4(l + b + h)

Cube: With side ‘a’
(a) Volume = a³
(b) Total surface area = 6a²
(c) Lateral surface area = 4a²

(d) Diagonal = \(\sqrt{3} a\)
(e) Perimeter = 12a

Right circular cylinder: With radius ‘r’ and height ‘h’
(a) Volume = πr²h
(b) Curved surface area = 2πrh

(c) Total surface area = 2πr(h + r)

Right circular cone: With radius ‘r’, height ‘h’ and slant height ‘l’
(a) Volume = \(\frac{1}{3}\) πr² h or \(\frac{1}{3}\) x (Area of the base) x height

(b) Curved surface area = πrl, where \(l=\sqrt{h^{2}+r^{2}}\)
(c) Total surface area = πr(l + r)

Sphere: With radius ‘r’
(a) Volume =\(\frac{4}{3}\) πr³

(b) Surface area = 4πr²

Hemisphere: With radius ‘r’
(a) Volume = \(\frac{2}{3}\)πr³
(b) Curved surface area = 2πr²

(c) Total surface area = 3πr²

On this page, you will find Constructions Class 9 Notes Maths Chapter 12 Pdf free download. CBSE NCERT Class 9 Maths Notes Chapter 12 Constructions will seemingly help them to revise the important concepts in less time.

CBSE Class 9 Maths Chapter 12 Notes Constructions

Constructions Class 9 Notes Understanding the Lesson

• Geometrical construction means using only a ruler and a pair of compasses as geometrical instruments.
• Protractor may be used for drawing non-standard angles.

Construction of bisector of a line segment using compass

• Draw a line segment, say AB.
• With both the end points (A and B) of line segment as centre and taking radius of more than half of the measure of line segment, draw the arcs on both sides, which cut at two points on opposite side, say P and Q.
  
• Join these two points.
  This line (PQ) is the required bisector.

Construction of the bisector of a given angle (say ∠ABC)

• With A as centre and a small radius draw an arc, cutting AB at P and AC at Q.
• With P as centre and the same radius as above, draw an arc.
• With Q as centre and with same radius, draw another arc, cutting the previous arc at D.
  
• Join AD.

AD is the required bisector of ∠BCA.

• Construction of some Angies and Triangles ;
• Constructions of some standard angles such as 30°, 45°, 60°, 75°, 90°, 120° etc. are possible using a
  ruler and a pair of compasses. :
• Construction of a triangle is possible, when its base, a base angle and the sum of other two sides are given.
• Construction of a triangle is possible, when its base, a base angle and the difference of other two sides are given.
• Construction of a triangle is possible when its perimeter

On this page, you will find Circles Class 9 Notes Maths Chapter 11 Pdf free download. CBSE NCERT Class 9 Maths Notes Chapter 11 Circles will seemingly help them to revise the important concepts in less time.

CBSE Class 9 Maths Chapter 11 Notes Circles

Circles Class 9 Notes Understanding the Lesson

Circle is the collection of all points in a plane, which are equidistant from a fixed point in the plane. The fixed point is called the centre O and the given distance is called the radius r of the circle.

Concentric circles: Circles having same centre and different radii are called concentric circles.

Arc: A continuous piece of a circle is called an arc of the circle.

Chord: A line segment joining any two points on a circle is called the chord of the circle.

Diameter: A chord passing through the centre of a circle is called the diameter of the circle.

• Semicircle: A diameter of a circle divides it into two equal parts which are arc. Each of these two arcs is called semicircle.
• Angle of semicircle is right angle.
• If two arcs are equal, then their corresponding, chords are also equal.

Theorem 10.1: Equal chords of a circle subtend equal angle at the centre of the circle.
Theorem 10.2: If the angles subtended by the chords of a circle at the centre are equal, then the chords are equal.
Theorem 10.3: The perpendicular drawn from centre to the chord of circle bisects the chord.

Theorem 10.4: The line drawn through the centre of a circle to bisect a chord is perpendicular to the chord. Theorem 10.5: There is one and only one circle passing through three non-collinear points.
Theorem 10.6: Equal chords of circle are equidistant from centre.

Theorem 10.7: Chords equidistant from the centre of a circle are equal in length.

• If two circles intersect in two points, then the line through the centres is perpendicular to the common chord.

Theorem 10.8: The angle subtended by an arc at the centre of circle is twice the angle subtended at remaining part of circumference.
Theorem 10.9: Any two angles in the same segment of the circle are equal.
Theorem 10.10: If a line segment joining two points subtends equal angles at two other points on the same side of the line containing the line segment, the four points lie on a circle (i.e., they are concyclic).

Cyclic Quadrilateral: If all the vertices of a quadrilateral lie on the circumference of circle, then quadrilateral is called cyclic.

Theorem 10.11: In a cyclic quadrilateral the sum of opposite angles is 180°.
Theorem 10.12: In a quadrilateral if the sum of opposite angles is 180°, then quadrilateral is cyclic.

• The exterior angle of a cyclic quadrilateral is equal to the interior opposite angle.

On this page, you will find Areas of Parallelograms and Triangles Class 9 Notes Maths Chapter 10 Pdf free download. CBSE NCERT Class 9 Maths Notes Chapter 10 Areas of Parallelograms and Triangles will seemingly help them to revise the important concepts in less time.

CBSE Class 9 Maths Chapter 9 Notes Areas of Parallelograms and Triangles

Areas of Parallelograms and Triangles Class 9 Notes Understanding the Lesson

1. Area of a parallelogram = base x height
= DC x AE

2. Area of a triangle = \(\frac{1}{2}\)base x height
\(\frac{1}{2}\) BC x AD

3. Area of a trapezium = x (Sum of parallel sides) x Distance between them
\(\frac{1}{2}\) (AB + DC) x AE

4. Area of a rhombus =\(\frac{1}{2}\) \(\frac{1}{2}\) x product of diagonals A B
\(\frac{1}{2}\) x AC x BD

5. Two figures are said to be on the same base and between the same parallels, if they have a common side (base) and the vertices (or the vertex) opposite to the common base of each figure He on a line parallel to the base.

Theorem 9.1: Parallelograms on the same base and between the same parallels are equal in area.
ar(ABCD) = ar(EFCD)

Theorem 9.2: Triangles on the same base and between the same parallels are equal in area.
ar(ΔABC) = ar(ΔPBC)

Theorem 9.3: Two triangles having the same base and equal areas lie between the same parallels.
If a triangle and a parallelogram are on the same base and between the same parallels, then

(i) Area of triangle = \(\frac{1}{2}\) x area of the parallelogram
ar(ΔPDC) = \(\frac{1}{2}\) ar(||gmABCD)

(ii) A diagonal of parallelogram divides it into two triangles of equal areas.
ar(ΔABD) = ar(ΔBCD)

(iii) If each diagonal of a quadrilateral separates it into two triangles of equal area, then the quadrilateral is a parallelogram.

(iv) A median AD of a ΔABC divides it into two triangles of equal areas.
ar(ΔABD) = ar(ΔACD)

On this page, you will find Quadrilaterals Class 9 Notes Maths Chapter 9 Pdf free download. CBSE NCERT Class 9 Maths Notes Chapter 9 Quadrilaterals will seemingly help them to revise the important concepts in less time.

CBSE Class 9 Maths Chapter 9 Notes Quadrilaterals

Quadrilaterals Class 9 Notes Understanding the Lesson

Quadrilateral
A plane figure bounded by four line segments is called quadrilateral.

Properties:

• It has four sides.
• It has four vertices or comers.
• It has two diagonals.
• The sum of four interior angles is equal to 360°.

In quadrilateral ABCD, AB, BC, CD and DA are sides; AC and BD are diagonals and
∠ABC + ∠BCD + ∠CDA + ∠DAB = 360°.

Types of Quadrilaterals
1. Parallelogram: A quadrilateral whose each pair of opposite sides are parallel.

• AB || DC
• AD || BC

2. Rectangle: A parallelogram whose one angle is 90°. Diagonals are equal.

3. Rhombus: A parallelogram whose adjacent sides are equal.
Note: Diagonal bisect each other at 90°.

4. Square: A rectangle whose adjacent sides are equal (four sides are equal). Diagonal bisect each other at 90°.

5. Trapezium: A quadrilateral whose one pair of opposite sides are parallel. AB || DC

6. Kite: It has two pair of adjacent sides that are equal in length but opposite sides are unequal.

Note:

• One of the diagonal bisects the other at right angle.
• One pair of opposite angles are equal.

Properties of a Parallelogram

• Opposite sides are equal.
  e.g., AB = DC and AD = BC
• Consecutive angles are supplementary.
  e.g., ∠A + ∠D = 180°
• Diagonals of parallelogram bisect each other.
• Diagonal divide it into two congruent triangles. A B

Theorem 8.1: A diagonal of a parallelogram divides it into two congruent triangles.
Theorem 8,2: In a parallelogram, opposite sides are equal.
Theorem 8.3: If each pair of opposite sides of a quadrilateral is equal, then it is a parallelogram.
Theorem 8.4: In a parallelogram, opposite angles are equal.
Theorem 8.5: If in a quadrilateral, each pair of opposite angles of a quadrilateral is equal then it is a parallelogram.
Theorem 8.6: The diagonals of a parallelogram bisect each other.
Theorem 8.7: If the diagonals of quadrilateral bisect each other, then it is a parallelogram.
Theorem 8.8: A quadrilateral is a parallelogram if a pair of opposite sides is equal and parallel.

Mid-point Theorem
Theorem 8.9: The line segment joining the mid-points of two sides of a triangle is parallel to the third.
Given: A triangle ABC, E and F are mid-points of sides AB and AC respectively.
i.e., AE = EB and AF = FC
To Prove:
(i) EF || BC
(ii) EF = \(\frac{1}{2}\) BC
Construction: Draw a line through C parallel to AB and extend EF which intersect at D.

Proof: (i) In AAEF and ACDF,
AF = CF (F is the mid-point of AC)
∠AFE = ∠CFD (Vertically opposite angles)
∠EAF = ∠DCF (Alternate interior angles)
∴ ΔAEF = ΔCDF (by ASA congruency)
∴ AE = CD (by CPCT)
and BE = CD (AE = BE)
EF = FD (by CPCT);
Hence, BCDE is a parallelogram.
ED || BC )
∴ EF || BC

(ii) BCDE is a parallelogram.
DE = BC
EF + FD = BC
2EF = BC
EF=\(\frac{1}{2}\)BC

Converse of Mid-Point Theorem

Theorem 8.10: The line drawn through the mid-point of one side of a triangle, parallel to another side  bisects the third side. ‘
Given: ΔABC in which E is the mid point of AB.
EF || BC
To Prove: AF = FC
Construction: Draw CD || AB and extend EF which intersect at D.
Proof: EF || BC
∴ ED || BC
AB || CD
⇒ BE || CD
∴ BCDE is a parallelogram.

Now in ΔAEF and ΔCDF, ∠AFE = ∠CFD (Vertically opposite angles)
∠EAF = ∠DCF (Alternate interior angles)
AE = CD (BE = AE opposite side of a parallelogram and BE = CD
∴ AAEF ≅ ACDF (by AAS congruency)
Hence AF = FC (by CPCT)

On this page, you will find Linear Equations in Two Variables Class 9 Notes Maths Chapter 8 Pdf free download. CBSE NCERT Class 9 Maths Notes Chapter 8 Linear Equations in Two Variables will seemingly help them to revise the important concepts in less time.

CBSE Class 9 Maths Chapter 8 Notes Linear Equations in Two Variables

Linear Equations in Two Variables Class 9 Notes Understanding the Lesson

1. Equation: An equation is a mathematical statement that two things are equal. It consists of two expressions one on each side of an equals sign. For example,7x + 9 = 0

2. An equation in a statement of an equality containing one or more variables.
7x + 3y = 10

3. Linear equation in one variable: A linear equation or first degree equation, in the single variable x is an equation that can be written in the form ax + b = 0 where a, and b are equal numbers, when a≠0.
Examples:

• 2x+3=0
• 3y + 4 = \(\frac{y}{3}\)
• 7x-\(\frac{9}{2}\) =0
• 3x -7y = 73

These equations are solved by applying the properties of real numbers and properties of equality.

4. Solution of a linear equation: The value of the variable which when substituted in place of variable makes both sides of the given equation equal, is called the solution of given equation. These values of variables is also known as root of the equation.
Example:
3x + 4y – 5
Let x – 3, and y = -1
Putting x- 3 and y = -1 in the given equation 3 x 3 + 4 x (-1) = 5
⇒ 9 – 4 = 5
⇒ 5 = 5
∴ LHS = RHS
Hence (3, -1) is a solution of given equation.

5. Linear equation in two variables: A linear equation in two variables is a first degree equation which can be written in the form ax + by + c – 0 and a, b both are non-zero real number. Where a, b and c are real numbers.
Examples:

• 3x + 2y – 9 = 0
• 7x – 4y + 6 = 0

6. Graph of a Linear Equation in two Variables
Graph of a linear equation in two variables is a straight line.

Steps of graphing a line

• If the equation is not in slope intercept form, i.e., y = mx + c, then write the equation in such form.
• Plot they intercept at (0, 6).
• Plot two or three more points by counting the rise and run from the y intercepts.

While solving the equation we should put the following points in our mind.                         •

• We should add or subtract the same number on both the sides of the equation.
• We should multiply or divide by the same non-zero real number on both sides of the equation.

Note:

• A linear equation in one variable has only one solution.
• A linear equation in two variables has infinitely many solutions.

(a) If the slope is positive, count upward for the rise and to the right for the run (also down and left)
Example;    y = \(\frac{2}{3}\) x + 1

(b) If the slope is negative, count downward for the rise and to the right for the run (also up and left)                  Example:    y =\(\frac{2}{3} \)x + 1

7. Draw a line through the points and place arrows on the ends. Extend the line to cover the whole grid (not just connect the two points)

Note:

• The graph of every first degree equation in two variables is a straight line.
• Equation of x-axis is y = 0 (:Hi) Equation of y-axis is x = 0
• The graph of x = a is a straight line parallel to y-axis.
• The graph of y = b is a straight line parallel to x-axis.
• Graph of the equation y = mx (i.e., has no intercepts) is a straight line passing through origin.
  Every point which lies on the graph of the linear equation in two variables is a solution of linear equation.
• Graph of linear equation in one variable
• If given equation is in variable x only then its value represented graphically is on x-axis.
• If the given equation is in variable y only then its value represented graphically is on y-axis.

8. Graph of linear equation in one variable

• If given equation is in variable x only then its value represented graphically is on x-axis.
• If the given equation is in variable y only then its value represented graphically is on y-axis.

For example, 2x = 5 ⇒ x=\(\frac{5}{2}\)
Representation: In one variable,

In Cartesian plane or in two variables,

Draw a line through \(x=\frac{5}{2}\) parallel to y-axis. In such representation, the equation has many solutions.

On this page, you will find Heron’s Formula Class 9 Notes Maths Chapter 7 Pdf free download. CBSE NCERT Class 9 Maths Notes Chapter 7 Heron’s Formula will seemingly help them to revise the important concepts in less time.

CBSE Class 9 Maths Chapter 7 Notes Heron’s Formula

Heron’s Formula Class 9 Notes Understanding the Lesson

1. Area of triangle with base ‘b’ and altitude ‘h’ is
Area = \(\frac{1}{2}\)(b x h)

2. Area of an isosceles triangle with equal sides ‘a’ each and third side b is
Area \(=\frac{b}{4} \sqrt{4 a^{2}-b^{2}}\)

3. Area of an equilateral triangle with side ‘a’ each is
Area=\(\frac{\sqrt{3}}{4} a^{2}\)

4. Area of a triangle by Heron’s formula when sides a, b and c are given is
Area = \(\sqrt{s(s-a)(s-b)(s-c)}\)
Where s = semi-perimeter = \frac{a+b+c}{2}

5. Area of rhombus
Area= \(\frac{1}{2} d_{1} \times d_{2}\)
where d₁ and d₂ are the lengths of its diagonals.

6. Area of trapezium
Area=\(\frac{1}{2}\) (a+b) h
where a and b are parallel sides and h is distance between two parallel sides.

On this page, you will find Coordinate Geometry Class 9 Notes Maths Chapter 6 Pdf free download. CBSE NCERT Class 9 Maths Notes Chapter 6 Coordinate Geometry will seemingly help them to revise the important concepts in less time.

CBSE Class 9 Maths Chapter 6 Notes Coordinate Geometry

Coordinate Geometry Class 9 Notes Understanding the Lesson

Rene Descartes was a French mathematician. He introduced an idea of Carterian Coordinate System for describing the position of a point in a plane. The idea which has given rise to an important branch of Mathematics known as Coordinate Geometry.

1. Cartesian coordinate system: A system which describe the position of a point in a plane is called Cartesian system.

2. Cartesian coordinate axis: Let us draw a horizontal line XX’ and a vertical line YY’ in a plane. Both the lines intersect each other at 90°, then the plane is divided into four parts.

The lines XX’ and YY’ are called axes i.e., XX’ is the x-axis and YY’ is y-axis.

3. Origin: The point where both the axis intersect each other is known as origin.

4. Quadrant
When XX’ and YY’ intersect each other then the plane is divided into four parts. These parts are called quadrants. The plane is known as Cartesian plane or XY plane.

5. Coordinate Geometry: It is a branch of geometry in which geometric problems are solved through algebra by using coordinate system.

6. Cartesian Coordinate (Rectangular Coordinate) System

In this system, the position of a point P is determined by knowing the distances from two perpendicular lines passing through the fixed point O is called origin.
The position of the point P from origin on x-axis is called x-coordinate and the position of P from origin on y-axis is called y-coordinate.

Abscissa: The distance of a point P from y-axis is called abscissa.

Ordinate: The distance of a point P from x-axis is called its ordinate.

Abscissa and ordinate together determine the position of a point in a plane, and it is called coordinates of the point. If a and b are respectively abscissa and ordinate, then the coordinates are (a, b).

Note:

• In first quadrant values of x and y are both positive.
• In second quadrant value of x is negative whereas the value of y is positive.
• In third quadrant value of x and y both are negative.
• In fourth quadrant, the value of x is positive and value ofy is negative.
• Perpendicular distance of a point from x-axis = (+)y-coordinate.
• Perpendicular distance of a point from y-axis = (+)x-coordinate.
• A point which lies on x-axis has coordinates of the form (a, 0).
• A point which lies on y-axis has coordinates of the form (0, b).
• Distance of a point P(x, y) from origin 0(0, 0) =\(\sqrt{x^{2}+y^{2}}\)
  e.g., distance of a point A(4,5) from origin, OA = \(\sqrt{4^{2}+5^{2}}\)
  \(=\sqrt{16+25}=\sqrt{41}\)units

On this page, you will find Triangles Class 9 Notes Maths Chapter 5 Pdf free download. CBSE NCERT Class 9 Maths Notes Chapter 5 Lines and Angles will seemingly help them to revise the important concepts in less time.

CBSE Class 9 Maths Chapter 5 Notes Triangles

Triangles Class 9 Notes Understanding the Lesson

Two geometric figures are said to be congruent if they have exactly the same shape and size.
Note: Congruent means equal in all respect. When one figure is kept over another then it should superimpose on the other to cover it exactly.

If a 500-rupee note is placed over another 500-rupee note then they cover each other.
If 5-rupee coin is placed over another 5-rupee coin of same year, then they cover each other completely.
Congruence of line segments: Two line segments are congruent if they are of the same length. Length of AB = length of CD

Hence, \(\overline{\mathrm{AB}} \cong \overline{\mathrm{CD}}\)

Congruence of angles: Two angles are congruent if they have equal degree measures.

Hence, \(\angle \mathrm{ABC} \cong \angle \mathrm{CDE}\)

Congruence of squares: Two squares are said to be congruent, if they have equal sides.
Hence,

Note: Congruent plane figures are equal in area.

Congruence of circles: Two circles are congruent if they have equal radii.
Hence, Circle C₁ ≅ Circle C₂

Congruent Polygons
Two polygons are said to be congruent if they are the same size and shape. For existence of congruency,
(a) their corresponding angles are equal, and
(b) their corresponding sides are equal.

Congruence Triangles
Two triangles are congruent if they will have exactly the same three sides and three angles.

Axiom 7,1: SAS (Side-Angle-Side) Congruence rule: Two triangles are said to be congruent if two sides and the included angle of one triangle are equal to A D
the two sides and the included angle of the other.

In ΔABC and ΔDEF,
AB = DE
∠ABC = ∠DEF
BC = EF
ΔABC ≅ ΔDEF (by SAS)

Theorem 7.1: ASA (Angle-Side-Angle)
Congruence rule: Two triangles are said to be congruent, if two angles and the included side of one triangle are equal to two angles and the included side of other triangle.
In Δ ABC and Δ DEF,
∠ABC = ∠DEF
BC = EF
∠ACB = ∠DFE
∴ΔABC = ΔDEF (by ASA)

Given: AABC and ADEF in which
∠ABC = ∠DEF, ∠ACB = ∠DFE and BC = EF
To Prove: ΔABC = ΔDEF
Proof: There are three cases arises for primary two congruence of the two triangles.

Case I: Let AB = DE
In ΔABC and ΔDEF,
AB = DE (assumed)
∠ABC = ∠DEF (given)
BC = EF (given)
So ΔABC ≅ ΔDEF (by SAS congruency)

Case II: Let AB > DE. So we can take a point P on AB such that PB = DE.
Now in ΔPBC and ΔDEF
PB = DE (by construction)
∠PBC = ∠DEF (given)
BC = EF (given)
∴ ΔPBC ≅ ΔDEF (by SAS Congruency)
∠PCB = ∠DFE …(1) (by CPCT)
∴ ∠ACB = ∠DFE …(2)
From eqn (1) and (2),
∠PCB = ∠ACB
which is not possible. This is only possible if point P coincides with A.
Hence AB = DE (PB=AB)
So ΔABC = ΔDEF (by SAS congruency)

Case III: If AB < DE, so we take a point Q on DE such that QE = AB
Now in ΔABC and ΔQEF,
AB = QE (by construction)
∠ABC = ∠QEF (given)
BC = EF (given)
Hence ΔABC = ΔQEF (by SAS congruency)
∠ACB = ∠QFE (by CPCT)
But ∠ACB = ∠DFE
Hence ∠QFE = ∠DFE
which is only possible if point Q coincides with D.
∴ AB = DE
Hence ΔABC ≅ ΔDEF (by SAS congruency)

Corollary: AAS (Angle-Angle-Side) congruence rule:
Two triangles are said to be congruent if two angles and one side of one triangle is equal to two angles and one side of another triangle.
In A ABC and A DEF,
∠ACB = ∠DFE . ∠ABC – ∠DEF
AB = DE
∴ ΔABC = ΔDEF (by AAS)

Given: ΔABC and ΔDEF
In which ∠A = ∠D, ∠B = ∠E
and BC = EF
To Prove: ΔABC ≅ ΔDEF
Proof: In ΔABC and ΔDEF
∠1 = ∠4 … (1) (given)
and ∠2 = ∠3 … (2) (given)
Adding eqn. (1) and (2),
∠1 + ∠2 = ∠3 + ∠4
⇒ 180° – (∠1 + ∠2) = 180° – (∠3 + ∠4) (by angle sum property)
∠ACB = ∠DFE
Hence ΔABC ≅ ΔDEF

Theorem 7.2 Angle opposite to equal sides of an isosceles triangle are equal.
If AB = AC, then
∠ABC = ∠ACB
Given: ABC is a triangle in which
AB = AC
To Prove: ∠B = ∠C


Construction:
Draw AD angle bisector of ∠A.
Proof: In ΔBAD and ΔCAD,
AB = AC (given)
∠BAD = ∠CAD (by construction)
AD = AD (common)
.∴ΔBAD = ΔCAD (by SAS)
Hence ∠B = ∠C (by CPCT)

Theorem 7.3. The sides opposite to equal angles of a triangle are equal.
Given: ΔABC in which ∠B = ∠C
To Prove: AB = AC
Construction:
Draw AD bisector of ∠A which meets BC at D.
Proof: In ΔBAD and ΔCAD,
∠B = ∠C
∠BAD – ∠CAD
AD = AD
∴ΔBAD ≅ ΔCAD
Hence AB – AC
Theorem SSS (Side-Side-Side)

Congruence rule: Two triangles are said to be congruent if all sides (three) of one triangle are equal to the all sides (three sides) of another triangles then the two triangle are congruent.
In Δ ABC and Δ DEF,
AB = DF
BC = EF
AC = DE
ΔABC ≅ΔDEF

Theorem 7.5: RHS (Right angle-Hypotenuse-Side)
Congruence rule: If in two right triangles the hypotenuse and one side of one triangle are equal to the hypotenuse and one side of another triangle, their the two triangles are congruent.

In ΔABC and ΔDEF,
∠ABC = ∠DEF
AC = DF
AB = DE
∴ ΔABC ≅ ΔDEF (by R.H.S)

Inequalities in a Triangle
Theorem 7.6: If two sides of a triangle are unequal, the angle opposite to the longer side is greater.
∠ABC > ∠BAC
∴ AC > BC
Given: ΔABC in which
AC > AB
To Prove:
∠ABC > ∠ACB

Construction:
Take a point D on AC such that AD = AB and join BD.
Proof: In Δ ABD,
AB – AD (by construction)
∴  ∠1 = ∠2 …(1) (Angle opposite to equal sides are equal)
∠2 > ∠3 ……..(2)  ∠1 is exterior angle of ΔBCD)

From eqn. (1) and (2),
∠1 > ∠3
Hence ∠ABC > ∠ACB.

Theorem 7.7: In a triangle, side opposite to greater angle is longer.
If ∠ABC > ∠BAC
∴ AC > BC
Given: Δ ABC in which
∠ABC > ∠ACB
To Prove: AC > AB

Proof: Here three cases arises.

• AC = AB
• AC < AB
• AC < AC

Case I: If AC = AB
∠ABC – ∠ACB (Angle opposite to equal sides are equal)
But ∠ABC > ∠ACB (given)
This is a contradiction.
Hence AC ≠AB
∴ AC > AB

Case II: AC < AB
∠ACB > ∠ABC (Angle opposite to longer side is greater)
This is contradiction of given hypothesis.
Hence only one possibility is left.
i.e. AC > AB (It must be true)
Hence AC > AB

Theorem 7.8: The sum of any two sides of a triangle is greater than the third side.
(i) AB + AC > BC
(ii) AB + BC > AC
(iii) BC + AC > AB
Given: ΔABC
Prove that:
(i) AB + BC > AC
(ii) AB + AC > BC
(iii) AC + BC > BC

Construction: BA produce to D such that AD = AC. Join CD.
Proof: In ΔACD,
AC = AD (by construction)
∠2 = ∠1 (Angle opposite to equal sides are equal)
∠2 + ∠3 > ∠1 (∠2 + ∠3 = ∠BCD)
Hence ∠BCD > ∠BDC

Hence BD > BC
⇒ AB + AC > BC (AD = AC by construction)
Similarly, AB + BC > AC
and AC + BC > AB

Median of a triangle: A line segment which joins the mid-point of the side to the opposite vertex. AD is median. D is the mid-point of BC.

Centroid: The point of intersection of all three medians of a triangle is known as its centroid.
Note: Centroid G divides the medians in the ratio 2: 1, i.e., AG : GD = 2: 1

Altitude: Perpendicular drawn from a vertex to the opposite side.

Orthocentre: The point at which all the three altitudes intersect each other is known as orthocentre.

Incentre: The point at which the bisectors of internal angles of a triangle intersect each other is called incentre.

Circumcentre: The point at which perpendicular bisectors of the sides of a triangle intersect each other is called circumcentre.