Prasanna

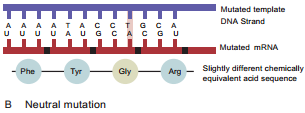
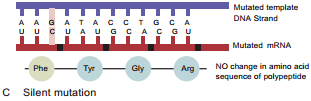
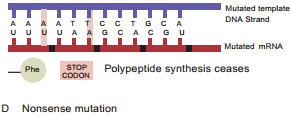
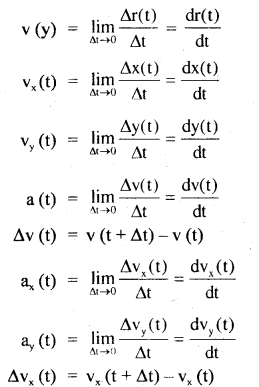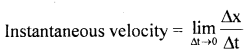By going through these CBSE Class 11 Physics Notes Chapter 5 Law of Motion, students can recall all the concepts quickly.

Law of Motion Notes Class 11 Physics Chapter 5

→ Inertia is proportional to the mass of the body.

→ The force causes acceleration.

→ In the absence of force, a body moves along a straight-line path.

→ If the net external force on a body is zero, its acceleration is zero. Acceleration can be non-zero only if there is a net external force on the body.

→ If a body moves along a curved path, then it is certainly acted upon by a force.

→ C.G.S. and S.I. absolute units of force are dyne and newton (N) respectively and 1 N = 10⁵ dynes.

→ C.G.S. and S.I. gravitational units of force are gm wt. and kg wt. (i.e. kilogram weight) respectively and 1 kg wt = kgf.

→ 1 gm wt = 1 gmf, 1 kg f = 10³ gm f.

→ 1 kg f = 9.8 N.

→ 1 gm f = 1 gm wt = 980 dyne.

→ Impulse = change in momentum.

→ Four types of forces exist in nature, they are gravitational force (F_g), electromagnetic force (F_em), weak force (F_w), and nuclear force (F_n).

→ F_g: F_em: F_w: F_n:: 1: 1025: 1036: 1038.

→ Rocket works on the principle of conservation of linear momentum.

→ Rocket ejects gases backward and as a result, acquires a forward momentum.

→ If Δm is the mass of the gas ejected backward in time At with speed u, then the force acting on the rocket will be:
F = u \(\frac{\Delta m}{\Delta t}\)

→ When a force acting on a particle is always perpendicular to its velocity, then the path followed by the particle is a circle.

→ In a uniform circular motion, the magnitude of velocity always remains constant and only its direction changes continuously.

→ If a body moves with a vertical acceleration a, then its apparent weight is given by:
R = m (g – a)

→ The weight of a body measured by the spring balance in a lift is equal to the apparent weight.

→ The apparent weight of a body falling freely is zero because for it, a = g. It is the case of weightlessness.

→ If the lift falls with a < g, the apparent weight of the body decreases.

→ If the lift accelerates upwards, the apparent weight of the body increases.

→ The true weight of the body = mg.

→ If the lift rises or falls with constant speed, then apparent weight = true weight

→ If the person climbs up along the rope with acceleration ‘a’, then tension in the rope will be T = m (g + a).

→ If the person climbs down along the rope with acceleration ‘a’, then tension in the rope will be T = m (g – a).

→ If the person climbs up or down the rope with uniform velocity, then tension in the string, T = mg.

→ If a body starting from rest moves along a smooth inclined plane of length l, height h and having an angle of inclination 0, then:
1. Its acceleration down the plane is g sin θ.

2. Its velocity at the bottom of the inclined plane will be
\(\sqrt{2 \mathrm{gh}}=\sqrt{2 \mathrm{~g} l \sin \theta}\)

3. Time taken to reach the bottom will be:
t = \(\sqrt{\frac{2 l}{g \sin \theta}}=\left(\frac{2 l^{2}}{g h}\right)^{\frac{1}{2}}\)

= \(\left(\frac{2 \mathrm{~h}}{\mathrm{~g} \sin \theta}\right)^{\frac{1}{2}}=\frac{1}{\sin \theta}\left(\frac{2 \mathrm{~h}}{\mathrm{~g}}\right)^{\frac{1}{2}}\)

4. If the angle of inclination is changed keeping the length constant, then:
\(\frac{\mathrm{t}_{1}}{\mathrm{t}_{2}}=\left(\frac{\sin \theta_{2}}{\sin \theta_{1}}\right)^{\frac{1}{2}}\)

5. If the angle of inclination is changed keeping the height constant, then
\(\frac{\mathrm{t}_{1}}{\mathrm{t}_{2}}=\frac{\sin \theta_{2}}{\sin \theta_{1}}\)

→ A system or a body is said to be in equilibrium when the net force acting on it is zero.

→ If the vector sum of a number of forces acting on a body is zero, then it is said to be in equilibrium.

→ Friction acts opposite to the direction of motion of the body and parallel to the surfaces in contact.

→ Friction depends on the nature of surfaces in contact.

→ Friction is more when the surfaces in contact are rough.

→ Friction is a necessary evil it causes the dissipation of energy. But we need.

→ Friction is of different types such as static friction, kinetic (sliding or rolling) friction, dry friction, wet friction.

→ Static friction is a variable force.

→ The maximum value of static friction is called limiting friction.

→ Static friction is equal and opposite to the force applied to the body.

→ When the applied force is equal to the limiting friction, the body begins to slide.

→ The kinetic friction is less than the limiting friction.

→ The friction on a rolling body is called rolling friction.

→ The rolling friction is less than sliding friction.

→ Friction is a self-adjusting force.

→ The limiting friction is directly proportional to the normal reaction i. e. F ∝ R.

→ The net reactive force acting perpendicular to the surface is called normal reaction (R) and is equal to the force with which the two bodies are pressed against each other.

→ The ratio of limiting friction (F) to the normal reaction (R) is called the coefficient of limiting friction (μ_l) i.e. μ_l = F/R.

→ The limiting friction is independent of the shape or area of surfaces in contact if R = constant.

→ μ_l is a dimensionless constant. It depends on the nature of the surfaces in contact. It is independent of the normal reaction.

→ No work is done against static friction.

→ The kinetic friction opposes the motion of the body.

→ Static friction is the frictional force that comes into play when a body tends to move on the surface of another body.

→ Static friction is due to the interlocking of microscopic projections on the surface of the body.

→ The change from static to kinetic friction is by a stick and slip process. The slip is a break away from the static condition.

→ Sticking is caused by the second interlocking.

→ Kinetic friction is a constant force.

→ It is independent of the applied force.

→ The coefficient of kinetic friction is equal to the ratio of kinetic friction (F_k) to the normal reaction (R) i.e. μ_k = F_k/R.

→ F_k is independent of the area of contact between two bodies.

→ Work is done against kinetic friction.

→ Coefficient of rolling friction (μ_r) = \(\frac{\mathrm{F}_{\mathrm{r}}}{\mathrm{R}}=\frac{\text { rolling friction }}{\text { normal reaction }}\)

→ μ_r < μ_k < μ_s.

→ The friction between two solid surfaces is called dry friction.

→ The friction between a solid surface and a liquid surface is called wet friction.

→ The dry friction causes squeaking of the surfaces trying to move over each other.

→ The dry friction can also cause pleasant sound e.g. the bow under-going stick and slip motion on the string of violin causes pleasant sound.

→ Friction can be decreased by converting dry friction to wet friction.

→ Friction may increase if the surfaces are highly polished. This happens due to cold welding together of the polished surfaces.

→ The angle between the normal reaction and the resultant force of friction and the normal reaction is called the angle of friction (θ).

→ µ = tan θ i.e. coefficient of friction = tan θ.

→ The angle of the inclined plane at which the body placed on it just begins to slide down is called the angle of repose (α) or angle of sliding.

→ µ = tan α.

→ Also α = θ.

→ When a body rotates, all its particles describe circular paths about a line called the axis of rotation.

→ The centers of circles described by the different particles of the rotating body lie on the axis of rotation.

→ The Axis of rotation is perpendicular to the plane of rotation.

→ For uniform circular motion, we have

1. a_c = v²/r = rω²
2. v = rω
3. a ∝ r

where α = angular acceleration.

→ When a body rotates with uniform velocity, its different particles have centripetal acceleration directly proportional to the radius i.e. a_c ∝ r.

→ There can be no circular motion without centripetal force.

→ Centripetal force can be a mechanical, electrical, or magnetic force.

→ In a uniform circular motion, the magnitude of momentum, velocity, and kinetic energy remains constant.

→ Centrifugal force is the pseudo force that is equal and opposite to the centripetal force. It is directed away from the center along the radius.

→ The centrifugal force appears to act on the agency which exerts the centripetal force.

→ The centrifugal force cannot balance the centripetal force because they act on different bodies.

→ The railway tracks and roads are banked for safe turning. The banking angle θ for safe turning is tan θ = \(\frac{\mathbf{v}^{2}}{r g}\) . Also tan θ = \(\frac{\mathrm{h}}{\mathrm{d}}\)
where d = width of road
h = height of the outer edge of the road above the inner edge

→ Maximum speed of the car without overturning when it moves on a circular banked road of radius r is
u_max = \(\sqrt{\frac{\mathrm{grd}}{\mathrm{h}}}\)
when d = \(\frac{1}{2}\) of the distance between two wheels of the car.

→ When a particle of mass m, tied to a string of length is rotated in a horizontal plane with a speed ‘y’, the tension is given by
T = \(\frac{m v^{2}}{r}\)

→ When the string breaks, the particle moves away from the center but tangentially.

→ K.E. of a body rotating in a vertical plane is different at different points.

→ The angle through which the outer edge of the road track is raised above the inner edge is called the angle of banking of roads/ tracks.

→ For safe going of the vehicle round the circular level road, the required condition is:
μ ≤ \(\frac{\mathrm{v}^{2}}{\mathrm{rg}}\)

→ A simple pendulum oscillates in a vertical plane. It will oscillate only if its motion is in the lower semi-circle.

→ For oscillation, the velocity at the lowest point L must be such that the velocity reduces to zero at points M₁ and M₂.

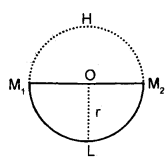
Thus, \(\frac{1}{2}\)mv_e² = mgr
or
v_e = \(\sqrt{2 \mathrm{gr}}\)
i.e v_e ≤ \(\sqrt{2 \mathrm{gr}}\)

→ If v_e > \(\sqrt{2 \mathrm{gr}}\) it will not then oscillate in the lower semi-circle.

→ Minimum velocity that a body should have at the lowest point (L) and highest point (H) of a vertical circle for looping it are
v₁ = \(\sqrt{5 \mathrm{gr}}\) and v₂ = \(\sqrt{\mathrm{gr}}\)
where v₁ and v₂ are velocities at L and H points respectively. Maximum speed with which a vehicle can take a safe turn on a level road is v = \(\sqrt{\mu \mathrm{gr}}\).

→ Maximum speed of the vehicle with which it can take a safe turn on a banked road is given by
v = \(\sqrt{rgθ}\)

→ Sufficient force of friction is there between the tyres of the vehicle and the banked road, then the maximum speed of the vehicle for taking a safe turn is given by

v_max = \(\left(rg\frac{\mu_{\mathrm{s}}+\tan \theta}{1-\mu_{\mathrm{s}} \tan \theta}\right)^{\frac{1}{2}}\)

→ The first law is also called the law of inertia according to which the state of rest or uniform motion of a body remains the same unless acted upon by an external force.

→ The action and reaction always occur in pairs.

→ The position of an event or particle is measured by using a system of coordinates called a frame of reference.

→ There are two types of frame of reference

1. inertial and
2. non-inertial (or accelerated frame of reference).

→ A frame of reference with uniform motion with respect to another inertial frame of reference is also the inertial frame of reference in which the body is situated and obey’s Newton’s law of motion.

→ Inertia: A body at rest or in uniform motion continues in its state unless acted upon by an external force.

→ Force: Force is the action that changes or tends to change the state of rest or uniform motion of a rigid body along a straight line.

→ Rigid body: A body whose various particles move through the same distance parallel to each other under the action of external force i.e. there is no relative motion amongst the various particles of the body under the action of an external force is called a rigid body.

→ Linear momentum (p).: The quantity of motion possessed by a body is called its momentum Mathematically the linear momentum of the body is equal to the product of its mass and velocity i.e.
p = mv

→ Retardation: The quantity of hindrance in the motion of a body is called retardation and the force which retards the body is called retarding force.

→ Newton’s first law of motion: A body continues in its state of rest or uniform motion along a straight line in the absence of external force.

This is called Newton’s first law of motion.
\(\overrightarrow{\mathrm{F}}\) ∞ \(\frac{\mathrm{d}(\overrightarrow{\mathrm{p}})}{\mathrm{dt}}\)
or
\(\overrightarrow{\mathrm{F}}\) = k m\(\overrightarrow{\mathrm{a}}\)

In non-relativistic dynamics \(\frac{\Delta \overrightarrow{\mathrm{v}}}{\Delta \mathrm{t}}=\overrightarrow{\mathrm{a}}\), the acceleration of the body or particle.

When force F, mass m and acceleration arc measured in Newton, kilogram and meter per second respectively. i.e. in S.L units. so that
\(\overrightarrow{\mathrm{F}}\) = m \(\overrightarrow{\mathrm{a}}\)

Its scalar from is F = ma

→ Newton (N): It is the SI unit of measurement of force. One newton is that force that causes an acceleration of 1 ms 2 in a rigid body of mass 1 kg.
∴ 1 N = 1 kg × 1 ms^-2

→ Impulse: The impact of force is called impulse. Mathematically impulse = F × Δt = force × time. So impulse = m(Δv).

→ The inertia of rest: The property of a body to be unable to change its state of rest itself is called the inertia of rest,

→ The inertia of motion: The property of a body by virtue of which it cannot change by itself its state of uniform motion is called inertia of motion.

→ The inertia of direction: The property of a body by virtue of which it cannot change its own direction of motion is called the direction of inertia.

→ Newton’s third law of motion: States that “To every action, there is an equal and opposite reaction.”
F_BA = – F_AB
where F_AB = force exerted on body 8 by body A, and F_BA = force exerted on body A by body B.

→ Law of conservation of linear momentum: The linear momentum of an isolated system of bodies or particles is always conserved, that is it remains constant.

→ Static equilibrium: A body is said to be in static equilibrium if the vector sum of all the forces acting on it is zero. This is a necessary and sufficient condition for a point object only.

→ Lubricants: The substances which are applied to the surfaces to reduce friction are called lubricants.

Important Formulae:
→ Linear momentum of a body of mass m and moving with a velocity v is: p = mv

→ Change in momentum, Δp = m Δ v

→ If two objects of masses M and m have same momentum, then
\(\frac{M}{m}=\frac{v}{V}\)

→ F = ma

→ Resultant of two forces F, and F2 acting simultaneously at angle θ is given by F = F₁ + F₂
The magnitude of F is given by parallelogram law of vectors
F = \(\sqrt{F_{1}^{2}+F_{2}^{2}+2 F_{1} F_{2} \cos \theta}\)

→ The orthogonal components of F and a are:
F = F_xi + F_yj + F_zk
and a = a_xi + a_yj + a_zk

→ Inertial mass, m₁ = \(\frac{\mathrm{F}}{\mathrm{a}}\)

→ Gravitational mass, mg = \(\frac{\mathrm{F}}{\mathrm{g}}\)

→ Impulse I = FΔt = mΔv

→ Newton’s third law of motion:
F₁₂ = – F₂₁
or
m₁a₁ = – m₂a₂

→ Equilibrium of body under three concurrent forces:
F₁ + F₂ + F₃ = 0
Or
F₃ = – (F₁ +F₂)

→ Simple pulley: a = acceleration of masses m₁ and m₂
= \(\left(\frac{m_{2}-m_{1}}{m_{1}+m_{2}}\right)\)
If m₂ > m₁

Tension in the string connecting the two masses and passing over the pulley is given by
T = \(\left(\frac{2 m_{1} m_{2}}{m_{1}+m_{2}}\right)\)g

→ Solving problems using Free Body Diagram Technique:

1. Draw a simple neat diagram of the system as per the given problem.
2. Isolate the object of interest. This is now called a free body.
3. Consider all the external forces acting on the free body and mark them by arrows touching the free body with their line of action clearly represented.
4. Now apply Newton’s second law of motion.
5. In a non-inertial frame consider the pseudo forces like real forces acting on the object in addition to other external forces. The direction of such a force will be opposite to the direction of acceleration of the frame of reference.

By going through these CBSE Class 11 Physics Notes Chapter 4 Motion in a Plane, students can recall all the concepts quickly.

Motion in a Plane Notes Class 11 Physics Chapter 4

→ All physical quantities having direction are not vectors.

→ The following quantities are neither scalars nor vectors: Relative density, density, frequency, stress, strain, pressure, viscosity, modulus of elasticity, Poisson’s ratio, specific heat, latent leat, a moment of Inertia, loudness, spring constant, Boltzman constant, Stefan’s constant, Gas constant, Gravitational constant, Plank’s constant, Rydberg’s constant etc.

→ A vector can have only two rectangular components in a plane and only three rectangular components in space.

→ Vectors cannot be added or subtracted or divided algebraically.

→ Division of two vectors is not allowed.

→ A vector can have any number of components (even infinite in number but a minimum of two components).

→ Two vectors can be added graphically by using head to tail method or by using the parallelogram or triangle law method.

→ A vector multiplied by a real number gives another vector having a magnitude equal to real number times the magnitude of the given vector and having direction same or opposite depending upon whether the number is positive or negative.

→ Multiplication of a vector by -1 reverses its direction.

→ If A + B = C or A + B + C = 0, then A, B and C are in one place.

→ Vector addition obeys commutative law
i.e. A + B = B + A

→ Vector addition obeys associative law
i.e. (A + B) + C = A + (B + C)

→ Subtraction of B from A is defined as the sum of
– B + A i.e. A – B = A + (-B)

→ The angle between two equal vectors is zero.

→ The angle between -ve vectors is 180°.

→ Unit vector  = \(\frac{\mathbf{A}}{|\mathbf{A}|}\) .

→ The magnitude of  = 1.

→ The direction of A is the same as that of the given vector along which it acts.

→ The resultant of two vectors of unequal magnitudes can never be a null vector.

→ î, ĵ, k̂ are the unit vectors acting mutually perpendicular to each other along X, Y and Z axes respectively and are called orthogonal unit vectors.

→ î.î = ĵ.ĵ = k̂.k̂ = 1

→ î.ĵ = ĵ.k̂ = k̂.î = 0

→ î × î = ĵ × ĵ = k̂ × k̂-= 0

→ î × ĵ = k̂, ĵ × k̂ = î, k̂ × î = ĵ

→ A × A = 0 , Also A – A = 0
But A × A ≠ A – A as A × A ⊥ A and A – A is collinear with A.

→ The cross product:

1. Is not commutative (i.e. don’t obey commutative law):
   i. e. A × B ≠ B × A
   = B × A (anticommutative law)
2. obeys distributive law i.e.
   A × (B + C) = A × B + A × C

→ Vectors lying in the same plane are called co-planer vectors.

→ Vectors are added according to triangle law, parallelogram law, and polygram law of vector addition.

→ The maximum resultant of two vectors A and B is
|R_max| = |A| + |B|

→ The minimum resultant of two vectors A and B is
|R_max| = |A| – |B|

→ The minimum number of vectors lying in the same plane whose results can be zero is 3.

→ The minimum number of vectors that are not co-planar and their results can be zero is 4.

→ A minimum number of collinear vectors whose resultant can be zero is 2.

→ A vector in component form is A = A_xî + A_yĵ +A_zk̂

→ Magnitude of A is = \(\sqrt{A_{x}^{2}+A_{y}^{2}+A_{z}^{2}}\).

→ A projectile is any object thrown with some initial velocity and then it moves under the effect of gravity alone.

→ The trajectory is the path followed by the projectile during its flight.

→ Its trajectory is always parabolic in nature.

→ Two-dimensional motion: The motion of an object in a plane is a two-dimensional motion such as the motion of an arrow shot at some angle and then moving under gravity.

→ Three-dimensional motion: The motion of an object in space is called a three-dimensional motion, for example, the motion of a free gas molecule.

→ Scalar quantities: The quantities which do not need direction for their description are called scalar quantities. Only the magnitude of the quantity is needed to express them correctly. Such quantities are distance, mass, density, energy, temperature etc.

→ Vector quantities: The quantities which need both magnitude and direction for their correct description are called vector quantities. They also obey the law of the addition of vectors. For example displacement, velocity, acceleration, force, momentum etc. are vector quantities.

→ Triangle law of vector addition: If the two vectors are represented by the two adjacent sides of a triangle taken in order, their resultant is given in magnitude and direction by the third side of the triangle taken in the opposite order.

→ Parallelogram law of vector addition: If two vectors acting simultaneously at a point are represented by the two adjacent sides of a parallelogram, then their resultant is completely given in magnitude and direction by the diagonal of the parallelogram passing through that point.

→ Unit vector: A unit vector is a vector in the direction of a given vector whose magnitude is unity. It is represented by a cap or a hat over letter e.g. n̂, î, ĵ, k̂, x̂, ŷ, ẑ etc. The unit vectors in the cartesian coordinate system along the three axes are generally written as î, ĵ and k̂ such that |î| = |ĵ| = |k̂| = 1.

→ Uniform velocity: The uniform or constant velocity is the one in which the moving object undergoes equal displacements in equal intervals of time.

→ Speed: The magnitude of velocity is known as speed. It is the distance travelled divided by the time taken.

→ Uniform acceleration: When the velocity of an object changes by equal amounts in equal intervals of time, the object is said to be having uniform acceleration.

→ Projectile: Projectile is a particle or an object projected with some initial velocity and then left to move under gravity alone.

→ The uniform circular motion: The motion of an object in a circular path with constant speed and constant acceleration (magnitude) is called a uniform circular motion.

→ Equal vectors: Two vectors are said to be equal if they have the same magnitude and act in the same direction.

→ Negative vector: A vector having the same magnitude as the given vector but acting in exactly the opposite direction is called a negative vector.

→ Co-initial vectors: Vectors starting from the same initial point are called co-initial vectors.

→ Zero vector or Null vector: The vector whose magnitude is zero but the direction is uncertain (or arbitrary) is called a zero or null vector. It is represented by 0.

→ Collinear vectors: Two vectors acting along the same or parallel lines in the same or opposite directions are called collinear vectors.

→ Fixed vector: A vector whose tail point or initial point is fixed is called a fixed vector.

→ Free vector: A vector whose initial point or tail is not fixed is called a free vector.

→ Polygon law of addition of vectors: It states that if a number of vectors are represented by the sides of a polygon taken in the same order, then their resultant is given completely by the closing side of the polygon taken in the opposite order.

→ Rectangular components of a vector in a plane: The resolution of a vector into two mutually perpendicular components in a plane is called rectangular resolution and each component is called a rectangular component.

→ Rectangular components in a plane: The components of a vector along three mutually perpendicular axes are called the rectangular component of a vector in space.

→ Scalar product of vectors: If the multiplication of two vectors yields a scalar quantity, the multiplication is called a scalar or dot product. This is because of the fact that multiplication is denoted by a dot (.) between the multiplying vectors e.g. A.B = AB cos θ, where θ is the angle between the two vectors.

→ Cross or vector product: When the multiplication of two vectors is shown by a cross (×) between them, it is called a cross product. The resultant is also a vector quantity e.g. A × B = C. This multiplication is, therefore, also known as the vector product.

Important Formulae:
→ Uniform circular motion: Time period T second, frequency
v = \(\frac{1}{T}\)
Angular velocity ω = \(\frac{θ}{T}\),
ω = \(\frac{2 \pi}{\mathrm{T}}\) = 2πv,
v = \(\frac{1}{T}\),
θ = \(\frac{l}{r}\)
or
l = rθ.

→ Angular acceleration: α = \(\frac{\omega_{2}-\omega_{1}}{t_{2}-t_{1}}=\frac{d \omega}{d t}\)
average acceleration, a_av = \(\frac{v_{2}-v_{1}}{t_{2}-t_{1}}\)
Average angular acceleration,

→ Time for maximum height: t = \(\frac{\mathrm{u} \sin \theta}{\mathrm{g}}\)

→ Angle of projection of maximum horizontal range:
θ = \(\frac{π}{4}\) or 45°.

→ Angles for same range θ, (\(\frac{π}{2}\) – θ)

→ General position – velocity – acceleration relations:
Δr (t) = r(t + Δt) – r(t)
v(t) = \(\frac{\Delta \mathrm{x}(\mathrm{t})}{\Delta \mathrm{t}}\);

vx(t) = \(\frac{\Delta \mathrm{x}(\mathrm{t})}{\Delta \mathrm{t}}\),

vy(t) = \(\frac{\Delta \mathrm{y}(\mathrm{t})}{\Delta \mathrm{t}}\)

Δx(t) = x(t + Δt) – x(t)
Δy(t) = y(t + Δt) – y(t)

→ Since: A.A = A², so

• î.î =1,
• ĵ.ĵ =1,
• k̂.k̂ =1

As î, ĵ and k̂ are mutually perpendicular so
î.ĵ = ĵ.k̂ =0,
k̂.î = 0

→ A.(B + C) = A.B. +A.C

→ Vector product:
A × B = C = |A| |B|sin θ n̂
In cartesian coordinates,
A × B = (A_xî + A_yĵ + A_zk̂) × (B_xî + B_yĵ + B_zk̂)
= (A_yB_z – A_zB_y) î + (A_zB_x – A_xB_z)ĵ + (A_xB_y – A_yB_x)k̂
= \(\left|\begin{array}{ccc}
\hat{\mathrm{i}} & \hat{\mathbf{j}} & \hat{\mathbf{k}} \\
\mathrm{A}_{\mathrm{x}} & \mathrm{A}_{\mathrm{y}} & \mathrm{A} \\
\mathrm{B}_{\mathrm{x}} & \mathrm{B}_{\mathrm{y}} & \mathrm{B}
\end{array}\right|\)

→ A × B ≠ B × A
= -B × A

→ |A × B|² + |A . B|² = 2|\(\overrightarrow{\mathrm{A}}\)|²|\(\overrightarrow{\mathrm{B}}\)|²

→ Direction cosines:
cos α = \(\frac{A_{x}}{A}\) = l,
cos β = \(\frac{A_{y}}{A}\) = m, and
cos γ = \(\frac{A_{z}}{A}\) = n

→ l² + m² + n² = 1

→ Velocity: v = vxi + vyj

→ Speed: v = |v| = (v_x² + v_y²)^1/2 .

→ Distance travelled in time t:
x(t)î + y(t)ĵ = x(0)î + y(0)ĵ + (v_xî + v_yĵ)t

→ x (t) = x (0) + v_xt

→ y (t) = y (0) + v_yt

→ Average velocity:
V_average = \(\frac{\left|r\left(t^{\prime}\right)-r(t)\right|}{t^{\prime}-t}=\frac{r_{12}}{\left(t_{2}-t_{1}\right)}=\frac{\Delta r}{\Delta t}\)

→ Instantaneous velocity:

→ Scalar product of A and B is
A . B = AB cos θ, where θ = angle between A and B.

→ Scalar (or Dot) product always gives a scalar quantity.

→ When A. B = 0 then A and B are perpendicular to each other.

→ A . B in component form is
A.B = A_xB_x + A_yB_y + A_zB_z.

→ Cross product of A and B is
A × B = (AB sin θ) n̂ = C
where n̂ = unit vector ⊥ to the plane containing A and B i.e. n̂ acts along C.

→ If we move anticlockwise, n is vertically upward i.e. +ve.

→ If we move clockwise, n vertically downward i.e. -ve.

→ Maximum height attained by the projectile fired at an angle 0 with the horizontal with velocity u is
H = \(\frac{\mathbf{u}^{2} \sin ^{2} \theta}{2 \mathrm{~g}}\)

→ Time of flight = T = \(\frac{2 u \sin \theta}{g}\)

→ Time of maximum height attained = Time of ascent = Time of descent = \(\frac{u \sin \theta}{g}\)

→ Horizontal range of the projectile is R = \(\frac{\mathrm{u}^{2} \sin 2 \theta}{\mathrm{g}}\)

→The range of projectile is maximum if θ = 45°.

→ Rmax = \(\frac{\mathrm{u}^{2}}{\mathrm{~g}}\)

→ When the range is maximum, the maximum height attained by the projectile (Hm) is
H_m = \(\frac{u^{2}}{4 g}=\frac{R_{\max }}{4}\)

→ For R_max , T_max = \(\frac{\mathrm{u}}{\sqrt{2} \mathrm{~g}}\)

→ When θ = 90, H_max = \(\frac{u^{2}}{2 g}\) and is twice the maximum height attained by the projectile when range is maximum.

→ For θ = 90°, Time of flight is Maximum = \(\frac{2 \mathrm{u}}{\mathrm{g}}\)

→ Horizontal range is same for two angles of projections i.e. θ and 90 – θ with the horizontal.

→ If an object is moving in a plane with constant acceleration a, then a = \(\sqrt{\mathrm{a}_{\mathrm{x}}^{2}+\mathrm{a}_{\mathrm{y}}^{2}}\)

→ If r0 be the position vector of a particle moving in a plane at time t = 0, then at any other time t, its position vector will be
r = r_o + v_ot + \(\frac{1}{2}\) at²
where v0 = its velocity at t = 0.

→ Its velocity at time t will be v = v_o + at.

→ When the object moves in a circular path at constant speed, then its motion is called uniform circular motion. The angle described by the rotating particle is called angular displacement.

→ Angular displacement, Δθ = \(\frac{\Delta l}{\mathrm{r}}\) .

→ Angular velocity, ω = \(\frac{\Delta \theta}{\Delta \mathrm{t}}\)

Instantaneous angular velocity, ω = \(\frac{\mathrm{d} \theta}{\mathrm{dt}}\)

ω = \(\frac{2 \pi}{\mathrm{T}}\) = 2πv (∵ v = \(\frac{1}{T}\))

Angular velocity (ω) of a rigid body rotating about a given axis is constant, so v is different for different particles of the body.

Angular acceleration α = \(\frac{\mathrm{d} \omega}{\mathrm{dt}}=\frac{\mathrm{d}^{2} \theta}{\mathrm{dt}^{2}}\)

Tangential acceleration is a₁ = α × r and a_t is directed along the tangent to the circular path.

→ Centripetal acceleration (a_c) is given by a_c = \(\vec{\omega}\) × v and it is directed towards the centre of the circular path. Thus acceleration of the particle is
a = a_t + a_c
then a_t ⊥ a_c
∴ |a| = \(\sqrt{a_{1}^{2}+a_{c}^{2}}\)

→ Also \(\vec{\omega}\) ⊥v as to and a are parallel to Δθ. i.e. they are directed along the axis of rotation
Hence a_c = ω v sin 90
ac = ω v = ω . rω = rω²
= \(\frac{v^{2}}{r}\)

→ Centripetal force, F_c = ma_c = \(\frac{m v^{2}}{r}\) = mrω².

→ Fc is always directed towards the centre of the circular path.

→ The Axis of rotation is perpendicular to the plane of rotation.

→ There can be no circular motion without centripetal force. Centripetal force can be a mechanical, electrical or magnetic force in nature.

→ Fc is always ⊥ to the velocity of the particle.

→ θ, ω, α are called axial vectors or pseudo vectors.

→ Tangential acceleration is equal to the product of angular acceleration and the radius of the circular path i.e. at = rα.

By going through these CBSE Class 11 Physics Notes Chapter 3 Motion in a Straight Line, students can recall all the concepts quickly.

Motion in a Straight Line Notes Class 11 Physics Chapter 3

→ Mechanics is divided into three main branches: Statics, Kinematics and Dynamics.

→ Distance is a scalar quantity.

→ Displacement is a vector quantity.

→ An object is said to be in motion if it changes its position w.r.t. its surroundings as time passes.

→ An object is said to be at rest or it does not change its position w.r.t. its surroundings as time passes.

→ Both rest and motion are relative terms.

→ Distance travelled by a moving body can never be zero or negative i.e. it is always positive.

→ Displacement can be positive, negative or zero.

→ The magnitude of displacement = distance only if a body moves in a straight line without a change in direction.

→ The magnitude of the displacement of a body is the minimum possible distance, so distance ≥ displacement.

→ Speed is a scalar quantity.

→ Velocity is a vector quantity.

→ When a body moves with variable speed, then the average speed of the body is calculated as:
Average speed = \(\frac{\text { Total distance travelled by the body }}{\text { Total time taken }}\)

→ When a body moves with variable velocity, then the average velocity of the body is calculated as:
Average velocity = \(\frac{\text { Total displacement }}{\text { Total time taken }}\)

→ Distance travelled by an object in a given time interval is equal to the area under the velocity-time graph.

→ The direction of velocity and acceleration may not necessarily be the same.

→ The velocity and acceleration of a body may not be zero simultaneously. When the body is in equilibrium, its acceleration is zero.

→ In one, two and three dimensional motions, the object changes its position w.r.t. one, two and three coordinate axes respectively.

→ At a particular instant of time, any point may be chosen as a reference or zero points.

→ The events taking place before the zero time are assigned negative number and events after zero are assigned +ve number.

→ A suitable unit of time say, second, minute or hour may be chosen. In fact, zero points of time and unit of time are chosen according to one’s convenience.

→ The position is also measured with respect to a chosen zero position or origin on the path line.

→ Positions to the right of origin are represented by a positive number and a unit.

→ The position to the left of the origin is represented by a negative number and the unit.

→ For motion in the vertical direction, we can use ‘up’ or ‘down’ instead of ‘right’ and ‘left’.

→ The position is always stated with respect to time,

→ x (t) shows that x is a function of time t.

→ The shift in position x (t’) – x (t) is called the displacement.

→ The rate of change, of displacement, is called velocity.

→ The motion in which an object covers equal distances in equal intervals of time is called uniform motion.

→ Uniform motion may be represented by a straight line parallel to the time axis in a velocity-time graph.

→ It is also represented by a straight line inclined at some angle. The magnitude of velocity is speed.

→ The velocity of a body w.r.t. another body is called its relative velocity.

→ The x-t graph is a straight line parallel to the time axis for a stationary object.

→ Uniformly accelerated motion is a non-uniform motion.

→ When the velocity of the body decreases with time it is said to be decelerated or retarded.

→ When a particle returns to the starting point, its average velocity is zero but the average speed is not zero.

→ For one dimensional motion, the angle between acceleration and velocity is either zero or 180°. It may also change with time.

→ For two dimensional motion, the angle between acceleration and velocity is other than 0° or 180°. It may also change with time.

→ If the angle between a and v is 90°, the path of motion is a circle.

→ If the angle between a and v is other than 0° or 180°, the path of the particle is a curve.

→ For motion with constant acceleration, the graph between x and t is a parabola.

→ For uniform motion, the average velocity is equal to the instantaneous velocity.

→ Statics: It deals with the law of composition of forces and with the conditions of equilibrium of solid, liquid and gaseous states of the objects.

→ Kinematics: It is the branch of mechanics that deals with the study of the motion of objects without knowing the cause of their motion.

→ Dynamics: It is the branch of mechanics that deals with the study of the motion of objects by taking into account the cause of their motion.

→ Point object: It is defined as an object having its dimensions much smaller as compared to the distance covered by it.

→ Acceleration: It is defined as the change in velocity with time i. e.

→ Speed: Theatre of covering distance with time is called speed i.e.
speed = \(\frac{\text { Distance }}{\text { Time }}\)

→ Average speed: It is the ratio of total path length traversed and the corresponding time interval.

→ Velocity: The rate of change of displacement is called velocity.

→ Average velocity: When an object travels with different velocities, its rate of motion is measured by its average velocity.
Average velocity = \(\frac{x_{2}-x_{1}}{t_{2}-t_{1}}=\frac{\Delta x}{\Delta t}\)

→ Instantaneous velocity: The velocity of the object at any particular instant of time is known as instantaneous velocity.

→ V_inst = \(\frac{\mathrm{dx}}{\mathrm{dt}}\)

→ Uniform velocity: A motion in which the velocity of the moving object is constant is called uniform and the velocity is called the uniform velocity. In uniform motion, the object covers equal distances in equal intervals of time along a straight line.

→ Relative velocity: The rate of change in the relative position of an object with respect to the other object is known as the relative velocity of that object.

→ Acceleration: The time rate of change of velocity is known as acceleration.

→ Average acceleration: It is defined as the change in velocity divided by the time interval.
a_av = \(\frac{\text { Final velocity – Initial velocity }}{\text { Change in time }}=\frac{v_{2}-v_{1}}{t_{2}-t_{1}}=\frac{\Delta v}{\Delta t}\)

→ Instantaneous acceleration: The acceleration of an object at any instant of time is called instantaneous acceleration. It is also the limiting value of average acceleration.

→ Retardation: The negative acceleration due to which the body slows down is known as deacceleration or retardation.

→ Non-uniform motion: An object is said to have non-uniform motion when its velocity changes with time even though it has a constant acceleration.

Important Formulae:
→ Displacement in time from t to t’ = x(t’) – x (t)

→ Average velocity, vav = \(\frac{\mathrm{x}\left(\mathrm{t}^{\prime}\right)-\mathrm{x}(\mathrm{t})}{\mathrm{t}-\mathrm{t}}=\frac{\Delta \mathrm{x}}{\Delta \mathrm{t}}\)

→ The relative velocity of a body A w.r.t. another body B when they are moving along two parallel straight paths in the same direction is V_AB = V_A – V_B and if they are movinig in opposite direction, then V_AB = V_A – (-V_B) = V_A + V_B.

Average Speed V_av = \(\frac{\mathrm{S}_{1}+\mathrm{S}_{2}}{\left(\frac{\mathrm{S}_{1}}{\mathrm{v}_{1}}+\frac{\mathrm{S}_{2}}{\mathrm{v}_{2}}\right)}\)
Where S₁ is the distance travelled with velocity v₁ and S₂ is the distant travelled with velocity v₂.

→ If S₁ = S₂, then vav = \(\frac{2 v_{i} v_{2}}{v_{1}+v_{2}}=\frac{2}{\frac{1}{v_{1}}+\frac{1}{v_{2}}}\)

→ Average speed of a body when it travels with speeds v₁, v₂, v₃…..v_n in time intervals t₁, t₂, t₃,… t_n, respectively is given by
V_av = \(\frac{v_{1} t_{1}+v_{2} t_{2}+v_{3} t_{3}+\ldots .+v_{n} t_{n}}{t_{1}+t_{2}+t_{3}+\ldots+t_{n}}=\frac{\sum_{i=1}^{n} v_{i} t_{i}}{\sum_{i=1}^{n} t_{i}}\)

→ Distance travelled by a body moving with uniform velocity is S = ut.

→ Velocity of an object after a time t in uniformly accelerated motion is, v = u + at.

→ Distance covered by an object after a time t in accelerated motion is, S = ut + \(\frac{1}{2}\)at².

→ Velocity of an object after covering a distance S in uniformly accelerated motion is, v² – u² = 2aS.

→ Distance covered in nth second by a uniformly accelerated object
Snth = u + \(\frac{a}{2}\)(2n – 1)

→ Total time a flight = Time of Ascent + Time of descent.

→ Time of Ascent = Time of descent.

By going through these CBSE Class 11 Physics Notes Chapter 2 Units and Measurement, students can recall all the concepts quickly.

Units and Measurement Notes Class 11 Physics Chapter 2

→ Physical Quantity = numerical value × unit = nu

→ Numerical value (n) ∝ \(\frac{1}{\text { size of unit(u) }}\)

→ Physical quantities which are independent of each other are called fundamental quantities.

→ Units of fundamental quantities are called fundamental units.

→ There are four systems of units namely FPS, CGS, MKS, and S.I. system.

→ 1 a. m.u.= 1.66 × 10^-27kg.

→ The product of n and u is called the magnitude of the physical quantity.

→ Force, thrust, and weight have the same SI unit, i.e. Newton.

→ Pressure, stress, and coefficient of elasticity have the same SI unit, i.e. Pascal.

→ The standard unit must not change with time and space. That is why the atomic standards for length and time have been defined.

→ The dimensions of many physical quantities especially those of heat, electricity, thermodynamics, and magnetism in terms of mass, length, and time alone become irrational, so SI is adopted which uses 7 basic units and two supplementary units.

→ The first conference on weights and measures was held in 1889.

→ Sevres near Paris is the headquarter of the International Bureau of Weights and Measures.

→ SI system was first adopted in the 11th general Conference of Weights and Measures in 1960.

→ S.I. system is also known as the rationalized M.K.S. system.

→ The various units of the S.I. system are rational in nature.

→ The various units of the S.I. system are coherent in nature.

→ It is wrong to say that the dimensions of force are [MLT^-2]. On the other hand, we should say that the dimensional formula for force is [MLT^-2].

→ The dimensional formula for the dimensionless physical quantity is written as [M°L°T°].

→ The dimensions of a physical quantity don’t depend on the system of units.

→ The dimensional formula is very helpful in writing the unit of a physical quantity in terms of the basic units.

→ The pure numbers are dimensionless.

→ Physical quantities defined as the ratio of two similar quantities are dimensionless.

→ The physical relations involving logarithm, exponential, trigonometric ratios, numerical factors, etc. cannot be derived by the method of dimensional analysis.

→ Physical relations involving addition or subtraction sign cannot be derived by the method of dimensional analysis.

→ If units or dimensions of two physical quantities are the same, these need not represent the same physical characteristics.

→ Torque and work have the same dimensions but have different physical characteristics.

→ Measurement is most accurate if its observed value is very close to the true value.

→ Significant figures are the number of digits up to which we are sure about their accuracy.

→ Significant figures don’t change if we measure a physical quantity in different units.

→ Significant figures retained after the mathematical operation (like addition, subtraction, multiplication, or division) should be equal to the minimum significant figures involved in any physical quantity in the given operation.

→ Error = Actual value: Observed value.

→ Absolute error: Δx_i = \(\overline{\mathrm{x}}\) – x_i

→ The absolute error in each measurement is equal to the least count of the measuring instrument.

→ Mean absolute error
Δx = \(\frac{1}{x} \sum_{i=1}^{n}\)(Δx₁)

→ When we add or subtract two measured quantities, the absolute error in the final result is equal to the sum of the absolute errors in the measured quantities.

→ When multiply or divide two measured quantities, the relative error in the final result is equal to the sum of the relative errors in the measured quantities.

→ For greater accuracy, the quantity with higher power should have the least error.

→ Smaller is the least count higher is the accuracy of measurement.

→ The relative error is a dimensionless quantity.

→ The unit and dimensions of the error are the same as that of the quantity itself.

→ The larger the number of significant digits after the decimal point in measurement, the higher is the accuracy of measurement.

→ Physical quantities: Physical quantities may be defined as the quantities in terms of which physical laws can be expressed and which can be measured directly or indirectly.

→ Subjective methods: The methods of measurement which depend on our senses are called subjective methods.

→ Objective methods: The methods of measurement which make use of scientific instruments are called objective methods.

→ Fundamental quantities: The quantities which are independent of each other and which are not generally defined in terms of other physical quantities are known as fundamental or basic quantities.

→ Derived quantities: The quantities whose defining operations are based on the fundamental physical quantities are called derived quantities.

→ Unit: A unit is defined as the reference standard of measurement.

→ If a number is without a decimal point and ends in one or more zeros, then all the zeros at the end of the number may not be significant.

→ To make the number, of Significant digits clear, it is suggested that the number may be written in exponential form.

→ For example, 20300 may be expressed as 203.00 × 10², to suggest that all the zeros at the end of 20300 are significant.

→ Fundamental or basic units: The basic units are those which can neither be derived from one another nor can be resolved into further units! For example units of length, mass and time, etc. These are 7 in number.

→ Derived units: The units of all those physical quantities which can be expressed in terms of fundamental units are called derived units. For example, units of velocity, force, and energy, etc.

→ Size of a physical quantity: The size of a physical quantity is determined by a unit and the number of times that unit is to be repeated to represent the complete quantity.
Size of a physical quantity = nu;
n = number of times the chosen unit is contained in the physical quantity,
u = size of the unit.

→ System of units: Complete set of units both for fundamental and derived quantities is known as a system of units.

→ S.I. Units: Systeme international of units, in short, is called S.I. units.
It has seven fundamental units namely

1. unit of length is meter (m),
2. kilogram (kg) unit of mass,
3. second (s) unit of time,
4. ampere (A) unit of current,
5. Kelvin (K) unit of temperature,
6. Candela (cd) unit of light intensity and
7. mol (mole) for a unit of amount of substance.

→ There are two supplementary units for measuring: (a) plane angle and solid angle. These are radian (rad) and steradian (sr) respectively.

→ θ(rad) = \(\frac{\text { arc }}{\text { radius }}=\frac{l}{r}\)

→ Ω(sr) = \(\frac{\text { surface area }}{(\text { radius })^{2}}=\frac{\Delta \mathrm{A}}{\mathrm{r}^{2}}\)

→ Length: It is defined as a measure of separation between two points in space.

→ Mass: It is the amount of substance contained in the body. Inertial mass: It is the mass of the body which is a measure of inertia F
∴ m = \(\frac{F}{a}\)

→ Gravitational mass: It is the mass of the body that determines the gravitational pull due to the earth acting on the body.
∴ m = \(\frac{W}{g}\)

→ Fermi (F): It is a unit of extremely small distances:
1 F = 10^-15 m.

→ Angstrom (A): It is the unit of length at the atomic level:
1 A = 10^-10 m ,

→ Astronomical unit (AU): It is the unit of length at a large scale:
1 A.U. = 1.496 × 10¹¹ m= 1.5 × 10¹¹ m.

→ Light year- It is defined as the distance traveled by light in one year
1 L.Y. = 9.46 × 10¹⁵ m.

→ Meter (m): Metre is the unit of length and is defined as the space occupied by 1,650,763.73 wavelengths of orange-red light emitted by krypton: 86 kept “at the triple point of nitrogen (radiation emitted due to transition between the levels 2P₁₀ and 5d₅).

→ Kilogram (kg): Kilogram is the unit of measurement of mass. It is the mass of international prototype platinum-iridium cylinders kept in the International Bureau of Weights and Measures at Sevres, France.

→ Second(s): It is the unit of time. A second is the duration of time corresponding to 9,192,631,770 vibrations corresponding to the transition between two hyperfine levels of cesium-133 atom in the ground state.

→ Ampere(A): An ampere of current is defined as the constant current, which when flowing through two straight parallel conductors of infinite length and negligible area of cross-section placed lm apart in air produces a force of 2 × 10^-7 Nm^-1.

→ Parsec: This unit is used to measure very large distances i.e., the distance between stars or galaxies.
1 Parsec = 3.08 × 10¹⁶m

→ Atomic mass unit (AMU): It is the unit of mass at the atomic and subatomic levels.
1 amu = \(\frac{\left(\text { mass of }_{6} C^{12} \text { atom }\right)}{12}\)

→ Dimensions: The dimensions of a physical quantity are the powers to which the fundamental units of length, mass and time have to be raised to obtain its units, e.g., dimensions of force [MLT^-2] are 1 in mass 1 in length and -2 in time.

→ Dimensional formula: Dimensional formula of a physical quantity is defined as the expression that indicates which of the fundamental units of mass, length, and time appear into the derived unit of that physical quantity and with what powers.

→ Dimensional equation: The equation obtained by equating the physical quantity to its dimensional formula is called the dimensional equation of that physical quantity.

→ Dimensional variables: The variable quantities which have dimensions are called dimensional variables! For example, velocity, force, momentum, etc.

→ Dimensionless variables: These are variable physical quantities that do not have dimensions. For example, relative density, specific heat, strain, etc.

→ Dimensional constants: Those constants which have dimensions are called dimensional constants. For example, gravitational constant, Planck’s constant.

→ Dimensionless constants: Those constants which do not have, dimensions are dimensionless constants. For example, all trigonometric functions, natural numbers 1, 2, 3…. π, e.

→ Significant figure: The significant figures are a measure of the accuracy of a particular measurement of a physical quantity. Significant figures in measurement are those digits in a physical quantity that are known reliably plus the one-digit which is uncertain.

→ Error: It is the difference between a true and measured value of a physical quantity.

→ Discrepancy: The difference between the two measured values of a physical quantity is known as a discrepancy.

→ Constant error: It is an error in measurements. It arises due to some constant causes such as faulty calibration on the instrument. This error remains constant in all observations.

→ Systematic error: This error is also a measurement error. The error is one that always produces an error of the same sign. This error may be due to imperfect technique, due to alteration of the quantity being measured, or due to carelessness and mistakes on the part of the observer.

→ Instrumental error: This is a constant type of error. These are errors of an apparatus and that of the measuring instruments used e.g., zero error in vernier calipers or screw gauge.

→ Error due to least count: This also is another type of constant error. The error due to the limitations imposed by the least counts of the measuring instruments comes under this heading.

→ Observational or Personal Error: This is a subheading of systematic error. This error is due to the experimental arrangement or due to the habits of the observer.

→ Error due to physical conditions: These errors are due to the experimental arrangement or due to the habits of the observer. These are also systematic errors.

→ Error due to unavoidable situations: These errors are due to the imperfectness of the apparatus or of non-availability of ideal conditions.

→ Random errors: The errors due to unknown causes are random errors.

→ Gross error: These types of errors are because of the carelessness of the observer.
These errors may be due to

• negligence towards sources of error due to overlooking of sources of error by the observer;
• the observer, without caring for least count, takes wrong observations;
• wrong recording of the observation.

→ Absolute error: The magnitude of the difference between the true value and the measured value is called absolute error.

→ A relative error: It is defined as the ratio of the mean absolute error to the true value.

→ Percentage error: The relative error expressed in percentage is percentage error.

→ Standard error: The error which takes into account all the factors affecting the accuracy of the result is known as the standard error.

→ Standard deviation: The root means the square value of deviations (the deviation of different sets of observations from the arithmetic mean) is known as standard deviation.
Standard deviation σ = \(\sqrt{\frac{\left(\mathrm{x}_{1}-\overline{\mathrm{x}}\right)^{2}+\left(\mathrm{x}_{2}-\overline{\mathrm{x}}\right)^{2}+\left(\mathrm{x}_{\mathrm{n}}-\overline{\mathrm{x}}\right)^{2}}{\mathrm{n}}}=\sqrt{\frac{\mathrm{S}}{\mathrm{n}}}\)

→ Probable error: The error calculated by using the principle of probability are probable errors. According to Bessels formula

→ Probable error e = ± 0.6745\(\sqrt{\frac{S}{n(n-1)}}\)

→ Standard error = \(\sqrt{\frac{\mathrm{S}}{n(n-1)}}\)

Important Formulae:
→ t = Size of oleic acid molecule = thickness of film of oleic acid
= \(\frac{\text { Volume of film }}{\text { Area of film }}\)

→ Inertial mass determination:
\(\frac{m_{1 i}}{m_{2 i}}=\frac{T_{1}^{2}}{T_{2}^{2}}\) where T₁ and T₂ are of the time of oscillation of inertia balance with inertial masses.

→ Gravitational mass determination:
\(\frac{\mathrm{w}_{1}}{\mathrm{w}_{2}}=\frac{\mathrm{m}_{\mathrm{g}_{1}}}{\mathrm{~m}_{\mathrm{g}_{2}}}\)
where m_g1 and m_g2 are gravitational masses.

→ Height by triangulation method:

1. The height of an accessible object, h = x tanθ, where θ = angle of elevation of the object at the point of observation at a distance x from it.
2. The height of the inaccessible object is:
   h = \(\frac{x}{\cot \theta_{2}-\cot \theta_{1}}\)
   where θ₁ and θ₂ are the angles made at two points of observation at distance x from each other.

→ Distance of stars (parallax method):
S = \(\frac{\mathrm{b}}{\theta}\), θ = Φ₁, + Φ₂, where Φ₁, and Φ₂, are the angles subtended by star on observer on Earth with an interval of 6 months.
θ = angle of parallax.
b = basis = distance between two points on the surface of earth.

→ n₂ = n₁ \(\left[\frac{\mathrm{m}_{1}}{\mathrm{~m}_{2}}\right]^{a}\left[\frac{\mathrm{L}_{1}}{\mathrm{~L}_{2}}\right]^{b}\left[\frac{\mathrm{T}_{1}}{\mathrm{~T}_{2}}\right]^{\mathrm{c}}\)

→ Distance by reflection method (Radar) is given by
d = \(\frac{c \times t}{2}\) where
c = velocity of light in vacuum
t = time in which it is covered twice.

→ d = \(\frac{\mathrm{ut}}{2}\) for Sonar, where u = velocity of sound waves.

→ Diameter of moon is D = Sθ, where θ is the angle made by the diameter of moon at the observer, S = distance of observer from the moon, D = diameter of moon or an astronomical object.

→ Radius of atom is r = \(\left(\frac{M}{2 \pi N \rho}\right)^{1 / 3}\)
Where N = Avogadro’s number
M = molecular weight of the substance
ρ = density of substance.

→ Relative error = \(\frac{\Delta \mathrm{x}}{\mathrm{x}}\)

→ % error = \(\frac{\Delta \mathrm{x}}{\mathrm{x}}\) × 100

→ Error in sum or difference form, ± Δz = ± Δp ± Δq

→ Maximum error in product or quotient form, \(\frac{\Delta z}{z}=\frac{\Delta p}{p}+\frac{\Delta q}{q}\)

→ % Error in power form,\(\frac{\Delta \mathrm{z}}{\mathrm{z}}\) × 100 = n\(\frac{\Delta \mathrm{p}}{\mathrm{p}}\) × 100

By going through these CBSE Class 11 Physics Notes Chapter 1 Physical World, students can recall all the concepts quickly.

Physical World Notes Class 11 Physics Chapter 1

→ Physics deals with nature and natural phenomenon.

→ Science is the knowledge acquired by man in an organised way.

→ The various steps involved in acquiring knowledge are:

1. systematic observations
2. reasoning
3. model making
4. a theoretical prediction.

→ The theory is the explanation of the behaviour of a physical system using a limited number of laws.

→ A theory is valid if it is able to explain satisfactorily most of the relevant measurements.

→ There is a certain amount of overlapping between Physics, Chemistry and Biology.

→ Advances in Physics are directly related to the advances in experimental observations.

→ Advances in Physics lead to the development of concepts.

→ A wide diversity in the physical world can be understood on the basis of a few concepts.

It is due to three reasons:
(a) Strict regularities and laws help in quantitative measurements.
(b) There is a small number of common and basic principles covering enormous diversities of scales of the phenomenon.
(c) It is easier to understand a phenomenon by separating important features from unimportant features.

→ The technological development of any society is very closely related to the application of Physics and other branches of science.

→ Measurements are the heart of Physics. In fact, Physics is also defined as the science of measurements.

→ Motion, energy, gravitation, properties of matter in bulk and their atomic origin, study of details of mechanical oscillations and waves, description of matter with a microscope all form a systematic study.

→ Science: An organised attempt of man to know and the knowledge he acquires is science.

→ Physics: It is the subject which deals with nature and natural phenomenon and their quantitative measurements.

→ Scientific method: Scientific method involves systematic observation, reasoning, model making and theoretical prediction altogether.

→ Theory: A scientific theory is the explanation of the natural phenomenon in terms of a limited number of laws.

→ Geocentric theory: It is a theory in which the earth is assumed to be at the centre of the universe.

→ Heliocentric theory: The sun is at the centre of the world consisting of Earth and other planets.

→ Corpuscular theory of light: Newton assumed light to be made up of corpuscles or particles.

→ Hydroelectric energy: Conversion of gravitational energy into ‘ electric energy through water.

→ Thermal power: Conversion of chemical energy of coal by burning it into electric energy.

→ Geothermal energy: It is the heat in the depth of the Earth.

→ Gravitational force: The force is an attraction between two masses is called the gravitational force. This force of attraction between the two bodies is directly proportional to the product of their masses and inversely proportional to the square of the distance between them. The constant of proportionality is called the Gravitational constant or constant of gravitation G.
F = G\(\frac{m_{1} m_{2}}{r^{3}}\)r̂
Its scalar form is F = G\(\frac{m_{1} m_{2}}{r^{2}}\)

→ Constant of gravitation ‘G’: It is equal to the force of attraction acting between two masses each of 1 kg placed 1 m apart in the air.

→ Electromagnetic force: The combined electrostatic and magnetic force between charged particles and magnetic poles is called electromagnetic force.

→ Nuclear or strong forces: The strong attractive forces between particles in a nucleus are called nuclear forces. This force can act within a distance of 10-15m. These forces are charge independent
i. e. even a proton attracts another proton.

→ Weak forces: The forces of interaction between elementary particles are weaker than the strong forces and these activities within a distance of about 10^-12 m.