Category: CBSE Class 10

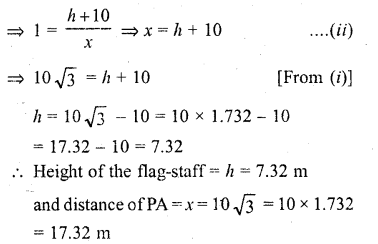
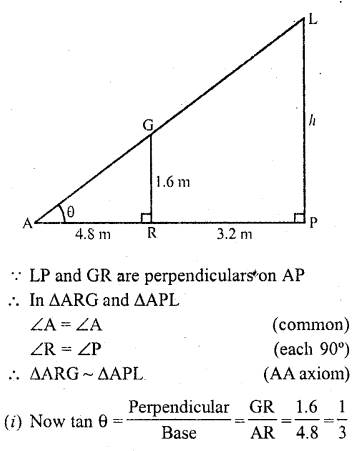
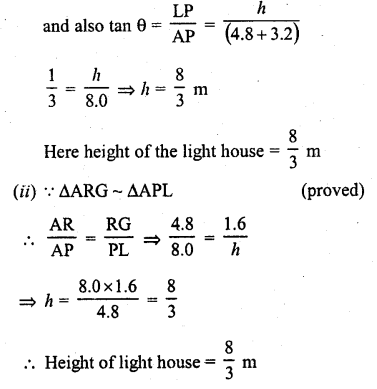
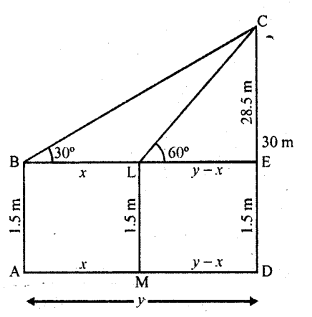
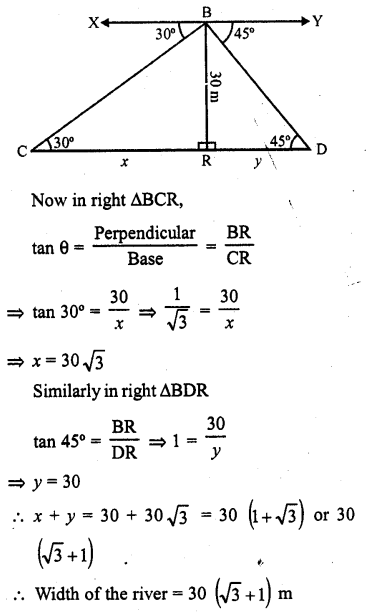
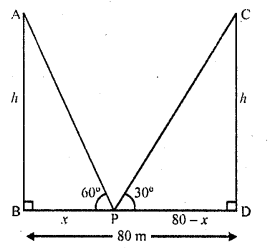
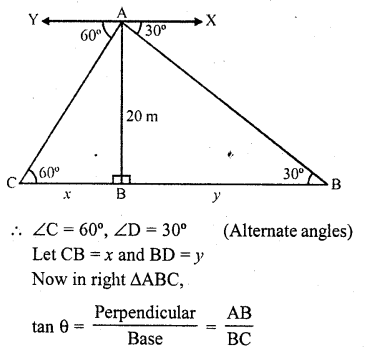
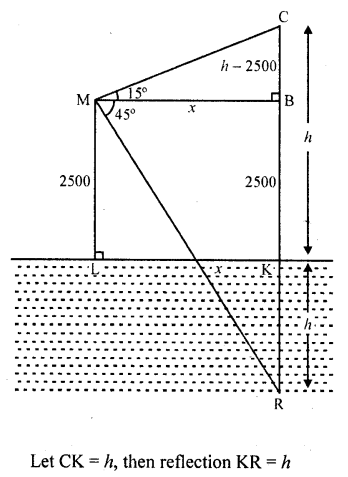
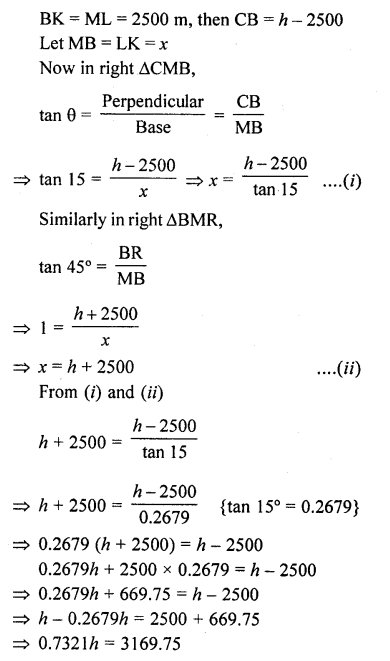
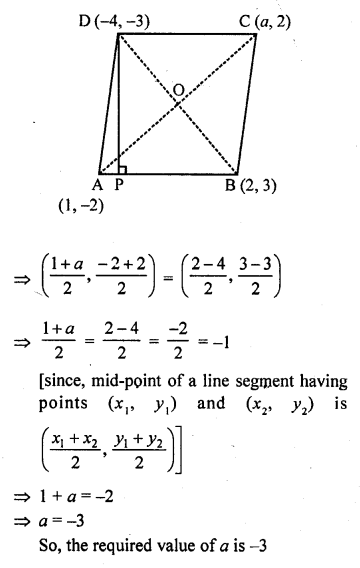
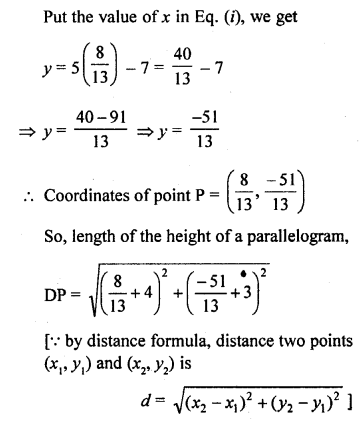
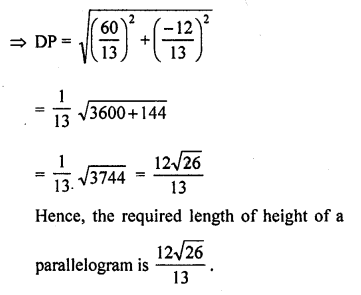
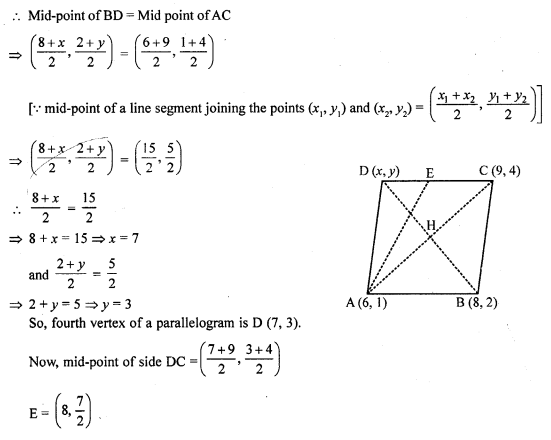
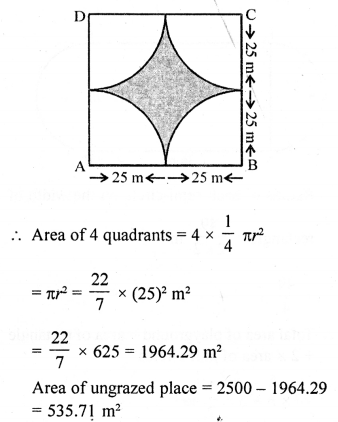
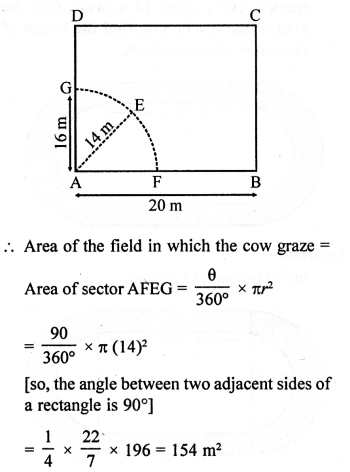
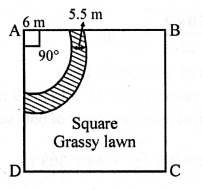
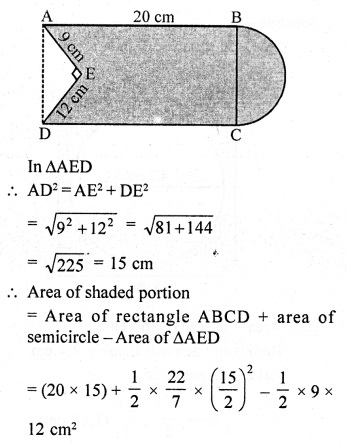
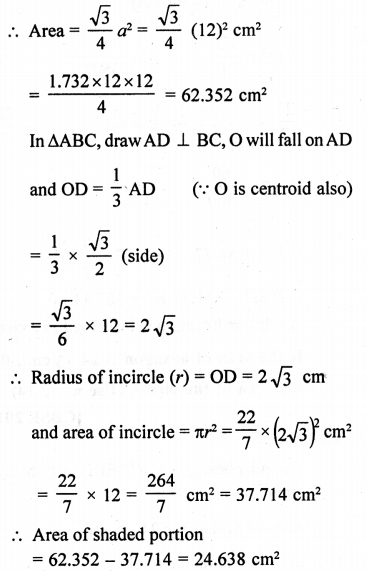
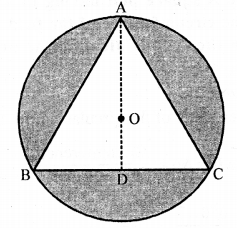
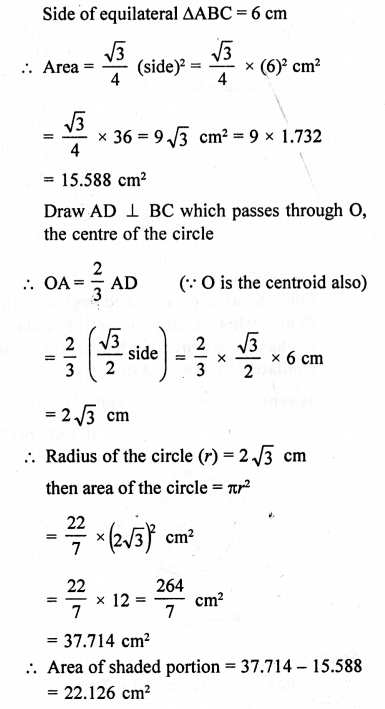
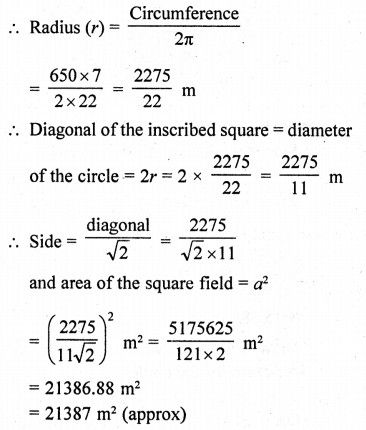
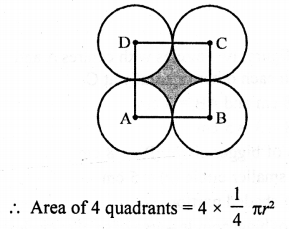
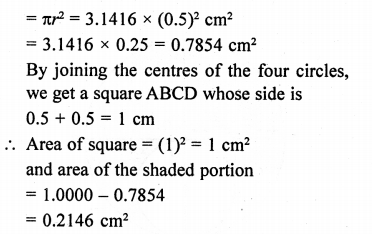
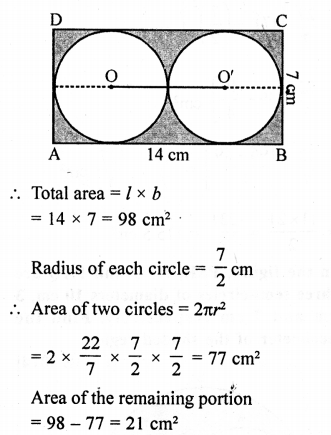
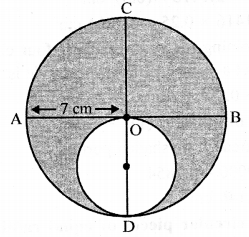
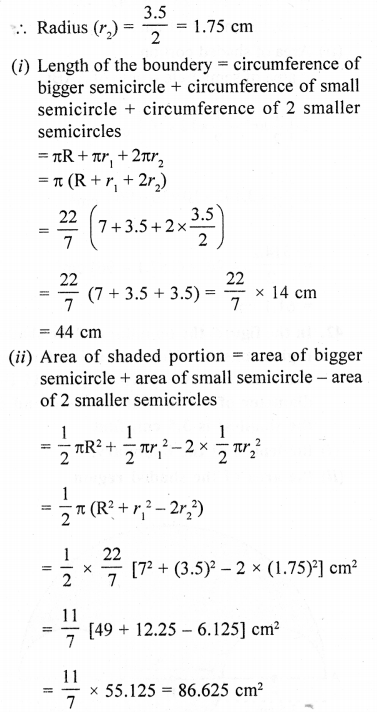
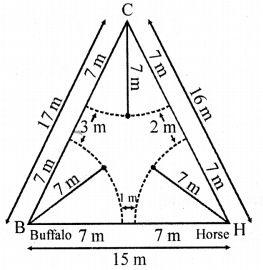
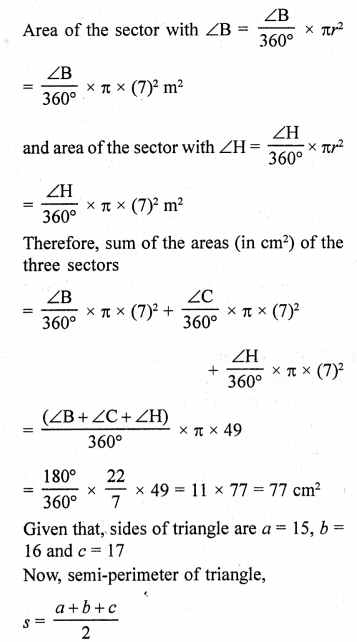
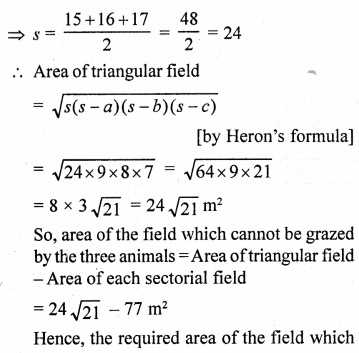
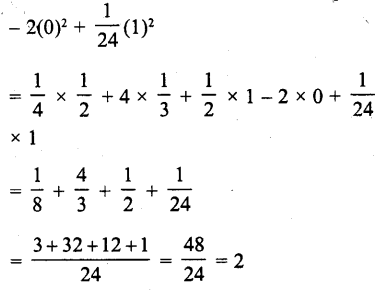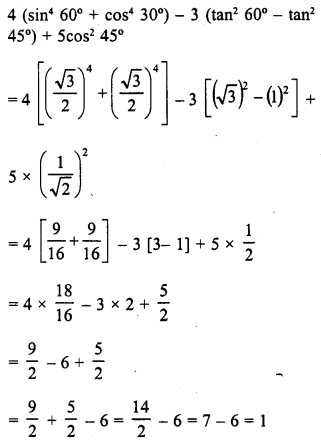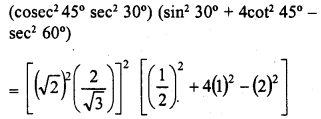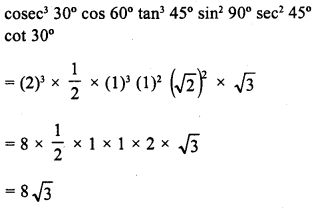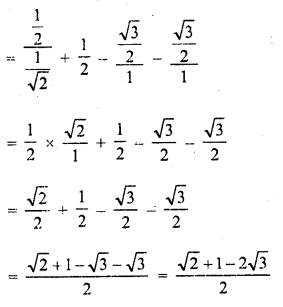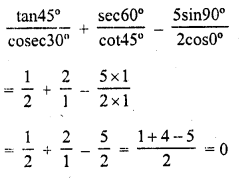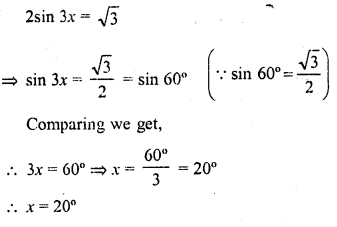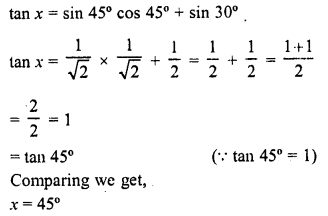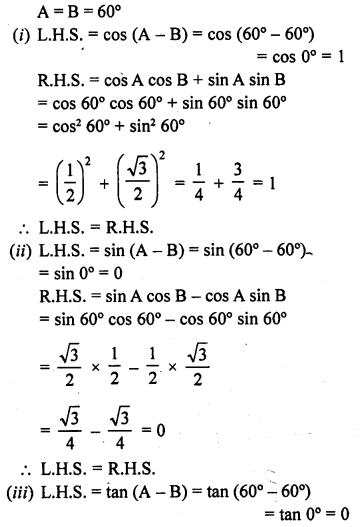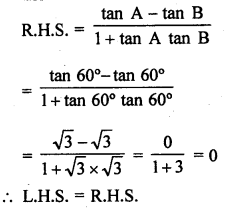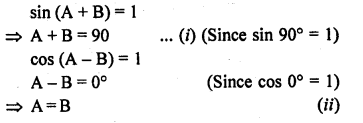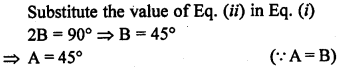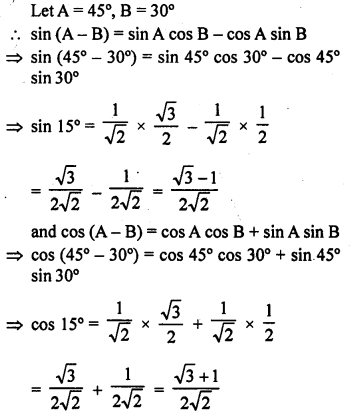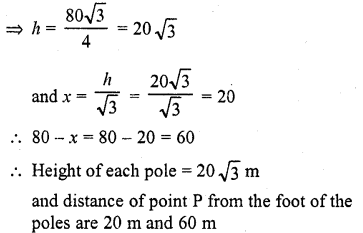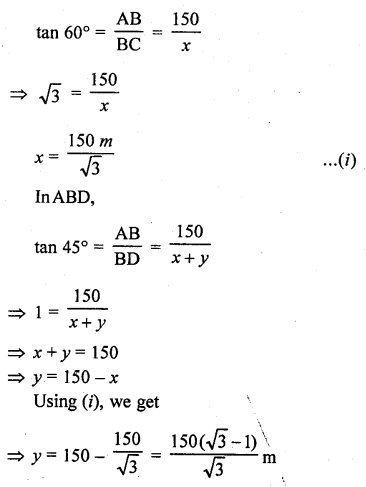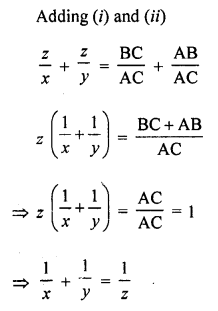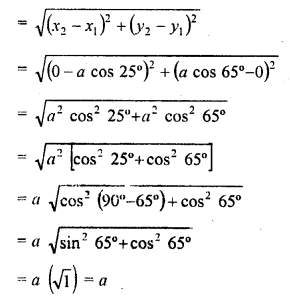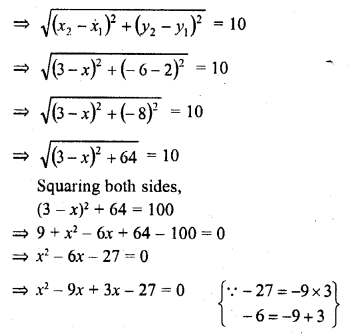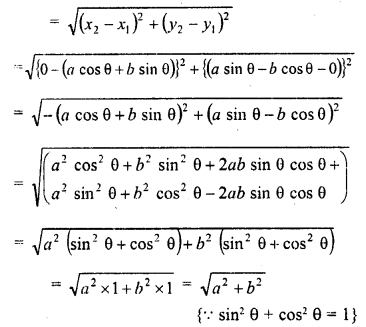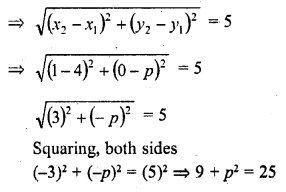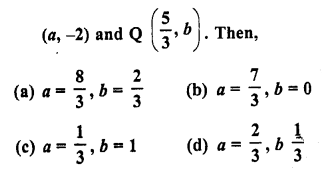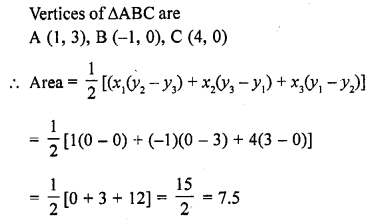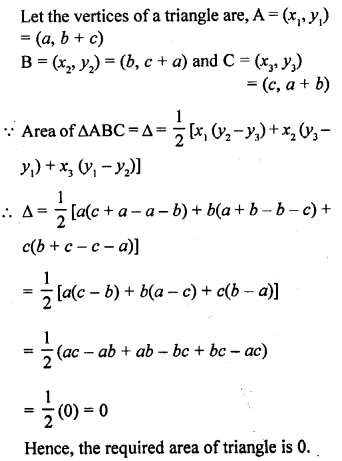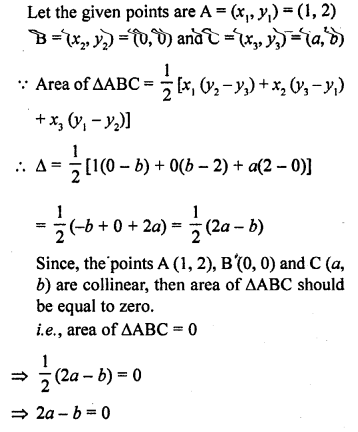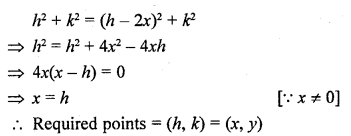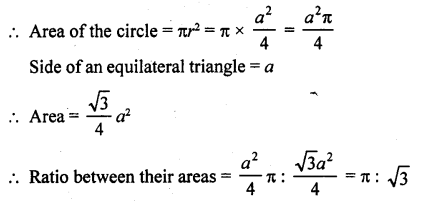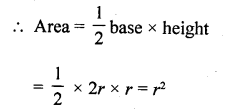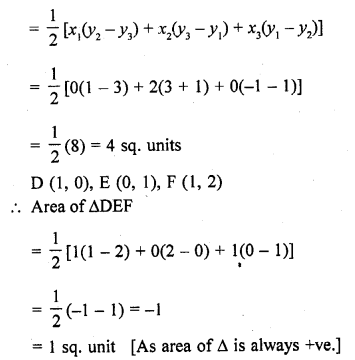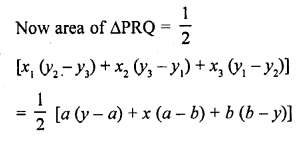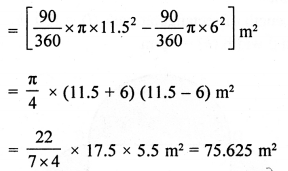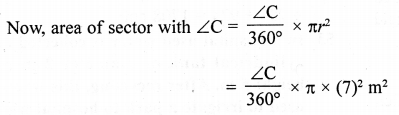RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.6

These Solutions are part of RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.6

Other Exercises

• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.1
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.2
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.3
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.4
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.5
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.6
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.7
• RD Sharma Class 10 Solutions Chapter 7 Triangles Revision Exercise
• RD Sharma Class 10 Solutions Chapter 7 Triangles VSAQS
• RD Sharma Class 10 Solutions Chapter 7 Triangles MCQS

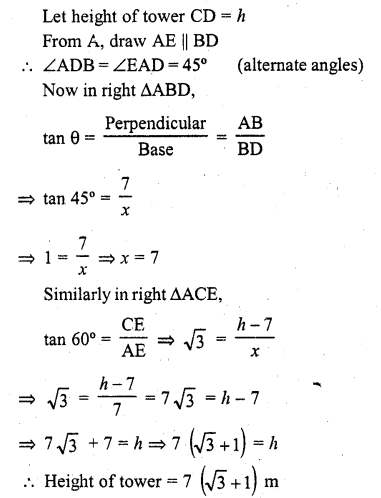
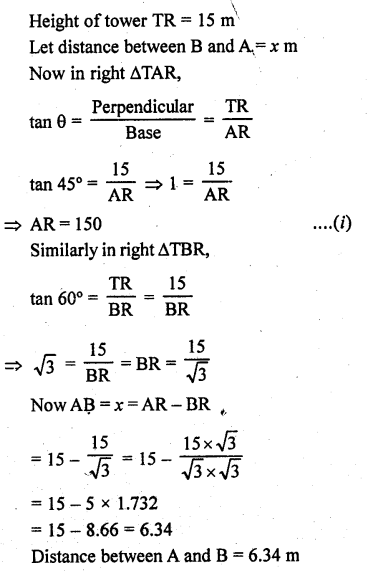
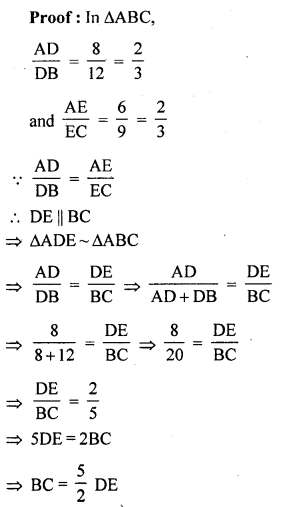
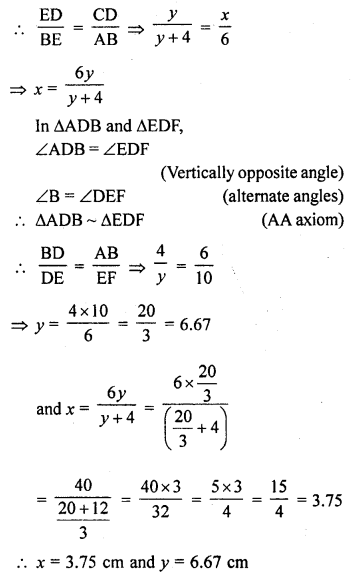
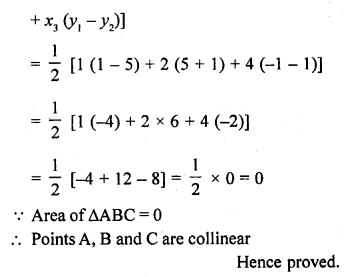
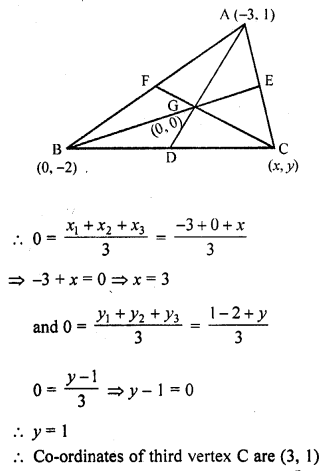
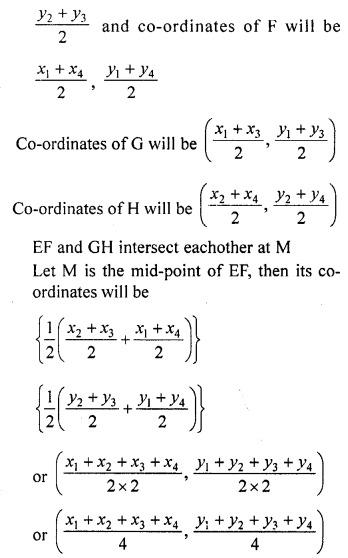
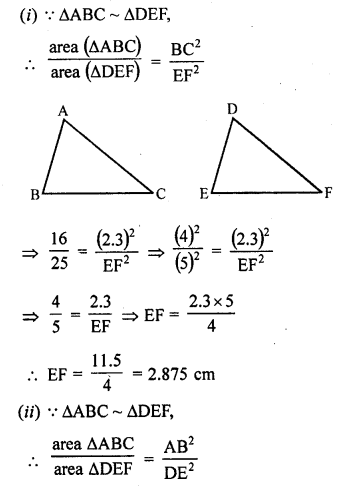
Question 1.
Triangles ABC and DEF are similar.
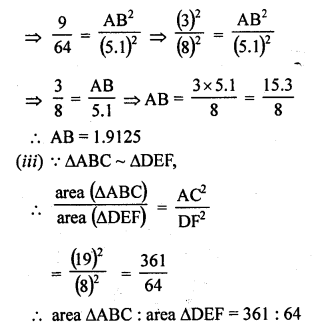
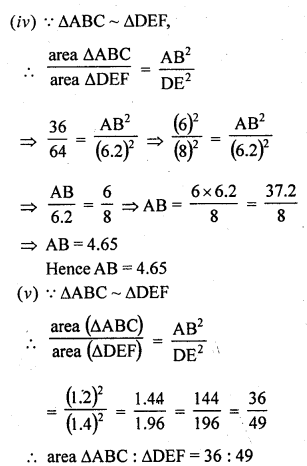
(i) If area (∆ABC) = 16 cm², area (∆DEF) = 25 cm² and BC = 2.3 cm, find EF. (C.B.S.E. 1992)
(ii) If area (∆ABC) = 9 cm², area (∆DEF) = 64 cm² and DE = 5.1 cm, find AB.
(iii) If AC = 19 cm and DF = 8 cm, find the ratio of the area of two triangles. (C.B.S.E. 1992C)
(iv) If area (∆ABC) = 36 cm², area (∆DEF) = 64 cm² and DE = 6.2 cm, find AB. (C.B.S.E. 1992)
(v) If AB = 1.2 cm and DE = 1.4 cm, find the ratio of the areas of ∆ABC and ∆DEF. (C.B.S.E. 1991C)
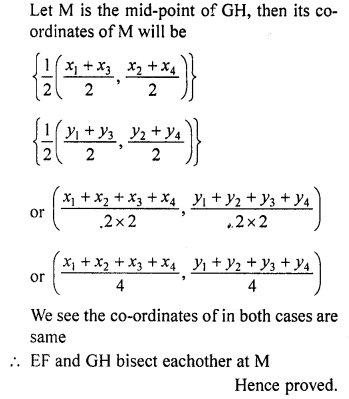
Solution:

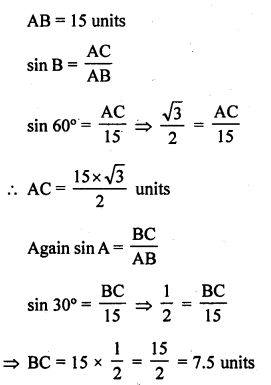
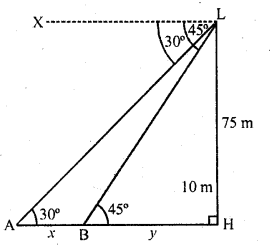
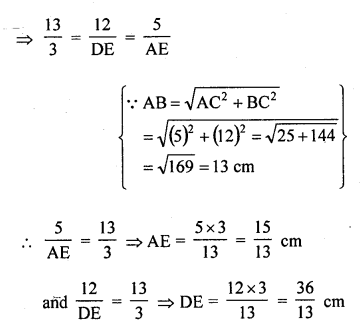
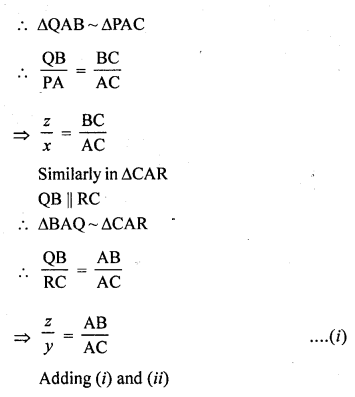
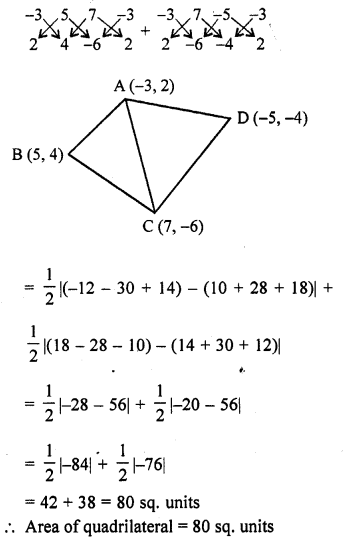
Question 2.
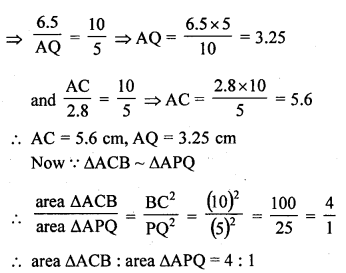
In the figure, ∆ACB ~ ∆APQ. If BC = 10 cm, PQ = 5 cm, BA = 6.5 cm and AP = 2.8 cm, find CA and AQ. Also, find the area (∆ACB) : area (∆APQ).

Solution:

Question 3.
The areas of two similar triangles are 81 cm² and 49 cm² respectively. Find the ratio of their corresponding heights, what is the ratio of their corresponding medians ?
Solution:
Areas of two similar triangles are 81 cm² and 49 cm²
The ratio of the areas of two similar triangles are proportion to the square of their corresponding altitudes and also squares of their corresponding medians
Ratio in their altitudes = √81 : √49 = 9 : 7
Similarly, the ratio in their medians = √81 : √49 = 9 : 7

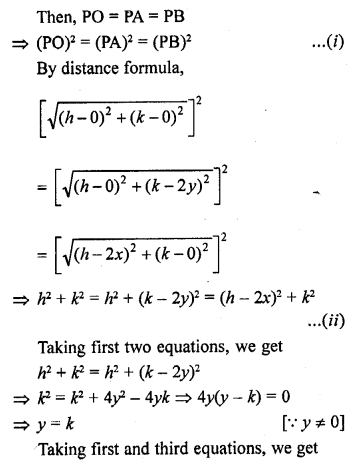
Question 4.
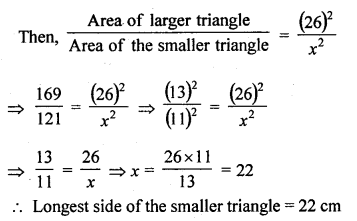
The areas of two similar triangles are 169 cm² and 121 cm² respectively. If the longest side of the larger triangle is 26 cm, find the longest side of the smaller triangle.
Solution:
Triangles are similar Area of larger triangle = 169 cm²
and area of the smaller triangle =121 cm²
Length of longest sides of the larger triangles = 26 cm
Let the length of longest side of the smaller triangle = x

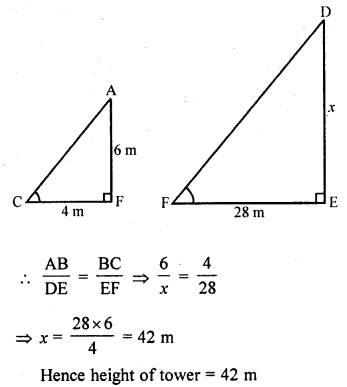
Question 5.
The areas of two similar triangles are 25 cm² and 36 cm² respectively. If the altitude of the first triangle is 2.4 cm, find the corresponding altitude of the other.
Solution:
Area of first triangle = 25 cm²
Area of second = 36 cm²
Altitude of the first triangle = 2.4 cm
Let altitude of the second triangle = x
The triangles are similar

Question 6.
The corresponding altitudes of two similar triangles are 6 cm and 9 cm respectively. Find the ratio of their areas.
Solution:
Length of the corresponding altitude of two triangles are 6 cm and 9 cm
triangles are similar

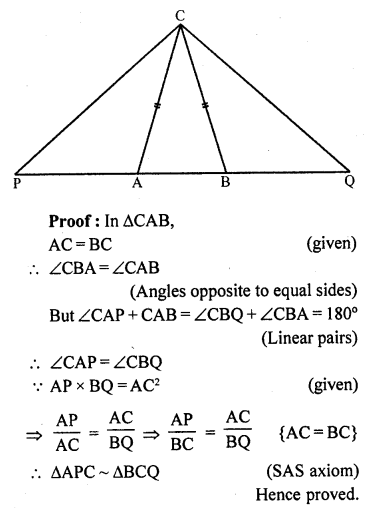
Question 7.
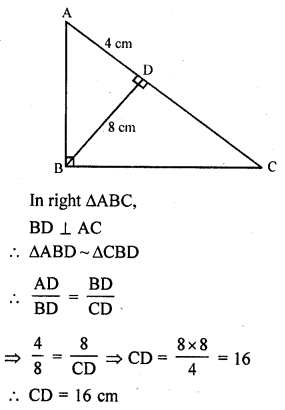
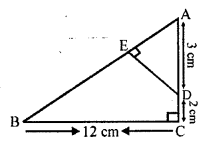
ABC is a triangle in which ∠A = 90°, AN ⊥ BC, BC = 12 cm and AC = 5 cm. Find the ratio of the areas of the ∆ANC and ∆ABC.
Solution:
In ∆ABC, ∠A = 90°
AN ⊥ BC
BC = 12 cm, AC = 5 cm

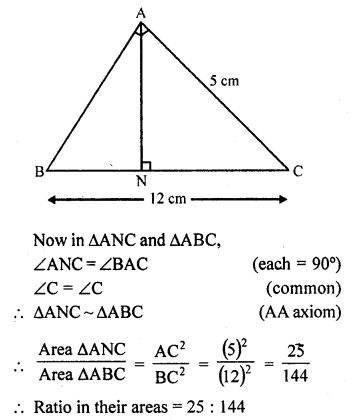
Question 8.
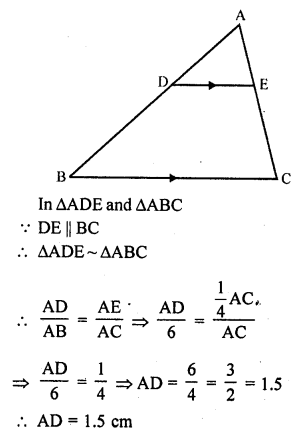
In the figure, DE || BC
(i) If DE = 4 cm, BC = 6 cm and area (∆ADE) = 16 cm², find the area of ∆ABC.
(ii) If DE = 4 cm, BC = 8 cm and area of (∆ADE) = 25 cm², find the area of ∆ABC. (C.B.S.E. 1991)
(iii) If DE : BC = 3 : 5, calculate the ratio of the areas of ∆ADE and the trapezium BCED

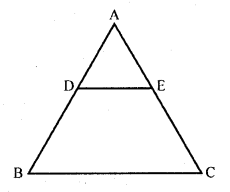
Solution:

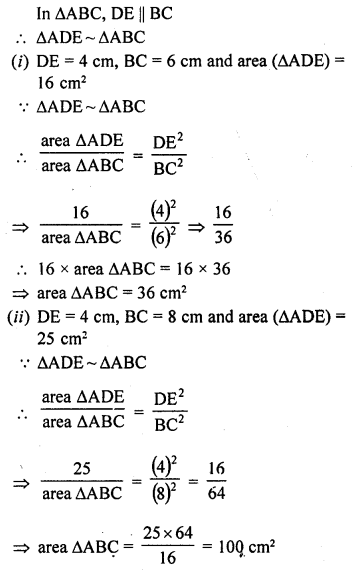
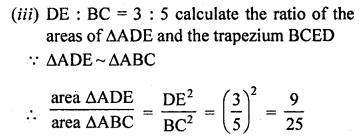
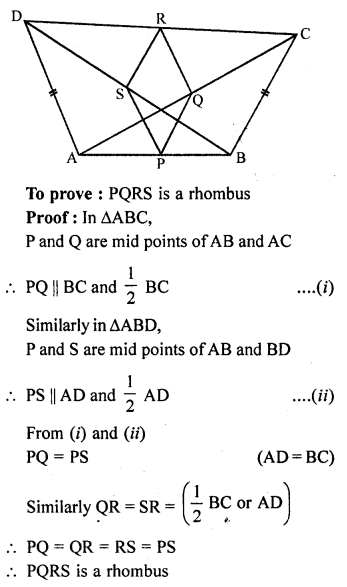
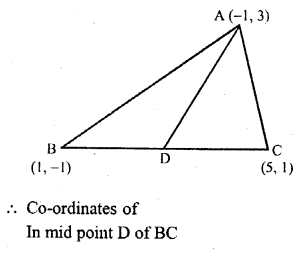
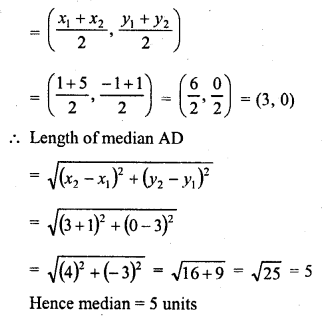
Question 9.
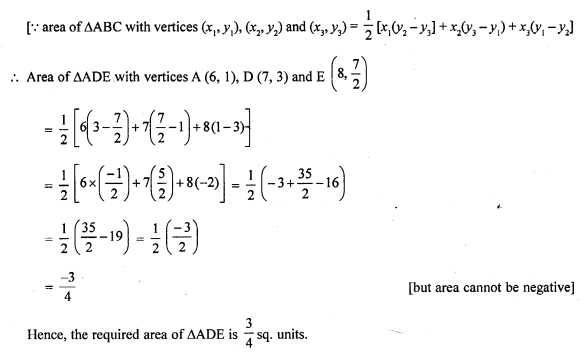
In ∆ABC, D and E are the mid-points of AB and AC respectively. Find the ratio of the areas of ∆ADE and ∆ABC.
Solution:
In ∆ABC, D and E are the mid points of AB and AC respectively

Question 10.
The areas of two similar triangles are 100 cm² and 49 cm² respectively. If the altitude of the bigger triangle is 5 cm, find the corresponding altitude of the other. (C.B.S.E. 2002)
Solution:
∆ABC ~ ∆DEF
area ∆ABC = 100 cm²
and area ∆DEF = 49 cm²

Question 11.
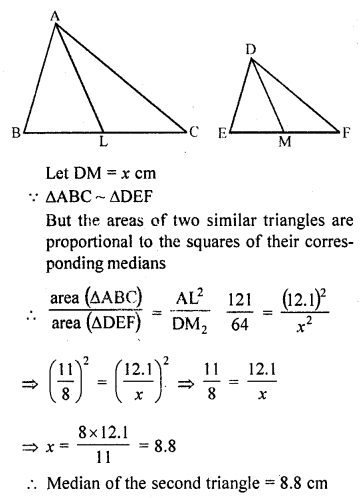
The areas of two similar triangles are 121 cm² and 64 cm² respectively. If the median of the first triangle is 12.1 cm, find the corresponding median of the other. (C.B.S.E. 2001)
Solution:
∆ABC ~ ∆DEF
area of ∆ABC = 121 cm² area of ∆DEF = 64 cm²
AL and DM are the medians of ∆ABC and ∆DEF respectively
AL = 12.1 cm

Question 12.
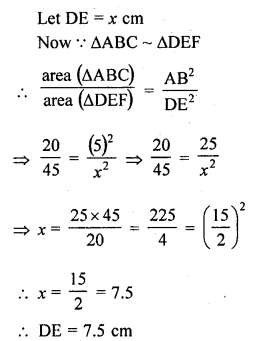
In ∆ABC ~ ∆DEF such that AB = 5 cm and (∆ABC) = 20 cm² and area (∆DEF) = 45 cm², determine DE.
Solution:
∆ABC ~ ∆DEF
area (∆ABC) = 20 cm²
area (∆DEF) = 45 cm²

Question 13.
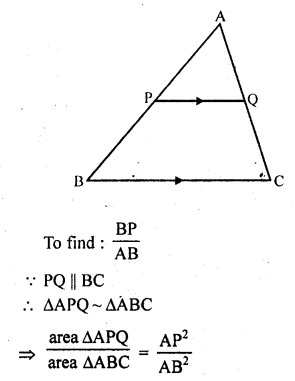
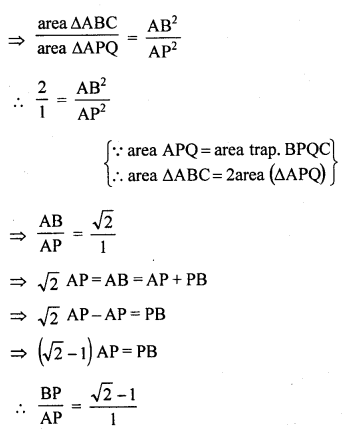
In ∆ABC, PQ is a line segment intersecting AB at P and AC at Q such that PQ || BC and PQ divides ∆ABC into two parts equal in area. Find \(\frac { BP }{ AB }\).
Solution:
In ∆ABC, PQ || BC and PQ divides ∆ABC in two parts ∆APQ and trap. BPQC of equal in area
i.e., area ∆APQ = area BPQC

Question 14.
The areas of two similar triangles ABC and PQR are in the ratio 9 : 16. If BC = 4.5 cm, find the length of QR. (C.B.S.E. 2004)
Solution:
∆ABC ~ ∆PQR
area (∆ABC) : area (∆PQR) = 9 : 16
and BC = 4.5 cm

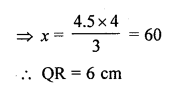
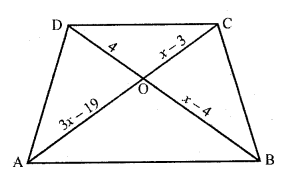
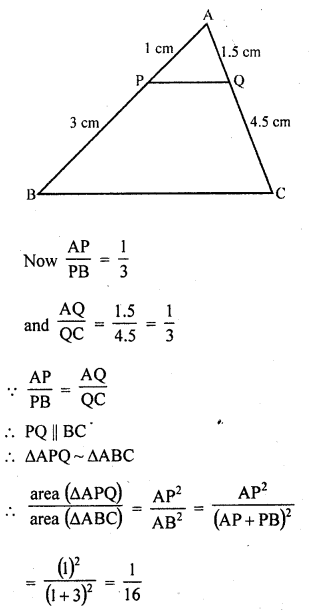
Question 15.
ABC is a triangle and PQ is a straight line meeting AB in P and AC in Q. If AP = 1 cm, PB = 3 cm, AQ = 1.5 cm, QC = 4.5 cm, prove that area of ∆APQ is one sixteenth of the area of ∆ABC. (C.B.S.E. 2005)
Solution:
In ∆ABC, P and Q are two points on AB and AC respectively such that
AP = 1 cm, PB = 3 cm, AQ = 1.5 cm and QC = 4.5 cm

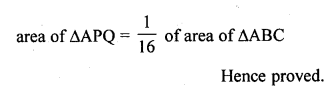
Question 16.
If D is a point on the side AB of ∆ABC such that AD : DB = 3 : 2 and E is a point on BC such that DE || AC. Find the ratio of areas of ∆ABC and ∆BDE. (C.B.S.E. 2006C)
Solution:
In ∆ABC, D is a point on AB such that AD : DB = 3 : 2

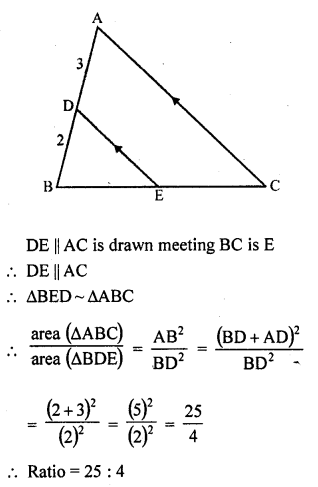
Question 17.
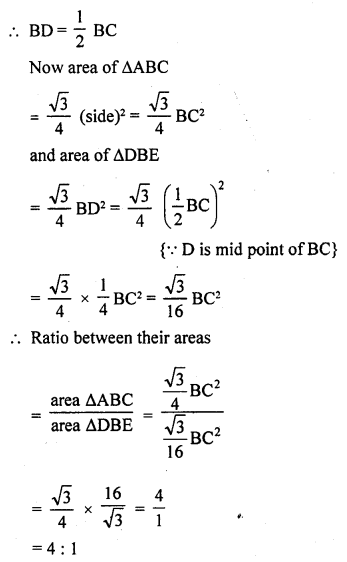
If ∆ABC and ∆BDE are equilateral triangles, where D is the mid point of BC, find the ratio of areas of ∆ABC and ∆BDE. [CBSE 2010]
Solution:
∆ABC and ∆DBE are equilateral triangles Where D is mid point of BC

Question 18.
Two isosceles triangles have equal vertical angles and their areas are in the ratio 36 : 25. Find the ratio of their corresponding heights.
Solution:
Two isosceles triangles have equal vertical angles
So their base angles will also be the equal to each other
Triangles will be similar Now, ratio in their areas = 36 : 25

Question 19.
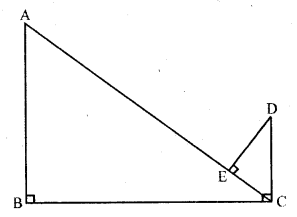
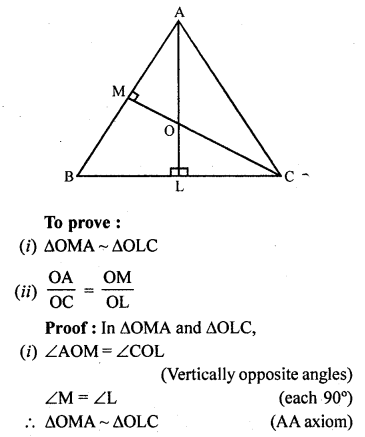
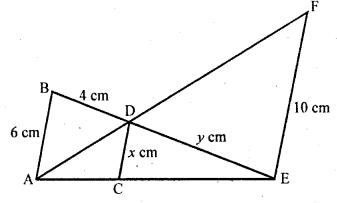
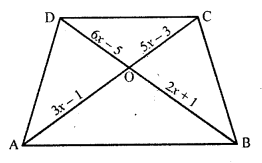
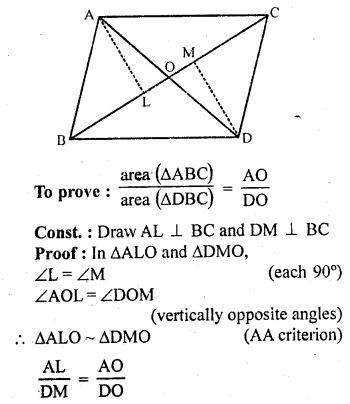
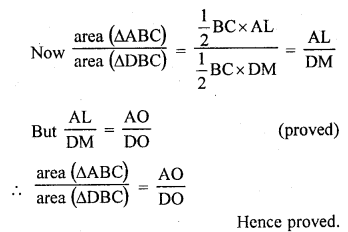
In the figure, ∆ABC and ∆DBC are on the same base BC. If AD and BC intersect

Solution:
Given : Two ∆ABC and ∆DBC are on the same base BC as shown in the figure
AC and BD intersect eachother at O

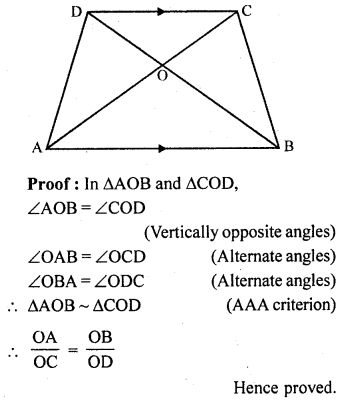
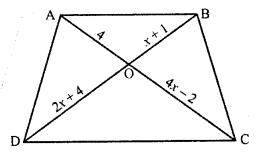
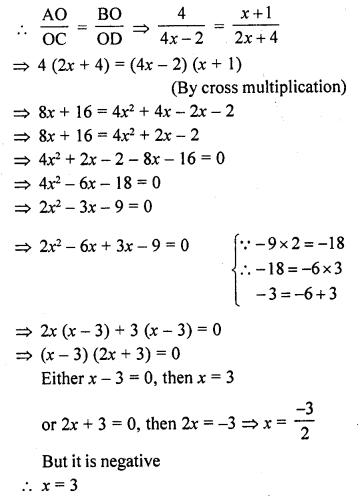
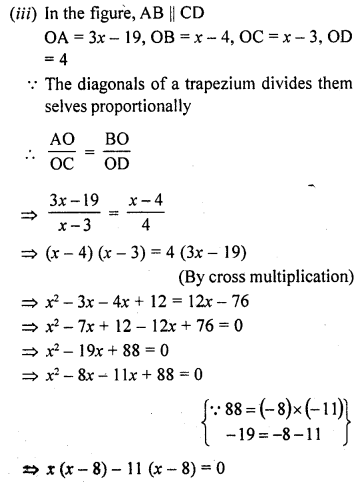
Question 20.
ABCD is a trapezium in which AB || CD. The diagonals AC and BD intersect at O. Prove that
(i) ∆AOB ~ ∆COD
(ii) If OA = 6 cm, OC = 8 cm, find

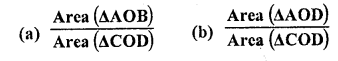
Solution:
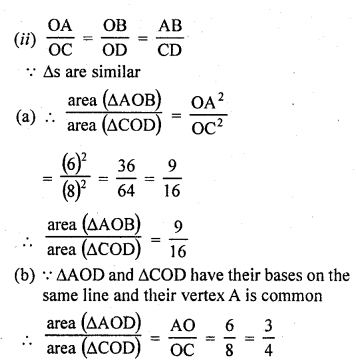
Given : ABCD is a trapezium in which AB || CD
Diagonals AC and BD intersect each other at O

Question 21.
In ∆ABC, P divides the side AB such that AP : PB = 1 : 2. Q is a point in AC such that PQ || BC. Find the ratio of the areas of ∆APQ and trapezium BPQC.
Solution:
In ∆ABC, P is a point on AB such that AP : PQ = 1 : 2
PQ || BC
Now we have to find the ratio between area ∆APQ and area trap BPQC

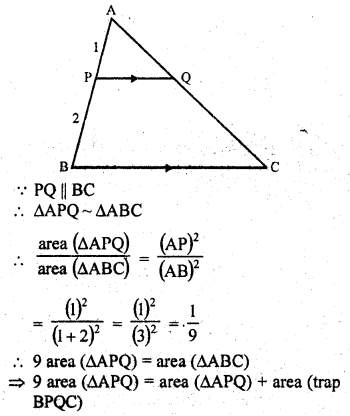
Question 22.
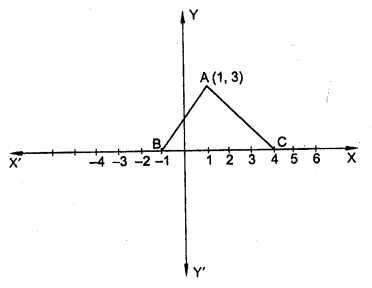
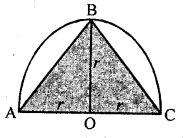
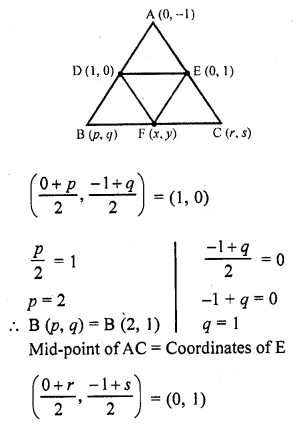
AD is an altitude of an equilateral triangle ABC. On AD as base, another equilateral triangle ADE is constructed. Prove that Area (∆ADE) : Area (∆ABC) = 3 : 4. [CBSE 2010]
Solution:
Given: In equilateral ∆ABC, AD ⊥ BC and with base AD, another equilateral ∆ADE is constructed

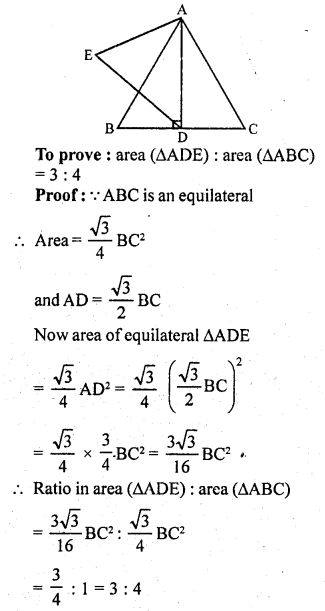
Hope given RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.6 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 10 Solutions Chapter 10 Trigonometric Ratios Ex 10.2

These Solutions are part of RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 10 Trigonometric Ratios Ex 10.2

Other Exercises

• RD Sharma Class 10 Solutions Chapter 10 Trigonometric Ratios Ex 10.1
• RD Sharma Class 10 Solutions Chapter 10 Trigonometric Ratios Ex 10.2
• RD Sharma Class 10 Solutions Chapter 10 Trigonometric Ratios Ex 10.3
• RD Sharma Class 10 Solutions Chapter 10 Trigonometric Ratios VSAQS
• RD Sharma Class 10 Solutions Chapter 10 Trigonometric Ratios MCQS

Evaluate each of the following (1-19) : 1.
Question 1.
sin45° sin30° + cos45° cos30°.
Solution:
sin 45° sin 30° + cos 45° cos 30°

Question 2.
sin60° cos30° + cos60° sin30°.
Solution:

Question 3.
cos60° cos45° – sin60° sin45°.
Solution:

Question 4.
sin²30° + sin²45° + sin²60° + sin²290°.
Solution:

Question 5.
cos²30° + cos²45° + cos²60° + cos²90°.
Solution:

Question 6.
tan²30° + tan²60° + tan²45°.
Solution:

Question 7.
2sin²30° – 3cos²45° + tan²60°.
Solution:

Question 8.
sin²30°cos²4S° + 4tan²30° + \(\frac { 1 }{ 2 }\) sin²90° -2cos²90° + \(\frac { 1 }{ 24 }\) cos20°.
Solution:

Question 9.
4 (sin⁴ 60° + cos⁴ 30°) – 3 (tan² 60° – tan² 45°) + 5cos² 45°
Solution:

Question 10.
(cosecc² 45° sec² 30°) (sin² 30° + 4cot² 45° – sec² 60°).
Solution:

Question 11.
cosec³ 30° cos 60° tan³ 45° sin2 90° sec² 45° cot 30°.
Solution:

Question 12.
cot² 30° – 2cocs² 60° – \(\frac { 3 }{ 4 }\)sec2 45° – 4sec² 30°.
Solution:

Question 13.
(cos0° + sin45° + sin30°) (sin90° – cos45° + cos60°)
Solution:

Question 14.

Solution:

Question 15.

Solution:

Question 16.
4 (sin⁴ 30° + cos² 60°) – 3 (cos² 45° – sin² 90°) – sin² 60°
Solution:

Question 17.

Solution:

Question 18.

Solution:

Question 19.

Solution:

Find the value of x in each of the following : (20-25)

Question 20.
2sin 3x = √3
Solution:

Question 21.
2sin \(\frac { x }{ 2 }\) = 1
Solution:

Question 22.
√3 sin x=cos x
Solution:

Question 23.
tan x = sin 45° cos 45° + sin 30°
Solution:

Question 24.
√3 tan 2x = cos 60° +sin 45° cos 45°
Solution:

Question 25.
cos 2x = cos 60° cos 30° + sin 60° sin 30°
Solution:

Question 26.
If θ = 30°, verify that :

Solution:

Question 27.
If A = B = 60°, verify that:
(i) cos (A – B) = cos A cos B + sin A sin B
(ii) sin (A – B) = sin A cos B – cos A sin B tan A – tan B
(iii) tan (A – B) = \(\frac { tanA-tanB }{ 1+tanA-tanB }\)
Solution:

Question 28.
If A = 30° and B = 60°, verify that :
(i) sin (A + B) = sin A cos B + cos A sin B
(ii) cos (A + B) = cos A cos B – sin A sin B
Solution:

Question 29.
If sin (A + B) = 1 and cos (A,-B) = 1,0° < A + B < 90°, A > B find A and B.
Solution:

Question 30.

Solution:

Question 31.

Solution:

Question 32.
In a ∆ABC right angle at B, ∠A = ∠C. Find the values of
(i) sin A cos C + cos A sin C
(ii) sin A sin B + cos A cos B
Solution:

Question 33.
Find acute angles A and B, if sin (A + 2B)=\(\frac { \sqrt { 3 } }{ 2 }\) and cos (A + 4B) = 0, A > B.
Solution:

Question 34.
In ΔPQR, right-angled at Q, PQ = 3 cm and PR = 6 cm. Determine ∠P and ∠R.
Solution:

Question 35.
If sin (A – B) = sin A cos B – cos A sin B and cos (A – B) = cos A cos B + sin A sin B, find the values of sin 15° and cos 15°.
Solution:

Question 36.
In a right triangle ABC, right angled at ∠C if ∠B = 60° and AB – 15 units. Find the remaining angles and sides.
Solution:

Question 37.
If ΔABC is a right triangle such that ∠C = 90°, ∠A = 45° and BC = 7 units. Find ∠B, AB and AC.
Solution:

Question 38.
In a rectangle ABCD, AB = 20 cm, ∠BAC = 60°, calculate side BC and diagonals AC and BD.
Solution:

Question 39.
If A and B are acute angles such that

Solution:

Question 40.
Prove that (√3 + 1) (3 – cot 30°) = tan³ 60° – 2sin 60°. [NCERT Exemplar]
Solution:

Hope given RD Sharma Class 10 Solutions Chapter 10 Trigonometric Ratios Ex 10.2 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 10 Solutions Chapter 12 Heights and Distances Ex 12.1

These Solutions are part of RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 12 Heights and Distances Ex 12.1

Other Exercises

• RD Sharma Class 10 Solutions Chapter 12 Heights and Distances Ex 12.1
• RD Sharma Class 10 Solutions Chapter 12 Heights and Distances VSAQS
• RD Sharma Class 10 Solutions Chapter 12 Heights and Distances MCQS

Question 1.
A tower stands vertically on the ground. From a point on the ground, 20 m away from the foot of the tower, the angle of elevation of the top of the tower is 60°. What is the height of the tower ?
Solution:
Let TS is the tower and P is a point which is 20 m away from the foot of the tower and angle of elevation of T is 60°

Question 2.
The angle of elevation of a ladder leaning against a wall is 60° and the foot of the ladder is 9.5 m away from the wall. Find the length of the ladder.
Solution:
Let LM is the ladder which makes an angle of 60° with the wall LM

Question 3.
A ladder is placed along a wall of a house such that its upper end is touching the top of the wall. The foot of the ladder is 2 m away from the wail and the ladder is making an angle of 60° with the level of the ground. Determine the height of the wall.
Solution:
Let LM be the ladder which makes an angle of 60° with the wall LN and at a distance of 2 m from the foot of the wall

Question 4.
An electric pole is 10 m high. A steel wire tied to top of the pole is affixed at a point on the ground to keep the pole up right. If the wire makes an angle of 45° with the horizontal through the foot of the pole, find the length of the wire.
Solution:
Let AB be the pole and a wire AC is tied to the top of the pole with a point C on the ground which makes an angle of 45° with the ground

Question 5.
A kite is flying at a height of 75 metres from the ground level, attached to a string inclined at 60° to the horizontal. Find the length of the string to the nearest metre.
Solution:
Let K be the kite flying in the sky at a height of 75 m from the ground LM. The string KL makes an angle of 60° to the ground
Let length of string KL = x m
KM = 75 m

Question 6.
A ladder 15 m long just reaches the top of a vertical wall. If the ladders makes an angle of 60° with the wall, then find the height of the wall.
Solution:
Given that, the height of the ladder = 15 m
Let the height of the vertical wall = h
and the ladder makes an angle of elevation 60° with the wall i.e., θ = 60°.

Question 7.
A vertical tower stands on a horizontal plane and is surmounted by a vertical flag-staff. At a point on the plane 70 metres away from the tower, an observer notices that the angles of elevation of the top and the bottom of the flag-staff are respectively 60° and 45°. Find the height of the flag-staff and that of the tower.
Solution:
Let TR be the tower and FT is the flag on it. P is an point on the ground 70 m away from the foot of the tower. From P, the angle of elevation of the top and bottom of the flag are 60° and 45° respectively Let h be the height of flag staff and x be the height of the tower

Question 8.
A vertically straight tree, 15 m high, is broken by the wind in such a way that its top just touches the ground and makes an angle of 60° with the ground. At what height from the ground did the tree break ? [CBSE 1995]
Solution:
Let TR be the tree whose height is 15 m
Let it is broken from A and its top T touches the ground at B making an angle of 60° with the ground

Question 9.
A vertical tower stands on a horizontal plane and is surmounted by a vertical flag-staff of height 5 metres. At a point on the plane, the angles of elevation of the bottom and the top of the flag-staff are respectively 30° and 60°. Find the height of the tower. (C.B.S.E. 1995)
Solution:
Let TR be the tower and FT is a flag staff on it. A is a point on the ground such that it makes angles of elevation of the bottom and top of the flag staff of 30° and 60° respectively

Question 10.
A person observed the angle of elevation of the top of a tower as 30°. He walked 50 m towards the root of the tower along level ground and found the angle of elevation of the top of the tower as 60°. Find the height of the tower.
Solution:
Let TR be the tower. A person at A on the ground observes the angle of elevation of the top T of the tower as 30° and then moves towards the foot of the tower. At a distance of 50 m at B, the angle of elevation becomes 60°

Let h be the height of the tower and BR = x, then AR = (50 + x)

Question 11.
The shadow of a tower, when the angle of elevation of the sun is 45°, is found to be 10 m longer than when it was 60°. Find the height of the tower.
Solution:
Let TR be we know
Let the shadow of TR at the elevation of the sun 45° be x m and at 60°, will be (x – 10) m Now in right ΔTAR,

Question 12.
A parachutist is descending vertically and makes angles of elevation of 45° and 60° at two observing points 100 m apart from each other on the left side of himself. Find the maximum height from which he falls and the distance of the point where he falls on the ground from the just observation point.
Solution:
Let P be the parachutist landing to Q on the ground
A and B are two observations such that AB = 10 m

Let h be the height of the parachutist from the ground and x be the distance of B from Q and (100 + x) is the distance from A to Q Now in right ΔAPQ,

Question 13.
On the same side of a tower, two objects are located. When observed from the top of the tower, their angles of depression are 45° and 60°. If the height of the tower is 150 m, find the distance between the objects. (C.B.S.E. 1992)
Solution:
Let TR be the tower and A, B are two objects which makes angles of elevation with top of the tower as 45° and 60° respectively

Question 14.
The angle of elevation of a tower from a point on the same level as the foot of the tower is 30°. On advancing 150 metres towards the foot of the tower, the angle of elevation of the tower becomes 60°. Show that the height of the tower is 129.9 metres (Use\(\sqrt { 3 } \) = 1-732).          (C.B.S.E. 2006)
Solution:
Let TR be the tower. A is a point on the same level which makes an angle of elevation of 30° with the top of the tower TR, and 150 m from A towards the foot of the tower the angle of elevaton is 60°, Let TR = h m

Question 15.
The angle of elevation of the top of a tower as observed from a point in a horizontal plane through the foot of the tower is 32°. When the observer moves  towards the tower a distance of 100 m, he finds the angle of elevation of the top to be 63°. Find the height of the tower and the distance of the first position from the tower. [Take tan 32° = 0.6248 and tan 63° = 1.9626]              (C.B.S.E. 2001C)
Solution:
Let PQ be the tower and from a points A, and B the angles of elevations of top P of the tower are 32° and 63° respectively and AB= 100 m

Question 16.
The angle of elevation of the top of a tower from a point A on the ground is 30°. On moving a distance of 20 metres towards the foot of the tower to a point B the angle of elevation increases to 60°. Find the height of the tower and the distance of the tower from the point A. (C.B.S.E. 2002)
Solution:
Let CD be the tower and from a point A on the same ground, the angle of elevation of the top of the tower is 30°. B is another point such that AB = 20 m and from B, the angle of elevation is 60°

Let h be the height of the tower CD and x is the length of AD
∴  BD = (x – 20) m
Now in right ΔCAD

Question 17.
From the top of a building 15 m high the angle of elevation of the top of a tower is found to be 30°. From the bottom of the same building, the angle of elevation of the top of the tower is found to be 60°. Find the height of the tower and the distance between the tower and building. (C.B.S.E. 2002)
Solution:
Let TR be the tower and AB be the building The angles of elevation of the top of the tower T, from A is 30° and from B is 60°
Height of AB = 15 m

Let the height of tower TR = h and the distance between the tower and building = x
In right ΔTBR,

Question 18.
On a horizontral plane there is a vertical tower with the flag pole on the top of the tower. At a point 9 metres away from the foot of the tower the angle of elevation of the top and bottom of the flag pole are 60° and 30° respectively. Find the height of the tower and the flag pole mounted on it.      (C.B.S.E. 2005)
Solution:
Let TR be the tower and F is the flag pole on it. A is a point 9 m away from the foot of the tower and angles of elevation of the top and bottom of the flag pole from A are 60° and 30° respectively

Question 19.
A tree breaks due to storm and the broken part bends so that the top of the tree touches the ground making an angle of 30° with the ground. The distance between the foot of the tree to the point where the top touches the ground is 8 in. Find the height of the tree.
Solution:
Let TR be the tree and it is broken from A and broken part of the tree makes an angle of 30° on the ground at B

Question 20.
From a point P on the ground the angle of elevation of a 10 m tall building is 30°. A flag is hoisted at the top of the building and the angle of elevation of the top of the flag-staff from P is 45°. Find the length of the flag-staff and the distance of the building from the point P. (Take \(\sqrt { 3 } \)  = 1.732
Solution:
Let BA is the building such that BA = 10 in CB is a flag-staff on the building P is a point on the ground such that the angles of the elevation of the top of the building is 30° and that of the top of the flag-staff is 45°

Question 21.
A 1.6 m tall girl stands at a distance of 3.2 m from a lamp-post and casts a shadow of 4.8 m on the ground. Find the height of the lamp-post by using
(i) trigonometric ratios
(ii) property of similar triangles.
Solution:
Let LP is the lamp-post and GR is the girl who is at a distance of 3.2 m
From the lamp-post and its shadow is AR which is 4.8 m long. Let ∠A = 0
Height of girl GR = 1.6 m and let height of lamp post = h

Question 22.
A 1.5 m tall boy is standing at some distance from a 30 m tall building. The angle of elevation from his eyes to the top of the building increasees from 30° to 60° as he walks towards the building. Find the distance he walked towards the building.
Solution:
Let BA is the boy whose height is 1.5 m and CD is building whose height is 30 m Angle of elevation of C from B (eyes of the boy) is 30°

and on moving some distance towards the building at L, the angle of elevation of C is 60°
Let AM = BL = x and AD = y
Then LE = MD = y – x
and CE = CD – ED = 30 – 1.5 = 28.5 m
Now in right ΔCBE

Question 23.
The shadow of a tower standing on a level ground is found to be 40 m longer when Sun’s altitude is 30° than when it was “60°. Find the height of the tower.
Solution:
Let TR be the tower and RB and RA are its shadows at the elevation of 60° and 30° respectively. Such that BA = 40 m

Let h be the height of the tower and let shadow RA = x m
Then shadow RB = (x – 40) m
Now in right ΔTAR,

Question 24.
From a point on the ground the angles of elevation of the bottom and top of a transmission tower fixed at the top of 20 m high building are 45° and 60° respectively. Find the height of the transmission tower.
Solution:
BC is the building and AB is the transmission on it the height of the building is 20 m From a point P, the angles of elevation of B and A are 45° and 60° respectively

Let height of the transmission AB = h and let PC = x
Now in right ΔBPC,

Question 25.
The angles of depression of the top and bottom of 8 m tali building from the top of a multistoried building are 30° and 45° respectively. Find the height of the multistoried building and the distance Between the two buildings.
Solution:
Let AB be the multistoried building and CD is another building. From A, the angles of depression of the top C and bottom D of the other building are 30° and 45° respectively Height of building is 8 m i.e. CD = 8 m

Question 26.
A statue 1.6 m tall stands on the top of pedestal. From a point on the ground, the angle of elevation of the top of the statue is 60° and from the same point the angles of elevation of the top of thd\pedestal is 45°. Find the height of the pedestal. (C.B.S.E. 2008)
Solution:
Let AB be the statue standing on the top of a pedestal BC
From a point P on the ground the angles of elevation of the top of the statue is 60° and top of the pedestal BC is 45°
Let height of BC = h and PC = x

Question 27.
A T.V. Tower stands vertically on a bank of a river. From a point on the other bank directly opposite the tower, the angle of elevation of the top of the tower is 60°. From a point 20 m away this point on the same bank, the angle of elevation of the top of the tower is 30°. Find the height of the tower and the width of the river.
Solution:
Let AB is the T.V. tower and CB is the,width of the river. D is a point which is 20 m away from C
Now angles of elevation from A to C and D are 60° and 30° respectively.

Question 28.
From the top of a 7 m high building, the – angle of elevation of the top of a cable tower is 60° and the angle of depression of its foot is 45°. Determine the height of the tower.
Solution:
Let AB be the building and CD be the cable tower
From the top of building, the angle of elevation of the top of tower is 60° and angle of depression of the foot of the tower is 45° Now AB = 7 m

Question 29.
As observed from the top of a 75 m tall lighthouse, the angles of depression of two ships are 30° and 45°. If one ship is exactly behind the other on the same side of the lighthouse, find the distance between the two ships.
Solution:
Let LH is die light house and A, B are two ships From L, angles of depression of the ships A and B are 30° and 45° respectively

Question 30.
The angle of elevation of the top of the building from the foot of the tower is 30° and the angle of the top of the tower from the foot of the building is 60°. If the tower is 50 m high, find the height of the building.
Solution:
Let AB be the tower and CD is the building The angle of elevation of the top of the building from the foot of the tower is 30° and the angle of the top of the tower from the foot of the building is 60°

Question 31.
From a point on a bridge across a river the angles of depression of the banks onopposite side of the river are 30° and 45° respectively. If bridge is at the height of 30 m from the banks, find the width of the river.
Solution:
Let BR is the bridge with a height of 30 m and angles of depression from B the top of the bridge to two given points C and D on the opposite sides of the river are 30° and 45° respectively CD is the width of the river Let CR = x and DR = y

Question 32.
Two poles of equal heights are standing opposite to each other on either side of the road which is 80 m wide. From a point between them on the road the angles of elevation of the top of the poles are 60° and 30° respectively. Find the height of the poles and the distances of the point from the poles.
Solution:
AB and CD are two equal poles on either side of the road BD which is 80 m wide P is a point on the road such that the angles of elevation of the tops of the poles are 60° and 30° respectively

Let the length of each pole in h and let BP = x, then DP = 80 – x
In right ΔABP,

Question 33.
A man sitting at a height of 20 m on a tall tree on a small island in the middle of a river observes two poles directly opposite to each other on the two banks of the river and in line with the foot of tree. If the angles of depression of the feet of the poles from a point at which the man is sitting on the tree on either side of the river are 60° and 30° respectively. Find the width of the river.
Solution:
Let AB be the tree on which a man is sitting the tree is on a small island in the middle of the river. C and D are the foot of the poles on either bank of the river The angles of depression from A to the poles C and D are 60° and 30° respectively and AB = 20 m

Question 34.
A vertical tower stands on a horizontal plane and is surmounted by a flag-staff of height 7 m. From a point on the plane, the angle of elevation of the bottom of the flag-staff is 30° and that of the top of the flag-staff is 45°. Find the height of the tower.
Solution:
Let AB be a flag post on a building BC From a point P on the same ground, angle of elevations of A and B are 45° and 30° respectively

Question 35.
The length of the shadow of a tower standing on level plane is found to be 2x metres longer when the sun’s altitude is 30° than when it was 45°. Prove that the height of tower is x (\(\sqrt { 3 } \) +1) metres.
Solution:
Let PQ be the tower whose shadow is QA at the elevation of 45° and QB at the elevation of 30° such that AB = 2x

Question 36.
A tree breaks due to the storm and the broken part bends so that the top of the tree touches the ground making an angle of 30° with the ground. The distance from the foot of the tree to the point where the top touches the ground is 10 Find the height of the tree.
Solution:
Let TR be the tree arid it is broken at S and its broken parts touches the ground at P making ah angle of 30° elevation angle,
PR = 10 m
Let length of the tree TR = h and let TS = SP = x

Question 37.
A balloon is connected to a meteoro­logical ground station by a cable of length 215 m inclined at 60° to the horizontal. Determine the height of the balloon from the ground. Assume that there is no slack in the cable.
Solution:
Let B is the balloon which is connected by the cable BC which is 215 m long and makes an angle of elevation of 60° with the ground Let h be the height of the balloon

Question 38.
Two men on either side of the cliff 80 m high observes the angles of elevation of the top of the cliff to be 30° and 60° respectively. Find the distance between the two men.
Solution:
Let CL is the cliff and A and B are two men on either side of the cliff making angles of elevation with C as 30“ and 60“ respectively Height of cliff CL = 80 m

Question 39.
Find the angle of elevation of the sun (sun’s altitutde) when the length of the shadow of a vertical pole is equal to its height.
Solution:
Let the height of pole AB = h m
Then its shadow = h m

Question 40.
An aeroplane is flying at a height of 210 m. Flying at this height at some instant the angles of depression of two points in a line in opposite directions on both the banks of the river are 45° and 60°. Find the width of the river. (Use \(\sqrt { 3 } \)  = 1.73)          [CBSE 2015]
Solution:
Height of the aeroplane = 210 m
Let AB is the height of aeroplane and C and D are the points on the opposite banks of a river.

Question 41.
The angle of elevation of the top of a chimney from the top of a tower is 60° and the angle of depression of the foot of the chimney from the top of the tower is 30°. If the height of the tower is 40 m, find the height of the chimney. According to pollution control norms, the minimum height of a smoke emitting chimney should be 100 m. State if the height of the above mentioned chimriey meets the pollution norms. What value is discussed in this question?  [CBSE 2014]
Solution:
Let CD be the tower area AB be the chimney angle of elevation of the top of tower with the top of the chimney is 60° and foot of chimney with the top of tower is 30°
CD = 40 m

Question 42.
Two ships are there in the sea on either side of a light house in such a way that the ships and the light house are in the same straight line. The angle of depression of two ships are observed from the top of the light house are 60° and 45° respectively. If the height of the light house is 200 m, find the distance between the two ships. (Use \(\sqrt { 3 } \) = 1.73)
Solution:
Let AB be the light house and C and D are two ships which make angle of depression with the top A of the light house of 60° and 45°
AB = 20 m

Question 43.
The horizontal distance between two poles is 15 m. The angle of depression of the top of the first pole as seen from the top of the second pole is 30°. If the height of the second pole is 24 m, find the height of the first pole. (\(\sqrt { 3 } \) = 1.732)                [CBSE 2013]
Solution:
Let AB and CD are two poles and distance between them = 15 m, and AB = 24 m Angle of elevation from top of pole CD, to pole AB = 30°
From C, draw CE || DB

Question 44.
The angles of depression of two ships from the top of a light house and on the same side of it are found to be 45° and 30° respectively. If the ships are 200 m apart, find the height of the light house.
Solution:
Let PQ be the light house and A and B are two ships on the same side of the light house Angle of depression from top of the light house of the two ships are 30° and 45°

Question 45.
The angles of elevation of the top of a tower from two points at’a distance of 4 m and 9 m from the base of the tower and in the same straight line with it are complementary. Prove that the height of the tower is 6 m.
Solution:
Let TR be the tower. A and B are two points which make the angled of elevation with top of tower as θ and 90° – θ (∵ angles are complementary)
Let height of tower TR = h and AR = 9 m, BR = 4m

Question 46.
From the top of a 50 m high tower, the angles of depression of the top and bottom of a pole are observed to be 45° and 60° respectively. Find the height of the pole.
Solution:
Let AB be the tower and CD is the pole. Angles of depression from the top A to the top and bottom of the pole are 45° and 60° respectively
AB = 50 m, let CD = h and BD = EC = x
∵ CE || DB
∴ EB = CD = h
and AE = 50 – h

Question 47.
The horizontal distance between two trees of different heights is 60 m. The angle of depression of the top of the first tree when seen from the top of the second tree is 45°. If the height of the second tree is 80 m, find the height of the first tree.
Solution:
Let AB and CD be the two trees
AB = 80 m, angle of depression from A of C is 45°. Draw CE || DB

Question 48.
A flag-staff stands on the top of a^5 m high tower. From a point on the ground, the angle of elevation of the top of the flag-staff is 60° and from the same point, the angle of elevation of the top of the tower is 45°. Find the height of the flag­staff.
Solution:
Let FT is the flag-staff situated on the top of the tower TR. A is any point on the same plane which makes angles of elevation with top of the flag-staff and top of the tower are 60° and 45° respectively.

Question 49.
The angle of elevation of the top of a vertical tower PQ from a point X on the ground is 60°. At a point Y, 40 m vertically above X, the angle of elevation of the top is 45°. Calculate the height of the tower.
Solution:
Let TR is the tower From a point X, the angle of elevation of T is 60° and 40 m above X, from the point Y, the angle of elevation is 45°

Question 50.
As observed from the top of a 150 m tall light house, the angles of depression of two ships approaching it are 30° and 45°. If one ship is directly behind the other, find the distance between the two ships.
Solution:
Let LH be the light house, A apd B ate two ships making angles of elevation with the top of the light house as 30° and 45° respectively.
LH = 150 m.

Question 51.
The angles of elevation of the top of a rock from the top and foot of a 100 m high tower are respectively 30° and 45°. Find the height of the rock.
Solution:
Let RS is the rock and TP is the tower. The angles of elevation of the top of rock with the top and foot of the tower are 30° and 45° respectively

Height of TP = 100 m
Let height of rock RS = h
From T, draw TQ || PS                                 _
Then QS = TP = 100 m                               ‘
and RQ = h – 100
Let PS = TQ = x
Now in right ΔRPS

Question 52.
A straight highway leads to the foot of a tower of height 50 m. From the top of the tower, the angles of depression of two cars standing on the highway are 30° and 60° respectively. What is the distance between the two cars and how far is each car from the tower ?
Solution:
Let TR be the tower and A and B are two cars on the road making angles of elevation with T the top of tower as 30° and 60°
Height of the tower TR = 50 m
Let AR = x and BR = y
Now in right ΔTAR,

Question 53.
From the top of a building AB, 60 m high, the angles of depression of the top and bottom of a vertical lamp post CD are observed to be 30° and 60° respectively. Find
(i) the horizontal distance between AB and CD.
(ii) the height of the lamp post
(iii) the difference between the heights of the building and the lamp post. [CBSE 2009]
Solution:
Let AB is the building and CD is the verticle lamp
From A, the top of the building angles of depression of C and D are 30° and 60° respectively
Height of building AB = 60 m

Let height of CD = h
Draw CE || DB || AX
∴ ∠ACE = ∠XAC = 30° and ∠ADB = ∠XAD = 60°
EB = CD = h and AE = 60 – h
Let DB = CE = x
Now in right ΔACE,

Question 54.
Two boats approach a light house in mid­sea from opposite directions. The angles of elevation of the top of the light house from two boats are 30° and 45° respectively. If the distance between two boats is 100 m, find the height of the light house. [CBSE 2014]
Solution:
Let LH is the light house and A and B are two boats on the opposite directions of the light house which are making angle of elevation of the top L of the light house as 30° and 45°

Question 55.
The angle of elevation of the top of a hill at the foot of a tower is 60° and the angle of elevation of the top of the tower from the foot of the hill is 30°. If the tower is 50 m high, what is the height of the hill ?[CBSE 2006C, 2013]
Solution:
Let TR be the tower, HL is the hill and angles of elevation of top of the hill is 60° and top of the tower is 30

Question 56.
A moving boat is observed from the top of a 150 m high cliff moving away from the cliff. The angle of depression of the boat changes from 60° to 45° in 2 minutes. Find the speed of the boat in m/h.   [CBSE 2017]
Solution:
Let the distance BC be x m and CD be y m.

Question 57.
From the fop of a 120 m ifigh tower, a man observes two cars on the opposite sides of the tower and in straightline with the base of tower with angles of depression as 60° and 45°. Find the distance between the cars. (Take \(\sqrt { 3 } \)  = 1.732) [CBSE 2017]
Solution:
Let BD be the tower and A and C be the two points on ground.

Then, BD, the height of the tower = 120m
∠BAD = 45°, ∠BCD = 60°

Question 58.
Two points A and B are on the same side of a tower and in the same straight line with its base. The angles of depression of these points from the top of the tower are 60° and 45° respectively. If the height of the tower is 15 m, then find the distance between these points.  [CBSE2017]
Solution:
Let TR be the tower and A, B are two objects which makes angles of elevation with top of the tower as 45° and 60° respectively

Question 59.
A fire in a building B is reported on telephone to two fire stations P and Q, 20 km apart from each other on a straight road. P observes that the fire is at an angle of 60° to the road and Q observes that it is at an angle of 45° to the road. Which station should send its team and how much will this team have to travel ?
Solution:
Let B is the building one fire and P and Q the fire stations which are 20 km apart i.e., PQ = 20 km.


P and Q observes the angles with B, as 60° and 45° respectively.
Draw BA ⊥ PQ
Let AB = h Now in right ΔBAQ

Question 60.
A man on the deck of a ship is 10 m above the water level. He observes that the angle of elevation of the top of a cliff is 45° and the angle of depression of the base is 30°. Calculate the distance of the cliff from the ship and the height of the cliff.
Solution:
Let M is a man on the deck MN such that MN = 10 m, AB is the cliff
From M the angle of elevation of A is 45°
and angle of depression of B is 30°

Question 61.
A man standing on the deck of a ship, which is 8 m above water level. He observes the angle of elevation of the top of a hill as 60° and the angle of depression of the base of the hill as 30°. Calculate the distance of the hill from the ship and the height of the hill.
Solution:
Let M is the man on the deck MN such that MN = 8 m. AB is the hill From M, the angle of elevation of A is 60° and angle of depression of B is 30°

Question 62.
There are two temples, one on each bank of a river, just opposite to each other. One temple is 50 m high. From the top of this temple, the angles of depression of the top and the foot of the other temple are 30° and 60° respectively. Find the width of the river and the height of the other temple.
Solution:
Let AB and CD are two temples on the banks of the river.
AB = 50 m
From A, the angles of depression of the top and botttom of the other temple are 30° and 60° respectively.

Question 63.
The angle of elevation of an aeroplane from a point on the ground is 45°. After a flight of 15 seconds, the elevation changes to 30°. If the aeroplane is flying at a height of 3000 metres, find the speed of the aeroplane.
Solution:
Let A is the plane flying in the sky at is height of 3000 m i.e., AB = 3000 m
P is a point on the ground which from an angle of elevation of 45° at A and then after a flight of 15 seconds at A’ the angle of elevation because 30°

Question 64.
An aeroplane flying horizontally 1 km above the ground is observed at an elevation of 60°. After 10 seconds, its elevation is observed to be 30°. Find the the speed of the aeroplane in km/hr.
Solution:
Let A be the aeroplane and AB is the height which 1 km and make an angle of elevations of 60° from a point P on the ground After moving 10 second’s flight, the angle of elevation becomes 30° from P

A’B’=AB =1 km = 1000 m
Let PB = y and BB’ = x
Now in right ΔAPB,

Question 65.
A tree standing on a horizontal plane is leaning towards east. At two points situated at distance a and b exactly due west on it, the angles of elevation of the top are respectively a and p. Prove that the height of the top from the ground is

Solution:
Let CD is the tree which is leaning towardsEast and A and B are two points on the West making angles of elevation with top C of the tree as α and β
A and B are at the distance of a and b from the foot of the tree CD, then AD = a, BD = b

Question 66.
The angle of elevation of a stationery cloud from a point 2500 m above a lake is 15° and the angle of depression of its reflection in the lake is 45°. What is the height of the cloud above the lake level? (Use tan 15° = 0.268)
Solution:
Let C is the cloud over a lake LK
From a point M which, is 2500 m above the lake level, angle of elevation of C is 15° and angle of depression of the reflection of C in the lake which is R is 45°

Question 67.
If the angle of elevation of a cloud from a point h metres above a lake is a and the angle of depression of its reflection in the lake be p, prove that the distance of the cloud from the point of \(\frac { 2h\sec { \alpha } }{ \tan { \beta -\tan { \alpha } } }\)  [CBSE 2004]
Solution:
Let C be the cloud and from a point M which h m is above the lake level angle of elevation is α and angle of reflection of the cloud C, is β

Question 68.
From an aeroplane vertically above a straight horizontal road, the angles of depression of two consecutive mile stones on opposite sides of the aeroplane are observed to be a and p. Show that the height in miles of aeroplane above the road is given by \(\frac { \tan { \alpha } \tan { \beta } }{ \tan { \alpha } +\tan { \beta } }\)   [CBSE 2004]
Solution:
Let A is aeroplane and C and D are two such points that the angles of depression from A are a and p respectively and CD = 1 km
Let height of the plane be h

Question 69.
PQ is a post of given height a and AB is a tower at some distance. If a and p are the angles of elevation of B, the top of the tower, at P and Q respectively. Find the height of the tower and its distance from the post.
Solution:
Let PQ is post and AB is the tower Angles of elevation of B, from P and Q are a and P respectively

Question 70.
A ladder rests against a wail at an angle a to the horizontal. Its foot is pulled away from the wall through a distance a, so that it slides a distance b down the wall making an angle p with the horizontal.
Show that \(\frac { a }{ b }\)  = \(\frac { \cos { \alpha } -\cos { \beta } }{ \sin { \beta } -\sin { \alpha } }\)
Solution:
In the figure, AC and ED is the same stair, so AC = ED

Question 71.
A tower subtends an angle a at a point A in the plane of its base and the angle of depression of the foot of the tower at a point b metres just above A is p. Prove that the height of the tower is b tan α cot β.
Solution:
Let TR is the tower which subtends angle α at a point A on the same plane
AB = b and angle of depression of R from B is β

Question 72.
An observer, 1.5 m tall, is 28.5 m away from a tower 30 m high. Determine theangle of elevation of the top of the tower from his eye.
Solution:
Let TR is the tower and CD is the observer who is 28.5 m away from the tower TR
Height of the tower TR = 30 m
and height of observer CD = 1.5 m

Question 73.
A carpenter makes stools for electricians with a square top of side 0.5 m and at a height of 1.5 m above the ground. Also, each leg is inclined at an angle of 60° to the ground. Find the length of each leg and also the lengths of two steps to be put at equal distances.
Solution:
Let AC be the leg of stool whose top in a square shaped of side AB = 0.5 m
Height of stool AL = 1.5 m, and angle of inclination by the leg of the stool = 60°

Question 74.
A boy is standing on the ground and flying a kite with 100 m of string at an elevation of 30°. Another boy is standing on the roof of a 10 m high building and is flying his kite at an elevation of 45°. Both the boys are on opposite sides of both the kites. Find the length of the string that the second boy must have so that the two kites meet.
Solution:
Let K be the kite. A and B are two boys flying kites. Boy B is standing on a building 10 m high
The string AK of kite of boy A is 100 m Let h be the height of the kite and x is the length of string of kite of second boy B

Question 75.
From the top of a light house, the angles of depression of two ships on the opposite sides of it are observed to be α and β. If the height of the light house be h metres and the line joining the ships passes through the foot of the light house, show that the distance between the ship is \(\frac { h(\tan { \alpha } +\tan { \beta } ) }{ \tan { \alpha } \tan { \beta } }\)   meters.
Solution:
Let LH be the light house and A and B are two ships which make angles of elevation with L are a and p respectively

Question 76.
From the top of a tower hmetre high, the angles of depression of two objects, which are in the line with the foot of the tower are a and β (β > α). Find the distance between the two objects. [NCERT Exemplar]
Solution:
Let the distance between two objects is x m
and CD = y m
Given that, ∠BAX = α = ∠ABD                              [alternate angle]
∠CAY = β = ∠ACD                                                   [alternate angle]
and the height of tower, AD = h m Now, in ΔACD,

Question 77.
A window of a house is h metre above the ground. From the window, the angles of elevation and depression of the top and bottom of another house situated on the opposite side of the lane are found to be α and β respectively. Prove that the height of the house is h( 1 + tan α tan β) metres. [NCERT Exemplar]
Solution:
Let the height of the other house = OQ = H
and OB = MW = x m
Given that, height of the first house = WB = h = MO
and ∠QWM = α, ∠OWM =β= ∠WOB                                 [alternate angle]
Now, in ΔWOB,

Question 78.
The lower window of a house is at a height of 2 m above the ground and its upper window is 4 m vertically above the lower window. At certain instant the angles of elevation of a balloon from these windows are observed to be 60° and 30° respectively. Find the height of the balloon above the ground.[NCERT Exemplar]
Solution:
Let the height of the balloon from above the ground is H.
A and OP = w₂R = w₁Q = x
Given that, height of lower window from above the ground = w₂P = 2 m = OR
Height of upper window from above the lower window = w₁w₂ = 4 m = QR
∴ BQ = OB – (QR + RO)
BQ = H – (4 + 2)
BQ = H – 6

Hope given RD Sharma Class 10 Solutions Chapter 12 Heights and Distances Ex 12.1 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.5

These Solutions are part of RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.5

Other Exercises

• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.1
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.2
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.3
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.4
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.5
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.6
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.7
• RD Sharma Class 10 Solutions Chapter 7 Triangles Revision Exercise
• RD Sharma Class 10 Solutions Chapter 7 Triangles VSAQS
• RD Sharma Class 10 Solutions Chapter 7 Triangles MCQS

Question 1.
In the figure, ∆ACB ~ ∆APQ. If BC = 8 cm, PQ = 4 cm, BA = 6.5 cm and AP = 2.8 cm, find CA and AQ. (C.B.S.E. 1991)
Solution:
In the figure,
∆ACB ~ ∆APQ
BC = 8 cm, PQ = 4 cm, BA = 6.5 cm and AP = 2.8 cm

Question 2.
In the figure, AB || QR. Find the length of PB. (C.B.S.E. 1994)

Solution:
In the figure,
In ∆PQR, AB || QR
AB = 3 cm, QR =9 cm, PR = 6 cm
In ∆PAB and ∆PQR
∠P = ∠P (common)
∠PAB = ∠PQR (corresponding angles)
∠PBA = ∠PRQ (corresponding angles)
∠PAB = ∠PQR (AAA axiom)

Question 3.
In the figure, XY || BC. Find the length of XY. (C.B.S.E. 1994C)
Solution:
In the figure
In ∆ABC XY || BC
AX = 1 cm, BC = 6 cm

Question 4.
In a right angled triangle with sides a and b and hypotenuse c, the altitude drawn on hypotenuse is x. Prove that ab = cx.
Solution:
Given : In right ∆ABC, ∠B is right angle BD ⊥ AC
Now AB = a, BC = b, AC = c and BD = x

Question 5.
In the figure, ∠ABC = 90° and BD ⊥ AC. If BD = 8 cm and AD = 4 cm, find CD.
Solution:

Question 6.
In the figure, ∠ABC = 90° and BD ⊥ AC. If AB = 5.7 cm, BD = 3.8 cm and CD = 5.4 cm, find BC.
Solution:
In right ∆ABC, ∠B = 90°
BD ⊥ AC
AB = 5.7 cm, BD = 3.8 and CD = 5.4 cm

Question 7.
In the figure, DE || BC such that AE = \(\frac { 1 }{ 4 }\) AC. If AB = 6 cm, find AD.
Solution:
In the figure, in ∆ABC, DE || BC
AE = \(\frac { 1 }{ 4 }\) AC, AB = 6 cm

Question 8.
In the figure, if AB ⊥ BC, DC ⊥ BC and DE ⊥ AC, prove that ∆CED ~ ∆ABC.

Solution:
Given : In the figure AB ⊥ BC, DC ⊥ BC and DE ⊥ AC
To prove : ∆CED ~ ∆ABC.
Proof: AB ⊥ BC
∠B = 90°
and ∠A + ∠ACB = 90° ….(i)
DC ⊥ BC
∠DCB = 90°
=> ∠ACB + ∠DCA = 90° ….(ii)
From (i) and (ii)
∠A = ∠DCA
Now in ∆CED and ∆ABC,
∠E = ∠B (each 90°)
∠DEA or ∠DCE = ∠A (proved)
∆CED ~ ∆ABC (AA axiom)
Hence proved.

Question 9.
Diagonals AC and BD of a trapezium ABCD with AB || DC intersect each other at the point O. Using similarity criterion
for two triangles, show that \(\frac { OA }{ OC }\) = \(\frac { OB }{ OD }\)
Solution:
Given : ABCD is a trapezium in which AB || DC and diagonals AC and BD intersect each other at O
To Prove : \(\frac { OA }{ OC }\) = \(\frac { OB }{ OD }\)

Question 10.
In ∆ABC and ∆AMP are two right triangles, right angled at B and M respectively such that ∠MAP = ∠BAC. Prove that
(i) ∆ABC ~ ∆AMP
(ii) \(\frac { CA }{ PA }\) = \(\frac { BC }{ MP }\).
Solution:
Given : In ∆ABC and ∆AMP,
∠B = ∠M = 90°
∠MAP = ∠BAC

Question 11.
A vertical stick 10 cm long casts a shadow of 8 cm long. At the same time a tower casts a shadow 30 m long. Determine the height of the tower. (CB.S.E. 1991)
Solution:
The shadows are casted by a vertical stick and a tower at the same time
Their angles will be equal

Question 12.
In the figure, A = CED, prove that ∠CAB ~ ∠CED. Also find the value of x.

Solution:
Given : In ∆ABC,
∠CED = ∠A
AB = 9 cm, BE = 2 cm, EC = 10 cm, AD = 7 cm and DC = 8 cm
To prove :
(i) ∆CAB ~ ∆CED
(ii) Find the value of x
Proof: BC = BE + EC = 2 + 10 = 12 cm
AC = AD + DC = 7 + 8 = 15 cm
(i) Now in ∆CAB and ∆CED,
∠A = ∠CED (given)
∠C = ∠C (common)
∆CAB ~ ∆CED (AA axiom)

Question 13.
The perimeters of two similar triangles are 25 cm and 15 cm respectively. If one side of first triangle is 9 cm, what is the corresponding side of the other triangle? (C.B.S.E. 2002C)
Solution:
Let perimeter of ∆ABC = 25 cm
and perimeter of ∆DEF = 15 cm
and side BC of ∆ABC = 9 cm
Now we have to find the side EF of ∆DEF
∆ABC ~ ∆DEF (given)

Question 14.
In ∆ABC and ∆DEF, it is being given that: AB = 5 cm, BC = 4 cm and CA = 4.2 cm; DE = 10 cm, EF = 8 cm and FD = 8.4 cm. If AL ⊥ BC and DM ⊥ EF, find AL : DM.
Solution:
In ∆ABC and ∆DEF,
AB = 5 cm, BC = 4 cm, CA = 4.2 cm, DE = 10 cm, EF = 8 cm and FD = 8.4 cm
AL ⊥ BC and DM ⊥ EF

Question 15.
D and E are the points on the sides AB and AC respectively of a ∆ABC such that: AD = 8 cm, DB = 12 cm, AE = 6 cm and CE = 9 cm. Prove that BC = \(\frac { 5 }{ 2 }\) DE.
Solution:
Given : In ∆ABC, points D and E are on the sides AB and AC respectively
and AD = 8 cm, DB = 12 cm, AE = 6 cm and CE = 9 cm


Hence proved

Question 16.
D is the mid-point of side BC of a ∆ABC. AD is bisected at the point E and BE produced cuts AC at the point X. Prove that BE : EX = 3 : 1.
Solution:
Given : In ∆ABC, D is mid point of BC, and E is mid point of AD
BE is joined and produced to meet AC at X
To prove : BE : EX = 3 : 1
Construction : From D, draw DY || BX meeting AC at Y


Hence proved.

Question 17.
ABCD is a parallelogram and APQ is a straight line meeting BC at P and DC produced at Q. Prove that the rectangle obtained by BP and DQ is equal to the rectangle contained AB and BC.
Solution:
Given : ABCD is a parallelogram.
APQ is a straight line which meets BC at P and DC on producing at Q

Question 18.
In ∆ABC, AL and CM are the perpendiculars from the vertices A and C to BC and AB respectively. If AL and CM intersect at O, prove that :
(i) ∆OMA ~ ∆OLC
(ii) \(\frac { OA }{ OC }\) = \(\frac { OM }{ OL }\)
Solution:
Given : In ∆ABC, AL ⊥ BC, CM ⊥ AB which intersect each other at O

(ii) \(\frac { OA }{ OC }\) = \(\frac { OM }{ OL }\)
Hence proved.

Question 19.
ABCD is a quadrilateral in which AD = BC. If P, Q, R, S be the mid-points of AB, AC, CD and BD respectively, show that PQRS is a rhombus.
Solution:
Given : In quadrilateral ABCD, AD = BC
P, Q, R and S are the mid points of AB, AC, CD and AD respectively
PQ, QR, RS, SP are joined

Question 20.
In an isosceles ∆ABC, the base AB is produced both the ways to P and Q such that AP x BQ = AC². Prove that ∆APC ~ ∆BCQ.
Solution:
Given : In ∆ABC, AC = BC
Base AB is produced to both sides and points P and Q are taken in such a way that
AP x BQ = AC²
CP and CQ are joined
To prove : ∆APC ~ ∆BCQ

Question 21.
A girl of height 90 cm is walking away from the base of a lamp-post at a speed of 1.2 m/sec. If the lamp is 3.6 m above ground, find the length of her shadow after 4 seconds.
Solution:
Let AB be the lamp post and CD be the girl and AB = 3.6 m, CD = \(\frac { 90 }{ 100 }\) = 9 m
Distance covered in 4 seconds = 1.2 m x 4 = 4.8 m
BD = 4.8 m

x = 1.6
Length of her shadow = 1.6 m

Question 22.
A vertical stick of length 6 m casts a shadow 4 m long on the ground and at the same time a tower casts a shadow 28 m long. Find the height of the tower.
Solution:
Let AB be stick and DE be tower.
A stick 6 m long casts a shadow of 4 m i.e., AB = 6 m and BC = 4 m
Let DE casts shadow at the same time which is EF = 28 m
Let height of tower DE = x
Now in ∆ABC and ∆DEF,
∠B = ∠E (each 90°)
∠C = ∠F (shadows at the same time)
∆ABC ~ ∆DEF (AA criterion)

Question 23.
In the figure, ∆ABC is right angled at C and DE ⊥ AB. Prove that ∆ABC ~ ∆ADE and hence find the lengths of AE and DE.

Solution:
Given: In the figure, ∆ABC is a right angled triangle right angle at C.
DE ⊥ AB
To prove:
(i) ∆ABC ~ ∆ADE
(ii) Find the length of AE and DE
Proof: In ∆ABC and ∆ADE,
∠ACB = ∠AED (each 90°)
∠BAC = ∠DAE (common)
∆ABC ~ ∆ADE (AA axiom)

Question 24.
In the figure, PA, QB and RC are each perpendicular to AC. Prove that

Solution:
Given : In the figure, PA, QB and RC are perpendicular on AC and PA = x, QB = z and RC = y



Hence proved.

Question 25.
In the figure, we have AB || CD || EF. If AB = 6 cm, CD = x cm, EF = 10 cm, BD = 4 cm and DE = y cm, calculate the values of x and y.

Solution:
In the figure, AB || CD || EF
AB = 6 cm, EF = 10 cm, BD = 4 cm, CD = x cm and DE = y cm
In ∆ABE, CE || AB
∆CED ~ ∆AEB

Hope given RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.5 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.4

These Solutions are part of RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.4

Other Exercises

• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.1
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.2
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.3
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.4
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.5
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.6
• RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.7
• RD Sharma Class 10 Solutions Chapter 7 Triangles Revision Exercise
• RD Sharma Class 10 Solutions Chapter 7 Triangles VSAQS
• RD Sharma Class 10 Solutions Chapter 7 Triangles MCQS

Question 1.
(i) In figure, if AB || CD, find the value of x.

(ii) In figure, if AB || CD, find the value of x.

(iii) In figure, AB || CD. If OA = 3x – 19, OB = x – 4, OC = x – 3 and OD = 4, find x. (C.B.S.E. 2000C)

Solution:
(i) In the figure, AB || CD
The diagonals of a trapezium divides each other proportionally




=> (x – 8)(x – 11) = 0
Either x – 8 = 0, then x = 8 or x – 11 =0, then x = 11
x = 8 or 11

Hope given RD Sharma Class 10 Solutions Chapter 7 Triangles Ex 7.4 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry MCQS

These Solutions are part of RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry MCQS

Other Exercises

• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.1
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.2
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.3
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.4
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.5
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry VSAQS
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry MCQS

Mark the correct alternative in each of the following :
Question 1.
The distance between the points (cosθ, sinθ) and (sinθ, -cosθ) is
(a) √3
(b) √2
(c) 2
(d) 1
Solution:
(b) Distance between (cosθ, sinθ) and (sinθ, -cosθ)

Question 2.
The distance between the points (a cos 25°, 0) and (0, a cos 65°) is
(a) a
(b) 2a
(c) 3a
(d) None of these
Solution:
(a) Distance between (a cos 25°, 0) and (0, a cos 65°)

Question 3.
If x is a positive integer such that the distance between points P (x, 2) and Q (3, -6) is 10 units, then x =
(a) 3
(b) -3
(c) 9
(d) -9
Solution:
(c) Distance between P (x, 2) and Q (3, -6) = 10 units

=> x (x – 9) + 3 (x – 9) = 0
(x – 9) (x + 3) = 0
Either x – 9 = 0, then x = 9 or x + 3 = 0, then x = -3
x is positive integer
Hence x = 9

Question 4.
The distance between the points (a cosθ + b sinθ, 0) and (0, a sinθ – b cosθ) is
(a) a² + b²
(b) a + b
(c) a² – b²
(d) √(a²+b²)
Solution:
(d) Distance between (a cosθ + b sinθ, 0) and (0, a sinθ – b cosθ)

Question 5.
If the distance between the points (4, p) and (1, 0) is 5, then p =
(a) ±4
(b) 4
(c) -4
(d) 0
Solution:
(a) Distance between (4, p) and (1, 0) = 5

Question 6.
A line segment is of length 10 units. If the coordinates of its one end are (2, -3) and the abscissa of the other end is 10, then its ordinate is
(a) 9, 6
(b) 3, -9
(c) -3, 9
(d) 9, -6
Solution:
(b) Let the ordinate of other end = y
then distance between (2, -3) and (10, y) = 10 units

Question 7.
The perimeter of the triangle formed by the points (0, 0), (1, 0) and (0, 1) is
(a) 1 ± √2
(b) √2 + 1
(c) 3
(d) 2 + √2
Solution:
(d) Let the vertices of ∆ABC be A (0, 0), B(1, 0) and C (0, 1)

Question 8.
If A (2, 2), B (-4, -4) and C (5, -8) are the vertices of a triangle, then the length of the median through vertices C is
(a) √65
(b) √117
(c) √85
(d) √113
Solution:
(c) Let mid point of A (2, 2), B (-4, -4) be D whose coordinates will be

Question 9.
If three points (0, 0), (3, √3) and (3, λ) form an equilateral triangle, then λ =
(a) 2
(b) -3
(c) -4
(d) None of these
Solution:
(d) Let the points (0, 0), (3, √3) and (3, λ) from an equilateral triangle
AB = BC = CA
=> AB² = BC² = CA²
Now, AB² = (x₂ – x₁)² + (y₂ – y₁)²

Question 10.
If the points (k, 2k), (3k, 3k) and (3, 1) are collinear, then k
(a) \(\frac { 1 }{ 3 }\)
(b) \(\frac { -1 }{ 3 }\)
(c) \(\frac { 2 }{ 3 }\)
(d) \(\frac { -2 }{ 3 }\)
Solution:
(b) Let the points A (k, 2k), B (3k, 3k) and C (3, 1) be the vertices of a ∆ABC

Question 11.
The coordinates of the point on x-axis which are equidistant from the points (-3, 4) and (2, 5) are
(a) (20, 0)
(b) (-23, 0)
(c) (\(\frac { 4 }{ 5 }\) , 0)
(d) None of these
Solution:
(d)

Question 12.
If (-1, 2), (2, -1) and (3, 1) are any three vertices of a parallelogram, then
(a) a = 2, b = 0
(b) a = -2, b = 0
(c) a = -2, b = 6
(d) a = 0, b = 4
Solution:
(d) In ||gm ABCD, diagonals AC and AD bisect each other at O
O is mid-point of AC

Question 13.
If A (5, 3), B (11, -5) and P (12, y) are the vertices of a right triangle right angled at P, then y =
(a) -2, 4
(b) -2, 4
(c) 2, -4
(d) 2, 4
Solution:
(c) A (5, 3), B (11, -5) and P (12, y) are the vertices of a right triangle, right angle at P

Question 14.
The area of the triangle formed by (a, b + c), (b, c + a) and (c, a + b) is
(a) a + b + c
(b) abc
(c) (a + b + c)²
(d) 0
Solution:
(d) Vertices of a triangle are (a, b + c), (b, c + a) and (c, a + b)

Question 15.
If (x, 2), (-3, -4) and (7, -5) are coliinear, then x =
(a) 60
(b) 63
(c) -63
(d) -60
Solution:
(c) Area of triangle whose vertices are (x, 2), (-3, -4) and (7, -5)

Question 16.
If points (t, 2t), (-2, 6) and (3, 1) are collinear, then t =
(a) \(\frac { 3 }{ 4 }\)
(b) \(\frac { 4 }{ 3 }\)
(c) \(\frac { 5 }{ 3 }\)
(d) \(\frac { 3 }{ 5 }\)
Solution:
(b) The area of triangle whose vertices are (t, 2t), (-2, 6) and (3, 1)

Question 17.
If the area of the triangle formed by the points (x, 2x), (-2, 6) and (3, 1) is 5 square units, then x =
(a) \(\frac { 2 }{ 3 }\)
(b) \(\frac { 3 }{ 5 }\)
(c) 2
(d) 5
Solution:
(c) Area of triangle whose vertices are (x, 2x), (-2, 6) and (3, 1)

Question 18.
If points (a, 0), (0, b) and (1, 1) are collinear, then \(\frac { 1 }{ a }\) + \(\frac { 1 }{ b }\) =
(a) 1
(b) 2
(c) 0
(d) -1
Solution:
(a) The area of triangle whose vertices are (a, 0), (0, b) and (1, 1)

Question 19.
If the centroid of a triangle is (1, 4) and two of its vertices are (4, -3) and (-9, 7), then the area of the triangle is
(a) 183 sq. units
(b) \(\frac { 183 }{ 2 }\) sq. units
(c) 366 sq. units
(d) \(\frac { 183 }{ 4 }\) sq. units
Solution:
(b) Centroid of a triangle = (1, 4)
and two vertices of the triangle are (4, -3) and (-9, 7)
Let the third vertex be (x, y), then

= \(\frac { 183 }{ 2 }\) sq. units

Question 20.
The line segment joining points (-3, -4) and (1, -2) is divided by y-axis in the ratio
(a) 1 : 3
(b) 2 : 3
(c) 3 : 1
(d) 2 : 3
Solution:
(c) The point lies on y-axis
Its abscissa will be zero
Let the point divides the line segment joining the points (-3, -4) and (1, -2) in the ratio m : n

Question 21.
The ratio in which (4, 5) divides the join of (2, 3) and (7, 8) is
(a) -2 : 3
(b) -3 : 2
(c) 3 : 2
(d) 2 : 3
Solution:
(d) Let the point (4, 5) divides the line segment joining the points (2, 3) and (7, 8) in the ratio m : n

Question 22.
The ratio in which the X-axis divides the segment joining (3, 6) and (12, -3) is
(a) 2 : 1
(b) 1 : 2
(c) -2 : 1
(d) 1 : -2
Solution:
(a) The point lies on x-axis
Its ordinate is zero
Let this point divides the line segment joining the points (3, 6) and (12, -3) in the ratio m : n

Question 23.
If the centroid of the triangle formed by the points (a, b), (b, c) and (c, a) is at the origin, then a³ + b³ + c³ =
(a) abc
(b) 0
(c) a + b + c
(d) 3 abc
Solution:
(d) Centroid of the triangle formed by the points (a, b), (b, c) and (c, a) is origin (0, 0)

Question 24.
If points (1, 2), (-5, 6) and (a, -2) are collinear, then a =
(a) -3
(b) 7
(c) 2
(d) -2
Solution:
(b) The area of a triangle whose vertices are (1, 2), (-5, 6) and (a, -2)

Question 25.
If the centroid of the triangle formed by (7, x), (y, -6) and (9, 10) is at (6, 3), then (x, y) =
(a) (4, 5)
(b) (5, 4)
(c) (-5, -2)
(d) (5, 2)
Solution:
(d) Centroid of (7, x), (y, -6) and (9, 10) is (6, 3)

Question 26.
The distance of the point (4, 7) from the x-axis is
(a) 4
(b) 7
(c) 11
(d) √65
Solution:
(b) The distance of the point (4, 7) from x-axis = 7

Question 27.
The distance of the point (4, 7) from the y-axis is
(a) 4
(b) 7
(c) 11
(d) √65
Solution:
(a) The distance of the point (4, 7) from y-axis = 4

Question 28.
If P is a point on x-axis such that its distance from the origin is 3 units, then the coordinates of a point Q on OY such that OP = OQ, are
(a) (0, 3)
(b) (3, 0)
(c) (0, 0)
(d) (0, -3)
Solution:
(a) P is a point on x-axis and its distance from 0 is 3
Co-ordinates of P will be (3, 0)
Q is a point on OY such that OP = OQ
Co-ordinates of Q will be (0, 3)

Question 29.
If the point (x, 4) lies on a circle whose centre is at the origin and radius is 5, then x =
(a) ±5
(b) ±3
(c) 0
(d) ±4
Solution:
(b) Point A (x, 4) is on a circle with centre O (0, 0) and radius = 5

Question 30.
If the point P (x, y) is equidistant from A (5, 1) and B (-1, 5), then
(a) 5x = y
(b) x = 5y
(c) 3x = 2y
(d) 2x = 3y
Solution:
(c)

Question 31.
If points A (5, p), B (1, 5), C (2, 1) and D (6, 2) form a square ABCD, then p =
(a) 7
(b) 3
(c) 6
(d) 8
Solution:
(c) Vertices of a square are A (5, p), B (1, 5), C (2, 1) and D (6, 2)
The diagonals bisect each other at O
O is the mid-point of AC and BD
O is mid-point of BD, then

Question 32.
The coordinates of the circumcentre of the triangle formed by the points O (0, 0), A (a, 0) and B (0, b) are
(a) (a, b)
(b) (\(\frac { a }{ 2 }\) , \(\frac { b }{ 2 }\))
(c) (\(\frac { b }{ 2 }\) , \(\frac { a }{ 2 }\))
(d) (b, a)
Solution:
(b) Let co-ordinates of C be (x, y) which is the centre of the circumcircle of ∆OAB
Radii of a circle are equal

Question 33.
The coordinates of a point on x-axis which lies on the perpendicular bisector of the line segment joining the points (7, 6) and (-3, 4) are
(a) (0, 2)
(b) (3, 0)
(b) (0, 3)
(d) (2, 0)
Solution:
(d) The given point P lies on x-axis
Let the co-ordinates of P be (x, 0)
The point P lies on the perpendicular bisector of of the line segment joining the points A (7, 6), B (-3, 4)

Question 34.
If the centroid of the triangle formed by the points (3, -5), (-7, 4), (10, -k) is at the point (k, -1), then k =
(a) 3
(b) 1
(c) 2
(d) 4
Solution:
(c) O (k, -1) is the centroid of triangle whose vertices are

Question 35.
If (-2, 1) is the centroid of the triangle having its vertices at (x, 0), (5, -2), (-8, y), then x, y satisfy the relation
(a) 3x + 8y = 0
(b) 3x – 8y = 0
(c) 8x + 3y = 0
(d) 8x = 3y
Solution:
(-2, 1) is the centroid of triangle whose vertices are (x, 0), (5, -2), (-8, y)

Question 36.
The coordinates of the fourth vertex of the rectangle formed by the points (0, 0), (2, 0), (0, 3) are
(a) (3, 0)
(b) (0, 2)
(c) (-2, 3)
(d) (3, 2)
Solution:
(c) Three vertices of a rectangle are A (0, 0), B (2, 0), C (0, 3)
Let fourth vertex be D (x, y)
The diagonals of a rectangle bisect eachother at O
O is the mid-point of AC, then
Coordinates of O will be (\(\frac { 0+0 }{ 2 }\) , \(\frac { 0+3 }{ 2 }\))

Question 37.
The length of a line segment joining A (2, -3) and B is 10 units. If the abscissa of B is 10 units, then its ordinates can be
(a) 3 or -9
(b) -3 or 9
(c) 6 or 27
(d) -6 or-27
Solution:
(a) Abscissa of B is 10 and co-ordinates of A are (2, -3)
Let ordinates of B be y, then

Question 38.
The ratio in which the line segment joining P(x₁, y₁) and Q (x₂, y₂) is divided by x-axis is
(a) y₁ : y₂
(b) -y₁ : y₂
(c) x₁ : x₂
(d) -x₁ : x₂
Solution:
(b) Let a point A on x-axis divides the line segment joining the points P (x₁, y₁), Q (x₂, y₂) in the ratio m₁ : m₂ and
let co-ordinates of A be (x, 0)

Question 39.
The ratio in which the line segment joining points A (a₁, b₁) and B (a₂, b₂) is divided by y-axis is
(a) -a₁ : a₂
(b) a₁ : a₂
(c) b₁ : b₂
(d) -b₁ : b₂
Solution:
(a) Let the point P on y-axis, divides the line segment joining the point A (a₁, b₁) and B (a₂, b₂) is the ratio m₁ : m₂ and
let the co-ordinates of P be (0, y), then

Question 40.
If the line segment joining the points (3, -4) and (1, 2) is trisected at points P

Solution:
(b)

Question 41.
If the coordinates of one end of a diameter of a circle are (2, 3) and the coordinates of its centre are (-2, 5), then the coordinates of the other end of the diameter are [CBSE 2012]
(a) (-6, 7)
(b) (6, -7)
(c) (6, 7)
(d) (-6, -7)
Solution:
(a) Let AB be the diameter of a circle with centre O

Question 42.
The coordinates of the point P dividing the line segment joining the points A (1, 3) and B (4, 6) in the ratio 2 : 1 are
(a) (2, 4)
(b) (3, 5)
(c) (4, 2)
(d) (5, 3) [CBSE 2012]
Solution:
(b) Point P divides the line segment joining the points A (1, 3) and B (4, 6) in the ratio 2 : 1
Let coordinates of P be (x, y), then

Question 43.
In the figure, the area of ∆ABC (in square units) is [CBSE 2013]
(a) 15
(b) 10
(c) 7.5
(d) 2.5

Solution:
(c)

Question 44.
The point on the x-axis which is equidistant from points (-1, 0) and (5, 0) is
(a) (0, 2)
(b) (2, 0)
(c) (3, 0)
(d) (0, 3) [CBSE 2013]
Solution:
(c) Let the point P (x, 0) is equidistant from the points A (-1, 0), B (5, 0)

Question 45.
If A (4, 9), B (2, 3) and C (6, 5) are the vertices of ∆ABC, then the length of median through C is
(a) 5 untis
(b) √10 units
(c) 25 units
(d) 10 units [CBSE 2014]
Solution:
(b) A (4, 9), B (2, 3) and C (6, 5) are the vertices of ∆ABC
Let median CD has been drawn C (6, 5)

Question 46.
If P (2, 4), Q (0, 3), R (3, 6) and S (5, y) are the vertices of a paralelogram PQRS, then the value of y is
(a) 7
(b) 5
(c) -7
(d) -8 [CBSE 2014]
Solution:
(a) P (2, 4), Q (0, 3), R (3, 6) and S (5, y) are the vertices of a ||gm PQRS

Question 47.
If A (x, 2), B (-3, -4) and C (7, -5) are collinear, then the value of x is
(a) -63
(b) 63
(c) 60
(d) -60 [CBSE 2014]
Solution:
(a) A (x, 2), B (-3, -4) and C (7, -5) are collinear, then area ∆ABC = 0
Now area of ∆ABC

Question 48.
The perimeter of a triangle with vertices (0, 4) and (0, 0) and (3, 0) is
(a) 7 + √5
(b) 5
(c) 10
(d) 12 [CBSE 2014]
Solution:
(d) A (0, 4) and B (0, 0) and C (3, 0) are the vertices of ∆ABC

Question 49.
If the point P (2, 1) lies on the line segment joining points A (4, 2) and B (8, 4), then
(a) AP = \(\frac { 1 }{ 3 }\) AB
(b) AP = BP
(c) BP = \(\frac { 1 }{ 3 }\) AB
(d) AP = \(\frac { 1 }{ 2 }\) AB
Solution:
(d) Given that, the point P (2, 1) lies on the line segment joining the points (4, 2) and B (8, 4), which shows in the figure below:

Question 50.
A line intersects the y-axis and x-axis at P and Q, respectively. If (2, -5) is the mid-point of PQ, then the coordinates of P and Q are, respectively
(a) (0, -5) and (2, 0)
(b) (0, 10) and (-4, 0)
(c) (0, 4) and (-10, 0)
(d) (0, -10) and (4, 0)
Solution:
(d) Let the coordinates of P and Q (0, y) and (x, 0), respectively.
So, the mid-point of P (0, y) and Q (x, 0) is

2 = \(\frac { x + 0 }{ 2 }\) and -5 = \(\frac { y + 0 }{ 2 }\)
=> 4 = x and -10 = y
=> x = 4 and y = -10
So, the coordinates of P and Q are (0, -10) and (4, 0).

Hope given RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry MCQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry VSAQS

These Solutions are part of RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry VSAQS

Other Exercises

• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.1
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.2
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.3
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.4
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.5
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry VSAQS
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry MCQS

Answer each of the following questions either in one word or one sentence or as per requirement of the questions :
Question 1.
Write the distance between the points A (10 cosθ, 0) and B (0, 10 sinθ).
Solution:
Distance between the points A (10 cosθ, 0) and B (0, 10 sinθ)

Question 2.
Write the perimeter of the triangle formed by the points O (0, 0), A (a, 0), and B (0, b).
Solution:
The vertices of a ∆OAB, O (0, 0), A (a, 0), and B (0, b)
Now length of OA

Question 3.
Write the ratio in which the line segment joining points (2, 3) and (3, -2) is divided by x-axis.
Solution:
The required point is on x-axis
Its ordinate will be 0
Let the point be (x, 0) and let this point divides the join of the points (2, 3) and (3, -2) in the ratio m : n

Question 4.
What is the distance between the points (5 sin 60°, 0) and (0, 5 sin 30°) ?
Solution:
Distance between the given points

Question 5.
If A (-1, 3), B (1, -1) and C (5, 1) are the vertices of a triangle ABC, what is the length of the median through vertex A ?
Solution:
The vertices of ∆ABC are A (-1, 3), B (1, -1) and C (5, 1)
Let AD be the median

Question 6.
If the distance between points (x, 0) and (0, 3) is 5, what are the value of x ?
Solution:
Distance between (x, 0) and (0, 3) = 5

Question 7.
What is the area of the triangle formed by the points O (0, 0), A (6, 0) and B (0, 4) ?
Solution:
The vertices of the triangle OAB are O (0, 0), A (6, 0) and B (0, 4)

Question 8.
Write the coordinates of the point dividing line segment joining points (2, 3) and (3, 4) internally in the ratio 1 : 5.
Solution:
Let the coordinates of the required point be (x, y), then

Question 9.
If the centroid of the triangle formed by points P (a, b), Q (b, c) and R (c, a) is at the origin, what is the value of a + b + c ?
Solution:
Vertices of ∆PQR are P (a, b), Q (b, c) and R (c, a) and its centroid = O (0, 0)

Question 10.

Solution:

Question 11.
Write the coordinates of a point on x- axis which is equidistant from the points (-3, 4) and (2, 5).
Solution:
The point is on x-axis
Its ordinates of the point P is (x, 0)
P is equidistant from A (-3, 4) and B (2, 5)

Question 12.
If the mid-point of the segment joining A (x, y + 1) and B (x + 1, y + 2) is C (\(\frac { 3 }{ 2 }\) , \(\frac { 5 }{ 2 }\)) find x, y.
Solution:
C (\(\frac { 3 }{ 2 }\) , \(\frac { 5 }{ 2 }\)) is mid point of the line segment

Question 13.
Two vertices of a triangle have co-ordinates (-8, 7) and (9, 4). If the centroid of the triangle is at the origin, what are the co-ordinates of the third vertex ?
Solution:
Two vertices of a triangle are (-8, 7) and (9, 4)
Let the third vertex be (x, y)
Centroid of the triangle is (0, 0)

Question 14.
Write the coordinates the reflections of points (3, 5) in x and y-axes.
Solution:
Reflection of P (3, 5) in x-axis is will be (3, -5)
and reflection of P in y-axis will be (-3, 5)

Question 15.
If points Q and R reflections of point P (-3, 4) in X and Y axes respectively, what is QR ?
Solution:
Reflection of point P (-3, 4) in X-axis will be Q with coordinates Q (-3, -4) and reflection in Y-axis will be R (3, 4)

Question 16.
Write the formula for the area of the triangle having its vertices at (x₁, y₁), (x₂, y₂) and (x₃, y₃).
Solution:

Question 17.
Write the condition of collinearity of points (x₁, y₁), (x₂, y₂) and (x₃, y₃).
Solution:
Three points (x₁, y₁), (x₂, y₂) and (x₃, y₃). are said to be collinear if the area of the triangle formed by these point = 0 i.e.,

Question 18.
Find the values of x for which the distance between the point P (2, -3) and Q (x, 5) is 10.
Solution:
Distance between P (2, -3) and Q (x, 5) = 10

Question 19.
Write the ratio in which the line segment joining the points A (3, -6) and B (5, 3) is divided by X-axis.
Solution:
The point lies on x-axis
Its ordinate will be = 0
Let the point P (x, 0) divides the line segment joining the points A (3, -6) and B (5, 3) in the ratio m : n.

Question 20.
Find the distance between the points (\(\frac { -8 }{ 5 }\) , 2) and (\(\frac { 2 }{ 5 }\) , 2). (C.B.S.E. 2009)
Solution:

Question 21.
Find the value of a so that the point (3, a) lies on the line represented by 2x – 3y + 5 = 0. (C.B.S.E. 2009)
Solution:

Question 22.
What is the distance between the points A (c, 0) and B (0, – c) ? [CBSE 2010]
Solution:

Question 23.
If P (2, 6) is the mid-point of the line segment joining A (6, 5) and B (4, y), find y. [CBSE 2010]
Solution:
P (2, 6) is the mid-point of the line segment A (6, 5) and b (4, y)

Question 24.
If the distance between the points (3, 0) and (0, y) is 5 units and y is positive, then what is the value of y ? [CBSE 2010]
Solution:
Distance between (3, 0) and (0, y) is 5 units

Question 25.
If P (x, 6) is the mid-point of the line segment joining A (6, 5) and B (4, y), find y. [CBSE 2010]
Solution:
P (x, 6) is the mid-point of the line segment joining the points A (6, 5), B (4, y)

Question 26.
If P (2, p) is the mid-point of the line segment joining the points A (6, -5) and B (-2, 11), find the value of p. [CBSE 2010]
Solution:
P (2, p) is the mid-point of the line segment joining the points A (6, -5) and B (-2, 11)

Question 27.
If A (1, 2), B (4, 3) and C (6, 6) are the three vertices of a parallelogram ABCD, find the coordinates of fourth vertex D. [CBSE 2010]
Solution:
vertices of a parallelogram Let co-ordinates of D be (x, y)
Diagonals AC and BD bisect each other at O
Co-ordinates of O will be

Question 28.
What is the distance between the points A (sinθ – cosθ, 0) and B (0, sinθ + cosθ)? [CBSE 2015]
Solution:

Question 29.
What are the coordinates of the point where the perpendicular bisector of the line segment joining the points A (1, 5) and B (4, 6) cuts the y-axis?
Solution:
Firstly, we plot the points of the line segment on the paper and join them.


Now, we draw a straight line on paper passes through the mid-point P.
We see that the perpendicular bisector cuts the y-axis at the point (0, 13).
Hence, the required point is (0, 13).

Question 30.
Find the area of the triangle with vertices (a, b + c), (b, c + a) and (c, a + b).
Solution:

Question 31.
If the points A (1, 2), O (0, 0) and C (a, b) are collinear, then find a : b.
Solution:

=> 2a = b
Hence, the required relation is 2a = b

Question 32.
Find the coordinates of the point which is equidistant from the three vertices A (2x, 0), O (0, 0) and B (0, 2y) of ∆AOB.
Solution:
Let the coordinate of the point which is equidistant from the three vertices O (0, 0), A (0, 2y) and B (2x, 0) is P (h, k).

Question 33.
If the distance between the points (4, k), and (1, 0) is 5, then what can be the possible value of k? [CBSE 2017]
Solution:
Let the points x (4, k) and y (1, 0)
It is given that the distance xy is 5 units.
By using the distance formula,

Hope given RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry VSAQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles VSAQS

These Solutions are part of RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles VSAQS

Other Exercises

• RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles Ex 13.1
• RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles Ex 13.2
• RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles Ex 13.3
• RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles Ex 13.4
• RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles VSAQS
• RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles MCQS

Answer each of the following questions either in one word or one sentence or as per requirement of the questions :
Question 1.
What is the ratio of the areas of a circle and an equilateral triangle whose diameter and a side are respectively equal?
Solution:
Diameter of a circle and side of an equilateral triangle are same
Let the diameter of the circle = a
Then radius (r) = \(\frac { a }{ 2 }\)

Question 2.
If the circumference of two circles are in the ratio 2 : 3, what is the ratio of their areas ?
Solution:
Let R and r be the radii of two circles, then the ratio between their circumferences = 2πR : 2πr

Question 3.
Write the area of the sector of a circle whose radius is r and length of the arc is l.
Solution:
Let arc l subtends angle 9 at the centre of the circle
Now radius of a circle = r
and length of arc =l

Question 4.
What is the length (in terms of π) of the arc that subtends an angle of 36° at the centre of a circle of radius 5 cm?
Solution:
Radius of the circle = 5 cm
Angle at the center = 36°

Question 5.
What is the angle subtended at the centre of a circle of radius 6 cm by an arc of length 3π cm ?
Solution:
Let the arc subtends angle θ at the centre of a circle
Radius of circle (r) = 6 cm
Length of arc = 3π cm

Question 6.
What is the area of a sector of a circle of radius 5 cm formed by an arc of length 3.5 cm ?
Solution:
Radius of the circle (r) = 5 cm
Length of arc (l) = 3.5 cm
Let angle 9 be subtended by the arc at the centre

Question 7.
In a circle of radius 10 cm, an arc subtends an angle of 108° at the centre. What is the area of the sector in terms of π ?
Solution:
Radius of the circle = 10 cm
Angle at the centre = 108°

Question 8.
If a square is inscribed in a circle, what is the ratio of the areas of the circle and the square ?
Solution:
A square ABCD is inscribed in a circle with centre O

Let the radius of the circle = r
Then its area = πr²
Now diagonal of the square = diameter of the circle = 2r

Question 9.
Write the formula for the area of a sector of angle θ (in degrees) of a circle of radius r.
Solution:
Area of a sector of a circle whose radius = r

Question 10.
 Write the formula for the area of a segment in a circle of radius r given that the sector angle is 0 (in degrees).
Solution:
Radius of the circle = r
and angle subtended by the sector at the centre = θ
Area of the segment

Question 11.
If the adjoining figure is a sector of a circle of radius 10.5 cm, what is the perimeter of the sector ? (Take π= 22/7)

Solution:
Radius of the circle = 10.5 cm
Angle at the centre of the circle = 60°

Question 12.
If the diameter of a semi-circular protractor is 14 cm then find its perimeter. (C.B.S.E. 2009)
Solution:
Diameter of semicircular protractor = 14 cm

∴ Radius (r) =  \(\frac { 14 }{ 2 }\) = 7 cm
Now perimeter of protractor

Question 13.
An arc subtends an angle of 90° at the centre of the circle of radius 14 cm. Write the area of minor sector thus formed in terms of π.
Solution:
AB is an arc of the circle with centre O and radius 14 cm and subtends an angle of 90° at the centre O.

Question 14.
Find the area of the largest triangle that can be inscribed in a semi-circle of radius r units. [CBSE 2015]
Solution:
Radius of semicircle = r

In semicircle ΔABC is the largest triangle whose base is AC = 2 x r = 2r units
and height OB = r units

Question 15.
Find the area of a sector of circle of radius 21 cm and central angle 120°.
Solution:

Question 16.
What is the area of a square inscribed in a circle of diameter p cm?
Solution:
Diameter AC of the circle is p.
Also AC is diagonal of square ABCD.
Each angle of square is of 90°

Question 17.
Is it true to say that area of a segment of a circle is less than the area of its corresponding sector? Why?
Solution:
False.
It is true only in the case of minor segment. But in case of major segment, area is always greater than the area of sector.

Question 18.
If the numerical value of the area of a circle is equal to the numerical value of its circumference, find its radius.
Solution:
∵ Numerical value of area of circle = Numerical value of circumference
∴  πr² = 2πr
or r = 2 units

Question 19.
How many revolutions a circular wheel of radius r metres makes in covering a distance of s metres?
Solution:
Radius of circular of wheel (r) = r m
Circumference of a circular wheel = 2πr
Distance to be covered = Sm

Question 20.
Find the ratio of the area of the circle circumscribing a square to the area of the circle inscribed in the square.
Solution:
Let each side of of square = x
∴ Diameter of inner circle = x
Radius r = \(\frac { x }{ 2 }\)
Diameter of outer circle = AD

Hope given RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles VSAQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.5

These Solutions are part of RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.5

Other Exercises

• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.1
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.2
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.3
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.4
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.5
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry VSAQS
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry MCQS

Question 1.
Find the area of a triangle whose vertices are :
(i) (6, 3), (-3, 5) and (4, -2)
(ii) (\({ at }_{ 1 }^{ 2 }\), 2at₁), (\({ at }_{ 2 }^{ 2 }\), 2at₂) and (\({ at }_{ 3 }^{ 2 }\), 2at₃)
(iii) (a, c + a), (a, c) and (-a, c – a)
Solution:
(i) Co-ordinates of ∆ABC are A (6, 3), B (-3, 5) and C (4, -2)

Question 2.
Find the area of the quadrilaterals, the coordinates of whose vertices are
(i) (-3, 2), (5, 4), (7, -6) and (-5, -4)
(ii) (1, 2), (6, 2), (5, 3) and (3, 4)
(iii) (-4, -2), (-3, -5), (3, -2), (2, 3) (C.B.S.E. 2009)
Solution:
(i) Let vertices of quadrilateral ABCD be A (-3, 2), B (5, 4), C (7, -6) and D (-5, -4)
Join AC

Question 3.
The four vertices of a quadrilaterals are (1, 2), (-5, 6), (7, -4) and (k, -2) taken in order. If the area of the quadrilateral is zero, find the value of k ?
Solution:
Let the vertices of quadrilateral ABCD be
A (1, 2), B (-5, 6), C (7, -4) and D (k, -2)
Join AC

Question 4.
The vertices of ∆ABC are (-2, 1), (5, 4) and (2, -3) respectively. Find the area of the triangle and the length of the altitude through A.
Solution:
Vertices of ∆ABC are A (-2, 1), B (5, 4) and C (2, -3) and AD ⊥ BC, let AD = h
Now area of ∆ABC

Question 5.
Show that the following sets of points are collinear
(a) (2, 5), (4, 6) and (8, 8)
(b) (1, -1), (2, 1) and (4, 5)
Solution:
We know that points are collinear if the area of the triangle formed by them is zero
(a) Vertices of ∆ABC are (2, 5), (4, 6) and (8, 8)

Question 6.
Find the area of a quadrilateral ABCD, the coordinates of whose varities are A (-3, 2), B (5, 4), C (7, -6) and D (-5, -4). [CBSE 2016]
Solution:
Area of quadrilateral ABCD
= area of ∆ABC + area of ∆ACD

Question 7.
In ∆ABC, the coordinates of vertex A are (0, -1) and D (1, 0) and E (0, 1) respectively the mid-points of the sides AB and AC. If F is the mid-point of side C, find the area of ∆DEF. [CBSE 2016]
Solution:
Let B (p, q), C (r, s) and F (x, y)
Mid-point of AB = Coordinates of D

Question 8.
Find the area of the triangle PQR with Q (3, 2) and the mid-points of the sides through Q being (2, -1) and (1, 2). [CBSE 2015]
Solution:
In ∆PQR, L and N are mid points of QR and QP respectively coordinates of Q are (3, 2) of L are (2, -1) and of N are (1, 2)

Question 9.
If P (-5, -3), Q (-4, -6), R (2, -3) and S (1, 2) are the vertices of a quadrilateral PQRS, find its area. [CBSE 2015]
Solution:
P (-5, -3), Q (-4, -6), R (2, -3) and S (1,2) are the vertices of a quadrilateral PQRS
Join PR which forms two triangles PQR and PSR

Question 10.
If A (-3, 5), B (-2, -7), C (1, -8) and D (6, 3) are the vertices of a quadrilateral ABCD, find its area. [CBSE 2014]
Solution:
A (-3, 5), B (-2, -7), C (1,-8) and D (6, 3) are the vertices of a quadrilateral ABCD
Join AC

Question 11.
For what value of ‘a’ the points (a, 1), (1, -1) and (11, 4) are collinear?
Solution:
Let the vertices of ∆ABC are A (a, 1), B (1, -1) and C (11, 4)

Question 12.
Prove that the points (a, b), (a₁, b₁) and (a – a₁, b – b₁) are collinear if ab₁ = a₁b.
Solution:

Question 13.
If the vertices of a triangle are (1, -3), (4, p) and (-9, 7) and its area is 15 sq. units, find the value(s) of p. [CBSE 2012]
Solution:
The vertices of a triangle are (1, -3), (4, p) and (-9, 7) and area of triangle = 15 sq. units

Question 14.
If (x, y) be on the line joining the two points (1, -3) and (-4, 2), prove that x + y + 2 = 0.
Solution:
Point (x, y) be on the line joining the two points (1, -3) and (-4, 2)
Points (x, y), (1, -3) and (-4, 2) are collinear
Let the points (x, y) (1, -3) and (-4, 2) are the vertices of a triangle, then

Question 15.
Find the value of k if points (k, 3), (6, -2) and (-3, 4) are collinear. [CBSE 2008]
Solution:
Let the points (k, 3), (6, -2) and (-3, 4) be the vertices of a triangle, then

Question 16.
Find the value of k, if the points A (7, -2), B (5, 1) and C (3, 2k) are collinear. [CBSE 2010]
Solution:
Points A (7, -2), B (5, 1) and C (3, 2k) are collinear
area of ∆ABC = 0

Question 17.
If the point P (m, 3) lies on the line segment joining the points A (\(\frac { -2 }{ 5 }\) , 6) and B (2, 8), find the value of m.
Solution:

Question 18.
If R (x, y) is a point on the line segment joining the points P (a, b) and Q (b, a), then prove that x + y = a + b. [CBSE 2010]
Solution:
Point R (x, y) lies on the line segment joining the points P (a, b) and Q (b, a)
Area of ∆PRQ = 0

Question 19.
Find the value of k, if the points A (8, 1), B (3, -4) and C (2, k) are collinear. [CBSE 2010]
Solution:
The points A (8, 1), B (3, -4) and C (2, k) are collinear
Area of ∆ABC = 0

Question 20.
Find the value of a for which the area of the triangle formed by the points A (a, 2a), B (-2, 6) and C (3, 1) is 10 square units.
Solution:

Question 21.
If a ≠ b ≠ 0, prove that the points (a, a²), (b, b²), (0, 0) are never collinear. [CBSE 2017]
Solution:

Question 22.
The area of a triangle is 5 sq. units. Two of its vertices are at (2, 1) and (3, -2). If the third vertex is (\(\frac { 7 }{ 2 }\) , y), find y. [CBSE 2017]
Solution:

Question 23.
Prove that the points (a, 0), (0, b) and (1, 1) are collinear if, \(\frac { 1 }{ a }\) + \(\frac { 1 }{ b }\) = 1.
Solution:
Let the points are A (a, 0), B (0, b) and C (1, 1) which form a triangle

Question 24.
The point A divides the join of P (-5, 1) and Q (3, 5) in the ratio k : 1. Find the two values of k for which the area of ∆ABC where B is (1, 5) and C (7, -2) is equal to 2 units.
Solution:
Let the coordinates of A be (x, y) which divides the join of P (-5, 1) and Q (3, 5) in the ratio. Then

Question 25.
The area of a triangle is 5. Two of its vertices are (2, 1) and (3, -2). The third vertex lies on y = x + 3. Find the third vertex.
Solution:
Let the coordinates of third vertex of the triangle be (x, y) and other two vertices are (2, 1) and (3, 2)

Question 26.
If a ≠ b ≠ c, prove that the points (a, a²), (b, b²), (c, c²) can never be collinear.
Solution:

Question 27.
Four points A (6, 3), B (-3, 5), C (4, -2) and D (x, 3x) are given in such a way that \(\frac { \triangle DBC }{ \triangle ABC } =\frac { 1 }{ 2 }\) , find x?
Solution:
Let A (6, 3), B (-3, 5), C (4, -2) and D (x, 3x) are the vertices of quadrilateral ABCD
AC and BD are joined

Question 28.
If three points (x₁, y₁), (x₂, y₂), (x₃, y₃) lie on the same line, prove that

Solution:
Let the points (x₁, y₁), (x₂, y₂), (x₃, y₃) are the vertices of a triangle

Question 29.
Find the area of a parallelogram ABCD if three of its vertices are A (2, 4), B (2 + √3, 5) and C (2, 6). [CBSE 2013]
Solution:
Three vertices of a ||gm ABCD are A (2, 4), B (2 + √3 , 5) and C (2, 6).
Draw one diagonal AC of ||gm ABCD

Question 30.
Find the value (s) of k for which the points (3k – 1, k – 2), (k, k – 7) and (k – 1, -k – 2) are collinear. [CBSE 2014]
Solution:

Question 31.
If the points A (-1, -4), B (b, c) and C (5, -1) are collinear and 2b + c = 4, find the values of b and c. [CBSE 2014]
Solution:

Question 32.
If the points A (-2, 1), B (a, b) and C (4, -1) are collinear and a – b = 1, find the values of a and 6. [CBSE 2014]
Solution:
Points A (-2, 1), B (a, b) and C (4, -1) are
collinear if area ∆ABC = 0
Now area of ∆ABC

Question 33.
If the points A (1, -2), B (2, 3), C (a, 2) and D (-4, -3) form a parallelogram, find the value of a and height of the parallelogram taking AB as base. [NCERT Exemplar]
Solution:
In parallelogram, we know that, diagonals bisects each other
i.e., mid-point of AC = mid-point of BD

Question 34.
A (6, 1), B (8, 2) and C (9, 4) are three vertices of a parallelogram ABCD. If E is the mid-point of DC, find the area of ∆ADE. [NCERT Exemplar]
Solution:
Given that, A (6,1), B (8,2) and C (9,4) are three vertices of a parallelogram ABCD.
Let the fourth vertex of parallelogram be (x, y).
We know that, the diagonal of a parallelogram bisect each other.

Question 35.
If D (\(\frac { -1 }{ 2 }\), \(\frac { 5 }{ 2 }\)) E (7, 3) and F (\(\frac { 7 }{ 2 }\), \(\frac { 7 }{ 2 }\)) are the mid-points of sides of ∆ABC, find the area of ∆ABC. [NCERT Exemplar]
Solution:

Hope given RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.5 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.4

These Solutions are part of RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.4

Other Exercises

• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.1
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.2
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.3
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.4
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.5
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry VSAQS
• RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry MCQS

Question 1.
Find the centroid of the triangle whose vertices are :
(i) (1, 4), (-1, -1), (3, -2)
(ii) (-2, 3), (2, -1), (4, 0)
Solution:

Question 2.
Two vertices of a triangle are (1, 2), (3, 5) and its centroid is at the origin. Find the Co-ordinates of the third vertex.
Solution:
Centroid of a triangle is O(0, 0) ….(i)
Co-ordinates of two vertices of a ∆ABC are A (1, 2) and B (3, 5)
Let the third vertex be (x, y)

Question 3.
Find the third vertex of a triangle, if two of its vertices are at (-3, 1) and (0, -2) and the centroid is at the origin.
Solution:
Let two vertices of a ∆ABC be A (-3, 1) and B (0, -2) and third vertex C be (x, y)
Centroid of the ∆ABC is O (0, 0)

Question 4.
A (3, 2) and B (-2, 1) are two vertices of a triangle ABC whose centroid G has the coordinates (\(\frac { 5 }{ 3 }\) , \(\frac { -1 }{ 3 }\)) . Find the coordinates of the third vertex C of the triangle. [CBSE 2004]
Solution:
A (3, 2) and B (-2, 1) are the two vertices of ∆ABC whose centroid is G (\(\frac { 5 }{ 3 }\) , \(\frac { -1 }{ 3 }\))
Let third vertex C be (x, y)

Question 5.
If (-2, 3), (4, -3) and (4, 5) are the mid-points of the sides of a triangle, find the co-ordinates of its centroid.
Solution:
In ∆ABC, D, E and F are the mid-points of the sides BC, CA and AB respectively.
The co-ordinates of D are (-2, 3), of E are (4,-3) and of F are (4, 5)
Let the co-ordinates of A, B and C be (x₁, y₁), (x₂, y₂), (x₃, y₃) respectively

Question 6.
Prove analytically that the line segment joining the middle points of two sides of a triangle is equal to half of the third side.
Solution:
In ∆ABC,
D and E are the mid points of the sides AB and AC respectively

Question 7.
Prove that the lines joining the middle points of the opposite sides of a quadrilateral and the join of the middle points of its diagonals meet in a point and bisect one another.
Solution:
Let A (x₁, y₁), B (x₂, y₂), C (x₃, y₃) and D (x₄, y₄) be the vertices of quadrilateral ABCD
E and F are the mid points of side BC and AD respectively and EF is joined G and H are the mid points of diagonal AC and BD.
GH are joined

Question 8.
If G be the centroid of a triangle ABC and P be any other point in the plane, prove that PA² + PB² + PC² = GA² + GB² + GC² + 3GP².
Solution:
In AABC, G is the centroid of it Let P (h, x) is any point in the plane
Let co-ordinates of A are (x₁, y₁) of B are (x₂, y₂) and of C are (x₃, y₃)


Hence proved.

Question 9.
If G be the centroid of a triangle ABC, prove that AB² + BC² + CA² = 3 (GA² + GB² + GC²)
Solution:
Let the co-ordinates of the vertices of ∆ABC be A (x₁, y₁), B (x₂, y₂), C (x₃, y₃) and let G be the centroid of the triangle



Hence proved.

Question 10.
In the figure, a right triangle BOA is given. C is the mid-point of the hypotenuse AB. Show that it is equidistant from the vertices O, A and B.
Solution:

In right ∆OAB, co-ordinates of O are (0, 0) of A are (2a, 0) and of B are (0, 2b)
C is the mid-point of AB
Co-ordinates of C will be

We see that CO = CA = CB
Hence C is equidistant from the vertices O, A and B.
Hence proved.

Hope given RD Sharma Class 10 Solutions Chapter 6 Co-ordinate Geometry Ex 6.4 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles Ex 13.4

These Solutions are part of RD Sharma Class 10 Solutions. Here we have given RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles Ex 13.4

Other Exercises

• RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles Ex 13.1
• RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles Ex 13.2
• RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles Ex 13.3
• RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles Ex 13.4
• RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles VSAQS
• RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles MCQS

Question 1.
A plot is in the form of a rectangle ABCD having semi-circle on BC as shown in the figure. If AB = 60 m and BC = 28 m, find the area of the plot.

Solution:
Plot is formed of a rectangle ABCD and one semicircle on BC as diameter
∴  AB (l) = 60 m,
BC (b) = 28 m
∴ Radius of semicircle (r) = \(\frac { 1 }{ 2 }\) BC
= \(\frac { 1 }{ 2 }\) x 28 = 14m
Area of plot = area of rectangle ABCD + area of semicircle

Question 2.
A play ground has the shape of a rectangle, with two semi-circles on its smaller sides as diameters, added to its outside. If the sides of the rectangle are 36 m and 24.5 m, find the area of the playground. (Take π = 22/7).
Solution:
Length of rectangle (l) = 36 m 49
and width (b) = 24.5 =  \(\frac { 49 }{ 2 }\) m

Question 3.
Find the area of the circle in which a square of area 64 cm2 is inscribed. (Use π = 3.14)
Solution:
Area of square = 64 cm²
∴  Side of square = \(\sqrt { Area } \)  = \(\sqrt { 64 } \)  = 8 cm
∵ The square in inscribed in the circle
∴  Radius of the circle will be = \(\frac { 1 }{ 2 }\) diagonal of
the square (r)=\(\frac { 1 }{ 2 }\) x \(\sqrt { 2 } \)a = \(\frac { 1 }{ 2 }\) x \(\sqrt { 2 } \) x 8 cm = 4\(\sqrt { 2 } \)
∴ Area of the circle = πr²
= 3.14 x (4\(\frac { 1 }{ 2 }\) x \(\sqrt { 2 } \) x 8 cm = 4\(\sqrt { 2 } \))² cm²
= 3.14 x 32 = 100.48 cm²

Question 4.
A rectangular piece is 20 m long and 15 m wide. From its four corners, quadrants of radii 3.5 m have been cut. Find the area of the remaining part.
Solution:
Length of rectangular piece (l) = 20 m
and width (b) = 15m

Radius of each quadrant (r) = 3.5 m = \(\frac { 7 }{ 2 }\) m
Now area of the rectangle = l x b = 20 x 15 = 300 m²
and area of 4 quadrants = 4 x \(\frac { 1 }{ 4 }\) πr²

Question 5.
In the figure, PQRS is a square of side 4 cm. Find the area of the shaded square.


Solution:
Side of the square PQRS (a) = 4 cm
∴ Area of total square = a² = 4×4=16 cm²
Radius of each of the four quadrants at the corners = 1 cm

Question 6.
Four cows are tethered at four corners of a square plot of side 50 m, so that they just cannot reach one another. What area will be left ungrazed ? (See figure)

Solution:
side of square (a) = 50 m
∴ Area of the square field = a² = (50)² m² = 2500 m²
Radius of each quadrant (r) = \(\frac { 50 }{ 2 }\) = 25 m

Question 7.
A cow is tied with a rope of length 14 m at the corner of a rectangular field of dimensions 20 m x 16 m, find the area of the field in which the cow can graze. [NCERT Exemplar]
Solution:
Let ABCD be a rectangular field of dimensions 20 m x 16 m.
Suppose, a cow is tied at a point A.  Let length of rope AE = 14m =r (say).

Question 8.
A calf is teid with a rope of length 6 m at the corner of a square grassy lawn of side 20 m. If the length of the rope is increased by 5.5 m, find the increase in area of the grassy lawn in which the calf can graze. [NCERT Exemplar]
Solution:
Let the calf be tied at the corner A of the square lawn.

Then, the increase in area = Difference of the two sectors of central angle 90° each and radii 11.5 m (6 m + 5.5 m) and 6 m, which is the shaded region in the figure.

Question 9.
A square water tank has its side equal to 40 m. There are four semi-circular grassy plots all round it. Find the cost of turfing the plot at ?1.25 per square metre (Take π = 3.14).
Solution:
Side of square tank (a) = 40 m
Radius of each semicircular grassy plots = \(\frac { 40 }{ 2 }\) = 20

Question 10.
A rectangular park is 100 m by 50 m. It is surrounded by semi-circular flower beds all round. Find the cost of levelling the semi-circular flower beds at 60 paise per square metre. (Use π = 3.14).
Solution:
Length of rectangular park (l) = 100 m
and width (b) = 50 m

Radius of each semicircular beds along the
lengths side (R) = \(\frac { 100 }{ 2 }\)  = 50 m
and radius of each semicircular beds along
the width side (r) = \(\frac { 50 }{ 2 }\) = 25 m

Question 11.
The inside perimeter of a running track (shown in the figure) is 400 m. The length of each of the straight portion is 90 m and the ends are semi-circles. If the track is everywhere 14 m wide, find the area of the track. Also find the length of the outer running track.

Solution:

Question 12.
 Find the area of the figure, in square cm, correct to one place of decimal. (Take π = 22/7).


Solution:
Join AD
ABCD is a square whose each side = 10 cm

Area of square = a² = (10)² = 100 cm²
Area of half semicircle whose radius is \(\frac { 10 }{ 2 }\) = 5

Question 13.
In the figure, from a rectangular region ABCD with AB = 20 cm, a right triangle AED with AE = 9 cm and DE = 12 cm, is cut off. On the other end, taking BC as diameter, a semicircle is added on outside the region. Find the area of the shaded region. (Use π = 22/7). [CBSE 2014]

Solution:
AB = 20 cm, AE = 9 cm, DE = 12 cm
∠AED = 90°

Question 14.
From each of the two opposite corners of a square of side 8 cm, a quadrant of a cirlce of radius 1.4 cm is cut. Another circle of diameter 4.2 cm is also cut from the centre as shown in the figure. Find the area of the remaining (shaded) portion of the square. (Use π = 22/7). [CBSE 2010]


Solution:
Side of a square ABCD = 8 cm

Question 15.
In the figure, ABCD is a rectangle with AB = 14 cm and BC = 7 cm. Taking DC, BC and AD as diameters, three semi¬circles are drawn as shown in the figure. Find the area of the shaded region.


Solution:
In the figure, ABCD is a rectangle AB = 14 cm abd BC = 7 cm

Two semicircles are draw on AD and BC as diameter and thid semicircle is drawn on Cd as diameter
Now area of rectangle ABCD = l x b = 14 x 7 = 98 cm²
Area of two semicircles on AD and BC

Question 16.
In the figure, ABCD is a rectangle, having AB = 20 cm and BC = 14 cm. Two sectors of 180° have been cut off. Calculate :
(i) the area of the shaded region.
(ii) the length of the boundary of the shaded region.

Solution:
ABCD is a rectangle whose Length AB = 20 cm
and width BC = 14 cm
∴ Area of the rectangle = l x b = 20 x 14 = 280 cm²

Question 17.
In the figure, the square ABCD is divided into five equal parts, all having same area. The central part is circular and the lines AE, GC, BF and HD lie along the diagonals AC and BD of the square. If AB = 22 cm, find :
(i) the circumference of the central part.
(ii) the perimeter of the part ABEF.

Solution:
Side of the square ABCD = 22 cm
∴  Area = (side)² = (22)² = 484 cm²
∵ The squre is divided into 5 parts equal in area
∴ Area of each part  = \(\frac { 484 }{ 5 }\) cm²

Question 18.
In the given figure, Find the area of the shaded region. (Use π = 3.14).  [CBSE 2015]

Solution:
Side of large square = 14 cm
Radius of each semicircle = \(\frac { 4 }{ 2 }\) = 2 cm
Side of square = 4 cm
Area of square =  4 x 4=16 cm²
∴ Area of semicircles = 4 x \(\frac { 1 }{ 2 }\) πr²
= 2 x 3.14 x 2 x 2
= 8 x 3.14
= 25.12 cm²
∴ Area of shaded region = Area of large square – Area of central portion
= (14)2-(16+ 25.12) cm²
= 196-41.12 cm²
= 154.88 .cm²

Question 19.
In the Figure, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the
(i) quadrant OACB
(ii) shaded region.

Solution:
Radius of outer quadrant (R) = 3.5 cm
and radius of inner quadrant = 2 cm
∴  Area of shaded portion
= Area of outer quadrant – area of inner quadrant

Question 20.
In the figure, a square OABC is inscribed in a quadrant OPBQ of a circle. If OA = 21 cm, find the area of the shaded region.[CBSE 2013]

Solution:
In the figure, OPQ is a quadrant in which
OABC is a square OA = 21 cm
Join OB,

Question 21.
In the figure, OABC is a square of side 7 cm. If OAPC is a quadrant of a cirice with centre O, then find the area of the shaded region. (Use π = 22/7) [CBSE 2012]

Solution:
In square OABC, whose side is 7 cm, OAPC
is a quadrant
Area of square = (side)²
= (7)² = 49 cm²
and radius of quadrant = 7 cm

Question 22.
In the figure, OE = 20 cm. In sector OSFT, square OEFG is inscribed. Find the area of the shaded region.

Solution:
In the figure OSFT is a quadrant and OEFG
is a square inscribed in it
The side of the square is OE = 20 cm

Question 23.
Find the area of the shaded region in the figure, if AC = 24 cm, BC = 10 cm and O is the centre of the circle. (Use π = 3.14)

Solution:
In right AABC
AB²=AC² + BC² (Pythagoras Theorem)
= (24)²+ (10)²
= 576 + 100 = 676
= (26)²
∴ AB = 26 cm
∴ Diameter of circle = 26 cm
and radius (r)= \(\frac { 26 }{ 2 }\) = 13 cm
Now area of shaded portion
= Area of semicircle – area of right triangle ABC

Question 24.
A circle is inscribed in an equilateral triangle ABC is side 12 cm, touching its sides (see figure). Find the radius of the inscribed circle and the area of the shaded part.

Solution:
Each side of the equilateral triangle ABC (a) = 12 cm

Question 25.
In the figure, an equilateral triangle ABC of side 6 cm has been inscribed in a circle. Find the area of the shaded region. (Take π = 3.14)

Solution:

Question 26.
A circular field has a perimeter of 650 m. A square plot having its vertices on the circumference of the field is marked in the field. Calculate the area of the square plot.
Solution:
Perimeter of the circular field = 650 m

Question 27.
Find the area of a shaded region in the figure, where a circular arc of radius 7 cm has been drawn with vertex A of an equilateral triangle ABC of side 14 cm as centre, (use π = 22/7 and \(\sqrt { 3 } \)  = 1.73) [CBSE 2015]

Solution:
Radius of circular arc (r) = 7 cm
and side of equilateral AABC (a) = 14 cm and each angle = 60°

Area of shaded region
=Area of circle + Area of equilateral triangle – 2 area of sector EAF

Question 28.
A regular hexagon is inscribed in a circle. If the area of hexagon is 24\(\sqrt { 3 } \) cm², find the area of the circle. (Use π = 3.14) [CBSE 2015]
Solution:
A regular hexagon ABCDEF is inscribed in a circle
Area of hexagon = 24 \(\sqrt { 3 } \) cm²
Let r be the radius of circle
∴ Side of regular hexagon = r
Area of equilateral ΔOAB = \(\frac { \sqrt { 3 } }{ 3 }\) r² sq. units

Question 29.
ABCDEF is a regular hexagon with centre O (see figure). If the area of triangle OAB is 9 cm², find the area of :
(i) the hexagon and
(ii) the circle in which the hexagon is inscribed.

Solution:
O is the centre of the regular hexagon ABCDEF and area of the AOAB = 9 cm²
∵ By joining the vertices of the hexagon with O,
(i) We get 6 equal equilateral triangles
∴ Area of hexagon = 9 cm² x 6 = 54 cm²
(ii) Radius of the circle when this hexagon is inscribed in it will be = OB =AB  =r

Question 30.
Four equal circles, each of radius 5 cm, touch each other as shown in the figure. Find the area included between them. (Take π = 3.14).

Solution:
Radius of each circle = 5 cm
∵ The four circles touch eachother externally
∴ By joining their centres, we get a square whose side will be 5 + 5 = 10 cm

Now area of square so formed = a² = (10)² = 100 cm²
and area of 4 quadrants = 4 x \(\frac { 1 }{ 4 }\) πr²= πr²
= 3.14 x (5)2 cm² = 3.14 x 25 cm² = 78.5 cm²
∴  Area of the part included between the circles
= 100 – 78.5
= 21.5 cm²

Question 31.
Four equal circles, each of radius ‘a’ touch each other. Show that the area between them is \(\frac { 6 }{ 7 }\)a² . (Take π = \(\frac { 22 }{ 7 }\)
Solution:
Four circles each of radius ‘a’ touch each other at A, B, C and D respectively.
Their centes are P, Q, R and S respectively
By joining PQ, QR, RS, SD

Question 32.
A child makes a poster on a chart paper drawing a square ABCD of side 14 cm. She draws four circles with centre A, B, C and D in which she suggests different ways to save energy. The circles are drawn in such a way that each circle touches externally two of the three remaining circles in the given figure. In the shaded region she write a message ‘Save Energy’. Find the perimeter and area of the shaded region. (Use π = 22/7) [CBSE 2015]

Solution:
Radius of circular arc (r) = 7 cm
Side of square ABCD (a) = 14 cm

Question 33.
The diameter of a coin is 1 cm (see figure). If four such coins be placed on a table so that the rim of each touches that of the other two, find the area of the shaded region (Take π = 3.1416).


Solution:
Diameter of each coin = 1 cm
∴ Radius (r) = \(\frac { 1 }{ 2 }\) cm = 0.5 cm

Question 34.
Two circular pieces of equal radii and maximum area, touching each other are cut out from a rectangular card board of dimensions 14 cm x 7 cm. Find the area of the remaining card board. (Use π = 22/7) [CBSE 2013]
Solution:
Length of rectangle = 14 cm
and breadth = 7 cm

Question 35.
In the figure, AB and CD are two diameters of a circle perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region.

Solution:
Radius of larger circle (R) = 7 cm
and radius of smaller circle (r) =\(\frac { 7 }{ 2 }\) cm
Area of shaded portion = Area of larger circle – area of smaller circle

Question 36.
In the figure, PSR, RTQ and PAQ are three semi-circles of diameters 10 cm, 3 cm and 7 cm respectively. Find the perimeter of the shaded region. [CBSE 2014]

Solution:
Radius of larger semicircle (r₁) =\(\frac { 10 }{ 2 }\) = 5 cm
Radius of large semicircle = (r₂) = \(\frac { 7 }{ 2 }\) cm
Radius of small semicircle (r₃) = \(\frac { 3 }{ 2 }\) cm

Question 37.
In the figure, two circles with centres A and B touch each other at the point C. If AC = 8 cm and AB = 3 cm, find the area of the shaded region.

Solution:
In the figure, two circles with centres A and B touch each-other internally at C
AC = 8 cm and AB = 3 cm
∴  BC = 8 – 3 = 5 cm
∴  Radius of bigger circle (R) = 8 cm
and of smaller circle (r) = 5 cm
∴  Area of shaded portion =Area of bigger circle – area of smaller circle

Question 38.
In the figure, ABCD is a square of side 2a. Find the ratio between
(i) the circumferences
(ii) the areas of the incircle and the circum- circle of the square.

Solution:
A square ABCD is inscribed a circle
The side of the square = 2a
and one circle is inscribed in the square ABCD
Now diameter of the outer circle is AC

Question 39.
 In the figure, there are three semicircles, A, B and C having diameter 3 cm each, and another semicircle E having a circle D with diameter 4.5 cm are shown. Calculate :
(i) the area of the shaded region
(ii) the cost of painting the shaded region at the rate of 25 paise per cm², to the nearest rupee.


Solution:

Question 40.
 In the figure, ABC is a right-angled triangle, ∠B = 90°, AB = 28 cm and BC = 21 cm. With AC as diameter a semicircle is drawn and with BC as radius a quarter circle is drawn. Find the area of the shaded region correct to two decimal places.

Solution:
In right ΔABC,
AB = 28 cm, BC = 21 cm

Question 41.
In the figure, O is the centre of a circular arc and AOB is a straight line. Find the perimeter and the area of the shaded region correct to one decimal place. (Take π = 3.142)

Solution:
A semicircle is drawn on the diameter AB
ΔACB is drawn in this semicircle in right ΔACB
AC = 12 cm and BC = 16 cm

Question 42.
In the figure, the boundary of the shaded region consists of four semi-circular arcs, the smallest two being equal. If the diameter of the largest is 14 cm and of the smallest is 3.5 cm, find
(i) the length of the boundary,
(ii) the area of the shaded region.

Solution:
Diameter of the biggest semicircle = 14 cm 14
∴  Radius (R) = \(\frac { 14 }{ 2 }\) = 7 cm
Diameter of the small semicircle = 7 cm 7
∴  Radius (r₁) = \(\frac { 7 }{ 2 }\) cm
and diameter of each smaller circles = 3.5 cm

Question 43.
In the figure, AB = 36 cm and M is mid¬point of AB. Semi-circles are drawn on AB, AM and MB as diameters. A circle with centre C touches all the three circles. Find the area of the shaded region.

Solution:
In the figure, there are three semicircles one bigger and two smaller There is one small circle also
Now diameter of bigger semicircle = 36 cm
∴   Radius (R) = \(\frac { 36 }{ 2 }\) = 18 cm
and diameter of each smaller semicircle = 18 cm

Question 44.
In the figure, ABC is a right angled triangle in which ∠A = 90°, AB = 21 cm and AC = 28 cm. Semi-circles are described on AB, BC and AC as diameters. Find the area of the shaded region.

Solution:
In the figure, ABC is a right triangle in a semicircle ∠A = 90°, AB = 21 cm and AC  = 28 cm

Semicircles are drawn on BC and AC as diameters
In right ΔABC
BC² = AB²+AC² (Pythagoras Theorem)
= 21²+ 28²
= 441 + 784= 1225 = (35)²
∴  BC = 35 cm
Now radius of bigger semicircle (R) = \(\frac { 35 }{ 2 }\) cm,
of semicircle at AB = \(\frac { 21 }{ 2 }\) cm and of
semicircle on AC = \(\frac { 28 }{ 2 }\) cm = 14 cm
Now area of shaded portion
= Area of semicircle on AB as diameter + area of semicircle on AC as diameter + area of ΔABC – area of semicircle on BC as diameter

Question 45.
In the figure, shows the cross-section of railway tunnel. The radius OA of the circular part is 2 m. If ∠AOB = 90°, calculate :
(i) the height of the tunnel
(ii) the perimeter of the cross-section
(iii) the area of the cross-section.

Solution:
Radius of the circular part of the tunnel = 2 m
i.e. OA = OB = 2 m
and ∠AOB = 90°
OD ⊥ AB
∴ D in mid-point of AB
∴ In right ΔAOB,

Question 46.
In the figure, shows a kite in which BCD is the shape of a quadrant of a circle of radius 42 cm. ABCD is a square and ΔCEF is an isosceles right angled triangle whose equal sides are 6 cm long. Find the area of the shaded region.

Solution:
ABCD is a square with side = 42 cm
BCD is a quadrant in which ∠BCD = 90° and radius = 42 cm
ΔCEF is an isosceles right triangle in which CE = CF = 6 cm

Question 47.
In the figure, ABCD is a trapezium of area 24.5 cm2. In it, AD || BC, ∠DAB = 90°, AD = 10 cm and BC = 4 cm. If ABE is a quadrant of a circle, find the area of the shaded region. (Take π – (22/7). [ cbse2014]

Solution:
In the figure, ABCD is a trapezium
Area = 24.5 cm2, AD || BC
∠DAB = 90°, AD = 10 cm BC = 4 cm

Question 48.
In the figure, ABCD is a trapezium with AB || DC, AB = 18 cm, DC = 32 cm and the distance between AB and DC is 14 cm. Circles of equal radii 7 cm with centres A, B, C and D have been drawn. Then, find the area of the shaded region of the figure. (Use π  = 22/7). [CBSE 2014]

Solution:
In trapezium ABCD
AB || DC
AB = 18 cm, DC = 32 cm Height = 14 cm
Radius of each at the corner of trapezium = 7 cm

Question 49.
From a thin metallic piece, in the shape of a trapezium ABCD, in which AB || CD and ∠BCD = 90°, a quarter circle BEFC is removed (see figure). Given AB = BC = 3.5 cm and DE = 2 cm, calculate the area of the remaining piece of the metal sheet.

Solution:
ABCD is a trapezium in shape in which
AB || CD and ∠BCD = 90°
AB = BC = 3.5 cm, DE = 2 cm
∴ DC = DE + EC = DE + BC = 2 + 3.5 = 5.5 cm
Now area of trapezium ABCD = \(\frac { 1 }{ 2 }\) (AB + CD) x BC (∵ BC is height)

Question 50.
In the figure, ABC is an equilateral triangle of side 8 cm. A, B and C are the centres of circular arcs of radius 4 cm. Find the area of the shaded region correct upto 2 decimal places. (Take π = 3.142
and \(\sqrt { 3 } \)  = 1.732).

Solution:
In the figure, ABC is an equilateral triangle with 8 cm as side with centres A, B and C,
circular arcs drawn of radius 4 cm
Each side of ΔABC = 8 cm

Question 51.
Sides of a triangular field are 15 m, 16 m and 17 m. With the three corners of the field a cow, a buffalo and a horse are tied separately with ropes of length 7 m each to graze in the field. Find the area of the field which cannot be grazed by three animals. (NCERT Exemplar)
Solution:
Given that, a triangular field with the three comers of the field a cow, a buffalo and a horse are tied separately with ropes. So, each animal grazed the field in each corner of triangular field as a sectorial form.
Given, radius of each sector (r) = 7m

Question 52.
In the given figure, the side of a square is 28 cm, and radius of each circle is half of the length of the side of the square where O and O’ are centres of the circles. Find the area of shaded region. [CBSE 2017]

Solution:

Question 53.
In a hospital used water is collected in a cylindrical tank of diameter 2 m and height 5 m. After recycling, this water is used to irrigate a park to hospital whose length is 25 m and breadth is 20 m. If tank is filled completely then what will be the height of standing water used for irrigating the park? [CBSE 2017]
Solution:
Diameter of cylinder (d) = 2 m
Radius of cylinder (r) = 1 m
Height of cylinder (H) = 5 m
Volume of cylindrical tank, Vc = = πr²H = π X (1) 2 X 5= 5πm
Length of the park (L) = 25 m
Now water from the tank is used to irrigated the park. So, volume of cylindrical tank = Volume of water in the park.
⇒ 5π = 25 x 20 x h
⇒ 5π/25 x 20 = h
⇒ h = π/100 m
⇒ h = 0.0314 m
Through recycling of water, better use of the natural resource occurs without wastage. It helps in  reducing and preventing pollution.
It thus helps in conserving water. This keeps the greenery alive in urban areas like in parks gardens etc.

Hope given RD Sharma Class 10 Solutions Chapter 13 Areas Related to Circles Ex 13.4 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.