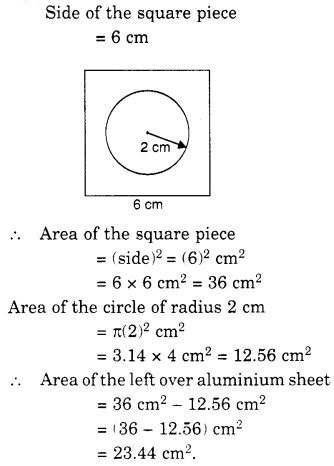
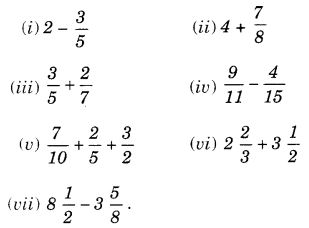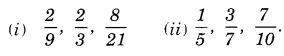NCERT Solutions for Class 7 Science Chapter 4 Heat are part of NCERT Solutions for Class 7 Science. Here we have given NCERT Solutions for Class 7 Science Chapter 4 Heat.
| Board | CBSE |
| Textbook | NCERT |
| Class | Class 7 |
| Subject | Science |
| Chapter | Chapter 4 |
| Chapter Name | Heat |
| Number of Questions Solved | 11 |
| Category | NCERT Solutions |
NCERT Solutions for Class 7 Science Chapter 4 Heat
Question 1.
State similarities and differences between the laboratory thermometer and the clinical thermometer.
Answer:
Similarities:
- Both contain mercury in bulbs.
- Both use the Celsius scale on a glass tube.
Differences:
- The temperature on the laboratory thermometer falls by itself, but not so in the clinical thermometer.
- The range of a laboratory thermometer is much higher than a clinical thermometer.
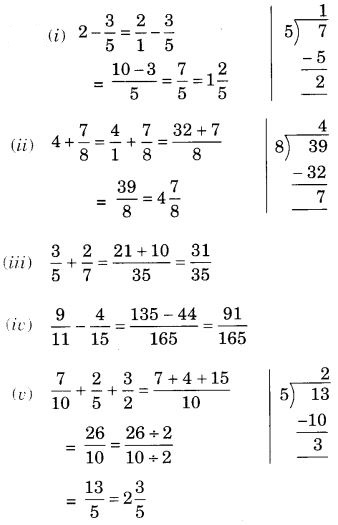
Question 2.
Give two examples each of conductors and insulators of heat.
Answer:
- Conductors of heat: Copper, iron.
- Insulators: Plastic, wood (dry).
Question 3.
Fill in the blanks:
- The hotness of an object is determined by its …….
- The temperature of boiling water cannot be measured by a ………. thermometer.
- Temperature is measured in degree …………
- No medium is required for transfer of heat by the process of ………..
- A cold steel spoon is dipped in a cup of hot milk. It transfers heat to its other end by the process of ………
- Clothes of ……….. colours absorb heat better than clothes of light colours.
Answer:
- temperature
- clinical
- Celsius
- radiation
- conduction
- dark
Question 4.
Match the following:
| (i) Land breeze blows during | (a) summer |
| (ii) Sea breeze blows during | (b) winter |
| (iii) Dark-coloured clothes are preferred during | (c) day |
| (iv) Light-coloured clothes are preferred during | (d) night |
Answer:
| (i) Land breeze blows during | (d) night |
| (ii) Sea breeze blows during | (c) day |
| (iii) Dark-coloured clothes are preferred during | (b) winter |
| (iv) Light-coloured clothes are preferred during | (a) summer |
Question 5.
Discuss why wearing more layers of clothing during winter keeps us warmer than wearing just one thick piece of clothing.
Answer:
If there are more than one layer of clothes, air would be trapped in between them. Air is a poor conductor of heat. This increases the insulation and thus comfortability of the clothes as a whole.
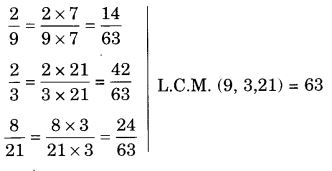
Question 6.
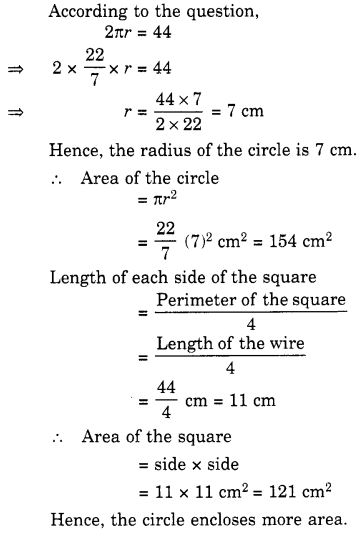
Look at Fig., mark where the heat is being transferred by conduction, by convection, and by radiation.

Fig. Heat transferred by conduction, by convection and by radiation
Answer:
In this figure, the heat is transferred at three points in different ways:
- by radiation: from the flame to the bottom of the pan.
- by conduction: from lower surface to upper surface of the pan.
- by convection: from the base to the upper region of pan water.
Question 7.
In places of the hot climate, it is advised that the outer walls of houses be painted white. Explain.
Answer:
Objects with white surfaces reflect more heat and absorb less. So, less heat from outside would enter the house.
Question 8.
One liter of water at 30°C is mixed with one liter of water at 50°C. The temperature of the mixture will be
(a) 80°C
(b) more than 50°C but less than 80°C
(c) 20°C
(d) between 30°C and 50°C
Answer:
(d) between 30°C and 50°C.
Question 9.
An iron ball at 40°C is dropped in a mug containing water at 40°C. The heat will
(a) flow from the iron ball to water.
(b) not flow from iron ball to water or from water to iron ball.
(c) flow from water to iron ball.
(d) increase the temperature of both.
Answer:
(b) not flow from iron ball to water or from water to iron ball.
Question 10.
A wooden spoon is dipped in a cup of ice cream. Its other end
(a) becomes cold by the process of conduction.
(b) becomes cold by the process of convection.
(c) becomes cold by the process of radiation.
(d) does not become cold,
Answer:
(d) does not become cold.
Question 11.
Stainless steel pans are usually provided with copper bottoms. The reason for this could be that
(a) copper bottom makes the pan more durable.
(b) such pans appear colourful.
(c) copper is a better conductor of heat than stainless steel.
(d) copper is easier to clean than stainless steel.
Answer:
(c) copper is a better conductor of heat than stainless steel.
We hope the NCERT Solutions for Class 7 Science Chapter 4 Heat help you. If you have any query regarding NCERT Solutions for Class 7 Science Chapter 4 Heat, drop a comment below and we will get back to you at the earliest.