Category: CBSE Class 9

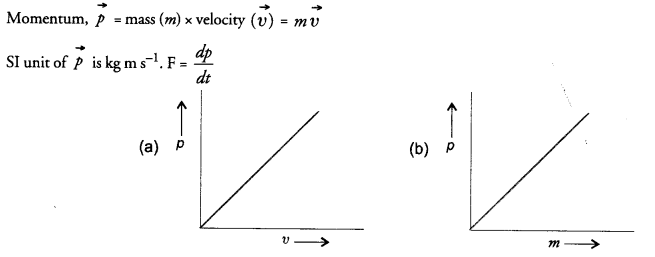
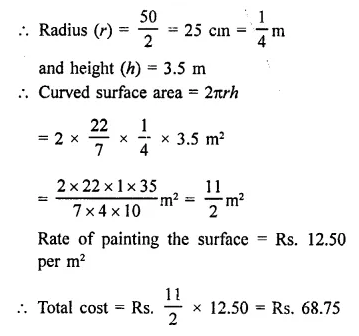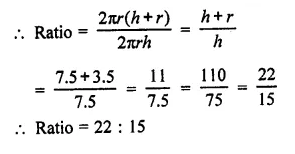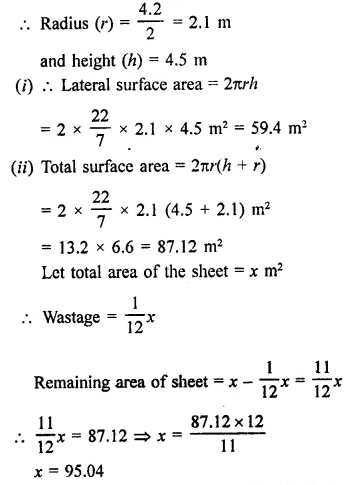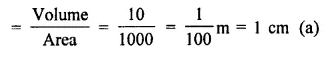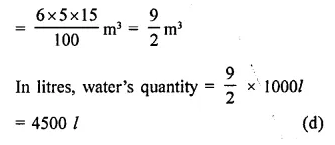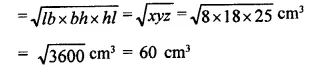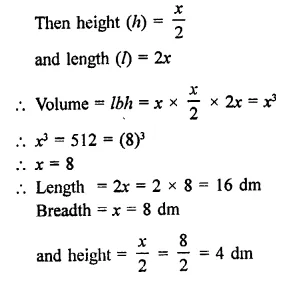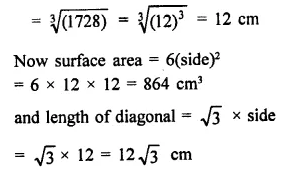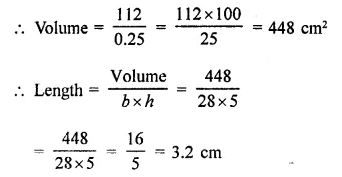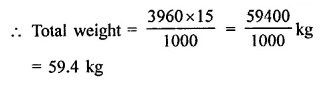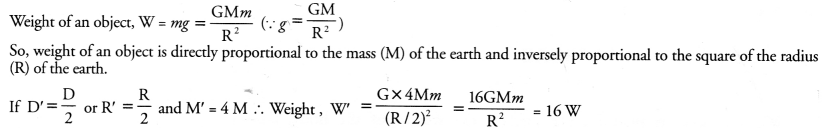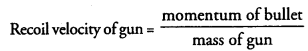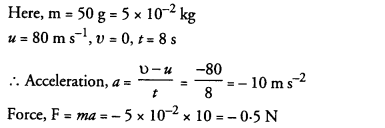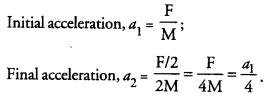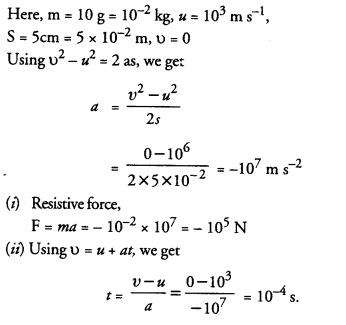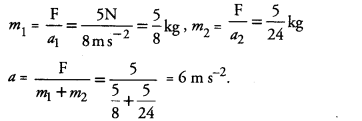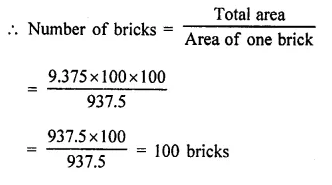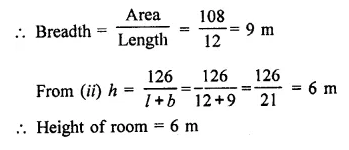RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder Ex 19.2

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder Ex 19.2

Other Exercises

• RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder Ex 19.1
• RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder Ex 19.2
• RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder VSAQS
• RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder MCQS

Question 1.
A soft drink is available in two packs – (i) a tin can with a rectangular base of length 5 cm and width 4 cm, having a height of 15 cm and
(ii) a plastic cylinder with circular base of diameter 7 cm and height 10 cm. Which container has greater capacity and by how much? [NCERT]
Solution:
In first case, in a rectangular container of soft drink, the length of base = 5 cm
and Width = 4 cm
Height = 15 cm
∴ Volume of soft drink = lbh = 5 x 4 x 15 = 300 cm³
and in second case, in a cylindrical container, diameter of base = 7 cm

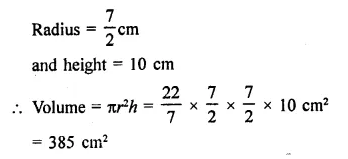
∴ The soft drink in second container is greater and how much greater = 385 cm – 380 cm² = 85 cm²

Question 2.
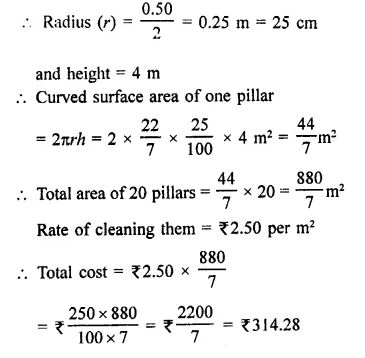
The pillars of a temple are cylindrically shaped. If each pillar has a circular base of radius 20 cm and height 10 m. How much concrete mixture would be required to build 14 such pillars? [NCERT]
Solution:
Radius of each pillar (r) = 20 cm

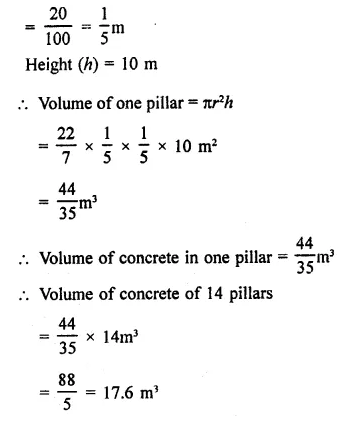
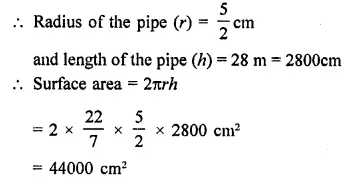
Question 3.
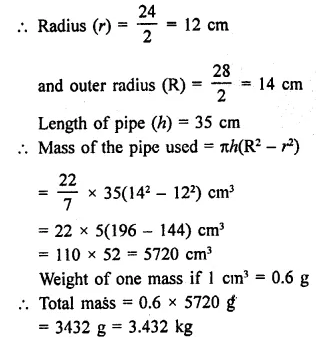
The inner diameter of a cylindrical wooden pipe is 24 cm and its outer diameter is 28 cm. The length of the pipe is 35 cm. Find the mass of the pipe, if 1 cm³ of wood has a mass of 0.6 gm. [NCERT]
Solution:
Inner diameter of a cylindrical wooden pipe = 24 cm

Question 4.
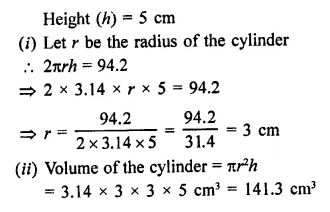
If the lateral surface of a cylinder is 94.2 cm² and its height is 5 cm, find:
(i) radius of its base
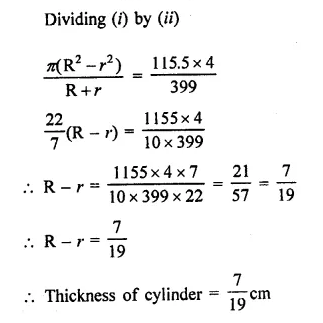
(ii) volume of the cylinder [Use π = 3.14] [NCERT]
Solution:
Lateral surface area of a cylinder = 94.2 cm2

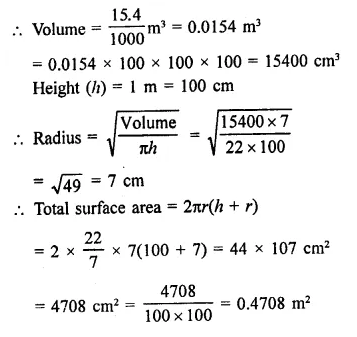
Question 5.
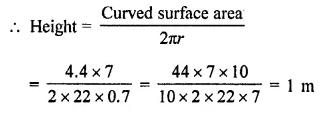
The capacity of a closed cylindrical vessel of height 1 m is 15.4 litres. How many square metres of metal sheet would be needed to make it? [NCERT]
Solution:
The capacity of a closed cylindrical vessel = 15.4 l

Question 6.
A patient in a hospital is given soup daily in a cylindrical bowl of diameter 7 cm. If the bowl is filled with soup to a height of 4 cm, how much soup the hospital has to prepare daily to serve 250 patients? [NCERT]
Solution:
Diameter of the cylindrical bowl = 7 cm
∴ Radius (r) = \(\frac { 7 }{ 2 }\)cm
Level of soup in it = 4 cm
∴ Volume of soup in one bowl for one patient

Question 7.
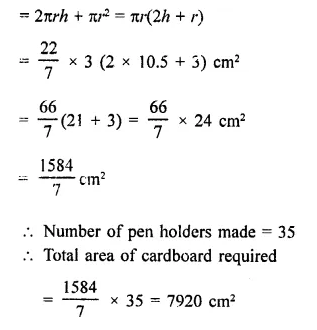
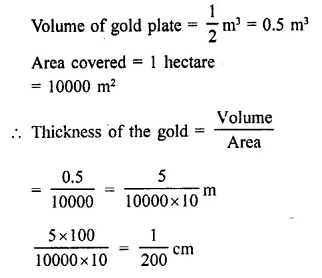
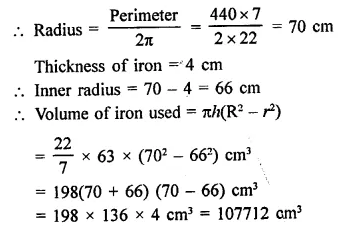
A hollow garden roller, 63 cm wide with a girth of 440 cm, is made of 4 cm thick iron. Find the volume of the iron.
Solution:
Width of hollow cylinder (A) = 63 cm
Girth = 440 cm

Question 8.
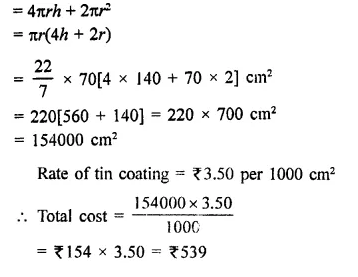
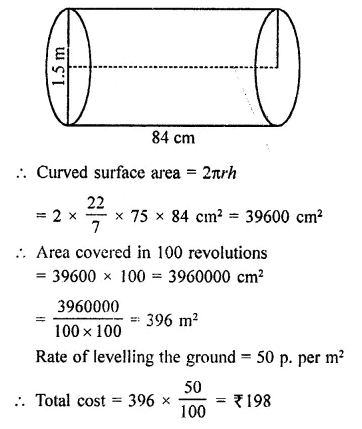
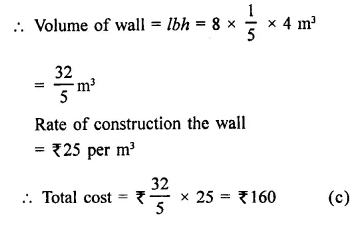
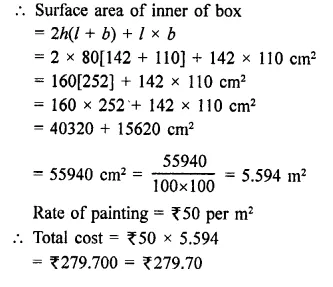
The cost of painting the total outside surface of a closed cylindrical oil tank at 50 paise per square decimetre is ₹ 198. The height of the tank is 6 times the radius of the base of the tank. Find the volume corrected to 2 decimal places.
Solution:
Rate of painting = 50 paise per dm²
Total cost = ₹198

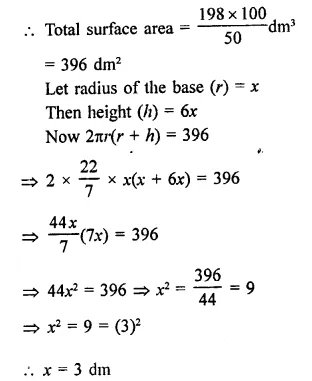
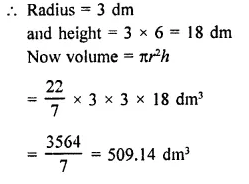
Question 9.
The radii of two cylinders are in the ratio 2 : 3 and their heights are in the ratio 5:3. Calculate the ratio of their volumes and the ratio of their curved surfaces.
Solution:
Ratio in radii of two cylinders = 2:3
and ratio in their heights = 5:3
Let radius of the first cylinder (r₁) = 2x
and radius of second cylinder (r₂) = 3x
and height of first cylinders (h₁) = 5y
and height of second cylinder (h₂) = 3y
(i) Now volume of the first cylinder = πr²h = π(2x)² x 5y = 20πx²2y
and volume of tlie second cylinder = π(3x)² x 3y = π x 9×2 x 3y = 27πx²y
Now ratio in their volume
= 20πx²y : 21πx²y = 20 : 27
(ii) Curved surface area of first cylinder = 2πrh = 2π x 2x x 5y =20πxy
and curved surface area of second cylinder = 2π x 3x x = 1 8πxy
∴ Ratio in their curved surface area
= 20πxy : 18πxy = 10 : 9

Question 10.
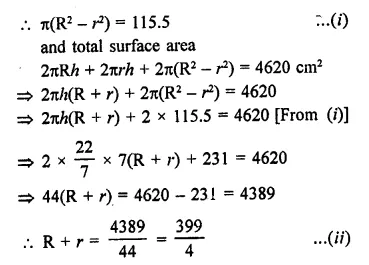
The ratio between the curved surface area and the total surface area of a right circular cylinder is 1 : 2. Find the volume of the cylinder, if its total surface area is 616 cm².
Solution:
Ratio in curved surface area and total surface area of a cylinder =1:2
Total surface area = 616 cm²

Question 11.
The curved surface area of a cylinder is 1320 cm² and its base had diameter 21 cm. Find the height and the volume of the cylinder. [Use π = 22/7]
Solution:
Curved surface area of a cylinder = 1320 cm²
Diameter of the base = 21 cm

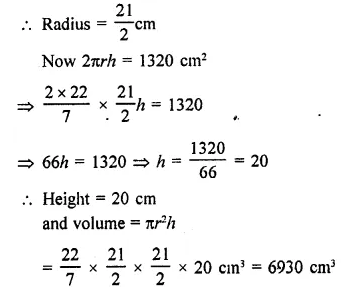
Question 12.
The ratio between the radius of the base and the height of a cylinder is 2 : 3. Find the total surface area of the cylinder, if its volume is 1617 cm³.
Solution:
Ratio between radius and height of a cylinder = 2:3
Volume =1617 cm³
Let radius (r) = 2x
Then height (h) = 3x
∴ Volume = πr²h

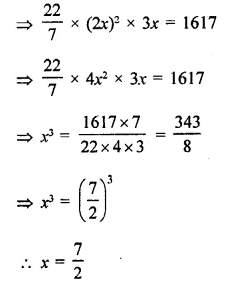
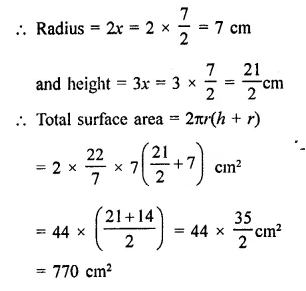
Question 13.
A rectangular sheet of paper, 44 cm x 20 cm, is rolled along its length of form a cylinder. Find the volume of the cylinder so formed.
Solution:
Length of sheet = 44 cm
Breadth = 20 cm
By rolling along length, the height of cylinder (h) = 20cm
and circumference of the base = 44cm

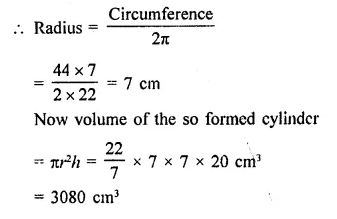
Question 14.
The curved surface area of a cylindrical pillar is 264 m2 and its volume is 924 m3. Find the diameter and the height of the pillar.
Solution:
Curved surface area of a pillar = 264 m²
and volume = 924 m³
Let r be the radius and It be height, then 2πrh = 264

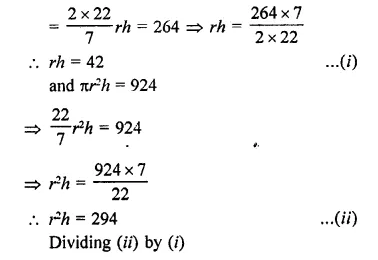
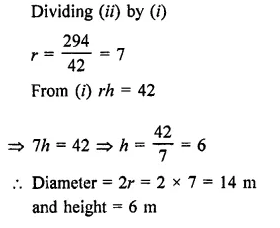
Question 15.
Two circular cylinders of equal volumes have their heights in the ratio 1 : 2. Find the ratio of their radii.
Solution:
Volumes of two cylinders are equal Ratio in their height h₁ :h₂ = 1: 2

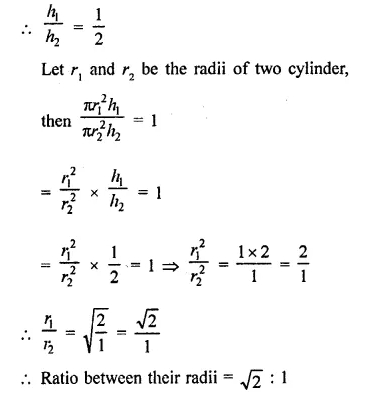
Question 16.
The height of a right circular cylinder is 10.5 m. Three times the sum of the areas of its two circular faces is twice the” area of the curved surface. Find the volume of the cylinder.
Solution:
Height of a right circular cylinder = 10.5 m
3 x sum of areas of two circular faces
= 2 x area of curved surface
Let r be that radius,

Question 17.
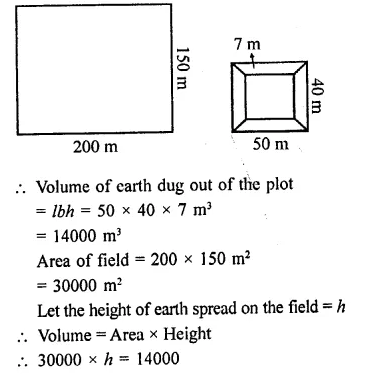
How many cubic metres of earth must be dugout to sink a well 21 m deep and 6 m diameter? Find the cost of plastering the inner surface of the well at ₹9.50 per m².
Solution:
Diameter of a well = 6 m
∴ Radius (r) = \(\frac { 6 }{ 2 }\) = 3 m
Depth (h) = 21 m
∴ Volume of earth dugout = πr²h

Question 18.
The trunk of a tree is cylindrical and its circumference is 176 cm. If the length of the trunk is 3 m. Find the volume of the timber that can be obtained from the trunk.
Solution:
Circumference of a cylindrical trunk of a tree = 176 cm

Question 19.
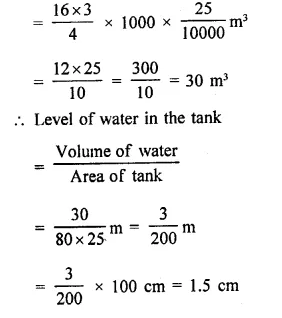
A cylindrical container with diameter of base 56 cm contains sufficient water to submerge a rectangular solid of iron with dimensions 32 cm x 22 cm x 14 cm. Find the rise in the level of the water when the solid is completely submerged.
Solution:
Diameter of cylindrical container = 56 cm
∴ Radius (r) = \(\frac { 56 }{ 2 }\) = 28 cm
Dimensions of a rectangular solid are = 32 cm x 22 cm x 14 cm
∴ Volume of solid = lbh
= 32 x 22 x 14 = 9856 cm³
∴ Volume of water in the container = 9856 cm³
Let h be the level of water, then
πr2h = 9856

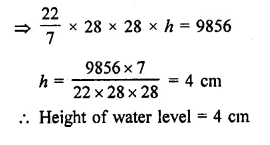
Question 20.
A cylindrical tube, open at both ends, is made of metal. The internal diameter of the tube is 10.4 cm and its length is 25 cm. The thickness of the metal is 8 mm everywhere. Calculate the volume of the metal.
Solution:
Length of metallic tube = 25 cm
Inner diameter = 10.4 cm
∴ Radius (r) = \(\frac { 10.4 }{ 2 }\) = 5.2 cm
Thickness of metal = 8 mm
∴ Outer radius (R) = 5.2 + 0.8 = 6.0 cm
Volume of metal used = π(R² – r²) x h

Question 21.
From a tap of inner radius 0.75 cm, water flows at the rate of 7 m per second. Find the volume in litres of water delivered by the pipe in one hour.
Solution:
Inner radius of a tap = 0.75 cm
Speed of flow of water in it = 7 m/s
Time = 1 hour
∴ Length of flow of water (h)
= 7 x 60 x 60 m = 25200 m
∴ Volume of water = πr²h

Question 22.
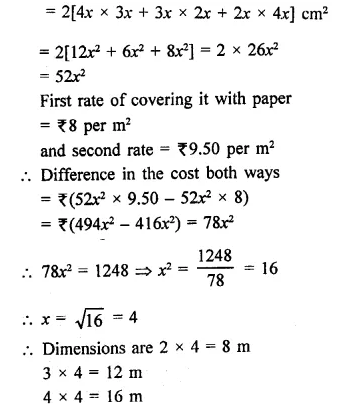
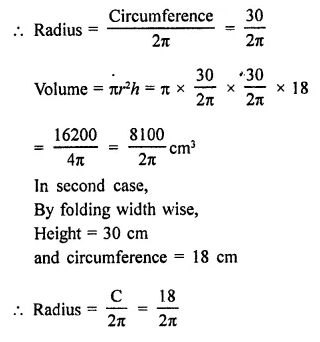
A rectangular sheet of paper 30 cm x 18 cm can be transformed into the curved surface of a right circular cylinder in two ways i.e., either by rolling the paper along its length or by rolling it along its breadth. Find the ratio of the volumes of the two cylinders thus formed.
Solution:
Size of rectangular sheet = 30 cm x 18 cm
∴ Length of sheet = 30 cm
and breadth = 18 cm
By folding length wise,
Height = 18 cm
and circumference = 30 cm

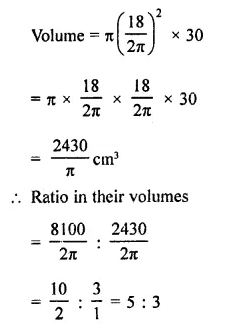
Question 23.
How many litres of water flow out of a pipe having an area of cross-section of 5 cm² in one minute, if the speed of water in the pipe is 30 cm/sec?
Solution:
Area of the cross-section of the pipe = 5 cm²
Speed of water flow = 30 cm/sec
Period = 1 minute
∴ Flow of water in 1 minute = 30 x 60 cm = 1800 cm
Area of mouth of pipe = 5 cm²
∴ Volume = 1800 x 5 = 9000 cm³
Volume of water in litres = 9000 ml

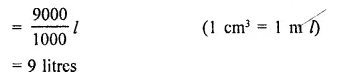
Question 24.
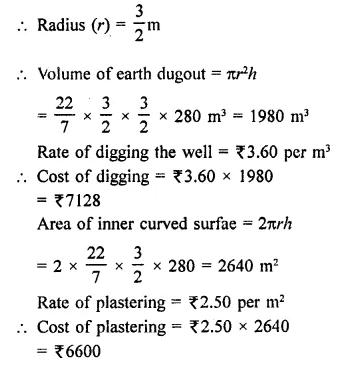
Find the cost of sinking a tubewell 280 m deep, having diameter 3 m at the rate of ₹3.60 per cubic metre. Find also the cost of cementing its inner curved surface at ₹2.50 per square metre.
Solution:
Depth of well (h) = 280 m
Diameter = 3 m

Question 25.
Find the length of 13.2 kg of copper wire of diameter 4 mm, when 1 cubic cm of copper weighs 8.4 gm.
Solution:
Weights of copper wire = 13.2 kg
Diamter = 4 mm

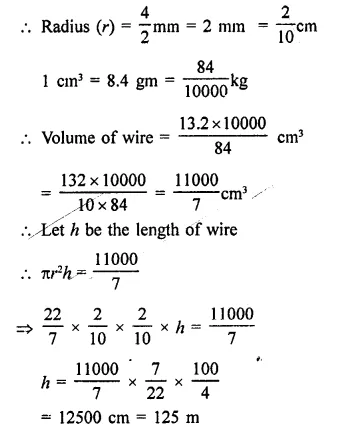
Question 26.
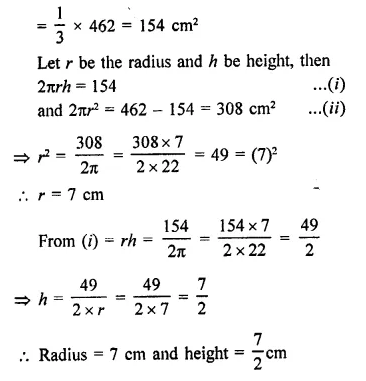
A solid cylinder has a total surface area of 231 cm². Its curved surface area is \(\frac { 2 }{ 3 }\) of the total surface area. Find the volume of the cylinder.
Solution:
Surface area of solid cylinder = 231 cm²
and curved surface area = \(\frac { 2 }{ 3 }\) of 231 cm²

Question 27.
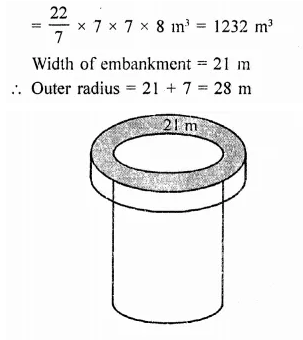
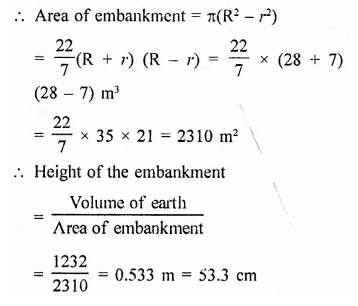
A well with 14 m diameter is dug 8 m deep. The earth taken out of it has been evenly spread all around it to a width of 21 m to form an embankment. Find the height of the embankment.
Solution:
Diameter of a well = 14 m
∴ Radius (r) = y = 7 m
Depth (h) = 8 m
∴ Volume of the earth dugout = πr²h

Question 28.
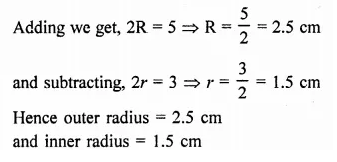
The difference between inside and outside surfaces of a cylindrical tube 14 cm long is 88 sq. cm. If the volume of the tube is 176 cubic cm, find the inner and outer radii of the tube.
Solution:
Length of cylindrical tube = 14 cm
Difference betveen the outer surface and inner surface = 88 cm²
and volume of the tube = 176 cm³
Let R and r be the outer and inner radius of the tube

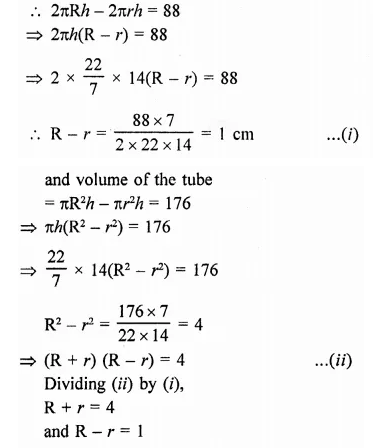
Question 29.
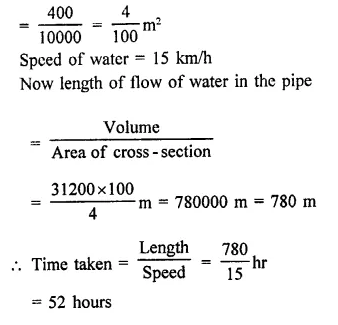
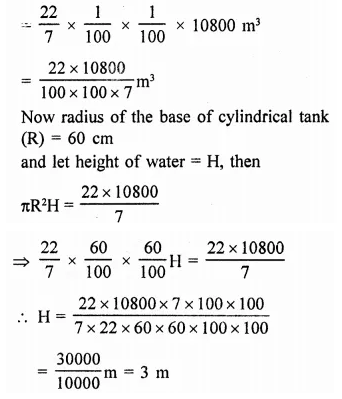
Water flows out through a circular pipe whose internal diameter is 2 cm, at the rate of 6 metres per second into a cylindrical tank. The radius of whose base is 60 cm. Find the rise in the level of water in 30 minutes?
Solution:
Internal diameter of the pipe = 2 cm
∴ Radius (r) = \(\frac { 2 }{ 2 }\) = 1 cm
Speed of water flow = 6m per second Water in 30 minutes (h) = 6 x 60 x 30 m = 10800 m
Volume of water = πr²h

Question 30.
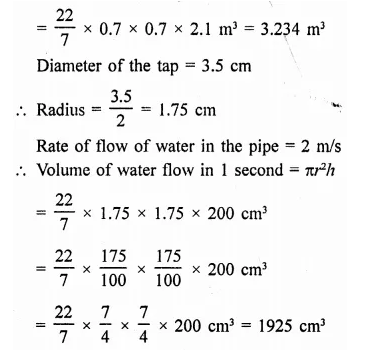
A cylindrical water tank of diameter 1.4 m and height 2.1 m is being fed by a pipe of diameter 3.5 cm through which water flows at the rate of 2 metre per second. In how much time the tank will be filled?
Solution:
Diameter of cylindrical tank = 1.4 m
∴ Radius (r) = \(\frac { 1.4 }{ 2 }\) = 0.7 m
and height (h) = 2.1 m
∴ Volume of water in the tank = πr²h

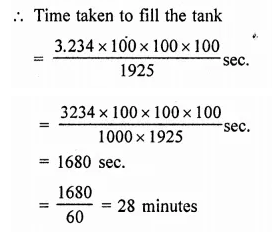
Question 31.
The sum of the radius of the base and height of a solid cylinder is 37 m. If the total surface area of the solid cylinder is 1628 m². Find the volume of the cylinder.
Solution:
Sum of radius and height of a cylinder = 37 m
Let r be the radius and h be the height, then r + h = 37m …(i)
Total surface area of a solid cylinder = 1628m³

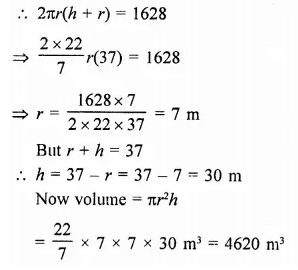
Question 32.
A well with 10 m inside diameter is dug 8.4 m deep. Earth taken out of it is spread all around it to a width of 7.5 m to form an embankment. Find the height of the embankment.
Solution:
Diameter of the well = 10 m 10
∴ Radius (r) = \(\frac { 10 }{ 2 }\) = 5 m
Depth (h) = 8.4 m
∴ Volume of earth dugout = πr²h

Hope given RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder Ex 19.2 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder Ex 19.1

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder Ex 19.1

Other Exercises

• RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder Ex 19.1
• RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder Ex 19.2
• RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder VSAQS
• RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder MCQS

Question 1.
Curved surface area of a right circular cylinder is 4.4 m2. If the radius of the base of the cylinder is 0.7 m, find its height. [NCERT]
Solution:
Curved surface area of a cylinder = 4.4 m²
Radius (r) = 0.7 m

Question 2.
In a hot water heating system, there is a cylindrical pipe of length 28 m and diameter 5 cm. Find the total radiating surface in the system. [NCERT]
Solution:
Diameter of the pipe = 5 cm

Question 3.
A cylindrical pillar is 50 cm in diameter and 3.5 m in height. Find the cost of painting the curved surface of the pillar at the rate of 12.50 per m². [NCERT]
Solution:
Diameter of cylindrical pillar = 50 cm

Question 4.
It is required to make a closed cylindrical tank of height 1 m and base diameter 140 cm from a metal sheet. How many square metres of the sheet are required for the same? [NCERT]
Solution:
Height of cylinder (h) = 1 m = 100 cm
Diameter of box = 140 cm

Question 5.
The total surface area of a hollow cylinder which is open from both sides is 4620 sq. cm, area of base ring is 115.5 sq. cm and height 7 cm. Find the thickness of the cylinder.
Solution:
Total surface area of a hollow cylinder open from both sides = 4620 cm²
Area of base of ring = 115.5 cm²
Height (h) = 7 cm
Let outer radius (R) = R
and inner radius = r

Question 6.
Find the ratio between the total surface area of a cylinder to its curved surface area, given that its height and radius are 7.5 cm and 3.5 cm.
Solution:
Radius of the cylinder (r) = 3.5 cm
and height (h) = 7.5 cm
Total surface area = 2πr (h + r)
and curved surface area = 2πrh

Question 7.
A cylindrical vessel, without lid, has to be tin-coated on its both sides. If the radius of the base is 70 cm and its height is 1.4 m, calculate the cost of tin-coating at the rate of ₹3.50 per 1000 cm².
Solution:
Radius of the base of a cylindrical vessel (r) = 70 cm
and height (h) = 1.4 m = 140 cm
Total surface area (excluding upper lid) on both sides = 2πrh x 2 + πr² x 2

Question 8.
The inner diameter of a circular well is 3.5 m. It is 10 m deep. Find:
(i) inner curved surface area.
(ii) the cost of plastering this curved surface at the rate of ₹40 per m². [NCERT]
Solution:
Inner diameter of a well = 3.5 m

Question 9.
The students of a Vidyalaya were asked to participate s a competition for making and decorating pen holders in the shape of a cylinder with a base, using cardboard. Each pen holder was to be of radius 3 cm and height 10.5 cm. The Vidyalaya was to supply the competitors with cardboard. If there were 35 competitors, how much cardboard was required to be bought for the competition? [NCERT]
Solution:
Radius of cylinderical pen holder (r) = 3 cm
Height (h) = 10.5 cm
∴ Surface area of the pen holder

Question 10.
The diameter of roller 1.5 m long is 84 cm. If it takes 100 revolutions to level a play¬ground, find the cost of levelling this ground at the rate of 50 paise per square metre.
Solution:
Diameter of a roller = 1.5 m
∴ Radius = \(\frac { 1.5 }{ 2 }\) = 0.75 m = 75 cm
and length (h) = 84 cm

Question 11.
Twenty cylindrical pillars of the Parliament House are to be cleaned. If the diameter of each pillar is 0.50 m and height is 4 m. What will be the cost of cleaning them at the rate of ₹2.50 per square metre? [NCERT]
Solution:
Number of pillars = 20
Diameter of one pillar = 0.50 m

Question 12.
A solid cylinder has total surface area of 462 cm². Its curved surface area is one- third of its total surface area. Find the radius and height of the cylinder.
Solution:
Total surface of solid cylinder = 462 cm²
Curved surface area = \(\frac { 1 }{ 3 }\) of total surface area

Question 13.
The total surface area of a hollow metal cylinder, open at both ends of external radius 8 cm and height 10 cm is 338 π cm². Taking r to be inner radius, obtain an equation in r and use it to obtain the thickness of the metal in the cylinder.
Solution:
Total surface area of a hollow metal cylinder = 338π cm²
Let R be the outer radius, r be inner radius and h be the height of the cylinder of the cylinder
∴ 2πRh + 2πrh + 2πR² – 2πr² = 338π
R = 8 cm, h = 10 cm
⇒ 2πh (R + r) + 2π(R² – r²) = 338π
⇒ Dividing by 2π , we get
⇒ h(R + r) + (R² – r²) = 169
⇒ 10(8 + r) + (8 + r) (8 – r) = 169
⇒ 80 + 10r + 64 – r² = 169
⇒ 10r – r² + 144 – 169 = 0
⇒ r² – 10r + 25 = 0
⇒ (r-5)² = 0
⇒ r = 5
∴ Thickness of the metal = R – r = 8 – 5 = 3 cm

Question 14.
Find the lateral curved surface area of a cylinderical petrol storage tank that is 4.2m in diameter and 4.5 m high. How much steel was actually used, if \(\frac { 1 }{ 12 }\) of steel actually used was wasted in making the closed tank? [NCERT]
Solution:
Diameter of a cylinderical tank = 4.2 m

Hope given RD Sharma Class 9 Solutions Chapter 19 Surface Areas and Volume of a Circular Cylinder Ex 19.1 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube MCQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube MCQS

Other Exercises

• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.1
• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.2
• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube VSAQS
• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube MCQS

Mark correct alternative in each of the following:
Question 1.
The length of the longest rod that can be fitted in a cubical vessel of edge 10 cm long, is
(a) 10 cm
(b) 10\(\sqrt { 2 } \) cm
(c) 10\(\sqrt { 3 } \) cm
(d) 20 cm
Solution:
Edge of cuboid (a) = 10 cm
∴ Longest edge = \(\sqrt { 3 } \) a cm
= \(\sqrt { 3 } \) x 10 = 10\(\sqrt { 3 } \) cm (c)

Question 2.
Three equal cubes are placed adjacently in a row. The ratio of the total surface area of the resulting cuboid to that of the sum of the surface areas of three cubes, is
(a) 7 : 9
(b) 49 : 81
(c) 9 : 7
(d) 27 : 23
Solution:
Let a be the side of three equal cubes
∴ Surface area of 3 cubes
= 3 x 6a² = 18a²
and length of so formed cuboid = 3a
Breadth = a
and height = a
∴ Surface area = 2(lb + bh + hl)
= 2[3a x a + a x a+a x 3a] = 2[3a² + a² + 3a²] = 2 x 7a² = 14a²
∴ Ratio in the surface areas of cuboid and three cubes = 14a² : 18a²= 7:9 (a)

Question 3.
If the length of a diagonal of a cube is 8 \(\sqrt { 3 } \) cm, then its surface area is
(a) 512 cm²
(b) 384 cm²
(c) 192 cm²
(d) 768 cm²
Solution:
Length of the diagonal of cube = 8 \(\sqrt { 3 } \) cm

Question 4.
If the volumes of two cubes are in the ratio 8:1, then the ratio of their edges is
(a) 8 : 1
(b) 2\(\sqrt { 2 } \) : 1
(c) 2 : 1
(d) none of these
Solution:
Let volume of first cube = 8x³
and of second cube = x³

Question 5.
The volume of a cube whose surface area is 96 cm², is
(a) 16\(\sqrt { 2 } \) cm³
(b) 32 cm³
(c) 64 cm³
(d) 216 cm³
Solution:
Surface area of a cube = 96 cm²

Question 6.
The length, width and height of a rectangular solid are in the ratio of 3 : 2 : 1. If the volume of the box is 48 cm³, the total surface area of the box is
(a) 27 cm²
(b) 32 cm²
(c) 44 cm²
(d) 88 cm²
Solution:
Ratio in the dimensions of a cuboid =3 : 2 : 1
Let length = 3x
Breadth = 2x
and height = x
Then volume = lbh = 3x x 2x x x = 6×3
∴ 6x³ = 48 ⇒ x³= \(\frac { 48 }{ 6 }\) = 8 = (2)³
∴ x = 2
∴ Length (l) = 3 x 2 = 6 cm
Breadth (b) = 2 x 2 = 4 cm
Height (h) = 1 x 2 = 2 cm
Now surface area = 2[lb + bh + hl]
= 2[6 x 4 + 4 x 2 + 2 x 6] cm²
= 2[24 + 8-+ 12] = 2 x 44 cm²
= 88 cm² (d)

Question 7.
If the areas of the adjacent faces of a rectangular block are in the ratio 2:3:4 and its volume is 9000 cm3, then the length of the shortest edge is
(a) 30 cm
(b) 20 cm
(c) 15 cm
(d) 10 cm
Solution:
Ratio in the areas of three adjacent faces of a cuboid = 2 : 3 : 4
Volume = 9000 cm³
Let the area of faces be 2x, 3x, Ax and
Let a, b, and c be the dimensions of the cuboid, then
∴ 2x = ab, 3x = be, 4x = ca
∴ ab x be x ca = 2x x 3x x 4x
a²b²c² = 24 x 3
But volume = abc = 9000 cm³

Question 8.
If each edge of a cube, of volume V, is doubled, then the volume of the new cube is
(a) 2V
(b) 4V
(c) 6V
(d) 8V
Solution:
Let a be the edge of a cube whose Volume = V
∴ a3 = V
By doubling the edge, we get 2a
Then volume = (2a)3 = 8a³
∴ Volume of new cube = 8a³ = 8V (d)

Question 9.
If each edge of a cuboid of surface area S is doubled, then surface area of the new cuboid is
(a) 2S
(b) 4S
(c) 6S
(d) 8S
Solution:
Let each edge of a cube = a
Then surface area = 6a²
∴ S = 6a²
Now doubling the edge, we get
New edge of a new cube = 2a
∴ Surface area = 6(2a)²
= 6 x 4a² = 24a²
= 4 x 6a² = 4S (b)

Question 10.
The area of the floor of a room is 15 m2. If its height is 4 m, then the volume of the air contained in the room is
(a) 60 dm³
(b) 600 dm³
(c) 6000 dm³
(d) 60000 dm³
Solution:
Area of a floor of a room = 15 m²
Height (h) = 4 m
∴ Volume of air in the room = Floor area x Height
= 15 m² x 4 m = 60 m³
= 60 x 10 x 10 x 10 dm² = 60000 dm² (d)

Question 11.
The cost of constructing a wall 8 m long, 4 m high and 20 cm thick at the rate of ₹25 per m³ is
(a) ₹16
(b) ₹80
(c) ₹160
(d) ₹320
Solution:
Length of wall (l) = 8 m
Breadth (b) = 20 cm = \(\frac { 1 }{ 5 }\) m
Height (h) = 4 m

Question 12.
10 cubic metres clay in uniformaly spread on a land of area 10 acres. The rise in the level of the ground is
(a) 1 cm
(b) 10 cm
(c) 100 cm
(d) 1000 cm
Solution:
Volume of clay = 10 m³
Area of land = 10 acres
= 10 x 100 = 1000 m²
∴ Rise of level by spreading the clay

Question 13.
Volume of a cuboid is 12 cm³. The volume (in cm³) of a cuboid whose sides are double of the above cuboid is
(a) 24
(b) 48
(c) 72
(d) 96
Solution:
Volume of cuboid = 12 cm³
By doubling the sides of the cuboid the
volume will be = 12 cm³ x 2 x 2 x 2
= 96 cm³ (d)

Question 14.
If the sum of all the edges of a cube is 36 cm, then the volume (in cm3) of that cube is
(a) 9
(b) 27
(c) 219
(d) 729
Solution:
Sum of all edges of a cube = 36 cm
No. of edge of a cube are 12
∴ Length of its one edge = \(\frac { 36 }{ 12 }\) = 3 cm
Then volume = (edge)³ = (3)³ cm³
= 27 cm³ (b)

Question 15.
The number of cubes of side 3 cm that can be cut from a cuboid of dimensions 9 cm x 9 cm x 6 cm, is
(a) 9
(b) 10
(c) 18
(d) 20
Solution:
Dimensions of a cuboid = 9 cm x 9 cm x 6 cm

Question 16.
On a particular day, the rain fall recorded in a terrace 6 m long and 5 m broad is 15 cm. The quantity of water collected in the terrace is
(a) 300 litres
(b) 450 litres
(c) 3000 litres
(d) 4500 litres
Solution:
Dimension of a terrace = 6mx5m
Level of rain on it = 15 cm
∴ Volume of water collected on it

Question 17.
If A₁, A₂ and A₃ denote the areas of three adjacent faces of a cuboid, then its volume is

Solution:
Let l, b, h be the dimensions of the cuboid
∴ A₁= lb, A₂ = bh, A₃ = hl
∴ A₁ A₂ A₃ = lb.bh.hl = l₂b₂h₂

Question 18.
If l is the length of a diagonal of a cube of volume V, then

Solution:
Volume of a cube = V
and longest diagonal = l

Question 19.
If V is the volume of a cuboid of dimensions x, y, z and A is its surface area, then \(\frac { A }{ V }\)

Solution:
A is surface area, V is volume and x, y and z are the dimensions
Then V = xyz
A = 2[xy + yz + zx]

Question 20.
The sum of the length, breadth and depth of a cuboid is 19 cm and its diagonal is 5\(\sqrt { 5 } \) cm. Its surface area is
(a) 361 cm²
(b) 125 cm²
(c) 236 cm²
(d) 486 cm²
Solution:
Let x, y, z be the dimensions of a cuboid,
then x + y + z = 19 cm

Question 21.
If each edge of a cube is increased by 50%, the percentage increase in its surface area is
(a) 50%
(b) 75%
(c) 100%
(d) 125%
Solution:
Let in first case, edge of a cube = a
Then surface area = 6a²
In second case, increase in side = 50%

Question 22.
A cube whose volume is 1/8 cubic centimeter is placed on top of a cube whose volume is. 1 cm³. The two ,cubes are then placed on top of a third cube whose volume is 8 cm³. The height of the stacked cubes is
(a) 3.5 cm
(b) 3 cm
(c) 7 cm
(d) none of these
Solution:
Volume of first cube = \(\frac { 1 }{ 2 }\) cm³

Hope given RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube MCQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube VSAQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube VSAQS

Other Exercises

• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.1
• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.2
• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube VSAQS
• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube MCQS

Question 1.
If two cubes of side 6 cm are joined face to face, then find the volume of the resulting cuboid.
Solution:
Side of a cube = 6 cm
∴ By joining two such cubes, the length of so
formed cuboid (l) = 6 x 2 = 12 cm
Breadth (b) = 6 cm
Height (h) = 6 cm
∴ Volume = lbh = 12 x 6 x 6 cm³
= 432 cm³

Question 2.
Three cubes of metal whose edges are in the ratio 3 : 4 : 5, are melted down into a single cube whose diagonals is 12 \(\sqrt { 3 } \) cm. Find the edges of three cubes.
Solution:
Ratio in the sides of three cubes = 3 : 4 : 5
Let side of first cube = 3x
and side of second cube = 4x
and side of third cube = 5x
∴ Sum of volume of three cubes
= (3x)³ + (4x)³ + (5x)³
= 27x³ + 64x³ + 125x³ = 216x³
∴ Volume of the cube formed Jby melting these three cubes = 216x³

∴ Side of first cube = 3x = 3 x 2 = 6 cm
Side of second cube = 4x = 4×2 = 8 cm
and side of third cube = 5.r = 5 x 2 = 10 cm

Question 3.
If the perimeter of each face of a cube is 32 cm, find its lateral surface area. Note that four faces which meet the base of a cube are called its lateral faces.
Solution:
Perimeter of each face of a cube = 32 cm
∴ Length of edge = \(\frac { 32 }{ 4 }\) = 8 cm
and lateral surface area of the cube = 4 x (side)²
= 4 x 8 x 8 = 256 cm²

Question 4.
Find the edge of a cube whose surface area is 432 m².
Solution:

Question 5.
A cuboid has total surface area of 372 cm² and its lateral surface area is 180 cm², find the area of its base.
Solution:
Total surface area of a cuboid = 372 cm²
and lateral surface area = 180 cm²
∴ Area of base and roof = 372 – 180 = 192 cm²
and area of base = \(\frac { 192 }{ 2 }\) = 96 cm²

Question 6.
Three cubes of each side 4 cm are joined end to end. Find the surface area of the resulting cuboid.
Solution:
By joining three cubes of side 4 cm each, end is end, we get a cuboid
Length of cuboid = 4 x 3 = 12 cm
Breadth = 4 cm
and height = 4 cm

∴ Surface area = 2(lb + bh + hl)
= 2[12 x4+4×4 + 4x 12] cm²
= 2[48 + 16 + 48] cm²
= 2 x 112 = 224 cm²

Question 7.
The surface area of a cuboid is 1300 cm2. If its breadth is 10 cm and height is 20 cm, find its length.
Solution:
Surface area of a cuboid = 1300 cm²
Breadth (b) = 10 cm
and height (h) = 20 cm
Let l be the length, then
= 2 (lb + bh + hl) = 1300
lb+ bh + hl = \(\frac { 1300 }{ 2 }\) = 650
l x 10 + 10 x 20 + 20 x l = 650
10l + 20l + 200 = 650
⇒ 30l = 650 – 200 = 450
⇒ l = \(\frac { 450 }{ 30 }\) = 15
∴ Length of cuboid = 15 cm

Hope given RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube VSAQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.2

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.2

Other Exercises

• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.1
• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.2
• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube VSAQS
• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube MCQS

Question 1.
A cuboidal water tank is 6 m long, 5 m wide and 4.5 m deep. How many litres of water can it hold? [NCERT]
Solution:
Length of water tank (l) = 6 m
Breadth (b) = 5 m
and depth (h) = 4.5 m

∴ Volume of water in it = lbh
= 6 x 5 x 4.5 m³ = 135 m³
Capacity of water in litres = 135 x 1000 litres (1 m³ = 1000 l)
= 135000 litres

Question 2.
A cubical vessel is 10 m long and 8 m wide. How high must it be made to hold 380 cubic metres of a liquid? [NCERT]
Solution:
Length of vessal (l) = 10 m
Breadth (b) = 8 m
Volume = 380 m³

Question 3.
Find the cost of digging a cuboidal pit 8 m long, 6 m broad and 3 m deep at the rate of ₹30 per m³. [NCERT]
Solution:
Length of pit (l) = 8m
Width (b) = 6 m
and depth (h) = 3 m
∴ Volume of earth digout = lbh
= 8 x 6 x 3 = 144 m³
Cost of digging the pit at the rate of ₹30 per m³
= 144 x 30 = ₹4320

Question 4.
If the areas of three adjacent faces of a cuboid are 8 cm², 18 cm² and 25 cm². Find the volume of the cuboid.
Solution:
Let x, y, z be the three adjacent faces of the cuboid, then
x = 8 cm², y = 18 cm², z = 25 cm²
and let l, b, h are the dimensions of the cuboid, then
x = lb = 8 cm²
y = bh = 18 cm²
z = hl = 25 cm²
∴ Volume = lbh

Question 5.
The breadth of a room is twice its height, one half of its length and the volume of the room is 512 cu. dm. Find its dimensions.
Solution:
Let breadth of a room (b) = x

Question 6.
Three metal cubes with edges 6 cm, 8 cm and 10 cm respectively are melted together and formed into a single cube. Find the volume, surface area and diagonal of the new cube.
Solution:
Edge of first cube = 6 cm
Edge of second cube = 8 cm
and edge of third cube = 10 cm
∴ Volume of 3 cubes = (6)³ + (8)³ + (10)3 cm³
= 216 + 512 + 1000 cm³
= 1728 cm³
∴ Edge of so formed cube

Question 7.
Two cubes, each of volume 512 cm³ are joined end to end. Find the surface area of the resulting cuboid.
Solution:
Volume of each volume = 512 cm³
∴ Side (edge) = \(\sqrt [ 3 ]{ 512 }\)
=\(\sqrt [ 3 ]{ { 8 }^{ 3 } }\)  = 8 cm
Now by joining two cubes, then Length of so formed cuboid (l)
= 8 + 8 = 16 cm
Breadth (b) = 8 cm
and height (h) = 8 cm
∴ Surface area = 2(lb + bh + hl)
= 2[16 x 8 + 8 x 8 + 8 x 16] cm²
= 2[128 + 64 + 128] cm²
= 2 x 320 = 640 cm²

Question 8.
A metal cube of edge 12 cm is melted and formed into three smaller cubes. If the edges of the two smaller cubes are 6 cm and 8 cm, find the edge of the third smaller cube.
Solution:
Edge of metal cube = 12 cm
∴ Its volume = (Edge)³ = (12)³ cm3³
= 1728 cm³
It is melted and form 3 cubes
Edge of one smaller cube = 6 cm
and edge of second smaller cube = 8 cm
∴ Volume of two smaller cubes = (6)³ + (8)³ cm³
= 216 + 512 cm³ = 728 cm³
∴ Volume of third smaller cube = 1728 – 728 = 1000 cm³
∴ Edge of the third cube = \(\sqrt [ 3 ]{ 1000 }\)
= \(\sqrt [ 3 ]{ (10)3 }\)  cm = 10 cm

Question 9.
The dimensions of a cinema hall are 100 m, 50 m and 18 m. How many persons can sit in the hall, if each person requires 150 m3 of air?
Solution:
Length of cinema hall (l) = 100 m
Breadth (b) = 50 m
and height (h) = 18 m
∴ Volume of air in it = lbh
= 100 x 50 x 18 m³ = 90000 m³
Air required for one person = 150 m³
∴ Number of persons in the hall = \(\frac { 90000 }{ 150 }\) = 600 persons

Question 10.
Given that 1 cubic cm of marble weighs 0.25 kg, the weight of marble block 28 cm in width and 5 cm thick is 112 kg. Find the length of the block.
Solution:
Weight of 1 cm3 = 0.25 kg
Breadth of the block (b) = 28 cm
Thickness (h) = 5 cm
and total weight of the block = 112 kg

Question 11.
A box with lid is made of 2 cm thick wood. Its external length, breadth and height are 25 cm, 18 cm and 15 cm respectively. How much cubic cm of a liquid can be placed in it? Also, find the volume of the wood used in it.
Solution:
Outer length of the closed wooden box (l) = 25 cm
Breadth (b) = 18 cm
and height (h) = 15 cm
Width of wood = 2 cm

∴ Inner length = 25 – 2×2 = 25- 4 = 21cm
Breadth =18- 2×2 = 18-4 = 14 cm
and height =15- 2×2 = 15- 4=11 cm
Now outer volume = 25 x 18 x 15 cm³ = 6750 cm³
and inner volume = 21 x 14 x 11 cm³ = 3234 cm³
(i) Inner volume = 3234 cm³
(ii) Volume of wood = 6750 – 3234 = 3516 cm³

Question 12.
The external dimensions of a closed wooden box are 48 cm, 36 cm, 30 cm. The box is made of 1.5 cm thick wood. How many bricks of size 6 cm x 3 cm x 0.75 cm can be put in this box?
Solution:
External length of a closed wooden box (L) = 48 cm
Width (B) = 36 cm
and height (H) = 30 cm
Thickness of wood = 1.5 cm

∴ Internal length (l) = 48 – 2 x 1.5 cm = 48 – 3 = 45 cm
Width (b) = 36 – 2 x 1.5 cm
= 36 – 3 = 33 cm
and height (h) = 30 – 2 x 1.5 cm
= 30 – 3 = 27 cm
Now volume of internal box
= lbh = 45 x 33 x 27 cm³
Volume of one bricks = 6 x 3 x 0.75 cm³

Question 13.
A cube of 9 cm edge is immersed completely in a rectangular vessel containing water. If the dimensions of the base are 15 cm and 12 cm, find the rise in water level in the vessel.
Solution:
Edge of a cube = 9 cm
Volume of cube = (9)³ cm³
= 729 cm³
Now length of vessel (l) = 15 cm
and breadth (b) = 12 cm

Question 14.
A field is 200 m long and 150 m broad. There is a plot, 50 m long and 40 m broad, near the field. The plot is dug 7 m deep and the earth taken out is spread evenly on the field. By how many metres is the level of the field raised? Give the answer to the second place of decimal.
Solution:
Length of a field (l) = 200 m
Breadth (b) = 150 m
Length of plot = 50 m
and breadth = 40 m
Depth of plot = 7 m

Question 15.
A field is in the form of a rectangle of length 18 m and width 15 m. A pit, 7.5 m long, 6 m broad and 0.8 m deep, is dug in a comer of the field and the earth taken out is spread- over the remaining area of the field. Find out the extent to which the level of the field has been raised.
Solution:
Length of a field (L) = 18m
and width (B) = 15 m
Length of pit (l) = 7.5 m
Breadth (b) = 6 m
and depth (h) = 0.8 m

∴ Volume of earth dugout = lbh
= 7.5 x 6 x 0.8 m³
= 45 x 0.8 = \(\frac { 45 x 4 }{ 5 }\) = 36 m3
Total area of the field = L x B
= 18 x 15 = 270 m²
and area of pit = lb = 7.5 x 6 = 45 m²
∴ Remaining area of the field excluding pit
= 270 – 45 = 225 m²
Let by spreading the earth on the remaining part of the field, the height = h
= 225 x h = 36
⇒ h = \(\frac { 36 }{ 225 }\) = \(\frac { 4 }{ 25 }\)= 0.16 m = 16 cm
∴ Level of field raised = 16 cm

Question 16.
A village having a population of 4000 requires 150 litres of water per head per day. It has a tank measuring 20 m x 15m x 6 m. For how many days will the water of this tank last? [NCERT]
Solution:
Population of a village = 4000
Water required per head per day = 150 litres
∴ Total water required = 4000 x 150 litres = 600000 litres
Dimensions of a tank = 20mx 15mx6m
∴ Volume of tank = 20 x 15 x 6 m3 = 1800 m³
Capacity of water in litres = 1800 x 1000 litres (1 m³ = 1000 litres)
= 1800000 litres
The water will last for = \(\frac { 1800000 }{ 600000 }\) = 3 days

Question 17.
A child playing with building blocks, which are of the shape of the cubes, has built a structure as shown in the figure. If the edge of each cube is 3 cm, find the volume of the structure built by the child. [NCERT]

Solution:
No. of cubes at the given structure = 1+ 2 + 3+ 4 + 5 = 15
Edge of one cube = 3 cm
∴ Volume of one cube = (3)³ = 3 x 3 x 3 cm³ = 27 cm³
∴Volume of the structure = 27 x 15 cm³ = 405 cm³

Question 18.
A godown measures 40 m x 25 m x 10 m. Find the maximum number of wooden crates each measuring 1.5 m x 1.25 m x 0.5 m that can be stored in the godown. [NCERT]
Solution:
Length of godown (L) = 40 m
Breadth (B) = 25 m
and height (H) = 10 m
∴ Volume of godown = LBH
= 40 x 25 x 10 = 10000 m³
Dimension of one wooden crates = 1.5 m x 1.25 m x 0.5 m
∴Volume of one crate = 1.5 x 1.25 x 0.5 m³ = 0.9375 m³
∴ Number of crates to be stored in the

Question 19.
A wall of length 10 m was to be built across an open ground. The height of the wall is 4 m and thickness of the wall is 24 cm. If this wall is to be built up with bricks whose dimensions are 24 cm x 12 cm x 8 cm, how many bricks would be required? [NCERT]
Solution:
Length of wall (L) = 10 m = 1000 cm
Height (H) = 4 m = 400 cm
Thickness (B) = 24 cm = 24 cm
∴ Volume of wall = LBH = 1000 x 24 x 400 cm3 = 9600000 cm³
Dimensions of one brick = 24 cm x 12 cm x 8 cm = 2304 cm³

Question 20.
If V is the volume of a cuboid of dimensions a, b, c and S is its surface area, then prove that

Solution:
a, b, c are the dimensions of a cuboid S is the surface area and V is the volume
∴ V = abc and S = 2(lb + bc + ca)

Question 21.
The areas of three adjacent faces of a cuboid are x, y and z. If the volume is V, prove that V² = xyz.
Solution:
Let a, b, c are the dimensions of a cuboid then,
x = ab, y = bc, z = ca
and V = abc
Now L.H.S. = V²
= (abc)² = a²b²c²
= ab.bc.ca = xyz = R.H.S.
Hence V² = xyz

Question 22.
A river 3 m deep and 40 m wide is flowing at the rate of 2 km per hour. How much water will fall into the sea in a minute? [NCERT]
Solution:
Speed of water in a river = 2 km/hr

Question 23.
Water in a canal 30 dm wide and 12 dm deep, is flowing with a velocity of 100 km per hour. How much area will it irrigate in 30 minutes if 8 cm of standing water is desired?
Solution:
Width of canal (b) = 30 dm = 3 m
Depth (h) = 12 dm = 1.2 m
Speed of water = 100 km/hr
Length of water flow in 30 minutes = \(\frac { 1 }{ 2 }\) hr

Question 24.
Half cubic metre of gold-sheet is extended by hammering so as to cover an area of 1 hectare. Find the thickness of the gold- sheet.
Solution:

Question 25.
How many cubic centimetres of iron are there in an open box whose external dimensions are 36 cm, 25 cm and 16.5 cm, the iron being 1.5 cm thick throughout? If 1 cubic cm of iron weighs 15 g, find the weight of the empty box in kg.
Solution:
External length of open box (L) = 36 cm
Breadth (B) = 25 cm
and Height (H) = 16.5 cm
Width of iron sheet used = 1.5 cm
∴ Inner length (l) = 36 – 1.5 x 2 = 36 – 3 = 33 cm
Breadth (b) = 25 – 2 x 1.5 = 25 – 3 = 22 cm
and Height (h) = 16.5 – 1.5 = 15 cm
∴ Volume of the iron used = Outer volume – Inner volume
= 36 x 25 x 16.5 – 33 x 22 x 15
= 14850 – 10890 = 3960 cm3
Weight of 1 cm3 = 15 g

Question 26.
A rectangular container, whose base is a square of side 5 cm, stands on a horizontal table, and holds water upto 1 cm from the top. When a cube is placed in the water it is completely submerged, the water rises to the top and 2 cubic cm of water overflows. Calculate the volume of the cube and also the length of its edge.
Solution:
Base of the container = 5 cm x 5 cm
Level of water upto 1 cm from the top After placing a cube in it, the water rises to the top and 2 cubic cm of water overflows,
(i) ∴ Volume of water = 5 x 5 x 1 + 2 = 25 + 2 = 27 cm³
∴ Volume of cube = 27 cm³

Question 27.
A rectangular tank is 80 m long and 25 m broad. Water-flows into it through a pipe whose cross-section is 25 cm², at the rate of 16 km per hour. How much the level of the water rises in the tank in 45 minutes.
Solution:
Length of tank (l) = 80 m
Breadth (b) = 25 m
Area of cross section of the month of pipe = 25 cm²
and speed of water-flow =16 km/h
∴ Volume of water is 45 minutes

Question 28.
Water in a rectangular reservoir having base 80 m by 60 m is 6.5 m deep. In what time can the water be emptied by a pipe of which the cross-section is a square of side 20 cm, if the water runs through the pipe at the rate of 15 km/hr.
Solution:
Length of reservoir (l) = 80 m
Breadth (b) = 60 m
and depth (h) = 6.5 m
∴ Volume of water in it = lbh = 80 x 60 x 6.5 m³ = 31200 m³
Area of cross-section of the month of pipe = 20 x 20 = 400 cm²

Hope given RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.2 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.1

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.1

Other Exercises

• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.1
• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.2
• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube VSAQS
• RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube MCQS

Question 1.
Find the lateral surface area and total surface area of a cuboid of length 80 cm, breadth 40 cm and height 20 cm.
Solution:
Length of cuboid (l) = 80 cm
Breadth (b) = 40 cm
Height (h) = 20 cm
(i) ∴ Lateral surface area = 2h(l + b)
= 2 x 20(80 + 40) cm²
= 40 x 120 = 4800 cm²
(ii) Total surface area = 2(lb + bh + hl)
= 2(80 x 40 + 40 x 20 + 20 x 80) cm²
= 2(3200 + 800 + 1600) cm²
= 5600 x 2 = 11200 cm²

Question 2.
Find the lateral surface area and total surface area of a cube of edge 10 cm.
Solution:
Edge of cube (a) = 10 cm
(i) ∴ Lateral surface area = 4a²
= 4 x (10)² = 4 x 100 cm²= 400 cm²
(ii) Total surface area = 6a² = 6 x(10)² cm²
= 6 x 100 = 600 cm²

Question 3.
Find the ratio of the total surface area and lateral surface area of a cube.
Solution:
Let a be the edge of the cube, then Total surface area = 6a2²
and lateral surface area = 4a²
Now ratio between total surface area and lateral surface area = 6a² : 4a² = 3 : 2

Question 4.
Mary wants to decorate her Christmas tree. She wants to place the tree on a wooden block covered with coloured paper with picture of Santa Claus on it. She must know the exact quantity of paper to buy for this purpose. If the box has length, breadth and height as 80 cm, 40 cm and 20 cm respectively. How many square sheets of paper of side 40 cm would she require?   [NCERT]
Solution:
Length of box (l) = 80 cm
Breadth (b) = 40 cm
and height (h) = 20 cm
∴ Total surface area = 2(lb + bh + hl)
= 2[80 x 40 + 40 x 20 + 20 x 80] cm²
= 2[3200 + 800 + 1600] cm² = 2 x 5600 = 11200 cm²
Size of paper sheet = 40 cm
∴ Area of one sheet = (40 cm)² = 1600 cm²
∴ No. of sheets required for the box = 11200 = 1600 = 7 sheets

Question 5.
The length, breadth and height of a room are 5 m, 4 m and 3 m respectively. Find the cost of white washing the walls of the room and the ceiling at the rate of ₹7.50 m².
Solution:
Length of a room (l) = 5m
Breadth (b) = 4 m
and height (h) = 3 m
∴ Area of 4 walls = 2(l + b) x h
= 2(5 + 4) x 3 = 6 x 9 = 54 m²
and area of ceiling = l x b = 5 x 4 = 20 m²
∴ Total area = 54 + 20 = 74 m2
Rate of white washing = 7.50 per m²
∴ Total cost = ₹74 x 7.50 = ₹555

Question 6.
Three equal cubes are placed adjacently in a row. Find the ratio of total surface area of the new cuboid to that of the sum of the surface areas of the three cubes.
Solution:
Let each side of a cube = a cm
Then surface area = 6a² cm²
and surface area of 3 such cubes = 3 x 6a² = 18a² cm²
By placing three cubes side by side we get a cuboid whose,
Length (l) = a x 3 = 3a
Breadth (b) = a
Height (h) = a
∴ Total surface area = 2(lb + bh + hf)
= 2[3a x a+a x a+a x 3a] cm²
= 2[3a² + a² + 3a²] = 14 a²
∴ Ratio between their surface areas = 14a² : 18a² = 7 : 9

Question 7.
A 4 cm cube is cut into 1 cm cubes. Calculate the total surface area of all the small cubes.
Solution:
Side of cube = 4 cm
But cutting into 1 cm cubes, we get = 4 x 4 x 4 = 64
Now surface area of one cube = 6 x (1)²
= 6 x 1=6 cm²
and surface area of 64 cubes = 6 x 64 cm² = 384 cm²

Question 8.
The length of a hall is 18 m and the width 12 m. The sum of the areas of the floor and the flat roof is equal to the sum of the areas of the four walls. Find the height of the hall.
Solution:
Let h be the height of the room
Length (l) = 18 m
and width (b) = 12 m
Now surface area of floor and roof = 2 x lb = 2 x 18 x 12 m²
= 432 m²
and surface area of 4-walls = 2h (l + b)
= 2h(18 + 12) = 2 x 30h m² = 60h m²
∵ The surface are of 4-walls and area of floor and roof are equal
∴ 60h = 432
⇒ h = \(\frac { 432 }{ 60 }\) = \(\frac { 72 }{ 10 }\) m
∴ Height = 7.2m

Question 9.
Hameed has built a cubical water tank with lid for his house, with each other edge 1.5m long. He gets the outer surface of the tank excluding the base, covered with square tiles of side 25 cm. Find how much he would spend for the tiles, if the cost of tiles is ₹360 per dozen. [NCERT]
Solution:
Edge of cubical tank = 1.5 m
∴ Area of 4 walls = 4 (side)² = 4(1.5)² m² = 4 x 225 = 9 m²
Area of floor = (1.5)² = 2.25 m²
∴ Total surface area = 9 + 2.25 = 11.25 m²
Edge of square tile = 25 m = 0.25 m²
∴ Area of 1 tile = (0.25)2 = .0625 m²

Question 10.
Each edge of a cube is increased by 50%. Find the percentage increase in the surface area of the cube.
Solution:
Let edge of a cube = a
Total surface area = 6a2
By increasing edge at 50%,

Question 11.
A closed iron tank 12 m long, 9 m wide and 4 m deep is to be made. Determine the cost of iron sheet used at the rate of ₹5 per metre sheet, sheet being 2 m wide.
Solution:
Length of iron tank (l) = 12 m
Breadth (b) = 9 m
Depth (h) = 4 cm

Question 12.
Ravish wanted to make a temporary shelter for his car by making a box-like structure with tarpaulin that covers all the four sides and the top of tire car (with the front face as a flap which can be rolled up). Assuming that the stitching margins are very small, and therefore negligible, how much tarpaulin would be required to make (he shelter of height 2.5 m with base dimensions 4 m x 3 m? [NCERT]
Solution:
Length of base (l) = 4m
Breadth (b) = 3 m
Height (h) = 2.5 m

Question 13.
An open box is made of wood 3 cm thick. Its external length, breadth and height are 1.48 m, 1.16 m and 8.3 dm. Find the cost of painting the inner surface of ₹50 per sq. metre.
Solution:
Length of open wood box (L) = 1.48 m = 148 cm
Breadth (B) = 1.16 m = 116 cm
and height (H) = 8.3 dm = 83 cm
Thickness of wood = 3 cm

Question 14.
The dimensions of a room are 12.5 m by 9 m by 7 m. There are 2 doors and 4 windows in the room; each door measures 2.5 m by 1.2 m and each window 1.5 m by 1 m. Find the cost of painting the walls at ₹3.50 per square metre.
Solution:
Length of room (l) = 12.5 m
Breadth (b) = 9 m
and height (h) = 7 m
∴ Total area of walls = 2h(l + b)
= 2 x 7[12.5 + 9] = 14 x 21.5 m² = 301 m²
Area of 2 doors of 2.5 m x 1.2 m
= 2 x 2.5 x 1.2 m² = 6 m²
and area of 4 window of 1.5 m x 1 m
= 4 x 1.5 x 1 = 6 m²
∴ Remaining area of walls = 301 – (6 + 6)
= 301 – 12 = 289 m²
Rate of painting the walls = ₹3.50 per m²
∴ Total cost = 289 x 3.50 = ₹1011.50

Question 15.
The paint in a certain container is sufficient to paint on area equal to 9.375 m2. How many bricks of dimension 22.5 cm x 10 cm x 7.5 cm can be painted out of this container? [NCERT]
Solution:
Area of place for painting = 9.375 m²
Dimension of one brick = 22.5 cm x 10 cm x 7.5 cm
∴ Surface area of one bricks = 2 (lb + bh + hl)
= 2[22.5 x 10 + 10 x 7.5 + 7.5 x 22.5] cm2
= 2[225 + 75 + 168.75]
= 2 x 468.75 cm² = 937.5 cm²

Question 16.
The dimensions of a rectangular box are in the ratio of 2 : 3 : 4 and the difference between the cost of covering it with sheet of paper at the rates of ₹8 and ₹9.50 per m2 is ₹1248. Find the dimensions of the box.
Solution:
Ratio in the dimensions of a cuboidal box = 2 : 3 : 4
Let length (l) = 4x
Breadth (b) = 3.v
and height (h) = 2x
∴ Total surface area = 2 [lb + bh + hl]

Question 17.
The cost of preparing the walls of a room 12 m long at the rate of ₹1.35 per square metre is ₹340.20 and the cost of matting the floor at 85 paise per square metre is ₹91.80. Find the height of the room.
Solution:
Cost of preparing walls of a room = ₹340.20

Question 18.
The length and breadth of a hall are in the ratio 4 : 3 and its height is 5.5 metres. The cost of decorating its walls (including doors and windows) at ₹6.60 per square metre is ₹5082. Find the length and breadth of the room
Solution:
Ratio in length and breadth = 4:3
and height (h) = 5.5 m
Cost of decorating the walls of a room including doors and windows = ₹5082
Rate = ₹6.60 per m²

Question 19.
A wooden bookshelf has external dimensions as follows: Height =110 cm, Depth = 25 cm, Breadth = 85 cm (see figure). The thickness of the plank is 5 cm everywhere. The external faces are to be polished and the inner faces are to be painted. If the rate of polishing is 20 paise per cm2 and the rate of painting is 10 paise per cm2. Find the total expenses required for polishing and painting the surface of the bookshelf.  [NCERT]
Solution:
Length (l) = 85 cm
Breadth (b) = 25 cm
and height (h) = 110 cm
Thickness of plank = 5 cm
Surface area to be polished
= [(100 x 85) + 2 (110 x 25) + 2 (85 x 25) + 2 (110 x 5) + 4 (75 x 5)]
= (9350 + 5500 + 4250 + 1100 + 1500) cm² = 21700 cm²

Hope given RD Sharma Class 9 Solutions Chapter 18 Surface Areas and Volume of a Cuboid and Cube Ex 18.1 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles VSAQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles VSAQS

Other Exercises

• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.1
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.2
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.3
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.4
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.5
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles VSAQS
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles MCQS

Question 1.
In the figure, two circles intersect at A and B. The centre of the smaller circle is O and it lies on the circumference of the larger circle. If ∠APB = 70°, find ∠ACB.

Solution:
Arc AB subtends ∠AOB at the centre and ∠APB at the remaining part of the circle
∴ ∠AOB = 2∠APB = 2 x 70° = 140°
Now in cyclic quadrilateral AOBC,
∠AOB + ∠ACB = 180° (Sum of the angles)
⇒ 140° +∠ACB = 180°
⇒ ∠ACB = 180° – 140° = 40°
∴ ∠ACB = 40°

Question 2.
In the figure, two congruent circles with centre O and O’ intersect at A and B. If ∠AO’B = 50°, then find ∠APB.

Solution:
Two congruent circles with centres O and O’ intersect at A and B

∠AO’B = 50°
∵ OA = OB = O’A = 04B (Radii of the congruent circles)

Question 3.
In the figure, ABCD is a cyclic quadrilateral in which ∠BAD = 75°, ∠ABD = 58° and ∠ADC = IT, AC and BD intersect at P. Then, find ∠DPC.

Solution:
∵ ABCD is a cyclic quadrilateral,

∴ ∠BAD + ∠BCD = 180°
⇒ 75° + ∠BCD – 180°
⇒ ∠BCD = 180°-75°= 105° and ∠ADC + ∠ABC = 180°
⇒ 77° + ∠ABC = 180°
⇒ ∠ABC = 180°-77°= 103°
∴ ∠DBC = ∠ABC – ∠ABD = 103° – 58° = 45°
∵ Arc AD subtends ∠ABD and ∠ACD in the same segment of the circle 3
∴ ∠ABD = ∠ACD = 58°
∴ ∠ACB = ∠BCD – ∠ACD = 105° – 58° = 47°
Now in ∆PBC,
Ext. ∠DPC = ∠PBC + ∠PCB
=∠DBC + ∠ACB = 45° + 47° = 92°
Hence ∠DPC = 92°

Question 4.
In the figure, if ∠AOB = 80° and ∠ABC = 30°, then find ∠CAO.

Solution:
In the figure, ∠AOB = 80°, ∠ABC = 30°
∵ Arc AB subtends ∠AOB at the centre and
∠ACB at the remaining part of the circle

∴ ∠ACB = \(\frac { 1 }{ 2 }\)∠AOB = \(\frac { 1 }{ 2 }\) x 80° = 40°
In ∆OAB, OA = OB
∴ ∠OAB = ∠OBA
But ∠OAB + ∠OBA + ∠AOB = 180°
∴ ∠OAB + ∠OBA + 80° = 180°
⇒ ∠OAB + ∠OAB = 180° – 80° = 100°
∴ 2∠OAB = 100°
⇒ ∠OAB = \(\frac { { 100 }^{ \circ } }{ 2 }\)  = 50°
Similarly, in ∆ABC,
∠BAC + ∠ACB + ∠ABC = 180°
∠BAC + 40° + 30° = 180°
⇒ ∠BAC = 180°-30°-40°
= 180°-70°= 110°
∴ ∠CAO = ∠BAC – ∠OAB
= 110°-50° = 60°

Question 5.
In the figure, A is the centre of the circle. ABCD is a parallelogram and CDE is a straight line. Find ∠BCD : ∠ABE.

Solution:
In the figure, ABCD is a parallelogram and
CDE is a straight line

∵ ABCD is a ||gm
∴ ∠A = ∠C
and ∠C = ∠ADE (Corresponding angles)
⇒ ∠BCD = ∠ADE
Similarly, ∠ABE = ∠BED (Alternate angles)
∵ arc BD subtends ∠BAD at the centre and
∠BED at the remaining part of the circle

Question 6.
In the figure, AB is a diameter of the circle such that ∠A = 35° and ∠Q = 25°, find ∠PBR.

Solution:
In the figure, AB is the diameter of the circle such that ∠A = 35° and ∠Q = 25°, join OP.

Arc PB subtends ∠POB at the centre and
∠PAB at the remaining part of the circle
∴ ∠POB = 2∠PAB = 2 x 35° = 70°
Now in ∆OP,
OP = OB radii of the circle
∴ ∠OPB = ∠OBP = 70° (∵ ∠OPB + ∠OBP = 140°)
Now ∠APB = 90° (Angle in a semicircle)
∴ ∠BPQ = 90°
and in ∆PQB,
Ext. ∠PBR = ∠BPQ + ∠PQB
= 90° + 25°= 115°
∴ ∠PBR = 115°

Question 7.
In the figure, P and Q are centres of two circles intersecting at B and C. ACD is a straight line. Then, ∠BQD =

Solution:
In the figure, P and Q are the centres of two circles which intersect each other at C and B
ACD is a straight line ∠APB = 150°
Arc AB subtends ∠APB at the centre and
∠ACB at the remaining part of the circle
∴ ∠ACB = \(\frac { 1 }{ 2 }\) ∠APB = \(\frac { 1 }{ 2 }\) x 150° = 75°
But ∠ACB + ∠BCD = 180° (Linear pair)
⇒ 75° + ∠BCD = 180°
∠BCD = 180°-75°= 105°
Now arc BD subtends reflex ∠BQD at the centre and ∠BCD at the remaining part of the circle
Reflex ∠BQD = 2∠BCD = 2 x 105° = 210°
But ∠BQD + reflex ∠BQD = 360°
∴ ∠BQD+ 210° = 360°
∴ ∠BQD = 360° – 210° = 150°

Question 8.
In the figure, if O is circumcentre of ∆ABC then find the value of ∠OBC + ∠BAC.

Solution:
In the figure, join OC

∵ O is the circumcentre of ∆ABC
∴ OA = OB = OC
∵ ∠CAO = 60° (Proved)
∴ ∆OAC is an equilateral triangle
∴ ∠AOC = 60°
Now, ∠BOC = ∠BOA + ∠AOC
= 80° + 60° = 140°
and in ∆OBC, OB = OC
∠OCB = ∠OBC
But ∠OCB + ∠OBC = 180° – ∠BOC
= 180°- 140° = 40°
⇒ ∠OBC + ∠OBC = 40°
∴ ∠OBC = \(\frac { { 40 }^{ \circ } }{ 2 }\)  = 20°
∠BAC = OAB + ∠OAC = 50° + 60° = 110°
∴ ∠OBC + ∠BAC = 20° + 110° = 130°

Question 9.
In the AOC is a diameter of the circle and arc AXB = 1/2 arc BYC. Find ∠BOC.

Solution:
In the figure, AOC is diameter arc AxB = \(\frac { 1 }{ 2 }\) arc BYC 1
∠AOB = \(\frac { 1 }{ 2 }\) ∠BOC
⇒ ∠BOC = 2∠AOB
But ∠AOB + ∠BOC = 180°
⇒ ∠AOB + 2∠AOB = 180°
⇒ 3 ∠AOB = 180°
∴ ∠AOB = \(\frac { { 180 }^{ \circ } }{ 3 }\)  = 60°
∴ ∠BOC = 2 x 60° = 120°

Question 10.
In the figure, ABCD is a quadrilateral inscribed in a circle with centre O. CD produced to E such that ∠AED = 95° and ∠OBA = 30°. Find ∠OAC.

Solution:
In the figure, ABCD is a cyclic quadrilateral
CD is produced to E such that ∠ADE = 95°
O is the centre of the circle

∵ ∠ADC + ∠ADE = 180°
⇒ ∠ADC + 95° = 180°
⇒ ∠ADC = 180°-95° = 85°
Now arc ABC subtends ∠AOC at the centre and ∠ADC at the remaining part of the circle
∵ ∠AOC = 2∠ADC = 2 x 85° = 170°
Now in ∆OAC,
∠OAC + ∠OCA + ∠AOC = 180° (Sum of angles of a triangle)
⇒ ∠OAC = ∠OCA (∵ OA = OC radii of circle)
∴ ∠OAC + ∠OAC + 170° = 180°
2∠OAC = 180°- 170°= 10°
∴ ∠OAC = \(\frac { { 10 }^{ \circ } }{ 2 }\) = 5°

Hope given RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles VSAQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.