Category: ICSE Class 10

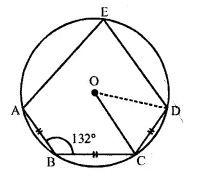
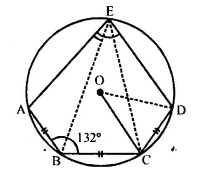
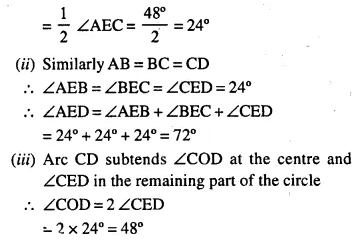
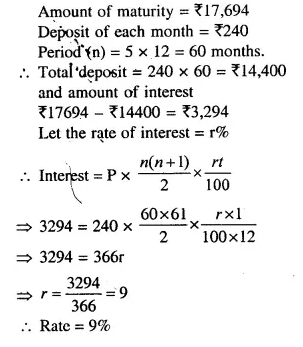
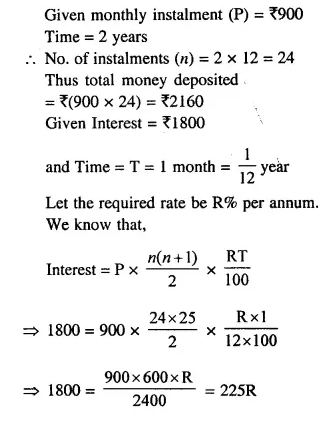
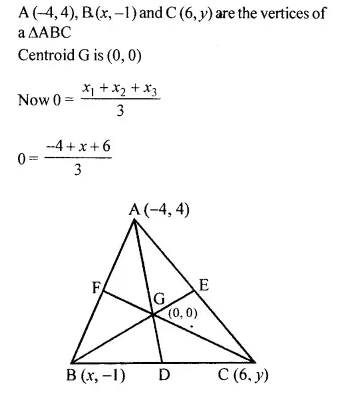
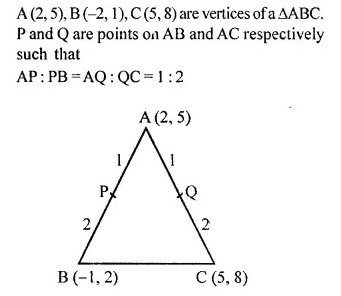
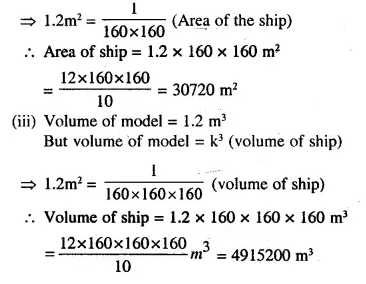
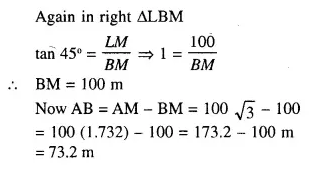
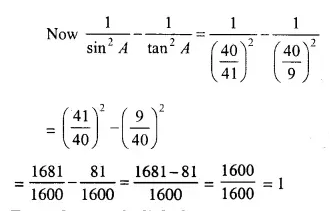
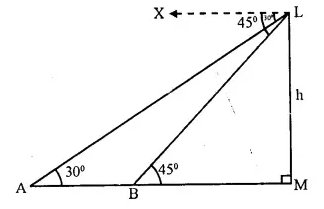
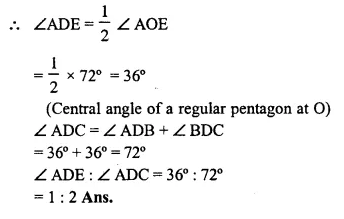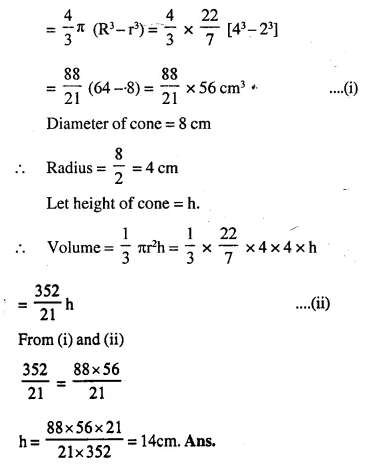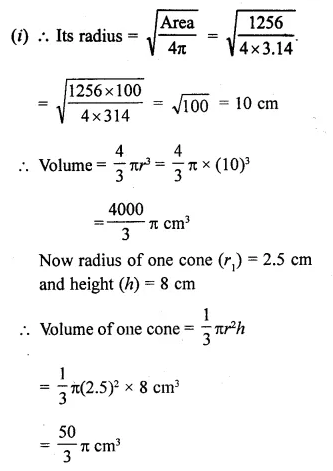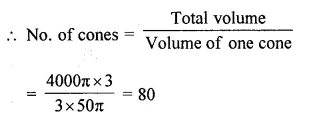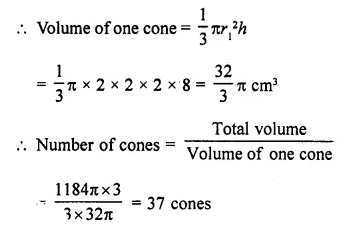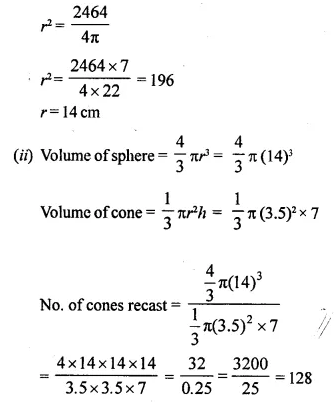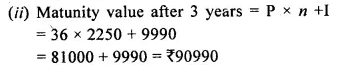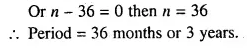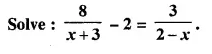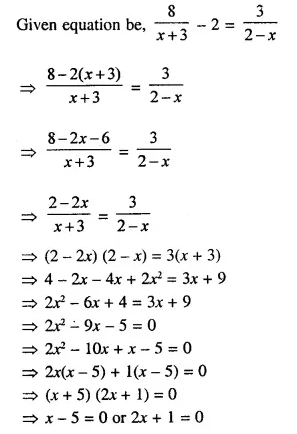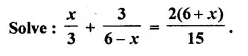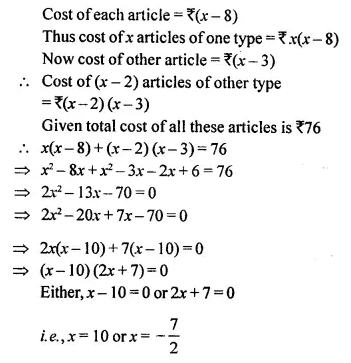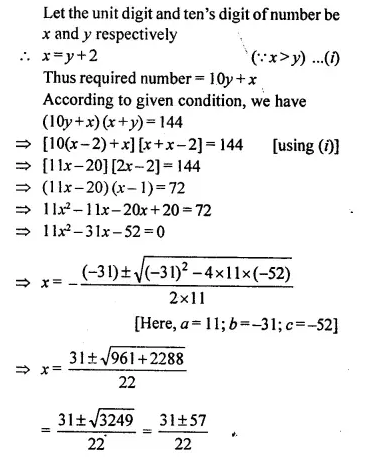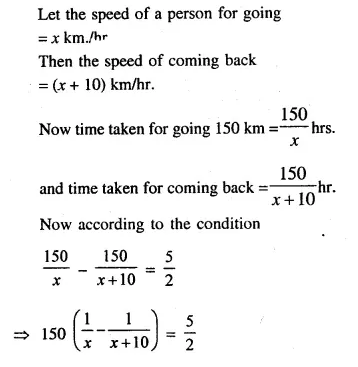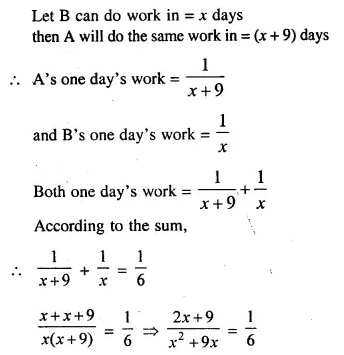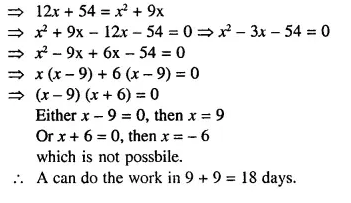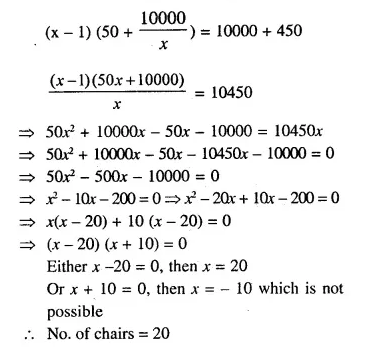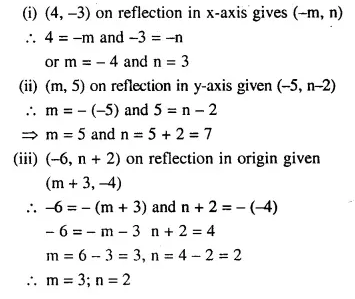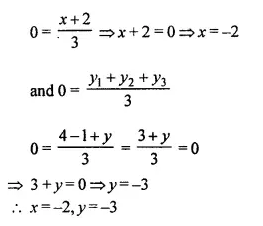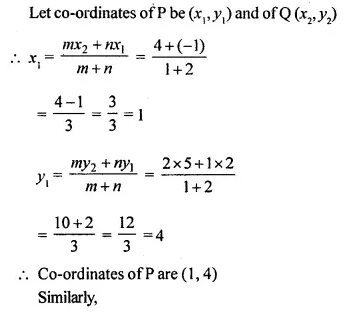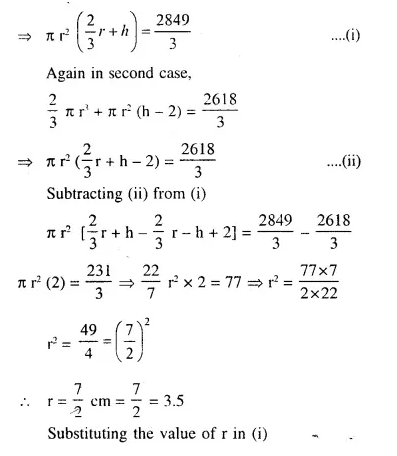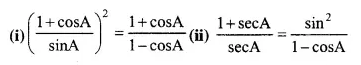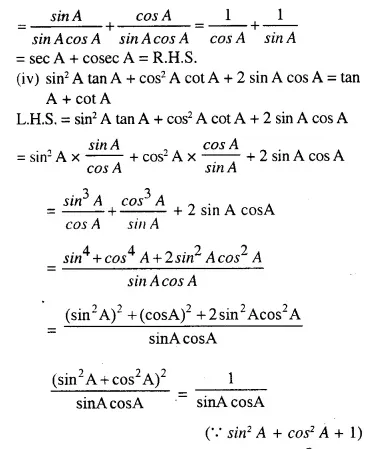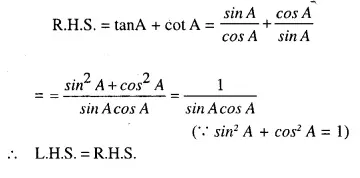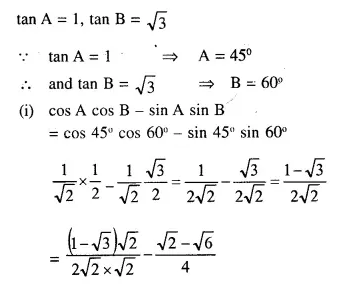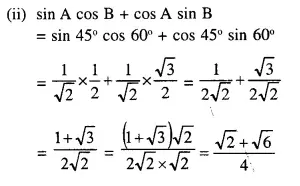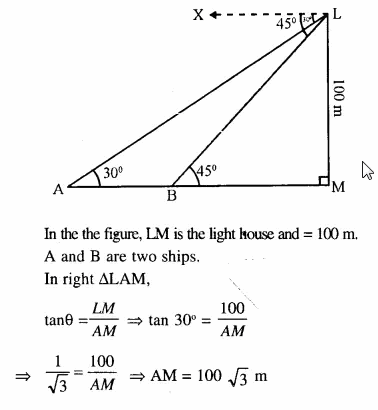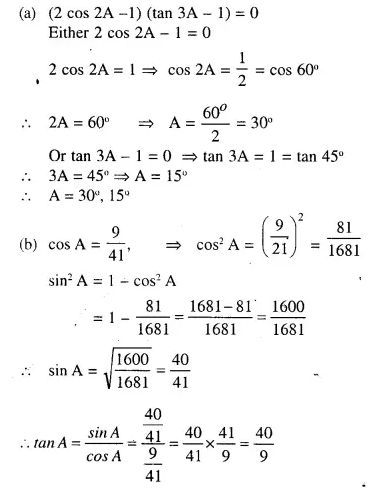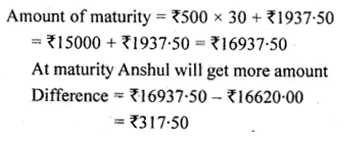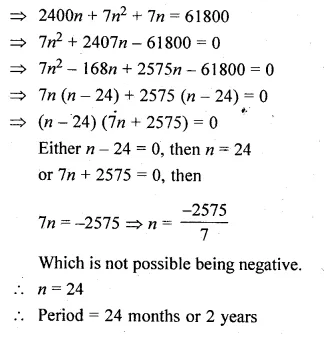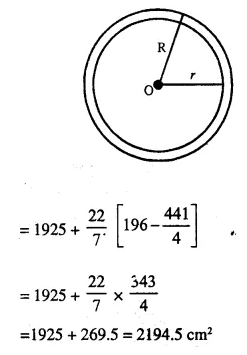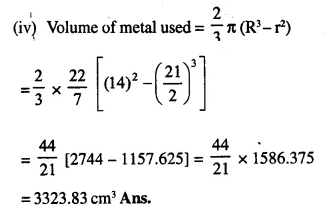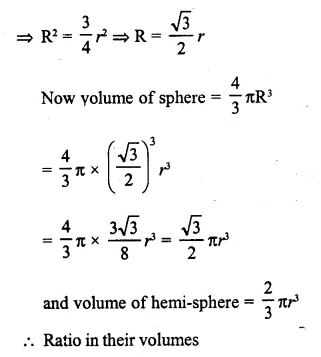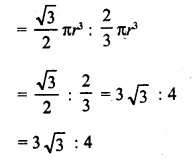Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere (Surface Area and Volume) Ex 20E

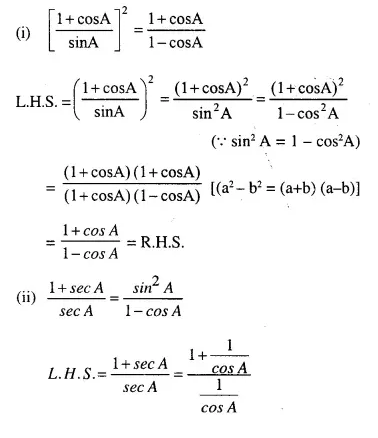
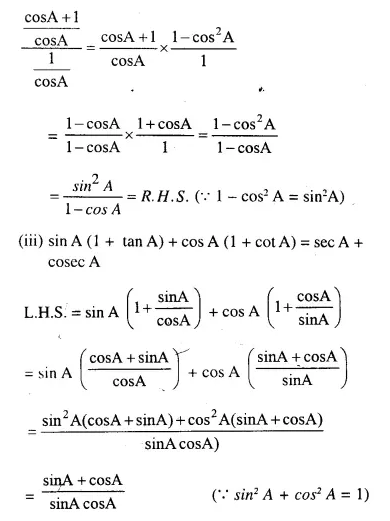
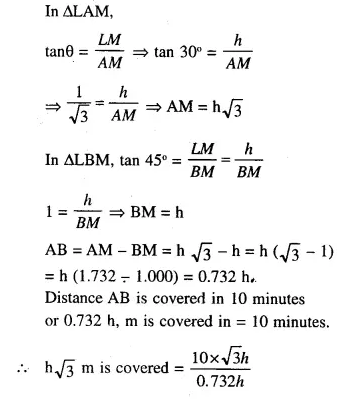
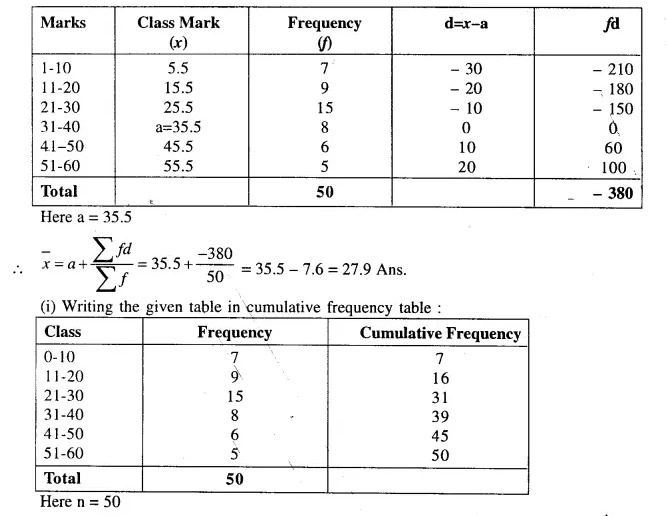
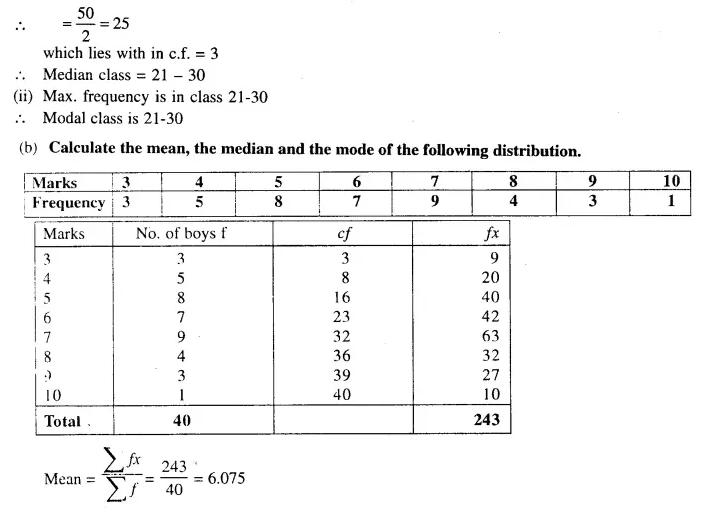
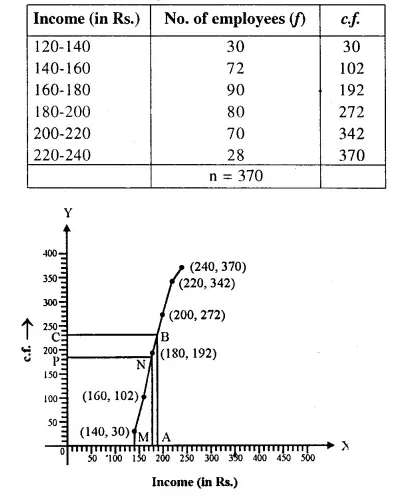
These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21E

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20E
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20F
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20G

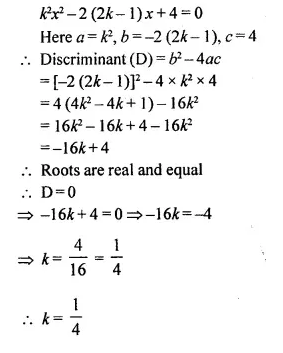
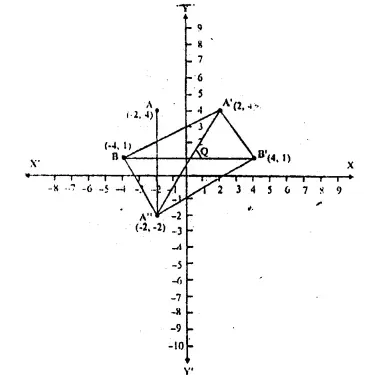
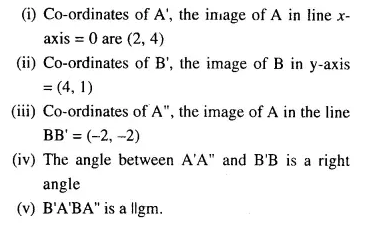
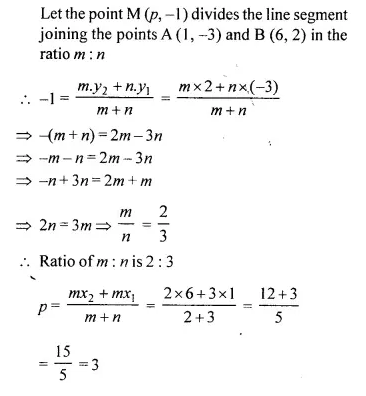
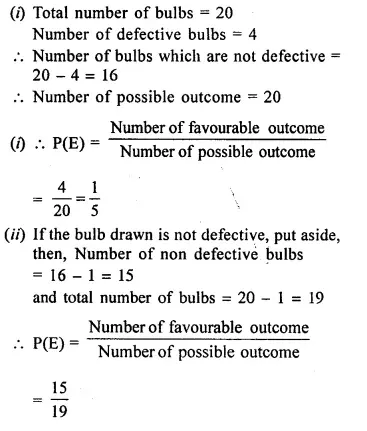
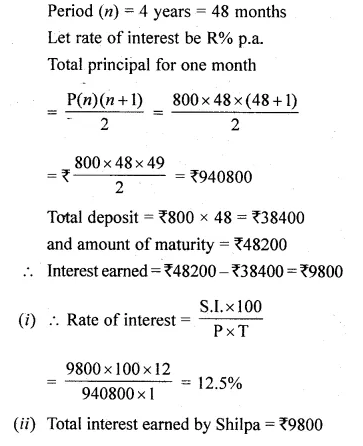
Question 1.
A cone of height 15 cm and diameter 7 cm is mounted on a hemisphere of same diameter. Determine the volume of the solid thus formed.
Solution:
Height of cone = 15 cm
and radius of base = \(\frac { 7 }{ 2 }\)cm.

Question 2.
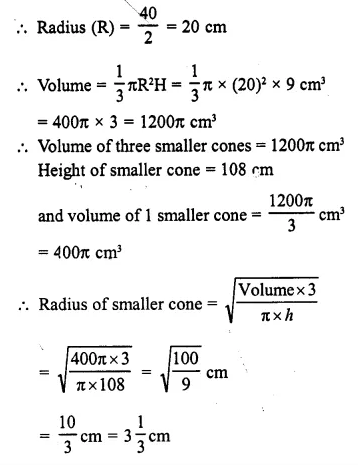
A buoy is made in the form of hemisphere surmounted by a right, cone whose circular base coincides with the plane surface of hemisphere. The radius of the base of the cone is 3.5 metres and its volume is two-thirds of the hemisphere. Calculate the height of the cone and the surface area of the buoy, correct to two places of decimal.
Solution:
Radius of hemispherical part (r)

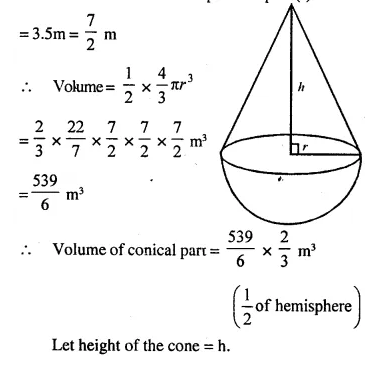
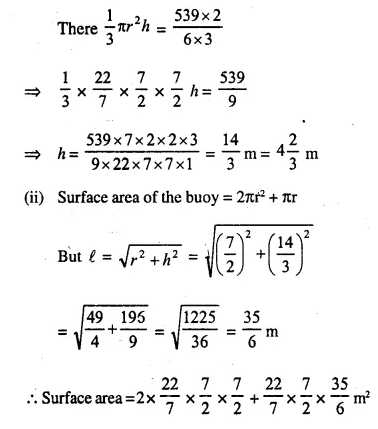
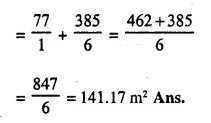
Question 3.
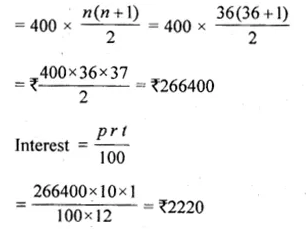
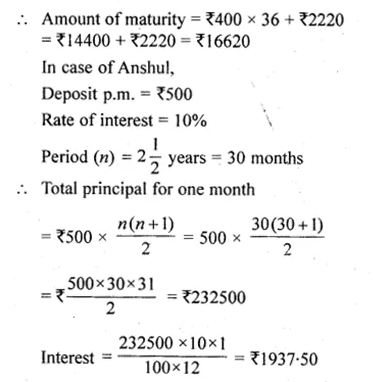
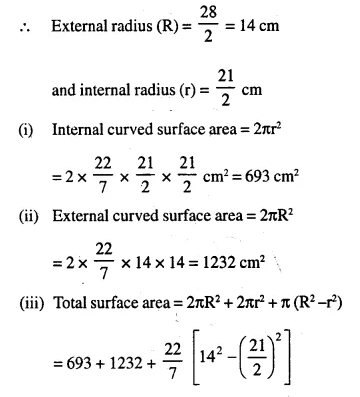
 From a rectangular solid of metal 42 cm by 30 cm by 20 cm, a conical cavity of diam­eter 14 cm and depth 24 cm is drilled out. Find:
(i) the surface area of remaining solid,
(ii) the volume of remaining solid,
(iii) the weight of the material drilled out if it weighs 7 gm per cm³.
Solution:
Length of rectangular solid (l) = 30 cm
Breadth (b) = 20 cm
and height (h) = 42 cm
Diameter of the cone = 14 cm

(i)  Surface area of remaining solid Surface area of rectangular solid + Surface area of curved surface of cone – Surface area of the base of the cone
= 2 (lb + bh + hl) + πrl – πr²

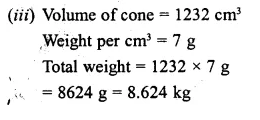
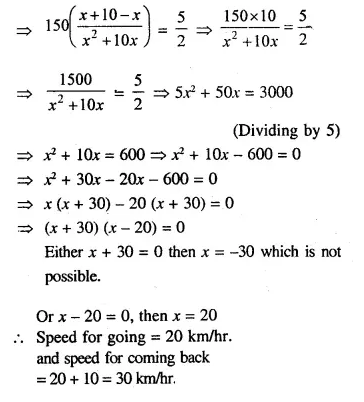
Question 4.
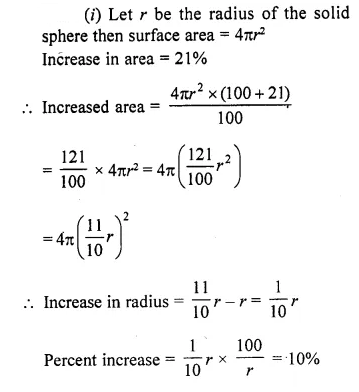
A cubical block of side 7 cm is surmounted by a hemisphere of the largest size. Find the surface area of the resulting solid.
Solution:
Side of a cubical block = 7 cm
Radius of the hemisphere = \(\frac { 7 }{ 2 }\)cm
Now total surface area of the block

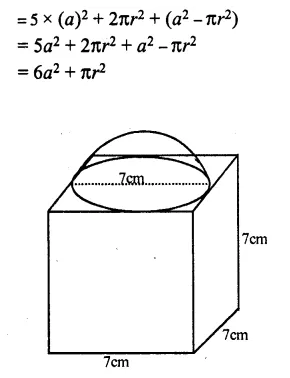
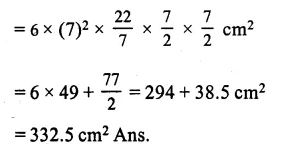
Question 5.
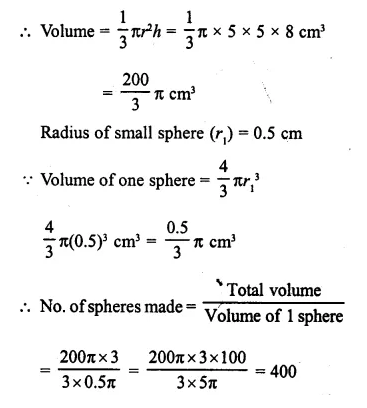
A vessel is in the form of an inverted cone. Its height is 8 cm and the radius of its top, which is open, is 5 cm. It is filled with water up to the rim. When lead shots each of which is a sphere of radius 0.5 cm are dropped into the vessel, one- fourth of the water flows out. Find the number of lead shots dropped in the vessel.
Solution:
Radius of conical vessel (R) = 5 cm
and height (h) = 8 cm

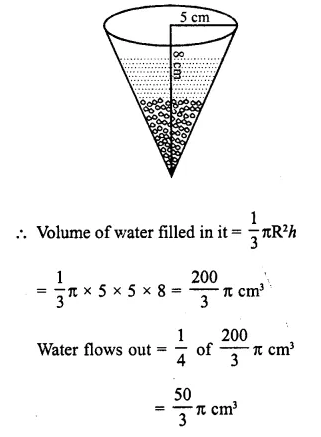
Question 6.
A hemi-spherical bowl has neligible thickness and the length of its circumference is 198 cm. Find the capacity of the bowl.
Solution:
Upper circumference of the hemi-spherical bowl = 198 cm

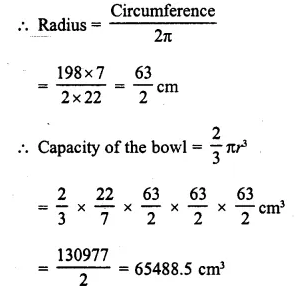
Question 7.
Find the maximum volume of a cone that can be carved out of a solid hemisphere of radius r cm.
Solution:
Radius of solid hemisphere = r
Radius of the cone carved out of the hemisphere = r
and height (h) = r

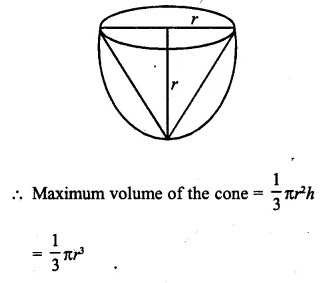
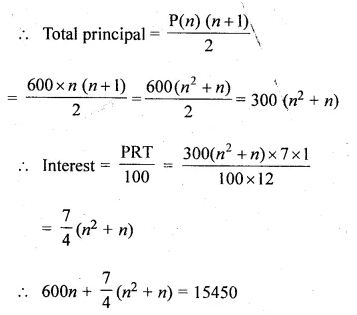
Question 8.
The radii of the bases of two solid right circular cones of same height are r₁ and r₂ The cones are melted and recast into a solid sphere of radius R. Find the height of each cone in terms of r_1, r₂ • and R.
Solution:

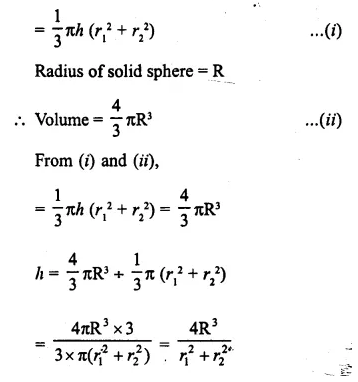
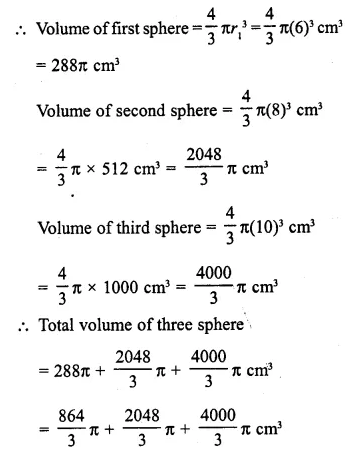
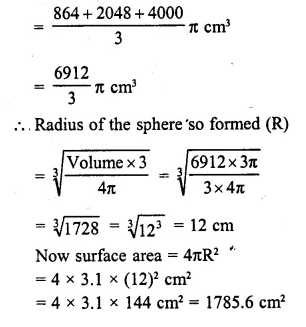
Question 9.
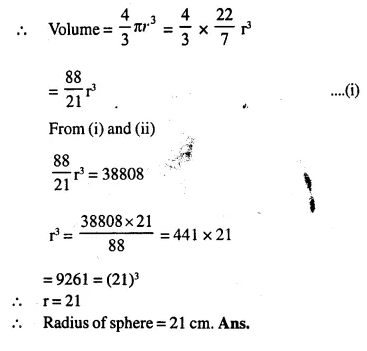
A solid metallic hemisphere of diameter 28 cm is melted and recast into a number of identical solid cones, each of diameter  14 cm and height 8 cm. Find the number * of cones so formed.
Solution:
Diameter of solid hemisphere = 28 cm

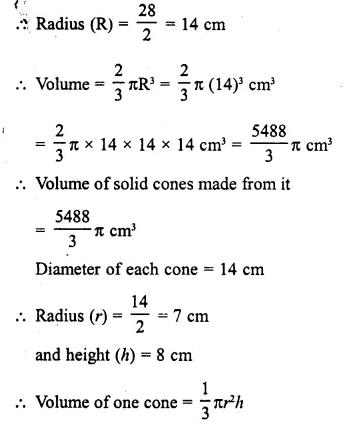
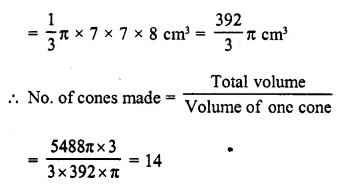
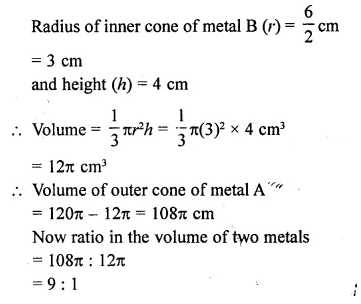
Question 10.
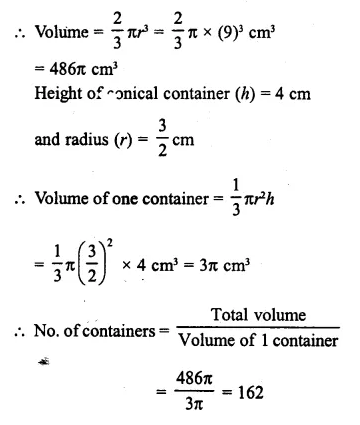
A cone and a hemisphere have the same base and the same height. Find the ratio between their volumes.
Solution:
Let radius of the base of cone = r
and height = h
Then radius of hemisphere = r

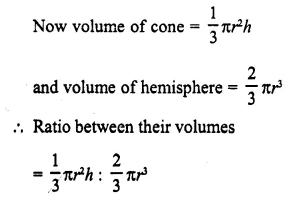
Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20E are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 18 Tangents and Intersecting Chords Ex 18B 

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 18 Tangents and Intersecting Chords Ex 18B.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 18 Tangents and Intersecting Chords Ex 18A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 18 Tangents and Intersecting Chords Ex 18B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 18 Tangents and Intersecting Chords Ex 18C

Question 1.
(i) In the given figure 3 x CP = PD = 9 cm and AP = 4.5 cm. Find BP.

(ii) In the given figure, 5 x PA = 3 x AB = 30 cm and PC = 4 cm. Find CD.

(iii) In the given figure, tangent PT = 12.5 cm and PA = 10 cm; find AB.

Solution:

Question 2.
In the figure given below, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find:
(i) AB.
(ii) the length of tangent PT. (2014)

Solution:
PT is tangent and PDC is secant out to the circle
∴ PT² = PC x PD
PT² = (5 + 7.8) x 5 = 12.8 x 5
PT² = 64 ⇒ PT = 8 cm
In ΔOTP
PT² + OT² = OP²
8²+x² = (x + 4)²
⇒ 64 +x² = x² + 16 + 8x
64- 16 = 8x
⇒ 48 = 8x
x = \(\frac { 48 }{ 8 }\) = 6 cm
∴ Radius = 6 cm
AB = 2 x 6 = 12 cm

Question 3.
In the following figure, PQ is the tangent to the circle at A, DB is the diameter and O is the centre of the circle. If ∠ ADB = 30° and ∠ CBD = 60°, calculate
(i) ∠ QAB
(ii) ∠ PAD
(iii) ∠ CDB.

Solution:
(i) PAQ is a tangent and AB is the chord, ∠ QAB = ∠ ADB (Angles in the alternate segment)
= 30°
(ii) OA = OD (Radii of the same circle)
∴ ∠ OAD = ∠ ODA = 30°
But OA ⊥ PQ
∴ ∠ PAD = ∠ OAP – ∠OAD = 90° – 30° = 60°
(iii) BD is diameter
∴ ∠ BCD = 90° (Angle in semi circle)
Now in ∆ BCD,
∠ CDB + ∠ CBD + ∠ BCD = 180°
⇒ ∠ CDB + 60° + 90° = 180°
⇒ ∠ CDB = 180°- (60° + 90°) = 180° – 150° = 30°

Question 4.
If PQ is a tangent to the circle at R; calculate:
(i) ∠ PRS
(ii) ∠ ROT.
Given O is the centre of the circle and angle TRQ = 30°.
Solution:

PQ is tangent and OR is the radius
∴ OR ⊥ PQ
∴ ∠ORT = 90° =
∠TRQ = 90° – 30° = 60°
But in ∆ OTR, OT = OR (Radii of the circle)
∴ ∠ OTR = 60° or ∠STR = 60°
But ∠PRS = ∠STR (Angles in the alternate segment) = 60°
In ∆ ORT, ∠ OTR = 60°, ∠ TOR = 60°
∴ ∠ROT= 180°-(60°+ 60°)= 180°-120° = 60°

Question 5.
AB is the diameter and AC is a chord of a circle with centre O such that angle BAC = 30°. The tangent to the circle at C intersect AB produced in D. Show that BC = BD.
Solution:


Given: In a circle, O is the centre,
AB is the diameter, a chord AC such that ∠ BAC = 30°
and a tangent from C, meets AB in D on producing. BC is joined.
To Prove: BC = BD
Construction: Join OC
Proof : ∠ BCD = ∠ BAC =30°(Angle in alternate segment)
Arc BC subtends ∠ DOC at the centre of the circle and ∠ BAC at the remaining part of the circle.
∴ ∠ BOC = 2 ∠ BAC = 2 x 30° = 60°
Now in ∆ OCD,
∠ BOC or ∠ DOC = 60° (Proved)
∠ OCD = 90° (∵ OC ⊥ CD)
∴ ∠ DOC + ∠ ODC = 90°
⇒ 60° + ∠ ODC = 90°
∴ ∠ ODC = 90°- 60° = 30°
Now in ∆BCD,
∵∠ ODC or ∠ BDC = ∠ BCD (Each = 30°)
∴ BC = BD Q.E.D.

Question 6.
Tangent at P to the circumcircle of triangle PQR is drawn. If this tangent is parallel to side QR, show that ∆PQR is isosceles.
Solution:

In a circumcircle of ∆PQR, a tangent TPS is drawn through P which is parallel to QR
To prove : ∆PQR is an isosceles triangle.
Proof:
∵ TS \(\parallel\) QR
∠TPQ = ∠PQR (Alternate angles) ….(i)
∵ TS is tangent and PQ is the chord of the circle
∴ ∠TPQ = ∠RP (Angles in the alternate segment) ….(ii)
From (i) and (ii),
∠PQR = ∠QRP
∴ PQ = PR (Opposite sides of equal angles)
∴ ∆PQR is an isosceles triangle
Hence proved

Question 7.
Two circles with centres O and O’ are drawn to intersect each other at points A and B. Centre O of one circle lies on the circumference of the other circle and CD is drawn tangent to the circle with centre O’ at A. Prove that OA bisects angle BAC.

Solution:

Given: Two circles with centre O and O’ intersect each other at A and B, O lies on the circumference, of the other circle. CD is a tangent at A to the second circle. AB, OA are joined.
To Prove: OA bisects ∠ BAC.
Construction: Join OB, O’A, O’B and OO’
Proof: CD is the tangent and AO is the chord
∠ OAC = ∠ OBA …(i)
(Angles in alt. segment)
In ∆ OAB, OA = OB (Radii of the same circle)
∴ ∠ OAB = ∠ OBA ….(ii)
From (i) and (ii),
∠ OAC = ∠ OAB
∴ OA is the bisector of ∠ BAC Q.E.D.

Question 8.
Two circles touch each other internally at a point P. A chord AB of the bigger circle intersects the other circle in C and D. Prove that: ∠ CPA = ∠DPB.

Solution:
Given:Two circles touch each other internally at P. A chord AB of the bigger circle intersects the smaller circle at C and D. AP, BP, CP and DP are joined.
To Prove: ∠ CPA = ∠ DPB
Construction: Draw a tangent TS at P to the circles given.
Proof:
∵ TPS is the tangent, PD is the chord.
∴ ∠ PAB = ∠ BPS …(i) ( Angles in alt. segment)
Similarly we can prove that
∠ PCD = ∠ DPS …(ii)
Subtracting (i) from (ii), we gel
∠ PCD – ∠ PAB = ∠ DPS – ∠ BPS
But in ∆ PAC,
Ext. ∠ PCD = ∠ PAB + ∠ CPA
∴ ∠ PAB + ∠ CPA – ∠ PAB = ∠ DPS – ∠ BPS
∠ CPA = ∠ DPB           Q.E.D.

Question 9.
In a cyclic quadrilateral ABCD, the diagonal AC bisects the angle BCD. Prove that the diagonal BD is parallel to the tangent to the circle at point A.
Solution:

Given: ABCD is a cyclic quadrilateral and diagonal AC bisects ∠ BCD. AT A. a tangent TAS is drawn. BD is joined.
To Prove: TS || BD.
Proof: ∠ ADB = ∠ ACB …….(i) (Angles in the same segment)
Similarly ∠ ABD = ∠ ACD ……..(ii)
But ∠ ACB = ∠ ACD (AC is the bisector of ∠ BCD)
∴ ∠ ADB = ∠ ABD |From (i) and (ii)]
TAS is a tangent and AB is chord
∴ ∠ BAS = ∠ ADB (Angles in all segment)
But ∠ ADB = ∠ ABD (Proved)
∴ ∠ BAS = ∠ ABD
But these are alternate angles.
∴ TS || BD.      Q.E.D.

Question 10.
In the figure, ABCD is a cyclic quadrilateral with BC = CD. TC is tangent to the circle at point C and DC is produced to point G. If ∠ BCG = 108° and O is the centre of the circle,
Find:
(i) angle BCT
(ii) angle DOC

Solution:

Join OC, OD and AC.
(i) ∠ BCG + ∠ BCD = 180° (Linear pair)
⇒ 108° + ∠ BCD = 180°
(∵∠ BCG = 108° given)
∴∠ BCD = 180° – 108° = 72°
BC = CD (given)
∴ ∠ DCP = ∠ BCT
But ∠ BCT + ∠ BCD + ∠ DCP = 180°
∴∠ BCT + ∠ BCT + 72° = 180°
(∵∠ DCP = ∠ BCT)
2 ∠ BCT = 180° – 72° = 108°
∴∠ BCT = \(\frac { { 108 }^{ \circ } }{ 2 }\) = 54°
(ii) PCT is the tangent and CA is chord
∴ ∠ CAD = ∠ BCT = 54°
But arc DC subtends ∠ DOC at the centre and
∠ CAD at the remaining part of the circle
∴ ∠ DOC = 2 ∠ CAD = 2 x 54° = 108°.

Question 11.
Two circles intersect each other at points A and B. A straight line PAQ cuts the circles at P and Q. If the tangents at P and Q intersect at point T; show that the points P, B, Q and T arc concyclic.
Solution:
Given: Two circles intersect each other at point A and B. PAQ is a line which intersects circles at P, A and Q. At P and Q, tangents are drawn to the circles which meet at T.
To Prove: P, B, Q, T are concyclic.

Construction: Join AB, BP and BQ.
Proof: TP is the tangent and PA. a chord
∴ ∠ TPA = ∠ ABP
(angles in alt. segment)
Similarly we can prove that
∠ TQA = ∠ ABQ …,(ii)
Adding (i) and (ii), we get
∠ TPA + ∠ TQA = ∠ ABP + ∠ ABQ
But in ∆ PTQ,
∠ TPA + ∠ TQA + ∠ PTQ = 180°
⇒ ∠ TPA + ∠ TQA = 180° – ∠ PTQ
⇒ ∠ PBQ = 180°- ∠ PTQ
⇒ ∠ PBQ + ∠PTQ = 180°
But there are the opposite angles of the quadrilateral
∴ Quad. PBQT is a cyclic
Hence P, B. Q and T are concyclic     Q.E.D.

Question 12.
In the figure; PA is a tangent to the circle. PBC is secant and AD bisects angle BAC.
Show that triangle PAD is an isosceles triangle. Also shaw that:

Solution:
Given: In a circle PA is the tangent, PBC is the secant and AD is the bisector of ∠BAC which meets the secant at D.
To Prove:
(i) ∆ PAD is an isosceles triangle.
(ii) ∠CAD = \(\frac { 1 }{ 2 }\) [(∠PBA – ∠PAB)]
Proof:
(i) PA is the tangent and AB is chord.
∠PAB = ∠C ….(i)
(Angles in the alt. segment)
AD is the bisector is ∠BAC
∴ ∠1 = ∠2 ….(ii)
In ∆ ADC,
Ext. ∠ADP = ∠C + ∠1
= ∠PAB + ∠2 = ∠PAD
∴ ∆ PAD is an isosceles triangle.
(ii) In A ABC,
Ext. ∠PBA = ∠C + ∠BAC
∴∠BAC = ∠PBA – ∠C
⇒ ∠1 + ∠2 = ∠PBA – ∠PAB [from (i)]
⇒ 2 ∠1 = ∠PBA – ∠PAB
⇒ ∠1 = – [∠PBA – ∠PAB]
⇒ ∠CAD = – [∠PBA – ∠PAB] Q.E.D.

Question 13.
Two circles intersect each other at points A and B. Their common tangent touches the circles v at points P and Q as shown in the figure . Show that the angles PAQ and PBQ arc supplementary. [2000]

Solution:
Given: Two circles intersect each other at A and
B. A common tangent touches the circles at P and
Q. PA. PB, QA and QB are joined.
To Prove: ∠ PAQ + ∠ PBQ = 180°
or ∠ PAQ and ∠ PBQ are supplementary.
Construction: Join AB.
Proof: PQ is the tangent and AB is the chord
∴ ∠ QPA = ∠ PBA (alternate segment) ,…(i)
Similarly we can prove that
∠ PQA = ∠ QBA ,…(ii)
Adding (i) and (ii), we get
∠ QPA + ∠ PQA = ∠ PBA + ∠ QBA
But ∠ QPA + ∠ PQA = 180° – ∠ PAQ ,…(iii) (In ∆ PAQ)
and ∠ PBA + ∠ QBA = ∠ PBQ ,…(iv)
from (iii) and (iv)
∠ PBQ = 180° – ∠ PAQ
⇒ ∠ PBQ + ∠ PAQ = 180°
= ∠ PAQ + ∠ PBQ = 180°
Hence ∠ PAQ and ∠ PBQ arc supplementary Q.E.D.

Question 14.
In the figure, chords AE and BC intersect each other at point D.
(i) If ∠ CDE = 90°.
AB = 5 cm, BD = 4 cm and CD 9 cm;
Find DE.
(ii) If AD = BD, show that AE = BC.

Solution:

Question 15.
Circles with centres P and Q intersect at points A and B as shown in the figure. CBD is a line segment and EBM is tangent to the circle, with centre Q, at point B. If the circles arc congruent; show that CE = BD.

Solution:

Given: Two circles with centre P and Q intersect each other at A and B. CBD is a line segment and EBM is tangent to the circle with centre Q, at B. Radii of the cirlces are equal.
To Prove: CE = BD
Construction: Join AB and AD.
Proof: EBM is the tangent and BD is the chord
∴ ∠ DBM = ∠ BAD (Anglesi in alt. segment)
But ∠ DBM = ∠ CBE (Vertically opposite angles)
∴ ∠ BAD = ∠ CBE
∵ In the same circle or congruent circles, if angles are equal, then chords opposite to them are also equal.
∴ CE = BD Q.E.D.

Question 16.
In the following figure O is the centre of ti.e circle and AB is a tangent to it at point B. ∠BDC = 65°, Find ∠BAO

Solution:
Given: ∠BDC = 65° and AB is tangent to circle with centre O.
∵ OB is radius
⇒ OB ⊥ AB
In ∆BDC
∠DBC + ∠BDC + ∠B CD = 180°
90° + 65° + ∠BCD =180°
⇒ ∠BCD = 25°
∵ OE = OC = radius
⇒ ∠OEC = ∠OCE
⇒ ∠OEC = 25°
Also, ∠BOE = ∠OEC + ∠OCE
[Exterior angle = sum of opposite interior angles in a ∆]
⇒ ∠BOE = 25°+ 25°
⇒∠BOE = 50°
⇒ ∠BOA = 50°
In ∆AOB
∠AOB + ∠BAO + ∠OBA = 180°
50° + ∠BAO + 90° = 180°
⇒ ∠BAO = 40°

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 18 Tangents and Intersecting Chords Ex 18B  are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere (Surface Area and Volume) Ex 20D

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21D

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20E
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20F
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20G

Question 1.
A solid sphere of radius 15 cm is melted and recast into solid right circular cones of radius 2.5 cm and height 8 cm. Calculate the number of cones recast.
Solution:

Question 2.
A hollow sphere of internal and external diameters 4 cm and 8 cm respectively is melted into a cone of base diameter 8 cm. Find the height of the cone. [2002]
Solution:
External diameter = 8cm
∴ Radius (R) = \(\frac { 8 }{ 2 }\) = 4 cm
Internal diameter = 4 cm
∴ Radius (r) = \(\frac { 4 }{ 2 }\) = 2cm.
Volume of metal used in hollow sphere

Question 3.
The radii of the internal and external surfaces of a metallic spherical shell are 3 cm and 5 cm respectively. It is melted and recast into a solid right circular cone of height 32 cm. Find the diameter of the base of the cone.
Solution:
Inner radius of spherical shell (r) = 3 cm
and outer radius (R) = 5 cm

Question 4.
Total volume of three identical cones is the same as that of a bigger cone whose height is 9 cm and diameter 40 cm. Find the radius of the base of each smaller cone, if height of each is 108 cm.
Solution:
Height of bigger cone (H) = 9 cm
Diameter 40 cm

Question 5.
A solid rectangular block of metal 49 cm by 44 cm by 18 cm is melted and formed into a solid sphere. Calculate the radius of the sphere.
Solution:
Dimensions of rectangular block of metal = 49cm x 44 cm x 18 cm.
∴ Volume = 49 x 44 x 18 cm³ = 38808 cm³
Let radius of solid sphere = r

Question 6.
A hemisphere bowl of internal radius 9 cm is full of liquid. This liquid is to be filled into conical shaped small containers each of diameter 3 cm and height 4 cm. How many containers are necessary to empty the bowl?
Solution:
Internal radius of hemispherical bowl (r) = 9 cm

Question 7.
A hemispherical bowl of diameter 7.2 cm is filled completely with chocolate sauce. This sauce is poured into an inverted cone of radius 4.8 cm. Find the height of the cone if it is completely filled. [2010]
Solution:
Diameter of hemispherical bowl = 7.2 cm

Question 8.
A solid cone of radius 5 cm and height 8 cm is melted and made into small spheres of radius 0.5 cm. Find the number of spheres formed. [2011]
Solution:
Radius of solid cone = (r) = 5 cm
and height (h) = 8 cm

Question 9.
The total area of a solid metallic sphere is 1256 cm². It is melted and recast into solid right circular cones of radius 2.5 cm and height 8 cm. Calculate:,
(i)  the radius of the solid sphere,
(ii) the number of cones recast.   Take π = 3.14              [2000]
Solution:
Total area of solid sphere = 125

Question 10.
A solid metallic cone, with radius 6 cm and height 10 cm, is made of some heavy metal A. In order to reduce its weight, a conical hole is made in the cone as shown and it is completely filled with a lighter metal B. The conical hole has a diameter of 6 cm and depth 4 cm. Calculate the ratio of the volume of metal A to the volume of the metal B in the solid.


Solution:
Radius of solid metallic cone A(R) = 6 cm
and height (H) = 10 cm

Question 11.
A hollow sphere of internal and external radii 6 cm and 8 cm respectively is melted and recast into small cones of base radius 2 cm and height 8 cm. Find the number of cones.           [2012]
Solution:
Inner radius of a hollow sphere (r) = 6 cm
and outer radius (R) = 8 cm

Question 12.
The surface area of a solid metallic sphere is 2464 cm². It is melted and recast into solid right circular cones of radius 3.5 cm and height 7 cm. Calculate:
(i) the radius of the sphere.
(ii) the number of cones recast. (Take π = 22/7)
Solution:
(i) Surface area=4πr²=2464 cm² (given)

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapterwise Revision Exercises

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapterwise Revision Exercises.

Sales-Tax (VAT) Chapterwise Revision Exercises

Question 1.
A man purchased a pair of shoes for ₹809.60 which includes 8% rebate on the marked price and then 10% sales tax on the remaining price. Find the marked price of the pair of shoes.
Solution:

Question 2.
The catalogue price of an article is ₹36,000. The shopkeeper gives two successive discounts of 10% each. He further gives an off-season discount of 5 % on the balance. If sales-tax at the rate 10% is charged on the remaining amount, find :
(i) the sales tax charged
(ii) the selling price of the article including sales-tax.
Solution:

Question 3.
A sells an article to B for ₹80,000 and charges sales-tax at 8%. B sells the same article to C for ₹1,12,000 and charges sales- tax at the rate of 12%. Find the VAT paid by B in this transaction.
Solution:

Question 4.
The marked price of an article is ₹1,600. Mohan buys this article at 20% discount and sells it at its marked price. If the sales-tax at each stage is 6%; find :
(i) the price at which the article can be bought,
(ii) the VAT (value added tax) paid by Mohan.
Solution:

Question 5.
A sells an old laptop to B for ₹12,600; B sells it to C for ₹14,000 and C sells the same laptop to D for ₹16,000.
If the rate of VAT at each stage is 10%, find the VAT paid by :
(i) B
(ii) C
Solution:

Banking Chapterwise Revision Exercises

Question 6.
Ashok deposits ₹3200 per month in a cumulative account for 3 years at the rate of 9% per annum. Find the maturity value of this account
Solution:

Question 7.
Mrs. Kama has a recurring deposit account in Punjab National Bank for 3 years at 8% p.a. If she gets ₹9,990 as interest at the time of maturity, find:
(i) the monthly instalment.
(ii) the maturity value of the account.
Solution:

Question 8.
A man has a 5 year recurring deposit account in a bank and deposits ₹240 per month. If he receives ₹17,694 at the time of maturity, find the rate of interest.
Solution:

Question 9.
Sheela has a recurring deposit account in a bank of ₹2,000 per month at the rate of 10% per anum. If she gets ₹83,100 at the time of maturity, find the total time (in years) for which the account was held.
Solution:

Question 10.
A man deposits ₹900 per month in a recurring account for 2 years. If he gets 1,800 as interest at the time of maturity, find the rate of interest .
Solution:

Shares And Dividend Chapterwise Revision Exercises

Question 11.
What is the market value of 4 \(\frac { 1 }{ 2 }\) % (₹100) share, when an investment of ₹1,800 produces an income of ₹72 ?
Solution:

Question 12.
By investing ₹10,000 in the shares of a company, a man gets an income of ₹800; the dividend being 10%. If the face-value of each share is ₹100, find :
(i) the market value of each share.
(ii) the rate per cent which the person earns on his investment.
Solution:

Question 13.
A man holds 800 shares of ₹100 each of a company paying 7.5% dividend semiannually.
(i) Calculate his annual dividend.
(ii) If he had bought these shares at 40% premium, what percentage return does he get on his investment ?
Solution:

Question 14.
A man invests ₹10,560 in a company, paying 9% dividend, at the time when its ₹100 shares can be bought at a premium of ₹32. Find:
(i) the number of shares bought by him;
(ii) his annual income from these shares and
(iii) the rate of return on his investment .
Solution:

Question 15.
Find the market value of 12% ₹25 shares of a company which pays a dividend of ₹1,875 on an investment of ₹20,000.
Solution:

Linear Inequations Chapterwise Revision Exercises

Question 16.
The given diagram represents two sets A and B on real number lines.

(i) Write down A and B in set builder notation.
(ii) Represent A ∪ B, A ∩ B, A’ ∩ B, A – B and B – A on separate number lines.
Solution:

Question 17.
Find the value of x, which satisfy the inequation:

Graph the solution set on the real number line .
Solution:

Question 18.
State for each of the following statements whether it is true or false :
(a) If (x – a) (x – b) < 0, then x < a, and x < b.
(b) If a < 0 and b < 0, then (a + b)² > 0.
(c) If a and b are any two integers such that a > b, then a² > b².
(d) If p = q + 2, then p > q.
(e) If a and b are two negative integers such that a < b , then \(\frac { 1 }{ a }\) < \(\frac { 1 }{ b }\)
Solution:

Question 19.
Given 20 – 5x < 5(x + 8), find the smallest value of x when :
(i) x \(\epsilon\) I
(ii) x \(\epsilon\) W
(iii) x \(\epsilon\) N
Solution:

Question 20.
If x \(\epsilon\) Z, solve : 2 + 4x < 2x – 5 < 3x. Also, represent its solution on the real number line.
Solution:

Quadratic Equation Chapterwise Revision Exercises

Question 21.

Solution:

Question 22.

Solution:

Question 23.
Find the value of k for which the roots of the following equation are real and equal k²x² – 2 (2k -1) x + 4 = 0
Solution:

Question 24.

Solution:

Question 25.
If -5 is a root of the quadratic equation 2x² +px -15 = 0 and the quadratic equation p(x² + x) + k = 0 has equal roots, find the value of k.
Solution:

Problems On Quadratic Equations Chapterwise Revision Exercises

Question 26.
x articles are bought at ₹(x – 8) each and (x – 2) some other articles are bought at ₹(x – 3) each. If the total cost of all these articles is ₹76, how many articles of first kind were bought ?
Solution:

Question 27.
In a two digit number, the unit’s digit exceeds its ten’s digit by 2. The product of the given number and the sum of its digits is equal to 144. Find the number.
Solution:

Question 28.
The time taken by a person to cover 150 km was 2.5 hours more than the time taken in return journey. If he returned at a speed of 10 km/hour more than the speed of going, what was the speed per hour in each direction ?
Solution:

Question 29.
A takes 9 days more than B to do a certain piece of work. Together they can do the work in 6 days. How many days will A alone take to do the work ?
Solution:

Question 30.
A man bought a certain number of chairs for ₹10,000. He kept one for his own use and sold the rest at the rate ₹50 more than he gave for one chair. Besides getting his own chair for nothing, he made a profit of ₹450. How many chairs did he buy ?
Solution:

Question 31.
In the given figure; the area of unshaded portion is 75% of the area of the shaded portion. Find the value of x.

Solution:

Ratio And Proportion Chapterwise Revision Exercises

Question 32.

Solution:

Question 33.
If a:b = 2:3,b:c = 4:5 and c: d = 6:7, find :a:b :c :d.
Solution:

Question 34.

Solution:

Question 35.
Find the compound ratio of:
(i) (a-b) : (a+b) and (b²+ab): (a²-ab)
(ii) (x+y): (x-y); (x²+y²): (x+y)² and (x²-y²)²: (x⁴-y⁴)
(iii) (x²– 25): (x²+ 3x – 10); (x²-4): (x²+ 3x+2) and (x + 1): (x² + 2x)
Solution:

Question 36.
The ratio of the prices of two fans was 16: 23. Two years later, when the price of the first fan had risen by 10% and that of the second by Rs. 477, the ratio of their prices became 11: 20. Find the original prices of two fans.
Solution:
The ratio of prices of two fans = 16 : 23
Let the price of first fan = 6x
then price of second fan = 23x

Remainder And Factor Theorems Chapterwise Revision Exercises

Question 37.
Given that x + 2 and x – 3 are the factors of x³ + ax + b, calculate the values of a and b. Also find the remaining factor.
Solution:

Question 38.
Use the remainder theorem to factorise the expression 2x³ + 9x² + 7x – 6 = 0 Hense, solve the equation 2x³ + 9x² + 7 x – 6 = 0
Solution:

Question 39.
When 2x³ + 5x² – 2x + 8 is divided by (x – a) the remainder is 2a³ + 5a². Find the value of a.
Solution:

Question 40.
What number should be added to x³ – 9x² – 2x + 3 so that the remainder may be 5 when divided by (x – 2) ?
Solution:

Question 41.
Let R₁ and R₂ are remainders when the polynomials x³ + 2x² – 5ax – 7 and x³ + ax² – 12x + 6 are divided by (x +1) and (x – 2) respectively. If 2R₁ + R₂ = 6; find the value of a.
Solution:

Matrices Chapterwise Revision Exercises

Question 42.

Solution:

Question 43.

Solution:

Question 44.

Solution:

Question 45.

Solution:

Arithmetic Progression (A.P.) Chapterwise Revision Exercises

Question 46.
Find the 15th term of the A.P. with second term 11 and common difference 9.
Solution:

Question 47.
How many three digit numbers are divisible by 7 ?
Solution:

Question 48.
Find the sum of terms of the A.P.: 4,9,14,…….,89.
Solution:

Question 49.
Daya gets pocket money from his father every day. Out of the pocket money, he saves ₹2.75 on first day, ₹3.00 on second day, ₹3.25 on third day and so on. Find:
(i) the amount saved by Daya on 14th day.
(ii) the amount saved by Daya on 30th day.
(iii) the total amount saved by him in 30 days.
Solution:

Question 50.
If the sum of first m terms of an A.P. is n and sum of first n terms of the same A.P. is m. Show that sum of first (m + n) terms of it is (m + n).
Solution:

Geometric Progression (GP) Chapterwise Revision Exercises

Question 51.
3^rd term of a GP. is 27 and its 6th term is 729; find the product of its first and 7^th terms.
Solution:

Question 52.
Find 5 geometric means between 1 and 27.
Solution:

Question 53.
Find the sum of the sequence 96 – 48 + 24…. upto 10 terms.
Solution:

Question 54.
Find the sum of first n terms of:
(i) 4 + 44 + 444 + …….
(ii) 0.7 + 0.77 + 0.777 + …..
Solution:

Question 55.
Find the value of 0.4.
Solution:

Reflection Chapterwise Revision Exercises

Question 56.
Find the values of m and n in each case if:
(i) (4, -3) on reflection in x-axis gives (-m, n)
(ii) (m, 5) on reflection in y-axis gives (-5, n-2)
(iii) (-6, n+2) on reflection in origin gives (m+3, -4)
Solution:

Question 57.
Points A and B have the co-ordinates (-2,4) and (-4,1) respectively. Find :
(i) The co-ordinates of A’, the image of A in the line x = 0.
(ii) The co-ordinates of B’, the image of Bin y-axis.
(iii) The co-ordinates of A”, the image of A in the line BB’,
Hence, write the angle between, the lines A’A” and B B’. Assign a special name to the figure B’ A’ B A”
Solution:

Question 58.
Triangle OA₁B₁ is the reflection of triangle OAB in origin, where A, (4, -5) is the image of A and B, (-7, 0) is the image of B.
(i) Write down the co-ordinates of A and B and draw a diagram to represent this information.
(ii) Give the special name to the quadrilateral ABA₁ B₁. Give reason.
(iii) Find the co-ordinates of A₂, the image of A under reflection in x-axis followed by reflection in y-axis.
(iv) Find the co-ordinates of B₂, the image of B under reflection in y-axis followed by reflection in origin.
(v) Does the quadrilateral obtained has any line symmetry ? Give reason.
(vi) Does it have any point symmetry ?
Solution:

Section And Mid-Point Formulae Chapterwise Revision Exercises

Question 59.
In what ratio does the point M (P, -1) divide the line segment joining the points A (1,-3) and B (6,2) ? Hence, find the value of p.
Solution:

Question 60.
A (-4,4), B (x, -1) and C (6,y) are the vertices of ∆ABC. If the centroid of this triangle ABC is at the origin, find the values of x and y.
Solution:

Question 61.
A (2,5), B (-1,2) and C (5,8) are the vertices of a triangle ABC. Pand Q are points on AB and AC respectively such that AP: PB=AQ: QC = 1:2.
(a) Find the co-ordinates of points P and Q
(b) Show that BC = 3 x PQ.
Solution:

Question 62.
Show that the points (a, b), (a+3, b+4), (a -1, b + 7) and (a – 4, b + 3) are the vertices of a parallelogram.
Solution:

Equation Of straight Line Chapterwise Revision Exercises

Question 63.
Given points A(l, 5), B (-3,7) and C (15,9).
(i) Find the equation of a line passing through the mid-point of AC and the point B.
(ii) Find the equation of the line through C and parallel to AB.
(iii) The lines obtained in parts (i) and (ii) above, intersect each other at a point P. Find the co-ordinates of the point P.
(iv) Assign, giving reason, a special names of the figure PABC.
Solution:

Question 64.
The line x- 4y=6 is the perpendicular bisector of the line segment AB. If B = (1,3); find the co-ordinates of point A.
Solution:

Question 65.
Find the equation of a line passing through the points (7, -3) and (2, -2). If this line meets x- axis at point P and y-axis at point Q; find the co-ordinates of points P and Q.
Solution:

Question 66.
A (-3,1), B (4,4) and C (1, -2) are the vertices of a triangle ABC. Find:
(i) the equation of median BD,
(ii) the equation of altitude AE.
Solution:

Question 67.
Find the equation of perpendicular bisector of the line segment joining the points (4, -3) and (3,1).
Solution:

Question 68.
(a) If (p +1) x + y = 3 and 3y – (p -1) x = 4 are perpendicular to each other find the value of p.
(b) If y + (2p +1) x + 3 = 0 and 8y – (2p -1) x = 5 are mutually prependicular, find the value of p.
Solution:

Question 69.
The co-ordinates of the vertex A of a square ABCD are (1, 2) and the equation of the diagonal BD is x + 2y = 10. Find the equation of the other diagonal and the coordinates of the centre of the square.
Solution:

Similarity Chapterwise Revision Exercises

Question 70.
M is mid-point of a line segment AB; AXB and MYB are equilateral triangles on opposite sides of AB; XY cuts AB at Z. Prove that AZ = 2ZB.
Solution:

Question 71.
In the given figure, if AC = 3cm and CB = 6 cm, find the length of CR.

Solution:

Question 72.
The given figure shows a trapezium in which AB is parallel to DC and diagonals AC and BD intersect at point O. If BO : OD = 4:7; find:
(i) ∆AOD : ∆AOB
(ii) ∆AOB : ∆ACB
(iii) ∆DOC : ∆AOB
(iv) ∆ABD : ∆BOC
Solution:

Question 73.
A model of a ship is made to a scale of 1 : 160. Find :
(i) the length of the ship, if the length of its model is 1.2 m.
(ii) the area of the deck of the ship, if the area of the deck of its model is 1.2 m².
(iii) the volume of the ship, if the volume of its model is 1.2m³.
Solution:

Question 74.
In trapezium ABCD, AB || DC and DC = 2 AB. EF, drawn parallel to AB cuts AD in F and BC in E such that 4 BE = 3 EC. Diagonal DB intersects FE at point G Prove that: 7 EF = 10 AB.

Solution:

Loci Chapterwise Revision Exercises

Question 75.
In triangle ABC, D is mid-point of AB and CD is perpendicular to AB. Bisector of ∠ABC meets CD at E and AC at F. Prove that:
(i) E is equidistant from A and B.
(ii) F is equidistant from AB and BC.
Solution:

Question 76.
Use graph paper for this questions. Take 2 cm = 1 unit on both axes.
(i) Plot the points A (1,1), B (5,3) and C (2,7)
(ii) Construct the locus of points equidistant from A and B.
(iii) Construct the locus of points equidistant from AB and AC.
(iv) Locate the point P such that PA = PB and P is equidistant from AB and AC.
(v) Measure and record the length PA in cm.
Solution:

Circles Chapterwise Revision Exercises

Question 77.
In the given figure, ∠ADC = 130° and BC = BE. Find ∠CBE if AB ⊥ CE.

Solution:

Question 78.
In the given figure, ∠OAB=30° and ∠OCB= 57°, find ∠BOC and ∠AOC.

Solution:

Question 79.
In the given figure, O is the centre of the circle. If chord AB = chord AC, OP⊥ AB and OQ⊥ AC; show that: PB=QC.
Solution:

Question 80.
In the given figure, AB and XY are diameters of a circle with centre O. If ∠APX=30°, find:
(i) ∠AOX
(ii) ∠APY
(iii) ∠BPY
(iv) ∠OAX

Solution:

Question 81.
(a) In the adjoining figure; AB = AD, BD = CD and ∠DBC = 2 ∠ABD.
Prove that: ABCD is a cyclic quadrilateral.

(b) AB is a diameter of a circle with centre O, Chord CD is equal to radius OC. AC and BD produced intersect at P. Prove that ∠APB = 60°.

Solution:

Tangents And Intersecting Chords Chapterwise Revision Exercises

Question 82.
In the given figure, AC=AB and ∠ABC=72°.
OA and OB are two tangents. Determine:
(i) ∠AOB
(ii) angle subtended by the chord AB at the centre.

Solution:

Question 83.
In the given figure, PQ, PR and ST are tangents to the same circle. If ∠P = 40° and ∠QRT = 75°, find a, b and c.

Solution:

Question 84.
In the given figure, ∠ABC = 90° and BC is diameter of the given circle. Show that:
(i) AC x AD =AB²
(ii) AC x CD = BC²

Solution:

Question 85.
In the given figure; AB, BC and CA are tangents to the given circle. If AB = 12 cm, BC = 8 cm and AC=10 cm, find the lengths of AD,BE = CF.

Solution:

Question 86.
(a) AB and CD are two chords of a circle intersecting at a point P inside the circle. If:
(i) AB = 24 cm, AP = 4 cm and PD = 8 cm, determine CP.
(ii) AP = 3 cm, PB = 2.5 cm and CD = 6.5 cm determine CP.
(b) AB and CD are two chords of a circle intersecting at a point P outside the circle. If:
(i) PA = 8 cm, PC – 5 cm and PD = 4 cm, determine AB.
(ii) PC = 30 cm, CD = 14 cm and PA = 24 cm, determine AB.
Solution:

Construction Chapterwise Revision Exercises

Question 87.
Construct a triangle ABC in which AC = 5 cm, BC = 7 cm and AB = 6 cm.
(i) Mark D, the mid point of AB.
(ii) Construct a circle which touches BC at C and passes through D.
Solution:

Question 88.
Using ruler and compasses only, draw a circle of radius 4 cm. Produce AB, a diameter of this circle up to point X so that BX = 4cm. Construct a circle to touch AB at X and to touch the circle, drawn earlier externally.
Solution:

Mensuration Chapterwise Revision Exercises

Question 89.
A cylindrical bucket 28 cm in diameter and 72 cm high is full of water. The water is emptied into a rectangular tank 66 cm long and 28 cm wide. Find the height of the water level in the tank.
Solution:

Question 90.
A tent is of the shape of right circular cylinder upto height of 3 metres and then becomes a right circular cone with a maximum height of 13.5 metres above the ground. Calculate the cost of painting the inner surface of the tent at Rs. 4 per sq. metre, if the radius of the base is 14 metres.
Solution:

Question P.Q.
In the given figure, diameter of the biggest semi-circle is 108cm, and diameter of the smallest circle is 36 cm. Calculate the area of the shaded portion.

Solution:

Question 91.
A copper wire of diameter 6 mm is evenly wrapped on the cylinder of length 18 cm and diameter 49 cm to cover the whole surface. Find:
(i) the length
(ii) the volume of the wire
Solution:

Question 92.
A pool has a uniform circular cross-section of radius 5 m and uniform depth 1.4m. It is filled by a pipe which delivers water at the rate of 20 litres per sec. Calculate, in minutes, the time taken to All the pool. If the pool is emptied in 42 min. by another cylindrical pipe through which water flows at 2 m per sec, calculate the radius of the pipe in cm.
Solution:

Question 93.
A test tube consists of a hemisphere and a cylinder of the same radius. The volume of water required to fill the whole tube is 2849/3cm³ and 2618/3cm³ of water are required to fill the tube to a level which is 2 cm below the top of the tube. Find the radius of the tube and the length of its cylinderical part.
Solution:

Question 94.
A sphere is placed in an inverted hollow conical vessel of base radius 5 cm and vertical height 12 cm. If the highest point of the sphere is at the level of the base of the cone, find the radius of the sphere. Show that the volume of the sphere and the conical vessel are as 40 : 81.
Solution:

Question 95.
The difference between the outer and the inner curved surface areas of a hollow cylinder, 14cm. long is 88sq. cm. Find the outer and the inner radii of the cylinder given that the volume of metal used is 176 cu. cm.
Solution:

Trigonometry Chapterwise Revision Exercises

Question 96.

Solution:

Question 97.
If tan A = 1 and tan B = √3 ; evaluate :
(i) cos A cos B – sin A sin B
(ii) sin A cos B + cos A sin B
Solution:

Question 98.
As observed from the top of a 100 m high light house, the angles, of depression of two ships approaching it are 30° and 45°. If one ship is directly behind the other, find the distance between the two ships.
Solution:

Question 99.

Solution:

Question 100.
From the top of a light house, it is observed that a ship is sailing directly towards it and the angle of depression of the ship changes from 30° to 45° in 10 minutes. Assuming that the ship is sailing with uniform speed; calculate in how much more time (in minutes) will the ship reach to the light house.
Solution:
Let LM be the height of light house = h
Angle of depression changes from 30° to 45° in 10 minutes.

Statistics Chapterwise Revision Exercises

Question 101.
Calculate the mean mark in the distribution given below :

Also state (i) median class (ii) the modal class.
Solution:

Question 102.
Draw an ogive for the following distribution :

Use the ogive drawn to determine :
(i) the median income,
(ii) the number of employees whose income exceeds Rs. 190.
Solution:

Question 103.
The result of an examination are tabulated below :

Draw the ogive for above data and from it determine :
(i) the number of candidates who got marks less than 45.
(ii) the number of candidates who got marks more than 75.
Solution:

Probability Chapterwise Revision Exercises

Question 104.
A bag contains a red ball, a blue ball and a yellow ball, all the balls being of the same size. If a ball is drawn from the bag, without looking into it, find the probability that the ball drawn is
(i) yellow
(ii) red
(iii) blue
Solution:

Question 105.
A bag contains 6 red balls, 8 blue balls and 10 yellow balls, all the balls being of the same size. If a ball is drawn from the bag, without looking into it, find the probability that the ball drawn is
(i) yellow
(ii) red
(iii) blue
(iv) not yellow
(v) not blue
Solution:

Question 106.
Two dice are thrown at the same time. Write down all the possible outcomes. Find the probability of getting the sum of two numbers appearing on the top of the dice as :
(i) 13
(ii) less than 13
(iii) 10
(iv) less then 10
Solution:

Question 107.
Five cards : the ten, jack, queen, king and ace. of diamonds are well-shuffled with their face downwards. One card is then picked up at random.
(i) What is the probability that the card is the queen ?
(ii) If the queen is drawn and put aside, what is the probability that the second card picked up is (a) an ace ? (b) a queen ?
Solution:

Question 108.
(i) A lot of 20 bulbs contains 4 defective bulbs, one bulb is drawn at random, from the lot. What is the probability that this bulb is defective ?
(ii) Suppose the bulb drawn in (i) is not defective and is not replaced. Now one bulb is drawn at random from the rest. What is the probability that this bulb is not defective?
Solution:

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapterwise Revision Exercises  are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

 

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere (Surface Area and Volume) Ex 20C

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21C

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20E
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20F
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20G

Question 1.
The surface area of a sphere is 2464 cm², find its volume.
Solution:
Surface area of sphere = 2464 cm²
Let radius = r

Question 2.
The volume of a sphere is 38808 cm³; find its diameter and the surface area.
Solution:
Volume of sphere = 38808 cm³
Let radius of shpere = r

Question 3.
A spherical ball of lead has been melted and made into identical smaller balls with radius equal to half the radius of the original one. How many such balls can be made ?
Solution:
Let the radius of spherical ball = r

Question 4.
How many balls each of radius 1 cm can be made by melting a bigger ball whose diameter is 8 cm.
Solution:

Question 5.
Eight Metallic sphere; each of radius 2 mm, are melted and cast into a single sphere. Calculate the radius of the new sphere.
Solution:
Radius of metallic sphere = 2mm = \(\frac { 1 }{ 5 }\) cm

Question 6.
The volume of one sphere is 27 times that of another sphere. Calculate the ratio of their:
(i) radii,
(ii) surface areas.
Solution:
Volume of first sphere = 27 x volume of second sphere.
Let radius of first sphere = r₁
and radius of second sphere = r₂

Question 7.
If the number of square centimetres on the surface of a sphere is equal to the number of cubic centimetres in its volume, what is the diameter of the sphere ?
Solution:

Question 8.
A solid metal sphere is cut through its centre into 2 equal parts. If the diameter of the sphere is 3\(\frac { 1 }{ 2 }\) cm, find the total surface area of each part correct to two decimal places.
Solution:
A solid sphere is cut into two equal hemispheres.

Question 9.
The internal and external diameters of a hol­low spectively. Find:
(i) internal curved suface area,
(ii) external curved surface area,
(iii) total surface area,
(iv) volume of material of the vessel.
Solution:
Internal diameter of hollow hemispher = 21cm
and external diameter = 28 cm

Question 10.
A solid sphere and a solid hemi-sphere have the same total surface area. Find the ratio between their volumes.
Solution:
Let radius of a sphere = R
∴  Surface area = 4πR²
and radius of hemi-sphere = r
∴ Surface area = 3πr²
∵ Their surface area are equal
4πR² = 3πr² ⇒ 4R² = 3r²

Question 11.
Metallic spheres of radii 6 cm, 8 cm and 10 cm respectively are melted and recasted into a single solid sphere. Taking π = 3.1, find the surface area of solid sphere formed.
Solution:
Radius of first sphere (r₁) = 6 cm
Radius of second sphere (r₂) = 8 cm
Radius of third sphere (r₃) = 10 cm

Question 12.
The surface area of a solid sphere is increased by 21% without changing its shape. Find the percentage increase in its:
(i) radius                            
(ii) volume
Solution:
(i)  Let r be the radius of the solid sphere then surface area = 4πr²
Increase in area = 21%

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 20 Cylinder, Cone and Sphere Ex 20C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.