Category: Uncategorized

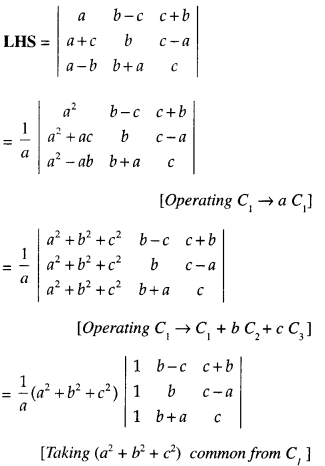
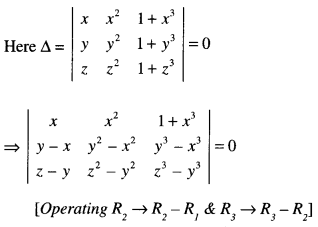
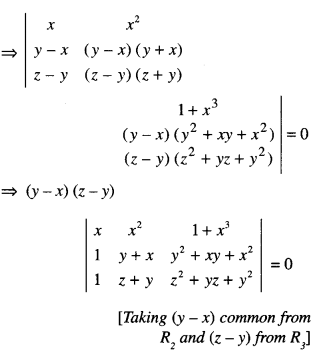
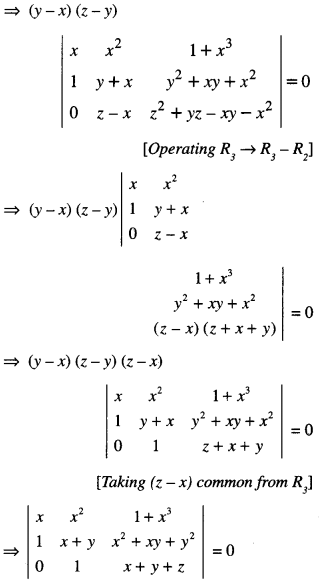
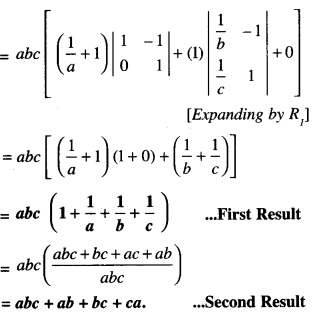
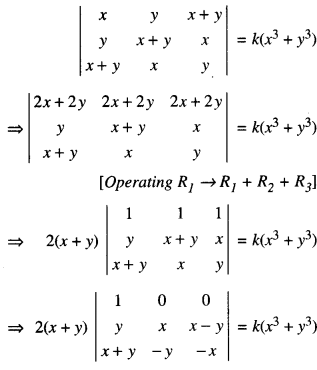
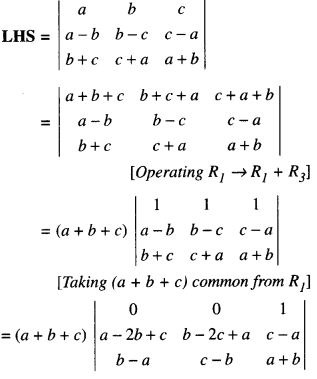
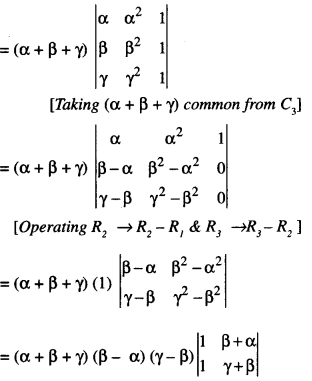
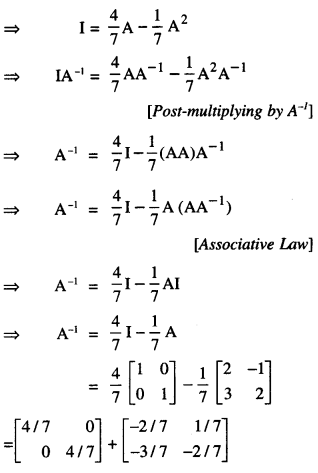
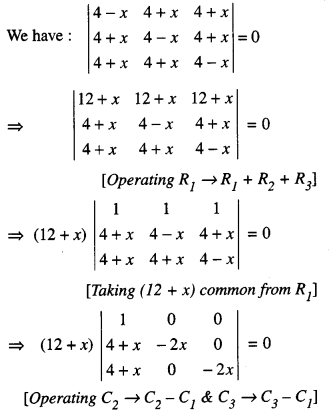
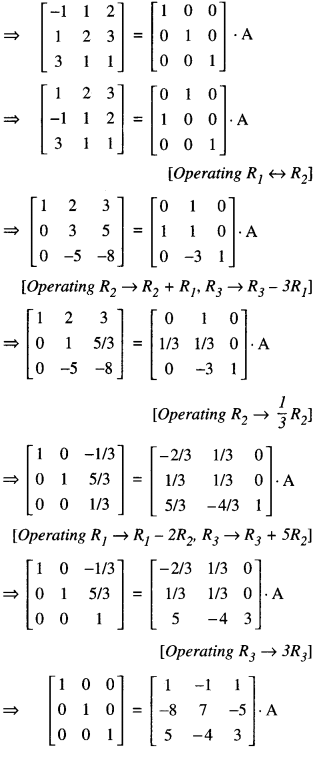
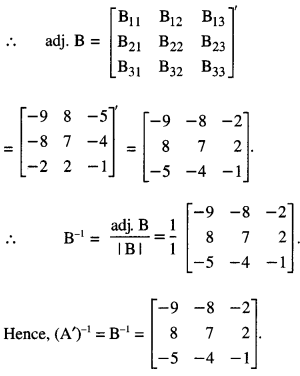
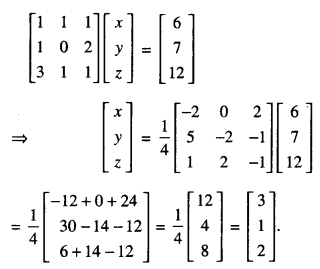
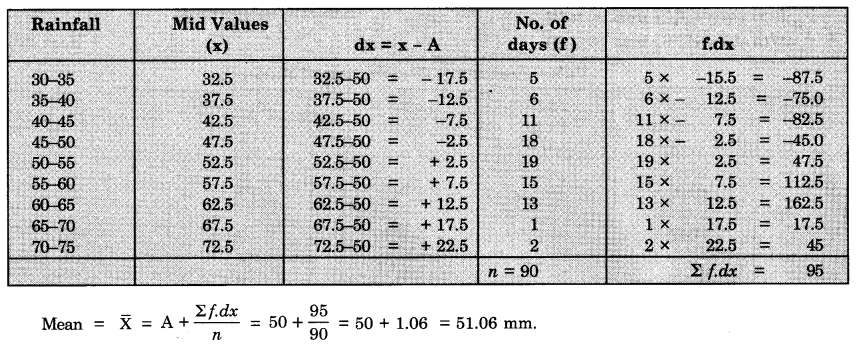
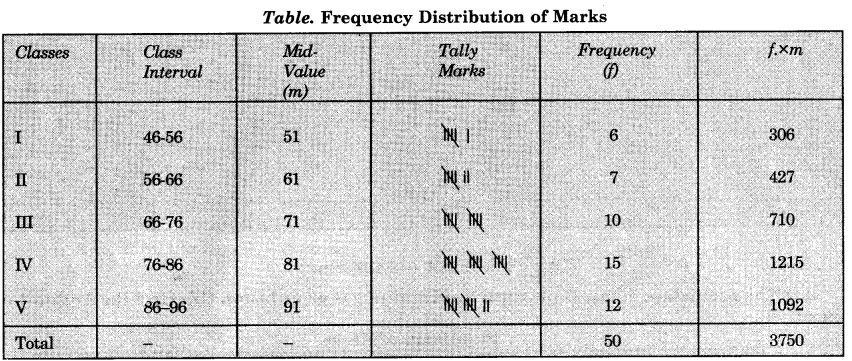
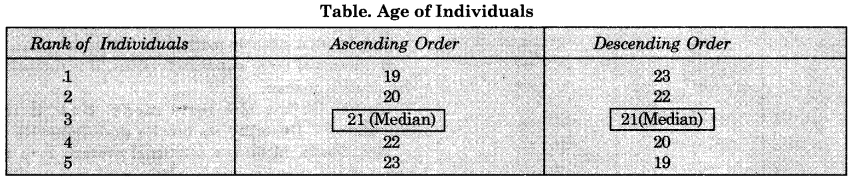
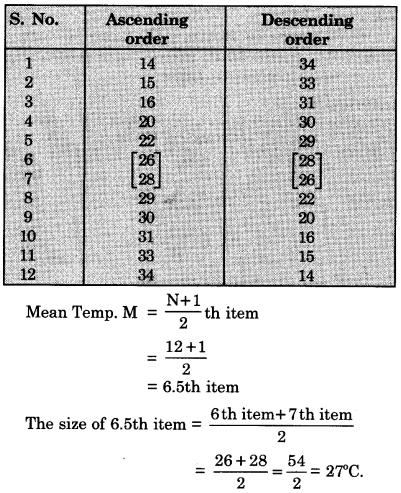
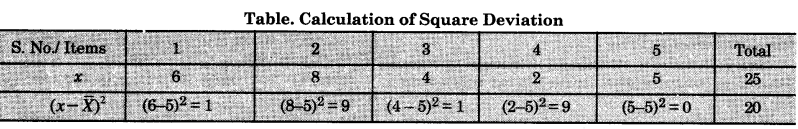
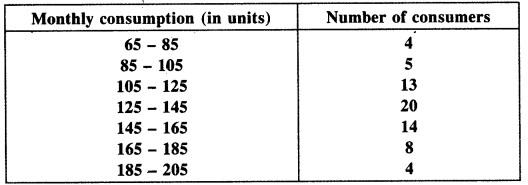
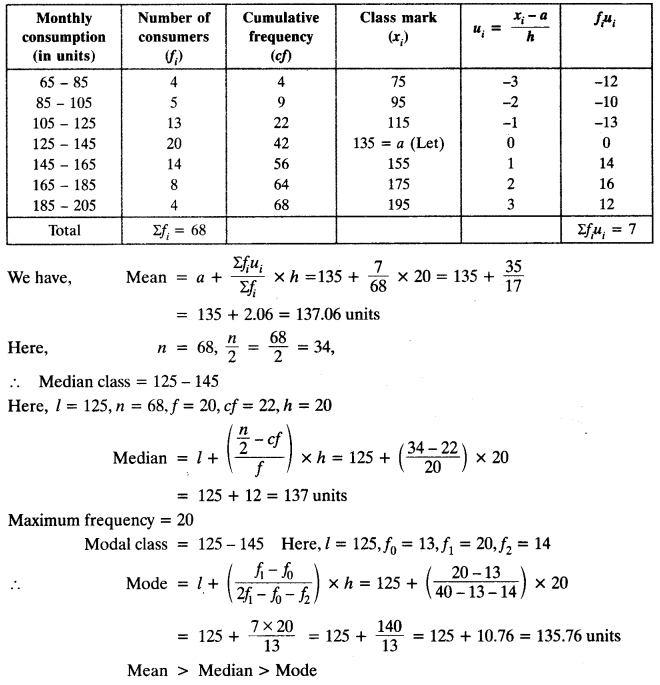
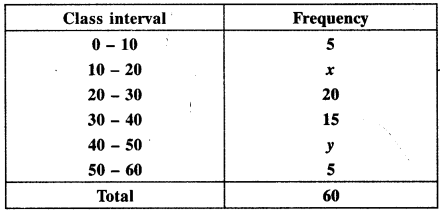
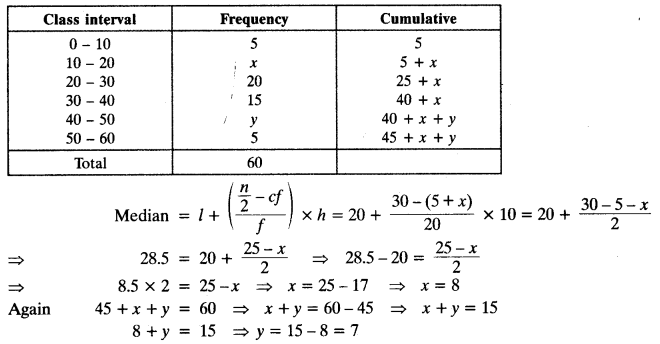
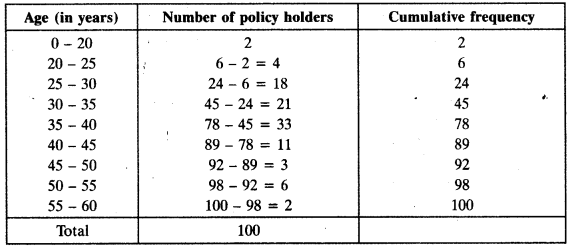
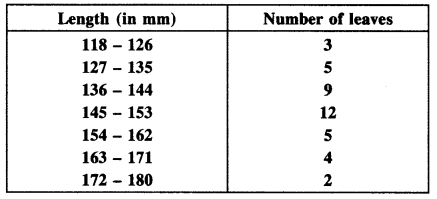
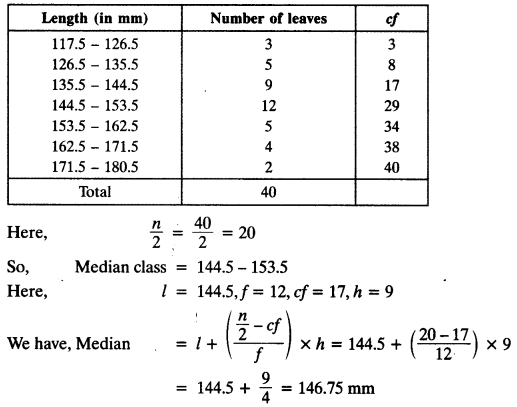
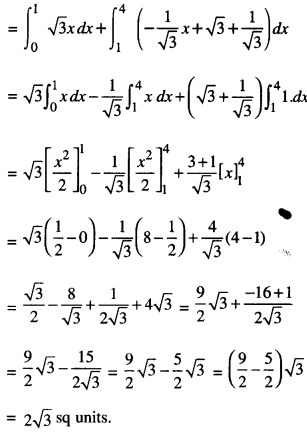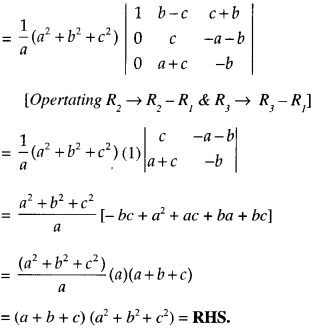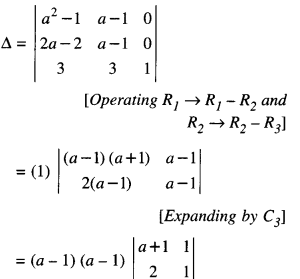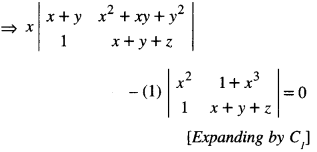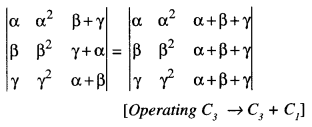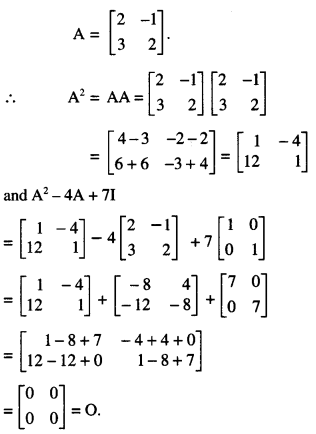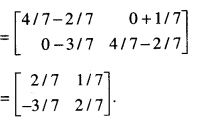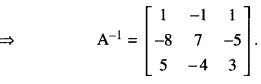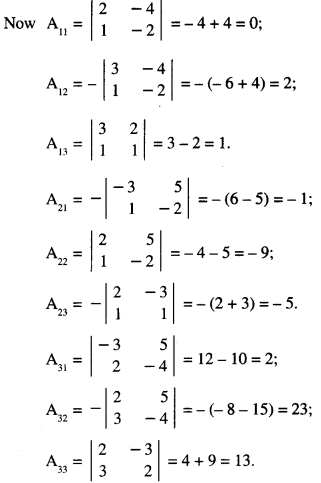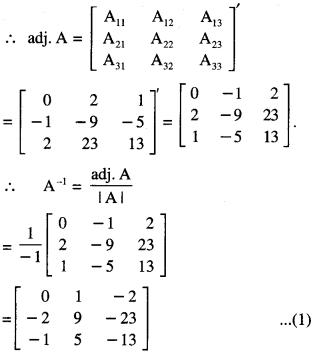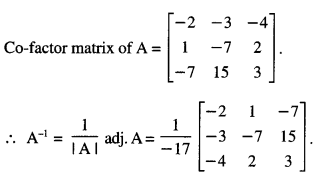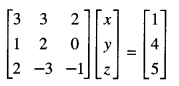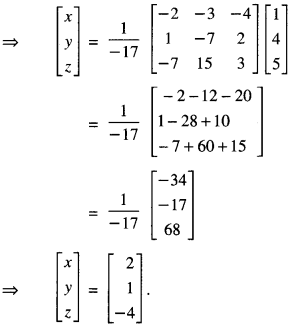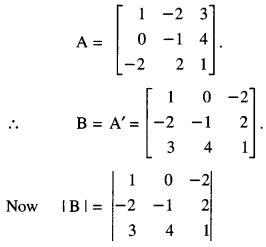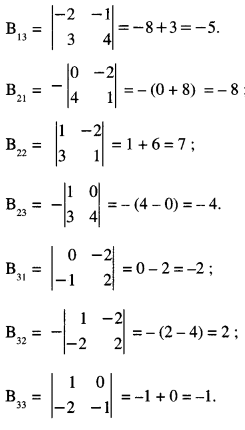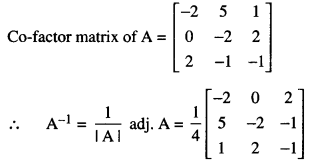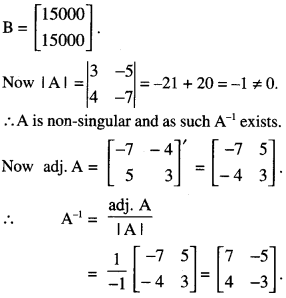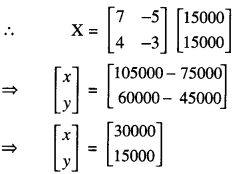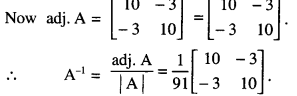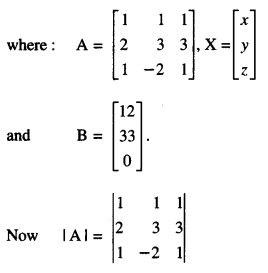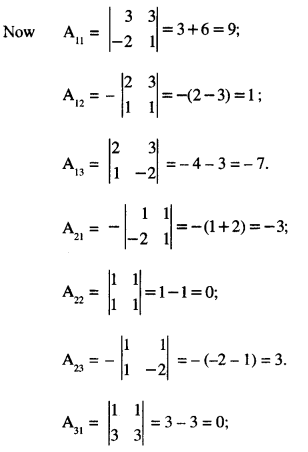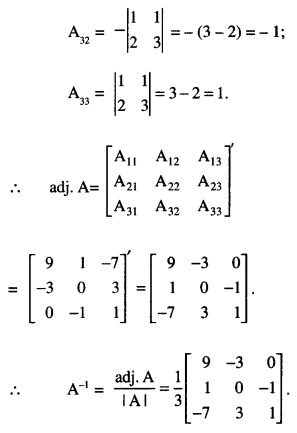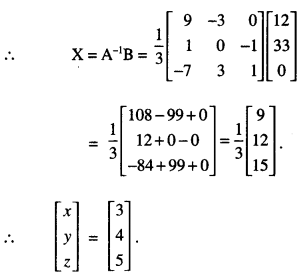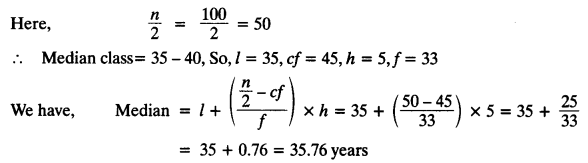Here we are providing Class 12 Maths Important Extra Questions and Answers Chapter 8 Applications of the Integrals. Class 12 Maths Important Questions are the best resource for students which helps in Class 12 board exams.

Class 12 Maths Chapter 8 Important Extra Questions Applications of the Integrals

Applications of the Integrals Important Extra Questions Very Short Answer Type

Question 1.
Find the area of region bounded by the curve y = x² and the line y = 4.
Answer:
\(\frac { 32 }{ 2 }\) sq. units.

Question 2.
Find the area bounded by the curve y = x³, x = 0 and the ordinates x = -2 and x = 1.
Answer:
\(\frac { 17 }{ 4 }\) sq. units.

Question 3.
Find the area bounded between parabolas y² = 4x and x² = 4y.
Answer:
\(\frac { 16 }{ 3 }\) sq. units.

Question 4.
Find the area enclosed between the curve y = cos x, 0 ≤ x ≤ \(\frac{\pi}{4}\) and the co-ordinate axes.
Answer:
\(\frac { 1 }{ 2 }\) sq. units.

Question 5.
Find the area between the x-axis curve y = cos x when 0≤ x < 2.
Answer:
4 sq. units

Question 6.
Find the ratio of the areas between the centre y = cos x and y = cos 2x and x-axis for x = 0 to
x = \(\frac{\pi}{3}\)
Answer:
2:1.

Question 7.
Find the areas of the region:
{(x,y): x² + y² ≤ 1 ≤ x + 4}
Ans.
\(\frac{1}{2}\) (π – 1) sq. units.

Applications of the Integrals Important Extra Questions Long Answer Type 2

Question 1.
Find the area enclosed by the circle:
x² + y² = a². (N.C.E.R.T.)
Solution:
The given circle is
x² + y² = a² ………….(1)
This is a circle whose centre is (0,0) and radius ‘a’.

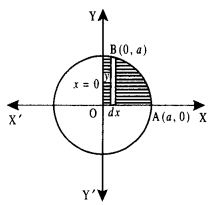
Area of the circle=4 x (area of the region OABO, bounded by the curve, x-axis and ordinates x = 0, x = a)
[ ∵ Circle is symmetrical about both the axes]
= 4 \(\int_{0}^{a}\) ydx [Taking vertical strips] o
= \(4 \int_{0}^{a} \sqrt{a^{2}-x^{2}} d x\)
[ ∵ (1) ⇒ y = ± \(\sqrt{a^{2}-x^{2}}\)
But region OABO lies in 1st quadrant, ∴ y is + ve]

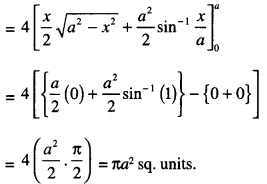
Question 2.
Using integration, find the area of the region in the first quadrant enclosed by the x-axis, the liney = x and the circle x² + y² = 32. (C.B.S.E. 2018)
Solution:
We have :
y = x …(l)
and x² + y² = 32 …(2)
(1) is a st. line, passing through (0,0) and (2) is a circle with centre (0,0) and radius 4√2 units. Solving (1) and (2) :
Putting the value of y from (1) in (2), we get:
x² + x² = 32
2x² = 32
x² = 16
x = 4.
[∵ region lies in first quadrant]
Also y = 4
Thus the line (1) and the circle (2) meet each other at B (4,4), in the first quadrant.
Draw BM perp. to x – axis.

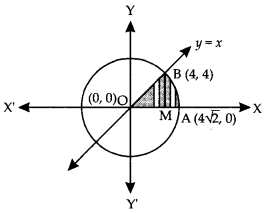
∴ Reqd. area = area of the region OMBO + area of the region BMAB …(3)
Now, area of the region OMBO

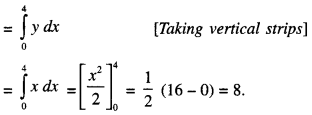
Again, area of the region BMAB

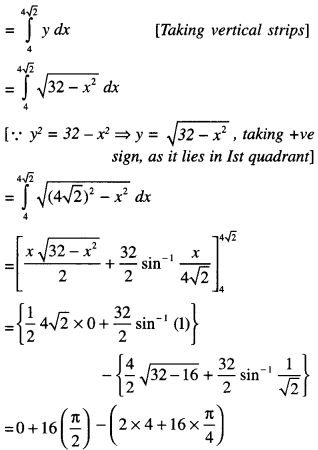
= 8π – (8 + 4π) = 4π – 8
∴ From (3),
Required area = 8 + (4π – 8) = 4π sq.units.

Question 3.
Find the area bounded by the curves y = √x , 2y + 3 = Y and Y-axis. (C.B.S.E. Sample Paper 2018-19)
Solution:
The given curves are
y = √x ………….(1)
and 2y + 3 = x …(2)
Solving (1) and (2), we get;
\(\sqrt{2 y+3}\) = y
Squaring, 2y + 3 = y²
⇒ y²2 – 2y – 3 = 0
⇒ (y + 1)(y-3) = 0 ⇒ y = -1, 3
⇒ y = 3 [∵ y > 0]
Putting in (2),
x = 2(3) + 3 = 9.
Thus, (1) and (2) intersects at (9, 3).

Question 4.
Find the area of region:
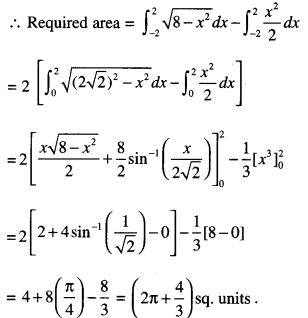
{(x,y): x² + y² < 8, x² < 2y}. (C.B.S.E. Sample Paper 2018-19)
Solution:
The given curves are ;
x² + y² = 8 ………… (1)
x² = 2y ………… (2)

Solving (1) and (2):
8 – y²= 2y
⇒ y² + 2y – 8 = 0
⇒ (y + 4)(y – 2) = 0
= y = -4,2
⇒ y = 2. [∵ y > 0]
Putting in (2), x² = 4
⇒ x = -2 or 2.
Thus, (1) and (2) intersect at P(2, 2) and Q(-2, 2).

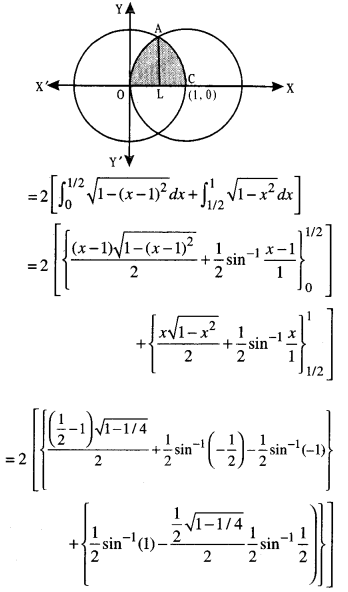
Question 5.
Using integration, find the area of the region enclosed between the two circles:
x² + y² = 1 and (x – 1)² + y² = 1. (C.B.S.E. 2019 C, C.B.S.E. 2019)
Solution:
The given circles are x² + y² =1 …(1)
and (x – 1)² + y² = 1
(1) is a circle with centre (0,0) and radius 1.
(2) is a circle with centre (1,0) and radius 1.
Solving (1) and (2):
(2)-(1) gives: -2x + 1 =0 ⇒ x = \(\frac { 1 }{ 2 }\).
Putting in (1), \(\frac { 1 }{ 4 }\) + y² = 1
y² = \(\frac { 3 }{ 4 }\)
⇒ y = ± \(\frac{\sqrt{3}}{2}\)
Thus, the circles intersect at A (\(\frac{1}{2}, \frac{\sqrt{3}}{2}\)) and B(\(\frac{1}{2},-\frac{\sqrt{3}}{2}\))

Reqd. area = 2 (shaded area)
= 2 (area (OAL) + area (ALC))

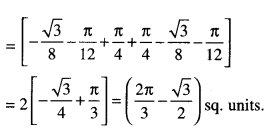
Question 6.
Using integration, find the area of the region: {(x, y); 9x² + 4y² ≤ 36,3x + 2y ≥ 6}. (C.B.S.E. 2019(C))
Solution:
We have: 9x² + 4y² = 36
\(\frac{x^{2}}{4}+\frac{y^{2}}{9}\) = 1 …(1) ,
which is an upward ellipse
and 3x + 2y = 6 =» \(\frac{x}{2}+\frac{y}{3}\) = 1 …(2),
which is a st. line.
Reqd. area is the shaded area, as shown in the figure:

Question 7.
Using the method of integration, find the area of the region bounded by the lines:
3x – 2y + 1 = 0,2x + 3y – 21 = 0 and x – 5y + 9 = 0.   (A.I.C.B.S.E. 2019, C.B.S.E. 2012)
Solution:
Let the sides AB, BC and CA of ΔABC be:
3x – 2y + 1 = 0 …(1)
2x + 3y – 21 = 0 …(2)
and x – 5y + 9 = 0 …(3) respectively.
Solving (3) and (1), we get A as (1,2).
Solving (1) and (2), we get B as (3,5).
Solving (2) and (3), we get C as (6,3).

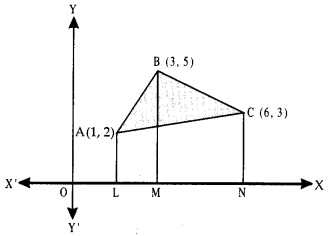
Now ar (ΔABC) = ar (trap ALMB) + ar (trap BMNC) – ar (trap ALNC)

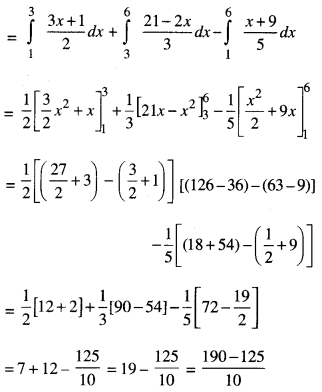
= \(\frac{65}{10}\) = 6.5sq . units.

Question 8.
Find the area lying above the x-axis and included between the circle x² + y² = Sir and the parabola y² = 4x. (N.C.E.R. I; C.B.S.E. 2019,19 C)
Solution:
The given circle is x² + y² – 8x = 0
i.e. (x-4)² + y² = 16 ….. (1)
It has centre (4,0) and radius 4 units.
The given parabola is y² = 4x ….(2)

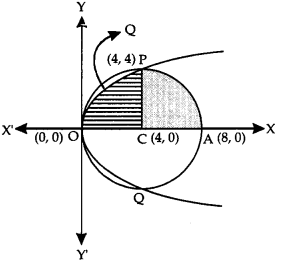
Solving (1) and (2) :
x² – 8x + 4x = 0 =
⇒ x² – 4x = 0
⇒ x(x-4) = 0
⇒ x = 0,4.
When x = 0,y = 0.
When x = 4,y² = 16
⇒ y = ±4.

Thus (1) intersects (2) at O (0, 0) and P (4, 4) above the x-axis.
∴ Area of the region OC APQO
= Area of the region OCPQO + Area of the region C APC
= \(\int_{0}^{4} y_{1} d x+\int_{4}^{8} y_{2} d x\)
where yv y2 are ordinates of points on (2) and (1) respectively.
= \(\int_{0}^{4} \sqrt{4 x} d x+\int_{4}^{8} \sqrt{4^{2}-(x-4)^{2}} d x\)
[∵ Thinking +ve values as region lies above x-axis]

[Putting x-4 = t in 2nd integral so that dx = dt. When x = 4, t = 0; when x – 8, t = 4]

Question 9.
Using integration, find the area of the region bounded by the parabola y² = 4x and the circle 4x² + 4y² = 9. (Outside Delhi 2019)
Answer:

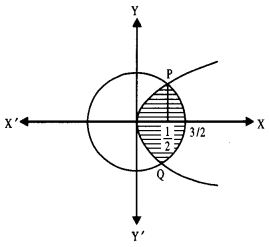
To find the points of intersection of the curves.
y² = 4x …(1)
and 4x² + 4y² = 9 …(2)
From (1) and (2),
4x² + 16x = 9
⇒ 4x²+ 10x – 9 = 0.
Solving, x = \(\frac { 1 }{ 2 }\)

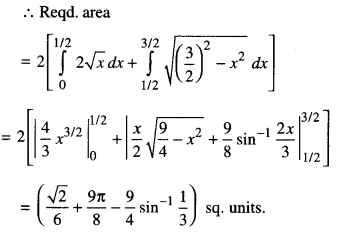
Question 10.
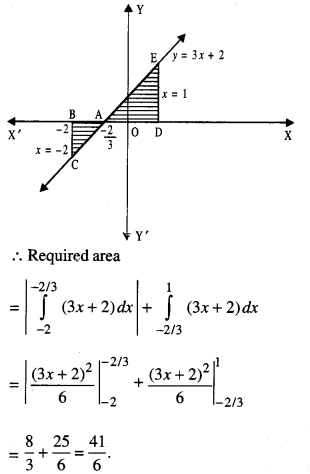
Using integration, find the area of the region bounded by the iiney = 3x +2, the x-axis and the ordinates x = -2 and x = 1. (Outside Delhi 2019)
Answer:
The region is as shown in the figure.

Question 11.
Using integration, find the area of the region:
{(x,y): x² + y² ≤ 1, x + y ≥ 1 x ≥ 0, y ≥ 0}
Answer:
We have: x² + y² = 1 …(1)
and x + y = 1 …(2)
Solving (1) and (2), x² + (1 – x)² = 1 ⇒
2x² – 2x = 0
2x(x-1) = 0
x = 0
x = 1.
or

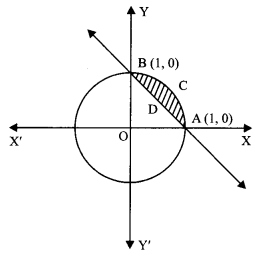
Required area = Shaded area ACBDA
= ar(OACBO – ar(OADBO)

Question 12.
Find the area enclosed between the parabola 4y = 3x²and the straight line 3x – 2y +12 = 0.
(A.I.C.B.S.E. 2017)
Answer:
The given parabola is 4y – 3x²
i.e. x² = \(\frac{4 y}{3}\)…(1),
which is an upward parabola.
The given line is 3x – 2y + 12 = 0 ……….. (2)
Solving (1) and (2) :
From(1), y = \(\frac{3 x^{2}}{4}\) …(3)
Putting in (2),
3x – 2(\(\frac{3 x^{2}}{4}\)) + 12 = 0
x – \(\frac{x^{2}}{4}\) + 4 = 0
⇒ x² – 2x – 8 =0
⇒ (x-4)(x + 2)-0
⇒ x = – 2, 4.
When x = -2, then from (3),
y = \(\frac { 3 }{ 4 }\)(4) = 3.
When x = 4, then from (3),
y = \(\frac { 3 }{ 4 }\)(16) = 12.
Thus parabola (1) and line (2) meet each other at A (-2, 3) and B (4,12).

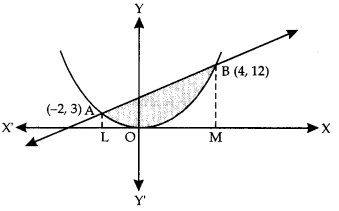
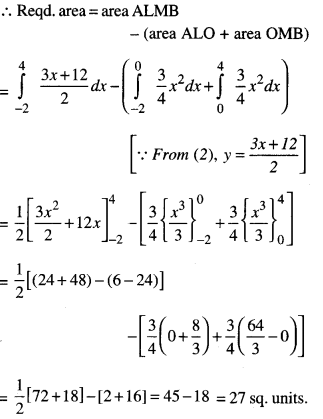
Question 13.
Using integration, find the area of the region :
{(x, y : |x-1| ≤ y ≤ \(\sqrt{5-x^{2}}\)} (C.B.S.E. 2010)
Or
Sketch the region bounded by the curves:
y= \(\sqrt{5-x^{2}}\) and y = |x-1|and find its area, using integration.
(A.I.C.B.S.E. 2015)
Solution:
The given curves are : x² + y² = 5

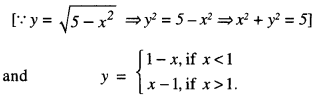
The reqd. region is shown as shaded in the following figure:

y =x-1 meets x² + y² = 5 at B(2,1).
y = 1-x meets x² + y² = 5 atC(-1,2)
y = x -1 and y = 1 -xmeet at A(1, 0).

Reqd. area = ar (MCBLM) – ar (CMAC) – ar (ALBA)

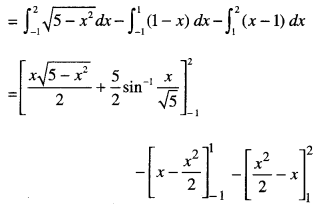
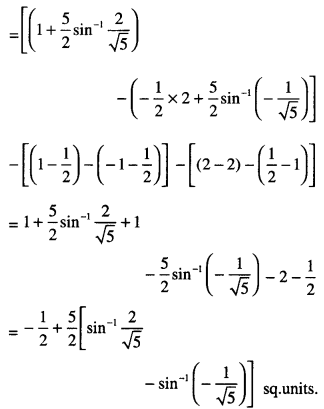
Question 14.
Using integration, find the area of the triangle formed by positive x-axis and tangent and
normal to the circle x² + y² = 4 at (1, 73). (C.B.S.E. 2015)
Solution:
The given circle is
x² + y² = 4 ……. (1)
Diff. w.r.t. x,
2x + 2y \(\frac{d y}{d x}\) = 0
\(\frac{d y}{d x}\) = \(\frac{-x}{y}\)

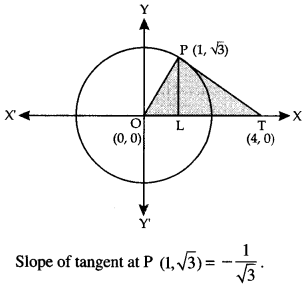
Slope of normal at P (1, √3) =√3 .
∴ The equation of the tangent at P is :

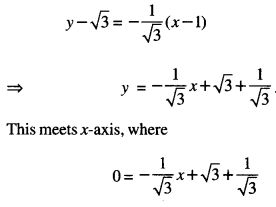
⇒ 0 = -x + 3 + 1
⇒ x = 4.
Thus T is (4,0).
The equation of the normal at P is :
y – √3= √3(x-l)
⇒ y = √3x.
This meets x-axis i.e. y = 0, where x = 0.
Thus O is (0,0).
Now ar (ΔOPT) = ar (OPL) + ar (PLT)

By going through these CBSE Class 11 Maths Notes Chapter 2 Relations and Functions Class 11 Notes, students can recall all the concepts quickly.

Relations and Functions Notes Class 11 Maths Chapter 2

Ordered Pair: Two elements a and b, listed in a specific order, form an ordered pair, denoted by (a,b).
In general, (a,b) ≠ (b, a) and (a₁ b₁) = (a₂, b₂) ⇔ a₁ = a₂ and b₁ = b₂.

Cartesian Product of Sets : If A and B are two non-empty sets, then the set of all ordered pairs (a, b) such that a ∈ A and b ∈ B, is called the cartesian product of A and B, to be denoted by A x B.

Thus, A x B = {(a, b): a e A and b ∈ B)
Remarks : (i) If A = Φ or B = Φ, then A x B = Φ
(ii) If A ≠ Φ and B ≠ Φ, then A x B ≠ Φ.
(iii) A x B ≠ B x A, in general.
(.iv) If A and B are finite sets, then n(A x B) = n(A).n(B).
(v) If either A or B is an infinite set, then A x B is an infinite set.
(vi) If A = B, then A x B is expressed as A².
(vii) In general, if A₁ A₂ ………. A_n are n sets, then (a₁, a₂,…, a_n) is called an ra-tuple, where a_i = A_i, i = 1,2,…, n and the set of all such n-tuples, is called the cartesian product of A₁, A₂,…, A_n. It is denoted by x A₁ x A₂ x …, x A_n = ( a₁, a₂,…, a_n): a_i ∈ A_i, 1 ≤ i ≤ n).

Relation :

(i) Definition : A relation R from a non-empty set A to a non-empty set B is a subset of the cartesian product A x B. The subset is derived by describing a relationship between the first element and the second element of the ordered pair in A x B.
(ii) Image : The second element of each ordered pair of the relation R is called the image of the first element.
(iii) Domain : The set of all first elements of the ordered pairs in a relation R from a set A to a set B is called the domain of the relation R.
(iv) Co-domain : The set B is known as the co-domain of relation R.
Note that range ⊆ co-domain.

Functions : Let X and Y be two non-empty sets. A function or mapping ‘f’ from X into Y written as f: X → F is a rule by which each element x ∈ X is associated to a unique element y ∈ Y. We then say that f is a mapping of X into Y or f is a function of X to Y.

Element y ∈ Y to which the element x ∈ X is associated is called f-image of x or image of x or the value of the function at x and x is called pre-image of y. The set X is called domain and Y is called the co-domain of the function. The set formed by all the f – images of the elements of Y is called the range of the function and is denoted by f(X) or {f(X)}. Clearly, range is the subset of Y.

The numbers x and y, where y is an image ofx are also denoted by ordered pair ix,y) or [x, fix)).

Some Functions With Graphs

1. Constant function : The constant function is defined by y = f(x) = c, where c is a real number.
The graph of this function is set of all points (x, c) i.e., the straight line parallel to x-axis.
Domain = All reals
Range = {c}.

2. Absolute value function : The absolute value function is defined as y = f(x) = | x |. This function can also be written as
|x| = \(\left\{\begin{array}{ll}
x, & \text { if } x \geq 0 \\
-x, & \text { if } x<0 \end{array}\right.\)

The graph of this function is set of all points of the form (x, |x |). Domain = All reals. Range = All non-negative reals.

3. The greatest integer function : The greatest integer function is defined as y = f(x) = [x]. [x] means the greatest integer n such that n ≤ x. For example : [3.5] = 3, [4] = 4, [-5.1] =-6. The graph of [x] gives Domain = Set of ail reals Range = Set of integers.

4. Signum function : Let R be the set of real numbers. Then, the function f: R → R defined by f(x) = \(\left\{\begin{array}{ll} 1, & \text { if } x>0 \\
0, & \text { if } x=0 \\
-1, & \text { if } x<0
\end{array}\right.\) is known as signum function.

Domain of f = R.
Range of f = {- 1, 0, 1}.

5. Identity function : Let R be the set of real numbers. A real valued function f is defined as f: R → R by y = f(x) = x for each value of x e R. Such a function is called the identity function.
Domain of f = R.
Range of f = R.

6. Polynomial function: A function f: R → R is said to be the polynomial function, if for each x ∈ R, y = f(x) = a₀ + a₁x + a₂x² + … + a_nxⁿanxn, where n is non-negative integer and a₀, a₁, a₂,…, a_n ∈ R.

7. Rational functions : These functions are of type \(\frac{f(x)}{g(x)}\), where f(x) and g(x) are polynomial functions of* defined in a domain where g(x) ≠ 0.

Algebra Of Real Functions

(i) Addition of two real functions : Let f: X → R and g : X→ R be any two real functions, where X ⊂ R. Then, we define(f+g):X ⊂ R by (f+g)(x) = f(x)+g(x) for all x ∈ X.

(ii) Subtraction of a real function from another : Let f: X → R and g: X → R be two real functions, where X ⊂ R. Then, we define (f -g)x: x → R by (f-g)x – f(x)-g(x) for all x ∈ X.

(iii) Multiplication by Scalar: Let f: R → R be a real valued function and c be a scalar, where c is real number. Then, the product cf is a function from X to R defined by (f)(x) = cf(x), x ∈ X.

(iv) Multiplication of two real functions : The product of two real functions, f: x → R and g : x → R is a function fg : X → R defined by (fg){x) = f(x)g(x), for all x ∈ X.

(v) Quotient of two real functions: Let fandg be two real functions defined from X → R, where X⊂R. The quotient of f by g is function defined by \(\left[\frac{f}{g}\right][x]=\frac{f(x)}{g(x)}\), for all x ∈ X such that g(x) ≠ 0.

Here we are providing Class 12 Maths Important Extra Questions and Answers Chapter 4 Determinants. Class 12 Maths Important Questions are the best resource for students which helps in Class 12 board exams.

Class 12 Maths Chapter 4 Important Extra Questions Determinants

Determinants Important Extra Questions Very Short Answer Type

Question 1.
Find the co-factor of the element a₂₃ of the determinant:
\(\left|\begin{array}{lll}
5 & 3 & 8 \\
2 & 0 & 1 \\
1 & 2 & 3
\end{array}\right|\)
(C.B.S.E. 2019 C)
Solution:
Co-factor of a₂₃ = (-1)^{2 + 3} \(\left|\begin{array}{ll}
5 & 3 \\
1 & 2
\end{array}\right|\)
= (-1)⁵ (5 x 2 – 1 x 3)
= (-1) (10-3)
= (-1) (7) = -7.

Question 2.
If A and B are invertible matrices of order 3, |A| = 2 and |(AB)^-1| = \(-\frac{1}{6}\) Find |B|.
(C.B.S.E. Sample Paper 2018-19)
Solution:


Hence |B| = 3

Question 3.
Check whether (l + m + n) is a factor of the determinant \(\left|\begin{array}{ccc}
l+m & m+n & n+l \\
n & l & m \\
2 & 2 & 2
\end{array}\right|\) or not.
Given reason.
(C.B.S.E. Sample Paper 2020)
Solution:
Given

Hence, (l + m + n) is a factor of given determinant.

Question 4.
If A is a square matrix of order 3, with |A| = 9, then write the value of |2 . adj. A|.   (A.I.C.B.S.E. 2019)
Solution:
| 2 – adj. A| = 2³ | A |^3-1
= 8(9)²
= 648.

Question 5.
If A and B are square matrices of the same order 3, such that |A| = 2 and AB = 2I, write the value of IBI. (C.B.S.E. 2019)
Solution:
We have : AB = 2I
∴ |AB| = |2I|
⇒ |A||B| = |2I|
⇒ 2|B|= 2(1).
Hence,|B| = 1.

Question 6.
A is a square matrix with |A| = 4. Then find the value of |A. (adj. A) |.
(A.I.C.B.S.E. 2019)
Solution:
|A . (adj. A) | = |A|ⁿ
= 4ⁿ or 16 or 64.

Question 7.
If Δ = \(\left|\begin{array}{lll}
5 & 3 & 8 \\
2 & 0 & 1 \\
1 & 2 & 3
\end{array}\right|\) , write:
(i) the minor of the element a₂₃ (C.B.S.E. 2012)
(ii) the co-factor of the element a₃₂. (C.B.S.E. 2012)
Solution:
(i) a₂₃ = \(\left|\begin{array}{ll}
5 & 3 \\
1 & 2
\end{array}\right|\)
= (5) (2) – (1) (3)
= 10 – 3 = 7.

(ii) a₃₂ = (-1)³⁺² \(\left|\begin{array}{ll}
5 & 8 \\
2 & 1
\end{array}\right|\)
= (-1)⁵ [(5) (1) – (2) (8)]
= (-1)⁵ (5 – 16)
= (- 1) (- 11) = 11.

Question 8.
Find the adjoint of the matrix A = \(\left[\begin{array}{cc}
2 & -1 \\
4 & 3
\end{array}\right]\) (A.I.C.B.S.E. 2010)
Solution:
Here |A| = \(\left[\begin{array}{cc}
2 & -1 \\
4 & 3
\end{array}\right]\)
Now A₁₁ = Co-factor of 2 = 3,
A₁₂ = Co-factor of – 1 = – 4,
A₂₁ = Co-factor of 4 = 1
and A₂₂ = Co-factor of 3 = 2
∴ Co-factor matrix = \(\left[\begin{array}{rr}
3 & -4 \\
1 & 2
\end{array}\right]\)
Hence, adj. A = \(\left[\begin{array}{rr}
3 & -4 \\
1 & 2
\end{array}\right]\) = \(\left[\begin{array}{rr}
3 & 1 \\
-4 & 2
\end{array}\right]\)

Question 9.
Given A = \(\left[\begin{array}{rr}
2 & -3 \\
-4 & 7
\end{array}\right]\) compute A^-1 and show that 2A^-1 = 9I – A. (C.B.S.E. 2018)
Solution:
(i) We have: A = \(\left[\begin{array}{rr}
2 & -3 \\
-4 & 7
\end{array}\right]\)
∴ |A| = \(\left|\begin{array}{cc}
2 & -3 \\
-4 & 7
\end{array}\right|\)
= (2) (7) – (-4) (-3)
= 14 – 12 = 2 ≠ 0.
∴ A^-1 exists and
A^-1 = \(\frac{1}{|\mathrm{~A}|}\) adj A = \(\frac{1}{2}\left[\begin{array}{ll}
7 & 3 \\
4 & 2
\end{array}\right]\)

(ii) RHS = 9I – A

Question 10.
For what value of ‘x’, the matrix \(\left[\begin{array}{cc}
5-x & x+1 \\
2 & 4
\end{array}\right]\) is singular? (C.B.S.E. 2011)
Solution:
The matrix \(\left[\begin{array}{cc}
5-x & x+1 \\
2 & 4
\end{array}\right]\) is singular
⇒ \(\left[\begin{array}{cc}
5-x & x+1 \\
2 & 4
\end{array}\right]\) = 0
⇒ 4(5 -x) – 2 (x + 1) = 0
⇒ 20 – 4x – 2x – 2 = 0
⇒ 18 – 6x = 0
⇒ 6x = 18.
Hence, x = 3.

Determinants Important Extra Questions Long Answer Type 1

Question 1.
Using properties of determinants, prove the following :

(C.B.S.E. 2019)
Solution:

[Operating C₃ → C₃ + C₂]
= (a + b) (a + c) (2) [c + b]
= 2(a + b) (b + c) (c + a) = RHS.

Question 2.
If f(x) = \(\left|\begin{array}{rrr}
a & -1 & 0 \\
a x & a & -1 \\
a x^{2} & a x & a
\end{array}\right|\), using properties of determinants, find the value   (C.B.S.E. 2015)
Solution:
We have


[Operating C₂ → C₂ + C₁]
= a[(a + x) a + (ax + x²)]
= a[a² + ax + ax + x²]
= a(x² + 2 ax + a²)
= a(x + a)².
f(2x) = a(2x + a)²,
f (2x) -f(x) = a[(2x + a)² – (x + a)²]
= a[(4x² + 4 ax + a²) – (x² + 2ax + a²)]
= a(3x² + 2 ax)
= ax(3x + 2a).

Question 3.
Using properties of determinants, prove that:
\(\left|\begin{array}{ccc}
1 & 1 & 1+3 x \\
1+3 y & 1 & 1 \\
1 & 1+3 z & 1
\end{array}\right|\) = 9(3xyz + xy + yz + zx) (C.B.S.E. 2018)
Solution:

1 . (0 + 9yz) + 3x(3 z + 9yz + 3y)
= 9(3xyz + xy + yz + zx) = RHS

Question 4.
Using properties of determinants, prove that:
\(\left|\begin{array}{ccc}
a & b-c & c+b \\
a+c & b & c-a \\
a-b & b+a & c
\end{array}\right|\) = (a + b + c) (a² + b² + c²) (C.B.S.E. Sample Paper 2018-19)
Solution:

Question 5.
Using properties of determinants, prove that:
\(\left|\begin{array}{ccc}
a^{2}+2 a & 2 a+1 & 1 \\
2 a+1 & a+2 & 1 \\
3 & 3 & 1
\end{array}\right|\) = (a – 1)³. (C.B.S.E. 2019)
Solution:

[Taking (a-1) common form R₁ and R₂]
= (a – 1)² [a + 1 -2]
= (a – 1)² (a – 1)
= (a – 1)³.

Question 6.
If x, y, z are different and \(\left|\begin{array}{lll}
x & x^{2} & 1+x^{3} \\
y & y^{2} & 1+y^{3} \\
z & z^{2} & 1+z^{3}
\end{array}\right|\) = 0 show that :
(i) 1 + xyz = 0
(ii) xyz = -1
Solution:



[Dividing by (x – y) (y – z) (z – x)
because x ≠ y y ≠ z z ≠ x

⇒ x [(x + y) (x + y + z) – (1) (x² + xy + y²)]
– [x² (x + y + z) – (1) (1 + x³)] = 0
⇒ x[x² + xy + zx + xy + y² + yz – x² – xy – y²] – [x³ + x²y + x²z – 1 – x³] = 0
⇒ x[xy + yz + zx]- [x²y + x²z – 1] = 0
⇒ x²y + xyz + zx² – x²y – x²z + 1 = 0
⇒ 1 + xyz = 0 or xyz = -1, which is

Question 7.
Prove that \(\begin{array}{c}
\left|\begin{array}{ccc}
1+a & 1 & 1 \\
1 & 1+b & 1 \\
1 & 1 & 1+c
\end{array}\right| \\
=a b c\left(1+\frac{1}{a}+\frac{1}{b}+\frac{1}{c}\right)
\end{array}\) = abc + ab + bc + ca (N.C.E.R.T.; A.I.C.B.S.E. 2013, 12, 10 C)
Solution:

Question 8.
Using properties of determinants, find the value of k if
\(\left|\begin{array}{ccc}
x & y & x+y \\
y & x+y & x \\
x+y & x & y
\end{array}\right|\)= k(x³ + y³) (A.I.C.B.S.E. 2019)
Solution:
We have :

[Operating C₂ → C₂ – C₁ & C₃ → C₃ – C₁]
⇒ 2(x + y) (1) [-x² + y (x – y] = k(x³ + y³)
⇒ – 2(x + y) (x² – xy + y²) = k(x³ + y³)
⇒ – 2(x³ + y³) = k(x³ + y³).
Hence, k = – 2.

Question 9.
Using properties of determinants, prove the
following:
\(\left|\begin{array}{ccc}
a & b & c \\
a-b & b-c & c-a \\
b+c & c+a & a+b
\end{array}\right|\) = a³ + b³ + c³ – 3abc. (C.B.S.E. 2019)
Solution:

[Operating C₁ → C₁ – C₂ and C₂ → C₂ – C₃]
= (a + b + c) [(a – 2b + c) (c – b) – (b – 2c + a) (b – a)]
= (a + b + c)[a² + b² + c² – ab – bc – ca]
= a³ + b³ + c³ – 3abc = RHS

Question 10.
Prove the following, using properties of determinants:
\(\left|\begin{array}{ccc}
\alpha & \alpha^{2} & \beta \\
\beta & \beta^{2} & \gamma+\alpha \\
\gamma & \gamma^{2} & \alpha+\beta
\end{array}\right|\) = (β-γ)(γ-α)(α-β)(α+β+γ) (C.B.S.E. 2019 C)
Solution:


= (α + β + γ)(α – β)(β – γ)(γ + β – β – α)
= (β – γ)(γ – α)(α – β)(α + β + γ)
which is true.

Question 11.
Find the minors and co-factors of all elements of the determinant \(\left|\begin{array}{rr}
1 & -2 \\
4 & 3
\end{array}\right|\) . (N.C.E.R.T.)
Solution:
By definition,
M₁₁ = Minor of a₁₁ = 3
A₁₁ = Co-factor of a₁₁
= (- 1)¹⁺¹ M₁₁= (- 1)² 3 = 3

M₁₂ = Minor of a₁₂ = 4
A₁₂ = Co-factor of a₁₂
= (-1)^{1 + 2} M₁₂ = (-1)³ 4 = -4

M₂₁ = Minor of a₂₁ = – 2
A₂₁ = Co-factor of a₂₁
= (- 1)²⁺¹ M₂₁
= (- 1)³ (- 2)
= (- 1) (- 2) = 2

M₂₂ = Minor of = 1
A₂₂ = Co-factor of a₂₂
= (-1)²⁺² M₂₂ = (-1)⁴ (1) = 1.

Question 12.
For the matrix A = \(\left[\begin{array}{rr}
2 & -1 \\
3 & 2
\end{array}\right]\), show that A² – 4A + 7I = 0. Hence, obtain A^-1.
Solution:

(ii) A² – 4A + 7I = 0
⇒ 7I = 4A-A²

Question 13.
Using properties of determinants, find the value of x for which
\(\left|\begin{array}{lll}
4-x & 4+x & 4+x \\
4+x & 4-x & 4+x \\
4+x & 4+x & 4-x
\end{array}\right|\) = 0
(A.I.C.B.S.E. 2019)
Solution:

⇒ (12 + x) [4x²] = 0
⇒ x² (x + 12) = 0.
Hence, x = 0 or x = – 12.

Question 14.
If A = \(\left[\begin{array}{rrr}
1 & -2 & 3 \\
0 & -1 & 4 \\
-2 & 2 & 1
\end{array}\right]\), find (A’)^-1 (C.B.S.E. 2015)
Solution:
We have


= (1)( – 1 – 8) – O – 2( – 8 + 3)
= – 9 + 10 = 1 ≠ 0
B exists.= (1)( – 1 – 8) – 0 – 2( – 8 + 3)
= -9 + 10 = 1 ≠ 0
B^-1 exists.

Question 15.
Using matrices, solve the following system of linear equations :
x + 2y -3z = – 4
2x + 3y + 2z = 2
3x – 3y – 4z = 11.   (A.I.C.B.S.E. 2019)
Solution:
We have A= \(\left[\begin{array}{ccc}
1 & 2 & -3 \\
2 & 3 & 2 \\
3 & -3 & -4
\end{array}\right]\)
∴ |A| = \(\left|\begin{array}{ccc}
1 & 2 & -3 \\
2 & 3 & 2 \\
3 & -3 & -4
\end{array}\right|\)
=(1)( – 12 + 6) – (2)( – 8 – 6)+( – 3)( – 6 – 9)
= – 6 + 28 + 45 = 73 – 6 = 67.
∴ A is non-singular A exists.
Now, A₁₁ = -6, A₁₂ = 14. A₁₃ = -15
A₂₁ = 17, A₂₂ = 5, A₂₃ = 9
A₃₁ = 13, A₃₂ = – 8, A₃₃ = – 1


The given equation can be written as AX = B.
⇒ X = A^-1B
Hence x = 3, y = -2 and z = 1

Determinants Important Extra Questions Long Answer Type 2

Question 1.
Using the properties of determinants, prove that:
\(\mid \begin{array}{ccc}
(y+z)^{2} & x^{2} & x^{2} \\
y^{2} & (z+x)^{2} & y^{2} \\
z^{2} & z^{2} & (x+y)^{2}
\end{array}\) = 2xyz(x + y + z)³
(CBSE. Sample Paper 2019-20)
Solution:


= 2(x + y + z)² [xyz² + x²yz + xy²z
+ y² z² – y² z² ]
= 2xyz (x + y + z)² (x + y + z)
= 2xyz (x + y + z)³ = RHS.

Question 2.
If A = \(\left[\begin{array}{ccc}
2 & 3 & 4 \\
1 & -1 & 0 \\
0 & 1 & 2
\end{array}\right]\), find A^-1 Hence , solve the system of equations.
x – y = 3;
2x + 3y + 4z = 17;
y + 2z = 7.
(C.B.S.E. 2019)
Solution:
(i) |A| = \(\left|\begin{array}{ccc}
2 & 3 & 4 \\
1 & -1 & 0 \\
0 & 1 & 2
\end{array}\right|\)
=2( – 2 – 0) – 3(2 – 0)+4(1 +0)
= – 4 – 6 + 4= – 6 ≠ 0
∴ A is non-singular and as such A exists.
Now, A₁₁ = -2, A₁₂ = -2. A₁₃ = 1
A₂₁ = -2, A₂₂ = 4, A₂₃ = -2
A₃₁ = 4, A₃₂ = 4, A₃₃ = -5

(II) The given system of equations can be written as AX = B,

Hence x = 2, y = -1 and z = 4.

Question 3.
Obtain the inverse of the following matrix using elementary operations :
A = \(\left[\begin{array}{ccc}
-1 & 1 & 2 \\
1 & 2 & 3 \\
3 & 1 & 1
\end{array}\right]\) (C.B.S.E. 2019)
Solution:
We know that A = I₃A

Question 4.
If A = \(\left[\begin{array}{rrr}
2 & -3 & 5 \\
3 & 2 & -4 \\
1 & 1 & -2
\end{array}\right]\) , find A^-1. Use it to solve the system of equations:
2x – 3y + 5z = 11
3x + 2y – 4z = – 5
x + y – 2z = -3.   (C.B.S.E. 2019,18)
Solution:
(I) |A| = \(\left[\begin{array}{rrr}
2 & -3 & 5 \\
3 & 2 & -4 \\
1 & 1 & -2
\end{array}\right]\)
= 2 (- 4 + 4) + 3 (- 6 + 4) + 5 (3 – 2)
= 2 (0) + 3 (- 2) + 5 (1)
= 0 – 6 + 5 = -1 ≠ 0.
A is non-singular and as such A^-1 exists.

(II) The given system of equations is :
2x – 3y + 5z = 11
3x + 2y – 4z = -5
x + y – 2z = – 3.
These equations can be written as AX = B,
where A = \(\left[\begin{array}{rrr}
2 & -3 & 5 \\
3 & 2 & -4 \\
1 & 1 & -2
\end{array}\right]\), X = \(\left[\begin{array}{l}
x \\
y \\
z
\end{array}\right]\)
and B = \(\left[\begin{array}{r}
11 \\
-5 \\
-3
\end{array}\right]\)
Since A is non – singular, (∵ |A|≠ 0)
∴ the given system has a unique solution given byX = A^-1B
[∵ AX = B ⇒ A^-11 (AX) = A^-1B
⇒ (A^-1A)X = A^-1B
⇒ IX = A^-1B ⇒ X = A^-1B]

Hence, x = 1, y = 2 and z = 3.

Question 5.
If A = \(\left[\begin{array}{rrr}
3 & 1 & 2 \\
3 & 2 & -3 \\
2 & 0 & -1
\end{array}\right]\) find A^-1 the system of equations:
3x + 3y + 2z = 1
x + 2y = 4
2x – 3y – z = 5.   (C.B.S.E. Sample Paper 2018-19)
Solution:
We have A = \(\left[\begin{array}{rrr}
3 & 1 & 2 \\
3 & 2 & -3 \\
2 & 0 & -1
\end{array}\right]\)
|A| = 3[-2+0] -1 [-3+6] + 2[0-4]
= -6 – 3 – 8 = -17 ≠ 0
∴ A^-1 exits

(ii) The given system of equation is

(A’)X = B
X = (A’)^-1B
X = (A^-1)^tB [ ∵ (A^t)^-1 = (A^-1)^t]

Hence x = 2, y =1, z = -4

Question 6.
If A = \(\left[\begin{array}{rrr}
1 & -2 & 3 \\
0 & -1 & 4 \\
-2 & 2 & 1
\end{array}\right]\), find (A’)^-1 (C.B.S.E 2015)
Solution:
We have :

= (1)( – 1 – 8) – 0 – 2( – 8 + 3)
= – 9 + 10 = 1 ≠ 0
B^-1 exists.
Now B₁₁ = \(\left|\begin{array}{rr}
-1 & 2 \\
4 & 1
\end{array}\right|\) = – 1 – 8 = – 9;
B₁₂= \(-\left|\begin{array}{rr}
-2 & 2 \\
3 & 1
\end{array}\right|\) = – ( – 2 – 6) = 8;

Question 7.
If A = \(\), find A^-1
Hence, solve the system of equations :
x + y + z = 6,
x + 2z = 7,
3x + y + z = 12. (C.B.S.E. 2019)
Solution:
(i) Here, A = \(\left[\begin{array}{lll}
1 & 1 & 1 \\
1 & 0 & 2 \\
3 & 1 & 1
\end{array}\right]\)
∴ |A| = 1(0-2) -(1) (1-6) + 1(1-0)
= – 2 + 5 + 1 = 4 ≠ 0
∴ A^-1 exists.

(ii) For given system of equations can be written as :

Hence x = 3, y = 1 and z = 2.

Question 8.
Find the inverse of the following matrix, using elementary operations :
A = \(\left[\begin{array}{ccc}
1 & 2 & -2 \\
-1 & 3 & 0 \\
0 & -2 & 1
\end{array}\right]\)
Solution:
We know that A = I₃A

Question 9.
Using elementary transformations, find the inverse of the matrix :
A = \(\left[\begin{array}{lll}
8 & 4 & 3 \\
2 & 1 & 1 \\
1 & 2 & 2
\end{array}\right]\) and use it to solve the following system of equations:
8x + 4y + 3z = 19
2x + y + z = 5
x + 2y + 2z = 7. (C.B.S.E. 2016)
Solution:
(i) We know that AA^-1 = I

(ii) The given system of equations can be written as AX = B …(1),
Where A = \(\left[\begin{array}{lll}
8 & 4 & 3 \\
2 & 1 & 1 \\
1 & 2 & 2
\end{array}\right]\), X = \(\left[\begin{array}{l}
x \\
y \\
z
\end{array}\right]\) and B = \(\left[\begin{array}{c}
19 \\
5 \\
7
\end{array}\right]\)
From AX = B
⇒ A^-1(AX) = A^-1B
⇒ (A^-1A)X = A^-1B
⇒ IX = A^-1B
⇒ X = A^-1B

Hence x = 1, y = 2, z = 1.

Question 10.
If A = \(\left[\begin{array}{rrr}
2 & 3 & 10 \\
4 & -6 & 5 \\
6 & 9 & -20
\end{array}\right]\) , find A^-1. Using A-1 , solve the system of equations:

Solution:
The given system of equation is:

These equations can be written as:
AX = B ……….(4)

= 2(120 – 45) – 3 (- 80 – 30) + 10 (36 + 36)
= 150 + 330 + 720= 1200 ≠ 0.
∴ A is non-singular and as such A^-1 exists.


From (4)
⇒ A^-1(AX) = A^-1B
⇒ (A^-1A)X = A^-1B
⇒ IX = A^-1B
⇒ X = A^-1B

Hence x = 2, y = -3 and z = 5.

Question 11.
The monthly incomes of Aryan and Babban are in the ratio 3 : 4 and their monthly expenditures are in the ratio 5 : 7. If each saves ₹ 15,000 per month, find their monthly incomes, using matrix method. (C.B.S.E. 2016)
Solution:
Let ₹3x and ₹4x be the monthly income of Aryan and Babban respectively.
Let ₹5y and ₹7y be the monthly expenditure of Aryan and Babban respectively.
By the question,
3x – 5y = 15000 …(1)
and 4x – 7y = 15000 …(2)
These equations can be written as AX = B …..(3)
where A = \(\left[\begin{array}{ll}
3 & -5 \\
4 & -7
\end{array}\right]\), X = \(\left[\begin{array}{l}
x \\
y
\end{array}\right]\) and

From (3),
⇒ A^-1(AX) = A^-1B
⇒ (A^-1A)X = A^-1B
⇒ IX = A^-1B
⇒ X = A^-1B

⇒ x = 30,000.
Hence,monthly income of Aryan
= 3(30,000) = ₹ 90,000
and monthly income of Babban
= 4(30,000) = ₹ 1,20,000.

Question 12.
A typist charges ₹ 145 for typing 10 English and 3 Hindi pages, while charges for typing 3 English and 10 Hindi pages are ₹180. Using matrices, And the charges of typing one English and one Hindi page separately. (A.I.C.B.S.E. 2016)
Solution:
Let ₹x and ₹y be the charges for 1 page of En¬glish and Hindi respectively.
By the question,
10x + 3y = 145 …(1)
and 3x + 10y = 180 …(2)
These can be written as AX = B …(3),
Where A = \(\left[\begin{array}{rr}
10 & 3 \\
3 & 10
\end{array}\right]\), X = \(\left[\begin{array}{l}
x \\
y
\end{array}\right]\)
and B = \(\left[\begin{array}{l}
145 \\
180
\end{array}\right]\)
now |A| = \(\left|\begin{array}{rr}
10 & 3 \\
3 & 10
\end{array}\right|\) = 100 – 9 = 91 ≠ 0
∴ A is non-singular and as such A^-1 exits.

From (3)
⇒ A^-1(AX) = A^-1B
⇒ (A^-1A)X = A^-1B
⇒ IX = A^-1B
⇒ X = A^-1B

Thus x = 10 and y = 15
Hence, the charges are ₹ 10 and ₹ 15 per page of English and Hindi respectively.

Question 13.
The management committee of a residential colony decided to award some of its members (say x) for honesty, some (say y) for helping others and some others (say z) for supervising the workers to keep die colony neat and clean. The sum of all the awardees is 12. Three times the sum of the awardees for cooperation and supervision added to two times the number of awardees for honesty is 33. If the sum of the number of awardees for honesty and supervision is twice the number of awardees for helping others. Using matrix method, find the number of awardees of each category.   (A.I.C.B.S.E. 2013)
Solution:
Let x, y and z refer to honesty, co-operation and supervision respectively.
∴ x + y + z = 12 …(1)
3(y + z) + 2x = 33
i.e. 2x + 3y + 3z = 33 …(2)
and x + z = 2y
i.e. x – 2y + z = 0 …(3)
These can be written as AX = B – (4),

= (1) (3 + 6) – (1) (2 – 3) + (1) (-4-3)
= 9 + 1 – 7 = 3 ≠ 0.
∴ A is non-singular and as such A^-1 exists.


From (4)
⇒ A^-1(AX) = A^-1B
⇒ (A^-1A)X = A^-1B
⇒ IX = A^-1B
⇒ X = A^-1B

Thus, x = 3, y = 4 and z = 5.
Hence, the number of awardees are 3, 4 and 5 in three categories.

Here we are providing Class 11 Political Science Important Extra Questions and Answers Chapter 6 Judiciary. Political Science Class 11 Important Questions with Answers are the best resource for students which helps in class 11 board exams.

Class 11 Political Science Chapter 6 Important Extra Questions Judiciary

Judiciary Important Extra Questions Very Short Answer Type

Question 1.
What is Judiciary?
Answer:
Judiciary is the important organ of the Government which is concerned with settling the disputes and dispensation of Justice at different levels for justice. Earlier all the judicial powers used to be vested in the king under the Monarchy system but now in democratic setup Judiciary is structural as a separate organ of the Government.

Question 2.
Why we need Judiciary?
Answer:
In any group or society, disputes are bound to occur due to different individual and group interests of the people which need to be settled up to the satisfaction of all the concerned by an independent competent and impartial body. To serve these needs, a separate organ of the Government is structural as the judiciary in modern democracies. Judiciary, besides settling the disputes, performs a number of other functions for the society.

Question 3.
Write three main functions of the Judiciary
Answer:

1. Settling the disputes among the people
2. It protects the Rule of Law and to ensure the supremacy of Law
3. Safeguarding the rights of the people and the constitution.

Question 4.
What do you mean by the independence of the judiciary?
Answer:
Basically, independence of judiciary means that judiciary is allowed to work independently, impartially without undue interference of executive and legislature. Independence of Judiciary also means that the judges should be appointed on merit with definite qualifications and experience and they should enjoy long tenure. They should not be removed in an arbitrary manner. The judges should be paid good salaries and facilities. The decisions should be respected.

Question 5.
How the judges are appointed in India?
Answer:
The judges are appointed for Supreme court and“High Court by the President of India with the consultation of the Chief Justice of India and the Chief Justice of concerned High Court (in a case of High Court Judges) The constitution of India has provided, qualification and experience for the appointment of judges.

Question 6.
How the judges can be removed?
Answer:
It is provided in the Constitution that the judges can be removed from the office by impeachment in which the charges are levelled in one house of the Parliament and are listened and examined in next house. If the charges are proved correct, the judges stand impeached.

Question 7.
What do you mean by the integrated judiciary?
Answer:
We in India have integrated judiciary which means that at the apex is the Supreme Court and at the bottom is the lower court (District Court). It is a Pyramid (ladder) like structure. The Supreme Court has administrative and supervisory control on lower courts and it listens to the appeal against the decisions of the lower courts.

Question 8.
Discuss the composition of the Supreme Court of India.
Answer:
Supreme Court of India consists of 26 judges including Chief Justice, who are appointed by the President of India. To become a judge in the Supreme Court one should have experience of Judge for 5 years in the High Court or 10 years of an advocate in the Supreme Court. He should be a distinguished jurist in the eye of President.

Question 9.
What is original jurisdiction of Supreme Court of India?
Answer:
In original jurisdiction of Supreme Court of India following cases are taken up.

1. Disputes between Centre and State Governments.
2. Disputes between the two states.
3. Disputes related to Fundamental Rights
4. Disputes in which issue of interpretation of the constitution is involved.

Question 10.
What is the jurisdiction of the High Court?
Answer:
Almost every state has its High Court. It stands in the middle of the judicial system. High Courts have the following jurisdiction:

1. Original Jurisdiction
2. Appellate Jurisdiction
3. Supervisory Jurisdiction
4. Administrative Jurisdiction
5. Court of record

Question 11.
What is District Court?
Answer:
District Court is at the lowest level of Indian judicial system. Its judges are appointed by the Governor with the consultation of Chief Justice of High Courts. Its functions are as under:

1. Deals with the cases arising in the District
2. Considers appeals on decisions given by lower courts
3. Decides cases involving serious criminal offences.
4. District Cases have administrative control on subordinate courts.

Question 12.
What is the role of Indian Judiciary?
Answer:

1. It settles disputes
2. It interprets the Constitution and acts its guardian
3. It protects the Fundamental Rights of the people.
4. It protects the federal system.
5. It helps in the development of the constitution

Question 13.
What do you understand by Judicial activism?
Answer:
The term Judicial activism refers to the working of Judiciary beyond its given area. It is said that when executive and legislature fail, the judiciary start. It is called Judicial activism. In our constitution procedure established by law is allowed under which the judiciary can examine whether a particular law is according to the provisions of the Constitution or not. But our judiciary is going beyond its limit and is commenting on every policy and political matter which is referred to as Judicial activism.

Question 14.
What is the Power of Judicial Activism?
Answer:
The power of Judicial Review is the power of judiciary under which it can examine the constitutional validity of an order issued by the executive and legislation passed by the legislature. If the court finds it contrary to the provisions of the Constitution it can declare them as unconstitutional. On the basis of this power judicial is playing a very effective role in checking the executive and legislature but in the process, it is going beyond its jurisdiction Hence it is called as Judicial activism.

Question 15.
What is PIL Public Interest Litigation?
Answer:
PIL Public’ Interest Litigation means taking up the case of such weaker and poor people to the court who are unable to take up their case themselves due to some reasons.

Judicial activism has a manifold impact on the Indian Political System. It has democratised the Judicial systems. It has forced accountability and terror.

Judiciary Important Extra Questions Short Answer Type

Question 1.
Mention essential conditions for the independence of the judiciary.
Answer:
Following are some main essential conditions for the independence of the judiciary.

1. The judiciary should be the product of constitutional law.
2. Judiciary should be free from undue interference of Executive and Legislature.
3. High qualifications and experiences should be set for the appointment of judges.
4. The judge’s pay and allowance and other service conditions should be attractive so that man of high integrity enters in this profession.
5. The tenure of the service of the judges should be long and for the security of service, they should not be dependent on the where is and fancies of the executive and Legislature.
6. The decisions of the Judiciary should be respected and accepted.

Question 2.
How the constitution of India has ensured independent of the judiciary?
Answer:
Constitution of India has ensured the independence of the judiciary by the following provisions:

1. The legislature is not involved in the appointment of Judges
2. The judges have fixed and long tenure and the process of removal of judges by impeachment is long and difficult.
3. Judiciary is not financially dependent on the executive or legislature.
4. Judiciary has the power to penalise those who commit contempt of Court.
5. Parliament cannot discuss the conduct of judges.
6. Decisions of the judiciary are immense from personal criticism.

Question 3.
How Indian Judiciary is integrated Judiciary?
Answer:
Integrated Judiciary is one which is structurally and functionally. related at different levels. India judiciary is also integrated because it is also a ladder-like structure. At its apex in the Supreme Court of India.

In the middle (State Level) are the High Courts and the Lower Level (District Level) and the District and other local courts. The Supreme Court of India which is at the apex of the Judicial system exercises, administrative, supervisory control in the Lower Courts. It has also appellate jurisdictions on lower courts. It has also appellate jurisdiction on lower courts. Its decisions are binding on all courts. It can transfer judges from one court to another. It can transfer a case from one court to another court. It can more any case from any court to itself.

Question 4.
Write the composition of the Supreme Court of India.
Answer:
Supreme Court of India consists of 26 judges including one Chief Justice. To became the Judge one should have following qualifications

1. He should be a citizen of India.
2. He should have worked as a judge in any High Court for five years.
3. He should have worked as an advocate in the Supreme court for 10 years.
4. He should be a distinguished judge in the eye of the President.

All the judges of Supreme Court are appointed by the President of India with the consultation of Chief Justice of India.

The Judges of the Supreme Court get retirement at the age of 65 years.

Question 5.
Explain the Jurisdiction of the Supreme Court of India.
Answer:
Supreme Court of India has the following Jurisdiction:

1. Original Jurisdiction Under this jurisdiction the Supreme Court listens to the case related to
a. Dispute between centre and state
b. Dispute between two states
c. Fundamental Rights
4. Interpretation of the constitution

2. Appellate Jurisdiction: Under this jurisdiction Supreme Court listen to the appeal against the decisions of the High Courts in all the civil, criminal and constitutional matters.

3. Advisory Jurisdiction: Under this jurisdiction, the Supreme Court can give its opinion to the President on any issue on which he seeks its opinion.

Question 6.
Write the functions of High Courts.
Answer:
Almost every state has its own High Court. Its main functions are as under:

1. It can hear the appeals against the decisions of the lower courts
2. It can issue writs for restoring Fundamental Rights
3. It can deal with cases which fall within the jurisdiction of the State.
4. It has supervisory and administrative jurisdiction over the lower courts under which it exercises, superintendence and control over courts below it.
5. It decides the service conditions of the employees of the lower courts.

Question 7.
What is impeachment?
Answer:
Impeachment is a method of removal of the judges by the Parliament on the charges like corruption, misuse of power and violation of the Constitution.

The impeachment motion of the removal of the judge with the charges is introduced in either house. If that motion is passed by 2/3 majority of the present and voting of the members, then it is sent to another house when it is heard. The judge can explain his/her position in that house. If the charges levelled in the previous house are proved in the second house by 2/3 majority of the present and voting and majority of the house. The judge stands impeached and he has to vacate the office. So far only one case of the removal of a judge of Supreme Court came up for consideration before the Parliament which could not get the support of the majority of the total strength of the House.

Question 8.
What is Judicial Review? How does it work in India?
Answer:
Judicial Review is the power of the judiciary to test the constitutional validity which means it tests whether a law or executive is in accordance with the provisions of the Constitution. If the imposed legislation or order of the executive is not according to the provisions of the constitution, it can declare them as unconstitutional.

In India judicial review is borrowed from the USA but with a difference. In the USA the power of Judicial review works on the principle of Due process of Law, while in India the power of Judicial review works on the Principle of Procedure established by Law. On the process of Due process of Law Supreme Court of USA can comment on Justness and Unjustness of the policy matters while as per the procedure established by Law Indian Judiciary is not supposed to comment on policy matters.

Question 9.
What is the role of the Supreme Court in India?
Answer:
Oft the basis of the power of Judicial review the Supreme Court is playing a very effective role in a different way on which we can enumerate as following

1. It is the final authority of the interpretation of the constitution.
2. It checks the arbitrariness of executives and legislature.
3. It protects the Fundamental Rights of the people.
4. It strengthens democracy.
5. It protects the Indian Federal System
6. It checks the bureaucracy
7. It acts as guardian of the constitution
8. It helps in the evolution of the Constitution.

Question 10.
Explain the Advisory Jurisdiction of Supreme Court of India.
Answer:
Besides the original and appellate jurisdiction, the Supreme Court of India possesses Advisory Jurisdiction also. Under this jurisdiction, the President of India can seek opinion on any matter which involves the interpretation of the Constitution. However, the Supreme Court of India is not bound to give its advice nor the President of India is bound to accept the advice of the Supreme Court which he has sought. The utility of this jurisdiction is twofold. Number one it gives the opportunity to government to seek the legal opinion of the Supreme Court on the matter of greater importance and consequences. Secondly in the light of the advice of Supreme Court government can make necessary changes.

Question 11.
What do you mean by Judicial Activism?
Answer:
The term Judicial activism is much talked about in legal, political and academic circle in India. On the basis of Judicial activism, the courts have given revolutionary decisions is different areas which have demoralised the legislature and executive who are particularly vocal infusing the concept of Judicial activism. However, it is found to be more people-friendly.

Judicial activism means working of Judiciary beyond its limit, which is permitted under the principle of procedure established by law which says

Under this jurisdiction, the Supreme Court listens to the case related to
a. Dispute between the centre and state
b. The dispute between two states
c. Fundamental Rights
d. Interpretation of the constitution

2. Appellate Jurisdiction: Under this jurisdiction Supreme Court listen to the appeal against the decisions of the High Courts in all the civil, criminal and constitutional matters.

3. Advisory Jurisdiction: Under this jurisdiction, the Supreme Court can give its opinion to the President on any issue on which he seeks its opinion.

Question 12.
What are PIL(Public Interest Litigation) cases?
Answer:
PIL (Public Interest Litigation) is the chief instrument through which the Judicial activism has flourished in India.

PIL are those, cases which have been filed not by the aggrieved persons or parties but by the other, spirited persons and organisations on their behalf in Larger Public interests. Supreme Court of India took up the cases about rights of Prisoners which opened the gates for others.

Many Voluntary organisations have sought judicial intervention for the protection of existing rights and interest of the poor people and for improving the conditions of the poor people, protection of the environment and may other issues in the interest of public and \yeaker sections of the society. Justice P.N. Bhagwati had played a pioneering role in. the field of PIL (Public Interest Litigation case).

Question 13.
What is the importance and impact of PIL Public Interest Litigation Court?
Answer:
PIL is a revolutionary development in Indian Judiciary and is the consequence of Judicial activism and is an indicator of the changes which are taking place in social and economic set up in India.

Through the PIL, the courts have expanded the idea of rights, clean air, pure drinking water, decent living and dignified living are accepted as the essential rights of every man in the society. It was therefore felt by the courts that the individuals are part of the society must have the right to seeks Justice when such rights are involved. Through PIL Judiciary has become more liberal and human.

Judicial activism has a manifold impact on Indian Political System. People have largely found it and accepted as people-friendly. It has also made the executive more conscious and accountable to the people. Transparency in official working is the impact of Judicial activism; In fact, through Judicial activism, Judiciary has put the executives on their toes.

Question 14.
Mention some negative impact of Judicial activism.
Answer:
No doubt that Judicial activism in India is people friendly but it is certainly a violation of the principle procedure established by law because judicial activism is based on the process of law which does not prevail in India.

There is the negative side of Judicial activism which are as under:

1. It is the violation of principle separation of power
2. It is an attack on the autonomy of executive and legislative.
3. An ultimate representative of the people of the interest of the people is legislative which are elected by the people.
4. It will lead to high handedness of Judiciary.
5. It will lead to conflict between the legislature and judiciary and will be a hindrance in the working of the Government.

Question 15.
Explain the conflict between the Judiciary and Parliament over the issue of amendment of Fundamental Rights.
Answer:
Since the very implementation of the Constitution, there has been conflicting between the Parliament and Judiciary over the amendment of Fun-damental Rights. Some of the causes of this conflict are used under.

1. Shankari Prasad Case 1951
2. Golak Nath case 1967
3. Keshwanand Bharti Case 1973
4. Minerva Mill Case 1980

Now it is the latest position that Parliament can amend every part of the Constitution including the Fundamental Rights but cannot amend the basic structure of the constitution.

Judiciary Important Extra Questions Long Answer Type

Question 1.
Write the composition and role of the Supreme Court of India.
Answer:
Judiciary in India occupies the place of dignity and prestige. We have an independent, impartial and powerful judiciary. It is an integrated one. At its apex is Supreme Court which has administrative and Supervisory control on the lower courts.

The Supreme Court consists of 26 Judges including the Chief Justice of India. The Chief Justice of India is appointed by the President of India by the principle of seniority. Other Judges are appointed by the President with the consultation of Chief Justice of India. The Judges of the Supreme Court enjoy long tenure. They get retirement at the age of 65 years. They can be removed from the officers by way of impeachment by Parliament. Supreme Court of India has a power of Judicial review and exercises the jurisdiction in the following areas:

1. Original Jurisdiction
2. Appellate Jurisdiction
3. Advisory Jurisdiction
4. Miss.

Supreme Court of India has assumed more and m^fe powers and role in Indian Political System. It is working beyond its/jurisdiction and has the renowned name of Judicial activism. L.N. Bhagwati has filter done revolutionary step by accepting the principle ofPIL (Public Interest Litigations) which means by filing the case by an others-the aggrieved person who an unable to move to the courts themselves. Judiciary has played’ important role in

1. Checking the arbitrations of executive/ln India
2. It is defending the democracy in India
3. It is protecting the Fundamental Rights of the people.
4. It is working as guardian of the Constitution

Here we are providing 1 Mark Questions for Economics Class 12 Chapter 4 Poverty are the best resource for students which helps in class 12 board exams.

One Mark Questions for Class 12 Economics Chapter 4 Poverty

Question 1.
What do you mean by poverty?
Answer:
Poverty is the inability to secure the minimum consumption requirements for life, health and efficiency.

Question 2.
What proportion of the world’s poor live in India?
Answer:
One-fifth of the world’s poor live in India.

Question 3.
How many children under the age of five die annually in India according to UNICEF?
Answer:
About 2.3 million children under the age of five die India per annum according to UNICEF.

Question 4.
Name the two key features of poorest households.
Answer:
The two key features of poorest households are hunger and starvation.

Question 5.
What are the factors responsible alarming malnutrition among the poor?
Answer:
Ill health, disability and serious illness are the factors responsible for alarmingly high malnutrition among the poor.

Question 6.
Define poverty line.
Answer:
Poverty line estimates the minimum level of income that is considered appropriate to secure basic necessities of life.

Question 7.
What was the percentage of population below poverty line in 2011-12 in India?
Answer:
22 percent of India’s population lived below poverty line in 2011 -12.

Question 8.
Name the two types of poverty.
Answer:
The two types are absolute poverty and relative poverty.

Question 9.
Define absolute poverty.
Answer:
Absolute poverty determines the minimum physical quantities of requirement for a subsistence level, with the help of poverty line.

Question 10.
What is relative poverty?
Answer:
Relative poverty refers to lack of resources in relation to different classes,: regions and countries.

Question 11.
State the minimum calorie requirement (per day) of a person in rural area and a person in urban area
Answer:
The minimum calorie intake (per day) for a rural person is estimated at 2,400 calories while that for a person in urban area is 2,100 for a person.

Question 12.
How is the extent of poverty worked out in India?
Answer:
The extent of poverty in India is worked out with the help of “Head Count Ratio”.

Question 13.
Define Head Count Ratio.
Answer:
Head Count Ratio is the proportion of persons living below the poverty line.

Question 14.
Name some factors, other than income and expenditure, which are associated with poverty.
Answer:
Some factors, other than income and expenditure, which are associated with poverty, include accessibility to basic education, health care, drinking water and sanitation

Question 15.
Name the state in India which had the highest poverty in 2011-2012.
Answer:
In 2011 -2012, Chhattisgarh had the highest poverty in India.

Question 16.
List any two causes of poverty in India.
Answer:
Causes of poverty in India are:
(i) Lack of quality education
(ii) No or limited access to health care
(iii) Unequal distribution of income and wealth

Question 17.
Why are casual labourers among the most vulnerable group in society?
Answer:
Casual labourers are among the most vulnerable in society as they suffer lack of job security, assets, skills, opportunities and have no surplus to sustain them.

Question 18.
Give two examples of self-employment programmes initiated by the government to alleviate poverty.
Answer:
Two self-employment programmes initiated by the government include are Rural Employment Generation Programme (REGP) and Prime Minister’s Rozgar Yojana (PMRY).

Question 19.
Name the three major programmes that aim at improving the food and nutritional status of the poor.
Answer:
Three major programmes that aim at improving the food and nutritional status of the poor are:
(i) Public Distribution System
(ii) Integrated Child Development Scheme
(iii) Mid-day Meal

Here we are providing 1 Mark Questions for Political Science Class 12 Chapter 9 Globalisation  are the best resource for students which helps in class 12 board exams.

One Mark Questions for Class 12 Political Science Chapter 9 Globalisation

Question 1.
What is meant by flows in relation to globalisation ? (C.B.S.E. 2008)
Answer:
In globalisation, flows represent the flow or movement of ideas, capital, commodities and people from one part of the world to another.

Question 2.
What caused India’s resistance to Globalisation ?
Answer:
Globalisation has been opposed in India because the gap between the rich and the poor exapanded considerably.

Question 3.
How far is it correct to say that globalisation results in the erosion of state sovereignty ? (C.B.S.E. 2009) (Imp)
Answer:
Globalisation has adversely affected the sovereignty of state in many ways. Interdependence of the state compelled the state to surrender part of sovereignty i.e.. external sovereignty.

Question 4.
How far is it correct to say that globalisation actually increases the activities of the state ? (C.B.S.E. 2009) (Imp)
Answer:
It is true to a great extent that globalisation has increased the activities of the state.

Question 5.
Give any one example to show that events taking place in one part of the world could have an impact on another part of the world. (C.B.S.E. 2017)
Answer:
Policies made by International Monetary Fund (IMF) and World Trade Organization effect the whole world.

Question 6.
How far do you agree with the statement that cultural globalization is dangerous not only for the poor countries but for the entire globe? (C.B.S.E. 2017)
Answer:
The effects of globalization are not confined only to the sphere of politics and economy, but its cultural effect give birth to cultural homogenisation. The culture of the politically and economically dominant society leaves its imprint on a less powerful society. This is dangerous not only for the poor countries but for the whole humanity. It leads to the shrinking of the rich cultural heritage of the entire globe.

Question 7.
Distinguish between cultural homogenization and cultural heterogenisation. (C.B.S.E. 2017)
Answer:
The cultural effect of globalisation leads to the rise of a uniform culture and it is given the name cultural ‘homogenisation’. In this culture of the politically and economically dominant society leave its imprint on the less powerful society—it is homogenisation. The process of adoption of elements of global culture to local culture is known as cultural heterogenization.

Question 8.
Give a suitable example to show that globalisation need not always be positive. (C.B.S.E. 2017)
Answer:
In globalisation market based economy and capitalism has made rich more riches and poor more poorer.

Question 9.
Assess the impact of Valentine’s Day on Indian culture. (C.B.S.E. 2017)
Answer:
Celebrations of Valentine Day is the cultural impact of globalisation which was never a part of Indian society in the past. Now a days press, T.V. students of school and colleges and advertising agencies and marketing etc. try to make this event very colourful. Many organisation and political parties oppose these celebration of Valentine Day.

Question 10.
Why does mineral Industry invite criticism and resistance in various parts of the globe? Give any one major reason. (C.B.S.E. 2017)
Answer:
There is great criticism and resistance in various parts of the globe for mineral industry for the possession of developed nations on it.

Question 11.
When the first meeting of WSF was held?
(a) Port Alerge in 2001
(b) Mumbai in 2000
(c) New Delhi in 2004
(d) London in 1998.
Answer:
(a) Port Alerge in 2001

Question 12.
Globalisation begin in :
(a) 1981
(b) 2000
(c) 1989
(d) 1991
Answer:
(d) 1991

Question13.
When the Seventh meeting of WSF was held ?
(a) Nairobi in January 2007
(b) New York in January 2007
(c) New Delhi in January 2007
(d) Paris in March 2007.
Answer:
(a) Nairobi in January 2007

Question 14.
In which meeting there was a protests alleging that unfair trading practised by the developed countries ?
(a) WTO ministerial meetings at Seattle in 1992.
(b) IMF meetings at Washington in 1994.
(c) WTO ministerial meeting at Paris in 1990.
(d) WTO ministerial meeting at London in 1988.
Answer:
(a) WTO ministerial meetings at Seattle in 1992.

Question 15.
Which of the following statements is not true ?
(a) Global economy is the most important feature of globalisation.
(b) Global movements have nothing to do with globalisation.
(c) Competitive economy is a basic feature of globalisation.
(d) WTO is an indication of globalisation process.
Answer:
(b) Global movements have nothing to do with globalisation.

Here we are providing 1 Mark Questions for Political Science Class 12 Chapter 8 Environment and Natural Resources are the best resource for students which helps in class 12 board exams.

One Mark Questions for Class 12 Political Science Chapter 8 Environment and Natural Resources

Question 1.
Why is it said that history of : Nffhlj Petroleum is also the history of war | and struggle ? (C.B.S.E. 2016)
Answer:
Petroleum is very useful and valuable element in this Modern-World. One can not think of any life and working without this essential element. Due to its essentiality and importance history of Petroleum is the history of war and struggle.

Question 2.
Who are considered as ‘indigenous people’ in India? (C.B.S.E. 2019)
Answer:
In India, scheduled tribes are considered as Indigenous people.

Question 3.
Write the U.N. definition of Indigenous populations. (C.B.S.E. 2019)
Answer:
According to U.N. “Indigenous people are comprising the descendants of people who inhabited the present territory of a country at the time when persons of a different culture or ethnic origin arrived there from other parts of the world and overcame them.”

Question 4.
What aroused a sense of common concern among the indigenous communities of the world during the 1970s? (C.B.S.E. 2019)
Answer:
Capitalist and M.N.C are draining their natural resources by illegal means and sometimes people are also compelled for immigration from their native places.

Question 5.
Why are India and China exempted from Kyoto Protocol? (C.B.S.E. 2008 Outside Delhi)
Answer:
India and China were exempted from the requirement of Kyoto Protocol because their contribution to the emission of greenhouses gases during the industrialisation period was insignificant.

Question 6.
Highlight the major objective of UNFCCC. (C.B.S.E. Sample Q.P. 2017)
Answer:
Protection of climate system on the basis of equality and in accordance with their common but differentiated.

Question 7.
Why should the ‘environmental concern’ be a part of Contemporary Global Politics ? (C.B.S.E. 2009)
Answer:
Environmental concern must be a part of Contemporary Global Politics because traditional patterns of economic growth are not sustainable in the long term. A balance has to be created between economic growth and ecological conservation.

Question 8.
The Earth Summit was held at :
(a) London
(b) New York
(c) New Delhi
(d) Rio-de-Janeiro.
Answer:
(d) Rio-de-Janeiro.

Question 9.
World Environment Day is celebrated each year on :
(a) 5 March
(b) 5 June
(c) 5 January
(d) 7 June.
Answer:
(b) 5 June

Question 10.
The United Nations Framework Convention on Climate Change was held in:
(a) 2004
(b) 1997
(c) 2001
(d) 1992.
Answer:
(d) 1992.

Question 11.
India signed and ratified the 1997 Kyoto Protocol in :
(a) August 2002
(b) July 2006
(c) August 2000
(d) August 2005.
Answer:
(a) August 2002

Question 12.
The World Council of Indigenous Peoples was formed in :
(a) 1950
(b) 1975
(c) 1990
(d) 2007.
Answer:
(b) 1975

Question 13.
Earth Summit was attended by :
(a) 170 states
(b) 59 states
(c) 191 states
(d) 184 states.
Answer:
(a) 170 states

Here we are providing 1 Mark Questions for Political Science Class 12 Chapter 6 International Organisations are the best resource for students which helps in class 12 board exams.

One Mark Questions for Class 12 Political Science Chapter 6 International Organisations

Question 1.
Who is the present Secretary General of the United Nations ? (C.B.S.E. 2014)
Answer:
Antonio Guterres from Portugal, is the present Secretary General of U.N.

Question 2.
Mention any two agencies of | the United Nations. (C.B.S.E. 2014) :
Answer:
(i) UNESCO
(ii) ILO are the two agencies of the United Nations.

Question 3.
What is World Health Organisation (WHO)? (Imp.) (C.B.S.E. 2010)
Answer:
World Health Organisation was established in 1940. Its main aim is to improve the health standard of the people of the world. It has three organs.
(i) World Health Council,
(ii) Executive Board and
(iii) Secretariat.

Question 4.
Write the full form of I.M.F. (C.B.S.E. 2010 Delhi)
Answer:
International Monetary Fund.

Question 5.
Highlight any one benefit of having an international organization. (C.B.S.E. 2019)
Answer:
International organization can maintain peace and order in the world.

Question 6.
How many permanent members and how many non-permanent members are there in the U.N. Security Council? (C.B.S.E. 2013)
Answer:
There are 5 permanent and 10 non-permanent members in the U.N. security council.

Question 7.
Mention any one objective of the United Nations ? (C.B.S.E. 2013)
Or
What is the main objectives of the United Nations. (C.B.S.E. 2014)
Answer:
To save succeeding generations for the scourage of war.

Question 8.
Correct the following statement and rewrite.
Eight temporary members of the U.N. Security Council are elected by the general assembly for a period of two years. (C.B.S.E. 2012 Delhi)
Answer:
Ten temporary members of the U.N. Security Council are elected by the general assembly for a period of two years.

Question 9.
Mention the main function of the World Trade Organisation. Imp. (C.B.S.E. 2013)
Answer:
The World Trade Organisation sets the rules for global trade.

Question 10.
Mention any one function of the World Bank. (C.B.S.E. 2013)
Answer:
World Bank provides loans and grants to the member countries.

Choose the correct answer :

Question 11.
India became the member of U.N. in :
(a) 1945
(b) 1947
(c) 1950
(d) 1962.
Answer:
(a) 1945

Question 12.
Who was the first woman President of the U.N. General Assembly?
(a) Sarojini Naidu
(b) Aruna Asaf Ali
(c) Vijay Lakshmi Pandit
(d) Raj Kumari Amrit Kaur.
Answer:
(c) Vijay Lakshmi Pandit

Question 13.
Which one of the following is not a permanent member of the U.N. Security Council ?
(a) Russia
(b) India
(c) China
(d) France.
Answer:
(b) India

Question 14.
Who blocked the Second term for Boutros Ghali as Secretary General ?
(a) USA
(b) India
(c) France
(d) China.
Answer:
(a) USA

Question 15.
“The United Nations was not created to take humanity to the heaven, but to save it from the hell.” Who made this statement ?
(a) Pt. Jawaharlal Nehru
(b) Kofi Annan
(c) Ban Ki-moon
(d) Dag Hammarskjold.
Answer:
(d) Dag Hammarskjold.

Here we are providing Class 12 Geography Important Extra Questions and Answers Chapter 2 Data Processing. Geography Class 12 Important Questions are the best resource for students which helps in class 12 board exams.

Class 12 Geography Chapter 2 Important Extra Questions Data Processing

Data Processing Important Extra Questions Short Answer Type

Question 1.
What do you mean by ‘Measures of central tendency’ ?
Answer:
“Measures of Central Tendency is an important technique for statistical analysis. Sometimes, it becomes necessary to obtain a single representative value for the entire data. This value enables one to make comparisons between different types of data. As these  figures are average and lie in the middle of the values, it is known as ‘measures of central tendency.’ The summary values that are representative of the various distributions are known as measures of central tendency. The commonly used measures of central tendency are :

• Arithmetic mean or the Average
• Median
• Mode.

Question 2.
What is a Mean ? What are its types ?
Answer:
Mean or average is a quotient of the sum of several quantities. It is a number that by and large represents a series of numbers. So, average can be taken as the central value or tendency of the population, in this case, all the students of your class.

Mean or average can be of three types :
1. Arithmetic
2. Geometric, and
3. Harmonic mean.

Question 3.
Define the term Arithmetic ‘mean’. How is it determined ?
Answer:
Mean
The most frequently used measure of central tendency is the mean or the average. The mean is simply the sum of all the values of a series divided by their number. Example. Suppose the monthly income of five agricultural labour families in a village is ₹ 100, ₹ 80, ₹ 120, ₹ 90 and ₹ 60. The mean monthly income of these families
\(\overline{\mathrm{X}}=\frac{100+80+120+90+60}{5}=\frac{450}{5}=₹ 90\)

Procedure:
(i) This is a simple measure of average.
(ii) Add all the values to get Σx
(iii) Divide it by the number of items (N)
\(\text { Mean }=\bar{x}=\frac{\Sigma x}{N}\)

(1) For Ungrouped data. Supposing there are ‘n’
agricultural labour families in a village and their income of the 1st, 2nd, 3rd and the nth family is Xj, X2, X3 Xn. The mean would be
\(\begin{aligned}
\overline{\mathrm{X}} &=\frac{\mathrm{X}_{1}+\mathrm{X}_{2}+\mathrm{X}_{3} \ldots \ldots \ldots . .+\mathrm{X}_{n}}{n}=\frac{\Sigma \mathrm{X}}{n} \\
\text { where } \overline{\mathrm{X}} &=\text { mean }
\end{aligned}\)
∑ X = the sum of all values
n = no. of items

(2) For Grouped data. In this case, the mean is calculated by the following two methods:
(i) Direct method
(ii) Short-cut method.
Example. Calculate the mean for the data given in the following table:

S. No.	Land Holding (Acres)	Number of Farmers
1.	0 – 5	4
2.	5 – 10	5
3.	10 – 15	8
4.	15 – 20	10
5.	20 – 25	3
	Total	30

Procedure.
(i) This is the simplest method where the frequency (l) of each item is given.
(ii) Find out the mid-value of each class interval. Multiply it by the frequency to find out f(X).
(iii) Add all the values of f(X) and divide it by the number of items.
\(\overline{\mathrm{X}}=\frac{\Sigma f(\mathrm{X})}{\mathrm{N}}\)
Solution:
(i) Direct Method

Land Holding	Number of Farmers (f)	Mid-Values (X)	f(X)
0 – 5		2.5	4 x 2 – 5 = 10.0
5 – 10	5	7.5            ‘	5 x 7 – 5 = 37 – 5
10 – 10	8	12.5	8 x 12 – 5= 1000
15 – 20	10	17.5	10 x 17 – 5 = 175 0
20 – 25	3	22-5	3 x 22 – 5 = 67 – 5
		n=30	∑f(x) = 3900

\(\text { Mean }=\overline{\mathrm{X}}=\frac{\Sigma f x}{n}=\frac{390}{30}=13 \text { acres. }\)

(ii) Short-cut Method

Procedure :
(i) Find out the mid-value (x) of each class interval.
(ii) Assume a mid-value as assumed Mean.
(iii) Find out the deviation of different values (dX) from the assumed Mean by subtracting the assumed average (A) from the mid-values (X).
(iv) Multiply (dx) by frequency (f) and add these
\(\overline{\mathrm{X}}=\mathrm{A}+\frac{\Sigma f d x}{\mathrm{~N}}\)
Assumed Mean = 12.5 acres.

Land Holdings	Mid­ values (x)	dx = X-A	Number of Farmers (f)	f.dx
0 – 5	2.5	-10	4	-10
5 – 10	7.5	-5	5	-25
10 – 15	12.5	0	8	0
15 – 20	17.5	5	10	50
20 – 25	22.5	10	3	30
			n = 30	Σ.f.dx- 15

\(\begin{aligned}
\bar{X} &=A+\frac{\Sigma f d x}{n} \\
&=12 \cdot 5+\frac{15}{30}=13 \text { acres. }
\end{aligned}\)

Question 4.
Calculate the mean for the following data.
Answer:

Marks obtained	No. of Students
0-10	5
10-20	10
20-30	40
30 – 40	20
40 – 50	25

Solution:
(i) Direct Method

Marks Obtained	Mid-values (X)	No. of Students (f)	f.x.
0-10	5	5	5×5 ~25
10-20	15	10	15 x 10 = 150
20-30	25	40	25 x 40= 1000
30 – 40	35	20	35 x 20 = 700
40 – 50	45	25	45 x 25 = 1125
		n = 100	∑fx = 3000

\(\begin{aligned}
\text { Mean } &=\overline{\mathrm{X}}=\frac{\Sigma f x}{n} \\
&=\frac{3000}{10}=30
\end{aligned}\)

(ii) Short-cut method :
Assumed Mean = 25

Marks Obtained	Mid-values X	dx = X – A	No. of Students (f)	f.dx
0 – 10	5	-20	5	-100
10 – 20	15	-10	10	-100
20 – 30	25	0	40	0
30 – 40	35	10	20	200
40 – 50	45	20	25	500
			n = 100	∑  fdx = 500

\(\begin{aligned}
\text { Mean }=\bar{X} &=A+\frac{\Sigma f d x}{n} \\
&=25+\frac{500}{100}=25+5=30 \text { marks. }
\end{aligned}\)

Question 5.
Calculate the mean from the given data of rainfall of a place.

Rainfall (Millimetres)	No. of Days
30 – 35	5
35 – 40	6
40 – 45	11
45 – 50	18
50 – 55	19
55 – 60	15
60 – 65	13
65 – 70	1
70-75	2

Solution:
(i) Direct Method :

Rainfall	Mid­ values (x)	No.of Days (f)	f.x
30-35	32.5	5	162.5
35-40	37.5	6	225.0
40-45	42.5	11	467.5
45 – 50	47.5	18	855.0
50 – 55	52.5	19	997.5
55 — 60	57.5	15	862.5
60-65	62.5	13	812.5
65 – 70	67.5	1	67.5
70-75	72.5	2	145.0
		n = 90	∑ fx = 4595.0

\(\begin{aligned}
\text { Mean }=\overline{\mathrm{X}} &=\frac{\Sigma f x}{n} \\
&=\frac{4595}{90}=51.06 \mathrm{~mm} .
\end{aligned}\)

(ii) Short-cut method :

Question 6.
The percentage of marks obtained by 50 students is given below. Calculate the Arithmetic mean.
Answer:
Percentage of Marks

59	47	87	46(L)	91
68	80	65	50	69
95	77	96(H)	94	82
84	91	49	92	74
88	83	79	88	79
76	94	63	82	57
81	78	87	71	65
75	62	83	69	80
79	58	67	55	76
93	63	70	63	56

Answer:
We calculate the A.M. in the following steps:
Step-1. The lowest (L) and highest (H) values are L = 46, H = 96
Step-2. Calculate the range (R) by deducting L from H
i.e. R = H – L
∴ R = 96 – 46 = 50

Step-3. Divide the range by the desired number of classes (N) to determine the class intervals (C.I.)
\(\begin{array}{l}
\mathrm{C.I.}=\frac{\mathrm{R}}{\mathrm{N}} \\
\text { C.I. }=\frac{50}{5}=10
\end{array}\)
taking N = 5*
The number of classes should neither be too small nor too large.

Step-4. Determine the class the limits (lower and upper boundaries) by adding C.I. as many times with L as the number of classes till the H value is obtained.
Thus, the lowest limit = 46 (Lowest value)
next limit = 46 + (1 x 10) = 56
next limit = 46 + (2 x 10) = 66
next limit = 46 + (3 x 10) = 76
next limit = 46 + (4 x 10) = 86
and the highest limit = 46 + (5 x 10)
= 96 (Highest value)
So, 1st class will be from 46 to 56 Ilnd class will be from 56 to 66 Illrd class will be from 66 to 76 IVth class will be from 76 to 86
Vth class will be from 86 to 96.

Step-5. Arrange the data in the frequency table with 5 classes by tally methods

Step-6. Now the frequencies are multiplied by the respective mid-values and the products which are added to get
∑ f x m.

Step-7. The A.M. is calculated using
\(\begin{aligned}
\overline{\mathrm{X}} &=\frac{\Sigma f \times m}{\Sigma f} \\
\mathrm{A.M.} &=\frac{3750}{50}=75
\end{aligned}\)

Question 7.
Describe the merits and demerits of Arithmetic Mean.
Answer:
Merits of Arithmetic Mean.
1. Simplicity. It is the most simple of all the methods of central tendency and is easy to understand.
2. Representative Value. This is the representative value of all the values of a series.
3. Certain Value. It has a definite value and remains same all the time.
4. Stable Value. It has a stable value. The changes in the values of samples do not affect it.
5. Basis of Comparison. It can be used to compare the value of a series.
6. Fair Value. It gives a fair wholesome idea of all values.
7. Balanced Value. It balances the values of entire distribution.

Demerits of Arithmetic Mean
1. Effect of Extreme Values. The mean is affected by extreme values of the series because there is a wide gap. For example, the monthly salary of a company manager is ₹ 50,000/-. The monthly salary of the other three employees is ₹ 3500/-, ₹ 1500/-,₹ 1000/. The average salary will be:
\(\begin{array}{l}
=\frac{50000+3500+1500+1000}{4} \\
=\frac{56000}{4}=₹ 14000
\end{array}\)
This is not a representative value.
2. The Arithmetic mean is not present in the series.
3. Arithmetic mean sometimes gives a distorted picture.

Question 8.
What is Geometric Mean ? How is it calculated ?
Answer:
Geometric Mean (G.M.)
Geometric Mean (G.M.) is used to compute the rate of growth of any item.
The G.M. of n values, for example,
x₁, x₂, x₃  ………………..  x_n is defined as the nth root of the
product of these values.
So, we may express this mathematically as :
\(\text { G. M. }=\left(x_{1} \cdot x_{2} \cdot x_{3} \ldots x_{n}\right)^{\frac{1}{n}}\)
The G.M may be calculated using following example:
Growth rates of the economy in 4 years are given as 4%, 8%, 8% and 16% respectively.
\(\text { So G.M. }=(4 \times 8 \times 8 \times 16)^{1 / 4}\)
\(=(4096)^{\frac{1}{4}}\)

Uses of G.M.
G.M. is more suitable average if data are given in terms of ratios or in terms of change in which use of A.M. may lead to inconsistent results.

Question 9.
Define the term Median. How is it calculated ?
Answer:
Median. Median is used as a measure of central tendency. Median is the positional average. It is defined as the middle most value of variables. It is denoted as M. It is the mid-value of a series. Median is that value which divides a series into two equal parts. One- half includes the values which are more than the median while the second half includes the values which are less than the median. For example—40, 42, 38, 45, 50, 25, 55 has a median of 45.

Example. Calculate the median when age of five individuals A,B, C, D and E are respectively 20, 21, 19, 23 and 22 years.
We determine the median in the following steps: Step-1. Arrange the data is ascending or descending order in a tabular format:

Step – 2. The value of the middle number is the median.
Step – 3. The middle number is calculated by

Therefore, the value of 3rd number will be the median.
Median = 21 years
\(\mathrm{M}=\frac{n+1}{2} \text { th item }\)
Where n = 4 (even), \(\text { so } \frac{n+1}{2}=\frac{5+1}{2}=3\)
So, if we have say 4 members, 19, 20, 21 and 22, in this case we will add the values of 2nd and 3rd number and divide the total by 2 :
\(\frac{20+21}{2}=20.5 \text { years}\)

In that case Median = 20.5 years.
Uses of Median
1. Median is the middle most value, so the extreme values do not vitiate its representative character as in case of A.M.
2. The median is the centre of gravity for the entire distribution.
3. Use of median is however not very common. The Median (M) for a large number of distributions

Question 10.
The monthly income of 12 households in a locality are ₹ 140,150,130,135,170,190, 500, 210, 205,195, 290, 200.
Find out the median income.
Answer:
(1) Arrange the income in an ascending order as :
130, 135, 140, 150, 170, 190, 195, 200, 205, 210, 290 and 500.
(2) Arrange the incomes in descending order as 500, 290. 210, 205, 200,195,190, 170, 150,140, 135 and 130

Question 11.
The following table gives the mean monthly temperatures of Delhi. Find out the median temperature of Delhi.

Sr. No.	1	2	3	4	5	6	7	8	9	10	11	12
Month	J	F	M	A	M	J	J	A	S	O	N	D
Temp. (°C)	14	16	22	2$	33	34	31	30	29	26	20	15

Answer:
Arrange the figures in ascending order and descending order.

Question 12.
Describe the merits and demerits of Median.
Answer:
Merits of median :
1. It is easy to calculate the median.
2. It can be calculated in the absence of complete data.
3. It is not affected by extreme values of a series.
4. It can be easily calculated in an open ended series.
5. It can be calculated graphically.
6. It has a definite value.
7. It is the representative value of a series and in the centre of gravity for the entire distribution of a series.

We can determine the Mode in the following steps—

Demerits
1. It takes more time to arrange the series in ascending and descending order.
2. It does not depend on all the values of a series.
3. It is not an exact value as it does not consider the extreme values.
4. It is an approximate value when the values are even.
5. It is not a suitable method for irregular values.
6. In case of large number of values, it is difficult to calculate median.

Question 13.
Define the term mode. How is it calculated ? Describe its merits and demerits.
Answer:
Mode. Mode is a positional average. It is a value of the variable which occurs most frequently. Mode is a measure of central tendency and is the most frequently occurring value in a series. It is a typical value around which most of the items tend to cluster. It is the representative value of a series around which there is maximum concentration.

Inspection method to determine the mode. In this method, the value is determined which occurs mostly in a series. When the frequency of a discrete series increases or decreases regularly, the mode is clearly known.

Example :

Wages (₹)	No. of workers
10	2
20	3
30	7
40	8
50	4
60	5

It is evident that the frequency of ₹ 40 is the maximum. Therefore, the mode is Z = ₹ 40.
Example. Determine the mode from the following distributions—
2, 10, 5, 7, 7, 9, 2, 7, 11, 17, 7, 8

SI. No./Type	1	2	3	4	5	6	7	8	9	10	11	12
Ascending	2	2	5	7	‘ 7	7	7	8	9	10	11	17
Descending	17	11	10	9	8	7	7	7	7	5	2	2

Step 1. Arrange the data in ascending and descending order in Table.
Step 2. In both the ascending and descending order value ‘7’ is occurring most frequently i.e. 4 times.
So the mode is 7.

Merits of Mode.
(i) It is easy to understand mode by inspection method
(ii) Extreme values do not affect mode
(iii) It is the representative value of a series
(iv) It can be calculated in the absence of complete data
(v) Mode can be used in certain types of distributions, in which values are whole numbers
(vi) It can be shown on a graph also.

Demerits of Mode
(i) It becomes uncertain value when two variables occur equal times
(ii) It is not based on all the variables
(iii) It sometimes gives a distorted picture.

Question 14.
What do you mean by measures of deviation ?
Answer:
Measures of Deviation
Deviation or Dispersion simply means scattering or variation of the variables about a central value.
We can take four alternative measures of deviation—

• Range
• Mean Deviation or Absolute Deviation
• Standard Deviation
• Quartile Deviation

Question 15.
What is range ? How is mean deviation calculated ?
Answer:
Range (R)
The range is the simplest of the measures of deviation. It is defined as the difference between the highest (H) and the lowest (L) values of a given set of measurements.
Range = Highest – Lowest

Step 3. So the mean deviation is
\(\frac{\Sigma|X-\bar{X}|}{N}=\frac{12}{5}\)

Question 16.
What do you mean by Standard Deviation and Quartile Deviation ?
Answer:
Standard Deviation
Another measure of deviation calculated using A.M.
\((\overline{\mathrm{X}})\) is Standard Deviation (σ) This is also known as Root Mean Square Deviation. This can be mathematically formulated as:
\((\sigma)=\sqrt{\left[\frac{\sum(\mathrm{X}-\overline{\mathrm{X}})^{2}}{\mathrm{~N}}\right]}\)

Example. Determine the range for the following percentage of marks obtained by 5 students 83, 96, 72, 46, 68 respectively.
Answer:
We determine the range in the following manner—
Step 1. Determine the highest (H) and lowest (L) values in this case H = 96 and L – 46.
Step 2. We calculate the range using formula R = H – L
(Range) R = 96 — 46 = 50%

Use of Range
A range is rather a crude measure of deviation because it does not indicate anything about the way the values are distributed within the range.

Mean Deviation Or Absolute Deviation
Mean Deviation (M.D.) is the absolute deviation (i.e. ignoring sign) from Mean \((\overline{\mathrm{X}})\)
We can mathematically put M.D. as—
\(\text { M.D. }=\frac{\Sigma|X-\bar{X}|}{N}\)
Where X = any value of population
\((\overline{\mathrm{X}})\) = The mean of population
N = Total number of population

Example.
Determine the Mean Deviation (M.D.) for the following population 6, 8, 4, 12, 5.
Answer:
We calculate the M.D. in the following steps:

Step 1. Calculate A.M. (X) as mentioned earlier.
\(\overline{\mathrm{X}} \text { (Arithmetic Mean) }=\frac{6+8+4+12+5}{5}=\frac{35}{5}=7\)

Step 2. Deduct the value of \((\overline{\mathrm{X}})\) from individual member in a tabular format. The square of the S.D. is known as ‘variance’.

Example. Calculate Standard Deviation of the following distribution of 6,8,4,2,5.
Answer:
We calculate the S.D. (σ) in the following steps:
Step 1. Determine the A.M \((\overline{\mathrm{X}})\)
\(\overline{\mathrm{X}}=\frac{6+8+4+2+5}{5}=5\)

Step 2. Determine the square deviations from A.M. in a tabular format as shown in table below.
\(\sigma=\sqrt{\frac{20}{5}}=\sqrt{4}=2\)

So the Standard Deviation of the above distribution is
σ = 2

Quartile Deviation

Median (M) divides the total distribution into two equal halves but quartiles divide the total distribution into 4 equal parts. This is called Partition value also. Any series has three Quartiles Q₁, Q₂ and Q₃.

1. The lower quartile (Q₁) is such that 14th of the total distribution will be less than it and %th of the total distribution will be above this value.
2. The middle Quartile (Q₂) is such that 54 of the total distribution will be below it and 14 above it. Thus, middle quartile (Q₂) is nothing but the median (Me).
3. The upper quartile Q₃ will divide the entire distribution in such a manner that %th will be less than this and 14th above it. The quartile deviation (Q.D.) is called as semi interquartile range which may be expressed as—
   \(\text { Q.D. }=\frac{\mathrm{Q}_{3}-\mathrm{Q}_{1}}{2}\)

Example Determine the Quartile Deviation for the following distribution
46, 32, 25, 50, 72, 35, 75, 65, 58
Answer:
We can determine the Q.D. in the following steps:
Step. 1. Arrange the distribution in ascending order and rank them serially as below:

Step 2. The middle quartile (Q₂) or the median (Me) is the middle most member, i.e.

Question 17.
What is Correlation ? How is correlation coefficient determined ?
Answer:
Correlation
A distribution of two sets of variables is known as bivariate distribution where these two variables are said to be correlated if the change of one variable results in a corresponding change in the other variable. The first variable causing a change of second variable is known as independent variable (X) and the other variable is known as dependent variable (Y).

As estimation of the strength and direction of the association between two variables is called simple or bivariate correlation. The most commonly used measures of correlation is known as Pearson’s product moment correlation co-efficient (r).

The value of r ranges between + 1 to – 1. Thus, when:

• r = + 1. The correlation is perfect and positive.
• r – 0. No correlation or Zero correlation.
• r = – 1. The correlation is perfect and negative.

The product moment correlation co-efficient may be mathematically expressed as:
\(r=\frac{N \sum X Y-\sum X \sum Y}{\sqrt{\left[N \sum X^{2}-\left(\sum X\right)^{2}\right]\left[N \sum Y^{2}-\left(\sum Y\right)^{2}\right]}}\)

Where X is the independent variable
Y is the dependent variable
N is the total number of distribution.

Question 18.
Calculate the Pearson’s Correlation Coefficient from the following size of plots and selling prices:

Plot size (ha)	10	16	19	22	28
Price (₹)	45	55	75	100	105

Answer:
Step—I. Arrange the data sequentially in a proper table showing separately the two variables. Add them separately.

Step-2. Using formula we calculate Pearson’s Product moment correlation co-efficient. So it may be said that price of land is perfectly and positively correlated with the size of the land.

So it may be said that price of land is perfectly and positively correlated with the size of the land.

Question 19.
Compute the Standard Deviation from the following data
15, 18, 20, 12, 10, 9, 11.

Find out the root deviation from the Table.

Standard Deviation (S.D.)
\(=\rho=\sqrt{\frac{\Sigma(\mathrm{X}-\overline{\mathrm{X}})^{2}}{\mathrm{~N}}}=\sqrt{\frac{86}{7}}=\sqrt{12.28}=3.5 \)

Question 20.
The table below shows the relation between teachers and students of a school. Find out the nature of correlation between the teachers and students.

Answer:
Show the two variables separately. Multiply X and Y. Find out the squares of each.

Here we are providing Class 12 Geography Important Extra Questions and Answers Chapter 4 Human Settlements. Geography Class 12 Important Questions are the best resource for students which helps in class 12 board exams.

Class 12 Geography Chapter 4 Important Extra Questions Human Settlements

Human Settlements Important Extra Questions Very Short Answer Type

Question 1.
What is a settlement ?
Answer:
A settlement is a cluster of dwellings of different sizes.

Question 2.
Name any two modern towns built by the British in modern style? (Sample Paper 2018-19)
Answer:
Mumbai, Chennai.

Question 3.
Which town was developed as centre of modern industries after 1850? (Sample Paper 2018-19)
Answer:
Jamshedpur.

Question 4.
Name any two towns of India, initially developed as educational centres. (C.B.S.E. Outside Delhi 2017 Set-III)
Answer:
Varanasi, Aligarh, Pilani etc., developed as educational centres.

Question 5.
Name the local names of hamleted settlements.
Answer:
Panna, Para, Palli, Nagla and Dhani.

Question 6.
Why is India a popular tourist destination in the world. (CBSE 2018)
Answer:
Because of favourable climatic conditions, medical services, heritage home, national parks, etc.

Question 7.
Name the areas of dispersed settlements are found.
Or
Name any one state of India, where dispersed settlements are found. (Outside Delhi 2019)
Answer:
Meghalaya, Uttarakhand, Himachal Pradesh and Kerala.

Question 8.
Name two most ancient town in India. (C.B.S.E. 2014)
Answer:
Varanasi and Ayodhaya.

Question 9.
Name three nodal towns of India.
Answer:
Mumbai, Chennai and Kolkata.

Question 10.
Name three satellite towns around Delhi.
Answer:
Ghaziabad, Rohtak and Gurgaon.

Question 11.
What is the population size of the class I cities in India ?
Answer:
1,00,000 persons and above.

Question 12.
Which type of the rural settlement in India includes Panna, Para, Palli, Nagla, Dhani, etc.
Answer:
Hamleted settlement.

Question 13.
Give any two examples of mining towns in India, (C.B.S.E. 2013)
Or
Name any two towns of India, initially developed as mining towns. (C.B.S.E. Outside Delhi 2017 Set-11)
Answer:
Jharia and Raniganj.

Question 14.
Name any one area of hamleted settlement in India. (Outside Delhi 2019)
Answer:
Chattisgarh, Lower Valleys of Himalayas.

Human Settlements Important Extra Questions Short Answer Type

Question 1.
On what factors does the location of rural settlements depend ?
Answer:
There are various factors and conditions responsible for having different types of rural settlements in India.
These include:
(i) physical features – nature of terrain, altitude, climate and availability of water
(ii) cultural and ethnic factors social structure, caste and religion
(iii) security factors – defence against thefts and robberies.

Question 2.
Name the main types of rural settlements in India.
Answer:
Rural settlements in India can broadly be put into four types:
(i) Clustered, agglomerated or nucleated,
(ii) Semi-clustered or fragmented,
(iii) Hamleted, and
(iv) Dispersed or isolated.

Question 3.
What are administrative towns? State one example from India. (Sample Paper 2017-18)
Answer:
Towns supporting administrative headquarters of higher order are called administrative towns e.g. Chandgara.

Question 4.
What is the meaning of a settlement ? What is its base ?
Answer:
Human Settlement means cluster of dwellings or any type of size where human beings live. For this purpose, people may erect houses and other structures and command some area or territory as their economic support-base. Thus, the process of settlement inherently involves grouping of people and apportioning of territory as their resource base.

Question 5.
What is the basic difference between rural and urban settlements ? (C.B.S.E. Delhi 2017)
Answer:
The basic difference between rural and urban settlements is as follows :
The rural settlements derive their life support or basic economic needs from land based primary economic activities, whereas, urban settlements, depend on processing of raw materials and manufacturing of finished goods on the one hand and a variety of services on the other.

Question 6.
How does an agglomeration develop ?
Answer:
Majority of metropolitan and mega cities are urban agglomerations. An urban agglomeration may consist of any one of the following three combinations :
(i) a town and its adjoining urban outgrowths
(ii) two or more contiguous towns with or without their outgrowths, and
(iii) a city and one or more adjoining towns with their outgrowths together forming a contiguous spread.

Question 7.
State any three characteristics of clustered rural settlements in India.
Answer:
(i) This is a cluster of compact houses.
(ii) The General living area is separated from farming area.
(iii) These settlements are rectangular and linear in shape.

Question 8.
Classify Indian Towns on the basis of their evolution in three different periods. Name one town of each period. (C.B.S.E. 2009)
Answer:
The Indian towns are classified into three groups on the basis of their Evolution in different periods.

Types of Town Example :

• Ancient Towns — Pataliputra
• Medieval Towns — Agra
• Modern Towns — Chandigarh.

Question 9.
What are salient characteristics of Indian cities ?
Answer:
Indian Cities : Salient Characteristics.

Salient features of the Indian cities are as follows :
1. Most towns and cities are over-grown villages and have much rural semblance behind their street frontages.

2. People are even more rural in their habits and attitude, which reflects their socio-economic outlook in housing and other aspects.

3. Sizeable chunk of cities are full of slums largely due to the influx of immigrants without much infrastructure.

4. Several cities have distinct marks of earlier rulers and old functions.

5. Functional segregation is distinctly rudimentary, non-comparahle to western cities.

6. Social segregation of population is based either on caste, religion, income or occupation.

Question 10.
Classify Town and Cities on basis of population size.
Answer:
Towns and Cities based on Population Size
Census of India classifies urban centres into six classes. Urban centre with population of more than one lakh is called a city and less than one lakh is called a town. Cities accommodating population between one to five million are called metropolitan cities and more than five million are mega cities. Majority of metropolitan and mega cities are urban agglomerations. An urban agglomeration may consist of any one of the following three

Combinations :
(i) a town and its adjoining urban outgrowths
(ii) two or more contiguous towns with or without their outgrowths, and
(iii) a city and one or more adjoining towns with their outgrowths.

It is evident that majority of urban population lives in 423 cities, i.e., only 8.2 per cent of all urban places. They support 60.3 per cent of the total urban population of the country. Out of 423 cities, 35 cities / urban agglomerations have population more than 1 million each, thus they are metropolitan cities. Six of them are mega cities with population over five million each. More than one-fifth (21.0%) of urban population lives in these mega cities.

More than half (55.2%) of the towns (with population less than 20 thousand each) accommodate only 11.0 percent of urban population. One-fourth (26.78%) of urban population lives in middle-sized towns of the country. These medium towns recorded highest growth during the last decade, raising their share in total urban population from 24.3 per cent to 26.8 percent.

Question 11.
Differentiate between Hamletled and Dispersed Survival Settlements of India. (C.B.S.E. 2016)
Answer:
Hamletled settlements: When a village is fragmented on social and ethnic factors, its units are separated from each other. They bear a common name. These units are called pauna, Para, Palli, nagla and dhani. Such villages are more frequently found in the middle and lower Ganga plains.

Dispersed settlements: Isolated settlements are called dispersed settlements. These are found in forests, on hill slopes and fragmente fields. These include hamlets of few huts. Dispersion of settlements is caused by extremely fragmented nature of the terrain. Many areas of Meghalaya, Uttaranchal, Himachal Pradesh and Kerala have this type of settlement.

Question 12.
Discuss the factors that determine the type of rural settlements. (C.B.S.E. 2011)
Answer:
The rural settlements vary in size, shape and lay-out plans. The types of rural settlement depends upon the following factors :
(i) Physical Factors. Physical factors such as relief, altitude, drainage, water table, climate and soil play an important role in determining the type of settlement. In dry areas, the houses are clustered around a source of water.

(ii) Cultural Factors. Ethnic and cultural factors such as tribal, caste or communal identity are also important in determining the lay-out of a rural settlement. The nucleus of the settlement is occupied by land-owners. Harijan Dwellings are located on the periphery away from the main settlement. The settlement is divided into several units.

(iii) Historical factors. The Northern plains of India have been exposed to frequent invasions from invadors and conquerors. The villagers preferred to live in compact settlement in order to defend against the invaders.

Question 13.
Study the diagram given below and answer the questions that follow: (CBSE 2016)
.
(5.1) Identify and name the given rural settlement pattern.
(5.2) In which type of areas do we find such types of settlement patterns?
(5.3) Give an important characteristic of this type of settlement pattern.
Answer:
(5.1.) It is a cross shaped settlement.
(5.2) These settlements emerge at places where two roads converge on the third.
(5.3) Houses extend in all the four directions.

Human Settlements Important Extra Questions Long Answer Type

Question 1.
What is a Town ? Classify Towns according to urban Historians and describe their evolution.
Or
“Examine flourished since prehistoric times in India.” (Delhi 2019)
Or
Examine the level of urbanisation in India after Independence. (Delhi 2019)
Or
“Towns flourished since prehistoric times in India.” Examine. (Delhi 2019)
Answer:
Definition of Town
Town is defined in different ways in different countries. In India, the census of India 2001 identifies two types of towns : Statutory and Census :

Statutory Towns : Ail places which have municipal, or corporation, or cantonment board, or a notified town area committee.

Census Towns: All other places which satisfy the following criteria :

• A minimum population of 5,000 persons;
• At least 75 percent of male wmrking population engaged in non-agricultural pursuits;
• A density of population of atleast 400 persons per sq. km.

Evolution of towns in india

Towns flourished since prehistoric times in India. Even at the time of Indus valley civilisation, towns like Harappa and Mohanjodaro were in existence. The second phase of urbanisation began around 600 B.C.E. It continued with periodic ups and downs until the arrival of Europeans in India in the 18th century. Urban historians classify towns of India as :
(1) Ancient towns
(2) Medieval towns, and
(3) Modern towns.

(1) Ancient Towns : At least 45 towns have historical background and have been in existence at least for over 2000 years. Most of them developed as religious and cultural centres. Varanasi is one of the important towns among these. Ayodhya, Prayagraj (Allahabad), Pataliputra (Patna), Mathura and Madurai are some other ancient towns.

(2) Medieval Towns : About 100 of the existing towns have their roots in the medieval period. Most of them developed as headquarters of principalities and kingdoms. Most of them are fort towns and came up on the ruins of earlier existing towns. Important among them are Delhi, Hyderabad, Jaipur, Lucknow, Agra and Nagpur.

(3) Modern Towns : The British and other Europeans modified the urban scene. As an external force, starting their foothold on coastal locations, they first developed some trading ports such as Surat, Daman, Goa, Puducherry, etc. The British later consolidated their hold from three principal nodes – Mumbai (Bombay), Chennai (Madras) and Kolkata (Calcutta) – and built them in the British fashion.

Rapidly extending their domination either directly or through super control over the princely states, they established their administrative centres, hill-towns as summer resorts, and added new civil, administrative and military areas to them. Towns based on modern industries also evolved after 1850. Jamshedpur can be cited as an example.

After independence, a large number of towns emerged as administrative headquarters (Chandigarh, Bhubaneshwar, Gandhinagar, Dispur, etc.) and industrial centres (Durgapur, Bhilai, Sindri, Barauni, etc.). Some old towns also developed as satellite towns around metropolitan cities such as Ghaziabad, Rohtak, Gurgaon, etc. around Delhi. With increasing investment in rural areas, a large number of medium and small towns have developed all over the country.

Human Settlements Important Extra Questions HOTS

Question 1.
‘Towns act as nodes of economic growth.’ Discuss.
Answer:
Cities act as nodes of economic growth provide goods and services not only to urban dwellers but also to the people of the rural settlements in their hinterlands in return for food and raw materials. This functional relationship between the urban and rural settlements takes place through transport and communication network.

Question 2.
‘Rural and urban settlements differ in their way of life, attitude and outlook.’ Explain.
Answer:
Rural and urban settlements differ also in their way of life, attitude and outlook. Rural people are less mobile and therefore social relations among them are intimate. They employ simple techniques to perform their activities and their pace of life is slow. In urban areas, on the other hand, way of life is complex and fast and social relations are formal and institutionalized.

 

NCERT Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.3 are part of NCERT Solutions for Class 10 Maths. Here we have given NCERT Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.3.

Board	CBSE
Textbook	NCERT
Class	Class 10
Subject	Maths
Chapter	Chapter 14
Chapter Name	Statistics
Exercise	Ex 14.3
Number of Questions Solved	7
Category	NCERT Solutions

NCERT Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.3

Question 1.
The following frequency distribution gives the monthly consumption of electricity of 68 consumers of a locality. Find the median, mean and mode of the data and compare them.

Solution:

Question 2.
If the median of the distribution given below is 28.5, find the values of x and y.

Solution:
Given, Median = 28.5 which lies in the class (20 – 30).
Here, l = 20, f = 20, cf = 5 + x, h = 10, n = 60

Question 3.
A life insurance agent found the following data for distribution of ages of 100 policy holders. Calculate the median age, if policies are given only to persons having age 18 years onwards but less than 60 years.

Solution:

Question 4.
The lengths of 40 leaves of a plant are measured correct to nearest millimetre, and the data obtained is represented in the following table:

Find the median length of the leaves.
(Hint: The data needs to be converted to continuous classes for finding the median since the formula assumes continuous classes. The classes then change to 117.5 – 126.5,126.5 -135.5,…, 171.5 -180.5.)
Solution:

Question 5.
The following table gives the distribution of the lifetime of 400 neon lamps:

Find the median lifetime of a lamp.
Solution:

Question 6.
100 surnames were randomly picked up from a local telephone directory and the frequency distribution of the number of letters in the English alphabet in the surnames was obtained as follows:

Determine the median number of letters in the surnames. Find the mean number of letters in the surnames. Also, find the modal size of the surnames.
Solution:

For mean

Question 7.
The distribution below gives the weight of 30 students of a class. Find the median weight of the students.

Solution:

We hope the NCERT Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.3, help you. If you have any query regarding NCERT Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.3, drop a comment below and we will get back to you at the earliest.