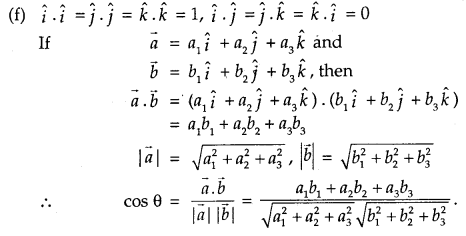Prasanna

Here we are providing Class 12 Maths Important Extra Questions and Answers Chapter 12 Linear Programming. Class 12 Maths Important Questions are the best resource for students which helps in Class 12 board exams.

Class 12 Maths Chapter 12 Important Extra Questions Linear Programming

Linear Programming Important Extra Questions Very Short Answer Type

Question 1.
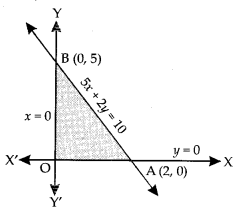
Draw the graph of the following LPP:
5x + 2y ≤ 10, x ≥ 0,y ≥ 0.
Solution:
Draw the line AB : 5.v + 2y = 10 …(1),
which meets x-axis at A (2, 0) and y-axis at B (0,5).
Also x = 0 is y-axis and y = 0 is x-axis.
Hence, the graph of the given LPP is as shown (shaded):

Question 2.
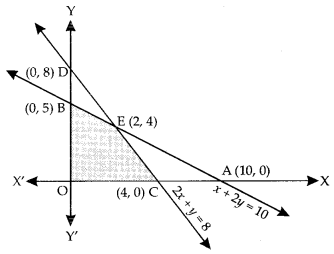
Solve the system of linear inequations: x + 2y ≤ 10; 2x + y ≤ 8.
Solution:
Draw the st. lines x + 2y = 10 and 2x + y = 8.
These lines meet at E (2,4).
Hence, the solution of the given linear inequations is shown as shaded in the following figure :

Question 3.
Find the linear constraints for which the shaded area in the figure below is the solution set:

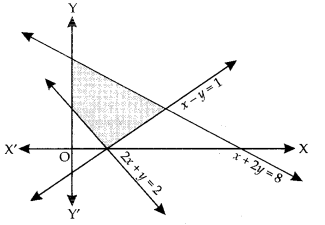
Solution:
From the above shaded portion, the linear constraints are :
2x + y ≥ 2,x – y ≤ 1,
x + 2y ≤ 8, x ≥ 0, y ≥ 0.

Question 4.
A small firm manufactures neclaces and bracelets. The total number of neclaces and bracelets that it can handle per day is at most 24. It takes one hour to make a bracelet and half an hour to make a neclace. The maximum number of hours available per day is 16. If the profit on a neclace is ₹100 and that on a bracelet is ₹300. Formulate an LPP for finding how many of each should be produced daily to maximize the profit ?
It is being given that at least one of each must be produced. (C.B.S.E. 2017)
Solution:
Let ‘x’ neclaces and ‘y’ bracelets be manufactured per day.
Then LPP problem is:
Maximize Z = 100x+300y
Subject to the constraints : x + y ≤ 24,
(1) (x) + \(\frac { 1 }{ 2 }\)y ≤ l6,
i.e. 2x + y ≤ 32
and x ≥ 1
and y ≥ 1
i.e. x – 1 ≥ 0
and y – 1 ≥ 0.

Question 5.
Old hens can be bought for ?2.00 each and young ones at ?5.00 each. The old hens lay 3 eggs per week and the young hens lay 5 eggs per week, each egg being worth 30 paise. A hen costs ₹1.00 per week to feed. A man has only ₹80 to spend for hens. Formulate the problem for maximum profit per week, assuming that he cannot house more than 20 hens.
Solution:
Let ‘x’ be the number of old hens and ‘y’ the number of young hens.
Profit = (3x + 5y) \(\frac { 30 }{ 100 }\) – (x + y) (1)
= \(\frac{9 x}{10}+\frac{3}{2}y\)x – y
= \(\frac{y}{2}-\frac{x}{10}=\frac{5 y-x}{10}\)
∴ LPP problem is:
Maximize Z = \(\frac{5 y-x}{10}\) subject to:
x ≥ 0,
y ≥ 0,
x + y ≤ 20 and
2x + 5y ≤ 80.

Linear Programming Important Extra Questions Very Long Answer Type 2

Question 1.
Maximize Z-5x + 3y
subject to the constraints:
3x + 5y ≤ 15, 5x + 2y ≤ 10, x ≥ 0,y ≥ 0. (N.C.E.R.T)
Solution:
The system of constraints is :
3x + 5y ≤ 15 …(1)
5x + 2y ≤ 10 …(2)
and x ≥ 0, y≥ 0 …(3)
The shaded region in the following figure is the feasible region determined by the system of constraints (1) – (3):

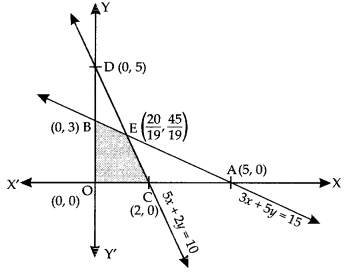
It is observed that the feasible region OCEB is bounded. Thus we use Corner Point Method to determine the maximum value of Z, where :
Z = 5x + 3y …(4)

The co-ordinates of O, C, E and B are (0, 0), (2,0), \(\left(\frac{20}{19}, \frac{45}{19}\right)\) (Solving 3x + 5y = 15 and 5x + 2y – 10) and (0, 3) respectively.
We evaluate Z at each comer point:

Comer Point	Corresponding Value of Z
O: (0,0)	0
C: (2,0)	10
E(\(\left(\frac{20}{19}, \frac{45}{19}\right)\))	\(\frac{20}{19}\) (Maximum)
B(0.3)	9

Hence’ Z_max = at the Point \(\left(\frac{20}{19}, \frac{45}{19}\right)\)

Question 2.
Minimize Z = 3x + 2y subject to the constraints:
x +y ≥ 8, 3x + 5y ≤ 15, x ≥ 0, y ≥ 0. (N.C.E.R.T.)
Solution:
The system of constraints is :
x +y ≥ 8, , x ≥ 0, y ≥ 0…(1)
3x + 5y ≤ 15 …(2)
and x ≥ 0, y ≥ 0 …(3)

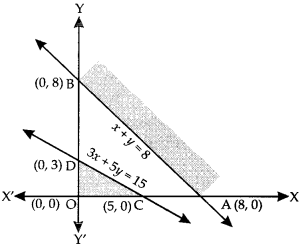
It is observed that there is no point, which satisfies all (1) – (3) simultaneously.
Thus there is no feasible region.
Hence, there is no feasible solution.

Question 3.
Determine graphically the minimum value of the objective function :
Z = – 50x + 20y
subject to the constraints:
2x-y ≥ – 5, 3x +y ≥ 3, 2x – 3y ≤ 12, x,y ≥ 0. (N.C.E.R.T.)
Sol. The system of constraints is :
2x-y ≥ – 5 …(1)
3x +y ≥ 3 …(2)
2x – 3y ≤ 12 …(3)
and x,y ≥ 0 …(4)
The shaded region in the following figure is the feasible region determined by the system of constraints (1) – (4).

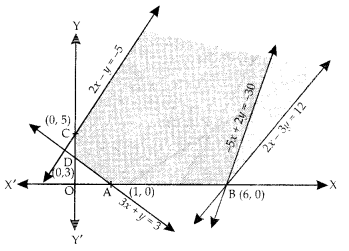
It is observed that the feasible region is unbounded.
We evaluate Z = – 50x + 20y at the corner points :
A (1, 0), B (6, 0), C (0, 5) and D (0, 3) :

Corner Point	Corresponding Value of Z
A: (1,0)	-50
B : (6, 0)	– 300 (Minimum)
C : (0, 5)	100
D : (0, 3)	60

From the table, we observe that – 300 is the minimum value of Z.
But the feasible region is unbounded.
∴ – 300 may or may not be the minimum value of Z. ”

For this, we draw the graph of the inequality.
– 50x + 20y < – 300
i.e. – 5x + 2y < – 30.
Since the remaining half-plane has common points with the feasible region,
∴ Z = – 50x + 20y has no minimum value.

Question 4.
Minimize and Maximize Z = 5x + 2y subject to the following constraints : x – 2y ≤ 2, 3x + 2y < 12, -3x + 2y ≤ 3, x ≥ 0, y ≥ 0. (A.I.C.B.S.E. 2015)
Solution:
The given system of constraints is :
x – 2y ≤ 2 …(1)
3x + 2y < 12 …(2)
-3x + 2y ≤ 3 …(3)
and x ≥ 0, y ≥ 0.

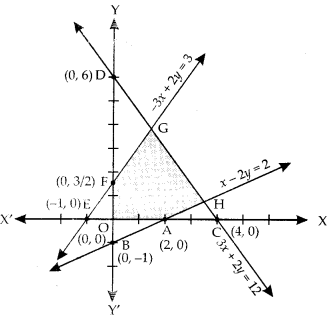
The shaded region in the above figure is the feasible region determined by the system of constraints (1) – (4). It is observed that the feasible region OAHGF is bounded. Thus we use Corner Point Method to determine the maximum and minimum value of Z, where
Z = 5x + 2y …(5)

The co-ordinates of O, A, H, G and F are :
(0, 0). (2. 0), (\(\frac{7}{2}, \frac{3}{4}\)) and (\(\frac{3}{2}, \frac{15}{4}\)), \(\frac { 3 }{ 2 }\))
respectively. [Solving x

2y = 2 and 3x + 2y = 12 for
H and -3x + 2y = 3 and
3x + 2y = 12 for G]
We evaluate Z at each cormer point:

Corner Point	Corresponding value of Z
O: (0,0)	0 (Minimum)
A: (2,0)	10
H(\(\frac{7}{2}, \frac{3}{4}\))	19 (Maximum)
G(\(\frac{3}{2}, \frac{15}{4}\))	15
F: (0, \(\frac{3}{2}\)))	3

Hence, Z_max = 19 at (\(\frac{7}{2}, \frac{3}{4}\)) and
Z_max = 0at (0,0)

Question 5.
A dealer in rural area wishes to purchase a number of sewing machines. He has only ₹5760.00 to invest and has a space for at most 20 items. An electronic sewing machine costs him ₹360.00 and a manually operated sewing machine ₹240.00. He can sell electronic sewing machine at a profit of ₹22.00 and a manually operated sewing machine at a profit of ₹18.00. Assuming that he can sell all the items that he can buy, how should he invest his money in order to maximize his profit. Make it a linear programming problem and solve it graphically. (C.B.S.E. 2014)
Solution:
Let ‘x’ be the number of electronic operated machines and ‘y’ that of manually operated machines be purchased.
Then the LPP problem is as follows :
Maximize:
Z = 22 + 18y
Subject to:
x + y ≤ 20 …(1)
360x + 240y ≤ 5760
i.e. 3x + 2y ≤ 48 …(2)
and x ≥ 0, y ≥ 0 …(3)
The shaded region of the figure represents the feasible region OCEB, which is bounded.

Applying Corner Point Method, we have:

Corner Point	Corresponding value of Z
O: (0,0) C:(16,0) E: (8,12) B: (0,20)	0 352 392 (Maximum) 360

Thus, Z is maximum at E (8, 12).
Hence, the dealer should invest in 8 electronic and 12 manually operated machines.

Question 6.
A manufacturer produces nuts and bolts. It takes 1 hour of work on machine A and 3 hours on machine B to produce a package of nuts. It takes 3 hours on machine A and 1 hour on machine B to produce a package of bolts. He earns a profit of ₹ 35 per package of nuts and ₹ 14 per package of bolts. How many packages of each should be produced each day so as to maximise his profit, if he operates each machine for atmost 12 hours a day? Convert it into an LPP and solve graphically.
Solution:
Let ‘x’ and ‘y’ be the number of packages of nuts and bolts respectively.
We have the following constraints :
x ≥ 0 …(1)
y ≥ 0 …(2)
x + 3y ≤ 12 ….(3)
3x + y ≤ 12 …(4)
Now the profit,P= 35x+ 14y …..(5)
We are to maximize P subject to constraints (1) -(4).
Draw the line AB (x + 3y = 12)
Draw the line CD (3x + y = 12)
These meet at E (3, 3).
The shaded region in the figure represents the feasible region, which is bounded.

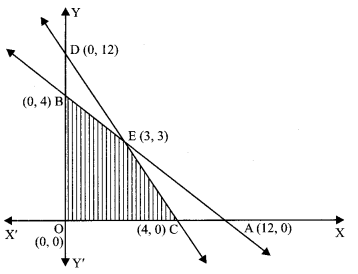
Applying Corner Point Method, we have :

Corner Point	P = 35x + 14y
O: (0,0)	0
C : (4, 0)	140
E: (3,3)	147 (Maximum)
B : (0,4)	56

Hence, max. profit is ₹ 147 and it is obtained when 3 packages each of Nuts and Bolts are produced daily.

Question 7.
Two tailors A and B earn ₹ 150 and ₹ 200 per day respectively. A can stitch 6 shirts and 4 pants per day, while B can stitch 10 shirts and 4 pants per day. Form a L.P.P. to minimize the labour cost to produce (stitch) at least 60 shirts and 32 pants and solve it graphically.
Solution:
Let the tailor A work for ‘x’ days and B for ‘y’ days.

Thus, we have the following constraints:
x ≥ 0 …(1)
y ≥ 0 …(2)
6x + 10y ≥ 60
i.e. 3x + 5y ≥ 30 …….(3)
4x + 4y ≥ 32
i.e. x + y ≥ 8 ……(4)
The objective function, or the cost Z is:
Z = 150x + 200 y ……..(5)
For the solution set, we draw the lines:
x = 0, y – 0, 3x + 5y = 30, x + y = 8

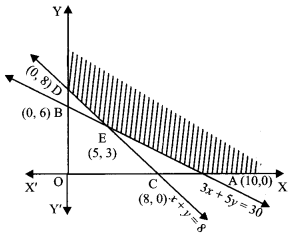
The feasible region (shaded) is unbounded. Let us evaluate Z at the comer points:
A (10,0), D(0, 8) and E(5, 3)
[Solving x + y – 8, Sx + 5y – 30; x – 5,y = 3]

Applying Comer Point Method, we have:

Corner Point	Z = 150 x + 200y
A : (10, 0) E: (5,3) D: (0, 8)	1500 1350 (Minimum) 1600

Hence, the tailor A should work for 5 days and B for 3 days.
To Check: Draw 150x + 200y < 1350 i.e. 3x + 4y < 27.
Since there is no region common with feasible region,
∴ Minimum value is ₹ 1350.

Question 8.
A dietician wishes to mix two types of food in such a way that the vitamin contents of the mixture contains at least 8 units of vitamin A and 10 units of vitamin C. Food I contains 2 units/kg of vitamin A and 1 unit/kg of vitamin C. It costs ₹50 per kg to produce food I. Food II contains 1 unit/kg of vitamin A and 2 units/kg of vitamin C and it costs ₹70 per kg to produce food n. Formulate this problem as a LPP to minimise the cost of a mixture that will produce the required diet. Also find the minimum cost
Solution:
Let the quantity of Food I = x kg
and the quantity of Food II = y kg.
Then the LPP problem is as below :
Z = 50x+70y …(1)
Subject to 2x + y ≥ 8 …(2)
x + 2y ≥ 10 ….(3)
and x ≥ 0, y ≥0 ……..(4)
For the solution, we draw the lines :
x = 0,y = 0,2x + y = 8 and x + 2y = 10

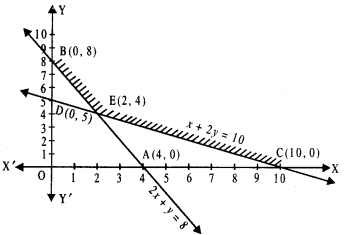
The feasible regin is as shown with vertices C(10,0), E(2,4) and B(0,8).
Applying Corner Point Method, we have

Corner Point	Z = 50x + 70y
C: (10,0) E:(2,4) B: (0,8)	500 380 (Minimum) 560

Thus minimum cost is ₹38Q when 2 kg of Food I and 4 kg of Food II are mixed.

Question 9.
A manufacturer makes two types of toys A and B. Three machines are needed for this purpose and the time (in minutes) required for each toy on the machines is given below:

Types of Toys	Machines
	I	II	III
A	20	10	10
B	10	20	30

The machines I, II and III are available for a maximum of 3 hours, 2 hours and 2 hours 30 minutes respectively. The profit on each toy of type A is ₹50 and that of type Bis ₹60. Formulate the above problem as a LPP and solve it graphically to maximize profit.
(C.B.S.E. Sample Paper 2018-19)
Solution:
Let ‘x’ and ‘y’ be the number of toys of type A and type B respectively.
Then maximize :
P = 50x + 60y …(1)
Subject to constraints :
20x +10v ≤ 180 …(2)
10x + 20y ≤ 120 …….(3)
10x + 30y ≤ 150 ……(4)
and x ≥ 0, y ≥ 0 …(5)

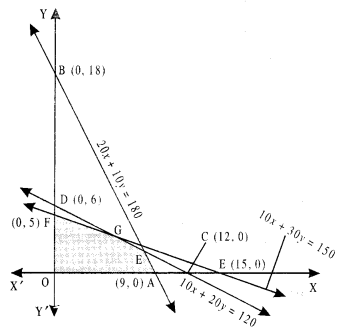
Applying Corner Point Method we have :

Corner point	P = 50jc + 60y
O: (0,0) F:(0,5) G: (6,3) H: (8,2) A: (9,0)	0 300 480 520 (Maximize) 450

Hence, maximum profit is ₹520 when x = 8 and y = 2. i.e., when 8 toys of type A and 2 toys of type B are made.

Question 10.
A factory manufactures two types of screws A and B, each type requiring the use of two machines, an automatic and a hand- operated. It takes 4 minutes on the automatic and 6 minutes on the hand operated machines to manufacture a packet of screws ‘A’ while it takes 6 minutes on the automatic and 3 minutes on the hand-operated machine to manufacture a packet of screws ‘B\ Each machine is available for at most 4 hours on any day. The manufactures can sell a packet of screws ‘A’ at a profit of 70 paise and screws ‘B’ at a profit of ₹ 1. Assuming that he can sell all the screws he manufactures, how many packets of each type should the factory owner produce in a day in order to maximize his profit? Formulate the above LPP and solve it graphically and find the maximum profit (C.B.S.E. 2018)
Solution:
Let the factory manufacture ‘x’ of type ‘A’ and ‘y’ of type ‘B’
Clearly x ≥ 0 …(1)
and y ≥ 0 …(2)
Since the machines can operate for at the most 4 hours a day,
4x + 6y ≤ 240
i.e., 2x + 3y ≤ 120 …(3)
and 6x + 3y ≤ 240
i.e., 2x + y ≤ 80 …(4)
The objective function or the profit, P, is:
P = 0.7 x + y …(5)

We drawn the lines :
x = 0,y = 0,
2x + 3y = 120
and 2x + y – 80

The feasible region is shown shaded OCPB is bounded, where O is (0, 0), C is (40, 0), B is (0, 40) and P is (30, 20).
[Solving 2x + y – 80 and 2x + 3y = 120; x = 30, y – 20]

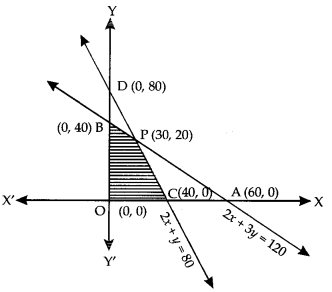
Applying Corner Point Method, we have :

Comer point	P = 0.7x + y
O: (0, 0) C : (40, 0) P : (30, 20) B: (0,40)	0 28 41 (Maximum) 40

Hence, in order to maximize profit 30 packets of screw ‘A’ and 20 packets of screw ‘B’ should be manufactured and maximum profit = ₹ 41.

Question 11.
A small firm manufactures chairs and tables. Market demand and available resources indicate that the continued production of chairs and tables should not exceed 50 units per day. It takes 30 minutes to manufacture a chair and 1 hour to manufacture a table. A maximum of 40 man-hours per day are available. The profit on each chair is ₹ 40 and profit on each table is ₹ 60. Determine how many each of chairs and tables should be manufactured per day in order to maximize the profit. What is the maximum profit? Formulate LPPand solve graphically.
Solution:
Let ‘x’ and ‘y’ be the number of chairs and tables respectively.
We have: x ≥ 0 ………..(1)
y ≥ 0 ………(2)
x + y ≤ 50 ……(3)
and \(\frac{x}{2}\) + y ≤ 40
⇒ x + 2y < 80 …(4)
The objective function, or the profit, Z is
Z = 40 x + 60 y …(5)

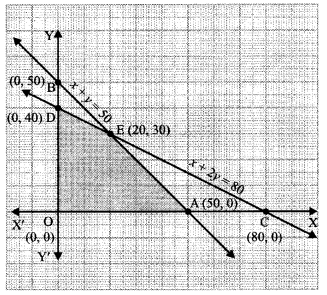
We have to maximize Z subject to (1) – (4).
For solution set, we draw the lines:
x = 0, y – 0, x + y = 50 and x + 2y – 80.
The lines x + y = 50 and x + 2y = 80 meet at E (20, 30).

The shaded portion represents the feasible region, which is bounded.
Applying Corner Point Method, we have:

Corner Point	Z = 40x + 60y
0 =(0,0) A: (50, 0) E: (20,30) B: (0,40)	0 2000 2600 (Maximum) 2400

Hence, the maximum profit is ₹ 2600 when 20 chairs and 30 table are manufactured.

We have given detailed NCERT Solutions for Class 10 Sanskrit Grammar Book सङ्केताधारितम् औपचारिकम् अनौपचारिक च पत्रम् Questions and Answers come in handy for quickly completing your homework.

Sanskrit Vyakaran Class 10 Solutions सङ्केताधारितम् औपचारिकम् अनौपचारिक च पत्रम्

अतिरिक्त-उदाहरणानि

1. अस्वस्थतायाः हेतोः दिनद्वयस्य अवकाशार्थम् प्रधानाचार्य प्रति लिखिते प्रार्थनापत्रे मञ्जूषायाः पदानि चित्वा रिक्तस्थानानि पूरयत-
(अस्वस्थ होने के कारण दो दिन के अवकाश के लिए प्रधानाचार्य को लिखे गए प्रार्थना-पत्र में मञ्जूषा से पद चुनकर खाली स्थान भरिए।)

परीक्षाभवनम्

आदरणीयाः (i) _______________।
विषय- अवकाशप्राप्तये निवेदनम्।
सविनयं (ii) ____________ अस्ति यत् अहं (iii) ____________ आक्रान्तः अस्मि। एतस्मात् कारणात् विद्यालयम् आगन्तुम् (iv) _______________ अस्मि। अतः मह्यम् (v) _______________ अवकाशं प्रदाय (vi) _______________ अनुगृह्णन्तु। एतयोः दिवसयोः अध्ययनस्य या (vii) _______________ भविष्यति ताम् (viii) _______________ यतिष्ये।
(ix) _______________

भवदीयः शिष्यः
क, ख, ग

तिथि: (x) _______

मञ्जूषा- दूरीकर्तुम्, हानिः, धन्यवादः, दिनद्वयस्य, प्रधानाचार्याः, असमर्थः, माम्, तीव्रज्वरेण, निवेदनम्, 20-8-20……….
उत्तराणि:
(i) प्रधानाचार्याः
(ii) निवेदनम्
(iii) तीव्रज्वरेण
(iv) असमर्थः
(v) दिनद्वयस्य
(vi) माम्
(vii) हानिः
(viii) दूरीकर्तुम्
(ix) धन्यवादः

2. विद्यालयस्य प्रधानाचार्यां प्रति लिखिते शुल्कक्षमाप्रार्थनापत्रे मञ्जूषायाः समुचितपदैः रिक्तस्थानानि पूरयत-
(विद्यालय की प्रधानाचार्या को लिखे गए शुल्क माफी प्रार्थना-पत्र में मञ्जूषा के समुचित पदों से खाली स्थान भरिए।)

परीक्षाभवनात्

आदरणीयाः (i) _______________
विषय- शुल्कक्षमापनार्थं निवेदनम्
(ii) _______________

(iii) _______________ निवेदनम् अस्ति यत् मम पिता एकस्मिन् सर्वकारविद्यालये (iv) _______________ अस्ति। तस्य मासिकं (v) _______________ द्विसहस्र रूप्यकाणि अस्ति। अस्माकं परिवारे (vi) _______________ सन्ति। अस्मिन् सीमिते वर्तने विद्यालयस्य (vii) _______________ अतीव कठिनम् अस्ति। कृपया तदर्थं (viii) _______________ प्रदाय (ix) _______________ माम् अनुगृह्णन्तु।
(x) _______________

भवदीया
आज्ञाकारिणी शिष्या
क, ख, ग

तिथि : 24-7-20……..

मञ्जूषा- सधन्यवादम्, भवत्यः, शिक्षाशुल्कप्रदानम्, वर्तनम्, लिपिकः, महोदयाः, प्रधानाचार्याः सदस्याः, सविनयम्, स्वीकृतिम्
उत्तराणि:
(i) प्रधानाचार्याः
(ii) महोदयाः
(iii) सविनयम्
(iv) लिपिकः
(v) वर्तनम्
(vi) सदस्याः
(vii) शिक्षाशुल्कप्रदानम्
(viii) स्वीकृतिम्
(ix) भवत्यः
(x) सधन्यवादम्

3. सुकन्या कोलकातानगरे छात्रावासे निवसति। सा विद्यालयतः शैक्षिकभ्रमणाय भुवनेश्वरं गन्तुम् इच्छति। एतदर्थम् अनुमतिं राशिं च प्राप्तुं सा नवदिल्लीस्थं पितरं प्रति पत्रम् एकं लिखति। मञ्जूषातः पदानि विचित्य पत्रे रिक्तस्थानानि पूरयन्तु।

छात्रावासः
राजकीयः विद्यालयः
(i) _______________
तिथि: 25-2-20XX

माननीयाः पितृवर्याः,

सादरं प्रणमामि।

भवतः पत्रं प्राप्तम्। मम (ii) _______________ परीक्षा समाप्ता। परीक्षापत्राणि अतिशोभनानि जातानि। यावत् परीक्षापरिणामः न आगच्छति तावत् |आगामिमासस्य प्रथमसप्ताहे (iii) _______________ विद्यालयस्य अध्यापिकाः अस्मान् शैक्षिकभ्रमणाय (iv) _______________ नेष्यन्ति। अहम् अपि ताभिः (v) _______________ गन्तुम् इच्छामि। एतदर्थम् मया कक्षाध्यापिकायै (vi) _______________ रूप्यकाणि दातव्यानि सन्ति। अतः यदि अनुमतिः (vii) _______________ तर्हि अहम् अपि गच्छेयम्। ज्ञानवर्धनार्थम् एतत् अतिरिच्य अवसरं च पश्यामि। अतः कृपया उपर्युक्तां राशिं प्रेषयित्वा माम् अनुगृहीतां कुर्वन्तु।

सर्वेभ्यः मम (viii) _______________ निवेदनीयाः।

भवतां प्रिया (ix) _______________
सुकन्या

वेष्टनम्-
श्री देवदत्त, महापात्रः
1/30, साकेत, नव दिल्ली – (x) ____________

मञ्जूषाः- प्रणामाः, सह, कोलकातातः, स्यात्, पञ्चशतम्, 110019, भुवनेश्वरम्, प्रथमसत्रीया, मम, पुत्री
उत्तराणि:
(i) कोलकातातः
(ii) प्रथमसत्रीया
(iii) मम
(iv) भुवनेश्वरम्
(v) सह
(vi) पञ्चशतम्
(vii) स्यात्
(viii) प्रणामाः
(ix) पुत्री
(x) 110019

4. सौम्यः दिल्ली नगरे वसति। तस्य मित्रं सुमतिः असमप्रदेशे वसति। सौम्येन गणतन्त्रदिवसस्य शोभायात्रायां भागः गृहीतः। सः स्वानुभवान् स्वमित्रं सुमतिं प्रति पत्रे लिखति। मञ्जूषातः पदानि विचित्य पत्रं पूरयन्तु।

ए-14, वसन्तविहारः
नवदिल्लीतः
तिथि: 05-05-20XX

प्रियसखि सुमते,
(i) ____________
अत्र कुशलं तत्रास्तु। अहं (ii) ____________ सज्जायां व्यस्तः आसम्। अतः विलम्बेन तव पत्रस्य उत्तरं ददामि। अस्मिन् वर्षे मयापि गणतन्त्रदिवसस्य शोभायात्रायां भागः (iii) ____________।
अस्माकं विद्यालयस्य (iv) ____________ राजपथे स्वकलायाः प्रदर्शनम् अकरोत्। अहं गरबानृत्यस्य (v) ____________ आसम्। छात्राणां राष्ट्रगानस्य ओजस्वी ध्वनिः (vi) ____________ गुञ्जितम् अकरोत्। स्वराष्ट्रस्य सैन्यबलानां पराक्रमप्रदर्शनानि विचित्रवर्णानि परिदृश्यानि (vii) ____________ च दृष्ट्वा अहं गौरवान्वितः(viii) ____________ बाल्यावस्थायाः स्वप्नः तत्र पूर्जातः।
(ix) ____________ वन्दनीयौ।

भवत्याः (x) ____________
सौम्य

मञ्जूषाः- पितरौ, गृहीतः, नमस्ते, मम, सुहृद्, राजपथम्, वाद्यवृन्दम्, लोकनृत्यानि, गणतन्त्रदिवस समारोहस्य, प्रमुख-सञ्चालकः।
उत्तराणि:
(i) नमस्ते
(ii) गणतन्त्रदिवस समारोहस्य
(iii) गृहीतः
(iv) वाद्यवृन्दम्
(v) प्रमुख-सञ्चालक:
(vi) राजपथम्
(vii) लोकनृत्यानि
(viii) मम
(ix) पितरौ
(x) सुहृद्।

5. सुजाता दिल्लीमहानगरे वसति। तस्याः सखी दिव्यामहादेवन् चेन्नईनगरे निवसति। सुजाताया: विद्यालये पर्यावरणविषये एका गोष्ठी जाता। गोष्ठी वर्णयन्ती सुजाता दिव्यायै पत्रम् एकं लिखति। मञ्जूषातः समुचितपदानि चित्वा पत्रं पूरयन्तु।

20, वसन्तविहारः
नवदिल्लीतः
तिथि: 20-05-20XX

(i) _______ दिव्ये,
नमस्ते!

ह्यः अस्माकं विध्यालये (ii) _______ एका महत्त्वपूर्णा गोष्ठी अभवत्। तत्र महानगरेषु वर्धमानं प्रदूषणं दृष्ट्वा ‘पर्यावरणस्य (iii) _______ उपयानां विषये चर्चा अभवत्। गोष्ठ्यां वक्तृणां विचारसारः आसीत् यत् गृहात् बहिः, (iv) _______ रथ्यासु च अवकरः न क्षेपणीयः। (v) _______ समये-समये जलशुद्धिः करणीया। मार्गेषु, उद्यानेषु, विद्यालयेषु च अधिकाधिकं (vi) _______ आरोपणीयाः। जनजागरणार्थं “वृक्षो रक्षति रक्षितः”, “जलम् एव जीवनम्” एतादृशानि महावाक्यानि पटट्किासु लिखित्वा यत्र तत्र (vii) _______। ध्वनिप्रदूषणं निवारयितुं ध्वनिप्रसारकयन्त्राणां (viii) _______ प्रयोगः करणीयः। अस्मिन् विषये भवत्याः विद्यालये किं किं भवति इति मां विस्तरेण लिखतु।

पितरौ वन्दनीयौ।

भवत्याः सखी,
(ix) _______

वेष्टनम्
(x) _______
50/1, चेन्ना स्वामी नगरम्, चेन्नई

मञ्जूषाः- सुजाता, नदीजलेषु, स्नेहस्निग्धे, पर्यावरणविषये, न्यूनतमः, वृक्षाः, रक्षणाय, दिव्यामहादेवन्, टङ्कनीयानि, जलप्रदूषण-निवारणाय।
उत्तराणि:
(i) स्नेहस्निग्धे
(ii) पर्यावरणविषये
(iii) रक्षणाय
(iv) नदीजलेषु
(v) जलप्रदूषण-निवारणाय
(vi) वृक्षाः
(vii) टङ्कनीयानि
(viii) न्यूनतमः
(ix) सुजाता
(x) दिव्यामहादेवन्।

6. विकासः नैनीतालस्य एकस्मिन् विद्यालये पठति। सः छात्रावासात् अमेरिका-वास्तव्यं मित्रं जोसेफ नैनीतालविषये पत्रम् एकं लिखति। मञ्जूषातः पदानि विचित्य पत्रे रिक्तस्थानानि पूरयन्तु।

छात्रावासः
(i) _______
तिथि: 20-01-20XX

प्रिय (ii) _______ जोसेफ,
(iii) _______
गतमासे आवयोः दूरभाषेण वार्तालापः अभवत्। (iv) _______ एव अत्र हिमपातः आरभत। तं द्रष्टुम् अनेके यात्रिणः दूरतः आगताः आसन्। नैनीसरः अत्र एक विशेषम् आकर्षणस्थलम् अस्ति, तस्य अपि जलं (v) _______ आसीत्। तदा तत्र नौकाविहारः तु सम्भवः नासीत् किन्तु (vi) _______ आनन्दिताः आसन्। भयङ्करे शीतेऽपि यात्रिणः ‘चाइना-पीक’ इति स्थानं द्रष्टुम् गताः आसन्। अत्रत्यानि प्राकृतिकदृश्यानि आकर्षकाणि मनोरमाणि च सन्ति।

यात्रिणां समूह (vii) _______ स्थानीयाः आपणिकाः प्रमुदिताः आसन्। भोजनालयेषु आवासः दुर्लभः अभवत् निवासाय (viii) _______ भाटकं प्रवृद्धम् आसीत्। अहम् इच्छामि यत् ईदृशे अवसरे भवान् अपि भारते आगच्छेत् पर्वतीयस्थलस्य च आनन्दम् (ix) _______।

अग्रिम दिसम्बरमासे जनवरीमासे वा भवान् अत्र आगमिष्यति इति मे हार्दिकी कामना।

सधन्यवादम्,

(x) _______ मित्रम्,
विकासः

मञ्जूषाः- भवताम्, दृष्ट्वा, नैनीतालम्, अनुभवेत्, मित्र, प्रकोष्ठानाम्, हिमीभूतम्, नमो नमः, दिनद्वयानन्तरम्, स्केटिंगप्रेमिणः।
उत्तराणि:
(i) नैनीतालम्
(ii) मित्र
(iii) नमो नमः
(iv) दिनद्वयानन्तरम्
(v) हिमीभूतम्
(vi) स्केटिंगप्रेमिणः
(vii) दृष्ट्वा
(viii) प्रकोष्ठानाम्
(ix) अनुभवेत्
(x) भवताम्।

परीक्षापयोगिनी विविध पत्राणि

1. भवान् राकेशः। भवतः विद्यालयः छात्रान् शिक्षा-यात्रायै जोधपुरम् नेष्यति। पितुः आज्ञाप्राप्त्यर्थम् लिखिते पत्रे मञ्जूषापदानां सहायतया रिक्तस्थानपूर्तिं कुरुत।
(आप राकेश हैं। आपका विद्यालय छात्रों को शिक्षा-यात्रा के लिए जोधपुर ले जाएगा। पिता जी की आज्ञा प्राप्ति के लिए लिखे इस पत्र के रिक्त स्थानों को मंजूषा की सहायता से पूर्ण करें। You are Rakesh. Your school will take the students Jodhpur on an educational trip. Complete the letter written to your father to seek his permission by filling up the blanks with the words given in the box.)

8, संस्कृतिः (i) _______
दिनाङ्क: 3.8.20…………

आदरणीयाः (ii) _______।
सादरं (iii) _______।
भवताम् पत्रम् प्राप्तम् (iv) _______ च ज्ञाता। मम विद्यालयः शरदावकाशे छात्रान् (v) _______ जोधपुरम् नेष्यति। अहम् अपि एतत् (vi) _______ नगरं द्रष्टुम् इच्छामि । मह्यम् (vii) _______ पञ्चशतम् रूप्यकाणि प्रेषयन्तु
(viii) _______ शेषं कुशलम्। (ix) _______ मम प्रणामाः अनुजाय च स्नेहः।

(x) _______ पुत्रः
राकेशः

मञ्जूषा- यात्राव्ययार्थम्, भवताम्, पितृमहोदयाः, छात्रावासतः, भवन्तः, ऐतिहासिकम्, नमोनमः, अम्बायै, कुशलता, शिक्षा-यात्रायै।
उत्तराणि:

8, संस्कृतिः (i) छात्रावासतः
दिनाङ्कः 3.8.20…….

आदरणीयाः (ii) पितृमहोदयाः।
सादरं (iii) नमोनमः।
भवताम् पत्रम् प्राप्तम् (iv) कुशलता च ज्ञाता। मम विद्यालयः शरदावकाशे छात्रान् (v) शिक्षा-यात्रायै जोधपुरम् नेष्यति। अहम् अपि एतत् (vi) ऐतिहासिकम् नगरं द्रष्टुम् इच्छामि। मह्यम् (vii) यात्राव्ययार्थम् पञ्चशतम् रूप्यकाणि प्रेषयन्तु (viii) भवन्तः। शेषं कुशलम्। (ix) अम्बायै मम प्रणामाः अनुजाय च स्नेहः।

(x) भवताम् पुत्रः
राकेशः

2. भवान् सोमरत्नः।मातुःस्वास्थ्यं ज्ञातुम् भगिनीं लतां प्रति लिखिते पत्रे मञ्जूषायां दत्तैः पदैः रिक्तस्थानानि पूरयत।
(आप सोमरत्न हैं। माता जी का स्वास्थ्य जानने के लिए बहन लता को लिखे पत्र में मंजूषा में दिए गए पदों की सहायता से खाली स्थानों को भरिए। You are Somratna. Complete the letter written to your sister Lata to know how your mother is by filling up the blanks with the words given in the box.)

(i) ____________
शिमलानगरम्
दिनाङ्कः 23.12.20……..

समादरणीये (ii) ____________।
नमोनमः।
पत्रेण ज्ञातम् यत् अम्बा (iii) ____________ अस्ति । अहम् अतीव (iv) ____________ अस्मि परम् परीक्षाकारणात् गृहम् (v) ____________ सक्षमः अस्मि । भवती (vi) ____________ तस्यै (vii) ____________ यच्छतु, भगवत्कृपया सा शीघ्रम् (viii) ____________ भविष्यति। कृपया पत्रोत्तरम् (ix) ____________ प्रेषयत्।

तव भ्राता
(x) ____________

मञ्जूषा- औषधिम्, सोमरत्नः, आगन्तुं, भगिनि, चिन्तितः, यथासमयम्, ज्वरपीडिता, छात्रावासतः, शीघ्रम्, स्वस्था।
उत्तराणि:

(i) छात्रावासतः
शिमलानगरम्
दिनाङ्क: 23.12.20….

समादरणीये (ii) भगिनी।
नमोनमः।
पत्रेण ज्ञातम् यत् अम्बा (iii) ज्वरपीडिता अस्ति। अहम् अतीव (iv) चिन्तितः अस्मि परम् परीक्षाकारणात् गृहम् (0) आगन्तुं सक्षमः न अस्मि। भवती (vi) यथासमयम् तस्यै (vii) औषधिम् यच्छतु, भगवत्कृपया सा शीघ्रम् (viii) स्वस्था भविष्यति। कृपया पत्रोत्तरम् (ix) शीघ्रम् प्रेषयतु।

तव भ्राता
(x) सोमरत्नः

3. भवान् प्रतापः। भवता संस्कृतनाटके श्री कृष्णस्य भूमिकायाः अभिनयः कृतः सर्वोत्तम-अभिनयस्य पुरस्कारः च प्राप्तः। तत् सूचयता भवता स्वपितरं प्रति लिखिते पत्रे मञ्जूषातः रिक्तस्थानानि पूरयित्वा पत्रं पुनः लिखत।
(आप प्रताप हैं। आपने संस्कृत नाटक में श्री कृष्ण की भूमिका की है और सर्वोत्तम अभिनय हेतु पुरस्कार प्राप्त किया है। उसे सूचित करते हुए अपने पिता जी को लिखे पत्र में मंजूषा से शब्दों को लेकर खाली स्थानों को भरकर पत्र को फिर से लिखिए। You are Pratap. You have played the role of Shri Krishna in a Sanskrit drama and won the prize for the best acting. Rewrite the letter written to your father to tell him about this by filling up the blanks with the words given in the box.)

छात्रावासः
नवोदयविद्यालयः
(i) _______________।
दिनाङ्कः 11.7.20……..

परमपूज्यपितृचरणयोः
(ii) _______________ वन्दनम्। अहम् अत्र भवतः आशीर्वादेन (iii) _______________ अस्मि। मम विद्यालये एका संस्कृतनाटक-प्रतियोगिता (iv) _______________। अहम् तस्यां श्रीकृष्णस्य अभिनयं कृतवान्। (v) _______________ सभां प्रविश्य अहं पाण्डवानां कृते (vi) _______________ ग्रामान् एव (vii) _______________। दुर्योधनः माम् ग्रहीतुं यत्नं कृतवान्। सर्वैः दर्शकैः (viii) _______________ कृतम्। अहम् सर्वोत्कृष्ट-अभिनेतुः पुरस्कार प्राप्तवान्।
(ix) _______________ मातृचरणाभ्याम्।

भवदीयः प्रियः पुत्रः
(x) _______________

मञ्जूषा- याचितवान्, स्वस्थः, सादरं, जयपुरतः, प्रतापः, पञ्च, अभवत्, नमः, करतलवादनं, दुर्योधनस्य।
उत्तराणि:

छात्रावासः
नवोदयविद्यालयः
(i) जयपुरतः।
दिनाङ्कः 11.7.20…….

परमपूज्यपितृचरणयोः
(ii) सादरं वन्दनम्। अहम् अत्र भवतः आशीर्वादेन (iii) स्वस्थः अस्मि। मम विद्यालये एका संस्कृतनाटक-प्रतियोगिता (iv) अभवत्। अहम् तस्यां श्रीकृष्णस्य अभिनयं कृतवान्। (v) दुर्योधनस्य सभां प्रविश्य अहं पाण्डवानां कृते (vi) पञ्च ग्रामान् एव (vii) याचितवान्। दुर्योधनः माम् ग्रहीतुं यत्नं कृतवान्। सर्वैः दर्शकैः (viii) करतलवादनं कृतम्। अहम् सर्वोत्कृष्टअभिनेतुः पुरस्कार प्राप्तवान्।
(ix) नमः मातृचरणाभ्याम्।

भवदीयः प्रियः पुत्रः
(x) प्रतापः

4. भवान् नवेन्दुः जन्मदिवसोत्सवे कालघटिकायाः पुरस्कारं वाञ्छति। पितरं प्रति लिखितं पत्रं मञ्जूषायाः उचितं पदं चित्वा पत्रं पूरयत।
(आप नवेन्दु जन्मदिवस के उत्सव पर घड़ी भेंट चाहते हैं। पिता को लिखे पत्र की मंजूषा से उचित पदों को लेकर पत्र को पूर्ण कीजिए। You are Navendu. You want a watch as your birthday gift. Complete the letter written to your father with the words taking from the box.)

(i) _______________
दिनाङ्क: 16.8.20…….

परमादरणीयाः (ii) _______________
सादरं प्रणमामि।
(iii) _______________ कुशलं तत्रास्तु। भवता स्वपत्रे पृष्टं यत् जन्मदिवसस्य (iv) _______________ अहं किम् वाञ्छामि? अहं तु (v)
_______________ कालघटिकाम् इच्छामि। छात्रावासे सर्वाणि कार्याणि (vi) _______________ भवन्ति परं निश्चितसमयात् भोजनव्यवस्था न वर्तते। प्रातः पञ्चवादने जागरणम् (vii) _______________। अतः नियमपालनाय घटिका अत्यावश्यकी। अनया (viii) _______________ अपि दैनिककार्येषु नियमितः भविष्यामि। शेषं सर्वं कुशलम्। मम जनन्यै अग्रजाय च सादरं (ix) _______________

भवदीयः प्रियपुत्रः
(x) _______________

मञ्जूषा- अत्र, यथासमयम्, अत्यावश्यकम्, प्रणामाः, छात्रावासः, उपहाररूपेण, एकां, पितृमहाभागाः, अन्येषु, नवेन्दुः।
उत्तराणि:

(i) छात्रावासः
दिनाङ्कः 16.8.20…….

परमादरणीयाः (ii) पितृमहाभागाः
सादरं प्रणमामि।
(iii) अत्र कुशलं तत्रास्तु। भवता स्वपत्रे पृष्टं यत् जन्मदिवसस्य (iv) उपहाररूपेण अहं किम् वाञ्छामि? अहं तु (v) एकां कालघटिकाम् इच्छामि। छात्रावासे सर्वाणि कार्याणि (vi) यथासमयम् भवन्ति परं निश्चितसमयात् भोजनव्यवस्था न वर्तते।
प्रातः पञ्चवादने जागरणम् (vii) अत्यावश्यकम्। अतः नियमपालनाय घटिका अत्यावश्यकी। अनया (viii) अन्येषु अपि दैनिककार्येषु नियमितः भविष्यामि।
शेषं सर्वं कुशलम्। मम जनन्यै अग्रजाय च सादरं (ix) प्रणामाः।

भवदीयः प्रियपुत्रः
(x) नवेन्दुः

5. भवान् सुन्दरः। भवतः अनुजः नैनीताल नगरस्य वुडफोर्ट नामके विद्यालये दशमी कक्षायां पठति। तस्य समाचार ज्ञातुं प्रयत्नशीलः भवान्। अतः तस्य विद्यालयस्य प्रधानाचार्य प्रति एकं पत्रं लिखितुम् इच्छति भवान्। तत्पत्रं मञ्जषायाः पदानां सहायतया सम्परयत।
(आप सुन्दर हैं। आपका छोटा भाई नैनीताल नगर के वुडफोर्ट नामक विद्यालय में दशमी कक्षा में पढ़ता है। उसका समाचार जानने के लिए आप प्रयत्न कर रहे हैं। इसके लिए उस विद्यालय के प्रधानाचार्य को एक पत्र आप लिखना चाहते हैं। उस पत्र को मंजूषा के पदों से आप लिखिए। You are Sunder. Your younger brother studies in 10th standard in Woodfort School of Nainital district. You want to know about his healthwealth, etc. For this, you want to write a letter to the Principal of his school. Complete this letter with the help of words in box.)

1/360 गोलागोकर्णपुरम्।
(i) _______________
दिनाङ्क : 23-05-20…
सेवायाम्,
श्रीमन्तः प्रधानाचार्याः महोदयाः,
(ii) _______________
नैनीतालम् (उत्तराखण्डम्)
सादरं नमोनमः।
महाभागाः,
सादरं निवेदनं वर्तते यत् मम अनुजः देवर्षिः भवतां विद्यालयस्य (iii) _______________ कक्षायां पठति। विगत मासस्य तस्य कञ्चिदपि (iv) _______________ न आगच्छत्। सः किं करोति? कथं पठति? तस्य (v) _______________ कथं वर्तते? इत्यादयः। गृहस्य सर्वे जनाः एतदर्थं (vi) _______________ सन्ति। मातुः रोदनं निरन्तरम् एव भवति। अतः भवद्भ्यः (vii) _______________ अस्ति यत् इदं पत्रं प्राप्य भवान् तस्मै (viii) _______________ दातुं कथयतु। भवतां कृपा भविष्यति यावत् तस्य पत्रं न आगमिष्यति तावद् गृहे (ix) _______________ न भविष्यति। कृपया पत्रं दातुं शीघ्रमेव वदन्तु भवन्तः।

भवतां कृपाकांक्षी
सुन्दरः

मञ्जूषा- सधन्यवादम्, कर्णपुरम्, स्वास्थ्य, वुडफोर्ट विद्यालयः, दशमी, दुःखिताः, स्वसमाचार, समाचार, प्रार्थना, शान्तिः
उत्तराणि:

1/360 गोलागोकर्णपुरम्।
(i) कर्णपुरम्
दिनाङ्क : 23-05-20… सेवायाम्,
(ii) वुडफोर्ट विद्यालयः
नैनीतालम् (उत्तराखण्डम्)
सादरं नमोनमः।
महाभागां,
सादरं निवेदनं वर्तते यत् मम अनुजः देवर्षिः भवतां विद्यालयस्य (iii) दशमी कक्षे पठति। विगत मासस्य तस्य कञ्चिदपि (iv) समाचारं न आगच्छत्। सः किं करोति? कथं पठति? तस्य (v) स्वास्थ्यं कथं वर्तते? इत्यादयः। गृहस्य सर्वे जनाः एतदर्थं (vi) दुःखिताः सन्ति। मातुः रोदनं निरन्तरम् एव भवति। अतः भवद्भ्यः (vii) प्रार्थना अस्ति यत् इदं पत्रं प्राप्य भवान् तस्मै (viii) स्वसमाचारं दातुं कथयतु। भवतां कृपा भविष्यतिः यावत् तस्य पत्रं न आगमिष्यति तावद् गृहे (ix) शान्तिः न भविष्यति।
कृपया पत्रं दातुं शीघ्रमेव वदन्तु भवन्तः।
(x) सधन्यवादम्

भवतां कृपाकांक्षी
सुन्दरः

6. भवती जागृतिः शिक्षा शुल्क-आवास शुल्क-परीक्षा शुल्कादीनामर्थे धनं प्रेषणार्थं स्व पितरं पत्रं लिखितुम् इच्छति। मञ्जूषायाः सहायतया भवती तत्सम्पूरयत।
(आप जागृति शिक्षा, आवास तथा परीक्षा शुल्क आदि हेतु धन भेजने के लिए पिता जी को पत्र लिखना चाहती हैं। मंजूषा की सहायता से उसे पूर्ण कीजिए। You are Jagriti and you want to write a letter to your father for sending you money for your house, education and examination fees. With the help of word box, complete their letter.)

छात्रावासः (कन्यार्थम्)
कन्या गुरुकुल विद्यालयः
द्रौणस्थली (उत्तराखण्ड:)
दिनाङ्क : 20-06-20……..
सेवायाम्,
पूजास्पदं पितृचरणाः,
सादरं प्रणामम्।
आशास्यते तत्र परिवारे सर्वे जनाः (i) _______________ भविष्यन्ति। अहम् अपि अत्र स्वस्था अस्मि। अत्र (ii) _______________ वातावरणम् अतीव शोभनं लाभप्रदं चास्ति। काचिद् अपि (iii) _______________ न करणीया। पितृचरणाः! भवन्तः तु जानान्ति एव यत् अस्मिन् मासे (iv) _______________ सर्वे शुल्काः देयाः सन्ति। अतः (v) _______________ आवास शुल्कं परीक्षा शुल्कं च कृत्वा पञ्चसहस्रं (vi) _______________ दातव्यानि सन्ति। कृपया एते शुल्काः अस्मिन् मासे एव देयाः इति अवधार्य शीघ्रमेव (vii) _______________ एतानि रुप्यकाणि प्रेषयतु। येन मम (viii) _______________ व्यवधानं न भवेत्। अत्र मम अध्ययनं (ix) _______________ भवति। किञ्चिदपि कष्टं नास्ति। स्वसमाचारं लिखतु भवान्।

भवदीया पुत्री
(x) _______________

मञ्जूषा- जागृतिः, छात्रावासस्य, भवान्, पठने, गुरुकुलस्य, सम्यक्, स्वस्थाः, चिन्ता, शिक्षाशुल्कं, रुप्यकाणि।
उत्तराणि:
छात्रावासः (कन्यार्थम्) कन्या गुरुकुल विद्यालयः
द्रौणस्थली (उत्तराखण्डः)
दिनाङ्क : 20-06-20……..
सेवायाम्
पूजास्पदं पितृचरणाः,
सादरं प्रणामम्।
आशास्यते तत्र परिवारे सर्वे जनाः (i) स्वस्थाः भविष्यन्ति। अहम् अपि अत्र स्वस्था अस्मि। अत्र (ii) छात्रावासस्य वातावरणम् अतीव शोभनं लाभप्रदं चास्ति। काचिद् अपि (iii) चिन्ता न करणीया। पितृचरणाः! भवन्तः तु जानान्ति एव यत् अस्मिन् मासे (iv) गुरुकुलस्य सर्वे शुल्काः देयाः सन्ति। अतः (v) शिक्षाशुल्कं आवास शुल्कं परीक्षा शुल्कं च कृत्वा पञ्चसहस्रं (vi) रुप्यकाणि दातव्यानि सन्ति। कृपया एते शुल्काः अस्मिन् मासे एव देयाः इति अवधार्य शीघ्रमेव (vii) भवान् एतानि रुप्यकाणि प्रेषयतु। येन मम (viii) पठने व्यवधानं न भवेत्। अत्र मम अध्ययन (ix) सम्यक् भवति।
किञ्चिदपि कष्टं नास्ति। स्वसमाचारं लिखतु भवान्।

भवदीया पुत्री
(x) जागृतिः

7. भवतः नाम धर्मधरः आर्यः वर्तते। भवान् स्व मातुः स्वास्थ्य विषये स्वमातुलं अर्जुन देव आर्यम् एकं पत्रं लिखितुम् इच्छति। मञ्जूषायाः सहायतया तत् सम्पूर्य लिखतु भवान्।
(आपका नाम धर्मधर आर्य है। आप अपनी माता जी के स्वास्थ्य के विषय में अपने मामा अर्जुन देव आर्य को एक पत्र लिखना चाहते हैं। मंजूषा की सहायता से उसे पूर्ण करके आप लिखिए। Your name is Dharmdhar Arya. You want to write a letter to your maternal uncle, Arjun Dev Arya asking him about your mother’s health. With the help of words in box, complete this letter.)

परीक्षा भवनम्
देवानन्दः विज्ञानशाला
राजकोट (गुजरात)
दिनाङ्क : 20-01-20…
सेवायाम्
समादरणीयाः मातुल चरणाः (i) ____________।
सादरं प्रणामम्।
अत्र सर्वं कुशलं वर्तते। तत्रस्थानां सर्वेषां (ii) ____________ कुशलतां कामये।
मातुल! समाचारोऽयं यत् इदानीं (iii) ____________ अस्वस्थाः सन्ति। तस्याः हृदये शनैः-शनैः पीड़ा भवति। यस्याः (iv) ____________ योग्येन चिकित्सकेन भवति। सम्प्रति सा भवन्तम् अधिकं (v) ____________। यदि भवतां दृष्ट्या अन्यः कश्चिद् योग्यः (vi) ____________ भवेत् तर्हि अपि कथयतु भवान्। अहं तां तत्र नीत्वा उपचारं कारयिष्यामि। अधुना मातुः पाचनक्रिया अपि (vii) ____________ अभवत्। तस्याः भोजनस्य पाचनकार्यं तु (viii) ____________ एव न। अतः आगते समये पाचने सहायकं किञ्चिद् (ix) ____________ अपि भवान अत्र नयेत्। अहं भवताम् आभारी भविष्यामि। कृपया शीघ्रमेव आगच्छतु भवान्।

भवदीयः भागिनीसुतः
(x) ____________

मञ्जूषा- औषधिम्, अर्जुन देव आर्याः, चिकित्सा, दुर्बला, धर्मधर आर्यः, स्मरति, जनानां, भवति, मातृचरणाः, चिकित्सकः।
उत्तराणि:
परीक्षा भवनम्
देवानन्दः विज्ञानशाला
राजकोट (गुजरात)
दिनाङ्क : 20-01-20……
सेवायाम् समादरणीयाः मातुल चरणाः (i) अर्जुन देव आर्याः!
सादरं प्रणामम्।
अत्र सर्वं कुशलं वर्तते। तत्रस्थानां सर्वेषां (ii) जनानां कुशलतां कामये।
मातुल! समाचारोऽयं यत् इदानीं (iii) मातृचरणाः अस्वस्थाः सन्ति। तस्याः हृदये शनैः-शनैः पीड़ा भवति। यस्याः (iv) चिकित्सा योग्येन चिकित्सकेन भवति। सम्प्रति सा भवन्तम् अधिकं (v) स्मरति । यदि भवतां दृष्ट्यां अन्यः कश्चिद् योग्यः (vi) चिकित्सकः भवेत् तर्हि अपि कथयतु भवान्। अहं तां तत्र नीत्वा उपचारं कारयिष्यामि। अधुना मातुः पाचनक्रिया अपि (vii) दुर्बला अभवत्। तस्याः भोजनस्य पाचनकार्यं तु (viii) भवति एव न। अतः आगते समये पाचने सहायकं किञ्चिद् (ix) औषधिम् अपि भवान् अत्र नयेत्।
अहं भवताम् आभारी भविष्यामि। कृपया शीघ्रमेव आगच्छतु भवान्।

भवदीयः भागिनीसुतः
(x) धर्मधर आर्यः

8. विद्यालयस्य वार्षिकोत्सवम् अधिकृत्य मित्रं प्रति लिखिते पत्रे रिक्तस्थानानि पूरयत।
(विद्यालय के वार्षिकोत्सव का वर्णन करके मित्र को लिखे गए पत्र में रिक्त स्थान भरिए। Complete the letter, written to your friend describing about annual function of your school.)

(i) ____________
तिथि: 20.3.20……

प्रिय मित्र (ii) ____________
अद्य तव पत्रं (iii) ____________ समाचारः अयम् यत् गते सप्ताहे विद्यालयस्य (iv) ____________ आसीत्। अहं सर्वे च अध्यापकाः (v) ____________ आसन्। शिक्षानिदेशकः कार्यक्रमस्य (vi) ____________ आसीत्। सः अस्माकम् (vii) ____________ प्राशंसत्। सः योग्येभ्यः छात्रेभ्यः (viii) ____________ अयच्छत्। पितृभ्याम् मम (ix) ____________ निवेदयतु।

(x) भवतः ____________
क०ख०ग०

मञ्जूषा- वार्षिकोत्सवः, कार्यक्रमम्, मित्रम्, प्रणामः, परीक्षाभवनम्, व्यस्ताः, मुख्यातिथिः, पारितोषिकानि, प्राप्तम्, राम।
उत्तराणि:
(i) परीक्षाभवनम्
तिथि: 20.3.20……
प्रिय मित्र (ii) राम,
अद्य तव पत्रं (iii) प्राप्तम्। समाचारः अयम् यत् गते सप्ताहे विद्यालयस्य (iv) वार्षिकोत्सवः आसीत्। अहं सर्वे च अध्यापकाः (v) व्यस्ताः आसन्। शिक्षानिदेशक: कार्यक्रमस्य (vi) मुख्यातिथिः आसीत्। सः अस्माकम् (vii) कार्यक्रमम् प्राशंसत्। सः योग्येभ्यः छात्रेभ्यः (viii) पारितोषिकानि अयच्छत्। पितृभ्याम् मम (ix) प्रणामः निवेदयतु।

(x) भवतः मित्रम्,
क०ख०ग०

9. मित्रं प्रति लिखितं निम्नलिखितं पत्रम् मञ्जूषायां प्रदत्तैः पदैः पूरयत।
(मित्र को लिखे गए निम्नलिखित पत्र को मञ्जूषा में दिए गए पदों से भरिए। Complete the following letter, with suitable words from the box, written to a friend.)

अरुणाचलप्रदेशः
तिथि: 20.2.20……

प्रिय (i) ____________ भास्कर,
सस्नेह नमस्ते।
(ii) ____________ अहं मित्रैः सह शैक्षिकभ्रमणाय ‘दार्जिलिङ्ग इति’ पर्वतीयस्थलं गतवान्। (iii) ____________ स्थानस्य सौन्दर्यम् अद्भुतम् (iv) ____________ अस्ति। विशालैः (v) ____________ सुसज्जिता इयं देवभूमिः एव अस्ति। एवं प्रतीयते यत् (vi) ____________ अन्यस्मिन् एव संसारे वसामः। इमं सुरम्यं प्रदेशं दृष्ट्वा इदम् असत्यम् एव प्रतीयते यत् पर्वताः केवलं दूरतः एव (vii) ____________ पर्वता: तु सदैव रम्याः एव भवन्ति। अहं त्वया सह अपि एकवारं तत्र पुनः (viii) ____________ इच्छामि। आशासे आवां शीघ्रं गमिष्यावः। अधुना विरमामि। सर्वेभ्यः मम (ix) ____________ कथनीयः।

(x) ____________ मित्रम्,
शैलेन्द्रः

मञ्जूषा- गन्तुम्, वृक्षैः, नमस्कारः, मित्र, भवतः, अस्य, एव, वयं, रम्याः, गतसप्ताहे।
उत्तराणि:

अरुणाचलप्रदेशः
तिथि: 20.2.20……

प्रिय (i) मित्र भास्कर,
सस्नेह नमस्ते।
(ii) गतसप्ताहे अहं मित्रैः सह शैक्षिकभ्रमणाय ‘दार्जिलिङ्ग इति’ पर्वतीयस्थलं गतवान्। (iii) अस्य स्थानस्य सौन्दर्यम् अद्भुतम् (iv) एव अस्ति। विशालैः (v) वृक्षैः सुसज्जिता इयं देवभूमिः एव अस्ति। एवं प्रतीयते यत् (vi) वयं अन्यस्मिन् एव संसारे वसामः। इमं सुरम्यं प्रदेशं दृष्ट्वा इदम् असत्यम् एव प्रतीयते यत् पर्वताः केवलं दूरतः एव (vii) रम्याः। पर्वताः तु सदैव रम्याः एव भवन्ति । अहं त्वया सह अपि एकवारं तत्र पुनः (viii) गन्तुम् इच्छामि। आशासे आवां शीघ्रं गमिष्यावः। अधुना विरमामि। सर्वेभ्यः मम (ix) नमस्कार: कथनीयः।

(x) भवतः मित्रम्,
शैलेन्द्रः

10. मित्रं प्रति लिखितं निम्नलिखितं पत्रम् मञ्जूषायां प्रदत्तैः उचितैः शब्दैः पूरयत।
(मित्र को लिखे गए निम्नलिखित पत्र को मञ्जूषा में दिए गए शब्दों से भरिए। Complete the following letter, with suitable words from the box, written to a friend.)

(i) ____________
तिथि: 28.6.20……

प्रियमित्र दिवाकर,
(ii) ____________ नमस्ते।
(iii) ____________ अहं मित्रैः सह जन्तुशालां द्रष्टुं काननवनम् अगच्छम्। तत्र (iv) ____________ अनेकान् पशून् अपश्याम। सर्वे पशवः इतस्ततः भ्रमन्ति स्म। सिंहाः उच्चैः (v) ____________ मयूराः नृत्ये (vi) ____________ आसन्। वस्तुतः मयूर (vii) ____________ कुत्र जन्तुशालायाः भव्यशोभा? तत्र आम्रवृक्षाः अपि आसन्, कोकिला (viii) ____________। वस्तुतः यत्र आम्रवृक्षाः (ix) ____________ कोकिला तु भविष्यति एव। अग्रे पुनः लेखिष्यामि। (x) ____________ मम नमस्कार: कथनीयः।

भवदीयम् अभिन्नमित्रम्
शेखरः

मञ्जूषा- विना, तत्र, वयम्, सस्नेह, चेन्नईतः, सर्वेभ्यः, अगर्जन्, ह्यः, मग्नाः, अपि।
उत्तराणि:

(i) चेन्नईतः
तिथि: 28.6.20……

प्रियमित्र दिवाकर,
(ii) सस्नेह नमस्ते।
(iii) ह्यः अहं मित्रैः सह जन्तुशालां द्रष्टुं काननवनम् अगच्छम् । तत्र (iv) वयम् अनेकान् पशून् अपश्याम। सर्वे पशवः इतस्ततः भ्रमन्ति स्म। सिंहाः उच्चैः (v) अगर्जन्। मयूराः नृत्ये (vi) मग्नाः आसन्। वस्तुतः मयूरं (vii) विना कुत्र जन्तुशालायाः भव्यशोभा? तत्र आम्रवृक्षाः अपि आसन्, कोकिला (viii) अपि। वस्तुतः यत्र आम्रवृक्षाः (ix) तत्र कोकिला तु भविष्यति एव। अग्रे पुनः लेखिष्यामि। (x) सर्वेभ्यः मम नमस्कार: कथनीयः।

भवदीयम् अभिन्नमित्रम्
शेखरः

11. मित्रं प्रति परीक्षासफलतायाम् लिखितं पत्रं मञ्जूषायां प्रदत्तैः शब्दैः पूरयत।
(परीक्षा में सफल होने पर मित्र को लिखे गए पत्र को मञ्जूषा में दिए गए उचित शब्दों से भरिए। Complete this letter, with suitable words from the box, written to your friend on his success in the examination.)

(i) ____________।
तिथि: 22.9.20…..

(ii) ____________
(iii) ____________।
भवतः परीक्षासफलतापत्रम् अधुनैव (iv) ____________। भवतः उत्तीर्णतां ज्ञात्वा मयि अति (v) ____________ अस्ति। अहोरात्रं प्रयासं विधाय भवान् 95 प्रतिशतम् (vi) ____________ लब्धवान्। त्वं मम परिवारजनस्य (vii) ____________अर्हसि। पत्रसमाप्तौ तुभ्यं पुनः वर्धापनं (viii) ____________। (ix) ____________ सादरं नमः।

भवतः (x) ____________
अ०ब०स०

मञ्जूषा- परीक्षाभवनात्, सन्तोषः, अङ्कान्, मित्रम्, साधुवादान्, कामये, प्राप्तम्, पितृभ्याम्, प्रियमित्र, सप्रेम नमस्कारम्।
उत्तराणि:

(i) परीक्षाभवनात्।
तिथि: 22.9.20……

(ii) प्रियमित्र,
(iii) सप्रेम नमस्कारम्।
भवत: परीक्षासफलतापत्रम् अधुनैव (iv) प्राप्तम्। भवतः उत्तीर्णतां ज्ञात्वा मयि अति (५) सन्तोषः अस्ति । अहोरात्रं प्रयासं विधाय भवान् 95 प्रतिशतम् (vi) अङ्कान् लब्धवान्। त्वं मम परिवारजनस्य (vii) साधुवादान् अर्हसि। पत्रसमाप्तौ तुभ्यं पुनः वर्धापनं (viii) कामये। (ix) पितृभ्याम् सादरं नमः।

भवतः (x) मित्रम्
अ०ब०स०

12. लघुभ्रातरं सोमेशं द्धद्दप्रति लिखिते पत्रे मञ्जूषायां प्रदत्तैः शब्दैः रिक्तस्थानपूर्तिं कुरुत।
(छोटे भाई सोमेश को लिखे गए पत्र को मञ्जूषा में दिए गए उचित शब्दों से रिक्त स्थान की पूर्ति कीजिए। Complete this letter, with suitable words from the box, written to your younger brother Somesh.)

(i) ____________
आगरा।
तिथि: 22.9.20……

प्रिय (ii) ____________ सोमेश,
सस्नेहं आशीर्वादम्।
(iii) ____________ अहं तव पत्रं प्राप्तवान्। (iv) ____________ परीक्षापरिणामः (v) ____________ असति, परं गणितविषये भवता न्यूनाः अङ्काः (vi) ____________ इति सखेदं मया अधीतम्। (vii) ____________ प्रतिदिनंप्रातः काले उत्थाय गणितस्य (viii)
____________ कुरु, गणिताध्यापकं च पुनः पुनः प्रश्नान् पृच्छ। अभ्यासेन एव सर्वाणि कार्याणि सिध्यन्ति। (ix) ____________ चरणयोः सादरं प्रणामः।

भवदीयः अग्रजः,
(x) ____________

मञ्जूषा- पित्रोः, त्वं, अभ्यासं, छात्रावासतः, अनुज, ह्यः, शोभनः, श्रीशः, तव, प्राप्ताः।
उत्तराणि:

(i) छात्रावासतः
आगरा।
तिथि: 22.9.20……

प्रिय (ii) अनुज सोमेश,
सस्नेहं आशीर्वादम्।
(iii) ह्यः अहं तव पत्रं प्राप्तवान्। (iv) तव परीक्षापरिणामः (v) शोभनः अस्ति, परं गणितविषये भवता न्यूनाः अङ्काः (vi) प्राप्ताः इति सखेदं मया अधीतम्। (vii) त्वं प्रतिदिनं प्रातः काले उत्थाय गणितस्य (viii) अभ्यासं कुरु, गणिताध्यापकं च पुनः पुनः प्रश्नान् पृच्छ। अभ्यासेन एव सर्वाणि कार्याणि सिध्यन्ति।
(ix) पित्रो: चरणयोः सादरं प्रणामः।

भवदीयः अग्रजः,
(x) श्रीशः

13. विद्यालयस्य वार्षिकोत्सवम् अधिकृत्य मित्रं प्रति लिखिते पत्रे मञ्जूषाशब्दैः रिक्तस्थानपूर्तिं कुरुत।
(विद्यालय के वार्षिकोत्सव को अधिकृत करके मित्र को लिखे गए पत्र में मञ्जूषा के शब्दों से रिक्त स्थान की पूर्ति कीजिए।) (Complete this letter, with suitable words from the box, written to your friend specially mentioning the annual function of the school.)

(i) ____________
कुरुक्षेत्रम्।
तिथि: 10.10.20……

प्रिय (ii) ____________
(iii) ____________ तव पत्रम् अधिगतम्। मया पत्रेण ज्ञातं यत् गत सप्ताहे विद्यालयस्य (iv) ____________ आसीत्। त्वं सर्वे अध्यापकाः च (v) ____________ अतिष्ठन्। कार्यक्रमस्य मुख्यातिथि: (vi) ____________ आसीत्। सः (vii) ____________ कार्यक्रमस्य प्रशंसाम् अकरोत्। (viii) ____________ छात्रेभ्यः पुरस्कारान् प्रायच्छत्। पितृभ्यां सादरं (ix) ____________

भवत: (x) ____________
क०ख०ग०

मञ्जूषा- शिक्षानिदेशकः, मया, परीक्षाभवनम्, वार्षिकोत्सवः, व्यस्ताः, प्रणामाः, युष्माकम्, योग्येभ्यः, प्रियमित्रम्, मित्र।
उत्तराणि:

(i) परीक्षाभवनम्
कुरुक्षेत्रम्।
तिथि: 10.10.20……

प्रिय (ii) मित्र
(iii) मया तव पत्रम् अधिगतम्। मया पत्रेण ज्ञातं यत् गत सप्ताहे विद्यालयस्य (iv) वार्षिकोत्सवः आसीत्। त्वं सर्वे अध्यापका: च (v) व्यस्ताः अतिष्ठन्। कार्यक्रमस्य मुख्यातिथिः (vi) शिक्षानिदेशकः आसीत्। सः (vii) युष्माकम् कार्यक्रमस्य प्रशंसाम् अकरोत्। (viii) योग्येभ्यः छात्रेभ्यः पुरस्कारान् प्रायच्छत्। पितृभ्यां सादरं (ix) प्रणामाः।

भवतः (x) प्रियमित्रम्,
क०ख०ग०

14. भवतः नाम अर्णवः। भवान् छात्रावासे निवसति। आगरायाः ताजमहलम्’ इति स्थाने शैक्षिकभ्रमणाय गन्तुं भवान् इच्छति। तदर्थं धनप्रेषणार्थं पितरं प्रति अधः अपूर्णं पत्रं लिखितम्। मञ्जूषायाः सहायतया उचितशब्दैः रिक्तस्थानानि पूरयित्वा पुनः पत्रम् उत्तरपुस्तिकायां लिखत।
(आपका नाम अर्णव है। आप छात्रावास में रहते हैं। आप शैक्षिक भ्रमण के लिए आगरा स्थित ताजमहल जाना चाहते हैं। इसके लिए धन भेजने हेतु पिता जी को नीचे अपूर्ण पत्र लिखा गया है। मञ्जूषा के उचित शब्दों की सहायता से रिक्त स्थान भरकर पत्र पुनः उत्तर पुस्तिका में लिखिए। You are Arnav. You reside at a hostel. You want to visit Taj Mahal of Agra on an educational tour. The following letter written to the father for sending money is incomplete. Re-write the letter in answer book, after completing with suitable words from the box.)

20, नवोदयविद्यालयछात्रावासः,
(i) ____________,
तिथिः 4.11.20……

परम-आदरणीयाः पितृमहाभागाः,
सादरं (ii) ____________।
सविनयं निवेदनम् अस्ति यत् मम मासिकी परीक्षा (iii) ____________ जाता। मम उत्तरपत्राणि (iv) ____________ अभवन्। अस्मिन् ग्रीष्मावकाशे अहं गृहं न (v) ____________ यतः विद्यालयेन एकस्याः (vi) ____________ आयोजनं कृतम्। एषा यात्रा आगरा-ताजमहलं द्रष्टुम् (vii) ____________ अस्ति। यात्रा-व्ययार्थं पञ्चशतं (viii) ____________ भवन्तः प्रेषयन्तु। शेषं सर्वं कुशलम्। (ix) ____________ अग्रजाय च मम प्रणामाः।

(x) ____________ प्रियपुत्रः,
अर्णवः

मञ्जूषा- भवदीयः, समाप्ता, शैक्षिकयात्रायाः, मुम्बईनगरम्, रुप्यकाणि, जनन्यै, आगमिष्यामि, प्रणामाः, शोभनानि, आयोजिता।
उत्तराणि:

20, नवोदयविद्यालयछात्रावासः,
(i) मुम्बईनगरम्,
तिथिः 4.11.20……

परम-आदरणीयाः पितृमहाभागाः,
सादरं (ii) प्रणामाः।
सविनयं निवेदनम् अस्ति यत् मम मासिकी परीक्षा (iii) समाप्ता जाता। मम उत्तरपत्राणि (iv) शोभनानि अभवन्। अस्मिन् ग्रीष्मावकाशे अहं गृहं न (v) आगमिष्यामि यतः विद्यालयेन एकस्याः (vi) शैक्षिकयात्रायाः आयोजनं कृतम्। एषा यात्रा आगराताजमहलं द्रष्टुम् (vii) आयोजिता अस्ति। यात्रा-व्ययार्थं पञ्चशतं (viii) रुप्यकाणि भवन्तः प्रेषयन्तु। शेषं सर्वं कुशलम्। (ix) जनन्यै अग्रजाय च मम प्रणामाः।

(x) भवदीयः प्रियपुत्रः,
अर्णवः

15. स्वविद्यालयस्य वर्णनं कुर्वन् मित्रं संजीवं प्रति अधः लिखितं पत्रम् उत्तरपुस्तिकायां रिक्तस्थानपूर्तिं कृत्वा पुनः लिखत। सहायतायै मञ्जूषायां पदानि दत्तानि।
(अपने विद्यालय का वर्णन करते हुए मित्र संजीव को नीचे लिखे गए पत्र में रिक्त स्थान पूर्ति करके पुनः उत्तर पुस्तिका में लिखिए। सहायता के लिए मञ्जूषा में पद दिए गए हैं। Re-write the following letter in your answer book after filling the blanks, written to your friend Sanjeev, describing your school. For your help, words are given in the box.)

परीक्षाभवनम्,
तिथि: 10.10.20……

प्रिय (i) ____________
सस्नेहं नमस्कारः।
भवतः पत्रं प्राप्तम्। (ii) ____________ प्रासीदत्। यथा भवता कथितं तथा अहं पत्रोत्तरे स्वविद्यालयस्य वर्णनं (iii) ____________ मम (iv) ____________ अतीव विशालः सुन्दरः च अस्ति । अत्र त्रिसहस्रं छात्राः (v) ____________ पठन्ति। (vi) ____________ पुस्तकानां पत्र-पत्रिकाणां च सुव्यवस्था अस्ति। (vii) ____________ वालीबाल-बैडमिन्टन-क्रिकेट रज्जु आकर्षणादि खेलानाम् उत्तमः प्रबन्धः अस्ति। परिषदः परीक्षापरिणामः प्रतिवर्ष (viii) ____________ भवति। मातपित्रोः (ix) ____________ प्रणामाः।

भवतः (x) ____________
क०ख०ग०

मञ्जूषा- पुस्तकालये, चरणयोः, अभिन्नमित्रम्, संजीव, शतप्रतिशतम् , मनोयोगेन, मनः, करोमि, क्रीडाक्षेत्रे, विद्यालयः।
उत्तराणि:

परीक्षाभवनम्,
तिथि: 10.10.20……

प्रिय (i) संजीव , सस्नेह नमस्कारः। भवतः पत्रं प्राप्तम्। (ii) मनः प्रासीदत्। यथा भवता कथितं तथा अहं पत्रोत्तरे स्वविद्यालयस्यवर्णनं (ii) करोमि। (iv) विद्यालयः अतीव विशालः सुन्दरः च अस्ति। अत्र त्रिसहस्र छात्राः (v) मनोयोगेन पठन्ति। (vi) पुस्तकालये पुस्तकानां पत्र-पत्रिकाणां च सुव्यवस्था अस्ति। (vii) क्रीडाक्षेत्रे वालीबाल-बैडमिन्टन-क्रिकेट रज्जु आकर्षणादि खेलानाम् उत्तमः प्रबन्धः अस्ति। परिषदः परीक्षापरिणामः प्रतिवर्ष (viii) शतप्रतिशतम् भवति। मातपित्रोः (ix) चरणयोः प्रणामाः।

भवतः (x) अभिन्नमित्रम्
क०ख०ग०

16. रुग्णतायाः कारणात् अवकाशप्राप्तये प्रधानाचार्य प्रति पत्रम्-
(बीमार होने की वजह से अवकाश प्राप्त करने के लिए प्रधानाचार्य को पत्र- Left Application to the Principal for approving leave caused by illness.)

सेवायाम्
प्रधानाचार्य-महोदयाः
केन्द्रीय विद्यालयः
केशव पुरम्, सैक्टर:-द्वितीयाः
नव दिल्ली – 110022
विषयः-(i) ____________ निवेदनम मान्यवराः महोदयाः
सविनयं (ii) ____________ यत् अहं शिरोवेदनया (iii) ____________ अस्मि । एतस्मात्कारणात् (iv) ____________ विद्यालयम् (v) ____________ (vi) ____________। अतः प्रार्थये (vii) ____________ दिनद्वयस्य (viii) ____________ रदाय अनुग्रहं (ix) _________ भवन्तः ।
धन्यवादाः

भवतां (x) ____________ शिष्यः
नरेन्द्रः
कक्षा 10
वर्ग: ‘ब’

दिनाङ्कः ____________

मञ्जूषा- कुर्वन्तु, विनीतः, निवेदमस्ति, पीड़ितः, अद्य, आगन्तुमसमर्थः, अस्मि, यत्, अवकाशं, अवकाशप्राप्तये,।
उत्तराणि:
सेवायाम्
प्रधानाचार्य-महोदयाः
केन्द्रीय-विद्यालयः
आर. के. पुरम्, सैक्टर:-द्वितीयः
नव दिल्ली – 110022
विषयः – (i) अवकाशप्राप्तये निवेदनम्
मान्यवराः महोदयाः
सविनयं (ii) निवेदमस्ति यत् अहं शिरोवेदनया (iii) पीड़ितः अस्मि। एतस्मात्कारणात् (iv) अद्य विद्यालयम् (७) आगन्तुमसमर्थः (vi) अस्मि। अतः प्रार्थये (vii) यत् दिनद्वयस्य (viii) अवकाशं प्रदाय अनुग्रहं (ix) कुर्वन्तु भवन्तः। धन्यवादाः

भवतां (x) विनीतः शिष्यः
नरेन्द्रः
कक्षा 10
वर्ग: ‘ब’

दिनाङ्कः ____________

17. शुल्कक्षमापनार्थं प्रधानाचार्य प्रति पत्रम्-
(शुल्क माफी के लिए प्रधानाचार्या को पत्र-Letter to the Principal for remission of fee)
सेवायाम्
प्रधानाचार्य-महोदयाः
केन्द्रीय विद्यालयः
आर. के. पुरम्, सैक्टर:-चतुर्थः
नव दिल्ली – 110022
विषयः-शुल्कक्षमापनार्थ (i) ____________
(ii) ____________ महोदयाः
सविनयं (iii) ____________ यत् अहं भवतः (iv) ____________ दशमकक्षायाः ‘स’ वर्गस्य (v) ____________ अस्मि। मम पिता (vi) ____________ विद्यालये द्वारपालः अस्ति। तस्य मासिकवेतनम् द्विसहस्ररूप्यकमात्रम् अस्ति। (vii) ____________ कुटुम्बे पञ्च सदस्याः सन्ति। अनेन वेतनेन कुटुम्बस्य निर्वाहः (viii) ____________भवति। अतः शुल्कक्षमापनार्थं प्रार्थये।
आक्षासे यत् मदीयाम् इमां (ix) ____________ स्वीकृत्य शुल्कक्षमापनद्वारा माम् (x) ____________ श्रीमन्तः।
धन्यवादाः।

भवतां विनीतः शिष्यः
सुरेन्द्रः
कक्षा 10
वर्ग: ‘ब’

दिनाङ्कः ____________

मञ्जूषा – निवेदनम्, उपकरिष्यन्ति, प्रार्थना, काठिन्येन, अस्माकं, एकस्मिन्, छात्रः, मान्यवराः, निवेदनमस्ति, विद्यालये।
उत्तराणि:
सेवायाम्
प्रधानाचार्य-महोदयाः
केन्द्रीय विद्यालयः
आर. के. पुरम्, सैक्टर:-चतुर्थः
नव दिल्ली – 110022
विषयः – शुल्कक्षमापनार्थं (i) निवेदनम्
(ii) मान्यवराः महोदयाः
सविनयं (iii) निवेदनमस्ति यत् अहं भवतः (iv) विद्यालये दशमकक्षायाः ‘स’ वर्गस्य (५) छात्रः अस्मि। मम पिता (vi) एकस्मिन् विद्यालये द्वारपालः अस्ति। तस्य मासिकवेतनम् द्विसहस्ररूप्यकमात्रम् अस्ति। (vii) अस्माकं कुटुम्बे पञ्च सदस्याः सन्ति। अनेन वेतनेन कुटुम्बस्य निर्वाहः (viii) काठिन्येन भवति। अतः शुल्कक्षमापनार्थं प्रार्थये। आक्षासे यत् मदीयाम् इमां (xi) प्रार्थनां स्वीकृत्य शुल्कक्षमापनद्वारा माम् (x) उपकरिष्यन्ति श्रीमन्तः।
धन्यवादाः।

भवतां विनीतः शिष्यः
सुरेन्द्रः
कक्षा 10
वर्ग: ‘ब’

दिनाङ्कः ____________

By going through these CBSE Class 12 Maths Notes Chapter 11 Three Dimensional Geometry, students can recall all the concepts quickly.

Three Dimensional Geometry Notes Class 12 Maths Chapter 11

1. Direction cosines of a line:
Let AB be a line in space.
Through O, draw a line OP parallel to AB. Let OP makes angles α, β, and γ with OX, OY, and OZ respectively.

Cosines of the angles α, β, and γ,
i.e., cos α, cos β, and cos γ are known as the direction cosines of line AB.
Let l = cos α, m = cos β, n = cos γ
⇒ l, m, n are the direction cosines of the line AB Let us consider the ray BA. OQ is drawn parallel to BA. Now, OQ makes angles n – α, n – β, and n – γ with coordinates axes OX, OY, and OZ respectively.

∴ Direction cosines of BA are cos(π – α), cos (π – β) and cos (π – γ),
i.e., – cos α, – cos β, – cos γ or -l, -m, -n.

→ Relation between l, m, and n
AB is any ray having direction cosines l, m, n. Now OP is drawn parallel to AB, where P is the point (x, y, z). Let PM be drawn perpendicular to OY.

In ΔOPM OP = r (say). PM⊥OY.
∴ ∠PMO = 90°
Also, ∠POM = β.
∴ \(\frac{\mathrm{OM}}{\mathrm{OP}}\) = β
∴ \(\frac{y}{r}\) = m,
∴ y = rm

Similarly, x = rl and z = rn.
Now, OP² = x² + y² + z²
r² = (rl)² + (rm)² + (rn)²
or
r² = r²(l² + m² + n²)
⇒ l² + m² + n² = 1.

→ Direction Ratios of a line
Definition: The numbers which are proportional to the direction cosines of a line are known as direction ratios of the line.

Let Z, m, n be the direction cosines of a line.
Multiplying each by r, we ger rl, rm, rn the direction ratios.
Let rl = a,rm = b and rn – c.
Squaring and adding
r²l² + r²m² + r²n² = a² + b² + c²
∴ r²(l² + m² + n²) = a² + b² + c²
∵ l² + m² + n² = 1
∴ l = \(\frac{a}{r}=\frac{a}{\sqrt{a^{2}+b^{2}+c^{2}}}\)

Similarly, m = \(\frac{b}{\sqrt{a^{2}+b^{2}+c^{2}}}\) and n = \(\frac{c}{\sqrt{a^{2}+b^{2}+c^{2}}}\)

Thus, if a, b and c are the direction ratios of a line, the direction cosines are \(\frac{a}{\sqrt{a^{2}+b^{2}+c^{2}}}\), \(\frac{b}{\sqrt{a^{2}+b^{2}+c^{2}}}\) and \(\frac{c}{\sqrt{a^{2}+b^{2}+c^{2}}}\).

→ Direction cosines of the line passing through the points P(x₁, y₁, z₁) and Q(x₂, y₂, z₂)
Direction ratios of the line PQ are x₂ – x₁, y₂ – y_1, z₂ – z₁
∴ PQ = \(\sqrt{\left(x_{2}-x_{1}\right)^{2}+\left(y_{2}-y_{1}\right)^{2}+\left(z_{2}-z_{1}\right)^{2}}\)
∴Direction cosines of PQ are \(\frac{x_{2}-x_{1}}{\mathrm{PQ}}\), \(\frac{y_{2}-y_{1}}{\mathrm{PQ}}\), \(\frac{z_{2}-z_{1}}{P Q}\)

2. Angle between the two lines
1. Let l_1, m₁, n₁, and l₂, m₂, n₂ be the direction cosines of the lines OP and OQ.

The angle θ between these lines is given by
cos θ = l₁l₂ + m₁m₂ + n₁n₂
and sin θ = \(\sqrt{\left(m_{1} n_{2}-m_{2} n_{1}\right)^{2}+\left(n_{1} l_{2}-n_{2} l_{1}\right)^{2}+\left(l_{1} m_{2}-l_{2} m_{1}\right)^{2}}\)

2. If a₁, b₁, c₁ and a₂, b₂, c₂ are the direction ratios of two lines, then

3. Two lines are perpendicular to each other,
if θ = \(\frac{π}{2}\) ⇒ cos θ = cos \(\frac{π}{2}\) = 0
⇒ l₁l₂ + m₁m₂ + n₁n₂ = 0
or
a₁a₂ + b₁b₂ + c₁c₂ = 0

4. When the lines are parallel
θ = 0 ⇒ sin θ = sin 0 = 0.

STRAIGHT LINE
3. Equation of a line through a given point:
(a) Let the line passes through \(\vec{a}\) and is parallel to vector \(\vec{b}\)

Then, the equation of the line is
\(\vec{r}\) – \(\vec{a}\) = λ\(\vec{b}\)
or
\(\vec{r}\) = \(\vec{a}\) + λ\(\vec{b}\)

(b) Let the point A be (x₁, y₁, z₁) and a, b, c are the direction ratios of the line. The equation of the line is
\(\frac{x-x_{1}}{a}=\frac{y-y_{1}}{b}=\frac{z-z_{1}}{c}\)
If l, m, n are the direction cosines, then equation of the line is
\(\frac{x-x_{1}}{l}=\frac{y-y_{1}}{m}=\frac{z-z_{1}}{n}\)

4. Equation of the line passing through two points:
(a) Let \(\vec{a}_{1}\), \(\vec{a}_{2}\) be the position vectors of two points P and Q respectively.
⇒ \(\vec{b}\) = \(\vec{a}_{1}\) – \(\vec{a}_{2}\)

∴ Equation of PQ is
\(\vec{r}\) = \(\vec{a}\) + λ(\(\vec{a}_{2}\) – \(\vec{a}_{1}\))

(b) Direction ratios of the line passing through P(x₁, y₁, z₁) and Q(x₂, y₂, z₂) are x₂ – x₁, y₂ – y₁ and z₂ – z₁
∴ Equation of the line PQ is
\(\frac{x-x_{1}}{x_{2}-x_{1}}=\frac{y-y_{1}}{y_{2}-y_{1}}=\frac{z-z_{1}}{z_{2}-z_{1}}\)

5. Angle between two straight lines:

(a) Let the two lines be
\(\vec{r}\) = \(\vec{a}_{1}\) + λ\(\vec{b}_{1}\)
\(\vec{r}\) = \(\vec{a}_{2}\) + λ\(\vec{b}_{2}\)
If θ be the angle between them, then
cos θ = \(\frac{\vec{b}_{1} \vec{b}_{2}}{\left|\vec{b}_{1}\right|\left|\vec{b}_{2}\right|}\)

(b) Let the lines be
\(\frac{x-x_{1}}{a_{1}}=\frac{y-y_{1}}{b_{1}}=\frac{z-z_{1}}{c_{1}}\)
and \(\frac{x-x_{2}}{a_{2}}=\frac{y-y_{2}}{b_{2}}=\frac{z-z_{2}}{c_{2}}\)
i.e., a1, b1, c1 and a2, b2, c2 are the direction ratios of the lines.
∴ cos θ = \(\frac{a_{1} a_{2}+b_{1} b_{2}+c_{1} c_{2}}{\sqrt{a_{1}^{2}+b_{1}^{2}+c_{1}^{2}} \sqrt{a_{2}^{2}+b_{2}^{2}+c_{2}^{2}}}\)
If l1, m1, n1 and l₂, m₂, n₂ are the direction cosines, then
cos θ = l₁l₂ + m1m₂ +n1n₂

6. Shortest Distance:
(a)Let \(\vec{r}\) = \(\vec{a}_{1}\) + λ\(\vec{b}_{1}\) and
\(\vec{r}\) = \(\vec{a}_{2}\) + λ\(\vec{b}_{2}\) be the two non-intersecting lines.
The shortest distance between the given lines = \(\left|\frac{\left(\vec{b}_{1} \times \vec{b}_{2}\right) \cdot\left(\vec{a}_{2}-\vec{a}_{1}\right)}{\left|\vec{b}_{1} \times \vec{b}_{2}\right|}\right|\)

(b) Let the lines be

If the lines are intersecting, then lines are coplanar.
⇒ S.D. = 0
⇒ (\(\vec{b}_{1} \times \vec{b}_{2}\)) – (\(\vec{a}_{1} \times \vec{a}_{2}\)) = 0
or
\(\left|\begin{array}{ccc}
x_{2}-x_{1} & y_{2}-y_{1} & z_{2}-z_{1} \\
a_{1} & b_{1} & c_{1} \\
a_{2} & b_{2} & c_{2}
\end{array}\right|\) = 0

7. Distance between parallel lines:
Let the parallel lines be
\(\vec{r}\) = \(\vec{a}_{1}\) + λ\(\vec{b}_{1}\) and
\(\vec{r}\) = \(\vec{a}_{2}\) + μ\(\vec{b}_{2}\)

Shortest distance d between these lines is given by,
d = \(\left|\frac{\vec{b} \times\left(\vec{a}_{1}-\vec{a}_{2}\right)}{|\vec{b}|}\right|\)

PLANES
8. Different forms of equations of a plane:
1. Normal Form.
(a) Let the plane ABC be at a distance d from the origin. ON is the normal to the plane in the directon n̂. Equation of the plane is \(\vec{r}\). n̂ = d.

(b) If l, m, n are the direction cosines of the normal to the plane which is at distance d from the origin. The equation of the plane is lx + my + nz = d.
However, general form of the equation of a plane are \(\vec{r}\) .\(\overrightarrow{\mathrm{N}}\) = D and Ax + By + Cz + D = 0.

2. (a) Let the plane passes through a point A and let it perpendicular to the vector \(\overrightarrow{\mathrm{N}}\)
∴ Equation of the plane
(\(\vec{r}\) – \(\vec{a}\)).\(\overrightarrow{\mathrm{N}}\) = 0.

(b) If a plane passes through (x₁, y₁, z₁) and perpendicular to the line with direction ratios a, b, c the equation of the plane is
a(x – x₁) + b(y – y₁)+c(z – z₁) =0

3. Equation of the plane passing through three points.
(a) Let the three points be A, B and C whose position vectors be, \(\vec{a}\), \(\vec{b}\) and \(\vec{c}\)
The equation of the plane is
\((\vec{r}-\vec{a}) \cdot[(\vec{b}-\vec{a}) \times(\vec{c}-\vec{a})]\) = 0

(b) Let the three points through which the plane is passing be A(x₁, y₁, z₁), B(x₂, y₂, z₂), and C(x₃, y₃, z₃).
Then, equation of the plane is
\(\left|\begin{array}{llll}
x & y & z & 1 \\
x_{1} & y_{1} & z_{1} & 1 \\
x_{2} & y_{2} & z_{2} & 1 \\
x_{3} & y_{3} & z_{3} & 1
\end{array}\right|\) = 0
or
\(\left|\begin{array}{ccc}
x-x_{1} & y-y_{1} & z-z_{1} \\
x_{2}-x_{1} & y_{2}-y_{1} & z_{2}-z_{1} \\
x_{3}-x_{1} & y_{3}-y_{1} & z_{3}-z_{1}
\end{array}\right|\) = 0

4. Intercepts form of the equation of the plane
Let the plane make the intercepts a, b, and c on coordinate axes OX, OY, and OZ respectively.

Then, the equation of the plane is
\(\frac{x}{a}+\frac{y}{b}+\frac{z}{c}\) = 1

9. Plane passing through the intersection of the two planes:
Let the equation of two planes be
\(\vec{r}\). \(\vec{n}_{1}\) = d1 and
\(\vec{r}\) .\(\vec{n}_{2}\) = d2

The equation of the plane passing through the line of intersection of the given planes is
(\(\vec{r}\). \(\vec{n}_{1}\) – d1) + λ(\(\vec{r}\) .\(\vec{n}_{2}\) – d2) = 0
or
\(\vec{r}\) .(\(\vec{n}_{1}\) + λ\(\vec{n}_{2}\)) = (d1 + λd2)

(b) Equation of the plane passing through the line of intersection of the planes
a₁x + b₁y + c₁z + d₁ = 0 and a₂x + b₂y + c₂z + d₂ = 0 is (a₁x + b₁ y + c₁z + d₁ + λ(a₂x + b₂y + c₂z + d₂) = 0
or
(a₁ + λa₂)x + (b₁ + λb₂)y + (c₁ + λc₂)z + d₁ + λd₂ = 0,
where λ is determined according to the given condition.

10. Coplanarity of two lines:

(a) The two lines
\(\vec{r}\) = \(\vec{a}_{1}\) + λ\(\vec{b}_{1}\) and
\(\vec{r}\) = \(\vec{a}_{2}\) + λ\(\vec{b}_{2}\) intersect each other, if \(\left(\vec{a}_{2}-\vec{a}_{1}\right) \cdot\left(\vec{b}_{2} \times \vec{b}_{1}\right)\) = 0

11. The angle between the planes:
Definition : The angle between the two planes is the angle between their normals. The angle 0 between the planes
\(\vec{r}\) = \(\vec{n}_{1}\) = d1 and \(\vec{r}\) = \(\vec{n}_{1}\) = d2 is given by cos θ = \(\frac{\left|\vec{n}_{1} \cdot \vec{n}_{2}\right|}{\left|\vec{n}_{1}\right|\left|\vec{n}_{2}\right|}\)

(b) If the planes are
a₁x + b₁y + C₁z + d₁ = 0
a₂x + b₂y + c₂z + d₂ = 0, then the angle θ between these planes is given by
cos θ = \(\frac{a_{1} a_{2}+b_{1} b_{2}+c_{1} c_{2}}{\sqrt{a_{1}^{2}+b_{1}^{2}+c_{1}^{2}} \sqrt{a_{2}^{2}+b_{2}^{2}+c_{2}^{2}}}\)

(c) The planes are perpendicular to each other, if θ = \(\frac{π}{2}\)
∴ cos \(\frac{π}{2}\) = 0 ⇒ \(\vec{n}_{1}\).\(\vec{n}_{2}\) = 0
or
a₁a₂ + b₁b₂ + c₁c₂ = 0

(d) The planes a₁x + b₁y +c₁z + d₁ = 0
and a₂x + b₂y + c₂z + d₂ = O
are parallel, if \(\frac{a_{1}}{a_{2}}=\frac{b_{1}}{b_{2}}=\frac{c_{1}}{c_{2}}\).

(e) Equation of the plane parallel to
\(\vec{r} \cdot \vec{n}\) = d is \(\vec{r} \cdot \vec{n}\) = λ
Plane parallel to
ax + by + cz + d = 0 is ax + by + cz + λ = 0.

12. Distance of a point from th plane:
(a) Let the plane be \(\vec{r} \cdot \vec{n}\) = d.
∴ Perpendicular distance of the point \(\vec{a}\) from the plane
=|d – \(\vec{a} \cdot \hat{n}\)|

(b) Let the equation of the plane
be Ax + By + Cz + D= O.
The distance of the point (x1, y1, z1) from this plane
= \(\left|\frac{A x_{1}+B y_{1}+C z_{1}+D}{\sqrt{A^{2}+B^{2}+C^{2}}}\right|\)

13. Angle between a line and a plane:
Definition: The angle between a line and plane is said to be the complement of the angle between the line and the normal to the plane.

(a) Let the line and plane be
\(\vec{r}\) = \(\vec{a}\) + λ\(\vec{b}\) and \(\vec{r} \cdot \vec{n}\) = d.

If θ be the angle between the plane and the line, then
sin θ = \(\frac{\vec{b} \cdot \vec{n}}{|\vec{b}||\vec{n}|}\)

(b) ¡et the line and the plane be \(\frac{x-x_{1}}{a}=\frac{y-y_{1}}{b}=\frac{z-z_{1}}{c}\) and Ax + By + Cz + D = O.
The angle Φ between them is given by
sin Φ = \(\frac{a \cdot \mathrm{A}+b \cdot \mathrm{B}+c \cdot \mathrm{C}}{\sqrt{a^{2}+b^{2}+c^{2}} \sqrt{\mathrm{A}^{2}+\mathrm{B}^{2}+\mathrm{C}^{2}}}\).

1. IMPORTANT RESULT

l² + m² + n² = 1, where < l, m, n > are direction-cosines of a st. line.

2. DIRECTION-RATIOS

The direction-ratios of the line joining of the points (x₁, y₁, z₁) and (x₂, y₂, z₂) are :
<x₂-x₁, y₂-y₁ ,z₂-z₁ >.

3. (i) Angle between two lines. The angle between two lines having direction-cosines
< l₁ m₁ n₁ > and < l₂, m₂, n₂ > is given by :
cos θ = |l₁l₂+ m₁m₂ + n₁n₂|.

(ii) The lines are:
(a) perpendicular l₁l₂+ m₁m₂ + n₁n₂ = 0.
(b) parallel iff l₁ = l_2, m₁ = m₂, + n₁ = n₂ 

4. SHORTEST DISTANCE

The shortest distance between two lines :
\(\vec{r}=\overrightarrow{a_{1}}+\lambda \overrightarrow{b_{1}} \text { and } \vec{r}=\overrightarrow{a_{2}}+\mu \overrightarrow{b_{2}} \text { is }\left|\frac{\left(\overrightarrow{b_{1}} \times \overrightarrow{b_{2}}\right) \cdot\left(\overrightarrow{a_{2}}-\overrightarrow{a_{1}}\right)}{\left|\overrightarrow{b_{1}} \times \overrightarrow{b_{2}}\right|}\right|\)

5. EQUATIONS OF PLANES

(i) Equation of a plane, which is at a distance ‘p’ from the origin and perpendicular to the unit
vector \(\hat{n}\) is \(\vec{r} \cdot \hat{n}=p\)

(ii) General Form. The general equation of first degree i.e.ax + by + cz + d- 0 represents a plane,

(iii) One-point Form. The equation of a plane through (*,, y,, z,) and having <a,b,c> as direction-
ratios of the normal is a (x – x₁) + b (y – y₁) + c (z – z₁) = 0.

(iv) Three-point Form. The equation of the plane through (x₁, y₁, z₁), (x₂, y₂, z₂) and (x₃, y₃, z₃) is:
\(\left|\begin{array}{ccc}
x-x_{1} & y-y_{1} & z-z_{1} \\
x-x_{2} & y-y_{2} & z-z_{2} \\
x-x_{3} & y-y_{3} & z-z_{3}
\end{array}\right|=0\)

(v) Intercept Form. Equation of the plane, which cuts off intercepts a, b, c on the axes, is :
\(\frac{x}{a}+\frac{y}{b}+\frac{z}{c}\) = 1

6. ANGLE BETWEEN TW O PLANES
The angle between the planes :
a₁x + b₁y + c₁z + d₁ = 0 and a₂x + b₂y + c₂z + d₂ = 0 is given by :
cos θ = \(\frac{\left|a_{1} a_{2}+b_{1} b_{2}+c_{1} c_{2}\right|}{\sqrt{a_{1}^{2}+b_{1}^{2}+c_{1}^{2}} \sqrt{a_{2}^{2}+b_{2}^{2}+c_{2}^{2}}}\)

7. BISECTING PLANES
The equations of the planes bisecting the planes :
a₁x + b₁y + c₁z + d₁ = 0 and a₂x + b₂y + c₂z + d₂ = 0 are:
\(\frac{a_{1} x+b_{1} y+c_{1} z+d_{1}}{\sqrt{a_{1}^{2}+b_{1}^{2}+c_{1}^{2}}}=\pm \frac{a_{2} x+b_{2} y+c_{2} z+d_{2}}{\sqrt{a_{2}^{2}+b_{2}^{2}+c_{2}^{2}}}\)

By going through these CBSE Class 12 Maths Notes Chapter 10 Vector Algebra, students can recall all the concepts quickly.

Vector Algebra Notes Class 12 Maths Chapter 10

Some Basic Concepts:
1. Vector (Definition): A quantity that has magnitude, as well as direction, is called a vector.
\(\overrightarrow{\mathrm{AB}}\) is a directed line segment.

It is a vector \(\overrightarrow{\mathrm{AB}}\) and its direction is from A to B.
→ Initial Points: The point A wherefrom the vector \(\overrightarrow{\mathrm{AB}}\) starts is known as the initial point.

→ Terminal point: Point B, where it ends is said to be the terminal point.

→ Magnitude: The distance between initial point and terminal point of a vector is the magnitude (or length) of the vector \(\overrightarrow{\mathrm{AB}}\) . It is denoted by |\(\overrightarrow{\mathrm{AB}}\)| or |\(\overrightarrow{\mathrm{a}}\)| or simply as a.

2. Position Vector: Consider a point F(x, y, z) in space. The vector \(\overrightarrow{\mathrm{OP}}\) with an initial point as origin O and terminal point P, is called the position vector of P. It may be denoted by \(\overrightarrow{\mathrm{p}}\).

3. Direction Cosines: Let OX, OY, OZ be the coordinates axes and F(x, y, z) be any point in the space. Let OP makes angles α, β, γ with coordinates axes OX, OY, OZ respectively.

The angles α, β, γ are known as direction angles. The cosine of these angles, i.e., cos α, cos β, cos γ is called direction cosines of line OP. These direction cosines are denoted by Z, m, n, i.e., Z = cos α, m = cos β, and n = cos γ.

4. Relation between l, m, n, and Direction Ratios: The perpendiculars PA, PB, PC are drawn on coordinate axes OX, OY, OZ respectively. Let OP = r,
In ΔOAP, ∠A = 90°, cos α = \(\frac{x}{r}\) = l ∴ x = Ir
In ΔABP, ∠B = 90°, cos β = \(\frac{y}{r}\) = m ∴ y = mr
In ΔOCP, ∠C = 90°, cos γ = \(\frac{z}{r}\) = n ∴ z = nr

Thus, the coordinates of P may be expressed as (lr, mr, nr).
Also, OP² = x² + y² + z² = r² = (lr)² + (mr)² + (nr)²
⇒ l² + m² + n² = 1.
When l, m, n are multiplied by a scalar k, we get the direction ratios, i.e., lk, mk, nk are the direction ratios of OA.

Types of vectors:
1. Zero Vector or Null Vector: A vector whose initial and terminal points coincide is known as zero vector (\(\overrightarrow{0}\)).

2. Unit Vector: A vector whose magnitude is unity is said to be a unit vector. It is denoted as â, so that | â | = 1.

3. Co-initial Vectors: Two or more vectors having the same initial point are called co-initial vectors.

4. Collinear Vectors: If two or more vectors are parallel to the same line, such vectors are known as collinear vectors.

5. Equal Vectors: If two vectors \(\vec{a}\) and \(\vec{b}\) have the same magnitude and direction, regardless of the positions of their initial points, such vectors are said to be equal, i.e., \(\vec{a}\) = \(\vec{b}\) .

6. Negative of a Vector: A vector whose magnitude is the same as that of a given vector (say \(\overrightarrow{\mathrm{AB}}\)), but the direction is opposite to that of it, is known as negative of vector \(\overrightarrow{\mathrm{AB}}\). i.e., \(\overrightarrow{\mathrm{BA}}\) = – \(\overrightarrow{\mathrm{AB}}\).

6. Sum of Vectors:
1. Sum of Vectors \(\vec{a}\) and \(\vec{b}\):
Let the vectors \(\vec{a}\) and \(\vec{b}\) be so positioned that initial point of one coincides with terminal point of the other i.e., let \(\vec{a}\) = \(\overrightarrow{\mathrm{AB}}\) and \(\vec{b}\) = \(\overrightarrow{\mathrm{BA}}\) .

Then, the vector \(\vec{a}+\vec{b}\) is represented by the third side of ΔABC
i. e.,
\(\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}=\overline{\mathrm{AC}}\) ……..(1)
or
\(\overrightarrow{\mathrm{AC}}+\overrightarrow{\mathrm{AB}}=\overline{\mathrm{BC}}\)
and
\(\overrightarrow{\mathrm{AC}}+\overrightarrow{\mathrm{BC}}=\overline{\mathrm{AB}}\)

This is known as the triangle law of addition.
Further, \(\overrightarrow{\mathrm{AC}}\) = – \(\overrightarrow{\mathrm{CA}}\)
So, (1) may be written as
\(\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}\) = – \(\overrightarrow{\mathrm{CA}}\)

∴ \(\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}+\overrightarrow{\mathrm{CA}}\) = \(\overrightarrow{0}\)

When sides of a triangle ABC are taken in order, i.e., initial and terminal points coincides, then
\(\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}+\overrightarrow{\mathrm{CA}}\) = \(\overrightarrow{0}\)

2. Parallelogram law of Addition:
If the two vectors \(\vec{a}\) and \(\vec{b}\) are represented by the two adjacent sides OA and OB of a parallelogram OACB, then their sum \(\vec{a}\) + \(\vec{b}\) is represented in magnitude and direction by the diagonal OC of the parallelogram through their common point O.

i.e., \(\overline{\mathrm{OA}}+\overrightarrow{\mathrm{OB}}=\overrightarrow{\mathrm{OC}}\)

Properties of Vector Addition:
Property 1: For two vectors \(\vec{a}\) and \(\vec{b}\), the sum is commutative i.e.,
\(\vec{a}+\vec{b}\) = \(\vec{b}+\vec{a}\)

Property 2: For three vectors \(\vec{a}\), \(\vec{b}\) and \(\vec{c}\) the sum of vectors is associative,- i.e.,
\((\vec{a}+\vec{b})+\vec{c}\) =\(\vec{a}+(\vec{b}+\vec{c})\)

7. Multiplication of a vector by a scalar:
Let \(\vec{a}\) be the given vector and λ be a scalar.
Product of λ and \(\vec{a}\) = λ\(\vec{a}\).
(i) When λ is +ve, \(\vec{a}\) and λ \(\vec{a}\) have the same sense of direction.
(ii) When λ is -ve, \(\vec{a}\) and λ\(\vec{a}\) are of the opposite directions.
Also, |λ\(\vec{a}\)| = |λ| |\(\vec{a}\)|

→ Additive Inverse of Vector \(\vec{a}\)
If there exists a vector – \(\vec{a}\) such that
\(\vec{a}\) + (- \(\vec{a}\)) = \(\vec{a}\) – \(\vec{a}\) = \(\vec{0}\), then – \(\vec{a}\) is called the additive inverse of \(\vec{a}\).

→ Unit Vector \(\vec{a}\)
Let λ = \(\frac{1}{|\vec{a}|}\) . So, | λ\(\vec{a}\)| = |λ| |\(\vec{a}\)|
= \(\frac{1}{|\vec{a}|}\) × |\(\vec{a}\)| = 1
∴ \(\frac{1}{|\vec{a}|}\) × |\(\vec{a}\)| = a, where |\(\vec{a}\)| ≠ 0 and \(\vec{a}\) is the unit vector.

8. Components of Vector:
Let us take the points A(1, 0, 0), B(0,1, 0) and 0(0, 0,1) on the co-ordinate axes OX, OY and OZ respectively.
Obviously |\(\overrightarrow{\mathrm{OA}}\)| = 1, |\(\overrightarrow{\mathrm{OB}}\)| = 1 and |\(\overrightarrow{\mathrm{OC}}\)| = 1.

Vectors \(\overrightarrow{\mathrm{OA}}\), \(\overrightarrow{\mathrm{OB}}\) and \(\overrightarrow{\mathrm{OC}}\) each having magnitude 1 are known as unit vectors. They are denoted by î, ĵ and k̂ respectively.

Consider the vector \(\overrightarrow{\mathrm{OP}}\), where P is the point (x, y, z). Now, OQ, OR, OS are the projections of OP on co-ordinates axes.

∴ OQ = x, OR = y, OS = z
\(\overrightarrow{\mathrm{OQ}}\) = xî, \(\overrightarrow{\mathrm{OR}}\) =yĵ, \(\overrightarrow{\mathrm{OS}}\) = zk̂.
⇒ \(\overrightarrow{\mathrm{OP}}\) – xî + yĵ +zk̂.
Also, |\(\overrightarrow{\mathrm{OP}}\)| = \(\sqrt{x^{2}+y^{2}+z^{2}}\) = |\(\vec{r}\)|

x, y, z are called the scalar components and xî, yĵ, and zk̂ are the vector components of vector \(\overrightarrow{\mathrm{OP}}\)

Some properties:
Let \(\vec{a}\) =a₁î + a₂ĵ + a₃k̂ and \(\vec{b}\) =b₁î +b₂ĵ +b₃k̂
1. \(\vec{a}\) + \(\vec{b}\) =(a₁ î + a₂ĵ + a₃k̂) + (b₁ î +b₂ĵ +b₃k̂)
⇒ (a₁ + b₁)î + (a₂ + b₂)ĵ + (a₃ + b₃)k̂

2. \(\vec{a}\) – \(\vec{b}\) or (a₁î + a₂ĵ +a₃k̂) = (b₁î + b₂ĵ + b₃k̂)

3. λ\(\vec{a}\) = λ(a₁î + a₂ĵ + a₃k̂)
⇒ (λa₁)î + (λa₂)ĵ+ (λa₃)k̂

4. \(\vec{a}\) and \(\vec{b}\) are collinear, if and only if there exists a non-zero scalar X such that \(\vec{b}\) = λ\(\vec{a}\), i.e.,

9. Vector joining two points:
Let P₁(x₁, y₁, z₁) and P₂(x₂, y₂, z₂) be the two points. Then, the vector joining the points P₁ and P₂ is \(\overline{\mathrm{P}_{1} \mathrm{P}_{2}}\)
Join P₁ and P₂ with O.

Now,

10. Dividing a line segment in a given ratio:
1. A line segment PQ is divided by a point R in the ratio m: n internally.

i.e., \(\frac{\mathrm{PR}}{\mathrm{RQ}}=\frac{m}{n}\)
If \(\vec{a}\) and \(\vec{b}\) are the position vectors of P and Q then the position vector \(\vec{r}\) of R is given by
\(\vec{r}\) = \(\frac{m \vec{b}+n \vec{a}}{m+n}\)

2. When R divides PQ externally,
\(\frac{\mathrm{PR}}{\mathrm{RQ}}=\frac{m}{n}\)

Replacing n by -n,
\(\vec{r}\) = \(\frac{m \vec{b}-n \vec{a}}{m-n}\)

Product of two vectors (Dot Product)
1. Scalar (or Dot) Product:
Scalar product of two vectors \(\vec{a}\) and \(\vec{b}\) is defined as \(\vec{a}\) . \(\vec{b}\) = | \(\vec{a}\) | \(\vec{b}\) | cos 0, where 0 is the angle between a and b (0,< θ < π).

2. From definition we derive:
(a) \(\vec{a}\).\(\vec{b}\) is a scalar quantity.

(b) When θ = 0, \(\vec{a}\).\(\vec{b}\) = |\(\vec{a}\)| |\(\vec{a}\).\(\vec{b}\)|.
Also, \(\vec{a}\). \(\vec{a}\) = |\(\vec{a}\)|2 = |\(\vec{a}\)|.

(c) When θ = \(\frac{π}{2}\)
⇒ When \(\vec{a}\) ⊥ \(\vec{a}\), \(\vec{a}\). \(\vec{b}\) = 0.

(d) When either \(\vec{a}\) = 0 or \(\vec{b}\) = 0, \(\vec{a}\). \(\vec{b}\) = 0.

(e) cos θ = \(\frac{\vec{a} \cdot \vec{b}}{|\vec{a}||\vec{b}|}\)

3. Properties of scalar Product
(a) Scalar product is commutative, i.e.,
\(\vec{a}\) . \(\vec{b}\) = \(\vec{b}\).\(\vec{a}\)

(b) If a is scalar, then
(α \(\vec{a}\) ). \(\vec{b}\) = α(\(\vec{a}\).\(\vec{b}\)) – \(\vec{a}\). (α\(\vec{b}\))

11. Projection of vector along a directed line:
Let the vector \(\overrightarrow{\mathrm{AB}}\) makes ar angle θ with directed line l.
Projection of AB on l = |\(\overrightarrow{\mathrm{AB}}\)| cos θ = \(\overrightarrow{\mathrm{AC}}\) = \(\overrightarrow{\mathrm{P}}\).

The vector is \(\overrightarrow{\mathrm{P}}\) is called the projection vector. Its magnitude is
|\(\overrightarrow{\mathrm{P}}\)|, which is known as projection of vector \(\overrightarrow{\mathrm{AB}}\).

The angle θ between \(\overrightarrow{\mathrm{AB}}\) and \(\overrightarrow{\mathrm{AC}}\) is given by
cos θ = \(\frac{\overrightarrow{\mathrm{AB}} \cdot \overrightarrow{\mathrm{AC}}}{|\overline{\mathrm{AB}}||\overline{\mathrm{AC}}|}\)

Now, projection AC = \(\overrightarrow{\mathrm{AB}}\) cos θ = cos θ \(\frac{\overline{\mathrm{AB}} \cdot \overrightarrow{\mathrm{AC}}}{|\mathrm{AC}|}\)
= \(\overrightarrow{\mathrm{AB}}\).(\(\frac{\overrightarrow{\mathrm{AC}}}{|\overrightarrow{\mathrm{AC}}|}\))

If, \(\overrightarrow{\mathrm{AB}}\) = \(\vec{a}\) and \(\overrightarrow{\mathrm{AC}}\) = \(\vec{p}\), then
AC = \(\vec{a}\)(\(\frac{\vec{p}}{|\vec{p}|}\)) = \(\vec{a}\).\(\vec{p}\)

Thus, the projection of \(\vec{a}\) on \(\vec{b}\)
= \(\vec{a}\)(\(\frac{\vec{b}}{|\vec{b}|}\)) = \(\vec{a}\).\(\vec{b}\).

Note: If α, β, γ are the direction angles of the vector
\(\vec{a}\) = (a₁î + a₂ĵ + a₃k̂), the direction cosines of a are given as

cos α = \(\frac{\vec{a} \cdot \hat{i}}{|\vec{a}||\hat{i}|}=\frac{a_{1}}{|\vec{a}|}\)
cos β = \(\frac{a_{2}}{|\vec{a}|}\)
cos γ = \(\frac{a_{3}}{|\vec{a}|}\)

12. Vector Product of two vectors:
1. Definition: The vector product of two non-zero vectors \(\vec{a}\) and \(\vec{b}\), denoted by \(\vec{a}\) × \(\vec{b}\) is defined as
\(\vec{a}\) × \(\vec{b}\) = |\(\vec{a}\) ||\(\vec{b}\) |sin θ.n̂
where θ is the angle between \(\vec{a}\) and \(\vec{b}\), 0 ≤ θ ≤ π. Unit vector n̂ is perpendicular to both vectors \(\vec{a}\) and \(\vec{b}\), such that \(\vec{a}\), \(\vec{b}\) and n̂ form a right handed system.

2. Note:
(a) \(\vec{a}\) × \(\vec{b}\) = | \(\vec{a}\) | | \(\vec{b}\) | sin θ. n̂

1. If \(\vec{a}\) = 0 or \(\vec{b}\) = 0, \(\vec{a}\) × \(\vec{a}\) = \(\vec{0}\)
2. \(\vec{a}\) || \(\vec{b}\), \(\vec{a}\) × \(\vec{b}\) = 0

(b) \(\vec{a}\) × \(\vec{b}\) = – \(\vec{b}\) × \(\vec{a}\)
\(\vec{a}\) × \(\vec{b}\) ≠ \(\vec{b}\) × \(\vec{a}\)
⇒ vector product is not commutative.

(c) When θ = \(\frac{π}{2}\), \(\vec{a}\) × \(\vec{b}\) = |\(\vec{a}\)| × |\(\vec{b}\)| × n̂
or
| \(\vec{a}\) × \(\vec{b}\) | = |\(\vec{a}\)||\(\vec{b}\)|

(d) î × î = ĵ × ĵ = k̂ × k̂ = 0
and î × ĵ = k̂, ĵ × k̂ = ĵ, k̂ × î = ĵ.
⇒ĵ × î = – k̂, k̂ × ĵ = -î , î × k̂ = – ĵ.

(e) sin θ = \(\frac{|\vec{a} \times \vec{b}|}{|\vec{a}||\vec{b}|}\)

(f) If \(\vec{a}\) and \(\vec{b}\) represent adjacent sides of a parallelogram, then its area |\(\vec{a}\) × \(\vec{b}\)|.

(g) If \(\vec{a}\) and \(\vec{b}\) represent adjacent sides of a triangle, then its area = \(\frac{1}{2}\) |\(\vec{a}\) × \(\vec{b}\)|

(h) Distributive Property
\(\vec{a}\) × (\(\vec{b}\) + \(\vec{c}\)) = \(\vec{a}\) × \(\vec{b}\) + \(\vec{b}\) x× \(\vec{c}\)
1. Let α be a scalar, then
α(\(\vec{a}\) × \(\vec{b}\)) = (α\(\vec{a}\)) × \(\vec{b}\) = \(\vec{a}\) × (α\(\vec{b}\))

2. \(\vec{a}\) = (a₁ î + a₂ ĵ + a₃ k̂) and
\(\vec{b}\) = (b₁ î + b₂ ĵ + b₃ k̂), then

\(\vec{a}\) × \(\vec{a}\) = \(\left|\begin{array}{lll}
\hat{i} & \hat{j} & \hat{k} \\
a_{1} & a_{2} & a_{3} \\
b_{1} & b_{2} & b_{3}
\end{array}\right|\)

1. PROPERTIES OF \TXHORS UNDER ADDITION

(i) Commutative Law. \(\vec{a}+\vec{b}=\vec{b}+\vec{a}\)
(ii) Associative Law, \(\vec{a}+(\vec{b}+\vec{c})=(\vec{a}+\vec{b})+\vec{c}\)
(iii) Additive Identity. \(\vec{a}+\overrightarrow{0}=\vec{a}=\overrightarrow{0}+\vec{a}\)
(iv) Additive Inverse. \(\vec{a}+(-\vec{a})=\overrightarrow{0}=(-\vec{a})+\vec{a}\)

2. \(\vec{AB}\) = (POSITION VECTOR OF B)-(POSITION VECTOR OF A).

3. SCALAR PRODUCT

(i) Def. The scalar product of \(\vec{a}\) and \(\vec{b}\) is defined as \(\vec{a} \cdot \vec{b}=|\vec{a}||\vec{b}| \cos \theta\), where ‘θ’ is the angle between \(\vec{a}\) and \(\vec{b}\)
(ii) Condition of Perpendicularity. If \(\vec{a}\) and \(\vec{b}\) are perpendicular, then \(\vec{a} \cdot \vec{b}\) = 0.
(iii) If \(\hat{i}, \hat{j}, \hat{k}\) are unit vectors, which are perpendicular to each other, then :
\(\hat{i} \cdot \hat{j}=\hat{j} \cdot \hat{k}=\hat{k} \cdot \hat{i}=0\) and \(\hat{i}^{2}=\hat{j}^{2}=\hat{k^{2}}\)

(iv) Properties:
(a) CommutativeLaw. \(\vec{a}\cdot \vec{b}=\vec{b} \cdot \vec{a}\)

(b) Associative Law, does not hold.

(c) Distributive Laws, \(\vec{a} \cdot(\vec{b}+\vec{c})=\vec{a} \cdot \vec{b}+\vec{a} \cdot \vec{c}\) and \((\vec{b}+\vec{c}) =\vec{b} \cdot \vec{a}+\vec{c} \cdot \vec{a}\)

(d) \((m\vec{a}) \cdot \vec{b}=m(\vec{a} \cdot \vec{b})=\vec{a} \cdot(m \vec{b})\), m being a scalar.

(e) \(\vec{a} \cdot \vec{a}=a^{2}\)

4. VECTOR PRODUCT

(i) Definition. The vector product of \(\vec{a}\) and \(\vec{b}\) is given by: \({a} \times \vec{b}=|\vec{a} \| \vec{b}| \sin \theta \hat{n}\) , where ‘θ’ the angle between \(\vec{a}\) and \(\vec{b}\) and \(\hat{n}\) is a unit vector perpendicular to the plane of \(\vec{a}\) and \(\vec{b}\) .

(ii) When two vectors are parallel, then \(\vec{a} \times \vec{b}=\overrightarrow{0}\)

(iii) If \(\hat{i}, \hat{j}, \hat{k}\) are unit vectors, which are perpendicular to each other, then :
\(\hat{i} \times \hat{j}=\hat{k}=-\hat{j} \times \hat{i} ; \text { etc. } \hat{i} \times \hat{i}=\hat{j} \times \hat{j}=\hat{k} \times \hat{k}=\overrightarrow{0}\)

(iv) Properties :

(a) Commutative Law does not hold.

(b) \((m \vec{a}) \times \vec{b}=m(\vec{a} \times \vec{b})=\vec{a} \times(m b)\), m being a scalar.

(c) If \(\vec{a}=a_{1} \hat{i}+a_{2} \hat{j}+a_{3} \hat{k}, \vec{b}=b_{1} \hat{i}+b_{2} \hat{j}+b_{3} \hat{k}\), then \(\vec{a} \times \vec{b}\) = \(\left|\begin{array}{ccc}
\hat{i} & \hat{j} & \hat{k} \\
a_{1} & a_{2} & a_{3} \\
b_{1} & b_{2} & b_{3}
\end{array}\right|\)

(d) Distributive Law. \(\vec{a} \times(\vec{b}+\vec{c})=\vec{a} \times \vec{b}+\vec{a} \times \vec{c}\)

5. SCALAR TRIPLE PRODUCT

(i) Def. If \(\vec{a}, \vec{b}, \vec{c}\) are three vectors, then the scalar product of \(\vec{a} \times \vec{b}\) with \(\vec{c}\) is called scalar triple product

(ii) Properties:
(a) Condition for three vectors to be coplanar. If \(\vec{a}, \vec{b}, \vec{c}\) are all coplanar vectors, then \(\left[\begin{array}{lll}
\rightarrow & \rightarrow & \rightarrow \\
a & b & c
\end{array}\right]\) = 0

(b) If any two of the three vectors \(\vec{a}, \vec{b}, \vec{c}\) are equal, then \([\vec{a} \vec{b} \vec{c}]\) = 0

(c) If any two of the three vectors \(\vec{a}, \vec{b}, \vec{c}\) are parallel, then \(\left[\begin{array}{ccc}
\rightarrow & \rightarrow & \rightarrow \\
a & b & c
\end{array}\right]\) = 0

(d) In the scalar triple product, the position of dot and cross can be interchanged at pleasure provided the cyclic order of the vectors is maintained.

(e) In the scalar triple product, the change in the cyclic order of die vectors changes the sign of the product.

By going through these CBSE Class 9 Sanskrit Notes Chapter 3 गोदोहनम् Summary, Notes, word meanings, translation in Hindi, students can recall all the concepts quickly.

Class 9 Sanskrit Chapter 3 गोदोहनम् Summary Notes

गोदोहनम् Summary

यह नाटयांश कृष्णचंद्र त्रिपाठी महोदय द्वारा रचित ‘चतुर्म्यहम्’ पुस्तक से सम्पादित करके लिया गया है। इस नाटक में ऐसे व्यक्ति की कथा है जो धनी और सुखी होने की इच्छा से प्रेरित होकर एक महीने के लिए गाय का दूध निकालना बंद कर देता है ताकि गाय के शरीर में इकट्ठा पर्याप्त दूध एक ही बार में बेचकर सम्पत्ति कमाने में समर्थ हो सके।

परन्तु मास के अन्त में जब वह दूध दुहने का प्रयत्न करता है तब वह दूध की बूंद भी प्राप्त नहीं कर पाता है। दूध प्राप्त करने के स्थान पर वह गाय के प्रहारों द्वारा खून से लथपथ हो जाता है और जान जाता है कि प्रतिदिन का कार्य यदि मास भर के लिए इकट्ठा किया जाता है तब लाभ के स्थान पर हानि ही होती है।

गोदोहनम् Word Meanings Translation in Hindi

1. (प्रथमम् दृश्यम्)

(मल्लिका मोदकानि रचयन्ती मन्दस्वरेण शिवस्तुतिं करोति)
(ततः प्रविशति मोदकगन्धम् अनुभवन् प्रसन्नमना चन्दनः।)

चन्दनः – अहा! सुगन्धस्तु मनोहरः ( विलोक्य ) अये मोदकानि रच्यन्ते? (प्रसन्नः भूत्वा) आस्वादयामि तावत्।
(मोदकं गृहीतुमिच्छति)
मल्लिका – (सक्रोधम् ) विरम। विरम। मा स्पृश! एतानि मोदकानि।
चन्दनः – किमर्थं क्रुध्यसि! तव हस्तनिर्मितानि मोदकानि दृष्ट्वा अहं जिह्वालोलुपतां नियन्त्रयितुम् अक्षमः
अस्मि, किं न जानासि त्वमिदम्?
मल्लिका – सम्यग् जानामि नाथ! परम् एतानि मोदकानि पूजानिमित्तानि सन्ति।
चन्दनः – तर्हि, शीघ्रमेव पूजनं सम्पादय। प्रसादं च देहि।

शब्दार्था:-
मोदकानि – लड्डुओं को
रचयन्ती – बनाती हुई
मन्दस्वरेण – धीमी आवाज़ से
मोदकगन्धम् – लड्डुओं की सुगन्ध को
प्रसन्नमना – प्रसन्न मन वाला
रच्यन्ते – बनाए जा रहे हैं
आस्वादयामि – स्वाद लेता हूँ
तावत् – तो/ तब तक
गृहीतुम् – लेने के लिए
सक्रोधम् – क्रोध के साथ
स्पृश – छुओ
हस्तनिर्मितानि – हाथों से बने हुए नियन्त्रयितुम् काबू करने में
अक्षमः – असमर्थ हूँ
पूजानिमित्तानि – पूजा के लिए
सम्पादय – करो।

(पहला दृश्य)

अर्थ –
(मल्लिका लड्डुओं को बनाती हुई धीमी आवाज़ में शिव की स्तुति करती है।)
(उसके बाद लड्डुओं की सुगन्ध को अनुभव करते हुए प्रसन्न मन से चन्दन प्रवेश करता है।)

चन्दन – वाह! सुगन्ध तो मनोहारी है (देखकर) अरे लड्डू बनाए जा रहे हैं? (प्रसन्न होकर) तो स्वाद लेता हूँ। (लड्डू को लेना चाहता है)
मल्लिका – (क्रोध के साथ) रुको। रुको। मत छुओ! इन लड्डुओं को।
चन्दन – क्यों गुस्सा करती हो! तुम्हारे हाथों से बने लड्डुओं को देखकर मैं जीभ की लालच पर काबू रखने में असमर्थ हूँ, क्या तुम यह नहीं जानती हो?
मल्लिका – अच्छी तरह से जानती हूँ स्वामी। परन्तु ये लड्डू पूजा के लिए हैं।
चन्दन – तो जल्दी ही पूजा करो और प्रसाद को दो।

सन्धिः विच्छेदः वा –
पदानि – सन्धिविच्छेदः
सुगन्धस्तु – सुगन्धः + तु
सम्यग् जानामि – सम्यक् + जानामि

प्रकृति प्रत्ययोः विभाजनम् –

पदानां वाक्यप्रयोगः –

पद परिचयः –

2. मल्लिका – भो! अत्र पूजनं न भविष्यति। अहं स्वसखिभिः सह श्वः प्रातः काशीविश्वनाथमन्दिरं प्रति गमिष्यामि, तत्र गङ्गास्नानं धर्मयात्राञ्च वयं करिष्यामः।
चन्दनः – सखिभिः सह! न मया सह! (विषादं नाटयति)
मल्लिका – आम्। चम्पा, गौरी, माया, मोहिनी, कपिलाद्याः सर्वाः गच्छन्ति। अतः, मया सह तवागमनस्य औचित्यं नास्ति। वयं सप्ताहान्ते प्रत्यागमिष्यामः। तावत्, गृह व्यवस्था, धेनोः दुग्धदोहनव्यवस्थाञ्च परिपालय।

शब्दार्थाः-
पूजनम् – पूजा को
स्वसखिभिः सह – अपनी सखियों के साथ
विषादम् – दु:ख को
नाटयति – अभिनय करता है
आगमनस्य – आने का
औचित्यम् – उचित कारण
प्रत्यागमिष्यामः – वापस आ जाएँगी
तावत् – तब तक
परिपालय – संभालो
दुग्धदोहनव्यवस्थाम् – दूध को दुहने की व्यवस्था को।

अर्थ
मल्लिका – अरे! यहाँ पूजा नहीं होगी। मैं अपनी सखियों के साथ कल सुबह काशीविश्वनाथ मंदिर की ओर जाऊँगी, वहाँ हम गंगा स्नान और धार्मिक यात्रा करेंगी।
चन्दन – सखियों के साथ! मेरे साथ नहीं! (दु:ख का अभिनय करता है)
मल्लिका – हाँ। चंपा, गौरी, माया, मोहिनी, कपिला आदि सब जाती हैं। अतः मेरे साथ तुम्हारे आने का कोई औचित्य (उचित रूप) नहीं है। हम सप्ताह के अन्त में (तक) वापस आ जाएँगी। तब तक घर की व्यवस्था और गाय के दूध को दुहने की व्यवस्था को चलाना।

सन्धिः विच्छेदः वा –
पदानि – सन्धिःविच्छेदःवा
धर्मयात्राञ्च – धर्मयात्राम् + च विषादं
नाटयति – विषादम् + नाटयति
कपिलाद्याः – कपिल + आद्याः
तवागमनस्य – तव + आगमनस्य
नास्ति – न + अस्ति
सप्ताहान्ते – सप्ताह + अन्ते
प्रत्यागमिष्यामः – प्रति + आगमिष्यामः
दुग्धदोहन व्यवस्थाञ्च – दुग्धदोहन व्यवस्थाम् + च

उपसर्ग विभाजनम्

कारकाः उपपदविभक्तयश्च

पदपरिचयः

3. (द्वितीयम् दृश्यम्)
चन्दनः – अस्तु। गच्छ। सखिभिः सह धर्मयात्रया आनन्दिता च भव। अहं सर्वमपि परिपालयिष्यामि। शिवास्ते सन्तु पन्थानः।
चन्दनः – मल्लिका तु धर्मयात्रायै गता। अस्तु। दुग्धदोहनं कृत्वा ततः स्वप्रातराशस्य प्रबन्धं करिष्यामि। (स्त्रीवेषं धृत्वा, दुग्धपात्रहस्तः नन्दिन्याः समीपं गच्छति)
उमा – मातुलानि! मातुलानि!
चन्दनः – उमे! अहं तु मातुलः। तव मातुलानी तु गङ्गास्नानार्थं काशीं गता अस्ति। कथय! किं ते प्रियं करवाणि?
उमा – मातुल! पितामहः कथयति, मासानन्तरम् अस्मत् गृहे महोत्सवः भविष्यति। तत्र त्रिशत-सेटक्रमितं दुग्धम् अपेक्षते। एषा व्यवस्था भवद्भिः करणीया।
चन्दनः – (प्रसन्नमनसा) त्रिशत-सेटककपरिमितं दुग्धम्! शोभनम्। दुग्धव्यवस्था भविष्यति एव इति पितामहं प्रति त्वया वक्तव्यम्।
उमा – धन्यवादः मातुल! याम्यधुना। (सा निर्गता)

शब्दार्था: –
अस्तु – ठीक है
धर्मयात्रया – धर्म की यात्रा से
परिपालयिष्यामि – संभाल लूँगा
शिवा: – कल्याणकारी
पन्थान: – मार्ग (रास्ते)
स्वप्रातराशस्य – अपने नाश्ते का
दुग्धपात्रहस्तः – हाथ में दूध के पात्र वाला
मातुलानि! – मामी!
गता – गई हुई
मासानन्तरम् – महीने बाद
अस्मत् – हमारे
अपेक्षते – चाहिए
भवद्भिः – आपके द्वारा
सेटक – लीटर (सेर)
वक्तव्यम् – कहना
यामि – जाती हूँ
निर्गता – निकल गई।

अर्थ – (दूसरा दृश्य)

चन्दन – ठीक है। जाओ। और सखियों के साथ धर्मयात्रा से आनन्दित होओ। मैं सभी को पाल लूँगा। तुम्हारे रास्ते शुभ हों।
चन्दन – मल्लिका तो धर्मयात्रा हेतु गई। ठीक है। गाय का दूध दुहकर उससे अपने नाश्ते (जलपान) का प्रबन्ध करूँगा। (स्त्री का वेश धारण करके, दूध के हाथ में लिए नन्दिनी गाय के पास जाता है)
उमा – मामी! मामी!
चन्दन – हे उमा! मैं तो मामा हूँ। तुम्हारी मामी तो गंगा स्नान के लिए काशी गई है। कहो! क्या तुम्हारा भला करूँ?
उमा – मामा! दादाजी कहते हैं, महीने भर बाद हमारे घर में उत्सव होगा। तब तीन सौ लीटर तक दूध चाहिए। यह व्यवस्था आपको ही करनी है।
चन्दन – (प्रसन्न मन से) तीस लीटर मात्रा (माप) में दूध! सुन्दर। दूध की व्यवस्था हो जाएगी ही ऐसा दादा जी को तुम कह दो।
उमा – धन्यवाद मामा! अब जाती हूँ। (वह चली गई) सन्धिः विच्छेदः वा

सन्धिःविच्छेदःवा –
शिवास्ते – शिवाः + ते
गङ्गा – गम् + गा
स्नानार्थम् – स्नान + अर्थम्
मासानन्तरम् – मास + अनन्तरम्
महोत्सवः – महा + उत्सवः
याम्यधुना – यामि + अधुना

प्रकृति – प्रत्यय-विभाजनम् –

विशेषण – विशेष्य – चयनम् –
विशेषणानि – विशेष्याः
शिवाः – पन्थानः
दुग्धपात्रहस्तः – चन्दनः
त्रिशत-सेटकमितम् – दुग्धम्
एषा – व्यवस्था

उपपदविभक्तयः कारकाश्च –

4. (तृतीयं दृश्यम्)

चन्दनः – (प्रसन्नो भूत्वा, अलिषु गणयन्) अहो! सेटक-त्रिशतकानि पयांसि! अनेन तु बहुधनं लप्स्ये।
(नन्दिनीं दृष्ट्वा) भो नन्दिनि! तव कृपया तु अहं धनिकः भविष्यामि। (प्रसन्नः सः धेनोः बहुसेवां करोति)
चन्दनः – (चिन्तयति) मासान्ते एव दुग्धस्य आवश्यकता भवति। यदि प्रतिदिनं दोहनं करोमि तर्हि दुग्धं
सुरक्षितं न तिष्ठति। इदानीं किं करवाणि? भवतु नाम मासान्ते एव सम्पूर्णतया दुग्धदोहनं करोमि।
(एवं क्रमेण सप्तदिनानि व्यतीतानि। सप्ताहान्ते मल्लिका प्रत्यागच्छति)
मल्लिका – (प्रविश्य) स्वामिन्! प्रत्यागता अहम्। आस्वादय प्रसादम्।
(चन्दनः मोदकानि खादति वदति च)
चन्दनः – मल्लिके! तव यात्रा तु सम्यक् सफला जाता? काशीविश्वनाथस्य कृपया प्रियं निवेदयामि।
मल्लिका – (साश्चर्यम् ) एवम्। धर्मयात्रातिरिक्तं प्रियतरं किम्?

शब्दार्था: –
अगुलिषु – अंगुलियों पर
गणयन् – गिनते हुए
लप्स्ये – प्राप्त करूँगा
दोहनम् – दुहना (दूध दुहने का काम)
तिष्ठति – रहता है
सम्पूर्णतया – पूरी तरह से
क्रमश: – क्रमानुसार (एक के बाद एक)
प्रत्यागच्छति – वापस आती है
प्रत्यगता – वापस आ गई
आस्वादय – स्वाद (आनंद) लो
प्रियतरम् – अधिक प्यारी।

अर्थ – (तृतीय दृश्य)

चन्दन – (प्रसन्न होकर, अंगुलियों पर गिनता हुआ) अरे! तीन सौ लीटर दूध। इससे तो बहुत धन प्राप्त करूँगा (पाऊँगा)।
(नन्दिनी को देखकर) हे नन्दिनी! तुम्हारी कृपा से तो मैं धनी हो जाऊँगा। (प्रसन्न होकर वह गाय की बहुत सेवा करता है)
चन्दन – (सोचता है) मास (महीने) के अन्त में ही दूध की जरूरत है। यदि हर रोज़ दुहने का काम करता हूँ
तो दूध सुरक्षित नहीं रहता है। अब क्या करूँ? ठीक है, महीने के अन्त में ही पूरी तरह से दूध को दुहने का काम करता हूँ (करूँगा)।
(इस प्रकार क्रमानुसार सात दिन बीत गए। सप्ताह के अन्त में मल्लिका वापस आती है)
मल्लिका – (प्रवेश करके) स्वामी! मैं वापस आ गई। प्रसाद को खाओ।
(चन्दन लड्डुओं को खाता है और बोलता है।)
चन्दन – मल्लिका! तुम्हारी यात्रा तो ठीक प्रकार से सफल हो गई? काशी विश्वनाथ की कृपा से प्रिय निवेदन करता हूँ।
मल्लिका – (आश्चर्य के साथ) ऐसा है! धर्मयात्रा के अतिरिक्त अधिक प्रिय क्या है?

सन्धिः विच्छेदः वा –
पदानि – सन्धिःविच्छेदः
प्रसन्नोभूत्वा  – प्रसन्नः + भूत्वा
मासान्ते – मास + अन्ते
सप्ताहान्ते – सप्ताह + अन्ते
प्रत्यागच्छति – प्रति + आगच्छति
प्रत्यागता – प्रति + आगता
साश्चर्यम् – स + आश्चर्यम्
धर्मयात्रातिरिक्तम् – धर्मयात्रा + अतिरिक्तम्

प्रकृति – प्रत्ययोः – विभाजनम्

विशेषण-विशेष्य-चयनम्

उपसर्ग – पदानाञ्च विभाजनम्

5. चन्दनः – ग्रामप्रमुखस्य गृहे महोत्सवः मासान्ते भविष्यति। तत्र त्रिशत-सेटकमितं दुग्धम् अस्माभिः दातव्यम् अस्ति ।
मल्लिका – किन्तु एतावन्मानं दुग्धं कुतः प्राप्स्यामः।
चन्दनः – विचारय मल्लिके! प्रतिदिनं दोहनं कृत्वा दुग्धं स्थापयामः चेत् तत् सुरक्षितं न तिष्ठति। अत एव
दुग्धदोहनं न क्रियते। उत्सवदिने एव समग्रं दुग्धं धोक्ष्यावः।
मल्लिका – स्वामिन्! त्वं तु चतुरतमः। अत्युत्तमः विचारः। अधुना दुग्धदोहनं विहाय केवलं नन्दिन्याः सेवाम् एव करिष्यावः। अनेन अधिकाधिकं दुग्धं मासान्ते प्राप्स्यावः। (द्वावेव धेनोः सेवायां निरतौ भवतः। अस्मिन् क्रमे घासादिकं गुडादिकं च भोजयतः। कदाचित् विषाणयोः तैलं लेपयतः तिलकं धारयतः, रात्रौ नीराजनेनापि तोषयतः)
चन्दनः – मल्लिके! आगच्छ। कुम्भकार प्रति चलावः।
दुग्धार्थं पात्रप्रबन्धोऽपि करणीयः। (द्वावेव निर्गतौ)

अर्थ
चन्दन – गाँव के प्रधान के घर में महीने के अन्त में महोत्सव होगा। वहाँ तीन सौ लीटर दूध हमें देना है।
मल्लिका – किन्तु इतनी मात्रा में दूध हमें कहाँ से मिलेगा?
चन्दन – सोचो मल्लिका! हर रोज दुह करके हम दूध को रखते हैं यदि वह सुरक्षित न रहे तो। इसलिए गाय का दोहन कार्य नहीं किया जा रहा है। उत्सव के दिन ही सारा दूध दुह लेंगे।
मल्लिका – हे स्वामी! तुम तो बहुत चतुर हो। बहुत सुन्दर विचार है। अब दूध दुहना छोड़कर केवल नन्दिनी की
सेवा ही करेंगे। इससे अधिक से अधिक दूध को महीने के अन्त में प्राप्त करेंगे। (दोनों ही गाय की सेवा में लगे होते हैं। इसी तरह वे घास व गुड़ आदि खिलाते हैं। कभी सीगों पर
तेल लगाते हैं, तिलक लगाते हैं, रात में जल आदि से भी सन्तुष्ट करते हैं।)
चन्दन – मल्लिका! आओ। कुंभार के घर की ओर चलते हैं। दूध के लिए बर्तन का प्रबन्ध भी करना है। (दोनों ही निकलते हैं) सन्धिः विच्छेदः वा

सन्धिः विच्छेदः वा –

प्रकृति – प्रत्ययोः – विभाजनम् –

विशेषण-विशेष्य-चयनम् –

उपपदविभक्तीनां प्रयोगः –

पद परिचयः –

6. (चतुर्थं दृश्यम्)

कुम्भकारः – (घटरचनायां लीनः गायति)

ज्ञात्वाऽपि जीविकाहेतोः रचयामि घटानहम्।
जीवनं भङ्गरं सर्वं यथैष मृत्तिकाघटः॥

शब्दार्थाः-
ज्ञात्वा – जानकर
जीविकाहेतोः – जीवन का कारण
मृत्तिकाघट: – मिट्टी का घड़ा
घटान् – घड़ों को
जीवनम् – जीवन
एषः – यह।

अर्थ – (चौथा दृश्य)

कुंभार – (घड़े बनाने में व्यस्त है और गाता है)
मैं जानकर भी जीविका (जीवन के लिए साधन) के कारण ही इन घड़ों को बनाता हूँ। सारा जीवन टूटकर नष्ट होने योग्य हैं जैसे यह मिट्टी का घड़ा टूटकर समाप्त होने योग्य है।

सन्धिः विच्छेदः वा –
पदानि – सन्धिःविच्छेदः
ज्ञात्वाऽपि – ज्ञात्वा + अपि
घटानहम् – घटान् + अहम्
भगुरम् – भम् + गुरम्
यथैष – यथा + एष

विशेषण – विशेष्य – चयनम्
विशेषणम् – विशेष्यः
सर्वम्, भगुरम् – जीवनम्
एष – मृत्तिकाघटः

7. चन्दनः – नमस्करोमि तात! पञ्चदश घटान् इच्छामि। किं दास्यसि?
देवेश – कथं न? विक्रयणाय एव एते। गृहाण घटान्। पञ्चशतोत्तर-रूप्यकाणि च देहि।
चन्दनः – साधु। परं मूल्यं तु दुग्धं विक्रीय एव दातुं शक्यते।
देवेशः – क्षम्यतां पुत्र! मूल्यं विना तु एकमपि घटं न दास्यामि।
मल्लिका – (स्वाभूषणं दातुमिच्छति) तात! यदि अधुनैव मूल्यम् आवश्यकं तर्हि, गृहाण एतत् आभूषणम्।
देवेशः – पुत्रिके! नाहं पापकर्म करोमि। कथमपि नेच्छामि त्वाम् आभूषणविहीनां कर्तुम्। नयतु यथाभिलषितान् घटान्। दुग्धं विक्रीय एव घटमूल्यम् ददातु।
उभौ – धन्योऽसि तात! धन्योऽसि।

शब्दार्था:-
पञ्चदश – पन्द्रह
दास्यसि – दोगे
विक्रयणाय – बेचने के लिए
विक्रीय – बेचकर, दातुम्
शक्यते – दिया जा सकता है
क्षम्यताम् – क्षमा करें
स्वाभूषणम् – अपने ज़ेवर को
अधुनैव – इसी समय ही
गृहाण – ले लो
पुत्रिके – बेटी,
आभूषणविहीनाम् – जेवरों से रहित
नयतु – ले जाओ
यथाभिलाषात् – इच्छानुसार
विक्रीय – बेचकर।

अर्थ
चन्दन – हे तात! नमस्कार करता हूँ। पन्द्रह घड़े चाहता हूँ। क्या दोगे? ।
देवेश – क्यों नहीं? बेचने के लिए ही हैं ये। घड़ों को ले लो। और पाँच सौ रुपये दे दो।
चन्दन – ठीक है। परन्तु मूल्य (दाम) तो दूध को बेचकर ही दिया जा सकता है।
देवेश – हे पुत्र क्षमा करो! बिना मूल्य (दान) के एक भी घड़ा नहीं दूंगा।
मल्लिका – (अपने आभूषणों को देना चाहती है) हे तात! यदि अभी कीमत देना आवश्यक (ज़रूरी) है तो, यह जेवर ले लो।
देवेश – बेटी! मैं पाप का काम नहीं करता हूँ। तुम्हें आभूषण विहीन कभी भी नहीं करना चाहता हूँ। इच्छानुसार
घड़ों को ले जाओ। दूध को बेचकर ही घड़ों की कीमत दे देना।
दोनों – तात! आप धन्य हो! धन्य हो।

सन्धिः विच्छेदः वा –
पदानि – सन्धिःविच्छेदः
नमस्करोमि – नमः + करोमि
पञ्चशतोत्तर – पञ्चशत + उत्तर
मूल्यं विना – मूल्यम् + विना
स्वाभूषणम् – स्व + आभूषणम्
अधुनैव – अधुना + एव
नाहम् – न + अहम्
नेच्छामि – न + इच्छामि
यथाभिलषितान् – यथा + अभिलषितान्
धन्योऽसि – धन्यो + असि / धन्यः + असि उपसर्गचयनम्

विशेषण-विशेष्य-चयनम् –
विशेषणानि – विशेष्याः
पञ्चदश – घटान्
पञ्चशतोत्तर – रूप्यकाणि
एकम् – घटम्
आवश्यकम् – मूल्यम्
एतत् – आभूषणम्
आभूषणविहीनाम् – त्वाम्
यथाभिलषितान् – घटान्

8. (पञ्चमं दृश्यम्)
(मासानन्तरं सन्ध्याकालः। एकत्र रिक्ताः नूतनघटाः सन्ति। दुग्धक्रेतारः अन्ये च ग्रामवासिनः अपरत्र आसीनाः)

चन्दनः – (धेनुं प्रणम्य, मङ्गलाचरणं विधाय, मल्लिकाम् आह्वयति) मल्लिके! सत्वरम् आगच्छ।
मल्लिका – आयामि नाथ! दोहनम् आरभस्व तावत्।।
चन्दनः – (यदा धेनोः समीपं गत्वा दोग्धुम् इच्छति, तदा धेनुः पृष्ठपादेन प्रहरति। चन्दनश्च पात्रेण सह पतति) नन्दिनि! दुग्धं देहि। किं जातं ते? (पुनः प्रयासं करोति) (नन्दिनी च पुनः पुनः पादप्रहारेण ताडयित्वा चन्दनं रक्तरञ्जितं करोति) हा! हतोऽस्मि। (चीत्कारं कुर्वन् पतति) (सर्वे आश्चर्येण
चन्दनम् अन्योन्यं च पश्यन्ति)

शब्दार्थाः
मासान्तरम् – महीने भर बाद
रिक्ताः – खाली
एकत्र – इकट्ठा
दुग्धक्रेतारः – दूध खरीदने वाले
अपरत्र – दूसरी ओर
आसीनाः – खड़े हैं
विधाय – करके
आह्वयति – बुलाता है
सत्वरम् – शीघ्र/जल्दी
दोहनम् – दुहने के काम को
आरभस्व – शुरू करो
दोग्धुम् – दुहना
पृष्ठपादेन – पीछे के पैर से
प्रहरति – मारती है
ते – तुम्हें
जातम् – हो गया है
पादप्रहारेण – पैर की चोट से
रक्तरञ्जितम् – लहुलुहान
ततोऽस्मि – मारा गया हूँ
चीत्कारम् – चीख,
अन्योन्यम्-एक-दूसरे को।
(पाँचवाँ दृश्य)

अर्थ-
(महीने भर बाद का सायंकाल। इकट्ठे ही खाली नये घड़े हैं। दूध खरीदने वाले और दूसरे गाँववासी दूसरी ओर खड़े हैं।)

चन्दन – (गाय को प्रणाम करके, मंगलाचरण (पूजा) करके, मल्लिका को बुलाता है) हे मल्लिका! जल्दी आओ।
मल्लिका – आती हूँ स्वामी! तब तक दुहना शुरू करो।
चन्दन: (जब गाय के पास जाकर दुहना चाहता है, तब गाय पिछले पैर से मारती है और चन्दन बर्तन के साथ गिरता है) हे नन्दिनी! दूध दो। तुम्हें क्या हो गया? फिर से कोशिश करता है) (और नन्दिनी बार-बार पैर की चोट से मारकर चन्दन को लहुलुहान कर देती है) हाय! मैं मार दिया गया। (चीखते हुए गिरता है) (सभी आश्चर्य से चन्दन और एक-दूसरे को देखते हैं)

सन्धिः विच्छेदः वा –
पदानि – सन्धिः विच्छेदः
मासानन्तरम् – मास + अनन्तरम्
मङ्गलाचरणम् – मङ्गल + आचरणम्
चन्दनश्च – चन्दनः + च
हतोऽस्मि – हतो + अस्मि/हतः + अस्मि

9. मल्लिका – (चीत्कारं श्रुत्वा, झटिति प्रविश्य) नाथ! किं जातम्? कथं त्वं रक्तरञ्जितः?
चन्दनः – धेनुः दोग्धुम् अनुमतिम् एव न ददाति। दोहनप्रक्रियाम् आरभमाणम् एव ताडयति माम्। (मल्लिका धेनुं स्नेहेन वात्सल्येन च आकार्य दोग्धुं प्रयतते। किन्तु, धेनुः दुग्धहीना एव इति अवगच्छति।) मल्लिका – (चन्दनं प्रति) नाथ! अत्यनुचितं कृतम् आवाभ्याम् यत्, मासपर्यन्तं धेनोः दोहनं कृतम्। सा पीडाम् अनुभवति। अत एव ताडयति।
चन्दनः – देवि! मयापि ज्ञातं यत्, अस्माभिः सर्वथा अनुचितमेव कृतं यत्, पूर्णमासपर्यन्तं दोहनं न कृतम्। अत एव, दुग्धं शुष्कं जातम्। सत्यमेव उक्तम्
कार्यमद्यतनीयं यत् तदद्यैव विधीयताम्।
विपरीते गतिर्यस्य स कष्टं लभते ध्रुवम्॥
मल्लिका – आम् भर्तः! सत्यमेव। मयापि पठितं यत्
सुविचार्य विधातव्यं कार्यं कल्याणकाटिणा।
यः करोत्यविचार्यैतत् स विषीदति मानवः॥
किन्तु प्रत्यक्षतया अद्य एव अनुभूतम् एतत्।
सर्वे, – दिनस्य कार्यं तस्मिन्नेव दिने कर्तव्यम्। यः एवं न करोति सः कष्टं लभते ध्रुवम्।

शब्दार्थाः –
चीत्कारम् – चीख को
झटिति – शीघ्र (जल्दी)
जातम् – हुआ
आरभमाणम् – शुरू करने वाले को
स्नेहेन – प्रेम से
वात्सल्येन – पुचकार से
आकार्य – बुलाकर
दुग्धहीना – दूध से रहित
अवगच्छति – समझती है
मासपर्यन्तम् – महीने भर तक
दोहनम् – दुहना
ज्ञातम् – जान लिया
पूर्णमासपर्यन्तम् – पूरे महीने तक
शुष्कम् – सूखा (सूखता)
यत् – जो
अद्यतनीयं – आज का
तत् – उसे
अद्यैव – आज ही
विधीयताम् – करना चाहिए
विपरीते – उल्टा/उल्टी/ विरुद्ध
लभते – पता है
ध्रुवम् निश्चय से, भर्तः!-हे स्वामी
सुविचार्य – अच्छी तरह
सोच – विचार करके
विद्यातव्यम् – करना चाहिए
कल्याणकाक्षिणा – कल्याण चाहने वाले के द्वारा
अविचार्य – बिना विचार करके
विषीदति – दु:खी होता है
मानव – मनुष्य
प्रत्यक्षतया – प्रत्यक्ष रूप से
कर्तव्यम् – करना चाहिए, ध्रुवम् निश्चय से

अर्थ
मल्लिका – (चीख को सुनकर, जल्दी प्रवेश करके) स्वामी! क्या हुआ? कैसे तुम लहुलुहान हो गए?
चन्दन – गाय दूध दुहने की अनुमति ही नहीं दे रही है। दुहने का काम शुरू करते ही मुझे मारती है।
(मल्लिका गाय को प्यार और पुचकार से बुलाकर दुहने की कोशिश करती है किन्तु गाय तो दुधविहीन है यह समझ जाती है।)
मल्लिका – (चन्दन की ओर) हे स्वामी! हम दोनों ने बहुत अनुचित किया कि महीने भर तक गाय का दोहन नहीं
किया। वह पीड़ा (दर्द) को अनुभव कर रही है। इसलिए मारती है।
चन्दन – हे देवी! मुझे भी लगा कि हमने पूरी तरह से अमुचित ही किया कि पूरे महीने तक दोहन नहीं किया। इसीलिए दूध सूख गया। सत्य ही कहा गया है-आज का जो काम है, उसे आज ही करना चाहिए। जिसकी गति (काम करने का तरीका) उल्टी होती है, वह निश्चय ही कष्ट पाता है।
मल्लिका – हाँ स्वामी! सच ही। मैंने भी पढ़ा है यत्-कल्याण चाहने वाले मनुष्य के द्वारा अच्छी तरह से सोच-विचार कर काम को करना चाहिए। जो व्यक्ति बिना विचार किए काम करता है, वह दुखी होता है। किन्तु प्रत्यक्ष रूप से आज ही यह अनुभव किया। दिन का काम उसी दिन ही करना चाहिए। जो ऐसा नहीं करता है वह निश्चित कष्ट को अनुभव करता है।

सन्धिः विच्छेदः वा –
पदानि – सन्धिःविच्छेदः/सन्धिः
किं जातम् – किम् + जातम्
अत्यनुचितम् – अति + अनुचितम्
मयापि – मया + अपि
तदचैव – तत् + अद्य + एव
गतिर्यस्य – गतिः + यस्य
स कष्टम् – स: + कष्टम्
कष्टं लभते – कष्टम् + लभते
अद्य + एव – अद्यैव
तस्मिन्नेव – तस्मिन् + एव
कल्याणकाक्षिणा – कल्याणकाम् + क्षिणा
करोत्यविचार्यैतत् – करोति + अविचार्य + एतत्
विधातव्यं कार्यम् – विधातव्यम् + कार्यम्

विशेषण – विशेष्य – चयनम्
विशेषणम् – विशेष्यः
रक्तरञ्जितः – त्वम्
आरभमाणम् – माम्
अद्यतनीयम् – कार्यम्
ध्रुवम् – कष्टम्
दुग्धहीना – धेनुः
पूर्णमासपर्यन्तम् – दोहनम्
शुष्कम् – दुग्धम्

प्रकृति-प्रत्ययो:-विभाजनम् पदानि

10. (जवनिका पतनम्)
(सर्वे मिलित्वा गायन्ति)
आदानस्य प्रदानस्य कर्तव्यस्य च कर्मणः।
क्षिप्रमक्रियमाणस्य कालः पिबति तद्रसम्॥

शब्दार्थाः-
जवनिका – परदा
पतनम् – गिरता है
आदानस्य – लेने के
प्रदानस्य – देने के
कर्तव्यस्य – करने योग्य
कर्मणः – कर्म के
क्षिप्रम् – शीघ्र
अक्रियामाणस्य – न करने वाले के
काल: – समय
पिबति – पी जाता है
तत् – उसके
रसम् – रस/आनन्द को।।

अर्थ —
(परदा गिरता है।
(सभी मिलकर गाते हैं)
लेने के, देने के और कहने योग्य (अन्य) कर्म के शीघ्र न किए जाने पर समय उसका रस (आनन्द) पी जाता

Here we are providing Class 11 Biology Important Extra Questions and Answers Chapter 8 Structural Organisation in Animals. Important Questions for Class 11 Biology are the best resource for students which helps in Class 11 board exams.

Class 11 Biology Chapter 8 Important Extra Questions Structural Organisation in Animals

Structural Organisation in Animals Important Extra Questions Very Short Answer Type

Question 1.
What is a ligament?
Answer:
It is a connective tissue that joins one bone to another bone.

Question 2.
Name the tissue which forms the inner lining of blood vessels
Answer:
Squamous epithelium

Question 3.
Name the functional unit of a muscle.
Answer:
Sarcomere.

Question 4.
Name any two granulocytes
Answer:
Eosinophils and basophils.

Question 5.
What are cell junctions?
Answer:
Cell junctions are the structures that hold the adjacent cells of tissue together when they are not separated widely by extracellular material.

Question 6.
Name the tissue that lines the intestinal wall.
Answer:
Columnar epithelium.

Question 7.
State the function of neuroglial cells.
Answer:
Serves as packing and supporting material between the nerve cells.

Question 8.
Name few epidermal structures.
Answer:
Hair, nails, claws and scales.

Question 9.
Define organ.
Answer:
Organ: A number of tissues together form an organ that works as a unit for the benefit of an organism.

Question 10.
What is the function of the ligament?
Answer:
The ligament is a dense fibrous connective tissue that connects bones at the joints.

Question 11.
Give an example of a heterochrony gland.
Answer:
Pancreas.

Question 12.
Name the cells from which thrombocytes are produced.
Answer:
Megakaryocytic cells.

Question 13.
Where are W.B.C. formed?
Answer:
In the bone marrow, lymph nodes and spleen.

Question 14.
What are the endocrine glands?
Answer:
They are ductless glands that secrete hormones directly into the bloodstream.

Question 15.
What is the function of antithrombin in our blood?
Answer:
It acts as an anticoagulant that prevents the clotting of blood in our blood vessels.

Question 16.
In which type of nerve fibre, the nerve impulse will move faster?
Answer:
Myelinated nerve fibre.

Question 17.
State the role of ligaments in the human body.
Answer:
It joins bones at the joints

Question 18.
Name the protein found in the white fibres,
Answer:
Collagen.

Question 19.
What do ESR and EDTA stand for?
Answer:
Erythrocytes Sedimentation Rate, Ethylene Diamine Tetra Acetic Acid.

Question 20.
What are myoepithelial cells?
Answer:
Cells showing the peculiar characteristics of both muscles and epithelial tissues.

Question 21.
What do fibroblasts synthesise? j
Answer:
The fibroblast in areolar tissue synthesizes collagen and elastin proteins.

Question 22.
How is coagulation prevented in an uninjured blood vessel?
Answer:
It is due to the secretion of heparin by most cells.

Question 23.
Name the two fundamental types of smooth muscles.
Answer:

1. Single unit smooth muscle.
2. Multi-unit smooth muscle.

Question 24.
What is double circulation?
Answer:
The blood passes twice through the heart to move through the body.

Question 25.
What is the trachea?
Answer:
It is a tube that is a part of the breathing apparatus of air-breathing animals.

Question 26.
What is clitellum?
Answer:
It is a prominent circular band of glandular tissue which surrounds the 14th to 16th segments in the earthworm body.

Question 27.
What is worm casting?
Answer:
It is the insoluble and undigested food that is given out along with soil through the anus.

Question 28.
What is the scientific name of an earthworm?
Answer:
Pheretima Posthuma.

Question 29.
Why is cockroach called Urecotelic?
Answer:
It is called Urecotelic because it excretes in the form of uric acid.

Question 30.
What is the function of Malpighian tubules?
Answer:
It helps in excretion.

Question 31.
What is the trachea?
Answer:
It is a tube that is a part of the breathing apparatus of air-breathing animals.

Question 32.
What are nephridia?
Answer:
Excretory organs of earthworm.

Question 33.
Define the term—hermaphrodite.
Answer:
It is the condition in which both testes and ovaries are produced by the same individual

Question 34.
Name the mouthparts of earthworm.
Answer:
Mandibles, maxillae, labium, labrum and hypopharynx.

Question 35.
Does the rat possess tonsils?
Answer:
No, the rat does not possess tonsils.

Question 36.
What is the functional unit of the cockroach eye?
Answer:
Ommatidium.

Question 37.
What is coelom?
Answer:
It is the true body cavity, space between the body wall and the alimentary canal.

Question 38.
State the difference between male and female frog.
Answer:
The male frog bears copulatory pads and well developed local sacs whereas they are absent in the female.

Question 39.
What are Pilitactiles?
Answer:
They are bristles found on both the sides of nostrils in the rat.

Question 40.
Name the larval stage in the life history of the frog.
Answer:
Tadpole.

Structural Organisation in Animals Important Extra Questions Short Answer Type

Question 1.
What is the utility of transitional epithelium in constituting the surface layer of the urinary bladder?
Answer:
Transitional epithelium is. highly stretchable and it does not allow the urinary bladder to get torn off when it is completely filled with urine. It undergoes considerable expansion to accommodate the maximum quantity of urine.

Question 2.
Classify the muscular tissue into different types.
Answer:
The muscular tissues can be divided into two broad categories depending upon their cytologic characteristic.
These are

1. Striated muscles and
2. Non-striated.

Striated muscle cells, in turn, may be subdivided into two additional categories-skeletal and cardiac.

So these are summarised into three different types:

1. Striated or striped-skeletal and voluntary muscle fibres.
2. Non-striated or unstriped plain or smooth and involuntary muscle fibres.
3. Cardiac – striated and involuntary muscle fibres.

Question 3.
What is the major function of haemoglobin?
Answer:
Functions of haemoglobin:

1. It is essential for oxygen carrier from the lungs to the tissue in the form of oxyhaemoglobin, Each molecule of haemoglobin carries about 1.33 ml of oxygen.
2. It plays an important part in the transport of CO₂ as Carbomino haemoglobin from tissue to the lungs. About 23 per. cent of CO₂ is transported back to the lungs through the haemoglobin.
3. It acts as a buffer arid regulates’•the blood reactions by maintaining a constant pH.

Question 4.
What are the general characteristics of epithelial tissue?
Answer:

1. It consists of closely packed cells of various types and shapes which forms the lining membrane of several organs.
2. The cells of the epithelial membrane are arranged in one or more layers resting upon the thin non-cellular basement membrane and these cells are supported by intercellular cementing substance and close vascular connective tissues.
3. The basement membrane is formed of protein fibres interspersed in the matrix of polysaccharide. The matrix is secreted by underlying connective tissue.
4. The cementing substance between the cells is formed of mucoprotein containing hyaluronic and calcium salts.

Question 5.
What are oligodendrocytes? What their functions?
Answer:
Oligodendrocytes are a type of neuroglia cells that hold the neurons in position in the central nervous system. Their process spirally wraps around the nerve fibres to form a myelin sheath in the absence of Schwann cells in the central nervous system.

Question 6.
Why does oedema occur in persons suffering from a dietary deficiency of proteins?
Answer:
Albumin and globulin proteins are necessary for retaining water in the blood plasma by their, osmotic effects. In the dietary deficiency of proteins, enough water is not retained in the blood plasma and it gets filtered out from the blood into the tissue and leads to the swelling of hands and feet.

Question 7.
Name the specific tissue that forms the outermost exposed surface of the human skin. State any two advantages of this tissue being there.
Answer:
Keratinised squamous epithelium. It contains insoluble protein-keratin which is impervious to water and so prevents the loss of water. It also provides protection to the skin against mechanical pressure, friction, injury and water loss.

Question 8.
Write a short note on erythropoiesis.
Answer:
The formation of new erythrocytes is called erythropoiesis and if takes place from the haemopoietic tissue. The haemopoietic tissue in the young foetus is the liver and spleen whereas in the adults it is the bone marrow, of long bones.

The haemopoietic tissue synthesises millions of RBCs every minute to replace the worn-out erythrocytes promoted by erythropoietin, a glycoprotein produced by renal tissue in response to the liberation of ACTH. The deficiency of iron, folic add and vitamin B leads to anaemia, megaloblastic anaemia and pernicious anaemia etc. 12

Question 9.
How is blood prevented from clotting in the blood banks?
Answer:
In the blood banks, the various anticoagulants like.

1. Sodium oxalate, sodium citrate and EDTA (Ethylene diamine tetraacetic acid) are used which prevent coagulation by removal of calcium ions.
2. Clotting can also be prevented by removing free calcium ions from the whole blood as this metallic ion is a critical requirement in several stages of the coagulation mechanism.

Question 10.
How does saltatory conduction take place along a nerve fibre?
Answer:
Along with a myelinated nerve fibre, the conduction of impulse is called saltatory conduction. This is so because the ionic changes and consequent depolarisation taking place only at the nodes of Ranvier free from myelin sheath leading to the jumping of action potential from one node to the next.

Question 11.
State the important function of platelets.
Answer:

1. During injury to any tissue, the platelets disintegrate and liberate a thromboplastin which is concerned with the activation of prothrombin to thrombin.
2. The platelets are quite sticky and they get adhered to the roughened surfaces of foreign bodies as bacteria. This response tends to increase the bodily defence as leukocytes can phagocytize these microorganisms more easily.

Question 12.
Write a note on the body wall of the earthworm.
Answer:
The body wall of the earthworm: The body wall of the earthworm is covered by a thin non-cellular cuticle. The cuticle is followed by an epidermis, two muscle layers and innermost coelomic epithelium. The epidermis consists of a single layer of columnar epithelial cells. It contains many other types of cells, including the secretory gland cells. Muscle layers are made of circular and longitudinal muscle fibres.

Question 13.
How does the earthworm move?
Answer:
Earthworm moves with the help of an S-shaped stopped setae, embedded in the epidermal pit at the middle of each segment. These are made up of chitin and help in locomotion by gripping the earth. Locomotion is thus, performed by contraction and relaxation of the muscles and used of setae for gripping on-die soil.

Question 14.
What is the main difference between the blood of earth¬worm and man?
Answer:
The blood of the earthworm is red due to haemoglobin that is dissolved in plasma. Corpuscles are colourless and nucleated. Man blood is also red but haemoglobin is dissolved in RBGs. The corpuscles are denucleated and biconcave disc-like.

Question 15.
Name the main excretory organ in the cockroach. How does it help in excretion?
Answer:
The main excretory organ in the cockroach is the malpighian tubules. Each malpighian tubule is lined by glandular, ciliated cells with a brush border. They absorb the nitrogenous waste product and convert them into uric acid through various biochemical processes. The uric acid is excreted through the hindgut. The fat bodies, nephrocytes, cuticle and Urecose glands also help in the excretion

Question 16.
What is the mosaic vision?
Answer:
Each ommatidium produces a separate image or a small part of the object present just in front of it. Thus, the image of the object formed in a compound eye is actually made of several small pieces. Such a picture or image is called a mosaic image and the vision is called mosaic vision. The nature of the image depends upon the intensity of light. In bright light, an apposition image is formed and in dim light, it is superposition.

Question 17.
Write briefly about the female reproductive system in the cockroach.
Answer:
It consists of two large ovaries lying laterally in the 4th, 5th and 6th segment. Each ovary is made up of a group of 8 ovarian tubules containing a chain of developing ova. Oviducts of each ovary unite into a single median oviduct that is also called the vagina that opens into the genital chamber. The female accessory glands are a pair of branched, collateral glands, that open into the dorsal side of the genital chamber:

Question 18.
What is a portal system?
Answer:
It consists of a vein and its capillaries that collect deoxygenated blood from some organ and pour it into some other organ instead of supplying it directly to the heart. Such a vein is called a portal vein and these veins along with their capillaries constitute the portal system.

Question 19.
Write briefly about the brain of the frog.
Answer:
The frog’s brain consists, of the fore-brain, mid-brain and hind-brain. It is enclosed in a bony structure, which has two occipital condyles for attachment with the first vertebra. Fore-brain is made up of olfactory lobes, paired cerebral hemispheres and unpaired diencephalon.

The mid-brain has characteristic paired ‘optic lobes. The hind-brain consists of the cerebellum and medulla oblongata. The medulla oblongata passes out through the foramen magnum and continues into the spinal cord that is contained in the vertebral column.

Question 20.
What is the interaction of frog with mankind?
Answer:

1. Frogs eat up insects and thus, protect the crop.
2. Frogs are largely used as experimental material for teaching and research.
3. The muscular legs of the frog are largely used as food by man in some parts of India and many other countries.

Question 21.
What are nephridia? In which animal they are present? Name a different kind of nephridia by indicating their location.
Answer:
Nephridia are the excretory organs and they are found in earth-worm.

There are three types of nephridia:

1. Septal nephridia: These are present on both sides of the intersegmental septa and open into the intestine.
2. Integumentary nephridia: These are found attached to the lining of the body wall and open on the body surface.
3. Pharyngeal nephridia: These are found in the 4th, 5th and 6th segments in the form of paired tufts.

Question 22.
What are the ear ossicles in the rat?
Answer:
Ear ossicles in the rat:
The ear of a rat is divided into three parts:

1. external ear,
2. the middle ear, and
3. internal ear.

The outermost is the external ear or pinna has the middle ear three small bones called ear ossicles. The third internal ear is related to hearing and equilibrium. The semicircular canals are lined with sensory epithelial cell in the internal ear of the rat.

Question 23.
How is earthworm known as the “friend of farmers”?
Answer:

1. Earthworm makes small burrows in the soil and so the soil becomes porous facilitating respiration and penetration of developing plant roots.
2. They chum up the soil on swallowing at their mouth end and pass the soil out at their anal end. Thus, the soil becomes finer and capable of holding more soil water.
3. They cultivate the soil by bringing its deeper layer of the surface.
4. Earthworms are also used as food in some countries. Many tribal communities in India use earthworms in the form of medicine to cure bladder stones, jaundice, piles, diarrhoea etc.

Question 24.
Name the main excretory organ in the cockroach. How does it help in excretion?
Answer:
The main excretory organ in the cockroach is the malpighian tubules.

Each malpighian tubule is lined by glandular, ciliated cells with a brush border. They absorb the nitrogenous waste product and convert them into uric acid through various biochemical processes. The uric acid is excreted through the hindgut. The fat bodies, nephrocytes, cuticle and Urecose glands also help in excretion.

Question 25.
Describe briefly the male reproductive organs in the cockroach.
Answer:
The male reproductive organs consist of a pair of testes lying one on each lateral side in the 4th-5th abdominal segments, A vas deferens, a thin tube arises from each testis and opens into the male gonophore situated ventral to the anus. A mushroom-shaped gland is present at the 6th-7th abdominal segments that function as an accessory reproductive gland.

The external genitalia is represented by chitinous asymmetrical structures, surrounding the male gonopore, at the end of the abdomen, called male gonapophysis or phylloxera. The sperms are stored in seminal vesicles and are glued together in the form of bundles called spermatophores.

Question 26.
Give the possible reason for the following statements.
(i) A transparent nictitating membrane is present in the eyes of a frog.
Answer:
Nictitating membrane protects the eyes of the frog when it undergoes mud during hibernation, aestivation and swimming.

(ii) The first finger of the male frog is swollen.
Answer:
The first finger in the male frog is swollen in the form of a copulatory pad to hold up the female frog firmly during copulation.

(iii) The skin of the frog is moist and slippery
Answer:
The skin of the frog is moist and slippery to perform the cutaneous respiration as well as to slip away from the grip of predators.

(iv) Webs are present between the toes of frog,
Answer:
The webs are present between the toes because these act as paddles when the frog is swimming.

Question 27.
Name the three types of respiration in the frog. How does frog respire during hibernation?
Answer:
The three types of respiration in a frog are:

1. Cutaneous respiration
2. Buccal respiration
3. Pulmonary respiration

The frog’s skin is moist, slimy and highly vascularized which is especially useful for respiration in hibernation and aestivation. The oxygen of the atmosphere enters the thin film of skin moisture where it goes to the blood capillaries of the skin. The oxygen mixes with the blood and passes to the different organs of the body. The carbon dioxide formed in the body organs is taken up by the blood capillaries to the skin from where it diffuses out into the air.

Structural Organisation in Animals Important Extra Questions Long Answer Type

Question 1.
Enumerate the various functions of epithelial tissue.
Answer:
Function:

1. Protection: It protects the underlying or overlying soft tissues against heat, injury, chemicals, virus and bacteria etc.
2. Absorption: It absorbs the digestive food especially with columnar cells of the intestine.
3. Secretion: The glandular epithelial cells lining the inner cavities secrete various substances like mucous, enzymes and hormones which are necessary for various metabolic activities.
4. Excretion: The epithelial cells of kidney tubules and sweat glands help in the excretion of wastes from the body.
5. Sensation: The nerve ending in the epithelial cells of the retina, olfactory organs and nasal chamber etc. receive the stimuli from the external atmosphere and transfer them to the brain for interpretation.
6. Other functions: The trachea contains the ciliated epithelium to facilitate the transport of mucous and other substances from one part of the body to another. The lungs’ epithelium helps in the exchange of gases during respiration and the germinal epithelium of testes and ovaries form the sperms and ova respectively. The epithelium also forms the exoskeleton structure as scales, feathers, hairs, nails, claws, horns etc.

Question 2.
What is a gland? Differentiate between simple and compound exocrine gland.
Answer:
Any organ or structure that secretes specific useful substances is known as a gland.

A gland develops from the epithelium tissues and is generally cubical, short columnar or polyhedral in shape.
1. Simple exocrine gland: Simple exocrine gland has no branch but consists of a single unbranched duct lined by epithelial cells. The secretory part of the gland also consists of epithelial cells arranged in a simple tube, coiled tube in a flask which send their secretion into a single duct.

So these are simple tubular glands, simple coiled tubular glands and simple alveolar glands respectively.

2. Compound exocrine gland: Compound exocrine gland has a branched system of ducts. The secretory part consists of tubules-Com-pound tables, many acini or alveoli-compound alveolar glands or a combination of both tubules and acini-compound tubuloalveolar gland.

Question 3.
What is connective tissue? Give its important functions.
Answer:
Connective tissue is mesodermal in origin and form of matrix, fibres and cells. It constitutes the extracellular ground substance and fills up the intercellular spaces between the cells.

Functions of connective tissue: The connective tissue perform various functions and these are:

1. It serves the function of packing material nearly for all organs.
2. It binds one tissue or organ to another and serves the purpose of a strong elastic rope.
3. It provides skeletal support and shape to the body.
4. It protects the vital organs of the cranial and thoracic cavities, deep blood vessels and nerves from mechanical injuries.
5. The adipose connective tissue stores fat and represent stored energy.
6. It provides defence against foreign particles like bacteria. The phagocytes of leucocytes ingest the bacteria and germs and protect the body against infection.
7. The lymphocytes from the antibodies against the action of antigens and provide immunity to the body.

Question 4.
What is adipose tissue? Where does it lie in the human body? Give its important functions.
Answer:
Adipose tissue: It is the specialized form of areolar tissue where it contains fat cells or adipocytes in the matrix. Each fat cell is large, rounded occupied by big fat droplets and its nucleus and cytoplasm are pushed towards the periphery of the cell. This imparts a ‘signet’ ring-like look to the fat cell.

These fat cells can easily be stained by Sudan III or osmic acid. The matrix is supported by a loose framework of areolar tissue containing fibroblasts, macrophage, white collagen fibres of small size, elastic fibres; lymphatics and blood vessels.

The lactating tissue lies in the subcutaneous tissue of the skin, in the mesentery and in the perinephric and sub periodical tissue of the body. The lactating mammary gland of human also contain abundant amounts of adipose tissue but these are quite lacking in penis, scrotum, eyelids and in the cranial cavity.

Adipose tissue

Functions: It synthesises, stores and metabolises the fat and forms the insulating layer beneath the skin. It collects around the viscera especially kidneys and prevents them from shock and injury.

Question 5.
Plasma contains three classes of proteins. What are these? Give their functions.
Answer:
The major classes of plasma proteins are

1. Albumin,
2. Globulins and
3. Fibrinogen

Functions of Plasma Proteins:

1. The immune bodies are mainly an important constituent of the globulin fraction which provide defence against infection.,
2. Fibrinogen and prothrombin are necessary for the coagulation of blood.
3. The plasma proteins normally have an osmotic effect of 25 mm Hg and thus influence the exchange of fluid between blood and tissue spaces.
4. Plasma proteins mainly globulins are responsible for the viscosity of blood and this help in maintaining peripheral resistance and arterial blood pressure for efficient heart functioning.
5. Plasma, proteins act as buffers in maintaining acid-base balance.
6. Albumin and globulin proteins retain water in the blood plasma by their osmotic effects and their deficiency lead to oedema.
7. Plasma proteins especially the fibrinogen are concerned with erythrocyte sedimentation rate.
8. Plasma proteins help in the transport of certain substances like hormones, enzymes, iron and copper etc. in the blood.
9. Plasma proteins distribute heat uniformly all over the body.

Question 6.
Describe briefly the external features of cockroach.
Answer:
The cockroach is a nocturnal cursorial and omnivorous insect. It is generally reddish-brown in colour and its entire body is covered by tough chitin. Its body is divided into head, thorax and abdomen.

Head: It is somewhat pear-shaped and is six segmented. It lies at a right angle to the body with the broad side upwards. It articulates with the thorax by the flexible neck. On each side of the head is a large compound eye. A pair of antennae articulate Inspite close to the notches of the compound eyes.

The top of the head is termed a vertex. Mouthparts are chewing and surrounded by five kinds of appendages. These include, the labrum, mandibles, first maxillae, second maxillae and hypopharynx. The neck is slender, flexible and supported by chitinous cervical plates.

Thorax: The thorax consists of three segments – Prothorax, mesothorax and metathorax. Each segment of the thorax is covered by four sclerites. There is a tergum on the dorsal side, a sternum on the ventral side and a pleuron on either lateral side.

The thorax bears three pairs of jointed walking legs, a pair per segment and two pairs of wings in meso and metathorax. Each leg is made of a number of parts-coxa; the trochanter, femur, tibia and tarsus. Their leg is made of a number of parts coxa, trochanter, femur, tibia and tarsus: They are formed as lateral expansions of the

(a) External features of cockroach

integument between the tergum and the pleuron. Each wing is supported by a network of hollow veins. The mesothorax wings are narrow, thick, opaque and leathery and not used in flight.
Head

(b) External features of cockroach

Abdomen: The abdomen is ten segmented and covered by four sclerites. There are is all ten-terga, but 9th terga of the male and 8th and 9th terga of the female are covered by 7th tergum. In the female-only the first seven sterna are visible, the seventh, eighth and ninth sterna together form a brood pouch.

The 10th tergum bears a pair of a long tapering structure called anal cerci. In male 9th sternum bears a pair of short unjointed anal styles. They are absent in females. The gonapophyses are very small, irregular arising from the 9th sternum in the male and from the eighth and ninth sterna in the female.

At the posterior end of the abdomen, below the tenth terga, is situated the anus. There, are ten pairs of spiracles on the lateral sides of the body, two on the thorax, eight on the abdomen.

Question 7.
Describe briefly the alimentary canal and digestive glands of the frog.
Answer:
The alimentary canal of the frog is short. It starts with a mouth that is terminal in position. It opens into the buccopharyngeal cavity which contains the maxillary and vomerine teeth and carries the openings of the eustachian tube, vocal sac, gullet and glottis. The gullet opens in a narrow and short tubed Oesophagus which continues In the large stomach. Stomach walls arc highly muscular that help In converting the food into chyme.

The stomach is followed by a coiled small intestine. The small intestine bears a number of finger-like folds called villi and microvilli which increase the surface area for absorption of digested food. Intestine continues into a wider section, opening into the cloaca. The urinary bladder opens into the cloaca. The urinary opens into the cloacal chamber through the ureter.

Alimentary canal and digestive glands of the frog

The gastric glands of the stomach bring about the digestion of protein. The liver secretes bile that is temporarily stored in the gall bladder. Bile helps in the digestion of food by changing its pH from acidic to alkaline and by emulsifying the fat. The liver does not secrete any enzyme. The pancreas is situated in a thin mesentery and lies parallel to the stomach.

It secretes pancreatic juice that causes the digestion of protein starch and fats with the help of trypsin, amylase and lipase. Similarly, intestinal juices with their peptidases, lipase and sugar enzymes bring about the digestion of peptides, fats and sugars.

Question 8.
Describe briefly the blood vascular system of the cockroach.
Answer:
The cockroach has an open circulatory system. Blood is colourless and contains plasma with colourless cells, the leucocytes. It does not contain haemoglobin and thus, plays no role in respiration.

The heart is thirteen chambered which is long, narrow, muscular and tube-like structure. The three chambers of the heart lie in the thorax and ten in the abdomen. The posterior end is closed and the anterior end is continued forwards as the anterior aorta. It opens into a haemocoel in the head.

There is a small hole, the Ostia at the posterior side of each chamber. Ostia is guided by valves to allow blood flow only in one direction i.e., from the haemocoel to the inner chamber of the heart.

All the visceral organs are bathed in blood. It consists of a colourless liquid part, plasma containing many corpuscles called haemocytes.

Question 9.
Describe the male reproductive organs in the frog.
Answer:
It consists of a pair of the yellowish testis that is attached to the upper part of the kidneys by a double fold of peritoneum called mesorchium.

Reproductive organs of the male frog

Each testis contains about 10 to 12 vasa efferentia that run through the mesorchium and enter the kidneys of their side. In kidneys, these open into the bidder’s canal that finally joins with the urinogenital ducts. This cones of the kidneys and finally opens into the cloaca. The cloaca is a small, medium chamber that is used to pass faecal matter, urine and sperms to the exterior.

Question 10.
Draw a labelled diagram of the reproductive organs of an earthworm.
Answer:
Reproduction in Earthworm: The earthworm is hermaphrodite (bisexual). The testes and ovaries are present in the same individual. There are two testes present in the 10th and 11th segments. Ducts that arise from them are called vasa deferentia. The run-up to the 18th segment where they join the ventral side of the 17th and 19th segments.

The prostate and spermatic duct open to the exterior by a pair of male genital pores on the ventrolateral side of the 18th segment. The spermathecae are found one in each of the 6th to 9th segments. They are 4 pairs, they receive and store spermatozoa during copulation.

Two ovaries are attached at the intersegmental septum of the 12th and 13th segments. Ovarian funnels are found beneath the ovaries. They continue into oviducts, join together and open on the ventral side as a single female genital pore on the 14th segment. The development is direct. There are no larval forms in earthworm.

The reproductive system of earthworm

Question 11.
Describe the alimentary canal of an earthworm and interactions with mankind.
Answer:
Alimentary canal of earthworm: Alimentary canal is a straight tube starting from 1st segment to the last segment of the body. It consists of the mouth, buccal cavity, pharynx, oesophagus, gizzard, stomach, intestine and anus. The mouth lies in the first segment.

The mouth opens into the buccal cavity (1 – 3 segment). The buccal cavity leads into the pharynx. The pharynx leads into the oesophagus which is continued into the gizzard. The stomach extends from the 9th 14th segment. The intestine starts from the 15th segment and continues up to the last segment. The alimentary canal opens to the outside by an aperture called the anus.

Interaction of earthworm with mankind:

1. Earthworms are the friends of farmers. They make burrows in the soil and make it porous to facilitate respiration and penetration of plant root. Earthworm increases the fertility of the soil by vermicomposting.
2. In China, Japan, Burma, Australia, earthworms are used for food.
3. Earthworms are used as bait for game fishing.
4. In India, earthworms are used for the treatment of stones, jaundice, piles, diarrhoea.
5. Earthworms also cause harm by making burrows in the land (damage tender plant).

Alimentary canal of the earthworm

By going through these CBSE Class 11 Maths Notes Chapter 7 Permutations and Combinations Class 11 Notes, students can recall all the concepts quickly.

Permutations and Combinations Notes Class 11 Maths Chapter 7

Fundamental Principle of Counting (F.P.C.): If an event can occur in m different ways, following which another event can occur in n different ways, following which another event can occur in r different ways and so forth, then the total number of different ways of occurrence of the events in the given order is m x n x r x …

Note : The principle is also known as multiplication principle.

Factorial Notation:
Important Definition : Factorial is a convenient notation for representing the product of first natural numbers. The notation for the product uses a mark of exclamation (!) suffixed to the highest natural number in the product. Thus

4! = 4 × 3 × 2 × 1.
6! = 6 × 5 × 4 × 3 × 2 × 1.
In general, n! = n(n – l)(ra – 2)… (3)(2)(1).
It is read as ‘factorial n

Important formula:
(i) 6(5!) = 6(5 × 4 × 3 × 2 × 1)
= 6!
n[(n-l)!] = n!
(it) 0! = 1. [By definition]

Permutations:
Important Definition : A permutation is an arrangement in definite order of a number of objects taken when some or all at a time.

Important Formulae : (i) The number of permutations of n different objects taken all at a time, denoted by nPn is given by ⁿP_n = n(n – l)(n – 2)… 3.2.1
= n!

(ii) The number of permutations of n different objects taken r at a time denoted by ⁿP_r (n > r) is given by
ⁿP_r or P(n, r) = n(n – 1)(n – 2)… (n + 1 – r).
or ⁿP_r = \(\frac{n !}{(n-r) !}\) (when all objects are different)

(iii) Value of 0!
Putting r = n in \(\frac{n !}{(n-r) !}\), we have :
ⁿP_n= \(\frac{n !}{(n-n) !}\) or n! = \(\frac{n !}{0 !}\)
or 0! = \(\frac{n !}{n !}\) = 1
∴ 0! = 1.

(iv) Permutations of n objects all at a time, when n1 are alike, n2 are alike, and so on but different from n1 and n2 are given by
P = \(\frac{n !}{n_{1} ! n_{2} ! n_{3} !}\)

(v) Circular permutations : If we consider arrangements of objects in a circle, instead of a line, then we speak of circular permutations.

If n distinct objects are arranged in a circle, each object can shift position n times and returns to its original without disturbing the arrangement. Thus, each circular permutation gives n linear permutations and the number of distinguishable circular permutations, p is given by p = \(\frac{n !}{n}\) = (n – 1)!.

Combinations :
Important Definition: (i) A combination is a selection of some or all of a number of different objects. In a combination, the order of selection of the objects is immaterial.

(ii) The difference between a permutation and a combination of the objects is the “Order” does matter in a permutation, while it does not matter in case of a combination.

(iii) Relation between permutations and combinations is
C(n, r) = \(\frac{\mathrm{P}(n, r)}{r !}\), where C(n,r)
is the number of combinations of n objects taken r at a time.

or P(n,r) = (r!)C(n, r)
Note : ⁿP_r and ⁿC_r may also be denoted as P(n, r) and C(n, r) respectively.

Important Formulae:
(i) C(n, r) = \(\)
Note : (a) C(n, n)= 1 (b) C(n, 0) = \(\frac{n !}{0 !(n-0) !}\) = 1.
(ii) Complementary combinations
C (n, r) = C(n, n-r)
(;iii) Pascal’s rule
C(n, r) + C(n, r – 1) = C(n + 1, r).

We have given detailed NCERT Solutions for Class 10 Sanskrit Grammar Book अव्ययाः Questions and Answers come in handy for quickly completing your homework.

Sanskrit Vyakaran Class 10 Solutions अव्ययाः

अभ्यास:

प्रश्न 1.
समुचितैः अव्ययैः (मञ्जूषातः गृहीत्वा) रिक्तस्थानानि पूरयत-

(i) सः ___________ वनं गतवान्।
(ii) सः ___________ गच्छति?
(iii) गजः ___________ चलति।
(iv) सः ___________ स्वपिति।
(v) सिंहः ___________ गर्जति।
(vi) सः ___________ विजेष्यते।
(vii) परिश्रमं कुरु, ___________ अनुत्तीर्णः भविष्यसि।
(viii) गृहात् ___________ मा गच्छ।
(ix) सः ___________ माम् उद्वेजयति।
(x) कोलाहलं ___________ कुरु।

मञ्जूषा- मा, बहिः, मुहुर्मुहुः, अन्यथा, एकदा, शनैः-शनैः, चिरम्, नूनम्, उच्चैः, कुत्र

उत्तराणि:
(i) एकदा
(ii) कुत्र
(iii) शनैः-शनैः
(iv) चिरम्
(v) उच्चैः
(vi) नूनम्
(vii) अन्यथा
(viii) बहिः
(ix) मुहुर्मुहुः
(x) मा

प्रश्न 2.
अधोलिखितेषु वाक्येषु अव्ययपदं चित्वा लिखत-

(i) यावत् परीक्षाकालः नायाति तावत् परिश्रमं कुरु।
(ii) अस्माभिः सर्वदा सत्यं वक्तव्यम्।
(iii) कालः वृथा न यापनीयः।
(iv) अहं सम्प्रति गृहं गन्तुम् इच्छामि।
(v) त्वं कुतः समायात्?
(vi) अहं श्वः ग्रामं गमिष्यामि।
(vii) तौ परस्परम् आलपतः।
(viii) अद्यप्रभृति अहं धूमपानं न करिष्यामि।
(ix) धनं विना जीवनं वृथा भवति।
(x) अथ रामायणकथा आरभ्यते।
उत्तराणि:
(i) यावत्, तावत्
(ii) सर्वदा
(iii) वृथा
(iv) सम्प्रति
(v) कुतः
(vi) श्वः
(vii) परस्परम्
(viii) अद्यप्रभृति
(ix) वृथा
(x) अथ

प्रश्न 3.
कोष्ठकेभ्यः शुद्धम् अव्ययपदं चित्वा रिक्तस्थानं पूरयत्-

(i) अहम् ___________ भ्रमणाय गमिष्यामि। (श्वः / ह्यः)
(ii) त्वम् कस्य ___________ गच्छसि? (परित: / पुरतः)
(iii) विद्यालयम् ___________ उद्यानम् अस्ति। (परित: / एव)
(iv) सः यदा आगमिष्यति ___________ अहं गमिष्यामि। (तदैव / तथैव)
(v) परिश्रमं कुरु ___________ अनुत्तीर्णः भविष्यसि। (सर्वदा / अन्यथा)
(vi) त्वं ___________ कुत्र गच्छसि? (जातु / साम्प्रतम्)
(vii) यूयम् ___________ ध्यानेन पठत। (बाढम् / नूनम्)
(viii) श्यामः ___________ पठति श्यामा न। (एव / विना)
(ix) छात्राः पुस्तकम् ___________ न शोभन्ते। (यदि / विना)
(x) यथा वप्स्यसि ___________ फलं प्राप्स्यसि। (तदा / तथा)
उत्तराणि:
(i) श्वः
(ii) पुरतः
(iii) परितः
(iv) तदैव
(v) अन्यथा
(vi) साम्प्रतम्
(vii) नूनम्
(viii) एव
(ix) विना
(x) तथा

अभ्यासः

प्रश्न 1.
इमं संवादम् उचितैः अव्ययपदैः पूरयत। उत्तराणि समक्षं प्रदत्तस्थाने लिखत अधा प्रदत्ता (अव्ययानां सूची अधः प्रदत्ता)-

मञ्जूषा – अधुना, अपि, बहिः, नूनम्, उच्चैः

रमा – विश्रुत! मेघाः (i) ___________ गर्जन्ति।
विश्रुतः – आम् (ii) ___________ वृष्टिः भविष्यति।
रमा – अवश्यम्, त्वम् गृहात् (iii) ___________ मा गच्छ।
विश्रुतः – तर्हि अहम् (iv) ___________ दूरदर्शने एव नाटकम् पश्यामि।
रमा – विश्रुत! समाचारम् अपि शृणु, दशमकक्षायाः परीक्षापरिणामस्य सूचना (v) ___________ संभवेत्!
उत्तराणि:
(i) उच्चैः
(ii) नूनम्
(iii) बहिः
(iv) अधुना
(v) अपि।

प्रश्न 2.
अधोलिखिते संवादे उचित-अव्ययपदैः रिक्तस्थानानि पूरयत। (अव्ययानां सूची अधः प्रदत्ता)-

मञ्जूषा – उच्चैः, किमर्थम्, एव, बहिः, अपि

पिता – पुत्र! एषः कोलाहल: (i) ___________ भवति?
पुत्र – (ii) ___________ एका नटी स्वनृत्यकौशलं प्रदर्शयति।
पिता – किम् कश्चित् गायति, वादयति (iii) ___________?
पुत्र – आम्, नटः (iv) ___________ गायति, ढोलकं वादयति च।
पिता – अभ्यासेन (v) ___________ कौशलं प्राप्यते।
उत्तराणि:
(i) किमर्थम्
(ii) बहिः
(iii) अपि
(iv) उच्चैः
(v) एव।

प्रश्न 3.
अधोलिखितम् अनुच्छेदम् उचितैः अव्ययपदैः पूरयित्वा पुनः लिखत-

मञ्जूषा – यदा, तदा, नूनम् एव, अद्य, अपि, यत्र, तत्र, सर्वदा

(i) विद्यालये ___________ वनमहोत्सवः मान्यते।
(ii) छात्रैः गृहेभ्यः ___________ एकः पादपः आनीतः।
(iii) छात्रैः आनीतेषु पादपेषु फलिनः वृक्षाः ___________ सन्ति।
(iv) ___________ वृक्षाः सन्ति ___________ हरीतिमा वर्तते।
(v) ___________ वृक्षेषु फलानि आगमिष्यन्ति ___________ केचन अन्ये एव छात्राः तानि उपभोक्ष्यन्ति।
उत्तराणि:
(i) अद्य
(ii) नूनम् एव
(iii) अपि
(iv) यत्र, तत्र
(v) यदा, कदा।

प्रश्न 4.
अधोलिखितानि वाक्यानि मञ्जूषायाः सहायतया उचितैः अव्ययपदैः पूरयित्वा पुनः लिखत-

मञ्जूषा – यावत्-तावत्, शनैः शनैः, श्वः, विना, मा, उच्चैः, एव

(i) ___________ मम मातुल: काश्मीरात् आगमिष्यति।
(ii) असत्यं ___________ वद।
(iii) ___________ स्थास्यन्ति गिरयः ___________ रामायणी कथा प्रचरिष्यति।
(iv) कच्छपः ___________ चलति।
(v) अस्माकं जीवनं जलं ___________ असम्भवम्।
उत्तराणि:
(i) श्वः
(ii) मा
(ii) यावत्-तावत्
(iv) शनैः शनैः
(v) विना।

प्रश्न 5.
अधोलिखितानि वाक्यानि मञ्जूषायाः सहायतया उचितैः अव्ययपदैः पूरयित्वा पुनः लिखत-

मञ्जूषा – इतस्ततः, सर्वत्र, यथा, तथा, वृथा, उपरि, अधः, कुत्र

(i) वृक्षाणाम् ___________ वानराः कूर्दन्ति।
(ii) ईश्वरः ___________ व्यापकः अस्ति।
(iii) ___________ अश्वाः दग्धाः।
(iv) ___________ च पञ्त्वं गताः।
(v) समुद्रेषु वृष्टिः ___________।
उत्तराणि:
(i) उपरि, इतस्ततः
(ii) सर्वत्र
(iii) यथा
(iv) तथा
(v) वृथा।

प्रश्न 6.
उचितैः अव्ययपदैः इमे संवादाः पूरयन्ताम् (अव्ययानां सूची मञ्जूषायां दत्ता)-

मञ्जूषा – शनैः शनैः, अपि, शीघ्रम्-शीघ्रम्, एव, नूनम्।

विभः – एतत् उपवनम्। यस्य हस्ते करण्डक: (i) ___________ एषः मालाकारः।
रश्मिः – आम्! अहं तं जानामि। एषः मालाकारः (ii) ___________ अस्ति।
विभः – पश्य, उपवने जनाः (iii) ___________ चलन्ति।
रश्मिः – परम् अहं पश्यामि यद् वृद्धाः तु (iv) ___________ चलन्ति।
विभः – पश्य, तत्र महिलाः (v) ___________ भ्रमन्ति।
उत्तराणि:
(i) नूनम्
(ii) एव
(iii) शीघ्रम्-शीघ्रम्
(iv) शनैः शनैः
(v) अपि।

प्रश्न 7.
अयं संवादः उचितैः अव्ययपदैः पूरणीयः। अव्ययपदानि मञ्जूषायाः ग्रहीतव्यानि।

मञ्जूषा – श्वः, तत्र, अत्र, इतस्ततः, अपि

अर्णवः – (i) ___________ एकं विशालम् उपवनम् अस्ति।
प्रत्यूषः – तत्र वृक्षेषु वानराः (ii) ___________ कूर्दन्ति।
अर्णवः – पश्य, (iii) ___________ मयूराः अपि नृत्यन्ति।
प्रत्यूषः – शृणु, वृक्षशाखासु कोकिलाः (iv) ___________ मधुरं गायन्ति।
अर्णवः – आगच्छ, अधुना गृहं गच्छाव। (v) ___________ पुनः आगमिष्यावः।
उत्तराणि:
(i) अत्र
(ii) इतस्ततः
(iii) तत्र
(iv) अपि
(v) श्वः।

प्रश्न 8.
अयं संवादः उचितैः अव्ययपदैः पूरणीयः। अव्ययपदानि मञ्जूषायाः ग्रहीतव्यानि।

मञ्जूषा – विना, सह, नूनम्, यदा, अधुना

मोहनः – राम! त्वं गृहात् बहिः गच्छ।
रामः – अहं त्वां (i) ___________ न गमिष्यामि।
मोहनः – किमर्थम् मया एव (ii) ___________ गमिष्यसि?
रामः – (iii) ___________ रात्रिः अस्ति। अहम् अन्धकारात् त्रस्यामि।
मोहनः – अहं तु न त्रस्यामि। (iv) ___________ त्वया सह गमिष्यामि।
रामः – (v) ___________ मेघाः गर्जन्ति तदा मयूराः नृत्यन्ति।
उत्तराणि:
(i) विना
(ii) सह
(iii) अधुना
(iv) नूनम्
(v) यदा।

प्रश्न 9.
अयं संवादः उचितैः अव्ययपदैः पूरणीयः। अव्ययपदानि अधोदत्तायाः मञ्जूषायाः ग्रहीतव्यानि।

मञ्जूषा – एव, अलम्, उच्चैः, अधुना, शनैः

अध्यापकः – अनिल! अत्र आगच्छ।
अनिलः – आगतः अस्मि, श्रीमन्तः! (i) ___________ किं करवाणि?
अध्यापकः – (ii) ___________ भ्रमणेन। अत्र उपविश।
अनिलः – आम्, उपविशामि। यद् भवन्तः कथयिष्यन्ति तद् (iii) ___________ करिष्यामि।
अध्यापकः – एतं पाठम् (iv) ___________ पठ। पाठम् (v) ___________ न पठ शीघ्रं न पठ।
उत्तराणि:
(i) अधुना
(ii) अलम्
(iii) एव
(iv) उच्चैः
(v) शनैः।

प्रश्न 10.
मञ्जूषायां दत्तानि पदानि संयोज्य वाक्यानि रचयत-

(i) _______________________
(ii) _______________________
(iii) _______________________
(iv) _______________________
(v) _______________________
उत्तराणि:
(i) अहम् ह्यः देवालयम् अगच्छम्।
(ii) अहम् श्वः तरणताले तरिष्यामि।
(iii) अहम् अधुना भोजनम् खादामि।
(iv) अहम् यदा-कदा उपवने भ्रमामि।
(v) अहम् पठितुं विद्यालयम् गच्छामि।

प्रश्न 11.
अधोलिखिते अनुच्छेदे रिक्तस्थानानि उचित-अव्ययपदैः पूरयत। (अव्ययानाम् सूची अधः प्रदत्ता)-

मञ्जूषा – पुरा, एकदा, तत्र, च, एव

(i) ___________ श्रीकृष्णः सुदामा (ii) ___________ शैशवात् मित्रे आस्ताम्। तौ बाल्ये गुरोः संदीपनस्य आश्रमे (iii) ___________ अवसताम्। (iv) ___________ गुरुपत्न्याः आदेशेन तौ काष्ठानि आनेतुं वनं गतौ। वने काष्ठानां कृन्तनकार्ये तयोः सूर्यास्तः अभवत्। तौ हिंसकप्राणिभ्यः भीतौ एकं वृक्षम् आरुह्य (v) ___________ स्थितौ।
उत्तराणि:
(i) पुरा
(ii) च
(iii) एव
(iv) एकदा
(v) तत्र।

प्रश्न 12.
इमां कथां उचितैः अव्ययपदैः पूरयत। अव्ययानां सूची अधः प्रदत्ता।

मञ्जूषा – यदि, एव, सहसा, तु, अधः
आम्रवृक्षस्य (i) ___________ उपविष्टः एकः नरः कोमलासु लतासु विशालफलानि दृष्ट्वा अकथयत्, “मूर्खः ईश्वरः लतासु विशालानि फलानि रोपयति, विशाले वृक्षे तु लघूनि फलानि। (ii) ___________ पवनस्य वेगेन एकं आम्रफलं तस्य शिरसि अपतत्। सः अचिन्तयत् (iii) ___________ वृक्षे विशालफलम् स्यात् तर्हि मम शिरः तु भग्नम् (iv) ___________ भवेत्। ननु अहमेव मूर्खः, ईश्वरः (v) ___________ बुद्धिमान् अस्ति।
उत्तराणि:
(i) अधः
(ii) सहसा
(iii) यदि
(iv) एव
(v) तु।

प्रश्न 13.
अधोलिखितम् अनुच्छेदम् अव्ययपदैः पूरयत। अव्ययानां सूची अधः प्रदत्ता-

मञ्जूषा – विना, अधुना, सदैव, वृथा, एव, अपि, यत्र-यत्र, इव, कदापि, नूनम्

ते जनाः (i) ___________ धन्याः ये (ii) ___________ निरुत्साहिताः न भवन्ति। ते (iii) ___________ धन्याः, ये (iv) ___________ न वदन्ति। ते (v) ___________ गच्छन्ति तत्र-तत्र साफल्यम् (vi) ___________ न प्राप्नुवन्ति अपितु सूर्यः (vii) ___________ अन्येभ्यः प्रकाशम् (viii) ___________ छन्ति। ते सदैव चिन्तयन्तिकार्यं (ix) ___________ तु जीवनम् एव व्यर्थम्। तेषाम् अस्मिन् कथने एव विश्वासः भवति यत् कार्य तु (x) ___________ एव करणीयम् श्वः न।
उत्तराणि:
(i) नूनम्
(ii) कदापि
(iii) अपि
(iv) वृथा
(v) यत्र-यत्र
(vi) सदैव
(vii) इव
(viii) एव
(ix) विना
(x) अधुना।

प्रश्न 14.
अधोलिखितानि वाक्यानि अव्ययपदैः पूरयत। अव्ययानां सूची अधः मञ्जूषायां प्रदत्ता-

मञ्जूषा – पुनः, इति, अपि, विना, अतः, ह्यः, इतस्ततः, उच्चैः, एव, तत्र-तत्र

(i) ___________ वयं जन्तुशालां द्रष्टुं काननवनम् अगच्छाम। तत्र वयं अनेकान् जन्तून् अपश्याम। सर्वे पशवः (ii) ___________ भ्रमन्ति स्म। सिंहाः (iii) ___________ गर्जन्ति स्म, मयूराः (iv) ___________ नृत्ये मग्नाः आसन्। वस्तुतः मयूरं (v) ___________ कुत्र जन्तुशालायाः शोभा? तत्र आम्रवृक्षाः आसन् (vi) ___________ कोकिलाः अपि। सत्यम् (vii) ___________ यत्र-यत्र आम्रवृक्षाः (viii) ___________ कोकिलाः तु भविष्यन्ति एव। अग्रिमे मासे वयम् (ix) ___________ गमिष्यामः (x) ___________ मया निश्चितम्।
उत्तराणि:
(i) ह्यः
(ii) इतस्ततः
(iii) उच्चैः
(iv) अपि
(v) विना
(vi) अतः
(vii) एव
(viii) तत्र-तत्र
(ix) पुनः
(x) इति।

प्रश्न 15.
अधोलिखितानि वाक्यानि अव्ययपदैः पूरयत। अव्ययानां सूची अधः मञ्जूषायां प्रदत्ता-

अव्ययानां सूची – बहिः, उभयतः, तथा, यथा, तावत्, पत्र, उच्चैः, अधुना, अपि, इव

उपवनं (i) ___________ नदी वहति। कक्षायाः (ii) ___________ मा गच्छ। यावत् कथा श्रूयते (iii) ___________मा गच्छ। (iv) ___________ त्वं चलसि (v) ___________ सा न चलति। मेघाः (vi) ___________ गर्जन्ति। पुरा (vii) ___________ स्रोतः (viii) ___________ तत्र सिकता। किं त्वं (ix) ___________ सा (x) ___________ पठसि।
उत्तराणि:
(i) उभयतः
(ii) बहिः
(iii) तावत्
(iv) यथा
(v) तथा
(vi) उच्चैः
(vii) यत्र
(viii) अधुना
(ix) अपि
(x) इव।

प्रश्न 16.
अधोलिखितानि वाक्यानि अव्ययपदैः पूरयत। अव्ययानां सूची अधः मञ्जूषायां प्रदत्ता-

अव्ययानां सची – खलु, यदा, यावत्, बहिः, तावत्, अपि, तत्र, विना, इव, तदा

त्वं (i) ___________ विद्यालयं गच्छसि (ii) ___________ अध्यापकं पाठयति। विद्या (iii) ___________ संसारे किमपि न लभ्यते। (iv) ___________ विद्या पठ्यते (v) ___________ बालकस्य जीवन सुखमयं भवति। विद्यां प्राप्य मानवाः गृहाद् (vi) ___________ (vii) ___________ गच्छन्ति। (viii) ___________ विदेशेषु भारतीयानां महान् आदरः भवति। (ix) ___________ विद्याधनम् (x) ___________ प्रकाशते।
उत्तराणि:
(i) यदा
(ii) तदा
(iii) विना
(iv) यावत्
(v) तावत्
(vi) बहिः
(vii) अपि
(viii) तत्र
(ix) खलु
(x) इव।

प्रश्न 17.
अधोलिखितानि वाक्यानि अव्ययपदैः पूरयत। अव्ययानां सूची अधः मञ्जूषायां प्रदत्ता-

अव्ययानां सूची। – इतस्ततः, तत्र, परम्, विना, तदैव, यथा, सह, तथा, तु ह्यः

(i) ___________ वयं बुद्धोद्याने भ्रमितुम् अगच्छाम। तत्र वयम् अनेकान् जनान् अपश्याम ये (ii) ___________ अभ्रमन्। (iii) ___________ छात्राः पुस्तकानि अपठन्। उद्यानरक्षक: (iv) ___________पादपेभ्यः जलम् अयच्छत्। (v) ___________ एक: रक्षकः सर्वान् जनान् अपश्यत्। (vi) ___________। एकः भीतः शिशुः तत्र संप्राप्तः। यः मातरं (vii) ___________ व्याकुलः आसीत्। (viii) ___________ सायंकालः अभवत् (ix) ___________ सः अतीव अरोदीत्। आरक्षकः तं स्वेन (x) ___________ अनयत्।
उत्तराणि:
(i) ह्यः
(ii) इतस्ततः
(iii) तत्र
(iv) तु
(v) परम्
(vi) तदैव
(vii) विना
(vii) यथा
(ix) तथा
(x) सह।

प्रश्न 18.
अधोलिखितानि वाक्यानि मञ्जूषायाः सहायतया उचितैः अव्ययपदैः पूरयित्वा उत्तरपुस्तिकायां लिखत-

मञ्जूषा – यावत्-तावत् यत्र-तत्र, यदा-तदा, पुरा-न, ह्यः श्वः

(i) ___________ रविवासरः आसीत, ___________ मङ्गलवासरः भविष्यति।
(ii) ___________ मम माता अनुमतिं दास्यति ___________ अहं नाटकं द्रष्टुं गमिष्यामि।
(iii) ___________ स्थास्यन्ति सरितः महीतले ___________ रामायणी कथा लोकेषु प्रचरिष्यति।
(iv) कालिदासः ___________ अभवत् परं तस्य निश्चितकालं ___________ जाने।
(v) ___________ आम्रवृक्षाः ___________ कोकिलाः सन्ति।
उत्तराणि:
(i) ह्य:-श्वः
(ii) यदा-तदा
(iii) यावत्-तावत्
(iv) पुरा-न
(v) यत्र-तत्र।

प्रश्न 19.
निम्नलिखितवाक्यानि मञ्जूषायाः सहायतया उचितैः अव्ययपदैः पूरयित्वा उत्तरपुस्तिकायां लिखत-

मञ्जूषा – उपरि, अधः, मा, इतस्ततः, विना, उच्चैः, सदैव, अद्य, तत्र-तत्र

(i) ___________ वने पशुमहोत्सवः अस्ति। मञ्चस्य (ii) ___________ वनराजः सिंहः तिष्ठति। आकाशे (iii) ___________ मेघाः सन्ति। मेघान् दृष्ट्वा मयूराः वृक्षाणाम् (iv) ___________ नृत्यन्ति। पशवः (v) ___________ भ्रमन्ति। कोलाहलं श्रुत्वा सिंहः (vi) ___________ गर्जति आदिशति च, “भोः कोलाहलं” (vii) ___________ कुरुत। अस्माकं जीवनं वृक्षान् (viii) ___________ असम्भवम्। वने अस्माभिः (ix) ___________ वृक्षाः रक्षणीयाः। सत्यमुक्तम् यत्र-यत्र धूमः (x) ___________ वह्निः अस्ति।”
उत्तराणि:
(i) अद्य
(ii) उपरि
(iii) सर्वत्र
(iv) अधः
(v) इतस्ततः
(vi) उच्चैः
(vi) मा
(viii) विना
(ix) सदैव
(x) तत्र-तत्र।

प्रश्न 20.
अधोलिखितवाक्यानि मञ्जूषायाः सहायतया अनुच्छेदं उचितैः अव्ययपदैः पूरयित्वा उत्तरपुस्तिकायां लिखत-

मञ्जूषा – सह, उच्चैः, बहिः, इतस्ततः, अपि

नगरात (i) ___________ एकं वनम् अस्ति। तत्र दिने (ii) ___________ अन्धकारः भवति। जनाः प्रायः कुक्कुरैः (iii) ___________ एव तत्र प्रविशन्ति। कुक्कुराश्च जीवान् दृष्ट्वा (iv) ___________शब्दं कुर्वन्ति। गच्छ्त्वा वन्याः पशव: (v) ___________ धावन्ति।
उत्तराणि:
(i) बहि:
(ii) अपि
(iii) सह
(iv) उच्चैः
(v) इतस्ततः।

प्रश्न 21.
मञ्जूषातः उचित-अव्ययपदानि चित्वा रिक्तस्थानानि पूरयत।

मञ्जूषा – नूनम्, उच्चैः, अद्य, बहिः, मा, उपरि, अधः, अपि, अतः, विना, इतस्ततः, च, सर्वत्र।

अनुच्छेदः वने (i) ___________ पशुमहोत्सवः अस्ति। मञ्चस्य (ii) ___________ सिंहः विराजते। आकाशे मेघाः (iii) ___________ सन्ति। मेघान् दृष्ट्वा मयूराः वृक्षाणाम् (iv) ___________ नृत्यन्ति। पशवः (v) ___________ भ्रमन्ति। सर्पः कोटरात् (vi) ___________ आगच्छति। सर्पात् भीताः पश्वः कोलाहलं कुर्वन्ति। कोलाहलं श्रुत्वा सिंहः (vii) ___________ गर्जति आदिशति च-भोः। कोलाहलं (viii) ___________ कुरुत।शृणुत-अस्माकं जीवनं वृक्षान् (ix) ___________ असम्भवम्। वृक्षाः फलानि छायां (x) ___________ दत्वा अस्मान् उपकुर्वन्ति (xi) ___________ अस्माभिः वने (xii) ___________ वृक्षाः रक्षणीयाः।
उत्तराणि:
(i) अद्य
(ii) उपरि
(iii) अपि
(iv) अधः
(v) इतस्ततः
(vi) बहिः
(vii) उच्चैः
(viii) मा
(ix) विना
(x) च
(xi) अतः
(xii) नूनम्।

प्रश्न 22.
(क) मञ्जूषायां दत्तान् शब्दान् संयोज्य कानिचित् वाक्यानि रचयत।

(i) _______________________
(ii) _______________________
(iii) _______________________
(iv) _______________________
(v) _______________________
उत्तराणि:
(i) अहम् ह्यः देवालयम् अगच्छम्।
(ii) अहम् श्वः तरणताले तरिष्यामि।
(iii) अहम् अधुना भोजनं खादामि।
(iv) अहम् यदा-कदा उपवने भ्रमामि।
(v) अहम् पठितुम् विद्यालयम् गच्छामि।

(ख) ‘ह्यः-श्वः’ इति प्रयोगेण शृङ्खलां पूरयत। (ह्यः-श्वः के प्रयोग से श्रृंखला बनाइए)-

(ह्यः अर्थात् बीता हुआ कल तथा श्वः अर्थात् आने वाला कल-इनके प्रयोग से शृंखला को पूरा किया गया है)
यथा-
(i) ह्यः रविवासरः आसीत् श्वः मङ्गलवासरः भविष्यति।
(ii) ___________ श्वः गुरुवासरः भविष्यति।
(iii) ह्यः शुक्रवासरः आसीत् ___________।
(iv) ___________ श्वः शनिवासरः भविष्यति।
(v) ह्यः सोमवासरः आसीत् ___________।
उत्तराणि:
(ii) ह्यः मङ्गलवासरः आसीत्।
(iii) श्वः रविवासरः भविष्यति।
(iv) ह्यः गुरुवासरः आसीत्।।
(v) श्वः बुधवासरः भविष्यति।

(ग) उदाहरणम् अनुसृत्य अव्ययपदैः संवादं पूरयत।

अध्यापकः – भास्कर, कोकिलः कदा कूजति?
भास्करः – यदा वसन्तागमनं भवति तदा कोकिलः कूजति।
अध्यापकः – शोभनम्। कृषकाः कदा नन्दन्ति?
भास्करः – (i) ___________ सुवृष्टिः भवति ___________ कृषकाः नन्दन्ति।
अध्यापकः – साधु! तमः कदा नश्यति?
भास्करः – (ii) ___________ भानुः उदयति ___________ तमः नश्यति।
अध्यापकः – अतिशोभनम्! मयूराः कदा नृत्यन्ति?
भास्करः – (iii) ___________ मेघाः गर्जन्ति ___________ मयूराः नृत्यन्ति।
अध्यापकः – सुष्ठु! मेघाः कदा अपसरन्ति?
भास्करः – (iv) ___________ वातः चलति ___________ मेघाः अपसरन्ति।
उत्तराणि:
(i) यदा, तदा
(ii) यदा, तदा
(iii) यदा, तदा
(iv) यदा, तदा।

(घ) उदाहरणम् अनुसृत्य ‘यावत्-तावत्’ इति युग्मस्य रिक्तस्थानेषु उचितं प्रयोगं कुरुत।

यथा-
(i) यावत् दीयते तावत् धनस्य सदुपयोगः भवति।
(ii) ___________ परोपकारः क्रियते ___________ शरीरस्य सदुपयोगः।
(iii) ___________ स्थास्यन्ति गिरयः ___________ रामायणी कथा प्रचरिष्यति।
(iv) ___________ एव मार्जारः प्रविशति ___________ सर्वे मूषकाः पलायिताः।
उत्तराणि:
(i) यावत्, तावत्
(ii) यावत्, तावत्
(iii) यावत्, तावत्
(iv) यावत्, तावत्।

प्रश्न 23.
उदाहरणम् अनुसृत्य वाक्यपरिवर्तनम् कुरुत। (उदाहरण का अनुसरण करके वाक्य परिवर्तन करें)

उत्तराणि:
(i) मा
(ii) मा
(iii) मा
(iv) मा।

प्रश्न 24.
अधुना ‘इव’ अव्ययस्य प्रयोगेण अधोलिखितपदानां समुचितमेलनं क्रियताम्। (यहाँ ‘इव’ अव्यय के प्रयोग से अधोलिखित पदों का उचित मिलान किया गया है।)-

उत्तराणि:
(ii) करः कमलम् इव
(iii) मुखम् पल्लवः इव
(iv) चरणम् व्याघ्रः इव

प्रश्न 25.
अधोलिखितेषु हास्यालापेषु अव्ययपदानि रेखाङ्कितानि कृत्वा अधः लिखत।

(i) याचकः – श्रेष्ठिन्! एकं पणं देहि।
श्रेष्ठी – अत्र कोऽपि मानवः न अस्ति।
याचकः – कृपया त्वम् एव अस्मिन् समये मानवः भव।
(ii) अनिलः – राजेश! नूनम् आवां मित्रे स्वः। जानासि मित्रस्य लक्षणम्?
राजेशः – सम्यक् जानामि।
अनिलः – अहिं त्वदर्थे मृत्युनापि योद्धं सज्जोऽस्मि। (सहसा व्याघ्रः दृष्टिपथे समायाति)
अनिलः – रे वीर! रक्ष माम्, एष व्याघ्रः मां खादति।
राजेशः – अरे! मया मृत्युना योद्धं कथितं न तु व्याघ्रण।
अव्ययानि –

उत्तराणि:
अत्र, अपि, न, एव, नूनम्, सम्यक्, किम्, कर्तुं, सहसा, योद्ध।

प्रश्न 26.
अव्ययपदानि रेखाङ्कितानि कुरुत।

(i) अलसस्य कुतः विद्या, अविद्यस्य कुतः धनम्।
अधनस्य कुतः मित्रम्, अमित्रस्य कुतः सुखम्।।

(ii) शनैः कन्था शनैः पन्थाः शनैः पर्वतलङ्घनम्।
शनैः विद्या शनैः वित्तम् पञ्चैतानि शनैः शनैः।

(iii) त्वमेव माता च पिता त्वमेव,
त्वमेव बन्धुश्च सखा त्वमेव।
त्वमेव विद्या द्रविणं त्वमेव,
त्वमेव सर्वं मम देवदेव।।

(iv) वृथा वृष्टिः समुद्रेषु, वृथा तृप्तस्य भोजनम्।
वृथा दानं समर्थस्य, वृथा दीपो दिवाऽपि च।।

(v) यावत् स्थास्यन्ति गिरयः सरितश्च महीतले।
तावद् रामायणी कथा, लाकेषु प्रचरिष्यति।।
उत्तराणि:
(i) कुतः
(ii) शनैः
(iii) एव, च
(iv) वृथा च
(v) यावत्, तावत्।

बहुविकल्पीय प्रश्नाः

1. प्रदत्तेषु उत्तरेषु यत् उत्तरम् शुद्धम् अस्ति तत् विकल्पात् चीयताम्।
(दिए गए उत्तरों में से जो उत्तर शुद्ध है उसे विकल्प से चुनिए। Choose the appropriate answer from the options given below.)

प्रश्न 1.
अहं पश्यामि यद् वृद्धाः तु ___________ चलन्ति।
(क) शनैः
(ख) शीघ्रम्
(ग) नीचैः
(घ) नूनम्।
उत्तराणि:
(क) शनैः

प्रश्न 2.
त्वं ___________ कुक्कुरो भव।
(क) सदा
(ख) पुनः
(ग) कदापि
(घ) अपि।
उत्तराणि:
(घ) अपि।

प्रश्न 3.
तत्र वृक्षेषु वानराः ___________ कूर्दन्ति।
(क) इतस्ततः
(ख) सर्वत्र
(ग) इत्यत्र
(घ) इदानीम्।
उत्तराणि:
(क) इतस्ततः

प्रश्न 4.
मूर्खाः जनाः संसारे ___________ जल्पन्ति।
(क) एव
(ख) बहुधा
(ग) अपि
(घ) वृथा।
उत्तराणि:
(घ) वृथा।

प्रश्न 5.
पश्य, ___________ मयूराः अपि नृत्यन्ति परं तत्र शुकाः वदन्ति।
(क) कदा
(ख) अत्र
(ग) तदा
(घ) यथा।
उत्तराणि:
(ख) अत्र

प्रश्न 6.
वृक्ष शाखासु कोकिला: ___________ मधुरं गायन्ति।
(क) अपि
(ख) नैव
(ग) ह्यः
(घ) एव।
उत्तराणि:
(क) अपि

प्रश्न 7.
___________ गृहं गच्छावः श्वः पुनः आगमिष्यावः।
(क) ह्यः
(ख) श्वः
(ग) अधुना
(घ) वृथा।
उत्तराणि:
(ग) अधुना

प्रश्न 8.
यत्र कमलानि ___________ भ्रमराः।
(क) तत्र
(ख) यत्र-तत्र
(ग) अत्र-तत्र
(घ) यथा।
उत्तराणि:
(क) तत्र

प्रश्न 9.
यथा राजा ___________ प्रजा।
(क) यथा-तथा
(ख) तथा
(ग) तथा-यथा
(घ) यत्र।
उत्तराणि:
(ख) तथा

प्रश्न 10.
अहं ___________ तत्र गमिष्यामि।
(क) कदा
(ख) अपि
(ग) ह्यः
(घ) पुरा।
उत्तराणि:
(ख) अपि

प्रश्न 11.
अहं ___________ तत्र न अगच्छम्।
(क) श्वः
(ख) अधुना
(ग) इव
(घ) सहसा।
उत्तराणि:
(ख) अधुना

प्रश्न 12.
ते ___________ एव अत्रागमिष्यन्ति।
(क) अधुना
(ख) ह्यः
(ग) श्वः
(घ) नूनम्।
उत्तराणि:
(घ) नूनम्।

प्रश्न 13.
___________ एकं विशालं भवनम् अस्ति।
(क) तत्र
(ख) उपरि
(ग) नीचैः
(घ) अत्र-तत्र।
उत्तराणि:
(क) तत्र

प्रश्न 14.
___________ एक: जलाशयः आसीत्।
(क) उपरि
(ख) नीचैः
(ग) अत्र
(घ) पुरा।
उत्तराणि:
(घ) पुरा।

प्रश्न 15.
त्वम् ___________ पठ।
(क) अपि
(ख) तदा
(ग) न
(घ) नूनम्।
उत्तराणि:
(क) अपि

प्रश्न 16.
छात्राः ___________ किं करिष्यन्ति?
(क) ह्यः
(ख) सहसा
(ग) इदानीम्
(घ) वृथा।
उत्तराणि:
(ग) इदानीम्

प्रश्न 17.
___________ अशोक: नाम महान् सम्राट् आसीत्।
(क) पुरा
(ख) अधुना
(ग) तदा
(घ) कदा।
उत्तराणि:
(क) पुरा

प्रश्न 18.
___________ ईश्वरभक्ताः तादृशाः न सन्ति।
(क) ह्यः
(ख) अधुना
(ग) श्वः
(घ) शनैः।
उत्तराणि:
(ख) अधुना

प्रश्न 19.
___________ वर्षा भवति तथा एव कृषकाः मोदन्ते।
(क) प्रातः
(ख) यदा
(ग) सदा
(घ) यथा।
उत्तराणि:
(घ) यथा।

प्रश्न 20.
यथा देश: ___________ वेशः।
(क) तदा
(ख) यदा
(ग) तथा
(घ) तत्र।
उत्तराणि:
(ग) तथा

प्रश्न 21.
ते पर्वतात् ___________ न सन्ति।
(क) उपरि
(ख) तत्र
(ग) अधः
(घ) उच्चैः।
उत्तराणि:
(घ) उच्चैः।

प्रश्न 22.
विद्यालयस्य छात्राः कथम् ___________ धावन्ति?
(क) यथा-तथा
(ख) विना
(ग) इतस्ततः
(घ) अपि।
उत्तराणि:
(ग) इतस्ततः

प्रश्न 23.
दुष्टाः ___________ एव जल्पन्ति।
(क) वृथा
(ख) इतस्ततः
(ग) तत्र
(घ) शनैः।
उत्तराणि:
(क) वृथा

प्रश्न 24.
त्वमपि रामम् ___________ भव।
(क) अधुना
(ख) सहसा
(ग) पुरा
(घ) इव।
उत्तराणि:
(घ) इव।

प्रश्न 25.
वृद्धा ___________ स्वकार्याणि करोति।
(क) नूनम्
(ख) शनैः
(ग) एव
(घ) इदानीम्।
उत्तराणि:
(ख) शनैः

प्रश्न 26.
संस्कारात्। ___________ जनः द्विज उच्यते।
(क) एव
(ख) अपि
(ग) इव
(घ) अत्र-तत्र।
उत्तराणि:
(क) एव

प्रश्न 27.
चन्द्रगुप्तः सम्राट् चाणक्यं ___________ किमपि न अकरोत्।
(क) पुरा
(ख) विना
(ग) इतस्ततः
(घ) सहसा।
उत्तराणि:
(ख) विना

प्रश्न 28.
वेदमन्त्राणाम् उच्चारणम् ___________ कर्तव्यम्।
(क) इव
(ख) अपि
(ग) एव
(घ) उच्चैः।
उत्तराणि:
(घ) उच्चैः।

प्रश्न 29.
यथा पिता पुत्रोऽपि ___________ एव दृश्यते।
(क) तदा
(ख) इतस्ततः
(ग) तथा
(घ) इदानीम्।
उत्तराणि:
(ग) तथा

प्रश्न 30.
___________ त्वम् स्वकार्यं कुरु।
(क) विना
(ख) इदानीम्
(ग) वृथा
(घ) शनैः।
उत्तराणि:
(ख) इदानीम्

प्रश्न 31.
इदं कार्यं त्वया ___________ कर्तव्यम्।
(क) एव
(ख) विना
(ग) नूनम्
(घ) वृथा।
उत्तराणि:
(क) एव

प्रश्न 32.
अभिमन्युः तदा ___________ अवदत्।
(क) एव
(ख) कथम्
(ग) इव
(घ) अधुना।
उत्तराणि:
(क) एव

प्रश्न 33.
दुष्टैः सह समयं ___________ न करणीयम्।
(क) विना
(ख) अधुना
(ग) सहसा
(घ) वृथा।
उत्तराणि:
(घ) वृथा।

प्रश्न 34.
अहम् ___________ तव स्वामी अतः ममाज्ञा पालनीया।
(क) अपि
(ख) सहसा
(ग) एव
(घ) वृथा।
उत्तराणि:
(ग) एव

प्रश्न 35.
चिकित्सालये ___________ न वक्तव्यम्।
(क) उच्चैः
(ख) वृथा
(ग) एव
(घ) सम्प्रति।
उत्तराणि:
(क) उच्चैः

प्रश्न 36.
मम मातामही ___________ वृद्धा अस्ति।
(क) शनैः
(ख) अपि
(ग) उच्चैः
(घ) वृथा।
उत्तराणि:
(ख) अपि

प्रश्न 37.
दुष्टानां जन्म ___________ वृथा भवति।
(क) एव
(ख) शनैः
(ग) इव
(घ) सहसा।
उत्तराणि:
(क) एव

प्रश्न 38.
___________ मया पित्राज्ञा पालनीया।
(क) इदानीम्
(ख) पुरा
(ग) यथा
(घ) तत्र।
उत्तराणि:
(क) इदानीम्

प्रश्न 39.
जगति ___________ पितरौ कुरुतः ___________ एव सन्ततयः कुर्वन्ति।
(क) यथा-तथा
(ख) अत्र-तत्र
(ग) कुत्र-यत्र
(घ) कुतः-ततः।
उत्तराणि:
(क) यथा-तथा

प्रश्न 40.
___________ विदधीत न क्रियाम्।
(क) वृथा
(ख) सहसा
(ग) इतस्ततः
(घ) पुरा।
उत्तराणि:
(ख) सहसा

प्रश्न 41.
जीवः जीवस्य ___________ भोजनं भवति।
(क) एव
(ख) विना
(ग) अपि
(घ) वृथा।
उत्तराणि:
(क) एव

प्रश्न 42.
छात्राणां कृते ___________ कार्यं नोचितम्।
(क) उच्चैः
(ख) शनैः
(ग) अधुना
(घ) सहसा।
उत्तराणि:
(ख) शनैः

प्रश्न 43.
सर्वे जीवाः मिलित्वा ___________ मोदन्ते।
(क) इदानीम्
(ख) पुरा
(ग) शनैः
(घ) उच्चैः।
उत्तराणि:
(क) इदानीम्

प्रश्न 44.
छात्राः सम्प्रति ___________ कथं भ्रमन्ति?
(क) अधुना
(ख) इतस्ततः
(ग) पुरा
(घ) शनैः।
उत्तराणि:
(ख) इतस्ततः

प्रश्न 45.
द्वारपाल: ___________ अपि तत्र तिष्ठति।
(क) अधुना
(ख) नीचैः
(ग) शनैः
(घ) उच्चैः।
उत्तराणि:
(क) अधुना

प्रश्न 46.
ईश्वरेण दत्तम् इदं जीवन ___________ न कर्तव्यम्।
(क) शनैः
(ख) उच्चैः
(ग) नूनम्
(घ) वृथा।
उत्तराणि:
(घ) वृथा।

प्रश्न 47.
अलङ्कारैः विभूषितः ___________ गुणहीन: न शोभते।
(क) अपि
(ख) एवम्
(ग) पुरा
(घ) एव।
उत्तराणि:
(क) अपि

प्रश्न 48.
___________ तुभ्यं रोचते त्वं तथैव कुरु।
(क) तत्र
(ख) अत्र
(ग) यथा
(घ) परम्।
उत्तराणि:
(ग) यथा

प्रश्न 49.
जनानां समृद्धिः ___________ संवर्धिता वर्तते।
(क) अधुना
(ख) ह्यः
(ग) पुरा
(घ) इव।
उत्तराणि:
(क) अधुना

प्रश्न 50.
यात्रायां ___________ कोलाहलं न कर्तव्यम्।
(क) सहसा
(ख) वृथा
(ग) इव
(घ) शनैः।
उत्तराणि:
(ख) वृथा

प्रश्न 51.
___________ भारतम् अतीव समृद्धम् आसीत्।
(क) अधुना
(ख) इतस्ततः
(ग) पुरा
(घ) शनैः।
उत्तराणि:
(ग) पुरा

प्रश्न 52.
वर्तमानकाले ___________ सर्वत्र विलासिता एव दृश्यते।
(क) यथा-तथा
(ख) सम्प्रति
(ग) अत्र-तत्र
(घ) शनैः।
उत्तराणि:
(ग) अत्र-तत्र

प्रश्न 53.
त्वम् ___________ बन्धुः च सखा त्वमेव।
(क) एव
(ख) अपि
(ग) नूनम्
(घ) सहसा।
उत्तराणि:
(क) एव

प्रश्न 54.
धनाभावे रोदनं ___________ भवति।
(क) वृथा
(ख) एव
(ग) नूनम्
(घ) इतस्ततः।
उत्तराणि:
(क) वृथा

प्रश्न 55.
शनैः कन्थाः ___________ पन्थाः शनैः पर्वत लङ्घनम्।
(क) अधुना
(ख) उच्चैः
(ग) विना
(घ) शनैः।
उत्तराणि:
(घ) शनैः।

प्रश्न 56.
दैत्यानाम् ___________ पर्वताः दूरतः एव भयातुरम् कुर्वन्ति।
(क) शनैः
(ख) सहसा
(ग) इव
(घ) इदानीम्।
उत्तराणि:
(ग) इव

प्रश्न 57.
मधुरा दिव्या वाणी ___________ गीर्वाण कथ्यते।
(क) एव
(ख) नूनम्
(ग) पुरा
(घ) इव।
उत्तराणि:
(क) एव

प्रश्न 58.
भारतभूमिः ___________ दर्शनीया अस्ति।
(क) सम्प्रति
(ख) इतस्ततः
(ग) नूनम्
(घ) विना।
उत्तराणि:
(ग) नूनम्

प्रश्न 59.
___________ कृतं कार्यं नूनं कष्टकरं भवति।
(क) शनैः
(ख) सहसा
(ग) इव
(घ) पुरा।
उत्तराणि:
(ख) सहसा

प्रश्न 60.
श्रीरामम् उपेत्य भरतः ___________ अवदत्।
(क) अपि
(ख) वृथा
(ग) उच्चैः
(घ) इदानीम्।
उत्तराणि:
(ग) उच्चैः

प्रश्न 61.
लङ्लकारस्य प्रयोगः भूतकाले ___________ भवति।
(क) अधुना
(ख) विना
(ग) एव
(घ) अपि।
उत्तराणि:
(ग) एव

प्रश्न 62.
श्रीरामः यथा आदर्शपुत्रः आसीत् ___________ एव आदर्शपिता अपि।
(क) तत्र
(ख) तथा
(ग) यत्र
(घ) सहसा।
उत्तराणि:
(ख) तथा

प्रश्न 63.
संसारे सूर्यः ___________ जीवनाधारः वर्तते।
(क) अधुना
(ख) एव
(ग) पुरा
(घ) अपि।
उत्तराणि:
(ख) एव

प्रश्न 64.
___________ सूर्यः जगत् प्रकाशयति ___________ चन्द्रः अपि।
(क) अत्र-तत्र
(ख) यथा-तथा
(ग) यत्र-तत्र
(घ) इत:-ततः।
उत्तराणि:
(ख) यथा-तथा

प्रश्न 65.
त्वया ___________ कोलाहलं परित्यज्य सुप्तव्यम्।
(क) अधुना
(ख) शनैः
(ग) उच्चैः
(घ) सहसा।
उत्तराणि:
(क) अधुना

प्रश्न 66.
प्राणं ___________ कथं जीवनम्?
(क) शनैः
(ख) विना
(ग) इदानीम्
(घ) वृथा।
उत्तराणि:
(ख) विना

प्रश्न 67.
क्रीडाङ्गणे ___________ रामः तु तत्र कृष्ण: वर्तेते।
(क) तथा
(ख) यथा
(ग) यथा-तथा
(घ) यत्र।
उत्तराणि:
(घ) यत्र।

प्रश्न 68.
स्वर्णशलाकाम् ___________ तत् विभाति।
(क) इव
(ख) पुरा
(ग) इदानीम्
(घ) नूनम्।
उत्तराणि:
(क) इव

प्रश्न 69.
रात्रौ कुक्कुराः ___________ भाषन्ते।
(क) पुरा
(ख) इतस्ततः
(ग) इदानीम्
(घ) विना।
उत्तराणि:
(ख) इतस्ततः

प्रश्न 70.
___________ कलियुगस्य सप्तविंशतितमा चतुर्युगी वर्तते।
(क) पुरा
(ख) यथा
(ग) तथा
(घ) इदानीम्।
उत्तराणि:
(घ) इदानीम्।

प्रश्न 71.
वर्षायां मेघाः ___________ गर्जन्ति।
(क) नीचैः
(ख) उच्चैः
(ग) अधुना
(घ) इव।
उत्तराणि:
(ख) उच्चैः

प्रश्न 72.
अर्जुनेन सह कृष्णः ___________ आसीत्।
(क) अपि
(ख) नूनम्
(ग) वृथा
(घ) विना।
उत्तराणि:
(क) अपि

प्रश्न 73.
परीक्षाकाले ___________ शीतः भविष्यति एव।
(क) अधुना
(ख) इतस्ततः
(ग) अपि
(घ) इव।
उत्तराणि:
(ग) अपि

प्रश्न 74.
रात्रौ तु चन्द्रः वर्तते ___________ न।
(क) एव
(ख) नूनम्
(ग) इदानीम्
(घ) सम्प्रति।
उत्तराणि:
(ग) इदानीम्

प्रश्न 75.
त्वाम् ___________ को उद्धरिष्यति माम्?
(क) एव
(ख) विना
(ग) इतस्ततः
(घ) पुरा।
उत्तराणि:
(ख) विना

प्रश्न 76.
प्रातः उद्याने ___________ न चलितव्यम्।
(क) उच्चैः
(ख) नूनम्
(ग) शनैः
(घ) इदानीम्।
उत्तराणि:
(ग) शनैः

प्रश्न 77.
धरायाम् ___________ सर्वे जीवाः संवसन्ति।
(क) अपि
(ख) एव
(ग) अत्र-तत्र
(घ) इदानीम्।
उत्तराणि:
(ख) एव

प्रश्न 78.
सहसा उत्थाय भीमः ___________ अवदत्।
(क) शनैः
(ख) एव
(ग) उच्चैः
(घ) विना।
उत्तराणि:
(ग) उच्चैः

प्रश्न 79.
केनचिदपि समय ___________ न कर्तव्यम्।
(क) नूनम्
(ख) वृथा
(ग) विना
(घ) सहसा।
उत्तराणि:
(ख) वृथा

प्रश्न 80.
ईश! ___________ त्वमेव मम पिता वर्तसे।
(क) वृथा
(ख) पुरा
(ग) अपि
(घ) नूनम्।
उत्तराणि:
(घ) नूनम्।

प्रश्न 81.
___________ वृथा भ्रमतां छात्राणां संस्काराः हसन्ति।
(क) इतस्ततः
(ख) नूनम्
(ग) सहसा
(घ) शनैः।
उत्तराणि:
(क) इतस्ततः

प्रश्न 82.
___________ देवासुर-संग्रामोऽभवत्।
(क) सम्प्रति
(ख) इदानीम्
(ग) पुरा
(घ) अधुना।
उत्तराणि:
(ग) पुरा

प्रश्न 83.
___________ जनाः परिश्रमेण कार्यं करिष्यन्ति तावत् सुखानि प्राप्स्यन्ति।
(क) यावत्
(ख) तावत्
(ग) इदीनीम्
(घ) अधुना।
उत्तराणि:
(क) यावत्

प्रश्न 84.
यावत् जगति सदाचारः आचरिष्यति ___________ संसारे आनन्दं भविष्यति।
(क) एव
(ख) पुरा
(ग) तावत्
(घ) अधुना।
उत्तराणि:
(ग) तावत्

2. मञ्जूषायां प्रदत्तैः अव्ययैः सहायतया वाक्यानां रिक्तस्थानानि पूरयत।
(मञ्जूषा में दिए गए अव्ययों की सहायता से वाक्यों के रिक्त स्थानों की पूर्ति कीजिए। Fill in the blanks with the help of indeclinables given in the box.)

प्रश्न 1.
(क) तस्याः मुखम् चन्द्रम् इव ___________ न प्रतीयते।
(ख) ___________ रामकथा समाप्यते।
(ग) रामः श्याम: च ___________ पठतः?
(घ) ___________ कः तिष्ठति?
(ङ) वृथा ___________ वद।

मञ्जूषा – बहिः, मा, कुत्र, कदापि, इति।

उत्तराणि:
(क) कदापि
(ख) इति
(ग) कुत्र
(घ) बहिः
(ङ) मा।

प्रश्न 2.
(क) त्वम् ___________ तेन सह गच्छ।
(ख) तस्य मुखम् कमलम् इव ___________ नास्ति।
(ग) त्वम् दिल्लीनगरम् ___________ गमिष्यसि?
(घ) यत् वस्तु लाभदायकम् ___________ तत् एव आनय।
(ङ) अहम् ___________ पठिष्यामि।

मञ्जूषा – सम्प्रति, इति, कदा, कदापि, श्वः।

उत्तराणि:
(क) सम्प्रति
(ख) कदापि
(ग) कदा
(घ) इति
(ङ) श्वः

प्रश्न 3.
(क) सः ___________ एव अत्र आगच्छत्।
(ख) ___________ अहम् आगमिष्यामि तावत् त्वम् अत्र उपविश।
(ग) अहम् तु पठामि परं त्वम् ___________ न पठसि?
(घ) श्यामः अवदत् ___________ त्वं यत् पश्यसि तत् एव वद।
(ङ) त्वम् ___________ पठिष्यसि?

मञ्जूषा – किमर्थम्, यावत्, कुतः, ह्यः, यत्, कदा।

उत्तराणि:
(क) ह्यः
(ख) यावत्
(ग) किमर्थम्
(घ) यत्
(ङ) कदा।

प्रश्न 4.
(क) कुम्भं करोति ___________ कुम्भकारः।
(ख) असत्यं ___________ वद।
(ग) त्वया ___________ आयातः?
(घ) अहम् ___________ अगच्छम्? इति न जानामि।
(ङ) ___________ अहम् लिखामि तावत् त्वम् पठ।

मञ्जूषा – मा, यावत्, कदा, कुतः, इति।

उत्तराणि:
(क) इति
(ख) मा
(ग) कदा
(घ) कुतः
(ङ) यावत्।

प्रश्न 5.
(क) सः आत्मानम् रामः ___________ मानयति।
(ख) एषः सिंहः ___________ आगच्छत्?
(ग) विद्यालयात् ___________ मा गच्छ।
(घ) ___________ त्वम् कुत्र आसी:?
(ङ) ___________ इतस्ततः भ्रमणं मा कुरु।

मञ्जूषा – इति, सम्प्रति, कुतः, बहिः, ह्यः।

उत्तराणि:
(क) इति
(ख) कुतः
(ग) बहिः
(घ) ह्यः
(ङ) सम्प्रति।

प्रश्न 6.
(क) अहम् ___________ विद्यालयं न आगमिष्यामि।
(ख) ___________ अवकाशः भविष्यति।
(ग) मृषा ___________ वद।
(घ) धनात् विना ___________ सुखम्?
(ङ) त्वम् तत्र ___________ गच्छसि?

मञ्जूषा – किमर्थम्, श्वः, कुत, मा, श्वः।

उत्तराणि:
(क) श्वः
(ख) श्वः
(ग) मा
(घ) कुतः
(ङ) किमर्थम्।

प्रश्न 7.
(क) सः ___________ आगमिष्यति।
(ख) त्वं ___________ मृषा मा वद।
(ग) ग्रामात् ___________ देवालयः अस्ति।
(घ) त्वम् असत्यं ___________ वद।
(ङ) ___________ मम अध्यापक: न अपाठयत्।

मञ्जूषा – श्वः, कदापि, ह्यः, मा, बहिः।

उत्तराणि:
(क) श्वः
(ख) कदापि
(ग) बहिः
(घ) मा
(ङ) ह्यः।

प्रश्न 8.
(क) विद्यालये ___________ वनमहोत्सवः मान्यते।
(ख) छात्रैः गृहेभ्यः ___________ एकः पादपः आनीतः।
(ग) छात्रैः आनीतेषु पादपेषु फलिनः वृक्षाः विद्यालयात् ___________ रोपणीयाः।
(घ) ___________ वृक्षाः सन्ति तत्र दुर्दिवसा: ___________?
(ङ) एतेषु वृक्षेषु फलानि ___________ न आगमिष्यन्ति।

मञ्जूषा – यदा-कदा, कदापि, ह्यः, यत्र-कुत्र, बहिः।

उत्तराणि:
(क) कदा
(ख) ह्यः
(ग) बहिः
(घ) यत्र-कुत्र
(ङ) कदापि।

प्रश्न 9.
(क) ___________ मम मातुलः काश्मीरात् आगमिष्यति।
(ख) असत्यं ___________ वद।
(ग) ___________ स्थास्यन्ति गिरयः तावत् रामायणी कथा प्रचरिष्यति।
(घ) कच्छप: ___________ न धावति।
(ङ) अस्माकं जीवन ___________ वर्तते?

मञ्जूषा – यावत्, श्वः, मा, कदापि, किमर्थम्।

उत्तराणि:
(क) श्वः
(ख) मा
(ग) यावत्
(घ) कदापि
(ङ) किमर्थम्।

प्रश्न 10.
(क) त्वम् एवं ___________ वद।
(ख) सः ___________ असत्यं न अवदत्।
(ग) ___________ शीतकालो वर्तते।
(घ) ___________ मम परीक्षा भविष्यति।
(ङ) सः ___________ पठति इति न ज्ञायते।

मञ्जूषा – कदापि, कदा, श्वः मा, सम्प्रति।

उत्तराणि:
(क) मा
(ख) कदापि
(ग) सम्प्रति
(घ) श्वः
(ङ) कदा।

प्रश्न 11.
ते जनाः (i) ___________ धन्याः ये (ii) ___________ निरुत्साहिताः न भवन्ति। ते (iii) ___________ न धन्याः, ये (iv) ___________ सत्यं वदन्ति। ते (v) ___________ गच्छन्ति, साफल्यम् प्राप्नुवन्ति। ते सदैव चिन्तयन्ति इति कार्यं विना तु जीवनम् एव व्यर्थम्।

मञ्जूषा – यत्र-कुत्र, सम्प्रति, कदापि, यदा-कदा, कदापि।

उत्तराणि:
(i) सम्प्रति
(ii) कदापि
(iii) कदापि
(iv) यदा-कदा
(v) यत्र-कुत्र।

प्रश्न 12.
(i) ___________ वयं जन्तुशालां द्रष्टुं नन्दन काननवनम् अगच्छाम। तत्र वयं अनेकान् जन्तून् अपश्याम। सर्वे पशवः (ii) ___________ भ्रमन्ति स्म। सिंहाः(iii) ___________ नासन्। मयूराः अपि (iv) ___________ अनृत्यत्। वस्तुतः मयूरं विना (v) ___________ जन्तुशालायाः शोभा? अग्रिमे मासे वयम् पुन: गमिष्यामः।

मञ्जूषा – ह्यः, यत्र-कुत्र, बहिः, दा-कदा, कुतः।

उत्तराणि:
(i) ह्यः
(ii) यत्र-कुत्र
(iii) बहिः
(iv) यदा-कदा
(v) कुतः।

प्रश्न 13.
(क) ___________ कथा श्रूयते तावत् मा गच्छ।
(ख) ___________ एव अहं वार्तालापं करोमि।
(ग) ___________ सा न चलति।
(घ) मेघाः ___________ गर्जन्ति।
(ङ) सः ___________ अस्मत् स्थानात् गतः।

मञ्जूषा – यदा-कदा, कदा, यावत्, सम्प्रति, यत्र-कुत्र।

उत्तराणि:
(क) यावत
(ख) यदा-कदा
(ग) सम्प्रति
(घ) यत्र-कुत्र
(ङ) कदा।

प्रश्न 14.
(क) त्वं ___________ विद्यालयं गच्छसि?
(ग) विद्याध्ययनं ___________ तपः कुर्यात्।
(ख) ___________ अध्यापकः पाठयति।
(घ) ___________ विद्या पठ्यते तावत् छात्राः तपन्ति।
(ङ) ___________ जीवनं सुखमयं भवति।

मञ्जूषा – कदा, इति, यावत्, सम्प्रति, यावत्।

उत्तराणि:
(क) कदा
(ख) सम्प्रति
(ग) यावत्
(घ) यावत्
(ङ) इति।

3. तालिकाप्रदत्तपदानि संयोज्य षट्वाक्यानि रचयत।
(तालिका में दिए गए पदों को जोड़कर छह वाक्य बनाइए। Frame six sentences with the help of the words given in the table.)

प्रश्न 1.

उत्तराणि:

प्रश्न 2.

उत्तराणि:

प्रश्न 3.

उत्तराणि:

प्रश्न 4.

उत्तराणि:

प्रश्न 5.

उत्तराणि:

By going through these CBSE Class 11 Maths Notes Chapter 5 Complex Numbers and Quadratic Equations Class 11 Notes, students can recall all the concepts quickly.

Complex Numbers and Quadratic Equations Notes Class 11 Maths Chapter 5

Imaginary Numbers: A number whose square is negative, is known as an imaginary number, e.g. : \(\sqrt{-1}, \sqrt{-2}\), etc.
\(\sqrt{-1}\) is denoted by the Greek letter i (iota).

Powers of i : i¹ = – 1; i²= – 1; i³ = — i, i⁴ = 1, etc.

An Important Result : For any two real numbers a and b,
\(\sqrt{a} \times \sqrt{b}=\sqrt{a b}\) is true only when at least one of a and b is either 0 or positive.
In fact, \(\sqrt{-a} \times \sqrt{-b}=(i \sqrt{a})(i \sqrt{b})=i^{2} \sqrt{a b}=-\sqrt{a b}\), where a and b are positive real numbers.

Complex Number : Any number of the form x + iy, where x, y are real numbers and i \(\sqrt{-1}\) is called a complex number.

It is denoted by z, i.e., z = x + iy. The real and imaginary parts of a complex number z are denoted by Re(z) and Im(z) respectively. Thus, if z = x + iy, then Re(z) = x and Im(z) = y.

Note : Every real number is a complex number, for if a e R, it can be written as a – a + i0.

Purely Real and Purely Imaginary Numbers : A complex number z is said to be
(i) purely real, if Im(z) = 0.
(ii) purely imaginary, if Re(z) = 0.

Conjugate of a Complex Number : The conjugate of a complex number z, denoted by \(\bar{z}\) is the complex number obtained by changing the sign of imaginary part of z i.e., if z = a + ib, then \(\bar{z}\) = a-ib.

Modulus of a Complex Number: The modulus of a complex number, z- a + ib, denoted by |z |, is defined as |z | = \(\sqrt{x^{2}+y^{2}}\)

Sum, Difference and Product of Complex Numbers : For any complex numbers z₁ = a + ib and z₂ = c + id, it is defined as
(i) Z₁ + z₂ = (a + ib) + (c + id) = (a + c) + i(b + d).
(ii) z₁ – z₂ = (a + ib) – (c + id) = (a -c) + i(b – d).
(iii) z₁z₂ = (a + ib)(c + id) = (ac -bd) + i(ad – bc).

Properties of Complex Numbers:

1. A real number can never be equal to an imaginary number.
2. (a + ib) = 0 ⇔ a = 0 and 6 = 0.
3. Two complex numbers are equal only when their real and imaginary parts are separately equal.
i.e., if a + ib = c + id, then a = c and b = d.

4. If z is a complex number, then :
(i) (\(\bar{z}\)) = z
(ii) (z + \(\bar{z}\)) is real.
(iii) (z – \(\bar{z}\)) is zero or an imaginary number.
(iv) z + \(\bar{z}\) = 2Re(z) and (z – \(\bar{z}\)) = 2iIm(z).
(v) z\(\bar{z}\) – |z |² and, therefore, z\(\bar{z}\) is real.

5. If z₁ and z₂ are complex numbers, then :
(i) \(\left(\overline{z_{1}+z_{2}}\right)=\overline{z_{1}}+\overline{z_{2}}\)
(ii) \(\left(\overline{z_{1}-z_{2}}\right)=\overline{z_{1}}-\overline{z_{2}}\)
(iii) \(\left(\overline{z_{1} z_{2}}\right)=\overline{z_{1}} \cdot \overline{z_{2}}\)
(iv) \(\left|z_{1} z_{2}\right|=\left|z_{1}\right|\left|z_{2}\right|\)

6. For any complex number z, |z | = 0 ⇔ z = 0.
7. The sum and product of two complex numbers are real if and only if they are conjugate of each other.
8. The conjugate and the reciprocal of a non-zero complex number z are equal if and only if | z | = 1.
9. Reciprocal or multiplicative inverse of a + ib
= \(\frac{1}{a+i b}=\frac{1}{a+i b} \times \frac{a-i b}{a-i b}=\frac{a}{a^{2}+b^{2}}-i\left(\frac{b}{a^{2}+b^{2}}\right)\)

10. For any integer k,
(i) i^2k = (-1)^k , i^{2k+ 1} = (- 1)^ki
(ii) i^4k = (i⁴)^k = 1, i^{4k+ 1} = i^4k+2 = i² = -1,
i^4k+3 = i³ = i² x i = -i

Geometrical Representation of a Complex Number : To every complex number z = x + iy, there is one and only one point P(x, y) in the plane, and conversely to every point (x,y) in the plane, there is one and only one complex number z = x + iy.

The plane, representing the complex numbers, as an ordered pair of real numbers is called the Complex plane or Gaussian plane or Argand plane.

Modulus and Amplitude of a Complex Number : Let a poin^ P represents a complex number z = x + iy in the complex plane. Then, the length OP is called the modulus of the complex number z and is denoted by |z|.

∴ |z| = OP = |x + iy|
= \(\sqrt{x^{2}+y^{2}}\) .

The angle XOP = θ is called the amplitude or argument of 2 and is written as amp. z or arg. z. The value of θ satisfying – π < θ < π is called the principal value of amp z.

Polar Form of a Complex Number : Let z – a + ib be represented by P(a, b).
Let OP = r, ∠XOP = θ.
Then, a – rcos θ,
b = rsinθ
= r² = a² + b²
⇒ r = |2|
and tanθ = \(\frac{b}{a}\)
⇒ cosθ = \(\frac{a}{r}\) ,sinθ = \(\frac{b}{r}\).
The form 2 = r(cos θ + isin θ) is called the Polar Form.

Polynomial Equation : A polynomial equation of a degree x has n roots.

Solutions of Quadratic Equation: The solutions of quadratic equation
ax² + bx + c = 0,
where a, b, c ∈ R, a ≠ 0, b2² – 4ac < 0 are given by
x = \(\frac{-b \pm\left(\sqrt{4 a c-b^{2}}\right) i}{2 i}\)

D = b² – 4ac is called the discriminant of the quadratic equation.

By going through these CBSE Class 11 Maths Notes Chapter 4 Principle of Mathematical Induction Class 11 Notes, students can recall all the concepts quickly.

Principle of Mathematical Induction Notes Class 11 Maths Chapter 4

Mathematical induction is a technique for proving general results or theorem involving positive integers.

The word induction means the method of inferring a general statement from the validity of particular cases.

Statement : A sentence which can be judged to be true or false is called a statement.
A statement holding for n ∈ N is generally denoted by P(n).

Principle of mathematical induction : Let P(n) be a statement involving the natural number n. Then,
(i) P( 1) is true and
(ii) P(k + 1) is true whenever P(k) is true, then P(n) is true for all natural numbers n.

For proving that statement P(n) holds for all n ∈ N :
Following steps are applied :

Step 1 : Verification that P(n.) holds for n = 1, i.e., P( 1) is true.
Step 2 : Suppose that P(re) holds for every k ∈ N.
Step 3 : Prove that P(n) holds for n = k + 1 also.

By going through these CBSE Class 11 Maths Notes Chapter 3 Trigonometric Functions Class 11 Notes, students can recall all the concepts quickly.

Trigonometric Functions Notes Class 11 Maths Chapter 3

Important Definitions:

Angle: An angle is considered as a figure obtained by rotating a given ray about its initial point. The original position of the ray is called the initial side and the final position is called the terminal side of the angle. The point of rotation is called the vertex.

Positive Angle : If the direction of the rotation is anticlockwise, the angle is said to be positive.

Negative Angle : If the direction of the rotation is clockwise, the angle is said to be negative.

Degree measure of an angle: If a rotation from initial side of the terminal side is (\(\frac{1}{360}\))th of a revolution, the angle is said to have a measure of one degree, written as 1°.

1° = 60′ [One degree = 60 minutes]
1′ = 60″ [One minute = 60 seconds]

Radian Measure : An angle with its vertex at the centre of a circle which intercepts an arc equal in length to the radius of the circle is said to have a measure of 1 radian.

Important Formulae:

1. θ = \(\frac{l}{r}\)
   where θ = angle subtended by arc of length l at the centre of the circle and
   r = radius of the circle.
2. π radians = 180°.
3. 1 radian = \(\left(\frac{180}{\pi}\right)^{\circ}\)
4. 1° = radian = 0.01746 (app.)

Circular Functions: Let a circle of unit radius be drawn with centre O. A line segment OP = 1 is rotated from the initial position OX, in the anticlockwise direction.

Let OP makes an angle θ with OX. The x-coordinate of P gives the value of cos θ and y – coordinate will give the value of sin θ. P may be any where on the circle and hence θ may acquire any value from 0 to 2π radians. Thus, we get the value of cos θ and sin θ for any value of θ.
These trigonometric functions are known as circular functions.

Important Formulae :

1. sin² θ – co² θ = 1
2. 1 + tan² θ = sec² θ
3. 1 + cot² θ = cosec² θ

Periodic Functions : A function f is said to be periodic, if there exists a real number T > 0 such that f(x + T) = f(x) for all values of x. Then, T is called the period of the function.
Trigonometric actions are also periodic.
Here, cos (2nπ + x) = cos x
sin (2 nn + x) = sin x

Trigonometric Ratios (T-Ratios) Of Angle (-x)

1. sin(-x) = -sin x,
2. cos (- x) = cos x,
3. tan (-x) = -tan x,
4. cosec (- x) = – cosec x,
5. sec(-x) = sec x,
6. cot (- x) = – cot x.

T-Ratios of (\(\frac{\pi}{2}\) – x)

1. sin (\(\frac{\pi}{2}\)-x) = cos x
2. cosec (\(\frac{\pi}{2}\)-x) = sec x
3. cos (\(\frac{\pi}{2}\)-x) = sin x
4. tan (\(\frac{\pi}{2}\)-x) = cot x
5. sec (\(\frac{\pi}{2}\)-x) = cosec x
6. cot (\(\frac{\pi}{2}\)-x) = tan x

T-Ratios of (\(\frac{\pi}{2}\) + x)

1. sin (\(\frac{\pi}{2}\)-x) = cos x
2. cos (\(\frac{\pi}{2}\)-x) = -sin x
3. tan (\(\frac{\pi}{2}\)-x) = -cot x
4. cosec (\(\frac{\pi}{2}\)-x) = sec x
5. sec (\(\frac{\pi}{2}\)-x) = -cosec x
6. cot (\(\frac{\pi}{2}\)-x) = -tan x

T-Ratios of (π -x)

1. sin (π – x) = sin x,
2. cos (π – x) = – cos x,
3. tan (π – x) = – tan x,
4. cosec (π – x) = cosec x,
5. sec (π – x) = – sec x,
6. cot (π – x) = – cot x.

T-Ratios of (π+ x)

1. sin (π + x) = – sin x,
2. cos (π + x) = – cos x,
3. tan (π + x) = tan x,
4. cosec (π + x) = – cosec x,
5. sec (π + x) = – sec x,
6. cot (π + x) = cot x.

T-Ratios of (2π – x)

1. sin (2π – x) = – sin x,
2. cos (2n – x) = cos x,
3. tan (2π – x) = – tan x,
4. cosec (2π – x) = – cosec x,
5. sec (2π – x) = sec x,
6. cot (2π -x) = – cot x.

T-Ratios of (2π + x)

1. sin (2π + x) = sin x,
2. cos (2π + x) = cos x,
3. tan (2π + x) = tan x,
4. cosec (2π + x) = cosec x,
5. sec (2π + x) = sec x,
6. cot (2π+x)=cotx.

Note : To learn these formulae by heart, we adopt the following method :

I. First of all, we determine the sign of t-ratio under consideration.
The phrase Add Sugar To Coffee is quite helpful. We write Add, Sugar, To,
Coffee in I, II, III, IV quadrants respectively.

1. The letter A of word ‘add’ indicates all the f-ratios of angles lying in the first quadrant are positive.
2. The letter S of word ‘sugar’ indicates sin and cosec of the angle lying in II quadrant are positive and rest of t-ratios are negative.
3. T of word ‘to’ indicates tan and cot of the angle lying in the third quadrant are positive and rest of the t-ratios of such angles are negative.
4. C of the word coffee shows that cos and sec of the angles lying in IV quadrant are positive and rest of t-ratios are negative of such angles.

II. (i) For -angle – x, π – x, π + x, 2π – x, 2π + x, the t-ratios remains to be the same.
sin (- x) = – sin x, tan (π + x) = tan x, cos (2π -x) = cos x.

(ii) For angles \(\frac{\pi}{2}\) – x, \(\frac{\pi}{2}\) + x, \(\frac{3\pi}{2}\) – x, \(\frac{3\pi}{2}\)+ x the t-ratio changes from cos to sin , tan to cot and sec to cosec and vice versa.
e.g., sin(\(\frac{\pi}{2}\) + x) = cosx, tan(\(\frac{\pi}{2}\) – x) = cot x,
sec(\(\frac{\pi}{2}\) + x) = -cosec x etc.

T-Ratios of (x + y)

1. sin (x + y) = sin x cos y + cos x sin y
2. cos (x + y) = cos x cos y – sin x sin y
3. tan (x + y) = \(\frac{\tan x+\tan y}{1-\tan x \tan y}\)
4. cot(x + y) = \(\frac{\cot x \cot y-1}{\cot x+\cot y}\)

T-Ratios of (x – y)

1. sin Or – y) = sin x cos y – cos x- sin y
2. cos (x -y) = cos x cos y + sin x sin y
3. tan(x – y) = \(\frac{\tan x-\tan y}{1+\tan x \tan y}\)
4. cot(x – y) = \(\frac{\cot x \cot y+1}{\cot y-\cot x}\)

T-Ratios of (2x)

1. sin 2x = 2sin x cos x = \(\frac{2 \tan x}{1+\tan ^{2} x}\)
2. cos 2x = cos² x – sin² x = 2cos² x – 1 = 1 – 2sin² x
   = \(\frac{1-\tan ^{2} x}{1+\tan ^{2} x}\)
3. tan 2x = \(\frac{2 \tan x}{1-\tan ^{2} x}\)

T-Ratios of (3x)

1. sin 3x = 3sin x – 4sin³ x.
2. cos 3x = 4cos³ x – 3 cos x.
3. tan3 x = \(\frac{3 \tan x-\tan ^{3} x}{1-3 \tan ^{2} x}\)

Sum and Difference of two like ratios :

1. sm x + sin y = 2sin\(\frac{x+y}{2}\) cos \(\frac{x-y}{2}\)
2. sin x – sin y = 2cos \(\frac{x+y}{2}\) sin \(\frac{x-y}{2}\)
3. cos x + cos y = 2cos \(\frac{x+y}{2}\) cos\(\frac{x-y}{2}\)
4. cos x – cos y = – 2sin\(\frac{x+y}{2}\) sin\(\frac{x-y}{2}\)

Product of T-Ratios:

1. 2 sin x cos y = sin (x + y) + sin (x – y).
2. 2 cos x sin y = sin (x + y) – sin (x – y).
3. 2 cos x cos y = cos (x + y) + cos (x – y).
4. 2 sin x sin y = cos (x – y) – cos (x + y).

General solution of an equation :

1. If sin x = 0, then x = nπ, n ∈ Z.
2. If cos x = 0, then x = (2n + 1)\(\frac{\pi}{2}\), n ∈ Z.
3. If tan x = 0, then x = nπ, n ∈ Z.
4. sin x = sin y ⇒ x = nπ + (- 1 )ⁿy, n ∈ Z.
5. cos x = cos y ⇒ x = 2nπ ±y, n ∈ Z.
6. tan x = tan y ⇒ x = nπ+y, n ∈ Z,
   where y is the principal value of x.