NCERT Solutions for Class 9 Science Chapter 14 Natural Resources
These Solutions are part of NCERT Solutions for Class 9 Science. Here we have given NCERT Solutions for Class 9 Science Chapter 14 Natural Resources. LearnInsta.com provides you the Free PDF download of NCERT Solutions for Class 9 Science (Biology) Chapter 14 – Natural Resources solved by Expert Teachers as per NCERT (CBSE) Book guidelines. All Chapter 14 – Natural Resources Exercise Questions with Solutions to help you to revise complete Syllabus and Score More marks.
More Resources
- NCERT Solutions for Class 9 Science
- HOTS Questions for Class 9 Science
- Value Based Questions in Science for Class 9
- NCERT Exemplar Solutions for Class 9 Science
- Previous Year Question Papers for CBSE Class 9 Science
NCERT TEXT BOOK QUESTIONS
IN TEXT QUESTIONS
Question 1.
How is our atmosphere different from the atmosphere of Venus and Mars ?
Answer:
| Atmosphere over Earth | Atmosphere over Venus and Mars | ||
| 1. | Nitrogen and Oxygen. | It contains nitrogen and oxygen. | The two are absent |
| 2. | Carbon Dioxide. | Carbon dioxide content is little (0-03%). | Carbon dioxide is the major component of atmosphere forming 95-97%. |
| 3. | Water Vapours. | The atmosphere contains water vapours which form a component of water cycle. | The atmosphere does not contain water vapours. Living beings, being absent, have no role in |
| 4. | living Beings. | Composition of atmosphere is maintained by living beings. | determining composition of atmosphere. |
Question 2.
How does atmosphere act as a blanket ?
Answer:
Atmosphere or air is a bad conductor of heat. It, therefore, functions as a blanket.
- It does not allow sudden increase in temperature during the daylight hours when sun shines overhead.
- There is no sudden cooling during night. Atmosphere slows down the escape of heat to the outer space from the area of earth under darkness.
- Atmosphere maintains the average temperature of the earth fairly steady not only during the day but throughout the year.
Question 3.
What causes winds ?
Answer:
Winds are basically caused by heating of air in certain parts. The hot air rises upwards. This creates an area of low pressure. Cooler air from adjacent higher pressure areas passes into this area. This creates wind. The factors which control movement of winds in different directions in different parts of the earth are
- Uneven heating of land in different parts of earth
- Differences in heating and cooling of land and water.
- Barrier of mountains
- Rotation of earth.
Question 4.
How are clouds formed ? (CCE 2011)
Answer:
Clouds are wet air masses that float in the direction of prevailing wind. They develop when water vapours are formed in large number. There is evaporation from the surface of water bodies and wet areas due to their heating during the daytime. Plants also give out water vapours in transpiration while animals do so in exhaled air and perspiration. Air also heats up during daytime. The hot air along with water vapour rises up. At a height, air expands and becomes cool. Cooling causes the water vapours to condense. Suspended particles of dust and other materials function as nuclei around which water vapours condense. When a large wet air mass collects, cloud is formed.
Question 5.
Answer:
List any three human activities that you think would lead to air pollution.
- Burning of fossil fuels in industries, vehicles and thermal plants.
- Processing industries like textiles, asbestos, flour mills.
- Stone crushing.
Question 6.
Why do organisms need water ? (CCE 2011)
Answer:
Organisms need water due to following reasons :
- Component of Living Matter: Water is a major component (60-90%) of living matter.
- Solvent: Water is a general solvent for chemicals found in the living world.
- Reaction Medium: All biochemical reactions occur in the medium of water.
- Transport: Substances are transported in the body of a living organisms only in the dissolved state.
- Turgidity: Cells, cell organelles, tissues and other structures maintain their shape only when they contain sufficient water to make them turgid.
- Temperature Buffer: Water protects the body from sudden changes of temperature.
- Wastes: It helps in separation and elimination of metabolic wastes.
Question 7.
What is the major source of fresh water in the city/town/village where you live ?
Answer:
Ground water which is pumped out by tube wells (In some it is local reservoir, canal or river.
Question 8.
Do you know of any activity which may be polluting this water source ?
Answer:
Dumping of industrial wastes from where pollutants seep into soil to reach ground water (sewage and industrial effluents in case of canal or river water).
Question 9.
How is soil formed ? What is the function of humps in soil ? (CCE 2011, 2012)
Answer:
Soil is formed through two processes of weathering and humification.
Weathering
It is pulverisation of rocks or breaking of rocks into fine particles. There are three types of weathering — physical (atmospheric changes and mechanical forces), chemical and biological. Sun, water, wind and living organisms perform them.
- Sun: It causes expansion of rocks by heating. Cooling causes their contraction. Different parts of rocks expand and contract at different rates. Uneven expansion and contraction produces cracks leading to fragmentation of rocks.
- Water:
- Wetting and Drying: Certain rock components can pick up and lose moisture. They undergo swelling and contraction resulting in fragmentation of rocks.
- Frost Action: Water seeping in cracks would swell up and exert a great pressure if it freezes due to low temperature. The rock would undergo fragmentation.
- Abrasion: Running water carrying rock fragments would break and grind rocks occurring in the pathway. Rain and hail also cause rock breaking.
- Wind: Dust and fine sand carried by wind cause abrasion of the rock surface when wind strikes the same.
- Living Organisms: Lichens secrete chemicals to dissolve minerals from the rock surface. This produces crevices where dust collects. Mosses grow there. They cause deepening of crevices and development of small cracks. Roots of short lived plants widen these cracks. Roots of larger plants cause fragmentation of rocks by entering the cracks and growing in size.
Humification
Partially decomposed organic matter or humus mixes with weathered rock particles to form soil. Humus helps in formation of soil crumbs which are essential for maintaining proper hydration and aeration of soil.
Question 10.
What are the methods of preventing or reducing soil erosion ?
Answer:
Soil Erosion: It is removal of top soil by agency of wind or water. Wind and water are also the agencies which cause weathering of rocks and carrying the fine particles to other places for the formation of soil. Removal of top soil by water or wind leaves the underneath subsoil and rocky base exposed. Very little plant growth occurs there.
Factors Promoting Soil Erosion
- It destroys herbs, grasses and seedlings. The soil is exposed. Trampling by animals causes compaction of soil which reduces its porosity and percolation.
- Removal of litter or scraping of forest floor leaves the ground bare for action of agencies causing erosion.
- There is decreased absorption of water as the latter does not stay for long on the slope. Run off water passing along the slope gathers speed and develops high cutting and carrying capacity.
- Felling of Trees: Felling of trees in excess of regeneration capacity of a forest causes deforestation. It also leaves large area bare for action of wind and water. Deforestation or clearing of forest not only destroys biodiversity but also leads to large scale soil erosion.
- Clean Tilling: Clean tilling of crop fields exposes the soil to erosion.
- Heavy Rain and Strong Winds. Uncovered soil is eroded quickly by heavy rain and strong wind.
Question 11.
What are two different states in which water is found during the water cycle ?
Answer:
Liquid and vapour, occasionally solid (snow) as well.
Question 12.
Name two biologically important compounds that contain both oxygen and nitrogen. . (CCE 2014)
Answer:
Proteins and nucleic acids.
Question 13.
List any three human activities which would lead to an increase in the carbon dioxide content of air.
Answer:
- Increasing combustion of fossil fuels (coal, petroleum, natural gas) in homes, industries, transportation and power projects.
- Increasing use of wood for cooking and heating.
- Deforestation leading to reduced utilisation of carbon dioxide in photosynthesis.
Question 14.
What is green house effect ? (CCE 2011, 2012, 2013)
Answer:
Green house effect is keeping an area warm by allowing the solar radiations to pass in but preventing long waves to escape due to presence of radiatively active gases and glass panes.
Question 15.
What are the two forms of oxygen found in the atmosphere ? (CCE 2011, 2014)
Answer:
- Diatomic oxygen, O2
- Triatomic oxygen or ozone, O3.
NCERT CHAPTER END EXERCISES
Question 1.
Why is the atmosphere essential for life ? (CCE 2012)
Answer:
- Oxygen: Atmosphere contains oxygen which is essential for combustion and respiration of most organisms.
- Carbon Dioxide: Atmosphere provides carbon dioxide for photosynthesis of plants.
- Protection: Atmosphere filters out lethal cosmic rays and high energy ultraviolet rays.
- Temperature Buffer: Atmosphere does not allow daytime temperature to rise abnormally nor does it allow night time temperature to fall down drastically. This provides favourable temperature for the living organisms.
- Other Functions: Air currents help in dispersal of spores and other dissemules. Water cycle operates through atmosphere and produces rain to replenish fresh water over land.
Question 2.
Why is water essential for life ?
Answer:
Organisms need water due to following reasons :
- Component of Living Matter: Water is a major component (60-90%) of living matter.
- Solvent: Water is a general solvent for chemicals found in the living world.
- Reaction Medium: All biochemical reactions occur in the medium of water.
- Transport: Substances are transported in the body of a living organisms only in the dissolved state.
- Turgidity: Cells, cell organelles, tissues and other structures maintain their shape only when they contain sufficient water to make them turgid.
- Temperature Buffer: Water protects the body from sudden changes of temperature.
- Wastes: It helps in separation and elimination of metabolic wastes.
Question 3.
How are living organisms dependent on soil ? Are organisms that live in water totally independent of soil as a resource ? ‘
Answer:
All terrestrial organisms depend upon plants for their food and its contained energy. Plants are dependent on soil for anchorage, absorption of water and nutrients. Without them plants cannot manufacture food. So, all living terrestrial organisms depend upon soil.
Aquatic organisms are apparently not connected with soil. However, aquatic autotrophs require inorganic nutrients for manufacture of food. Nutrients reach water bodies only when rain water passes over and inside the soil. Therefore, aquatic organisms are not totally independent of soil as a resource.
Question 4.
You have seen weather reports on television and in newspapers. How do you think we are able to predict the weather ?
Answer:
Weather reports depict areas of low and high pressure, prevailing direction of wind, dryness or wetness of air masses, clouds and their progress, presence and progress of any cyclone, etc. Predictions are then made whether a particular area will or will not receive rain, have calm weather or high speed wind and dust storm.
Question 5.
We know that many human activities lead to increasing levels of pollution of air, water bodies and soil. Do you think that isolating these activities to specific and limited areas would help in reducing pollution ?
Answer:
Restricting pollution related activities to specific and limited areas will not reduce pollution in those areas. The same may rather increase. There are two benefits of such a practice
- Joint pollution treatment plants can be installed
- The residential and commercial areas away from such pollution generating regions will be comparatively free from pollution.
Question 6.
Write a note on how forests influence the quality of our air, soil and water resources. (CCE 2012)
Answer:
Air Resources:
- Oxygen-Carbon Dioxide Balance. Forests maintain the optimum level of oxygen and carbon dioxide. They function as sink for excess carbon dioxide being produced due to excessive combustion. Forests also release a lot of more oxygen to compensate for excess being consumed elsewhere in respiration and combustion.
- Control of Air Pollution. Both suspended particles and gaseous pollutants are picked up by forest plants.
Soil Resources: Roots of the forest plants hold the soil firmly. Forest cover protects the soil from direct pounding by rain drops. Forest soil is also sufficiently porous to reduce run off and increase infiltration of rain water. All the three factors prevent soil erosion.
Water Resources:
- Rainfall. Forests help in increasing the amount and periodicity of rain fall.
- Forest trees retain a lot of water at their bases. Percolation of water into interior of earth produces springs which form rivulets with perennial flow of water.
SELECTION TYPE QUESTIONS
Alternate Response Type Questions :
(True/False, Right/Wrong, Yes/No)
Question 1.
As 75% of earth’s surface is covered with water, the outer crust of earth is called hydrosphere.
Question 2.
Combustion consumes oxygen and liberates carbon dioxide.
Question 3.
Winds develop due to uneven heating of earth.
Question 4.
Carbon monoxide and carbon dioxide produce acid rain.
Question 5.
The amount of rainfall directly influences the abundance and diversity of like forms.
Question 6.
Soil has no role in supplying nutrients to aquatic biota
Question 7.
Pesticides and fertilizers are harmful to soil as they kill the microorganisms involved in recycling of nutrients.
Question 8.
Green house gases are the ones which allow the heat emitted by earth to pass out.
Matching Type Questions :
Question 9.
Match the articles of the column I and column II (Single Matching)
| Column I | Column II |
| (a) Chlorofluorocarbons | (i) Bacteria |
| (b) Carbon dioxide | (ii) Fossil fuels |
| (c) Nitrogen fixation | (iii) Ozone depletion |
| (d) Nitrogen and sulphur oxides | (iv) Green house gas |
Question 10.
Match the contents of columns I, II and III (Double matching)
| Column I | Column II | Column III |
| (a) Food | (i) Sun, water and wind | (p) Soil |
| (b) Abiotic | (ii) Resources | (q) Living organisms |
| (c) Carbon dioxide | (iii) Air and Water | (r) Energy |
| (d) Paedogenesis | (iv) Photosynthesis | (s) Shells |
Question 11.
Match the pollutants with the type of pollution—air (A), water (W) and soil (S) (Key or check List matching)
| Pollutants | Pollution |
|
(a) Hydrogen sulphide (b) SPM (c) Algal bloom (d) Raw manure |
Question 12.
Match Stimulus with Appropriate Response.
| Conservation Practice | Soil-A | Water-B | Air-C |
|
(i) Pollution under control certificate (ii) Vegetation cover (iii) Terracing (iv) Sewage treatment |
Fill In the Blanks
Question 13. The earth ……………… and energy from sun are necessary to meet basic requirements of life forms.
Question 14. Surface temperature of moon varies from …………….. to 110°C.
Question 15. …………….. makes soil porous and allows water and air to penetrate deep underground.
Question 16. Carbon occurs in elemental form in ………………. and graphite.
Question 17. CFCs are carbon compounds having both ……………. and chlorine.
Answers:
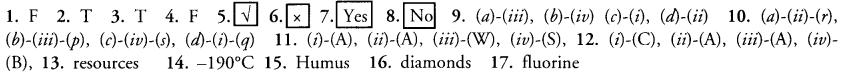
SOME TYPICAL QUESTIONS
Question 1.
Name the different physical divisions of biosphere.
Answer:
Three – atmosphere (air), hydrosphere (water) and lithosphere (land).
Question 2.
Differentiate between renewable and non-renewable resources.
Answer:
| Renewable Resources | Non-renewable Resources |
| 1. Replacement. The resources are replenished within reasonable time.
2. Use. The resources can be used forever provided the use is limited. 3. Life. They are both abiotic and biotic. 4. Availability. It can be increased only by enhancing replenishment. |
Replenishment is absent.
The resources will ultimately dwindle, and get exhausted. They are abiotic. This is not possible. Increased exploitation will result in quick exhaustion. |
Question 3.
Name the parts of India falling under wet zone.
Answer:
Wet zone (rainfall over 200 cm/yr) comprises Western Ghats, Andaman-Nicobar and North East India.
Question 4.
Name two diseases caused by
(a) Infectious agents in polluted water,
(b) Toxic chemicals in polluted water.
Answer:
(a) Infectious Agents. Jaundice, dysentery.
(b) Toxic Agents. Minamata (mercury), itai-itai (cadmium).
Question 5.
What is ozone hole ? Where is it found ? What is its effect ? (CCE 2011)
Answer:
Ozone hole is thinning of ozone in the stratosphere where it is normally present in high concentration as ozone layer. Ozone hole is formed during spring time over antarctica and to a small extent over north pole. Thinning of ozone layer or ozone hole increases the passage of harmful ultravoilet rays to earth. This has increased incidence of skin cancers, defective eye sight, reduced immunity, increased number of mutations and reduced crop yield in southern countries of southern hemisphere.
NCERT Solutions for Class 9 Science Chapter 14 Natural Resources
Hope given NCERT Solutions for Class 9 Science Chapter 14 are helpful to complete your science homework.
If you have any doubts, please comment below. Learn Insta try to provide online science tutoring for you.