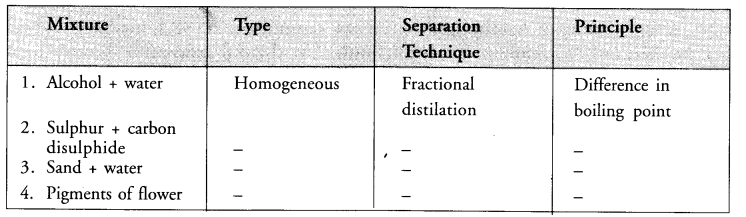
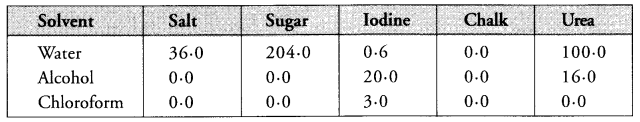
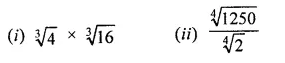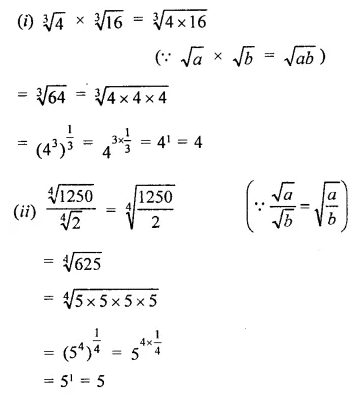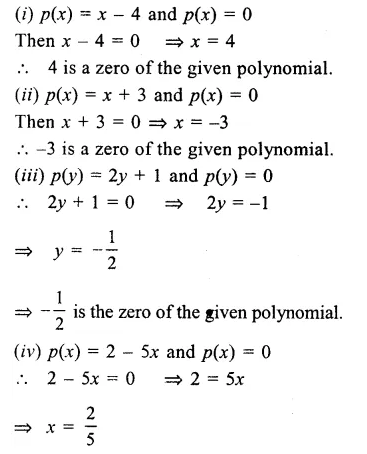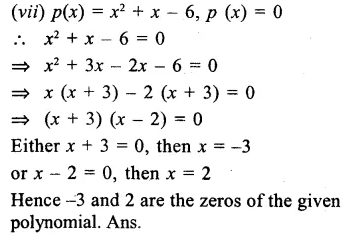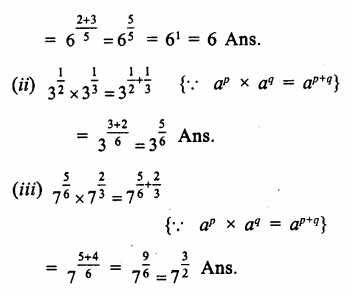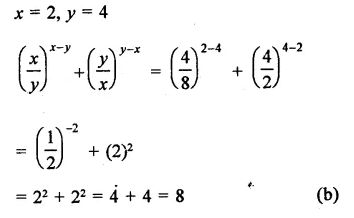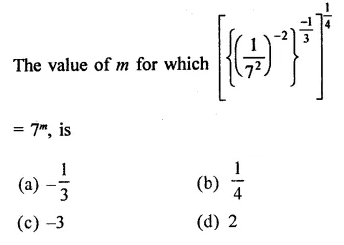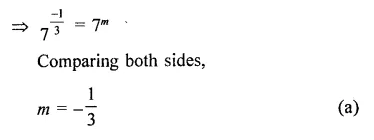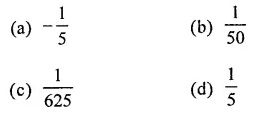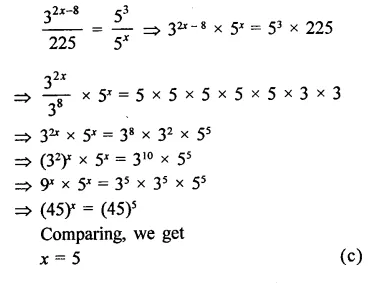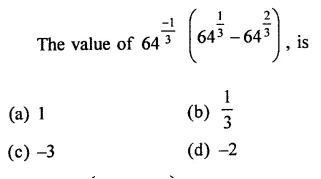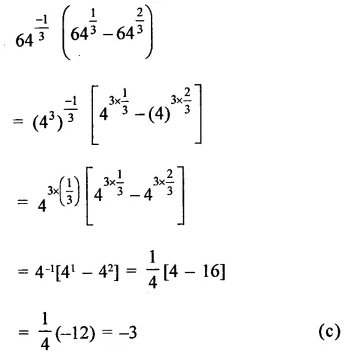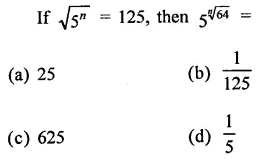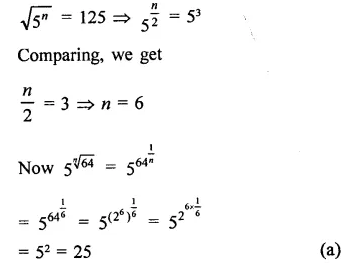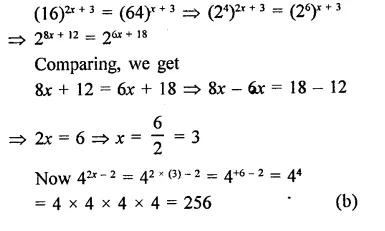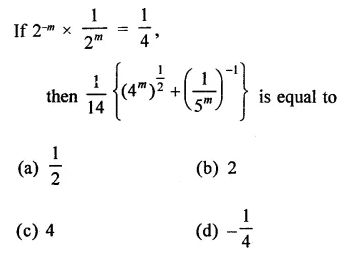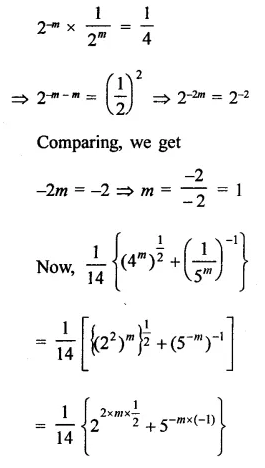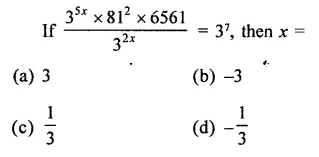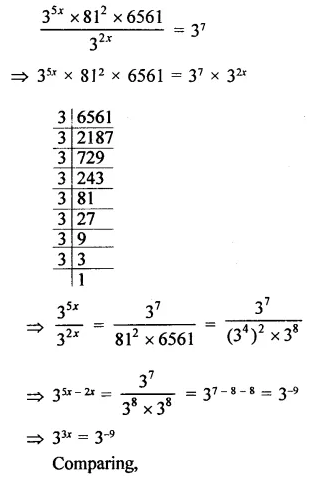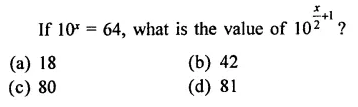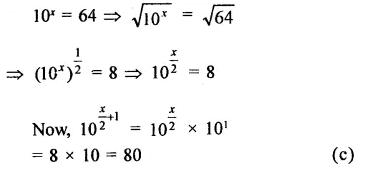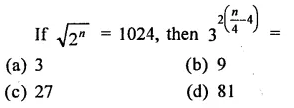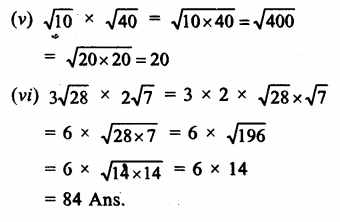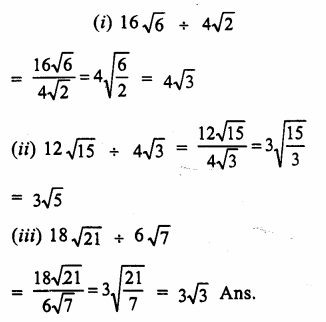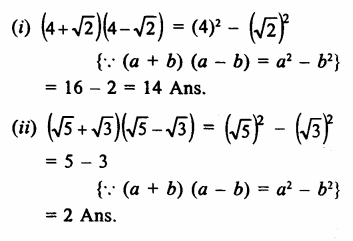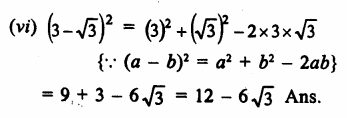HOTS Questions for Class 9 Science Chapter 1 Matter in Our Surroundings

HOTS Questions for Class 9 Science Chapter 1 Matter in Our Surroundings

These Solutions are part of HOTS Questions for Class 9 Science. Here we have given HOTS Questions for Class 9 Science Chapter 1 Matter in Our Surroundings

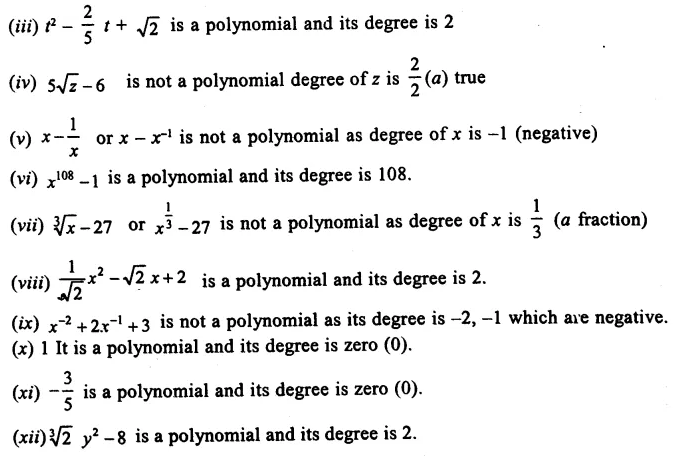
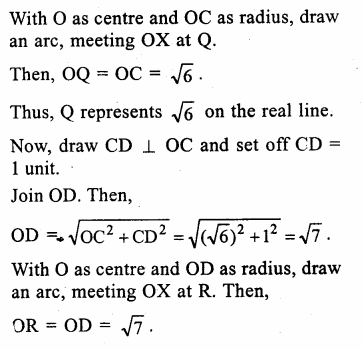
Question 1.
The diagram shows an experiment in which gases hydrogen and carbon dioxide are placed in two jars as shown in the figure. If the lid separating the two jars be removed, what will the constituents in the gas jar A after a few minutes ?
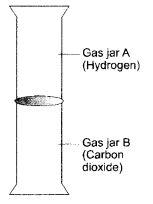
HOTS Questions for Class 9 Science Chapter 1 Matter in Our Surroundings image - 1
(a) carbon dioxide only
(b) hydrogen only
(c) mixture of carbon dioxide and hydrogen.
Answer:
(c) The gas jar A will contain both the gases carbon dioxide and hydrogen. Actually, the molecules of the gas present in one jar will move into the empty spaces present in the other jar and vice versa.

More Resources

Question 2.
The given graph shows the heating curve for a pure substance. The temperature rises with time as the substance is heated :
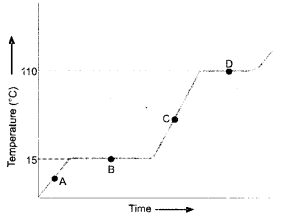
HOTS Questions for Class 9 Science Chapter 1 Matter in Our Surroundings image - 2

  1. What is the physical state of the substance at the points A, B, C and D ?
  2. What is the melting point of the substance ?
  3. What is its boiling point ?
  4. What happens to the temperature while the substance is changing state ?
  5. The substance is not water. How can you judge from the graph ?

Answer:

  1. At point A : The substance is in the solid state.
    At point B : The substance has started melting. It exists both in the solid and liquid states.
    At point C : The substance is in the liquid state.
    At point D : The substance has started boiling. It exists both in the liquid and gaseous states.
  2. The melting point of the substance is 15°C.
  3. The boiling point of the substance is 110°C.
  4. The temperature remains the same during the change of state.
  5. Had substance been water, its melting point should have been 0°C and boiling point 100°C. It is therefore, not water.

Question 3.
In severe cold weather, a family burnt wood in the room during the night by keeping the windows close. After sometime, they felt suffocated. They immediately opened the windows and got relief. What did actually happen ?
Answer:
When wood burns, the carbon present is oxidised to carbon dioxide (CO2) which is non-poisonous. When the windows are close, the air or oxygen cannot enter the room. In the incomplete supply of oxygen, carbon is oxidised to carbon monoxide (CO) which is a highly poisonous gas. It caused suffocation in a close room. On opening the windows, the poisonous gas slowly diffused out the room and fresh air came inside. That is how the poisonous effect of carbon monoxide gas was neutralised and the family got relief.

Question 4.
Small quantities of water and ether are placed on the palms of both the hands. Which will experience more cooling ?
Answer:
The palm containing ether will experience extra cooling. Actually ether is more volatile than water. Therefore, it will evaporate at a faster rate than water. Since cooling is always caused during evaporation, the palm containing ether will become cooler.

Hope given HOTS Questions for Class 9 Science Chapter 1 Matter in Our Surroundings are helpful to complete your science homework.

If you have any doubts, please comment below. Learn Insta try to provide online science tutoring for you.

HOTS Questions for Class 9 Science Chapter 2 Is Matter Around Us Pure

HOTS Questions for Class 9 Science Chapter 2 Is Matter Around Us Pure

These Solutions are part of HOTS Questions for Class 9 Science. Here we have given HOTS Questions for Class 9 Science Chapter 2 Is Matter Around Us Pure.

Question 1.
A house wife churned full cream with a milk churner

  1. What will she observe after churning the milk ?
  2. What could be the possible reason for the observation ?

Answer:

  1. Churning of milk is a centrifugation process. As a result, lighter particles of cream or butter will move upwards and collect at the top. Heavier residual particles will remain at the bottom. This is a very common process used to separate cream from milk or butter from yogurt.
  2. The separation is based on the principle that lighter particles move upwards while denser particle downwards upon centrifugation.

More Resources

Question 2.
Based on separation techniques, complete the following. The first one is done for you.
HOTS Questions for Class 9 Science Chapter 2 Is Matter Around Us Pure image - 1
Answer:
2. Homogeneous, Evaporation, Difference in nature.
3. Hetereogeneous, Filtration, Difference in solubility in water
4. Homogeneous, Chromatography, Difference in adsorption of different components.

Question 3.
The table given below shows number of grams of five different solids dissolving in 100 g of the solvents : water, alcohol and chloroform (all at 20°C).
HOTS Questions for Class 9 Science Chapter 2 Is Matter Around Us Pure image - 2

  1. Which solid dissolves best in water at 20°C ?
  2. Which solid is maximum soluble in alcohol ?
  3. Which solid is insoluble in all the three solvents ?

Answer:

  1. Sugar is best soluble in water at 20°C
  2. Iodine is maximum soluble in alcohol.
  3. Chalk is insoluble in all the three solvents.

Question 4.
Some solids dissolve easily in liquids while the others donot

  1. What is the name given to the liquids which dissolve solids ?
  2. What is the name given to the clear liquid formed when a solid dissolves in a liquid ?
  3. What is the name given to the liquid which contains in it some suspended particles ?

Answer:

  1. The liquids are known as solvents
  2. The clear liquid is called solution or true solution. .
  3. The liquid is known as dispersion medium or dispersing medium.

Question 5.
Butter is an example of one type of colloidal solution. Name it. Give a reason for your choice.
Answer:
The colloidal solution is an example in which solid acts as the dispersion medium while liquid as the dispersed phase. It is also called gel.
Reason for the choice. On pressing butter, liquid drops come out of it leaving behind a solid. This clearly shows that butter is a gel.

Question 6.

  1. The solubility of sodium chloride in water increases with rise in temperature while that of lithium carbonate decreases. Assign reason.
  2. Water containing 88-8% oxygen and 11-2% hydrogen is often used as a fire extinguisher. Can a mixture containing the two gases in the same ratio by mass be used for extinguishing fire ?
  3. The melting point of a solid when determined experimentally comes out to be 160°C. But its actual melting point as given in standard books is 150°C. Predict the nature of the solid.

Answer:

  1. When sodium chloride is dissolved in water, the process is endothermic in nature. This means that heat energy is absorbed in the process. Therefore, solubility increases with rise in the temperature. In case of lithium carbonate, the process of dissolution is exothermic. This means that heat is evolved in the process. Therefore, its solubility in water decreases with rise in temperature.
  2. No, it cannot be used. Actually, in water the two elements are chemically combined with each other. They therefore, lose their identity. But in the mixture, no chemical combination between hydrogen and oxygen has taken place. Even water cannot be formed on mixing the gases. Therefore, the mixture does not extinguish any fire.
  3. Since the experimentally determined melting point of the solid is more than the standard value of the melting point, this means that the solid is not in pure state. It has some impurities present. Please note that the purity of a solid can be determined by finding its melting point and comparing it with the standard value.

Hope given HOTS Questions for Class 9 Science Chapter 2 Is Matter Around Us Pure are helpful to complete your science homework.

If you have any doubts, please comment below. Learn Insta try to provide online science tutoring for you.

RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1

RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1

Question 1.
Simplify each of the following:
RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1 Q1.1
Solution:
RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1 Q1.2

Question 2.
Simplify the following expressions:
(i)  (4 + \(\sqrt { 7 } \)) (3 + \(\sqrt { 2 } \))
(ii) (3 + \(\sqrt { 3 } \) )(5- \(\sqrt { 2 } \))
(iii) (\(\sqrt { 5 } \) -2)(\(\sqrt { 3 } \) – \(\sqrt { 5 } \))
Solution:
RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1 Q2.1

Question 3.
Simplify the following expressions:
(i)  (11 + \(\sqrt { 11 } \)) (11 – \(\sqrt { 11 } \))
(ii) (5 + \(\sqrt { 7 } \) ) (5 – \(\sqrt { 7 } \) )
(iii) (\(\sqrt { 8 } \) – \(\sqrt { 2 } \)) (\(\sqrt { 8 } \)+ \(\sqrt { 2 } \))
Solution:
RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1 Q3.1
RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1 Q3.2

Question 4.
Simplify the following expressions:
(i) (\(\sqrt { 3 } \)+\(\sqrt { 7 } \))2
(ii) (\(\sqrt { 5 } \) – \(\sqrt { 3 } \) )2
(iii) (2\(\sqrt { 5 } \) + 3 \(\sqrt { 2} \))2
Solution:
(i)
RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1 Q4.1
RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1 Q4.2
Hope given RD Sharma Class 9 Solutions Chapter 3 Rationalisation Ex 3.1 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2B

RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2B

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2B.

Other Exercises

Question 1.
Solution:
p(x) = 5 – 4x + 2x2
RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2B Q1.1

Question 2.
Solution:
p(y) = 4 + 3y – y2 + 5y3
RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2B Q2.1

Question 3.
Solution:
f(t)=4t2-3t+6
RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2B Q3.1

Question 4.
Solution:
RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2B Q4.1
RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2B Q4.2

Question 5.
Solution:
RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2B Q5.1
RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2B Q5.2
RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2B Q5.3

Hope given RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2A

RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2A

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2A.

Other Exercises

Question 1.
Solution:
(i) x5-2x3+x+7 is a polynomial and its degree is 5
(ii) y3-√3y is a polynomial and its degree is 3
RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2A 1

Question 2.
Solution:
(i) Degree is 1
(ii) degree is 3
(iii) degree is zero
(iv) degree is 7
(v) degree is 10
(vi) degree is 2

Question 3.
Solution:
(i) Coefficient of x3 is -5.
(ii) Coefficient of x is -2√2
(iii) Coefficient of x2 is \(\frac { \pi }{ 3 } \)
(iv) Coefficient of x2 is 0

Question 4.
Solution:
(i) Example of a binomial of degree 27 is 4x27 – 5
(ii) Example of a monomial of degree 16 is x16
(iii) Example of trinomial of degree 3 is 2x3 + 7x + 4

Question 5.
Solution:
(i) 2x2 + 4x is a quadratic polynomial. (Degree 2)
(ii) x – x3 is a cubic polynomial (Degree 3)
(iii) 2 – y – y2 is a quadratic polynomial (Degree 2)
(iv) – 7 + z is a linear polynomial (Degree 1)
(v) 5t is a linear polynomial (Degree 1)
(vi) p3 is a cubic polynomial (Degree 3)

 

Hope given RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1F

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1F

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1F.

Other Exercises

Question 1.
Solution:
We know that
ap x aq = ap+q
∴ Therefore
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1F 1
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1F 2

Question 2.
Solution:
We know that
ap ÷ aq = ap-q
Therefore
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1F 3

Question 3.
Solution:
We know that
ap x bp = (ab)p
Therefore
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1F 4

Question 4.
Solution:
We know that
(ap)q =apq
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1F 5

Question 5.
Solution:
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1F 6

Question 6.
Solution:
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1F 7

Question 7.
Solution:
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1F 8

Hope given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1F are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS

RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS

Mark the correct alternative in each of the following:
Question 1.
The value of {2 – 3 (2 – 3)3}3 is
(a) 5
(b) 125
(c) \(\frac { 1 }{ 5 }\)
(d) -125
Solution:
{2 – 3 (2 – 3)3}3 = {2 – 3 (-1)3}3
= {2 – 3 x (-1)}3
= (2 + 3)3 = (5)3
= 125    (b)

Question 2.
The value of x – yx-y when x = 2 and y = -2 is
(a) 18
(b) -18
(c) 14
(d) -14
Solution:
x = 2, y = -2
x-yx-y = 2 – (-2)2 – (-2)
= 2 – (-2)2 + 2 = 2 – (-2)4
= 2 – (+16) = 2 – 16 = -14        (d)

Question 3.
The product of the square root of x with the cube root of x, is
(a) cube root of the square root of x
(b) sixth root of the fifth power of x
(c) fifth root of the sixth power of x
(d) sixth root of x
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q3.1

Question 4.
The seventh root of x divided by the eighth root of x is
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q4.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q4.2

Question 5.
The square root of 64 divided by the cube root of 64 is
(a) 64
(b) 2
(c) \(\frac { 1 }{ 2 }\)
(d) 64\(\frac { 2 }{ 3 }\)
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q5.1

Question 6.
Which of the following is (are) not equal to
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q6.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q6.2

Question 7.
When simplified (x–1 + y–1)–1 is equal to
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q7.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q7.2

Question 8.
If 8x+1 = 64, what is the value of 3 2x +1?
(a) 1
(b) 3
(c) 9
(d) 27
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q8.1

Question 9.
If (23)2 = 4x then   3x =
(a) 3
(b) 6
(c) 9
(d) 27
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q9.1

Question 10.
If x-2= 64, then x\(\frac { 1 }{ 3 }\) + x°=
(a) 2
(b) 3
(c) \(\frac { 3 }{ 2 }\)
(c) \(\frac { 2 }{ 3 }\)
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q10.1

Question 11.
When simplified ( –\(\frac { 1 }{ 27 }\))\(\frac { -2 }{ 3 }\)
(a) 9
(b) -9
(c) \(\frac { 1 }{ 9 }\)
(d) –\(\frac { 1 }{ 9 }\)
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q11.1

Question 12.
Which one of the following is not equal to
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q12.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q12.2
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q12.3

Question 13.
Which one of the following is not equal to
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q13.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q13.2
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q13.3

Question 14.
If a, b, c are positive real numbers, then
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q14.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q14.2

Question 15.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q15.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q15.2

Question 16.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q16.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q16.2
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q16.3

Question 17.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q17.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q17.2

Question 18.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q18.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q18.2

Question 19.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q19.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q19.2

Question 20.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q20.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q20.2
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q20.3

Question 21.
The value of {(23 + 22)2/3+ (150 -29)1/2}2  is
(a) 196
(b) 289
(c) 324
(d) 400
Solution:
{(23 + 22)2/3 + (150 – 29)1/2}2
= [(23×4)\(\frac { 2 }{ 3 }\)  +(150 – 29)\(\frac { 1 }{ 2 }\) ]2
= [(27)\(\frac { 2 }{ 3 }\) + (121)\(\frac { 1 }{ 2 }\) ]2
= [(33)3 +(112)\(\frac { 1 }{ 2 }\)]2 = (9 + 11)2
= (20)2 = 400  (d)

Question 22.
(256)0.16x (256)0.09
(a) 4
(b) 16
(c) 64
(d) 256.25
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q22.1

Question 23.
If 102y = 25, then 10-y equals
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q23.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q23.2

Question 24.
If 9X + 2 = 240 + 9X. then x =
(a) 0.5
(b) 0.2
(c) 0.4
(d) 0.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q24.1

Question 25.
If x is a positive real number and x2 = 2, then x3 =
(a) \(\sqrt { 2 } \)
(b) 2\(\sqrt { 2 } \)
(c) 3\(\sqrt { 2 } \)
(d) 4
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q25.1

Question 26.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q26.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q26.2

Question 27.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q27.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q27.2

Question 28.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q28.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q28.2
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q28.3

Question 29.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q29.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q29.2

Question 30.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q30.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q30.2

Question 31.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q31.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q31.2

Question 32.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q32.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q32.2

Question 33.
If (16)2x + 3 = (64)x + 3 , then 42x – 2  =
(a) 64
(b) 256
(c) 32
(d) 512
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q33.1

Question 34.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q34.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q34.2
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q34.3

Question 35.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q35.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q35.2
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q35.3

Question 36.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q36.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q36.2
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q36.3

Question 37.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS 37.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS 37.2

Question 38.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q38.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q38.2

Question 39.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q39.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q39.2

Question 40.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q40.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q40.2
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS Q40.3

Hope given RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers MCQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1E.

Other Exercises

Rationalise the denominator of each of the followings :

Question 1.
Solution:
Here,RF of √7 is √7
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 1

Question 2.
Solution:
Here RF √3 is √3
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 2

Question 3.
Solution:
Here RF of \(\frac { 1 }{ \left( { 2+ }\sqrt { 3 } \right) }\) is \(\frac { 1 }{ \left( { 2- }\sqrt { 3 } \right) }\)
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 3

Question 4.
Solution:
Here RF is √5 + 2
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 4

Question 5.
Solution:
Here RF is 5 – 3√2
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 5

Question 6.
Solution:
Here RF is √6 + √5
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 6
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 7

Question 7.
Solution:
Here RF = √7 – √3
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 8

Question 8.
Solution:
Here RF = √3 – 1
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 9
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 10

Question 9.
Solution:
Here RF = (3-2√2)
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 11

Find the values of a and b in each of the following :

Question 10.
Solution:
\(\frac { \sqrt { 3 } +1 }{ \sqrt { 3 } -1 } \), RF = √3+1
(Multiplying and dividing by √3+1)
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 12

Question 11.
Solution:
\(\frac { 3+\sqrt { 2 } }{ 3-\sqrt { 2 } } \), RF is 3+√2
(Multiplying and dividing by 3+√2)
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 13

Question 12.
Solution:
In \(\frac { 5-\sqrt { 6 } }{ 5+\sqrt { 6 } } \), RF is (5-√6)
(Multiplying and dividing by 5-√6)
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 14

Question 13.
Solution:
In \(\frac { 5+2\sqrt { 3 } }{ 7+4\sqrt { 3 } } \), RF is 7-4√3
(Multiplying and dividing by 7-4√3)
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 15
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 16

Question 14.
Solution:
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 17
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 18

Question 15.
Solution:
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 19
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 20

Question 16.
Solution:
x = (4-√15)
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 21
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 22

Question 17.
Solution:
x = 2+√3
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 23

Question 18.
Solution:
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 24
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E 25

Hope given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1E are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1D.

Other Exercises

Question 1.
Solution:
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D 1
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D 2
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D 3

Question 2.
Solution:
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D 4
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D 5

Question 3.
Solution:
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D 6

Question 4.
Solution:
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D 7
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D 8
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D 9

Question 5.
Solution:
(i) Draw a line segment AB = 3.2 units (cm) and extend it to C such that BC = 1 unit.
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D 10
(ii) Find the mid-point O of AC.
(iii) With centre O and OA as radius draw a semicircle on AC
(iv) Draw BD ⊥ AC meeting the semicircle at D.
(v) Join BD which is √3.2 units.
(vi) With centre B and radius BD, draw an arc meeting AC when produced at E.
Then BE = BD = √3.2 units. Ans.

Question 6.
Solution:
(i) Draw a line segment AB = 7.28 units and produce is to C such that BC = 1 unit (cm)
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D 11
(ii) Find the mid-point O of AC.
(iii) With centre O and radius OA, draw a semicircle on AC.
(iv) Draw a perpendicular BD at AC meeting the semicircle at D
Then BD = √7.28 units.
(v) With centre B and radius BD, draw an arc which meet AC produced at E.
Then BE = BD = √7.28 units.

Question 7.
Solution:
(A) For Addition
(i) Closure property: The sum of two real numbers is always a real number.
(ii) Associative Law : (a + b) + c = a + (b + c), for all values of a, b and c.
(iii) Commutative Law : a + b = b + a for all real values of a and b.
(iv) Existance of Additive Identity : 0 is the real number such that: 0 + a = a + 0 = afor every real value of a.
(v) Existance of addtive inverse : For each real value of a, there exists a real value (-a) such that a + (-a) = (-a) + a = 0, Then (a) and (-a) are called the additive inverse of each other.
(v) Existence of Multiplicative Inverse. For each non zero real number a, there exists a real number \(\frac { 1 }{ a }\) such that a . \(\frac { 1 }{ a }\) = \(\frac { 1 }{ a }\) . a = 1
a and \(\frac { 1 }{ a }\) are called multiplicative inverse or reciprocal of each other.
(B) Multiplication
(i) Closure property: The product of two real numbers is always a real number.
(ii) Associative law : ab(c) = a(bc) for all real values of a, b and c
(iii) Commutative law : ab=ba for all real numbers a and b
(iv) Existance of Multiplicative Identity: clearly is a real number such that 1.a = a.1 = a for every value of a.

Hope given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS

RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS

Question 1.
Write (625)–1/4 in decimal form.
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q1.1

Question 2.
State the product law of exponents:
Solution:
xm x xn = xm +n

Question 3.
State the quotient law of exponents.
Solution:
xm ÷ xn = xm -n

Question 4.
State the power law of exponents.
Solution:
(xm)n =xm x n = xmn

Question 5.
If 24 x 42 – 16x, then find the value of x.
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q5.1

Question 6.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q6.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q6.2

Question 7.
Write the value of \(\sqrt [ 3 ]{ 7 }\)  x \(\sqrt [ 3 ]{ 49 }\) .
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q7.1

Question 8.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q8.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q8.2

Question 9.
Write the value of \(\sqrt [ 3 ]{ 125×27 }\)
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q9.1

Question 10.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q10.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q10.2

Question 11.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q11.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q11.2
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q11.3

Question 12.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q12.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q12.2

Question 13.
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q13.1
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q13.2

Question 14.
If (x – 1)3 = 8, what is the value of (x + 1)2?
Solution:
RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS Q14.1

Hope given RD Sharma Class 9 Solutions Chapter 2 Exponents of Real Numbers VSAQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1C

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1C

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1C.

Other Exercises

Question 1.
Solution:
Irrational numbers : Numbers which are not rational numbers, are called irrational numbers. Rational numbers can be expressed in terminating decimals or repeating decimals while irrational number can’t.
\(\frac { 1 }{ 2 } \) , \(\frac { 2 }{ 3 } \) , \(\frac { 7 }{ 5 } \) etc.are rational numbers and π, √2, √3, √5, √6….etc are irrational numbers

Question 2.
Solution:
(i) √4 = ±2, it is a rational number
(ii) √196 = ±14 it is a rational number
(iii) √21 It is irrational number.
(iv) √43 It is irrational number.
(v) 3 + √3 It is irrational number because sum of a rational and an irrational number is irrational
(vi) √7 – 2 It is irrational number because difference of a rational and irrational number is irrational
(vii) \(\frac { 2 }{ 3 } \)√6 . It is irrational number because product of a rational and an irrational number is an irrational number.
(viii) 0.\(\overline { 6 } \) = 0.6666…. It is rational number because it is a repeating decimal.
(ix) 1.232332333…. It is irrational number because it not repeating decimal
(x) 3.040040004…. It is irrational number because it is not repeating decimal.
(xi) 3.2576 It is rational number because it is a terminating decimal.
(xii) 2.3565656…. = 2.3 \(\overline { 56 } \) It is rational number because it is a repeating decimal.
(xiii) π It is an irrational number
(xiv) \(\frac { 22 }{ 7 } \). It is a rational number which is in form of \(\frac { p }{ q } \) Ans.

Question 3.
Solution:
(i) Let X’OX be a horizontal line, taken as the x-axis and let O be the origin. Let O represent 0.
Taken OA = 1 unit and draw AB ⊥ OA such that AB = 1 unit. Join OB, Then,
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1C 1
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1C 2
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1C 3

Question 4.
Solution:
Firstly we represent √5 on the real line X’OX. Then we will find √6 and √7 on that real line.
Now, draw a horizontal line X’OX, taken as x-axis
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1C 4
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1C 5
RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1C 6

Question 5.
Solution:
(i) 4 + √5 : It is irrational number because in it, 4 is a rational number and √5 is irrational and sum of a rational and an irrational is also an irrational.
(ii) (-3 + √6) It is irrational number because in it, -3 is a rational and √6 is irrational and sum or difference of a rational and irrational is an irrational.
(iii) 5√7 : It is irrational because 5 is rational and √7 is irrational and product of a rational and an irrational is an irrational.
(iv) -3√8 : It is irrational because -3 is a rational and √8 is an irrational and product of a rational and an irrational is also an irrational.
(v) \(\frac { 2 }{ \sqrt { 5 } } \) It is irrational because 2 is a rational and √5 is an irrational and quotient of a rational and an irrational is also an irrational.
(vi) \(\frac { 4 }{ \sqrt { 3 } } \) It is irrational because 4 is a rational and √3 is an irrational number and quotient of a rational and irrational is also an irrational.

Question 6.
Solution:
(i) True.
(ii) False, as the sum of two irrational number is irrational is not always true.
(iii) True.
(iv) False, as the product of two irrational numbers is irrational is not always true.
(v) True.
(vi) True.
(vii) False as a real number can be either rational or irrational.

Hope given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.