Tag: RS Aggarwal Maths Solutions Class 9

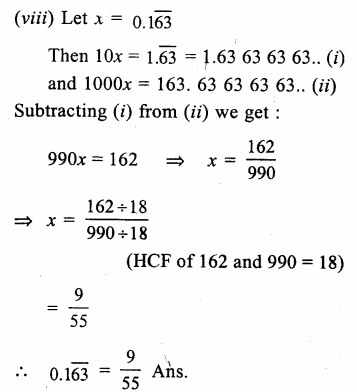
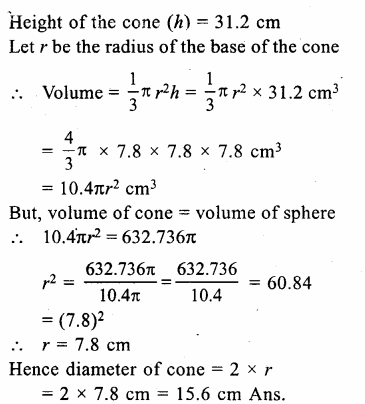
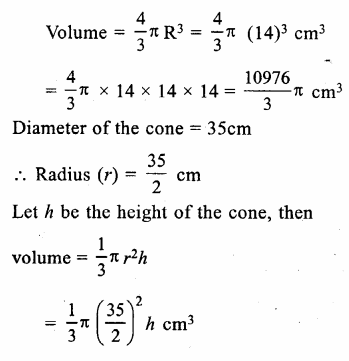
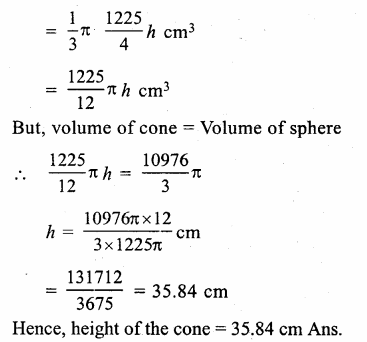
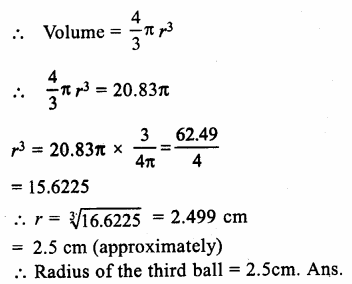
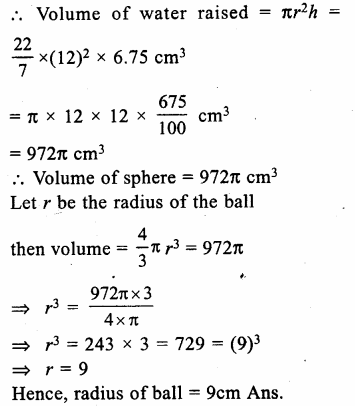
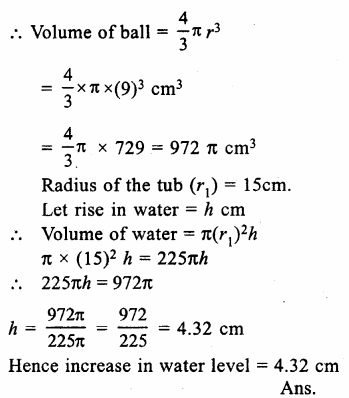
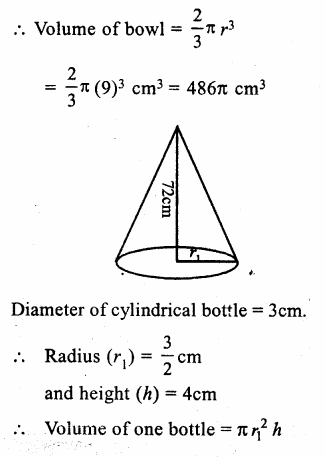
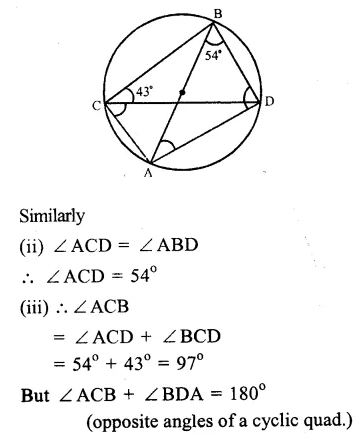
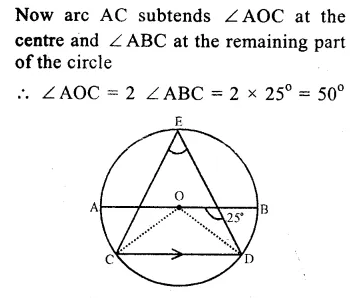
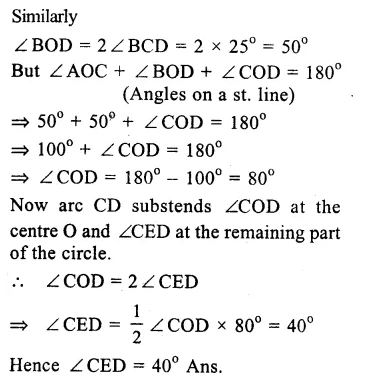
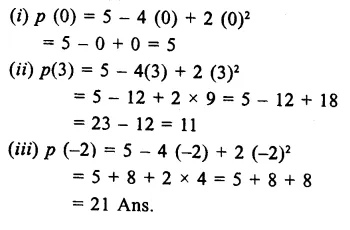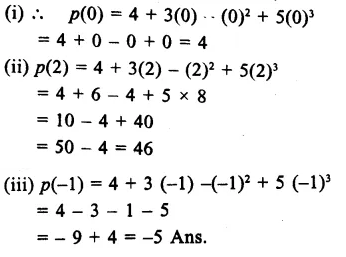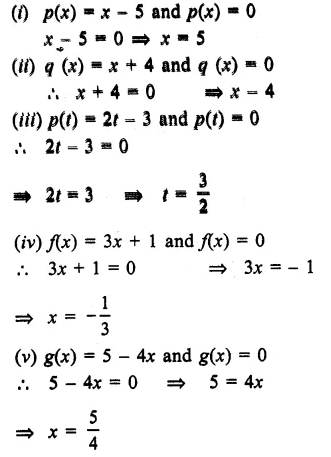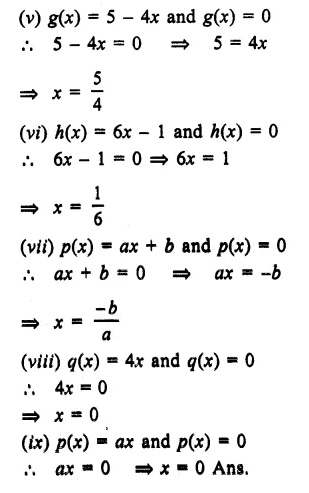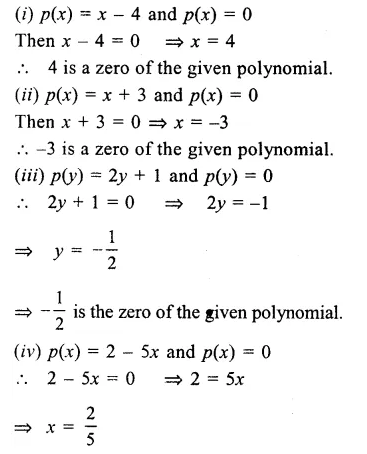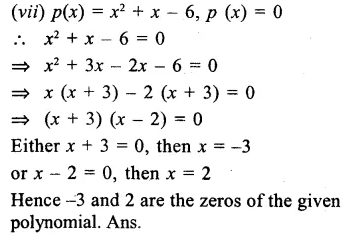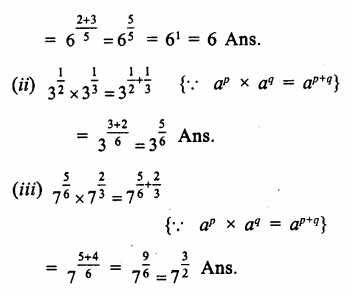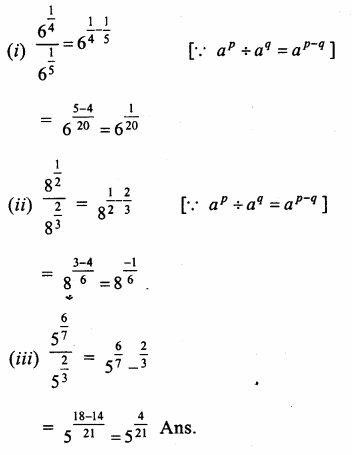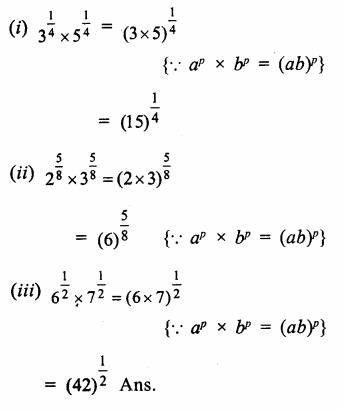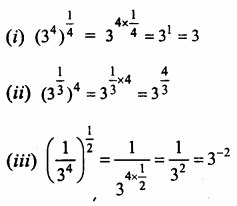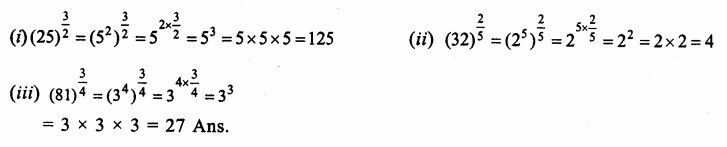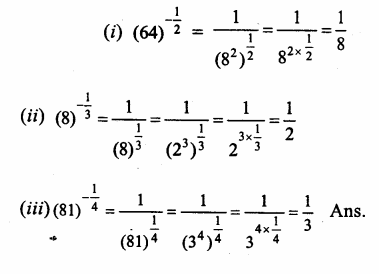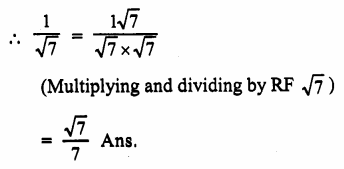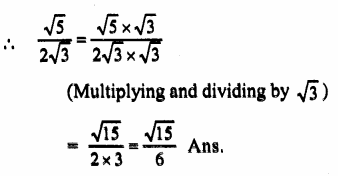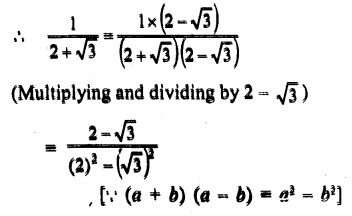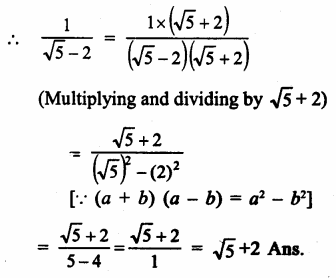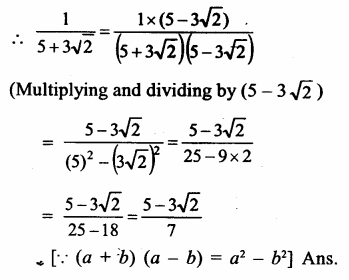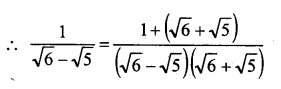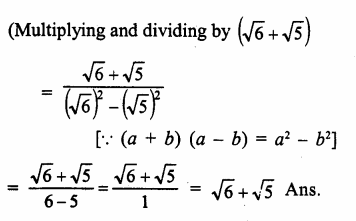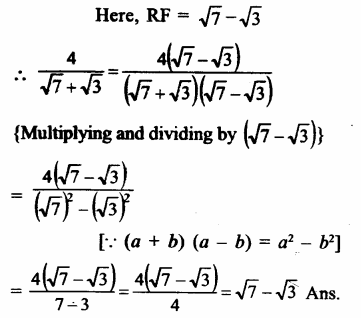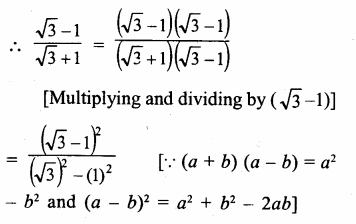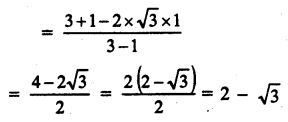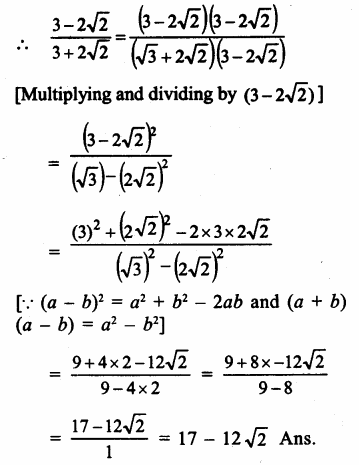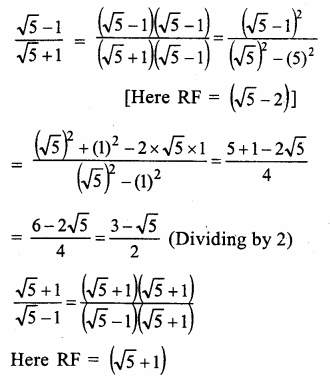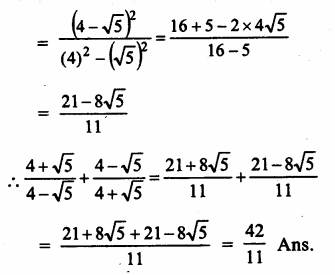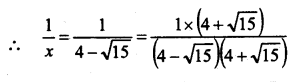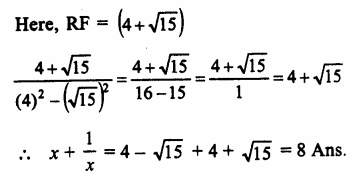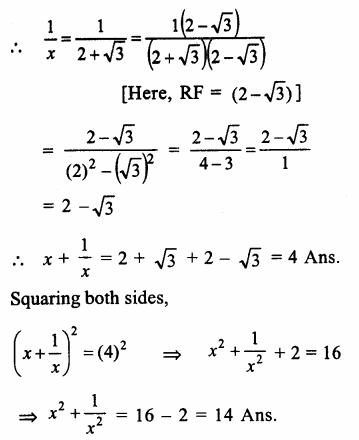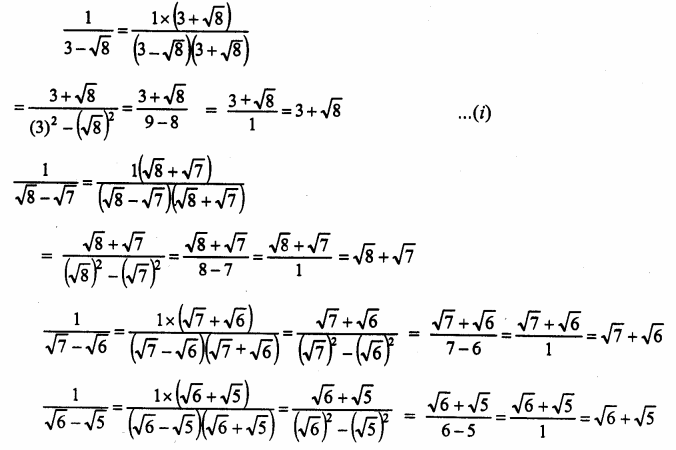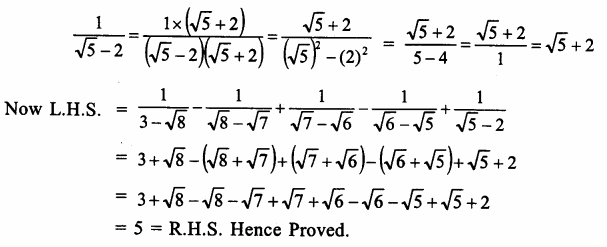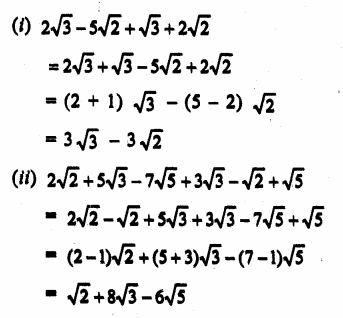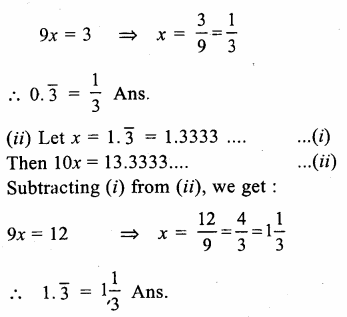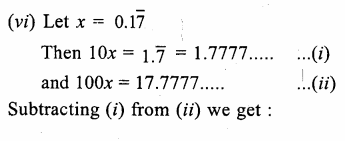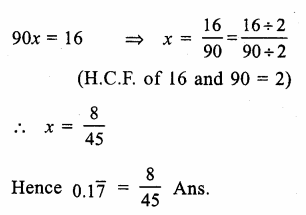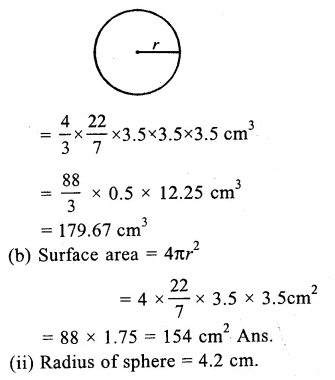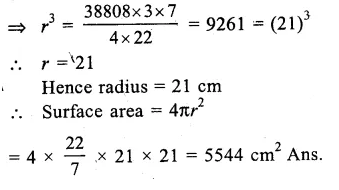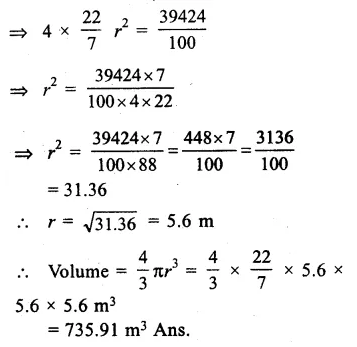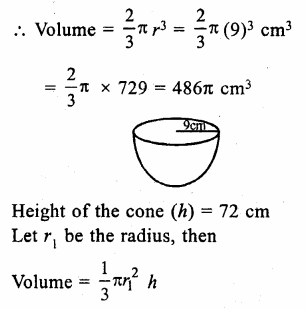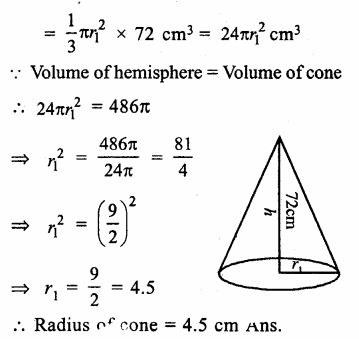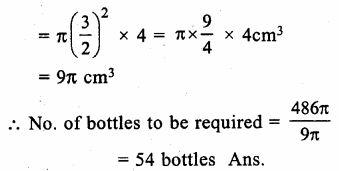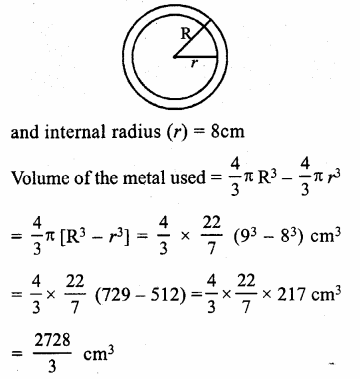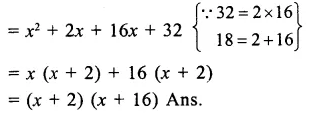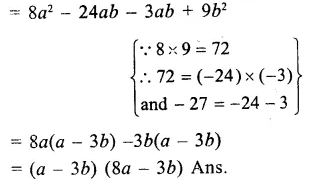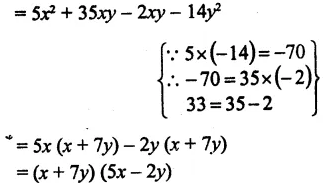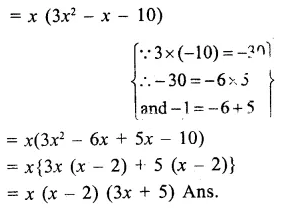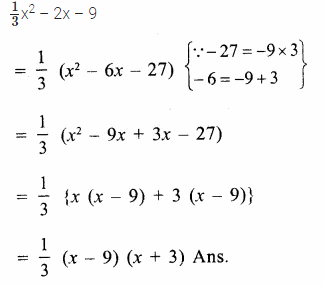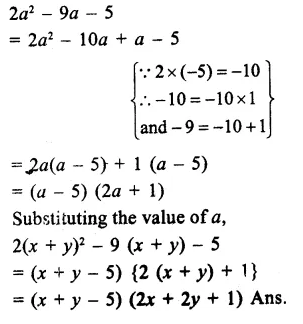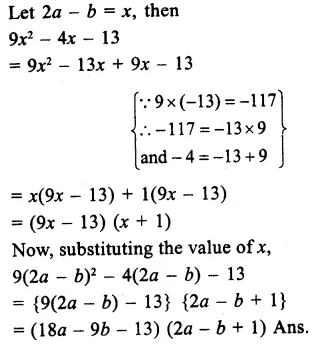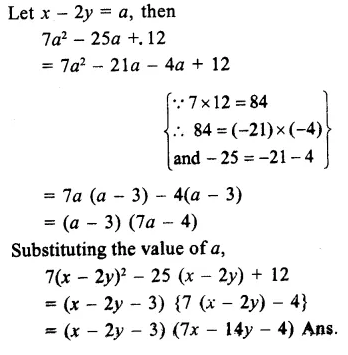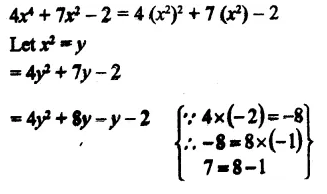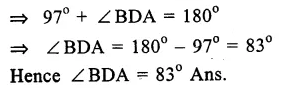RS Aggarwal Class 9 Solutions Chapter 3 Introduction to Euclid’s Geometry Ex 3A

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Class 9 Solutions Chapter 3 Introduction to Euclid’s Geometry Ex 3A.

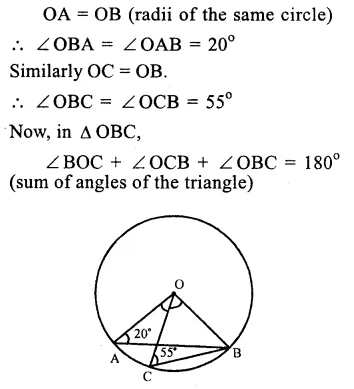
Question 1.
Solution:
A theorem is a statement that requires a proof while an axiom is the basic fact which is taken for granted without proof.

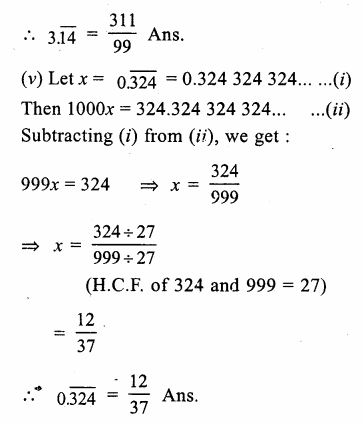
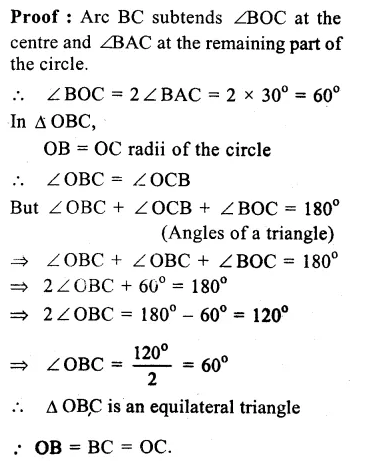
Question 2.
Solution:
(i) Line segment: The straight line between two points A and B is a called a line segment \(\overline { AB } \)

(ii) Ray : A line segment \(\overline { AB } \) when extended indefinitely is one direction is called a ray \(\overrightarrow { AB } \) It has no definitely length.

(iii) Intersecting lines : Two lines having one common point are called intersecting lines and the common point is called the point of intersection.

(iv) Parallel Lines : If two lines lying in the same plane do not intersect each other when produced on either side, then these two lines are called parallel lines. The distance between two parallel hues always remains the same.

(v) Half line : If we take a point P on a line \(\overleftrightarrow { AB } \), its divides the line into two parts. Each part is called half line or two ray i.e. \(\overrightarrow { PA } \) and \(\overrightarrow { PB } \) .

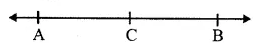
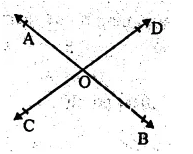
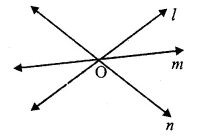
(vi) Concurrent lines : Three or more lines intersecting at the same point are called concurrent lines.

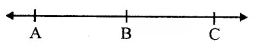
(vii) Collinear points : Three or more points lying on the same line are called collinear points.

(viii) Plane : A plane is a surface such that every point of the line joining any two points on it, lies on it.

Question 3.
Solution:
(i) Six points are : A, B, C, D, E and F
(ii) Five line segments are : \(\overline { EG }\), \(\overline { FH }\), \(\overline { EF }\), \(\overline { GH }\) and \(\overline { MN }\)
(iii) Four rays are : \(\overrightarrow { EP } \) , \(\overrightarrow { GR } \),\(\overrightarrow { GB } \) and \(\overrightarrow { HD } \)
(iv) Four lines are : \(\overrightarrow { AB } \),\(\overrightarrow { CD } \),\(\overrightarrow { PQ } \) and \(\overrightarrow { RS } \)
(v) Four collinear points are M, E, G, B. Ans

Question 4.
Solution:
(i) \(\overleftrightarrow { EF } \) and \(\overleftrightarrow { GH } \) is a pair of intersecting line whose point of intersection is R
and second pair of intersecting lines is \(\overleftrightarrow { AB } \) and \(\overleftrightarrow { CD } \) and point of intersection is P.
(ii) Three concurrent lines are \(\overleftrightarrow { AB } \), \(\overleftrightarrow { EF } \) and \(\overleftrightarrow { GH } \) and the point of intersection is R.
(iii) Three rays are \(\overleftrightarrow { RB } \),\(\overleftrightarrow { RH } \) and \(\overleftrightarrow { RG } \)
(iv) Two line segments are \(\overleftrightarrow { RQ } \) and \(\overleftrightarrow { RP } \)

Question 5.
Solution:
(i) Through a given point, infinitely many lines can be drawn.
(ii) Only one line can be drawn to pass through two given points.
(iii) Two lines can intersect each other at the most one point
(iv) A, B and C are three collinear points. Then the line segments will be \(\overline { AB } \), \(\overline { BC } \) and \(\overline { AC } \).

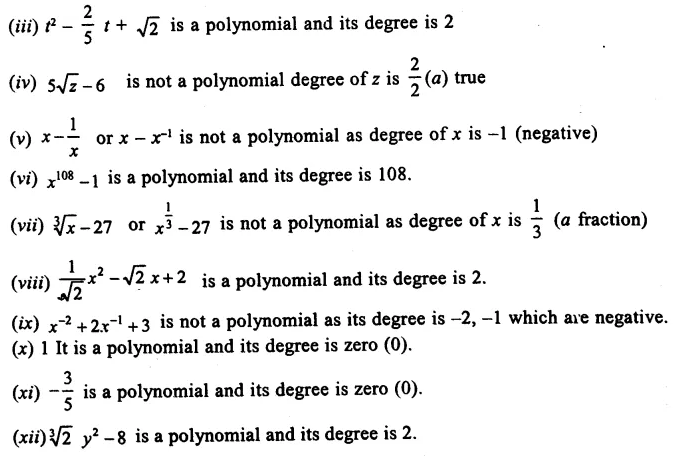
Question 6.
Solution:
(iv), (vi), (vii), (viii) and (ix) are true and others are not true.

 

Hope given RS Aggarwal Class 9 Solutions Chapter 3 Introduction to Euclid’s Geometry Ex 3A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2B

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2B.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2A
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2B
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2C
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2D
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2E
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2F
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2G
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2H
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2I
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2J
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2K

Question 1.
Solution:
p(x) = 5 – 4x + 2x²

Question 2.
Solution:
p(y) = 4 + 3y – y² + 5y³

Question 3.
Solution:
f(t)=4t²-3t+6

Question 4.
Solution:

Question 5.
Solution:

Hope given RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2A

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2A.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2A
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2B
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2C
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2D
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2E
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2F
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2G
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2H
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2I
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2J
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2K

Question 1.
Solution:
(i) x⁵-2x³+x+7 is a polynomial and its degree is 5
(ii) y³-√3y is a polynomial and its degree is 3

Question 2.
Solution:
(i) Degree is 1
(ii) degree is 3
(iii) degree is zero
(iv) degree is 7
(v) degree is 10
(vi) degree is 2

Question 3.
Solution:
(i) Coefficient of x³ is -5.
(ii) Coefficient of x is -2√2
(iii) Coefficient of x² is \(\frac { \pi }{ 3 } \)
(iv) Coefficient of x² is 0

Question 4.
Solution:
(i) Example of a binomial of degree 27 is 4x²⁷ – 5
(ii) Example of a monomial of degree 16 is x¹⁶
(iii) Example of trinomial of degree 3 is 2x³ + 7x + 4

Question 5.
Solution:
(i) 2x² + 4x is a quadratic polynomial. (Degree 2)
(ii) x – x³ is a cubic polynomial (Degree 3)
(iii) 2 – y – y² is a quadratic polynomial (Degree 2)
(iv) – 7 + z is a linear polynomial (Degree 1)
(v) 5t is a linear polynomial (Degree 1)
(vi) p³ is a cubic polynomial (Degree 3)

 

Hope given RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1F

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1F.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1A
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1B
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1C
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1D
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1E
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1F

Question 1.
Solution:
We know that
a^p x a^q = a^p+q
∴ Therefore

Question 2.
Solution:
We know that
a^p ÷ a^q = a^p-q
Therefore

Question 3.
Solution:
We know that
a^p x b^p = (ab)^p
Therefore

Question 4.
Solution:
We know that
(a^p)^q =a^pq

Question 5.
Solution:

Question 6.
Solution:

Question 7.
Solution:

Hope given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1F are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1E

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1E.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1A
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1B
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1C
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1D
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1E
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1F

Rationalise the denominator of each of the followings :

Question 1.
Solution:
Here,RF of √7 is √7

Question 2.
Solution:
Here RF √3 is √3

Question 3.
Solution:
Here RF of \(\frac { 1 }{ \left( { 2+ }\sqrt { 3 } \right) }\) is \(\frac { 1 }{ \left( { 2- }\sqrt { 3 } \right) }\)

Question 4.
Solution:
Here RF is √5 + 2

Question 5.
Solution:
Here RF is 5 – 3√2

Question 6.
Solution:
Here RF is √6 + √5

Question 7.
Solution:
Here RF = √7 – √3

Question 8.
Solution:
Here RF = √3 – 1

Question 9.
Solution:
Here RF = (3-2√2)

Find the values of a and b in each of the following :

Question 10.
Solution:
\(\frac { \sqrt { 3 } +1 }{ \sqrt { 3 } -1 } \), RF = √3+1
(Multiplying and dividing by √3+1)

Question 11.
Solution:
\(\frac { 3+\sqrt { 2 } }{ 3-\sqrt { 2 } } \), RF is 3+√2
(Multiplying and dividing by 3+√2)

Question 12.
Solution:
In \(\frac { 5-\sqrt { 6 } }{ 5+\sqrt { 6 } } \), RF is (5-√6)
(Multiplying and dividing by 5-√6)

Question 13.
Solution:
In \(\frac { 5+2\sqrt { 3 } }{ 7+4\sqrt { 3 } } \), RF is 7-4√3
(Multiplying and dividing by 7-4√3)

Question 14.
Solution:

Question 15.
Solution:

Question 16.
Solution:
x = (4-√15)

Question 17.
Solution:
x = 2+√3

Question 18.
Solution:

Hope given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1E are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1D

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1D.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1A
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1B
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1C
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1D
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1E
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1F

Question 1.
Solution:

Question 2.
Solution:

Question 3.
Solution:

Question 4.
Solution:

Question 5.
Solution:
(i) Draw a line segment AB = 3.2 units (cm) and extend it to C such that BC = 1 unit.

(ii) Find the mid-point O of AC.
(iii) With centre O and OA as radius draw a semicircle on AC
(iv) Draw BD ⊥ AC meeting the semicircle at D.
(v) Join BD which is √3.2 units.
(vi) With centre B and radius BD, draw an arc meeting AC when produced at E.
Then BE = BD = √3.2 units. Ans.

Question 6.
Solution:
(i) Draw a line segment AB = 7.28 units and produce is to C such that BC = 1 unit (cm)

(ii) Find the mid-point O of AC.
(iii) With centre O and radius OA, draw a semicircle on AC.
(iv) Draw a perpendicular BD at AC meeting the semicircle at D
Then BD = √7.28 units.
(v) With centre B and radius BD, draw an arc which meet AC produced at E.
Then BE = BD = √7.28 units.

Question 7.
Solution:
(A) For Addition
(i) Closure property: The sum of two real numbers is always a real number.
(ii) Associative Law : (a + b) + c = a + (b + c), for all values of a, b and c.
(iii) Commutative Law : a + b = b + a for all real values of a and b.
(iv) Existance of Additive Identity : 0 is the real number such that: 0 + a = a + 0 = afor every real value of a.
(v) Existance of addtive inverse : For each real value of a, there exists a real value (-a) such that a + (-a) = (-a) + a = 0, Then (a) and (-a) are called the additive inverse of each other.
(v) Existence of Multiplicative Inverse. For each non zero real number a, there exists a real number \(\frac { 1 }{ a }\) such that a . \(\frac { 1 }{ a }\) = \(\frac { 1 }{ a }\) . a = 1
a and \(\frac { 1 }{ a }\) are called multiplicative inverse or reciprocal of each other.
(B) Multiplication
(i) Closure property: The product of two real numbers is always a real number.
(ii) Associative law : ab(c) = a(bc) for all real values of a, b and c
(iii) Commutative law : ab=ba for all real numbers a and b
(iv) Existance of Multiplicative Identity: clearly is a real number such that 1.a = a.1 = a for every value of a.

Hope given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1C

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1C.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1A
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1B
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1C
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1D
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1E
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1F

Question 1.
Solution:
Irrational numbers : Numbers which are not rational numbers, are called irrational numbers. Rational numbers can be expressed in terminating decimals or repeating decimals while irrational number can’t.
\(\frac { 1 }{ 2 } \) , \(\frac { 2 }{ 3 } \) , \(\frac { 7 }{ 5 } \) etc.are rational numbers and π, √2, √3, √5, √6….etc are irrational numbers

Question 2.
Solution:
(i) √4 = ±2, it is a rational number
(ii) √196 = ±14 it is a rational number
(iii) √21 It is irrational number.
(iv) √43 It is irrational number.
(v) 3 + √3 It is irrational number because sum of a rational and an irrational number is irrational
(vi) √7 – 2 It is irrational number because difference of a rational and irrational number is irrational
(vii) \(\frac { 2 }{ 3 } \)√6 . It is irrational number because product of a rational and an irrational number is an irrational number.
(viii) 0.\(\overline { 6 } \) = 0.6666…. It is rational number because it is a repeating decimal.
(ix) 1.232332333…. It is irrational number because it not repeating decimal
(x) 3.040040004…. It is irrational number because it is not repeating decimal.
(xi) 3.2576 It is rational number because it is a terminating decimal.
(xii) 2.3565656…. = 2.3 \(\overline { 56 } \) It is rational number because it is a repeating decimal.
(xiii) π It is an irrational number
(xiv) \(\frac { 22 }{ 7 } \). It is a rational number which is in form of \(\frac { p }{ q } \) Ans.

Question 3.
Solution:
(i) Let X’OX be a horizontal line, taken as the x-axis and let O be the origin. Let O represent 0.
Taken OA = 1 unit and draw AB ⊥ OA such that AB = 1 unit. Join OB, Then,

Question 4.
Solution:
Firstly we represent √5 on the real line X’OX. Then we will find √6 and √7 on that real line.
Now, draw a horizontal line X’OX, taken as x-axis

Question 5.
Solution:
(i) 4 + √5 : It is irrational number because in it, 4 is a rational number and √5 is irrational and sum of a rational and an irrational is also an irrational.
(ii) (-3 + √6) It is irrational number because in it, -3 is a rational and √6 is irrational and sum or difference of a rational and irrational is an irrational.
(iii) 5√7 : It is irrational because 5 is rational and √7 is irrational and product of a rational and an irrational is an irrational.
(iv) -3√8 : It is irrational because -3 is a rational and √8 is an irrational and product of a rational and an irrational is also an irrational.
(v) \(\frac { 2 }{ \sqrt { 5 } } \) It is irrational because 2 is a rational and √5 is an irrational and quotient of a rational and an irrational is also an irrational.
(vi) \(\frac { 4 }{ \sqrt { 3 } } \) It is irrational because 4 is a rational and √3 is an irrational number and quotient of a rational and irrational is also an irrational.

Question 6.
Solution:
(i) True.
(ii) False, as the sum of two irrational number is irrational is not always true.
(iii) True.
(iv) False, as the product of two irrational numbers is irrational is not always true.
(v) True.
(vi) True.
(vii) False as a real number can be either rational or irrational.

Hope given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 1 Real Numbers Ex 1B

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1B.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1A
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1B
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1C
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1D
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1E
• RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1F

Question 1.
Solution:
We know that a fraction \(\frac { p }{ q } \) is terminating if prime factors of q are 2 and 5 only.
Hence.
(i) \(\frac { 13 }{ 80 } \) and \(\frac { 16 }{ 125 } \) are the terminating decimals.

Question 2.
Solution:

Question 3.
Solution:
(i) Let,x = 0.\(\overline { 3 } \) = 0.3333…(i)
Then, 10x = 3.3333….

Question 4.
Solution:
(i) True, because set of natural numbers is a subset of whole number.
(ii) False, because the number 0 does not belong to the set of natural numbers.
(iii) True, because a set of integers is a subset of a rational numbers.
(iv) False, because the set of rational numbers is not a subset of whole numbers.
(v) True, because rational number can be expressed as terminating or repeating decimals.
(vi) True, because every rational number can be express as repeating decimals.
(vii) True, because 0 = \(\frac { 0 }{ 1 } \), which is a rational number Ans.

Hope given RS Aggarwal Solutions Class 9 Chapter 1 Real Numbers Ex 1B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Online Education for RS Aggarwal Class 9 Solutions Chapter 13 Volume and Surface Area Ex 13D

These Solutions are part of Online Education RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Class 9 Solutions Chapter 13 Volume and Surface Area Ex 13D.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 13 Volume and Surface Area Ex 13A
• RS Aggarwal Solutions Class 9 Chapter 13 Volume and Surface Area Ex 13B
• RS Aggarwal Solutions Class 9 Chapter 13 Volume and Surface Area Ex 13C
• RS Aggarwal Solutions Class 9 Chapter 13 Volume and Surface Area Ex 13D

Question 1.
Solution:
(i) Radius of sphere = 3.5cm
(a) Volume = \(\frac { 4 }{ 3 } \) πr³

Question 2.
Solution:
Let r be the radius of the sphere and volume = 38808 cm³
∴\(\frac { 4 }{ 3 } \) πr³ = 38803
=> \(\frac { 4 }{ 3 } \) x \(\frac { 22 }{ 7 } \) r³ = 38803

Question 3.
Solution:
Let r be the radius of the sphere
∴ Volume = \(\frac { 4 }{ 3 } \) πr³

Question 4.
Solution:
Surface area of a sphere = 394.24 m²
Let r be the radius, then 4πr² = 394.24

Question 5.
Solution:
Surface area of sphere = 576π cm²
Let r be the radius, then 4r² = 576π

Question 6.
Solution:
Outer diameter of shell = 12cm,
Outer radius (R) = \(\frac { 12 }{ 2 } \) = 6cm
and inner diameter = 8cm

Question 7.
Solution:
Length of cuboid of (l) = 12cm
Breadth (b) = 11cm
and height (h) = 9cm

Question 8.
Solution:
Radius of sphere (r) = 8cm
Volume = \(\frac { 4 }{ 3 } \)πr³

Question 9.
Solution:
Radius of solid sphere (R) = 3cm.
Volume = \(\frac { 4 }{ 3 } \)π(R)³ = \(\frac { 4 }{ 3 } \)π(3)³ cm³

Question 10.
Solution:
Radius of metallic sphere (R) = 10.5cm

Question 11.
Solution:
Diameter of a cylinder = 8cm
Radius (r) = \(\frac { 8 }{ 2 } \) = 4cm

Question 12.
Solution:
Diameter of sphere = 6cm
Radius (R) = \(\frac { 6 }{ 2 } \) = 3cm

Question 13.
Solution:
Diameter of sphere = 18cm
Radius (R) = \(\frac { 18 }{ 2 } \) = 9cm.

Question 14.
Solution:
Diameter of the sphere = 15.6 cm
Radius (R) = \(\frac { 15.6 }{ 2 } \) = 7.8 cm

Question 15.
Solution:
Diameter of the canonball = 28cm
Radius (R) = \(\frac { 28 }{ 2 } \) = 14 cm

Question 16.
Solution:
Given,
Radius of spherical big ball (R) = 3cm

Question 17.
Solution:
Ratio in the radii of two spheres = 1:2
Let radius of smaller sphere = r then,
radius of bigger sphere = 2r

Question 18.
Solution:
Let r1 and r2 be the radii of two spheres

Question 19.
Solution:
Radius of the cylindrical tub = 12cm.
First level of water = 20cm
Raised water level = 6.75cm.

Question 20.
Solution:
Radius of the ball (r) = 9cm.
Volume of ball = \(\frac { 4 }{ 3 } \)πr³

Question 21.
Solution:
Given,
Radius of hemisphere of lead (r) = 9cm.

Question 22.
Solution:
Given,
Radius of hemispherical bowl (r) = 9cm

Question 23.
Solution:
External radius of spherical shell (R) = 9cm

Question 24.
Solution:
Inner radius (r) = 4 cm
Thickness of steel used = 0.5

Hope given RS Aggarwal Class 9 Solutions Chapter 13 Volume and Surface Area Ex 13D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Online Education for RS Aggarwal Class 9 Solutions Chapter 2 Polynomials Ex 2G

These Solutions are part of Online Education RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2G.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2A
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2B
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2C
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2D
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2E
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2F
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2G
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2H
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2I
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2J
• RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2K

Factorize :

Question 1.
Solution:
x² + 11x + 30

Question 2.
Solution:
x² + 18x + 32

Question 3.
Solution:
x² + 7x – 18

Question 4.
Solution:
x² + 5x – 6

Question 5.
Solution:
y² – 4y + 3

Question 6.
Solution:
x² – 21x + 108

Question 7.
Solution:
x² – 11x – 80

Question 8.
Solution:
x² – x – 156

Question 9.
Solution:
z² – 32z – 105

Question 10.
Solution:
40 + 3x – x²

Question 11.
Solution:
6 – x – x²

Question 12.
Solution:
7x² + 49x + 84

Question 13.
Solution:
m² + 17mn – 84n²

Question 14.
Solution:
5x² + 16x + 3

Question 15.
Solution:
6x² + 17x + 12

Question 16.
Solution:
9x² + 18x + 8

Question 17.
Solution:
14x² + 9x + 1

Question 18.
Solution:
2x² + 3x – 90

Question 19.
Solution:
2x² + 11x – 21

Question 20.
Solution:
3x² – 14x + 8

Question 21.
Solution:
18x² + 3x- 10

Question 22.
Solution:
15x² + 2x – 8

Question 23.
Solution:
6x² + 11x – 10

Question 24.
Solution:

Question 25.
Solution:
24x² – 41x + 12

Question 26.
Solution:
2x² – 7x – 15

Question 27.
Solution:
6x² – 5x – 21

Question 28.
Solution:
10x² – 9x – 7

Question 29.
Solution:
5x² – 16x – 21

Question 30.
Solution:
2x² – x – 21

Question 31.
Solution:
15x² – x – 28

Question 32.
Solution:
8a² – 27ab + 9b²

Question 33.
Solution:
5x² + 33xy -14y²

Question 34.
Solution:
3x³ – x² – 10x

Question 35.
Solution:

Question 36.
Solution:

Question 37.
Solution:
√2x² + 3x + √2

Question 38.
Solution:
√5x² + 2x – 3√5

Question 39.
Solution:
2a² + 3√3x + 3

Question 40.
Solution:
2√3x² + x – 5√3

Question 41.
Solution:
5√5x² + 20x + 3√5

Question 42.
Solution:
7√x² – 10x – 4√2

Question 43.
Solution:
6√3 x² – 47x + 5√3

Question 44.
Solution:
7x² + 2√14x + 2

Question 45.
Solution:
2(x + y)² – 9(x + y) – 5

Question 46.
Solution:
9(2a-b)²-4(2a-b)-13

Question 47.
Solution:
7(x – 2y)² – 25 (x – 2y) + 12

Question 48.
Solution:
4x⁴ + 7x²-2

Hope given RS Aggarwal Solutions Class 9 Chapter 2 Polynomials Ex 2G are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Online Education for RS Aggarwal Class 9 Solutions Chapter 11 Circle Ex 11B

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11B.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11A
• RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11B
• RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11C

Question 1.
Solution:
(i) O is the centre of the circle
∠OAB = 40°, ∠OCB = 30°
Join OB.

Question 2.
Solution:
O is the centre of the cirlce and ∠AOB = 70°
∵ Arc AB subtends ∠AOB at the centre and ∠ACB at the remaining part of the circle.
∵ ∠ACB = \(\frac { 1 }{ 2 } \) ∠AOB = \(\frac { 1 }{ 2 } \) x 70°
=> ∠ACB = 35°
or ∠OCA = 35°
In ∆OAC,
OA = OC (radii of the same circle)
∴ ∠OAC = ∠OCA = 35° Ans.

Question 3.
Solution:
In the figure, O is the centre of the circle. ∠PBC = 25°, ∠APB =110°
∠ APB + ∠ BPC = 180° (Linear pair)
=> 110° + ∠ BPC = 180°

Question 4.
Solution:
O is the centre of the circle
∠ABD = 35° and ∠B AC = 70°
BOD is the diameter of the circle
∠BAD = 90° (Angle in a semi circle)
But ∠ADB + ∠ABD + ∠BAD = 180° (Angles of a triangle)
=> ∠ADB + 35° + 90° = 180°
=> ∠ADB + 125° = 180°
=> ∠ADB = 180° – 125° = 55°
But ∠ACB = ∠ADB (Angles in the same segment of the circle)
∠ACB = 55° Ans.

Question 5.
Solution:
O is the centre of a circle and ∠ACB = 50°
∴ arc AB subtends ∠ AOB at the centre and ∠ ACB at the remaining part of the circle.
∴ ∠ AOB = 2 ∠ ACB
= 2 x 50° = 100
∴ OA = OB (radii of the same circle)
∴ ∠ OAB = ∠ OBA (Angles opposite to equal sides)
Now in ∆ OAB,
∠ OAB + ∠ OBA + ∠ AOB = 180°
=> ∠ OAB + ∠ OAB + ∠ AOB = 180° (∠OAB = ∠OBA)
=> 2 ∠ OAB + 100°= 180°
=> 2 ∠ OAB = 180° – 100° = 80°
=> ∠OAB = \(\frac { { 80 }^{ o } }{ 2 } \) = 40°
Hence, OAB = 40° Ans.

Question 6.
Solution:
(i) In the figure,
∠ABD = 54° and ∠BCD = 43°
∠BAD = ∠BCD (Angles in the same segment of a circle)
∠BAD = 43°

Question 7.
Solution:
Chord DE || diameter AC of the circle with centre O.
∠CBD = 60°
∠CBD = ∠ CAD
(Angles in the same segment of a circle)
∠CAD = 60°
Now in ∆ ADC,

Question 8.
Solution:
In the figure,
chord CD || diameter AB of the circle with centre O.
∠ ABC = 25°
Join CD and DO.
AB || CD
∠ ABC = ∠ BCD (alternate angles)

Question 9.
Solution:
AB and CD are two straight lines passing through O, the centre of the circle and ∠AOC = 80°, ∠CDE = 40°
∠ CED = 90° (Angle in a semi circle)

Question 10.
Solution:
O is the centre of the circle and ∠AOB = 40°, ∠BDC = 100°
Arc AB subtends ∠AOB at the centre and ∠ ACB at the remaining part of the circle
∠ AOB = 2 ∠ ACB

Question 11.
Solution:
Chords AC and BD of a circle with centre O, intersect each other at E at right angles.
∠ OAB = 25°. Join OB.
In ∆ OAB,
OA = OB (radii of the same circle)

Question 12.
Solution:
In the figure, O is the centre of a circle ∠ OAB = 20° and ∠ OCB = 55° .
In ∆ OAB,

Question 13.
Solution:
Given : A ∆ ABC is inscribed in a circle with centre O and ∠ BAC = 30°
To Prove : BC = radius of the circle
Const. Join OB and OC

Question 14.
Solution:
In a circle with centre O and PQ is its diameter. ∠PQR = 65°, ∠SPR = 40° and ∠PQM = 50°
(i) ∠PRQ = 90° (Angle in a semicircle) and ∠PQR + ∠RPQ + ∠PQR = 180° (Angles of a triangle)

Hope given RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.