Tag: Selina Publishers Mathematics for Class 10

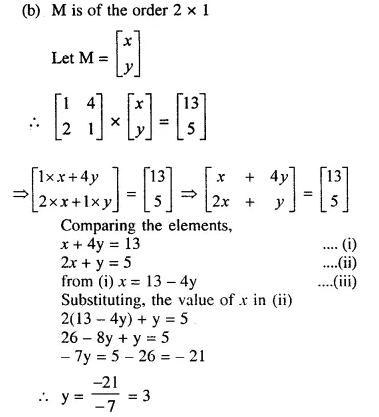
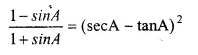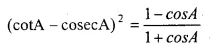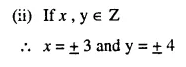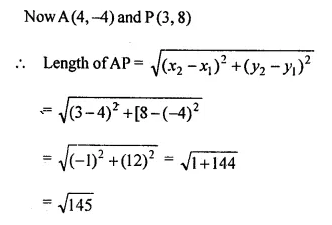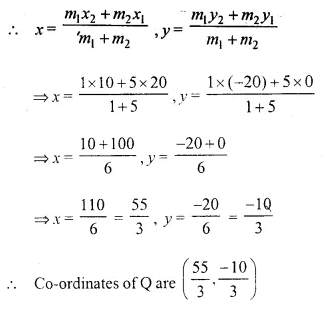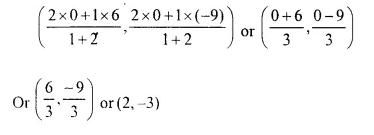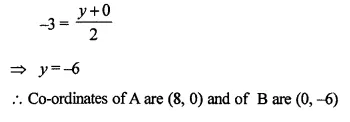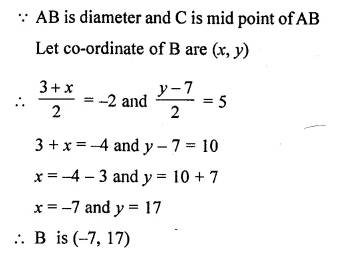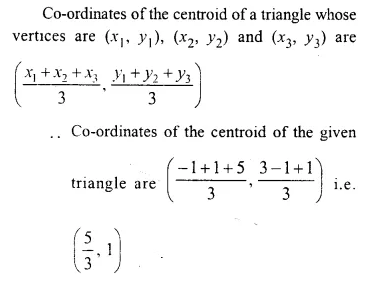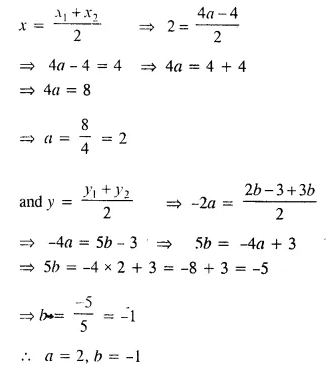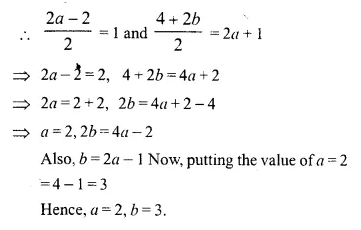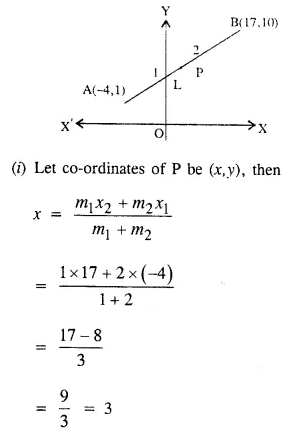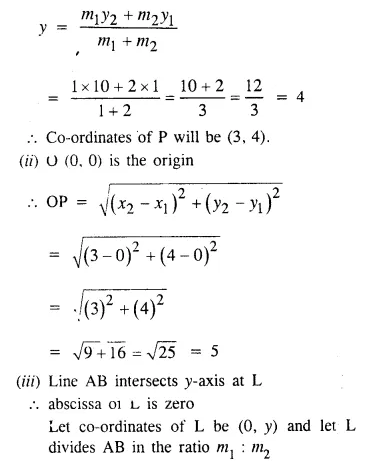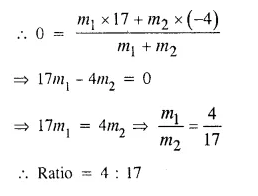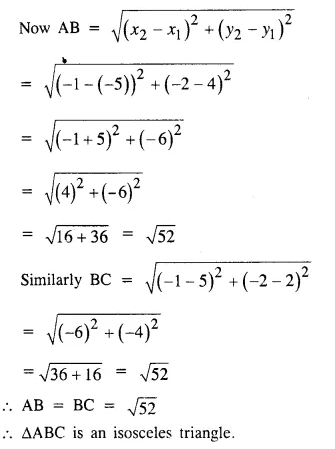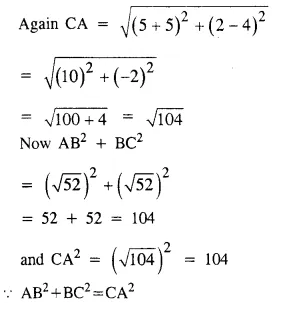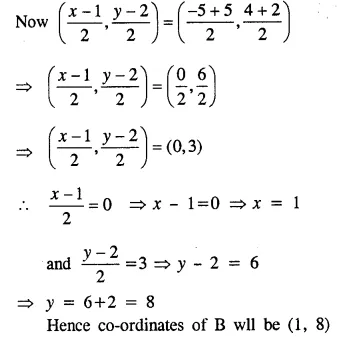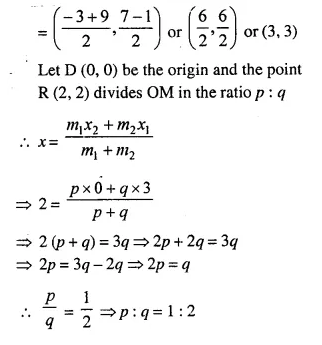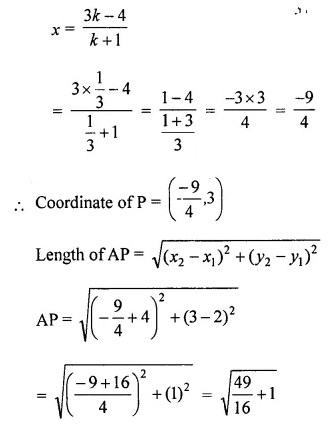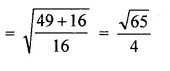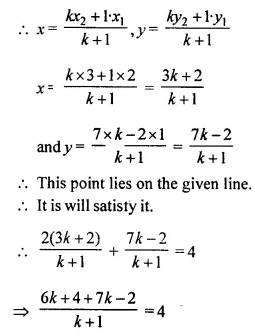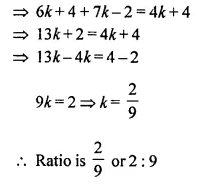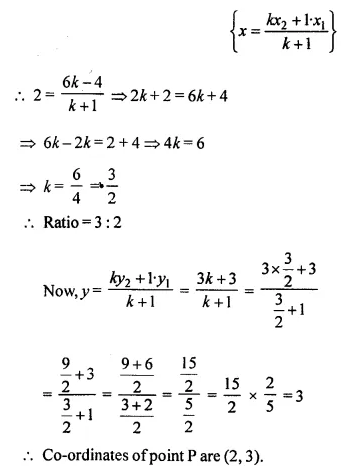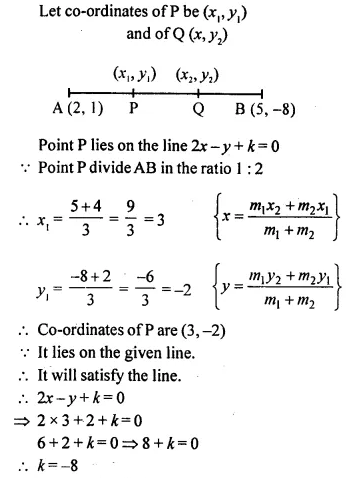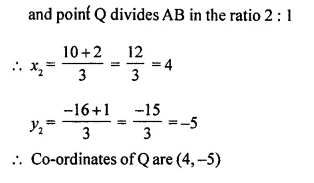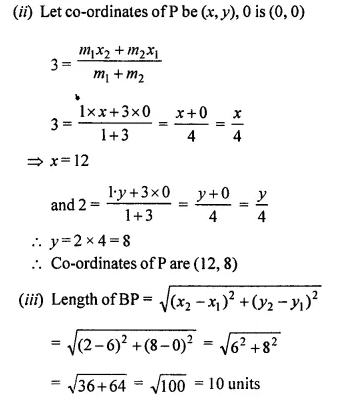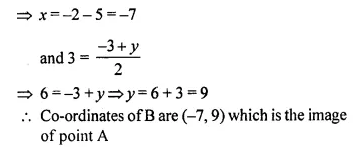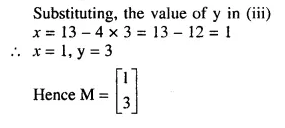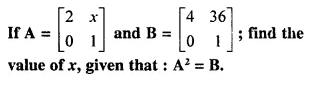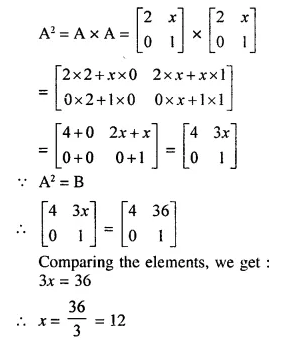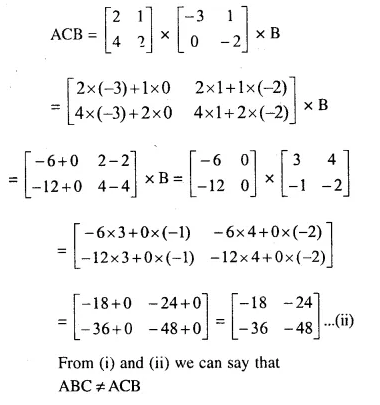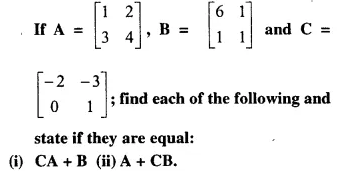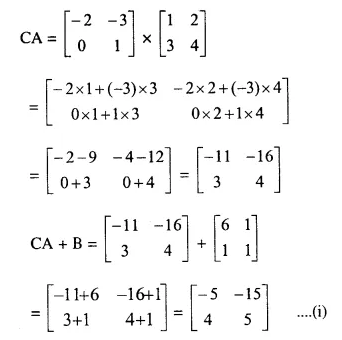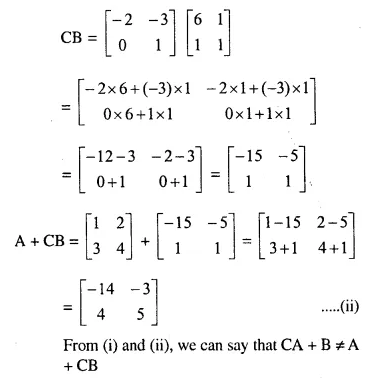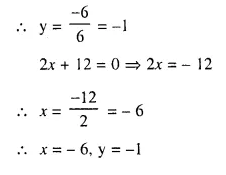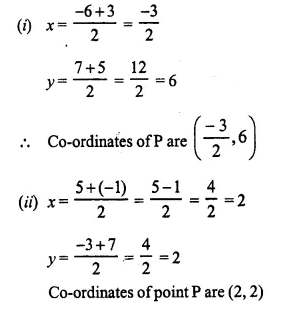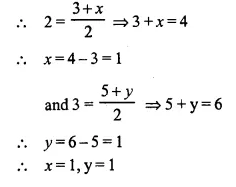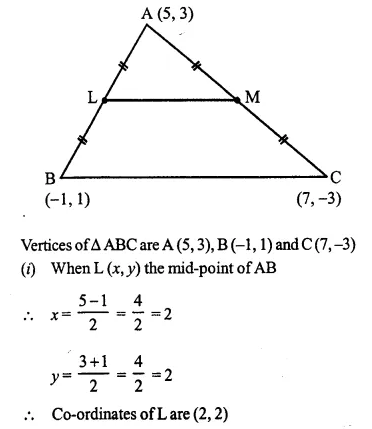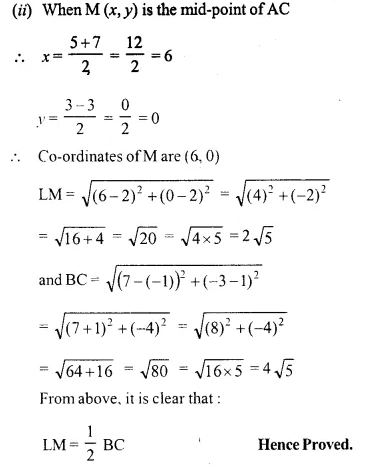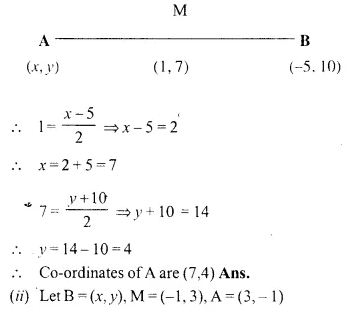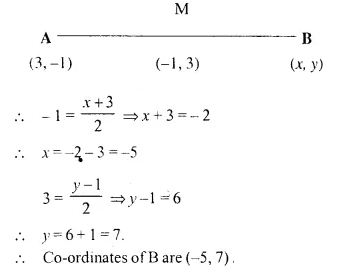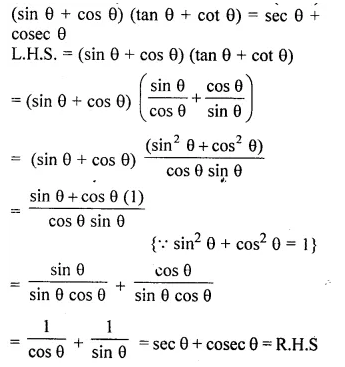Selina Concise Mathematics Class 10 ICSE Solutions Chapter 14 Equation of a Line Ex 14A

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 14 Equation of a Line Ex 14A.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 14 Equation of a Line Ex 14A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 14 Equation of a Line Ex 14B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 14 Equation of a Line Ex 14C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 14 Equation of a Line Ex 14D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 14 Equation of a Line Ex 14E

Question 1.
Find, which of the following points lie on the line x – 2y + 5 = 0 :
(i) (1, 3)
(ii) (0, 5)
(iii) (-5, 0)
(iv) (5, 5)
(v) (2, -1.5)
(vi) (-2, -1.5)
Solution:
Equation of given line x – 2y + 5 = 0
(i) Substituting x = 1, y = 3, in the given equation.
1 – 2 x 3 + 5 = 0 ⇒ 1 – 6 + 5 = 0 ⇒ 0 = 0, which is true.
(1, 3) satisfies the equation.
(ii) Substituting x = 0 , y = 5 in the given equation
0 – 2 x 5 + 5 = 0 ⇒ 0 – 10 + 5 = 0 ⇒ -5 = 0, which is not true.
( 0, 5) does not satisfy the equation.
(iii) Substituting x = – 5, y = 0 in the given equation
-5 – 2 x 0 + 5 = 0 ⇒ -5 – 0 + 5 = 0 ⇒ 0 = 0 which is true.
(-5, 0) satisfies the equation.
(iv) Substituting x = 5, y = 5 in the given equation.
– 5 – 2 x 5 + 5 = 0 ⇒ -5 – 10 + 5 = 0 ⇒ 0 = 0 which is true.
(5, 5) satisfies the equation.
(v) Substituting x = 2, y = -1.5 in the given equation.
2 – 2 x (- 1.5) + 5 = 0 ⇒ 2 + 3 + 5 = 0 ⇒ 10 = 0. which is not true.
(2, -1.5) does not satisfy the equation.
(vi) Substituting x = -2, y = -1.5 in the given equation
– 2 – 2 x (-1.5) + 5 = 0 ⇒ – 2 + 3 + 5 = 0 ⇒ 6 = 0, which is not true.
(-2, -1.5) does not satisfies the equation.

Question 2.
State, true or false :
(i) the line \(\frac { x }{ 2 }\) + \(\frac { y }{ 3 }\) = 0 passes through the point (2, 3).
(ii) the line \(\frac { x }{ 2 }\) + \(\frac { y }{ 3 }\) = 0 passes through the point (4, -6).
(iii) the point (8, 7) lies on the line y – 7 = 0
(iv) the point (-3, 0) lies on the line x + 3 = 0
(v) if the point (2, a) lies on the line 2x – y = 3, then a = 5.
Solution:
(i) Equation of the line is \(\frac { x }{ 2 }\) + \(\frac { y }{ 3 }\) = 0
and co-ordinates of point are (2, 3)
If the point is on the line, then it will satisfy the equation.

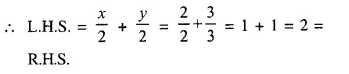
(2, 3) is not on the line
(ii) Equation of the line is \(\frac { x }{ 2 }\) + \(\frac { y }{ 3 }\) = 0
and co-ordinates of point are (4, -6)
If the point is on the line, then it will satisfy the equation

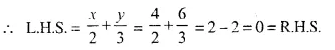
Hence, point (4, -6) is on the line.
(iii) Equation of line is y – 7 = 0 and the co-ordinates of point are (8, 7)
If the point is on the line, then it will satisfy the equation
L.H.S. = y – 7 = 7 – 7 = 0 = R.H.S.
Hence, point (8, 7) is on the line.
(iv) Equation of the line is x + 3 = 0 and co-ordinates of point are (-3, 0)
If the point is on the line, then it will satisfy the equation.
L.H.S. = x + 3 = -3 + 3 = 0 = R.H.S.
Hence, the point (-3, 0) is on the line.
(v) Equation of the line is 2x – y = 3
and co-ordinates of the point are (2, a)
If the point is on the line, then it will satisfy the equation.
L.H.S. = 2x – y = 2 x 2 – a = 4 – a
R.H.S. = 3
4 – a = 3 ⇒ 4 + 3 = a ⇒ a = 7
But a = 5 given, therefore it is not on the line.
(i) False (ii) True (iii) True (iv) True (v) False.

Question 3.
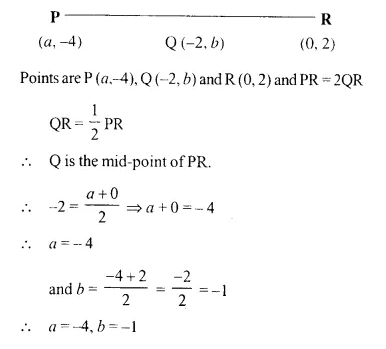
The line given by the equation 2x – \(\frac { y }{ 3 }\) = 7 passes through the point (k, 6); calculate the value of k.
Solution:

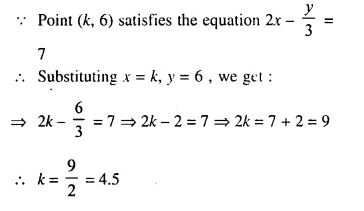
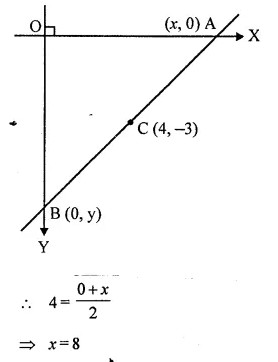
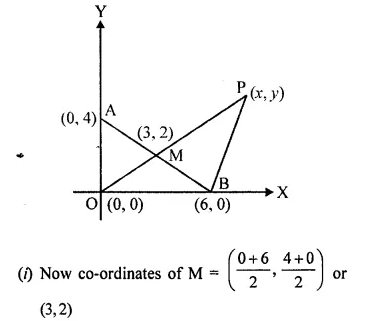
Question 4.
For what value of k will the point (3, -k) lie on the line 9x + 4y = 3 ?
Solution:
Point (3, -k) satisfies the equation 9x + 4y = 3
Substituting x = 3 , y = -k, we get :
9 x 3 + 4 (- k), = 3
⇒ 27 – 4k = 3
⇒ – 4k = 3 – 27
⇒ – 4k = – 24
⇒ k = 6

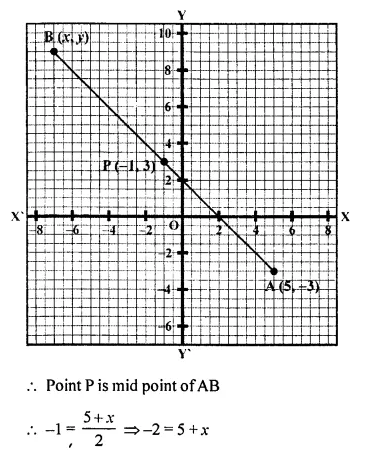
Question 5.
The line \(\frac { 3x }{ 5 }\) – \(\frac { 2y }{ 3 }\) + 1 = 0, contains the point (m, 2m – 1); calculate the value of m.
Solution:
Point (m, 2m -1) satisfies the equation

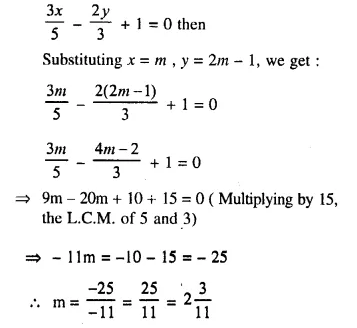
Question 6.
Does the line 3x – 5y = 6 bisect the join of (5, -2) and (-1, 2) ?
Solution:
Line 3x – 5y = 6 bisect the join of points (5, -2) and (-1, 2)
The mid-point of (5, -2) and (-1, 2) satisfies the equation.
Now, mid-point of (5, -2) and (-1, 2)

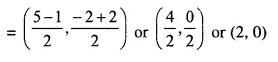
Now, substituting x = 2, y = 0, in the given equation
3 x 2 – 5 x 0 = 6 ⇒ 6 – 0 = 6 ⇒ 6 = 6 which is true. .
Given line bisect the join of points (5, -2) and (-1, 2)

Question 7.
(i) The line y = 3x – 2 bisects the join of (a, 3) and (2, -5), find the value of k.
(ii) The line x – 6y + 11 = 0 bisects the join of (8, -1) and ( 0, k). Find the value of k.
Solution:
(i) line y = 3x – 2 bisects the join of (a, 3) and (2, -5)
Mid-point join of there points satisfies it.
Now, mid-point of (a, 3) and (2, -5) is

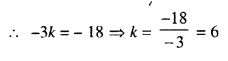
Question 8.
(i) The point (-3, -2) lies on the line ax + 3y + 6 = 0, calculate the value of ‘a’
(ii) The line y = mx + 8 contains the point (- 4, 4), calculate the value of ‘m’
Solution:
(i) Point (-3, 2) lies on the line ax + 3y + 6 = 0,
Then x = – 3, y = 2 satisfies it
a (-3) + 3(2) + 6 = 0
⇒ -3a + 6 + 6 = 0
⇒ -3a + 12 = 0
⇒ -3a = – 12
⇒ a = 4
(ii) line y = mx + 8 contains the point (-4, 4)
x = – 4, y = 4 satisfies it
4 = m (-4) + 8
⇒ 4 = -4m + 8
⇒ 4m = 8 – 4 = 4
⇒ m = 1

Question 9.
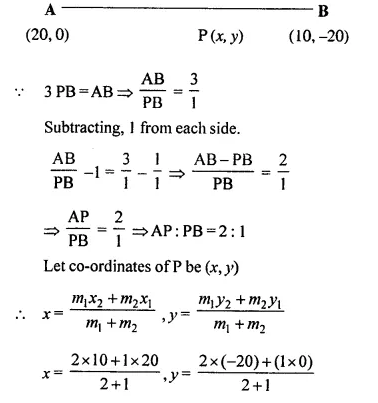
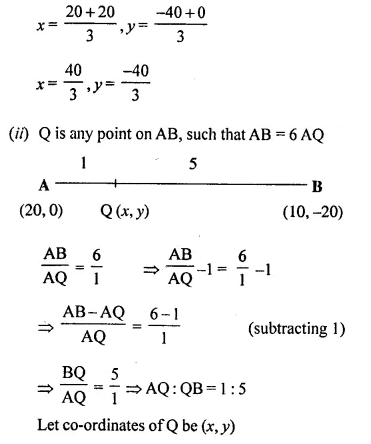
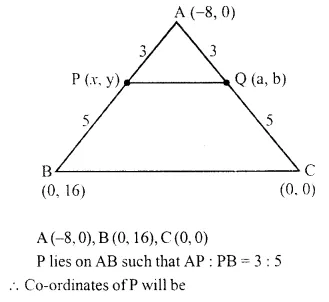
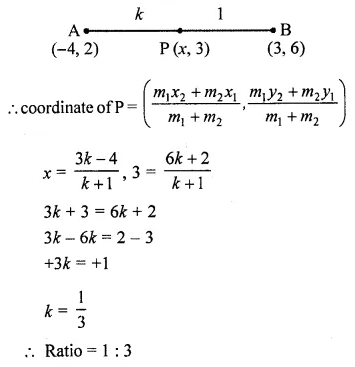
The point P divides the join of (2, 1) and (-3, 6) in the ratio 2 : 3. Does P lie on the line x – 5y + 15 = 0 ?
Solution:
P divides the line joining of the points (2, 1) and (-3, 6) in the ratio of 2 : 3,
co-ordinates of P will be

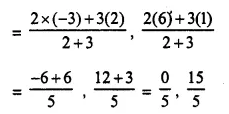
Now, substituting x = 0, y = 3 in the equation
x – 5y + 15 = 0
⇒ 0 – 5 x 3 + 15 = 0
⇒ 0 – 15 + 15 = 0
⇒ 0 = 0 which is true.
Point (0, 3) lies on the line.

Question 10.
The line segment joining the points (5, -4) and (2, 2) is divided by the point Q in the ratio of 1 : 2. Does the line x – 2y = 0 contain Q ?
Solution:
Point Q, divides the line segment joining the points (5, -4) and (2, 2) in the rates of 1 : 2
co-ordinates of Q will be,

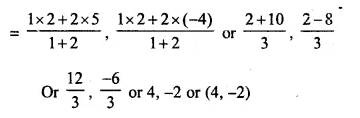
Now, substituting x = 4, y = – 2 in the equation
x – 2y = 0, we get
4 – 2 x (-2) = 0
⇒ 4 + 4 = 0
⇒ 8 = 0 which is not true.
Point Q does not lie on the line x – 2y = 0

Question 11.
Find the point of intersection of the lines : 4x + 3y = 1 and 3x – y + 9 = 0. If this point lies on the line (2k – 1) x – 2y = 4; find the value of k.
Solution:
4x + 3y = 1 …..(i)
3x – y = -9 …..(ii).
Multiplying (i) by 1 and (ii) 3
4x + 3y = 1
9x – 3y = -27
Adding, we get-:
1 3x = – 26 ⇒ x = -2
from (ii),
3x – y = – 9
3(-2) – y = -9
⇒ – 6 – y = -9
⇒ -y = -9 + 6 = -3
⇒ y = 3
The point of intersection is (-2, 3)
The line (2k – 1) x – 2y = 4 passes through that point also
It is satisfy it.
(2k – 1) (-2) – 2(3) = 4
⇒ -4k + 2 – 6 = 4
⇒ -4k – 4 = 4
⇒ -4k = 4 + 4 = 8
⇒ k = -2
Hence point of intersection is (-2, 3) and value of k = -2

Question 12.
Show that the lines 2x + 5y = 1, x – 3y = 6 and x + 5y + 2 = 0 are concurrent.
Solution:
2x + 5y = 1, x – 3y = 6 and x + 5y + 2 = 0 are concurrent
They will pass through the same point
Now 2x + 5y = 1 …..(i)
x – 3y = 6 ……(ii)
Multiply (i) by 3 and (ii) by 5, we get :
-6x + 15y = 3
5x – 15y = 30
Adding we get :
11x = 33 ⇒ x = 3
from (ii),
x – 3y = 6
⇒ 3 – 3y = 6
⇒ -3y = 6 – 3 = 3
⇒ y = -1
Point of intersection of first two lines is (3, -1)
Substituting the values in third line x + 5y + 2 = 0
L.H.S. = x + 5y + 2 = 3 + 5(-1) + 2 = 3 – 5 + 2 = 5 – 5 = 0 = R.H.S.
Hence the given three lines are concurrent.

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 14 Equation of a Line Ex 14A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity (With Applications to Maps and Models) Ex 15A

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15A.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15E

Question 1.
In the figure, given below, straight lines AB and CD intersect at P; and AC || BD. Prove that:
(i) ΔAPC and ΔBPD are similar.
(ii) If BD = 2.4 cm AC = 3.6 cm, PD = 4.0 cm and PB = 3.2 cm; find the lengths of PA and PC.

Solution:
Two line segments AB and CD intersect each other at P.
AC || BD To prove:
(i) ΔAPC ~ ΔBPD
(ii) If BD = 2.4cm, AC = 3.6cm, PD = 4.0 cm and PB = 3.2, find length of PA and PC
Proof:
(i) In ΔAPC and ΔAPD
∠APC = ∠BPD (Vertically opp. angles)
∠PAC = ∠PBD (Alternate angles)
ΔAPC ~ ΔBPD (AA axiom)

Question 2.
In a trapezium ABCD, side AB is parallel to side DC; and the diagonals AC and BD intersect each other at point P. Prove that:
(i) ΔAPB is similar to ΔCPD.
(ii) PA x PD = PB x PC.
Solution:
In trapezium ABCD AB || DC
Diagonals AC and BD intersect each other at P.

To prove:
(i) ΔAPB ~ ΔCPD.
(ii) PA x PD= PB x PC.
Proof: In ΔAPB and ΔCPD
∠APB = ∠CPD (Vertically opposite angles)
∠PAB = ∠PCD (Alternate angles)
ΔAPB ~ ΔCPD (AA axiom)
\(\frac { PA }{ PC }\) = \(\frac { PB }{ PD }\)
=> PA x PD = PB x PC
Hence proved.

Question 3.
P is a point on side BC of a parallelogram ABCD. If DP produced meets AB produced at point L, prove that:
(i) DP : PL = DC : BL.
(ii) DL : DP = AL : DC.
Solution:
P is a point on side BC of a parallelogram ABCD.
DP is produced to meet AB produced at L.

To prove:
(i) DP : PL = DC : BL
(ii) DL : DP = AL : DC.
Proof:
(i) In ΔBPL and ΔCPD
∠BPL = ∠CPD (Vertically opposite angles)
∠PBL = ∠PCD (Alternate angles)
ΔBPL ~ ΔCPD (AA axiom)

Question 4.
In quadrilateral ABCD, the diagonals AC and BD intersect each other at point O. If AO = 2CO and BO = 2DO; show that:
(i) ΔAOB is similar to ΔCOD.
(ii) OA x OD = OB x OC.
Solution:
Given : In quadrilateral ABCD, diagonals AC and BD intersect each other at O.
AO = 2CO, BO = 2DO.
To prove:
(i) ΔAOB is similar to ΔCOD.
(ii) OA x OD = OB x OC.

Question 5.
In ΔABC, angle ABC is equal to twice the angle ACB, and bisector of angle ABC meets the opposite side at point P. Show that:
(i) CB : BA = CP : PA
(ii) AB x BC = BP x CA
Solution:
In ΔABC,
∠ABC = 2∠ACB
Bisector of ∠ABC meets AC in P.

To prove:
(i) CB : BA = CP : PA
(ii) AB x BC = BP x CA
Proof:
(i) In ΔABC,
BP is the bisector of ∠ABC

Question 6.
In ΔABC; BM ⊥ AC and CN ⊥ AB; show that:

Solution:
In ΔABC,
BM ⊥ AC and CN ⊥ AB
To prove:

Question 7.
In the given figure, DE // BC, AE = 15 cm, EC = 9 cm, NC = 6 cm and BN = 24 cm.
(i) Write all possible pairs of similar triangles.
(ii) Find lengths of ME and DM.

Solution:
In the given figure,
DE || BC
AE = 15 cm, EC = 9 cm NC = 6 cm and BN = 24 cm
(i) Write all the possible pairs of similar triangles.
(ii) Find lengths of ME and DM
Proof:
(i) In ΔABC
DE || BC
Pairs of similar triangles are
(a) ΔADE ~ ΔABC
(b) ΔADM ~ ΔABN
(c) ΔAME ~ ΔANC
(ii) ΔAME ~ ΔANC
and ΔADM ~ ΔABN

Question 8.
In the given figure, AD = AE and AD² = BD x EC
Prove that: triangles ABD and CAE are similar.

Solution:
In the given figure,
AD = AE
AD² = BD x EC
To prove: ΔABD ~ ΔCAE
Proof: In ΔADC, AD = AE
∠ADE = ∠AED (Angles opposite to equal sides)
But ∠ADE + ∠ADB = ∠AED + ∠AEC = 180°
∠ADB = ∠AEC
AD² = BD x EC

Question 9.
In the given figure, AB // DC, BO = 6 cm and DQ = 8 cm; find: BP x DO.

Solution:
In the given figure, AB || DC,
BO = 6 cm, DQ = 8 cm
Find BP x DO
In ΔODQ and ΔOPB
∠DOQ = ∠POB (Vertically opposite angles)
∠DQO = ∠OPB (Alternate angles)

Question 10.
Angle BAC of triangle ABC is obtuse and AB = AC. P is a point in BC such that PC = 12 cm. PQ and PR are perpendiculars to sides AB and AC respectively. If PQ = 15 cm and PR = 9 cm; find the length of PB.
Solution:
In ΔABC, ∠ABC is an obtused angle,
AB =AC
P is a point on BC such that PC = 12 cm
PQ and PR are perpendiculars to the sides AB and AC respectively.
PQ = 15 cm and PR = 9 cm

Question 11.
State, true or false :
(i) Two similar polygons are necessarily congruent
(ii) Two congruent polygons are necessarily similar.
(iii) All equiangular triangles are similar.
(iv) All isosceles triangles are similar.
(v) Two isosceles-right triangles are similar.
(vi) Two isosceles triangles are similar, if an angle of one is congruent to the corresponding angle of the other.
(vii) The diagonals of a trapezium, divide each other into proportional segments.
Solution:
(i) False,
(ii) True,
(iii) True,
(iv) False,
(v) True,
(vi) True,
(vii) True.

Question 12.

Solution:

Question 13.
D is a point on the side BC of triangle ABC such that angle ADC is equal to angle BAC. Prove that CA² = CB x CD.
Solution:

Question 14.
In the given figure, ΔABC and ΔAMP are right angled at B and M respectively.
Given AC = 10 cm, AP = 15 cm and PM = 12 cm.
(i) Prove ΔABC ~ ΔAMP
(ii) Find AB and BC.
Solution:
(i) In ΔABC and ΔAMP,
∠A = ∠A (Common)
∠ABC = ∠AMP (Each = 90°)

From right triangle ABC, we have
AC² = AB² + BC² (Pythagoras Theorem)
⇒ 10² = AB² + 8²
⇒ 100 = AB² + 64
⇒ AB² = 100 – 64 = 36
⇒ AB = 6 cm
Hence, AB = 6 cm, BC = 8 cm

Question 15.
Given : RS and PT are altitudes of ΔPQR prove that:
(i) ΔPQT ~ ΔQRS,
(ii) PQ x QS = RQ x QT.
Solution:
Proof: In ΔPQT and ΔQRS,
∠PTQ = ∠RSQ (Each = 90°)
∠Q = ∠Q (Common)
ΔPQT ~ ΔQRS (AA postulate)

Question 16.
Given : ABCD is a rhombus, DPR and CBR are straight lines.

Prove that: DP x CR = DC x PR.
Solution:
Proof: In ΔAPD and ΔPRC
∠DPA = ∠CPR (Vertically opposite angles)
∠PAD = ∠PCR (Alternate angles)
ΔAPD ~ ΔPRC (AA Postulate)

Question 17.
Given: FB = FD, AE ⊥ FD and FC ⊥ AD.
Prove : \(\frac { FB }{ AD }\) = \(\frac { BC }{ ED }\)
Solution:

Question 18.
In ΔPQR, ∠Q = 90° and QM is perpendicular to PR, Prove that:
(i) PQ² = PM x PR
(ii) QR² = PR x MR
(iii) PQ² + QR² = PR²
Solution:
Given: In ΔPQR, ∠Q =90°
QM ⊥ PR.
To Prove:
(i) PQ2 = PM x PR
(ii) QR2 = PR x MR
(iii) PQ2 + QR2 = PR2
Proof: In ΔPQM and ΔPQR,
∠QMP = ∠PQR (each = 90°)
∠P = ∠P (Common)
ΔPQM ~ ΔPQR (AA postulate)

Question 19.
In ΔABC, ∠B = 90° and BD x AC.
(i) If CD = 10 cm and BD = 8 cm; find AD.
(ii) If AC = 18 cm and AD = 6 cm; find BD.
(iii) If AC = 9 cm, AB = 7 cm; find AD.
Solution:
In ΔABC, ∠B = 90°
∠A + ∠C = 90° …….(i)
and in ΔBDC, ∠D = 90°
∠CBD + ∠C = 90° ….(ii)
From (i) and (ii)
∠A + ∠C = ∠CBD + ∠C
∠A = ∠CBD
Similarly ∠C = ∠ABD
Now in ΔABD and ΔCBD,
∠A = ∠CBD and ∠ABD = ∠C
ΔABD ~ ΔCBD (AA Postulate)

Question 20.
In the figure, PQRS is a parallelogram with PQ = 16 cm and QR = 10 cm. L is a point on PR such that RL : LP = 2 : 3. QL produced meets RS at M and PS produced at N.

Find the lengths of PN and RM. [1997]
Solution:
In ΔLNP and ΔRLQ
∠LNP = ∠LQR (Alternate angles)
∠NLP = ∠QLR (Vertically opposite angles)
ΔLNP ~ ΔRLQ (AA Postulate)

Question 21.
In quadrilateral ABCD, diagonals AC and BD intersect at point E. Such that AE : EC = BE : ED. Show that ABCD is a parallelogram.
Solution:
Given : In quadrilateral ABCD, diagonals AC and BD intersect each other at E and

∠AEB = ∠CED (Vertically opposite angles)
ΔAEB ~ ΔCED (SAS axiom)
∠EAB = ∠ECB
∠EBA = ∠CDE
But, these are pairs of alternate angles
AB || CD …..(i)
Similarly we can prove that
AD || BC …..(ii)
from (i) and (ii)
ABCD is a parallelogram.

Question 22.
In ΔABC, AD is perpendicular to side BC and AD² = BD x DC. Show that angle BAC = 90°

Solution:
Given: In ΔABC, AD x BC and AD² = BD x DC
To Prove: ∠BAC = 90°
Proof:

Question 23.
In the given figure AB || EF || DC; AB = 67.5 cm. DC = 40.5 cm and AE = 52.5 cm.

(i) Name the three pairs of similar triangles.
(ii) Find the lengths of EC and EF.
Solution:
(i) In the figure AB || EF || DC
There are three pairs of similar triangles.
(i) ΔAEB ~ ΔDEC
(ii) ΔABC ~ ΔEEC
(iii) ΔBCD ~ ΔEBF
(ii) ΔAEB ~ ΔDEC

Question 24.
In the given figure, QR is parallel to AB and DR is parallel to QB.

Prove that PQ² = PD x PA.
Solution:
Given: In the figure QR || AB mid DR || QB.
To Prove: PQ² = PD x PA
Proof— In ΔPQB,
DR || QB (given)

Question 25.
Through the mid-point M of the side CD of a parallelogram ABCD, the line BM is drawn intersecting diagonal AC in L and AD produced in E.
Prove that : EL = 2 BL.
Solution:

Given: In ||gm. ABCD, M is the mid-point A of CD.
AC is the diagonal.
BM is joined and produced meeting AD produced in E and, intersecting AC in L.
To Prove: EL = 2 BL.
Proof: In ΔEDM, and ΔMBC,
DM = MC (M is mid-point of DC)
∠EMD = ∠CMD (vertically opposite angles)
∠EDM = ∠MCB (Alternate angles)
ΔEDM = ΔMBC (ASA postulate of congruency)
ED = CB = AD (c. p. c. t.)
EA = 2 AD = 2 BC
AB = BC (opposite sides of II gm)
∠DEM = ∠MBC (c. p. c. t.)
Now in ΔELA and ΔBLC,
∠ELA = ∠BLC (vertically opposite angles)
∠DEM or ∠AEL = ∠LBC (proved)
ΔELA ~ ΔBLC (AA postulate)

Question 26.
In the figure given below P is a point on AB such that AP : PB = 4 : 3. PQ is parallel to AC.

(i) Calculate the ratio PQ : AC, giving reason for your answer.
(ii) In triangle ARC, ∠ARC = 90° and in triangle PQS, ∠PSQ = 90°.
Given QS = 6 cm, calculate the length of AR. [1999]
Solution:
Given: In ΔABC, P is a point on AB such that AP : PB = 4 : 3
and PQ || AC is drawn meeting BC in Q.
CP is joined and QS ⊥ CP and AR ⊥ CP
To Find:
(i) Calculate the ratio between PQ : AC giving reason.
(ii) In ΔARC ∠ARC= 90°
and In ΔPQS, ∠PSQ = 90°, if QS = 6 cm, calculate AR.
proof:
(i) In ΔABC, PQ || AC.

Question 27.
In the right angled triangle QPR, PM is an altitude.

Given that QR = 8 cm and MQ = 3.5 cm. Calculate, the value of PR.
Given: In right angled ΔQPR, ∠P = 90° PM ⊥ QR, QR = 8 cm, MQ = 3.5 cm. Calculate PR [2000]
Solution:
In ΔPQM and ΔQPR,
∠PMQ = ∠QPR (each = 90°)
∠Q = ∠Q (common)
ΔPQM ~ ΔQPR (AA postulate)

Question 28.
In the figure given below, the medians BD and CE of a triangle ABC meet at G.
Prove that
(i) ΔEGD ~ ΔCGB
(ii) BG = 2 GD from (i) above.

Solution:
Given: In ΔABC, BD and CE are the medians of sides AC and AB respectively which intersect each at G.
To Prove:
(i) ΔEGD ~ ΔCGB
(ii) BG = 2 GD.
Proof: D and E are the mid points of AC and AB respectively.

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities (Including Trigonometrical Ratios of Complementary Angles and Use of Four Figure Trigonometrical Tables) Ex 21A

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21A.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21E

Prove the following Identities :
Question 1.

Solution:

Question 2.

Solution:

Question 3.

Solution:

Question 4.

Solution:

Question 5.
sin⁴A – cos⁴ A = 2 sin²A-1 
Solution:
L.H.S. = sin⁴ A – cos⁴A = (sin²A)²-(cos²A)²
= (sin²A + cos²A) (sin²A – cos²A)     [(a² – b² = (a + b) (a – b)]
= 1 (sin² A – cos²A) [∵ sin²A + cos²A = 1]
= sin² A – (1- sin²A) (∵ cos²A = 1 – sin²A)
= sin² A – 1 + sin² A
= 2 sin²A-1 = R.H.S.

Question 6.
(1 – tan A)² + (1 + tanA)² = 2sec²A
Solution:
LHS = (1 -tanA)² + (1 +tanA)²
= 1 + tan² A- 2 tan A + 1 + tan² A + 2 tanA
= 2 + 2 tan² A = 2 (1+tan²A)
= 2 sec²A (∵ l+tan²A=sec²A)
= R.H.S.

Question 7.
Cosec⁴ A – cosec² A = cot⁴ A + cot² A
Solution:
L.H.S. = cosec⁴ A -cosec² A
= (cosec²A)² – cosec²A
= (1 + cot²A)² – (1 + cot²A)
= 1 + cot⁴ A + 2 cot²A – 1- cot²A
= cot⁴ A + cot² A = R.H.S.

Question 8.
sec A (1-sin A) (sec A + tan A) = 1
Solution:

Question 9.
cosec A (1 + cos A) (cosec A – cot A) = 1
Solution:

Question 10.
sec² A + cosec²A = sec² A cosec² A
Solution:

Question 11.

Solution:

Question 12.
tan²A – sin²A = tan² A. sin² A
Solution:

Question 13.
cot² A – cos² A = cos² A. cot² A
Solution:

Question 14.
(cosecA + sinA) (cosec A – sinA) = cot² A + cos²A
Solution:
L.H.S. = (cosec A + sin A) (cosec A – sin A)
= (cosec²A – sin² A) [∵ (a + b) (a – b) = a²– b²]
= 1 + cot² A – sin² A = cot² A + 1 – sin²A
= cot² A + cos² A (∵ 1-sin²A = cos² A)
= R.H.S.

Question 15.
(sec A – cosA) (sec A + cosA) = sin² A + tan²A
Solution:
L.H.S. = (sec A-cos A) (sec A + cos A)
= sec² A – cos² A
= 1 + tan²A-cos² A
= 1-cos² A + tan² A
= sin² A + tan² A  (∵ 1- cos²A=sin²A)
= R.H.S.

Question 16.
(cos A + sin A)² + (cos A – sin A)² = 2
Solution:
LHS = (cos A + sin A)² + (cos A – sin A)²
= cos² A + sin² A + 2 cos A sin A + cos² A + sin² A – 2 cos A sin A
= 2 sin² A + 2 cos² A
= 2 (sin²A+cos²A)
= 2 x 1=2 = R.H.S. (∵ sin²A + cos² A = 1)

Question 17.
(cosec A – sin A) (sec A – cos A) (tan A + cot A) = 1
Solution:

Question 18.

Solution:

Question 19.

Solution:

Question 20.

Solution:

Question 21.
(sin A + cosec A)² + (cos A + sec A)² = 7 + tan² A + cot² A
Solution:
L.H.S. = (sin A + cosecA)² + (cosA+ secA)²
= sin² A + cosec² A + 2 sin A cosec A + cos² A + sec² A + 2 cos A sec A
= sin² A+cosec² A+2 sin A x \(\frac { 1 }{ sinA }\) + cos² A+sec²A + 2cosA x \(\frac { 1 }{ cosA }\)
= sin²A + cos² A + cosec² A + sec²A+ 2 + 2   (∵ sin² A + cos²A= 1)
= 1 +cosec²A + sec²A + 4
= (1 + cot² A) + (1 + tan² A) + 5 [∵ cosec²A = 1 + cot² A and sec² A = 1 + tan²A]
= 1 + cot² A + 1 + tan² A + 5
= 7 + tan²A + cot²A = R.H.S.

Question 22.
sec²A. cosec²A = tan²A + cot²A + 2
Solution:

Question 23.

Solution:

Question 24.

Solution:

Question 25.

Solution:

Question 26.

Solution:

Question 27.

Solution:

Question 28.

Solution:

Question 29.

Solution:

Question 30.

Solution:

Question 31.

Solution:

Question 32.

Solution:

Question 33.

Solution:

Question 34.

Solution:

Question 35.

Solution:

Question 36.

Solution:

Question 37.

Solution:

Question 38.
(1 +cot A-cosec A) (1 + tan A + sec A) = 2
Solution:

Question 39.

Solution:

Question 40.

Solution:

Question 41.

Solution:

Question 42.

Solution:

Question 43.

Solution:

Question 44.

Solution:

Question 45.

Solution:

Question 46.

Solution:

Question 47.

Solution:

Question 48.

Solution:

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9D

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9D.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9D

Question 1.
Find x and y, if:

Solution:

Question 2.
Find x and y, if :

Solution:

Question 3.

Solution:

Question 4.

(i) the order of the matrix X
(ii) the matrix X.
Solution:

Question 5.
Evaluate

Solution:

Question 6.

Solution:

Question 7.

Solution:

Question 8.

(i) A (BA)
(ii) (AB) A
Solution:

Question 9.
Find x and y, if

Solution:

Question 10.

Solution:

Question 11.

Solution:

Question 12.

Solution:

Question 13.

Solution:

Question 14.

Solution:

Question 15.

Solution:

Question 16.

Solution:

Question 17.

Solution:

Question 18.

Solution:

Question 19.

Solution:

Question 20.

Solution:

Question 21.

Solution:

Question 22.

Solution:

Question 23.

Solution:
(i) Order of matrix A is 2 x 2
Order of matrix B is 2 x 1
Order of matrix X is 2 x 1

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 13 Section and Mid-Point Formula Ex 13C

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 13 Section and Mid-Point Formula Ex 13C.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 13 Section and Mid-Point Formula Ex 13A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 13 Section and Mid-Point Formula Ex 13B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 13 Section and Mid-Point Formula Ex 13C

Question 1.
Given a triangle ABC in which A = (4, -4), B (0, 5) and C = (5, 10). A point P lies on BC such that BP : PC = 3 : 2. Find the length of line segment AP.
Solution:
B (0, 5), C (5, 10) and BP : PC = 3 : 2 Co-ordinates of P will be

Question 2.
A (20, 0) and B (10, – 20) are two fixed points, find the co-ordinates of the point P in AB such that 3PB = AB. Also, find the co-ordinates of some other point Q in AB such that AB = 6AQ.
Solution:
(i) A (20, 0), B (10, – 20)

Question 3.
A (-8, 0), B (0, 16) and C (0, 0) are the vertices of a triangle ABC. Point P lies on AB and Q lies on AC such that AP : PB = 3 : 5 and AQ : QC = 3 : 5. Show that: PQ = \(\frac { 3 }{ 8 }\) BC.
Solution:

Question 4.
Find the co-ordinates of points of trisection of the line segment joining the points (6, -9) and the origin.
Solution:
Points are A (6, -9) and O (0,0) let P and Q are points, which trisect AO

Question 5.
A line segment joining A (-1, \(\frac { 5 }{ 3 }\)) and B (a, 5) is divided in the ratio 1 : 3 at P, the point where the line segment AB intersects the y-axis.
(i) Calculate the value of ‘a’.
(ii) Calculate the co-ordinates of ‘P’. (1994)
Solution:

Question 6.
In what ratio is the line joining A (0, 3) and B (4, -1), divided by the x-axis ? Write the co-ordinates of the point where AB intersects the x-axis. [1993]
Solution:
Let the ratio be m₁ : m₂ when the x-axis intersects the line AB at P.

Question 7.
The mid point of the segment AB, as shown in diagram, is C (4, -3). Write down the co-ordinates of A and B. (1996)

Solution:
Let co-ordinates of A (x, 0) and B (0, y) and C (4, -3) the mid point of AB.

Question 8.
AB is a diameter of a circle with centre C = (-2, 5). If A = (3, -7). Find
(i) the length of radius AC
(ii) the coordinates of B.
Solution:

Question 9.
Find the co-ordinates of the centroid of a triangle ABC whose vertices are A (- 1, 3), B (1, – 1) and C (5, 1)
Solution:

Question 10.
The mid-point of the line segment joining (4a, 2b – 3) and (-4, 3b) is (2, -2a). Find the values of a and b.
Solution:
Let A and B are two points and P is its mid point then A is (4a, 2b -3), B(-4, 2b) and P is (2, -2a)

Question 11.
The mid point of the line segment joining (2a, 4) and (-2, 2b) is (1, 2a + 1). Find the value of a and b.
Solution:
The midpoint of the line segment joining (2a, 4) and (-2, 2b) is (1, 2a + 1)

Question 12.
(i) Write down the co-ordinates of the point P that divides the line joining A (-4, 1) and B (17, 10) in the ratio 1 : 2.
(ii) Calculate the distance OP, where O is the origin.
(iii) In what ratio does the y-axis divide the line AB ? [ICSE 1995]
Solution:
Point P, divides a line segment giving the points A (-4, 1) and B (17, 10) is the ratio 1 : 2.

Question 13.
Prove that the points A(-5, 4); B (-1, -2) and C (5, 2) are the vertices of an isosceles right-angled triangle. Find the co-ordinates of D. So that ABCD is a square. [1992]
Solution:
In ABC, the co-ordinates of A, B and C are (-5, 4), B(-1, -2) and C (5, 2) respectively.

ABC is also a right-angled triangle.
Hence ABC is an isosceles right angled triangle,
Let D be the fourth vertex of square ABCD and co-ordinates of D be (x,y)
Since the diagonals of a square bisect each other and let O be the point of intersection of AC and BD.
O is mid-point of AC as well as BD.

Question 14.
M is the mid-point of the line segment joining the points A (-3, 7) and B (9, -1). Find the co-ordinates of point M. Further, if R (2, 2) divides the line segment joining M and the origin in the ratio p : q, find the ratio p : q.
Solution:
Two points are given A (-3, 7) and B (9, -1)
M is the mid-point of line joining AB.
Co-ordinates of M wll be

Question 15.
Calculate the ratio in which the line joining A (-4, 2) and B (3, 6) is divided by point P (x, 3). Also find
(i) x
(ii) Length of AP. (2014)
Solution:
Let ratio = k : 1

Question 16.
Find the ratio in which the line 2x + y = 4 divides the line segment joining the points P (2, -2) and Q (3, 7).
Solution:
Let the given line 2x + y = 4 divides the line segment joining the points P (2, -2) and Q (3,7) in the ratio k : 1 at a point (x, y) on it.

Question 17.
If the abscissa of a point P is 2. Find the ratio in which this point divides the line segment joining the points (-4, 3) and (6, 3). Also, find the co-ordinate of point P.
Solution:
Abscissa of a point P is 2
Let co-ordinates of point P be (2, y)
Let point P (2, y) divides the line segment joining the points (-4, 3) and (6, 3) in the ratio k : 1

Question 18.
The line joining the points (2, 1) and (5, -8) is trisected at the points P and Q. If point P lies on the line 2x – y + k = 0, find the value of k, Also, find the co-ordinates of point Q.
Solution:
A line joining the points (2, 1) and (5, -8) is trisector at P and Q.

Question 19.
M is the mid-point of the line segment joining the points A (0, 4) and B (6, 0). M also divides the line segment OP in the ratio 1 : 3. Find:
(i) co-ordinates of M
(ii) co-ordinates of P
(iii) length of BP

Solution:
M is mid point of the line segment joining the points A (0, 4) and B (6, 0)
M divides the line segment OP in the ratio 1 : 3

Question 20.
Find the image of the point A (5, -3) under reflection in the point P (-1, 3).
Solution:
Image of the point A (5, -3) under reflection in the point P (-1, 3)
Let B (x, y) be the point of reflection of A (5, -3) under P(-1, 3)

Question 21.
A (-4, 2), B (0, 2) and C (-2, -4) are vertices of a triangle ABC. P, Q and R are mid-points of sides BC, CA and AB respectively. Show that the centroid of PQR is the same as the centroid of ABC.
Solution:
A (-4, 2), B (0, 2) and C (-2, -4) are the vertices of ABC.
P, Q and R are the mid-points of the sides BC, CA and AB respectively.
G is the centroid of medians AP, BQ and CR.
Co-ordinates of G are

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 13 Section and Mid-Point Formula Ex 13C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9C

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9C.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9D

Question 1.
Evaluate if possible :

If not possible, give a reason.
Solution:

(iv) It is not possible, because number of columns of the first matrix is not equal to number of rows of the second matrix.

Question 2.

Solution:

Question 3.

Solution:

Question 4.
Find x and y, if:

Solution:

Question 5.

Solution:

Question 6.

(i) AB
(ii) BA
(iii) A²
Solution:

Question 7.

Solution:

Question 8.

Solution:

Question 9.

Solution:


Comparing the elements, we get:
-2b =-2
b = 1
a = 2

Question 10.

Solution:

Question 11.

Solution:

Question 12.

Solution:

Question 13.

Solution:

Question 14.

Solution:

Question 15.

Simplify : A² + BC.
Solution:

Question 16.
Solve for x and y :

Solution:

Question 17.
In each case given below, find :
(a) the order of matrix M.
(b) the matrix M.

Solution:

Question 18.

Solution:

Question 19.

Solution:

Question 20.
If A and B are any two 2 x 2 matrices such that AB = BA = B and B is not a zero matrix, What can you say about the matrix A?
Solution:
AB = BA = B
But it is possible, when A = 0 or B = 0
But B is not a zero matrix (given)
A is a zero matrix or A is an identity matrix

Question 21.

Solution:

Question 22.

Solution:

Question 23.

Solution:

Question 24.

Solution:

Question 25.

Solution:

Question 26.

Solution:

Question 27.

Solution:

Question 28.

Solution:

Question 29.

Solution:

Question 30.

Solution:

Question 31.
State, with reason, whether the following are true or false. A, B and C are matrices of order 2 x 2.
(i) A + B = B + A
(ii) A – B = B – A
(iii) (B . C). A = B . (C . A)
(iv) (A + B) . C = A . C + B . C
(v) A . (B – C) = A . B – A . C
(vi) (A – B) . C = A . C – B . C
(vii) A² – B² = (A + B) (A – B)
(viii) (A – B)² = A² – 2 A . B + B²
Solution:
(i) True : Because addition of matrices is commutative.
(ii) False : Subtraction of matrices is not commutative.
(iii) True : Multiplication of matrices is associative.
(iv) True: Multiplication of matrices is distributive over addition.
(v) True : As given above in (iv)
(vi) True : As given above in (iv)
(vii) False : Laws of algebra for factorization and expansion are not applicable to matrices.
(viii) False, As given above in (vii)

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 13 Section and Mid-Point Formula Ex 13B

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 13 Section and Mid-Point Formula Ex 13B.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 13 Section and Mid-Point Formula Ex 13A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 13 Section and Mid-Point Formula Ex 13B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 13 Section and Mid-Point Formula Ex 13C

Question 1.
Find the mid-point of the line segment joining the points:
(i) (-6, 7) and (3, 5)
(ii) (5, -3), (-1, 7)
Solution:
Let P (x, y) be the mid-point in each case

Question 2.
Points A and B have co-ordinates (3, 5) and (x, y) respectively. The mid-point of AB is (2, 3). Find the values of x and y.
Solution:
Co-ordinates of A (3, 5), B (x, y) and mid-point M (2, 3)

Question 3.
A (5, 3), B (-1, 1) and C (7, -3) are the vertices of ABC. If L is the mid-point of AB and M is the mid-point of AC, show that LM = \(\frac { 1 }{ 2 }\) BC.
Solution:

Question 4.
Given M is the mid-point of AB, find the co-ordinates of:
(i) A; if M = (1, 7) and B = (-5, 10),
(ii) B; if A = (3, -1) and M (-1, 3).
Solution:
M is the mid-point of AB.
(i) Let A = (x, y), M = (1, 7) and B = (-5, 10)

Question 5.
P (-3, 2) is the mid-point of line segment AB as shown in the figure. Find the co-ordinates of points A and B.

Solution:

Point A is on y-axis
its abscissa is zero and point B is on x-axis
its ordinate is zero.
Now, let co-ordinates of A are (0, y) and ofB are (x, 0) and P (-3, 2) is the mid-point

Question 6.
In the given figure, P (4, 2) is the mid point of line segment AB. Find the co-ordinates of A and B.

Solution:

Points A and B are on x-axis and y-axis respectively
Ordinate of A is zero and abscissa of B is zero.
Let co-ordinates of A be (x, 0) and B (0, y)
and P (4, 2) is the mid-point

Question 7.
(-5, 2), (3, -6) and (7, 4) arc the vertices of a triangle. Find the length of its median through the vertex (3, -6) and (7, 4).
Solution:

Let A (-5, 2), B (3, -6) and C (7, 4) are the vertices of a ABC
Let L,M and N are the mid-points of sides BC, CA and AB respectively of ABC.
L is the mid-point of BC.
Co-ordinates of L will be

Question 8.
Given a line ABCD in which AB = BC = CD, B = (0, 3) and C = (1, 8). Find the co-ordinates of A and D.

Solution:

Question 9.
One end of the diameter of a circle is (-2, 5). Find the co-ordinates of the other end of it, if the centre of the circle is (2, -1).
Solution:

Question 10.
A (2, 5), B (1, 0), C (-4, 3) and D (-3, 8) are the vertices of quadrilateral ABCD. Find the co-ordinates of the mid-points of AC and BD. Give a special name to the quadrilateral.
Solution:
Co-ordinates of A = (2, 5), B = (1, 0), C = (-4, 3) and D = ( 3, 8)

Let the mid-point of AC is P (x1, y1) Co-ordinates of mid-point of AC will be

Co-ordinates of mid-points AC and BD are the same..
The quadrilateral is a parallelogram.

Question 11.
P (4, 2) and Q (-1, 5) are the vertices of parallelogram PQRS and (-3, 2) are the co-ordinates of the point of intersection of its diagonals. Find the co-ordinates of R and S.
Solution:
In the parallelogram PQRS and qo-ordinates of P are (4, 2) and of Q are (-1, 5).
The diagonals of || gm AC and BD intersect each other at O (-3, 2)

Question 12.
A (-1, 0), B (1, 3) and D (3, 5) are the vertices of a parallelogram ABCD. Find the co-ordinates of vertex C.
Solution:

Vertices of a parallelogram ABCD are A (-1, 0), B (1, 3) and D(3, 5)
Let co-ordinates of C be (x, y)
Let the diagonals AC and BD bisect each other at O. Then O is the mid-point of AC as well as of BD.
Co-ordinates of O, the mid-point of BD will be

Question 13.
The points (2, -1), (-1, 4) and (-2, 2) are the mid-points of the sides of a triangle. Find its vertices.
Solution:
Let D, E and F are the mid-points of sides BC, CA and AB of a ABC respectively.


Co-ordinates of A are (-5, 7), of B are (1, -3) and of C are (3, 1)

Question 14.
Points A (-5, x), B (y, 7) and C (1, -3) are collinear (i.e. lie on the same straight line) such that AB = BC. Calculate the values of x and y.
Solution:

Question 15.
Points P (a, -4), Q (-2, b) and R (0, 2) are collinear. If Q lies between P and R, such that PR = 2QR, calculate the values of ‘a’ and ‘b’:
Solution:

Question 16.
Calculate the co-ordinates of the centroid of the triangle ABC, if A = (7, -2), B = (0, 1) and C = (-1, 4).
Solution:

Question 17.
The co-ordinates of the centroid of a triangle PQR are (2, -5). If Q = (-6, 5) and R = (11, 8); calculate the co-ordinates of vertex P.
Solution:

Question 18.
A (5, x), B (-4, 3) and C (y, -2) are the vertices of the triangle ABC whose centroid is the origin. Calculate the values of x and y.
Solution:

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 13 Section and Mid-Point Formula Ex 13B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9B

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9B.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9D

Question 1.
Evaluate

Solution:

Question 2.
Find x and y if :

Solution:

Question 3.

Solution:

Question 4.

Solution:

Question 5.

Solution:

Question 6.

Solution:

Question 7.

Solution:

Question 8.

Solution:

Question 9.

Solution:

Question 10.
If I is the unit matrix of order 2 x 2; find the matrix M, such that:

Solution:

Question 11.

Solution:

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 9 Matrices Ex 9B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 22 Heights and Distances Ex 22C

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 22 Heights and Distances Ex 22C

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 22 Heights and Distances Ex 22A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 22 Heights and Distances Ex 22B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 22 Heights and Distances Ex 22C

Question 1.
Find AD.

Solution:

Question 2.
In the following diagram.
AB is a floor-board. PQRS is a cubical box with each edge = 1 m and ∠B = 60°. Calculate the length of the board AB.

Solution:

Question 3.
Calculate BC.

Solution:

Question 4.
Calculate AB .

Solution:

Question 5.
The radius of a circle is given as 15cm and chord AB subtends an angle of 131° at the centre C of the circle. Using trigonometry, Calculate :
(i) the length of AB;
(ii) the distance of AB from the centre C.
Solution:
Chord AD substends an angle of 131° at the centre. Join CA, CB and draw CD ⊥ AB which bisects AB at D.
(In ∆CAB)
∵ CA = CB (radii of the same circle)
∴ ∠CAB = ∠CBA
But ∠CAB + ∠CBA = 180°- 131° = 49°

Question 6.
At a point on level ground, the angle of elevation of a vertical tower is found to be such that its tangent is \(\frac { 5 }{ 12 }\). On walking 192 metres towards the tower; the tangent of the angle is found to be \(\frac { 3 }{ 4 }\). Find the height of the tower.
Solution:

Question 7.
A vertical tower stands on horizontal plane and is surmounted by a vertical flagstaff of height h metre. At a point on the plane, the angle of elevation of the bottom of the flagstaff is a and that of the top of flagstaff is β. Prove that the height of the tower is :

Solution:

Question 8.
With reference to the given figure, a man stands on the ground at point A, which is on the same horizontal plane as B, the foot of the vertical pole BC. The height of the pole is 10 m.
The man’s eye is 2 m above the ground. He observes the angle of elevation of C. The top of the pole, as x°, where tan x° = \(\frac { 2 }{ 5 }\) . calculate:

(i) the distance AB in m;
(ii) the angle of elevation of the top of the pole
when he is standing 15 m from the pole. Give your answer to the nearest degree. [1999]
Solution:

Question 9.
The angles of elevation of the top of a tower from two points on the ground at distances a and b metres from the base of the tower and in the same striaght line with it are complementary.Prove that height of the tower is \( \sqrt{ab} \) metre.
Solution:

Question 10.
From a window A. 10 m above the ground the angle of elevation of the top C of a tower is x°, where tan x = \(\frac { 5 }{ 2 }\) and the angle of depression of the foot D of the tower is y°, where tany° = \(\frac { 1 }{ 4 }\).(See the figure given below). Calculate the height CD of the tower in metres. [2000]

Solution:
Let CD be the height of the tower and height of window A from the ground = 10m
In right ∆AEC,

Question 11.
A vertical tower is 20 m high, A man standing at some distance from the tower knows that the cosine of the angle of elevation of the top of the tower is 0.53. How far is he standing from the foot of the tower ? [2001]
Solution:

Question 12.
A man standing on the bank of a river observes that the angle of elevation of a tree on the opposite bank is 60°. When he moves 50 m away from the bank, he finds the angle of elevation to be 30°. Calculate :
(i) the width of the river and
(ii) the height of the tree.
Solution:

Let TR be the tree of height x m and y be the width AR of the river,
then ∠B = 30° and A = ∠60° , AB = 50 m.
Now in right ∆ATR,

Question 13.
A 20 m high vertical pole and a vertical tower are on the same level ground in such a way that the angle of elevation of the top of the tower, as seen from the foot of the pole, is 60° and the angle of elevation of the top of the pole as seen from the foot of the tower is 30°. Find
(i) the height of the tower.
(ii) the horizontal distance between the pole and the tower.
Solution:
Let PQ is the pole and TS is the tower. PQ = 20 m.
Let TS = h and QS = x Angles of elevation from Q to T A T is 60° and from S to P is 30°.
In the ∆PQS

Question 14.
A vertical pole and a vertical tower are on the same level ground in such a way that from the top of the pole the angle of elevation of the top of the tower is 60° and the angle of depression of the bottom of the tower is 30°.
Find : (i) the height of the tower, if the height of the pole is 20m;
(ii) the height of the pole, if the height of the tower is 75 m.
Solution:
Let PQ is the pole and TW is the tower
Angle of elevation from T to P is 60° and angle of depression from P to W is 30°
∴ ∠PWQ = 30° = ∠RPW ( ∠ Altanate angles)
(i) In first case when height of pole OQ = 20m, Then in right ∆ PQW

Question 15.
From a point, 36 m above the surface of a lake, the angle of elevation of a bird is observed to be 30° and angle of depression of its image in the water of the lake is observed to be 60°. Find the actual height of the bird above the surface of the lake.
Solution:
Let AQ is the sea-level
P is a point 36 m above sea-level
∴ PQ = 36
Let B be the bird and R is its reflection in the water and angle of elevation of the bird B at P is 30° and angle of depression of the reflection of the bird at R is 60°

Question 16.

Solution:

Question 17.
As observed from the top of a 80 m tall lighthouse, the angles of depression of two ships, on the same side of the light house in horizontal line with its base , are 30° and 40° respectively . Find the distance between the two ships.Give your answer correct to the nearest meter. [2012]
Solution:

Question 18.
In the figure given, from the top of a building AB = 60 m high, the angles of depression of the top and bottom of a vertical lamp post CD are observed to be 30° and 60° respectively. Find :

(i) the horizontal distance between AB and CD.
(it) the height of the lamp post.
Solution:

Question 19.
An aeroplane at an altitude of 250 m observes the angle of depression of two boats on the opposite banks of a river to be 45° and 60° respectively. Find the width of the river. Write the answer correct to the nearest whole number. (2014)
Solution:

Question 20.
The horizontal distance between two towers is 120 m. The angle of elevation of the top and angle of depression of the bottom of the first tower as observed from the top of the second tower is 30° and 24° respectively. Find the height of the two towers. Give your answer correct to 3 significant figures. (2015)

Solution:
AB and CD are two towers which are 120 m apart
i.e. BD= 120m
Angles of elevation of the top and angle of depression of bottom of the first tower observed from the top of second tower is 30° and 24°

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 22 Heights and Distances Ex 22C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

 

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity (With Applications to Maps and Models) Ex 15D

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15D.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15E

Question 1.
A triangle ABC has been enlarged by scale factor m = 2.5 to the triangle A’ B’ C’. Calculate:
(i) the length of AB, if A’ B’ = 6 cm.
(ii) the length of C’ A’ if CA = 4 cm.
Solution:
Scale factor (k) = 2.5
∆ABC is enlarged to ∆A’B’C’
(i) A’B’ = 6 cm

Question 2.
A triangle LMN has been reduced by scale factor 0.8 to the triangle L’ M’ N’. Calculate:
(i) the length of M’ N’, if MN = 8 cm.
(ii) the length of LM, if L’ M’ = 5.4 cm.
Solution:
∆LMN has been reduced by the scale factor

Question 3.
A triangle ABC is enlarged, about the point O as centre of enlargement, and the scale factor is 3. Find:
(i) A’ B’, if AB = 4 cm.
(ii) BC, if B’ C’ = 15 cm.
(iii) OA, if OA’= 6 cm.
(iv) OC’, if OC = 21 cm.
Also, state the value of:
(a) \(\frac { OB’ }{ OB }\)
(b) \(\frac { C’A’ }{ CA }\)
Solution:
∆ABC is enlarged to ∆A’B’C’ about the point O as its centre of enlargement.
Scale factor = 3 = \(\frac { 3 }{ 1 }\)

Question 4.
A model of an aeroplane is made to a scale of 1 : 400. Calculate:
(i) the length, in cm, of the model; if the length of the aeroplane is 40 m.
(ii) the length, in m, of the aeroplane, if length of its model is 16 cm.
Solution:
Model of an aeroplane to the actual = 1 : 400

Question 5.
The dimensions of the model of a multistorey building are 1.2 m x 75 cm x 2 m. If the scale factor is 1 : 30; find the actual dimensions of the building.
Solution:
Dimensions of a model of multistorey building = 1.2 m x 75 cm x 2 m

Question 6.
On a map drawn to a scale of 1 : 2,50,000; a triangular plot of land has the following measurements : AB = 3 cm, BC = 4 cm and angle ABC = 90°.
Calculate:
(i) the actual lengths of AB and BC in km.
(ii) the area of the plot in sq. km.
Solution:
Scale of map drawn of a triangular plot = 1 : 2,50,000
Measurement of plot AB = 3 cm, BC = 4 cm
and ∠ABC = 90°

Question 7.
A model of a ship of made to a scale 1 : 300
(i) The length of the model of ship is 2 m. Calculate the lengths of the ship.
(ii) The area of the deck ship is 180,000 m². Calculate the area of the deck of the model.
(iii) The volume of the model in 6.5 m3. Calculate the volume of the ship. (2016)
Solution:

Question 8.
An aeroplane is 30 in long and its model is 15 cm long. If the total outer surface area of the model is 150 cm², find the cost of painting the outer surface of the aeroplane at the rate of ₹ 120 per sq.m. Given that 50 sq. m of the surface of the aeroplane is left for windows.
Solution:
Length of aeroplane = 30 m = 3000 cm
and length of its model = 15 cm
Surface area of model = 150 cm²
Scale factor (k) = \(\frac { 3000 }{ 15 }\) = \(\frac { 200 }{ 1}\)
Area of plane = k² x area of model = (200)² x 150 cm² = 40000 x 150 cm²
\(\frac { 40000 x 150 }{ 10000 }\) = 600 m² (1 m² = 10000 cm²)
Shape left for windows = 50 sq. m
Balance area = 600 – 50 = 550 sq. m
Race of painting the outer surface = ₹ 120 per sq.m
Total cost = ₹ 550 x 120 = ₹ 66000

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 15 Similarity Ex 15D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Chapter 21 Trigonometrical Identities (Including Trigonometrical Ratios of Complementary Angles and Use of Four Figure Trigonometrical Tables) Ex 21E

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21E.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21E

Question 1.
Prove the following identities :

Solution:

Solution:

Solution:

Solution:

Solution:


Solution:

Solution:

Solution:


Solution:

Solution:

Solution:

Solution:


Solution:

Solution:

Solution:


Solution:

Solution:

Question 2.
If sin A + cos A = p and sec A + cosec A = q then prove that: q(p² – 1) 2p
Solution:

Question 3.

Solution:

Question 4.

Solution:

Question 5.
If tan A=n tan B and sin A=m sin B, prove that:

Solution:

Question 6.
(i) If 2 sin A-1 = 0, show that:
sin 3 A = 3 sin A – 4 sin³ A.             [2001]
(ii) If 4cos² A-3 = 0, show that:
cos 3A = 4 cos³ A – 3 cos A
Solution:

Question 7.
Evaluate:

Solution:

Question 8.
Prove that:

Solution:

Question 9.
If A and B are complementary angles, prove that:
(i) cot B + cos B sec A cos B (1 + sin B)
(ii) cot A cot B – sin A cos B – cos sin B = 0
(iii) cosec² A + cosec² B = cosec² A cosec² B

Solution:

Question 10.
Prove that:


Solution:

Question 11.
If 4 cos² A – 3 = 0 and 0° ≤ A ≤ 90°, then prove that : 
(i) sin 3A = 3 sinA – 4 sin³A
(ii) cos 3A = 4 cos³ A – 3 cos A
Solution:

Question 12.
Find A, if 0° ≤ A ≤ 90° and :
(i) 2 cos² A – 1 = 0
(ii) sin 3A – 1 = 0
(iii) 4 sin² A – 3 = 0
(iv) cos² A – cos A = 0
(v) 2cos² A + cos A – 1 = 0
Solution:

Question 13.
If 0° < A < 90° ; find A, if :

Solution:

Question 14.
Prove that : (cosec A – sin A) (sec A – cos A) sec² A = tan A. (2011)
Solution:

Question 15.
Prove the identity : (sin θ + cos θ) (tan θ + cot θ) = sec θ + cosec θ. (2014)
Solution:

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 21 Trigonometrical Identities Ex 21E are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.