Category: CBSE Class 10

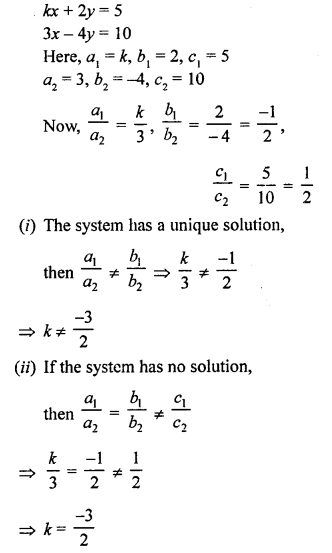
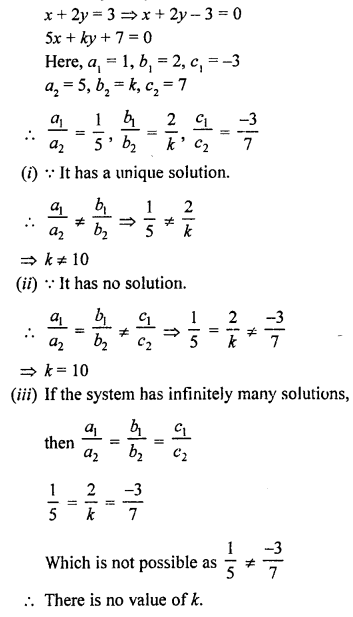
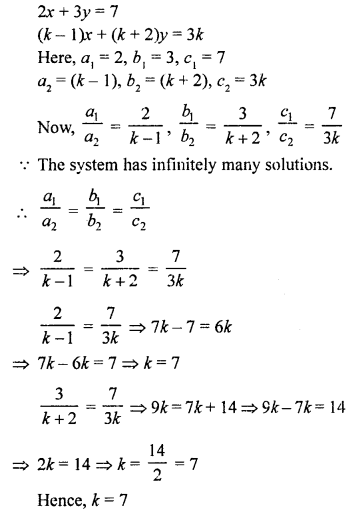
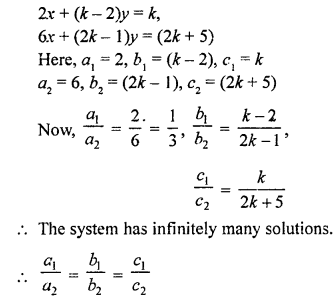
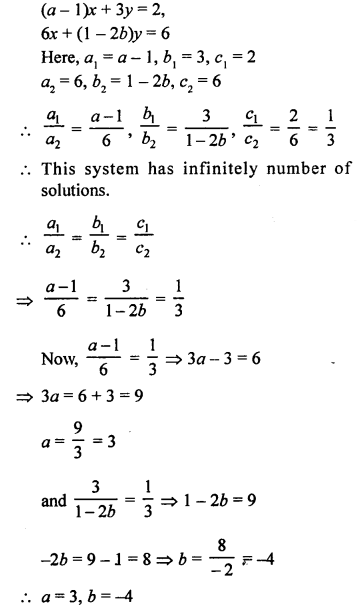
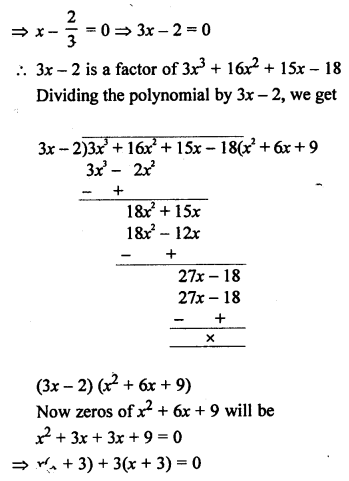
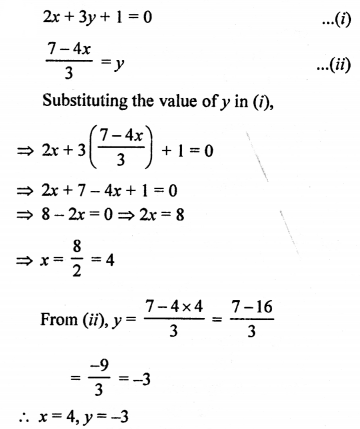
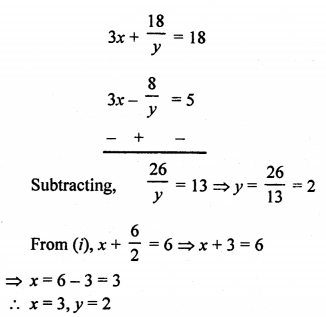
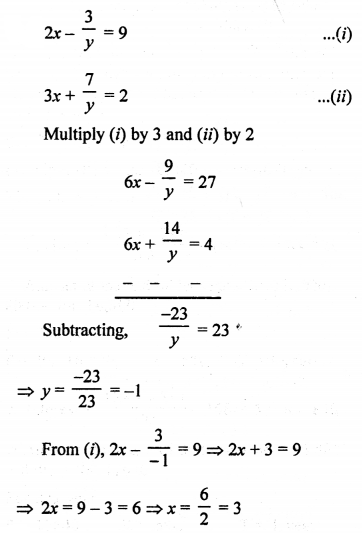
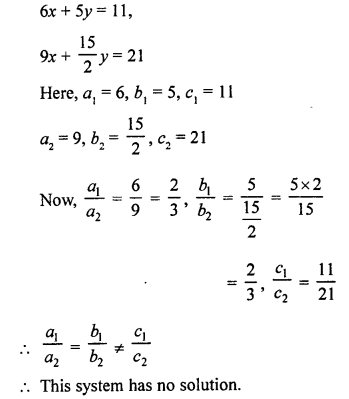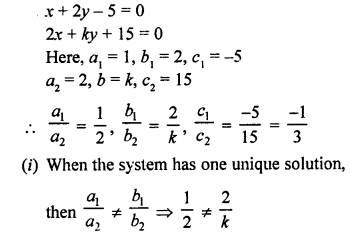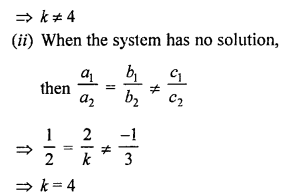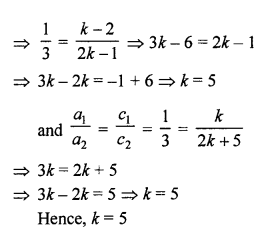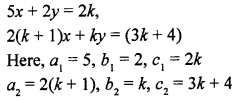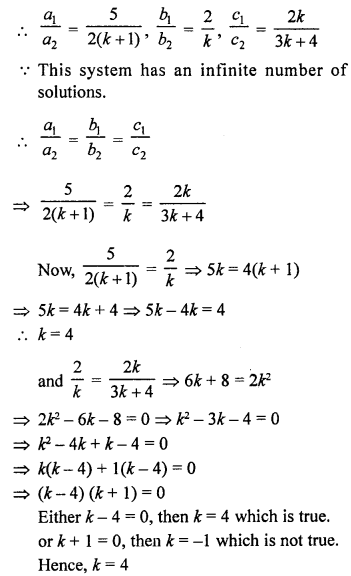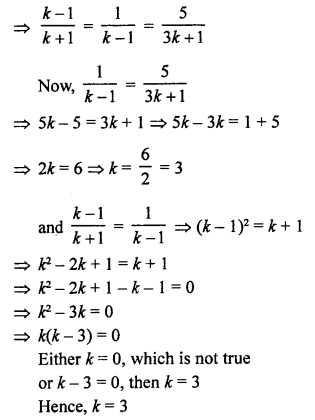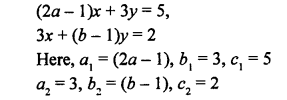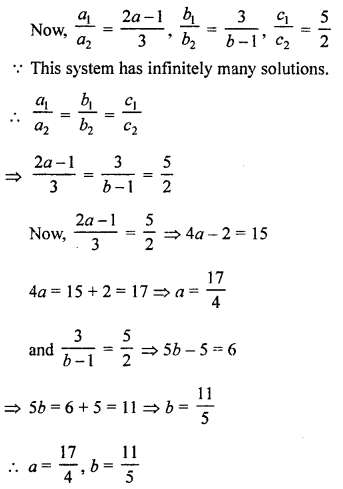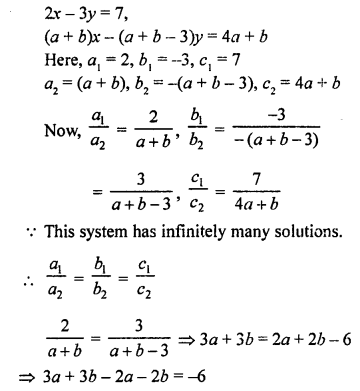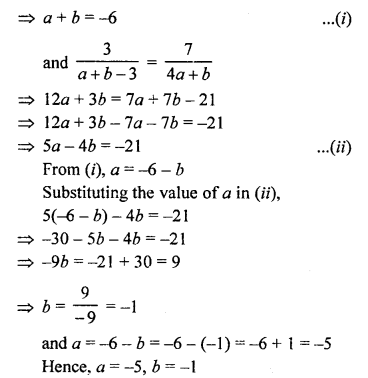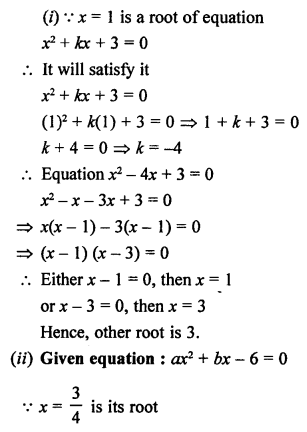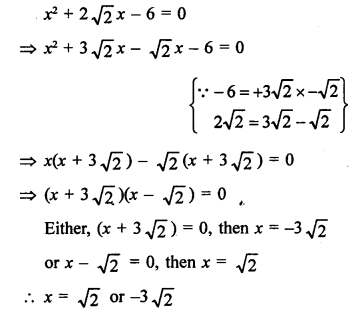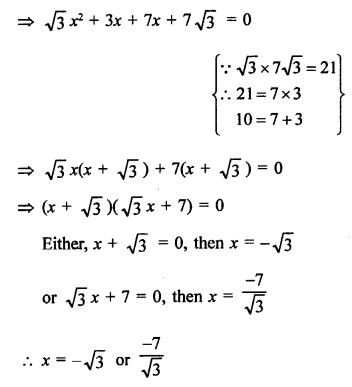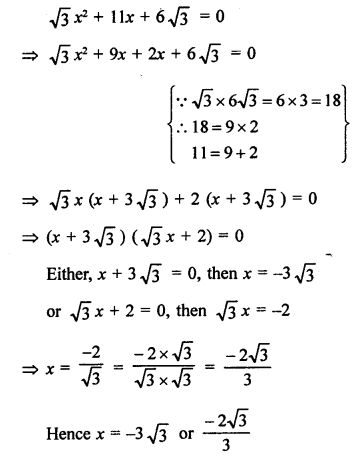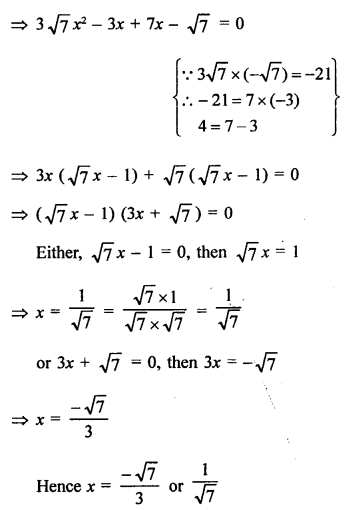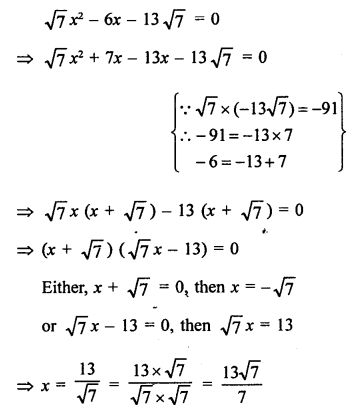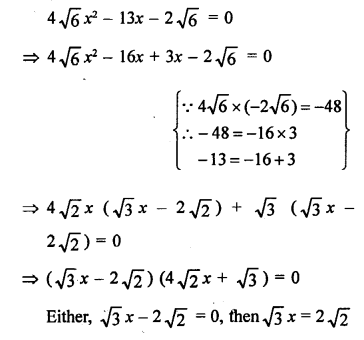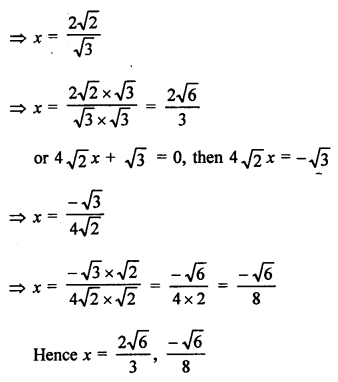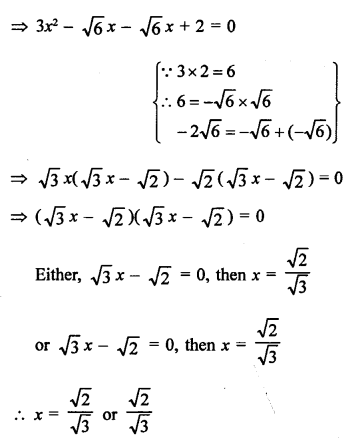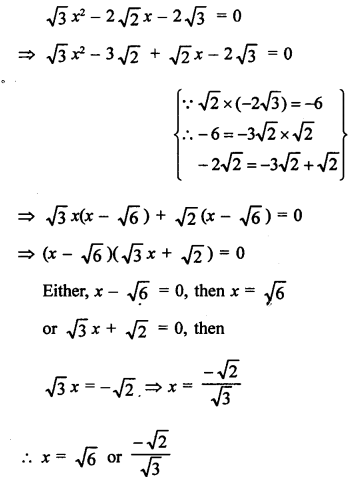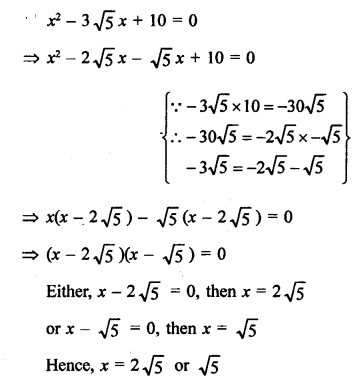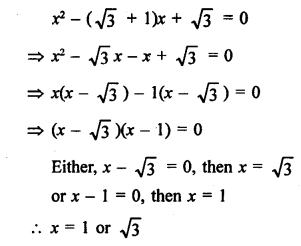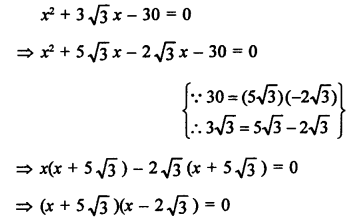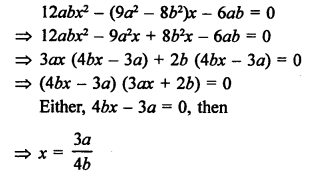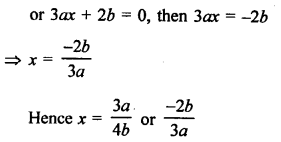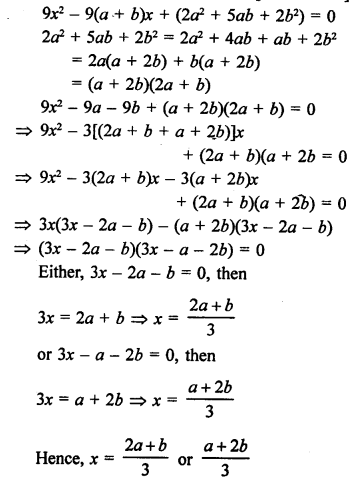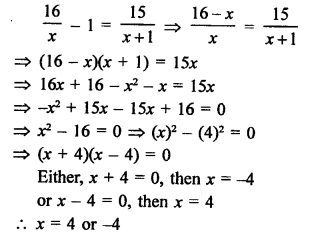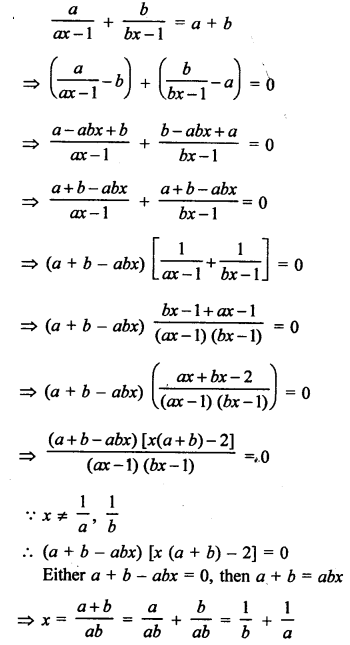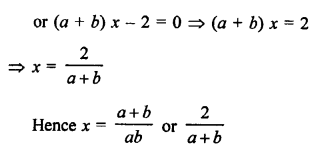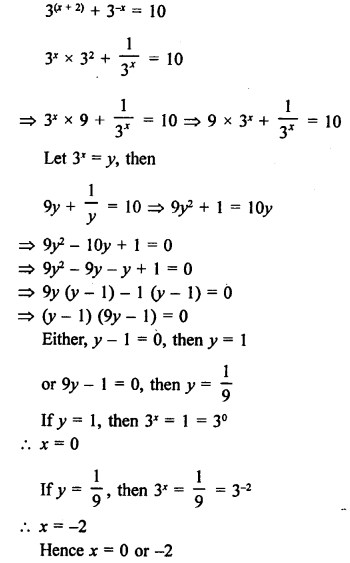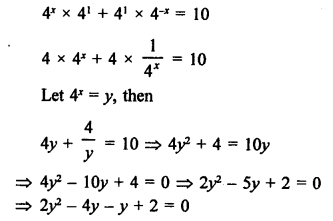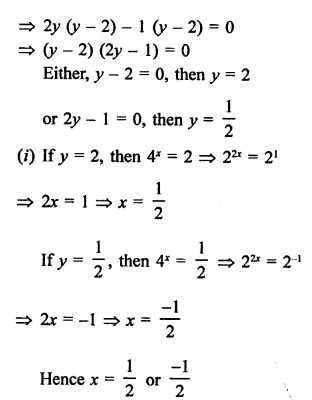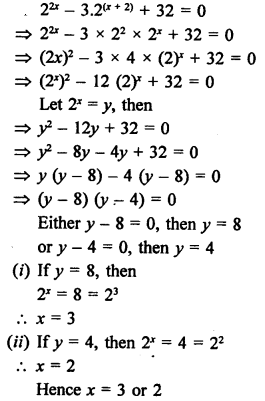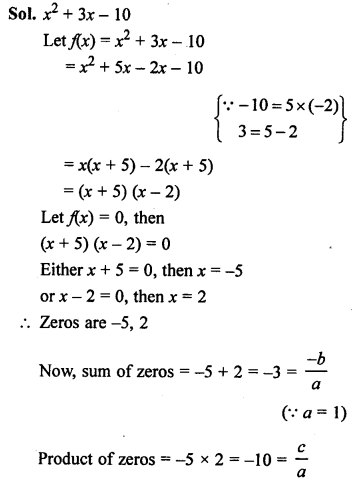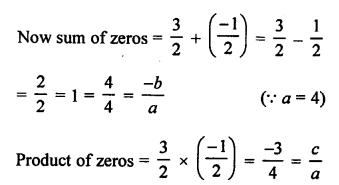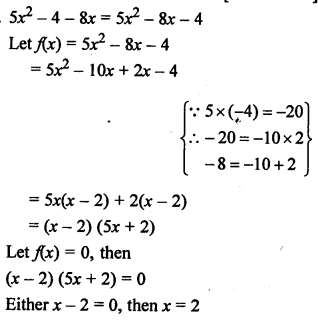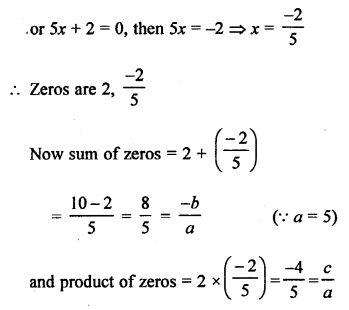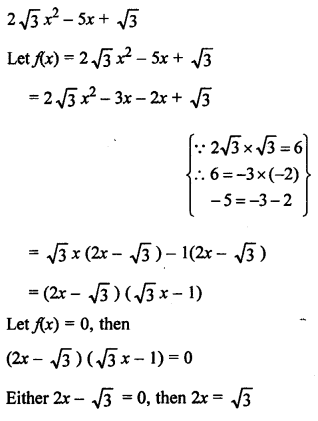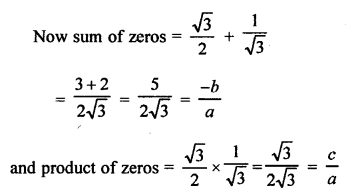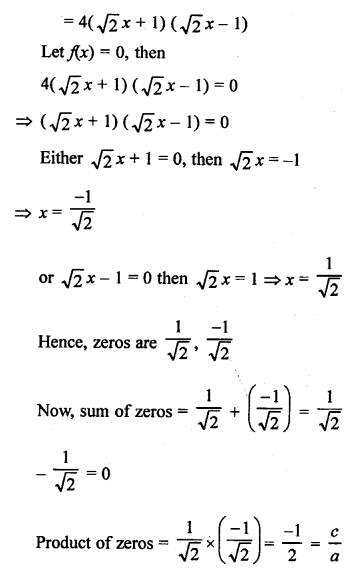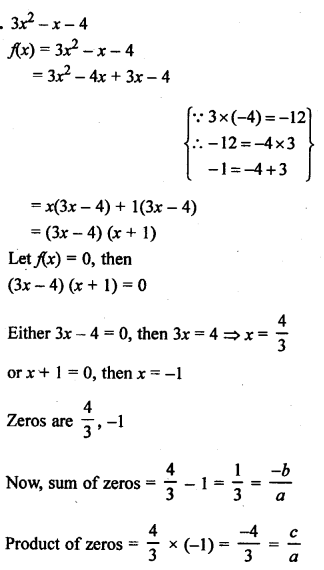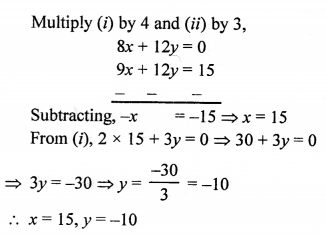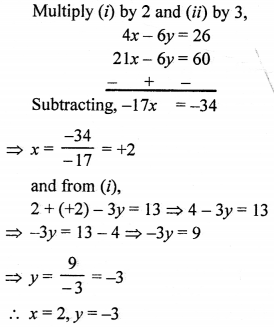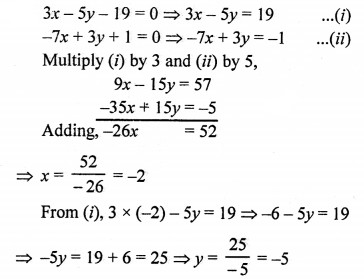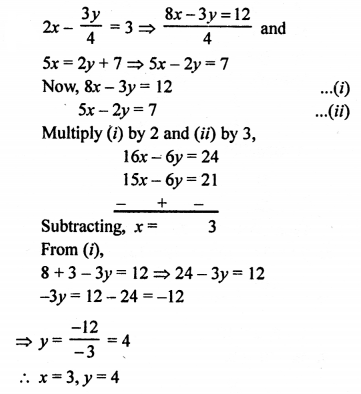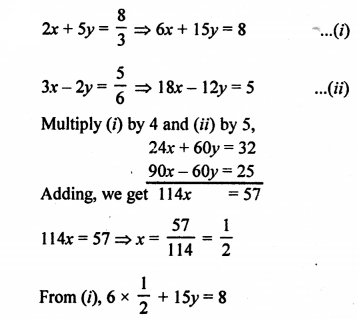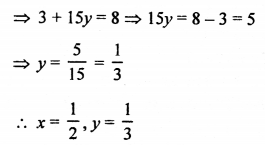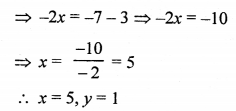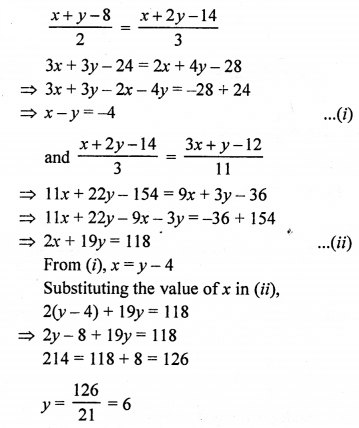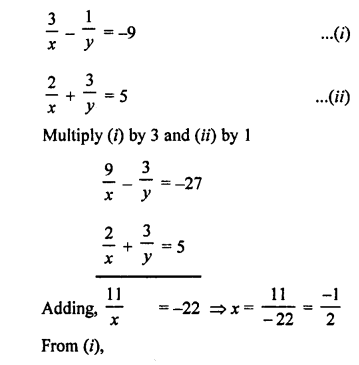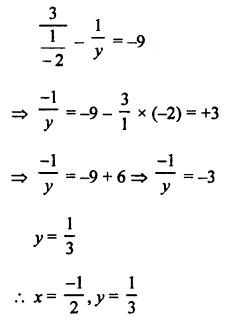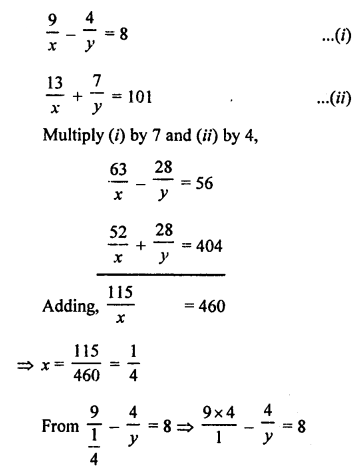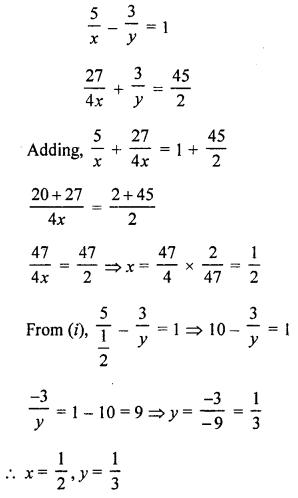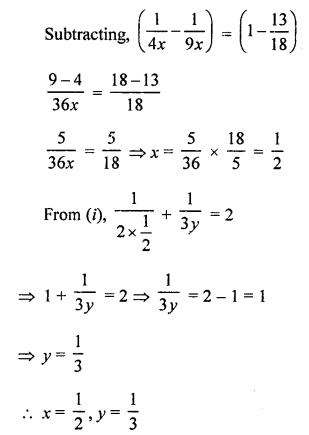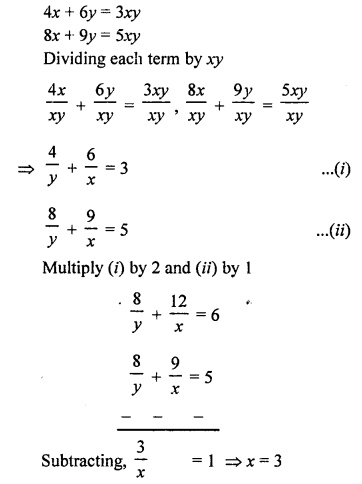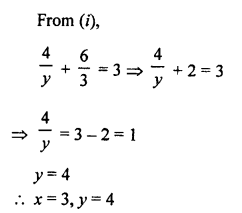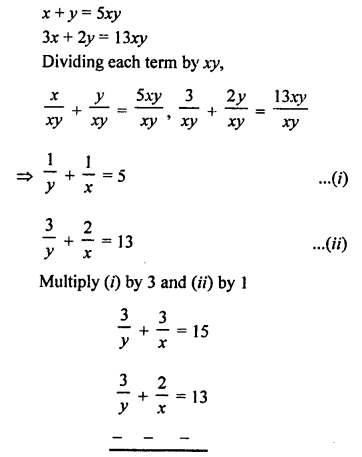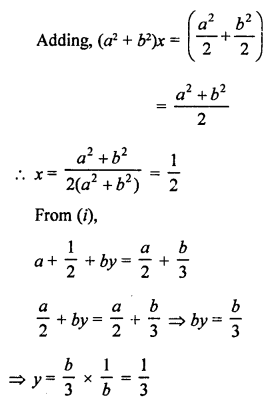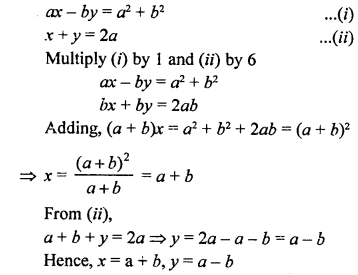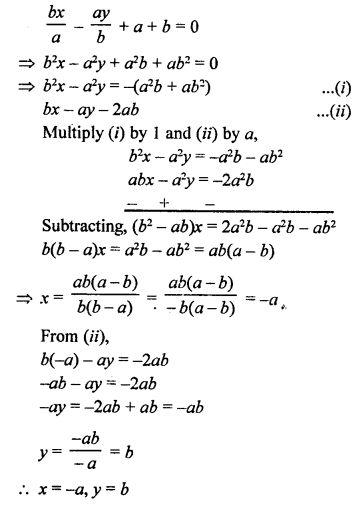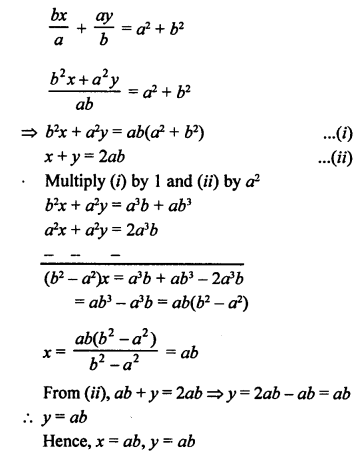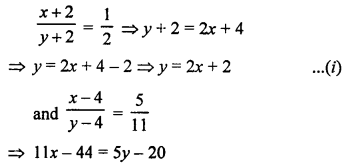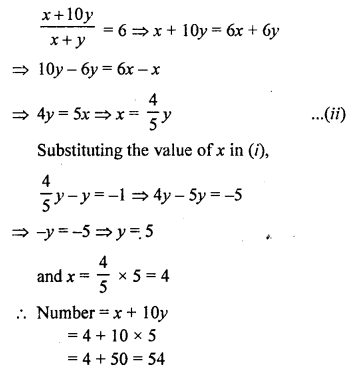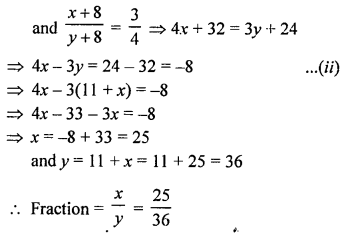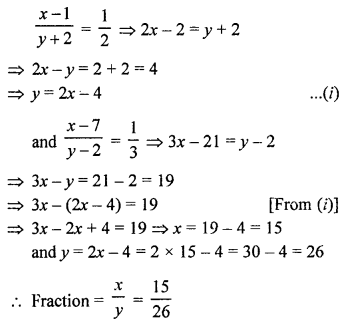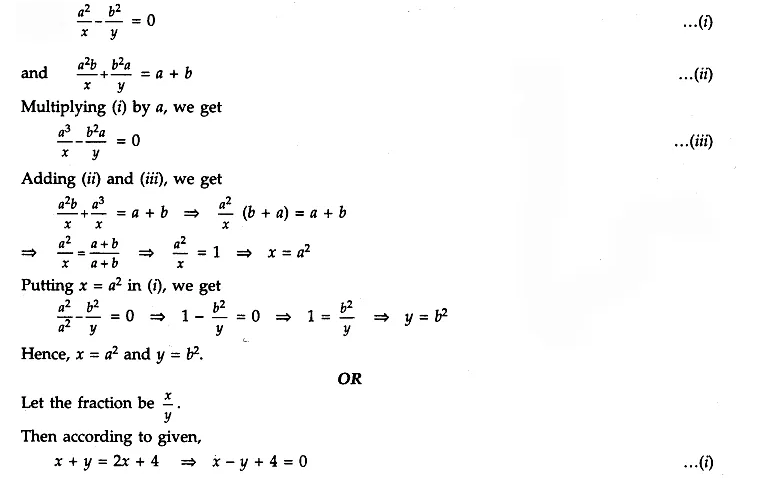NCERT Solutions for Class 10 Science Chapter 3 Metals and Non-metals

In this chapter, students will learn about the physical properties of metals, and non-metals, chemical properties of metals, how metals react with air, water, acid, other solutions, and metal salts, reactivity series.

Further students will come to know how metals and non-metals react, properties of ionic compounds, the occurrence of metals, extraction of metals, refining of metals, corrosion, prevention of corrosion. The chapter contains questions and answers exercise along with multiple choice questions.

These Solutions are part of NCERT Solutions for Class 10 Science. Here we have given NCERT Solutions for Class 10 Science Chapter 3 Metals and Non-metals. LearnInsta.com provides you the Free PDF download of NCERT Solutions for Class 10 Science (Chemistry) Chapter 3 – Metals and Non-metals solved by Expert Teachers as per NCERT (CBSE) Book guidelines. All Chapter 3 – Metals and Non-metals Exercise Questions with Solutions to help you to revise complete Syllabus and Score More marks.

NCERT Solutions for Class 10 Science Chapter 3 NCERT Questions

NCERT Solutions for Class 10 Science Chapter 3 In Text Book Questions

Question 1.
Give example of a metal which
(a) is a liquid at room temperature
(b) can be easily cut with a knife
(c) is the best conductor of heat
(d) is the poorest conductor of heat.
Answer:
(a) Mercury
(b) Sodium
(c) Silver
(d) Lead.

More Resources

• NCERT Solutions for Class 10 Science
• NCERT Exemplar Solutions for Class 10 Science
• HOTS Questions for Class 10 Science
• Value Based Questions in Science for Class 10
• Previous Year Question Papers for CBSE Class 10 Science

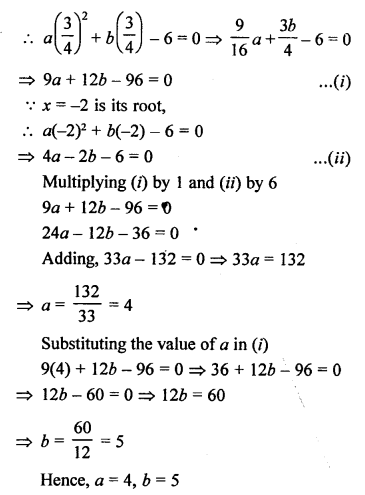
Question 2.
Explain the meaning of malleable and ductile.
Answer:
Malleable: The property due to which a substance can be beaten into sheets is known as malleability. Metals are malleable in nature.
Ductile. The property due to which a substance can be drawn into wires is known as ductility. Metals are ductile in nature.

Question 3.
Why is sodium kept immersed in kerosene oil ? (CBSE 2011)
Answer:
Sodium reacts with air and water both. It is a highly reactive metal. When kept in open, it readily combines with oxygen present in air to form its oxide. Similarly, it reacts with water vapours or moisture to form sodium hydroxide.

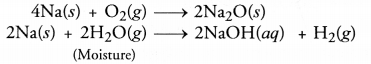
In order to preserve sodium metal, we generally keep it under kerosene so that neither air nor moisture may come in its contact.

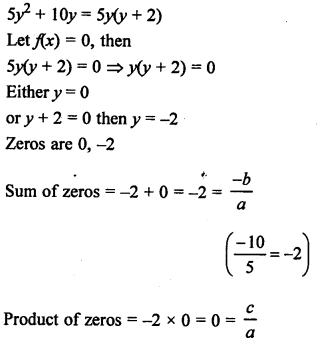
Question 4.
Write the equations for the reactions of
(a) iron with steam
(b) calcium with water
(c) potassium with water.
Answer:
(a) 3Fe(s) + 4H₂O (steam) ———–> Fe₃O₄(s) + 4H₂(g)
(b) Ca(s) + 2H₂O(aq) ———–> Ca(OH)₂(s) +H₂(g)
(c) 2K(s) + 2H₂O(aq) ———–> 2KOH(aq) + H₂(g)

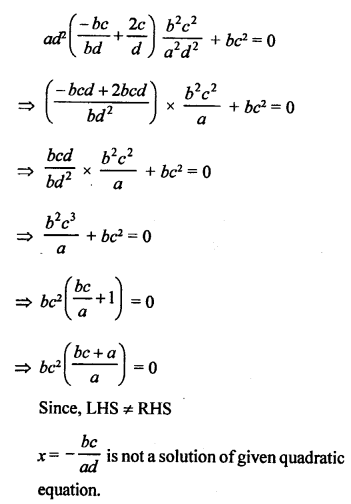
Question 5.
Samples of four metals A, B, C and D were taken and were added to the following solutions one by one. The results obtained have been tabulated as follows :

Metal	Solution to which metal is added
	Iron(II) sulphate	Copper(II) sulphate	Zinc sulphate	Silver nitrate
A	No reaction	Displacement	—	—
B	Displacement	—	No reaction „	—
C	No reaction	No reaction	No reaction	Displacement
D	No reaction	No reaction	No reaction	No reaction

Use the table given above to answer the following questions :
(a) Which is the most reactive metal ?
(b) What would you observe when B is added to solution of copper(II) sulphate ?
(c) Arrange the metals A, B, C and D in order of increasing reactivity. (CBSE 2011)
Answer:
Based on the activity series, the relative position of the metals in which involved in solutions is : Zn > Fe > Cu > Ag. On the basis of the results given in the table .

• Metal A is more reactive than copper and less reactive than iron.
• Metal B is more reactive than iron and less reactive than zinc. –
• Metal C is only more reactive than silver and less reactive than other metals.
• Metal D is the least reactive in nature.

In the light of above information, we can conclude that
(a) Metal B is the most reactive.
(b) Since B is more reactive than iron, it is also more reactive than copper. This means that it would displace copper from copper(II) sulphate solution. The blue colour of solution will slowly fade.
(c) The decreasing order of reactivity of metals is: B>A>C>D.

Question 6.
Which gas is produced when a reactive metal reacts with dilute hydrochloric acid ? Write the chemical reaction when iron reacts with dilute H₂SO₄. (CBSE 2010)
Answer:
Hydrogen gas (H₂) is produced when a reactive metal reacts with dilute hydrochloric acid. Iron and dilute H₂SO₄ react as follows :
Fe(s) + H₂SO₄(aq) ————> FeSO₄(aq) + H₂(g)
Hydrogen gas is evolved in this reaction also.

Question 7.
What would you observe when zinc is added to a solution of iron (II) sulphate ? Write the chemical reaction that takes place. (CBSE 2010)
Answer:
The green colour of the solution would slowly disappear. Zinc would gradually dissolve and iron would get precipitated at the bottom of the beaker.

Question 8.
(i) Write electron-dot structures for sodium, magnesium and oxygen.
(ii) Show the formation of Na₂O and MgO by the transfer of electrons.
(iii) What are the ions present in these compounds ?
Answer:
(i)

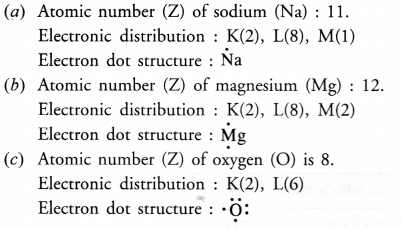
(ii) Formation of sodium oxide (Na₂O)

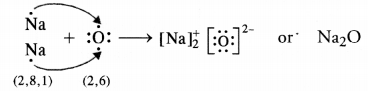
Formation of magnesium oxide (MgO)

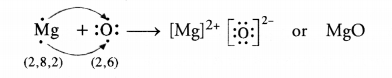
(iii) For answer, consult structures given above.

Question 9.
Why do ionic compounds have high melting points ? (CBSE 2014)
Answer:
In the formation of ionic compounds, positive ions (cations) and negative ions (anions) participate. These are closely packed and the ionic compounds exist as crystalline solids. They have strong inter ionic forces of attraction and have high melting and boiling points.

Question 10.
Define the following terms :

1. Minerals
2. Ores
3. Gangue.

Answer:

1. Minerals : These are the combined states of metals and non-metals present in earth’s curst.
2. Ores : The minerals from which metals can be conveniently and profitably extracted, are called ores.
3. Gangue : It represents the earthy impurities such as mud, sand and clay associated with the ore.

Question 11.
Name two metals which are formed in nature in free state.
Answer:
The metals are gold (Au) and platinum (Pt).

Question 12.
Which chemical process is used for obtaining a metal from its oxide ?
Answer:
The chemical process is known as reduction.

Question 13.
Metallic oxides of zinc, magnesium and copper were heated with the following metals. In which cases, will you find displacement reactions taking place ?

Metal	Zinc	Magnesium	Copper
Zinc oxide Magnesium oxide Copper oxide

Relative positions of these metals in the activity series are : Mg, Zn, Cu : In the light of this :
Answer:
Magnesium (Mg) will displace both zinc (Zn) and copper (Cu) from their oxides
Mg + ZnO ———-> MgO + Zn
Mg + CuO ———-> MgO + Cu
Zinc will displace copper from copper oxide.
Zn + CuO ———–> ZnO + Cu
Copper is least reactive and will not initiate displacement reaction.

Question 14.
Which metals do not corrode easily ?
Answer:
Metals such as gold (Au) and platinum (Pt) present at the bottom of the activity series do not corrode easily.

Question 15.
What are alloys ? (CBSE 2011)
Answer:
Alloys are the homogeneous mixture of two or more metals or even metals and non-metals.

NCERT Solutions for Class 10 Science Chapter 3 NCERT End Exercise

Question 1.
Which of the following will give displacement reactions ?
(a) NaCl solution and copper metal
(b) MgCl₂ solution and aluminium metal
(c) FeSO₄ solution and silver metal
(d) AgNO₃ solution and copper metal.
Answer:
(d). Only AgNO₃ solution will give displacement reaction with copper (Cu) because copper is placed above silver in the activity series.

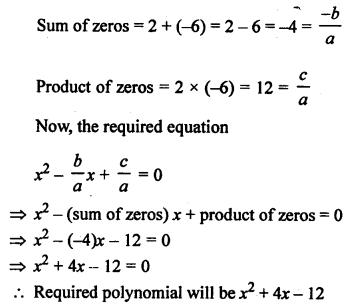
Question 2.
Which of the following methods is suitable for preventing an iron frying pan from rusting ?
(a) applying grease
(b) applying paint
(c) applying a coating of zinc
(d) all the above.
Answer:
Theoretically all the three methods are helpful for preventing an iron frying pan from rusting. However, the constituents of both grease and paint are mostly organic compounds. They cannot withstand the heat and do not last. Therefore, applying a coating of zinc (galvanisation) is the best method. Option (c) is correct.

Question 3.
An element reacts with oxygen to give a compound with high melting point. This compound is also water soluble. The element is likely to be :
(a) Calcium
(b) Carbon
(c) Silicon
(d) Iron
Answer:
(a). Calcium (Ca) combines with oxygen to form calcium oxide (CaO) with very high melting point. CaO dissolves in water to form calcium hydroxide

Question 4.
Food cans are coated with tin and not with zinc because
(a) Zinc is costlier than tin
(b) Zinc has higher melting point than tin
(c) Zinc is more reactive than tin
(d) Zinc is less reactive than tin.
Answer:
(c). Zinc is more reactive than tin and reacts with organic acids present in food to form poisonous compounds. Since tin is placed below zinc in the activity series, it is less reactive and does not react with the organic acids. Therefore, (c) is the correct option.

Question 5.
You are given a hammer, a battery, a bulb, wires and a switch :
(a) Flow could you use them to distinguish between samples of metals and non metals ?
(b) Assess the usefulness of these tests to distinguish between metals and non metals.
Answer:
(a) With the help of hammer, convert both the metal and non-metal (solid) in the form of plates or rods. Metal will readily form these since they are malleable. Non-metals being brittle will break when struck with hammer. They will form plates with difficulty Now construct a cell in both the cases using these plates as electrodes and switch on the current. If the bulb glows, this means that the electrodes are of metals. In case, this does not glow, this means that the electrodes are of non-metals.
(b) From these tests, we may conclude that

1. Metals are malleable while non-metals are not.
2. Metals are good conductors of electricity while non-meals are not (graphite is an exception).

Question 6.
What are amphoteric oxides ? Give examples of two amphoteric oxides.
Answer:
These are the oxides which can act both as acids and bases. For example, aluminium oxide (Al₂O₃) and zinc oxide (ZnO). The amphoteric character of the two oxides are shown by the following reactions.

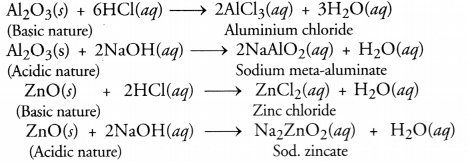
Question 7.
Name two metals which can displace hydrogen from dilute acids and two metals which can not do so.
Answer:
Sodium and calcium can displace hydrogen from dilute acids Copper and silver can not displace hydrogen from dilute acids.

Question 8.
In the electrolytic refining of metal M, name anode, cathode and electrolyte.
Answer:
Anode : Rod of the impure metal
Cathode : Rod of pure metal
Electrolyte : Aqueous solution of soluble salt of metal M.

Question 9.
Pratyush took sulphur powder on a spatula and heated it. He collected the gas evolved by inverting a test tube over it as shown in the figure.

What will be the action of gas on

1. dry litmus paper ?
2. moist litmus paper ?

Write a balanced chemical equation for the reaction taking place.
(CBSE 2011)
Answer:
The gas evolved upon heating sulphur powder on a spatula is sulphur dioxide

1. SO₂(g) has no action of dry litmus paper.
2. SO₂(g) dissolves in moisture (water) present in moist litmus paper to form sulphurous acid. In acidic solution, moist litmus paper will change to red.
   

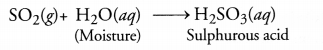
Question 10.
State two ways to prevent the rusting of iron.
Answer:

1. By applying a coating of grease or paint on the surface of iron.
2. By depositing a layer of zinc on the surface of iron. The process is called galvanisation.

Question 11.
What types of oxides are formed when non-metals combine with oxygen ?
Answer:
The oxides are generally acidic in nature which means that when dissolved in water, their solutions change blue litmus to red. For example,

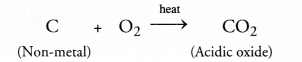
Question 12.
Give reasons for the following :
(a) Platinum, gold and silver are used to make jewellery.
(b) Sodium, potassium and lithium are stored under oil.
(c) Aluminium is a highly reactive metal but still used for making cooking utensils.
(d) Carbonate and sulphide ores are usually converted into oxides during the process of extraction. (CBSE 2013, 2014)
Answer:
(a) These metals placed at the bottom of the activity series are very little reactive in nature. Gold and
platinum are known as noble metals. They are not affected by air, water and by chemicals. Since they have bright lustre, jewellery can be made from these metals.
(b) There are reactive metals placed high in the activity series. In air, their surface gets tarnished due to presence oxygen, water vapours and carbon dioxide in air. With water, these react violently to evolve so much heat that is not possible to handle them. These metals are generally kept under kerosene which does not contain air and water.
(c) When exposed to air, the metal changes its oxide called aluminium oxide (Al₂CO₃). It gets deposited over the surface of the metal and forms a protective coating on the surface. Due to the presence of this layer, the metal becomes unreactive and can be used in making cooking utensils.
(d) Both carbonate and sulphide ores of metals cannot be directly reduced to metallic state. Flowever, metal oxides can be easily reduced with coke or other reducing agents. Both are therefore, converted into their respective oxides by calcination process (for carbonate ores) and by roasting process (for sulphide ores).
Metal oxides can be easily reduced to metallic form with coke (C) or any other suitable reducing agent. Therefore, carbonates and sulphides are converted to the oxide form by processes of calcination and roasting and are not directly reduced.

Question 13.
You must have seen tarnished copper vessels being cleaned with lemon or tamarind juice. Explain why these sour substances are effective in cleaning the vessels. (CBSE 2014)
Answer:
Copper metal slowly reacts with water, carbon dioxide and oxygen present in air to form basic copper carbonate which is green in colour. Its layer slowly gets deposited on the surface of the metal. Now lemon

juice contains citric acid while tartaric acid is present is tamrind. Both these acids react with basic copper carbonate to form soluble salts such as copper acetate (with citric acid) and copper tartarate (with tartaric acid). The equations for the reactions are complicated and are not given. These salts are removed from the surface of the copper metal and the surface of the metal shines.

Question 14.
A man went door to door posing as a goldsmith. He promised to bring back the glitter on dull gold ornaments. An unsuspecting lady gave a set of gold bangles to him which he dipped in a particular solution. The bangles sparked like new but their weight was reduced drastically. The lady got upset and after a futile argument, the man beat a hasty retreat. Can you predict the nature of the solution used by the impositer ?
Answer:
The man had actually used the solution of aqua regia (mixture of cone. HCl and cone. HNO₃ in the ratio of 3 : 1 by volume) which has dissolved gold forming soluble auric chloride (AuCl₃). Since gold actually reacted, there was a loss in weight of the gold bangles. With the removal of the dull layer of gold from the surface, there was original shine on the bangles. The chemical reactions have been

Question 15.
Give reason as to why copper is used to make hot water tanks and not steel (an alloy of iron).
Answer:
Copper is a better conductor of heat than steel which is an alloy of iron. Though copper is costlier than steel, it is used to make hot water tanks for storing hot water in preference to steel.

Question 16.
Differentiate between metals and non-metals on the basis of chemical properties.
For the distinction in the chemical characteristics,

Property	Metals	Non-metals
1. Nature of oxides	Oxides of the metals are generally basic in nature (Exception : ZnO and Al2O3 are amphoteric oxides).	Oxides of non-metals are mostly acidic in nature (Exception : CO and N2O are neutral oxides).
2. Electrochemical behaviour	Metals normally form cations by the loss of electrons. This means that these are electropositive in nature.	Non-metals normally form anions by the gain of electrons. This means that these are electronegative in nature.
3. Action with dilute acids	Active metals evolve hydrogen on reacting with dilute HCl and dilute H2SO4.	Non-metals do not react with dilute acids
4. Nature of compounds	The compounds of metals are mostly ionic in nature.	Compounds of non-metals are mostly covalent although there are many exceptions.
5. Oxidising and reducing nature	Metals act as reducing agents as their atoms lose electrons. For example, Na —— > Na+ + e–	Non-metals act as oxidising agents as their atoms accept electrons. For example, Cl + e–——–>Cl–

Hope given NCERT Solutions for Class 10 Science Chapter 3 are helpful to complete your science homework.

If you have any doubts, please comment below. Learn Insta try to provide online science tutoring for you.

NCERT Solutions for Class 10 Science Chapter 3 Metals and Non-metals

NCERT Solutions for Class 10 Science Chapter 2 Acids Bases and Salts

In this chapter, students will understand the chemical properties of acids, and bases, how acids and bases react with metals, how metal carbonates, and metal hydrogen carbonates react with acids, how acids and bases react with each other, the reaction of metallic oxides with acids. Students will also learn about what all acids and bases have in common, what happens to acids and bases in water solution, the importance of pH in everyday life, pH of salts.

These Solutions are part of NCERT Solutions for Class 10 Science. Here we have given NCERT Solutions for Class 10 Science Chapter 2 Acids Bases and Salts. LearnInsta.com provides you the Free PDF download of NCERT Solutions for Class 10 Science (Chemistry) Chapter 2 – Acids, Bases and Salts solved by Expert Teachers as per NCERT (CBSE) Book guidelines. All Chapter 2 – Acids, Bases and Salts Exercise Questions with Solutions to help you to revise complete Syllabus and Score More marks.

NCERT Solutions for Class 10 Science Chapter 2 In Text Book Questions

Question 1.
You have been provided with three test tubes. One of them contains distilled water and the other two contain an acidic solution and a basic solution respectively. If you are given only red litmus paper, how will you identify the contents of each test tube ?
Answer:
Take a small volume of all the three liquids in three test tubes. Dip red litmus paper strips separately in all the three. The tube in which red litmus strip turns blue, contains basic solution. Now remove the blue litmus paper and dip it one of the remaining test tubes. If the colour of the blue litmus paper changes to red, the tube contains acidic solution. In case, it remains blue then the tube contains distilled water.

More Resources

• NCERT Solutions for Class 10 Science
• NCERT Exemplar Solutions for Class 10 Science
• HOTS Questions for Class 10 Science
• Value Based Questions in Science for Class 10
• Previous Year Question Papers for CBSE Class 10 Science

Question 2.
Why should not curd and sour substances be kept in containers made up of brass or copper ?
Answer:
Both curd and sour substances contain some acids in them. They react with copper or brass vessels to form certain salts which are of poisonous nature. Therefore, it is not advisable to keep them in these containers.

Question 3.
Which gas is usually liberated when an acid reacts with a metal ? Illustrate with an example. How will you test the presence of this gas ?
Answer:
Metals are mostly reactive in nature. They react with dilute acids (HCl and H₂SO₄) to evolve hydrogen gas. For example,

The gas burns with a pop sound when a burning candle is brought near it.

Question 4.
A metallic compound ‘A’ reacts with dilute hydrochloric acid to produce effervescence. The gas evolved extinguishes a burning candle. Write a balanced chemical equation for the reaction if one of the compounds formed is calcium chloride.
Answer:
Since the gas is evolved with effervescence and extinguishes fire, it is expected to be CO₂ gas. As calcium chloride is formed as one of the products, this means that the substance A’ can be calcium carbonate. It reacts with dilute hydrochloric acid as :

Question 5.
Aqueous solutions of HCl, HNO₃ and H₂SO₄ etc. show acidic character while those of the compounds like ethyl alcohol (C₂H₅OH) and glucose (C₆H₁₂O₆) fail to do so. Explain.
Answer:
All the acids that are listed, have replaceable hydrogen atoms which they release in aqueous solution as H⁺ ions. Therefore, they show acidic character. However, both ethyl alcohol (C₂H₅OH) and glucose (C₆H₁₂O₆) do not have replaceable hydrogen atoms. They fail to evolve hydrogen gas and do not show any acidic character.

Question 6.
Why does aqueous solution of an acid (HA) conduct electricity ?
Answer:
Aqueous solution of an acid (HA) releases H+ ions or H₃O⁺ ions and anions (A^–) in solution. Since ions are the carrier of charge, the aqueous solution of an acid conducts electricity.

Question 7.
Why does not dry HCl gas change the colour of the dry litmus paper ? (CBSE 2013)
Answer:
Dry HCl gas fails to release any H⁺ ions which means that it is not acidic. It fails to change the colour of the dry litmus paper which has also no moisture present.

Question 8.
While diluting an acid, why is it not recommended that acid should be added to water and not water to the acid ? (CBSE 2011)
Answer:
Acids particularly the mineral acids like H₂SO₄, HNO₃ and HCl etc., have strong affinity for water. The dilution process is highly exothermic in nature. The heat evolved may crack or break the container and may also convert the acid into fog which is likely to pollute the atmosphere. In order to control the heat evolved, it is advisable to add acid drop by drop to water. In case water is added to acid, then the entire acid will get itself involved in the exothermic process. It may not be possible to control the heat evolved.

Question 9.
How is concentration of hydronium ions (H₃O⁺) affected when solution of an acid is diluted with water ?
Answer:
An acid dissociates into hydronium ions (H₃O⁺) and anions when dissolved in water. Upon dilution, the volume of the solution increases and the number of ions per unit volume decreases. Therefore, the concentration of H₃O⁺ ions per unit volume decreases.

Question 10.
How is concentration of hydroxyl (OH^–) ions affected when excess of base is dissolved in solution of sodium hydroxide ?
Answer:
Sodium hydroxide (NaOH) is a strong base. It immediately dissociates in solution to give OH^– ions and cations. Upon dissolving more of base in the solution, the concentration of OH^– ions further increases.

Question 11.
You have two solutions A and B. The pH of solution A is 6 and that of solution B is 8. Which solution has more hydrogen ion concentration ? Which of these is acidic and which one is basic ?
Answer:
The pH of a solution is inversely proportional to the concentration of H⁺ ions in solution. Lesser the pH of the solution, more will be the H⁺ ion concentration. The solution A with pH 6 has more H⁺ ion concentration than the solution with pH equal to 8. The solution A is acidic because its pH is less than 7 and the solution B is basic because its pH is more than 7.

Question 12.
What effect does concentration of H⁺(aq) ions have on acidic nature of absolution ?
Answer:
The acidic nature of a solution is directly related to the concentration of H⁺ ions. As the concentration of H⁺ ions increases, the acidic nature of solution also increases.

Question 13.
Do basic solutions also have H⁺(aq) ions ? If yes, then why are these basic ?
Answer:
Yes, basic solutions have also H⁺(aq) ions present in them. Actually, these solutions are prepared in water. Being a weak electrolyte, it dissociates to give H⁺ and OH^– ions. However, the number of H⁺ ions is very small as compared to the number of OH^– ions which are released by the base and also by water. Therefore, the solutions as a whole are of basic nature.

Question 14.
Under what soil conditions, do you think a farmer would spread or treat the soil of his fields with quick lime (calcium oxide) or slaked lime (calcium hydroxide) or chalk (calcium carbonate) ?
Answer:
A soil usually becomes acidic when there is either a high peat content, iron minerals or there is some rotting vegetable. In order to reduce the acidic strength, ‘liming of soil’ is usually done. For this, any of the substances that have been mentioned are added to the soil since these are of basic nature.

Question 15.
Name the substance which upon treating with chlorine gives bleaching powder. Write the chemical equation for the reaction. (CBSE 2011)
Answer:
Slaked lime is the substance which reacts with chlorine to give bleaching powder

Question 16.
Name the sodium compound used for softening hard water.
Answer:
Washing soda or sodium carbonate. It is chemically sodium carbonate decahydrate (Na₂CO₃.10H₂O). What will happen if the solution of sodium hydrogen carbonate is heated ?

Question 17.
Write the chemical equation involved.
Answer:
Carbon dioxide gas will evolve and sodium carbonate will be left.

Question 18.
Write the chemical equation for the reaction between Plaster of Paris and water.
Answer:

NCERT Solutions for Class 10 Science Chapter 2 NCERT End Exercise

Question 1.
A solution turns red litmus blue. Its pH is likely to be
(a) 2
(b) 4
(c) 7
(d) 10.
Answer:
The solution is basic. Its pH is likely to be 10.
Therefore, (d) is the correct answer.

Question 2.
A solution reacts with crushed egg-shells to give a gas that turns lime water milky. The solution contains
(a) NaCl
(b) HCl
(c) LiCl
(d) KCl.
Answer:
The crushed egg-shells consist of layer of calcium carbonate which reacts with dilute HCl to evolve CO₂(g). The gas turns lime water milky.
Therefore (b) is the correct choice.

Question 3.
10 mL of a solution of NaOH is found to be completely neutralised by 8 mL of a given solution of HCl. If we take 20 mL of the same solution of NaOH, the volume of HCl solution (the same solution as before) required to neutralise will be
(a) 4 mL
(b) 8 mL
(c) 12 mL
(d) 16 mL.
Answer:
10 mL of NaOH will require HCl = 8 mL and 20 mL of NaOH will require HCl=16 mL.
Therefore, (d) is the correct answer.

Question 4.
Which of the following types of medicines is used for treating indigestion ?
(a) Antibiotic
(b) Analgesic
(c) Antacid
(d) Antiseptic.
Answer:
Antacid is used for treating indigestion.
The correct answer is (c).

Question 5.
Write the word equations and the balanced equations for the reactions when :
(a) dilute sulphuric acid reacts with zinc granules.
(b) dilute hydrochloric acid reacts with magnesium ribbon.
(c) dilute sulphuric acid reacts with aluminium powder.
(d) dilute hydrochloric acid reacts with iron fillings.
(d) dilute hydrochloric acid reacts with iron fillings.
Answer:

Question 6.
Compounds like alcohol and glucose also contain hydrogen but are not characterised as acids. Describe an activity to prove it.
The chemical formula of ethyl alcohol is C₂H₅OH which is an alcohol and of glucose is C₆H₁₂O₆. Both are organic compounds and contain hydrogen atoms. However, they do not behave as acids. (CBSE 2011, 2013)
Answer:
This can be shown by the following activity :
In a glass beaker, take a dilute solution of glucose (C₆H₁₂O₆). Fix two small nails of iron in a rubber cork and place the cork in the beaker as shown in the figure. Connect the nails to the terminals of a 6 volt battery through a bulb. Switch on the current. The bulb will not glow. This shows that the electric current has not passed through the glucose solution. As the current is carried by the movement of ions, this shows that in solution, glucose has not given any H⁺ ions.

Now repeat the same experiment with ethyl alcohol (C₂H₅OH). The bulb will not glow in this case also. This shows that both of them do not behave as acids although they contain hydrogen atoms in their molecules.

Question 7.
Why does not distilled water conduct electricity whereas rain water does ?
Answer:
Pure water (or distilled water) is a very weak electrolyte and does not dissociate into ions. Therefore, it does not conduct electricity. However, rain water contains some dissolved acids like carbonic acid (H₂CO₃) and sulphurous acid (H₂SO₃). Actually air contains traces of both CO₂ and SO₂ gases which dissolve in rain water to produce corresponding acids. As a result, water becomes acidulated and gets ionised easily. Therefore, rain water conducts electricity.

Question 8.
Why does not an acid show any acidic behaviour in the absence of water ?
Answer:
An acid gets ionized only in aqueous solution i.e. in the presence of water. In other words, an acid releases H+ ions or shows acidic behaviour only in the presence of water.

Question 9.
Five solutions A, B, C, D and E when tested with universal indicator show pH as 4, 2, 12, 7 and 9 respectively. Which solution is :
(a) neutral
(b) strongly alkaline
(c) strongly acidic
(d) weakly alkaline
(e) weakly acidic
(f) Arrange the pH in increasing order of H⁺ ion concentration.
Answer:
(a) Neutral : D with pH = 7
(b) Strongly alkaline : C with pH = 12
(c) Strongly acidic : B with pH =2
(d) Weakly alkaline : E with pH = 9
(e) Weakly acidic : A with pH = 4
(f) Increasing order of H+ ions concentration :C<E<D<A<B

Question 10.
Equal lengths of magnesium ribbons are taken in test tubes A and B. Hydrochloric acid (HCl) is added to test tube A while acetic acid (CH₃COOH) is added to test tube B. In which case, fizzing occurs more vigorously and why ?
Answer:
Fizzing in the reaction is due to the evolution of hydrogen gas by the action of metal on the acid

Since hydrochloric acid is a stronger acid than acetic acid, fizzing occurs more readily in tube A than in tube B. Actually hydrogen gas will evolve at more brisk speed in test tube A.

Question 11.
Fresh milk has a pH of 6. How do you think the pH will change as it turns into curd ? Explain your answer. (CBSE 2011)
Answer:
When milk changes into curd, the pH decreases. Actually, lactose (carbohydrate) present in milk gets converted into lactic acid. As more of acid is formed, pH of the medium decreases.

Question 12.
A milkman adds a very small amount of baking soda to fresh milk.
(a) Why does he shift the pH of the fresh milk from 6 to slightly alkaline ?
(b) Why does this milk take a long time to set as curd ? (CBSE 2011)
Answer:
(a) We know that fesh milk is slightly acidic due to presence of lactic acid and its pH is 6. Upon
standing, its pH slowly decreases and it becomes sour since more of acid to released. The purpose of adding baking soda or sodium hydrogen carbonate (NaHCO₃) is to make medium slighly alkaline. The base released will neutralise the effect of lactic acid present in milk. This will check the milk from getting sour.
(b) When milk sets as curd, it becomes more acidic and pH decreases. In the alkaline medium, it takes longer time to achieve acidic medium back so that milk may set as curd.

Question 13.
Why should Plaster of Paris be stored in a moisture-proof container ?
Answer:
In the presence of moisture, Plaster of Paris gets hydrated and changes to Gypsum which is a hard mass.

It can be no longer be used for making moulds and statues. Therefore, Plaster of Paris is kept in moisture proof containers or bags.

Question 14.
What is neutralisation reaction ? Give two examples.
Answer:
Neutralisation reaction is the reaction between acid and base dissolved in aqueous solution to form salt and water.

Question 15.
Both NaCl and KNO₃ are neutral in nature. They neither change blue litmus red nor red litmus blue. That is why the reaction is called neutralisation reaction.
Give two important uses of washing soda and baking soda. (CBSE 2011)
Answer:
Uses of washing soda:

1. In the manufacture of glass, soap, paper and chemicals like caustic soda (NaOH) and borax (Na₂B₄O₇) etc.
2. As a cleansing agent for domestic purposes.

Uses of baking soda:

1. In baking powder used for preparing cakes.
2. In antacids to reduce acidity in the stomach.

NCERT Solutions for Class 10 Science Chapter 2 Acids, Bases and Salts

CBSE Sample Papers for Class 10 Maths Paper 2 is part of CBSE Sample Papers for Class 10 Maths Here we have given CBSE Sample Papers for Class 10 Maths Paper 2 According to new CBSE Exam Pattern, MCQ Questions for Class 10 Maths Carries 20 Marks.

CBSE Sample Papers for Class 10 Maths Paper 2

Board	CBSE
Class	X
Subject	Maths
Sample Paper Set	Paper 2
Category	CBSE Sample Papers

Students who are going to appear for CBSE Class 10 Examinations are advised to practice the CBSE sample papers given here which is designed as per the latest Syllabus and marking scheme as prescribed by the CBSE is given here. Paper 2 of Solved CBSE Sample Papers for Class 10 Maths is given below with free PDF download solutions.

Time: 3 Hours
Maximum Marks: 80

GENERAL INSTRUCTIONS:

• All questions are compulsory.
• This question paper consists of 30 questions divided into four sections A, B, C and D.
• Section A comprises of 6 questions of 1 mark each, Section B comprises of 6 questions of 2 marks each, Section C comprises of 10 questions of 3 marks each and Section D comprises of 8 questions 1 of 4 marks each.
• There is no overall choice. However, internal choice has been provided in one question of 2 marks, 1 three questions of 3 marks each and two questions of 4 marks each. You have to attempt only one of the alternatives in all such questions.
• In question of construction, drawings shall be neat and exactly as per the given measurements.
• Use of calculators is not permitted. However, you may ask for mathematical tables.

SECTION A

Question numbers 1 to 6 carry 1 mark each.

Question 1.
Find the value of k for which the following pair of linear equations has a unique solution:
2x + 3y = 7; (k – 1)x + (k + 2)y = 3k.

Question 2.
Find the nature of the roots of quadratic equation 2x² – √5 x + 1 = 0.

Question 3.
What is the probability that a non-leap year has 53 Mondays?

Question 4.
A die is thrown once. Find the probability of getting a prime number.

Question 5.
Find the mode of the data, whose mean and median are given by 10.5 and 11.5 respectively.

Question 6.
In the adjoining figure, DE || BC. If AD = x, DB = x – 2, AE = x + 2 and EC = x – 1, find the value of x.

SECTION B

Question numbers 7 to 12 carry 2 marks each.

Question 7.
Find HCF and LCM of 90 and 144 by method of prime factorisation.

Question 8.
Find the values of a and b for which the following pair of linear equations has infinitely many solutions:
3x – (a + 1)y = 2b – 1; 5x + (1 – 2a)y = 3b.

Question 9.
Without using trigonometric tables, evaluate the following:
(cos² 25° + cos² 65°) + cosec θ . sec (90° – θ) – cot θ tan (90° – θ).

Question 10.
ABC is a triangle and G (4, 3) is the centroid of the triangle. If A, B and C are the points (1, 3), (4, b) and (a, 1) respectively, find the values of a and b. Also find the length of side BC.

Question 11.
In the adjoining figure, DE || AC and \(\frac { BE }{ EC } =\frac { BC }{ CP } \) . Prove that DC || AP.

Question 12.
In the adjoining figure, a circle is inscribed in a quadrilateral ABCD in which ∠B = 90°. If AD = 23 cm, AB = 29 cm and DS = 5 cm, find the radius (r) of the circle.

SECTION C

Question numbers 13 to 22 carry 3 marks each.

Question 13.
If two zeroes of the polynomial x⁴ + 3x³ – 20x² – 6x + 36 are √2 and – √2 , find the other zeroes of the polynomial.

Question 14.
If α and β are zeroes of the polynomial 6x² – 7x – 3, then form a quadratic polynomial whose zeroes are \(\frac { 1 }{ \alpha } \) and \(\frac { 1 }{ \beta } \).

Question 15.
How many terms of the A.P. -6, \(\frac { 11 }{ 2 }\), -5,……. double answer.are needed to give the sum – 25? Explain the
OR
The 19th term of an AP is equal to three times its 6th term. If its 9th term is 19, find the AP.

Question 16.
The father’s present age is six times his son’s ages. Four years hence the age of the father will be four times his son’s age. Find the present ages of the father and son.
OR
The ratio of incomes of two persons is 9 : 7 and the ratio of their expenditures is 4 : 3. If each of them manages to save Rs 2000 per month, find their monthly incomes.

Question 17.
ABC is a right triangle, right angled at C. If p is the length of perpendicular from C to AB and a, b, c have usual meanings, then prove that \(\frac { 1 }{ { p }^{ 2 } } =\frac { 1 }{ { a }^{ 2 } } +\frac { 1 }{ { b }^{ 2 } } \)
OR
If the diagonals of a quadrilateral divide each other proportionally, prove that it is a trapezium.

Question 18.
PQ is a tangent to a circle with centre O at the point Q: A chord QA is ‘drawn parallel to PO. If AOB is a diameter of the circle, prove that PB is tangent to the circle at the point B.

Question 19.
The radii of the circular ends of a bucket of height 15 cm are 14 cm and r cm (r < 14 cm). If the volume of bucket is 5390 cm³, then find the value of r.

Question 20.
Find the area of the major segment APB in adjoining figure, of a circle of radius 35 cm and ∠AOB = 90°.

OR
In adjoining figure, a semicircle is drawn with O as centre and AB as diameter. Semicircles are drawn with AO and OB as diameters. If AB = 28 m, find the perimeter of the shaded region.

Question 21.
Prove that :

Question 22.
Prove that :

SECTION D

Question numbers 23 to 30 carry 4 marks each.

Question 23.
Prove that √5 is an irrational number and hence show that 2 + √5 is also an irrational number.

Question 24.
If two vertices of an equilateral triangle are (3, 0) and (6, 0), find the third vertex.
OR
The mid-points D, E and F of the sides AB, BC and CA of a triangle are (3, 4), (8, 9) and (6, 7) respectively. Find the coordinates of the vertices of the triangle.

Question 25.
Water is flowing at the rate of 15 km/hour through a pipe of diameter 14 cm into a cuboidal pond which is 50 m long and 44 m wide. In what time will the level of water in the pond rise by 21 cm?

Question 26.
While boarding an aeroplane, a passenger got hurt. The pilot showing promptness and concern, made arrangements to hospitalise the injured and so the plane started late by 30 minutes. To reach the destination, 1500 km away in time, the pilot increased the speed by 100 km/h. Find the original speed/hour of the plane.
Do you appreciate the values shown by pilot, namely promptness in providing help to the injured and his efforts to reach in time.

Question 27.
Draw a pair of tangents to a circle of radius 3 cm which are inclined at an angle of 60° to each other.

Question 28.
The angle of elevation of the top of a building from the foot of a tower is 30° aid the angle of elevation of the top of the tower from the foot of the building is 60°. If the tower is 50 m high, find the height of the building.
OR
The angles of depression of two ships from the top of a lighthouse and on the same side of it are found to be 45° and 30°. If the ships are 200 m apart, find the height of the lighthouse.

Question 29.
Three coins are tossed simultaneously, find the probability of getting:
(i) atleast one head
(ii) atmost two heads
(iii) exactly 2 heads
(iv) no head.

Question 30.
The mean of the following frequency distribution is 62.8 and the sum Of all the frequencies is 50. Compute the missing frequencies f1 and f2:

OR
The following frequency distribution gives the monthly consumption of electricity of 68 consumers of a locality. Find the median, mode and mean of the data:

Answers

Answer 1.
For unique solution, \(\frac { 2 }{ k-1 } \neq \frac { 3 }{ k+2 } \) ⇒ 2k + 4 ≠ 3k – 3
⇒ k ≠ 7
Hence, the given pair of linear equations will have unique solution for all real values of k except 7.

Answer 2.
Given 2x² – √5x + 1 = 0
D = (-√5)² – 4 x 2 x 1 = 5 – 8 = – 3
∵D < 0, therefore, given equation has no real roots.

Answer 3.
There are 365 days in a non-leap year.
365 days = 52 weeks + 1 day
∴ One day can be M, T, W, Th, F, S, Su = 7 ways
∴ P(53 Mondays in non-leap year) = \(\frac { 1 }{ 7 }\)

Answer 4.
Total number of outcomes = 6(1, 2, 3, 4, 5 or 6)
Favourable number of outcomes = 3(2, 3, 5)
∴ P(prime number) = \(\frac { 3 }{ 6 } =\frac { 1 }{ 2 } \)

Answer 5.
Mode = 3 Median – 2 Mean .
= 3 x 11.5 – 2 x 10.5 = 34.5 – 21 – 13.5
Hence, mode = 13.5

Answer 6.
∵ DE || BC
∴ By Basic Proportionality Theorem, we have

\(\frac { AD }{ DB } =\frac { AE }{ EC } \)
⇒ \(\frac { x }{ x-2 } =\frac { x+2 }{ x-1 } \)
⇒ x (x – 1) = (x – 2) (x + 2)
⇒ x² – x – x² – 4
⇒ -x = -4
⇒ x = 4.

Answer 7.
90 = 2 x 3 x 3 x 5
and 144 = 2 x 2 x 2 x 2 x 3 x 3
∴ HCF = 2 x 3 x 3 = 18
and LCM = 2 x 2 x 2 x 2 x 3 x 3 x 5 = 720
Hence, HCF = 18 and LCM = 720.

Answer 8.
Given 3x – (a + 1 )y – (2b – 1) = 0
and 5x + (1 – 2a)y – 3b = 0

Hence, a = 8 and b = 5.

Answer 9.
(cos² 25° + cos² 65°) + cosec θ . sec (90° – θ) – cot θ . tan (90° – θ)
= cos² 25° + cos² (90° – 25°) + cosec θ . cosec θ – cot θ . cot θ
= cos² 25° + sin² 25° + cosec² θ – cot² θ
= 1 + 1
= 2.

Answer 10.
Since G (4, 3) is the centroid of ∆ABC, we have

Answer 11.
In ∆ABC, DE || AC

Answer 12.
AR = AQ, DR = DS, BP = BQ (lengths of tangents)
but DS = 5 cm => DR = 5 cm.
AR = AD – DR = 23 cm – 5 cm = 18 cm => AQ = 18 cm.
BQ = AB – AQ = 29 cm – 18 cm = 11 cm.
As AB is tangent to the circle at Q, OQ ⊥ AB
=> ∠OQB = 90°.
Also ∠B = 90° (given) => OQBP is a rectangle.
But BP = BQ => OQBP is a square.
∴ Radius = r = OQ = BQ = 11 cm.

Answer 13.
∴ √2 and -√2 are the zero’s of the given polynomial.
∴ (x – √2) (x + √2) i.e. (x² – 2) is a factor of the given polynomial.

To find the other two zero’s, we proceed as follows:
x² + 3x – 18 = 0
⇒ (x + 6) (x – 3) = 0
⇒ x + 6 = 0 or x – 3 = 0
⇒ x = -6 or x = 3
Hence, other zero’s are -6 and 3.

Answer 14.
Given α and β are zero’s of quadratic polynomial 6x² – 7x – 3,

Answer 15.
Here, a = -6, d = \(-\frac { 11 }{ 2 }- (-6)\) = \(-\frac { 11 }{ 2 }+6\) = \(\frac { 1 }{ 2 }\) ,S_n = -25.
We are required to find n.

Answer 16.
Let the father’s present age be x years
and the son’s present age be y years.
According to given, x – 6y …(i)
4 years later,
father’s age = (x + 4) years
and son’s age = (y + 4) years
∴ (x + 4) = 4(y + 4)
=> x – 4y = 12 …(ii)
Putting the value of x from (i) in (ii), we get
6y – 4 y = 12 => 2y = 12 => y = 6
∴ x = 6 x 6 = 36
Hence, father’s present age = 36 years and son’s present age = 6 years
OR
Let the incomes per month of two persons be Rs x and Rs y respectively. As each person saves Rs 2000 per month, so their expenditures are Rs (x – 2000) and Rs (y – 2000) respectively.
According to given, we have
\(\frac { x }{ y } =\frac { 9 }{ 7 } \) i.e- 7x – 9y = 0 …(i)
and \(\frac { x-2000 }{ y-2000 } =\frac { 4 }{ 3 } \) i.e. 3x – 4y + 2000 = 0 …(ii)
Multiplying equation (i) by 3 and equation (ii) by 7, we get
21x – 27y = 0 …(iii) and 21x – 28y + 14000 = 0 …(iv)
Subtracting equation (iv) from equation (iii), we get
y – 14000 = 0 => y = 14000.
Substituting this value of y in (i), we get
7x – 9 x 14000 = 0 =>x = 18000.
Hence, the monthly incomes of the two persons are Rs 18000 and Rs 14000 respectively.

Answer 17.
In ∆ACB and ∆CDB,
∠ACB = ∠CDB (both 90°)
∠B = ∠B (common)
∴ ∆ACB ~ ∆CDB (by AA similarity criterion)

Answer 18.
Given a circle with centre O and PQ is tangent to the circle at the point Q from an external point P. Chord QA is parallel to PO and AOB is a diameter.
We need to prove that PB is tangent to the circle at the point B.
Join OQ and mark the angles as shown in the adjoining figure.

As QA || PO,
∠1 = ∠2 (alt. ∠s)
and ∠4 = ∠3 (corres. ∠s)
.But ∠2 = ∠3 (∵ in ∆OAQ, OA = OQ being radii)
∴ ∠1 = ∠4.
In ∆OPB and ∆OPQ,
OB = OQ (radii of same circle)
∠1 = ∠4 (proved above)
OP = OP (common)
∴ ∆OPB ≅ ∆OPQ (SAS congruence rule)
∴ ∠OBP = ∠OQP (c.p.c.t)
=> ∠OBP = 90° (tangent is ⊥ to radius ,OQ⊥PQ)
=> OB ⊥ PB i.e. radius is perpendicular to PB at point B.
Therefore, PB is tangent to the circle at the point B.

Answer 19.
Given h = 15 cm, R = 14 cm, ‘r’ = r cm and volume of bucket = 5390 cm³
∵Volume of bucket = volume of frustum of cone

∴r cannot be negative
∵Radius = r = 7 cm.

Answer 20.
Given r = 35 cm and ∠AOB = 90°
Area of minor segment = area of minor sector – area (∆OAB)

Answer 21.

Answer 22.

Answer 23.
Let √5 be a rational number, then
√5 = \(\frac { p }{ q }\), where p, q are integers, q ≠ 0 and p, q have no common factors (except 1)
=>\(5=\frac { { p }^{ 2 } }{ { q }^{ 2 } } \) => p² = 5q²
As 5 divides 5q², so 5 divides p², but 5 is prime.
=> 5 divides p
Let p = 5m, where m is an integer.
Substituting this value of p in (i), we get
(5m)² = 5q² => 25m² = 5q² => 5m² = q²
As 5 divides 5m², so 5 divides q², but 5 is prime
=> 5 divides q
Thus p and q have a common factor 5. This contradicts that p and q have no common factors (except 1)
Hence, √5 is not a rational number.
So, we conclude that √5 is an irrational number.
Let 2 + √5 be a rational number, say r
Then, 2 + √5 = r => √5 = r – 2
As r is rational, r – 2 is rational => √5 is rational
But this contradicts the fact that √5 is irrational.
Hence, our assumption is wrong. Therefore, 2 + √5 is an irrational number.

Answer 24.
Given vertices are A(3, 0) and B(6, 0) and let third vertex be C(x, y), then

OR
Let the vertices A, B and C of the triangle ABC be (x1 y1), (x2, y2) and (x3 y3) respectively.
Since points D and F are mid-points of the sides AB and
AC respectively, by mid-point theorem, DF || BC and
DF = \(\frac { 1 }{ 2 }BC\) but E is mid-point of BC, so DF || BE and
DF = BE.
Therefore, DBEF is a parallelogram.
Similarly, DECF and DEFA are parallelograms.
Since the diagonals of a parallelogram bisect each other, mid-points of diagonals BF and DE are same.

Answer 25.
Radius of pipe = \(\frac { 14 }{ 2 }\) cm = 7 cm = \(\frac { 7 }{ 100 }\) m = 0.07 m
As the water is flowing at the rate of 15 km per hour,
the length of water delivered by the circular pipe in 1 hour
= 15 km = 15000 m
Volume of water delivered by the circular pipe in 1 hour

Hence, the level of water in the pond rise by 21 cm in 2 hours.

Answer 26.
Let the original speed of the aeroplane be x km/h.
Time taken to cover the distance of 1500 km = \(\frac { 1500 }{ x }\) hours
New speed of the aeroplane = (x + 100) km/h.
Time taken to cover the distance of 1500 km at new speed = \(\frac { 1500 }{ x+100 }\) hours

=> x² + 100x – 300000 = 0
=> x² + 600x – 500x – 300000 = 0 => (x – 500) (x + 600) = 0
=> x = 500 or x = -600
But speed cannot be negative.
Hence, the original speed of the aeroplane = 500 km/h.
Yes, I appreciate the values shown by the pilot. Along with showing concern for the injured passenger he did not fail to perform his duty, by increasing the speed of the plane, he reached the destination on time.

Answer 27.
Steps of construction:
1. Draw a circle of radius 3 cm with O as its centre.
2. Draw any radius OA.
3. At O, construct ∠AOC = 120° to meet the circle at B.
4. At A, construct ∠OAR = 90°.
5. At B, construct ∠OBQ = 90° to meet AR at P.

Then PA and PB are tangents to the circle inclined at an angle of 60° to each other.
Justification:
As ∠APB and ∠AOB are supplementary, so ∠APB = 60°.

Answer 28.
Let CD = h metres be the height of the building and AB be the tower, then AB = 50 m.
Let BD = d metres be the distance between the foot of the tower and the foot of the building.
Given, ∠CBD = 30° and ∠ADB = 60°.
From right angled ∆CBD, we get

OR
Let the height of the lighthouse AB be h metres and C, D be the positions of two ships. The angles of depressions are shown in the adjoining figure.
Then ∠ACB = 45° and ∠ADB = 30°
Given CD = 200 m, let BC = x metres.
From right angled ∆ABC, we get

Answer 29.
When three coins are tossed simultaneously, the outcomes of the random experiment are:
HHH, HHT, HTH, THH, HTT, THT, TTH, TIT
It has 8 equally likely outcomes.
(i) The outcomes favourable to the event ‘atleast one head’ are
HHH, HHT, HTH, THH, HTT, THT, TTH; which are 7 in number.
∴ P(atleast one head) = \(\frac { 7 }{ 8 }\)
(ii) The outcomes favourable to the event ‘atmost two heads’ are
HHT, HTH, THH, THT, HTT, TTH, TTT; which are 7 in number.
∴P(atmost two heads) = \(\frac { 7 }{ 8 }\)
(iii) The outcomes favourable to the event ‘exactly 2 heads’ are
HHT, HTH, THH; which are 3 in number.
∴ P(exactly two heads) = \(\frac { 3 }{ 8 }\)
(iv) The only outcome favourable to the event ‘no head’ is TTT.
∴P(no head) = \(\frac { 1 }{ 8 }\)

Answer 30.
Given, sum of all frequencies = 50

We hope the CBSE Sample Papers for Class 10 Maths Paper 2 help you. If you have any query regarding CBSE Sample Papers for Class 10 Maths Paper 2, drop a comment below and we will get back to you at the earliest.

CBSE Sample Papers for Class 10 Maths Paper 1 is part of CBSE Sample Papers for Class 10 Maths Here we have given CBSE Sample Papers for Class 10 Maths Paper 1. According to new CBSE Exam Pattern, MCQ Questions for Class 10 Maths Carries 20 Marks.

CBSE Sample Papers for Class 10 Maths Paper 1

Board	CBSE
Class	X
Subject	Maths
Sample Paper Set	Paper 1
Category	CBSE Sample Papers

Students who are going to appear for CBSE Class 10 Examinations are advised to practice the CBSE sample papers given here which is designed as per the latest Syllabus and marking scheme as prescribed by the CBSE is given here. Paper 1 of Solved CBSE Sample Papers for Class 10 Maths is given below with free PDF download solutions.

Time: 3 Hours
Maximum Marks: 80

GENERAL INSTRUCTIONS:

• All questions are compulsory.
• This question paper consists of 30 questions divided into four sections A, B, C and D.
• Section A comprises of 6 questions of 1 mark each, Section B comprises of 6 questions of 2 marks each, Section C comprises of 10 questions of 3 marks each and Section D comprises of 8 questions 1 of 4 marks each.
• There is no overall choice. However, internal choice has been provided in one question of 2 marks, 1 three questions of 3 marks each and two questions of 4 marks each. You have to attempt only one of the alternatives in all such questions.
• In question of construction, drawings shall be neat and exactly as per the given measurements.
• Use of calculators is not permitted. However, you may ask for mathematical tables.

SECTION A

Question numbers 1 to 6 carry 1 mark each.

Question 1.
Given that LCM (91, 26) = 182, find HCF (91, 26).

Question 2.
Does the rational number \(\frac { 441 }{ { 2 }^{ 2 }.{ 5 }^{ 7 }.{ 7 }^{ 2 } } \) has a terminating or a non-terminating decimal representation?

Question 3.
If 1 is a zero of the polynomial p(x) = ax² – 3 (a – 1) x – 1, then find the value of a.

Question 4.
The nth turn of an AP is 7 – 4n. Find its common difference.

Question 5.
If adjoining figure, DE || BC and AD = 1 cm, BD = 2 cm. What is the ratio of the area of ∆ABC to the area of ∆ADE?

Question 6.
In adjoining figure, PA and PB are tangents to the circle drawn an external point P. CD is a third tangent touching the circle at PB = 10 cm and CQ = 2 cm, what is the length of PC?

SECTION B

Question numbers 7 to 12 carry 2 marks each.

Question 7.
Determine the values of m and n, so that the following system of linear equations has infinite number of solutions:
(2m – 1)x + 3y – 5; 3x + (n – 1)y – 2 = 0.

Question 8.
Find the roots of the quadratic equation: 4 √3 x² + 5x – 2 √3 =0.

Question 9.
A bag contains 5 red, 8 green and 7 white balls. One ball is drawn at random from the bag. Find the probability of getting:
(i) a white ball or a green ball.
(ii) neither a green ball nor a red ball.

Question 10.
Without using trigonometric tables, find the value of
\(\frac { cos{ 70 }^{ o } }{ sin{ 20 }^{ o } } \) + cos 57° . cosec 33° – 2 cos 60°.

Question 11.
In the adjoining figure, E is a point on the side CB produced of an isosceles triangle ABC with AB = AC. If AD ⊥ BC and EF ⊥ AC,
prove that: AB x EF = AD x EC.

Question 12.
Two circles touch externally. The sum of their areas is 58π cm² and the distance between their centres is 10 cm. Find the radii of the two circles.

SECTION C

Question numbers 13 to 22 carry 3 marks each.

Question 13.
Prove that

Question 14.
Prove that: (1 + cot A – cosec A) (1 + tan A + sec A) = 2.

Question 15.
Solve the following pair of linear equations:

OR
The sum of the numerator and denominator of a fraction is 4 more than twice the numerator. If the numerator and denominator both increased by 3, they are in the ratio 2 : 3. Determine the fraction.

Question 16.
Show that \(\frac { 1 }{ 2 }\) and \(\frac { -3 }{ 2 }\) are the zeroes of the polynomial 4x² + 4x – 3 and verify the relationship between zeroes and coefficients of the polynomial.
OR
Quadratic polynomial 2x² – 3x + 1 has zeroes as α and β. Form a quadratic polynomial whose zeroes are 3α and 3β.

Question 17.
Construct a ∆ABC in which CA = 6 cm, AB = 5 cm and ∠BAC = 45°, then construct a triangle similar to the given triangle whose sides are \(\frac { 6 }{ 5 }\) of the corresponding sides of the ∆ABC.

Question 18.
Find the values of k if the points A(k + 1, 2k), B(3k, 2k + 3) and C(5k – 1, 5k) are collinear. .
OR
If P(9a – 2, -b) divides the line segment joining A(3a + 1, -3) and B(8a, 5) in the ratio 3 : 1, find the values of a and b.

Question 19.
Prove that the points A (-3, 0), B (1, – 3) and C(4, 1) are the vertices of an isosceles right triangle.

Question 20.
Cards marked with all 2-digit numbers are placed in a box and are mixed thoroughly. One card is drawn at random. Find the probability that the number on the card is
(i) divisible by 10
(ii) a perfect square number
(iii) a prime number less than 25.
OR
The king, queen and jack of clubs are removed from a deck of 52 playing cards and the remaining cards are shuffled. A card is drawn from the remaining cards. Find the probability of getting a card of
(i) hearts
(ii) queen
(iii) clubs.

Question 21.
Find the median of the following data:

Question 22.
The following frequency distribution shows the number of rims scored by some batsmen of India in one-day cricket matches:

Find the mode for the above data.

SECTION D

Question numbers 23 to 30 carry 4 marks each.

Question 23.
Prove that n³ – n is divisible by 6 for every positive integer n.

Question 24.
A sum of Rs 1600 is to be used to give 10 cash prizes to students of a school for their overall academic performance. If each prize is Rs 20 less than its preceding prize, find the value of each of the prizes.
What is the importance of an academic prize in student’s life?

Question 25.
A motor boat whose speed is 20 km/h in still water takes 1 hour more to go 48 km upstream than to return downstream to the same spot. Find the speed of the stream.
OR
A shopkeeper buys some books for Rs 80. If he had bought 4 more books for the same amount, each book would have cost Rs 1 less. Find the number o.f books he bought.

Question 26.
Prove that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.

Question 27.
In the adjoining figure, XY and X’Y’ are two parallel tangents to a circle with centre O and another tangent AB with point of contact C intersects XY at A and X’Y’ at B. Prove that ∠AOB = 90°.

OR
The radii of two concentric circles are 13 cm and 8 cm. AB is a diameter of the bigger circle. BD is a tangent to the smaller circle touching it at D. Find the length AD.

Question 28.
The angles of elevation of the top of a tower from two points P and Q at distances of a and b respectively from the base and in the same straight line with it are complementary. Prove that the height of the tower is √ab .
OR
The angle of elevation of the top of a vertical tower from a point on the ground is 60°. From another point 10 m vertically above the first, its angle of elevation is 45°. Find the height of the tower.

Question 29.
In the adjoining figure, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm, find
(i) the area of the shaded region.
(ii) the perimeter of the shaded region.

Question 30.
A building is in the form of cylinder surmounted by a hemispherical dome (shown in the adjoining figure). The base diameter of the dome is equal to \(\frac { 2 }{ 3 }\) of the total height of the building. Find the height of the building if it contains \(67\frac { 1 }{ 21 }\) m³ of air.

Answers

Answer 1.
∵ HCF x LCM = 91 x 26
⇒HCF x 182 = 91 x 26
⇒HCF = \(\frac { 91X26 }{ 182 }\) = 13.

Answer 2.

Now numerator and denominator are co-prime and denominator is of the form 2^m x 5ⁿ. Hence, given rational number is terminating.

Answer 3.
Given 1 is a zero of p(x) = ax² – 3 (a – 1) x – 1
⇒ p(1) = 0 ⇒ a x 1² – 3(a – 1) x 1 – 1 = 0
⇒ a – 3a + 3 – 1 = 0
⇒ – 2a = – 2 ⇒ a = 1

Answer 4.
Given a_n = 7 – 4n ⇒a₂ = 7 – 4 x 2 = -1 and a₁ = 7 – 4 x 1 = 3
∴ common difference d = a₂ – a₁ = -1 – 3 = -4

Answer 5.

Area of ∆ABC : Area of ∆ADE = 9:1

Answer 6.
PC = PA – CA = PB – CQ = (10 – 2) cm = 8 cm (∵ PA = PB and CA = CQ)

Answer 7.
For infinite number of solutions
\(\frac { { a }_{ 1 } }{ { a }_{ 2 } } =\frac { { b }_{ 1 } }{ { b }_{ 2 } } =\frac { { c }_{ 1 } }{ { c }_{ 2 } } \)

Answer 8.
4√3 x² + 5x – 2√3 = 0
=> 4√3 x² + 8x – 3x – 2√3 = 0
=> 4x(√3x + 2) – √3(√3x + 2) = 0
=> (√3x + 2) (4x – √3 ) = 0
=> √3x + 2 = 0 or 4x – √3 = 0
\(x=-\frac { 2 }{ \sqrt { 3 } } or\frac { \sqrt { 3 } }{ 4 } \)

Answer 9.
Total number of possible outcomes = 5 + 8 + 7 = 20
(i) Number of favourable outcomes = number of white balls or number of green balls
= 7 + 8 = 15
∴ P (a white or a green ball) = \(\frac { 15 }{ 20 } =\frac { 3 }{ 4 } \)
(ii) Number of favourable outcomes = number of white balls = 7
∴ P (neither green nor red ball) = \(\frac { 7 }{ 20 }\)

Answer 10.
\(\frac { cos{ 70 }^{ o } }{ sin{ 20 }^{ o } } \) + cos 57° . cosec 33° – 2 cos 60°.

Answer 11.
Given, ∆ABC is an isosceles triangle with AB = AC.
⇒ ∠C = ∠B (angles opp. equal sides of a ∆ are equal)
In ∆ABD and ∆ECF,
∠ABD = ∠ECF (∵ ∠B = ∠C, proved above)
and ∠ADB = ∠EFC (each = 90°)
∴ ∆ABD ~ ∆ECF (by AA similarity criterion)
∴ \(\frac { AB }{ EC } =\frac { AD }{ EF } \) ⇒ AB x EF = AD x EC, as required.

Answer 12.
Let r₁ cm and r₂ cm be the radii of two circles, then
r₁ + r₂ = 10 ….(i)

If r₁ = 7, then r₂ = 3 and if r₁ = 3, then r₂ = 7.
Hence, the radii of two circles are 7 cm and 3 cm.

Answer 13.

Answer 14.
(1 + cot A – cosec A) (1 + tan A + sec A)

Answer 15.
Given

Answer 16.
Let f(x) = 4x² + 4x – 3, then

Answer 17.
Steps of construction:
1. Draw AB = 5 cm.
2. At the point A draw ∠BAX = 45°.
3. From AX cut off AC = 6 cm.
4. Join BC. ∆ABC is formed with given data.
5. Draw \(\overrightarrow { AL } \) making acute angle with AB as shown in the figure.
6. Draw 6 arcs at P1 P2, P3, P4, P5 and P6 such that AP1 = P1P2 = P2P3 = P3P4 = P4P5 = P5P6.
7. Join BP5.
8. Draw B’P6 || BP5 meeting AB produced at B’.
9. From B’, draw B’C’ || BC meeting AX at C’.
∆AB’C’ ~ ∆ABC.

Answer 18.
Since the given points A(k + 1, 2k), B(3k, 2k + 3) and C(5k – 1, 5k) are collinear, the area of triangle formed by them is zero

Answer 19.

∴AB² + BC² = AC² and AB = BC
Hence ABC is a right angled isosceles triangle , right angled at B

Answer 20.
Cards have numbers 10 to 99 (both inclusive) i.e. 10, 11, 12, … 99.
∴ Total number of cards = 90 (99 – 9 = 90)
(i) The numbers from 10 to 99 which are divisible by 10 are 10, 20, 30, …, 90
The number of’such numbers = 9.
∴ Required probability = \(\frac { 9 }{ 90 } =\frac { 1 }{ 10 } \)
(ii) The numbers from 10 to 99 which are perfect squares are 16, 25, 36, 49, 64, 81.
The number of such numbers = 6.
∴ Required probability = \(\frac { 6 }{ 90 } =\frac { 1 }{ 15 } \)
(iii) The numbers from 10 to 99 which are prime numbers less than 25 are 11, 13, 17, 19, 23.
The number of such numbers = 5.
∴ Required probability = \(\frac { 5 }{ 90 } =\frac { 1 }{ 18 } \)
OR
After removing king, queen and jack of clubs, the number of cards left = 49
(i) The number of cards of hearts left in the remaining cards = 13
∴ P (a card of hearts) = \(\frac { 13 }{ 49 }\)
(ii) The number of cards of queens left in the remaining cards = 13
∴ P (a card of queen) = \(\frac { 3 }{ 49 }\)
(iii) The number of cards of clubs left in the remaining cards = 10
∴ P (a card of clubs) = \(\frac { 10 }{ 49 }\)

Answer 21.

Answer 22.
The maximum class frequency is 10 and the class corresponding to this frequency is 6000 – 8000. So, the modal class is 6000 – 8000.
Here, l = 6000, h = 2000, f₁ = 10, f₀ = 8 and f₂ = 2

∴ Mode = 6400

Answer 23.
Given n is any positive integer. Applying Euclid’s lemma with divisor = 6, we get
n = 6q, 6q + 1, 6q + 2, 6q + 3, 6q + 4 or 6q + 5, where q is some whole number.
Six cases arise.
Case I.
If n = 6q, then
n³ – n = n(n – 1) (n + 1) = 6q(6q – 1 )(6q + 1), which is divisible by 6.

Case II.
If n = 6q + 1, then
n³ – n = n(n – 1 )(n + 1) = (6q + 1)(6q)(6q + 2)
= 12q(6q + 1)(3q + 1), which is divisible by 6.

Case III.
If n = 6q + 2, then
n³ – n – n(n – 1 )(n + 1) = (6q + 2)(6q + 1)(6q + 3)
= 6(3q + 1)(6q + 1)(2q + 1), which is divisible by 6.

Case IV.
If n – 6q + 3, then
n³ – n = n(n – 1 )(n + 1) = (6q + 3)(6q + 2)(6q + 4)
= 12(2q + 1)(3q + 1)(3q + 2), which is divisible by 6.

Case V.
If n = 6q + 4, then
n³ – n = n(n – 1 )(n + 1) = (6q + 4)(6q + 3)(6q + 5)
= 6(3q + 2)(2q + 1)(6q + 5), which is divisible by 6.

Case VI.
If n = 6q + 5, then
n³ – n = n(n – 1 )(n + 1) = (6q + 5)(6q + 4)(6q + 6)
= 12(69 + 5)(3q + 2)(q + 1), which is divisible by 6.
Thus, in all cases, n³ – n is divisible by 6.
Hence, for every positive integer n, n³ – n is divisible by 6.

Answer 24.
Let the first cash prize be Rs x.
It is given that each prize is Rs 20 less than its preceding prize.
These cash prizes form an AP with a = x, d = – 20, S_n = 1600 and n = 10.

⇒ 320 = 2x – 180
⇒ 2x = 500
⇒ x = 250.
Therefore, the prizes are 250, 250 – 20, 250 – 2 × 20, 250 – 3 × 20, 250 – 4 × 20, 250 – 5 × 20,
i.e., 250, 230, 210, 190, 170, 150, 130, 110, 90, 70
Hence, the values of cash prizes are
Rs 250, Rs 230, Rs 210, Rs 190, Rs 170, Rs 150, Rs 130, Rs 110, Rs 90 and Rs 70.
Academic prize generates spirit of competition. One starts doing hard work which leads to success.

Answer 25.
Let the speed of the stream be x km/h.
Given, speed of the motor boat in still water = 20 km/h.
Then, the speed of the boat upstream = (20 – x) km/h
and the speed of the bpat downstream = (20 + x) km/h
Time taken by the motor boat to cover 48 km upstream = \(\frac { 48 }{ 20-x }\) hours,
time taken by the motor boat to cover 48 km downstream = \(\frac { 48 }{ 20+x }\) hours.
According to given, \(\frac { 48 }{ 20-x }\) – \(\frac { 48 }{ 20+x }\) = 1
⇒ 48(20 + x) – 48(20 – x) = (20 – x) (20 + x) ⇒ 48(2x) = 20² – x²
⇒ x² + 96x – 400 = 0
⇒ (x – 4) (x + 100) = 0
⇒ x = A or x = – 100 but x being the speed of the stream cannot be negative
⇒ x = 4
Hence, the speed of the stream is 4 km/h.
OR
Let the number of books the shopkeeper bought be x.
Given, total cost of books = Rs 80
Then cost of one book = \(\frac { 80 }{ x }\)
When he had bought 4 more books for same amount i.e. Rs 80, then cost of one book = \(\frac { 80 }{ x+4 }\)
According to given \(\frac { 80 }{ x }\) – \(\frac { 80 }{ x+4 }\) = 1
⇒ 80(x + 4) – 80x = x(x + 4)
⇒ 320 = x² + 4x
⇒ x² + 4x – 320 = 0
⇒(x + 20) (x- 16) = 0
⇒ x = 16 or x = – 20 but x being the number of books cannot be negative
⇒ x = 16
Hence, the number of books he bought = 16.

Answer 26.
Given. ABC is a right triangle right angled at A so that BC is its hypotenuse.
To prove. BC² = AB² + AC².
Construction. From A, draw AD ⊥ BC.
Proof. In ∆DBA and ∆ABC,

∠ABD = ∠ABC (same angle)
and ∠ADB = ∠BAC (each = 90°)
∴ ∆DBA ~ ∆ABC (AA similarity criterion)
\(\frac { AB }{ BC } =\frac { BD }{ AB } \) => AB² = BD x BC …(i)
In ∆DAC and ∆ABC,
∠ACD = ∠ACB (same angle)
and ∠ADC = ∠BAC (each = 90°)
∴ ∆DAC ~ ∆ABC (AA similarity criterion)
\(\frac { AC }{ BC } =\frac { DC }{ AC } \) => AC² = DC x BC …(ii)
On adding (i) and (ii), we get
AB² + AC² = BD x BC + DC x BC
= (BD + DC) x BC = BC x BC
=> AB² + AC² = BC².
Hence, BC² = AB² + AC².

Answer 27.
Join OP, OQ and OC. Mark the angles as shown in the figure.

In ∆OAC and ∆OAP,
OA = OA (common)
OC = OP (radii of some circle)
AC = AP (tangent drawn from A)
∴ ∆OAC ≅ ∆OAP (by SSS rule of congruency)
∴∠1 = ∠2 => ∠PAC = 2∠1 …(i)
Similarly,
∴∆OBC ≅ ∆OBQ,
∴ ∠3 = ∠4 => ∠QBC = 2∠3 …(ii)
As XY || X’Y’ and AB is a transversal,
∠PAC + ∠QBC = 180° (sum of co-int ∠S)
=> 2∠1 + 2∠3 = 180° [using (i) and (ii)]
=> ∠1 + ∠3 = 90° …(iii)
In ∆OAB, ∠AOB + ∠1 + ∠3 = 180° (sum of angles of a ∆)
=> ∠AOB + 90° = 180° (using (iii))
=> ∠AOB = 90°.
OR

Let the line BD meet the bigger circle at E. Join AE. Let O be the centre of two concentric circles.
As AB is a diameter of the bigger circle, O is mid-point of AB.
BD is tangent to the smaller circle and OD is radius of smaller circle through the point of contact D, OD ⊥ BE.
Since BE is a chord of the bigger circle and OD ⊥ BE,
BD = DE
(∵ Perpendicular from the centre to a chord bisects it)
=> D is mid-point of BE
∴ OD = \(\frac { 1 }{ 2 }AE\)
(∵ Segment joining the mid-points of any two sides of a triangle is half of the third side)
=> AE = 2 OD => AE = (2 x 8) cm = 16 cm (∵ OD = 8 cm)
In ∆OBD; ∠ODB = 90°, by Pythagoras theorem, we get
OB² = BD² + OD² => 13² = BD² + 8² (∵ OB is radius of bigger circle, so OB = 13 cm)
=> BD² = 169 – 64 = 105 => BD = √105 cm
But DE = BD => DE = √105 cm
Now ∠AEB = 90° (∵ angle in a semicircle = 90°)
=> ∠AED = 90°
In ∆ADE; ∠AED = 90°, by Pythagoras theorem, we get
AD² = AE² + DE² => AD² = 16² + (√105)² = 256 + 105 = 361
=> AD = √361 cm => AD = 19 cm

Answer 28.
Let AB be the tower of height h (units).
AP = a, QA = b.
As the angles of elevation are complementary, if ∠APB = θ° then ∠AQB = 90° – θ°

Let CD be a vertical tower of height h metres. From a point A on the ground, the angle of elevation of the top is 60°. B is another point 10 m vertically above A and angle of elevation of the top of the tower from B is 45°.
Let AC = d metres
From B, draw BE ⊥ CD.
Then BE = AC and EC = BA = 10 m.
From right angled ∆ADC, we get

Answer 29.
(i) Radius of the circle = 3.5 cm = \(\frac { 7 }{ 2 }\)
∴ Area of the quadrant OACB = \(\frac { 1 }{ 4 }\) x πr²

Answer 30.
Let the radius of the spherical dome be r metres and the total height of the building be h metres.
Since the base diameter of the dome is \(\frac { 2 }{ 3 }\) of the total height of the building, therefore,

Hence , the height of the building = 6 m

We hope the CBSE Sample Papers for Class 10 Maths Paper 1 help you. If you have any query regarding CBSE Sample Papers for Class 10 Maths Paper 1, drop a comment below and we will get back to you at the earliest.

CBSE Sample Papers for Class 10 Maths Paper 6 is part of CBSE Sample Papers for Class 10 Maths . Here we have given CBSE Sample Papers for Class 10 Maths Paper 6. According to new CBSE Exam Pattern, MCQ Questions for Class 10 Maths Carries 20 Marks.

CBSE Sample Papers for Class 10 Maths Paper 6

Board	CBSE
Class	X
Subject	Maths
Sample Paper Set	Paper 6
Category	CBSE Sample Papers

Students who are going to appear for CBSE Class 10 Examinations are advised to practice the CBSE sample papers given here which is designed as per the latest Syllabus and marking scheme as prescribed by the CBSE is given here. Paper 6 of Solved CBSE Sample Papers for Class 10 Maths is given below with free PDF download solutions.

Time: 3 Hours
Maximum Marks: 80

GENERAL INSTRUCTIONS:

• All questions are compulsory.
• This question paper consists of 30 questions divided into four sections A, B, C and D.
• Section A comprises of 6 questions of 1 mark each, Section B comprises of 6 questions of 2 marks each, Section C comprises of 10 questions of 3 marks each and Section D comprises of 8 questions 1 of 4 marks each.
• There is no overall choice. However, internal choice has been provided in one question of 2 marks, 1 three questions of 3 marks each and two questions of 4 marks each. You have to attempt only one of the alternatives in all such questions.
• In question of construction, drawings shall be neat and exactly as per the given measurements.
• Use of calculators is not permitted. However, you may ask for mathematical tables.

SECTION A

Question numbers 1 to 6 carry 1 mark each.

Question 1.
If \(\frac { p }{ q }\) is a rational number (q ≠ 0), what is the condition on q so that the decimal representation of \(\frac { p }{ q }\) is terminating?

Question 2.
Find the value of k so that the following system has no solution:
3x – y – 5 = 0; 6x – 2y – k = 0.

Question 3.
Find the 10th term of the AP √2, √8, √18 , … .

Question 4.
In the adjoining figure, if ∠ATO = 40°, find ∠AOB.

Question 5.
If cos A = \(\frac { 3 }{ 5 }\), find 9 cot² A – 1.

Question 6.
In the adjoining figure, ∠M = ∠N = 46°. Express x in terms of a, b and c, where a, b and c are lengths of LM, MN and NK respectively.

SECTION B

Question numbers 7 to 12 carry 2 marks each.

Question 7.
For any natural number n check whether 6ⁿ end with digit 0.

Question 8.
Simplify:

Question 9.
If A, B and C are the interior angles of a triangle ABC, show that:

Question 10.
The angles of a cyclic quadrilateral ABCD are ∠A = (6x + 30)°, ∠B = (5x)°, ∠C = (x + 10)° and ∠D = (3y – 10)°. Find x and y.

Question 11.
Find the middle term(s) of the following AP:
213, 205, 197, …, 37.

Question 12.
The centre of a circle is (2a, a – 7). Find the values of a if the circle passes through the point (11, -9) and has diameter 10 √2 units.

SECTION C

Question numbers 13 to 22 carry 3 marks each.

Question 13.
If the roots of the equation (a – b) x² + (b – c) x + (c – a) = 0 are equal, prove that 2a = b + c.

Question 14.
The sum of first 7 terms of an AP is 63 and sum of its next 7 terms is 161. Find 28th term of AP.
OR
Find the sum of all multiples of 8 lying between 201 and 950.

Question 15.
Show that the square of any positive integer cannot be of the form 5m + 2 or 5m + 3 for some integer m.

Question 16.
In the adjoining figure, ∆ABC is right angled at C and DE ⊥ AB. Prove that ∆ABC ~ ∆ADE, and hence find the lengths of AE and DE.

Question 17.
In a quadrilateral ABCD, ∠A + ∠D = 90°. Prove that AC² + BD² = AD² + BC².

Question 18.
The average score of boys in the examination of a school is 71 and that of the girls is 73. The average score of the boys and girls in the examination is 71.8. Find the ratio of number of boys to the number of girls who appeared in the examination.
OR
Given below is the distribution of weekly pocket money received by students of a class. Calculate the pocket money that is received by most of the students.

Question 19.
If sec θ+ tan θ = p, prove that sin θ = \(\frac { { p }^{ 2 }-1 }{ { p }^{ 2 }+1 } \)
OR
Evaluate the following:
sec 41° sin 49° + cos 49° cosec 41° – \(\frac { 2 }{ \sqrt { 3 } } \) tan 20° . tan 60° . tan 70° – 3(cos² 45° – sin² 90°)

Question 20.
In the adjoining figure, O is the centre of a circle such that diameter AB = 13 cm and AC = 12 cm. BC is joined. Find the area of the shaded region. (Take π = 3.14)

Question 21.
In the adjoining figure, find the area of the shaded region, . enclosed between two concentric circles of radii 7 cm and 14 cm, where ∠AOC = 40°.

OR
In the adjoining figure, APB and AQO are semicircles and OA = OB. If the perimeter of the figure is 40 cm, find the area of the shaded region.

Question 22.
Construct a triangle with sides 5 cm, 5.5 cm and 6.5 cm. Now construct another triangle whose sides are \(\frac { 3 }{ 5 }\) times the corresponding sides of the given triangle. 5

SECTION D

Question numbers 23 to 30 carry 4 marks each.

Question 23.
In the adjoining figure, the vertices of ∆ABC are A(4, 6), B(1, 5) and C(7, 2). A line segment DE is drawn to intersect the sides AB and AC at D and E respectively such that \(\frac { AD }{ AB } =\frac { AE }{ AC } =\frac { 1 }{ 3 } \). Calculate the area of ∆ADE and compare it with area of ∆ABC.

OR
Find the point on the x-axis which is equidistant from the points (5, 4) and (-2, 3). Also find the area of the triangle formed by these points.

Question 24.
If α and β are the zeroes of the polynomial p(x) = 2x² + 5x + k satisfying the relation α² + β² + αβ = \(\frac { 21 }{ 4 }\), then find the value of k. Also find the zeroes of the polynomial p(x).
OR
If the remainder on division of x³ + 2x² + kx + 3 by x – 3 is 21, find the quotient and the value of k. Hence, find the zeroes of the cubic polynomial x³ + 2x² + kx – 18.

Question 25.
A number x is selected from the numbers 1, 2, 3 and 4. Another number y is selected at random from the numbers 1, 4, 9 and 16. Find the probability that product of x and y is
(i) less than 16
(ii) not less than 16.

Question 26.
Due to heavy floods in a State, thousands were rendered homeless. 50 schools collectively offered to the State Government to provide place and canvas for 1500 tents to be fixed by the Government and decided to share the whole expenditure equally. The lower part of each tent is cylindrical of base radius 2.8 m and height 3.5 m, with conical upper part of same base radius but of height 2.1 m. If the canvas used to make the tents costs Rs 120 per sq. m, find the amount shared by each school to set up the tents.
What value is generated by the above problem?

Question 27.
The median of the following data is 525. Find the values of x and y, if the total frequency is 100.

Question 28.
A rectangular park is to be designed whose breadth is 3 m less than its length. Its area is 4 square metres more than the area of a park that has already been made in the shape of an isosceles triangle with its base as the breadth of the rectangular park and of altitude 12 m (shown in the adjoining figure). Find the dimensions of the rectangular park.

Question 29.
In the adjoining figure, two equal circles with centres O and O’, touch each other at X. OO’ produced meets the circle with O’ at A. AC is tangent to the circle with centre O, at the point C. O’D is perpendicular to AC. Find the value of \(\frac { DO’ }{ CO }\)

OR
Prove that the tangent drawn at the mid-point of an arc of a circle is parallel to the chord joining the end points of the arc.

Question 30.
A bird is sitting on the top of a 80 m high tree. From a point on the ground, the angle of elevation of the bird is 45°. The bird flies away horizontally in such a way that it remained at a constant height from the ground. After 2 seconds, the angle of elevation of the bird from the same point is 30°. Find the speed of flying of the bird. (Take √3 = 1.732).

Answers

Answer 1.
q is of the form 2^m x 5ⁿ, where m, n are non-negative integers, where p, q are coprime.

Answer 2.
Given: 3x – y – 5 = 0
6x – 2y – k – 0
For no solution, we have

Answer 3.
Given AP is √2, √8, √18 ,……..
i.e. √2, 2√2, 3√2,……
∴ a = √2, d = √2
T₁₀ = a + (10 – 1 )d
= √2 + 9√2 = 10√2 = √200
Hence, 10th term of given AP is √200 .

Answer 4.
Given ∠ATO = 40°
and ∠OAT = 90° (tangent is perpendicular to the radius)
∴ ∠AOT = 180° – (90° + 40°)
= 50°
∠AOB = 2∠AOT = 2 x 50° = 100°

Answer 5.
∵ cos A = \(\frac { 3 }{ 5 }\)

Answer 6.
In ∆KPN and ∆KLM,
∠N = ∠M = 46° (given)
∠K = ∠K (common)
∴ ∆KPN ~ ∆KLM

Answer 7.
6ⁿ can end with digit 0 only if 6ⁿ is divisible by 2 and 5 both. .
But prime factors of 6ⁿ = 2ⁿ x 3ⁿ, so 6ⁿ is not divisible by 5.
∴ By Fundamental Theorem of Arithmetic, there is no natural number n for which 6ⁿ ends with digit zero.
Hence, 6ⁿ does not end with digit zero

Answer 8.

Answer 9.
As A, B and C are the interior angles of ∆ABC, A + B + C = 180

Answer 10.
In cyclic quadrilateral ABCD,
∠A + ∠C = 180°
=> (6x + 30)° + (x + 10)° = 180°
=> 7x = 140 => x = 20 …(i)
and ∠B + ∠D = 180°
=> (5x)° + (3y – 10)° = 180°
=> 5x + 3y = 190
=> 5 x 20 + 3y = 190 => 3y = 90 => y = 30 (using (i))
x = 20, y = 30.

Answer 11.
Given AP is 213, 205, 197, … 37.
Here, a = 213, d = -8, l = 37
∵ l = a + (n – 1 )d
=> 37 = 213 + (n – 1) (-8) => n – 1 = \(\frac { -176 }{ -8 }\) => n – 1 = 22
=> n = 23
Total number of terms = 23

Answer 12.
Radius of circle = \(\frac { 1 }{ 2 }\) x 10√2 = 5√2 .
The centre of the circle is C(2a, a – 7) and it passes through the point P(11, -9), so CP = radius of circle

Answer 13.
The given equation is (a – b)x² + (b – c) x + (c – a) = 0.
Comparing it with Ax² + Bx + C = 0, we get
A = a – b, B = b – c, C = c – a.
Discriminant = B² – 4AC = (b – c)² – 4(a – b) (c – a).
For equal roots, discriminant = 0
=> (b – c)² – 4(a – b) (c – a) = 0
=> b² – 2bc + c² – 4(ca – a² – bc + ab) = 0
=> 4a² + b² + c² – 4ab – 4ca + 2bc = 0
=> (2a – b – c)² = 0
=>2a – b – c = 0
=> 2a = b + c.

Answer 14.
Let the first term and common difference of AP be a and d respectively.
Given, S₇ = 63 => \(\frac { 7 }{ 2 }\) [2a + (7 – 1 )d] = 63
=> 2a + 6d = 18 …(i)
and sum of next 7 terms = 161
i.e. S₁₄ – S₇ = 161
=> S₁₄ = 161 + 63 => S14 = 224
=> \(\frac { 14 }{ 2 }\) [2a + (14 – 1 )d] = 224
=> 2 a + 13d = 32 … (ii)
Subtracting (i) from (ii), we get
2a + 13d – 2a – 6d = 32 – 18 => 7d = 14 => d = 2.
Substituting d = 2 in equation (i), we get
2A + 6 x 2 = 18 => 2a – 6 => a = 3.
Now, T₂₈ = 3 + (28 – 1) x 2 = 3 + 54 = 57.
Hence, 28th term of AP is 57.
OR
The multiples of 8 lying between 201 and 950 are 208, 216, 224, …, 944.
These numbers form are AP with a = 208, d = 8 and l = 944.
Let the number of these numbers be n, then
944 = 208 + (n – 1) x 8 => 736 = 8 (n – 1)
=> 92 = n – 1 => n = 93.
Sum of these integers = \(\frac { 93 }{ 2 }\) (208 + 944) = \(\frac { 93 }{ 2 }\) x 1152
= 93 x 576 = 53568.

Answer 15.
Let n be any positive integer. Applying Euclid’s division lemma with divisor = 5, we get
n = 5q, 5q + 1, 5q + 2, 5q + 3 or 5q + 4, where q is some whole number.
Now (5q)² – 25q² = 5 m, where m = 5q², which is an integer;
(5q + 1)² = 25q² + 10q + 1 = 5(5q² + 2q) + 1 = 5m + 1, where
m = 5q² + 2q, which is an integer;
(5q + 2)² = 25q² + 20q + 4 = 5(5q² + 4q) + 4 = 5m + 4, where
m = 5q² + 4q, which is an integer;
(5q + 3)² = 25q² + 30q + 9 = 5(5q² + 6q + 1) + 4 = 5m + 4, where
m = 5q² + 6q + 1, which is an integer;
(5q + 4)² = 25q² + 40q + 16 = 5(5q² + 8q + 3) + 1 = 5m + 1, where
m = 5q² + 8q + 3, which is an integer.
Thus, the square of any positive integer is of the form 5m, 5m + 1 or 5m + 4 for some integer m.
It follows that the square of any positive integer cannot be of the form 5m + 2 or 5m + 3 for some integer m.

Answer 16.
In ∆ABC and ∆ADE
∠BAC = ∠EAD (same angle)
∠ACB = ∠AED (each 90°)
∴ ∆ABC ~ ∆ADE (AA Similarity criterion)

Answer 17.

Given, in quadrilateral ABCD,
∠A + ∠D = 90° …(i)
Produce AB and DC to meet at the point E.
In ∆AED, ∠A + ∠D + ∠E = 180°
=> 90° + ∠E = 180° (using (i))
=> ∠E = 90°.
In ∆AED, ∠AED = 90°
∴ AD² = AE² + ED² …(ii)
In ∆BEC, ∠BEC = 90°
∴ BC² – BE² + EC² …(iii)
In ∆AEC, ∠AEC = 90°
∴ AC² = AE² + EC² …(iv)
In ∆BED, ∠BED = 90°
∴ BD² = BE² + ED² …(v)
On adding (iv) and (v), we get
AC² + BD² = (AE² + ED²) + (BE² + EC²)
=> AC² + BD² = AD² + BC² (using (ii) and (iii))

Answer 18.
Let the number of boys who appeared in the examination be x and that of the girls be y, so the total number of students who appeared in the examination = x + y.
Since the average score is boys in the examination is 71 and that of the girls is 73, therefore, the sum of scores of boys = 71x and the sum of scores of girls = 73y.
∴ The total sum of scores of boys and girls = 71x + 73y.
The average score of the boys and girls

Hence, the pocket money received by most of the students is Rs 86.32

Answer 19.
Given sec θ + tan θ = p

Answer 20.
In ∆ABC, ∠ACB = 90°. By Pythagoras theorem,
AB² = AC² + BC²
=> 13² = 12² + BC² (given AB = 13 cm and AC = 12 cm)
=> BC² = 25 => BC = 5 cm

Answer 21.
Let R and r be the radii of the outer and inner concentric circles then R = 14 cm and r = 7 cm.
The central angle made by the major sector of the outer and inner circles θ = 360° – 40° = 320°.
∴ Area of shaded region = area of major sector OAC – area of major sector OBD

Answer 22.
Steps of construction:
1. Draw AB = 6.5 cm.
2. With A as centre and radius 5.5 cm draw an arc.
3. With B as centre and radius 5 cm draw an arc to meet the previous arc at C.
4. Join AC and BC, then ABC is a triangle with AB = 6.5 cm, AC = 5.5 cm and BC = 5 cm.
5. Draw any ray AX making an acute angle with AB on the side opposite to the vertex C.
6. Locate 5 points A1, A2, A3, A4 and A5 on AX such that AA1 = A1 A2 = A2A3 = A3A4 = A4A5.
7. Join A5B. Through A3 draw a line parallel to A5B to intersect AB at B’.
8. Through B’ draw a line parallel to BC to intersect the AC at C’. Then AB’C’ is the required triangle.

Answer 23.
Given the vertices of ∆ABC are A(4, 6), B(1, 5) and C(7, 2).

Answer 24.
Given α and β are the zeroes of the polynomial p(x) = 2x² + 5x + k.
∴ Sum of the zeroes = α + β = \(\frac { -5 }{ 2 }\) …(i)
and product of the zeroes = αβ = \(\frac { k }{ 2 }\) …(ii)

Answer 25.
For the product of outcomes, we construct a table as under:

Total number of outcomes are 4 x 4 i.e. 16 which are all equally likely.
(i) Table shows that the outcomes favourable to the event ‘product xy less than 16’ are (1, 1), (1, 4), (1, 9), (2, 1), (2, 4), (3, 1), (3, 4) and (4, 1) i.e. products as 1, 2, 3, 4, 4, 8, 9, 12 which are 8 in numbers.
∴ Required probability = \(\frac { 8 }{ 16 }\) = \(\frac { 1 }{ 2 }\)
(ii) P(Product of x and y is not less than 16)
= 1 – P(Product of x and y is less than 16).
= \(1-\frac { 1 }{ 2 }\) = \(\frac { 1 }{ 2 }\).

Answer 26.
Let r and h be the radius and height of the cylindrical
part and l be the slant height of conical part.
Given r = 2.8 m, l = 3.5 m, height of cone = 2.1 m

Answer 27.
Construct the cumulative frequency distribution table as under:

It is given n = 100, so 76 + x + y = 100 => x + y = 24 …(i)
As the median is 525, which lies in the class 500 – 600, so the median class is 500 – 600.
∴I = 500, f = 20, c.f. = 36 + x and h = 100

=> 5(14 – x) = 25 => 14 – x = 5 =>x = 9
∴ From (i), 9 + y = 24 => y = 15.
Hence, x = 9 and y = 15.

Answer 28.

Let the breadth of the rectangular park be x metres, then its length = (x + 3) metres.
∴ Area of rectangular park = (x + 3) x m²
Base of isosceles triangle = x metres and its height = 12 m.
∴ Area of isosceles triangle = \(\left( \frac { 1 }{ 2 } \times x\times 12 \right) \) m² = 6x m².
According to given, (x + 3) x = 6x + 4
=> x² + 3x = 6x + 4 => x² – 3x – 4 = 0
=> x² – 4x + x – 4 = 0 => x(x – 4) + 1 (x – 4) = 0
=> (x – 4) (x + 1) = 0 =>x – 4 = 0 or x + 1 = 0
=> x = 4 or x = -1.
Since x is the breadth of the rectangular park, it cannot be negative.
Hence, the breadth of the park is 4 metres and its length = (x + 3) metres
= (4 + 3) metres = 7 metres.

Answer 29.
As AC is tangent to the circle with centre O at the point C and OC is radius,
OC ⊥ AC => ∠ACO = 90°.

But these are corresponding angles, therefore, AB || PQ.
Hence, the tangent drawn at the mid-point of an arc of a circle is parallel to the chord joining the end points of the arc.

Answer 30.
Let AB be the tree and B be the position of bird.
From a point C on the ground the angle of elevation of bird is 45°. Bird flies away horizontally and reach the point D in 2 seconds. The angle of elevation from point to the bird is now 30°.
Let AC = x m and AE = y m.

We hope the CBSE Sample Papers for Class 10 Maths Paper 6 help you. If you have any query regarding CBSE Sample Papers for Class 10 Maths Paper 6, drop a comment below and we will get back to you at the earliest.

CBSE Sample Papers for Class 10 Maths Paper 7 is part of CBSE Sample Papers for Class 10 Maths . Here we have given CBSE Sample Papers for Class 10 Maths Paper 7. According to new CBSE Exam Pattern, MCQ Questions for Class 10 Maths Carries 20 Marks.

CBSE Sample Papers for Class 10 Maths Paper 7

Board	CBSE
Class	X
Subject	Maths
Sample Paper Set	Paper 7
Category	CBSE Sample Papers

Students who are going to appear for CBSE Class 10 Examinations are advised to practice the CBSE sample papers given here which is designed as per the latest Syllabus and marking scheme as prescribed by the CBSE is given here. Paper 7 of Solved CBSE Sample Papers for Class 10 Maths is given below with free PDF download solutions.

Time: 3 Hours
Maximum Marks: 80

GENERAL INSTRUCTIONS:

• All questions are compulsory.
• This question paper consists of 30 questions divided into four sections A, B, C and D.
• Section A comprises of 6 questions of 1 mark each, Section B comprises of 6 questions of 2 marks each, Section C comprises of 10 questions of 3 marks each and Section D comprises of 8 questions 1 of 4 marks each.
• There is no overall choice. However, internal choice has been provided in one question of 2 marks, 1 three questions of 3 marks each and two questions of 4 marks each. You have to attempt only one of the alternatives in all such questions.
• In question of construction, drawings shall be neat and exactly as per the given measurements.
• Use of calculators is not permitted. However, you may ask for mathematical tables.

SECTION A

Question numbers 1 to 6 carry 1 mark each.

Question 1.
If the sum of zeroes of the quadratic polynomial 3x² – kx + 6 is 3, then find the value of k.

Question 2.
If 1 is a root of both the equations ay² + ay + 3 = 0 and y² + y + b = 0, then find the value of ab.

Question 3.
Consider the following distribution, find the frequency of class 30-40.

Question 4.
Cards marked with number 3, 4, 5, ..50 are placed in a box and mixed thoroughly. A card is drawn at random from the box. Find the probability that the selected card bears a perfect square number.

Question 5.
In which quadrant the point P that divides the line segment joining the points A(2, -5) and B(5, 2) in the ratio 2 : 3 lies?

Question 6.
If sec 2A = cosec (A – 27°), where 2A is an acute angle, find the measure of ∠A.

SECTION B

Question numbers 7 to 12 carry 2 marks each.

Question 7.
Find whether the following pair of linear equations is consistent or inconsistent:
2x – 3y = 8; 4x – by = 9.

Question 8.
The x-coordinate of a point P is twice its y-coordinate. If P is equidistant from Q(2, -5) and R(-3, 6), find the coordinates of P.

Question 9.
In the adjoining figure, AP and BP are tangents to a circle with centre O, such that AP = 5 cm and ∠APB = 60°, find the length of chord AB.

Question 10.
How many terms of the AP 18, 16, 14, … be taken so that their sum is zero?

Question 11.
Show that the mode of the sequences obtained by combining the two sequences S1 and S2 given below is different from that of S1 and S2 taken separately:
S1 : 3, 5, 8, 8, 9, 12, 13, 9, 9
S2 : 7, 4, 7, 8, 7, 8, 13.

Question 12.
In the adjoining figure, ABC and DBC are two right triangles. Prove that AP x PC = BP x PD.

SECTION C

Question numbers 13 to 22 carry 3 marks each.

Question 13.
Solve the following pair of equations
49x + 51y = 499
51x + 49y = 501.
OR
Sum of the digits of a two digit number is 8 and the difference between the number and that formed by reversing the digits is 18. Find the number.

Question 14.
Divide 56 in four parts in AP such that the ratio of the product of their extremes (1st and 4th) to the product of means (2nd and 3rd) is 5 : 6.

Question 15.
D and E are points on the sides AB and AC respectively of ∆ ABC such that DE is parallel to BC, and AD : DB = 4:5. CD and BE intersect each other at F. Find the ratio of the areas of ∆DEF and ∆CBF.

Question 16.
Find the area of ∆PQR with Q(3, 2) and the mid-points of the sides through Q being (2,-1) and (1, 2).
OR
The two opposite vertices of a square are (-1, 2) and (3, 2). Find the coordinates of the other two vertices.

Question 17.
Prove the following identity:

Question 18.
Three alarm clocks ring at intervals of 4, 12 and 20 minutes respectively. If they start ringing together, after how much time will they next ring together?

Question 19.
Prove that 3 + 2√3 is an irrational number.

Question 20.
In the adjoining figure, ABCD is a square of side 14 cm. Semicircles are drawn with side of square as diameter. Find the area of the shaded region.

Question 21.
In the adjoining figure shows two arcs PAQ and PBQ. Arc PAQ is a part of a circle with centre O and radius OP while arc PBQ is a semicircle drawn on PQ as diameter. If OP = PQ = 10 cm, show that the area of the shaded region is 25 (√3 – \(\frac { \pi }{ 6 } \))cm².

OR
Sides of a triangular field are 15 m, 16 m, 17 m. With the three comers of the field a cow, a buffalo and a horse are tied separately with ropes of length 7 m each to graze in the field. Find the area of the field which cannot be grazed by the three animals.

Question 22.
Two different dice are tossed together. Find the probability that the product of the two numbers on the top of dice is
(i) 6
(ii) a perfect square number.
OR
A carton consists of 100 shirts of which 88 are good, 8 have minor defects and 4 have major defects. Ramesh, a trader, will only accept the shirts which are good, but Kewal, another trader, will only reject the shirts which have major defects. One shirt is drawn at random from the carton. What is the probability that
(i) it is acceptable to Ramesh?
(ii) it is acceptable to Kewal?

SECTION D

Question numbers 23 to 30 carry 4 marks each.

Question 23.
If α and β are the zeroes of the polynomial p(x) = 3x² + 2x + 1, find the polynomial whose zeroes are

Question 24.
If sec θ – tan θ = x, show that sec θ + tan θ = \(\frac { 1 }{ x }\) and hence, find the values of cos θ and sin θ.
OR
If tan (A + B) = √3 , tan (A – B) = \(\frac { 1 }{ \sqrt { 3 } } \), 0° < A + B < 90°, A > B, find A and B. Also calculate tan A sin (A + B) + cos A tan (A – B).

Question 25.
From the top of a tower h metres high, the angles of depression of two objects, which are in line with the foot of tower are α and β (β>α). Find the distance between the two objects.

Question 26.
Sushant has a vessel of the form of an inverted cone, open at the top, of height 11 cm and radius of top as 2.5 cm and is full of water. Metallic spherical balls each of diameter 0.5 cm are put in the vessel due to which \(\frac { 2 }{ 5 }\) th of the water in the vessel flows out. Find how many balls were put in the vessel. Sushant made the arrangement so that the water that flows out irrigates the flower beds.
What value has been shown by Sushant?

Question 27.
A peacock is sitting on the top of a pillar, which is 9 m high. From a point 27 m away from the bottom of a pillar, a snake is coming to its hole at the base of a pillar, seeing the snake, the peacock pounces on it. If their speeds are equal, at what distance from the hole is the snake caught?
OR
Find the value(s) of p for which the quadratic equation
(2p + 1) x² – (7p + 2) x + (7p – 3) = 0 has equal roots. Also find these roots.

Question 28.
Draw two concentric circles of radii 3 cm and 5 cm. Taking a point on the outer circle, construct the pair of tangents to the other. Measure the length of a tangent and verify it by actual calculations.

Question 29.
In the adjoining figure, tangents PQ and PR are drawn from an external point P to a circle with centre O, such that ∠RPQ = 30°. A chord RS is drawn parallel to the tangent PQ. Find ∠RQS.

OR
In the adjoining figure, O is the centre of the circle. Determine ∠ACB, if PA and PB are tangents and ∠APB = 50°.

Question 30.
The following table gives the daily income of 50 workers of a factory. Draw both types (less than type and greater than type) ogives. Hence, obtain the median income.

Answers

Answer 1.
Given sum of zeroes of the polynomial 3x² – kx + 6 is 3
⇒ 3 = \(\frac { -(-k) }{ 3 }\) ⇒ k =9

Answer 2.
Given 1 is a root of both the equations => ay² + ay + 3 = 0 and y² + y + b =0
=> a.1² + a.1 + 3 = 0 and 1² + 1 + b = 0
=> a + a + 3 = 0 and 1 + 1 + b = 0
=> 2a + 3 = 0 and 2 + b = 0

Hence the values of ab is 3

Answer 3.

Hence, the frequency of class 30-40 is 3

Answer 4.
Total number of cards = 48
Perfect square number cards from 3 to 50 are 4, 9, 16, 25, 36 and 49. These are 6 in number.
∴ P(Perfect square number) = \(\frac { 6 }{ 48 }\) = \(\frac { 1 }{ 8 }\)

Answer 5.

Answer 6.
sec 2A = cosec (A – 27°) => cosec (90° – 2A) = cosec(A – 27°)
=> 90° – 2A = A – 27° => 3A = 117°
=> A = \(\frac { { 117 }^{ o } }{ 3 } \) => A = 39°
Hence, ∠A = 39°.

Answer 7.
The given pair of linear equations can be written as 2x – 3y – 8 = 0 and 4x – 6y – 9 = 0.

Answer 8.
Given the coordinates of Q(2, -5) and R(-3, 6).
Let the coordinates of P be (2y, y).
According to given, PQ = PR
=> (PQ)² = (PR)²
=> (2 – 2y)² + (- 5 – y)² = (- 3 – 2y)² + (6 – y)²
=> 4 – 8y + 4y² + 25 + 10y + y² – 9 + 12y + 4y² + 36 – 12y + y²
=> 2y + 29 = 45 => 2y = 16 => y = 8
Hence, coordinates of P are (16, 8).

Answer 9.
Given ∠APB = 60° and AP = 5 cm
In ∆APB, PA = PB (tangents drawn from an external point are equal)
∴∠PAB = ∠PBA (angles opposite to equal sides are equal)
Now, ∠PAB + ∠PBA + ∠APB = 180° (angle sum property of a triangle)
=> ∠PAB + ∠PAB + 60° = 180°
=> 2∠PAB = 120° => ∠PAB = 60°
=> ∠PAB = ∠PBA = ∠APB = 60°
∴∆APB is an equilateral triangle.
So, AB = AP = 5 cm.

Answer 10.
Given AP is 18, 16, 14, …
Here, a – 18, d = -2
If the sum of n terms of the given AP is zero, their S_n = 0

But n cannot be zero.
Hence, the required number of terms is 19.

Answer 11.
Given S1 : 3, 5, 8, 8, 9, 12, 13, 9, 9
S2 : 7, 4, 7, 8, 7, 8, 13
In S1 : Number 9 occurs maximum number of times i.e. 3 times
Hence, the mode of sequence S1 = 9
In S2 : Number 7 occurs maximum number of times i.e. 3 times
Hence, the mode of sequence S2 = 7
After combining sequences S1 and S2, we have
3, 4, 5, 7, 7, 7, 8, 8, 8, 8, 9, 9, 9, 12, 13, 13
Here number 8 occurs maximum number of times i.e. 4 times.
Hence, the mode of combined sequence = 8
Hence, mode of S1 and S2 taken combined is different from that of S1 and S2 taken separately.

Answer 12.
In ∆APB and ∆DPC,
∠BAP = ∠CDP (each 90°)
∠APB = ∠DPC (vert. opp. ∠s)
∴∆APB ~ ∆DPC (by AA similarity criterion)

Answer 13.
Given 49x + 51 y = 499
and 51x + 49y = 501
Adding (i) and (ii), we get
100x + 100y – 1000
=> x + y = 10
Subtracting (ii) from (i), we get
-2x + 2y = -2
=> -x + y = -1
Now, adding (iii) and (iv), we get
2y = 9 => y = \(\frac { 9 }{ 2 }\)
Putting y = \(\frac { 9 }{ 2 }\) in (iii), we get

OR
Let the two digit number be 10x + y, then
according to given, x + y = 8
and | 10x + y – (10y + x) | = 18
=> |9x – 9y| = 18 => |x – y| = 2 =>x – y = ±2
When x + y = 8 and x – y = 2, then
on adding these equations, we get 2x = 10 => x = 5.
Putting x = 5 in x + y = 8, we get y = 3
∴ The original number is 10 x 5 + 3 i.e. 53
When x + y = 8 and x – y = -2, then
on adding these equations, we get 2x – 6 => x = 3
Putting x = 3 in x + y = 8, we get y = 5
∴ The original number is 10 x 3 + 5 i.e. 35
Hence, the original number is 53 or 35.

Answer 14.
Let the four numbers in AP be
a – 3d, a – d, a + d and a + 3d, then
according to given,
a – 3d + a – d + a + d + a + 3d = 56
4a = 56 => a = 14

Answer 15.
Given AD : DB = 4 : 5

Answer 16.
Let D and E are the mid-points of the sides through Q.
Let the coordinates of P and R be (x, y) and (u, v) respectively
∵D is the mid-point of QP

Answer 17.

Answer 18.
To find the time when the clocks will next ring together, we have to find the LCM of 4, 12 and 20.
Prime factorisation 4, 12 and 20
4 = 2 x 2
12 = 2 x 2 x 3
20 = 2 x 2 x 5
∴ LCM of 4, 12 and 20 = 2 x 2 x 3 x 5 = 60
Hence, the alarm clocks will ring together again after 60 minutes i.e. one hour.

Answer 19.
Let us assume that 3 + 2√3 is a rational number, say r.

Answer 20.

Radius of each semicircle = \(\frac { 14 }{ 2 }\) cm = 7 cm
Mark the shaded regions I, II, III and IV (as shown in adjoining figure)
Area of region I + area of region III
= area of square ABCD – area of two semicircles each of radius 7 cm = (14 x 14 – 2.\(\frac { 1 }{ 2 }\) π x 72) cm²
= (196 – \(\frac { 22 }{ 7 }\) x 49) cm²
= (196 – 154) cm² = 42 cm²
Similarly, area of region II + area of region IV = 42 cm²
Required area = area of shaded regions I, II, III and IV
= (2 x 42) cm² = 84 cm²

Answer 21.
OQ = OP (radii of same circle)
Given OP = PQ = 10 cm => OP = OQ = PQ = 10 cm.
Thus, OPQ is an equilateral triangle of side 10 cm.
Also ∠POQ = 60° (angle of an equilateral triangle)
Area of shaded region = area of a semicircle with PQ as diameter i.e. radius 5 cm + area of equilateral AOPQ of side 10 cm – area of sector of a circle of radius 10 cm and central angle 60°

The region of the field left ungrazed by the three animals is shown shaded. Sum of areas of three sectors of radius 7 m (each) and having central angles as ∠A, ∠B and ∠C
= area of a sector of radius 7 m and having central angle as sum of ∠A, ∠B and ∠C
= area of a sector of radius 7 m and having central angle as ∠A + ∠B + ∠C i.e. an angle of 180°

Answer 22.
When two different dice are tossed together, the total number of outcomes is 36 and all the outcomes are equally likely.
(i) The outcomes favourable to the event ‘the product of two numbers on the top of two dice is 6’ are (1, 6), (6, 1), (2, 3) and (3, 2). These are 4 in number.
∴ P (product of two numbers is 6) = \(\frac { 4 }{ 36 }\) = \(\frac { 1 }{ 9 }\).
(ii) The outcomes favourable to the event ‘the product of two numbers on the top of dice is a perfect square number’ are (1, 1), (2, 2), (3, 3), (4, 4), (5, 5), (6, 6), (1, 4), (4, 1).
These are 8 in number.
∴ P (product of two numbers is a perfect square number) = \(\frac { 8 }{ 36 }\) = \(\frac { 2 }{ 9 }\).
OR
One shirt is drawn at random from a carton containing 100 shirts means all shirts are equally likely to be drawn. So, the sample space of the experiment has 100 equally likely outcomes.
(i) Since Ramesh accepts only good shirts and there are 88 good shirts in the carton. Therefore, the number of favourable (i.e. acceptable) outcomes to Ramesh = 88.
∴ P (acceptable to Ramesh) = \(\frac { 88 }{ 100 }\) = 0.88
(ii) As Kewal rejects the shirts which have major defects and the number of such shirts is 4, so the number of shirts acceptable to Kewal = 100 – 4 = 96.
Therefore, the number of favourable (acceptable) outcomes to Kewal = 96.
∴ P (acceptable to Kewal) = \(\frac { 96 }{ 100 }\) = 0.96

Answer 23.
Given a and (3 are the zeroes of the polynomial p(x) = 3x² + 2x + 1

Answer 24.
Given sec θ – tan θ = x
∵ sec² θ – tan² θ = 1
=> (sec θ tan θ) (sec θ + tan θ) = 1
x . (sec θ + tan θ) = 1
=> sec θ + tan θ = \(\frac { 1 }{ x }\) …(ii)
Adding (i) and (ii), we get
sec θ – tan θ + sec θ + tan θ = x + \(\frac { 1 }{ x }\)

Answer 25.
Let P be the top of the tower MP of height h metres, and A, B be the two objects. Let BM = x metres and AB = d metres. The angles of depression of the two objects are shown in the adjoining figure, then
∠MAP = α and ∠MBP = β.
From right angled ∆MBP, we get

Answer 26.
Volume of water in the cone
= Volume of cone of height 11 cm and radius 2.5 cm

Hence, the number of metallic balls put into the vessel = 440
Sushant’s love for plants, nature and environment has been depicted.

Answer 27.

Let CD be the pillar and initially the snake be at the point A and the peacock is sitting at the top of pillar i.e. at the point D. Then CD = 9 m and AC = 27 m.
Let the snake be caught at the point B. As the snake and peacock have equal speeds, they cover equal distance in same time.
Let AB = x metres, then BD = AB = x metres and BC = AC – AB = (27 – x) metres.
In ∆BCD, ∠C = 90°.
By Pythagoras theorem, we get
BD² = BC² + CD²
=> x² = (27 – x)² + 9² => x² = 729 – 54x + x² + 81
=> 54x = 810 => x = 15.
∴ BC = (27 – x) metres = (27 – 15) metres = 12 metres.
Hence, the snake is caught at a distance of 12 metres from its hole.
OR
The given equation is (2p + 1) x² – (7p + 2) x + (7p – 3) = 0 …(i)
Comparing it with ax² + bx + c = 0, we get
a = 2p + 1, b = – (7p + 2), c = 7p – 3.
Discriminant = b² – 4ac = (-(7p + 2))² – 4 (2p + 1) (7p – 3)
= 49p² + 28p + 4 – 4 (14p² – 6p + 7p – 3)
= 49p² + 28p + 4 – 56p² – 4p + 12
= -7p² + 24p + 16.
For equal roots, discriminant = 0
=> -7p² + 24p + 16 = 0 => 7p² – 24p – 16 = 0
=> 7p² – 28p + 4p – 16 = 0 => 7p (p – 4) + 4 (p – 4) = 0
=> (p – 4) (7p + 4) = 0 => p – 4 = 0 or 7p + 4 = 0

Answer 28.
Steps of construction:
1. Draw two concentric circles of radii 3 cm and 5 cm with point O as their centre.
2. Let P be a point on the outer circle. Join OP and draw its perpendicular bisector to meet OP at M.
3. Taking M as centre and OM (or MP) as radius, draw a circle. Let this circle intersect the smaller circle i.e. circle of radius 3 cm at points A and B.
4. Join PA and PB. Then PA and PB are the required tangents on measuring PA (or PB), we find that PA = 4 cm.

Calculation of length PA:
Join OA.
In ∆OAP, ∠OAP = 90° (angle in a semicircle)
By Pythagoras theorem, we get
PA² = OP² – OA² = 5² – 3² = 25 – 9 = 16
=> PA = 4 cm.

Answer 29.

Join QO and produce it to meet SR at M.
In ∆PRQ, PR = PQ (lengths of tangents)
=> ∠PRQ = ∠PQR …(i)
∠PQR + ∠PRQ + ∠RPQ = 180°
=> ∠PQR + ∠PQR + 30° = 180° (using (i))
=> 2∠PQR = 150° => ∠PQR = 75°.
As RS || PQ and RQ is transversal,
∠SRQ = ∠PQR
=> ∠SRQ = 75°
Since PQ is tangent to the circle with centre O at the point Q and OQ is radius, OQ ⊥ PQ.
Also RS || PQ (given), so QM ⊥ RS i.e. OM ⊥ RS
=> MR = MS (∵ perpendicular from centre to a chord bisects it)
In ∆QRM and ∆QSM,
MR = MS (proved above)
∠QMR = ∠QMS (each = 90°, as QM ⊥ RS)
QM = QM
∴∆QRM ≅ ∆QSM
∴ ∠MSQ = ∠MRQ (c.p.c.t.)
=> ∠RSQ = ∠SRQ.
In ∆QRS, ∠RQS + ∠SRQ + ∠RSQ = 180° => ∠RSQ + ∠SRQ + ∠SRQ = 180°
=> ∠RQS + 2 x 75° = 180° => ∠RQS = 30°.
OR

As PA is tangent to the circle at A and OA is radius, OA ⊥ AP
i.e. ∠OAP = 90°
Similarly, ∠OBP = 90°
In quadrilateral OAPB,
∠AOB + ∠OAP + ∠APB + ∠OBP = 360°
=> ∠AOB + 90° + 50° + 90° = 360°
=> ∠AOB = 130°
Reflex ∠AOB = 360° – 130° = 230°
∠ACB = \(\frac { 1 }{ 2 }\) of reflex ∠AOB
(∵ angle at the centre = double the angle at the remaining part of circle)
=> ∠ACB = \(\frac { 1 }{ 2 }\) x 230° = 115°

Answer 30.
For more than ogive:

Take 1 cm along x-axis = Rs 20 and 1 cm along y-axis = 10 workers.
Plot the points (100, 50), (120, 38), (140, 24), (160, 16), (180, 10) and (200, 0).
Join these points by a free-hand drawing. More than type ogive is drawn on the graph sheet. For less than type ogive:

Choose the same scale (as above). Plot the points (100, 0), (120, 12), (140, 26), (160, 34), (180, 40) and (200, 50). Join these points by a free hand drawing. Less than type ogive is drawn on the same graph sheet (with same axes).

The abscissa of point of intersection of more than ogive and less than ogive represents 138 Hence, the median daily income = Rs 138.

We hope the CBSE Sample Papers for Class 10 Maths Paper 7 help you. If you have any query regarding CBSE Sample Papers for Class 10 Maths Paper 7, drop a comment below and we will get back to you at the earliest.