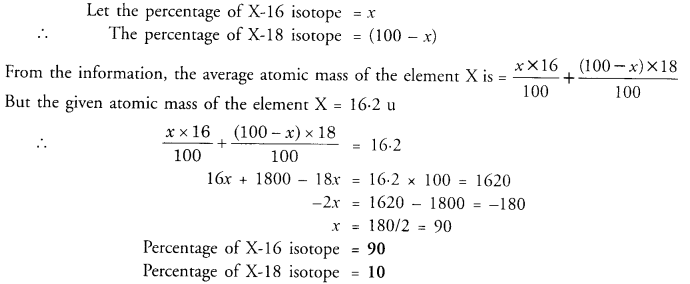Category: CBSE Class 9

Value Based Questions in Science for Class 9 Chapter 2 Is Matter Around Us Pure

These Solutions are part of Value Based Questions in Science for Class 9. Here we have given Value Based Questions in Science for Class 9 Chapter 2 Is Matter Around Us Pure

Question 1.
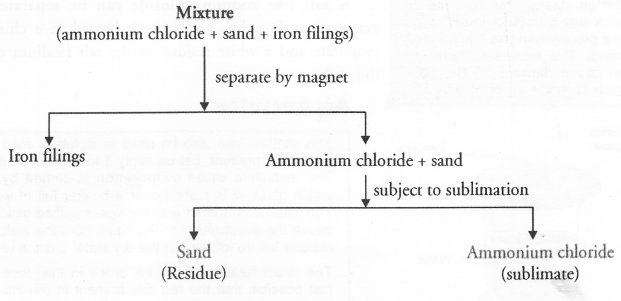
A student was asked by his teacher to separate an impure sample of sulphur containing sand as the impurity. He tried to purify it with the help of sublimation. But he was not successful. Particles of sulphur could not be separated completely from sand.

1. Why did not the sublimation process succeed ?
2. Suggest an alternate method to affect the separation.
3. What is the value based information associated with this ?

Answer:

1. Sulphur is not of volatile nature. Upon heating, it melts. Therefore, sublimation process could not succeed.
2. The student should have dissolved the impure sample in carbon disulphide. It is a liquid in which sulphur completely dissolves while iron does not. From the solution, sulphur can be recovered with the help of crystallization process.
3. The process of sublimation is useful only if one of the constituents present in the mixture can sublime while the others do not undergo sublimation.

More Resources

• Value Based Questions in Science for Class 9
• HOTS Questions for Class 9 Science
• NCERT Solutions for Class 9 Science
• NCERT Exemplar Solutions for Class 9 Science
• Previous Year Question Papers for CBSE Class 9 Science

Question 2:
Amit was asked by his teacher to separate a liquid mixture of acetone and ethyl alcohol. He set up a distillation apparatus and tried to distil the mixture. To his surprise, both the liquids got distilled. Teacher told Amit to repeat the experiment by using a fractionating column in the distillation flask. Amit followed the advice of the teacher and he was able to separate the two liquids.

1. Why was Amit not successful in separating the liquid mixture earlier ?
2. Why did teacher ask him to use the fractionating column ?
3. Which liquid was distilled first ?
4. As a student of chemistry, what value based information you have gathered ?

Answer:

1. The difference in boiling point temperatures of acetone (56°C) and ethyl alcohol (78°C) is only 22°C. Therefore, process of simple distillation fails in this case.
2. Fractionating column is quite effective in this case because it obstructs the distillation of ethyl alcohol which is high boiling and at the same time helps in the distillation of acetone which is low boiling.
3. Acetone was distilled first since it has comparatively low boiling point.
4. The process of simple distillation can be used only in case, the liquids present in the mixture differ in their boiling point by 25°C or more.

Question 3.
A housewife got a cut on her finger while working in the Kitchen. She tried to stop the bleeding by applying dettol on it but it was not effective. By chance, her friend was also there. She asked her to rub alum on the cut which she did. The bleeding immediately stopped.

1. Why was not dettol effective in stopping bleeding ?
2. Why was alum effective ?
3. What valuable service was done by the friend to the housewife ?

Answer:

1. Blood is a colloidal solution and the colloidal particles of RBCs carry charge. Dettol is an organic compound. It could not neutralise the charge on the colloidal particles. Therefore, bleeding did not stop.
2. Alum is a salt in which charged ions are present. When alum was applied on the cut, the charged ions neutralised the charge on the colloidal particles. In the absence of charge, blood became thick and bleeding stopped. In otherwords, blood got coagulated.
3. The friend has a proper knowledge of colloidal solutions. She must be a student of chemistry. Timely help by her stopped the bleeding. Otherwise, there would have been a further loss of blood.

Question 4.
Mallika’s mother was suffering from cold and cough. Mallika prepared tea for her mother. She boiled water in a pan, then she added tea leaves, sugar and milk to it. She filtered the tea in a cup and served to her mother.

1. Explain the values shown by Mallika.
2. Identify solute, solvent, residue and filtrate in this activity. (CBSE 2013)

Answer:

1. Mallika used the knowledge of chemistry to provide relief to her mother. Actually she prepared an extract of tea leaves which is helpful in curing cold and cough and gives warmth to the body.
2. Solute : tea leaves and sugar ; Solvent : water and milk; Filtrate : homogeneous mixture of water, milk, sugar and extract of tea leaves.

Question 5.
Nikhil’s father was suffering from high blood pressure and cardiac problems. Doctor suggested him to take low fat milk. Nikhil churned the milk and separated the butter (fat) from it and then he served that milk to his father. Answer the following questions based on above information :

1. Name the technique by which Nikhil separated butter from milk.
2. Write any other application of that technique.
3. Which values are reflected in Nikhil’s behaviour ?

Answer:

1. It is called centrifugation carried in a centrifugal machine.
2. Washing machines that are used for washing dirty clothes are centrifugal machines.
3. Nikhil had the proper knowledge of chemistry. He used this to separate fat from milk with the help of the centrifugal machine. In this way, he rendered proper service to his father.

Hope given Value Based Questions in Science for Class 9 Chapter 2 Is Matter Around Us Pure are helpful to complete your science homework.

If you have any doubts, please comment below. Learn Insta try to provide online science tutoring for you.

NCERT Exemplar Solutions for Class 9 Science Chapter 2 Is Matter Around Us Pure

These Solutions are part of NCERT Exemplar Solutions for Class 9 Science . Here we have given NCERT Exemplar Solutions for Class 9 Science Chapter 2 Is Matter Around Us Pure.

Question 1.
Which of the following statements are true for pure substances ?
(i) Pure substances contain only one kind of particles
(ii) Pure substances may be compounds or mixtures
(iii) Pure substances have the same composition throughout
(iv) Pure substances can be exemplified by all elements other than nickel.
(a) (i) and (ii)            (b) (i) and (iii)
(c) (iii) and (iv)          (d) (ii) and (iii).
Correct Answer:
(b) Both these statements are correct.

More Resources

• NCERT Exemplar Solutions for Class 9 Science
• NCERT Solutions for Class 9 Science
• Value Based Questions in Science for Class 9
• HOTS Questions for Class 9 Science
• Previous Year Question Papers for CBSE Class 9 Science

Question 2.
Rusting of an article made up of iron is called
(a) corrosion and it is a physical as well as chemical change
(b) dissolution and it is a physical change
(c) corrosion and it is a chemical change {d) dissolution and it is a chemical change.
Correct Answer:
(c) corrosion and it is a chemical change.

Question 3.
A mixture of sulphur and carbon disulphide is
(a) heterogeneous and shows Tyndall effect
(b) homogeneous and shows Tyndall effect
(c) heterogeneous and does not show Tyndall effect
(d) homogeneous and does not show Tyndall effect.
Correct Answer:
(d) Sulphur dissolves in carbon disulphide to form a solution which is homogeneous in nature. It does not show any Tyndall effect.

Question 4.
Tincture of iodine has antiseptic properties. This solution is made by dissolving
(a) iodine in benzene
(b) iodine in ether
(c) iodine in water
(d) iodine in alcohol.
Correct Answer:
(d) Tincture of iodine is a dilute solution of iodine formed by dissolving iodine in ethyl alcohol also called alcohol.

Question 5.
Which of the following are homogeneous mixtures in nature ?
(i) ice
(ii) wood
(iii) soil
(iv) air.
(a) (i) and (iii)        (b) (ii) and (iv)
(c) (i) and (iv)        (d) (iii) and (iv).
Correct Answer:
(c) Both ice and air are homogeneous mixtures.

Question 6.
Which of the following are physical changes ?
(i) Melting of iron metal
(ii) Rusting of iron
(iii) Bending of an iron rod
(iv) Drawing a wire of iron metal
(a) (i), (ii) and (iii)          (b) (i), (ii) and (iv)
(c) (i), (iii) and (iv)         (d) (ii), (iii) and (iv).
Correct Answer:
(c) All the three are examples of physical changes.

Question 7.
Which of the following are chemical changes ?
(i) Decaying of wood
(ii) Burning of wood
(iii) Growth of wood in a tree
(iv) Hammering of a nail into a piece of wood.
(a) (i) and (ii)             (b) (ii) and (iv)
(c) (iii) and (iv)           (d) (i), (ii) (iiii).
Correct Answer:
(d) All the three are chemical changes.

Question 8.
Two substances, A and B were made to react to form a third substance, A₂B according to the following reaction
2A + B —> A₂B
Which of the following statements concerning this
reaction are incorrect ?
(i) The product A₂B shows the properties of substances A and B
(ii) The product will always have a fixed composition
(iii) The product so formed cannot be classified as a compound
{iv) The product so formed is an element.
(a) (i), (ii) and (iii)           (b) (ii), (iii) and (iv)
(c) (i), (iii) and (iv)          (d) (ii), (iii) and (iv).
Correct Answer:
(c) Except for (ii) all other statements are incorrect.

Question 9.
Two chemical species X and Y combine together to form a product P which contains both X and Y
X + Y —> P
X and Y cannot be broken down into simpler substances by simple chemical reactions. Which of the following concerning the species X, Y and P are correct ?
(i) P is a compound
(ii) X and Y are compounds
(iii) X and Y are elements
(iv) P has a fixed composition
(a) (i), (ii) and {iii)               (b) (i), (ii) and (iv)
(c) (ii), (iii) and (iv)              (d) (i), (iii) and (iv).
Correct Answer:
(d) All these statements are correct.

Short Answer Questions

Question 10.
Suggest separation technique(s) one would need to employ to separate the following mixtures.

1. Mercury and water
2. Potassium chloride and ammonium chloride
3. Common salt, water and sand
4. Kerosene oil, water and salt.

Answer:

1. Separation can be done with a separating funnel. Mercury being heavy forms lower layer.
2. Sublimation can be used. Ammonium chloide sublimes.
3. Filtration followed by crystallisation techniques can be used here. Sand can be removed as an insoluble residue upon filtration. Upon evaporating the filtrate in a china dish to about one third followed by slow colling crystals of common salt (NaCl) are formed.
4. Kerosene oil can be separated with a separating funnel. Water and salt can be separated either by distillation (water distils) or by evaporation (water evaporates). Salt is left behind as residue.

Question 11.
Which of the tubes {a) and {b) will be more effective as a condenser in the distillation apparatus ?

Answer:
Condenser
(a) has a number of bends or obstructions. It will provide more opportunity to vapours to condense and act as a better condenser than
(b) which has no such obstructions.

Question 12.
Salt can be recovered from its solution by evaporation. Suggest some other technique for the same ?
Answer:
Crystallisation technique can also be used. For example, both these can be used to separate salts like sodium chloride (common salt) and potasium nitrate (nitre) from their aqueous solutions.

Question 13.
Sea-water can be classified as homogeneous as well as heterogeneous mixture. Comment.
Answer:
The sample of sea water from mid stream is transparent and has certain salts dissolved in water. It is therefore, homogeneous., However, the sample collected from near the seashore contains mud and many suspended particles. It is therefore, heterogeneous in nature.

Question 14.
While diluting a solution of salt in water, a student by mistake added acetone (boiling point 56°C). What technique can be employed to get back acetone ? Justify your choice.
Answer:
Acetone can be recovered by carrying out the distillation in a distillation flask. Its vapours will rise since it is volatile, get condensed and collected in a receiver. Salt being non-volatile in nature remains in the flask.

Question 15.
What would you observe when
(a) a saturated solution of potassium chloride prepared at 60°C is allowed to cool to room temperature.
(b) an aqueous sugar solution is heated to dryness
(c) a mixture of iron filings and sulphur powder is heated strongly.
Answer:
(a) Upon cooling, crystals of potassium chloride would separate.
(b) Initially, entire water slowly gets removed. Upon further heating, sugar gets charred and burning smell will be noticed.
(c) Iron sulphide is formed as a greyish black residue.

Question 16.
Explain why particles of a colloidal solution do not settle down when left undisturbed while in the case of a suspension, they do.
Answer:
In a colloidal solution, the particle size is smaller as compared to particle size in a suspension. The effect of gravity on these particles is less than in a suspension. Therefore, the particles in a suspension settle under the influence of gravity while they remain dispersed in a colloidal solution.

Question 17.
Smoke and fog both are aerosols. In what way are they different ?
Answer:
Air is the dispersion medium in both the cases. However, dispersed phases are not the same. In smoke, carbon particles act as the dispersed phase while water drops represent the dispersed phase in fog.

Question 18.
Classify the following as physical or chemical properties (CBSE 2012)

1. The composition of a sample of steel is : 98% iron. 1-5% carbon and 0-5% other elements.
2. Zinc dissolves in hydrochloric acid with the evolution of hydrogen gas.
3. Metallic sodium is soft enough to be cut with a knife.
4. Most metal oxides form alkalis on interacting with water.

Answer:

1. Physical
2. Chemical
3. Physical
4. Chemical.

Question 19.
The teacher instructed three students A’, ‘B’ and ‘C’ respectively to prepare a 50% (mass by volume) solution of sodium hydroxide (NaOH). ‘A’ dissolved 50 g of NaOH in 100 mL of water. ‘B’ dissolved 50 g of NaOH in 100 g of water while ‘C’ dissolved 50 g of NaOH in water to make 100 mL of solution. Which one of them has made the desired solution and why ?
Answer:
The student ‘C’ has prepared the desired solution since it represents mass/volume solution. The student A’ has taken into account volume of the solvent while the student ‘B’ has considered the mass of the solution

Question 20.
Name the process associated with the following :

1. Dry ice is kept at room temperature and under one atmospheric pressure.
2. A drop of ink placed on the surface of water contained in a glass spreads throughout the water.
3. A potassium permanganate crystal is in a beaker and water is poured into the beaker with stirring.
4. An acetone bottle is left open and the bottle, becomes empty.
5. Milk is churned to separate cream from it. .
6. Settling of sand when a mixture of sand and water is left undisturbed for some time.
7. Fine beam of light entering through a small hole in a dark room, illuminates the particles in its paths.

Answer:

1. Sublimation
2. Diffusion/Sedimentation
3. Dissolution/Dififusion
4. Evaporation/Diffusion
5. Centrifugation
6. Sedimentation
7. Scattering of light (Tyndall effect).

Question 21.
You are given two samples of water labelled as A and ‘B’. Sample A boils at 100°C and sample ‘B’ boils at 102°C. Which sample of water will not freeze at 0°C ? Comment.
Answer:
The standard boiling point temperature of water is 100°C. Therefore, the sample v/ith boiling point 100°C represents the pure form of water. The sample with boiling point 102° C is impure. Please note that impurities always raise the boiling point temperatures of liquids and lower their freezing point temperature. Therefore, the sample ‘B’ will freeze before 0°C.

Question 22.
What are the favourable qualities given to gold when it is alloyed with copper or silver for the purpose of making ornaments ?
Answer:
Pure gold (24 carats) is very soft and cannot be used for making ornaments. It is alloyed with a small quantity of copper or silver and becomes hard (22 carats). It can be drawn into wires (becomes ductile) or beaten into fine sheets (becomes malleable). It can, therefore, be used for making ornaments.

Question 23.
An element is sonorous and highly ductile. Under which category would you classify this element ? What other characteristics do you expect the element to possess ?
Answer:
The element is a metal. It is expected to possess the important characteristics of the metals.
Semi-Metals or Metalloids
There are a few elements which possess the characteristics of both metals and non-metals. These are actually border-line elements and are known as semi-metals. Semi-metals are also called metalloids. A few common examples are : Silicon, Arsenic, Antimony and Bismuth.

Question 24.
Give an example each for the mixture having the following characteristics. Suggest a suitable method to separate the components of these mixtures

1. A volatile and a non-volatile component.
2. Two volatile components with appreciable difference in boiling points.
3. Two immiscible liquids.
4. One of the components changes directly from solid to gaseous state.
5. Two or more coloured constituents soluble in same solvent.

Answer:

1. A mixture of naphthalene (volatile) and common salt (non-volatile). The separation can be done by sublimation.
2. A mixture of acetone (boiling point = 56°C) and water (boiling point = 100°C) separation can be done by distillation.
3. A mixture of kerosene oil and water. Separation can be done in a separating funnel.
4. Same as in (a).
5. A mixture of blue/black ink. Separation can be done by chromatography.

Question 25.
Fill in the blanks

1. A colloid is a …………… mixture and its components can be separated by the technique known as …………… .
2. Ice, water and water vapours look different and display different …………… properties but they are the …………… same.
3. A mixture of chloroform and water taken in a separating funnel is mixed and left undisturbed for some time. The upper layer in the separating funnel will be of …………… and the lower layer will be that of …………… .
4. A mixture of two or more miscible liquids for which the difference in the boiling points is less than 25 K, can be separated by the process called …………… .
5. When light is passed through water containing a few drops of milk, it shows a bluish tinge. This is due to the …………… of light by milk and the phenomenon is called …………… . This indicates that milk is a …………… solution.

Answer:

1. heterogenous, centrifugation
2. physical, chemically
3. water, chloroform (density of water is less than that of chloroform)
4. fractional distillation
5. scattering, Tyndall effect, colloidal (also called emulsion).

Question 26.
Sucrose (sugar) crystals obtained from sugarcane and beetroot are mixed together. Will it be a pure substance or a mixture ? Give reasons for the same.
Answer:
Both are chemically the same and represent a single chemical compound. Flence, they are pure substance.

Question 27.
Give some examples of Tyndall effect observed in your surroundings.
Answer:

1. Tyndall effect can be observed when sun light is made to enter the room in the morning time through a small slit. Dust particles with zig-zag motion can be seen.
2. Tyndall effect can be seen when sun light passes through the canopy of a dense forest.

Question 28.
Can we separate a mixture of alcohol and water by the use of a separating funnel ? If not, suggest an alternate method.
Answer:
No, the mixture of alcohol and water cannot be separated by the use of separating funnel because the two are completely miscible with each other. The separation can be done by fractional distillation.
The student should have dissolved the impure sample in carbon disulphide. It is a liquid in which sulphur completely dissolves while iron does not. From the solution, sulphur can be recovered with the help of crystallization process.

Question 29.
On heating, calcium carbonate gets converted into calcium oxide and carbon dioxide.

1. Is this a physical or a chemical change ?
2. Can you prepare one acidic and one basic solution by using the products formed in the above process ? If so, write the chemical equation involved.

Answer:

1. It is chemical change. The chemical equation for the reaction is :
   
2. By adding water to calcium oxide taken in a test tube, a basic solution results. It contains calcium hydroxide
   Calcium oxide + Water —-> Calcium hydroxide
   By passing carbon dioxide through water taken in a tube, carbonic acid is formed. The solution is of acidic nature
   Carbon dioxide + Water —-> Carbonic acid

Note:
All the three chemical equations are word equations. Symbol equations can also be written for these.
For example,

However, you will learn about these in the next class.

Question 30.
Non metals are usually poor conductors of heat and electricity. They are non-lustrous, non-sono- rous, non-malleable and are coloured.

1. Name a lustrous non-metal.
2. Name a non-metal which exists as a liquid at room temperature.
3. The allotropie form of a non-metal is a good conductor of electricity. Name the allotope.
4. Name a non-metal which is known to form the largest number of compounds.
5. Name a non-metal other than carbon which shows allotropy.
6. Name a non-metal which is required for combustion.

Answer:

1. Graphite (Carbon)
2. Bromine
3. Graphite(Carbon)
4. Carbon
5. Silicon
6. Coke (Carbon).

Question 31.
Classify the substances given in the figure into elements and compounds.

Answer:
Elements : Cu, Zn, Hg, Diamond.
Compounds : H₂O, CaCO₃, O₂, F₂
Note: Sand, NaCl (aq) and wood are the examples of mixtures.

Question 32.
Which of the following are not compounds ?

1. Chlorine gas
2. Potassium chloride
3. Iron
4. Iron sulphide
5. Aluminium
6. Iodine
7. Carbon
8. Carbon monoxide
9. Sulphur powder.

Answer:
Iron, aluminium, carbon and sulphur powder are not compounds. These are elements.

Long Answer Questions

Question 33.
Fractional distillation is suitable for separation of miscible liquids with a boiling point difference of about 25 K or less. What part of fractional distillation apparatus makes it efficient and possesses an advantage over a simple distillation process. Explain using a diagram.
Answer:
It is the fractionating column which fits into the distillation flask. It is packed with a number of glass beads. A the vapours of both the volatile liquids rise upwards in the distillation flask, the beads obstruct their movement i.e. it slows down. The vapours of higher boiling liquid condense to the liquid state and in doing so they release certain energy’ known as latent heat of condensation. The energy which is released is taken up by the molecules of lower boiling liquid. As a result, they vapourise more quickly and pass through the water condenser and condense as a liquid in the receiver. The higher boiling liquid remains in the distillation flask only. For further details about the fractional distillation

Question 34.
(a) Under which category of mixtures will you classify alloys and why ?
(b) A solution is always a liquid. Comment.
(c) Can a solution be heterogeneous ?
Answer:
(a) Aloys are regarded as homogeneous mixtures since the constituting elements are uniformly mixed in an alloy.
(b) This statement is not true. There may also be solid solutions (solid acts as solvent) and gaseous solutions (gas acts as solvent).
(c) No, a solution is always a homogeneous mixture of two or more substances.

Question 35.
Iron filings and sulphur were mixed together and divided into two parts ‘A’ and ‘B’. Part ‘A’ was heated strongly while Part ‘B’ was not heated. Dilute hydrochloric acid was added to both the Parts and evolution of gas was seen in both the cases. How will you identify the gases evolved ?
Answer:

1. In part A iron and sulphur will remain as such in the form of a mixture. On adding dilute hydrochlo¬ride acid iron will react to evolve hydrogen gas. The gas can be tested by bringing a burning splinter near its mouth. It will burn with a pop sound.
   
2. In part ‘B’ iron and sulphur will combine on heating to form greyish black solid known as iron sulphide.
   
3. When dilute hydrocloric acid is added to the greyish black mass taken in a tube, a gas with foul smell similar to that of the rotton eggs will evolve. This is hydrogen sulphide (H2S) Cover or Lid
   

Question 36.
A child wanted to separate the mixture of dyes constituting a sample of ink. He marked a line by the ink on the filter paper and placed the filter paper in a glass containing water as shown in the fig . The filter paper was removed when the water moved near the top of the filter paper.

1. What would you expect to see, if the ink contains three different coloured components ?
2. Name the technique used by the child.
3. Suggest one more application of this technique

Answer:

1. Three different bands will be seen on the filter papers.
2. The technique is known as paper chromatography.
3. It can be used to separate the coloured pigments from chlorophyll.

Question 37.
A group of students took an old shoe box and covered it with a black paper from all sides. They fixed a source of light (a torch) at one end of the box by making a hole in it and made another hole on the other side to view the light. They placed a milk sample contained in a beaker/tumbler in the box as shown in the figure.
They were amazed to see the milk taken in the tumbler was illuminated. They tried the same activity by taking a salt solution but found that light simply passed through it ?

1. Explain why the milk sample was illuminated. Name the phenomenon involved.
2. Same results were not observed with a salt solution. Explain.
3. Can you suggest two more solutions which would show the same effect as shown by the milk solution ?

Answer:

1. Milk sample is a colloidal sol also called emulsion. It got illuminated because of the scattering of light on passing through it. The phenomenon is known as Tyndall effect.
2. The salt solution is a true solution and not a colloidal solution. It will not show any Tyndall effect.
3. A solution of sulphur in water and a solution of starch in water.

Question 38.
Classify each of the following, as a physical or a chemical change. Give reasons.

1. Drying of a shirt in the sun.
2. Rising of hot air over a radiator.
3. Burning of kerosene in a lantern.
4. hange in the colour of black tea on adding lemon juice to it.
5. Churning of milk cream to get butter.

Answer:

1. It is a physical change. Moisture or water drops present on the shirt will evaporate or change into vapours.
2. It is a physical change. On cooling, the level of air over the radiator will fall.
3. It is a chemical change. The hydrocarbons present in kerosene will react chemically with oxygen to form new products.
4. It is a chemical change. The acid present in lemon juice will react with the constituents (e.g. caffeine) present in black tea.
5. It is a physical change. However, butter will not change to milk so easily.

Question 39.
During an experiment the students were asked to prepare a 10% (Mass/Mass) solution of sugar in water. Ramesh dissolved 10 g of sugar in 100 g of water while Sarika prepared it by dissolving 10 g of sugar in water to make 100 g of the solution.
(a) Are the two solutions of the same concentration
(b) Compare the mass % of the two solutions.
Answer:
(a) No, the two solutions have different concentrations.

Question 40.
You are provided with a mixture containing sand, iron filings, ammonium chloride and sodium chloride. Describe the procedure you would use to separate these constituents from the mixture ?
Answer:
Separate the constituents from a mixture containing ammonium chloride, sand and iron-filings.

Place the mixture on a paper or petridish. Move a magnet over it. Iron filings get attached to the magnet. Scrap off iron filings from the magnet and collect them separately. Transfer the remaining mixture to a china dish and subject it to the sublimation process. Ammonium chloride forms the sublimate while sand is left as residue in the dish.

Question 41.
Arun has prepared 0301% (by mass) solution of sodium chloride in water. Which of the following correctly represents the composition of the solution ?
(a) 1.00 g of NaCl + 100 g of water
(b) 0.11 g of NaCl + 100 g of water
(c) 0.01 g of NaCl + 99.99 g of water
(d ) 0.10 g of NaCl + 99.90 g of water.
Answer:
By definition 0.01% (by mass) solution means that 0.01 g of sodium chloride is dissolved in 0.99 g (by mass) of water. Therefore (c) represents the correct composition of the solution. This can be further verified as follows :

Question 42.
Calculate the mass of sodium sulphate required to prepare its 20% (mass percent) solution in 100 g of water ?
Answer:

Hope given NCERT Exemplar Solutions for Class 9 Science Chapter 2 Is Matter Around Us Pure are helpful to complete your science homework.

If you have any doubts, please comment below. Learn Insta try to provide online science tutoring for you.

NCERT Solutions for Class 9 Science Chapter 4 Structure of the Atom

These Solutions are part of NCERT Solutions for Class 9 Science. Here we have given NCERT Solutions for Class 9 Science Chapter 4 Structure of the Atom. LearnInsta.com provides you the Free PDF download of NCERT Solutions for Class 9 Science (Chemistry) Chapter 4 – Structure of The Atom solved by Expert Teachers as per NCERT (CBSE) Book guidelines. All Chapter 4 – Structure of The Atom Exercise Questions with Solutions to help you to revise complete Syllabus and Score More marks.

More Resources

• NCERT Solutions for Class 9 Science
• HOTS Questions for Class 9 Science
• Value Based Questions in Science for Class 9
• NCERT Exemplar Solutions for Class 9 Science
• Previous Year Question Papers for CBSE Class 9 Science

Practical Based Questions for Class 9 Science Chemistry

NCERT IN TEXT PROBLEMS

IN TEXT QUESTIONS

Question 1.
What are canal rays ?
Answer:
Canal rays also called anode rays, are seen moving from the anode towards the cathode in the specially designed discharge tube. However, they do not originate from the anode.

Question 2.
If an atom contains one electron and one proton, will it carry any charge or not ?
Answer:
No, it will not carry any charge because an electron has a unit negative charge and a proton has a unit
positive charge. They neutralise each other.

Question 3.
On the basis of Thomson’s model of an atom explain how the atom is neutral as a whole.
Answer:
According to Thomson’s Model of an atom, an atom may be regarded as a positively charged sphere in
which protons are present. The negatively charged electrons are believed to be studded or embedded in the sphere. Since the negative charges due to electrons and positive charges due to protons balance each other, the atom as a whole is neutral.

Question 4.
On the basis of Rutherford’s model of an atom, which sub-atomic particle is present in the nucleus of an atom ?
Answer:
The nucleus of atom is positively charged according to Rutherford’s model of an atom. All the protons in an atom are therefore, present in the nucleus.

Question 5.
Draw a sketch of Bohr’s model of an atom with three shells.
Answer:

Question 6.
What do you think would be the observation if the a-particle scattering experiment is carried out using a foil of a metal other than gold ?
Answer:
If the metal is a heavy one like gold (e.g. silver, platinum etc.) similar results will be obtained. However if the metal is quite light (e.g. sodium, magnesium etc.), it is just possible that the fast moving and massive α-particles (4u mass) may push the nucleus aside and pass through with slight deviations only.

Question 7.
Name the three sub – atomic particles in an atom.
Answer:
The sub-atomic particles in an atom are : protons, electrons and neutrons.

Question 8.
Helium atom has atomic mass of 4u and has two protons in its nucleus. How many neutrons does it have ?
Answer:
Mass number of helium is equal to its atomic mass but has no units.
∴ Mass number (A) of helium = 4
No. of protons in the nucleus = 2 Atomic number (Z) of the element = 2
No. of neutrons (n) = A – Z = 4 – 2 = 2.

Question 9.
Write the distribution of electrons in carbon and sodium atoms.
Answer:
Carbon(Z = 6) : 2 (K-shell) ; 4(L-shell)
Sodium (Z = 11) : 2 (K-shell) ; 10 (L-shell) ; 1 (M-shell)

Question 10.
If the K and L shells of an atom are full, then what would be the number of electrons in the atom ?
(CBSE 2014)
Answer:
Maximum no. of electrons in K-shell = 2
Maximum no. of electrons in L-shell = 8
Total no. of electrons in the atom = 2 + 8 = 10

Question 11.
If the number of electrons in an atom is 8 and the number of protons is also 8, then :
(i) What would be the atomic number of the atom ?
(ii) What is the charge on the atom ?
Answer:
(i) Atomic number (Z) of the atom = No. of protons = No. of electrons = 8
(ii) Charge on the atom = No charge
Actually, when the number of protons and electrons are the same, the atom is neutral.

Question 12.
For the symbols H, D and T, tabulate three sub-atomic particles found in each of them.
Answer:

Question 13.
Write the electronic configuration of any pair of isotopes and isobars.
Answer:
(i) Electronic configuration of pair of isotopes :

(ii) Electronic configuration of pair of isobars :
Isobars have same mass number but different atomic numbers. For example, elements calcium and argon are isobars :
Ca(Z = 20) : 2, 8, 8, 2
Ar (Z = 18) : 2, 8, 8.
Please note that both the elements have mass number (A) equal to 40.

NCERT END EXERCISE

Question 1.
Compare the properties of electrons, protons and neutrons.
Answer:

Question 2.
What are the limitations of J.J. Thomson’s model of an atom ?
Answer:
Limitations of Thomson’s model of an atom
(i) It could not explain the result of the scattering experiment performed by Rutherford.
(ii) It did not have any experimental evidence in its support.

Question 3.
What are the limitations of Rutherford’s model of an atom ?
Answer:
Limitations of Rutherford Model atom

1. Rutherford model of atom could not explain the stability of the atom.
2. Rutherford model of atom could not explain as to how the electrons are distributed in the extra nuclear portion in an atom.

Question 4.
Describe Bohr’s model of an atom.
Answer:
In 1913, Neils Bohr gave a theory regarding the distribution of electrons in the extra nuclear space in an atom. The main postulates of the theory are listed :

1. In the extra nuclear portion of an atom, the electrons revolve in well defined circular paths known as orbits.
2. These circular orbits are also known as energy levels or energy shells.
3. These have been designated as K, L, M, N, O, … (or as 1, 2, 3, 4, 5, …) based on the energy present.
4. The order of the energy of these energy shells is :
   K<L<M<N<0 <…. or 1< 2< 3 < 4<5 <….
5. While revolving in an orbit, the electron is not in a position to either lose or gain energy. In other words, its energy remains stationary. Therefore, these energy states for the electrons are also known as stationary states.

Question 5.
Compare all the proposed models of an atom given in this chapter.
Answer:
For Thomson’s Model of air atom: 

1. It could not explain the result of the scattering experiment performed by Rutherford.
2. It did not have any experimental evidence in its support.

For Rutherford’s Model of an atom:

1. Rutherford model of atom could not explain the stability of the atom.
2. Rutherford model of atom could not explain as to how the electrons are distributed in the extra nuclear portion in an atom.

For Bohr’s Model of air atom: 

1. It gave no idea about the shapes of the molecules formed by the combination of atoms.
2. According to Bohr’s theory, an electron in an atom follows a well defined circular path called orbit. However, the later studies have revealed that the path of the electron cannot be followed exactly. It is only of probable nature and not exact as stated by Bohr. However, it is not possible to discuss this concept at the present level of the students.

Question 6.
Summarise the rules for writing the distribution of electrons in various shells for first eighteen elements.
Answer:
As the electrons have negligible mass, the entire mass of the atom is that of protons and neutrons present in its nucleus. Since each proton and neutron has mass equal to 4 u, therefore, mass of an atom must be the same as the sum of protons and neutrons present in the nucleus. The latter is known as mass number of an element. It is denoted by the symbol A’. The mass number of an element may be defined as :
The sum of the number of protons and neutrons present in the nucleus of its atom.
Mass number (A) = No. of protons + No. of neutrons

Question 7.
Describe valency by taking the examples of silicon and oxygen.
Answer:
Valency of an element may be defined as the combining capacity of its atom with atoms of other elements in order to acquire 8 electrons (2 in some exceptional cases).
Valency of silicon (Si) : Atomic number of the element is 14. Its electronic distribution is ; K(2), L(8), M(4).
As silicon atom has four valence electrons, it can lose all of them. At the sametime, it can also gain four electrons to have a complete octet. Therefore, the valency of silicon is 4.
Valency of oxygen (O) : Atomic number of the element is 8. Its electronic distribution is ; K(2), L(6)
As oxygen atom has six valence electrons, it needs two more electrons to complete its octet (8-6 = 2). Therefore, valency of oxygen is 2.

Question 8.
Explain with examples
(i) Atomic number
(ii) Mass number
(iii) Isotopes
(iv) Isobars.
Answer:
(i) Atomic number:
Rutherford stated that the number of unit positive charges present in the nucleus of an atom is known as atomic number of the element It is denoted by the symbol Z.
                                        Atomic no. (Z)= No. ofprotons = No. of electrons
(ii) Mass number:
The mass number of an element may be defined as : the sum of the number of protons and neutrons present in the nucleus of its atom.
Mass number (A) = No. of protons + No. of neutrons
(iii) Isotopes:
Isotopes may be defined as the different atoms of the same element having same atomic number but different mass numbers.(iv) Isobars:
isobars may be defined as the atoms belonging to the different elements with same mass numbers but different atomic numbers.

Question 9.
Na⁺ ion has completely filled K and L shells. Explain. (CBSE 2013)
Answer:
Na⁺ ion is formed when Na atom loses one electron from the valence shell i.e. M shell. The Na⁺ ion formed has only two shells which are complete
Na : K(2) L(8) M(1) ;
Na⁺ : K(2) L(8)

Question 10.
If the element bromine is in the form two isotopes which are ⁷⁹₃₅Br (49.7%) and ⁸¹₃₅Br (30.3%), then calculate the average atomic mass of bromine.
Answer:

Question 11.
The average atomic mass of a sample of element X is 16.2 u. What are the percentages of isotopes ¹⁶₈X and ¹⁸₈X in the sample ? (CBSE 2016)
Answer:

Question 12.
If Z = 3, what would be the valency of the element ? Also name the element.
Answer:
The configuration of the element with Z = 3 is K(2) L(l). It has one electron in the valence shell i.e. L-shell. Its valency is one. The element is lithium (Li).

Question13.
Composition of the nuclei of two atomic species X and Y are given as under

Give the mass numbers of X and Y. What is the relation between the two species ?
Answer:
Mass number of X = protons + neutrons = 6 + 6 =12 Mass number of Y = 6 + 8 = 14
The species X and Y are the isotopes because their atomic numbers are same and their mass numbers are different.

Question 14.
For the following statements, write T for True and F for False.
(a) J.J. Thomson proposed that the nucleus of an atom contains only nucleons.
(b) A neutron is formed by an electron and proton combining together. Therefore, it is neutral.
(c) The mass of an electron is about 1/2000 times that of proton.
(d) An isotope of iodine is used for making tincture of iodine, which is used as medicine.
Answer:
(a) F
(b) F
(c) T
(d) F.

Question 15.
Rutherford’s alpha-particle scattering experiment was responsible for the discovery of
(a) Atomic Nucleus
(b) Electron
(c) Proton
(d) Neutron.
Answer:
(a) is the correct answer.

Question 16.
Isotopes of element have :
(a) the same physical properties
(b) different chemical properties
(c) different number of neutrons
(d) different atomic numbers.
Answer:
(c) Different number of neutrons.

Question 17.
Number of valence electrons in Cl^– ion are :
(a) 16
(b) 8
(c) 17
(d) 18.
Answer:
(d) CE ion has 18 electrons (17 + 1).

Question 18.
Which one of the following is a correct electronic configuration of sodium ?
(a) 2, 8
(b) 8, 2, 1
(c) 2, 1, 8
(d) 2, 8, 1
Answer:
(d) is the correct answer.

Question 19.
Complete the following table.

Answer:

VERY SHORT ANSWER QUESTIONS

Question 1.
Out of the three sub-atomic particles known, which is called universal particle ?
Answer:
Electron is known as universal particle.

Question 2.
Name a species which has
(i) one proton and one electron
(ii) one proton and two electrons
Answer:
(i) Protium or hydrogen (H)
(ii) Hydride ion (H^–).

Question 3.
How many electrons are present in the species He²⁺ ion ? Suggest another name for it.
Answer:
He²⁺ ion has no electrons. It is also called alpha (α) particle.

Question 4.
How many times is radius of extra nuclear protion more than that of the nucleus of an atom ?
Answer:
Radius of extra nuclear portion is nearly 10⁵ times more as compared to the nucleus.

Question 5.
Is atomic number of an atom always equal to the number of electrons ?
Answer:
No, it is the case when the atom has no charge. In case of cation (positively charged), atomic number is more than the number of electrons and in case of anion (negatively charged), it is less than the number of electrons.

Question 6.
Out of proton and neutron, which is heavier ?
Answer:
Neutron is slightly heavier (1.675 x 10^-27 kg) than proton (1.67 x 10^-27 kg).

Question 7.
Explain why chlorine has relative atomic mass of about 35.5 u ? (CBSE 2016)
Answer:
Chlorine always exists as two isotopes. These are Cl-35 and Cl-37 isotopes and are present in the ratio of 3:1. The atomic mass of the element is therefore, fractional and is regarded as the relative atomic mass.

Question 8.
Why is proton so named ?
Answer:
Proton (H⁺) is formed when hydrogen atom also called protium, loses an electron. Therefore, it is called proton.

Question 9.
The cation is represented as M³⁺. What is the difference between the number of protons and electrons present ?
Answer:
In cation M³⁺, there are three electrons less as compared to protons.

Question 10.
If K and L shells of an atom are completely filled, what will be its name ?
Answer:
The atom will belong to the noble gas element neon (Ne).

Question 11.
Do isobars have also identical chemical characteristics like isotopes ?
Answer:
No, these are not identical because the isobars have different atomic numbers as well as different electronic configurations. ‘

Question 12.
The electronic configuration of an element is : 2(K), 8(L), 5(M). Predict its valency.
Answer:
The valency of the element is 3. It is calculated as : 8 – 5 = 3.

Question 13.
Write two important postulates of Bohr’s model of an atom. (CBSE 2014)
Answer:
The 2 main postulates of the theory are listed :

1. In the extra nuclear portion of an atom, the electrons revolve in well defined circular paths known as orbits.
2. These circular orbits are also known as energy levels or energy shells.

Question 14.
What is the basic difference between the isotopes of an element ?
Answer:
Isotopes of an element differ in the number of neutrons leading to different mass numbers.

Question 15.
The element aluminium is written by the symbol fgAI. Write the number of protons, electrons and neutrons present in it.
Answer:
No. of protons= 13 ; No. of electrons = 13 ; No. of neutrons = 27 – 13 = 14.

Question 16.
What is the number of electrons in the valence shell of chlorine (Z = 17) ?
Answer:
The electronic distribution of the element is : K(2), L(8), M(7). This means that the valence shell of chlorine has 7 electrons.

Question 17.
Which radioisotope is used for the treatment of cancer ?
Answer:
Radioisotope Co-60 is used for the treatment of cancer.

Question 18.
Out of C-12 and C-14 isotopes of carbon, which is of radioactive nature ?
Answer:
C-14 isotope is of radioactive nature.

Question 19.
If an atom has one proton and one electron, state the charge on the atom. Justify your answer. (CBSE 2014)
Answer:
The atoms is neutral. One unit positive charge on proton cancels with one unit negative charge on electron.

Question 20.
The electronic configuration of an element Z is 2, 8, 6. How many electrons does it require to have a stable
configuration ?
Answer:
In order to have a stable configuration, the element Z must have 8 electrons in the valence shell. It needs (8 – 6) = 2 electrons for this purpose.

Question 21.
Will ¹²₆C and ¹⁴₆C have different valencies ?
Answer:
Both the species are the isotopes of the same element i.e. the carbon. Since their atomic numbers are same, their electronic configurations as well as valencies will also be the same.

Question 22.
The atom of an element A’ has three electrons in the outermost shell. It loses one of these to the atom of another element ‘B’. What will be the nature and value of charge on the ion which results from ‘A’ ?
Answer:
Since the atom A’ has lost one electron, the ion formed will be positive and will have +1 charge. It can be represented as A+.

Question 23.
The atom of an element has electronic configuration 2, 8, 6. Does it gain two electrons or lose six electrons to have the configuration of the nearest noble gas element ?
Answer:
The atom has six electrons in its valence shell. It is very difficult for it to lose six electrons. However, it can gain two electrons from a neighboring atom of another element. By doing so, it will have the electronic configuration of nearest noble gas element argon (2, 8, 8).

Question 24.
Do the elements X and represent pair of isotopes ?
Answer:
No, they do not because the two elements differ in their atomic numbers. Isotopes have the same atomic
numbers.

Question 25.
An element ‘X’ has mass number 4 and atomic number 2. Find the valency of ‘X’. (CBSE 2012)
Answer:
The electronic configuration : K(2). Since the K-shell is filled, the valency of ‘X’ = zero.

Question 26.
Name the particles which determine the mass of an atom. (CBSE 2014)
Answer:
Protons and neutrons present in the nucleus determine the mass of an atom.

SHORT ANSWER QUESTIONS

Question 27.
What is the number of valence electrons in
(i) Sodium ion (Na⁺)
(ii) oxide ion (O^2-) ? (CBSE 2012)
Answer:
(i) Sodium ion (Na⁺) :
No. of electrons : (11 – 1) = 10 ; Electronic configuration = 2, 8.
∴ Na⁺ ion has 8 valence electrons.
(ii) Oxide ion (O^2-) :
No. of electrons : (8 + 2) = 10 ; Electronic configuration = 2, 8
∴ O^2- ion has 8 valence electrons.
Both Na⁺ ion and O^2- ion are known as isoelectronic species as they have same number of electrons i.e. 10. Their electronic configuration is similar to that of neon (Z = 10) which is a noble gas element.
This means that O^2- ion, Na⁺ ion and Ne atom are isoelectronic species.

Question 28.
Elements A and ‘B’ have atomic numbers 18 and 16 respectively. Which of these two would be more reactive and why ?
Answer:
Electronic configuration of A (Z = 18) ; 2, 8, 8
Electronic configuration of B (Z = 16) ; 2, 8, 6
Element A’ has completely filled outermost shell (M-shell). It would be therefore, least reactive.
Element ‘B’ has incomplete outermost shell (M-shell has 6 electrons) only.
Therefore, element ‘B’ would be more reactive than element ‘A’.

Question 29.
Two atoms A and B have the following composition :

What are their mass numbers ? What is the relation between the species ?
Answer:
Mass number of A =17+18 = 35
Mass number of B = 17 + 20 = 37
The atoms A and B represent a pair of isotopes since thery have the same atomic number (17)

Question 30.
The composition of two atomic particles is given :

(i) What is the mass number of X ?
(ii) What is the mass number of Y
(iii) What is the relation between X and Y ?
(iv) Which element/elements do they represent ? (CBSE 2012)
Answer:
(i) Mass number of X = 8 + 8 =16
(ii) Mass number of Y = 8 + 9 = 17
(iii) The atomic particles represent a pair of isotopes.
(iv) The atomic number of the atomic particles is the same as the number of protons (8). The element with atomic number 8 is oxygen.

Question 31.
State whether the following statements are true or false.
(i) One mole of methane has four H atoms.
(ii) The isotopes of an element differ in the number of electrons.
(iii) The elements are identified by their atomic numbers.
(iv) The mass number of an element has specific units.
(v) The K shell of an element cannot have more than eight electrons.
Answer:
(i) False
(ii) False
(iii) True
(iv) False
(v) False

Question 32.
Name the elements which have the following electronic configuration :
(i) 2, 6
(ii) 2, 7
(iii) 2, 8, 1
(iv) 2, 8, 7
(v) 2, 8
Answer:
(i) oxygen
(ii) fluorine
(iii) sodium
(iv) chlorine
(v) neon.

Question 33.
Give three points of distinction between isotopes and isobars.
Answer:

Question 34.
The details about three atoms X, Y and Z are given. Give information about their atomic number, mass number and valency ?

Answer:

Question 35.
Why do elements helium, neon and argon have zero valencies ?
Answer:
The electronic configuration of three elements are given :

The first element helium (He) has only one shell and has maximum of two electrons in the only shell (K-shell) which it has.
Similarly, the next two elements neon (Ne) and argon (Ar) also have the maximum of eight electrons in their outermost shells L and M respectively.
This means that the atoms of all the three elements donot have any urge to either lose or gain electrons. Therefore, they show zero valencies. These are also called noble gas or inert gas elements as they donot take part in the chemical combination.

Question 36.
Give the electronic distribution in magnesium atom and magnesium ion. How do these electronic configuration differ ?
Answer:

Mg²⁺ ion has only two shells and its electronic configuration is similar to that of noble gas element neon (Ne). Mg atom has three shells with 2 electrons in the outer shell i.e. M-shell.

LONG ANSWER QUESTIONS

Question 37.
(a) Give the schematic atomic structures of chlorine atom and chloride ion.
(b) Mention two uses of isotopes in the field of medicine.
(c) How are the following pair of elements known as ?
⁴⁰₁₈Ar,   ⁴⁰₂₀Ca
Answer:
(a)

(b) (i) Radioisotope 1-131 is used to check the working of thyroid gland.
(ii) Radioisotope Co – 60 is used in cancer therapy.
(c) These are known as isobars. Since they have the same mass number but different atomic numbers.

Question 38.
Answer the following in one line or two :
(a) What is the maximum number of electrons that can be accomodated in the outermost energy shell in an atom ?
(b) On the basis of Thomsons model of an atom, explain how an atom is neutral as a whole.
(c) How many neutrons are present in hydrogen atom ?
(d) Do isobars belong to the same element ?
(e) An element has five electrons in the M-shell which is the outermost shell. Write its electronic configuration.
(CBSE 2011)
Answer:
(a) The outermost energy shell in an atom can have a maximum of eight electrons.
(b) According to Thomson’s model of an atoms, all the protons in an atom are present in the positively charged sphere while negatively charged electrons are studded in this sphere. Since the electrons and protons are equal in number each carrying one unit charge, the atom as a whole is electrically neutral.
(c) Hydrogen atom has no neutron.
(d) No, isolars belong to different elements since they differ in their atomic numbers.
(e) The electronic configuration of the element is K(2) L(8) M(5).

Question 39.
Which observations in scattering experiment led Rutherford to make the following conclusions :
(i) Most of the space in an atom is empty.
(ii) Whole mass of an atom is present in its centre.
(iii) Nucleus is positively charged.
Answer:
(i) As most of the alpha particles passed through undeflected, this means that they did not come across any obstruction in their path. Thus, most of the space in an atom is expected to be empty.
(ii)

1. As a few alpha particles suffered minor deflections and a very few major deflections, this means that these must have met with some obstructions in their path.
2. This obstruction must be :
   1. Very small : Only a few particles were obstructed by it.Massive : Each alpha particle has 4u mass and is quite heavy.
   2. It could easily pass through a light obstruction by pushing it aside.

(iii) Positively charged : Alpha particles have positive charge. Since they were repelled or deflected back, the obstruction must also carry same charge i.e., positive charge, (similarly charged particles always repel each other

Question 40.
With the help of suitable activities shows that
(i) Cathode rays travel in straight line
(ii) Cathode rays consist of material particles. (CBSE 2012)
Answer:
(i) Cathode rays always travel in straight line. In order to demonstrate this, place an object in the path of the cathode rays that are emitted in a discharge tube. The shadow of the object will immediately appear behind it. This shows that these rays are stopped by the object and thus, travel in straight line.

(ii) Cathode rays consist of negatively charged particles known as electrons which have definite mass. In order to demonstrate this, place a light paddle wheel of mica mounted on an axle in the path of cathode rays. It will start rotating. This shows that the cathode rays or electrons are material particles.

Question 41.
How did Bohr distribute electrons in different shells in an atom ?
Answer:
In 1913, Neils Bohr gave a theory regarding the distribution of electrons in the extra nuclear space in an atom. The main postulates of the theory are listed :

1. In the extra nuclear portion of an atom, the electrons revolve in well defined circular paths known as orbits.
2. These circular orbits are also known as energy levels or energy shells.
3. These have been designated as K, L, M, N, O, … (or as 1, 2, 3, 4, 5, …) based on the energy present.
4. The order of the energy of these energy shells is :
   K<L<M<N<0 <…. or 1< 2< 3 < 4<5 <….
5. While revolving in an orbit, the electron is not in a position to either lose or gain energy. In other words, its energy remains stationary. Therefore, these energy states for the electrons are also known as stationary states

Question 42.
The average atomic mass of a sample of an element ‘X’ is 16.2 u. What is the percentage of each isotope ¹⁶₈X and ¹⁸₈X in the sample ?
Answer:
Let the percentage of  ¹⁶₈X isotope = x
The percentage of  ¹⁸₈X isotope = 100 – x

Question 43.
Give reasons for the following :
(a) Isotopes of an element are chemically similar.
(b) An atom is electrically neutral.
(c) Noble gases show least reactivity
(d) Nucleus of an atom is heavy and and positively charged.
(e) Ions are more stable than atoms.
Answer:
(a) Isotopes of an element have same atomic number as well as electronic distribution. Since the chemical properties of the elements are related to their electronic distribution or configuration, the elements with similar configuration have similar properties. Thus, the isotopes of an element are chemically similar.
(b) In an atom, the number of protons in the nucleus is the equal to the number of electrons in the extra nuclear portion. Since each proton and each electron has the same charge but with opposite magnitude, atom is therefore, electrically neutral.
(c) The atoms of the noble gas elements have complete outermost electron shells. With the exception of helium (He) which has two electrons in its only shell (K-shell), the rest of the members of the family have eight electrons in their outermost or valence shell. This means that atoms of these elements have no urge to take part in chemical combination or they are least reactive in nature.
(d) Positively charged : Alpha particles have positive charge. Since they were repelled or deflected back, the obstruction must also carry same charge i.e., positive charge, (similarly charged particles always repel each other
(e) When an atom changes into ion (cation or anion) the valence shell of the ion has a complete octet, (i.e. it has eight electrons in the outermost or valence shell). In some ions, the valence shell is K-shell and it has only two electrons (e.g. Li⁺ ion or H^– ion). The ions are therefore, quite stable in nature.

Question 44.
The following data represents the distribution of electrons, protons and neutrons in atoms of four elements . A, B, C, D.

Answer the following questions :
(a) Describe the electronic distribution in atom of element B.
(b) Is element B a metal or a non-metal ? Why ?
(c) Which two elements form a pair of Isotopes ?
(d) Which two elements form in pair of Isobars ?
Answer:
(a) The electronic distribution of element B is 2, 8, 7 .
(b) The element B is non-metal (Cl). Its atom needs only one electron to have the configuration of nearest noble gas element argon (2, 8, 8).
(c) The elements B and C form pair of isotopes since both of them have same number of electrons 17 and also same atomic number (Z= 17)
(d) The elements A and D represent pair of isobars since both of them have same number (A = 40).

NCERT Solutions for Class 9 Science Chapter 4 Structure of The Atom

Hope given NCERT Solutions for Class 9 Science Chapter 4 are helpful to complete your science homework.

If you have any doubts, please comment below. Learn Insta try to provide online science tutoring for you.

RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.3

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.3

Other Exercises

• RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.1
• RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.2
• RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.3
• RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4
• RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.5
• RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials VSAQS
• RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials MCQS

In each of the following, using the remainder Theorem, find the remainder when f(x) is divided by g(x) and verify the result by actual division (1 – 8) :

Question 1.
f(x) = x³ + 4x² – 3x + 10, g(x) = x + 4
Solution:

Question 2.
f(x) – 4x⁴ – 3x³ – 2x² + x – 7, g(x) = x – 1
Solution:

Question 3.
f(x) = 2x⁴ – 6X³ + 2x² – x + 2, ,g(x) = x + 2
Solution:

Question 4.
f(x) = 4x³ – 12x² + 14x – 3, g(x) = 2x – 1
Solution:

Question 5.
f(x) = x³ – 6x² + 2x – 4, g(x) = 1 – 2x
Solution:

Question 6.
f(x) = x⁴ – 3x² + 4, g(x) = x – 2
Solution:

Question 7.

Solution:

Question 8.

Solution:

Question 9.
If the polynomials 2x³ + ax² + 3x – 5 and x³ + x² – 4x + a leave the same remainder when divided by x – 2, find the value of a.
Solution:
Let f(x) = 2x³ + ax² + 3x – 5
g(x) = x³+x²-4x + a
q(x) = x –  2 ⇒ x-2 = 0  ⇒x = 2
∴ Remainder =f(2) = 2(2)³ + a(2)² + 3 x 2-5
= 2 x 8 4-a x 4 + 3 x 2-5
= 16 + 4a + 6 – 5
= 4a +17
and g(2) = (2)³ + (2)² -4×2 + a
= 8 + 4 – 8 + a = a + 4
∵  In both cases, remainder are same
∴  4a + 17 = a + 4
⇒  4a – a = 4 – 17 ⇒  3a = -13
⇒ a = \(\frac { -13 }{ 3 }\)
Hence a = \(\frac { -13 }{ 3 }\)

Question 10.
If the polynomials ax³ + 3x² – 13 and 2x³ – 5x + a, when divided by (x – 2), leave the same remainders, find the value of a.
Solution:
Let p(x) = ax³ + 3x² – 13
q(x) = 2x³ –5x + a
and divisor g(x) = x – 2
x-2 = 0
⇒ x = 2
∴ Remainder = p(2) = a(2)³ + 3(2)² – 13
= 8a + 12 – 13 = 8a – 1
and q( 2) = 2(2)³ – 5×2 + a=16-10 + a
= 6 + a
∵  In each case remainder is same
∴ 8a – 1 = 6 + a
8a – a = 6 + 1
⇒  7a = 7
⇒ a = \(\frac { 7 }{ 7 }\)= 1
∴ a = 1

Question 11.
Find the remainder when x³ + 3x² + 3x + 1 is divided by

Solution:

Question 12.
The polynomials ax³ + 3a-² – 3 and 2x³ – 5x + a when divided by (x – 4) leave the remainders R₁ and R₂, respectively. Find the values of a in each case of the following cases, if
(i) R₁ = R₂
(ii) R₁ + R₂ = 0
(iii) 2R₁ – R₂ = 0.
Solution:

Hope given RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.3 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.