Tag: Maths Class 9 RD Sharma Solutions

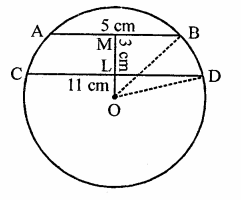
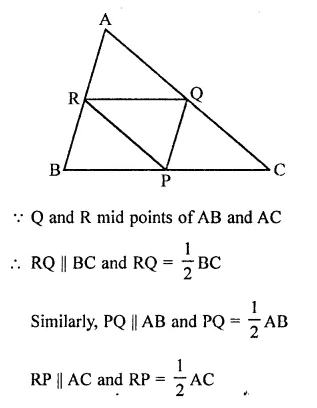
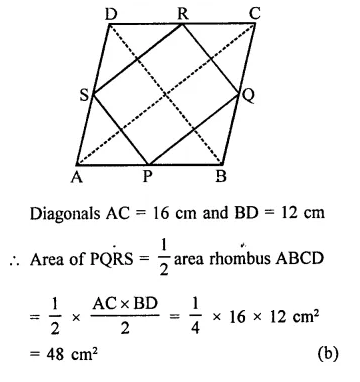
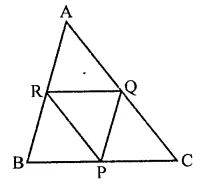
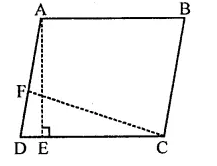
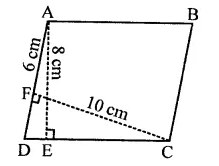
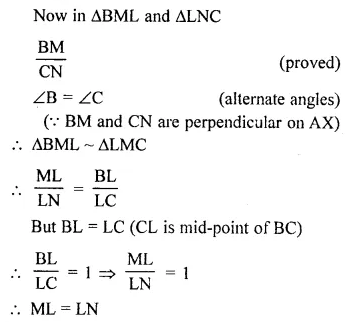
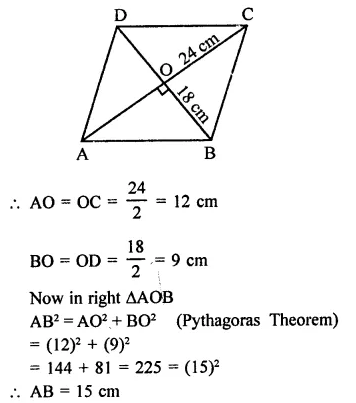
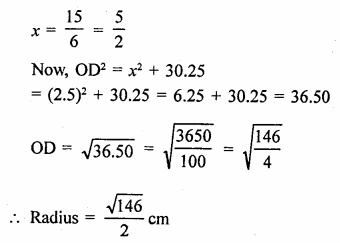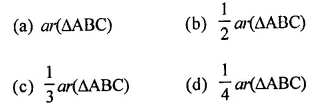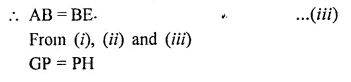RD Sharma Class 9 Solutions Chapter 16 Circles Ex 16.2

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 16 Circles Ex 16.2

Other Exercises

• RD Sharma Class 9 Solutions Chapter 16 Circles Ex 16.1
• RD Sharma Class 9 Solutions Chapter 16 Circles Ex 16.2
• RD Sharma Class 9 Solutions Chapter 16 Circles Ex 16.3

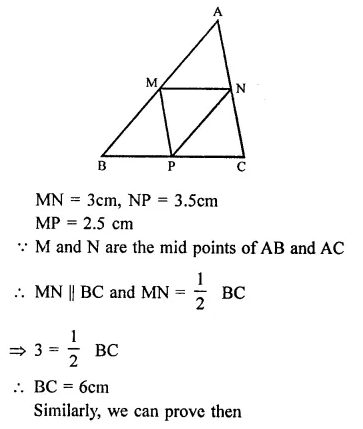
Question 1.
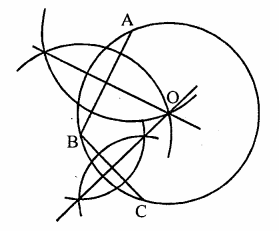
Draw an angle and label it as ∠BAC. Construct another angle, equal to ∠BAC.
Solution:
Steps of construction :
(i) Draw an angle BAC.
(ii) Draw a line DF.
(iii) With centre A and D, draw arcs of equal radius which intersect AC at L and AB at M and DF at P.
(iv) Cut off PQ = LM and join DQ and produce it to E, then
∠EDF = ∠BAC.

Question 2.
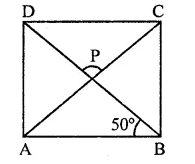
Draw an obtuse angle. Bisect it. Measure each of the angles so obtained.
Solution:
Steps of construction :
(i) Draw an angle ABC which is an obtuse i.e. more than 90°.
(ii) With centre B and a suitable radius draw an arc meeting BC at E and AB at F.
(iii) With centre E and F, and radius more than half of EF draw arcs intersecting each other at G.
(iv) Join BG and produce it to D.
ThenBD is die bisector of ∠ABC.
On measuring each part, we find each angle = 53°.

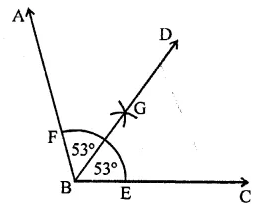
Question 3.
Using your protractor, draw an angle of measure 108°. With this angle as given, draw an angle of 54°.
Solution:
Steps of construction :
(i) With the help of protractor draw an angle ABC = 108°.
As 54° = \(\frac { 1 }{ 2 }\) x 108°
∴ We shall bisect it.

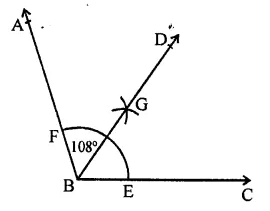
(ii) With centre B and a suitable radius, draw an arc meeting BC at E and AB at F.
(iii) With centre E and F, and radius more than half of EF, draw arcs intersecting each other at G
(iv) Join BG and produce it to D.
Then ∠DBC = 54°.

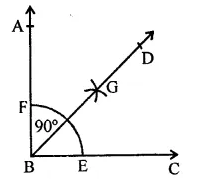
Question 4.
Using protractor, draw a right angle. Bisect it to get an angle of measure 45°.
Solution:
Steps of construction :
(i) Using protractor, draw a right angle ∆ABC.
i. e. ∠ABC = 90°.
(ii) With centre B and a suitable radius, draw an arc which meets BC at E and BA at F.
(iii) With centre E and F, draw arcs intersecting each other at G.
(iv) Join BG and produce it to D.
Then BD is the bisector of ∠ABC.
∴ ∠DBC =\(\frac { 1 }{ 2 }\) x 90° = 45°.

Question 5.
Draw a linear pair of angles. Bisect each of the two angles. Verify that the two bisecting rays are perpendicular to each other.
Solution:
Steps of construction :
(i) Draw a linear pair ∠DCA and ∠DCB.
(ii) Draw the bisectors of ∠DCA and ∠DCB. Forming ∠ECF on measuring we get ∠ECF = 90°.
Verification : ∵∠DCA + ∠DCB = 180°
⇒ \(\frac { 1 }{ 2 }\) ∠DCA + \(\frac { 1 }{ 2 }\) ∠DCB = 180° x \(\frac { 1 }{ 2 }\) = 90°
∴ ∠ECF = 90°
i.e. EC and FC are perpendicular to each other.

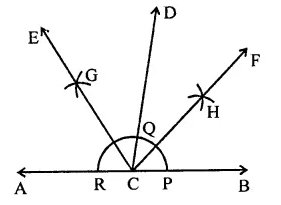
Question 6.
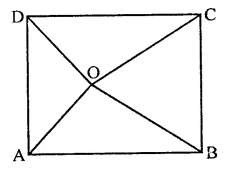
Draw a pair of vertically opposite angles. Bisect each of the two angles. Verify that the bisecting rays are in the same line
Solution:
Steps of construction :
(i) Draw two lines AB and CD intersecting each other at O.
(ii) Draw the bisector of ∠AOD and also the bisector of ∠BOC. Which are OP and OQ respectively. We see that OP and OQ are in the same straight line.

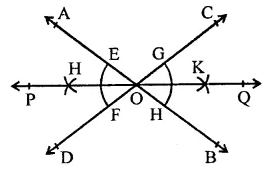
Question 7.
Using ruler and compasses only, draw a right angle.
Solution:
Steps of construction :
(i) Draw a line segment BC.
(ii) With centre B and a suitable radius, draw an arc meeting BC at E.
(iii) With centre E and same radius, cut off arcs EF and FG.
(iv) Bisect arc FG at H.
(v) Join BH and produce it to A.
Then ∠ABC = 90°.

Question 8.
Using ruler and compasses only, draw an angle of measure 135°.
Solution:
Steps of construction :
(i) Draw a line DC and take a point B on it.
(ii) With centre B and a suitable radius draw an arc meeting BC at P.
(iii) With centre P, cut off arcs PQ, QR and RS.
(iv) Bisect as QR at T and join BT and produce it to E.
(v) Now bisect the arc KS at RL.
(vi) Join BL and produce it to A.
Now ∠ABC = 135°.

Question 9.
Using a protractor, draw an angle of measure 72°. With this angle as given, draw angles of measure 36° and 54°.
Solution:
Steps of construction :
(i) Draw an angle ABC = 12° with the help of protractor.
(ii) With centre B and a suitable radius, draw an arc EF.
(iii) With centre E and F, draw arcs intersecting
each other at G and produce it to D.
Then BD is the bisector of ∠ABC.
∴ ∠DBC = 72° x \(\frac { 1 }{ 2 }\) = 36°.
(iv) Again bisect ∠ABD in the same way then PB is the bisector of ∠ABD.
∴ ∠PBC = 36° + \(\frac { 1 }{ 2 }\) x 36°
= 36° + 18° = 54°
Hence ∠PBC = 54°

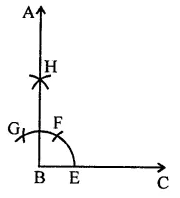
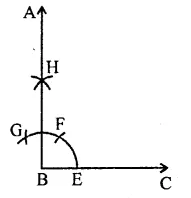
Question 10.
Construct the following angles at the initial point of a given ray and justify the construction:
(i) 45°
(ii) 90°
Solution:
(i) 45°
Steps of construction :
(a) Draw a line segment BC.
(b) With centre B and a suitable radius draw an arc meeting BC at E.
(c) With centre E, cut off equal arcs EF and FG.
(d) Bisect FG at H.
(e) Join BH and produce to X so that ∠XBC = 90°.
(f) Bisect ∠XBC so that ∠ABC = 45°.

(ii) 90°
Steps of construction :
(a) Draw a line segment BC.
(b) With centre B and a suitable radius, draw an arc meeting BC at E and with centre E cut off arcs from E, EF = FG
(c) Now bisect the arc EG at H.
(d) Join BH and produce it to A.
∴ ∠ABC = 90°.

Question 11.
Construct the angles of the following measurements:
(i) 30°
(ii) 75°
(iii) 105°
(iv) 135°
(v) 15°
(vi) 22 \(\frac { 1 }{ 2 }\)°
Solution:
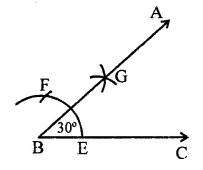
(i) 30°
Steps of construction :
(a) Draw a line segment BC.
(b) With centre B and a suitable radius draw an arc meeting BC at E.
(c) Cut off arcs EF and bisect it at G.
So that ∠ABC = 30°.

(ii) 75°
Steps of construction :
(a) Draw a line segment BC.
(b) With centre B and a suitable radius, draw an arc meeting BC at E.
(c) Cut off arc EF = FG from E.
(d) Bisect the arc FG at K and join BK so that ∠KBC = 90°.
(e) Now bisect arc HF at L and join BL and produce it to A so that ∠ABC = 75°.

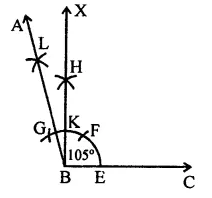
(iii) 105°
Steps of construction :
(a) Draw a line segment BC.
(b) With centre B and a suitable radius draw an arc meeting BC at E.
(c) From E, cut off arc EF = FG and divide FG at H.
(d) Join BH meeting the arc at K.
(e) Now bisect the arc KG at L.
Join BL and produce it to A.
Then ∠ABC = 105°.

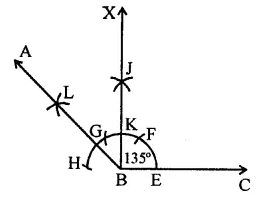
(iv) 135°
Steps of construction :
(a) Draw a line segment BC.
(b) With centre B and a suitable radius, draw an arc meeting BC at E.
(c) From E, cut off EF = FG = GH.
(d) Bisect arc FG at K, and join them.
(e) Bisect arc KH at L.
(f) Join BL and produce it to A, then ∠ABC = 135°.

(v) 15°
Steps of construction :
(a) Draw a line segment BC.
(b) With centre B and a suitable radius, draw an arc meeting BC at E.
(c) Cut off arc EF from E and bisect it at G. Then ∠GBC = 30°.
(d) Again bisect the arc EJ at H.
(e) Join BH and produce it to A.
Then ∠ABC = 15°.

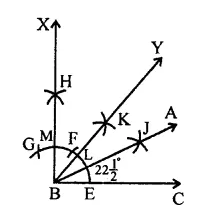
(vi) 22 \(\frac { 1 }{ 2 }\)°
Steps of construction :
(a) Draw a line segment BC.
(b) With centre B and a suitable radius, draw an arc and from E, cut off EF = FG
(c) Bisect FG at H so that ∠HBC = 90°.
(d) Now bisect ∠HBC at K. So that ∠YBC = 45°.
(e) Again bisect ∠YBC at J. So thttt ∠ABC = 22 \(\frac { 1 }{ 2 }\)°

Hope given RD Sharma Class 9 Solutions Chapter 16 Circles Ex 16.2 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.3

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.3

Other Exercises

• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.1
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.2
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.3
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.4
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.5
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles VSAQS
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles MCQS

Question 1.
Three girls Ishita, Isha and Nisha are playing a game by standing on a circle of radius 20 m drawn in a park. Ishita throws a ball to Isha, Isha to Nisha and Nisha to Ishita. If the distance between Ishita and Isha and between Isha and Nisha is 24 m each, what is the distance between Ishita and Nisha. [NCERT]
Solution:
∵ Distance between Isha and Ishita and Ishita and Nisha is same
∴ RS = SM = 24 m
∴They are equidistant from the centre
In right ∆ORL,
OL² = OR² – RL²


Hence distance between Ishita and Nisha = 38.4 m

Question 2.
A circular park of radius 40 m is situated in a colony. Three beys Ankur, Amit and Anand are sitting at equal distance on its boundary each having a toy telephone in his hands to talk to each other. Find thelength of the string of each phone. [NCERT]
Solution:
Radius of circular park = 40 m
Ankur, Amit and Anand are sitting at equal distance to each other By joining them, an equilateral triangle ABC is formed produce BO to L which is perpendicular bisector of AC

∴ BL = 40 + 20 = 60 m (∵ O is centroid of ∆ABC also)
Let a be the side of ∆ABC

Hence the distance between each other = 40\(\sqrt { 3 } \) m

Hope given RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.3 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.2

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.2

Other Exercises

• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.1
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.2
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.3
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.4
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.5
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles VSAQS
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles MCQS

Question 1.
The radius of a circle is 8 cm and the length of one of its chords is 12 cm. Find the distance of the chord from the centre.
Solution:
Radius of circle with centre O is OA = 8 cm
Length of chord AB = 12 cm
OC ⊥ AB which bisects AB at C

∴ AC = CB = 12 x \(\frac { 1 }{ 2 }\) = 6 cm
In ∆OAC,
OA2 = OC2 + AC2 (Pythagoras Theorem)
⇒ (8)2 = OC2 + (6)2
⇒ 64 = OC2 + 36
OC2 = 64 – 36 = 28
∴ OC = \(\sqrt { 28 } \) = \(\sqrt { 4×7 } \) cm
= 2 x 2.6457 = 5.291 cm

Question 2.
Find the length of a chord which is at a distance of 5 cm from the centre of a circle of radius 10 cm.
Solution:
Let AB be a chord of a circle with radius 10 cm. OC ⊥ AB
∴ OA = 10 cm
OC = 5 cm

∵ OC divides AB into two equal parts
i.e. AC = CB
Now in right AOAC,
OA2 = OC2 + AC2 (Pythagoras Theorem)
⇒ (10)2 = (5)2 + AC2
⇒ 100 = 25 + AC2
⇒ AC2 = 100 – 25 = 75
∴ AC = \(\sqrt { 75 } \)= \(\sqrt { 25×3 } \) = 5 x 1.732
∴ AB = 2 x AC = 2 x 5 x 1.732 = 10 x 1.732 = 17.32 cm

Question 3.
Find the length of a chord which is at a distance of 4 cm from the centre of the circle of radius 6 cm.
Solution:
In a circle with centre O and radius 6 cm and a chord AB at a distance of 4 cm from the centre of the circle
i.e. OA = 6 cm and OL ⊥ AB, OL = 4 cm

∵ Perpendicular OL bisects the chord AB at L 1
∴ AL = LB=\(\frac { 1 }{ 2 }\) AB
Now in right ∆OAL,
OA2 = OL2 + AL2 (Pythagoras Theorem)
(6)2 = (4)2 + AL2
⇒ 36=16+AL2
⇒ AL2 = 36 – 16 = 20
∴ AL = \(\sqrt { 20 } \) = \(\sqrt { 4×5 } \) = 2 x 2.236 = 4.472 cm
∴ Chord AB = 4.472 x 2 = 8.944 = 8.94 cm

Question 4.
Give a method to find the centre of a given circle.
Solution:
Steps of construction :

(i) Take three distinct points on the circle say A, B and C.
(ii) Join AB and AC.
(iii) Draw the perpendicular bisectors of AB and AC which intersect each other at O.
O is the required centre of the given circle

Question 5.
Prove that a diameter of a circle which bisects a chord of the circle also bisects the angle subtended by the chord at the centre of the circle.
Solution:
Given : In circle with centre O
CD is the diameter and AB is the chord
which is bisected by diameter at E
OA and OB are joined

To prove : ∠AOB = ∠BOA
Proof : In ∆OAE and ∆OBE
OA = OB (Radii of the circle)
OE = OE (Common)
AE = EB (Given)
∴ ∆OAE = ∆OBE (SSS criterian)
∴ ∠AOE = ∠BOE (c.p.c.t.)
Hence diameter bisect the angle subtended by the chord AB.

Question 6.
A line segment AB is of length 5 cm. Draw a circle of radius 4 cm passing through A and B. Can you draw a circle of radius 2 cm passing through A and B? Give reason in support of your answer.
Solution:
Steps of construction :
(i) Draw a line segment AB = 5 cm.
(ii) Draw a perpendicular bisector of AB.
(iii) With centre A and radius 4 cm, draw an arc which intersects the perpendicular bisector at O.
(iv) With centre O and radius 4 cm, draw a circle which passes through A and B.
With radius 2 cm, we cannot draw the circle passing through A and B as diameter
i. e. 2 + 2 = 4 cm is shorder than 5 cm.

Question 7.
An equilateral triangle of side 9 cm is inscribed in a circle. Find the radius of the circle.
Solution:
Steps of construction :
(i) Draw a line segment BC = 9 cm.
(ii) With centres B and C, draw arcs of 9 cm radius which intersect each other at A.
(iii) Join AB and AC.
∆ABC is the required triangle.
(iv) Draw perpendicular bisectors of sides AB and BC which intersect each other at O.
(v) With centre O and radius OB, draw a circle which passes through A, B and C.
This is the require circle in which ∆ABC is inscribed.

On measuring its radius, it is 5.2 cm

Question 8.
Given an arc of a circle, complete the circle.
Solution:
Steps of construction :
(i) Take three points A, B and C on the arc and join AB and BC.
(ii) Draw the perpendicular bisector of AB and BC which intersect each other at O.

(iii) With centre O and radius OA or OB, complete the circle.
This is the required circle.

Question 9.
Draw different pairs of circles. How many points does each pair have in common? What is the maximum number of common points?
Solution:
Below, three different pairs of circles are drawn:


(i) In the first pair, two circles do not intersect each other. Therefore they have no point in common. .
(ii) In the second pair, two circles intersect (touch) each other at one point P. Therefore they have one point in common.
(iii) In the third pair, two circles intersect each other at two points. Therefore they have two points in common.
There is no other possibility of two circles intersecting each other.
Therefore, two circles have at the most two points in common.

Question 10.
Suppose you are given a circle. Give a construction to find its centre.
Solution:
See Q. No. 4 of this exercise.

Question 11.
The length of two parallel chords of a circle are 6 cm and 8 cm. If the smaller chord is at a distance of 4 cm from the centre, what is the distance of the other chord from the centre? [NCERT]
Solution:
A circle with centre O and two parallel chords
AB and CD are AB = 6 cm, CD = 8 cm
Let OL ⊥ AB and OM ⊥ CD
∴ OL = 4 cm
Let OM = x cm
Let r be the radius of the circle

Question 12.
Two chords AB, CD of lengths 5 cm and 11 cm respectively of a circle are parallel. If the distance between AB and CD is 3 cm, find the radius of the circle.
Solution:
Let two chords AB and CD of length 5 cm and 11 cm are parallel to each other AB = 5 cm, CD = 11 cm
Distance between AB and LM = 3 cm
Join OB and OD
OL and OM are the perpendicular on CD and AB respectively. Which bisects AB and CD.
Let OL = x, then OM = (x + 3)

Now in right ∆OLD,
OD² = OL² + LD²
= x² + (5.5)²
Similarly in right ∆OMB,
OB² = OM² + MB² = (x + 3)² + (2.5)²
But OD = OB (Radii of the circle)
∴ (x + 3)² + (2.5)² = x² + (5.5)²
x² + 6x + 9 + 6.25 = x² + 30.25
6x = 30.25 – 6.25 – 9 = 15

Question 13.
Prove that the line joining the mid-point of a chord to the centre of the circle passes through the mid-point of the corresponding minor arc.
Solution:
Given : A circle with centre O and a chord AB
Let M be the mid point of AB and OM is joined and produced to meet the minor arc AB at N
To prove : M is the mid point of arc AB
Construction : Join OA, OB

Proof: ∵ M is mid point of AB
∴ OM ⊥ AB
In AOAM and OBM,
OA = OB (Radii of the circle)
OM = OM (common)
AM = BM (M is mid point of AB)
∴ ∆OAM = ∆OBM (SSS criterian)
∴ ∠AOM = ∠BOM (c.p.c.t.)
⇒ ∠AOM = ∠BOM
But these are centre angles at the centre made by arcs AN and BN
∴ Arc AN = Arc BN
Hence N divides the arc in two equal parts

Question 14.
Prove that two different circles cannot intersect each other at more than two points.
Solution:
Given : Two circles
To prove : They cannot intersect each other more than two points
Construction : Let two circles intersect each other at three points A, B and C

Proof : Since two circles with centres O and O’ intersect at A, B and C
∴ A, B and C are non-collinear points
∴ Circle with centre O passes through three points A, B and C
and circle with centre O’ also passes through three points A, B and C
But one and only one circle can be drawn through three points
∴Our supposition is wrong
∴ Two circle cannot intersect each other not more than two points.

Question 15.
Two chords AB and CD of lengths 5 cm and 11 cm respectively of a circle are parallel to each other and are opposite side of its centre. If the distance between AB and CD is 6 cm, find the radius of the circle. [NCERT]
Solution:
Let r be the radius of the circle with centre O.
Two parallel chords AB = 5 cm, CD = 11 cm
Let OL ⊥ AB and OM ⊥CD
∴ LM = 6 cm
Let OM = x, then
OL = 6 – x

Hope given RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.2 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.1

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.1

Other Exercises

• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.1
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.2
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.3
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.4
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.5
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles VSAQS
• RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles MCQS

Question 1.
Fill in the blanks: [NCERT]
(i) All points lying inside / outside a circle are called …….. points / ………. points.
(ii) Circles having the same centre and different radii are called …….. circles.
(iii) A point whose distance from the centre of a circle is greater than its radius lies in …….. of the circle.
(iv) A continuous piece of a circle is …….. of the circle.
(v) The longest chord of a circle is a ……… of the circle.
(vi) An arc is a …….. when its ends are the ends of a diameter.
(vii) Segment of a circle is the region between an are and ……..of the circle.
(viii)A circle divides the plane, on which it lies, in …….. parts.
Solution:
(i) All points lying inside / outside a circle are called interior points / exterior points.
(ii) Circles having the same centre and different radii are called concentric circles.
(iii) A point whose distance from the centre of a circle is greater than its radius lies in exterior of the circle.
(iv) A continuous piece of a circle is arc of the circle.
(v) The longest chord of a circle is a diameter of the circle.
(vi) An arc is a semi-circle when its ends are the ends of a diameter.
(vii) Segment of a circle is the region between an arc and centre of the circle.
(viii) A circle divides the plane, on which it lies, in three parts.

Question 2.
Write the truth value (T/F) of the following with suitable reasons: [NCERT]
(i) A circle is a plane figure.
(ii) Line segment joining the centre to any point on the circle is a radius of the circle.
(iii) If a circle is divided into three equal arcs each is a major arc.
(iv) A circle has only finite number of equal chords.
(v) A chord of a cirlce, which is twice as long is its radius is a diameter of the circle.
(vi) Sector is the region between the chord and its corresponding arc.
(vii) The degree measure of an arc is the complement of the central angle containing the arc.
(viii)The degree measure of a semi-circle is 180°.
Solution:
(i) True.
(ii) True.
(iii) True.
(iv) False. As it has infinite number of equal chords.
(v) True.
(vi) False. It is a segment not sector.
(vii) False. As total degree measure of a circle is 360°.
(viii) True.

 

 

Hope given RD Sharma Class 9 Solutions Chapter 15 Areas of Parallelograms and Triangles Ex 15.1 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals MCQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals MCQS

Other Exercises

• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.1
• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.2
• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.3
• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals VSAQS
• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals MCQS

Mark the correct alternative in each of the following:
Question 1.
Two parallelograms are on the same base and between the same parallels. The ratio of their areas is
(a) 1 : 2
(b) 2 : 1
(c) 1 : 1
(d) 3 : 1
Solution:
Two parallelograms which are on the same base and between the same parallels are equal in area
∴ Ratio in their areas =1 : 1 (c)

Question 2.
A triangle and a parallelogram are on the same base and between the same parallels. The ratio of the areas of triangle and parallelogram is
(a) 1 : 1
(b) 1 : 2
(c) 2 : 1
(d) 1 : 3
Solution:
A triangle and a parallelogram which are on the same base and between the same parallels, then area of triangle is half the area of the parallelogram
∴ Their ratio =1:2 (c)

Question 3.
Let ABC be a triangle of area 24 sq. units and PQR be the triangle formed by the mid-points of sides of ∆ABC. Then the area of ∆PQR is
(a) 12 sq. units
(b) 6 sq. units
(c) 4 sq. units
(d) 3 sq. units
Solution:
Area of ∆ABC = 24 sq. units

Question 4.
The median of a triangle divides it into two
(a) congruent triangle
(b) isosceles triangles
(c) right triangles
(d) triangles of equal areas
Solution:
The median of a triangle divides it into two triangles equal in area (d)

Question 5.
In a ∆ABC, D, E, F are the mid-points of sides BC, CA and AB respectively. If
ar(∆ABC) = 16 cm2, then ar(trapezium FBCE) =
(a) 4 cm²
(b) 8 cm²
(c) 12 cm²
(d) 10 cm²
Solution:
In ∆ABC, D, E and F are the mid points of sides BC, CA and AB respectively
ar(∆ABC) = 16 cm²

Question 6.
ABCD is a parallelogram. P is any point on CD. If ar(∆DPA) = 15 cm² and ar(∆APC) = 20 cm², then ar(∆APB) =
(a) 15 cm²
(b) 20 cm²
(c) 35 cm²
(d) 30 cm²
Solution:
In ||gm ABCD, P is any point on CD
AP, AC and PB are joined
ar(∆DPA) =15 cm²
ar(∆APC) = 20 cm²
Adding, ar(∆ADC) = 15 + 20 = 35 cm²

∵ AC divides it into two triangles equal in area
∴ ar(∆ACB) = ar(∆ADC) = 35 cm²
∵ ∆APB and ∆ACB are on the same base
AB and between the same parallels
∴ ar(∆APB) = ar(∆ACB) = 35 cm²(c)

Question 7.
The area of the figure formed by joining the mid-points of the adjacent sides of a rhombus with diagonals 16 cm and 12 cm is
(a) 28 cm²
(b) 48 cm²
(c) 96 cm²
(d) 24 cm²
Solution:
In rhombus ABCD,
P, Q, R and S are the mid points of sides AB, BC, CD and DA respectively and are joined in order to get a quad. PQRS

Question 8.
A, B, C, D are mid points of sides of parallelogram PQRS. If ar(PQRS) = 36 cm²,then ar(ABCD) =
(a) 24 cm²
(b) 18 cm²
(c) 30 cm²
(d) 36 cm²
Solution:
A, B, C and D are the mid points of a ||gm PQRS
Area of PQRS = 36 cm²

The area of ||gm formed by joining AB, BC, CD and DA

Question 9.
The figure obtained by joining the mid-points of the adjacent sides of a rectangle of sides 8 cm and 6 cm is
(a) a rhombus of area 24 cm²
(b) a rectangle of area 24 cm²
(c) a square of area 26 cm²
(d) a trapezium of area 14 cm²
Solution:
Let P, Q, R, S be the mid points of sides of a rectangle ABCD. Whose sides 8 cm and 6 cm

Their PQRS is a rhombus

Question 10.
If AD is median of ∆ABC and P is a point on AC such that ar(∆ADP) : ar(∆ABD) = 2:3, then ar(∆PDC) : ar(∆ABC) is
(a) 1 : 5
(b) 1 : 5
(c) 1 : 6
(d) 3 : 5
Solution:
AD is the median of ∆ABC,
P is a point on AC such that
ar(∆ADP) : ar(∆ABD) = 2:3
Let area of ∆ADP = 2×2
Then area of ∆ABD = 3×2

But area of AABD = \(\frac { 1 }{ 2 }\) area AABC
∴ Area ∆ABC = 2 x area of ∆ABD
= 2 x 3x² = 6x²
and area of ∆PDC = area ∆ADC – (ar∆ADP) = area ∆ABD – ar ∆ADP
= 3x² – 2x² = x²
∴ Ratio = x² : 6x²
= 1 : 6 (c)

Question 11.
Medians of AABC, intersect at G. If ar(∆ABC) = 27 cm2, then ar(∆BGC) =
(a) 6 cm2
(b) 9 cm2
(c) 12 cm2
(d) 18 cm2
Solution:
In ∆ABC, AD, BE and CF are the medians which intersect each other at G

Question 12.
In a ∆ABC if D and E are mid-points of BC and AD respectively such that ar(∆AEC) = 4 cm², then ar(∆BEC) =
(a) 4 cm²
(b) 6 cm²
(c) 8 cm²
(d) 12 cm²
Solution:
In ∆ABC, D and E are the mid points of BC and AD
Join BE and CE ar(∆AEC) = 4 cm²

In ∆ABC,
∵ AD is the median of BC
∴ ar(∆ABD) = ar(∆ACD)
Similarly in ∆EBC,
ED is the median
∴ ar(∆EBD) = ar(∆ECD)
and in ∆ADC, CE is the median
∴ ar(∆FDC) = ar(∆AEC)
= 4 cm
∴ar∆EBC = 2 x ar(∆EDC)
= 2 x 4 = 8 cm (c)

Question 13.
In the figure, ABCD is a parallelogram. If AB = 12 cm, AE = 7.5 cm, CF = 15 cm, then AD =
(a) 3 cm
(b) 6 cm
(c) 8 cm
(d) 10.5 cm

Solution:
In ||gm ABCD, AB = 12 cm AE = 7.5 cm
∴ Area of ||gm ABCD = base x height = AB x AE = 12 x 7.5 cm² = 90 cm²
Now area ||gm ABCD = 90 cm²
and altitude CF = 15 cm
∴ Base AD = \(\frac { Area }{ Altitude }\) = \(\frac { 90 }{ 15 }\) = 6 cm (b)

Question 14.
In the figure, PQRS is a parallelogram. If X and Y are mid-points of PQ and SR respectively and diagonal SQ is joined. The ratio ar(||gm XQRY) : ar(∆QSR) =
(a) 1 : 4
(b) 2 : 1
(c) 1 : 2
(d) 1 : 1

Solution:
In ||gm PQRS, X and Y are the mid points of PQ and SR respectively XY and SQ are joined.
∵ XY bisects PQ and SR
∴ PXYS and XQRY are also ||gms and ar(∆PXYS) = nr(∆XQRY)
∵ ||gm PQRS and AQSR are on the same base and between the same parallel lines

Question 15.
Diagonal AC and BD of trapezium ABCD, in which AB || DC, intersect each other at O. The triangle which is equal in area of ∆AOD is
(a) ∆AOB
(b) ∆BOC
(c) ∆DOC
(d) ∆ADC
Solution:
In trapezium ABCD, diagonals AC and BD intersect each other at O. AB || DC
∆ABC and ∆ABD are on the same base and between the same parallels

∴ ar(∆ABC) = or(∆ABD)
Subtracting ar(∆AOB)
ar(∆ABC) – ar(∆AOB) = ar(∆ADB) – ar(∆AOB)
⇒ ar(∆BOC) = ar(∆AOD)
ar(∆AOD) = ar(∆BOC) (c)

Question 16.
ABCD is a trapezium in which AB || DC. If ar(∆ABD) = 24 cm² and AB = 8 cm, then height of ∆ABC is
(a) 3 cm
(b) 4 cm
(c) 6 cm
(d) 8 cm
Solution:
In trapezium ABCD, AB || DC
AC and BD are joined
ar(∆ABD) = 24 cm2
AB = 8 cm,

Question 17.
ABCD is a trapezium with parallel sides AB = a and DC = b. If E and F are mid-points of non-parallel sides AD and BC respectively, then the ratio of areas of quadrilaterals ABFE and EFCD is
(a) a : b
(b) (a + 3b) : (3a + b)
(c) (3a + b) : (a + 3b)
(d) (2a + b) : (3a + b)
Solution:
In quadrilateral ABCD, E and F are the mid points of AD and BC
AB = a, CD = b

Let h be the height of trapezium ABCD then height of each quadrilateral
ABFE = altitude of quadrilateral EFCD = \(\frac { h }{ 2 }\)
Now area of trap. ABFE = \(\frac { 1 }{ 2 }\) (sum of parallel sides) x altitude

Question 18.
ABCD is a rectangle with O as any point in its interior. If or(∆AOD) = 3 cm2 and ar(∆BOC) = 6 cm2, then area of rectangle ABCD is
(a) 9 cm2
(b) 12 cm2
(c) 15 cm2
(d) 18 cm2
Solution:
In rectangle ABCD, O is any point
ar(∆AOD) = 3 cm2
and ar(∆BOC) = 6 cm2
Join OA, OB, OC and OD

We know that if O is any point in ABCD Then ar(AOB) + ar(COD) = ar(AOB) + ar(BOC)
= 3 + 6 = 9 cm
∴ ar(rect. ABCD) = 2 x 9 = 18 cm (d)

Question 19.
The mid-points of the sides of a triangle ABC along with any of the vertices as the fourth point make a parallelogram of area equal to

Solution:

P,Q and R the mid points of the sides of a ∆ABC then area of any parallelogram formed by the mid points and one vertex of the given triangle has area = \(\frac { 1 }{ 2 }\) area ∆ABC (b)

Question 20.
In the figure, ABCD and FECG are parallelograms equal in area. If ar(∆AQE) = 12 cm2, then ar(||gm FGBQ) =
(a) 12 cm2
(b) 20 cm2
(c) 24 cm2
(d) 36 cm2

Solution:
In the figure, ABCD and EFCG are parallelograms equal in area and ar(∆AQE) = 12 cm2
In ||gm AQED, AE is the diagonal
∴ ar(∆AQE) = \(\frac { 1 }{ 2 }\) ar(||gm AQED)
⇒ 12 cm2 = \(\frac { 1 }{ 2 }\) ar(||gm AQED)
∴ ar(||gm AQED) = 24 cm2
∵ ar ||gm ABCD = ar ||gm FECG
⇒ ar(||gm ∆QED) + ar(|| gm QBCE)
= ar(||gm QBCE) + ar(||gm FGBQ)
⇒ ar(||gm ∆QED) = ar(||gm FGBQ)
= 24 cm2 (c)

Hope given RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals MCQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.2

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.2

Other Exercises

• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.1
• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.2
• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.3
• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals VSAQS
• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals MCQS

Question 1.
In the figure, ABCD is a parallelogram, AE ⊥ DC and CF ⊥ AD. If AB = 16 cm, AE = 8 cm and CF = 10 cm, find AD. [NCERT]

Solution:
In ||gm ABCD,
Base AB = 16 cm
and altitude AE = 8 cm

∴ Area = Base x Altitude
= AB x AE
= 16 x 8 = 128 cm²
Now area of ||gm ABCD = 128 cm²
Altitude CF = 10 cm
∴ Base AD = \(\frac { Area }{ Altitude }\) = \(\frac { 128 }{ 10 }\) = 12.8cm

Question 2.
In Q. No. 1, if AD = 6 cm, CF = 10 cm, AE = 8 cm, find AB.
Solution:
Area of ||gm ABCD,

= Base x Altitude
= AD x CF
= 6 x 10 = 60 cm²
Again area of ||gm ABCD = 60 cm²
Altitude AE = 8 cm
∴ Base AB =\(\frac { Area }{ Altitude }\) = \(\frac { 60 }{ 8 }\) = \(\frac { 15 }{ 2 }\) cm = 7.5 cm

Question 3.
Let ABCD be a parallelogram of area 124 cm2. If E and F are the mid-points of sides AB and CD respectively, then find the area of parallelogram AEFD.
Solution:
Area of ||gm ABCD = 124 cm²
E and F are the mid points of sides AB and CD respectively. E, F are joined.

Draw DL ⊥ AB
Now area of ||gm ABCD = Base x Altitude
= AB x DL = 124 cm²
∵ E and F are mid points of sides AB and CD
∴ AEFD is a ||gm
Now area of ||gm AEFD = AE x DL
= \(\frac { 1 }{ 2 }\)AB x DL [∵ E is mid point of AB]
= \(\frac { 1 }{ 2 }\) x area of ||gm ABCD
= \(\frac { 1 }{ 2 }\) x 124 = 62 cm²

Question 4.
If ABCD is a parallelogram, then prove that ar(∆ABD) = ar(∆BCD) = ar(∆ABC) = ar(∆ACD) = \(\frac { 1 }{ 2 }\)ar( ||^gm ABCD).
Solution:
Given : In ||gm ABCD, BD and AC are joined

To prove : ar(∆ABD) = ar(∆BCD) = ar(∆ABC) = ar(∆ACD) = \(\frac { 1 }{ 2 }\)ar(||gm ABCD)
Proof: ∵ Diagonals of a parallelogram bisect it into two triangles equal in area When BD is the diagonal, then
∴ ar(∆ABD) = ar(∆BCD) = \(\frac { 1 }{ 2 }\)ar(||^gm ABCD) …(i)
Similarly, when AC is the diagonal, then
ar(∆ABC) = ar(∆ADC) = \(\frac { 1 }{ 2 }\)ar(||^gm ABCD) …(ii)
From (i) and (ii),
ar(∆ABD) = ar(∆BCD) = ar(∆ABC) = ar(∆ACD) = \(\frac { 1 }{ 2 }\) ar(||^gm ABCD)

Hope given RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.2 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.4

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.4

Other Exercises

• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.1
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.2
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.3
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.4
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables VSAQS
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables MCQS

Question 1.
In a ∆ABC, D, E and F are respectively, the mid-points of BC, CA and AB. If the lengths of side AB, BC and CA are 7cm, 8cm and 9cm, respectively, find the perimeter of ∆DEF.
Solution:
In ∆ABC, D, E and F are the mid-points of sides,
BC, CA, AB respectively
AB = 7cm, BC = 8cm and CA = 9cm
∵ D and E are the mid points of BC and CA
∴ DE || AB and DE =\(\frac { 1 }{ 2 }\) AB =\(\frac { 1 }{ 2 }\) x 7 = 3.5cm
Similarly,

Question 2.
In a triangle ∠ABC, ∠A = 50°, ∠B = 60° and ∠C = 70°. Find the measures of the angles of the triangle formed by joining the mid-points of the sides of this triangle.
Solution:
In ∆ABC,
∠A = 50°, ∠B = 60° and ∠C = 70°

D, E and F are the mid points of sides BC, CA and AB respectively
DE, EF and ED are joined
∵ D, E and F are the mid points of sides BC, CA and AB respectively
∴ EF || BC
DE || AB and FD || AC
∴ BDEF and CDEF are parallelogram
∴ ∠B = ∠E = 60° and ∠C = ∠F = 70°
Then ∠A = ∠D = 50°
Hence ∠D = 50°, ∠E = 60° and ∠F = 70°

Question 3.
In a triangle, P, Q and R are the mid-points of sides BC, CA and AB respectively. If AC = 21 cm, BC = 29cm and AB = 30cm, find the perimeter of the quadrilateral ARPQ.
Solution:
P, Q, R are the mid points of sides BC, CA and AB respectively
AC = 21 cm, BC = 29 cm and AB = 30°
∵ P, Q, R and the mid points of sides BC, CA and AB respectively.
∴ PQ || AB and PQ = \(\frac { 1 }{ 2 }\) AB

Question 4.
In a ∆ABC median AD is produced to X such that AD = DX. Prove that ABXC is a parallelogram.
Solution:
Given : In ∆ABC, AD is median and AD is produced to X such that DX = AD
To prove : ABXC is a parallelogram
Construction : Join BX and CX
Proof : In ∆ABD and ∆CDX
AD = DX (Given)
BD = DC (D is mid points)
∠ADB = ∠CDX (Vertically opposite angles)
∴ ∆ABD ≅ ∆CDX (SAS criterian)
∴ AB = CX (c.p.c.t.)
and ∠ABD = ∠DCX
But these are alternate angles

∴ AB || CX and AB = CX
∴ ABXC is a parallelogram.

Question 5.
In a ∆ABC, E and F are the mid-points of AC and AB respectively. The altitude AP to BC intersects FE at Q. Prove that AQ = QP.
Solution:
Given : In ∆ABC, E and F are the mid-points of AC and AB respectively.
EF are joined.
AP ⊥ BC is drawn which intersects EF at Q and meets BC at P.
To prove: AQ = QP
proof : In ∆ABC

E and F are the mid points of AC and AB
∴ EF || BC and EF = \(\frac { 1 }{ 2 }\)BC
∴ ∠F = ∠B
In ∆ABP,
F is mid point of AB and Q is the mid point of FE or FQ || BC
∴ Q is mid point of AP,
∴ AQ = QP

Question 6.
In a ∆ABC, BM and CN are perpendiculars from B and C respectively on any line passing through A. If L is the mid-point of BC, prove that ML NL.
Solution:
In ∆ABC,
BM and CN are perpendicular on a line drawn from A. L is the mid point of BC. ML and NL are joined.

Question 7.
In the figure triangle ABC is right-angled at B. Given that AB = 9cm. AC = 15cm and D, E are the mid points of the sides AB and AC respectively, calculate.
(i) The length of BC
(ii) The area of ∆ADC
Solution:
In ∆ABC, ∠B = 90°
AC =15 cm, AB = 9cm
D and E are the mid points of sides AB and AC respectively and D, E are joined.

Question 8.
In the figure, M, N and P are the mid points of AB, AC and BC respectively. If MN = 3 cm, NP = 3.5cm and MP = 2.5cm, calculate BC, AB and AC.

Solution:
In ∆ABC,
M, N and P are the mid points of side, AB, AC and BC respectively.

Question 9.
In the figure, AB = AC and CP || BA and AP is the bisector of exterior ∠CAD of ∆ABC. Prove that (i) ∠PAC = ∠BCA (ii) ABCP is a parallelogram.
Solution:
Given : In ABC, AB = AC

nd CP || BA, AP is the bisector of exterior ∠CAD of ∆ABC
To prove :
(i) ∠PAC = ∠BCA
(ii) ABCP is a ||gm
Proof : (i) In ∆ABC,
∵ AB =AC
∴ ∠C = ∠B (Angles opposite to equal sides) and ext.
∠CAD = ∠B + ∠C
= ∠C + ∠C = 2∠C ….(i)
∵ AP is the bisector of ∠CAD
∴ 2∠PAC = ∠CAD …(ii)
From (i) and (ii)
∠C = 2∠PAC
∠C = ∠CAD or ∠BCA = ∠PAC
Hence ∠PAC = ∠BCA
(ii) But there are alternate angles,
∴ AD || BC
But BA || CP
∴ ABCP is a ||gm.

Question 10.
ABCD is a kite having AB = AD and BC = CD. Prove that the figure formed by joining the mid-points of the sides, in order, is a rectangle.
Solution:
Given : In fne figure, ABCD is a kite in which AB = AD and BC = CD.
P, Q, R and S are the mid points of the sides AB, BC, CD and DA respectively.
To prove : PQRS is a rectangle.
Construction : Join AC and BD.

Proof: In ∆ABD,
P and S are mid points of AB and AD
∴ PS || BD and PS = \(\frac { 1 }{ 2 }\) BD …(i)
Similarly in ∆BCD,
Q and R the mid points of BC and CD
∴ QR || BD and
QR = \(\frac { 1 }{ 2 }\) BD …(ii)
∴ Similarly, we can prove that PQ || SR and PQ = SR …(iii)
From (i) and (ii) and (iii)
PQRS is a parallelogram,
∵ AC and BD intersect each other at right angles.
∴ PQRS is a rectangle.

Question 11.
Let ABC be an isosceles triangle in which AB = AC. If D, E, F be the mid-points of the sides BC, CA and AB respectively, show that the segment AD and EF bisect each other at right angles.
Solution:
In ∆ABC, AB = AC
D, E and F are the mid points of the sides BC, CA and AB respectively,
AD and EF are joined intersecting at O
To prove : AD and EF bisect each other at right angles.
Construction : Join DE and DF.

Proof : ∵ D, E and F are the mid-points of
the sides BC, CA and AB respectively
∴ AFDE is a ||gm
∴ AF = DE and AE = DF
But AF = AE
(∵ E and F are mid-points of equal sides AB and AC)
∴ AF = DF = DE = AE
∴AFDE is a rhombus
∵ The diagonals of a rhombus bisect each other at right angle.
∴ AO = OD and EO = OF
Hence, AD and EF bisect each other at right angles.

Question 12.
Show that the line segments joining the mid points of the opposite sides of a quadrilateral bisect each other.
Solution:
Given : In quad. ABCD,
P, Q, R and S are the mid points of sides AB, BC, CD and DA respectively.
PR and QS to intersect each other at O
To prove : PO = OR and QO = OS
Construction: Join PQ, QR, RS and SP and also join AC.
Proof: In ∆ABC
P and Q are mid-points of AB and BC
∴ PQ || AC and PQ = \(\frac { 1 }{ 2 }\) AC …(i)
Similarly is ∆ADC,
S and R are the mid-points of AD and CD
∴ SR || AC and SR = \(\frac { 1 }{ 2 }\) AC ..(ii)

from (i) and (ii)
PQ = SQ and PQ || SR
PQRS is a ||gm (∵ opposite sides are equal area parallel)
But the diagonals of a ||gm bisect each other.
∴ PR and QS bisect each other.

Question 13.
Fill in the blanks to make the following statements correct :
(i) The triangle formed by joining the mid-points of the sides of an isosceles triangle is …
(ii) The triangle formed by joining the mid-points of the sides of a right triangle is …
(iii) The figure formed by joining the mid-points of consecutive sides of a quadrilateral is …
Solution:
(i) The triangle formed by joining the mid-points of the sides of an isosceles triangle is an isosceles triangle.

(ii) The triangle formed by joining the mid-points of the sides of a right triangle is right triangle.

(iii) The figure formed by joining the mid-points of consecutive sides of a quadrilateral is a parallelogram.

Question 14.
ABC is a triangle and through A, B, C lines are drawn parallel to BC, CA and AB respectively intersecting at P, Q and R. Prove that the perimeter of ∆PQR is double the perimeter of ∆ABC.
Solution:
Given : In ∆ABC,
Through A, B and C, lines are drawn parallel to BC, CA and AB respectively meeting at P, Q and R.

To prove : Perimeter of ∆PQR = 2 x perimeter of ∆ABC
Proof : ∵ PQ || BC and QR || AB
∴ ABCQ is a ||gm
∴ BC = AQ
Similarly, BCAP is a ||gm
∴ BC = AP …(i)
∴ AQ = AP = BL
⇒ PQ = 2BC
Similarly, we can prove that
QR = 2AB and PR = 2AC
Now perimeter of ∆PQR.
= PQ + QR + PR = 2AB + 2BC + 2AC
= 2(AB + BC + AC)
= 2 perimeter of ∆ABC.
Hence proved

Question 15.
In the figure, BE ⊥ AC. AD is any line from A to BC intersecting BE in H. P, Q and R are respectively the mid-points of AH, AB and BC. Prove that PQR = 90°.
Solution:
Given: In ∆ABC, BE ⊥ AC
AD is any line from A to BC meeting BC in D and intersecting BE in H. P, Q and R are respectively mid points of AH, AB and BC. PQ and QR are joined B.

To prove : ∠PQR = 90°
Proof: In ∆ABC,
Q and R the mid points of AB and BC 1
∴ QR || AC and QR = \(\frac { 1 }{ 2 }\) AC
Similarly, in ∆ABH,
Q and P are the mid points of AB and AH
∴ QP || BH or QP || BE
But AC ⊥ BE
∴ QP ⊥ QR
∴ ∠PQR = 90°

Question 16.
ABC is a triangle. D is a point on AB such that AD = \(\frac { 1 }{ 4 }\) AB and E is a point on AC such that AE = \(\frac { 1 }{ 4 }\) AC. Prove that DE = \(\frac { 1 }{ 4 }\) BC.
Solution:
Given : In ∆ABC,
D is a point on AB such that
AD = \(\frac { 1 }{ 4 }\) AB and E is a point on AC such 1
that AE = \(\frac { 1 }{ 4 }\) AC
DE is joined.

To prove : DE = \(\frac { 1 }{ 4 }\) BC
Construction : Take P and Q the mid points of AB and AC and join them
Proof: In ∆ABC,
∵ P and Q are the mid-points of AB and AC

Question 17.
In the figure, ABCD is a parallelogram in which P is the mid-point of DC and Q is a point on AC such that CQ = \(\frac { 1 }{ 4 }\) AC. If PQ produced meets BC at R, prove that R is a mid-point of BC.

Solution:
Given : In ||gm ABCD,
P is the mid-point of DC and Q is a point on AC such that CQ = \(\frac { 1 }{ 4 }\) AC. PQ is produced meets BC at R.

To prove : R is mid point of BC
Construction : Join BD
Proof : ∵ In ||gm ABCD,
∵ Diagonal AC and BD bisect each other at O
∴ AO = OC = \(\frac { 1 }{ 2 }\) AC …(i)
In ∆OCD,
P and Q the mid-points of CD and CO
∴ PQ || OD and PQ = \(\frac { 1 }{ 2 }\) OD
In ∆BCD,
P is mid-poiht of DC and PQ || OD (Proved above)
Or PR || BD
∴ R is mid-point BC.

Question 18.
In the figure, ABCD and PQRC are rectangles and Q is the mid-point of AC.
Prove that (i) DP = PC (ii) PR = \(\frac { 1 }{ 2 }\) AC.

Solution:
Given : ABCD are PQRC are rectangles and Q is the mid-point of AC.
To prove : (i) DP = PC (ii) PR = \(\frac { 1 }{ 2 }\) AC
Construction : Join diagonal AC which passes through Q and join PR.

Proof : (i) In ∆ACD,
Q is mid-point of AC and QP || AD (Sides of rectangles)
∴ P is mid-point of CD
∴ DP = PC
(ii) ∵PR and QC are the diagonals of rectangle PQRC
∴ PR = QC
But Q is the mid-point of AC
∴ QC = \(\frac { 1 }{ 2 }\) AC
Hence PR = \(\frac { 1 }{ 2 }\) AC

Question 19.
ABCD is a parallelogram, E and F are the mid points AB and CD respectively. GFI is any line intersecting AD, EF and BC at Q P and H respectively. Prove that GP = PH.
Solution:
Given : In ||gm ABCD,
E and F are mid-points of AB and CD
GH is any line intersecting AD, EF and BC at GP and H respectively

To prove : GP = PH
Proof: ∵ E and F are the mid-points of AB and CD

Question 20.
BM and CN are perpendiculars to a line passing, through the vertex A of a triangle ABC. If L is the mid-point of BC, prove that LM = LN.
Solution:
In ∆ABC,
BM and CN are perpendicular on a line drawn from A.
L is the mid point of BC.
ML and NL are joined.

Hope given RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.4 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables VSAQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables VSAQS

Other Exercises

• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.1
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.2
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.3
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.4
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables VSAQS
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables MCQS

Question 1.
In a parallelogram ABCD, write the sum of angles A and B.
Solution:
In ||gm ABCD,
∠A + ∠B = 180°
(Sum of consecutive angles of a ||gm)

Question 2.
In a parallelogram ABCD, if ∠D = 115°, then write the measure of ∠A.
Solution:
In ||gm ABCD,
∠D = 115°
But ∠A + ∠D = 180°
(Sum of consecutive angles of a ||gm)

⇒ ∠A + 115°= 180° ∠A = 180°- 115°
∴ ∠A = 65°

Question 3.
PQRS is a square such that PR and SQ intersect at O. State the measure of ∠POQ.
Solution:
In a square PQRS,
Diagonals PR and QS intersects each other at O.

∵ The diagonals of a square bisect each other at right angles.
∴ ∠POQ = 90°

Question 4.
If PQRS is a square then write the measure of ∠SRP.
Solution:
In square PQRS,

Join PR,
∵Diagonals of a square bisect are opposite angles
∴∠SRP = \(\frac { 1 }{ 2 }\)x ∠SRQ
= \(\frac { 1 }{ 2 }\) x 90° = 45°

Question 5.
If ABCD is a rhombus with ∠ABC = 56°, find the measure of ∠ACD.
Solution:
In rhombus ABCD,
Diagonals bisect each other at 0 at right angles.
∠ABC = 56°
But ∠ABC + ∠BCD = 180° (Sum of consecutive angles)
⇒ 56° + ∠BCD = 180°
⇒ ∠BCD = 180° – 56° = 124°
∵ Diagonals of a rhombus bisect the opposite angle
∴ ∠ACD = \(\frac { 1 }{ 2 }\) ∠BCD = \(\frac { 1 }{ 2 }\) x 124°
= 62°

Question 6.
The perimeter of a parallelogram is 22 cm. If the longer side measures 6.5 cm, what is the measure of the shorter side.
Solution:
Perimeter of a ||gm ABCD = 22cm
∴ Sum of two consecutive sides = \(\frac { 22 }{ 2 }\)
= 11cm
i.e. AB + BC = 11 cm
AB = 6.5 cm and let BC = x cm
∴ 6.5 + x = 11 cm
x = 11 – 6.5 = 4.5
∴ Shorter side = 4.5 cm

Question 7.
If the angles of a quadrilateral are in the ratio 3 : 5 : 9 : 13. Then find the measure of the smallest angle.
Solution:
Ratio in the angles of a quadrilateral = 3 : 5 : 9 : 13
Let first angle = 3x
Second angle = 5x
Third angle = 9x
and fourth angle = 13x
∵ The sum of angles of a quadrilateral = 360°
∴ 3x + 5x + 9x + 13x = 360°
⇒ 30x = 360° ⇒ x = \(\frac { { 360 }^{ \circ } }{ 30 }\)  = 12
∴ Smallest angle = 3x = 3 x 12° = 36°

Question 8.
In parallelogram ABCD if ∠A = (3x – 20°), ∠B = (y + 15)°, ∠C = (x + 40°), then find the value of x and y.
Solution:
In a ||gm ABCD,
∠A = (3x – 20°), ∠B = y + 15°,
∠C = x + 40°
Now, ∠A = ∠C (Opposite angles of a ||gm)
⇒ 3x – 20 = x + 40°
⇒ 3x – x = 40° + 20° ⇒ 2x = 60°
⇒ x = \(\frac { { 60 }^{ \circ } }{ 2 }\)  = 30°
and ∠A + ∠B = 180° (Sum of the consecutive angles)
⇒ 3x-20° + y + 15° = 180°
⇒ 3x + y – 5° = 180°
⇒ 3 x 30° +y- 5° = 180°
⇒ 90° – 5° + y = 180
y = 180° – 90° + 5 = 95°
∴ x = 30°, y = 95°

Question 9.
If measures opposite angles of a parallelogram are (60 – x)° and (3x – 4)°, then find the measures of angles of the parallelogram.
Solution:
Opposite angles of a ||gm ABCD are (60 – x)° and (3x – 4°)
But opposite angles of a ||gm are equal, the
60° – x° = 3x – 4° ⇒ 60° + 4° = 3x + x
⇒ 4x = 64° ⇒ x = \(\frac { { 64 }^{ \circ } }{{ 4 }^{ \circ } }\)  = 16°
∴ ∠A = 60° – x = 60° – 16° = 44°
But ∠A + ∠B = 180° (sum of consecutive angle)
⇒ 44° + ∠B = 180°
⇒ ∠B = 180° – 44°
⇒ ∠B = 136°
But ∠A = ∠C and ∠B = ∠D (Opposite angles)
∴ Angles are 44°, 136°, 44°, 136°

Question 10.
In a parallelogram ABCD, the bisectors of ∠A also bisect BC at x, find AB : AD.
Solution:
In ||gm ABCD,
Bisectors of ∠A meets BC at X and BX = XC
Draw XY ||gm AB meeting AD at Y

Question 11.
In the figure, PQRS in an isosceles trapezium find x and y.
Solution:
∵ PQRS is an isosceles trapezium in which
SP = RQ and SR || PQ
∴ ∠P + ∠S = 180° (Sum of co-interior angles)
3x + 2x = 180° ⇒ 5x = 180°
⇒ x = \(\frac { { 180 }^{ \circ } }{ 5 }\)  = 36°

But ∠P = ∠Qm (Base angles of isosceles trapezium)
y = 2x = 2 x 36° = 12°
∴ y = 12°
Hence x = 36°, y = 12°

Question 12.
In the figure ABCD is a trapezium. Find the values of x and y.

Solution:
In trapezium ABCD,
AB || CD

∴ ∠A + ∠D = 180° (Sum of cointerior angles)
x + 20° + 2x + 10° = 180°
3x + 30° = 180°
⇒ 3x= 180° – 30°
3x = 150°
x = \(\frac { { 150 }^{ \circ } }{ 3 }\)  = 50°
Similarly, ∠B + ∠C = 180°
⇒ y + 92° = 180°
⇒ y = 180° – 92° = 88°
∴ x = 50°, y = 88°

Question 13.
In the figure, ABCD and AEFG are two parallelograms. If ∠C = 58°, find ∠F.

Solution:
In the figure, ABCD and AEFG are two parallelograms ∠C = 58°

∵ DC || GF and CB || FE (Sides of ||gms)
∴ ∠C = ∠F
But ∠C = 58°
∴ ∠F = 58°

Question 14.
Complete each of the following statements by means of one of those given in brackets against each:
(i) If one pair of opposite sides are equal and parallel, then the figure is ……… (parallelogram, rectangle, trapezium)
(ii) If in a quadrilateral only one pair of opposite sides are parallel, the quadrilateral is …….. (square, rectangle, trapezium)
(iii) A line drawn from the mid-point of one side of a triangle ………. another side intersects the third side at its mid-point, (perpendicular to, parallel to, to meet)
(iv) If one angle of a parallelogram is a right angle, then it is necessarily a …….. (rectangle, square, rhombus)
(v) Consecutive angle of a parallelogram are ……… (supplementary, complementary)
(vi) If both pairs of opposite sides of a quadrilateral are equal, then it is necessarily a ……… (rectangle, parallelogram, rhombus)
(vii) If opposite angles of a quadrilateral are equal, then it is necessarily a ………. (parallelogram, rhombus, rectangle)
(viii)If consecutive sides of a parallelogram are equal, then it is necessarily a …….. (kite, rhombus, square)
Solution:
(i) If one pair of opposite sides are equal and parallel, then the figure is parallelogram.
(ii) If in a quadrilateral only one pair of opposite sides are parallel, the quadrilateral is trapezium.
(iii) A line drawn from the mid-point of one side of a triangle parallel to another side intersects the third side at its mid-point,
(iv) If one angle of a parallelogram is a right angle, then it is necessarily a rectangle.
(v) Consecutive angle of a parallelogram are supplementary.
(vi) If both pairs of opposite sides of a quadrilateral are equal, then it is necessarily a parallelogram.
(vii) If opposite angles of a quadrilateral are equal, then it is necessarily a parallelogram.
(viii) If consecutive sides of a parallelogram are equal, then it is necessarily a rhombus.

Question 15.
In a quadrilateral ABCD, bisectors of A and B intersect at O such that ∠AOB = 75°, then write the value of ∠C + ∠D.
Solution:
In quadrilateral ABCD,
Bisectors of ∠A and ∠B meet at O and ∠AOB = 75°

In AOB, ∠AOB = 75°
∴ ∠OAB + ∠OBA = 180° – 75° = 105°
But OA and OB are the bisectors of ∠A and ∠B.
∴ ∠A + ∠B = 2 x 105° = 210°
But ∠A + ∠B + ∠C + ∠D = 360° (Sum of angles of a quad.)
∴ 210° + ∠C + ∠D = 360°
⇒ ∠C + ∠D = 360° – 210° = 150°
Hence ∠C + ∠D = 150°

Question 16.
The diagonals of a rectangle ABCD meet at O. If ∠BOC = 44° find ∠OAD.
Solution:
In rectangle ABCD,
Diagonals AC and BD intersect each other at O and ∠BOC = 44°

But ∠AOD = ∠BOC (Vertically opposite angles)
∴ ∠AOD = 44°
In ∆AOD,
∠AOD + ∠OAD + ∠ODA = 180° (Sum of angles of a triangle)
⇒ 44° + ∠OAD + ∠OAD = 180° [∵ OA = OD, ∠OAD = ∠ODA]
⇒ 2∠OAD = 180° – 44° = 136°
∴ ∠OAD = \(\frac { { 136 }^{ \circ } }{ 2 }\)  = 68°

Question 17.
If ABCD is a rectangle with ∠BAC = 32°, find the measure if ∠DBC.
Solution:
In rectangle ABCD,

Diagonals bisect each other at O
∠BAC = 32°
∵ OA = OB
∴ ∠OBA Or ∠DBA = ∠BAC = 32°
But ∠ABC = 90° (Angle of a rectangles)
∴ ∠DBC = ∠ABC – ∠DBA
= 90° – 32° = 58°

Question 18.
If the bisectors of two adjacent angles A and B of a quadrilateral ABCD intersect at a point O. Such that ∠C + ∠D = k(∠AOB), then find the value of k.
Solution:
In quadrilateral ABCD,
Bisectors of ∠A and ∠B meet at O
Such that ∠C + ∠D = k (∠AOB)

Question 19.
In the figure, PQRS is a rhombus in which the diagonal PR is produced to T. If ∠SRT = 152°, find x, y and z.

Solution:
In rhombus PQRS,
Diagonal PR and SQ bisect each other at right angles and PR is produced to T such that ∠SRT = 152°

But ∠SRT + ∠SRP = 180° (Linear pair)
⇒ 152° +∠SRP = 180°
⇒ ∠SRP =180°- 152° = 28°
But ∠SPR = ∠SRP (∵ PR bisects ∠P and ∠R)
⇒ z = 28°
y = 90° (∵ Diagonals bisect each other at right angles)
∠RPQ = z = 28°
∴ In ∆POQ,
z + x = 90° ⇒ 28° + x = 90°
⇒ x = 90° – 28° = 62°
∴ x = 62°, y = 90°, z = 28°

Question 20.
In the figure, ABCD is a rectangle in which diagonal AC is produced to E. If ∠ECD = 146°, find ∠AOB.

Solution:
In rectangle ABCD,
Diagonals AC and BD bisect each other at O
AC is produced to E and ∠DCE = 146°

∠DCE + ∠DCA = 180° (Linear pair)
⇒ 146°+ ∠DCA= 180°
⇒ ∠DCA = 180°- 146°
⇒ ∠DCA = 34°
∴ ∠CAB = ∠DCA (Alternate angles)
= 34°
Now in ∆AOB,
∠AOB = 180° – (∠DAB + ∠OBA)
= 180° – (34° + 34°)
= 1803 – 68° = 112°

Hope given RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables VSAQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.1

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.1

Other Exercises

• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.1
• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.2
• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.3
• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals VSAQS
• RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals MCQS

Question 1.
Which of the following figures lie on the same base and between the same parallels. In such a case, write the common base and two parallels: [NCERT]

Solution:
(i) ΔPCD and trapezium ABCD are on the same base CD and between the same parallels AB and DC.
(ii) Parallelograms ABCD and APQD are on the same base AD and between the same parallels AD and BQ.
(iii) Parallelogram ABCD and ΔPQR are between the same parallels AD and BC but they are not on the same base.
(iv) ΔQRT and parallelogram PQRS are on the same base QR and between the same parallels QR and PS.
(v) Parallelogram PQRS and trapezium SMNR on tire same base SR but they are not between the same parallels.
(vi) Parallelograms PQRS, AQRD, BCQR are between the same parallels. Also, parallelograms PQRS, BPSC, and APSD are between the same parallels.

 

Hope given RD Sharma Class 9 Solutions Chapter 14 Quadrilaterals Ex 14.1 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.3

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.3

Other Exercises

• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.1
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.2
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.3
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.4
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables VSAQS
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables MCQS

Question 1.
In a parallelogram ABCD, determine the sum of angles ZC and ZD.
Solution:
In a ||gm ABCD,
∠C + ∠D = 180°
(Sum of consecutive angles)

Question 2.
In a parallelogram ABCD, if ∠B = 135°, determine the measures of its other angles.
Solution:
In a ||gm ABCD, ∠B = 135°

∴ ∠D = ∠B = 135° (Opposite angles of a ||gm)
But ∠A + ∠B = 180° (Sum of consecutive angles)
⇒ ∠B + 135° = 180°
∴ ∠A = 180° – 135° = 45°
But∠C = ∠B = 45° (Opposite angles of a ||gm)
∴ Angles are 45°, 135°, 45°, 135°.

Question 3.
ABCD is a square, AC and BD intersect at O. State the measure of ∠AOB.
Solution:
In a square ABCD,
Diagonal AC and BD intersect each other at O
∵ Diagonals of a square bisect each other at right angle
∵∠AOB = 90°

Question 4.
ABCD is a rectangle with ∠ABD = 40°. Determine ∠DBC.
Solution:
In rectangle ABCD,

∠B = 90°, BD is its diagonal
But ∠ABD = 40°
and ∠ABD + ∠DBC = 90°
⇒ 40° + ∠DBC = 90°
⇒ ∠DBC = 90° – 40° = 50°
Hence ∠DBC = 50°

Question 5.
The sides AB and CD of a parallelogram ABCD are bisected at E and F. Prove that EBFD is a parallelogram.
Solution:
Given : In ||gm ABCD, E and F are the mid points of the side AB and CD respectively
DE and BF are joined
To prove : EBFD is a ||gm
Construction : Join EF

Proof : ∵ ABCD is a ||gm
∴ AB = CD and AB || CD
(Opposite sides of a ||gm are equal and parallel)
∴ EB || DF and EB = DF (∵ E and F are mid points of AB and CD)
∴ EBFD is a ||gm.

Question 6.
P and Q are the points of trisection of the diagonal BD of the parallelogram ABCD. Prove that CQ is parallel to AP. Prove also that AC bisects PQ.
Solution:
Given : In ||gm, ABCD. P and Q are the points of trisection of the diagonal BD

To prove : (i) CQ || AP
AC bisects PQ
Proof: ∵ Diagonals of a parallelogram bisect each other
∴ AO = OC and BO = OD
∴ P and Q are point of trisection of BD
∴ BP = PQ = QD …(i)
∵ BO = OD and BP = QD …(ii)
Subtracting, (ii) from (i) we get
OB – BP = OD – QD
⇒ OP = OQ
In quadrilateral APCQ,
OA = OC and OP = OQ (proved)
Diagonals AC and PQ bisect each other at O
∴ APCQ is a parallelogram
Hence AP || CQ.

Question 7.
ABCD is a square. E, F, G and H are points on AB, BC, CD and DA respectively, such that AE = BF = CG = DH. Prove that EFGH is a square.
Solution:
Given : In square ABCD
E, F, G and H are the points on AB, BC, CD and DA respectively such that AE = BF = CG = DH
To prove : EFGH is a square
Proof : E, F, G and H are points on the sides AB, BC, CA and DA respectively such that
AE = BF = CG = DH = x (suppose)
Then BE = CF = DG = AH = y (suppose)
Now in ∆AEH and ∆BFE

AE = BF (given)
∠A = ∠B (each 90°)
AH = BE (proved)
∴ ∆AEH ≅ ∆BFE (SAS criterion)
∴ ∠1 = ∠2 and ∠3 = ∠4 (c.p.c.t.)
But ∠1 + ∠3 = 90° and ∠2 + ∠4 = 90° (∠A = ∠B = 90°)
⇒ ∠1 + ∠2 + ∠3 + ∠4 = 90° + 90° = 180°
⇒ ∠1 + ∠4 + ∠1 + ∠4 = 180°
⇒ 2(∠1 + ∠4) = 180°
⇒ ∠1 + ∠4 = \(\frac { { 180 }^{ \circ } }{ 2 }\)  = 90°
∴ ∠HEF = 180° – 90° = 90°
Similarly, we can prove that
∠F = ∠G = ∠H = 90°
Since sides of quad. EFGH is are equal and each angle is of 90°
∴ EFGH is a square.

Question 8.
ABCD is a rhombus, EABF is a straight line such that EA = AB = BF. Prove that ED and FC when produced meet at right angles.
Solution:
Given : ABCD is a rhombus, EABF is a straight line such that
EA = AB = BF
ED and FC are joined
Which meet at G on producing

To prove: ∠EGF = 90°
Proof : ∵ Diagonals of a rhombus bisect
each other at right angles
AO = OC, BO = OD
∠AOD = ∠COD = 90°
and ∠AOB = ∠BOC = 90°
In ∆BDE,
A and O are the mid-points of BE and BD respectively.
∴ AO || ED
Similarly, OC || DG
In ∆ CFA, B and O are the mid-points of AF and AC respectively
∴ OB || CF and OD || GC
Now in quad. DOCG
OC || DG and OD || CG
∴ DOCG is a parallelogram.
∴ ∠DGC = ∠DOC (opposite angles of ||gm)
∴ ∠DGC = 90° (∵ ∠DOC = 90°)
Hence proved.

Question 9.
ABCD is a parallelogram, AD is produced to E so that DE = DC = AD and EC produced meets AB produced in F. Prove that BF = BC.
Solution:
Given : In ||gm ABCD,
AB is produced to E so that
DE = DA and EC produced meets AB produced in F.
To prove : BF = BC
Proof: In ∆ACE,

O and D are the mid points of sides AC and AE
∴ DO || EC and DB || FC
⇒ BD || EF
∴ AB = BF
But AB = DC (Opposite sides of ||gm)
∴ DC = BF
Now in ∆EDC and ∆CBF,
DC = BF (proved)
∠EDC = ∠CBF
(∵∠EDC = ∠DAB corresponding angles)
∠DAB = ∠CBF (corresponding angles)
∠ECD = ∠CFB (corresponding angles)
∴ AEDC ≅ ACBF (ASA criterion)
∴ DE = BC (c.p.c.t.)
⇒ DC = BC
⇒ AB = BC
⇒ BF = BC (∵AB = BF proved)
Hence proved.

Hope given RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.3 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables MCQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables MCQS

Other Exercises

• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.1
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.2
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.3
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables Ex 13.4
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables VSAQS
• RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables MCQS

Mark the correct alternative in each of the following:
Question 1.
The opposite sides of a quadrilateral have
(a) no common point
(b) one common point
(c) two common points
(d) infinitely many common points
Solution:
The opposite sides of a quadrilateral have no common point. (a)

Question 2.
The consecutive sides of a quadrilateral have
(a) no common point
(b) one common point
(c) two common points
(d) infinitely many common points
Solution:
The consecutive sides of a quadrilateral have one common point. (b)

Question 3.
PQRS is a quadrilateral, PR and QS intersect each other at O. In which of the following cases, PQRS is a parallelogram?
(a) ∠P = 100°, ∠Q = 80°, ∠R = 100°
(b) ∠P = 85°, ∠Q = 85°, ∠R = 95°
(c) PQ = 7 cm, QR = 7 cm, RS = 8 cm, SP = 8 cm
(d) OP = 6.5 cm, OQ = 6.5 cm, OR = 5.2 cm, OS = 5.2 cm
Solution:
PQRS is a quadrilateral, PR and QS intersect each other at O. PQRS is a parallelogram if ∠P = 100°, ∠Q = 80°, ∠R = 100° (a)

Question 4.
Which of the following quadrilateral is not a rhombus?
(a) All four sides are equal
(b) Diagonals bisect each other
(c) Diagonals bisect opposite angles
(d) One angle between the diagonals is 60°
Solution:
A quadrilateral is not a rhombus if one angle between the diagonals is 60°. (d)

Question 5.
Diagonals necessarily bisect opposite angles in a
(a) rectangle
(b) parallelogram
(c) isosceles trapezium
(d) square
Solution:
Diagonals necessarily bisect opposite angles in a square. (d)

Question 6.
The two diagonals are equal in a
(a) parallelogram
(b) rhombus
(c) rectangle
(d) trapezium
Solution:
The two diagonals are equal in a rectangle. (c)

Question 7.
We get a rhombus by joining the mid-points of the sides of a
(a) parallelogram
(b) rhombus
(c) rectangle
(d) triangle
Solution:
We get a rhombus by joining the mid points of the sides of a rectangle. (c)

Question 8.
The bisectors of any two adjacent angles of a parallelogram intersect at
(a) 30°
(b) 45°
(c) 60°
(d) 90°
Solution:
The bisectors of any two adjacent angles of a parallelogram intersect at 90°. (d)

Question 9.
The bisectors of the angle of a parallelogram enclose a
(a) parallelogram
(b) rhombus
(c) rectangle
(d) square
Solution:
The bisectors of the angles of a parallelogram enclose a rectangle. (c)

Question 10.
The figure formed by joining the mid-points of the adjacent sides of a quadrilateral is a
(a) parallelogram
(b) rectangle
(c) square
(d) rhombus
Solution:
The figure formed by joining the mid-points of the adjacent sides of a quadrilateral is a parallelogram. (a)

Question 11.
The figure formed by joining the mid-points of the adjacent sides of a rectangle is a
(a) square
(b) rhombus
(c) trapezium
(d) none of these
Solution:
The figure formed by joining the mid-points of the adjacent sides of a rectangle is a rhombus. (b)

Question 12.
The figure formed by joining the mid-points of the adjacent sides of a rhombus is a
(a) square
(b) rectangle
(c) trapezium
(d) none of these
Solution:
The figure formed by the joining the mid-points of the adjacent sides of a rhombus is a rectangle. (b)

Question 13.
The figure formed by joining the mid-points of the adjacent sides of a square is a
(a) rhombus
(b) square
(c) rectangle
(d) parallelogram
Solution:
Tire figure formed by joining the mid-points of the adjacent sides of a square is a square. (b)

Question 14.
The figure formed by joining the mid-points of the adjacent sides of a parallelogram is a
(a) rectangle
(b) parallelogram
(b) rhombus
(d) square
Solution:
The figure formed by joining the mid-points of the adjacent sides of a parallelogram is a parallelogram. (b)

Question 15.
If one angle of a parallelogram is 24° less than twice the smallest angle, then the measure of the largest angle of the parallelogram is
(a) 176°
(b) 68°
(c) 112°
(d) 102°
Solution:
Let the smallest angle be x
The largest angle = 2x – 24°
But sum of two adjacent angles = 180°

Question 16.
In a parallelogram ABCD, If ∠DAB = 75° and ∠DBC = 60°, then ∠BDC =
(a) 75°
(b) 60°
(c) 45°
(d) 55°
Solution:
In ||gm ABC,

∠A = 75°, ∠DBC = 60°
But ∠A + ∠B = 180° (Sum of two consecutive angles)
⇒ 75° + ∠B = 180°
⇒ ∠B = 180°- 75“= 105°
But ∠DBC = 60°
∴ ∠DBA = 105°-60° = 45°
But ∠BDC = ∠DBA (Alternate angles)
∴ ∠BDC = 45° (c)

Question 17.
ABCD is a parallelogram and E and F are the centroids of triangles ABD and BCD respectively, then EF =
(a) AE
(b) BE
(c) CE
(d) DE
Solution:
In ||gm ABCD, BD is joined forming two triangles ABD and BCD

E and F are the centroid of ∆ABD and ∆BCD
Now E and F trisect AC
i.e. AE = EF = FC
∴ EF = AE (a)

Question 18.
ABCD is a parallelogram, M is the mid¬point of BD and BM bisects ∠B. Then, ∠AMB =
(a) 45°
(b) 60°
(c) 90°
(d) 75°
Solution:
In ||gm ABCD, M is mid-point of BD and
BM bisects ∠B
AM is joined

∴AM bisects ∠A
But ∠A + ∠B = 180° (Sum of two consecutive angles)
∴ ∠AMB = 90° (c)

Question 19.
If an angle of a parallelogram is two-third of its adjacent angle, the smallest angle of the parallelogram is
(a) 108°
(b) 54°
(c) 12°
(d) 81°
Solution:
Let adjacent angle of a ||gm = x
Then second angle = \(\frac { 2 }{ 3 }\) x
∴ x+ \(\frac { 2 }{ 3 }\) x= 180°
(Sum of two adjacent angles of a ||gm is 180°)

Question 20.
If the degree measures of the angles of quadrilateral are Ax, lx, 9x and 10JC, what is the sum of the measures of the smallest angle and largest angle?
(a) 140°
(b) 150°
(c) 168°
(d) 180°
Solution:
Sum of the angles of a quadrilateral = 360°
∴ 4x + 1x + 9x + 10x = 360°
⇒ 30x = 360°
⇒ x = \(\frac { { 360 }^{ \circ } }{ 30 }\)  = 12°
Now sum of smallest and largest angle = 4 x 12° + 10 x 12°
= 48° + 120° = 168° (c)

Question 21.
If the diagonals of a rhombus are 18 cm and 24 cm respectively, then its side is equal to
(a) 16 cm
(b) 15 cm
(c) 20 cm
(d) 17 cm
Solution:
Diagonals of a rhombus are 18 cm and 24 cm But diagonals of a rhombus bisect each other at right angles

Question 22.
ABCD is a parallelogram in which diagonal AC bisects ∠BAD. If ∠BAC = 35°, then ∠ABC =
(a) 70°
(b) 110°
(c) 90°
(d) 120°
Solution:
In ||gm ABCD, AC is its diagonal which bisect ∠BAD

∠BAD = 35°
∴ ∠BAD = 2 x 35° = 70°
But ∠A + ∠B = 180° (Sum of consecutive angles)
⇒ 70° + ∠B = 180°⇒ ∠B = 180° – 70°
∴ ∠B = 110°
⇒ ABC = 110° (b)

Question 23.
In a rhombus ABCD, if ∠ACB = 40°, then ∠ADB =
(a) 70°
(b) 45°
(c) 50°
(d) 60°
Solution:
In rhombus ABCD, ∠ACB = 40°

∴ ∠BCD = 2 x ∠ACB
= 2 x 40° = 80°
But ∠BCD + ∠ADC = 180° (Sum of consecutive angles of ||gm)
⇒ 80° + ∠ADC = 180°
⇒ ∠ADC = 180° – 80° = 100°
∴ ∠ADB = \(\frac { 1 }{ 2 }\)∠ADC = \(\frac { 1 }{ 2 }\)x 100° = 50° (c)

Question 24.
In ∆ABC, ∠A = 30°, ∠B = 40° and ∠C = 110°. The angles of the triangle formed by joining the mid-points of the sides of this triangle are
(a) 70°, 70°, 40°
(b) 60°, 40°, 80°
(c) 30°, 40°, 110°
(d) 60°, 70°, 50°
Solution:
In ∆ABC,

∠A = 30°, ∠B = 40°, ∠C = 110°
D, E and F are mid-points of the sides of the triangle. By joining them in order,
DEF is a triangle formed
Now BDEF, CDFE and AFDE are ||gms
∴ ∠A = ∠D = 30°
∠B = ∠E = 40°
∠C = ∠F= 110°
∴ Angles are 30°, 40°, 110° (c)

Question 25.
The diagonals of a parallelogram ABCD intersect at O. If ∠BOC = 90° and ∠BDC = 50°, then ∠OAB =
(a) 40°
(b) 50°
(c) 10°
(d) 90°
Solution:
In ||gm ABCD, diagonals AC and BD intersect each other at O

BOC = 90°, ∠BDC = 50°
∵ ∠BOC = 90°
∴ Diagonals of ||gm bisect each other at 90°
∴∠COD = 90°
In ∆COD,
∠OCD = 90° – 50° = 40°
But ∠OAB = ∠OCD (Alternate angles)
∴∠OAB = 40° (a)

Question 26.
ABCD is a trapezium in which AB || DC. M and N are the mid-points of AD and BC respectively. If AB = 12 cm, MN = 14 cm, then CD =
(a) 10 cm
(b) 12 cm
(c) 14 cm
(d) 16 cm
Solution:
In trapezium AB || DC
M and N are mid-points of sides AD and BC and MN are joined
AB = 12 cm, MN = 14 cm

∵ MN = \(\frac { 1 }{ 2 }\)(AB + CD)
⇒ 2MN = AB + CD
⇒ 2 x 14 = 12 + CD
CD = 2 x 14 – 12 = 28 – 12 = 16 cm (d)

Question 27.
Diagonals of a quadrilateral ABCD bisect each other. If ∠A = 45°, then ∠B =
(a) 115°
(b) 120°
(c) 125°
(d) 135°
Solution:
Diagonals AC and BD of quadrilateral ABCD bisect each other at O

∴ AO = OC, BO = OD
∴ ABCD is a ||gm ∠A = 45°
But ∠A + ∠B = 180° (Sum of consecutive angles)
∴ ∠B = 180° – ∠A = 180° – 45°
= 135° (d)

Question 28.
P is the mid-point of side BC of a paralleogram ABCD such that ∠BAP = ∠DAP. If AD = 10 cm, then CD =
(a) 5 cm
(b) 6 cm
(c) 8 cm
(d) 10 cm
Solution:
In ||gm ABCD, P is mid-point of BC
AD = 10cm

∠BAP = ∠DAP
Through P, draw PQ || AB
∴ ABPQ is rhombus
∴ AB = BP = AQ
= \(\frac { 1 }{ 2 }\) AB = \(\frac { 1 }{ 2 }\) x 10 = 5 cm
But CD = AB (Opposite sides of ||gm)
∴ CD = 5 cm (a)

Question 29.
In ∆ABC, E is the mid-point of median AD such that BE produced meets AC at E If AC = 10.5 cm, then AF =
(a) 3 cm
(b) 3.5 cm
(c) 2.5 cm
(d) 5 cm
Solution:
In ∆ABC, E is the mid-point of median AD
Such that BE produced meets AC at F
AC = 10.5 cm
Draw DG || AF

In ∆ADG
E is mid-point of AD and EF || DG
∴ F is mid-point of AG
⇒ AF = FG …(i)
In ∆BCF
D is mid-point of BC and DG || BF
∴ G is mid-point of FC
∴ FG = GC …(i)
From (i) and (ii)
AF = FG = GC = \(\frac { 1 }{ 3 }\) AC
But AC = 10.5 cm
∴ AF = \(\frac { 1 }{ 3 }\) AC = \(\frac { 1 }{ 3 }\) x 10.5 = 3.5 cm (b)

Question 30.
ABCD is a parallelogram and E is the mid-point of BC. DE and AB when produced meet at F. Then, AF =

Solution:
In ||gm ABCD, E is mid-point of BC DE and AB are produced to meet at F

∵ E is mid point of BC
∴ BE = EC
In ∆BEF and ∆CDE
BE = EC
∠BEF = ∠CED (Vertically opposite angle)
and ∠EBF = ∠ECD (Alternate angles)
∴ ∆BEF ≅ ∆CDE (ASA criterian)
∴ DC = BF
But DC = AB
∴ AB = BF
AF = AB + BF = AB + AB
= 2AB (b)

Question 31.
In a quadrilateral ABCD, ∠A + ∠C is 2 times ∠B + ∠D. If ∠A = 140° and ∠D = 60°, then ∠B =
(a) 60°
(b) 80°
(c) 120°
(d) None of these
Solution:
In quadrilateral ABCD
⇒ ∠A + ∠C = 2(∠B + ∠D)
⇒ ∠A + ∠C = 2∠B + 2∠D
Adding 2∠A + 2∠C both sides
2∠A + 2∠C + ∠A + ∠C = 2∠A + 2∠B + 2∠C + 2∠D
⇒ 3∠A + 3∠C = 2(∠A + ∠B + ∠C + ∠D)
⇒ 3(∠A + ∠C) = 2 x 360° = 720°
∴ ∠A + ∠C = \(\frac { { 720 }^{ \circ } }{ 3 }\)  = 240°
⇒ 40° + ∠C = 240° (∵ ∠A = 40°)
∠C = 240° – 40° = 200°
Now 2(∠B + ∠D) = ∠A + ∠C = 240°
∠B + ∠D = \(\frac { { 240 }^{ \circ } }{ 2 }\)  = 120°
∴ ∠B = 60° = 120°
∴ ∠B = 60° (a)

Question 32.
The diagonals AC and BD of a rectangle ABCD intersect each other at P. If ∠ABD = 50°, then ∠DPC =
(a) 70°
(b) 90°
(c) 80°
(d) 100°
Solution:
In rectangle ABCD, diagonals AC and BD intersect each other at P

∠ABD = 50°
∴ ∠CAB = ∠ABD = 50° (∵ AP = BP)
Now in ∆APB
∠CAB + ∠ABD + ∠APB = 180° (Angles of a triangle)
⇒ ∠PAB + ∠PBA + ∠APB = 180°
⇒ 50° + 50° + ∠APB = 180°
⇒ ∠APB = 180° – 50° – 50° = 80°
But ∠DPC = ADB (Vertically opposite angles)
∴ ∠DPC = 80° (c)

Hope given RD Sharma Class 9 Solutions Chapter 13 Linear Equations in Two Variables MCQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.