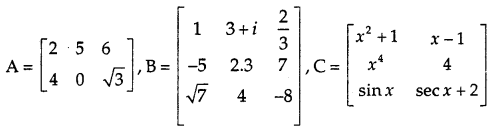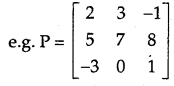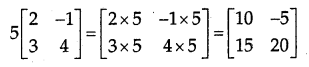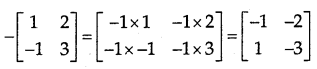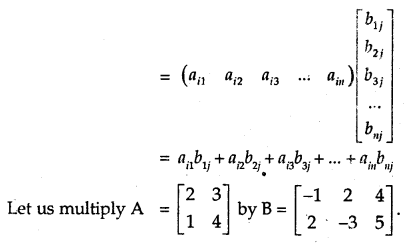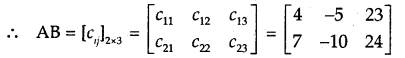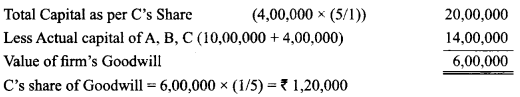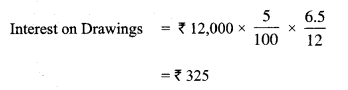Category: CBSE Class 12

By going through these CBSE Class 12 Maths Notes Chapter 4 Determinants, students can recall all the concepts quickly.

Determinants Notes Class 12 Maths Chapter 4

DETERMINANT:
Def.: Let A = [aij]n×n be a matrix of order n × n or simply as of order n. Now we can associate each square matrix with a unique number (real or complex). If M is a set of matrices and K is the set of real or complex numbers, then
f: M → K
or
f(A) = k, when A ∈ M and k ∈ K, which is written as
f(A) = | A | = det (A) = k.

Expansions of Determinants:
→ Determinant of order 1
Let A =[a]. Then, det A = a or | a | = a.

→ Determinant of order 2
Let A = \(\left[\begin{array}{ll}
a & b \\
c & d
\end{array}\right]\) is a matrix or order 2 × 2

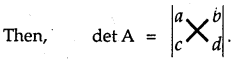
Multiply the elements along the arrow, the products are written with alternate sign +, -, i.e.,
\(\left|\begin{array}{ll}
a & b \\
c & d
\end{array}\right|\) = (ad – bc)
Product with element a₁₁ = a and, a₂₂ = d is taken positive.

→ Determinant of order n
Let the determinant be

To expand this determinant, we take the following steps:

1. Take up the elements of a row (or column). Let it be in ith a row. Its elements are a₁₁, a₂₂,…, a_ij…, ai_in.
2. Corresponding to element a_ij we find a determinant M_ij, which is obtained by deleting the elements of ith row and jth column. The determinant Mij is called the minor of a_ij.
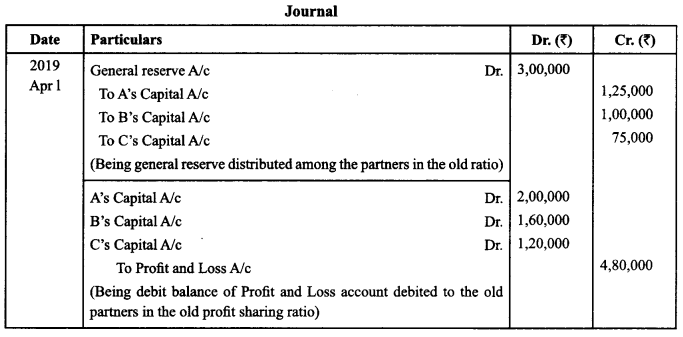
3. Sign of the product is (-1)^i+j. Thus, the expansion with the help of ith row = (-1)ⁱ⁺¹ a_i1 M_i1 + (-1)ⁱ⁺² a_i2 M_i2 +………… + (-1)^+j a_ij M_ij + ….. + (-1)i+n a_in M_in.

Now, consider the expansion of a determinant of third order,
i.e., \(\left|\begin{array}{lll}
a_{11} & a_{12} & a_{13} \\
a_{21} & a_{22} & a_{23} \\
a_{31} & a_{32} & a_{33}
\end{array}\right|\)

1. The elements in the first row are a₁₁, a₁₂ and a₁₃.
2. M₁₁ is obtained by deleting 1st row and 1st column.
   

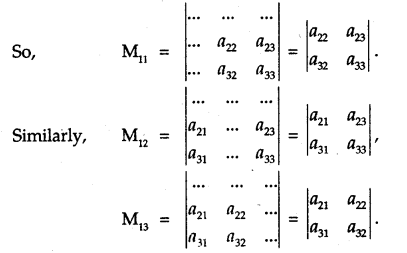
Adding the product elements and corresponding determinants with proper sign (-1)^i+j, we get the expansion of the determinant

The same result is obtained by taking the element of any other row or column. Similarly, the determinants of higher order may be expanded.

Properties of Determinants:
Property 1: If the rows and columns of a determinant are interchanged, the value of the determinant remains the same.
Thus, \(\left|\begin{array}{lll}
a_{1} & b_{1} & c_{1} \\
a_{2} & b_{2} & c_{2} \\
a_{3} & b_{3} & c_{3}
\end{array}\right|=\left|\begin{array}{lll}
a_{1} & a_{2} & a_{3} \\
b_{1} & b_{2} & b_{3} \\
c_{1} & c_{2} & c_{3}
\end{array}\right|\).

Property 2: If any two rows or columns of a determinant are interchanged, then sign of the determinant is changed.
Thus, \(-\left|\begin{array}{lll}
a_{2} & b_{2} & c_{2} \\
a_{1} & b_{1} & c_{1} \\
a_{3} & b_{3} & c_{3}
\end{array}\right|=\left|\begin{array}{lll}
a_{1} & b_{1} & c_{1} \\
a_{2} & b_{2} & c_{2} \\
a_{3} & b_{3} & c_{3}
\end{array}\right|\).

Property 3: If any two rows (columns) of a determinant are identical, the value of the determinant is zero.
Thus, \(\left|\begin{array}{lll}
a_{1} & a_{2} & a_{3} \\
a_{1} & a_{2} & a_{3} \\
b_{1} & b_{2} & b_{3}
\end{array}\right|\) = 0

Property 4: If each element of a row or column of a determinant is multiplied by a constant k, then its value is k times the given determinant.
Thus, \(\left|\begin{array}{lll}
k a_{1} & k a_{2} & k a_{3} \\
b_{1} & b_{2} & b_{3} \\
c_{1} & c_{2} & c_{3}
\end{array}\right|=k\left|\begin{array}{lll}
a_{1} & a_{2} & a_{3} \\
b_{1} & b_{2} & b_{3} \\
c_{1} & c_{2} & c_{3}
\end{array}\right|\).

Property 5: If the element of a row or column of a determinant are expressed as sum of two (or more terms), then the determinant can be expressed as sum of two (or more) determinants.

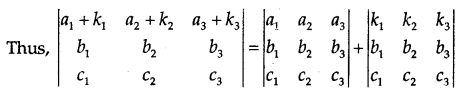
Property 6: If to each element of any row or column of a determinant, the equimultiples of corresponding elements of any other row or column are added, then the value of the determinant remains unchanged.

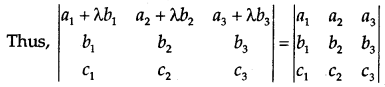
Area of a Triangle:
The area of a triangle whose vertices are (x₁, y₁), (x₂, y₂) and (x₃, y₃) is equal to \(\frac{1}{2}\) \(\left|\begin{array}{lll}
x_{1} & y_{1} & 1 \\
x_{2} & y_{2} & 1 \\
x_{3} & y_{3} & 1
\end{array}\right|\)

It may be noted:

• The area is positive. So, take the only absolute value.
• If the three points are collinear, the area of a triangle is taken as zero.

→ Minor of a determinant: In a determinant Δ, the minor of a_ij is obtained by deleting the ith row and jth column.
e.g. Minor of a₂₁ of \(\left|\begin{array}{lll}
a_{11} & a_{12} & a_{13} \\
a_{21} & a_{22} & a_{23} \\
a_{31} & a_{32} & a_{33}
\end{array}\right|\)

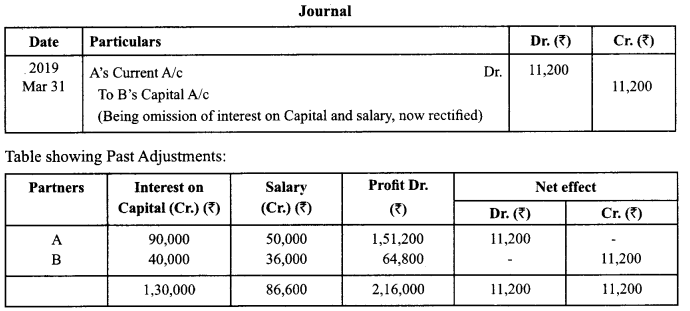
= M₂₁ = \(\left|\begin{array}{ccc}
\ldots & a_{12} & a_{13} \\
\ldots & \ldots & \ldots \\
\ldots & a_{32} & a_{33}
\end{array}\right|=\left|\begin{array}{ll}
a_{12} & a_{13} \\
a_{32} & a_{33}
\end{array}\right|\)

Co-factor of an element of Determinant:
Co-factor of an element a., of determinant | a_ij |
= (-1)^i+j M_ij where M_ij is the minor of a_ij.

In det. \(\left|\begin{array}{lll}
a_{11} & a_{12} & a_{13} \\
a_{21} & a_{22} & a_{23} \\
a_{31} & a_{32} & a_{33}
\end{array}\right|\), cofactor of a31 = (-1)3+1M31 = \(\left|\begin{array}{ll}
a_{12} & a_{13} \\
a_{22} & a_{23}
\end{array}\right|\)

→ Matrix of cofactors: By replacing the elements of a determinant with their cofactors, a matrix of cofactors is obtained.

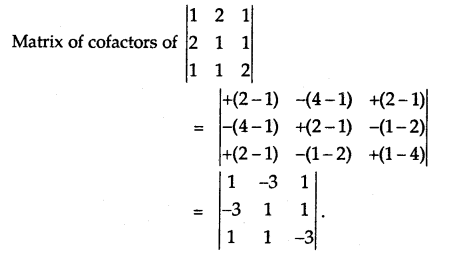
→ Adjoint of a Matrix: The adjoint of a square matrix is the transpose of the matrix of cofactors.
If A_ij, is the cofactor of a., of det. A = | a_ij |, then

→ Singular Matrix: If | A | =0, the square matrix A is said to be singular.

→ Non-singular Matrix: If | A | ≠ 0, the square matrix A is known as a non-singular matrix.

→ Invertible Matrix: If AB = BA = I, then A is called the inverse of A which is written as B = A^-1. In this case, the square matrix A is said to be invertible.

Some Theorems:

1. If A is a square matrix, then A (adj A) = (adj A) A = AI.
2. If A and B are non-singular matrices, then AB and BA are also non-singular matrices.
3. | AB | = | A | | B |.
4. A square matrix A is invertible, if and only if A is non-singular.
5. A^-1 = \(\frac{1}{|A|}\) adj A.
6. (AB)^-1 = B^-1A^-1.
7. (a) (A’)^-1 .= (A^-1)’.
   (b) (A^-1)^-1 = A.
   (c) (XYZ)^-1 = Z^-1 Y^-1 X^-1.

Linear System of Equations:
→ Consistent system: The system of equations is said to be consistent, if it has one or more than one solutions.

→ Inconsistent system: The system of equations is said to be inconsistent, if it has no solution.
Consider the system of equations:
a₁x + b₁y + c₁z = d₁
a₂x + b₂y + c₂z = d₂
a₃x + b₃y + c₃z = d₃

Let A = \(\left[\begin{array}{lll}
a_{1} & b_{1} & c_{1} \\
a_{2} & b_{2} & c_{2} \\
a_{3} & b_{3} & c_{3}
\end{array}\right]\), X = \(\left[\begin{array}{l}
x \\
y \\
z
\end{array}\right]\) and B = \(\left[\begin{array}{l}
d_{1} \\
d_{2} \\
d_{3}
\end{array}\right]\)

The given system of equations can be written is
\(\left[\begin{array}{lll}
a_{1} & b_{1} & c_{1} \\
a_{2} & b_{2} & c_{2} \\
a_{3} & b_{3} & c_{3}
\end{array}\right]\left[\begin{array}{l}
x \\
y \\
z
\end{array}\right]=\left[\begin{array}{l}
d_{1} \\
d_{2} \\
d_{3}
\end{array}\right]\)
or
AX = B.
∴ X = A-1B.

Consistency/Inconsistency of system cf equations:
(a) For a non-homogeneous system of equations AX ≠ O:

1. if | A | ≠ 0, AX = B has a unique solution.
2. If | A | = 0, let us find (adj A) B.
3. If (adj A)B ≠ 0, the system of equations is inconsistent.
4. If (adj A)B = 0, the system of equations has infinitely many solutions and hence consistent.

(b) For the homogeneous system of equations AX = O:

1. If | A | ≠ 0, the solution is x = 0, y = 0, z = 0. This is called the trivial solution. The system is consistent.
2. If | A | = 0, the system has infinitely many solutions. The system is consistent.

In such as case, we put one of the variables equal to k. Let z = k, then we find the values of x and y in terms of k.

1. DETERMINANT OF A SQUARE MATRIX

(i) If A = \(\left[\begin{array}{ll}
a_{11} & a_{12} \\
a_{21} & a_{22}
\end{array}\right]\), then det. A = \(\left|\begin{array}{ll}
a_{11} & a_{12} \\
a_{21} & a_{22}
\end{array}\right|\) = a₁₁a₂₂ – a₂₁a₁₂

(ii) If A = \(\left[\begin{array}{lll}
a_{11} & a_{12} & a_{13} \\
a_{21} & a_{22} & a_{23} \\
a_{31} & a_{32} & a_{33}
\end{array}\right]\), then det. A = a₁₁\(\left|\begin{array}{ll}
a_{22} & a_{23} \\
a_{32} & a_{33}
\end{array}\right|\) – a₁₂ \(\left|\begin{array}{ll}
a_{21} & a_{23} \\
a_{31} & a_{33}
\end{array}\right|\) + a₁₃ \(\left|\begin{array}{ll}
a_{21} & a_{22} \\
a_{31} & a_{32}
\end{array}\right|\)
= a₁₁a₂₂a₃₃ – a₂₃a₃₂a₁₁ – a₁₂a₂₁a₃₃ + a₁₂a₂₃a₁₁ + a₁₃a₂₁a₃₂ – a₁₃a₃₁a₂₂.

2. MINOR AND CO-FACTOR
(i) The minor of an element a_ij is a determinant, which is obtained by supressing die ith row and jth column. The minor of an element a_ij is denoted by M_ij.

(ii) The co-factor of an element is its minor with proper sign. The co-factor of an element a_ij is denoted by A_ij
A_ij =(-1)^i+jM_ij

3. PROPERTIES

(i) Reflection Property. The value of the determinant remains unaltered by interchanging its rows and columns.
(ii) Switching Property. If two adjacent rows (or columns) of a determinant are interchanged, then the sign of the determinant is changed.
(iii) Repetition Property. If two rows (or columns) of a determinant are identical, then its value is zero.
(iv) Scalar Multiple Property. If each element of a row (or column) of a determinant is multiplied f
by a constant ‘k’ then its value gets multiplied by the scalar ‘k’
(v) Sum Property. If each element of a row (or column) of a determinant is expressed as the sum
of two or more terms, then the determinant can be expressed as the sum of two or more determinants.
(vi) Invariance Property. If to any row (or column) of a determinant, a multiple of another row (or column) is added, the value of the determinant remains the same.
(vii) Factor Property. If a determinant Δ vanishes when for x is put a in those elements of Δ, which are polynomials in x, then (x – a) is a factor of Δ.

4. AREA OF A TRIANGLE

Area of a triangle whose vertices are (x₁, y₁), (x₂, y₂), (x₃, y₃) is given by:
D = \(\frac{1}{2}\left|\begin{array}{lll}
x_{1} & y_{1} & 1 \\
x_{2} & y_{2} & 1 \\
x_{3} & y_{3} & 1
\end{array}\right|\)
When the area of the triangle is zero, then the points are collinear.

5. ADJOINT OF A MATRIX

Let A = \(\left[\begin{array}{lll}
a_{11} & a_{12} & a_{13} \\
a_{21} & a_{22} & a_{23} \\
a_{31} & a_{32} & a_{33}
\end{array}\right]\), then adj, A = \(\left[\begin{array}{lll}
\mathrm{A}_{11} & \mathrm{~A}_{21} & \mathrm{~A}_{31} \\
\mathrm{~A}_{12} & \mathrm{~A}_{22} & \mathrm{~A}_{32} \\
\mathrm{~A}_{13} & \mathrm{~A}_{23} & \mathrm{~A}_{33}
\end{array}\right]\), where capital letters are co-factors of corresponding small letters.

6. INVERSE OF A MATRIX

Invertible Matrix. Any n-rowed square matrix A is said to be invertible if there exists an n-rowed matrix B such that
AB = BA = I_n
B is called the inverse of A and is denoted as A^-1.

Theorems.
(i) Inverse of every square matrix, if it exists, is unique.
(ii) A is invertible iff |A| ≠ 0
(iii) A^-1 = \(\frac{\operatorname{adj} . \mathrm{A}}{|\mathrm{A}|}\), if | A | ≠ 0.

PROPERTIES:

(i) (AB)^-1 =B^-1 A^-1
(ii) (A’)^-1 = (A^-1)’
(iii) (A^k)^-1 =(A^-1)^k, where k is any positive integer.

7. SINGULAR AND NON-SINGULAR MATRICES
A square matrix is said to be singular if |A| = 0 and non-singular if |A| ≠ 0.

8. SOLUTIONS OF EQUATIONS BY MATRIX METHOD To solve the equations :
\(\begin{array}{l}
a_{11} x_{1}+a_{12} x_{2}+\ldots \ldots+a_{1 n} x_{n}=b_{1} \\
a_{21} x_{1}+a_{22} x_{2}+\ldots \ldots+a_{2 n} x_{n}=b_{2} \\
\ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \\
a_{m 1} x_{1}+a_{m 2} x_{2}+\ldots \ldots+a_{m n} x_{n}=b_{m} .
\end{array}\)
Here X = A^-1B,
where A = \(\left[\begin{array}{cccc}
a_{11} & a_{12} & \ldots \ldots \ldots & a_{1 n} \\
a_{21} & a_{22} & \ldots \ldots \ldots & a_{2 n} \\
\ldots & \ldots \ldots \ldots \ldots & \\
a_{m 1} & a_{m 2} \ldots \ldots \ldots . & a_{m n}
\end{array}\right]\), X = \(\left[\begin{array}{c}
x_{1} \\
x_{2} \\
\cdots \\
x_{n}
\end{array}\right]\), B = \(\left[\begin{array}{c}
b_{1} \\
b_{2} \\
\ldots \\
b_{m}
\end{array}\right]\)

(i) If |A| ≠ 0, then the system is consistent and has a unique solution.
(ii) If | A | = 0 and (adj. A) B = O, (O being a zero matrix) then the system is consistent and has infinitely many solutions.
(iii) If | A | = 0 and (adj. A) B ≠ O, then the system is inconsistent and has no solution.

9. SOLUTION OF HOMOGENEOUS EQUATIONS
To solve the equations :
a₁x + b₁y + c₁z = 0
a₂x + b₂y + c₁z = 0
a₃x + b₃y + c₃z = 0.

Here AX = 0, where A = \(\left[\begin{array}{lll}
a_{1} & b_{1} & c_{1} \\
a_{2} & b_{2} & c_{2} \\
a_{3} & b_{3} & c_{3}
\end{array}\right]\) and X = \(\left[\begin{array}{l}
x \\
y \\
z
\end{array}\right]\)

(i) If |A| ≠ 0, then system has only trivial solution.
(ii) If |A| = 0, the system has infinitely many solutions.

By going through these CBSE Class 12 Maths Notes Chapter 3 Matrices, students can recall all the concepts quickly.

Matrices Notes Class 12 Maths Chapter 3

Matrix (Definition): A matrix is defined as a rectangular array (arrangement) of numbers or functions.A
The matrices are denoted by capital letters as shown below:

→ Elements: The numbers or functions in a matrix are called its elements. In matrix A; 2, 5, 6, 4, 0 and \(\sqrt{3}\) are the elements.

→ Row: The elements lying in a horizontal line form a row. Matrix B has 3 rows viz: first row is (1, 3 + 2i, \(\frac{2}{3}\)) is (-5, 2.3, 7) and third row is (\(\sqrt{7}\) 4 -8).

→ Column: The elements lying in a vertical line form a column. Matrix A has three columns viz: first column is \(\left(\begin{array}{l}
2 \\
4
\end{array}\right)\), second is \(\left(\begin{array}{l}
5 \\
0
\end{array}\right)\) and third is \(\left(\begin{array}{c}
6 \\
\sqrt{3}
\end{array}\right)\)

→ Order of matrix: A matrix, having m rows and n columns, is said to be of the order m × n. The matrix A is of order 2 × 3, B is of order 3 × 3 and C is of order 3 × 2.

In general, a matrix of order m × n, i.e., consisting of m rows and n columns is denoted by A = [a_ij]_m×n.

A number of elements in the matrix [a_ij]_m×n are m × n and nth element = a_ij is that element that lies in the ith row and jth column.

Types of matrices:
→ Square Matrix: If in a matrix, the number of rows is equal to the number of columns, then the matrix is called a square matrix.

has 3 rows and 3 columns. Therefore, it is a square matrix.

In general, [a_ij]_n×n is a square matrix of order n. the elements a₁₁, a₂₂, a₃₃,…,a_ii…, a_nn are the elements of main diagonal. Thus, in the matrix P; 2, 7 and 1 are the diagonal elements.

→ Row Matrix: A matrix, which has one row is known as row matrix. [3 -1 i 2] is a row matrix, which has only one row.

→ Column Matrix: A matrix having one column is said to be is a column matrix.\(\left[\begin{array}{c}
-1 \\
3 \\
2
\end{array}\right]\) is a column matrix, since there is only one column in it.

→ Diagonal Matrix: A square matrix is called a diagonal matrix, if its non-diagonal elements are zero, i.e., a_ij = 0, when i ≠ 0, e.g. \(\left[\begin{array}{ll}
2 & 0 \\
0 & 1
\end{array}\right]\) is a diagonal matrix.

→ Scalar Matrix: It is a square matrix whose (a) diagonal elements are non-zero and equal (b) non-diagonals elements are zero, Le, a_ij = k ≠ 0 when j = j, a_ij = 0, when i ≠ j.\(\left[\begin{array}{lll}
2 & 0 & 0 \\
0 & 2 & 0 \\
0 & 0 & 2
\end{array}\right]\) is scalar matrix.

→ Unit or Identity Matrix: It is a square matrix in which each diagonal element is 1. i.e., a_ij = 1 when i = j and aij = 0 when i ≠ j.\(\left[\begin{array}{lll}
1 & 0 & 0 \\
0 & 1 & 0 \\
0 & 0 & 1
\end{array}\right]\) is an Identity or Unit matrix.

→ Zero Matrix or Null Matrix: A matrix, in which all the elements are equal to zero, is called the zero matrix.\(\left[\begin{array}{lll}
0 & 0 & 0 \\
0 & 0 & 0 \\
0 & 0 & 0
\end{array}\right]\) is a zero matrix.

→ Comparable Matrices: Two matrices are said to be comparable, if they are of the same order. For example, \(\left[\begin{array}{ccc}
2 & 3 & -5 \\
4 & -2 & 6
\end{array}\right]\) and \(\left[\begin{array}{ccc}
i & 2 & x \\
3 & x^{2} & -1
\end{array}\right]\) are comparable matrices since each matrix is of order 2 × 3.

→ Equal Matrices: Two matrices are equal, if (a) they are of the same order (b) their corresponding elements are equal.

if p = 2, q = 3, r = 5, s = 7, t = 9 and u = 8.

OPERATIONS ON MATRICES:
→ Addition of Matrices: The sum of two matrices A and B of the same order is obtained by adding the corresponding elements. Thus,

→ Multiplication of a Matrix by a Scalar: If a matrix A = [a_ij]_m×n is multiplied by a scalar k, then the product kA is obtained by multiplying each element of A, by k. For example,

→ Negative of a Matrix: The negative of a matrix A = -A = (-1)A. For example,

→ Difference of two Matrices: If A and B are the matrices of the same order, then A – B = A + (-1)B = Sum of matrices A and -B.

→ Properties of Matrices Addition: Let A, B, and C be the matrices of the same order m × n.
(a) The commutation Law: A + B = B + A
(b) The Association Law: (A + B) + C = A + (B + C)
(c) The Existence of Additive Identity: Let Omxn be null matrix of order m × n.
A + O_m×n = O_m×n + A = A.
(d) The Existence of Additive Inverse: Let A = [aij]m×n. We have an order matrix – A = [-a_ij]_m×n such that A + (-A) = A – A = O_m×n
-A is called the additive inverse of A or negative of A.

→ Properties of Scalar Multiplication of a Matrix
Let A and B be the matrices of the same order m × n. Then,
(a) k(A + B) = kA + kB
(b) (k + l)A = kA+ lA

→ Multiplication of Matrices
Two matrices A and B are conformable for multiplication if the Tiber of columns in A is equal to the number of rows in B.
If A = [a_ij]_m×n then B = [b_ij]_n×p and AB = [c_ij]_m×p
C_ij = (ij), the element of AB = sum of the products of the elements of the ith row of A with corresponding elements oi jth column of B. Here, (ith row of A) (jth column of B).

No. of columns in A = No. of rows in B = 2 ⇒ A and B are comformable for multiplication. Let AB = [C_ij]_2×3
c₁₁ = (I row of A) × (I column of B)
= \(\left[\begin{array}{ll}
2 & 3
\end{array}\right]\left[\begin{array}{c}
-1 \\
2
\end{array}\right]\) = 2 × (-1) + 3 × 2 = -2 + 6 = 4

c₁₂ = (I row of A) × (II column of B)
= \(\left[\begin{array}{ll}
2 & 3
\end{array}\right]\left[\begin{array}{c}
2 \\
-3
\end{array}\right]\) = 2 × 2 + 3 × (-3) = 4 – 9 = -5

c₁₃ = (I row of A) × (III column of B)
= \(\left[\begin{array}{ll}
2 & 3
\end{array}\right]\left[\begin{array}{l}
4 \\
5
\end{array}\right]\) = 2 × 4 + 3 × 5 = 8 + 15 = 23

c₂₁ = (II row of A) × (I column of B)
= \(\left[\begin{array}{ll}
1 & 4
\end{array}\right]\left[\begin{array}{c}
-1 \\
2
\end{array}\right]\) = 1 × (-1) + 4 × 2 = -1 + 8 = 7

c₂₂ = (II row of A) × (III column of B)
= \(\left[\begin{array}{ll}
1 & 4
\end{array}\right]\left[\begin{array}{c}
2 \\
-3
\end{array}\right]\) = 1 × 2 + 4 × (-3) = 2 – 12 = -10

c₂₃ = (II row of A) × (III column j B)
= \(\left[\begin{array}{ll}
1 & 4
\end{array}\right]\left[\begin{array}{l}
4 \\
5
\end{array}\right]\) =1 × 4 + 4 × 5 = 4 + 20 = 24

→ Properties of Multiplication of Matrices
(a) The Associative Law:
Let A = [a_ij]_m×n, B = [b_ij]_n×p and C = [c_ij]_p×q.
Then, (AB)C = A(BC)

(b) The Distributive Law:

1. If A = [a_ij]_m×n, B = [b_ij]_n×p and C = [c_ij]_p×q, then A(B + C) = AB + AC.
2. If A = [a_ij]_m×n, B = [b_ij]_n×p and C = [c_ij]_p×q, then (A + B)C = AC + BC.

(c) The Existence of Multiplicative Identity:
Let A be a square matrix. There exists an identity matrix I of the same order such that IA = AI = A.

TRANSPOSE OF A MATRIX:
(a) Definition: Let A = [a_ij]_m×n. The matrix obtained by interchanging the rows and columns of A is called transpose of A. It is denoted by A’ or A^T.
For A = [a_ij]_m×n A’ = [aij]_n×m.

(b) Properties of Transpose of a Matrix Let A and B be the two matrices. Then,

1. (A’)’ = A
2. (kA)’ = kA’, where k is a scalar
3. (A + B)’ = A’ + B’ (whenever A + B is defined)
4. (AB)’ = B’A’ (whenever AB is defined)

SYMMETRIC AND SKEW SYMMETRIC MATRICES:
→ Symmetric Matrix: A square matrix A = [a_ij]_n×n is called symmetric, if A’ = A, i.e., for a_ji = a_ij e.g. \(\left[\begin{array}{ccc}
2 & 3 & 4 \\
3 & 1 & 5 \\
4 & 5 & -1
\end{array}\right]\) is a symmetric matrix.

→ Skew Symmetric Matrix: A square matrix A = [aij]n×n is skew symmetric, if A’ = -A for all i, j or a_ji = – a_ij\(\left[\begin{array}{ccc}
0 & 2 & -3 \\
-2 & 0 & 4 \\
3 & -4 & 0
\end{array}\right]\) a skew symmetric matrix.

→ Properties: Let A be a square matrix with real elements.
(a) A + A’ is symmetric.
(b) A – A’ is skew symrrtetric.
(c) A square matrix can be expressed as the sum of the symmetric and skew-symmetric matrix, i.e.,
A = \(\frac{1}{2}\) (A + A’) + \(\frac{1}{2}\) (A – A’)

ELEMENTARY TRANSFORMATIONS OF A MATRIX:
→ Interchange of ith row and jth row is denoted by R_i ↔ R_j. Similarly, interchange of the ith column with the jth column is denoted by C_i ↔ C_j.

→ Multiplication of each element of ith row by k is represented as R_i → kR_j. and when the ith column is multiplied by k, it is represented as C_i → kC_j.

→ Let the element of the ith row of A be added to the corresponding elements of the jth row multiplied by k. It is denoted by R_i → R_i + kR_j.

Similarly, in the case of columns when elements of the ith column are added to the corresponding elements of the jth column multiplied by k, then C_i → C_j + kC_j.

INVERTIBLE MATRICES:
(a) Definition: Let A be a square matrix of order n. If there exists another square matrix B of the same order such that AB = BA = I_n, then A is said to be invertible and B is called the inverse of A. It is denoted by A^-1. ⇒ B = A^-1.

(b) Inverse of a Matrix by Elementary Operations
Let B = A^-1 be the inverse of A.
i.e., I_n = BA
Multiplying I_n by A^-1, we get
A^-1I_n = I_n A^-1 = (BA)A^-1 = B(AA^-1)
= BI_n = B.
⇒ A^-1 = B.
e.g. Let us find the inverse of \(\left[\begin{array}{ll}
1 & 2 \\
2 & 2
\end{array}\right]\) by elementary operations we have: \(\left[\begin{array}{ll}
1 & 2 \\
2 & 2
\end{array}\right]=\left[\begin{array}{ll}
1 & 0 \\
0 & 1
\end{array}\right]\) A

By elementary transformation, we change the matrix \(\left[\begin{array}{ll}
1 & 2 \\
2 & 2
\end{array}\right]\) so that we get identity matrix.

1. MATRIX
Def. A system of mn-numbers (real or complex) arranged in the form of an ordered set of m horizontal lines (called rows) and n vertical lines (called columns) is called an m x n matrix.

2. TYPES OF MATRICES

(i) Rectangular Matrix. Any m x n matrix (m≠n) is called a rectangular matrix.
(ii) Square Matrix. Any n x n matrix is called a square matrix of order n.
(iii) Row Matrix. Any 1 x n matrix is called a row matrix.
(iv) Column Matrix. Any m x 1 matrix is called a column matrix.
(v) Diagonal Matrix. A square matrix A = [a_ij ] is said to be a diagonal matrix if a_ij = 0 when i ≠ j.
(vi) Scalar Matrix. A diagonal matrix is said to be a scalar matrix if all its diagonal elements are equal.
(vii) Identity Matrix. A diagonal matrix is said to be an identity matrix if each of its diagonal elements is unity.
(viii) Zero Matrix. A matrix is said to be a zero matrix if each of its elements is zero.
(ix) Triangular Matrices.

(a) A square matrix A = [a_ij ] is said to be upper triangular matrix if a_ij =0 for i > j.
(b) A square matrix A = [a_ij ] is said to be lower triangular matrix if a_ij =0 for i < j.

3. EQUALITY OF MATRICES
Two matrices A = [a_ij ] and B = [b_ij] are said to be equal iff (i) they are of the same order (ii) their corresponding elements are equal.

4. OPERATIONS ON MATRICES

(i) Addition of Matrices.
Let A = [a_ij]_{m x n} and B = [b_ij]_{m x n} be two matrices. Then the sum A + B = C = [c_ij]_{m x n} , where c_ij + a_ij + b_ij for 1 ≤ i ≤ m, 1 ≤ j ≤ n

Key Point
Addition is defined only for matrices, which are of the same order.

(ii) Multiplication of a matrix by a scalar.
Let A be any m x n matrix and k be any scalar. Then m x n matrix obtained by multiplying each element by k is said to be scalar multiple of A by k and is denoted by kA or Ak.

(iii) Multiplication of Matrices.
Let A = [a_ij] be mxn matrix and B = [b_jk] be nxp matrix such that the number of columns of A equals the number of rows of B. Then matrix C = [c_ik] which is of the order m x p such that:

c_ik = \(\sum_{j=1}^{n} a_{i j} b_{j k}\) where i = 1,2, ….. m; p = 1, 2, 3,…………………k is called the product of the matrices A and B and is written as C = AB.

Key Point
Product AB is defined iff number of columns of A = number of rows of B.

5. TRANSPOSE OF A MATRIX
(i) Def. If A=[a_ij]_{m x n}, then the transpose of A, denoted by A’ (or A^t or A^T) is defined by n x m matrix obtained from A by writing the rows of A as columns and columns of A as rows in the same order.

(ii) Properties:

(a) (A’)’=A 1
(b) (A + B)’=A’+B’, A and B being of same type «
(c) (kA)’ = kA’, k being any scalar »
(d) (AB)’ = B’A’.

6. SYMMETRIC AND SKEW-SYMMETRIC MATRICES

(i) Symmetric Matrix.
Def. A square matrix A = [a_ij] is said to be symmetric if (i,j)th element is the same as its (j, i)th element.

Key Point is
A is symmetric if A’= A.

(ii) Skew-Symmetric Matrix.

A square matrix A = [a_ij] is said to be skew-symmetric if (i,j)th element is negative of its . (j, i)th element.

Key Point
A is skew-symmetric if A’ = – A.

By going through these CBSE Class 12 Maths Notes Chapter 1 Relations and Functions, students can recall all the concepts quickly.

Relations and Functions Notes Class 12 Maths Chapter 1

RELATION
1. Types of Relations
→ Empty Relation: A relation in a set A is known as empty relation, if no element of A is related to any element of A, i.e., R = Φ ⊆ A × A. e.g.

Let the set A = {1, 2,3,4,5) and R is given by
R= {(a,b): a – b = 20}

There is no pair (a, b) that satisfies the condition
a – b = 20.
⇒ The relation R is the empty relation.

→ Universal Relation: A relation R in a set A is called a universal relation, if each element of A is related to every element of A, i.e.,
R = A × A. e.g.
Let the set A = {1, 2,3, 4,5} and R is given by R = {(a, b): ab > 0}
Here, R = {(a, b): ab > 0} is the whole set A × A as all pairs (a, b) in A × A satisfy ab > 0.
Thus, this is the universal relation.

→ A relation R in a set A is called
(a) reflexive: if (a, a) ∈ R for every a ∈ A.
(b) symmetric: ii (a, b) ∈ R implies that (b, a) ∈ R for all a, b ∈ A.
(c) transitive: if (a, b) ∈ R and (b, c) e R implies that (a, c) ∈ R for all a,b,c ∈ A.

→ Equivalence Relation: A relation R in A is an equivalence relation if R is reflexive, symmetric, and transitive. For example:
(1) Let T be the set of all triangles in a plane with R a relation in T given by
R = ((T₁, T₂): T₁ is similar to T₂)
(a) R is reflexive since every triangle is similar to itself.

(b) (T₁, T₂) ∈ R ⇒ T₁ is similar to T₂.
(T₂, T₃) ∈ R ⇒ T₂ is similar to T₁
Therefore, R is symmetric.

(c) (T₁, T₂) and (T₂, T₃) lies in R
⇒ T₁ is similar to T₂ and T₂ is similar to T₃, which means T₁ is similar to T₃,
i.e., (T₁, T₃) lies in R.
∴ R is transitive.
Now R is reflexive, symmetric, and transitive, therefore R is an equivalence relation.

(2) Consider the set A = {1,2,3,4} and the relation R = {(1,1), (2, 2), (3,3), (4, 4), (1, 2), (2, 3), (3, 4)}.
(a) Now (1,1), (2, 2), (3, 3), (4, 4) lie in R. Relation R is reflexive.
(b) (1, 2) lies in R but (2,1) does not lie in it.
∴ It is not symmetric.
(c) (1,2), (2, 3) lie in R but (1, 3) does not lie in it. Therefore, R is not transitive.
Here, R is reflexive but neither symmetric nor transitive. Therefore, R is not an equivalence relation.

2. Equivalence Class [a] containing a
For an arbitrary equivalence relation R in an arbitrary set X, R divides X into mutually disjoint subsets A_i, which are known as partitions or sub-divisions of X satisfying:
(a) All elements of A_i are related to each other for all i.
(b) No element of A_i is related to any element of A_j, i ≠ j.
(c) ∪ A_j = X and A_i ∩ A. = Φ, i ≠ j.

The subsets Af are said to be equivalence classes.
Example: Let R be the relation defined in the set A = {p, q, s, t, e, o, u} by
R = {(a, b): both a and b are either consonants or vowels,
Here, R is an equivalence relation.
(a) Any element ∈ A is either consonant or vowel,
i.e., (a, a) ∈ R ⇒ R is reflexive.

(b) If (a, b) ∈ R ⇒ a and b both are either consonants or vowels ⇒ (b, a) e R.
∴ R is symmetric.

(c) If (a, b) ∈ R and (b, c) ∈ R, then a, b; b, c both pairs are either consonants or vowels.
i.e., a, b, c all are either consonants or vowels.
⇒ (a, c) ∈ R.
∴ R is transitive.
Thus, R is an equivalence relation.

Further, all the elements of (p, q, s, t) are related to each other as all the elements of this subset are consonants.

Similarly, all the elements of {e, i, o, u } are related to each other as all of them are vowels. But no element of {p, q, s, t} can be related to any element of {e, i, o, u}, since the elements of {p, q, s, t} are all consonants and the elements of {e, i, o, u} are all vowels. {p, q, s, t} is an equivalence class.denoted by an element as {p}. Similarly, {e, i, o, u} is an equivalence class denoted by an element [e).

FUNCTIONS
1. Types of Functions
→ One-one (or Injective): A function f: X → Y is said to be one-one (or injective), if the images of the distinct elements of X under/are distinct, i.e., for every x₁, x₂ ∈ X, if f(x₁) = f(x₂) implies that x₁ = x₂.

Each element of X has a distinct image in Y. Such a function or a mapping is one-one.

→ Onto (or surjective): A function f: X →Y is called onto, if every element of Y is the image of some element of X under f, i.e., for all y ∈ Y, there exists an element x in X such that f(x) = y.

Corresponding to each element of Y, there is a pre-image in X. Such a mapping is onto.

→ One-one and Onto (Bijective): A function f: X to Y is known as one-one and onto (or bijective), if f is both one-one and onto.

Here,f is both one-one and onto. Therefore,f is said to be one-one onto function or bijective function.

2. Composition of Functions
Let f: A → B and g: B → C be the two functions. The composition of f and g is defined as. gof: A → C, such that
gof(x) = g{f(x)}, for all x ∈ A.

A function f: X → Y is said to be invertible if there exists a function g: Y → X such that gof = I_x and fog = I_y. The function g is called the inverse of f. It is denoted by f^-1.

Inverse or composite function: If f: X →Y and g: Y → Z be the two invertible functions, then gof is also invertible such that (gof)^-1 = f^-1og^-1

BINARY OPERATION
→ Binary Operation: A binary operation on a set A is a function X: A × A → A, defined by × (a,b) = a × b, e.g., ×: R × R → R is given by (a, b) → a + b. Here +, — and x are the functions but + : R × R →, R, written as (a, b) → \(\frac{a}{b}\) is not a function. It is not a binary operation, since it is not defined for b = O.

→ Commutative Binary Operation: A binary operation × on the set A is commutative,if for every a,b ∈ A, a × b = b × a.

→ Associative Binary Operation: A binary operation × on the set A is associative, if (a × b) × c = a × (b × c).
It may be noted that associative property, a × b × c × d, … is not defined unless brackets are used.

→ An Identity Element e for Binary Operation: Let ×: A × A → A be a binary operation. There exists an element e ∈ A such that a × e = a = e × a, for all a ∈ A.

The element e is known as the identity element. It should be noted that 0 is the identity element for addition but not for natural numbers N, since 0 ∉ N.

→ The inverse of an element a: Let ×: A × A → A be a binary operation with identity element e in A. An element a ∈ A is invertible w.r.t. binary operation ×, if there exists an element b in A such that a × b = e = b × a. The element b is said to be the inverse of a. It is denoted by a^-1, e.g.,

– a is the inverse of a for the operation of addition +.
\(\frac{1}{a}\) (a ≠ 0) is the inverse of a for multiplication.

1. RELATIONS

(i) Relation. A relation R from a set A to a set B is a subset of A x B.

(ii) Classification of Relations : a
(a) Reflexive Relation. A relation R in a set E is said to be reflexive if xRx ∀ x ∈ E.
(b) Symmetric Relation. A relation R in a set E is said to be symmetric if:
xRy = yRx ∀ x, y ∈ E.
(c) Transitive Relation. A relation R in a set E is said to be transitive if:
vRy and yRz ⇒ xRz ∀ x, y, z ∈ E.
(d) Equivalence Relation. A relation R in a set E is said to be an equivalence relation if it is :

• reflexive
• symmetric and
• transitive.

2. FUNCTIONS

(i) Let X and Y be two non-empty sets. Then ‘f’ is a rule, which associates to each element x in X . a unique element y in Y.
(a) The unique element y of Y is called the value of f at x.
(b) The element x of X is called pre-image of y.
(c) The set X is called the domain of f
(d) The set of images of elements of X under f is called the range of f.

(ii) (a) D_f = {x : x ∈ R, f(x) ∈ R}
(b) R_f = {f(x):x ∈ D_f}
(c) f is one-one iff x₁ = x₂
⇒ f(x₁) = f(x₂) for x₁, x₂ ∈ D_f
or iff x₁ ≠ x₂
⇒ f(x₁) ≠ f(x₂) for x₁, x₂ ∈ D_f
(d) f is invertible iff f is one-one onto and D_f^-1 = R_f, R_f^-1= DR_f.

3. ALGEBRA OF FUNCTIONS

Let f and g be two functions. Then
(i) (f+g) (x) =f(x) + g(x); D_f+g = D_f ∩ D_g
(ii) (f- g) (x) = f(x) – g(x); D_f-g = D_f ∩ D_g
(iii) (fg) (x) =f(x) g(x); D_fg = D_f ∩ D_g
(iv) \(\left(\frac{f}{g}\right) x=\frac{f(x)}{g(x)}\); D_f/g = D_f ∩ D_g – {x:x∈D_g, g(x) = 0}

By going through these CBSE Class 12 Maths Notes Chapter 13 Probability, students can recall all the concepts quickly.

Probability Notes Class 12 Maths Chapter 13

1. Conditional Probability:
Let E and F be two events with a random experiment. Then, the probability of occurrence of E under the condition that F has already occurred and P(F) ≠ 0 is called the conditional probability. It is denoted by P(E/F).

The conditional probability P(E/F) is given by
P(E/F) = \(\frac{\mathrm{P}(\mathrm{E} \cap \mathrm{F})}{\mathrm{P}(\mathrm{F})}\) , when P(F) ≠ 0.

→ Properties:

1. 0 ≤ P(E/F) ≤ 1
2. P(F/F) = 1
3. P(A ∪ B/F) = P(A/F) + P(B/F) – P(A n B/F)
   If A and B are disjoint events,
   then P(A ∪ B/F) = P(A/F) + P(B/F).
4. P(E/F) = 1 – P(E/F)

2. Multiplications Probability:
1. Multiplication Theorem on Probability:
Let E and F be two events associated with sample space S. P(E ∩ F) denotes the probability of the event that both E and F occur, which is given by P(E ∩ F) = P(E) P(F/E) = P(F) P(E/F) provided P(E) ≠ 0 and P(F) ≠ 0.
This result is known as the multiplication theorem on probability.

2. Multiplication rule of probability for more than two events:
Let E, F and G be the three events of sample space. Then, P(E ∩F ∩G) = P(E).P(F/E) P[G/(E ∩ F)]

3. Independent Events:

1. Two events E and F are said to be independent, if P(E/F) = P(E) and P(F/E) = P(F), provided P(E) ≠ 0 and P(F) ≠ 0.
   We know that P(E ∩ F)= P(E). P(F/E) and P(E ∩ F) = P(F). P(E/F).
2. Events E and F are independent if P(E ∩ F) = P(E) × P(F).
3. Three events E, F and G are said to be independent or mutually independent, if
   P(E∩F∩G)= P(E). P(F). P(G)

4. Partition of a Sample Space:
A set of events E₁, E₂,……….., E_n is said to represent a partition of sample S, if

1. E_i ∩ F_j = Φ, if i ≠ j, i, j = 1, 2,……….. ,n .
2. E₁ ∪ E₂ ∪ E₃ ∪ …. ∪ E_n = S
3. P(E_i) > 0 for all i = 1, 2,……., n

For example, E and E’ (complement of E) form a partition of sample space S, because
E∩E’ = Φ and E∪E’ = S.

5. Theorem of Total Probability:
Let E₁, E₂,…….., E_n be a partition of sample space and each event has a non-zero probability.
If A be any event associated with S, then
P(A) = P(E₁) P(A/E₁) + P(E₂) P(A/E₂) + P(E₃) P(A/E₃) +…….. + P(E_n) P(A/E_n)

6. Bayes’ Theorem:
Let E₁, E₂,…………, E_n be the n events forming a partition of sample space S, i.e., E₁, E₂,………, E( are pairwise disjoint and E₁ ∪ E₂ ∪………. ∪
E_n = S and A is any event of non-zero probability, then

7. A Few Terminologies:

1. Hypothesis: When Bayes’ Theorem is applied, the events E₁, E₂,……………., E_n is said to be a hypothesis.
2. Priori Probability: The probabilities P(E₁), P(E₂),…………., P(E_n) is called priori.
3. Posteriori Probability: The conditional probability P(E./A) is known as the posterior probability of hypothesis E.

8. Random Variable:
A random variable is a real-valued function whose domain is the sample space of a random experiment.
For example, let us consider the experiment of tossing a coin three times.

The sample space of the experiment is
S{TTT, TTH, THT, HTT, HHT, HTH, THH, HHH}

If x denotes the number of heads obtained, then X is the random variable for each outcome.
X(0) = {TTT}
X(1) = {TTH, THT, HTT}
X(2) = {HHT, HTH, THH}
X(3) = {HHH}

9. Probability Distribution of a Random Variable:
Let real numbers x₁, x₂,…………., x_n be the possible values of random variable and p₁, p₂,……………, p_n be probabilities corresponding to each value of the random variable X. Then the probability distribution is

It may be noted that

1. p_i > 0
2. Sum of probabilities p₁ + p₂ +………….+ p_n = 1

Example: Three cards are drawn successively with replacement from a well-shuffled pack of 52 cards. A random variable denotes the number of spades on three cards. Determine the probability distribution of X.
P(S) = P(Drawing a spade) = \(\frac{13}{52}=\frac{1}{4}\)
P(F) = P(Drawing not a space = 1 – \(\frac{1}{4}=\frac{3}{4}\)
P(X = 0) =P(FFF) = \(\left(\frac{3}{4}\right)^{3}=\frac{27}{64}\)
P(X = 1) = 3 × \(\left(\frac{1}{4} \times \frac{3}{4} \times \frac{3}{4}\right)=\frac{27}{64}\)

∵ X = 1 ⇒{SFF, FSF, FFS}
X = 2 ⇒ {SSF, SFS, FSS}
∴ P(X – 2) = 3 × \(\left(\frac{1}{4} \times \frac{1}{4} \times \frac{3}{4}\right)=\frac{9}{64}\)

When X = 3 ⇒ {SSS}
P(X = 3) = \(\frac{1}{4} \times \frac{1}{4} \times \frac{1}{4}=\frac{1}{64}\)
∴ Probability distribution is

10. Mean of Random Variable:
Let X be the random variable whose possible values are x₁, x₂,……….,x_n. If p₁, p₂,……….., p_n are the corresponding probabilities, then the mean of X denoted by p is given by
|
The mean of a random variable X is also called the expected value of X, denoted by E(x).
For the experiment of drawing a spade in three cards, the expected value ‘

11. Variance of a Random Variable:
Let X be the random variable with possible values of X: x₁ x₂,……x_n occur whose probabilities are p₁, p₂,………., p_n respectively.
Let μ = E(x) be the mean of X. The variance of X, denoted by Var (X) or σx2, is defined as

12. Bernoulli Trial:
Trials of a random experiment are said to be Bernoulli’s Trials if they satisfy the following conditions:

1. The trials should be independent.
2. Each trial has exactly two outcomes viz. success or failure.
3. The probability of success remains the same in each trial.
4. The number of trials is finite.

Example: An urn contains 6 red and 5 white balls. Four balls are drawn successively. Find whether the trials of drawing balls are Bernoulli trials when after each draw the ball draw is

1. replaced
2. not replaced in the urn.

1. If drawing a red ball with replacement is a success, in each trial the probability of success = \(\frac{6}{11}\).
Therefore, drawing a ball with replacement is a Bernoulli trial.

2. In the second attempt, when the ball is not replaced, the probability of success = \(\frac{5}{10}\).

In thired attempt, the probability of success = \(\frac{4}{9}\).
Thus, probability changes at each trial. Hence, in this case, it is not a Bernoulli trial.

13. Binomial Distribution:
The probability distribution of a number of successes in an experiment consisting of n Bernoulli trials is obtained by binomial expansion of (q + p)ⁿ.
Such a probability distribution may be written as:

This probability distribution is called binomial distribution with parameters n and p.

14. Probability Function:
The probability of x success is denoted by P(x). In a binomial distribution P(x) is given by
P(x) = ⁿC_x q^n-x p^x, x = 0,1, 2,…., n and q = 1 – p.
The function P(x) is known as the probability function of the binomial distribution.

1. DEFINITIONS

(i) Random Experiment of Trial. The performance of an experiment is called a trial.
(ii) Event. The possible outcomes of a trial are called events.
(iii) Equally likely Events. The events are said to be equally likely if there is no reason to expect
any one in preference to any other.
(iv ) Exhaustive Events. It is the total number of all possible outcomes of any trial.
(v) Mutually Exclusive Events. Two or more events are said to be mutually exclusive if they
cannot happen simultaneously in a trial. s:
(vi) Favourable Events. The cases which ensure the occurrence of the events are called favourable.
(vii) Sample Space. The set of all possible outcomes of an experiment is called a sample space.
( viii) Probability of occurrences of event A, denoted by P (A), is defined as :
P(A) = \(\frac{\text { No. of favourable cases }}{\text { No. of exhaustive cases }}=\frac{n(\mathrm{~A})}{n(\mathrm{~S})}\)

2. THEOREMS .

(i) In a random experiment, if S be the sample space and A an event, then :
(I) P (A) ≥ 0. (II) P (Φ) = 0 and (III) P (S) = 1.
(ii) If A and B are mutually exclusive events, then P (A ∩ B) = 0.
(iii) If A and B are two mutually exclusive events, then P (A) + P (B) – 1.
(iv) If A and B are mutually exclusive events, then : P (A ∪ B) = P ( A) + P ( B). s
(v) For any two events A and B. P (A ∪ B) = P (A) + P (B) – P (A ∩ B).
(vi) For each event A. P (\(\overline{\mathrm{A}}\)) = 1 – P (A), where (\(\overline{\mathrm{A}}\)) is the complementary event. 1;
( vii) 0 ≤ P (A) ≤ 1.

3. MORE DEFINITIONS
(i) Compound Event. The simultaneous happening of two or more events is called a compound event if they occur in connection with each other. I

(ii) Conditional Probability. Let A and B be two events associated with the same sample spat e then
P (A/B) = \(\frac { No. of elementary events favourable to B which are also favourable to A }{ No. of elementary events favourable to B }\)
Theorem. P (A/B) = \(\frac{P(A \cap B)}{P(B)}\)
P(B/A) = \(\frac{P(A \cap B)}{P(A)}\)

(iii) Independent Events. Two events are said to be independent if the occurrence of one does not a depend upon the occurrence of the other.
Theorem. P (A ∩ B) = P (A) P (B) when A, B are independent.

4. If A₁, A₁, …………A_r be r events, then the probability when at least one event happens
= 1 – \(\mathbf{P}\left(\overline{\mathbf{A}_{1}}\right) \mathrm{P}\left(\overline{\mathbf{A}_{2}}\right) \ldots \cdot \mathbf{P}\left(\overline{\mathrm{A}}_{r}\right)\)

5. BAYES’ FORMULA
If E₁, E₂,…., E_n are mutually exclusive and exhaustive events and A is any event that occurs with E₁, E₂, …. , E_n, then :
P(E₁/A) = \(\frac{\mathrm{P}\left(\mathrm{E}_{1}\right) \mathrm{P}\left(\mathrm{A} / \mathrm{E}_{1}\right)}{\mathrm{P}\left(\mathrm{E}_{1}\right) \mathrm{P}\left(\mathrm{A} / \mathrm{E}_{1}\right)+\mathrm{P}\left(\mathrm{E}_{2}\right) \mathrm{P}\left(\mathrm{A} / \mathrm{E}_{2}\right)+\ldots \ldots \ldots+\mathrm{P}\left(\mathrm{E}_{n}\right) \mathrm{P}\left(\mathrm{A} / \mathrm{E}_{n}\right)}\)

6. MEAN AND VARIANCE OF RANDOM VARIABLE.
Mean (μ) = Σx_ip_i
Variance (σ²) = Σ(x_i – μ)²p_i = Σx_i² p_i – μ²

Here we are providing 1 Mark Questions for Accountancy Class 12 Chapter 3 Reconstitution of Partnership Firm: Admission of a Partner are the best resource for students which helps in class 12 board exams.

Here we are providing 1 Mark Questions for Accountancy Class 12 Chapter 2 Accounting for Partnership: Basic Concepts are the best resource for students which helps in class 12 board exams.

Question 1.
Chhavi and Neha were partners in a firm sharing profits and losses equally. Chhavi withdrew a fixed amount at the beginning of each quarter. Interest on drawings is charged @ 6% p.a. At the end of the year, interest ‘ on Chhavi’s drawings amounted to ₹ 900. Pass necessary journal entry for charging interest on drawings.
Answer:

Question 2.
Dev withdrew ₹ 10,000 on 15th day of every month. Interest on drawings was to be charged @ 12% per
annum. Calculate interest on Dev’s drawings. (CBSE Outside Delhi 2019)
Answer:
Interest On Drawings = 1,20,000 x 12/100 x 6 x 12 = 7,200

Question 3.
Amit, a partner in a partnership firm withdrew ₹ 7,000 in the beginning of each quarter. For how many months would interest on drawings be charged₹ (CBSE SP 2019-20)
Answer:
7 1/2 months.

Question 4.
Raj and Seema started a partnership firm on 1st July, 2018. They agreed that Seema was entitled to a commission of 10% of the net profit after charging Raj’s salary of ₹ 2,500 per quarter and Seema’s commission. The net profit before charging Raj’s salary and Seema’s commission for the year ended 31st March, 2019 was ₹ 2,27,500. Calculate Seema’s commission. (CBSE Compt. 2019)
Answer:
Net profit before salary and commission = ₹ 2,27,500
Net Raj’s salary ₹ 2,500 x 3 = ₹ 7,500
Net profit after Raj’s salary but before Seema’s commission = ₹ 2,20,000
Seema’s commission = 10/110 of ₹ 2,20,000
= ₹ 20,000

Question 5.
A and B are partners in a firm sharing profits and losses in the ratio of 7 : 3. Their fixed capitals were : A ₹ 9,00,000 and B ₹ 4,00,000. The partnership deed provided the following: (CBSE Compt. 2019)
(i) Interest on capital @ 10% p.a.
(ii) A’s salary ₹ 50,000 per year and B’s salary ₹ 3,000 per month.
Profit for the year ended 31st March 2019 ₹ 2,78,000 was distributed without providing for interest on capital and partner’s salary.
Showing your working clearly, pass the necessary adjustment entry for the above omissions.
Answer:

Question 6.
Partners of ABC Corporation have agreed that D, a minor, should be admitted as a partner in the firm. What will be liability of D?
Answer:
Limited.

Question 7.
X, Y and Z are partners in a firm. The firm had adopted fixed capital method. Mention the account in which the interest on capital will be recorded:
Answer:
Capital Account.

Question 8.
A partnership deed provides for the payment of interest on capital but there was a loss instead of profits during the year 2010-11. Will the interest on capital be allowed?
Answer:
No.

Question 9.
Where is interest on a partner’s loan debited to Profit and Loss Account or Profit and Loss Appropriation Account?
Answer:
Profit and loss Account.

Question 10.
Is interest on a partner’s loan is payable even in case of loss to the firm?
Answer:
Yes.

Question 11.
Net profit of a firm is ₹ 30,000, partners’ salary is ₹ 12,000 and interest on capital is ₹ 20,000. Mention the amount of partners’ salary and interest on capital which should be debited to Profit and Loss Appropriation Account if both items are treated as appropriation.
Answer:
Partners’ salary ₹ 11,250, Interest on capital ₹ 18,750.
Note: In the ratio of salary and interest on capital i.e. 12,000 : 20,000 = 3:5.

Question 12.
Ram and Shyam are partners sharing profits/losses equally. Ram withdrew ₹ 1,000 p.m. regularly on the first day of every month during the year 2013-14 for personal expenses. If interest on drawings is charged @ 5% p.a. Calculate interest on the drawings of Ram.
Answer:

Question 13.
Verma and Kaul are partners in a firm. The partnership agreement provides that interest on drawings should be charged @ 6% p.a. Verma withdraws X 2,000 per month starting from April 01, 2013 to March 31, 2014. Kaul withdraw ₹ 3,000 per quarter, starting from April 01, 2013. Calculate interest on partner’s drawings.
Answer:

Question 14.
Himanshu withdraws ₹ 2,500 at the end of each month. The partnership deed provides for charging the interest on drawings @ 12% p.a. Calculate interest on Himanshu’s drawings for the year ending 31st December, 2013.
Answer:

Question 15.
Bharam is a partner in a firm. He withdraws ₹ 3,000 at the starting of each month for 12 months. The books . of the firm closes on March 31 every year. Calculate interest on drawings if the rate of interest is 10% p.a.
Answer:
Bharam withdraws ₹ 3,000 at the starting of each month.

Question 16.
Amit and Bhola are partners in a firm. They share profits in the ratio of 3 : 2. As per their partnership agreement, interest on drawings is to be charged @ 10% p.a. Their drawings during 2013 were ₹ 24,000 and ₹ 16,000, respectively. Calculate interest on drawings based on the assumption that the amounts were withdrawn evenly, throughout the year.
Answer:
Amit’s Drawings = ₹ 24,000

Note: In the absence of date of drawings, it is assumed drawings have been made in the middle of each month/period.

Question 17.
A, B and C were partners in a firm sharing profits in the ratio of 3 : 2 : 1. B was guaranteed a profit of X 2,00,000. During the year the firm earned a profit of ₹ 84,000. Calculate the net amount of Profit/Loss transferred to the capital accounts of A and C. (CBSE Sample Paper 2017-18)
Answer:
Net Amount of Loss transferred to:

• A’s Capital Account: ₹ 87,000
• C’s Capital Account: ₹ 29,000

Here we are providing 1 Mark Questions for Accountancy Class 12 Chapter 1 Accounting for Not for Profit Organisation are the best resource for students which helps in class 12 board exams.

Here we are providing 1 Mark Questions for Accountancy Class 12 Chapter 8 Financial Statements of a Company are the best resource for students which helps in class 12 board exams.

Here we are providing 1 Mark Questions for Accountancy Class 12 Chapter 7 Issue and Redemption of Debentures are the best resource for students which helps in class 12 board exams.

Here we are providing 1 Mark Questions for Accountancy Class 12 Chapter 6 Accounting for Share Capital are the best resource for students which helps in class 12 board exams.

Here we are providing 1 Mark Questions for Accountancy Class 12 Chapter 5 Dissolution of a Partnership Firm are the best resource for students which helps in class 12 board exams.