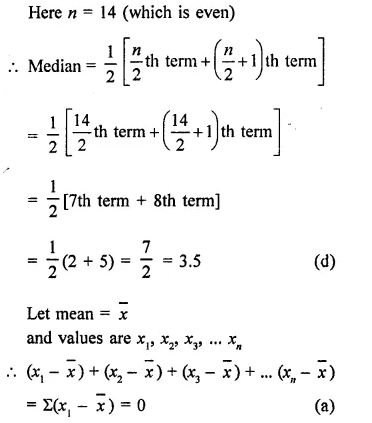
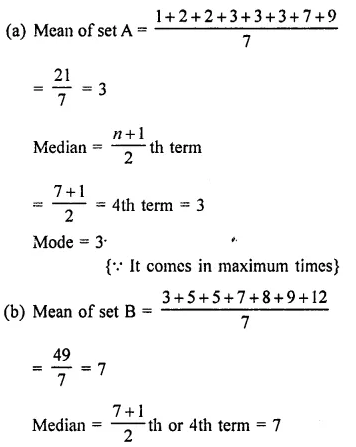
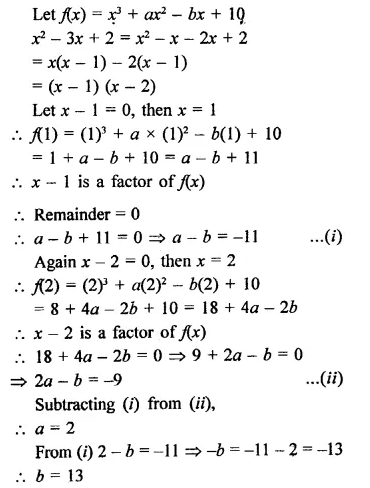
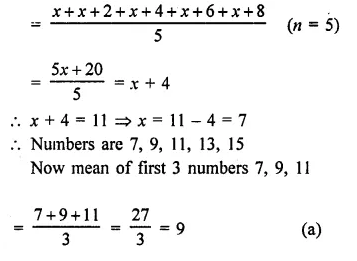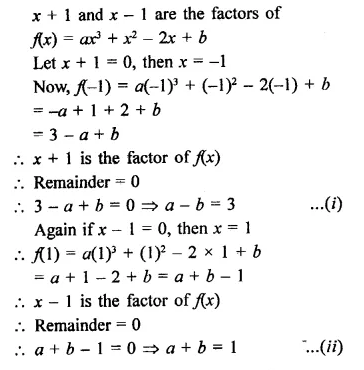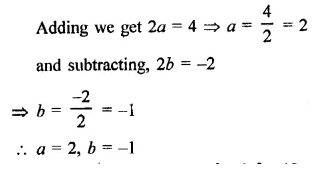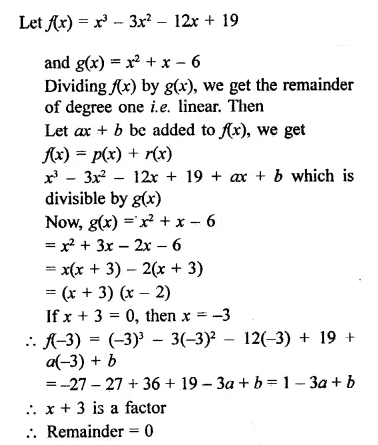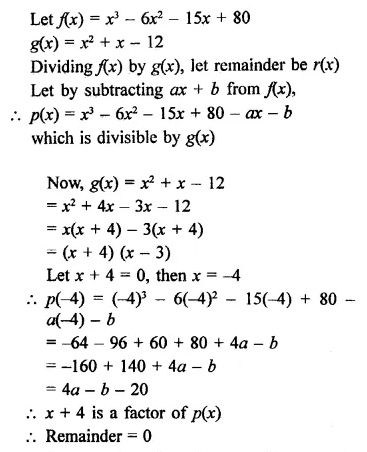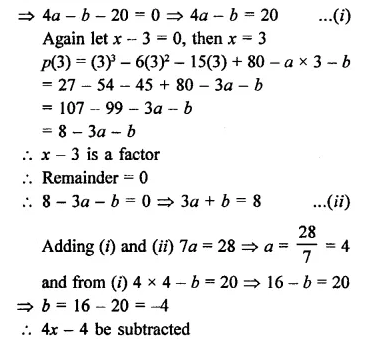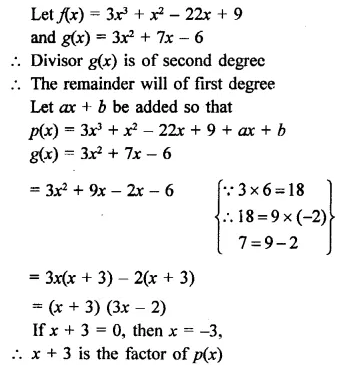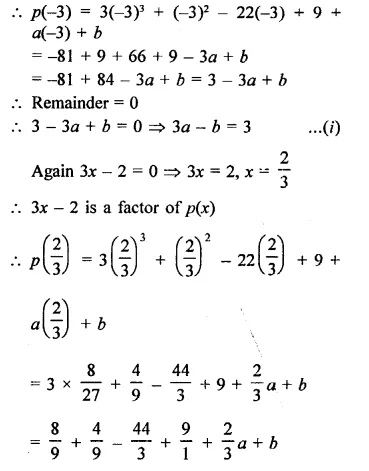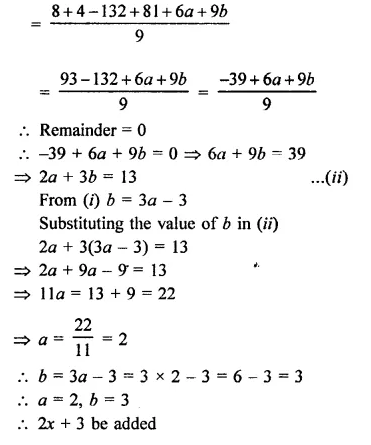Maths Class 9 RD Sharma Solutions – Page 7

RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency Ex 24.3

January 20, 2023

RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency Ex 24.3

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency Ex 24.3

Other Exercises

Find the median of the following data (1-8)

Question 1.
83, 37, 70, 29, 45, 63, 41, 70, 34, 54
Solution:
We know that median = \(\frac { 1 }{ 2 } \left[ \frac { n }{ 2 } th\quad term+\left( \frac { n }{ 2 } +1 \right) th\quad term \right] \)
(When n is even)
= \(\frac { n+1 }{ 2 } th\quad term\)
83, 37, 70, 29, 45, 63, 41, 70, 34, 54
Arranging in ascending order, 29, 34, 37, 41, 45, 54, 63, 70, 70, 83
Here n = 10 which an even
Median = \(\frac { 1 }{ 2 } \)[5th term + 6th term]
= \(\frac { 1 }{ 2 } \) (45+54) = \(\frac { 99 }{ 2 } \) = 49.5

Question 2.
133, 73, 89, 108, 94, 104, 94, 85, 100, 120
Solution:
133, 73, 89, 108, 94, 104, 94, 85, 100, 120
Arranging in ascending order, 73, 85, 89, 94, 94, 100, 104, 108, 120, 133
Here n = 10 which is an even
Median = \(\frac { 1 }{ 2 } \)[5th term + 6th term]
= \(\frac { 1 }{ 2 } \) (94+100) = \(\frac { 1 }{ 2 } \) x 194 = 97

Question 3.
31, 38, 27, 28, 36, 25, 35, 40
Solution:
31, 38, 27, 28, 36, 25, 35, 40
Arranging in ascending order, 25, 27, 28, 31, 35, 36, 38, 40
Here n = 8 which is even
Median = \(\frac { 1 }{ 2 } \)[4th term + 5th term]
= \(\frac { 1 }{ 2 } \) (31+35) = \(\frac { 1 }{ 2 } \) x 66 = 33

Question 4.
15, 6, 16, 8, 22, 21, 9, 18, 25
Solution:
15, 6, 16, 8, 22, 21, 9, 18, 25
Arranging in ascending order = 6, 8, 9, 15, 16, 18, 21, 22, 25
Here n = 9 which is odd
Median \(\frac { n+1 }{ 2 } th\quad term\) = \(\frac { 9+1 }{ 2 } th\quad term\) = \(\frac { 10 }{ 2 } th\quad \)
= 5th term = 16

Question 5.
41, 43, 127, 99, 71, 92, 71, 58, 57
Solution:
41, 43, 127, 99, 71, 92, 71, 58, 57
Arranging in ascending order = 41, 43, 57, 58, 71, 71, 92, 99, 127
Here n = 9 which is an odd
Median \(\frac { n+1 }{ 2 } th\quad term\) = \(\frac { 9+1 }{ 2 } th\quad term\) = \(\frac { 10 }{ 2 } th\quad\)
= 5th term = 71

Question 6.
25, 34, 31, 23, 22, 26, 35, 29, 20, 32
Solution:
25, 34, 31, 23, 22, 26, 35, 29, 20, 32
Arranging in ascending order = 20, 22, 23, 25, 26, 29, 31, 32, 34, 35
Here n = 10 which is even
Median = \(\frac { 1 }{ 2 } \left[ \frac { n }{ 2 } th\quad term+\left( \frac { n }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \) [5th term + 6th term]
= \(\frac { 1 }{ 2 } \) (26 + 29) = \(\frac { 1 }{ 2 } \) x 55 = \(\frac { 55 }{ 2 } \) = 27.5

Question 7.
12, 17, 3, 14, 5, 8, 7, 15
Solution:
12, 17, 3, 14, 5, 8, 7, 15
Arranging in ascending order = 3, 5, 7, 8, 12, 14, 15, 17
Here n = 8 which is odd
Median = \(\frac { 1 }{ 2 } \left[ \frac { n }{ 2 } th\quad term+\left( \frac { n }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \) [4th term + 5th term]
= \(\frac { 1 }{ 2 } \) (8+12) = \(\frac { 1 }{ 2 } \) x 20 = 10

Question 8.
92, 35, 67, 85, 72, 81, 56, 51, 42, 69
Solution:
92, 35, 67, 85, 72, 81, 56, 51, 42. 69
Arranging in ascending order = 35, 42, 51, 56, 67, 69, 72, 81, 85, 92
Here n = 10 which is even
Median = \(\frac { 1 }{ 2 } \left[ \frac { n }{ 2 } th\quad term+\left( \frac { n }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \) [5th term + 6th term]
= \(\frac { 1 }{ 2 } \) (67+69) = \(\frac { 1 }{ 2 } \) x 136 = 68

Question 9.
Numbers 50, 42, 35, 2x + 10, 2x – 8, 12, 11, 8 are written in descending order and their median is 25, find x.
Solution:
50, 42, 35, 2x + 10, 2x – 8, 12, 11, 8 are in descending order
Here n = 8 which is even
Now Median = \(\frac { 1 }{ 2 } \left[ \frac { n }{ 2 } th\quad term+\left( \frac { n }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \) [4th term + 5th term] = \(\frac { 1 }{ 2 } \)[2x + 10 + 2x – 8]
= \(\frac { 1 }{ 2 } \) [4x + 2] = 2x + 1
But median = 25
2x + 1 = 25
=> 2x = 25 – 1 = 24
=> \(\frac { 24 }{ 2 } \) = 12
Hence x = 12

Question 10.
Find the median of the following observations 46, 64, 87, 41, 58, 77, 35, 90, 55, 92, 33. If 92 is replaced by 99 and 41 by 43 in the above data, find the new median?
Solution:
46, 64, 87, 41, 58, 77, 35, 90, 55, 92, 33
Writing in ascending order = 33, 35, 41, 46, 55, 58, 64, 77, 87, 90, 92
Here n = 11 which is odd
Median = \(\frac { n+1 }{ 2 } \) th term
= \(\frac { 11+1 }{ 2 } \) = \(\frac { 12 }{ 2 } \)
= 6th term = 58
By replacing 92 by 93 and 41 by 43, then new order will be
33, 35, 43, 46, 55, 58, 64, 77, 87, 90, 99
Median = 6th term = 58

Question 11.
Find the median of the following data : 41, 43, 127, 99, 61, 92, 71, 58, 57. If 58 is replaced by 85, what will be the new median.
Solution:
41, 43, 127, 99, 61, 92, 71, 58, 57
Arranging in ascending order = 41, 43, 57, 58, 61, 71, 92, 99, 127
Here n = 9 which is odd
Median = \(\frac { n+1 }{ 2 } \) th term = \(\frac { 9+1 }{ 2 } \) th term
= \(\frac { 10 }{ 2 } \) = 5th term = 61
By change 58 by 92, we get new order = 41, 43, 57, 61, 71, 92, 92, 99, 127
Median = 5th term = 71

Question 12.
The weights (in kg) of 15 students are : 31, 35, 27, 29, 32, 43, 37, 41, 34, 28, 36, 44, 45, 42, 30. Find the median. If the weight 44 kg is replaced by 46 kg and 27 kg by 25 kg, find the new median.
Solution:
Weights of 15 students are 31, 35, 27, 29, 32, 43, 37, 41, 34, 28, 36, 44, 45, 42, 30
Writing in ascending order = 27, 28, 29, 30, 31, 32, 34, 35, 36, 37, 41, 42, 43, 44, 45
here n = 15 which is odd
n+1 15+1
Median = \(\frac { n+1 }{ 2 } \) th term = \(\frac { 15+1 }{ 2 } \)
= \(\frac { 16 }{ 2 } \)th term = 8th term = 35 kg
By replacing 44 kg by 46 kg and 27 kg by 25 kg we get new order,
25, 28, 29, 30, 31, 32, 34, 35, 36, 37, 41, 42, 43, 45, 46
Median = 8th term = 35 kg

Question 13.
The following observations have been arranged in ascending order. If the median of the data is 63, find the value of x: 29, 32, 48, 50, x, x + 2, 72, 78, 84, 95
Solution:
Median = 63
29, 32, 48, 50, x, x + 2, 72, 78, 84, 95
Here n = 10 which is even
median = \(\frac { 1 }{ 2 } \left[ \frac { n }{ 2 } th\quad term+\left( \frac { n }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \left[ \frac { 10 }{ 2 } th\quad term+\left( \frac { 10 }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \) [5th term + 6th term]
= \(\frac { 16 }{ 2 } \) [x+x+2] = \(\frac { 2x + 2 }{ 2 } \) = x + 1
x + 1 = 63 = x = 63 – 1 = 62
Hence x = 62

Hope given RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency Ex 24.3 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 10 Congruent Triangles VSAQS

January 20, 2023

RD Sharma Class 9 Solutions Chapter 10 Congruent Triangles VSAQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 10 Congruent Triangles VSAQS

Other Exercises

Question 1.
Define complementary angles.
Solution:
Two angles whose sum is 90°, are called complementary angles.

Question 2.
Define supplementary angles.
Solution:
Two angles whose sum is 180°, are called supplementary angles.

Question 3.
Define adjacent angles.
Solution:
Two angles which have common vertex and one arm common are called adjacent angles.

Question 4.
The complement of an acute angles is…….
Solution:
The complement of an acute angles is an acute angle.

Question 5.
The supplement of an acute angles is………
Solution:
The supplement of an acute angles is a obtuse angle.

Question 6.
The supplement of a right angle is…….
Solution:
The supplement of a right angle is a right angle.

Question 7.
Write the complement of an angle of measure x°.
Solution:
The complement of x° is (90° – x)°

Question 8.
Write the supplement of an angle of measure 2y°.
Solution:
The supplement of 2y° is (180° – 2y)°

Question 9.
If a wheel has six spokes equally spaced, then find the measure of the angle between two adjacent spokes.
Solution:
Total measure of angle around a point = 360°
Number of spokes = 6
∴ Angle between the two adjacent spokes = \(\frac { { 360 }^{ \circ } }{ 6 }\) = 60°

Question 10.
An angle is equal to its supplement. Determine its measure.
Solution:
Let required angle = x°
Then its supplement angle = 180° – x
x = 180° – x
⇒ x + x = 180°
⇒ 2x = 180° ⇒ x = \(\frac { { 180 }^{ \circ } }{ 2 }\) = 90°
∴ Required angle = 90°

Question 11.
An angle is equal to five times its complement. Determine its measure.
Solution:
Let required measure of angle = x°
∴ Its complement angle = 90° – x
∴ x = 5(90° – x)
⇒ x = 450° – 5x
⇒ x + 5x = 450°
⇒ 6x = 450°
⇒ x = \(\frac { { 450 }^{ \circ } }{ 6 }\) = 75°
∴ Required angle = 75°

Question 12.
How many pairs of adjacent angles are formed when two lines intersect in a point?
Solution:
If two lines AB and CD intersect at a point O, then pairs of two adjacent angles are, ∠AOC and ∠COB, ∠COB and ∠BOD, ∠BOD and DOA, ∠DOA and ∠ZAOC
i.e, 4 pairs
RD Sharma Class 9 Solutions Chapter 10 Congruent Triangles VSAQS Q12.1

Hope given RD Sharma Class 9 Solutions Chapter 10 Congruent Triangles VSAQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency MCQS

January 20, 2023

RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency MCQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency MCQS

Other Exercises

Mark the correct alternative in each of the following:

Question 1.
Which one of the following is not a measure of central value?
(a) Mean
(b) Range
(c) Median
(d) Mode
Solution:
Range (b)

Question 2.
The mean of n observations is \(\overline { X } \) . If k is added to each observation, then the new mean is
(a) \(\overline { X } \)
(b) \(\overline { X } \) + k
(c) \(\overline { X } \) – k
(d) k\(\overline { X } \)
Solution:
Mean of n observation = \(\overline { X } \)
By adding k to each observation the new mean will be \(\overline { X } \) + k (b)

Question 3.
The mean of n observations is \(\overline { X } \) . If each observation is multiplied by k, the mean of new observations is
(a) k\(\overline { X } \)
(b) \(\frac { \overline { X } }{ k } \)
(c) \(\overline { X } \) + k
(d) \(\overline { X } \) – k
Solution:
Mean of n observations = \(\overline { X } \)
By multiplying each observation by k,
the new mean = k\(\overline { X } \) (a)

Question 4.
The mean of a set of seven numbers is 81. If one of the numbers is discarded, the mean of the remaining numbers is 78. The value of discarded number is
(a) 98
(b) 99
(c) 100
(d) 101
Solution:
Mean of 7 numbers = 81
Total = 7 x 81 = 567
By discarding one number, the mean of the remaining 7 – 1 = 6 numbers = 78
Total = 6 x 78 = 468
Discarded number = 567 – 468 = 99 (b)

Question 5.
For which set of numbers do the mean, median and mode all have the same value?
(a) 2, 2, 2, 2, 4
(b) 1, 3, 3, 3, 5
(c) 1, 1, 2, 5, 6
(d) 1, 1, 1, 2, 5
Solution:
a) In set 2, 2, 2, 2, 4
RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency MCQS 5.1
Mode = 3 as it come in maximum times
This set has mean, median and mode same (b)

Question 6.
For the set of numbers 2, 2, 4, 5 and 12, which of the following statements is true?
(a) Mean = Median
(b) Mean > Mode
(c) Mean < Mode
(d) Mode = Median
Solution:
The given set is 2, 2, 4, 5, 12
RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency MCQS 6.1

Question 7.
If the arithmetic mean of 7, 5, 13, x and 9 is 10, then the value of x is
(a) 10
(b) 12
(c) 14
(d) 16
Solution:
Arithmetic mean of 7, 5, 13, x, 9 is 10
RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency MCQS 7.1

Question 8.
If the mean of five observations x, x + 2, x + 4, x + 6, x + 8, is 11, then the mean of first three observations is
(a) 9
(b) 11
(c) 13
(d) none of these
Solution:
Mean = 11
But mean of x, x + 2, x + 4, x+ 6, x + 8
RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency MCQS 8.1

Question 9.
Mode is
(a) least frequent value
(b) middle most value
(c) most frequent value
(d) none of these
Solution:
Mode is most frequent value (c)

Question 10.
The following is the data of wages per day: 5, 4, 7, 5, 8, 8, 8, 5, 7, 9, 5, 7, 9, 10, 8 The mode of the data is
(a) 7
(b) 5
(c) 8
(d) 10
Solution:
Wages per day
5, 4, 7, 5, 8, 8, 8, 5, 7, 9, 5, 7, 9, 10, 8
=> 4, 5, 5, 5, 5, 7, 7, 7, 8, 8, 8, 8, 9, 9, 10
Here 8 comes in maximum times
Mode = 8 (c)

Question 11.
The median of the following data :
is ,
(a) 0
(b) -1.5
(c) 2
(d) 3.5
Solution:
Arranging in ascending order,
-3, -3, -1, 0, 2, 2, 2, 5, 5, 5, 5, 6, 6, 6
RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency MCQS 11.1

Question 12.
The algebraic sum of the deviations of a set of n values from their mean is
(a) 0
(b) n – 1
(c) n
(d) n + 1
Solution:
The algebraic sum of deviation of a set of n values from that mean

Question 13.
A, B, C are three sets of values of X:
A : 2, 3, 7, 1, 3, 2, 3
B: 7, 5, 9, 12, 5, 3, 8
C: 4, 4, 11, 7 ,2, 3, 4
Which one of the following statements is
correct?
(a) Mean of A = Mode of C
(b) Mean of C = Median of B
(c) Median of B = Mode of A
(d) Mean, Median and Mode of A are equal.
Solution:
Arranging the sets in ascending order
A{2, 3, 7, 1,3,2,3)
= {1, 2, 2, 3, 3, 3, 7)
B = {7, 5, 9, 12, 5, 3, 8)
= {3, 5, 5, 7, 8, 9, 12)
C = {4, 4, 11,7,2,3,4)
= {2, 3, 4, 4, 4, 7, 11)
RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency MCQS 13.1
Mode = 5 {as it comes max times}
(c) Mean of set C = \(\\ \frac { 2+3+4+4+4+7+11 }{ 7 } \)
= \(\\ \frac { 35 }{ 7 } \) = 5
Median = \(\\ \frac { 7+1 }{ 2 } \) th =\(\\ \frac { 8 }{ 2 } \) =4th term = 4
Mode =4 {as it comes max times}
In set A,mean = median = mode = 3 (d)

Question 14.
The empirical relation between mean, mode and median is
(a) Mode = 3 Median — 2 Mean
(b) Mode 2 Median — 3 Mean
(c) Median 3 Mode — 2 Mean
(d) Mean = 3 Median —2 Mode
Solution:
The empirical relations between mean, mode
and median is
Mode = 3 Median — 2 Mean (a)

Question 15.
The mean of a, b, c, d and e is 28. If the mean of a, c, and e is 24, what is the mean of b and d?
(a) 31
(b) 32
(c) 33
(d) 34
Solution:
Mean of a, b, c, d and e = 28
Total of a, b, c, d and e = 28 x 5 = 140
Mean of a, c and e is = 24
Total of a, c, e = 24 x 3 = 72
Total of b and d = 140 – 72 = 68
Mean = \(\\ \frac { 68 }{ 2 } \) = 34 (d)

Hope given RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency MCQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency Ex 24.4

January 20, 2023

RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency Ex 24.4

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency Ex 24.4

Other Exercises

Question 1.
Find out the mode of the following marks obtained by 15 students in a class:
Marks : 4, 6, 5, 7, 9, 8, 10, 4, 7, 6, 5, 9, 8, 7, 7.
Solution:
Marks obtained are in ascending order,
4, 4, 5, 5, 6, 6, 7, 7, 7, 7, 8, 8, 9, 9, 10
Here we see that 7 is the number which is maximum times i.e. 4 times
Mode = 7

Question 2.
Find the mode for the following data:
125, 175, 225, 125, 225, 175, 325, 125, 375, 225, 125
Solution:
Arranging in ascending order,
125, 125, 125, 125, 175, 175, 225, 225, 225, 325, 375
We see that, 125 is the number which is in maximum times
Mode = 125

Question 3.
Find the mode for the following series:
7.5, 7.3, 7.2, 7.2, 7.4, 7.7, 7.7, 7.5, 7.3, 7.2, 7.6, 7.2
Solution:
Arranging in ascending order,
7.2, 7.2, 7.2, 7.2, 7.3, 7.3, 7.4, 7.5, 7.5, 7.6, 7.7, 7.7
We see that 7.2 comes in maximum times
Mode = 7.2

Question 4.
Find the mode of the following data in each case:
(i) 14, 25, 14, 28, 18, 17, 18, 14, 23, 22, 14, 18
(ii) 7, 9, 12, 13, 7, 12, 15, 7, 12, 7, 25, 18, 7
Solution:
(i) 14, 25, 14, 28, 18, 17, 18, 14, 23, 22, 14, 18
Arranging in ascending order,
14, 14, 14,. 14, 17, 18, 18, 18, 22, 23, 25, 28
Here we see that 14 comes in maximum times
Mode = 14
(ii) 7, 9, 12, 13, 7, 12, 15, 7, 12, 7, 25, 18, 7
Arranging in order,
7, 7, 7, 7, 7, 9, 12, 12, 12, 13, 15, 18, 25
Here we see that 7 comes in maximum times
Mode = 7

Question 5.
The demand of different shirt sizes, as obtained by a survey, is given below:
RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency Ex 24.4 5.1
Find the modal shirt sizes, as observed from the survey.
Solution:
From the given data

From above, we see that
Modal size is 39 as it has maximum times persons

Hope given RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency Ex 24.4 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS

January 20, 2023

RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS

Other Exercises

Question 1.
Define a triangle.
Solution:
A figure bounded by three lines segments in a plane is called a triangle.

Question 2.
Write the sum of the angles of an obtuse triangle.
Solution:
The sum of angles of an obtuse triangle is 180°.

Question 3.
In ∆ABC, if ∠B = 60°, ∠C = 80° and the bisectors of angles ∠ABC and ∠ACB meet at a point O, then find the measure of ∠BOC.
Solution:
In ∆ABC, ∠B = 60°, ∠C = 80°
OB and OC are the bisectors of ∠B and ∠C
∵ ∠A + ∠B + ∠C = 180° (Sum of angles of a triangle)
⇒ ∠A + 60° + 80° = 180°
⇒ ∠A + 140° = 180°
∴ ∠A = 180°- 140° = 40°
RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS Q3.1
= 90° + – x 40° = 90° + 20° = 110°

Question 4.
If the angles of a triangle are in the ratio 2:1:3. Then find the measure of smallest angle.
Solution:
Sum of angles of a triangle = 180°
Ratio in the angles = 2 : 1 : 3
Let first angle = 2x
Second angle = x
and third angle = 3x
∴ 2x + x + 3x = 180° ⇒ 6x = 180°
∴ x = \(\frac { { 180 }^{ \circ } }{ 6 }\) = 30°
∴ First angle = 2x = 2 x 30° = 60°
Second angle = x = 30°
and third angle = 3x = 3 x 30° = 90°
Hence angles are 60°, 30°, 90°

Question 5.
State exterior angle theorem.
Solution:
Given : In ∆ABC, side BC is produced to D
RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS Q5.1
To prove : ∠ACD = ∠A + ∠B
Proof: In ∆ABC,
∠A + ∠B + ∠ACB = 180° …(i) (Sum of angles of a triangle)
and ∠ACD + ∠ACB = 180° …(ii) (Linear pair)
From (i) and (ii)
∠ACD + ∠ACB = ∠A + ∠B + ∠ACB
∠ACD = ∠A + ∠B
Hence proved.

Question 6.
The sum of two angles of a triangle is equal to its third angle. Determine the measure of the third angle.
Solution:
In ∆ABC,
∠A + ∠C = ∠B
RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS Q6.1
But ∠A + ∠B + ∠C = 180° (Sum of angles of a triangle)
∴ ∠B + ∠A + ∠C = 180°
⇒ ∠B + ∠B = 180°
⇒ 2∠B = 180°
⇒ ∠B = \(\frac { { 180 }^{ \circ } }{ 2 }\) = 90°
∴ Third angle = 90°

Question 7.
In the figure, if AB || CD, EF || BC, ∠BAC = 65° and ∠DHF = 35°, find ∠AGH.
RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS Q7.1
Solution:
Given : In figure, AB || CD, EF || BC ∠BAC = 65°, ∠DHF = 35°

∵ EF || BC
∴ ∠A = ∠ACH (Alternate angle)
∴ ∠ACH = 65°
∵∠GHC = ∠DHF
(Vertically opposite angles)
∴ ∠GHC = 35°
Now in ∆GCH,
Ext. ∠AGH = ∠GCH + ∠GHC
= 65° + 35° = 100°

Question 8.
In the figure, if AB || DE and BD || FG such that ∠FGH = 125° and ∠B = 55°, find x and y.
RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS Q8.1
Solution:
In the figure, AB || DF, BD || FG

∠FGH = 125° and ∠B = 55°
∠FGH + FGE = 180° (Linear pair)
⇒ 125° + y – 180°
⇒ y= 180°- 125° = 55°
∵ BA || FD and BD || FG
∠B = ∠F = 55°
Now in ∆EFG,
∠F + ∠FEG + ∠FGE = 180°
(Angles of a triangle)
⇒ 55° + x + 55° = 180°
⇒ x+ 110°= 180°
∴ x= 180°- 110° = 70°
Hence x = 70, y = 55°

Question 9.
If the angles A, B and C of ∆ABC satisfy the relation B – A = C – B, then find the measure of ∠B.
Solution:
In ∆ABC,
∠A + ∠B + ∠C= 180° …(i)
and B – A = C – B
RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS Q9.1
⇒ B + B = A + C ⇒ 2B = A + C
From (i),
B + 2B = 180° ⇒ 3B = 180°
∠B = \(\frac { { 180 }^{ \circ } }{ 3 }\) = 60°
Hence ∠B = 60°

Question 10.
In ∆ABC, if bisectors of ∠ABC and ∠ACB intersect at O at angle of 120°, then find the measure of ∠A.
Solution:
In ∆ABC, bisectors of ∠B and ∠C intersect at O and ∠BOC = 120°
RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS Q10.1
But ∠BOC = 90°+ \(\frac { 1 }{ 2 }\)
90°+ \(\frac { 1 }{ 2 }\) ∠A= 120°
⇒ \(\frac { 1 }{ 2 }\) ∠A= 120°-90° = 30°
∴ ∠A = 2 x 30° = 60°

Question 11.
If the side BC of ∆ABC is produced on both sides, then write the difference between the sum of the exterior angles so formed and ∠A.
Solution:
In ∆ABC, side BC is produced on both sides forming exterior ∠ABE and ∠ACD
Ext. ∠ABE = ∠A + ∠ACB
and Ext. ∠ACD = ∠ABC + ∠A
RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS Q11.1
Adding we get,
∠ABE + ∠ACD = ∠A + ∠ACB + ∠A + ∠ABC
⇒ ∠ABE + ∠ACD – ∠A = ∠A 4- ∠ACB + ∠A + ∠ABC – ∠A (Subtracting ∠A from both sides)
= ∠A + ∠ABC + ∠ACB = ∠A + ∠B + ∠C = 180° (Sum of angles of a triangle)

Question 12.
In a triangle ABC, if AB = AC and AB is produced to D such that BD = BC, find ∠ACD: ∠ADC.
Solution:
In ∆ABC, AB = AC
AB is produced to D such that BD = BC
DC are joined
In ∆ABC, AB = AC
∴ ∠ABC = ∠ACB
In ∆ BCD, BD = BC
∴ ∠BDC = ∠BCD
and Ext. ∠ABC = ∠BDC + ∠BCD = 2∠BDC (∵ ∠BDC = ∠BCD)
⇒ ∠ACB = 2∠BCD (∵ ∠ABC = ∠ACB)
Adding ∠BDC to both sides
⇒ ∠ACB + ∠BDC = 2∠BDC + ∠BDC
⇒ ∠ACB + ∠BCD = 3 ∠BDC (∵ ∠BDC = ∠BCD)
⇒ ∠ACB = 3∠BDC
RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS Q12.1

Question 13.
In the figure, side BC of AABC is produced to point D such that bisectors of ∠ABC and ∠ACD meet at a point E. If ∠BAC = 68°, find ∠BEC.
RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS Q13.1
Solution:
In the figure,

side BC of ∆ABC is produced to D such that bisectors of ∠ABC and ∠ACD meet at E
∠BAC = 68°
In ∆ABC,
Ext. ∠ACD = ∠A + ∠B
⇒ \(\frac { 1 }{ 2 }\) ∠ACD = \(\frac { 1 }{ 2 }\) ∠A + \(\frac { 1 }{ 2 }\) ∠B
⇒ ∠2= \(\frac { 1 }{ 2 }\) ∠A + ∠1 …(i)
But in ∆BCE,
Ext. ∠2 = ∠E + ∠l
⇒ ∠E + ∠l = ∠2 = \(\frac { 1 }{ 2 }\) ∠A + ∠l [From (i)]
⇒ ∠E = \(\frac { 1 }{ 2 }\) ∠A = \(\frac { { 68 }^{ \circ } }{ 2 }\) =34°

Hope given RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4

January 19, 2023

RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4

Other Exercises

In each of the following, use factor Theorem to find whether polynomial g(x) is a factor of polynomial f(x) or, not: (1-7)
Question 1.
f(x) = x³ – 6x² + 11x – 6; g(x) = x – 3
Solution:
We know that if g(x) is a factor of p(x),
then the remainder will be zero. Now,
f(x) = x³ – 6x² + 11x – 6; g(x) = x -3
Let x – 3 = 0, then x = 3
∴ Remainder = f(3)
= (3)³ – 6(3)² +11 x 3 – 6
= 27-54 + 33 -6
= 60 – 60 – 0
∵ Remainder is zero,
∴ x – 3 is a factor of f(x)

Question 2.
f(x) = 3X⁴ + 17x³ + 9x² – 7x – 10; g(x) = x + 5
Solution:
f(x) = 3x⁴ + 17X³ + 9x² – 7x – 10; g(x) = x + 5
Let x + 5 = 0, then x = -5
∴ Remainder = f(-5) = 3(-5)⁴ + 17(-5)³ + 9(-5)² – 7(-5) – 10
= 3 x 625 + 17 x (-125) + 9 x (25) – 7 x (-5) – 10
= 1875 -2125 + 225 + 35 – 10
= 2135 – 2135 = 0
∵ Remainder = 0
∴ (x + 5) is a factor of f(x)

Question 3.
f(x) = x⁵ + 3x⁴ – x³ – 3x² + 5x + 15, g(x) = x + 3
Solution:
f(x) = x⁵ + 3X⁴ – x³ – 3x² + 5x + 15, g(x) = x + 3
Let x + 3 = 0, then x = -3
∴ Remainder = f(-3)
= (-3)⁵ + 3(-3)⁴ – (-3)³ – 3(-3)² + 5(-3) + 15
= -243 + 3 x 81 -(-27)-3 x 9 + 5(-3) + 15
= -243 +243 + 27-27- 15 + 15
= 285 – 285 = 0
∵ Remainder = 0
∴ (x + 3) is a factor of f(x)

Question 4.
f(x) = x³ – 6x² – 19x + 84, g(x) = x – 7
Solution:
f(x) = x³ – 6x² – 19x + 84, g(x) = x – 7
Let x – 7 = 0, then x = 7
∴ Remainder = f(7)
= (7)³ – 6(7)² – 19 x 7 + 84
= 343 – 294 – 133 + 84
= 343 + 84 – 294 – 133
= 427 – 427 = 0
∴ Remainder = 0
∴ (x – 7) is a factor of f(x)

Question 5.
f(x) = 3x³ + x² – 20x + 12, g(x) = 3x – 2
Solution:
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4 Q5.1

Question 6.
f(x) = 2x³ – 9x² + x + 12, g(x) = 3 – 2x
Solution:
f(x) = 2x³ – 9x² + x + 12, g(x) = 3 – 2x
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4 Q6.1

Question 7.
f(x) = x³ – 6x² + 11x – 6, g(x) = x² – 3x + 2
Solution:
g(x) = x² – 3x + 2
= x² – x – 2x + 2
= x(x – 1) – 2(x – 1)
= (x – 1) (x – 2)
If x – 1 = 0, then x = 1
‍∴ f(1) = (1)³ – 6(1)² + 11(1) – 6
= 1-6+11-6= 12- 12 = 0
‍∴ Remainder is zero
‍∴ x – 1 is a factor of f(x)
and if x – 2 = 0, then x = 2
∴ f(2) = (2)³ – 6(2)² + 11(2)-6
= 8 – 24 + 22 – 6 = 30 – 30 = 0
‍∴ Remainder = 0
‍∴ x – 2 is also a factor of f(x)

Question 8.
Show that (x – 2), (x + 3) and (x – 4) are factors of x³ – 3x² – 10x + 24.
Solution:
f(x) = x³ – 3x² – 10x + 24
Let x – 2 = 0, then x = 2
Now f(2) = (2)³ – 3(2)² – 10 x 2 + 24
= 8 – 12 – 20 + 24 = 32 – 32 = 0
‍∴ Remainder = 0
‍∴ (x – 2) is the factor of f(x)
If x + 3 = 0, then x = -3
Now, f(-3) = (-3)³ – 3(-3)² – 10 (-3) + 24
= -27 -27 + 30 + 24
= -54 + 54 = 0
∴ Remainder = 0
∴ (x + 3) is a factor of f(x)
If x – 4 = 0, then x = 4
Now f(4) = (4)³ – 3(4)² – 10 x 4 + 24 = 64-48 -40 + 24
= 88 – 88 = 0
∴ Remainder = 0
∴ (x – 4) is a factor of (x)
Hence (x – 2), (x + 3) and (x – 4) are the factors of f(x)

Question 9.
Show that (x + 4), (x – 3) and (x – 7) are factors of x³ – 6x² – 19x + 84.
Solution:
Let f(x) = x³ – 6x² – 19x + 84
If x + 4 = 0, then x = -4
Now, f(-4) = (-4)³ – 6(-4)² – 19(-4) + 84
= -64 – 96 + 76 + 84
= 160 – 160 = 0
∴ Remainder = 0
∴ (x + 4) is a factor of f(x)
If x – 3 = 0, then x = 3
Now, f(3) = (3)³ – 6(3)² – 19 x 3 + 84
= 27 – 54 – 57 + 84
= 111 -111=0
∴ Remainder = 0
∴ (x – 3) is a factor of f(x)
and if x – 7 = 0, then x = 7
Now, f(7) = (7)³ – 6(7)² – 19 x 7 + 84
= 343 – 294 – 133 + 84
= 427 – 427 = 0
∴ Remainder = 0
∴ (x – 7) is also a factor of f(x)
Hence (x + 4), (x – 3), (x – 7) are the factors of f(x)

Question 10.
For what value of a (x – 5) is a factor of x³ – 3x² + ax – 10?
Solution:
f(x) = x³ – 3x² + ax – 10
Let x – 5 = 0, then x = 5
Now, f(5) = (5)³ – 3(5)² + a x 5 – 10
= 125 – 75 + 5a – 10
= 125 – 85 + 5a = 40 + 5a
∴ (x – 5) is a factor of fix)
∴ Remainder = 0
⇒ 40 + 5a = 0 ⇒ 5a = -40
⇒ a = \(\frac { -40 }{ 5 }\)= -8
Hence a = -8

Question 11.
Find the value of a such that (x – 4) is a factor of 5x³ – 7x² – ax – 28.
Solution:
Let f(x) = 5x³ – 7x² – ax – 28
and Let x – 4 = 0, then x = 4
Now, f(4) = 5(4)³ – 7(4)² – a x 4 – 28
= 5 x 64 – 7 x 16 – 4a – 28
= 320 – 112 – 4a – 28
= 320 – 140 – 4a
= 180 – 4a
∴ (x – 4) is a factor of f(x)
∴ Remainder = 0
⇒ 180 -4a = 0
⇒ 4a = 180
⇒ a = \(\frac { 180 }{ 4 }\) = 45
∴ a = 45

Question 12.
Find the value of a, if x + 2 is a factor of 4x⁴ + 2x³ – 3x² + 8x + 5a.
Solution:
Let f(x) = 4x⁴ + 2x³ – 3x² + 8x + 5a
and Let x + 2 = 0, then x = -2
Now, f(-2) = 4(-2)⁴ + 2(-2)³ – 3(-2)² + 8 x ( 2) + 5a
= 4 x 16 + 2(-8) – 3(4) + 8 (-2) + 5a
= 64- 16- 12- 16 +5a
= 64 – 44 + 5a
= 20 + 5a
∴ (x + 2) is a factor of fix)
∴ Remainder = 0
⇒ 20 + 5a = 0 ⇒ 5a = -20
⇒ a =\(\frac { -20 }{ 5 }\) = -4
∴ a = -4

Question 13.
Find the value of k if x – 3 is a factor of k²x³ – kx² + 3kx – k.
Solution:
Let f(x) = k²x³ – kx² + 3kx – k
and Let x – 3 = 0, then x = 3
Now,f(3) = k²(3)³ – k(3)² + 3k(3) – k
= 27k² – 9k + 9k-k
= 27k²-k
∴ x – 3 is a factor of fix)
∴ Remainder = 0
∴ 27k² – k = 0
⇒ k(27k – 1) = 0 Either k = 0
or 21k – 1 = 0
⇒ 21k = 1
∴ k= \(\frac { 1 }{ 27 }\)
∴ k = 0,\(\frac { 1 }{ 27 }\)

Question 14.
Find the values of a and b, if x² – 4 is a factor of ax⁴ + 2x³ – 3x² + bx – 4.
Solution:
f(x) = ax⁴ + 2x³ – 3x² + bx – 4
Factors of x² – 4 = (x)² – (2)²= (x + 2) (x – 2)
If x + 2 = 0, then x = -2
Now, f(-2) = a(-2)⁴ + 2(-2)³ – 3(-2)² + b(-2) – 4
16a- 16 – 12-26-4
= 16a -2b-32
∵ x + 2 is a factor of f(x)
∴ Remainder = 0
⇒ 16a – 2b – 32 = 0
⇒ 8a – b – 16 = 0
⇒ 8a – b = 16 …(i)
Again x – 2 = 0, then x = 2
Now f(2) = a x (2)⁴ + 2(2)³ – 3(2)² + b x 2-4
= 16a + 16- 12 + 26-4
= 16a + 2b
∵ x – 2 is a factor of f(x)
∴ Remainder = 0
⇒ 16a + 2b = 0
⇒ 8a + b= 0 …(ii)
Adding (i) and (ii),
⇒ 16a = 16
⇒ a = \(\frac { 16 }{ 16 }\) = 1
From (ii) 8 x 1 + b = 0
⇒ 8 + b = 0
⇒ b = – 8
∴ a = 1, b = -8

Question 15.
Find α and β, if x + 1 and x + 2 are factors of x³ + 3x² – 2αx +β.
Solution:
Let f(x) = x³ + 3x² – 2αx + β
and Let x + 1 = 0 then x = -1
Now,f(-1) = (1)³ + 3(-1)² – 2α (-1) +β
= -1 + 3 + 2α + β
= 2 + 2α + β
∵ x + 1 is a factor of f(x)
∴ Remainder = 0
∴ 2 + 2α + β = 0
⇒ 2α + β = -2 …(i)
Again, let x + 2 = 0, then x = -2
Now, f(-2) = (-2)³ + 3(-2)² – 2α(-2) + β
= -8 + 12 + 4α+ β
= 4 + 4α+ β
∵ x + 2 is a factor of(x)
∴ Remainder = 0
∴ 4+ 4α + β = 0
⇒ 4α + β = -4 …(ii)
Subtracting (i) from (ii),
2α = -2
⇒ α = \(\frac { -2 }{ 2 }\) = -1
From (ii), 4(-1) + β = -4
-4 + β= -4
⇒ β =-4+ 4 = 0
∴ α = -1, β = 0

Question 16.
If x – 2 is a factor of each of the following two polynomials, find the values of a in each case:
(i) x³ – 2ax² + ax – 1
(ii) x⁵ – 3x⁴ – ax³ + 3ax² + 2ax + 4
Solution:
(i) Let f(x) = x³ – 2ax² + ax – 1 and g(x) = x – 2
and let x – 2 = 0, then x = 2
∴ x – 2 is its factor
∴ Remainder = 0
f(2) = (2)³ – 2a x (2)² + a x 2 – 1
= 8-8a+ 2a-1 = 7-6a
∴ 7 – 6a = 0
⇒ 6a = 7
⇒ a = \(\frac { 7 }{ 6 }\)
∴ a = \(\frac { 7 }{ 6 }\)
(ii) Let f(x) = x⁵ – 3x⁴ – ax³ + 3 ax² + 2ax + 4 and g(x) = x – 2
Let x – 2 = 0, then x=2
∴ f(2) = (2)⁵ – 3(2)⁴ – a(2³) + 3a (2)² + 2a x 2 + 4
= 32 – 48 – 8a + 12a + 4a + 4
= -12 + 8a
∴ Remainder = 0
∴ -12 + 8a = 0
⇒ 8a= 12
⇒ a = \(\frac { 12 }{ 8 }\) = \(\frac { 3 }{ 2 }\)
∴ Hence a = \(\frac { 3 }{ 2 }\)

Question 17.
In each of the following two polynomials, find the values of a, if x – a is a factor:
(i) x⁶ – ax⁵ + x⁴-ax³ + 3x-a + 2
(ii) x⁵ – a²x³ + 2x + a + 1
Solution:
(i) Let f(x) ⁼ x⁶– ax⁵+x⁴-ax³ + 3x-a + 2 and g(x) = x – a
∴ x – a is a factor
∴ x – a = 0
⇒ x = a
Now f(a) = a⁶-a x a⁵ + a⁴-a x a³ + 3a – a + 2
= a⁶-a⁶ + a⁴-a⁴ + 2a + 2
= 2a + 2
∴ x + a is a factor of p(x)
∴ Remainder = 0
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4 Q17.1

Question 18.
In each of the following, two polynomials, find the value of a, if x + a is a factor.
(i) x³ + ax² – 2x + a + 4
(ii) x⁴ – a²r + 3x – a
Solution:
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4 Q18.1

Question 19.
Find the values of p and q so that x⁴ + px³+ 2x² – 3x + q is divisible by (x² – 1).
Solution:
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4 Q19.1

Question 20.
Find the values of a and b so that (x + 1) and (x – 1) are factors of x⁴ + ax³ 3x² + 2x + b.
Solution:
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4 Q20.1

Question 21.
If x³ + ax² – bx + 10 is divisible by x² – 3x + 2, find the values of a and b.
Solution:
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4 Q21.1

Question 22.
If both x + 1 and x – 1 are factors of ax³ + x² – 2x + b, find the values of a and b.
Solution:
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4 Q22.1

Question 23.
What must be added to x³ – 3x² – 12x + 19 so that the result is exactly divisibly by x² + x – 6?
Solution:
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4 Q23.1

Question 24.
What must be subtracted from x³ – 6x² – 15x + 80-so that the result is exactly divisible by x² + x – 12?
Solution:
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4 Q24.1

Question 25.
What must be added to 3x³ + x² – 22x + 9 so that the result is exactly divisible by 3x²+ 7x – 6?
Solution:
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4 Q25.1

Hope given RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.4

January 19, 2023

RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.4

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.4

Other Exercises

Question 1.
Give the geometric representations of the following equations.
(a) on the number line
(b) on the cartesian plane.
(i) x – 2
(ii) y + 3 = 0
(iii) y = 3
(iv) 2x + 9 = 0
(v) 3x – 5 = 0
Solution:
(i) x = 2
(i) on the number line

(ii) x = 2 is a line parallel to 7-axis at a distance of 2 units to right of y-axis.
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.4 Q1.2
(ii) y = -3 is a line parallel to x-axis at a distance of 3 units below x-axis.

(iii) y = 3
(i) y = 3

(ii) y = 3 is a line parallel to x-axis at a distance of 3 units above x-axis.

x = -4.5 is a line parallel to 7-axis at a distance of 4.5 units to left of y-axis.
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.4 Q1.7

(ii) x = 1\(\frac { 2 }{ 3 }\) is a line parallel to y-axis at a distance of 1\(\frac { 2 }{ 3 }\) unit to right side of y-axis.

Question 2.
Give the geometrical representation of 2x + 13 = 0 as an equation in
(i) One variable
(ii) Two variables
Solution:
(i) In one variable,
2x + 13 = 0
⇒ 2x = – 13
⇒ x = \(\frac { -13 }{ 2 }\)
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.4 Q2.1
is a line parallel to y-axis at a distance of -6 \(\frac { 1 }{ 2 }\) units on the left side of y-axis.

Question 3.
Solve the equation 3x + 2 = x -8, and represent on
(i) the number line
(ii) the Cartesian plane.
Solution:
3x + 2 = x – 8
⇒ 3x – x = -8 – 2
⇒ 2x = -10
⇒ x = \(\frac { -10 }{ 2 }\) = -5
(i) on the number line s = -5
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.4 Q3.1
(ii) x = -5 is a line parallel to y-axis at a distance of 5 knot’s left of y-axis.

Question 4.
Write the equal of the line that is parallel to x-axis and passing through the points.
(i) (0, 3)
(ii) (0, -4)
(iii) (2, -5)
(iv)    (3, 4)
Solution:
∵ A line parallel to x-axis will be of the type y = a
∴ (i) y = 3
(ii) y = -4
(iii) y = -5 and y = 4 are equations of the lines parallel to x-axis

Question 5.
Write the equation of the line that is parallel to y-axis and passing through the points.
(i) (4, 0)
(ii) (-2, 0)
(iii) (3, 5)
(iv) (-4, -3)
Solution:
∵ A line parallel to y-axis will be of the type x = a
∴ (i) x = 4, (ii) x = -2, x = 3 and x = -4 are the equations of the lines parallel to y-axis.

Hope given RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.4 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3

January 19, 2023

RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3

Other Exercises

Question 1.
Draw the graph of each of the following linear equations in two variables.
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q1.1
Solution:
(i)x + y = 4
x = 4 – y
If y = 0, then x = 4
If y = 4, then x = 0
Now plot the points (4, 0) and (0, 4) on the graph and join them ro get the graph of the given equation

(ii)x – y = 2
x = 2 +y
If y = 0, then x = 2 and if y = 1,
Then x = 2 + 1 = 3
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q1.3
Now plot the points (2, 0) and (3, 1) on the graph and join them to get the graph of the equation.

(iii) -x+y = 6 ⇒ y = 6+x

If x = 0, then y = 6 + 0 = 6
If x = -1, then y = 6 – 1 = 5

Now plot the points (0, 6) and (-1, 5) on the graph and join them to get a graph of the line.
(iv) y = 2x
If x = 0, then y = 2 x 0 = 0
If x = 1, then y = 2 x 1 = 2
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q1.7

Now plot the points (0, 0) and (1, 2) on the graph and join them to get the graph of the line.

Now plot the points (5, 0) and (0, 3) on the graph and join them to get the graph of the line.

Now plot the points (4, 0) and (2, -3) on the graph and join them to get the graph of the line.
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q1.12

Now plot the points (-1, 2) and (2, 3) on the graph and join then to get the graph of the line.

Now plot the points (1, 0) and (-1, 1) on the graph and join then to get the graph of the line.

Question 2.
Give the equations of two lines passing through (3, 12). How many more such lines are there, and why ?
Solution:
∵ Points (3, 12) lies on the lines passing through the points
∴ Solutions is x = 3,y- 12
∴ Possible equation can be
x + y = 15
-x+y = 9
4x-y = 0
3x – y + 3 = 0

Question 3.
A three-wheeler scooter charges ₹15 for first kilometer and ₹8 each for every subsequent kilometer. For a distance of x km, an amount of ₹y is paid. Write the linear equation representing the above information.
Solution:
Charges for the first kilometer = ₹15
Charges for next 1 km = ₹8
Distance = x km
and total amount = ₹y
∴ Linear equation will be,
15 + (x- 1) x 8 =y
⇒ 15 + 8x – 8 = y
⇒ 7 + 8x = y
∴ y = 8x + 7

Question 4.
Plot the points (3, 5) and (-1, 3) on a graph paper and verify that the straight line passing through these points also passes through the point (1, 4).
Solution:
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q4.1
Points (3, 5) and (-1, 3) have been plotted on the graph and joined to get a line. We see that die point (1,4) also lies out.

Question 5.
From the choices given below, choose the equation whose graph is given in figure.
(i) y = x
(ii) x + y = 0
(iii) y = 2x
(iv) 2 + 3y = 7x
Solution:
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q5.1
From the graph, we see that Points (-1, 1) and (1, -1) be on the graph of the line these will satisfy the equation of the line
∴ -x = y ⇒ x+ y = 0
i.e, required equation
∵ x + y = 0 is the graph of the equation.

Question 6.
From the choices given below, choose the equation whose graph is given in figure.
(i) y = x + 2
(ii) y = x – 2
(ii) y = -x + 2
(iv) x + 2y = 6
Solution:
From the graph
Points (-1,3) and (2, 0) lie on the graph of the line
Now there points, by observation, satisfy the equation y= -x+2
∴ Required equation is y = -x + 2 whose graph is given.
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q6.1

Question 7.
If the point (2, -2) lies on the graph of the linear equation 5x + ky =4, find the value of k.
Solution:
∵ Point (2, -2) lies on the graph of the linear equation 5x + ky = 4
∴ It will satisfy it
∴ Now substituting the values of x and y 5 x 2 + k (-2) = 4
⇒ 10 – 2k = 4 ⇒ -2k = 4 – 10 = -6 -6
⇒ k= \(\frac { -6 }{ -2 }\) =3
Hence k = 3

Question 8.
Draw the graph of the equation 2x + 3p = 12. From the graph find the co-ordinates of the point.
(i) whose y -coordinates is 3
(ii) whose x-coordinates is -3
Solution:
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q8.1

Plot the points (6, 0) and (0, 4) on the graph and join them to get the graph if the line.
(i) If y = 3, then draw perpendicular from y = 3 to the line, which get meets it at P then x-coordinate of p will be
∴ coordinates of P are ( \(\frac { 3 }{ 2 }\) ,3)
(ii) If x = -3, draw perpendicular from x = -3 to the line, which meets it Q.
The y coordinates of Q will be y = 6
∴ co-ordinates of Q are (-3, 6)

Question 9.
Draw the graph of each of the equations given below. Also, find the coordinates of the points where the graph cuts the coordinates axes:
(i) 6x – 3y = 12
(ii) -x + 4y = 8
(iii) 2x + y = 6
(iv) 3x + 2y + 6 = 0
Solution:
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q9.1

Now plot the points of each equation and join then we get four lines as shown on the graphs.
Equation (i) cuts the axes at (2, 0) and (0, -4)
Equation (ii) cuts the axes at (-8, 0) and (0, 2)
Equation (iii) cuts the axes at (3, 0), (0, 6) and
Equation (iv), cuts the axes at (-2, 0) and (0,-3)
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q9.3

Question 10.
A lending library has a fixed charges for the first three days and an additional charge for each day thereafter. Aarushi paid ₹27 for a book kept for seven days. If fixed charges are ₹x and per day charges are ₹y. Write the linear equation representing the above information.
Solution:
Let fixed charges for first 3 days = ₹x
and additional charges for each day = ₹y
Total period = 7 days
and amount charges = ₹27
∴ x + (7 – 3) x y = 27
⇒ x + 4y = 27
Hence x + 4y = 27

Question 11.
A number is 27 more than the number obtained by reversing its digits. If its unit’s and ten’s digit are x and y respectively, write the linear equation representing the above statement.
Solution:
Let unit’s digit = x
and tens digit = y
∴ Number = x + 10y
By reversing the digits, units digit = y
and ten’s digit = x
∴ number = y + 10x
Now difference of these two numbers = 27 (x + 10y) – (y +10x) = 27
x + 10y – y – 10x = 27
⇒ -9x + 9y – 27 = 0
⇒ x-_y + 3 = 0 (Dividing by -9)
Hence equation is x – y + 3 = 0

Question 12.
The sum of a two digit number and the number obtained by reversing the order of its digits is 121. If units and ten’s digit of the number are x and y respectively, then write the linear equation representing the above statement.
Solution:
Let unit digit = x
and tens digit = y
∴ Number = x + 10y
By reversing the digits,
units digit = y
and tens digit = x
∴ Number =y+ 10x
Now sum of these two numbers = 121
∴ x + 10y + y + 10x = 121
⇒ 1 lx + 11y = 121
⇒ x + y = 11 (Dividing by 11)
∴ x + y = 11

Question 13.
Draw the graph of the equation 2x + y = 6. Shade the region bounded by the graph and the coordinate axes. Also find the area of the shaded region.
Solution:
2x + y = 6
⇒ y = 6 – 2x
If x = 0, then y = 6- 2 x 0 = 6 – 0 = 6
If x = 2, then y = 6- 2 x 2 = 6- 4 = 2
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q13.1
Now plot the points (0, 6) and (2, 2) on the graph and join them to get a line which intersects x-axis at (3, 0) and y-axis at (0,6)
Now co-ordinates if vertices of the shaded portion are (6, 0) (0, 0) and (3, 0) Now area of the shaded region.
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q13.2

Question 14.
Draw the graph of the equation \(\frac { x }{ 3 }\) + \(\frac { y }{ 4 }\) = 1 Also find the area of the triangle formed by the line and the co-ordinate axes.
Solution:
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q14.1
Now plot the points (3, 0) and (0, 4) and join them to get a line which interest x-axis at A (3, 0) and y-axis at B (0, 4)

Question 15.
Draw the graph of y = | x |
Solution:
y = | x |
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q15.1
⇒ y = x [∵ | x |=x]
∴ Now taking z points.

Now plot the points (1, 1) (2, 2) and (3, 3) and join them to get a graph of a line.

Question 16.
Draw the graph of y = | x | + 2
Solution:
y – | x | + 2
⇒ y = x + 2 [| x | = x]
If x = 0, then y = 0 + 2 = 2
If x = 1, then y = 1+2 = 3
If x = 2, then y = 2 + 2 = 4
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q16.1
Now plot the points (0, 2), (1, 3) and (2, 4) on the graph and join them to get a line.

Question 17.
Draw the graphs of the following linear equation on the same graph paper.
2x + 3y = 12, x -y = 1
Find the co-ordinates of the vertices of the triangle formed by the two straight lines and the y-axis. Also find the area of the triangle.
Solution:
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q17.1
Now plot the points (6, 0) (0, 4) on the graph to get a line.

Now plot the points (1, 0) and (2, 1) on the graph to get another line.
Area of the triangle FEB so formed,
= \(\frac { 1 }{ 2 }\) FB x FL = \(\frac { 1 }{ 2 }\) x 5 x 3
= \(\frac { 15 }{ 2 }\)
= 7.5 sq. units
co-ordinates of E, F, B are E (3, 2), (0, -1) and (0, 4)

Question 18.
Draw the graphs of the linear equations 4x – 3y + 4 = 0 and 4x + 3y – 20 = 0. Find the area bounded by these lines and x-axis.
Solution:
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q18.1

Now plot the points (5, 0) and (2, 4)and join them to get a line we see that the ΔABC is formed by bounding there line with x-axis.

Question 19.
The path of a train A is given by the equation 3x + 4y – 12 = 0 and the path of another train B is given by the equation 6jc + 8y – 48 = 0. Represent this situation graphically.
Solution:
Path of the train A = 3x + 4y – 12 = 0
Path of the train B = 6x + 8y – 48 = 0
Now, 3x + 4y – 12 = 0
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q19.1

Now plot the points (4, 0) and (0, 3) on the graph and join them to get a line, and 6x + 8y – 48 = 0
⇒ 6x = 48 – 8y

Now plot the points (0, 6) and (4, 3) on the graph and join them to get another line.

Question 20.
Ravish tells his daughter Aarushi, “Seven years ago, I was seven times as old as you were then. Also, three years from now, I shall be three times as old as you will be”. If present ages of Aarushi and Ravish are x and y years respectively, represent this situation algebraically as well as graphically.
Solution:
Present age of Aarushi = x years
and age of Ravish = y years
7 years ago,
age of Aarushi = x – 7
years and age of Ravish =y-7 years
∴ y- 7 = 7 (X – 7)
⇒ y – 7 = 7x – 49
⇒ 7x – y = -7 + 49 = 42
7x – y = 42
⇒ y = 7x – 42
If x = 6, then
y = 7 x 6 – 42 = 42 – 42 = 0,
If x = 7, then
y = 7 x 7 – 42 – 49 – 42 = -7
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q20.1
Plot the points (6, 0) (7, -7) on the graph and join them.
After 3 years,
age of Aarushi = x + 3
and age of Ravish = y + 3
⇒ y + 3 = 3(x + 3)
⇒ y + 3 = 3x + 9
⇒ y = 3x+ 9-3
⇒ y = 3x + 6
If x = -2, then y = 3 x (-2) + 6 =6-6=0
If x = 1, then y = 3 x (1) + 6 =3+6=9
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q20.2
Plot the points (1, 9), (-2, 0) on the graph Arundeep’s Mathematics (R.D.) 9th and join them to get another line.

Question 21.
Aarushi was driving a car with uniform speed of 60 km/h. Draw distance-time graph. From the graph, find the distance travelled by Aarushi in.
(i) 2\(\frac { 1 }{ 2 }\) Hours
(ii) \(\frac { 1 }{ 2 }\) Hour
Solution:
Speed of car = 60 km / h.
RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 Q21.1
Now plot the points (60, 1), (120, 2) are the graph and join then to get the graph of line.
From the graph, we see that

Hope given RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 10 Congruent Triangles Ex 10.1

January 19, 2023

RD Sharma Class 9 Solutions Chapter 10 Congruent Triangles Ex 10.1

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 10 Congruent Triangles Ex 10.1

Other Exercises

Question 1.
Write the complement of each of the following angles:
(i) 20°
(ii) 35°
(iii) 90°
(iv) 77°
(v) 30°
Solution:
We know that two angles are complement to each other if their sum is 90°. Therefore,
(i) Complement of 20° is (90° – 20°) = 70°
(ii) Complement of 35° is (90° – 35°) = 55°
(iii) Complement of 90° is (90° – 90°) = 0°
(iv) Complement of 77° is (90° – 77°) = 13°
(v) Complement of 30° is (90° – 30°) = 60°

Question 2.
Write the supplement of each of the following angles:
(i) 54°
(ii) 132°
(iii) 138°
Solution:
We know that two angles are supplement to each other if their sum if 180°. Therefore,
(i) Supplement of 54° is (180° – 54°) = 126°
(ii) Supplement of 132° is (180° – 132°) = 48°
(iii) Supplement of 138° is (180° – 138°) = 42°

Question 3.
If an angle is 28° less than its complement, find its measure.
Solution:
Let required angle = x, then
Its complement = x + 28°
∴ x + x + 28° = 90° ⇒ 2x = 90° – 28° = 62°
∴ x = \(\frac { { 62 }^{ \circ } }{ 2 }\) = 31°
∴ Required angle = 31°

Question 4.
If an angle is 30° more than one half of its complement, find the measure of the angle.
Solution:
Let the measure of the required angle = x
∴ Its complement = 90° – x
∴ x = \(\frac { 1 }{ 2 }\) (90° – x) + 30°
2x = 90° – x + 60°
⇒ 2x + x = 90° + 60°
⇒ 3x = 150°
⇒ x = \(\frac { { 150 }^{ \circ } }{ 3 }\) = 50°
∴ Required angle = 50°

Question 5.
Two supplementary angles are in the ratio 4 : 5. Find the angles.
Solution:
Ratio in two supplementary angles = 4 : 5
Let first angle = 4x
Then second angle = 5x
∴ 4x + 5x = 180
⇒ 9x = 180°
∴ x = \(\frac { { 180 }^{ \circ } }{ 9 }\) = 20°
∴ First angle = 4x = 4 x 20° = 80°
and second angle = 5x
= 5 x 20° = 100°

Question 6.
Two supplementary angles differ by 48°. Find the angles.
Solution:
Let first angle = x ”
Then second angle = x + 48°
∴ x + x + 48° = 180°⇒ 2x + 48° = 180°
⇒ 2x = 180° – 48° = 132°
x= \(\frac { { 132 }^{ \circ } }{ 2 }\) =66°
∴ First angle = 66°
and second angle = x + 48° = 66° + 48° = 114°
∴ Angles are 66°, 114°

Question 7.
An angle is equal to 8 times its complement. Determine its measure.
Solution:
Let the required angle = x
Then its complement angle = 90° – x
∴ x = 8(90° – x)
⇒ x = 720° – 8x ⇒ x + 8x = 720°
⇒ 9x = 720° ⇒ x = \(\frac { { 720 }^{ \circ } }{ 9 }\) = 80°
∴ Required angle = 80°

Question 8.
If the angles (2x – 10)° and (x – 5)° are complementary angles, find x.
Solution:
First complementary angle = (2x – 10°) and second = (x – 5)°
∴ 2x – 10° + x – 5° = 90°
⇒ 3x – 15° = 90° ⇒ 3x = 90° + 15° = 105°
∴ x = \(\frac { { 105 }^{ \circ } }{ 3 }\) = 35°
∴ First angle = 2x – 10° = 2 x 35° – 10°
= 70° – 10° = 60°
and second angle = x – 5 = 35° – 5 = 30°

Question 9.
If an angle differ from its complement by 10°, find the angle.
Solution:
Let required angle = x°
Then its complement angle = 90° – x°
∴ x – (90° – x) = 10
⇒ x – 90° + x = 10°⇒ 2x = 10° + 90° = 100° 100°
⇒ x = \(\frac { { 100 }^{ \circ } }{ 2 }\) = 50°
∴ Required angle = 50°

Question 10.
If the supplement of an angle is two-third of itself Determine the angle and its supplement.
Solution:
Let required angle = x
Then its supplement angle = 180° – x
∴ (180°-x)= \(\frac { 2 }{ 3 }\)x
540° – 3x = 2x ⇒ 2x + 3x = 540°
⇒ 5x = 540°⇒ x = \(\frac { { 540 }^{ \circ } }{ 5 }\) = 108°
-. Supplement angle = 180° – 108° = 72°

Question 11.
An angle is 14° more than its complementary angle. What is its measure?
Solution:
Let required angle = x
Then its complementary angle = 90° – x
∴ x + 14° = 90° – x
x + x = 90° – 14° ⇒ 2x = 76°
⇒ x = \(\frac { { 76 }^{ \circ } }{ 2 }\) = 38°
∴ Required angle = 38°

Question 12.
The measure of an angle is twice the measure of its supplementary angle. Find its measure.
Solution:
Let the required angle = x
∴ Its supplementary angle = 180° – x
∴ x = 2(180°-x) = 360°-2x
⇒ x + 2x = 360°
⇒ 3x = 360°
⇒ x = \(\frac { { 360 }^{ \circ } }{ 3 }\) = 120°
∴ Required angle = 120°

Question 13.
If the complement of an angle is equal to the supplement of the thrice of it. Find the measure of the angle.
Solution:
Let required angle = x
Then its complement angle = 90° – x
and supplement angle = 180° – x
∴ 3(90° – x) = 180° – x
⇒ 270° – 3x = 180° – x
⇒270° – 180° = -x + 3x => 2x = 90°
⇒ x = 45°
∴ Required angle = 45°

Question 14.
If the supplement of an angle is three times its complement, find the angle.
Solution:
Let required angle = x
Then its complement = 90°- x
and supplement = 180° – x
∴ 180°-x = 3(90°-x)
⇒ 180° – x = 270° – 3x
⇒ -x + 3x = 270° – 180°
⇒ 2x = 90° ⇒ x = \(\frac { { 90 }^{ \circ } }{ 2 }\) =45°
∴ Required angle = 45°

Hope given RD Sharma Class 9 Solutions Chapter 10 Congruent Triangles Ex 10.1 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry MCQS

January 19, 2023

RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry MCQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry MCQS

Other Exercises

Mark the correct alternative in each of the following:
Question 1.
If (4, 19) is a solution of the equation y = ax + 3, then a =
(a) 3
(b) 4
(c) 5
(d) 6
Solution:
∵ (4, 19) is a solution of equation
y = ax + 3
∴ x = 4, y= 19 will satisfy the equation
∴ 19 = a x 4 + 3 = 4a + 3
4a = 19-3 = 16 ⇒ a= \(\frac { 16 }{ 4 }\) = 4
∴ a = 4 (b)

Question 2.
If (a, 4) lies on the graph of 3x + y = 10, then the value of a is
(a) 3
(b) 1
(c) 2
(d) 4
Solution:
∵ (a, 4) is the solution of the equation 3x + y = 10
∴ x = a, y = 4 will satisfy the equation
∴ Substituting the value of x and y in the equation
3 xa + 4= 10 ⇒ 3a =10- 4 = 6
⇒ a = \(\frac { 6 }{ 3 }\) = 2
∴ a = 2 (c)

Question 3.
The graph of the linear equation 2x – y= 4 cuts x-axis at
(a) (2, 0)
(b) (-2, 0)
(c) (0, -4)
(d) (0, 4)
Solution:
∵ graph of the equation,
2x – y = 4 cuts x-axis
∴ y = 0
∴ 2x – 0 = 4 ⇒ 2x = 4
⇒ x = \(\frac { 4 }{ 2 }\) = 2
∴ The line cuts x-axis at (2, 0) (a)

Question 4.
How many linear equations are satisfied by x = 2 and y = -3 ?
(a) Only one
(b)   Two
(c) Three
(d)    Infinitely many
Solution:
∵ From a point, infinitely number of lines can pass.
∴ The solution x = 2, y = -3 is the solution of infinitely many linear equations.       (d)

Question 5.
The equation x – 2 = 0 on number line is represented by
(a) aline
(b) a point
(c) infinitely many lines
(d) two lines
Solution:
The equation x – 2 = 0
⇒ x = 2
∴ It is representing by a point on a number line. (b)

Question 6.
x = 2, y = -1 is a solution of the linear equation
(a) x   + 2y = 0
(b) x + 2y = 4
(c) 2x + y = 0
(d) 2x + y = 5
Solution:
x = 2, y = -1
Substituting the values of x and y in the equations one by one, we get (a) x + 2y = 0
⇒ 2 + 2(-1) = 0
⇒ 2 – 2 = 0
⇒ 0 = 0 which is true                             (a)

Question 7.
If (2k – 1, k) is a solution of the equation 10x – 9y = 12, then k =
(a) 1
(b) 2
(c) 3
(d) 4
Solution:
∵ (2k – 1, k) is a solution of the equation 10x – 9y = 12
Substituting the value of x and y in the equation
10(2k – 1) – 9k = 12
⇒ 20k – 10-9k= 12
⇒ 20k – 9k = 12 + 10
⇒ 11k = 22
⇒ k =\(\frac { 22 }{ 11 }\) = 2∴ k = 2                                                 (b)

Question 8.
The distance between the graph of the equation x = – 3    and x   = 2      is
(a) 1
(b) 2
(c) 3
(d) 5
Solution:
The distance between the graphs of the equation
x = -3 and x = 2 will be
2(-3) = 2+ 3 = 5 (b)

Question 9.
The distance   between the graphs of the equations y = -1 and y = 3    is
(a) 2
(b) 4
(c) 3
(d) 1
Solution:
The distance between the graphs of the equation
y = -1 and y = 3
is 3 – (-1) = 3 + 1 = 4                            (b)

Question 10.
If the graph of the equation 4x + 3y = 12 cuts the co-ordinate axes at A and B, then hypotenuse of right triangle AOB is of length
(a) 4 units
(b) 3 units
(c) 5 units
(d) none of these
Solution:
Equation is 4x + 3y = 12
If it cuts the x-axis, then y = 0
∴ 4x x 3 x 0 = 12
⇒ 4x = 12 ⇒ x = \(\frac { 12 }{ 4 }\) = 3
OA = 3 units
∴ The point of intersection of x-axis is (3, 0)
Again if it cuts the y-axis, then x = 0 , Y= 0
∴ 4x x 3 x 0 = 12
⇒ 4x = 12 ⇒ x = \(\frac { 12 }{ 3 }\) = 4
⇒ OB = 4 units
∴ The point of intersection is (0, 4)
∴ In right ΔAOB,
AB² = AO² + OB²= (3)² + (4)²
= 9 + 16 = 25
= (5)²∴ AB = 5 units (c)

Hope given RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry MCQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials MCQS

January 19, 2023

RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials MCQS

These Solutions are part of RD Sharma Class 9 Solutions. Here we have given RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials MCQS

Other Exercises

Mark the correct alternative in each of the following:
Question 1.
If x – 2 is a factor of x² + 3 ax – 2a, then a =
(a) 2
(b) -2
(c) 1
(d) -1
Solution:
∴ x – 2 is a factor of
f(x) = x² + 3 ax – 2a
∴ Remainder = 0
Let x – 2 = 0, then x = 2
Now f(2) = (2)² + 3a x 2 – 2a
= 4 + 6a – 2a = 4 + 4a
∴ Remainder = 0
∴ 4 + 4a = 0 ⇒ 4a = -4
⇒ a = \(\frac { -4 }{ 4 }\) = -1
∴ a= -1 (d)

Question 2.
If x³ + 6x² + 4x + k is exactly divisible by x + 2, then k =
(a) -6
(b) -7
(c) -8
(d) -10
Solution:
f(x) – x³ + 6x² + 4x + k is divisible by x + 2
∴ Remainder = 0
Let x + 2 = 0, then x = -2
∴ f(-2) = (-2)³ + 6(-2)² + 4(-2) + k
= -8 + 24-8 + k = 8 + k
∴ x + 2 is a factor
∴ Remainder = 0                                 .
⇒ 8 + k= 0 ⇒ k = -8
k = -8                                                (c)

Question 3.
If x – a is a factor of x³ – 3x² a + 2a²x + b, then the value of b is
(a) 0
(b) 2
(c) 1
(d) 3
Solution:
∴ x – a is a factor of x³ – 3x² a + 2a²x + b
Let f(x) = x³ – 3x² a + 2a²x+ b
and x – a = 0, then x = a
f(a) = a³ – 3a².a + 2a².a + b
= a³ – 3a³ + 2a³ + b = b
∵ x – a is a factor of f(x)
∴ b = 0 (a)

Question 4.
If x¹⁴⁰ + 2x¹⁵¹ + k is divisible by x + 1, then the value of k is
(a) 1
(b) -3
(c) 2
(d) -2
Solution:
∴ x + 1 is a factor of f(x) = x¹⁴⁰ + 2x¹⁵¹ + k
∴ Remainder will be zero
Let x + 1 = 0, then x = -1
∴ f(-1) = (-1)¹⁴⁰ + 2(-1)¹⁵¹ + k
= 1 + 2 x (-1) + k {∵ 140 is even and 151 is odd}
=1-2+k=k-1
∵ Remainder = 0
∴ k – 1=0 ⇒ k=1 (a)

Question 5.
If x + 2 is a factor of x² + mx + 14, then m =
(a) 7
(b) 2
(c) 9
(d) 14
Solution:
x + 2 is a factor of(x) = x² + mx + 14
Let x + 2 = 0, then x = -2
f(-2) = (-2)² + m{-2) + 14
= 4 – 2m + 14 = 18 – 2m
∴ x + 2 is a factor of f(x)
∴ Remainder = 0
⇒ 18 – 2m = 0
2m = 18 ⇒ m = \(\frac { 18 }{ 2 }\) = 9                       (c)

Question 6.
If x – 3 is a factor of x² – ax – 15, then a =
(a) -2
(b) 5
(c) -5
(d) 3
Solution:
x – 3 is a factor of(x) = x² – ax – 15
Let x – 3 = 0, then x = 3
∴ f(3) = (3)² – a(3) – 15
= 9 -3a- 15
= -6 -3a
∴ x – 3 is a factor
∴ Remainder = 0
-6 – 3a = 0 ⇒ 3a = -6
∴ a = \(\frac { -6 }{ 3 }\) = -2 (a)

Question 7.
If x⁵¹ + 51 is divided by x + 1, the remainder is
(a) 0
(b) 1
(c) 49
(d) 50
Solution:
Letf(x) = x⁵¹ + 51 is divisible by x + 1
Let x+1=0, then x = -1
∴ f(-1) = (-1)⁵¹ + 51 =-1+51 (∵ power 51 is an odd integer)
= 50 (d)

Question 8.
If x+ 1 is a factor of the polynomial 2x² + kx, then k =
(a) -2
(b) -3
(c) 4
(d) 2
Solution:
∴ x + 1 is a factor of the polynomial 2x² + kx
Let x+1=0, then x = -1
Now f(x) = 2x² + kx
∴ Remainder =f(-1) = 0
= 2(-1)² + k(-1)
= 2 x 1 + k x (-1) = 2 – k
∴ x + 1 is a factor of f(x)
∴ Remainder = 0
∴ 2 – k = 0 ⇒ k = 2                                 (d)

Question 9.
If x + a is a factor of x⁴ – a²x² + 3x – 6a, then a =
(a) 0
(b) -1
(c) 1
(d) 2
Solution:
x + a is a factor o f(x) = x⁴– a²x² + 3x – 6a
Let x + a = 0, then x = -a
Now, f(-a) – (-a)⁴ -a²(-a)² + 3 (-a) – 6a
= a⁴-a⁴-3a-6a = -9a
∴ x + a is a factor of f(x)
∴Remainder = 0
∴ -9a = 0 ⇒ a = 0                               (a)

Question 10.
The value of k for which x – 1 is a factor of 4x³ + 3x² – 4x + k, is
(a) 3
(b) 1
(c) -2
(d) -3
Solution:
x- 1 is a factor of f(x) = 4x³ + 3x² – 4x + k
Let x – 1 = 0, then x = 1
f(1) = 4(1 )³ + 3(1)² – 4 x 1 + k
= 4+3-4+k=3+k
∴ x- 1 is a factor of f(x)
∴ Remainder = 0
∴ 3 + k = 0 ⇒ k = -3 (d)

Question 11.
If x+2 and x-1 are the factors of x³+ 10x² + mx + n, then the values of m and n are respectively
(a) 5 and-3
(b) 17 and-8
(c) 7 and-18
(d) 23 and -19
Solution:
x+ 2 and x – 1 are the factors of
f(x) = x³ + 10x² + mx + n
Let x + 2 = 0, then x = -2
∴ f(-2) = (-2)³ + 10(-2)² + m(-2) + n
= -8 + 40 – 2m + n = 32 – 2m + n
∴ x + 2 is a factor of f(x)
∴ Remainder = 0
∴ 32 – 2m + n = 0 ⇒ 2m – n = 32   …(i)
Again x – 1 is a factor of f(x)
Let x-1=0, then x= 1
∴ f(1) = (1)³ + 10(1)² + m x 1 +n
= 1 + 10+ m + n =m + n+11
∴ x- 1 is a factor of f(x)
∴ m + n+ 11=0 ⇒ m+n =-11 …(ii)
Adding (i) and (ii),
3m = 32 – 11 = 21
⇒ m = \(\frac { 21 }{ 3 }\) = 7
and n = -11 – m = m = 7, n = -18
∴ m= 7, n = -18 (c)

Question 12.
Let f(x) be a polynomial such that f( \(\frac { -1 }{ 2 }\) )= 0, then a factor of f(x) is
(a) 2x – 1
(b) 2x + b
(c) x- 1
(d) x + 1
Solution:
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials MCQS Q12.1

Question 13.
When x³ – 2x² + ax – b is divided by x² – 2x-3, the remainder is x – 6. The value of a and b are respectively.
(a) -2, -6
(b) 2 and -6
(c) -2 and 6
(d) 2 and 6
Solution:
Let f(x) = x³ – 2x² + ax – b
and Dividing f(x) by x² – 2x + 3
Remainder = x – 6
Let p(x) = x³ – 2x² + ax – b – (x – 6) or x³– 2x² + x(a – 1) – b + 6 is divisible by x² – 2x+ 3 exactly
Now, x²-2x-3 = x²-3x + x- 3
= x(x – 3) + 1(x – 3)
= (x – 3) (x + 1)
∴ x – 3 and x + 1 are the factors of p(x)
Let x – 3 = 0, then x = 3
∴ p(3) = (3)³ – 2(3)² + (a-1)x3-b + 6
= 27-18 + 3a-3-b + 6
= 33-21+3 a-b
= 12 + 3 a-b
∴ x – 3 is a factor
∴ 12 + 3a-b = 0 ⇒ 3a-b = -12               …(i)
Again let x + 1 = 0, then x = -1
∴p(-1) = (-1)³ – 2(-l)² + (a – 1) X (-1) – b + 6
= -1-2 + 1 – a – 6 + 6
= 4 – a- b
∴ x + 1 is a factor
4-a-b = 0 ⇒ a + b = 4                  …(ii)
Adding (i) and (ii),
4 a = -12 + 4 = -8 ⇒ a = \(\frac { -8 }{ 4 }\) = -2
From (ii),
and -2 + 6 = 4⇒ 6 = 4 + 2 = 6
∴ a = -2, h = 6                       (b)

Question 14.
One factor of x⁴ + x² – 20 is x² + 5, the other is
(a) x² – 4
(b) x – 4
(c) x²-5
(d) x + 4
Solution:

RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials MCQS Q14.1

Question 15.
If (x – 1) is a factor of polynomial fix) but not of g(x), then it must be a factor of
(a) f(x) g(x)
(b) -f(x) + g(x)
(c) f(x) – g(x)
(d) {f(x) + g(x)}g(x)
Solution:
∴ (x – 1) is a factor of a polynomial f(x)
But not of a polynomial g(x)
∴ (x – 1) will be the factor of the product of f(x) and g(x) (a)

Question 16.
(x + 1) is a factor of xⁿ + 1 only if
(a) n is an odd integer
(b) n is an even integer
(c) n is a negative integer
(d) n is a positive integer
Solution:
∴ (x + 1) is a factor of xⁿ+ 1
Let x + 1 = 0, then x = -1
∴ f(x) = xⁿ + 1
and f(-1) = (-1)ⁿ + 1
But (-1)ⁿ is positive if n is an even integer and negative if n is an odd integer and (-1)ⁿ +1=0 {∵ x + 1 is a factor of(x)}
(-1)ⁿ must be negative
∴ n is an odd integer (a)

Question 17.
If x² + x + 1 is a factor of the polynomial 3x³ + 8x² + 8x + 3 + 5k, then the value of k is
(a) 0
(b) \(\frac { 2 }{ 5 }\)
(c) \(\frac { 5 }{ 2 }\)
(d) -1
Solution:
x² + x + 1 is a factor of
f(x) = 3x³ + 8x² + 8x + 3 + 5k
Now dividing by x² + x + 1, we get
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials MCQS Q17.1

Question 18.
If (3x – 1)⁷ = a₇x^₇ + a₆x⁶ + a₅x⁵ + … + a₁x + a₀, then a₇ + a₆ + a₅ + … + a₁ + a₀ =
(a) 0
(b) 1
(c) 128
(d) 64
Solution:
f(x) = [3(1) – 1]⁷ = a₇x⁷ + a₆x⁶ + a₅x⁵ + … + a₁x + a₀Let x = 1, then
f(1) = (3x- 1)⁷ = a₇(1)⁷ + a₆(1)⁶ + a₅(1)⁵ + … + a₁ x 1 + a₀⇒ (3 – 1)⁷ = a₇ x 1 + a₆ x 1+ a₅ x 1 + … + a₁x 1 +a₀⇒ (2)⁷ = a₇ + a₆ + a₅ + … + a₁ +a₀∴ a₇ + a₆ + a₅ + … + a₁ + a₀= 128 (c)

Question 19.
If both x – 2 and x – \(\frac { 1 }{ 2 }\) are factors of px² + 5x + r, then
(a) p = r
(b) p + r = 0
(c) 2p + r = 0
(d) p + 2r = 0
Solution:
RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials MCQS Q19.1

Question 20.
If x² – 1 is a factor of ax⁴ + bx³ + cx² + dx + e, then
(a) a + c + e- b + d
(b)a + b + e = c + d
(c)a + b + c = d+ e
(d)b + c + d= a + e
Solution:
X² – 1 is a factor of ax⁴ + bx³ + cx² + dx + e
⇒ (x + 1), (x – 1) are the factors of ax⁴ + bx³+ cx² + dx + e
Let f(x) = ax⁴ + bx³ + cx² + dx + e
and x + 1 = 0 then x = -1
∴ f(-1) = a(-1)⁴ + b(-1)³ + c(-1)² + d(-1) + e
= a- b + c- d+ e
∴ x + 1 is a factor of f(x)
∴ a-b + c- d+e = 0
⇒ a + c + e = b + d (a)

Hope given RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials MCQS are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Tag: Maths Class 9 RD Sharma Solutions

RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency Ex 24.3

RD Sharma Class 9 Solutions Chapter 10 Congruent Triangles VSAQS

RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency MCQS

RD Sharma Class 9 Solutions Chapter 24 Measures of Central Tendency Ex 24.4

RD Sharma Class 9 Solutions Chapter 11 Co-ordinate Geometry VSAQS

RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials Ex 6.4

RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.4

RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry Ex 7.3

RD Sharma Class 9 Solutions Chapter 10 Congruent Triangles Ex 10.1

RD Sharma Class 9 Solutions Chapter 7 Introduction to Euclid’s Geometry MCQS

RD Sharma Class 9 Solutions Chapter 6 Factorisation of Polynomials MCQS