Tag: RS Aggarwal Maths Solutions Class 9

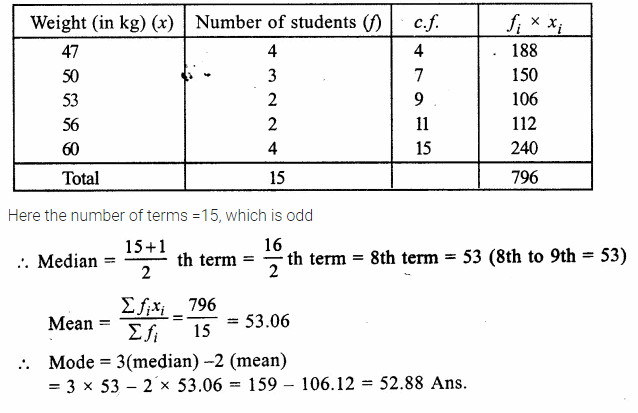
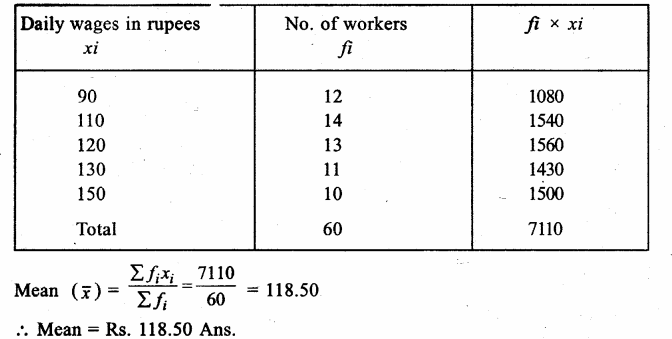
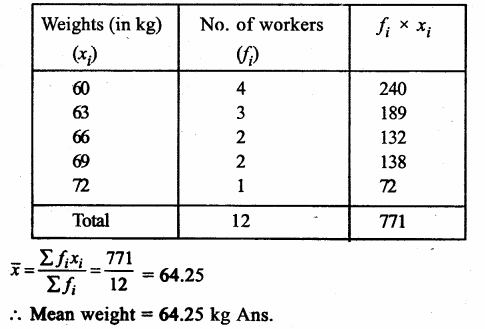
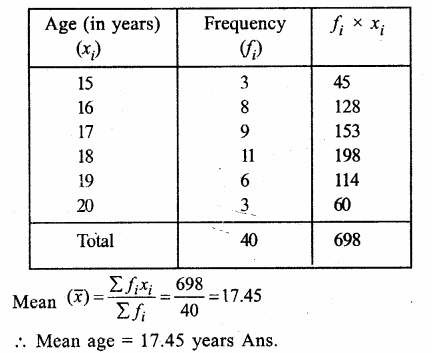
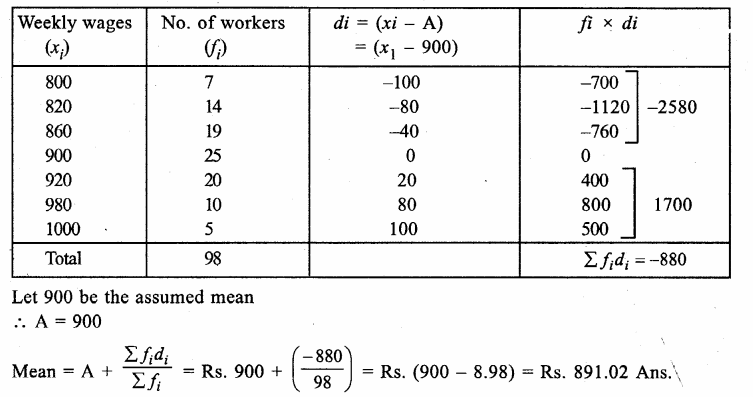
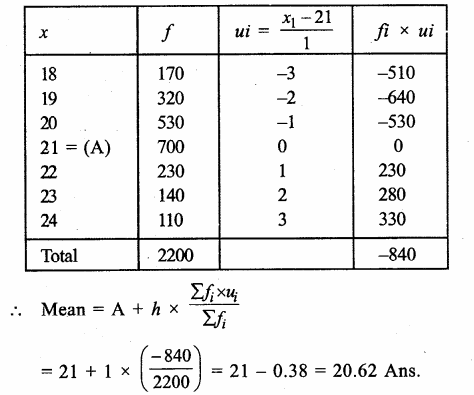
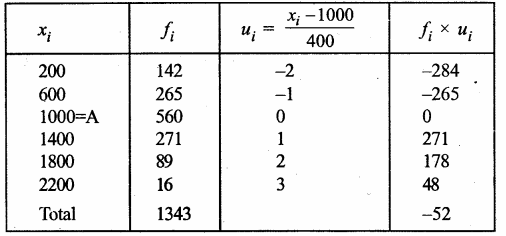
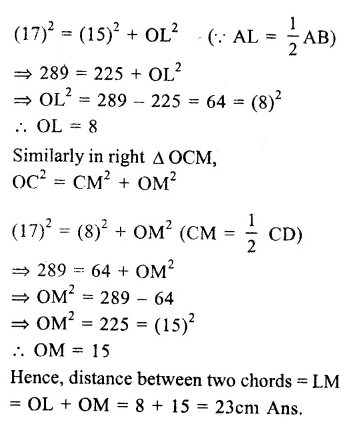
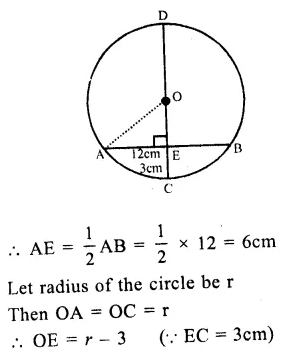
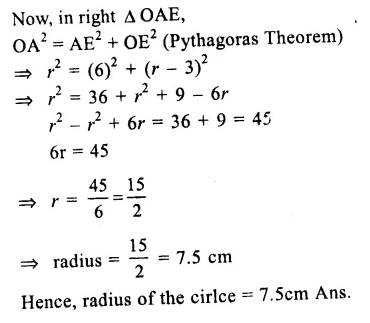
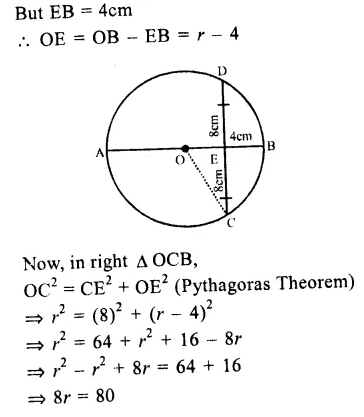
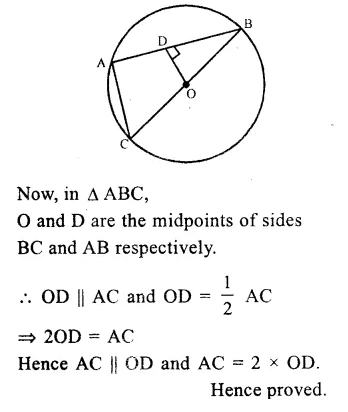
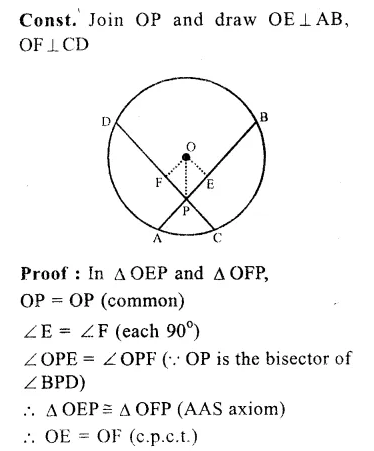
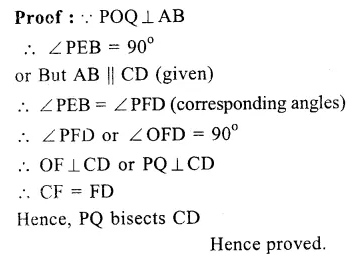
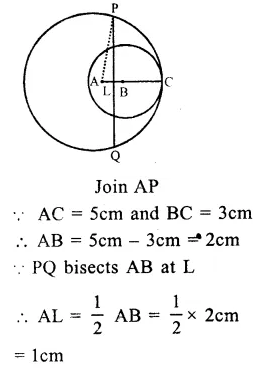
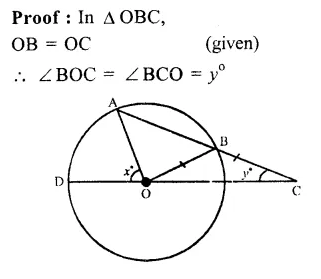
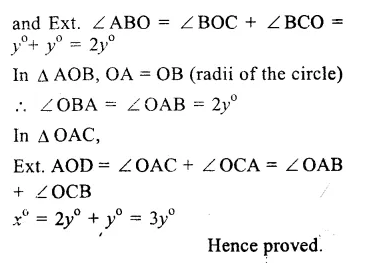
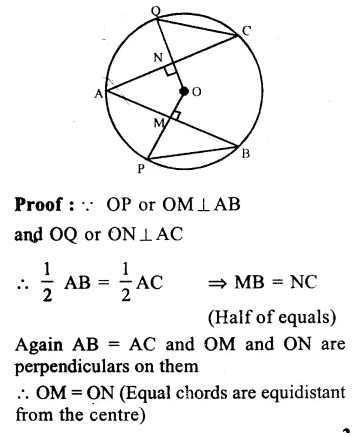
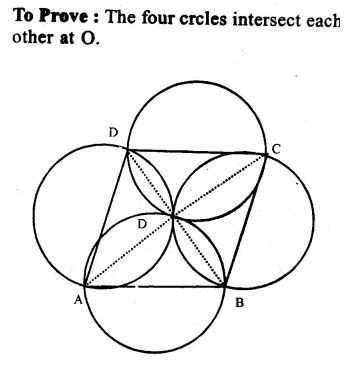
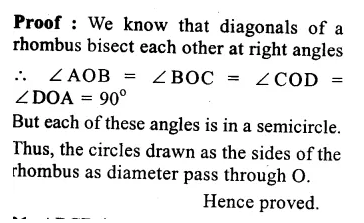
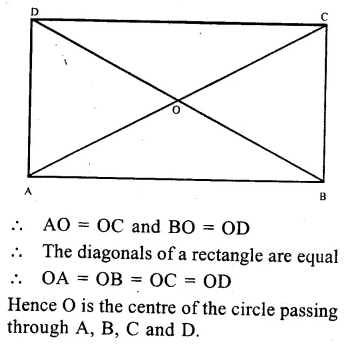
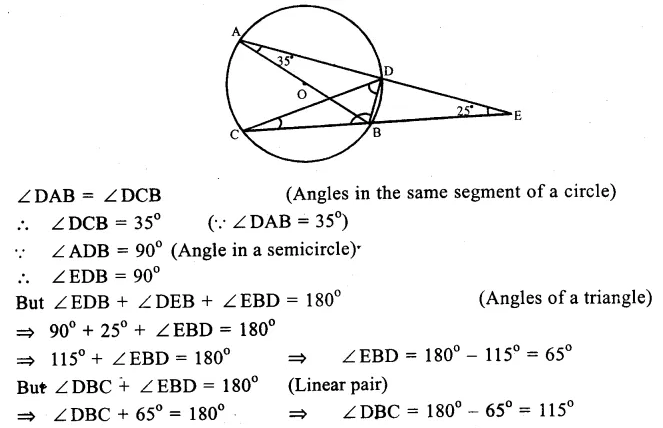
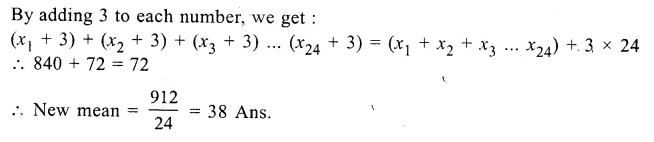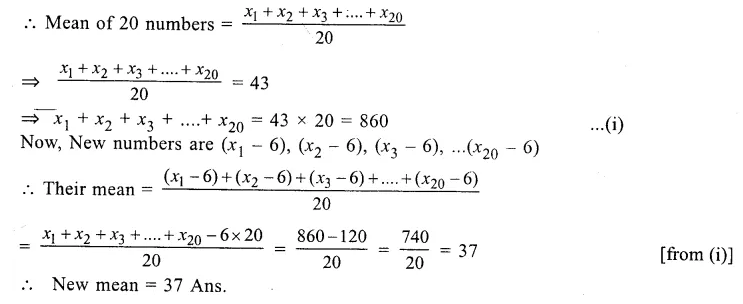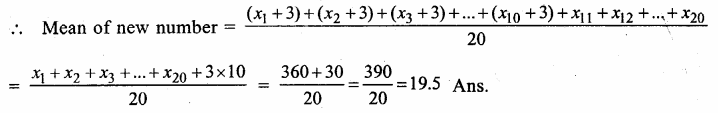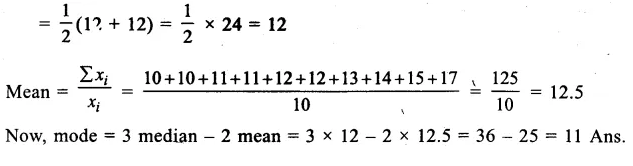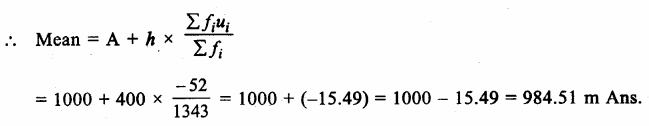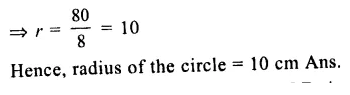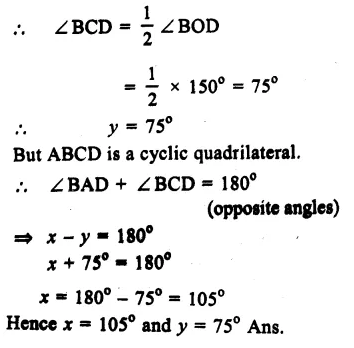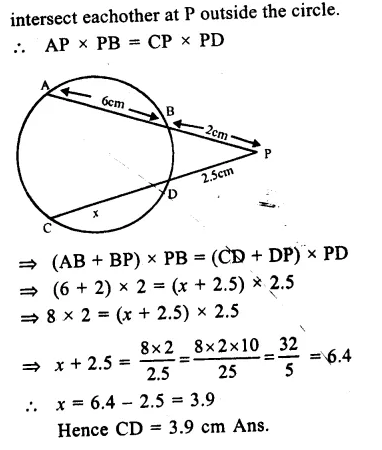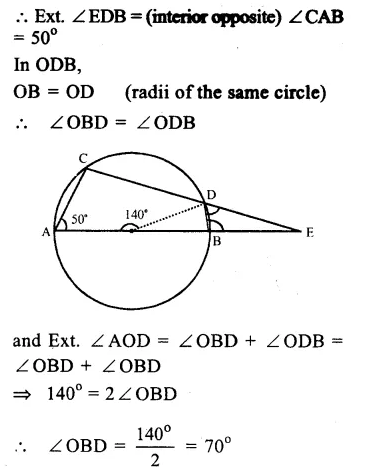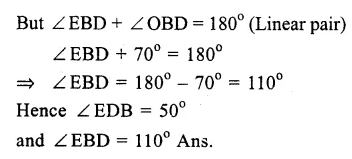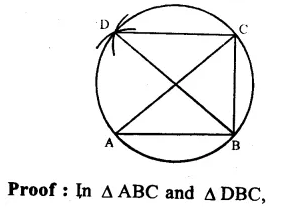RS Aggarwal Class 9 Solutions Chapter 4 Lines and Triangles Ex 4A

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 4 Lines and Triangles Ex 4A.

Other Exercises

RS Aggarwal Solutions Class 9 Chapter 4 Lines and Triangles Ex 4A
RS Aggarwal Solutions Class 9 Chapter 4 Lines and Triangles Ex 4B
RS Aggarwal Solutions Class 9 Chapter 4 Lines and Triangles Ex 4C
RS Aggarwal Solutions Class 9 Chapter 4 Lines and Triangles Ex 4D

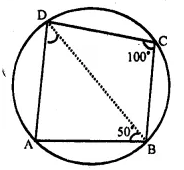
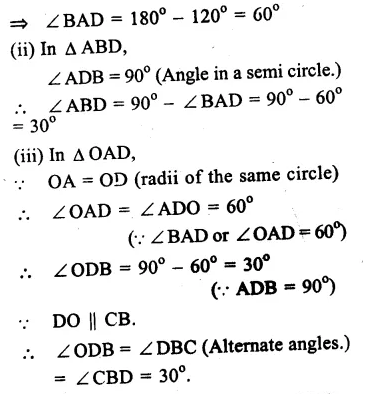
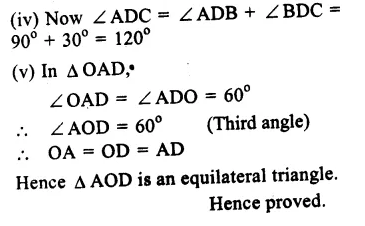
Question 1.
Solution:
(i)Angle : When two rays OA and OB meet at a point o, then ∠AOB is called an angle.

(ii) Interior of angle : The interior an angle is a set of all points in its plane which lie on the same side of OA as B and also on the same side of OB as A.
(iii) Obtuse angle : An angle greater than 90° but less than 180° is called an obtuse angle.
(iv) Reflex angle : An angle more than 180° but less than 360° is called a reflex angle.
(v) Complementary angles : Two angles are said to be complementary angles if their sum is 90°.
(vi) Supplementary angles : Two angles are said to be supplementary angles if their sum is 180°.

Question 2.
Solution:
∠A = 36°27’46”
∠B = 28° 43’39”
Adding, ∠A + ∠B = 64° 70′ 85″
We know that 60″ = 1′ and 60′ = 1°
∠A+ ∠B = 65° 11′ 25″ Ans.

Question 3.
Solution:
36° – 24° 28′ 30″
= 35° 59’60” – 25° 28’30”

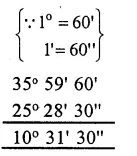
= 10° 31′ 30″ Ans.

Question 4.
Solution:
We know that two angles are complementary of their sum is 90°. Each of these two angles is complement to the other, therefore.
(i) Complement of 58° = 90° – 58° = 32°
(ii) Complement of 16° = 90° – 16° = 74°
(iii) Complement of \(\frac { 2 }{ 3 } \) of a right angle i.e.
of \(\frac { 2 }{ 3 } \) x 90° or 60° = 90° – 60° = 30°
= \(\frac { 2 }{ 3 } \) of right angle,
(iv) Complement of 46° 30′
= 90° – 46° 30′
= 43° 30′
(v) Complement of 52° 43′ 20°= 90° – 52° 43′ 20″
= 37° 16′ 40″
(vi) Complement of 68° 35′ 45″
= 90° – 68° 35′ 45″
= 21° 24′ 15″ Ans.

Question 5.
Solution:
We know that two angles are said to be supplement to each other of their sum is 180° therefore
(i) Supplement of 68° = 180° – 68° =112°
(ii) Supplement of 138° = 180° – 138° = 42°
(iii) Supplement of \(\frac { 3 }{ 5 } \) of a right angle or \(\frac { 3 }{ 5 } \) x 90° or 54°
= 180° – 54° = 126°
(iv) Supplement of 75° 36′ = 180° – 75° 36′ = 104° 24′
(v) Supplement of 124° 20′ 40″
= 180° – 124° 20′ 40″
= 55° 39′ 20″
(vi) Supplement of 108° 48′ 32″ = 180° – 108″ 48′ 32″ = 71° 11′ 28″ Ans.

Question 6.
Solution:
(i) Let the measure of required angle = x ,
their its complement = 90° – x
According to the condition,
x = 90° – x => 2x = 90°
=>x = \(\frac { { 90 }^{ o } }{ 2 } \) = 45°
Required angle = 45°
(ii) Let the measure of required angle = x then its supplement = 180° – x
According to the condition,
x = 180° – x => 2x = 180° = 90°
=>x = \(\frac {{ 180 }^{ o }}{ 2 }\) = 90°
Hence required angle = 90° Ans.

Question 7.
Solution:
Let required angle = x
then its complement = 90° – x
According to the condition,
x – (90° – x) = 36°
=> x – 90° + x = 36°
=> 2x = 36° + 90° = 126°
= \(\frac { { 126 }^{ o } }{ 2 } \) = 63°
Required angle = 63° Ans.

Question 8.
Solution:
Let the required angle = x
then its supplement = 180° – x
According to the condition,
(180° – x) – x = 25°
=> 180° – x – x = 25°
=> – 2x = 25° – 180°
=> – 2x = – 155°
=> x = \(\frac { { – 155 }^{ o } }{ – 2 } \)
= 77.5°
Hence required angle = 77.5° Ans.

Question 9.
Solution:
Let required angle = x
Then its complement = 90° – x
According to the condition,
x = 4 (90° – x) => x = 360° – 4x
=> x + 4x = 360° => 5x = 360°
x = \(\frac { { 360 }^{ o } }{ 5 } \) = 72°
Required angle = 72° Ans.

Question 10.
Solution:
Let required angle = x
Then its supplement = 180° – x
According to the condition,
x = 5 (180° – x)
=> x = 900° – 5x
=> x + 5x = 900°
=> 6x = 900°
=> x = \(\frac { { 900 }^{ o } }{ 6 } \) = 150°
Hence, required angle = 150° Ans

Question 11.
Solution:
Let required angle = x
then its supplement = 180° – x
and complement = 90° – x
According to the condition,
180° – x = 4 (90°- x)
=> 180° – x = 360° – 4x
=> – x + 4x — 360° – 180°
=> 3x= 180°
=> x = \(\frac { { 180 }^{ o } }{ 3 } \) = 60°
Required angle = 60° Ans.

Question 12.
Solution:
Let required angle = x
Then, its complement = 90° – x
and its supplement = 180° – x
According to the condition,
90° – x = \(\frac { 1 }{ 3 } \) (180° – x)
=> 90° – x = 60° – \(\frac { 1 }{ 3 } \) x
=> 90° – 60° = x – \(\frac { 1 }{ 3 } \) x
=> \(\frac { 2 }{ 3 } \) x = 30° =>x = \(\frac { { 30 }^{ o }X3\quad \quad }{ 2 } \) => x = 45° Ans.

Question 13.
Solution:
Let one angle = x
Then, its supplement = 180° – x
According to the condition,
x : (180° – x) = 3:2
=> \(\frac { x }{ { 180 }^{ o }-x } =\frac { 3 }{ 2 } \)
=>2x = 3(180°- x)
=> 2x = 540° – 3x
=> 2x + 3x = 540°
=> 5x = 540° => x = \(\frac { { 540 }^{ o } }{ 5 } \) = 108°
Angle = 108° and its supplement = 180° – 108° = 72°
Hence, angles are 108° and 72° Ans.

Question 14.
Solution:
Let angle = x
Then, its complementary angle = 90° – x
According to the condition,
x : (90° – x) = 4 : 5
=> \(\frac { x }{ { 90 }^{ o }-x } =\frac { 4 }{ 5 } \)
=> 5x = 4 (90° – x)
=> 5x = 360° – 4x
=> 5x + 4x = 360°
=> 9x = 360°
=> x = \(\frac { { 360 }^{ o } }{ 9 } ={ 40 }^{ o } \)
and its complement = 90° – 40° = 50°
Hence, angles are 40° and 50° Ans.

Question 15.
Solution:
Let the required angle = x
.’. its complement = 90° – x
and supplement = 180° – x
According to the condition,
7(90° – x) = 3(180° – x) – 10°
=> 630° – 7x = 3 (180° – x) – 10°
=> 630° – 7x = 540° – 3x – 10°
=> – 7x + 3x = 540° – 10° – 630°
– 4x = – 100°
x = \(\frac { { -100 }^{ o } }{ -4 } ={ 25 }^{ o }\)
Hence required angle = 25° Ans.

Hope given RS Aggarwal Solutions Class 9 Chapter 4 Lines and Triangles Ex 4A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 14 Statistics Ex 14D

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14D.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14A
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14B
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14C
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14D
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14E
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14F
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14G
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14H

Question 1.
Solution:
(i) First eight natural numbers are 1, 2, 3, 4, 5, 6, 7, 8
∴ Mean = \(\frac { 1+2+3+4+5+6+7+8 }{ 8 } \) = \(\frac { 36 }{ 8 } \) = \(\frac { 9 }{ 2 } \) = 4.5
(ii) First ten odd numbers are 1, 3, 5, 7, 9, 11, 13, 15, 17, 19
∴ Mean = \(\frac { 1+3+5+7+9+11+13+15+17+179 }{ 10 } \) = \(\frac { 100 }{ 10 } \) = 10
(iii) First five prime numbers are 2, 3, 5, 7, 11.
∴ Mean = \(\frac { 2+3+5+7+11 }{ 5 } \) = \(\frac { 28 }{ 5 } \) = 5.6
(iv) First six even numbers are 2, 4, 6, 8, 10, 12
∴ Mean = \(\frac { 2+4+6+8+10+12 }{ 10 } \) = \(\frac { 42 }{ 6 } \) = 7
(v) First seven multiples of 5 are 5, 10, 15, 20, 25, 30, 35
∴ Mean = \(\frac { 5+10+15+20+25+30+35 }{ 7 } \) = \(\frac { 140 }{ 7 } \) = 20
(vi) All the factors of 20 are, 1, 2, 4, 5, 10, 20
∴ Mean = \(\frac { 1+2+4+5+10+20 }{ 6 } \) = \(\frac { 42 }{ 6 } \) = 7

Question 2.
Solution:
No. of families (n) = 10
Sum of children (∑x1) = 2 + 4 + 3 + 4 + 2 + 0 + 3 + 5 + 1 + 6 = 30
∴ Mean \(\left( \overline { x } \right) =\frac { \sum { x1 } }{ n } =\frac { 30 }{ 10 } =3\)

Question 3.
Solution:
Here number of days (n) = 7
Number of books (∑x1) = 105 + 216 + 322 + 167 + 273 + 405 + 346 = 1834
∴ Mean \(\left( \overline { x } \right) =\frac { \sum { x1 } }{ n } =\frac { 1864 }{ 7 } =262 books\)

Question 4.
Solution:
Number of days (n) = 6
Sum of temperature (∑x) = 35.5 + 30.8 + 27.3 + 32.1 + 23.8 + 29.9 = 179.4°F
∴ Mean temperature = \(\frac { \sum { x } }{ n } =\frac { 179.4 }{ 6 } \)=29.9°F

Question 5.
Solution:
Number of students (n) = 12
Sum of marks (∑x) = 64 + 36 + 47 + 23 + 0 + 19 + 81 + 93 + 72 + 35 + 3 + 1 = 474
= \(\frac { \sum { x1 } }{ n } =\frac { 474 }{ 12 } \) = 39.5

Question 6.
Solution:
Here, n = 6
and arithmetic mean =13
Total sum = 13 x 6 = 78.
But sum of 7 + 9+ 11 + 13 + 21=61
Value of x = 78 – 61 = 17
Hence x = 17 Ans.

Question 7.
Solution:
Let x₁, x₂, x₃, … x₂₄ be the 24 numbers
\(\frac { x1+x2+x3+…..+x24 }{ 24 } =35\)
=> x₁ + x₂ + x₃ +….+ x₂₄ = 35 x 24

Question 8.
Solution:
Let x₁ + x₂ + x₃……..x₂₀ be the 20 numbers

Question 9.
Solution:
Let x₁, x₂, x₃ … x₁₅ be the numbers
Mean = \(\frac { x1+x2+x3+…..+x15 }{ 15 } = 27\)

Question 10.
Solution:
Let x₁, x₂, x₃ … x₁₂ be the numbers
Mean = \(\frac { x1+x2+x3+…..+x12 }{ 12 }\) = 40
=> x1+x2+x3+….+x12 = 40 X 12 =480
Now,new numbers are \(\frac { x1 }{ 8 } ,\frac { x2 }{ 8 } ,\frac { x3 }{ 8 } ,..\frac { x12 }{ 8 } \)
Mean = \(\frac { \frac { x1 }{ 8 } +\frac { x2 }{ 8 } +\frac { x3 }{ 8 } +…..+\frac { x12 }{ 8 } }{ 12 } \)
= \(\frac { 1 }{ 8 } \frac { \left( x1+x2+x3+….x12 \right) }{ 12 } \)
= \(\frac { 480 }{ 8X12 } \) = 5
Mean of new numbers =5

Question 11.
Solution:
Let x₁, x₂, x₃,…..x₂₀ are the numbers
Mean = \(\frac { x1+x2+x3+…x20 }{ 20 }\) = 18
=> x₁ + x₂ + x₃ +….+ x₂₀ = 18 X 20 =360

Question 12.
Solution:
Mean weight of 6 boys = 48 kg
Their total weight = 48 x 6 = 288 kg
Weights of 5 boys among them, are 51 kg, 45 kg, 49 kg, 46 kg and 44 kg
Sum of weight of 5 boys = (51 + 45 + 49 + 46 + 44) kg = 235 kg
Weight of 6th boy = 288 kg – 235 kg = 53 kg Ans.

Question 13.
Solution:
Mean of 50 students = 39
Total score = 39 x 50 = 1950
Now correct sum of scores = 1950 – Wrong item + Correct item= 1950 – 23 + 43
= 1950 + 20 = 1970
Correct mean = \(\frac { 1970 }{ 50 } \) = 39.4 Ans.

Question 14.
Solution:
Mean of 100 items = 64
The sum of 100 items = 64 x 100 = 6400
New sum of 100 items = 6400 + 36 + 90 – 26 – 9 = 6526 – 35 = 6491,
Correct mean = \(\frac { 6491 }{ 100 }\) =64.91 = 64.91 Ans.

Question 15.
Solution:
Mean of 6 numbers = 23
Sum of 6 numbers = 23 x 6 = 138
Excluding one number, the mean of remaining 5 numbers = 20
Total of 5 numbers = 20 x 5 = 100
Excluded number = 138 – 100 = 38 Ans.

Hope given RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 14 Statistics Ex 14E

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14E.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14A
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14B
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14C
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14D
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14E
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14F
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14G
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14H

Question 1.
Solution:
Mean marks of 7 students = 226
∴Total marks of 7 students = 226 x 1 = 1582
Marks obtained by 6 of them are 340, 180, 260, 56, 275 and 307
∴ Sum of marks of these 6 students = 340 + 180 + 260 + 56 + 275 + 307 = 1418
∴ Marks obtained by seventh student = 1582 – 1418 = 164 Ans.

Question 2.
Solution:
Mean weight of 34 students = 46.5 kg.
∴ Total weight of 34 students = 46.5 x 34 kg = 1581 kg
By including the weight of teacher, the mean weight of 35 persons = 500 g + 46.5 kg
= 46.5 + 0.5 = 47.0 kg
∴ Total weight = 47.0 x 35 = 1645 kg
∴ Weight of the teacher = (1645 – 1581) kg = 64 kg Ans.

Question 3.
Solution:
Mean weight of 36 students = 41 kg
∴ Total weight of 36 students = 41 x 36 kg = 1476 kg
After leaving one student, number of students = 36 – 1 =35
Their new mean = 41.0 – 200g = (41.0 – 0.2) kg = 40.8 kg.
∴ Total weight of 35 students = 40.8 x 35 = 1428 kg
∴ Weight of leaving student = (1476 – 1428) kg = 48 kg Ans.

Question 4.
Solution:
Average weight of 39 students = 40 kg
∴ Total weight of 39 students = 40 x 39 = 1560 kg
By admitting of a new student, no. of students = 39 + 1 =40
and new mean = 40 kg – 200 g = 40 kg – 0.2 kg = 39.8 kg
∴Weight of 40 students = 39.8 x 40 kg = 1592 kg
∴Weight of new student = 1592 – 1560 kg = 32 kg Ans.

Question 5.
Solution:
Average salary of 20 workers = Rs. 7650
∴Their total salary = Rs. 7650 x 20 = Rs. 153000
By adding the salary of the manager, their mean salary = Rs. 8200
∴Their total salary = Rs. 8200 x 21 = Rs. 172200
∴Salary of the manager = Rs. 172200 – Rs. 153000 = Rs. 19200 Ans.

Question 6.
Solution:
Average wage of 10 persons = Rs. 9000
Their total wage = Rs. 9000 x 10 = Rs. 90000
Wage of one person among them = Rs. 8100
and wage of new member = Rs. 7200
∴ New total wage = Rs. (90000 – 8100 + 7200) = Rs. 89100
Their new mean wage = Rs.\(\frac { 89100 }{ 10 } \) = Rs. 8910

Question 7.
Solution:
Mean consumption of petrol for 7 months of a year = 330 litres
Mean consumption of petrol for next 5 months = 270 litres
∴Total consumption for first 7 months = 7 x 330 = 2320 l
and total consumption for next 5 months = 5 x 270 = 1350 l
Total consumption for 7 + 5 = 12 months = 2310 + 1350 = 3660 l
Average consumption = \(\frac { 3660 }{ 12 } \) = 305 liters per month.

Question 8.
Solution:
Total numbers of numbers = 25
Mean of 15 numbers = 18
∴Total of 15 numbers = 18 x 15 = 270
Mean of remaining 10 numbers = 13
∴Total = 13 x 10 = 130
and total of 25 numbers = 270 + 130 = 400
∴Mean = \(\frac { 400 }{ 25 } \) = 16 Ans.

Question 9.
Solution:
Mean weight of 60 students = 52.75 kg.
Total weight = 52.75 x 60 = 3165 kg
Mean of 25 out of them = 51 kg.
∴Their total weight = 51 x 25 = 1275 kg
∴ Total weight of remaining 60 – 25 = 35 students = 3165 – 1275 = 1890 kg 1890
Mean weight = \(\frac { 1890 }{ 35 } \) = 54 kg Ans.

Question 10.
Solution:
Average increase of 10 oarsman = 1.5 kg.
∴ Total increased weight =1.5 x 10 = 15kg
Weight of out going oarsman = 58 kg .
∴ Weight of new oarsman = 58 + 15 = 73 kg Ans.

Question 11.
Solution:
Mean of 8 numbers = 35
∴Total of 8 numbers = 35 x 8 = 280
After excluding one number, mean of 7 numbers = 35 – 3 = 32
Total of 7 numbers = 32 x 7 = 224
Hence excluded number = 280 – 224 = 56 Ans.

Question 12.
Solution:
Mean of 150 items = 60
Total of 150 items = 60 x 150 = 9000
New total = 9000 + 152 + 88 – 52 – 8 = 9000 + 240 – 60 = 9180
∴New mean = \(\frac { 9180 }{ 150 } \) = 61.2 Ans.

Question 13.
Solution:
Mean of 31 results = 60
∴Total of 31 results = 60 x 31 = 1860
Mean of first 16 results = 58
∴Total of first 16 results = 58 x 16 = 928
and mean of last 16 results = 62
∴Total of last 16 results = 62 x 16 = 992
∴16th result = (928 + 992) – 1860 = 1920 – 1860 = 60 Ans.

Question 14.
Solution:
Mean of 11 numbers = 42
∴Total of 11 numbers = 42 x 11 = 462
Mean of first 6 numbers = 37
∴Total of first 6 numbers = 37 x 6 = 222
Mean of last 6 numbers = 46
∴Total of last 6 numbers = 46 x 6 = 276
∴6th number = (222 + 276) – 462 = 498 – 462 = 36 Ans.

Question 15.
Solution:
Mean weight of 25 students = 52 kg
∴ Total weight of 25 students = 52 x 25 = 1300 kg
Mean weight of first 13 students = 48 kg
∴ Total weight of first 13 students = 48 x 13 kg = 624 kg
Mean of last 13 students = 55 kg
∴Total of last 13 students = 55 x 13 kg = 715 kg
∴Weight of 13th student = (624 + 715) – 1300 = 1339 – 1300 = 39 kg Ans.

Question 16.
Solution:
Mean of 25 observations = 80
Total of 25 observations = 80 x 25 = 2000
Mean of another 55 observations = 60
∴ Total of these 55 observations = 60 x 55 = 3300
Total number of observations = 25 + 55 = 80
and total of 80 numbers = 2000 + 3300 = 5300
Mean of 80 observations = \(\frac { 5300 }{ 80 } \) = 66.25 Ans.

Question 17.
Solution:
Marks in English = 36
Marks in Hindi = 44
Marks in Mathematics = 75
Marks in Science = x
∴Total number of marks in 4 subjects = 36 + 44 + 75 + x = 155 + x
Average marks in 4 subjects = 50
∴Total marks = 50 x 4 = 200
∴155 + x = 200
=> x = 200 – 155
=> x = 45
Hence, marks in Science = 45 Ans.

Question 18.
Solution:
Mean of monthly salary of 75 workers = Rs. 5680
Total salary of 75 workers = Rs. 5680 x 75 = Rs. 426000
Mean salary of 25 among them = Rs. 5400
Total of 25 workers = Rs. 5400 x 25 = Rs. 135000
Mean salary of 30 among them = Rs. 5700
∴Total of 30 among them = Rs. 5700 x 30 = Rs. 171000
∴ Total salary of 25 + 30 = 55 workers = Rs. 135000 + 171000 = Rs. 306000
∴Total salary of remaining 75 – 55 = 20 workers = Rs. 426000 – 306000 = Rs. 120000
∴ Mean of remaining 20 workers = Rs. \(\frac { 120000 }{ 20 } \) = Rs. 6000 Ans.

Question 19.
Solution:
Let distance between two places = 60 km
∴Time taken at the speed of 15 km/h = \(\frac { 60 }{ 15 } \) = 4 hours
and time taken at speed of 10 km/h for coming back = \(\frac { 60 }{ 10 } \) = 6 hours
Total the taken = 4 + 6 = 10
hours and distance covered = 60 + 60 = 120 km
∴Average speed = \(\frac { 120 }{ 10 } \) =12 km/hr.

Question 20.
Solution:
No. of total students = 50
No. of boys = 40
∴ No. of girls = 50 – 40 = 10
Average weight of class = 44kg
∴Total weight of 50 students = 44 x 50 = 2200 kg
Average weight of 10 girls = 40 kg
∴Total weight = 40 x 10 = 400 kg
Total weight of 40 boys = 2200 – 400 = 1800 kg
∴Average weight of 40 boys = \(\frac { 1800 }{ 40 } \)kg = 45 kg Ans.

Hope given RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14E are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 14 Statistics Ex 14H

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14H.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14A
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14B
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14C
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14D
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14E
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14F
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14G
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14H

Question 1.
Solution:
Arranging the given data in ascending order :
0, 0, 1, 2, 3, 4, 5, 5, 6, 6, 6, 6
We see that 6 occurs in maximum times.
Mode = 6 Ans.

Question 2.
Solution:
Arranging in ascending order, we get:
15, 20, 22, 23, 25, 25, 25, 27, 40
We see that 25 occurs in maximum times.
Mode = 25 Ans.

Question 3.
Solution:
Arranging in ascending order we get:
1, 1, 2, 3, 3, 4, 5, 5, 6, 6, 7, 8, 9, 9, 9, 9, 9
Here, we see that 9 occurs in maximum times.
Mode = 9 Ans.

Question 4.
Solution:
Arranging in ascending order, we get:
9, 19, 27, 28, 30, 32, 35, 50, 50, 50, 50, 60
Here, we see that 50 occurs in maximum times.
Modal score = 50 scores Ans.

Question 5.
Solution:
Arranging in ascending order, we get:
10, 10, 11, 11, 12, 12, 13, 14,15, 17
Here, number of terms is 10, which is even
∴ Median = \(\frac { 1 }{ 2 } \left[ \frac { 10 }{ 2 } th\quad term+\left( \frac { 10 }{ 2 } +1 \right) th\quad term \right]\)
= \(\frac { 1 }{ 2 } \) (5th term + 6th term)

Question 6.
Solution:

Question 7.
Solution:
Writing its cumulative frequency table

Question 8.
Solution:
Writing its cumulative frequency table

Here, number of items is 40 which is even.
∴ Median = \(\frac { 1 }{ 2 } \left[ \frac { 40 }{ 2 } th\quad term+\left( \frac { 40 }{ 2 } +1 \right) th\quad term \right]\)
= \(\frac { 1 }{ 2 } \) (20th term + 21th term)
= \(\frac { 1 }{ 2 } \) (30 + 30) = \(\frac { 1 }{ 2 } \) x 60 = 30
Mean= \(\frac { \sum { { f }_{ i }{ x }_{ i } } }{ \sum { { f }_{ i } } } \) = \(\frac { 1161 }{ 40 } \) = 29.025
∴Mode = 3 median – 2 mean = 3 x 30 – 2 x 29.025 = 90 – 58.05 = 31.95

Question 9.
Solution:
Preparing its cumulative frequency table we get:

Here number of terms is 50, which is even

Question 10.
Solution:
Preparing its cumulative frequency table :

Question 11.
Solution:
Preparing its cumulative frequency table we have,

Question 12.
Solution:
Preparing its cumulative frequency table we have,

Hope given RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14H are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 15 Probability Ex 15A

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Class 9 Solutions Chapter 15 Probability Ex 15A.

Question 1.
Solution:
Number of trials = 500 times
Let E be the no. of events in each case, then
∴No. of heads (E1) = 285 times
and no. of tails (E2) = 215 times
∴ Probability in each case will be
∴(i)P(E1) = \(\frac { 285 }{ 500 } \) = \(\frac { 57 }{ 100 } \) = 0.57
(ii) P(E2) = \(\frac { 215 }{ 500 } \) = \(\frac { 43 }{ 100 } \) = 0.43

Question 2.
Solution:
No. of trials = 400
Let E be the no. of events in each case, then
No. of 2 heads (E1) = 112
No. of one head (E2) = 160 times
and no. of O. head (E3) = 128 times
∴ Probability in each case will be:
∴ (i)P(E1) = \(\frac { 112 }{ 400 } \) = \(\frac { 28 }{ 100 } \) = 0.28
(ii)P(E2) = \(\frac { 160 }{ 400 } \) = \(\frac { 40 }{ 100 } \)= 0.40
(iii) P(E3) = \(\frac { 128 }{ 400 } \) = \(\frac { 32 }{ 100 } \) = 0.32 Ans.

Question 3.
Solution:
Number of total trials = 200
Let E be the no. of events in each case, then
No. of three heads (E1) = 39 times
No. of two heads (E2) = 58 times
No. of one head (E3) = 67 times
and no. of no head (E4) = 36 times
∴ Probability in each case will be .
(i) P(E1) = \(\frac { 39 }{ 200 } \) = 0.195
(ii) P(E3) = \(\frac { 67 }{ 200 } \) = 0.335
(iii) P(E4) = \(\frac { 36 }{ 200 } \) = \(\frac { 18 }{ 100 } \) = 0.18
(iv) P(E2) = \(\frac { 58 }{ 200 } \) = \(\frac { 29 }{ 100 } \) = 0.29

Question 4.
Solution:
Solution No. of trials = 300 times
Let E be the no. of events in each case, then
No. of outcome of 1(E1) = 60
No. of outcome of 2(E2) = 72
No. of outcome of 3(E3) = 54
No. of outcome of 4(E4) 42
No. of outcome of 5(E5) = 39
No. of outcome of 6(E6) = 33
The probability of
(i) P(E3) = \(\frac { 54 }{ 300 } \) = \(\frac { 18 }{ 100 } \) = 0.18
(ii) P(E6) = \(\frac { 33 }{ 100 } \) = \(\frac { 11 }{ 100 } \)= 0.11
(iii) P(E5) = \(\frac { 39 }{ 300 } \) = \(\frac { 13 }{ 100 } \) = 0.13
(iv) P(E1) = \(\frac { 60 }{ 300 } \) = \(\frac { 20 }{ 100 } \)= 0.20 Ans.

Question 5.
Solution:
No. of ladies on whom survey was made = 200.
Let E be the no. of events in each case.
No. of ladies who like coffee (E1) = 142
No. of ladies who like coffee (E2) = 58
Probability of
(1) P(E1) = \(\frac { 142 }{ 200 } \) = \(\frac { 71 }{ 100 } \) = 0.71
(ii) P(E2) = \(\frac { 58 }{ 200 } \) = \(\frac { 29 }{ 100 } \) = 0.29 Ans.

Question 6.
Solution:
Total number of tests = 6
No. of test in which the students get more than 60% mark = 2
Probability will he
P(E) = \(\frac { 2 }{ 6 } \) = \(\frac { 1 }{ 3 } \)Ans.

Question 7.
Solution:
No. of vehicles of various types = 240
No. of vehicles of two wheelers = 64.
Probability will be P(E) = \(\frac { 84 }{ 240 } \) = \(\frac { 7 }{ 20 } \) = 0.35 Ans.

Question 8.
Solution:
No. of phone numbers are one page = 200
Let E be the number of events in each case,
Then (i) P(E5) = \(\frac { 24 }{ 200 } \) = \(\frac { 12 }{ 100 } \) = 0.12
(ii) P(E8) = \(\frac { 16 }{ 200 } \) = \(\frac { 8 }{ 100 } \) = 0.08 Ans.

Question 9.
Solution:
No. of students whose blood group is checked = 40
Let E be the no. of events in each case,
Then (i) P(E0) = \(\frac { 14 }{ 40 } \) = \(\frac { 7 }{ 20 } \) = 0.35
(ii) P(EAB) = \(\frac { 6 }{ 40 } \) = \(\frac { 3 }{ 20 } \) = 0.15 Ans.

Question 10.
Solution:
No. of total students = 30.
Let E be the number of elements, this probability will be of interval 21 – 30
P(E) = \(\frac { 6 }{ 30 } \) = \(\frac { 1 }{ 5 } \) = 0.2 Ans.

Question 11.
Solution:
Total number of patients of various age group getting medical treatment = 360
Let E be the number of events, then
(i) No. of patient which are 30 years or more but less than 40 years = 60.
P(E) = \(\frac { 60 }{ 360 } \) = \(\frac { 1 }{ 6 } \)
(ii) 50 years or more but less than 70 years = 50 + 30 = 80
P(E) = \(\frac { 80 }{ 360 } \) = \(\frac { 2 }{ 9 } \)
(iii) Less than 10 years = zero
P(E) = \(\frac { 0 }{ 360 } \) = 0
(iv) 10 years or more 90 + 50 + 60 + 80 + 50 + 30 = 360

Hope given RS Aggarwal Class 9 Solutions Chapter 15 Probability Ex 15A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 14 Statistics Ex 14F

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14F.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14A
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14B
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14C
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14D
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14E
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14F
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14G
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14H

Question 1.
Solution:

Question 2.
Solution:

Question 3.
Solution:

Question 4.
Solution:

Question 5.
Solution:
Mean = 8

Question 6.
Solution:
Mean = 28.25

Question 7.
Solution:
Mean = 16.6

Question 8.
Solution:
Mean = 50

Question 9.
Solution:

Question 10.
Solution:
Let assumed mean = 67

Question 11.
Solution:
Here h = 1, Let assumed mean (A) = 21

Question 12.
Solution:
Here h = 400 and let assumed mean (A) = 1000

Hope given RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14F are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 14 Statistics Ex 14G

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14G.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14A
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14B
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14C
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14D
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14E
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14F
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14G
• RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14H

Question 1.
Solution:
Arranging in ascending order, we get:
2,2,3,5,7,9,9,10,11
Here, number of terms is 9 which is odd.
∴ Median = \(\frac { n+1 }{ 2 } \) th term = \(\frac { 9+1 }{ 2 } \) th term = 5th term = 7 Ans.
(ii) Arranging in ascending order, we get: 6, 8, 9, 15, 16, 18, 21, 22, 25
Here, number of terms is 9 which is odd.
∴ Median = \(\frac { n+1 }{ 2 } \) th term = \(\frac { 9+1 }{ 2 } \) th term = 5th term = 16 Ans.
(iii) Arranging in ascending order, we get: 6, 8, 9, 13, 15, 16, 18, 20, 21, 22, 25
Here, number of terms is 11 which is odd.
∴ Median = \(\frac { 11+1 }{ 2 } \) th term = \(\frac { 12 }{ 2 } \) th term = 6th term = 16 Ans.
(iv) Arranging in ascending order, we get:
0, 1, 2, 2, 3, 4, 4, 5, 5, 7, 8, 9, 10
Here, number of terms is 13, which is odd.
Median = \(\frac { 13+1 }{ 2 } \) th term = \(\frac { 14 }{ 2 } \) th term = 7th term = 4 Ans.

Question 2.
Solution:
Arranging in ascending order, we get 9, 10, 17, 19, 21, 22, 32, 35
Here, number of terms is 8 which is even
∴Median = \(\frac { 1 }{ 2 } \left[ \frac { 8 }{ 2 } th\quad term+\left( \frac { 8 }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \) [4th term + 5th term] = \(\frac { 1 }{ 2 } \) (19 + 21) = \(\frac { 1 }{ 2 } \) x 40 = 20
(ii) Arranging in ascending order, we get:
29, 35, 51, 55, 60, 63, 72, 82, 85, 91
Here number of terms is 10 which is even
∴Median = \(\frac { 1 }{ 2 } \left[ \frac { 10 }{ 2 } th\quad term+\left( \frac { 10 }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \) (60 + 63) = \(\frac { 1 }{ 2 } \) x 123 = 61.5 Ans.
(iii) Arranging in ascending order we get
3, 4, 9, 10, 12, 15, 17, 27, 47, 48, 75, 81
Here number of terms is 12 which is even.
∴Median = \(\frac { 1 }{ 2 } \left[ \frac { 12 }{ 2 } th\quad term+\left( \frac { 12 }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \) (6th term + 7th term) = \(\frac { 1 }{ 2 } \) (15 + 17)= \(\frac { 1 }{ 2 } \) x 32
= 16 Ans.

Question 3.
Solution:
Arranging the given data in ascending order, we get :
17, 17, 19, 19, 20, 21, 22, 23, 24, 25, 26, 29, 31, 35, 40
∴ Median = \(\frac { 15+1 }{ 2 } \) th term = \(\frac { 16 }{ 2 } \) th term = 8th term = 23
∴ Median score = 23 Ans.

Question 4.
Solution:
Arranging in ascending order, we get:
143.7, 144.2, 145, 146.5, 147.3, 148.5, 149.6, 150, 152.1
Here, number of terms is 9 which is odd.
Median = \(\frac { 9+1 }{ 2 } \) th term = \(\frac { 10 }{ 2 } \) th term = 5th term = 147.3 cm
Hence, median height = 147.3 cm Ans.

Question 5.
Solution:
Arranging in ascending order, we get:
9.8, 10.6, 12.7, 13.4, 14.3, 15, 16.5, 17.2
Here number of terms is 8 which is even
∴Median = \(\frac { 1 }{ 2 } \left[ \frac { 8 }{ 2 } th\quad term+\left( \frac { 8 }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \)[4th term + 5th term]
= \(\frac { 1 }{ 2 } \) (13.4 + 14.3) = \(\frac { 1 }{ 2 } \) (27.7) = 13.85
∴ Median weight = 13.85 kg. Ans.

Question 6.
Solution:
Arranging in ascending order, we get:
32, 34, 36, 37, 40, 44, 47, 50, 53, 54
Here, number of terms is 10 which is even.
∴Median = \(\frac { 1 }{ 2 } \left[ \frac { 10 }{ 2 } th\quad term+\left( \frac { 10 }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \) [5th term + 6th term ] = \(\frac { 1 }{ 2 } \) (40 + 44) = \(\frac { 1 }{ 2 } \) x 84 = 42 .
∴ Median age = 42 years.

Question 7.
Solution:
The given ten observations are 10, 13, 15, 18, x + 1, x + 3, 30, 32, 35, 41
These are even
∴Median = \(\frac { 1 }{ 2 } \left[ \frac { 10 }{ 2 } th\quad term+\left( \frac { 10 }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \) [5th term + 6th term ] = \(\frac { 1 }{ 2 } \)(x + 1 + x + 3) = \(\frac { 1 }{ 2 } \)(2x + 4)
= x + 2
But median is given = 24
∴ x + 2 = 24 => x = 24 – 2 = 22
Hence x = 22.

Question 8.
Solution:
Preparing the cumulative frequency table, we have:

Here, number of terms (n) = 41, which is odd,
Median = \(\frac { 41+1 }{ 2 } \) th term = \(\frac { 42 }{ 2 } \) th term = 21st term = 50 (∵ 20th to 28th term = 50)
Hence median weight = 50 kg Ans.

Question 9.
Solution:
Arranging first in ascending order, we get:

Now preparing its cumulative frequency table

Here, number of terms is 37 which is odd.
Median = \(\frac { 37+1 }{ 2 } \) th term = \(\frac { 38 }{ 2 } \) th term = 19 th term = 22 (∵18th to 21st = 22)
Hence median – 22 Ans.

Question 10.
Solution:
first arranging in ascending order we get

Now preparing its cumulative frequency table,we find:

Here, number of terms is 43, which if odd.
Median = \(\frac { 43+1 }{ 2 } \) th term = \(\frac { 44 }{ 2 } \) th term = 22nd term = 25 25 (∵ 11th to 26th = 25)

Question 11.
Solution:
Arranging in ascending order,we get

Now preparing its cumulative frequency table, we find :

Here, number of terms = 50 which is even
∴Median = \(\frac { 1 }{ 2 } \left[ \frac { 50 }{ 2 } th\quad term+\left( \frac { 50 }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \) (154 + 155) = \(\frac { 1 }{ 2 } \) (309) = 154.5 (∵ 22nd to 25th = 154, 26th to 34th= 155)

Question 12.
Solution:
Arranging in ascending order, we get:

Now, preparing its cumulative frequency table.

Here, number of terms is 60 which is even
∴Median = \(\frac { 1 }{ 2 } \left[ \frac { 60 }{ 2 } th\quad term+\left( \frac { 60 }{ 2 } +1 \right) th\quad term \right] \)
= \(\frac { 1 }{ 2 } \) (30th term + 31st term)
= \(\frac { 1 }{ 2 } \) (20 + 23) = \(\frac { 1 }{ 2 } \) x 43 = 21.5 (∵ 18th to 30th term = 20, 31st term to 34th = 23)
Hence median = 21.5 Ans.

Hope given RS Aggarwal Solutions Class 9 Chapter 14 Statistics Ex 14G are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 11 Circle Ex 11A

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11A.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11A
• RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11B
• RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11C

Question 1.
Solution:
Let AB be a chord of a circle with centre O. OC⊥AB and OA be the radius of the circle, then
AB = 16cm, OA = 10cm .

OC ⊥ AB.
OC bisects AB at C
AC = \(\frac { 1 }{ 2 } \) AB = \(\frac { 1 }{ 2 } \) x 16 = 8cm

Question 2.
Solution:
Let AB be the chord of the circle with centre O and OC ⊥ AB, OA be the radius of the circle,
then OC = 3cm, OA = 5cm

Now in right ∆ OAC,
OA² = AC² = OC² (Pythagoras Theorem)

Question 3.
Solution:
Let AB be the chord, OA be the radius of
the circle OC ⊥ AB
then AB = 30 cm, OC = 8cm

Question 4.
Solution:
AB and CD are parallel chords of a circle with centre O.

Question 5.
Solution:
Let AB and CD be two chords of a circle with centre O.
OA and OC are the radii of the circle. OL⊥AB and OM⊥CD.

Question 6.
Solution:
In the figure, a circle with centre O, CD is its diameter AB is a chord such that CD⊥AB.
AB = 12cm, CE = 3cm.
Join OA.
∵ COD⊥AB which intersects AB at E

Question 7.
Solution:
A circle with centre O, AB is diameter which bisects chord CD at E
i.e. CE = ED = 8cm and EB = 4cm
Join OC.
Let radius of the circle = r

Question 8.
Solution:
Given : O is the centre of a circle AB is a chord and BOC is the diameter. OD⊥AB
To prove : AC || OD and AC = 20D
Proof : OD⊥AB
∵ D is midpoint of AB

Question 9.
Solution:
Given : O is the centre of the circle two
chords AB and CD intersect each other at P inside the circle. PO bisects ∠BPD.
To prove : AB = CD.

Question 10.
Solution:
Given : PQ is the diameter of the circle with centre O which is perpendicular to one chord AB and chord AB || CD.
PQ intersects AB and CD at E and F respectively
To prove : PQ⊥CD and PQ bisects CD.

Question 11.
Solution:
Two circles with centre O and O’ intersect each other.

To prove : The two circles cannot intersect each other at more than two points.
Proof : Let if opposite, the two circles intersect each other at three points P, Q and R.
Then these three points are non-collinear. But, we know that through three non- collinear points, one and only one circle can be drawn.
∵ Our supposition is wrong
Hence two circle can not intersect each other at not more than two points.
Hence proved

Question 12.
Solution:
Given : Two circles with centres O and O’ intersect each other at A and B. AB is a common chord. OO’ is joined.
AO and AO is joined.

Question 13.
Solution:
Given : Two equal circles intersect each other at P and Q.
A straight line drawn through
P, is drawn which meets the circles at A and B respectively
To prove : QA = QB

Question 14.
Solution:
Given : A circle with centre 0. AB and CD are two chords and diameter PQ bisects AB and CD at L and M
To Prove : AB || CD.

Question 15.
Solution:
Given : Two circles with centres A and B touch each other at C internally. A, B arc joined. PQ is the perpendicular bisector of AB intersecting it at L and meeting the bigger circle at P and Q respectively and radii of the circles are 5cm and 3cm. i.e. AC = 5cm,BC = 3cm
Required : To find the lenght of PQ

Question 16.
Solution:
Given : AB is a chord of a circle with centre O. AB is produced to C such that BC = OB, CO is joined and produced to meet the circle at D.
∠ ACD = y°, ∠ AOD = x°
To prove : x = 3y

Question 17.
Solution:
Given : O is the centre of a circle AB and AC are two chords such that AB = AC
OP⊥AB and OQ⊥AC.
which intersect AB and AC at M and N
respectively. PB and QC are joined.
To prove : PB = QC.

Question 18.
Solution:
Given : In a circle with centre O, BC is its diameter. AB and CD are two chords such that AB || CD.
To prove : AB = CD
Const. Draw OL⊥AB
OM⊥CD.
Proof : In ∆ OLB and ∆ OCM,
OB = OC (radii of the same circle)
∠ OLB = ∠ OMC (each 90°)
∠ OBL = ∠ OCM (alternate angles)

Question 19.
Solution:
Equilateral ∆ ABC in inscribed in a circle in which
AB = BC = CA = 9cm.

Question 20.

Solution:
Given : AB and AC are two equal chords of a circle with centre O
To Prove : O lies on the bisector of ∠ BAC

Question 21.
Solution:
Given : OPQR is a square with centre O, a circle is drawn which intersects the square at X and Y.
To Prove : Q = QY
Const. Join OX and OY

 

Hope given RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 13 Volume and Surface Area Ex 13C

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Class 9 Solutions Chapter 13 Volume and Surface Area Ex 13C.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 13 Volume and Surface Area Ex 13A
• RS Aggarwal Solutions Class 9 Chapter 13 Volume and Surface Area Ex 13B
• RS Aggarwal Solutions Class 9 Chapter 13 Volume and Surface Area Ex 13C
• RS Aggarwal Solutions Class 9 Chapter 13 Volume and Surface Area Ex 13D

Question 1.
Solution:
Radius of base (r) = 35cm
and height (h) = 84cm.

Question 2.
Solution:
Height of cone (h) = 6cm
Slant height (l) = 10cm.

Question 3.
Solution:
Volume of right circular cone = (100 π) cm³
Height (h) = 12cm.
Let r be the radius of the cone

Question 4.
Solution:
Circumference of the base = 44cm

Question 5.
Solution:
Slant height of the cone (l) = 25cm
Curved surface area = 550 cm²
Let r be the radius
πrl = curved surface area

Question 6.
Solution:
Radius.of base (r) = 35cm.
Slant height (l) = 37cm.
We know that

Question 7.
Solution:
Curved surface area = 4070 cm²
Diameter of the base = 70cm

Question 8.
Solution:
Radius of the conical tent = 7m
and height = 24 m.

Question 9.
Solution:
Radius of the first cone (r) = 1.6 cm.
and height (h) = 3.6 cm.

Question 10.
Solution:
Ratio in their heights =1:3
and ratio in their radii = 3:1
Let h1,h2 he their height and r1,r2 be their radii, then

The ratio between their volumes is 3:1
hence proved

Question 11.
Solution:
Diameter of the tent = 105m

Question 12.
Solution:
No. of persons to be s accommodated =11
Area to be required for each person = 4m²

Question 13.
Solution:
Height of the cylindrical bucket (h) = 32cm
Radius (r) = 18cm
Volume of sand filled in it = πr²h
= π x 18 x 18 x 32 cm³
= 10368π cm³
Volume of conical sand = 10368 π cm³
Height of cone = 24 cm

Question 14.
Solution:
Let h be the height and r be the radius of the cylinder and cone.
Curved surface area of cylinder = 2πrh
and curved surface area of cone = πrl

Question 15.
Solution:
Diameter of the pillar = 20cm
Radius (r) = \(\frac { 20 }{ 2 } \) = 10cm

Question 16.
Solution:
Height of the bigger cone (H) = 30cm
By cutting a small cone from it, then volume of smaller cone = \(\frac { 1 }{ 27 } \) of volume of big cone

Let radius and height of the smaller cone be r and h
and radius and height of the bigger cone be R and H.


Hence at the height of 20cm from the base it was cut off. Ans.

Question 17.
Solution:
Height of the cylinder (h) = 10cm.
Radius (r) = 6cm.
Height of the cone = 10cm

Question 18.
Solution:
Diameter of conical vessel = 40cm
Radius (r) = \(\frac { 40 }{ 2 } \) = 20cm
and depth (h) = 24cm.
.’. Volume = \(\frac { 1 }{ 3 } \) πr²h

Hope given RS Aggarwal Class 9 Solutions Chapter 13 Volume and Surface Area Ex 13C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 11 Circle Ex 11C

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11C.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11A
• RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11B
• RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11C

Question 1.
Solution:
In cyclic quad. ABCD, ∠ DBC = 60° and ∠BAC = 40°
∴∠ CAD and ∠ CBD are in the same segment of the circle.
∴∠ CAD = ∠ CBD or ∠ DBC
=> ∠ CAD = 60°
∴∠BAD = ∠BAC + ∠CAD
= 40° + 60° = 100°
But in cyclic quad. ABCD,
∠BAD + ∠BCD = 180°
(Sum of opposite angles)
=> 100° + ∠BCD = 180°
=> ∠BCD = 180° – 100°
∴ ∠ BCD = 80°
Hence (i) ∠BCD = 80° and
(ii) ∠CAD = 60° Ans.

Question 2.
Solution:
In the figure, POQ is diameter, PQRS is a cyclic quad, and ∠ PSR =150° In cyclic quad. PQRS.
∠ PSR + ∠PQR = 180°
(Sum of opposite angles)
=> 150° + ∠PQR = 180°
=> ∠PQR = 180°- 150° = 30°
=> ∠PQR =180° – 150° = 30°
Now in ∆ PQR,
∴∠ PRQ = 90° (Angle in a semicircle)
∴∠ RPQ + ∠PQR = 90°
=> ∠RPQ + 30° = 90°
=> ∠RPQ = 90° – 30° = 60° Ans.

Question 3.
Solution:
In cyclic quad. ABCD,
AB || DC and ∠BAD = 100°
∠ ADC = ∠BAD =180°
(co-interior angles)

=> ∠ ADC + 100° = 180°
=> ∠ADC = 180° – 100° = 80°
∴ ABCD is a cyclic quadrilateral.
∴ ∠BAD + ∠BCD = 180°
=> 100° + ∠ BCD = 180°
=> ∠BCD = 180° – 100°
=> ∠BCD = 80°
Similarly ∠ABC + ∠ADC = 180°
=> ∠ABC + 80° = 180°
=> ∠ABC = 180° – 80° = 100°
Hence (i) ∠BCD = 80° (ii) ∠ADC = 80° and (iii) ∠ABC = 100° Ans.

Question 4.
Solution:
O is the centre of the circle and arc ABC subtends an angle of 130° at the centre i.e. ∠AOC = 130°. AB is produced to P
Reflex ∠AOC = 360° – 130° = 230°
Now, arc AC subtends reflex ∠ AOC at the centre and ∠ ABC at the remaining out of the circle.

Question 5.
Solution:
In the figure, ABCD is a cyclic quadrilateral in which BA is produced to F and AE is drawn parallel to CD.
∠ABC = 92° and ∠FAE = 20°
ABCD is a cyclic quadrilateral.

Question 6.
Solution:
In the figure, BD = DC and ∠CBD = 30°
In ∆ BCD,
BD = DC (given)
∠ BCD = ∠ CBD
(Angles opposite to equal sides)
= 30°
But ∠BCD + ∠CBD + ∠BDC = 180° (Angles of a triangle)
=> 30°+ 30°+ ∠BDC = 180°
=> 60°+ ∠BDC = 180°
=> ∠ BDC =180° – 60° = 120°
But ABDC is a cyclic quadrilateral
∠BAC + ∠BDC = 180°
=> ∠BAC + 120°= 180°
=> ∠ BAC = 180° – 120° = 60°
Hence ∠ BAC = 60° Ans.

Question 7.
Solution:
(i) Arc ABC subtends ∠ AOC at the centre , and ∠ ADC at the remaining part of the circle.
∠ AOC = 2 ∠ ADC

Question 8.
Solution:
In the figure, ABC is an equilateral triangle inscribed is a circle
Each angle is of 60°.
∠ BAC = ∠ BDC
(Angles in the same segment)
∠BDC = 60°
BECD is a cyclic quadrilateral.
∠BDC + ∠BEC = 180°
(opposite angles of cyclic quad.)
=> 60°+ ∠BEC = 180°
=> ∠BEC = 180° – 60°= 120°
Hence ∠BDC = 60° and ∠BEC = 120° Ans.

Question 9.
Solution:
ABCD is a cyclic quadrilateral.
∠BCD + ∠BAD = 180°
(opposite angles of a cyclic quad.)
=> 100°+ ∠BAD = 180°
so ∠BAD = 180° – 100° = 80°
Now in ∆ ABD,
∠BAD + ∠ABD + ∠ADB = 180° (Angles of a triangle)

=> 80° + 50° + ∠ADB = 180°
=> 130°+ ∠ADB = 180°
=> ∠ADB = 180° – 130° = 50°
Hence, ∠ADB = 50° Ans.

Question 10.
Solution:
Arc BAD subtends ∠ BOD at the centre and ∠BCD at the remaining part of the circle.

Question 11.
Solution:
In ∆ OAB,
OA = OB (radii of the same circle)
∠OAB = ∠OBA = 50°
and Ext ∠BOD = ∠OAB + ∠OBA
=>x° = 50° + 50° – 100°
ABCD is a cyclic quadrilateral
∠BAD + ∠BCD = 180°
(opposite angles of a cyclic quad.)
=> 50°+ y° = 180°
=> y° = 180° – 50° = 130°
Hence x = 100° and y = 130° Ans.

Question 12.
Solution:
Sides AD and AB of cyclic quadrilateral ABCD are produced to E and F respectively.
∠CBF = 130°, ∠CDE = x.
∠CBF + ∠CBA = 180° (Linear pair)
=> 130°+ ∠CBA = 180°
=> ∠CBA = 180° – 130° = 50°
But Ext. ∠ CDE = Interior opp. ∠ CBA (In cyclic quad. ABCD)
=> x = 50° Ans.

Question 13.
Solution:
In a circle with centre O AB is its diameter and DO || CB is drawn. ∠BCD = 120°
To Find : (i) ∠BAD (ii) ABD
(iii) ∠CBD (iv) ∠ADC
(v) Show that ∆ AOD is an equilateral triangle.
(i) ABCD is a cyclic quadrilateral.
∠BCD + ∠BAD = 180°
120° + ∠BAD = 180°

Question 14.
Solution:
AB = 6cm, BP = 2cm, DP = 2.5cm
Let CD = xcm
Two chords AB and CD

Question 15.
Solution:
O is the centre of the circle
∠ AOD = 140° and ∠CAB = 50°
BD is joined.
(i) ABDC is a cyclic quadrilateral.

Question 16.
Solution:
Given : ABCD is a cyclic quadrilateral whose sides AB and DC are produced to meet each other at E.
To Prove : ∆ EBC ~ ∆ EDA
Proof : In ∆ EBC and ∆ EDA
∠ E = ∠ E (common)
∠ECB = ∠EAD
{Exterior angle of a cyclic quad, is equal to its interior opposite angle}
and ∠ EBC = ∠EDA
∆ EBC ~ ∆ EDA (AAS axiom)
Hence proved

Question 17.
Solution:
Solution Given : In an isosceles ∆ ABC, AB = AC
A circle is drawn x in such a way that it passes through B and C and intersects AB and AC at D and E respectively.
DE is joined.
To Prove : DE || BC
Proof : In ∆ ABC,
AB = AC (given)
∠ B = ∠ C (angles opposite to equal sides)
But ∠ ADE = ∠ C (Ext. angle of a cyclic quad, is equal E to its interior opposite angle)
∠ADE = ∠B
But, these are corresponding angles
DE || BC.
Hence proved.

Question 18.
Solution:
Given : ∆ ABC is an isosceles triangle in which AB = AC.
D and E are midpoints of AB and AC respectively.
DE is joined.
To Prove : D, B, C, E are concyclic.
Proof: D and E are midpoints of sides AB and AC respectively.
DE || BC
In ∆ ABC, AB = AC
∠B = ∠C
But ∠ ADE = ∠ B (alternate angles)
∠ADE =∠C
But ∠ADE is exterior angle of quad. DBCE which is equal to its interior opposite angle C.
DBCE is a cyclic quadrilateral.
Hence D, B, C, E are con cyclic.
Hence proved.

Question 19.
Solution:
Given : ABCD is a cyclic quadrilateral whose perpendicular bisectors l, m, n, p of the side are drawn

To prove : l, m, n and p are concurrent.
Proof : The sides AB, BC, CD and DA are the chords of the circle passing through the vertices’s of quad. A, B, C and D. and perpendicular bisectors of a chord always passes through the centre of the circle.
l,m, n and p which are the perpendicular bisectors of the sides of cyclic quadrilateral will pass through O, the same point Hence, l, m, n and p are concurrent.
Hence proved.

Question 20.
Solution:
Given : ABCD is a rhombus and four circles are drawn on the sides AB, BC, CD and DA as diameters. Diagonal AC and BD intersect each other at O.

Question 21.
Solution:
Given: ABCD is a rectangle whose diagonals AC and BD intersect each other at O.
To prove : O is the centre of the circle passing through A, B, C and D

Question 22.
Solution:
Construction.
(i) Let A, B and C are three points
(ii) With A as centre and BC as radius draw an arc
(iii) With centre C, and radius AB, draw another arc which intersects the first arc at D.
D is the required point.
Join BD and CD, AC and BA and CB

BC = BC (common)
AC = BD (const.)
AB = DC
∴ ∆ ABC ≅ ∆ DBC (SSS axiom)
∴ ∠BAC ≅ ∠BDC (c.p.c.t.)
But these are angles on the same sides of BC
Hence these are angles in the same segment of a circle
A, B, C, D are concyclic Hence D lies on the circle passing througtvA, B and C.
Hence proved.

Question 23.
Solution:
Given : ABCD is a cylic quadrilateral (∠B – ∠D) = 60°
To prove : The small angle of the quad, is 60°

Question 24.

Solution:
Given : ABCD is a quadrilateral in which AD = BC and ∠ ADC = ∠BCD
To prove : A, B, C and D lie on a circle

Question 25.
Solution:
Given : In the figure, two circles intersect each other at D and C
∠BAD = 75°, ∠DCF = x° and ∠DEF = y°

Question 26.
Solution:
Given : ABCD is a cyclic quadrilateral whose diagonals AC and BD intersect at O at right angle.

Question 27.
Solution:
In a circle, two chords AB and CD intersect each other at E when produced.
AD and BC are joined.

Question 28.
Solution:
Given : Two parallel chords AB and CD of a circle BD and AC are joined and produced to meet at E.

Question 29.
Solution:
Given : In a circle with centre O, AB is its diameter. ADE and CBE are lines meeting at E such that ∠BAD = 35° and ∠BED = 25°.
To Find : (i) ∠DBC (ii) ∠DCB (iii) ∠BDC
Solution. Join BD and AC,

Hope given RS Aggarwal Solutions Class 9 Chapter 11 Circle Ex 11C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 9 Solutions Chapter 9 Quadrilaterals and Parallelograms Ex 9A

These Solutions are part of RS Aggarwal Solutions Class 9. Here we have given RS Aggarwal Class 9 Solutions Chapter 9 Quadrilaterals and Parallelograms Ex 9A.

Other Exercises

• RS Aggarwal Solutions Class 9 Chapter 9 Quadrilaterals and Parallelograms Ex 9A
• RS Aggarwal Solutions Class 9 Chapter 9 Quadrilaterals and Parallelograms Ex 9B
• RS Aggarwal Solutions Class 9 Chapter 9 Quadrilaterals and Parallelograms Ex 9C

Question 1.
Solution:
We know that sum of angles of a quadrilateral is 360°
Now, sum of three angles = 56° + 115° + 84° = 255°
Fourth angle = 360° – 255° = 105° Ans.

Question 2.
Solution:
Sum of angles of a quadrilateral = 360°
Their ratio = 2 : 4 : 5 : 7
Let first angle = 2x
then second angle = 4x
third angle = 5x
and fourth angle = 7x
∴ 2x + 4x + 5x + 7x = 360°
=> 18x = 360°
=> x = \(\frac { { 360 }^{ o } }{ 18 } \) = 20°
Hence, first angle = 2x = 2 x 20° = 40°
Second angle = 4x = 4 x 20° = 80°
Third angle = 5x = 5 x 20° = 100°
and fourth angle = 7x = 7 x 20° = 140°Ans.

Question 3.
Solution:
In the trapezium ABCD
DC || AB
∴ ∠ A + ∠ D = 180° (Co-intericr angles)
∴ 55°+ ∠D = 180°
∠D = 180° – 55°
∴ ∠D = 125°
Similarly, ∠B + ∠C = 180°
(Co-interior angles)
=> 70° + ∠C = 180°
=> ∠C = 180° – 70°
∠C = 110°
Hence ∠C = 110° and ∠D = 125° Ans.

Question 4.
Solution:
Given : In the figure, ABCD is a square and ∆ EDC is an equilateral triangles on DC. AE and BE are joined.
To Prove : (i) AE = BE
(ii) ∠DAE = 15°

Question 5.
Solution:
Given : In the figure,
BM ⊥ AC, DN ⊥ AC.
BM = DN

Question 6.
Solution:
Given : In quadrilateral ABCD,
AB = AD and BC = DC
AC is joined.
To Prove : (i) AC bisects ∠ A and ∠ C
(ii) BE = DE
(iii) ∠ABC = ∠ADC
Const. Join BD.
Proof : (i) In ∆ ABC and ∆ ADB

Question 7.
Solution:
Given : In square ABCD,
∠ PQR = 90°
PB = QC = DR

Question 8.
Solution:
Given : In quadrilateral ABCD, O is any point inside it. OA, OB, OC and OD are joined.
To Prove : OA + OB + OC + OD > AC + BD

Question 9.
Solution:
Given : In quadrilateral ABCD, AC is its one diagonal.

Question 10.
Solution:
Given : A quadrilateral ABCD
To Prove : ∠A + ∠B + ∠C + ∠D = 360°
Const. Join AC.

 

Hope given RS Aggarwal Class 9 Solutions Chapter 9 Quadrilaterals and Parallelograms Ex 9A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.