Tag: RS Aggarwal Maths Solutions Class 6

RS Aggarwal Class 6 Solutions Chapter 20 Two-Dimensional Reflection Symmetry (Linear Symmetry) Ex 20

These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 20 Two-Dimensional Reflection Symmetry (Linear Symmetry) Ex 20

Mark against the correct answer in each of Q. 1 to Q. 8.

Question 1.
Solution:
(d)∵ A square has four lines of symmetry, two diagonals and two lines joining the mid-points of opposite sides.

Question 2.
Solution:
(c) ∵ A rectangle has two lines of symmetry, each one of which being the line joining of mid-points of opposite sides.

Question 3.
Solution:
(b) ∵ A rhombus has two lines of symmetry namely two diagonals.

Question 4.
Solution:
(d) Each diameter of a circle is its line of symmetry which are unlimited numbers.

Question 5.
Solution:
(a) ∵ A scalene triangle has no line of symmetry.

Question 6.
Solution:
(a) ∵ It is a figure of kite ; so AC is its line of symmetry.

Question 7.
Solution:
(c) ∵ Letter O has two lines of symmetry, one vertical and second horizontal

Question 8.
Solution:
(a) ∵ Letter Z has no line of symmetry.

Question 9.
Solution:

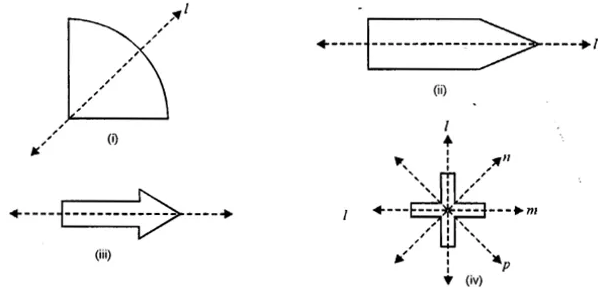
Question 10.
Solution:
(i) True (T) Parallelogram has no line of symmetry.
(ii) True (T) Bisector of an angle of*equal sides is the line of symmetry.
(iii) True (T)  Perpendiculars from each vertices’s of an equilateral-triangle to its opposite side is its line of symmetry.
(iv) False (F) Rhombus has two lines of symmetry which are its -diagonals.
(v) True (T) Square has four lines of symmetry, two diagonals and two perpendicular bisectors of opposite sides.
(vi) True (T) A rectangle has two lines of symmetry which are the perpendicular bisectors of its opposite sides.
(vii) True (T) H, I, O and X has two lines of symmetry.

 

Hope given RS Aggarwal Solutions Class 6 Chapter 20 Two-Dimensional Reflection Symmetry (Linear Symmetry) Ex 20 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 6 Solutions Chapter 19 Three-Dimensional Shapes Ex 19

These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 19 Three-Dimensional Shapes Ex 19

Tick the correct answer in each of Q. 1 to Q. 6.

Question 1.
Solution:
(c) ∵ A cuboid has three dimensions, length, breadth and height or depth.

Question 2.
Solution:
(b) ∵ Its six faces arc of square.

Question 3.
Solution:
(d) ∵ Its shape is of a cylinder as it is round in shape on either sides/faces.

Question 4.
Solution:
(c) ∵ Football is round as sphere.

Question 5.
Solution:
(b) A brick has length, breadth and height.

Question 6.
Solution:
(d) ∵ Its shape is like a cone. Ans.

Question 7.
Solution:
(i) solid
(ii) 6, 12 and 18
(iii) opposite
(iv) sphere
(v) cube
(vi) 4, 8
(vii) 3, 6
(viii) 6, 3, 2, 9 Ans.

Question 8.
Solution:
(a) A cone:
(i) Conical cup
(ii) An ice cream cup
(iii) Conical tent house
(iv) Conical vessel.
(b) A cuboid :
(i) A book,
(ii) A brick,
(iii) a box,
(iv) a briefcase.
(c) A cylinder
(i) Circular pipe
(ii) A jar or tumbler
(iii) A round powder tin
(iv) Circular pillar.

 

Hope given RS Aggarwal Solutions Class 6 Chapter 19 Three-Dimensional Shapes Ex 19 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 6 Solutions Chapter 5 Fractions Ex 5F

These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5F.

Other Exercises

• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5A
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5B
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5C
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5D
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5E
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5F
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5G

Find the difference:

Question 1.
Solution:
\(\frac { 5 }{ 8 } -\frac { 1 }{ 8 } \)
= \(\\ \frac { 5-1 }{ 8 } \)
= \(\frac { 4 }{ 8 } \)
= \(\frac { 4\div 4 }{ 8\div 4 } \)
= \(\frac { 1 }{ 2 } \)

Question 2.
Solution:
\(\frac { 7 }{ 12 } -\frac { 5 }{ 12 } \)

Question 3.
Solution:
\(4\frac { 3 }{ 7 } -2\frac { 4 }{ 7 } \)
= \(\frac { 31 }{ 7 } -\frac { 18 }{ 7 } \)

Question 4.
Solution:
\(\frac { 5 }{ 6 } -\frac { 4 }{ 9 } \)

Question 5.
Solution:
\(\frac { 1 }{ 2 } -\frac { 3 }{ 8 } \)

Question 6.
Solution:
\(\frac { 5 }{ 8 } -\frac { 7 }{ 12 } \)

Question 7.
Solution:
\(2\frac { 7 }{ 9 } -1\frac { 8 }{ 15 } \)
= \(\frac { 25 }{ 9 } -\frac { 23 }{ 15 } \)
(changing into improper fractions)

Question 8.
Solution:
\(3\frac { 5 }{ 8 } -2\frac { 5 }{ 12 } \)
= \(\frac { 29 }{ 8 } -\frac { 29 }{ 12 } \)

Question 9.
Solution:
\(2\frac { 3 }{ 10 } -1\frac { 7 }{ 15 } \)
= \(\frac { 23 }{ 10 } -\frac { 22 }{ 15 } \)

Question 10.
Solution:
\(6\frac { 2 }{ 3 } -3\frac { 3 }{ 4 } \)
= \(\frac { 20 }{ 3 } -\frac { 15 }{ 4 } \)

Question 11.
Solution:
\(7-5\frac { 2 }{ 3 } \)
= \(\frac { 7 }{ 1 } -\frac { 17 }{ 3 } \)
(changing into improper fractions)

Question 12.
Solution:
\(10-6\frac { 3 }{ 8 } \)
= \(\frac { 10 }{ 1 } -\frac { 51 }{ 8 } \)
(changing into improper fractions)

Simpilify

Question 13.
Solution:
\(\frac { 5 }{ 6 } -\frac { 4 }{ 9 } +\frac { 2 }{ 3 } \)

Question 14.
Solution:
\(\frac { 5 }{ 8 } +\frac { 3 }{ 4 } -\frac { 7 }{ 12 } \)

Question 15.
Solution:
\(2+\frac { 11 }{ 15 } -\frac { 5 }{ 9 } \)
= \(\frac { 90+33-25 }{ 45 } \)
(LCM of 15 and 9 = 45)

Question 16.
Solution:
\(5\frac { 3 }{ 4 } -4\frac { 5 }{ 12 } +3\frac { 1 }{ 6 } \)
= \(\frac { 23 }{ 4 } -\frac { 53 }{ 12 } +\frac { 19 }{ 6 } \)
(changing into improper fractions)

Question 17.
Solution:
\(2+5\frac { 7 }{ 10 } -3\frac { 14 }{ 15 } \)
= \(\frac { 2 }{ 1 } +\frac { 57 }{ 10 } -\frac { 59 }{ 15 } \)
(changing into improper fractions)

Question 18.
Solution:
\(8-3\frac { 1 }{ 2 } -2\frac { 1 }{ 4 } \)
= \(\frac { 8 }{ 1 } -\frac { 7 }{ 2 } -\frac { 9 }{ 4 } \)
(changing into improper fractions)

Question 19.
Solution:
\(8\frac { 5 }{ 6 } -3\frac { 3 }{ 8 } +2\frac { 7 }{ 12 } \)
= \(\frac { 53 }{ 6 } -\frac { 27 }{ 8 } +\frac { 31 }{ 12 } \)
(changing into improper fractions)

Question 20.
Solution:
\(6\frac { 1 }{ 6 } -5\frac { 1 }{ 5 } +3\frac { 1 }{ 3 } \)
= \(\frac { 37 }{ 6 } -\frac { 26 }{ 5 } +\frac { 10 }{ 3 } \)
(changing into improper fractions)

Question 21.
Solution:
\(3+1\frac { 1 }{ 5 } +\frac { 2 }{ 3 } -\frac { 7 }{ 15 } \)
= \(\frac { 3 }{ 1 } +\frac { 6 }{ 5 } +\frac { 2 }{ 3 } -\frac { 7 }{ 15 } \)

Question 22.
Solution:
By subtracting \(9 \frac { 2 }{ 3 } \) from 19, we get the required number

Question 23.
Solution:
By subtracting \(6 \frac { 7 }{ 15 } \) from \(8 \frac { 1 }{ 5 } \) we get the required number

Question 24.
Solution:
Sum of \(3 \frac { 5 }{ 9 } \) and \(3 \frac { 1 }{ 3 } \)
= \(\frac { 32 }{ 9 } +\frac { 10 }{ 3 } \)

Question 25.
Solution:
\(\\ \frac { 3 }{ 4 } \), \(\\ \frac { 5 }{ 7 } \)

Question 26.
Solution:
Milk bought by Mrs. Soni = \(7 \frac { 1 }{ 2 } \) litres
and milk consumed by here = \(5 \frac { 3 }{ 4 } \) litres

Question 27.
Solution:
Total time of film show = \(3 \frac { 1 }{ 3 } \) hours
Total spent on advertisement = \(1 \frac { 3 }{ 4 } \) hours
Duration of the film

Question 28.
Solution:
On a day, rickshaw pullar earned

Question 29.
Solution:
Total length of wire =\(2 \frac { 3 }{ 4 } \)-metres
Length of one piece = \(\\ \frac { 5 }{ 8 } \) metre
Length of the other piece

Hope given RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5F are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 6 Solutions Chapter 18 Circles Ex 18

These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 18 Circles Ex 18

Question 1.
Solution:
Method :

Take a point O on the paper as shown in the figure. With the help of the rular, open out compasses in such a way that the distance between the metal point and pencil point is 4 cm. Take the compasses in the same position and put its metal point at O and draw the circle.
Remove the compasses and again open out the compasses in such a way that the distance between the metal point and pencil point is 5.3 cm. Taking O as the centre, draw another circle. Again remove the compasses and similarly draw the third circle with radius 6.2 cm. Then the required circles are as shown in the figure which have radius OA = 4 cm., OB = 5.3 cm. and OC = 6.2 cm.

Question 2.
Solution:
Method : Take a point C on the paper. With the help of the rular, open out the compasses in such a way that the distance between its metal point and pencil point is 4.5 cm. Take the compasses in the same position and put its metal point at C and draw the circle. Mark points P, Q and R as shown in the figure as required.

Question 3.
Solution:
Method : Take a point O on the paper. With the help of the rular, open out the compasses in such a way that the distance between the metal point and pencil point is 4 cm. Take the compasses in the same position and put the metal point at O and draw the circle.

Take A and B any points on the circle and join AB. ThenAB is the chord of the circle. Mark points X and Y on the circle as shown. Then arc AXB and arc AYB are the required minor and major arcs respectively.

Question 4.
Solution:
(i) False
(ii) True
(iii) False
(iv) False
(v) True.

Question 5.
Solution:
Steps of construction :
(i) With centre O and radius 3.7 cm, draw a circle.
(ii) Take a point A on the circumference of the circle.
(iii) Join OA.
(iv) At O, draw another radius OB such that ∠AOB = 72° with the help of protractor. Then sector AOB is the required one.

Question 6.
Solution:
(i) > (ii) < (iii) > (iv) >. Ans.

Question 7.
Solution:
(i) Passes through
(ii) at the centre, on the circle
(iii) chord
(iv) arc
(v) sector.

 

Hope given RS Aggarwal Solutions Class 6 Chapter 18 Circles Ex 18 are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 6 Solutions Chapter 17 Quadrilaterals Ex 17B

These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 17 Quadrilaterals Ex 17B

Other Exercises

• RS Aggarwal Solutions Class 6 Chapter 17 Quadrilaterals Ex 17A
• RS Aggarwal Solutions Class 6 Chapter 17 Quadrilaterals Ex 17B

Objective questions
Mark against the correct answer in each of the following :

Question 1.
Solution:
(c) ∵ Sum of angles of a quadrilateral is 360°.

Question 2.
Solution:
Sum of 4 angles of a quadrilateral = 360° and three angles of a quadrilateral are 80°, 70° and 120°
∵ Fourth angle = 360° – (80° + 70° + 120°)
= 360° – 270° – 90° (c)

Question 3.
Solution:
Sum of angles of a quadrilateral = 360°
The ratio in there four angles is 3 : 4 : 5 : 6

Question 4.
Solution:
(d) Y Quadrilateral having one pair of parallel sides is a trapezium.

Question 5.
Solution:
(d) ∵ Quadrilateral having opposite sides parallel is called a parallelogram.

Question 6.
Solution:
(b) ∵ A trapezium having nonparallel sides equal is called an isosceles trapezium.

Question 7.
Solution:
(b) ∵ Diagonals of a rhombus bisect each other at right angles.

Question 8.
Solution:
(b) ∵ A square has four equal sides and also diagonals are equal.

Question 9.
Solution:
A quadrilateral having two pairs of equal adjacent sides but unequal opposite angles is called a kite. (c)

Question 10.
Solution:
A regular quadrilateral is a quadrilateral having equal sides and equal angles which is a square. (c)

Hope given RS Aggarwal Solutions Class 6 Chapter 17 Quadrilaterals Ex 17B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 6 Solutions Chapter 5 Fractions Ex 5E

These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5E.

Other Exercises

• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5A
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5B
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5C
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5D
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5E
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5F
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5G

Find the sum :

Question 1.
Solution:
\(\frac { 5 }{ 8 } +\frac { 1 }{ 8 } \)

Question 2.
Solution:
\(\frac { 4 }{ 9 } +\frac { 8 }{ 9 } \)

Question 3.
Solution:
\(1\frac { 3 }{ 5 } +2\frac { 4 }{ 5 } \)
\(\frac { 8 }{ 5 } +\frac { 14 }{ 5 } \)

Question 4.
Solution:
\(\frac { 2 }{ 5 } +\frac { 5 }{ 6 } \)
= \(\\ \frac { 4+15 }{ 18 } \) (LCM of 9 and 6 = 18)
= \(\\ \frac { 19 }{ 18 } \)
= \(1 \frac { 1 }{ 18 } \)

Question 5.
Solution:
\(\frac { 7 }{ 12 } +\frac { 9 }{ 16 } \)

Question 6.
Solution:
\(\frac { 4 }{ 15 } +\frac { 17 }{ 20 } \)

Question 7.
Solution:
\(2\frac { 3 }{ 4 } +5\frac { 5 }{ 6 } \)
= \(\frac { 11 }{ 4 } +\frac { 35 }{ 6 } \)

Question 8.
Solution:
\(3\frac { 1 }{ 8 } +1\frac { 5 }{ 12 } \)
= \(\frac { 25 }{ 8 } +\frac { 17 }{ 12 } \)

Question 9.
Solution:
\(2\frac { 7 }{ 10 } +3\frac { 8 }{ 15 } \)
= \(\frac { 27 }{ 10 } +\frac { 53 }{ 15 } \)

Question 10.
Solution:
\(3\frac { 2 }{ 3 } +1\frac { 5 }{ 6 } +2 \)
\(\frac { 11 }{ 3 } +\frac { 11 }{ 6 } +\frac { 2 }{ 1 } \)

Question 11.
Solution:
\(3+1\frac { 4 }{ 15 } +1\frac { 3 }{ 20 } \)
=\(\frac { 3 }{ 1 } +\frac { 19 }{ 15 } +\frac { 23 }{ 20 } \)

Question 12.
Solution:
\( 3\frac { 1 }{ 3 } +4\frac { 1 }{ 4 } +6\frac { 1 }{ 6 } \)
\(\frac { 10 }{ 3 } +\frac { 17 }{ 4 } +\frac { 37 }{ 6 } \)
(changing into improper fractions)

Question 13.
Solution:
\(\frac { 2 }{ 3 } +3\frac { 1 }{ 6 } +4\frac { 2 }{ 9 } +2\frac { 5 }{ 18 } \)
\(\frac { 2 }{ 3 } +\frac { 19 }{ 6 } +\frac { 38 }{ 9 } +\frac { 41 }{ 18 } \)

Question 14.
Solution:
\(2\frac { 1 }{ 3 } +1\frac { 1 }{ 4 } +2\frac { 5 }{ 6 } +3\frac { 7 }{ 12 } \)

Question 15.
Solution:
\(2+\frac { 3 }{ 4 } +1\frac { 5 }{ 6 } +3\frac { 7 }{ 16 } \)
\(\frac { 2 }{ 1 } +\frac { 3 }{ 4 } +\frac { 13 }{ 8 } +\frac { 55 }{ 16 } \)

Question 16.
Solution:
Cost of a pencil = Rs. \(3 \frac { 2 }{ 5 } \)
Cost of an eraser = Rs.\(2 \frac { 7 }{ 10 } \)

Question 17.
Solution:
Length of cloth for kurta = \(4 \frac { 1 }{ 2 } \) metres
Length of cloth for pyjamas = \(2 \frac { 2 }{ 3 } \) metres

Question 18.
Solution:
Distance travelled by Rickshaw = \(4 \frac { 3 }{ 4 } \) km
Distance travelled on foot = \(1 \frac { 1 }{ 2 } \) km

Question 19.
Solution:
Weight of empty cylinder = \(16 \frac { 4 }{ 5 } \) kg
Weight of gas filled in it = \(14 \frac { 2 }{ 3 } \) kg
Total. weight of cylinder with gas

Hope given RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5E are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 6 Solutions Chapter 5 Fractions Ex 5D

These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5D.

Other Exercises

• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5A
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5B
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5C
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5D
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5E
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5F
• RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5G

Question 1.
Solution:
(i) Like fraction : Fractions having the same denominators are called like fractions. For examples:
\(\frac { 2 }{ 11 } ,\frac { 3 }{ 11 } ,\frac { 4 }{ 11 } ,\frac { 5 }{ 11 } ,\frac { 8 }{ 11 } \)
(ii) Unlike fraction : Fraction having the different denominators, are called unlike fractions. For examples:
\(\frac { 1 }{ 3 } ,\frac { 4 }{ 7 } ,\frac { 5 }{ 9 } ,\frac { 3 }{ 8 } ,\frac { 7 }{ 11 } \)

Question 2.
Solution:
We know that like fractions have same denominator
Now \(\frac { 3 }{ 5 } ,\frac { 7 }{ 10 } ,\frac { 8 }{ 15 } ,\frac { 11 }{ 30 } \)
LCM of 5, 10, 15 and 30 = 30

Question 3.
Solution:
We know that like fraction have same denominators
\(\frac { 1 }{ 4 } ,\frac { 5 }{ 8 } ,\frac { 7 }{ 12 } ,\frac { 13 }{ 24 } \)
LCM of 4, 8, 12, 24 = 24

Question 4.
Solution:

Question 5.
Solution:

Compare the fractions given below :

Question 6.
Solution:
\(\frac { 4 }{ 5 } and\frac { 5 }{ 7 } \)
LCM of 5 and 7 = 35

Question 7.
Solution:
\(\frac { 3 }{ 8 } and\frac { 5 }{ 6 } \)
LCM of 8 and 6 = 24

Question 8.
Solution:
\(\frac { 7 }{ 11 } and\frac { 6 }{ 7 } \)
LCM of 11 and 7 = 77

Question 9.
Solution:
\(\frac { 5 }{ 6 } and\frac { 9 }{ 11 } \)
LCM of 6 and 11 = 66

Question 10.
Solution:
\(\frac { 2 }{ 3 } and\frac { 4 }{ 9 } \)
LCM of 3 and 9 = 9

Question 11.
Solution:
\(\frac { 6 }{ 13 } and\frac { 3 }{ 4 } \)
LCM of 13 and 4 = 52

Question 12.
Solution:
\(\frac { 3 }{ 4 } and\frac { 5 }{ 6 } \)
LCM of 4 and 6 = 12

Question 13.
Solution:
\(\frac { 5 }{ 8 } and\frac { 7 }{ 12 } \)
LCM of 8 and 12 = 24

Question 14.
Solution:
\(\frac { 4 }{ 9 } and\frac { 5 }{ 6 } \)
LCM of 9 and 6 = 18

Question 15.
Solution:
\(\frac { 4 }{ 5 } and\frac { 7 }{ 10 } \)
LCM of 5 and 10 = 10

Question 16.
Solution:
\(\frac { 7 }{ 8 } and\frac { 9 }{ 10 } \)
LCM of 8 and 10 = 40

Question 17.
Solution:
\(\frac { 11 }{ 12 } and\frac { 13 }{ 15 } \)
LCM of 12 and 15 = 60

Question 18.
Solution:
\(\frac { 1 }{ 2 } ,\frac { 3 }{ 4 } ,\frac { 5 }{ 6 } and\frac { 7 }{ 8 } \)
LCM of 2, 4, 6 and 8 = 24

Question 19.
Solution:
\(\frac { 2 }{ 3 } ,\frac { 5 }{ 6 } ,\frac { 7 }{ 9 } and\frac { 11 }{ 18 } \)
LCM of 3, 6, 9 and 18 = 18

Question 20.
Solution:
\(\frac { 2 }{ 5 } ,\frac { 7 }{ 10 } ,\frac { 11 }{ 15 } and\frac { 17 }{ 30 } \)
LCM of 5, 10, 15 and 30 = 30

Question 21.
Solution:
\(\frac { 3 }{ 4 } ,\frac { 7 }{ 8 } ,\frac { 11 }{ 16 } and\frac { 23 }{ 32 } \)
LCM of 4, 8, 16 and 32 = 32

Arrange the following fractions in the descending order :

Question 22.
Solution:
\(\frac { 3 }{ 4 } ,\frac { 5 }{ 8 } ,\frac { 11 }{ 12 } and\frac { 17 }{ 24 } \)
LCM of 4, 8, 12 and 24 = 24

Question 23.
Solution:
\(\frac { 7 }{ 9 } ,\frac { 5 }{ 12 } ,\frac { 11 }{ 18 } and\frac { 17 }{ 36 } \)
LCM of 9, 12, 18 and 36 = 36

Question 24.
Solution:
\(\frac { 2 }{ 3 } ,\frac { 3 }{ 5 } ,\frac { 7 }{ 10 } and\frac { 8 }{ 15 } \)
LCM of 3, 5, 10 and 15 = 30

Question 25.
Solution:
\(\frac { 5 }{ 7 } ,\frac { 9 }{ 14 } ,\frac { 17 }{ 21 } and\frac { 31 }{ 42 } \)
LCM of 7, 14, 21 and 42 = 42

Question 26.
Solution:
∴ the numerators are equal
∴ The fraction having small denominator is greater than the fraction having large denominator
∴ In descending order, we can write
\(\frac { 1 }{ 12 } ,\frac { 1 }{ 23 } ,\frac { 1 }{ 7 } ,\frac { 1 }{ 9 } ,\frac { 1 }{ 17 } ,\frac { 1 }{ 50 } \)

Question 27.
Solution:
Here, the numerators of all fractions are equal
∴ The fraction having small denominator is greater than the fraction having large denominator
Now in descending order is
\(\frac { 3 }{ 4 } ,\frac { 3 }{ 5 } ,\frac { 3 }{ 7 } ,\frac { 3 }{ 11 } ,\frac { 3 }{ 13 } ,\frac { 3 }{ 17 } \)

Question 28.
Solution:
Lalita read 30 pages of a book containing 100 pages
She read \(\\ \frac { 30 }{ 100 } \) = \(\\ \frac { 3 }{ 10 } \) part of the book and Sarita read \(\\ \frac { 2 }{ 5 } \) of the book
Now in \(\\ \frac { 3 }{ 10 } \) and \(\\ \frac { 2 }{ 5 } \), LCM of 10, 5 = 10
\(\\ \frac { 3 }{ 10 } \) = \(\\ \frac { 3 }{ 10 } \)
\(\\ \frac { 2 }{ 5 } \) = \(\\ \frac { 2\times 2 }{ 5\times 2 } \) = \(\\ \frac { 4 }{ 10 } \)
From above, Sarita read more
as \(\\ \frac { 4 }{ 10 } \) or \(\frac { 2 }{ 5 } >\frac { 3 }{ 10 } \)

Question 29.
Solution:
Rafiq exercised for \(\\ \frac { 2 }{ 3 } \) hour and Rohit exercised for \(\\ \frac { 3 }{ 4 } \) hour
In \(\\ \frac { 2 }{ 3 } \) and \(\\ \frac { 3 }{ 4 } \), LCM of 3 and 4 = 12
\(\\ \frac { 2 }{ 3 } \) = \(\\ \frac { 2\times 4 }{ 3\times 4 } \) = \(\\ \frac { 8 }{ 12 } \)
\(\\ \frac { 3 }{ 4 } \) = \(\\ \frac { 3\times 3 }{ 4\times 3 } \) = \(\\ \frac { 9 }{ 12 } \)
\(\frac { 9 }{ 12 } >\frac { 8 }{ 12 } \)
=> \(\frac { 3 }{ 4 } >\frac { 2 }{ 3 } \)
∴ Rohit exercised more time

Question 30.
Solution:
In VI A, 20 student passed out of 25 or \(\\ \frac { 20 }{ 25 } \) or \(\\ \frac { 4 }{ 5 } \) students passed
But in VI B, 24 out of 30 passed 24 or \(\\ \frac { 24 }{ 30 } \) or \(\\ \frac { 4 }{ 5 } \) students passed
Now \(\\ \frac { 4 }{ 5 } \) = \(\\ \frac { 4 }{ 5 } \)
∴ Both sections gave same result

Hope given RS Aggarwal Solutions Class 6 Chapter 5 Fractions Ex 5D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 6 Solutions Chapter 3 Whole Numbers Ex 3C

These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3C.

Other Exercises

• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3A
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3B
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3C
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3D
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3E
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3F

Question 1.
Solution:

Question 2.
Solution:

Question 3.
Solution:
(i) 463 – 9
= 464 – 1 – 9
= 464 – 10
= 454
(ii) 5632 – 99
= 5632 – 100 + 1
= 5633 – 100
= 5533
(iii) 8640 – 999
= 8640 – 1000 + 1
= 8641 – 1000
= 7641
(iv) 13006 – 9999
= 13006 – 10000 + 1
= 13007 – 10000
= 12007

Question 4.
Solution:
Smallest number of 7-digits = 1000000
Largest number of 4-digits = 9999
Required difference = (1000000 – 9999)
= 990001

Question 5.
Solution:
Deposit in the beginning = Rs. 136000
Next day withdrew = Rs. 73129
Amount left in the bank account = Rs. 136000 – 73129
= Rs. 62871

Question 6.
Solution:
Amount withdrawn from the bank = Rs. 1,00,000
Cost of TV set = Rs. 38750
Cost of refrigerator = Rs. 23890
Cost of jewellery = Rs. 35560
Total amount spent on her purchase = Rs. (38750 + 23890 + 35560)
= Rs. 98200
Amount left with her – Rs. 1,00,000 – Rs. 98200
= Rs. 1800

Question 7.
Solution:
Population of a town = 110500
Increase in 1 year = 3608
Persons left or died = 8973
The population at the end of year = 110500 + 3608 – 8973
= 114108 – 8973
= 105135

Question 8.
Solution:
(i) We have n + 4 = 9
n = 9 – 4 = 5
n = 5
(ii) n + 35 = 101
Subtracting 35 from both sides
n + 35 – 35 = 101 – 35
=> n = 66
n = 66
(iii) n – 18 = 39
Adding 18 to both sides
n – 18 + 18 = 39 + 18
=> n = 57
n = 57
(iv) n – 20568 = 21403 Adding 20568 to both sides
n – 20568 + 20568 = 21403 + 20568
n = 41971

Hope given RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 6 Solutions Chapter 3 Whole Numbers Ex 3B

These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3B.

Other Exercises

• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3A
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3B
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3C
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3D
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3E
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3F

Question 1.
Solution:
(i) 458 + 639 = 639 + 458 (Commulative law)
(ii) 864 + 2006 = 2006 + 864 (Commulative law)
(iii) 1946 + 984 = 984 + 1946 (Commulative law)
(iv) 8063 + 0 = 8063 (Additive property of zero)
(v) 53501 + (574 + 799) = 574 + (53501 + 799) (Associative law)

Question 2.
Solution:
(i) 16509 + 114 = 16623
Check : 16623 – 114 = 16509 which is given.
(ii) 2359 + 548 = 2907
Check: 2907 – 2359 = 548 which is given
(iii) 19753 + 2867 = 22620
Check : 22620 – 19753 = 2867 which is given

Question 3.
Solution:
(1546 + 498) + 3589
= 2044 + 3589
= 5633
and 1546 + (498 + 3589)
= 1546 + 4087
= 5633
Yes, the above two sum are equal.
The property used is associative law of addition.

Question 4.
Solution:
(i) 953 + 707 + 647
= (953 + 647) + 707
(by associative law)
= 1600 + 707
= 2307
(ii) 1983 + 647 + 217 + 353
= (1983 + 217) + (647 + 353)
= 2200 + 1000 = 3200
(iii) 15409 + 278 + 691 + 422
= (15409 + 691) + (278 + 422)
(by associative law)
= 16100 + 700
= 16800
(iv) 3259 + 10001 + 2641 + 9999
= (3259 + 2641) + (10001 + 9999)
(by associative law)
= 5900 + 20000
= 25900
(v) 1 +2 + 3 +4 + 96 + 97 + 98 + 99
= (1 +99) + (2 + 98) + (3 + 97) + (4 + 96)
= (100+ 100) + (100 +100)
= 200 + 200
= 400
(vi) 2 + 3 + 4 + 5 + 45 + 46 + 47 + 48
= (2 + 48) + (3 + 47) + (4 + 46) + (5 + 45)
= (50 + 50) + (50 + 50)
= 100 + 100 = 209

Question 5.
Solution:
(i) 6784 + 9999 = (6784 – 1) + (9999 + 1)
(Adding and subtracting 1)
= 6783 + 10000
= 16783
(ii) 10578 + 99999
(Adding and subtracting 1)
= (10578 – 1) + (99999 + 1)
= 10577 + 100000
= 110577

Question 6.
Solution:
Yes it is true, by the property of associative law of addition.

Question 7.
Solution:
The magic squares given are completed as under:


In each row/column, the sum = 46

Question 8.
Solution:
(i) The sum of two odd numbers is an odd number (F)
As sum of two odd numbers is alway an even number
(ii) The sum of two even number is an even number (T)
(iii) The sum of an even number and an odd number is an odd number (T)

Hope given RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 6 Solutions Chapter 3 Whole Numbers Ex 3A

These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3A.

Other Exercises

• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3A
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3B
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3C
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3D
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3E
• RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3F

Question 1.
Solution:
After 30999, three whole numbers will be
30999 + 1 = 31000
31000 + 1 = 31001
31001 + 1 = 31002
i.e., 31000, 31001 and 31002

Question 2.
Solution:
Before 10001, three whole numbers will be 10001 – 1 = 10000
10000 – 1 = 9999
9999 – 1 = 9998
i.e., 10000, 9999, 9998

Question 3.
Solution:
Between 1032 and 1209, whole number are 1209 – 1031
= 178

Question 4.
Solution:
The smallest whole number is 0

Question 5.
Solution:
The successor of
(i) 2540801 is 2540801 + 1 = 2540802
(ii) 9999 is 9999 + 1 = 10000
(iii) 50904 is 50904 + 1 = 50905
(iv) 61639 is 61639 + 1 = 61640
(v) 687890 is 687890 + 1 = 687891
(vi) 5386700 is 5386700 + 1 = 5386701
(vii) 6475999 is 6475999 + 1 = 6476000
(viii) 9999999 is 9999999 + 1 = 10000000

Question 6.
Solution:
Predecessor of
(i) 97 is 97 – 1 = 96
(ii) 10000 is 10000 – 1 = 9999
(iii) 36900 is 36900 – 1 = 36899
(iv) 7684320 is 7684320 – 1 = 7684319
(v) 1566391 is 1566391 – 1 = 1566390
(vi) 2456800 is 2456800 – 1 = 2456799
(vii) 100000 is 100000 – 1 = 99999
(viii) 1000000 is 1000000 – 1 = 999999

Question 7.
Solution:
Three consecutive whole numbers just preceding 7510001 are (7510001 – 1), (7510001 – 2), (7510001 – 3)
i.e. 7510000, 7509999, 7509998.

Question 8.
Solution:
(i) Zero is not a natural number. (F)
(ii) Zero is the smallest whole number (T)
(iii) No, it is false, as zero is not a natural number but it is a whole number.
(iv) Yes, it is true, as set of natural numbers is a subset of whole numbers.
(v) False, zero is the smallest whole number.
(vi) The natural number 1 has no predecessor as 0 is the predecessor of 1 (T)
Which is not a natural number
(vii) The whole number 1 has no predecessor (F)
Predecessor of 1 is 0 which is a whole number
(viii) The whole number 0 has no predecessor (T)
(ix) The predecessor of a two-digit number is never a single-digit number (F)
As predecessor of two digit number say 99 is 99 – 1 = 98
Which is also a two-digit number and of 10 is 10 – 1 = 9
which is single-digit number
(x) The successor of a two-digit number is always a two-digit number (F)
The successor of a two-digit number 99 is 99 + 1 = 100 which is a three digit number
(xi) 500 is the predecessor of 499 (F)
As predecessor of 499 is 499 – 1 = 498 not 500 as 500 is the successor of 499
(xii) 7000 is the successor of 6999 (T)

 

Hope given RS Aggarwal Solutions Class 6 Chapter 3 Whole Numbers Ex 3A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

RS Aggarwal Class 6 Solutions Chapter 2 Factors and Multiples Ex 2F

These Solutions are part of RS Aggarwal Solutions Class 6. Here we have given RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2F.

Other Exercises

• RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2A
• RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2B
• RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2C
• RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2D
• RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2E
• RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2F

Objective Questions
Tick the correct answer in each of the following :

Question 1.
Solution:
(c) Because sum of its digits is 8 + 3 + 4 + 7 + 9 + 5 + 6 + 0 = 42 which is divisible by 3.

Question 2.
Solution:
(a) Because sum of its digits is 8 + 5 + 7 + 6 + 9 + 0 + 1 = 36 which is divisible by 9.

Question 3.
Solution:
(d) Because the number formed by tens and ones digits is divisible by 4 i.e. 32 ÷ 4 = 8.

Question 4.
Solution:
(b) Because the number formed by hundred, tens and ones digits is divisible by 8 i.e. 176 ÷ 8 = 22.

Question 5.
Solution:
(a) Because its one digit is divisible by 2 and sum of its digits is 8 + 7 + 9 + 0 + 4 + 3 + 2 = 33,
which is divisible by 3. Hence it is divisible by 6.

Question 6.
Solution:
(c) Because the difference of the sums of its odd places digits and of its even places digits is (2 + 2 + 2 + 2) – (2 + 2 + 2 + 2) i.e. 8 – 8 = 0, which is zero and is divisible by 11.

Question 7.
Solution:
(d) Because 97 has no factors other than 1 and itself.

Question 8.
Solution:
(c) Because 179 has no factors other than 1 and itself.

Question 9.
Solution:
(c) Because 263 has no factors other than 1 and itself.

Question 10.
Solution:
(a), (b) Because the common factors of 9 and 10 are none but 1.

Question 11.
Solution:
(c) Because 32 has factors which are 2, 2, 2, 2, 2.

Question 12.
Solution:
(d) Because 18 is the highest common factor of 144 and 198.

Question 13.
Solution:
(a) Because 12 is the highest common factors of these numbers 144, 180 and 192.

Question 14.
Solution:
(b) Because 161 and 192 have no common factor other than 1, i.e., HCF of 161 and 192 is 1.

Question 15.
Solution:
(d) Because HCF of 289 and 391 is 289
and \(\frac { 289\div 17 }{ 391\div 17 } \) = \(\\ \frac { 17 }{ 23 } \)

Question 16.
Solution:
(d) Because dividing 134 and 167 by 33 remainder is 2 in each case.

Question 17.
Solution:
(c) Because 360 is the least multiple of 24, 36 and 40.

Question 18.
Solution:
(d) Because 540 is the least multiple of 12, 15, 20 and 27

Question 19.
Solution:
(c) Because 1263 – 3 = 1260 is divisible by 14, 28, 36 and 45.

Question 20.
Solution:
(c) Because HCF of two co-prime number is always 1.

Question 21.
Solution:
(c) Because HCF of a and b, two co-primes is 1.
LCM = a x b = ab.

Question 22.
Solution:
(c) Because LCM of two numbers = Product of these number ÷ their HCF i.e 2160 ÷ 12 = 180.

Question 23.
Solution:
(b) Because second number
= \( \frac { LCM\times HCF }{ 1st\quad number } \)
i.e., \(\\ \frac { 145\times 2175 }{ 725 } \)
= 435

Question 24.
Solution:
(c) Because LCM of 15, 20, 24. 32 and 36 = 1440.

Question 25.
Solution:
(d) Because LCM of 9, 12, 15 is 180. 180
180 minutes = \(\\ \frac { 180 }{ 60 } \)
= 3 hours.

Hope given RS Aggarwal Solutions Class 6 Chapter 2 Factors and Multiples Ex 2F are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.