Tag: Selina ICSE Solutions for Class 10 Maths

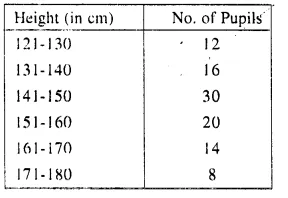
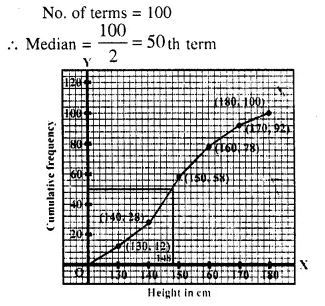
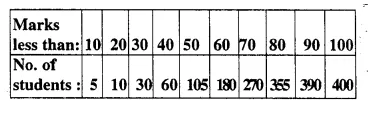
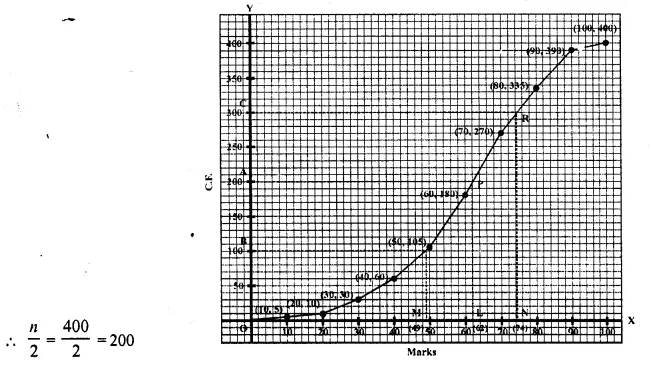
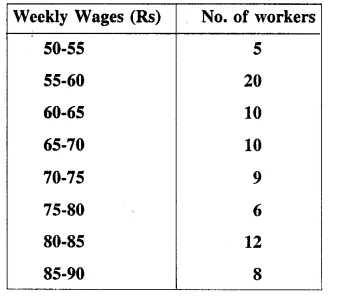
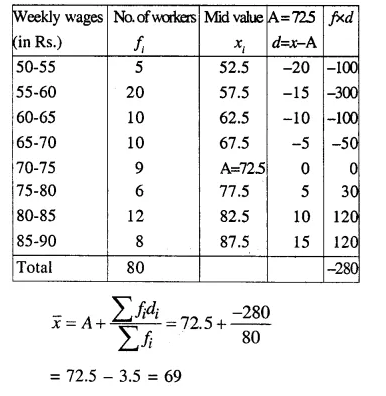
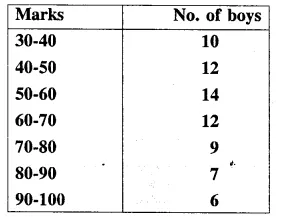
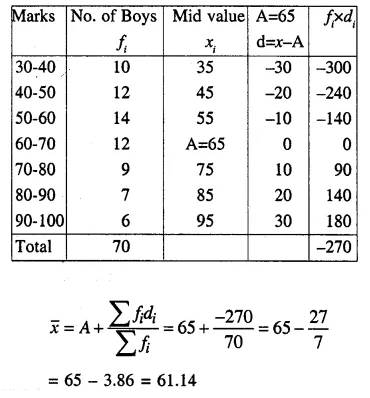
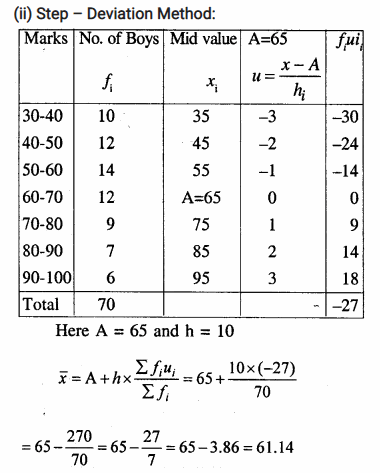
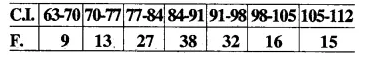
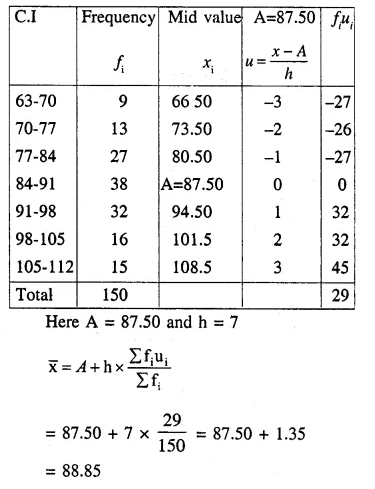
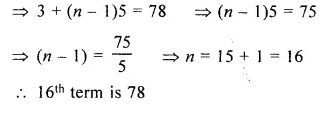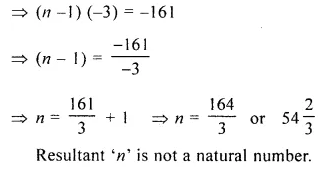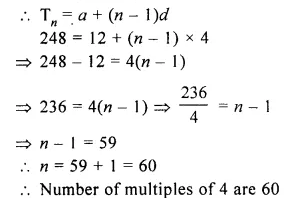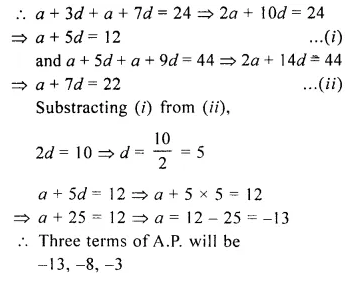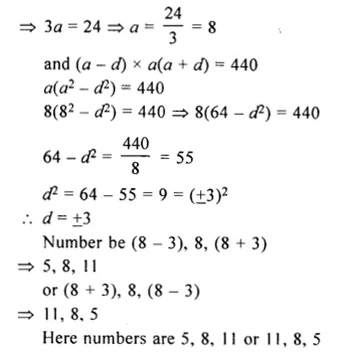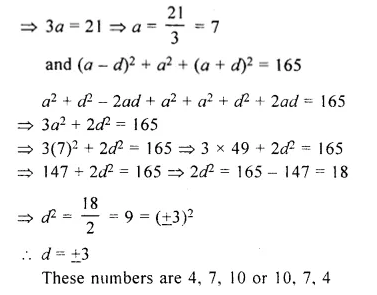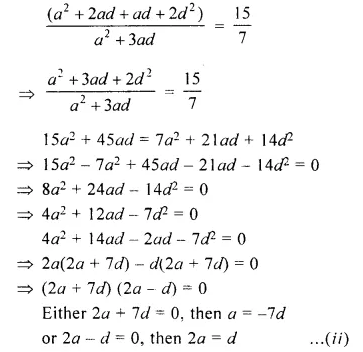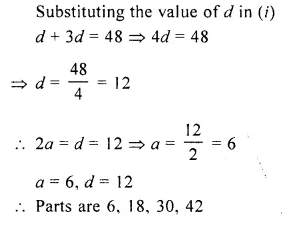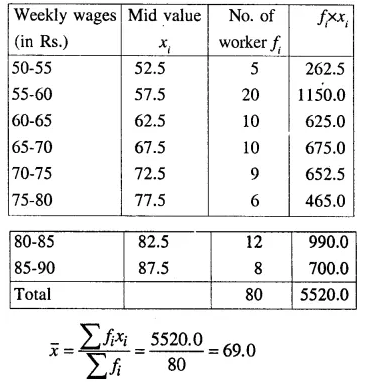Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11A

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11A

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Additional Questions

Question 1.
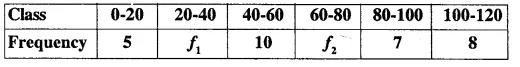
Find, which of the following sequence form a G.P. :
(i) 8, 24, 72, 216, ……
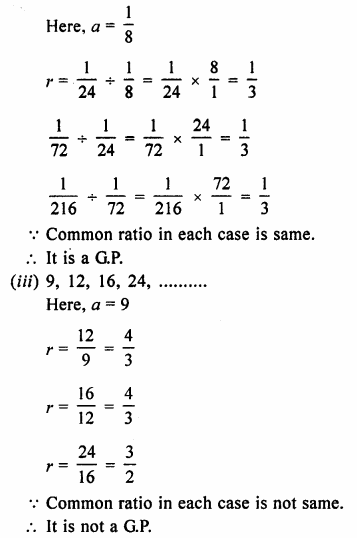
(ii) \(\\ \frac { 1 }{ 8 } \),\(\\ \frac { 1 }{ 24 } \),\(\\ \frac { 1 }{ 72 } \),\(\\ \frac { 1 }{ 216 } \)
(iii) 9, 12, 16, 24,…..
Solution:
(i) 8, 24, 72, 216,……
Here, a = 8

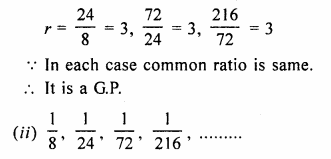
Question 2.
Find the 9th term of the series :
1, 4, 16, 64,…….
Solution:
In G.P. 1, 4, 16, 64,….
Here first term (a) = 1
and common ratio (r) = \(\\ \frac { 4 }{ 1 } \) = 4,
T₉ = ar^{n – 1} = 1 x 4^{9 – 1} = 1 x 4⁸ = 4⁸
= 4 x 4 x 4 x 4 x 4 x 4 x 4 x 4
= 65536

Question 3.
Find the seventh term of the G.P. :
1 , √3, 3, 3√3…….
Solution:
G.P. is 1 , √3, 3, 3√3
Here first term (a) = 1

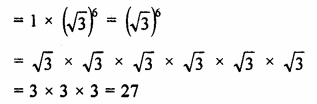
Question 4.
Find the 8th term of the sequence :
\(\\ \frac { 3 }{ 4 } \),\(1 \frac { 1 }{ 2 } \),3……
Solution:
G.P. = \(\\ \frac { 3 }{ 4 } \),\(1 \frac { 1 }{ 2 } \),3…….
= \(\\ \frac { 3 }{ 4 } \),\(\\ \frac { 3 }{ 2 } \),3…….

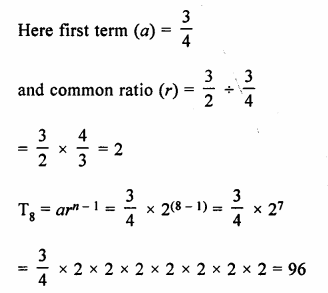
Question 5.
Find the 10th term of the G.P. :
12, 4, \(1 \frac { 1 }{ 3 } \),……
Solution:
G.P. = 12, 4, \(1 \frac { 1 }{ 3 } \),……..
= 12, 4, \(\\ \frac { 4 }{ 3 } \),…..

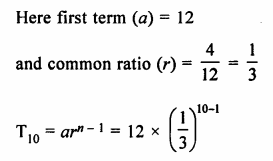
Question 6.
Find the nth term of the series :
1, 2, 4, 8 …….
Solution:
1, 2, 4, 8,……
Here, a = 1,r = \(\\ \frac { 2 }{ 1 } \) = 2

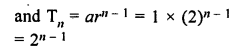
Question 7.
Find the next three terms of the sequence :
√5, 5, 5√5…..
Solution:
√5, 5, 5√5……
Here a = √5 and r = \(\frac { 5 }{ \surd 5 }\) = √5

Question 8.
Find the sixth term of the series :
2², 2³, 2⁴,….
Solution:
2², 2³, 2⁴,……
Here, a = 2², r = 2³ ÷ 2² = 2^{3 – 2} = 2¹ = 2

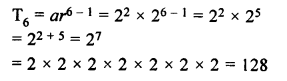
Question 9.
Find the seventh term of the G.P. :
√3 + 1, 1, \(\frac { \surd 3-1 }{ 2 } \),…….
Solution:
√3 + 1, 1, \(\frac { \surd 3-1 }{ 2 } \),…….

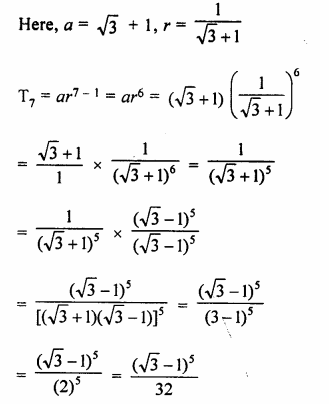
Question 10.
Find the G.P. whose first term is 64 and next term is 32.
Solution:
First term of a G.P. (a) = 64
and second term (ar) = 32
G.P. will be 64, 32, 16, 8, 4, 2, 1,…….

Question 11.
Find the next three terms of the series:
\(\\ \frac { 2 }{ 27 } \),\(\\ \frac { 2 }{ 9 } \),\(\\ \frac { 2 }{ 3 } \),…..
Solution:
G.P. is \(\\ \frac { 2 }{ 27 } \),\(\\ \frac { 2 }{ 9 } \),\(\\ \frac { 2 }{ 3 } \),…..
a = \(\\ \frac { 2 }{ 27 } \)

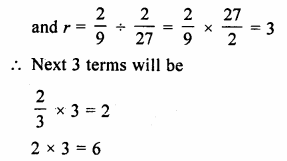
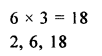
Question 12.
Find the next two terms of the series
2 – 6 + 18 – 54…..
Solution:
G.P. is 2 – 6 + 18 – 54 +………
Here a = 2 and r = \(\\ \frac { -6 }{ 2 } \) = – 3
Next two terms will be
– 54 x ( – 3) = + 162
162 x ( – 3) = – 486
Next two terms are 162 – 486

 

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems (Based on Quadratic Equations) Ex 6D

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6D.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6E

Question 1.
The sum S of n successive odd numbers starting from 3 is given by the relation :
S = n (n + 2). Determine n, if the sum is 168.
Solution:
S = n (n + 2) and S = 168
⇒ n (n + 2) = 168
⇒ n² + 2n – 168 = 0
⇒ n² + 14n – 12n – 168 = 0
⇒ n (n + 14) – 12 (n + 14) = 0
⇒ (n + 14) (n – 12) = 0
Either n + 14 = 0, then n = -14 which is not possible as n is positive.
or n – 12 = 0, then n = 12
Hence n = 12

Question 2.
A stone is thrown vertically downwards and the formula d = 16t² + 4t gives the distance, d metres, that it falls in t seconds. How long does it take to fall 420 metres ?
Solution:
d = 16t² + 4t, d = 420 m
Distance = 420 m.
6t² + 4t = 420
⇒ 16t² + 4t – 420 = 0
⇒ 4t² + t – 105= 0 (Dividing by 4)
⇒ 4t² + 21t – 20t – 105 = 0
⇒ t (4t + 21) – 5 (4t + 21) = 0
⇒ (4t + 21) (t – 5) = 0
Either 4t + 21 = 0, then 4t = -21 ⇒ t = \(\frac { -21 }{ 4 }\)
But it is not possible as time can not be negative.
or t – 5 = 0 , then t = 5
t = 5 seconds

Question 3.
The product of the digits of two digit number is 24. If its unit’s digit exceeds twice its ten’s digit by 2; find the number.
Solution:
Let ten’s digit = x
then unit’s digit = 2x + 2
According to the condition,
x (2x + 2) = 24
⇒ 2x² + 2x – 24 = 0
⇒ x² + x – 12 = 0 (Dividing by 2)
⇒ x² + 4x – 3x – 12 = 0
⇒ x (x + 4) – 3 (x + 4) = 0
⇒ (x + 4) (x – 3) = 0
Either x + 4 = 0, then x = – 4, which is not possible.
x – 3 = 0, then x = 3.
Ten’s digit = 3
and unit’s digit = 3 x 2 + 2 = 6 + 2 = 8
Number = 8 + 10 x 3 = 8 + 30 = 38

Question 4.
The ages of two sisters are 11 years and 14 years. In how many years time will the product of their ages be 304 ?
Solution:
Let the number of years = x
Age of first sister = 11 + x
and of second sister = 14 + x
Now according to the condition,
(11 + x) ( 14 + x) = 304
⇒ 154 + 11x + 14x + x² = 304
⇒ x² + 25x – 150 = 0
⇒ x² + 30x – 5x – 150 = 0
⇒ x (x + 30) – 5 (x + 30 ) = 0
⇒ (x + 30) (x – 5) = 0
Either x + 30 = 0 , then x = -30 But it is not possible as can’t be in negative
or x – 5 = 0 , then x = 5
Number of years = 5

Question 5.
One year ago, a man was 8 times as old as his son. Now his age is equal to the square of his son’s age. Find their present ages.
Solution:
One year ago, let the age of son = x years
and age of his father = 8x.
But present age of father is = (8x + 1) years
8x + 1 = (x + 1)²
⇒ x² + 2x + 1 = 8x + 1
⇒ x² + 2x + 1 – 8x – 1 = 0
⇒ x² – 6x = 0
⇒ x (x – 6) = 0
Either x = 0, which is not possible.
or x – 6 = 0, then x = 6
Present age of father = 8x + 1 = 8 x 6 + 1 = 48 + 1 = 49 years.
and age of son = x + 1 = 6 + 1 = 7 years

Question 6.
The age of a father is twice the square of the age of his son. Eight years hence, the age of the father will be 4 years more than three times the age of the son. Find their present ages.
Solution:
Let age of son = x
Then age of father will be = 2x²
8 years hence,
age of son = x + 8
and age of father = 2x² + 8
According to the condition,
2x² + 8 = 3 (x + 8) + 4
⇒ 2x² + 8 = 3x + 24 + 4
⇒ 2x² + 8 – 3x – 28 = 0
⇒ 2x² – 3x – 20 = 0
⇒ 2x² – 8x + 5x – 20 = 0
⇒ 2x (x – 4) + 5 (x – 4) = 0
⇒ (x – 4) (2x + 5) = 0
Either x – 4 = 0, then x = 4
or 2x + 5 = 0, then 2x – 5 ⇒ x = \(\frac { -5 }{ 2 }\)
Which is not possible being negative
x = 4
Present age of son = 4 years
and age of father = 2x² = 2 (4)² = 2 x 16 = 32 years

Question 7.
The speed of a boat in still water is 15 km/hr. It can go 30 km upstream and return down-stream to the original point in 4 hours 30 minutes, find the speed of the stream.
Solution:
Let the speed of stream = x km/hr.
Distance = 30 km.
Speed of boat in still water = 15 km/hr.

⇒ 9x² – 2025 + 1800
⇒ 9x² – 225 = 0
⇒ x² – 25 = 0
⇒ (x)² – (5)² = 0
⇒ (x + 5) (x – 5) = 0
Either x + 5 = 0, then x = -5 which is not possible.
or x – 5 = 0, then x = 5
Speed of stream = 5 km/hr.

Question 8.
Mr. Mehra sends his servant to the market to buy oranges worth Rs. 15. The servant having eaten three oranges on the way, Mr. Mehra pays 25 paise per orange more than the market price. Taking x to be the number of oranges which Mr. Mehra receives, form a quadratic equation in x. Hence, find the value of x.
Solution:
No. of oranges received by Mr. Mehra = x
No. of oranges eaten by the servant = 3
Total no. of oranges bought = x + 3
Total cost = Rs. 15
Price of one orange = Rs. \(\frac { 15 }{ x + 3 }\)
Now according to the sum,

⇒ x² + 3x = 180
⇒ x² + 3x – 180 = 0
⇒ x² + 15x – 12x – 180 = 0
⇒ x (x + 15) – 12 (x + 15) = 0
⇒ (x + 15) (x – 12) = 0
Either x + 15 = 0, then x = – 15 which is not possible
or x – 12 = 0, then x = 12
x = 12

Question 9.
Rs. 250 is divided equally among a certain number of children. If there were 25 children more, each would have received 50 paise less. Find the number of children.
Solution:
Let the number of children = x
Amount to be divided = Rs. 250

⇒ 6250 x 2 = x² + 25x
⇒ x² + 25x – 12500 = 0
⇒ x² + 125x – 100x – 12500 = 0
⇒ x (x + 125) – 100 (x + 125) = 0
⇒ (x + 125) (x – 100) = 0
Either x + 125 = 0 then x = -125 which is not possible.
or x – 100 = 0, then x = 100
No. of children = 100

Question 10.
An employer finds that if he increases the weekly wages of each worker by Rs. 5 and employs five workers less, he increases his weekly wage bill from Rs. 3,150 to Rs. 3,250. Taking the original weekly wage of each worker as Rs. x; obtain an equation in* and then solve it to find the weekly wages of each worker.
Solution:
In first case,
Let weekly wages of each employee = Rs. x
and number of employees = y
and weekly wages = 3150
xy = 3150 ⇒ y = \(\frac { 3150 }{ x }\) ….(i)
In second case,
Weekly wages = x + 5
and number of employees = y – 5
and weekly wages = 3250
(x + 5) (y – 5) = 3250
⇒ xy + 5y – 5x – 25 = 3200

⇒x (x + 70) – 45 (x + 70) = 0
⇒ (x + 70) (x – 45) = 0
Either x + 70 = 0, then x = -70 which is not possible being negative
or x – 45 = 0, then x = 45
Weekly wages per worker = Rs. 45

Question 11.
A trader bought a number of articles for Rs. 1,200. Ten were damaged and he sold each of the remaining articles at Rs. 2 more than what he paid for it, thus getting a profit of Rs. 60 on the whole transaction. Taking the number of articles he bought as x, form an equation in x and solve it.
Solution:
Let number of articles = x
C.P. = Rs. 1200
Profit = Rs. 60
S.P. = Rs. 1200 + 60 = Rs. 1260
No. of articles damaged = 10
Remaining articles = x – 10

⇒ 2x² – 80x – 12000 = 0
⇒ x² – 40x – 6000 = 0 (Dividing by 2)
⇒ x² – 100x + 60x – 6000 = 0
⇒ x (x – 100) + 60 (x – 100) = 0
⇒ (x – 100) (x + 60) = 0
Either x – 100 = 0, then x = 100
or x + 60 = 0, then x = – 60 which is not possible.
Number of articles = 100

Question 12.
The total cost price of a certain number of identical articles is Rs. 4,800. By selling the article at Rs. 100 each, a profit equal to the cost price of 15 articles is made. Find the number of articles bought.
Solution:
Total cost of some articles = Rs. 4800
Let number of articles = x
S.P. of one article = Rs. 100
S.P. of x articles = Rs. 100x
Profit = Cost price of 15 articles

⇒ x² = 48x + 720 (Dividing by 100)
⇒ x² – 48x – 720 = 0
⇒ x² – 60x + 12x – 720 = 0
⇒ x (x – 60) + 12 (x – 60) = 0
⇒ (x – 60) (x + 12) = 0
Either x – 60 = 0, then x = 60
or x + 12 = 0, then x = -12 Which is not possible.
x = 60
Number of articles = 60

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10F

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10F

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10E
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10F

Question 1.
The 6th term of an A.P. is 16 and the 14th term is 32. Determine the 36th term.
Solution:
Let the first term and common difference of an A.P. be a and d
As, we know that,

Question 2.
If the third and the 9th terms of an A.P. term is 12 and the last term is 106. Find the 29th term of the A.P.
Solution:
Let the first term and common difference of an A.P. be a and d.
As, we know that,
a_n = a + (n – 1 )d
a₃ = a + (3 – 1 )d = a + 2d
Similarly,

Question 3.
An A.P. consists of 50 terms of which 3rd term is 12 and the last term is 106. Find the 29th term of the A.P.
Solution:
Number of terms in an A.P. = 50
T₃= 12, l = 106
To find T₂₉
Let a be the first term and d be the common difference
=> a + 2d = 12 …(i)

Question 4.
Find the arithmetic mean of :
(i) – 5 and 41
(ii) 3x – 2y and 3x + 2y
(iii) (m + n)² and (m – n)²
Solution:
(i) Arithmetic mean between – 5 and 41
= \(\\ \frac { -5+41 }{ 2 } \)
= \(\\ \frac { 36 }{ 2 } \)
= 18

Question 5.
Find the sum of first 10 terms of the A.P. 4 + 6 + 8 +…..
Solution:
A.P. = 4 + 6 + 8 +…….
Here, a = 4, d = 6 – 4 = 2, n = 10

Question 6.
Find the sum of first 20 terms of an A.P. whose first term is 3 and the last term is 60.
Solution:
Sum of first 20 terms of an A.P. in which
a = 3 and a₂₀ = 60
a₂₀ = a + (20 – 1) x d
60 = 3 + 19 x d
19d = 60 – 3
d = \(\\ \frac { 57 }{ 19 } \)
= 3

Question 7.
How many terms of the series 18 + 15 + 12 +…..when added together will give 45 ?
Solution:
A.P. is 18 + 15 + 12 +…..
Here, a = 18, d = 15 – 18 = – 3
Given : S_n = 45

Question 8.
The nth term of a sequence is 8 – 5n. Show that the sequence is an A.P.
Solution:
Given, a_n = 8 – Sn
a₁ = 8 – 5 x (1) = 8 – 5 = 3
a₂ = 8 – 5 x (2) = 8 – 10 = – 2
a₃ = 8 – 5 x (3) = 8 – 15 = – 7
We see that

Question 9.
The the general term (nth term) and 23rd term of the sequence 3, 1, – 1, – 3,……
Solution:
The progression 3, 1, – 1, – 3,…..is A.P.
with first term (a) = 3 and common difference (d) = 1 – 3 = – 2

Question 10.
Which term of the sequence 3, 8, 13,…..is 78 ?
Solution:
Let 78 be the nth term
a = 3, d = 8 – 3 = 5, a_n = 78, n = ?
a + (n – 1)d = a_n

Question 11.
Is – 150 a term of 11, 8, 5, 2,….. ?
Solution:
11, 8, 5, 2,….1st term, a = 11
Common difference, d = 8 – 11 = – 3
a_n = – 150
=> a + (n – 1 )d = – 150
=> 11 + (n – 1) ( – 3) = – 150

Question 12.
How many two digit numbers are divisible by 3 ?
Solution:
Numbers divisible by 3 are 3, 6, 9, 12,….
Hence, lowest two digit number divisible by 3 = 12
and highest two digit number divisible by 3 = 99

Question 13.
How many multiples of 4 lie between 10 and 250 ?
Solution:
Multiples of 4 between 10 and 250 are
12, 16, 20, 24,……, 248
Here, a = 12, d = 4, l = 248

Question 14.
The sum of the 4th term and the 8th term of an A.P. is 24 and the sum of 6th term and the 10th term is 44. Find the first three terms of the A.P.
Solution:
In an A.P.
T₄ + T₈ = 24
T₆ + T₁₀ = 44
Let a be the first term and d be the common difference

Question 15.
The sum of first 14 terms of an A.P. is 1050 and its 14th term is 140. Find the 20th term.
Solution:
Given a₁₄ = 140
we know, a_n = a + (n – 1) x d

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10F are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems (Based on Quadratic Equations) Ex 6C

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6C.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6E

Question 1.
The speed of an ordinary train is x km/hr and that of an express train is (x + 25) km per hour.
(i) Find the time taken by each train to cover 300 km.
(ii) If the ordinary train takes 2 hrs more than the express train; calculate speed of the express train.
Solution:
Speed of an ordinary train = x km/ hr
and speed of an express train = (x + 25) km/hr.
(i) Time taken by ordinary train to cover 300 km = \(\frac { 300 }{ x }\) hr
(ii) Time taken by express train to cover 300 km = \(\frac { 300 }{ x + 25 }\) hr

⇒ 7500 = 2x² + 50x
⇒ 2x² + 50x – 7500 = 0
⇒ x² + 25x – 3750 = 0 (Dividing by 2)
⇒ x² + 75x – 50x – 3750 = 0
⇒ x (x + 75) -50 (x + 75) = 0
⇒ (x + 75) (x – 50) = 0
Either x + 75 = 0 then x = -75 But it is not possible,
or x – 50 = 0 then x = 50
Speed of an ordinary train = 50 km/ hr
and speed of express train = 50 + 25 = 75 km/ hr

Question 2.
If the speed of a car is increased by 10 km per hr, it takes 18 minutes less to cover a distance of 36 km. Find the speed of the car.
Solution:
Let the speed of ear = x km/hr
time taken to cover 36 km = \(\frac { 36 }{ x }\) hr
In second case,
Speed of car = (x + 10) km/hr

⇒ 3x² + 30 x = 3600
⇒ 3x² + 30x – 3600 = 0 .
⇒ x² + 10x – 1200 = 0 (Dividing by 3)
⇒ x² + 40x – 30x – 1200 = 0
⇒ x (x + 40) – 30 (x + 40) = 0
⇒ (x + 40) (x – 30) = 0
Either x + 40 = 0 then x = – 40 But it is not possible,
or x – 30 = 0, then x = 30
Speed of car = 30 km/hr.

Question 3.
If the speed of an aeroplane is reduced by 40 km/per hr, it takes 20 minutes more to cover 1200 km. Find the speed of the aeroplane.
Solution:
Let the speed of aeroplane = x km/hr.

⇒ x² – 40x = 144000
⇒ x² – 40x – 144000 = 0
⇒ x² – 400x + 360x – 144000 = 0
⇒ x (x – 400) + 360 (x – 400) = 0
Either x – 400 = 0, then x = 400
or x + 360 = 0, then x = – 360, But it is not possible.
x = 400
Hence speed of aeroplane = 400 km/hr.

Question 4.
A car covers a distance of 400 km at a certain speed. Had the speed been 12 km/h more, the time taken for the journey would have been 1 hour 40 minutes less. Find the original speed of the car.
Solution:
Let the original speed of the car be x km/h.

⇒ 1200x + 14400 – 1200x = 5x² + 60x
⇒ 14400 = 5x² + 60x
⇒ x² + 12x – 2880 = 0 (Dividing by 5)
⇒ x² + 60x – 48x – 2880 = 0
⇒ x (x + 60) – 48 (x + 60) = 0
⇒ (x + 60) (x – 48) = 0
⇒ x = 48 or x = -60
⇒ x = 48 (Rejecting x = -60, being speed)
Hence, original speed of the car = 48 km/h.

Question 5.
A girl goes to her friend’s house, which is at a distance of 12 km. She covers half of the distance at a speed of ‘x’ km/hr. and the remaining distance at a speed of (x + 2) km/hr. If she takes 2 hrs. 30 minutes to cover the whole distance; find ‘x’
Solution:
Distance = 12 km.
Speed for the first half distance = x km/hr.
and for the second half distance = (x + 2) km/hr.
Total time taken = 2 hrs. 30 minutes.

But it is not possible.
Speed for first half distance (x) = 4 km/hr

Question 6.
A car made a run of 390 km in ‘x’ hours. If the speed had been 4 km per hour more, it would have taken 2 hours less for the journey. Find ‘x’.
Solution:
Distance = 390 km
time = x hours

⇒ (x – 15) (x + 13) = 0
Either x – 15 = 0, then x = 15
or (x + 13) = 0 then x = -13 Which is not possible.
Value of x = 15

Question 7.
A goods train leaves a station at 6 p.m., followed by an express train which leaves at 8 p.m. and travels 20 km/hour faster than the goods train. The express train arrives at a station, 1040 km away, 36 minutes before the goods train. Assuming that the speeds of both the trains remain constant between the two stations; calculate their speeds.
Solution:
Departure of goods train = 6 p.m.
and departure of express train = 8 p.m.
Speed of express train is more than goods trains by 20 km/hr
Total distance = 1040 km
Let speed of goods train = x km/hr
Then speed of express train = (x + 20) km/hr
Difference of time taken = 8 p.m. – 6 p.m. + 36 minutes = 2 hours 36 minutes

⇒ x – (x + 100) – 80 (x + 100) = 0
⇒ (x + 100) (x – 80) = 0
Either x + 100 = 0, then x = -100 but it is not possible being negative
or x – 80 = 0, then x = 80
Speed of goods train = 80 km/hr
and speed of express train = 80 + 20 = 100 km/hr
⇒ 13x² + 507x – 35100 = 0
⇒ x² + 39x – 2700 = 0 (Dividing by 13)
⇒ x² + 75x – 36x – 2700 = 0
⇒ x (x + 75) – 36(x + 75) = 0
⇒ (x + 75) (x – 36) = 0
Either x + 75 = 0, then x = – 75 Which is not possible,
or x – 36 = 0 then x = 36
Speed of goods train = 36 km/hr
and speed of express train = 36 + 39 = 75 km/hr.

Question 8.
A man bought an article for Rs. x and sold it for Rs. 16. If his loss was x percent, find the cost price of the article.
Solution:
C.P. of article = Rs. x
S.R = Rs. 16
Loss = C.P. – S.P. = Rs. (x – 16)

⇒ x² – 100x + 1600 = 0
⇒ x² – 20x – 80x + 1600 = 0
⇒ x (x – 20) – 80 (x – 20) = 0
⇒ (x – 20) (x – 80) = 0
Either x – 20 = 0, then x = 20
or x – 80 = 0, then x = 80
Cost Price = Rs. 20 or Rs. 80

Question 9.
A trader bought an article for Rs. x and sold it for Rs. 52, thereby making a profit of (x – 10) per cent on his outlay. Calculate the cost price.
Solution:
Let C.P. = Rs. x
S.R = Rs. 52
Profit = S.P – C.P. = Rs. 52 – x

x² – 10x = 5200 – 100x
⇒ x² – 10x + 100x – 5200 = 0
⇒ x² + 90x – 5200 = 0
⇒ x2 + 130x – 40x – 5200 = 0
⇒ x (x + 130) – 40(x + 130) = 0
⇒ (x + 130) (x – 40 ) = 0
Either x + 130 = 0 , then x = – 130 which is not possible.
or x – 40 = 0 then x = 40
Cost price = Rs. 40

Question 10.
By selling a chair for Rs. 75, Mohan gained as much per cent as its cost. Calculate the cost of the chair.
Solution:
Let. C.P of chair = Rs. x
Profit = x %
S.P. = Rs. 75
Total profit = Rs. (75 – x)

⇒ x² = 7500 – 100x
⇒ x² + 100x – 7500 = 0
⇒ x²+ 150x – 50 – 7500 = 0
⇒ x (x + 150) – 50 (x + 150) = 0
⇒ (x + 150) (x – 50) = 0
Either x + 150 = 0, then x = -150 which is not possible
or x – 50 = 0, Then x = 50
Cost price of chair = Rs. 50

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10E

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10E

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10E
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10F

Question 1.
Two cars start together in the same direction from the same place. The first car goes at uniform speed of 10 km h^-1. The second car goes at a speed of 8 km h^-1 in the first hour and thereafter increasing the speed by 0.5 km h^-1 each succeeding hour. After how many hours will the two cars meet ?
Solution:
Speed of first car = 10 km/hr
Speed of second car = 8 km/hr in first hour

Question 2.
A sum of Rs 700 is to be paid to give seven cash prizes to the students of a school for their overall academic performance. If the cost of each prize is Rs 20 less than its preceding prize; find the value of each of the prizes.
Solution:
Total amount (S_n) = Rs 700
Cost of each prize is Rs 20 less than its preceding price or d = – 20
d = – 20 and n = 7
\({ S }_{ n }=\frac { n }{ 2 } \left[ 2a+(n-1)d \right] \)

Question 3.
An article can be bought by paying Rs 28,000 at once or by making 12 monthly installments. If the first installment paid is Rs 3,000 and every other installment is Rs 100 less than the previous one, find :
(i) amount of installment paid in the 9th month
(ii) total amount paid in the installment scheme.
Solution:
Total price of an article = Rs 28000
No. of installments (n) = 12
First installment (a) = RS 3000
d = Rs 100

Question 4.
A manufacturer of TV sets produces 600 units in the third year and 700 units in the 7th year. Assuming that the production increases uniformly by a fixed number every year, find :
(i) the production in the first year.
(ii) the production in the 10th year.
(iii) the total production in 7 years.
Solution:
A manufacture of TV sets, he produces
No. of units in 3rd year = 600
No. of units in 7th year = 700
Let a be the first term and d be the common difference, then

Question 5.
Mrs. Gupta repays her total loan of Rs 1.18,000 by paying installments every month. If the installment for the first month is Rs 1,000 and it increases by RS 100 every month, what amount will she pay as the 30th installment of loan? What amount of loan she still has to pay after the 30th installment?
Solution:
Total loan to be paid by Mrs. Gupta = Rs 118000
Installment for the first month (a) = Rs 1000

Question 6.
In a school, students decided to plant trees in and around the school to reduce air pollution. It was decided that the number of trees, that each section of each class will plant, will be five times of the class to which the respective section belongs. If there are 1 to 10 classes in the school and each class has three sections, find how many trees were planted by the students?
Solution:
Number of classes = 10
Number of sections of each class = 3
Total number of sections = 10 x 3 = 30
Each section plant tree = 5 times of the class
Each section of 1st class will plant = 1 x 15 = 15

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10E are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency (Mean, Median, Quartiles and Mode) Ex 24D

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Chapter 24 Measures of Central Tendency (Mean, Median, Quartiles and Mode) Ex 24D.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24E

Question 1.
Find the mode of the following data:
(i) 7,9,8,7,7,6,8,10,7 and 6
(ii) 9,11,8,11,16,9,11,5,3,11,17 and 8
Solution:
(i) Mode = 7
because it occurs 4 times
(ii) Mode =11
because it occurs 4 times

Question 2.
The following table shows the frequency distribution of heights of 50 boys:

Find the mode of heights.
Solution:
Mode is 122 because it occurs maximum times i.e its., frequency is 18.

Question 3.
Find the mode of following data, using a histogram:

Solution:
Mode class = 20 – 30
Mode = 24

We see in the histogram that line AD and CB intersect at P. Draw perpendicular Q to the horizontal x-axis. Which is the value of the mode = 24

Question 4.
The following table shows the expenditure of 60 boys on books. Find the mode of their expenditure:

Solution:
Model class is = 30 – 35
and Mode = 34

We see in the histogram that line AD and CB intersect at P. Draw perpendicular Q to the horizontal axis. Which is the value of the mode.

Question 5.
Find the median and mode for the set of numbers 2,2,3,5,5,5,6,8 and 9.
Solution:
Median = \(\frac { 9 +1 }{ 2 }\) = 5th term which is 5
Mode = 5, because it occurs in maximum times.

Question 6.
A boy scored following marks in various class tests during a term, each test being marked out of 20.
15,17,16,7,10,12,14,16,19,12,16.
(i) What are his modal marks ?
(ii) What are his median marks ?
(iii) What are his total marks ?
(iv) What are his mean marks ?
Solution:
Arranging the given data in ascending order : 7, 10,12, 12,14, 15,16,16, 16, 17,19.
(i) Mode = 16 as it occurs in maximum times.
(ii) Median= \(\frac { 11 +1 }{ 2 }\) = 6th term which is 15
(iii) Total marks = 7 + 10+ 12+ 12+ 14+ 15+ 16 + 16+ 16+ 17+ 19= 154

Question 7.
Find the mean, median and mode of the following marks obtained by 16 students in a class test marked out of 10 marks.
0,0,2,2,3,3,3,4,5,5,5,5,6, 6,7,8
Solution:

(ii) Median = Mean of 8th and 9th term
= \(\frac { 4 +5 }{ 2 }\) = \(\frac { 9 }{ 2 }\) = 4.5
(iii) Mode = 5 as it occurs in maximum times.

Question 8.
At a shooting competition the score of a com-petitor were as given below :

(i) What was his modal score ?
(ii) What was his median score ?
(iii) What was his total score ?
(iv) What was his mean score ?
Solution:

(i) Modal score =4 as its frequency is 7, the maximum.

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24D helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10D

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10D

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10E
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10F

Question 1.
Find three numbers in A.P. whose sum is 24 and whose product is 440.
Solution:
Let three numbers be a – d, a, a + d
a – d + a + a + d = 24

Question 2.
The sum of three consecutive terms of an A.P. is 21 and the slim of their squares is 165. Find these terms.
Solution:
Let three consecutive numbers in A.P. are
a – d, a, a + d
a – d + a + a + d = 21

Question 3.
The angles of a quadrilateral are in A.P. with common difference 20°. Find its angles.
Solution:
Let the angles of a quadrilateral are
a, a + d, a + 2d, a + 3d
d= 20°
a + a + d + a + 2d + a + 3d = 360°
(Sum of angles of a quadrilateral)
=> 4a + 6d = 360°
=> 4a + 6 x 20° = 360°
=> 4a + 120° = 360°
=> 4a = 360 – 120° = 240°
a = \(\\ \frac { 240 }{ 4 } \) = 60°
Angles are 60°, 80°, 100°, 120°

Question 4.
Divide 96 into four parts which are in A.P. and the ratio between product of their means to product of their extremes is 15 : 7.
Solution:
Number = 96
Let its four parts be a, a + d, a + 2d, a + 3d
a + a + d + a + 2d + a + 3d = 96
=> 4a + 6d = 96
=> 2a + 3d = 48 …(i)
Product of means : Product of extremes = 15 : 7

Question 5.
Find five numbers in A.P. whose sum is \(12 \frac { 1 }{ 2 } \) and the ratio of the first to the last terms is 2 : 3.
Solution:
Let 5 numbers in A.P. be
a, a + d, a + 2d, a + 2d, a + 4d
a + a + d + a + 2d + a + 3d + a + 4d =

Question 6.
Split 207 into three parts such that these parts are in A.P. and the product of the two smaller parts is 4623.
Solution:
Number = 207
Let part be a – d, a, a + d
a – d + a + a + d = 207

Question 7.
The sum of three numbers in A.P. is 15 the sum of the squares of the extreme is 58. Find the numbers
Solution:
Let three numbers in A.P. be a – d, a, a + d
a – d + a + a + d = 15
=> 3a = 15

Question 8.
Find four numbers in A.P. whose sum is 20 and the sum of whose squares is 120.
Solution:
Let four numbers in A.P. be
a – 3d, a – d, a + d, a + 3d
a – 3d + a – d + a + d + a + 3d = 20

Question 9.
Insert one arithmetic mean between 3 and 13.
Solution:
Let A be the arithmetic mean between 3 and 13
\(\left( A=\frac { a+b }{ 2 } \right) \)
A = \(\\ \frac { 3+13 }{ 2 } \)
= \(\\ \frac { 16 }{ 2 } \)
= 8

Question 10.
The angles of a polygon are in A.P. with common difference 5°. If the smallest angle is 120°, find the number of sides of the polygon.
Solution:
Angles of a polygon are in A.P.
and common difference (d) = 5°
Smallest angle (a) = 120°
Let n be the number of sides of the polygon then sum of angles = (2n – 4) x 90°
a = 120° and d = 5°

Question 11.
\(\\ \frac { 1 }{ a } \), \(\\ \frac { 1 }{ b } \) and \(\\ \frac { 1 }{ c } \) are in A.P
S.T : bc, ca and ab are also in A.P
Solution:
\(\\ \frac { 1 }{ a } \), \(\\ \frac { 1 }{ b } \) and \(\\ \frac { 1 }{ c } \) are in A.P
We have to show that

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems (Based on Quadratic Equations) Ex 6B

Question 1.
The sides of a right-angled triangle containing the right angle are 4x cm and (2x – 1) cm. If the area of the triangle is 30 cm²; calculate the lengths of its sides
Solution:


But -13 is not possible.
Sides are 5 cm, 12 cm and 13 cm.

Question 2.
The hypotenuse of a right-angled triangle is 26 cm and the sum of other two sides is 34 cm. Find the lengths of its sides.
Solution:

AC + BC = 34 cm
Let AC = x cm Then BC = (34 – x) cm
But AC² + BC² = AB² (By Pythagoras Theorem)
⇒ (x)² + (34 – x)² = (26)²
⇒ x² + 1156 + x² – 68x = 676
⇒ 2x² – 68x + 1156 – 676 = 0
⇒ 2x² – 68x + 480 = 0
⇒ x² – 34x + 240 = 0 (Dividing by 2)
⇒ x² – 24x – 10x + 240 = 0
⇒ x (x – 24) – 10 (x – 24) = 0
⇒ (x – 24) (x- 10) = 0
Either x – 24 = 0, then x = 24
or x – 10 = 0, then x = 10
If one side is 24 cm, then second will be 34 – 24 = 10 cm
If one side is 10 cm, then second side will be 34 – 10 = 24
Sides are 24 cm and 10 cm

Question 3.
The sides of a right-angled triangle are (x – 1) cm, 3x cm and (3x + 1) cm. Find :
(i) The value of x,
(ii) the lengths of its sides,
(iii) its area.
Solution:

AB = x – 1, BC = 3x and AC = 3x +1
According to Pythagoras Theorem,
AC² = AB² + BC²
⇒ (3x + 1)² = (x – 1)² + (3x)²
⇒ 9x² + 6x + 1 = x² – 2x + 1 + 9x²
⇒ 9x² + 6x + 1 – x² + 2x -1 – 9x² = 0
⇒ – x² + 8x = 0
⇒ x² – 8x = 0
⇒ x (x – 8) = 0
Either x = 0 but it is not possible.
or x – 8 = 0, then x = 8
(i) x = 8
(ii) Side AB = x – 1 = 8 – 1 = 7cm
BC = 3x = 3 x 8 = 24 cm
AC = 3x + 1 = 3 x 8 + 1 = 24 + 1 = 25 cm
(iii) Area = \(\frac { 1 }{ 2 }\) x AB x BC = \(\frac { 1 }{ 2 }\) x 7 x 24 cm² = 84 cm²

Question 4.
The hypotenuse of a right-angled triangle exceeds one side by 1 cm and the other side by 18 cm; find the lengths of the sides of the triangle.
Solution:

In right triangle ABC, ∠B = 90°
Let hypotenuse AC = x, then AB = x – 1 and BC = x – 18
Now, according to Pythagoras Theorem,
AC² = AB² + BC²
⇒ x² = (x – 1)² + (x – 18)²
⇒ x² = x² – 2x + 1 + x² – 36x + 324
⇒ x² = 2x² – 38x + 325
⇒ 2x² – 38x + 325 – x² = 0
⇒ x² – 38x + 325 = 0
⇒ x² – 13x – 25x + 325 =0
⇒ x (x – 13) – 25 (x – 13) = 0
⇒ (x – 13) (x – 25) = 0
Either x – 13 = 0, then x = 13 But it is not possible.
x – 18 = 13 – 18 = -5 cannot be possible.
or x – 25 = 0, then x = 25
AC = 25,
AB = x – 1 = 25 – 1 = 24
and BC = x – 18 = 25 – 18 = 7
Sides are 25, 24, 7

Question 5.
The diagonal of a rectangle is 60 m more than its shorter side and the larger side is 30 m more than the shorter side. Find the sides of the rectangle.
Solution:
Let shorter side of a rectangle (b) = x m
Longer side (l) = (x + 30) m
and length of diagonal = (x + 60) m
According to the condition,
(Diagonal)² = (Longer side)² + (Shorter side)² (Pythagoras Theorem)
⇒ (x + 60)² = (x + 30)² + x²
⇒ x² + 120x + 3600 = x² + 60x + 900 + x²
⇒ 2x² + 60x + 900 – x² – 120x – 3600 = 0
⇒ x² – 60x – 2700 = 0
⇒ x² – 90x + 30x – 2700 = 0 {-2700 = -90 x 30, -60 = -90 + 30}
⇒ x (x – 90) (x + 30) = 0
Either x – 90 = 0, then x = 90
or x + 30 = 0, then x = -30 but is not possible being negative
Length = x + 30 = 90 + 30 = 120 m
Breadth = x = 90 m

Question 6.
The perimeter of a rectangle is 104 m and its area is 640 m². Find its length and breadth.
Solution:

Perimeter = 104 m
⇒ 2 (l + b) = 104 m
l + b = 52 m
Let length of the rectangular plot = x m
Breadth = 52 – x
Area = l x b = x (52 – x)
But area of plot = 640 m²
x (52 – x) = 640
⇒ 52x – x² = 640
⇒ – x² + 52x – 640 = 0
⇒ x² – 52x + 640 = 0
⇒ x² – 20x – 32x + 640 = 0
⇒ x (x – 20) – 32 (x – 20) = 0
⇒ (x – 20) (x – 32) = 0
Either x – 20 = 0, then x = 20
or x – 32 = 0, then x = 32
Length = 32 m
and breadth = 52 – 32 = 20 m

Question 7.
A footpath of uniform width runs round the inside of a rectangular field 32 m long and 24 m wide. If the path occupies 208 m², find the width of the footpath.
Solution:

Length of field = 32m
and width = 24 m
Area of the field = 32 x 24 m² = 768m²
Area of path = 208 m²
Let width of path = x m
Inner length = 32 – 2x
and inner width = 24 – 2x
and inner area = (32 – 2x) (24 – 2x) m²
Area of path = 768 – (32 – 2x) (24 – 2x)
Now, according to the condition,
768 – (32 – 2x) (24 – 2x) = 208
⇒ 768 – (768 – 64x – 48x + 4x²) = 208
⇒ 768 – 768 + 64x + 48x – 4x² = 208
⇒ -4x² + 112x – 208 = 0
Dividing by -4 , we get:
⇒ x² – 28x + 52 = 0
⇒ x² – 26x – 2x + 52 = 0
⇒ x (x – 26) – 2 (x – 26) = 0
⇒ (x – 26 ) (x – 2) = 0
Either x – 26 = 0 , then x = 26 But it is not possible.
or x – 2 – 0 then x = 2
Width of path = 2 m

Question 8.
Two squares have sides x cm and (x + 4 ) cm. The sum of their areas is 656 sq. cm. Express this as an algebraic equation in x and solve the equation to find the sides of the squares.
Solution:
Side of first square = x cm
Area = x² cm²
Side of second square = (x + 4) cm
Area = (x + 4)² cm²
Sum of squares = 656 cm²
⇒ x² + (x + 4)² = 656
⇒ x² + x² + 8x + 16 – 656 = 0
⇒ 2x² + 8x – 640 = 0
⇒ x² + 4x- 320 = 0 (Dividing by 2)
⇒ x² + 20x – 16x – 320 = 0
⇒ x (x + 20) – 16 (x + 20) = 0
⇒ (x + 20) (x – 16) = 0
Either x + 20 = 0, then x = – 20 But it is not possible.
or x – 16 = 0, then x = 16
Side of first square = 16 cm
and side of second square = 16 + 4 = 20 cm

Question 9.
The dimensions of a rectangular field are 50 m by 40 m. A flower bed is prepared inside this field leaving a gravel path of uniform width all around the flower bed. The total cost of laying the flower bed and gravelling the path at Rs. 30 and Rs. 20 per square metre, respectively, is Rs. 52,000. Find the width of the gravel path.
Solution:

Length of the field (l) = 50 m
and width (b) = 40 m
Area of rectangular field = l x b = 50 x 40 = 2000 m²
Let width of gravel path be x
Then inner length = 50 – 2x
and width = 40 – 2x
Area of inner flower bed = (50 – 2x) (40 – 2x)
= 2000 – 80x – 100x + 4x² = 4x² – 180x + 2000 sq m
and Area of gravel path = 2000 – (4x² – 180x + 2000)
= 2000 – 4x² + 180x – 2000 = -4x² + 180x
Now rate of gravelling = Rs. 20 per m²
and rate of laying flowers = Rs. 30 per m²
and total cost = Rs. 52000
According to the condition,
(-4x² + 180x) x 20 + (4x² -180x + 2000) x 30 = 52000
-80x² + 3600x + 120x² – 5400x + 60000 = 52000
⇒ 40x² – 1800x – 60000 – 52000 = 0
⇒ 40x² – 1800x + 8000 = 0
⇒ x² – 45x + 200 = 0 (Dividing by 40)
⇒ x² – 40x – 5x + 200 = 0 (200= -40 x (- 5), -45 = -40 – 5)
⇒ x (x – 40) – 5 (x – 40) = 0
⇒ (x – 40) (x – 5) = 0
Either x – 40 = 0, then x = 40
or x – 5 = 0, then x = 5
But x = 40 is not possible
x = 5
Width of gravel path = 5 m

Question 10.
An area is paved with square tiles of a certain size and the number required is 128. If the tiles had been 2 cm smaller each way, 200 tiles would have been needed to pave the same area. Find the size of the larger tiles.
Solution:
No. of square tiles = 128
Let the size of square tile = x cm
Area of one square tile = x² cm²
Area of total tiles = 128 x x² = 128x² cm²
If the size of square tile is reduced by 2 cm
Then size of square tile = (x – 2) cm
Area of one tile = (x – 2)² cm²
Now number of tiles will be = 200
⇒ 128x² = 200 (x – 2)²
⇒128x² = 200x² – 800x + 800
⇒ 200x² – 800x + 800 – 128x² = 0
⇒ 72x² – 800x + 800 = 0
⇒ 9x² – 100x + 100 = 0 (Dividing by 8)
⇒ 9x² – 90x – 10x + 100 = 0
⇒ 9x (x – 10) – 10 (x – 10) = 0
⇒ (x – 10) (9x – 10) = 0
Either x- 10 = 0, then x = 10
or 9x – 10 = 0, then 9x = 10 ⇒ x = \(\frac { 10 }{ 9 }\)
Which is not possible x = 10
Size of square tile = 10 cm

Question 11.
A farmer has 70 m. of fencing, with which he encloses three sides of a rectangular sheep pen; the fourth side being a wall. If the area of the pen is 600 sq. m, find the length of its shorter side.
Solution:

Length of fencing = 70 m.
Area of rectangular pen = 600 sq. m
(i) Let the length of shorter side = x m
Length of larger side = 70 – 2x
Area of rectangular pen = x ( 70 – 2x) ….(ii)
From (i) and (ii)
x (70 – 2x) = 600
⇒ 70x – 2x² = 600
⇒ -2x² + 70x – 600 = 0
⇒ x² – 35x + 300 = 0 (Dividing by -2)
⇒ x² – 15x – 20x + 300 = 0
⇒ x (x – 15) – 20 (x – 15) = 0
⇒ (x – 15) (x – 20) = 0
Either x – 15 = 0 then x = 15
or x – 20 = 0 then x = 20
Shorter side = 15 m

Question 12.
A square lawn is bounded on three sides by a path 4 m wide. If the area of the path is \(\frac { 7 }{ 8 }\) that of the lawn, find the dimensions of the lawn.
Solution:

Let the side of square lawn = x m
Area of lawn = x² m²
Width of path = 4 m.
Area of path = 4 x x + 4 x x + (x + 8) x 4 = 8x + 4x + 32 = 12x + 32
But 12x + 32 = \(\frac { 7 }{ 8 }\) x2
⇒ 96x + 256 = 7x²
⇒ 7x² – 96x – 256 = 0
⇒ 7x² – 112x + 16x – 256 = 0
⇒ 7x (x – 16) + 16 (x – 16) = 0
⇒ (x – 16 ) (7x + 16) = 0
Either x – 16 = 0, then x = 16
0r 7x + 16 = 0, then 7x = -16 ⇒ x = \(\frac { -16 }{ 7 }\)
But it is not possible.
Side of square lawn = 16 m

Question 13.
The area of a big rectangular room is 300 m². If the length were decreased by 5m and the breadth increased by 5 m; the area would be unaltered. Find the length of the room.
Solution:
Area of big rectangular room = 300 m²
Let length of the room = x m.
Width = m
In second case,
Length = (x – 5) m
and width = (\(\frac { 300 }{ x }\) + 5) m

⇒ (x – 5) ( 300 + 5x) = 300x
⇒ 300x + 5x² – 1500 – 25x = 300x
⇒ 5x² + 300x – 25x – 300x – 1500 = 0
⇒ 5x² – 25x – 1500 = 0
⇒ x² – 5x – 300 = 0 (Dividing by 5)
⇒ x² – 20x + 15x – 300 = 0
⇒ x (x – 20) + 15 (x – 20) = 0
⇒ (x – 20) (x + 15) = 0
Either x – 20 – 0 then x = 20
or x + 15 = 0 then x = -15 But it is not possible.
Length of room = 20 m
and width = \(\frac { 300 }{ 20 }\) = 15 m

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency (Mean, Median, Quartiles and Mode) Ex 24C

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Chapter 24 Measures of Central Tendency (Mean, Median, Quartiles and Mode) Ex 24C.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24E

Question 1.
A student got the following marks in 9 questions of a question paper : 3, 5, 7, 3, 8, 0, 1, 4 and 6.
Find the median of these marks.
Solution:
Arranging the given data in descending order, we get:
8, 7, 6, 5,4,3, 3, 1,0
The middle term is 4 which is 5th terms
∴ Median = 4

Question 2.
The weights (in kg) of 10 students of a class are given below :
21, 28.5, 20.5, 24, 25.5, 22, 27.5, 28, 21, 24 Find the median of their weights.
Solution:
Arranging the given data in descending order.
We get 23.5, 28, 27.5, 25.5, 24, 24, 22, 21, 21, 20.5
the middle terms are 24 and 24, 5th and 6th terms.

Question 3.
The marks obtained by 19 students of a class are given below :
27, 36, 22, 31, 25, 26, 33, 24, 37, 32, 29, 28, 36, 35, 27, 26, 32, 35, 28. Find :
(i) Media
(ii) Lower quartile
(iii) Upper quartile
(iv) Inter – quartle range
Solution:
(i) Arranging in order say ascending, we get
22, 24, 25, 26, 26, 27, 27, 28, 28, 29, 31, 32, 32, 33, 35, 35,36, 36, 37
Middle term is 10th term i.e. 29
∴ Median = 29

Question 4.
From the following data, find :
(i) Median
(ii) Upper quartile
(iii) Inter-quartile range
25,10, 40, 88, 45, 60, 77, 36,18, 95, 56, 65, 7, 0, 38 and 83.
Solution:
(i) Arrange in ascending order, we get
0,7, 10, 18, 25, 36, 38, 40, 45, 56, 60, 65 ,77, 83, 88, 95

Question 5.
The ages of 37 students in a class are given in the following table :

Find the median.
Solution:

Question 6.
The weight of 60 boys are given in the following distribution table :

Find : (i) Median
(ii) Lower quartile
(iii) Upper quartile
(iv) Inter-quartile range
Solution:

Question 7.
Estimate the median for the given data by drawing ogive :

Solution:

Through mark of 25.5 on the y-axis, draw a line parallel to x-axis which meets the curve at A. From A, draw a perpendicular to x-axis, which meets x-axis at B.
∴ The value of B is the median which is 28.

Question 8.
By drawing an ogive; estimate the median for the following frequency distribution :

Solution:

Through mark of 28th on the y- axis, draw a line parallel to x-axis which meets the curve at A. From A, draw a perpendicular line segment to x- axis. Which meets it at B.

∴ The value of B is the median which is 18.4

Question 9.
From the following cumulative frequency table, draw ogive and then use it to find :
(i) Median,
(ii) Lower quartile,
(iii) Upper quartile.

Solution:

No. of terms = 80
Median = 40th term Through mark of 40 draw a line parallel to x-axis which meets the curve at A. From A, draw a perpendicular to x-axis which meets it at B
(ii) Lower quartile (Q1) = \(\frac { n }{ 4 }\) th term
= \(\frac { 80 }{ 4 }\) th term (Here n = 80 which is even)
= 20th term =18
(iii) Upper quartile (Q1) = \(\frac { 3 }{ 4 }\) nth term =\(\frac { 3 x80 }{ 4 }\) = 60th term = 66 .

∴ Value of B is the median which is 40.

Question 10.
In a school 100 pupils have heights as tabulated below:

Find the median height by drawing an ogive.
Solution:


Through mark 50, draw a line parallel to x- axis which meets the curve at A. From A, draw per-pendicular to x-axis which meets x-axis at B is the median which is 148 cm.

P.Q.
Attempt this question on a graph paper. The table shows the distribution of marks gained by a group of 400 students in an examination :


Using a scale of 2 cm to represent 10 marks and 2 cm to represent 50 students, plot these points and draw a smooth curve through the points
Estimate from the graph :
(i) Median marks
(ii) quartile marks. [1997]
Solution:
Plot the points (10, 5), (20, 10), (30, 30), (40, 60), (50,105), (60,180), (70,270), (80, 355), (90, 390), (100, 400) on the graph and join them with free hand to get an ogive (curve) as shown:
(i) Total students = 400

From 200 on y-axis draw a line parallel to x-axis meeting the curve at P. From P, draw PL perpendicular on x-axis then L is the median which is 62.
(ii) Lower quartile (Q1) = \(\frac { 1 }{ 4 }\) x n = \(\frac { 1 }{ 4 }\) x 400 =100
From 100 ony-axis, draw a line parallel to x-axis meeting the curve at Q, from Q, draw QM ⊥ x-axis.
M is the required lower quartile (Q1) which is 49 3 3
Upper quartile (Q3) = \(\frac { 3 }{ 4 }\) n = \(\frac { 3 }{ 4 }\) x 400 = 300
From 300 on y-axis, draw a line parallel to x-axis meeting the curve at R. From R draw RN perpendicular to x-axis N is the required upper quartile (Q3) which is = 74

P.Q.
Attempt this question on graph paper.

(i) Construct the ‘less than’ cumulative frequency curve for the above data, using 2 cm = 10 years on one axis and 2cm = 10 casualities on the other, (ii) From your graph determine :
(a) Median
(b) Lower quartile. (1995)
Solution:

No. of terms = 83
∴ Median \(\frac { 83 }{ 2 }\) =41.5 th term .
Through marks 41.5,draw a line segment par allel to x-axis which meets the curve at A. From A, draw a line segment perpendicular to x-axis meeting it at B.


Through 21 on the y-axis draw a line parallel to x-axis meeting the curve at M
From M, draw a perpendicular on x-axis which meets it at N.
∴N is the lower quartile which is 29 (approx)

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

 

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency (Mean, Median, Quartiles and Mode) Ex 24B

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Chapter 24 Measures of Central Tendency (Mean, Median, Quartiles and Mode) Ex 24B.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24E

Question 1.
The following table gives the ages of 50 students of a class. Find the arithmetic mean of their ages.

Solution:

Question 2.
The following table gives the weekly wages of workers in a factory.

Calculate the mean by using :
(i) Direct Method
(ii) Short-Cut Method
Solution:
(i) Direct Method:

(ii) Short cut method :

Question 3.
The following are the marks obtained by 70 boys in a class test :

Calculate the mean by :
(i) Short-Cut Method
(ii) Step-Deviation Method.
Solution:
(i) Short cut Method :

(ii) Step – Deviation Method:

Question 4.
Find mean by ‘step-deviation method :

Solution:

Question 5.
The mean of following frequency distribution is 21\(\frac { 1 }{ 7 }\) Find the value of ‘f ‘.

Solution:

Question 6.
Using step-deviation method, calculate the mean marks of the following distribution.

Solution:
Let Assumed mean = 72.5

Question 7.
Using the information given in the adjoining histogram; calculate the mean.
Solution:

Question 8.
If the mean of the following observations is 54, find the value of p.

Solution:
Mean = 54

⇒ 2106 + 54p = 2370 + 30p
⇒ 54p – 30p = 2370 – 2106 ⇒ 24p = 264
p = \(\frac { 264 }{ 24 }\) = 11
Hence p = 11

Question 9.
The mean of the following distribution is 62.8 and the sum of all the frequencies is 50. Find the missing frequencies f₁ and f₂.

Solution:
Mean = 62.8
and sum of frequencies = 50

Question 10.
Calculate the mean of the distribution given below using the short cut method.

Solution:

Question 11.
Calculate the mean of the following distribution :

Solution:

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10B

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10B

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10E
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10F

Question 1.
In an A.P., ten times of its tenth term is equal to thirty times of its 30th term. Find its 40th term.
Solution:
In an A.P.
10T₁₀ = 30T₃₀
We know that,
If m times of mth term = n times of nth term
Then its (m + n)th term = 0
∴ 10T₁₀ = 30T₃₀
Then T₁₀₊₃₀ = 0 or T₄₀ = 0

Question 2.
How many two-digit numbers are divisible by 3 ?
Solution:
Two digits numbers are 10 to 99
and two digits numbers which are divisible
by 3 are 12, 15, 18, 21,….99
Here a = 12, d = 3 and l = 99
Now, Tn = l = a + (n – 1 )d
99 = 12 + (n – 1) x 3
=> 99 – 12 = 3(n – 1)
=> 87 = 3(n – 1)
=> \(\\ \frac { 87 }{ 3 } \) = n – 1
=> n – 1 = 29
n = 29 + 1 = 30
Number of two digit number divisible by 3 = 30

Question 3.
Which term of A.P. 5, 15, 25, will be 130 more than its 31st term?
Solution:
A.P. is 5, 15, 25,….
Let T_n = T₃₁ + 130
In A.P. a = 5, d = 15 – 5 = 10
∴ T_n = a + 30d + 130 = 5 + 30 x 10 +130
= 5 + 300 + 130 = 435
∴ n + (n – 1)d = 435
=> 5 + (n – 1) x 10 = 435
=> (n – 1) x 10 = 435 – 5 = 430
n – 1 = \(\\ \frac { 430 }{ 10 } \) = 43
n = 43 + 1 = 44
∴ The required term is 44th.

Question 4.
Find the value of p, if x, 2x + p and 3x + 6 are in A.P.
Solution:
A.P. is x, 2x + p, 3x + 6
2x + p – x = 3x + 6 – 2x + p
x + p = x – p + 6
=>2p = 6
=>p = \(\\ \frac { 6 }{ 2 } \) = 3
Hence p = 3

Question 5.
If the 3rd and the 9th terms of an arithmetic progression are 4 and – 8 respectively, which term of it is zero?
Solution:
In an A.P.
T₃ = 4 and T₉ = – 8
Let a be the first term and d be the common difference, then

Question 6.
How many three-digit numbers ate divisible by 87 ?
Solution:
Three digits numbers are 100 to 999

999 – 42 = 957
Numbers divisible by 87 will be 174,….., 957
Let n be the number of required three digit number.
Here, a = 174 and d = 87
l = 957 = a + (n – l)d
= 174 + (n – 1) x 87
=> (n – 1) x 87 = 957 – 174 = 783
n – 1 = \(\\ \frac { 783 }{ 87 } \) = 9
n = 9 + 1 = 10
There are 10 such numbers.

Question 7.
For what value of n, the nth term of A.P. 63, 65, 67,….. and nth term of A.P. 3, 10, 17,…… are equal to each other?
Solution:
We are given,
nth term of 63, 65, 67, …….
=> nth term of 3, 10, 17,…….
=> In first A.P., a₁ = 63, d₁ = 2
and in second A.P., a₂ = 3, d₂ = 1

∴ Required nth term will be 13th

Question 8.
Determine the A.P. whose 3rd term is 16 and the 7th term exceeds the 5th term by 12.
Solution:
T₃ = 16, and T₇ = T₅ + 12
Let a be the first term and d be the common difference
T₃ = a + 2d – 16 …(i)
T₇ = T₅ + 12
a + 6d = a + 4d + 12
=> 6d – 4d = 12 => 2d – 12
=> d = \(\\ \frac { 12 }{ 2 } \) = 6
and in (i),
a + 6 x 2 = 16
=> a + 12 = 16
=>a = 16 – 12 = 4
A.P. will be 4, 10, 16, 22,……

Question 9.
If numbers n – 2, 4n – 1 and 5n + 2 are in A.P., find the value of n and its next two terms.
Solution:
n – 2, 4n – 1 and 5n + 2 are in A.P.
(4n – 1) – (n – 2) = (5n + 2) – (4n – 1)
=> 4n – 1 – n + 2 = 5n + 2 – 4n + 1
=> 4n – n – 5n + 4n = 2 + 1 + 1 – 2
=>2n = 2 =>n = \(\\ \frac { 2 }{ 2 } \) = 1
4n – 1 = 4 x 1 – 1 = 4 – 1 = 3
n – 2 = 1 – 2 = – 1
and 5n + 2 = 5 x 1 + 2 = 5 + 2 = 7
A.P. is – 1, 3, 7, ……
and next 2 terms will 11, 15

Question 10.
Determine the value of k for which k² + 4k + 8, 2k² + 3k + 6 and 3k² + 4k + 4 are in A.P.
Solution:
k² + 4k+ 8, 2k² + 3k + 6 and 3k² + 4k + 4 are in A.P.
(2k² + 3k + 6) – (k² + 4k + 8)
= (3k² + 4k + 4) – (2k² + 3k + 6)
=> 2k² + 3k + 6 – k² – 4k – 8
= 3k² + 4k + 4 – 2k² – 3k – 6
=> k² – k – 2 = k² + k – 2
=> k² – k – k² – k = – 2 + 2
=> – 2k = 0
=> k = 0
Hence k = 0

Question 11.
If a, b and c are in A.P. show that :
(i) 4a, 4b and 4c are in A.P.
(ii) a + 4, b + 4 and c + 4 are in A.P.
Solution:
a, b, c are in A.P.
2b = a + c
(i) 4a, 4b and 4c are in A.P.
If 2(4b) = 4a + 4c
If 8b = 4a + 4c
If 2b = a + c which is given
(ii) a + 4, b + 4 and c + 4 are in A.P.
If 2(b + 4) = a + 4 + c + 4
If 2b + 8 = a + c + 8
If 2b = a + c which is given

Question 12.
An A.P. consists of 57 terms of which 7th term is 13 and the last term is 108. Find the 45th term of this A.P.
Solution:
Number of terms in an A.P. = 57
T₇ = 13, l= 108
To find T₄₅
Let a be the first term and d be the common difference
=> a + 6d = 13 …(i)
a + (n – 1 )d= 108
=> a + (57 – 1 )d= 108
=> a + 56d = 108
Subtracting,

Question 13.
4th term of an A.P. is equal to 3 times its term and 7th term exceeds twice the 3rd term by 1. Find the first term and the common difference.
Solution:
In A.P
T₄ = 3 x T₁
T₇ = 2 x T₃ + 1
Let a be the first term and d be the common
difference

Question 14.
The sum of the 2nd term and the 7th term of an A.P. is 30. If its 15th term is 1 less than twice of its 8th term, find the A.P.
Solution:
In an A.P.
T₂ + T₇ = 30
T₁₅ = 2T₈ – 1
Let a be the first term and d be the common difference
a + d + a + 6d = 30

Question 15.
In an A.P., if mth term is n and nth term is m, show that its rth term is (m + n – r)
Solution:
In an A.P.
T_m = n and T_n = m
Let a be the first term and d be the common difference, then
T_m = a + (m – 1 )d = n

Question 16.
Which term of the A.P. 3, 10, 17,…..will be 84 more than its 13th term?
Solution:
A.P. is 3, 10, 17,….
Here, a = 2, d = 10 – 3 = 7

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 10 Arithmetic Progression Ex 10B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.